Recent Progress in X-ray and Neutron Phase Imaging with Gratings
Abstract
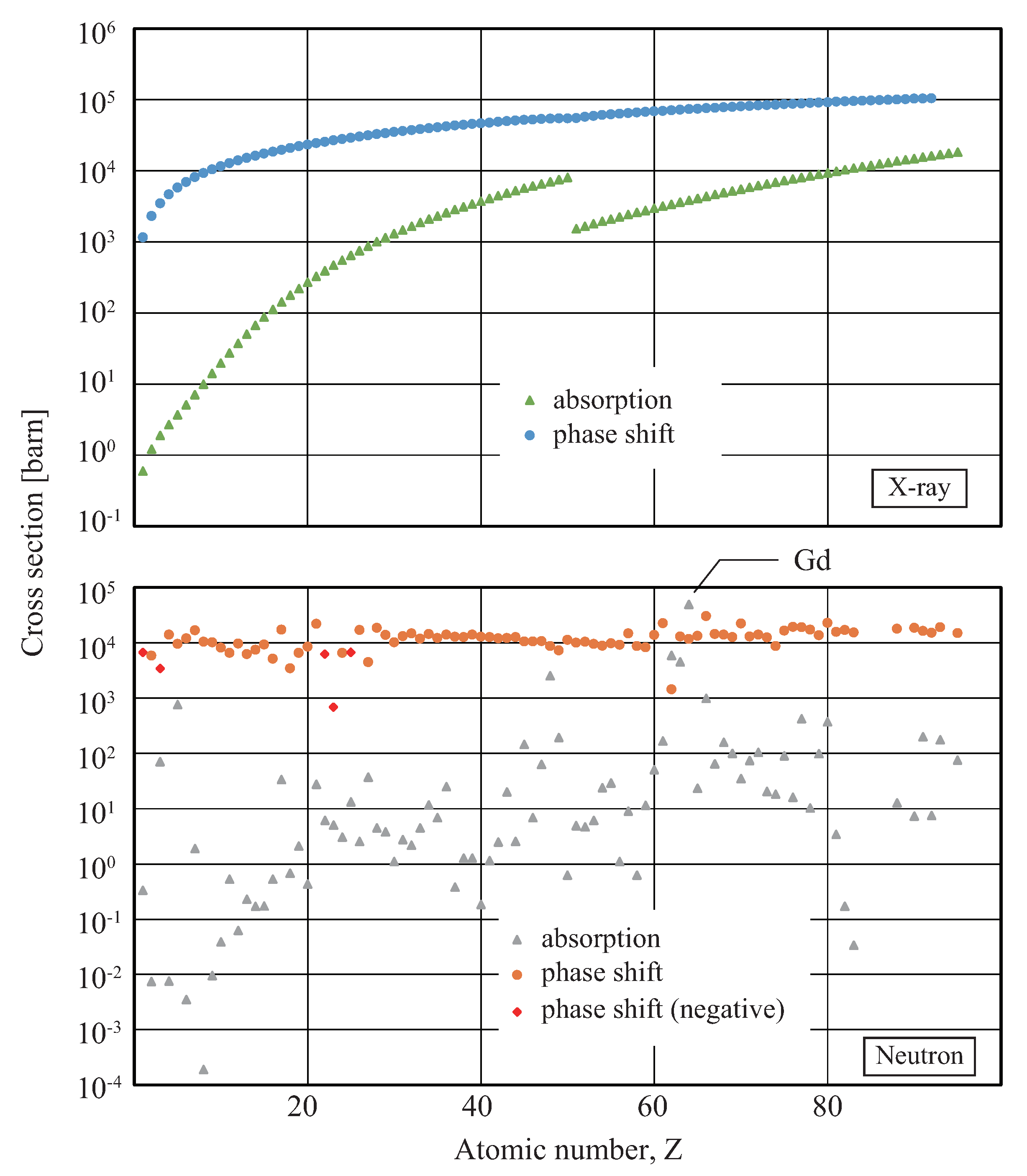
1. Introduction
2. Grating-Based Phase Imaging Method
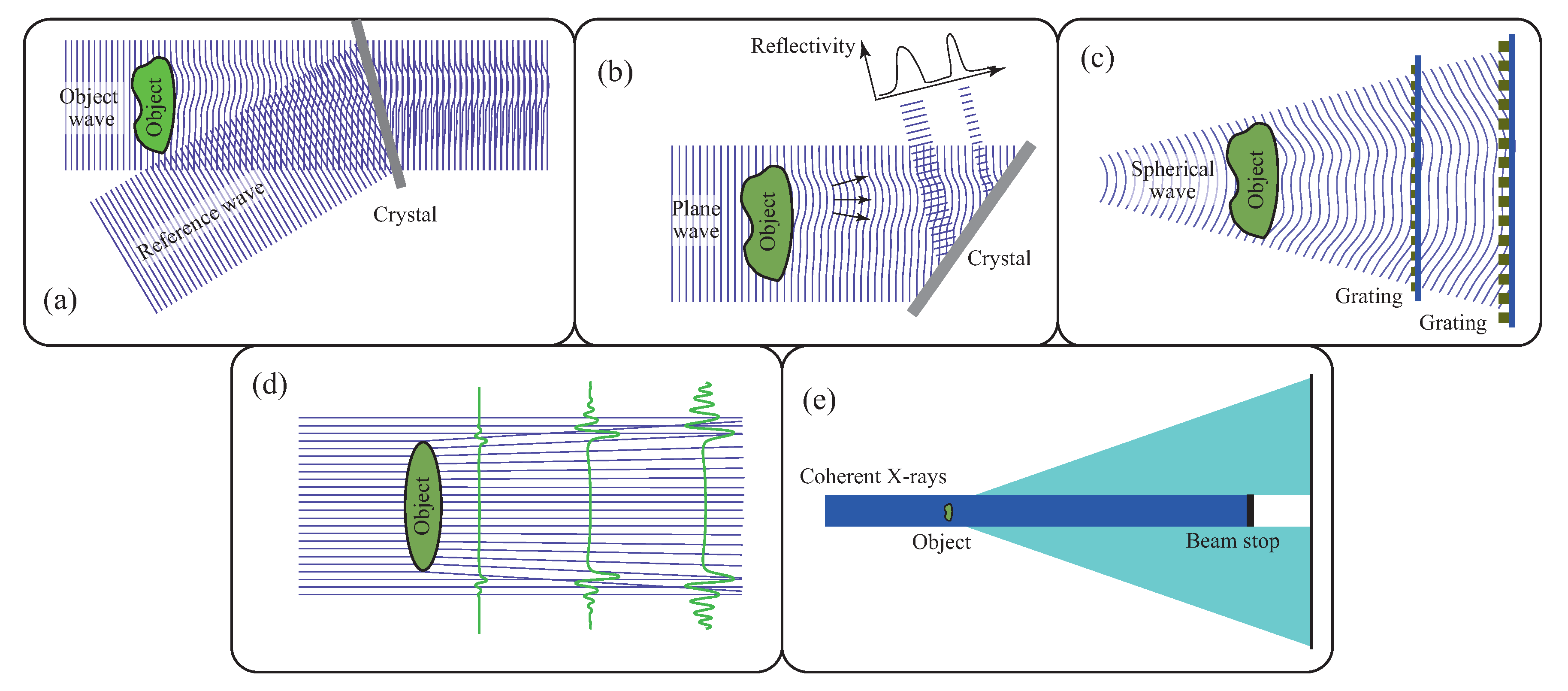
2.1. Methods to Generate Phase Contrast
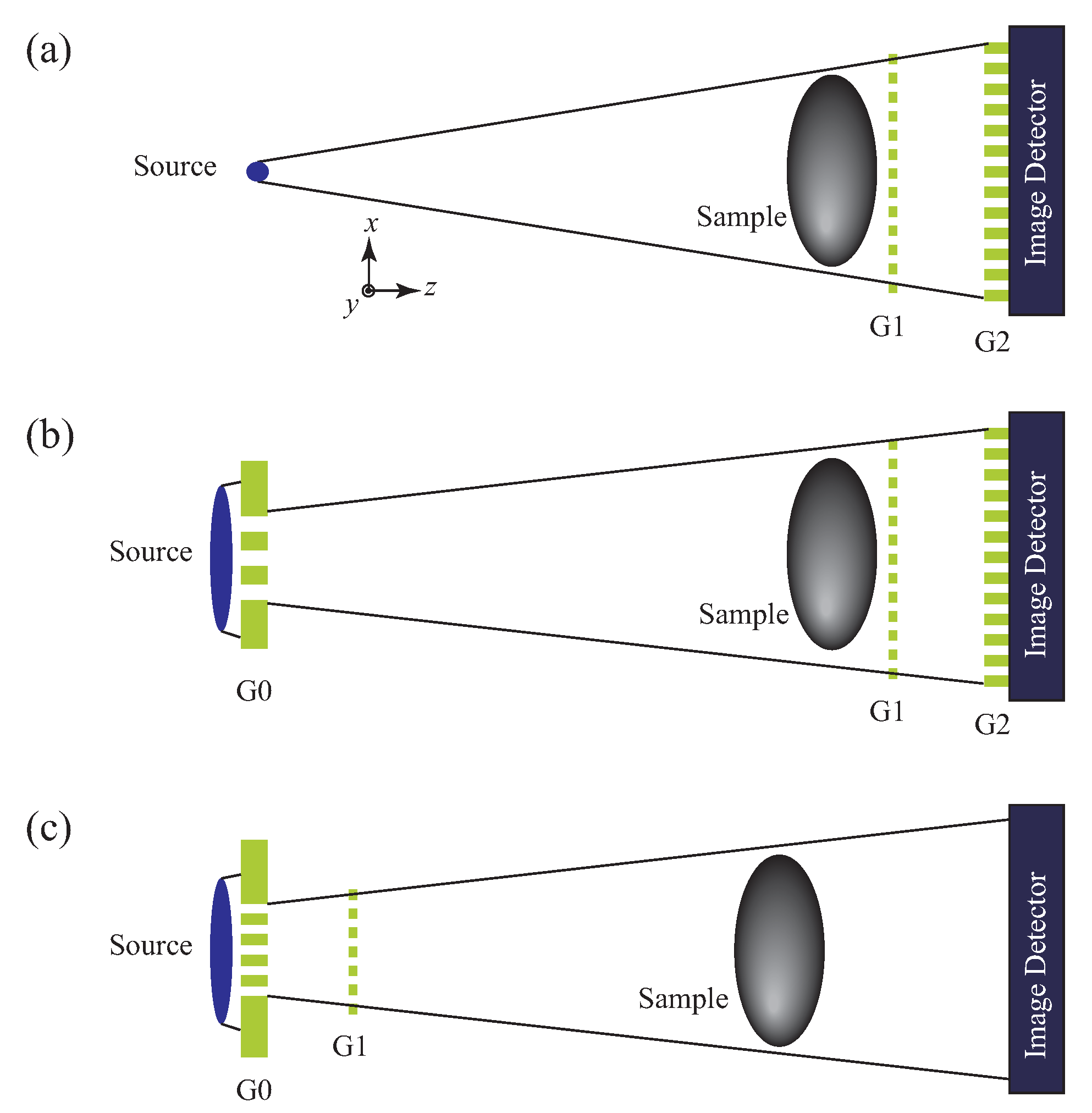
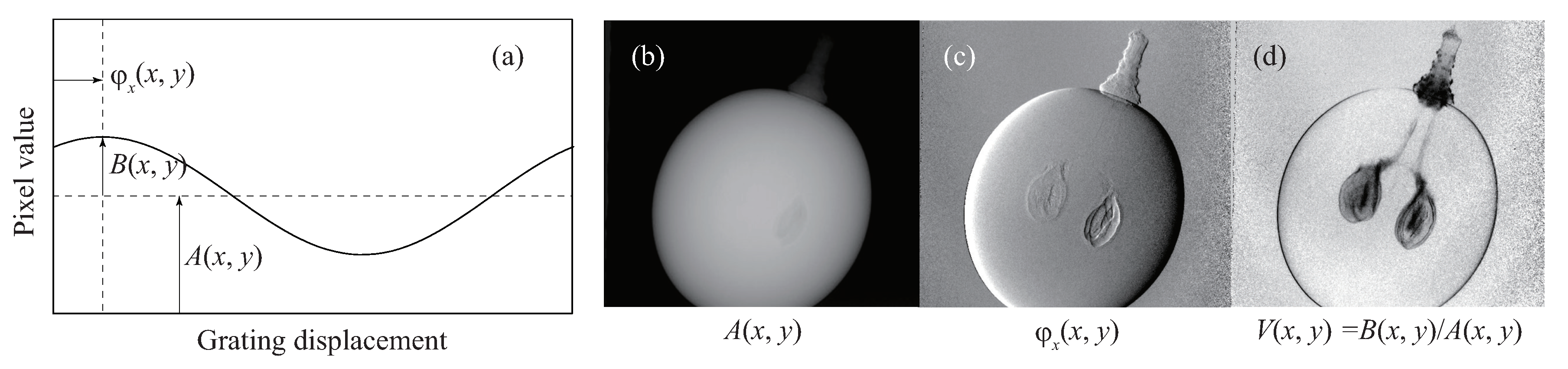
2.2. Principle of Talbot Interferometry and Phase Imaging
2.3. Visibility Reduction Induced by Scattering
2.4. Phase Tomography
2.5. Grating Fabrication for X-ray Talbot Interferometer
2.6. Extension from Talbot Interferometry
3. Development for X-ray Phase Imaging
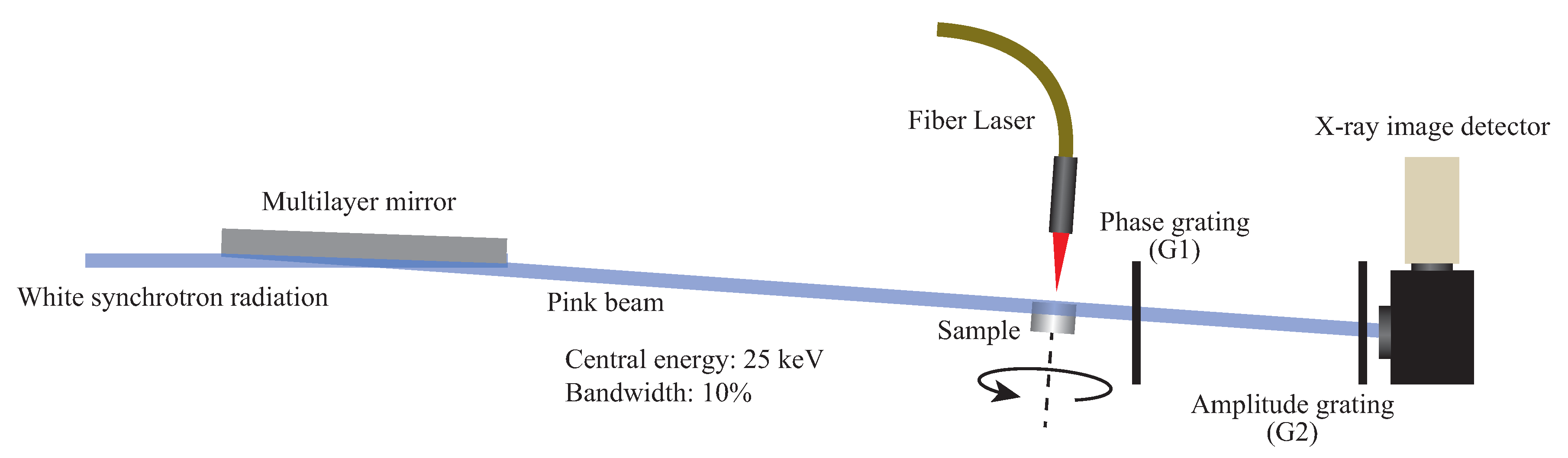
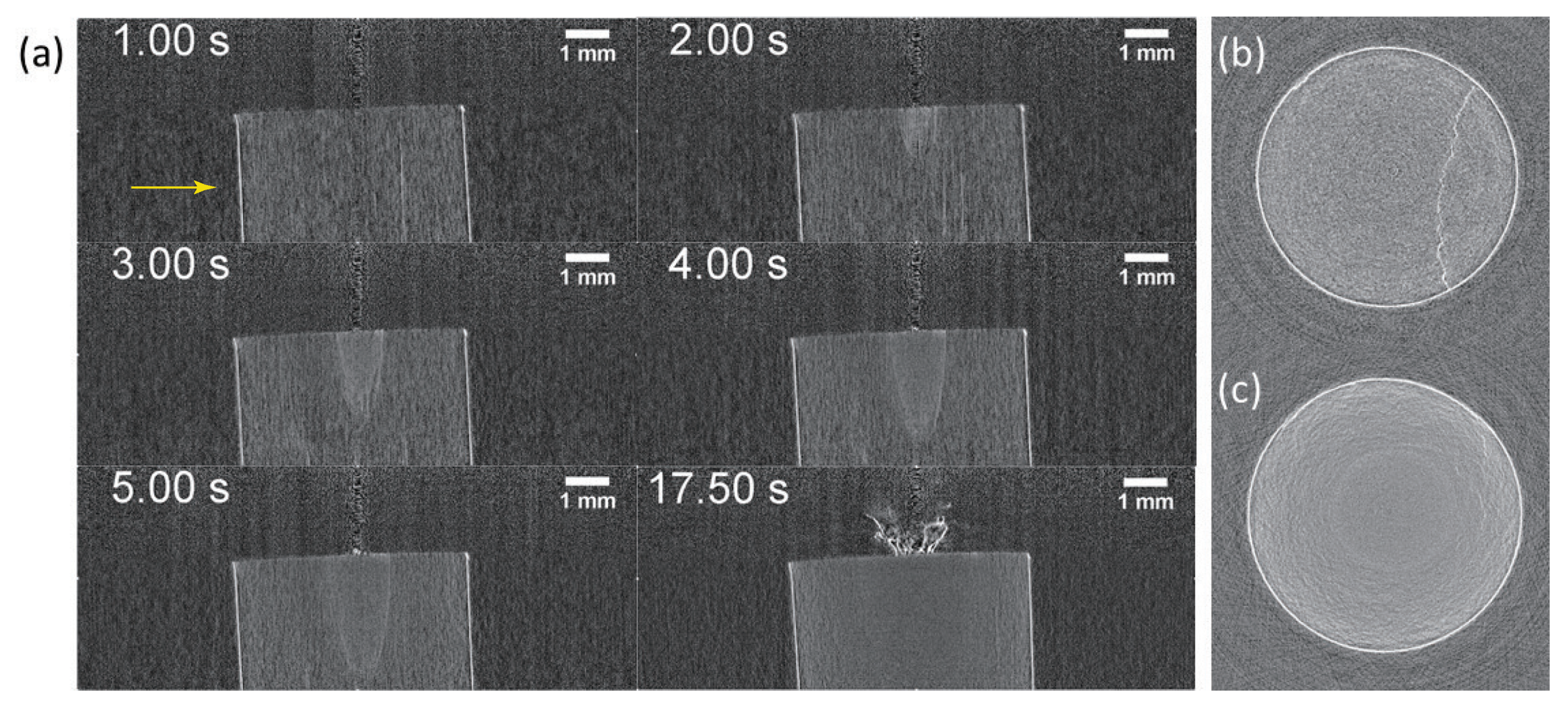
3.1. Four-Dimensional X-ray Phase Tomography
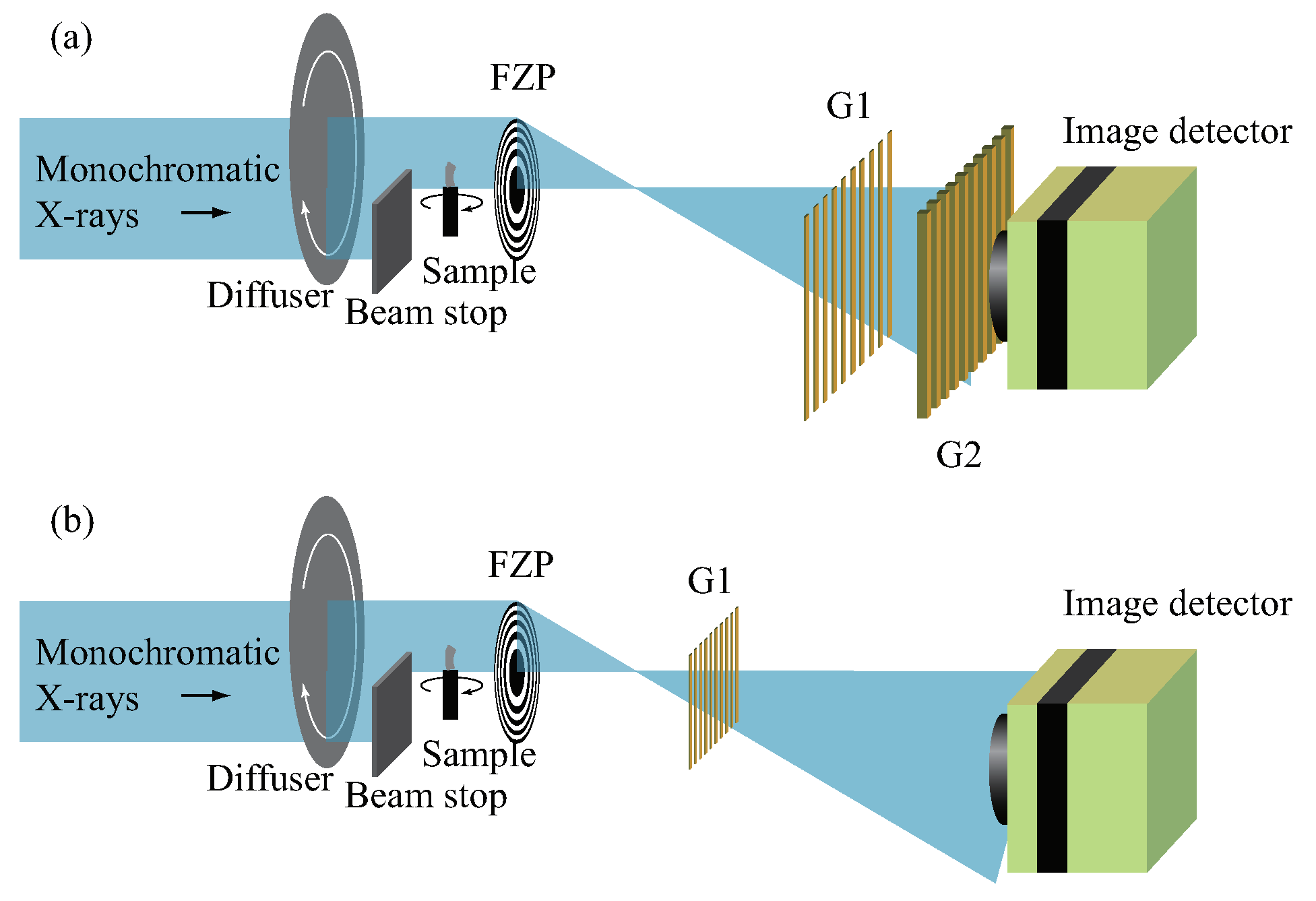
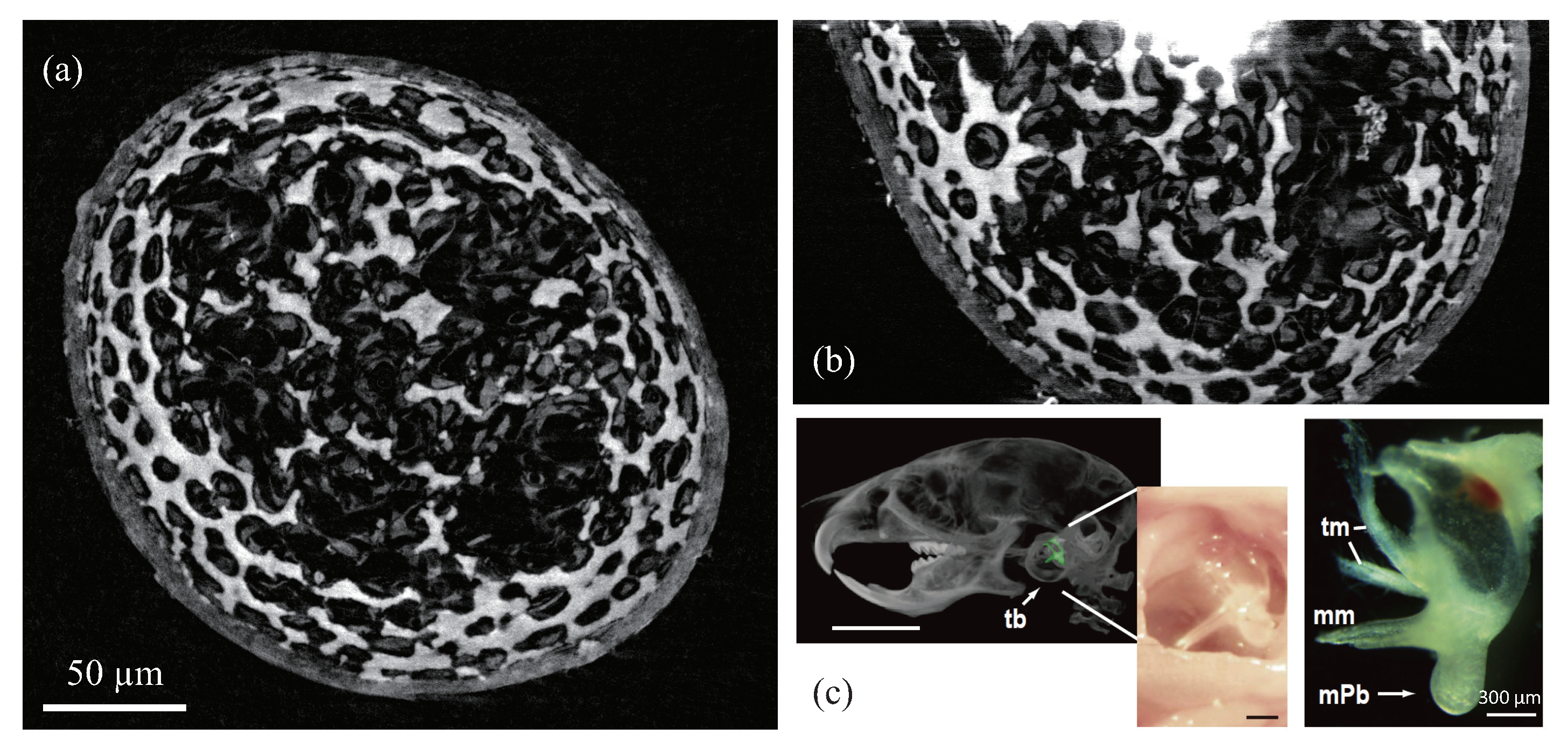
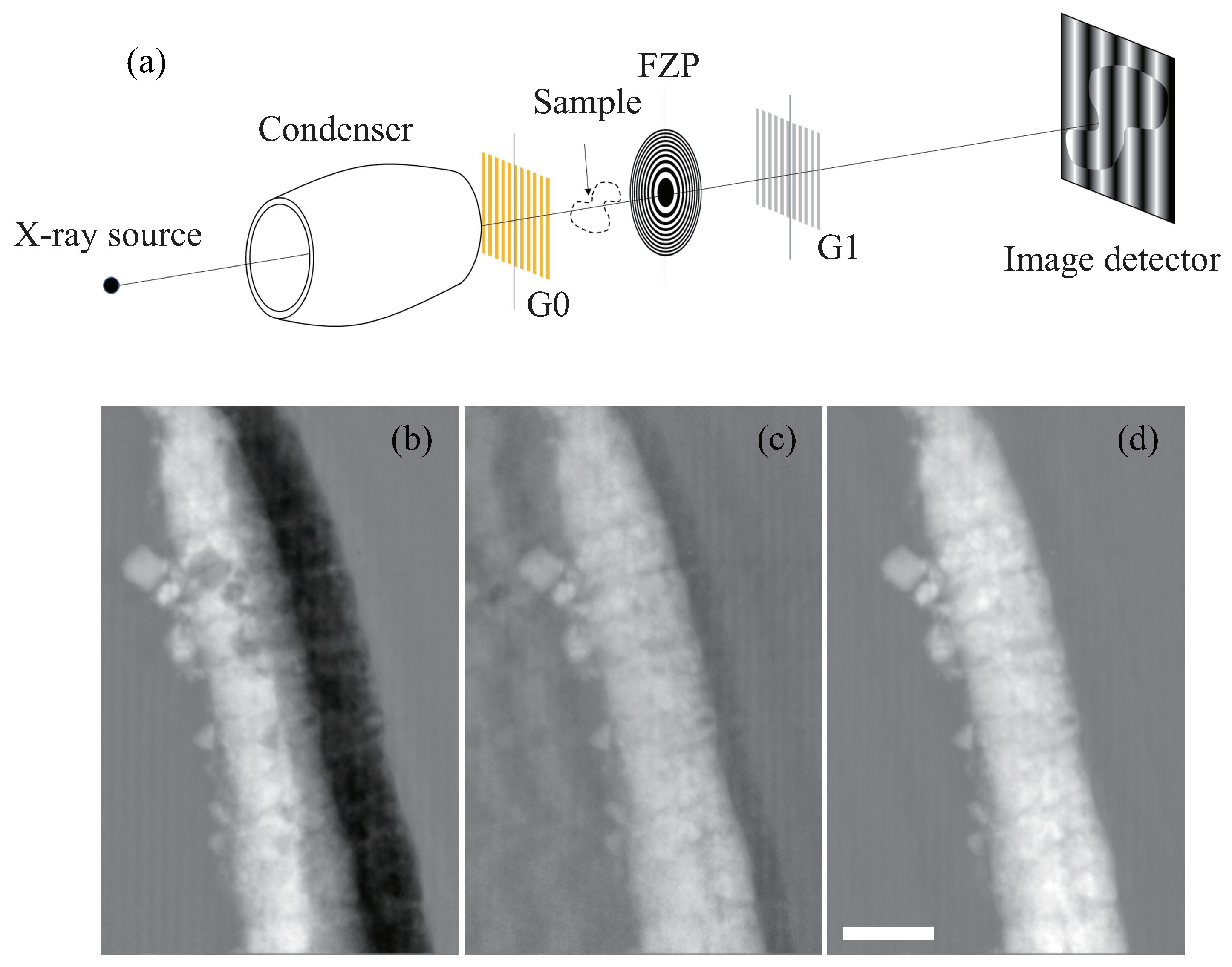
3.2. X-ray Phase Microscopy and Tomography
4. Development for Neutron Phase Imaging
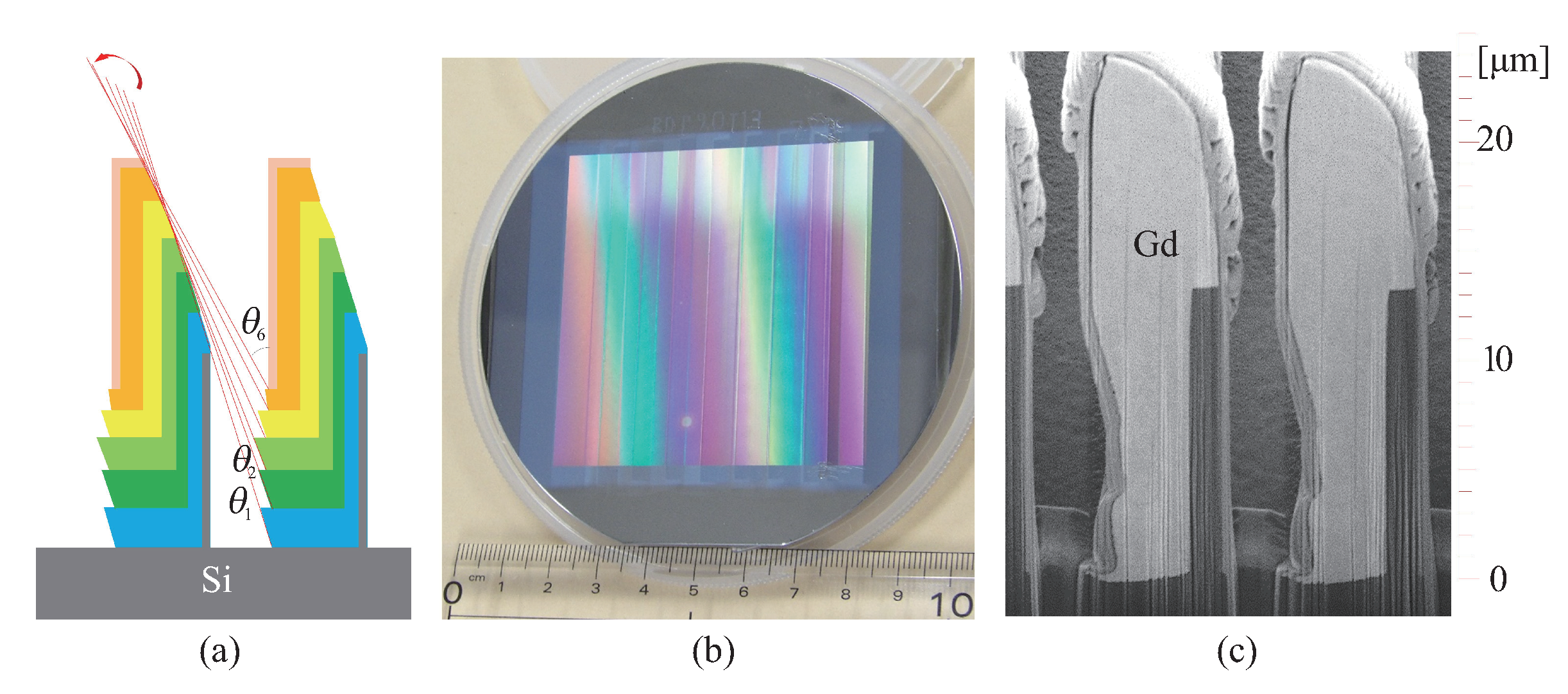
4.1. Neutron-Grating Fabrication
4.1.1. Gd-Based Metallic-Glass Imprinting
4.1.2. Improved Inclined Gd Evaporation
4.2. Neutron Phase Imaging at J-PARC
5. Summary
Funding
Acknowledgments
Conflicts of Interest
References
- X-ray Interactions with Matter. Available online: http://henke.lbl.gov/optical_constnts/ (accessed ona 10 February 2020).
- Sears, V.F. Neutron scattering lengths and cross sections. Neutron News 1992, 3, 26–37. [Google Scholar] [CrossRef]
- Zernike, F. Inflection theory of the cutting method and its improved form, the phase contrast method. Physica 1934, 1, 689–704. [Google Scholar] [CrossRef]
- Momose, A. Recent advances in X-ray phase imaging. Jpn. J. Appl. Phys. 2005, 44, 6355–6367. [Google Scholar] [CrossRef]
- Nugent, K. Coherent methods in the X-ray sciences. Adv. Phys. 2010, 59, 1–99. [Google Scholar] [CrossRef]
- Bravin, A.; Coan, P.; Suortti, P. X-ray phase-contrast imaging: From pre-clinical applications towards clinics. Phys. Med. Biol. 2013, 58, R1–R35. [Google Scholar] [CrossRef] [PubMed]
- Strobl, M.; Manke, I.; Kardjilov, N.; Hilger, A.; Dawson, M.; Banhart, J. Advances in neutron radiography and tomography. J. Phys. D Appl. Phys. 2009, 42, 243001. [Google Scholar] [CrossRef]
- Bonse, U.; Hart, M. An X-ray interferometer. Appl. Phys. Lett. 1965, 6, 155–156. [Google Scholar] [CrossRef]
- Momose, A. Demonstration of phase-contrast X-ray computed tomography using an X-ray interferometer. Nucl. Instrum. Methods A 1995, 352, 622–628. [Google Scholar] [CrossRef]
- Momose, A.; Takeda, T.; Itai, Y.; Hirano, K. Phase-contrast X-ray computed tomography for observing biological soft tissues. Nat. Med. 1996, 2, 473–475. [Google Scholar] [CrossRef]
- Rauch, H.; Treimer, W.; Bonse, U. Test of a single-crystal neutron interferometer. Phys. Lett. A 1974, 47, 369–371. [Google Scholar] [CrossRef]
- Davis, T.J.; Gao, D.; Gureyev, T.E.; Stevenson, A.W.; Wilkins, S.W. Phase-contrast imaging of weakly absorbing materials using hard X-rays. Nature 1995, 373, 595–598. [Google Scholar] [CrossRef]
- Ingal, V.N.; Beliaevskaya, E.A. X-ray phase-wave topography observation of the phase-contrast from a noncrystalline object. J. Phys. D Appl. Phys. 1995, 28, 2314–2317. [Google Scholar] [CrossRef]
- Chapman, D.; Thomlinson, W.; Johnston, R.E.; Washburn, D.; Pisano, E.; Gmür, N.; Zhong, Z.; Menk, R.; Arfelli, F.; Sayers, D. Diffraction enhanced X-ray imaging. Phys. Med. Biol. 1997, 42, 2015–2025. [Google Scholar] [CrossRef] [PubMed]
- Momose, A.; Kawamoto, S.; Koyama, I.; Hamaishi, Y.; Takai, K.; Suzuki, Y. Demonstration of X-ray Talbot interferometry. Jpn. J. Appl. Phys. 2003, 42, L866–L868. [Google Scholar] [CrossRef]
- Snigirev, A.; Snigireva, I.; Kohn, V.; Kuznetsov, S.; Schelokov, I. On the possibilities of X-ray phase contrast microimaging by coherent high-energy synchrotron radiation. Rev. Sci. Instrum. 1995, 66, 5486–5492. [Google Scholar] [CrossRef]
- Nugent, K.A.; Gureyev, T.E.; Cookson, D.F.; Paganin, D.; Barnea, Z. Quantitative phase imaging using hard X-rays. Phys. Rev. Lett. 1996, 77, 2961–2964. [Google Scholar] [CrossRef]
- Cloetens, P.; Barret, R.; Baruchel, J.; Guigay, J.P.; Schlenker, M. Phase objects in synchrotron radiation hard X-ray imaging. J. Appl. Phys. D Appl. Phys. 1996, 29, 133–146. [Google Scholar] [CrossRef]
- Wilkins, S.W.; Gureyev, T.E.; Gao, D.; Pogany, A.; Stevenson, A.W. Phase-contrast imaging using polychromatic hard X-rays. Nature 1996, 384, 335–338. [Google Scholar] [CrossRef]
- Allman, B.E.; McMahon, P.J.; Nugent, K.A.; Paganin, D.; Jacobson, D.L.; Arif, M.; Werner, S.A. Phase radiography with neutrons. Nature 2000, 408, 158–159. [Google Scholar] [CrossRef]
- Miao, J.; Charalambous, P.; Kirz, J.; Sayre, D. Extending the methodology of X-ray crystallography to allow imaging of micrometre-sized non-crystalline specimens. Nature 1999, 400, 342–344. [Google Scholar] [CrossRef]
- Thibault, P.; Dierolf, M.; Menze, A.; Bunk, O.; David, C. High-resolution scanning X-ray diffraction microscopy. Science 2008, 321, 379–382. [Google Scholar] [CrossRef] [PubMed]
- Bei, M.; Borland, M.; Cai, Y.; Elleaume, P.; Gerig, R.; Harkay, K.; Emery, L.; Hutton, A.; Hettel, R.; Nagaoka, R.; et al. The potential of an ultimate storage ring for future light sources. Nucl. Instrum. Methods A 2010, 622, 518–535. [Google Scholar] [CrossRef]
- Emma, P.; Akre, R.; Arthur, J.; Bionta, R.; Bostedt, C.; Bozek, J.; Brachmann, A.; Bucksbaum, P.; Coffee, R.; Decker, F.J.; et al. First lasing and operation of an angstrom-wavelength free-electron laser. Nat. Photon. 2010, 4, 641–647. [Google Scholar] [CrossRef]
- Yabashi, M.; Tanaka, H.; Ishikawa, T. Overview of the SACLA facility. J. Synchrotron Rad. 2015, 22, 477–484. [Google Scholar] [CrossRef]
- Altarelli, M. The European X-ray free-electron laser facility in Hamburg. Nucl. Instrum. Methods B 2011, 269, 2845–2849. [Google Scholar] [CrossRef]
- Chapman, H.; Nugent, K.A. Coherent lensless X-ray imaging. Nat. Photon. 2010, 4, 833–839. [Google Scholar] [CrossRef]
- Pfeiffer, F. X-ray ptychography. Nat. Photon. 2018, 12, 9–17. [Google Scholar] [CrossRef]
- Momose, A. Development toward high-resolution X-ray phase imaging. Microscopy 2017, 66, 155–166. [Google Scholar] [CrossRef]
- Pfeiffer, F.; Weitkamp, T.; Bunk, O.; David, C. Phase retrieval and differential phase-contrast imaging with low-brilliance X-ray sources. Nat. Phys. 2006, 2, 258–261. [Google Scholar] [CrossRef]
- Bruning, J.H.; Herriott, D.R.; Gallagher, J.E.; Rosenfeld, D.P.; White, A.D.; Brangaccio, D.J. Digital wavefront measuring interferometer for testing optical surfaces and lenses. Appl. Opt. 1974, 13, 2693–2703. [Google Scholar] [CrossRef]
- Pfeiffer, F.; Bech, M.; Bunk, O.; Kraft, P.; Eikenberry, E.F.; Brönnimann, C.H.; Grünzweig, C.; David, C. Hard-X-ray dark-field imaging using a grating interferometer. Nat. Mat. 2008, 7, 134–137. [Google Scholar] [CrossRef] [PubMed]
- Talbot, H.F. Facts relating to optical science. Phlos. Mag. 1836, 9, 401–407. [Google Scholar]
- Guigay, J.P. On Fresnel diffraction by one-dimensional periodic object, with application to structure determination of phase objects. Opt. Acta 1971, 18, 677–682. [Google Scholar] [CrossRef]
- Momose, A.; Yashiro, W.; Takeda, Y. X-ray phase imaging with Talbot interferometry. In Biomedical Mathematics: Promising Directions in Imaging, Therapy Planning, and Inverse Problems; Censor, Y., Jiang, M., Wang, G., Eds.; Medical Physics Publishing: Madison, WI, USA, 2009; pp. 281–320. [Google Scholar]
- Momose, A.; Yasihro, W.; Takeda, Y.; Suzuki, Y.; Hattori, T. Phase tomography by X-ray Talbot interferometry for biological imaging. Jpn. J. Appl. Phys. 2006, 45, 5254–5262. [Google Scholar] [CrossRef]
- Hashimoto, K.; Takano, H.; Momose, A. Improved reconstruction method for phase stepping data with stepping errors and dose fluctuations. submitted. [CrossRef]
- Yashiro, W.; Terui, Y.; Kawabata, K.; Momose, A. On the origin of visibility contrast in X-ray Talbot interferometry. Opt. Express 2010, 18, 16890–16901. [Google Scholar] [CrossRef]
- Lynch, S.K.; Pai, V.; Auxier, J.; Stein, A.F.; Bennett, E.E.; Kemble, C.K.; Xiao, X.; Lee, W.K.; Morgan, N.Y.; Wen, H.H. Interpretation of dark-field contrast and particle-size selectivity in grating interferometers. Appl. Opt. 2011, 50, 4310–4319. [Google Scholar] [CrossRef] [PubMed]
- Strobl, M. General solution for quantitative dark-field contrast imaging with grating interferometers. Sci. Rep. 2014, 4, 7243. [Google Scholar] [CrossRef] [PubMed]
- Grangeat, P. (Ed.) Tomography; John Wiley & Sons: Hoboken, NJ, USA, 2009. [Google Scholar]
- Faris, G.W.; Byer, R.L. Three-dimensional beam-deflection optical tomography of a supersonic jet. Appl. Opt. 1988, 27, 5202–5212. [Google Scholar] [CrossRef] [PubMed]
- Bech, M.; Bunk, O.; Donath, T.; Feidenhans’l, R.; David, C.; Pfeiffer, F. Quantitative X-ray dark-field computed tomography. Phys. Med. Biol. 2010, 55, 5529–5539. [Google Scholar] [CrossRef]
- Strobl, M.; Grünzweig, C.; Hilger, A.; Manke, I.; Kardjilov, N.; David, C.; Pfeiffer, F. Neutron dark-field tomography. Phys. Rev. Lett. 2008, 101, 123902. [Google Scholar] [CrossRef] [PubMed]
- Yashiro, W.; Momose, A. Effects of unresolvable edges in grating-based X-ray differential phase imaging. Opt. Express 2015, 23, 9233–9251. [Google Scholar] [CrossRef] [PubMed]
- Yashiro, W.; Vagovic, P.; Momose, A. Effect of beam hardening on a visibility-contrast image obtained by X-ray grating interferometry. Opt. Express 2015, 23, 23462–23471. [Google Scholar] [CrossRef]
- Matsumoto, M.; Takiguchi, K.; Tanaka, M.; Hunabiki, Y.; Takeda, H.; Momose, A.; Utsumi, Y.; Hattori, T. Fabrication of diffraction grating for X-ray Talbot interferometer. Microsys. Technol. 2007, 13, 543–546. [Google Scholar] [CrossRef]
- Mohr, J.; Grund, T.; Kunka, D.; Kenntner, J.; Leuthold, J.; Meiser, J.; Schulz, J.; Walter, M. High aspect ratio gratings for X-ray phase contrast imaging. AIP Conf. Proc. 2012, 1466, 41–50. [Google Scholar]
- Schröter, T.J.; Koch, F.J.; Mayer, P.; Kunka, D.; Meiser, J.; Willer, K.; Gromann, L.; De Marco, F.; Herzen, J.; Noel, P.; et al. Large field-of view tiled grating structures for X-ray phase-contrast imaging. Rev. Sci. Instrum. 2017, 88, 015104. [Google Scholar] [CrossRef] [PubMed]
- Kageyama, M.; Okajima, K.; Maesawa, M.; Nonoguchi, M.; Koike, T.; Noguchi, M.; Yamada, A.; Morita, E.; Kawase, S.; Kuribayashi, M.; et al. X-ray phase-imaging scanner with tiled bent gratings for large-field-of-view nondestructive testing. NDT E Int. 2019, 105, 19–24. [Google Scholar] [CrossRef]
- Tapfer, A.; Bech, M.; Velroyen, A.; Meiser, J.; Mohr, J.; Walter, M.; Schulz, J.; Pauwels, B.; Bruyndonckx, P.; Liu, X.; et al. Experimental results from a preclinical X-ray phase-contrast CT scanner. Proc. Natl. Acad. Sci. USA 2012, 109, 15691–15696. [Google Scholar] [CrossRef]
- Momose, A.; Yashiro, W.; Kido, K.; Kiyohara, J.; Makifuchi, C.; Ito, T.; Nagatsuka, S.; Honda, C.; Noda, D.; Hattori, T.; et al. X-ray phase imaging: From synchrotron to hospital. Phil. Trans. R. Soc. A 2014, 372, 20130023. [Google Scholar] [CrossRef][Green Version]
- Koehler, T.; Daerr, H.; Martens, G.; Kuhn, N.; Loescher, S.; van Stevendaal, U.; Roessl, E. Slit-scanning differential X-ray phase-contrast mammography: Proof-of-concept experimental studies. Med. Phys. 2015, 42, 1959–1965. [Google Scholar] [CrossRef]
- Hauke, C.; Bartl, P.; Leghissa, M.; Ritschl, L.; Sutter, S.M.; Weber, T.; Zeidler, J.; Freudenberger, J.; Mertelmeier, T.; Tadicke, M.; et al. A preclinical Talbot–Lau prototype for x-ray dark-field imaging of human-sized objects. Med. Phys. 2018, 45, 2565–2571. [Google Scholar] [CrossRef] [PubMed]
- Willer, K.; Fingerle, A.A.; Gromann, L.B.; De Marco, F.; Herzen, J.; Achterhold, K.; Gleich, B.; Muenzel, D.; Scherer, K.; Renz, M.; et al. X-ray dark-field imaging of the human lung—A feasibility study on a deceased body. PLoS ONE 2018, 13, e0204565. [Google Scholar] [CrossRef] [PubMed]
- Pfeiffer, F.; Grünzweig, C.; Bunk, O.; Frei, G.; Lehmann, E.; David, C. Neutron phase imaging and tomography. Phys. Rev. Lett. 2006, 96, 215505. [Google Scholar] [CrossRef] [PubMed]
- Donath, T.; Chabior, M.; Pfeiffer, F.; Bunk., O.; Reznikova, E.; Mohr, J.; Hempel, E.; Popescu, S.; Hoheisel, M.; Schuster, M.; et al. Inverse geometry for grating-based x-ray phase-contrast imaging. J. Appl. Phys. 2009, 106, 054703. [Google Scholar] [CrossRef]
- Momose, A.; Kuwabara, H.; Yasihro, W. X-ray phase imaging using Lau effect. Appl. Phys. Express 2011, 4, 066603. [Google Scholar] [CrossRef]
- Momose, A.; Yashiro, W.; Maikusa, H.; Takeda, Y. High-speed X-ray phase imaging and X-ray phase tomography with Talbot interferometer and white synchrotron radiation. Opt. Express 2009, 17, 12540–12545. [Google Scholar] [CrossRef]
- Momose, A.; Yashiro, W.; Harasse, S.; Kuwabara, H. Four-dimensional X-ray phase tomography with Talbot interferometry and white synchrotron radiation: Dynamic observation of a living worm. Opt. Express 2011, 19, 8423–8432. [Google Scholar] [CrossRef]
- Takeda, M.; Ina, H.; Kobayashi, S. Fourier-transform method of fringe-pattern analysis for computer-based topography and interferometry. J. Opt. Soc. Am. 1982, 72, 156–160. [Google Scholar] [CrossRef]
- Weitkamp, T.; Diaz, A.; David, C.; Pfeiffer, F.; Stampanoni, M.; Cloetens, P.; Ziegler, E. X-ray phase imaging with a grating interferometer. Opt. Express 2005, 13, 6296–6304. [Google Scholar] [CrossRef]
- Vegso, K.; Wu, Y.; Takano, H.; Hoshino, M.; Momose, A. Development of pink-beam 4D phase CT for -Situ Obs. Polym. Infrared Laser Irradiation. Sci. Rep. 2019, 9, 7404. [Google Scholar] [CrossRef]
- Kibayashi, S.; Harasse, S.; Yashiro, W.; Momose, A. High-speed X-ray phase tomography with Talbot interferometer and fringe-scanning method. AIP Conf. Proc. 2012, 1466, 261–265. [Google Scholar]
- Momose, A.; Vegso, K.; Takano, H.; Wu, Y.; Hashimoto, K.; Hoshino, M. Four-dimensional pink-beam X-ray phase tomography for studying laser processing. SPIE Proc. 2019, 11113, 111130I. [Google Scholar]
- Miao, H.; Gomella, A.A.; Chedid, N.; Chen, L.; Wen, H. Fabrication of 200 nm period hard X-ray phase gratings. Nano Lett. 2014, 14, 3453–3458. [Google Scholar] [CrossRef] [PubMed]
- Miao, H.; Gomella, A.A.; Harmon, K.J.; Bennett, E.E.; Chedid, N.; Znati, S.; Panna, A.; Foster, B.A.; Bhandarkar, P.; Wen, H. Enhancing tabletop X-ray phase contrast imaging with nano-fabrication. Sci. Rep. 2015, 5, 13581. [Google Scholar] [CrossRef] [PubMed]
- Miao, H.; Panna, A.; Gomella, A.A.; Bennett, E.E.; Znati, S.; Chen, L.; Wen, H. A universal moiré effect and application in X-ray phase-contrast imaging. Nat. Commun. 2016, 12, 830–834. [Google Scholar] [CrossRef] [PubMed]
- Takeda, Y.; Yashiro, W.; Hattori, T.; Takeuchi, A.; Suzuki, Y.; Momose, A. Differential phase X-ray imaging microscopy with X-ray Talbot interferometer. Appl. Phys. Express 2008, 1, 117002. [Google Scholar] [CrossRef]
- Nango, N.; Kubota, S.; Takeuchi, A.; Suzuki, Y.; Yashiro, W.; Momose, A.; Matsuo, K. Talbot-defocus multiscan tomography using the synchrotron X-ray microscope to study the lacuno-canalicular network in mouse bone. Biomed. Opt. Express 2013, 4, 917–923. [Google Scholar] [CrossRef]
- Momose, A.; Takano, H.; Hoshino, M.; Wu, Y.; Vegso, K. Recent advance in grating-based X-ray phase tomography. SPIE Proc. 2017, 103910, 103910Y. [Google Scholar]
- Matsuo, K.; Kuroda, Y.; Nango, N.; Shimoda, K.; Kubota, Y.; Ema, M.; Bakiri, L.; Wagner, E.F.; Takeda, Y.; Yashiro, W.; et al. Osteogenic capillaries orchestrate growth plate-independent ossification of the malleus. Development 2015, 142, 3912–3920. [Google Scholar] [CrossRef]
- Nango, N.; Kubota, S.; Hasegawa, T.; Yashiro, W.; Momose, A.; Matsuo, K. Osteocyte-directed bone demineralization along canaliculi. Bone 2016, 84, 279–288. [Google Scholar] [CrossRef]
- Yashiro, W.; Takeda, Y.; Takeuchi, A.; Suzuki, Y.; Momose, A. Hard-X-ray phase-difference microscopy using a Fresnel zone plate and a transmission grating. Phys. Rev. Lett. 2009, 103, 180801. [Google Scholar] [CrossRef] [PubMed]
- Kuwabara, H.; Yashiro, W.; Harasse, S.; Mizutani, H.; Momose, A. Hard-X-ray phase-difference microscopy with a low-brilliance laboratory X-ray source. Appl. Phys. Express 2011, 4, 062502. [Google Scholar] [CrossRef]
- Takano, H.; Wu, Y.; Irwin, J.; Maderych, S.; Leibowitz, M.; Tkachuk, A.; Kumer, A.; Hornberger, B.; Momose, A. Comparison of image properties in full-field phase X-ray microscopes based on grating interferometry and Zernike’s phase contrast optics. Appl. Phys. Lett. 2018, 113, 063105. [Google Scholar] [CrossRef]
- Takano, H.; Hashimoto, K.; Nagatani, Y.; Irwin, J.; Omlor, L.; Kumar, A.; Tkachuk, A.; Wu, Y.; Momose, A. Improvement in quantitative phase mapping by a hard x-ray microscope equipped with a Lau interferometer. Optica 2019, 6, 1012–1015. [Google Scholar] [CrossRef]
- Takano, H.; Hashimoto, K.; Nagatani, Y.; Wu, Y.; Momose, A. Development of laboratory-based x-ray phase tomographic microscope. SPIE Proc. 2019, 11113, 111130J. [Google Scholar]
- Grünzweig, C.; Pfeiffer, F.; Bunk, O.; Donath, T.; Kuhne, G.; Frei, G.; Dierolf, M.; David, C. Design, fabrication, and characterization of diffraction gratings for neutron phase contrast imaging. Rev. Sci. Instrum. 2008, 79, 053703. [Google Scholar] [CrossRef]
- Grünzweig, C.; David, C.; Bunk, O.; Dierolf, M.; Frei, G.; Kühne, G.; Kohlbrecher, J.; Schafer, R.; Lejcek, P.; Rønnow, H.M.R.; et al. Neutron decoherence imaging for visualizing bulk magnetic domain structures. Phys. Rev. Lett. 2008, 101, 025504. [Google Scholar] [CrossRef]
- Grünzweig, C.; David, C.; Bunk., O.; Dierolf, M.; Frei, G.; Kühne, G.; Schäfer, R.; Pofahl, S.; Rønnow, H.M.R.; Pfeiffer, F. Bulk magnetic domain structures visualized by neutron dark-field imaging. Appl. Phys. Lett. 2008, 93, 112504. [Google Scholar]
- Manke, I.; Kardjilov, N.; Schäfer, R.; Hilger, A.; Strobl, M.; Dawson, M.; Grünzweig, C.; Behr, G.; Hentschel, M.; David, C.; et al. Three-dimensional imaging of magnetic domains. Nat. Commun. 2010, 1, 125. [Google Scholar] [CrossRef]
- Reimann, T.; Mühlbauer, S.; Schulz, M.; Betz, B.; Kaestner, A.; Pipich, V.; Böni, P.; Grünzweig, C. Visualizing the morphology of vortex lattice domains in a bulk type-II superconductor. Nat. Commun. 2015, 6, 8813. [Google Scholar] [CrossRef]
- Valsecchi, J.; Harti, R.P.; Raventós, M.; Siegwart, M.D.; Morgano, M.; Boillat, P.; Strobl, M.; Hautle, P.; Holitzner, L.; Filges, U.; et al. Visualization and quantification of inhomogeneous and anisotropic magnetic fields by polarized neutron grating interferometry. Nat. Commun. 2019, 10, 3788. [Google Scholar] [CrossRef] [PubMed]
- Shinohara, T.; Kai, T.; Oikawa, K.; Segawa, M.; Harada, M.; Nakatani, M.; Ooi, M.; Aizawa, K.; Sato, H.; Kamiyama, T.; et al. Final design of the energy-resolved neutron imaging system “RADEN” at J-PARC. J. Phys. Conf. Ser. 2016, 746, 012007. [Google Scholar] [CrossRef]
- Seki, Y.; Shinohara, T.; Parker, J.D.; Ueno, W.; Samoto, T.; Yashiro, W.; Momose, A.; Otake, Y.; Kiyanagi, Y. Efficient phase imaging using wavelength-resolved neutron Talbot–Lau interferometry with TOF method. EPL 2018, 123, 12002. [Google Scholar] [CrossRef]
- Sadeghilaridjani, M.; Kato, K.; Shinohara, T.; Yashiro, W.; Momose, A.; Kato, H. High aspect ratio grating by isochronal imprinting of less viscous workable Gd-based metallic glass for neutron phase imaging. Intermetallics 2016, 78, 55–63. [Google Scholar] [CrossRef]
- Samoto, T.; Takano, H.; Momose, A. Evaluation of obliquely evaporated gadolinium gratings for neutron interferometry by X-ray microtomography. Mater. Sci. Semicond. Process. 2019, 92, 91–95. [Google Scholar] [CrossRef]
- Samoto, T.; Takano, H.; Momose, A. Gadolinium oblique evaporation approach to make large scale neutron absorption gratings for phase imaging. Jpn. J. Appl. Phys. 2019, 58, SDDF12. [Google Scholar] [CrossRef]
- Wang, W.H.; Dong, C.; Shek, C.H. Bulk metallic glasses. Mater. Sci. Eng. R 2004, 44, 45–89. [Google Scholar] [CrossRef]
- Seki, Y.; Shinohara, T.; Parker, J.D.; Yashiro, W.; Momose, A.; Kato, K.; Kato, H.; Sadeghilaridjani, M.; Otake, Y.; Kiyanagi, Y. Development of multi-colored neutron Talbot–Lau interferometer with absorption grating fabricated by imprinting method of metallic glass. J. Phys. Soc. Jpn. 2017, 86, 044001. [Google Scholar] [CrossRef]
- Seki, Y.; Shinohara, T.; Ueno, W.; Parker, J.D.; Samoto, T.; Yashiro, W.; Momose, A. Experimental evaluation of neutron absorption grating fabricated by oblique evaporation of gadolinium for phase imaging. Phys. Procedia 2017, 88, 217–223. [Google Scholar] [CrossRef]
- Parker, J.D.; Harada, M.; Hattori, K.; Iwaki, S.; Kabuki, S.; Kishimoto, Y.; Kubo, H.; Kurosawa, S.; Matsuoka, Y.; Miuchi, K.; et al. Spatial resolution of a mu PIC-based neutron imaging detector. Nucl. Instrum. Methods A 2013, 726, 155–161. [Google Scholar] [CrossRef]
- Sarapata, A.; Chabior, M.; Cozzini, C.; Sperl, J.I.; Bequé, D.; Langner, O.; Coman, J.; Zanette, I.; Ruiz-Yaniz, M.; Pfeiffer, F. Quantitative electron density characterization of soft tissue substitute plastic materials using grating-based X-ray phase-contrast imaging. Rev. Sci. Instrum. 2014, 85, 103708. [Google Scholar] [CrossRef] [PubMed]
- Ikematsu, K.; Takano, H.; Wu, Y.; Kimura, K.; Mamyrbayev, T.; Opolka, A.; Ershov, A.; Gutekunst, J.; Meyer, P.; Last, A.; et al. Super-resolution X-ray phase imaging with two dimensional biconcave parabolic multi lens array. in preparation.
- Wu, Y.; Takano, H.; Momose, A. Observation of structural degradation using grating-interferometer-based X-ray stroboscopic tomography. 2020; in preparation. [Google Scholar]
- Momose, A.; Ikematsu, K.; Wu, Y.; Kimura, K.; Takano, H. Sensitivity enhancement in X-ray Talbot interferometry by using parabolic gratings. 2020; in preparation. [Google Scholar]
- Yamagata, Y.; Hirota, K.; Ju, J.; Wang, S.; Morita, S.; Kato, J.; Otake, Y.; Taketani, A.; Seki, Y.; Yamada, M.; et al. Development of a neutron generating target for compact neutron sources using low energy proton beams. J. Radioanal. Nucl. Chem. 2015, 305, 787–794. [Google Scholar] [CrossRef]
- Jensen, T.H.; Bech, M.; Bunk, O.; Donath, T.; David, C.; Feidenhans’l, R.; Pfeiffer, F. Directional x-ray dark-field imaging. Phys. Med. Biol. 2010, 55, 3317–3323. [Google Scholar] [CrossRef]
- Malecki, A.; Potdevin, G.; Biernath, T.; Eggl, E.; Willer, K.; Lasser, T.; Maisenbacher, J.; Gibmeier, J.; Wanner, A.; Pfeiffer, F. X-ray tensor tomography. Europhys. Lett. 2014, 105, 38002. [Google Scholar] [CrossRef]
- Kagias, M.; Wang, Z.; Villanueva-Perez, P.; Jefimovs, K.; Stampanoni, M. 2D-Omnidirectional hard-X-ray scattering sensitivity in a single shot. Phys. Rev. Lett. 2016, 116, 093902. [Google Scholar] [CrossRef]
- Kagias, M.; Wang, Z.; Birkback, M.E.; Lauridsen, E.; Abis, M.; Lovric, G.; Jefimovs, K.; Stampanoni, M. Diffractive small angle X-ray scattering imaging for anisotropic structures. Nat. Commun. 2019, 10, 5130. [Google Scholar] [CrossRef]
- Thuering, T.; Barber, W.C.; Seo, Y.; Alhassen, F.; Iwanczyk, J.S.; Stampanoni, M. Energy resolved X-ray grating interferometry. Appl. Phys. Lett. 2013, 102, 191113. [Google Scholar] [CrossRef]
- Berujon, S.; Wang, H.; Sawhney, K. X-ray multimodal imaging using a random-phase object. Phys. Rev. A 2012, 86, 063813. [Google Scholar] [CrossRef]
- Morgan, K.S.; Paganin, D.M.; Siu, K.K.W. X-ray phase imaging with a paper analyzer. Appl. Phys. Lett. 2012, 100, 124102. [Google Scholar] [CrossRef]
- Olivo, A.; Speller, R. A coded-aperture technique allowing X-ray phase contrast imaging with conventional sources. Appl. Phys. Lett. 2007, 91, 074106. [Google Scholar] [CrossRef]


© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Momose, A.; Takano, H.; Wu, Y.; Hashimoto, K.; Samoto, T.; Hoshino, M.; Seki, Y.; Shinohara, T. Recent Progress in X-ray and Neutron Phase Imaging with Gratings. Quantum Beam Sci. 2020, 4, 9. https://doi.org/10.3390/qubs4010009
Momose A, Takano H, Wu Y, Hashimoto K, Samoto T, Hoshino M, Seki Y, Shinohara T. Recent Progress in X-ray and Neutron Phase Imaging with Gratings. Quantum Beam Science. 2020; 4(1):9. https://doi.org/10.3390/qubs4010009
Chicago/Turabian StyleMomose, Atsushi, Hidekazu Takano, Yanlin Wu, Koh Hashimoto, Tetsuo Samoto, Masato Hoshino, Yoshichika Seki, and Takenao Shinohara. 2020. "Recent Progress in X-ray and Neutron Phase Imaging with Gratings" Quantum Beam Science 4, no. 1: 9. https://doi.org/10.3390/qubs4010009
APA StyleMomose, A., Takano, H., Wu, Y., Hashimoto, K., Samoto, T., Hoshino, M., Seki, Y., & Shinohara, T. (2020). Recent Progress in X-ray and Neutron Phase Imaging with Gratings. Quantum Beam Science, 4(1), 9. https://doi.org/10.3390/qubs4010009




