Strengthening of Low-Strength Concrete Columns with Fibre Reinforced Polymers. Full-Scale Tests
Abstract
1. Introduction
2. Experimental Program
2.1. Main Parameters of Experimental Program
- The side-aspect ratio of the cross section or ratio to the sides of the concrete section (b/d). Specimens with a square section (b/d = 1) and a rectangular one (b/d = 1.5 and b/d = 2) were tested. Specifically, three sections were used: 300 × 300 mm2 (square section), 250 × 375 mm2 (rectangular, b/d = 1.5), and 200 × 400 mm2 (rectangular, b/d = 2).
- The radius of curvature of the corners (Rc). The corners of the rectangular columns must be rounded before applying strengthening to avoid premature failure of the FRP jacket. For this study, two radii of curvature were used: 20 and 40 mm.
- The amount of FRP reinforcement. Specimens were strengthened with 2 or 3 layers of CFRP (polymer reinforced with carbon fibres). The material has a nominal fibre thickness of 0.129 mm and its properties are described in Section 2.3 of this paper.
2.2. Specimen Preparation
2.3. FRP Strengthening
2.4. Test Set-Up and Instrumentation
3. Experimental Results
- The unconfined concrete compressive strength (fco) obtained by testing of cylindrical standard samples.
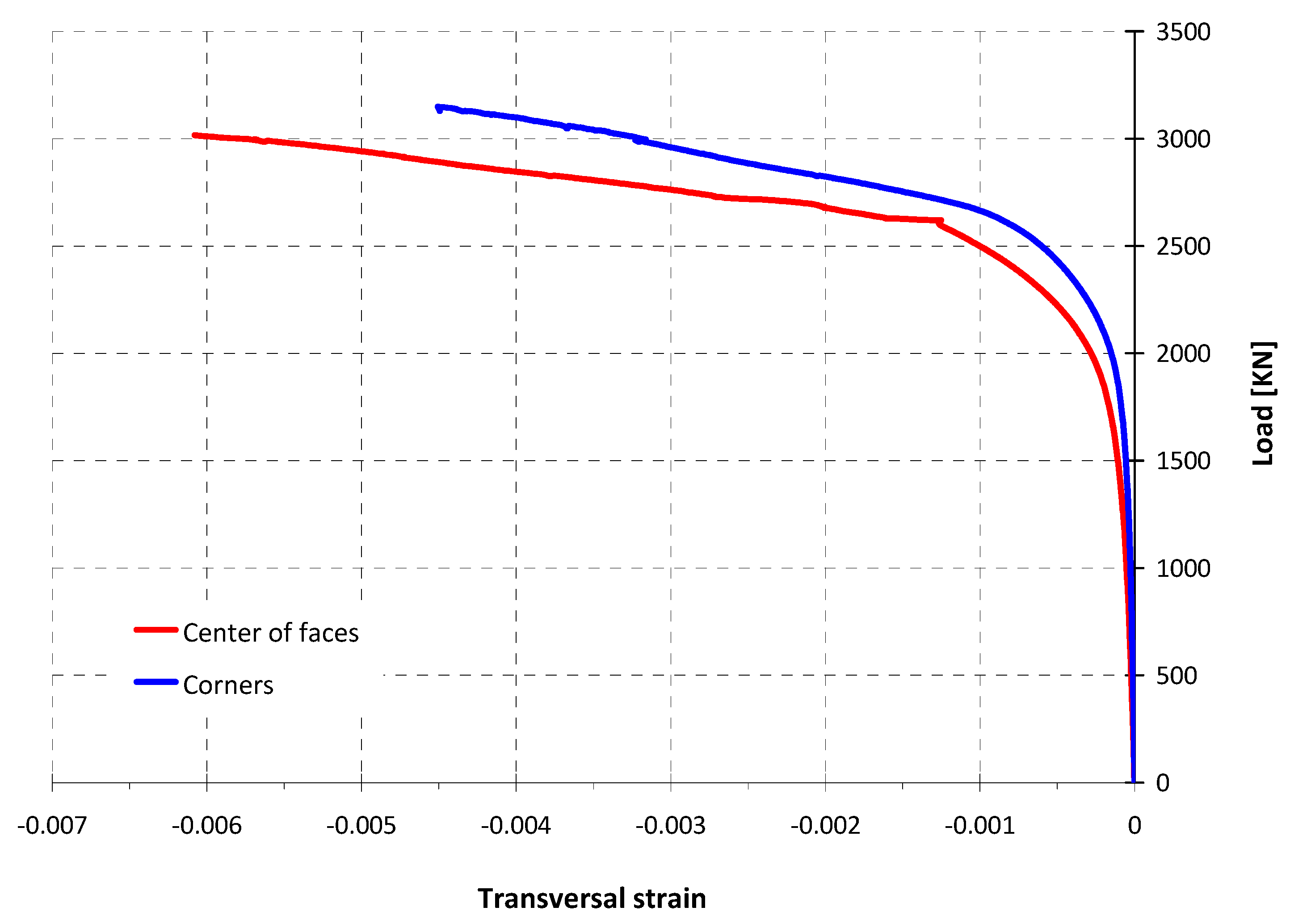
- The peak axial load (Qmax) or maximum load applied in the test.
- The confined concrete strength (fcc) or concrete axial stress at peak load. This value is computed as the difference between the peak load (Qmax) and the load carried by the longitudinal steel reinforcement, divided by the net area of concrete. The load carried by longitudinal steel bars is given as its total area multiplied by the steel yield stress.
- The rate of confined and unconfined concrete strength (fcc/fco) or strength enhancement ratio. It must be noted that the contribution of existing steel stirrups was neglected in this preliminary analysis.
- The ultimate axial strain (εcc). The axial strains are obtained as the average of measurements from the displacement sensors.
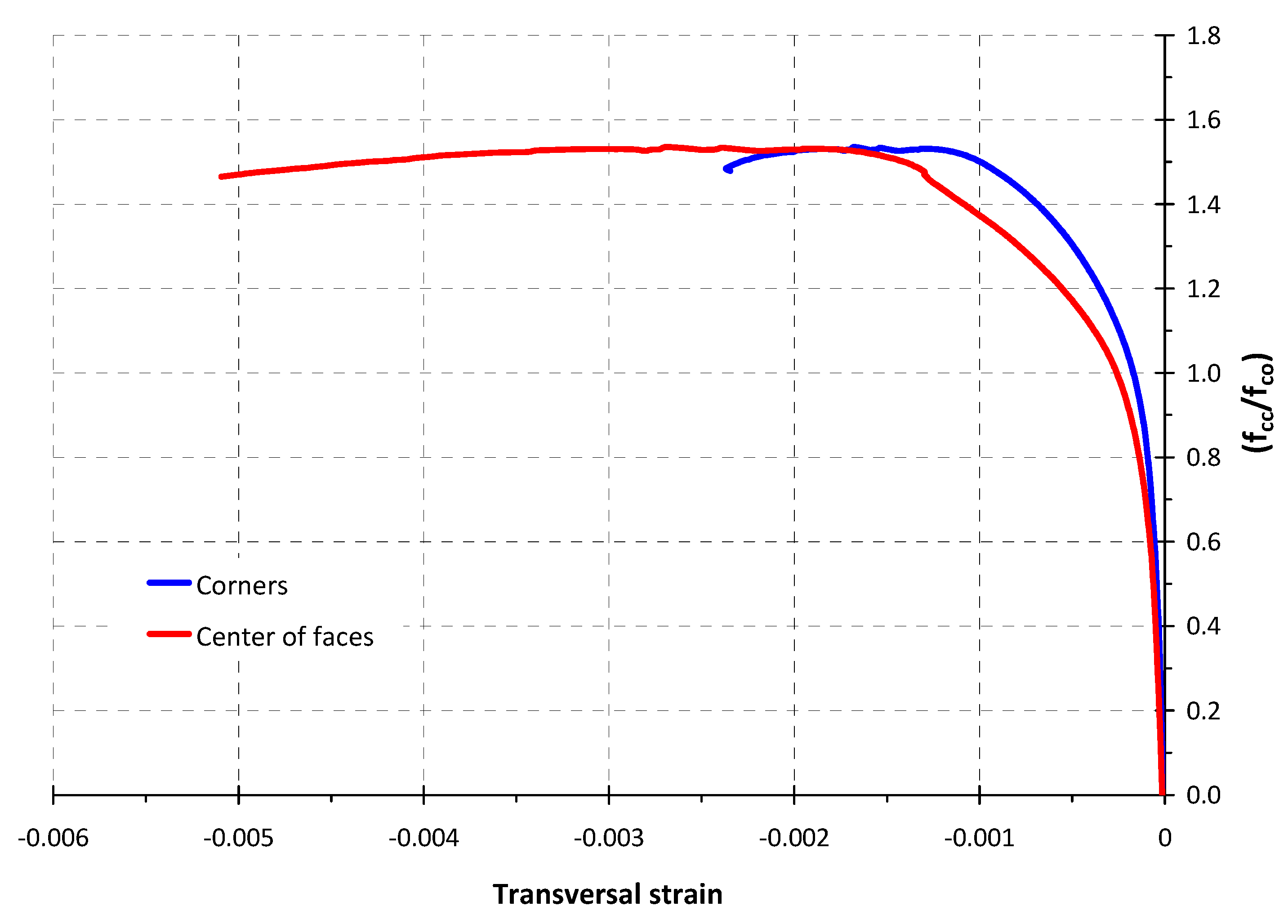
- The ultimate transversal strain, also called FRP effective strain (εf,eff). The transversal deformations are obtained as the average of measurements from the four side transversal measurements.
- The relationship between the FRP effective strain (εf,eff) and the ultimate strain of the fibre obtained by standard tensile tests of flat coupons (εf). This rate (εf,eff/εf) is commonly called the strain efficiency factor.
- The maximum transversal strain (εfmax) measured by any of the transversal strain gauges located at the centre of the four faces.
3.1. Failure Modes
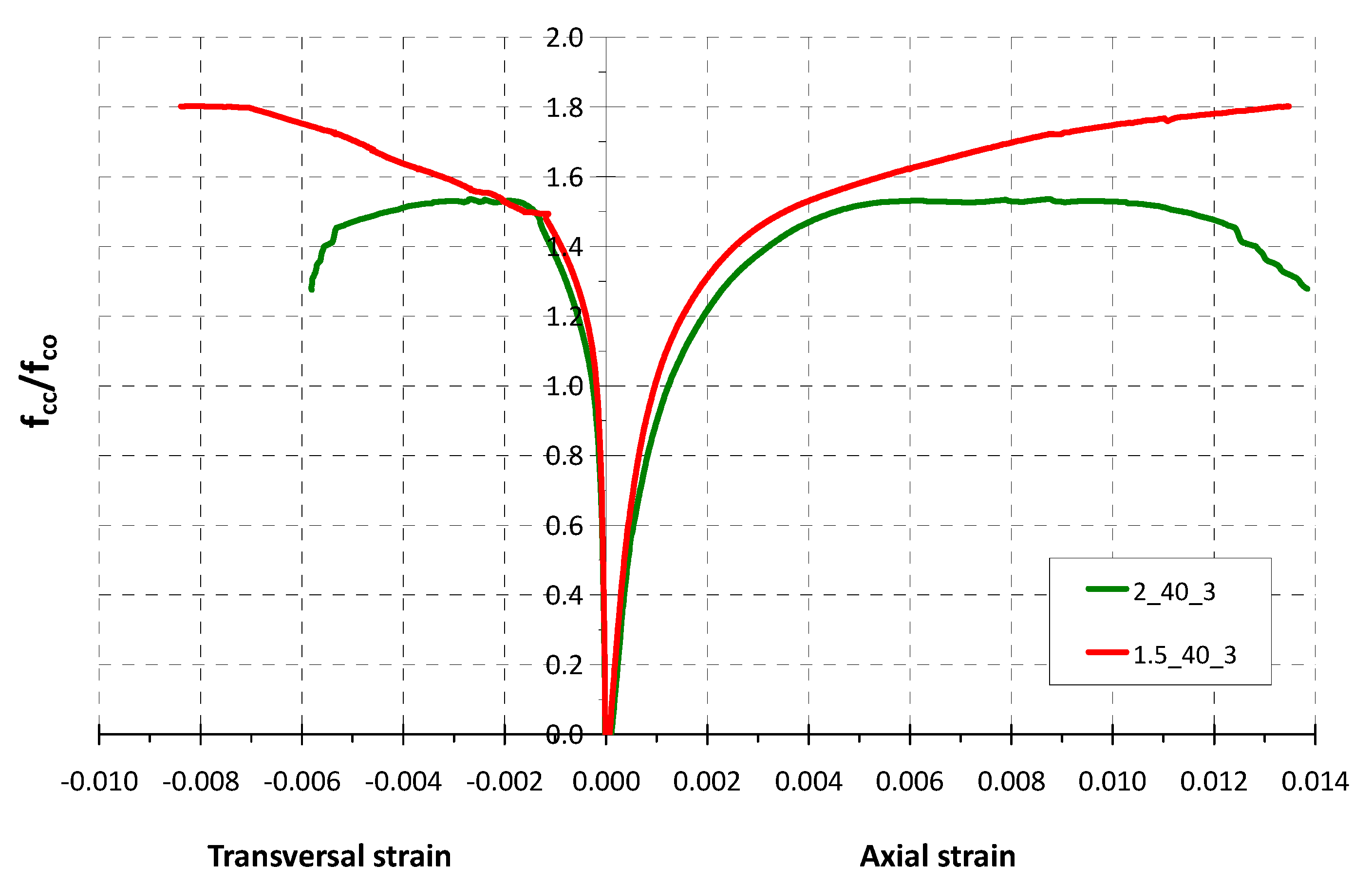
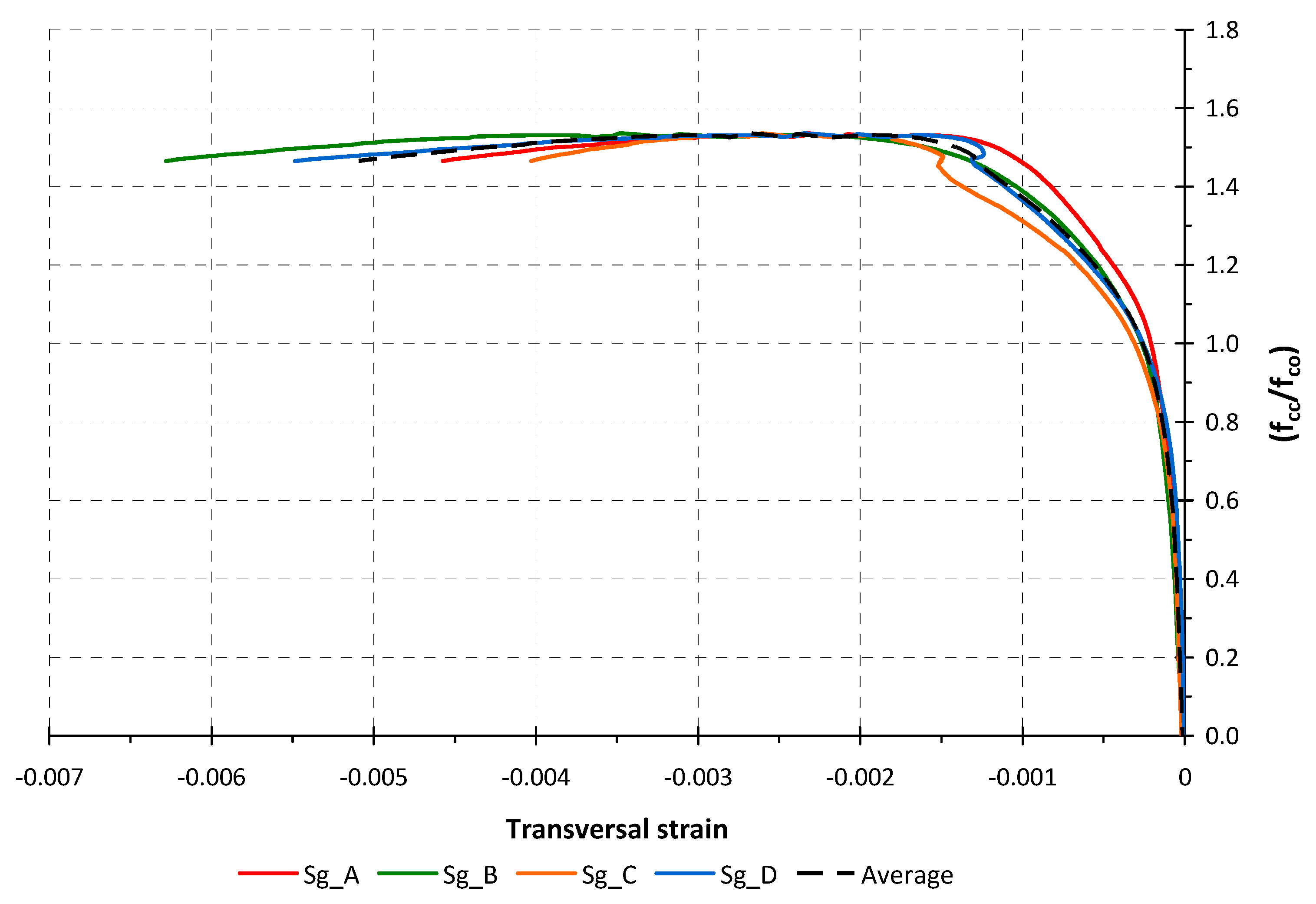
3.2. Strain-Stress Behaviour
3.3. FRP Jacket Strains Obtained by Testing
4. Comparison of Experimental Results with Recommendations of the Design Guides
5. Conclusions
- In the case of low strength concrete, the FRP confinement can significantly improve not only the strain capacity but also the strength of rectangular section RC columns with rounded corners. A strength improvement of 80% was obtained for a rectangular section specimen with a side-aspect rate (b/d) of 1.5 and 54% for a column with a side-aspect rate (b/d) of 2, both were reinforced with three layers of CFRP.
- The ultimate axial strain of concrete increased noticeably due to FRP confinement action, reaching values between 0.009 (0.9%) and 0.014 (1.4%) in the performed tests. For practical applications, high values of axial strains must be avoided. In this sense, some design guides (ACI, TR55) limit the ultimate concrete strain to 0.01.
- The failure usually occurs suddenly and explosively by tensile rupture of the jacket fibres to a strain value much lower than that obtained by tensile testing of FRP coupons.
- The strain efficiency factor (εf,eff/εf) is a key parameter for the calculation of FRP wrappings. The values obtained in the tests presented are lower than those usually recommended by the design guides (around 0.55–0.60). The strain efficiency factors (εf, eff/εf) from the tests were between 0.34 and 0.53 with an average value of 0.44.
- The results obtained so far in this experimental program show that the strain efficiency factor (εf,eff/εf) decreases with increasing cross section side-aspect ratio (b/d) although more tests are needed to support this finding.
- The predictions of the theoretical capacity of the FRP-confined columns using the formulation of three existing codes (without applying the safety factors for materials) show that these regulations appear to be conservative for low-strength concrete columns with a side-aspect ratio of the concrete section (b/d) greater than 1.5.
- The predictions obtained using the TR55 guide fit better the experimental results. The approach by the Concrete Society TR55 2012, whose formulation includes separately the FRP jacket ultimate strain and the effect of confinement stiffness, seems to be a valuable contribution, but further research is needed.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Lam, L.; Teng, J.G. Behaviour and modelling of fiber reinforced polymer-confined concrete. J. Struct. Eng. 2004, 130, 1713–1723. [Google Scholar]
- Ozbakkaloglu, T.; Lim, J.C.; Vincent, T. FRP-confined concrete in circular sections: Review and assessment of stress–strain models. Eng. Struct. 2013, 49, 1068–1088. [Google Scholar] [CrossRef]
- Mirmiran, A.; Shahawy, M.; Samaan, M.; El Echary, H.; Mastrapa, J.C.; Pico, O. Effect of Column Parameters on FRP-Confined Concrete. J. Compos. Constr. 1998, 2, 175–185. [Google Scholar] [CrossRef]
- Pessiki, S.; Harries, K.A.; Kestner, J.; Sause, R.; Ricles, J.M. The axial behaviour of concrete confined with fiber reinforced composite jackets. J. Compos. Constr. 2001, 5, 237–245. [Google Scholar] [CrossRef]
- Moran, D.A.; Pantelides, C.P. Stress-Strain Model for Fiber-Reinforced Polymer-Confined Concrete. J. Compos. Constr. 2002, 6, 233–240. [Google Scholar] [CrossRef]
- Wang, L.-M.; Wu, Y.-F. Effect of corner radius on the performance of CFRP-confined square concrete columns: Test. Eng. Struct. 2008, 30, 493–505. [Google Scholar] [CrossRef]
- Wu, Y.-F.; Wei, Y.-Y. Effect of cross-sectional aspect ratio on the strength of CFRP-confined rectangular concrete columns. Eng. Struct. 2010, 32, 32–45. [Google Scholar] [CrossRef]
- Vincent, T.; Ozbakkaloglu, T. Influence of fiber orientation and specimen end condition on axial compressive behaviour of FRP-confined concrete. Constr. Build. Mater. 2013, 47, 814–826. [Google Scholar] [CrossRef]
- Wu, Y.-F.; Jiang, J.-F. Effective strain of FRP for confined circular concrete columns. Compos. Struct. 2013, 95, 479–491. [Google Scholar] [CrossRef]
- Eid, R.; Paultre, P. Compressive behavior of FRP-confined reinforced concrete columns. Eng. Struct. 2017, 132, 518–530. [Google Scholar] [CrossRef]
- De Luca, A.; Nardone, F.; Matta, F.; Nanni, A.; Lignola, G.P.; Prota, A. Structural Evaluation of Full-Scale FRP-Confined Reinforced Concrete Columns. J. Compos. Constr. 2011, 15, 112–123. [Google Scholar] [CrossRef]
- Lam, L.; Teng, J. Design-oriented stress–strain model for FRP-confined concrete. Constr. Build. Mater. 2003, 17, 471–489. [Google Scholar] [CrossRef]
- Nisticò, N.; Monti, G. RC square sections confined by FRP: Analytical prediction of peak strength. Compos. Part. B Eng. 2013, 45, 127–137. [Google Scholar] [CrossRef]
- De Diego, A.; Arteaga, Á.; Fernández, J. Strengthening of square concrete columns with composite materials. Investigation on the FRP jacket ultimate strain. Compos. Part B Eng. 2019, 162, 454–460. [Google Scholar] [CrossRef]
- Chaallal, O.; Hassan, M.; Shahawy, M. Performance of axially loaded short columns strengthened with CFRP wrapping. J. Compos. Constr. ASCE 2003, 7, 200–208. [Google Scholar] [CrossRef]
- Lam, L.; Teng, J.G. Design-Oriented Stress-Strain Model for FRP-Confined Concrete in Rectangular Columns. J. Reinf. Plast. Compos. 2003, 22, 1149–1186. [Google Scholar] [CrossRef]
- Maalej, M.; Tanwongsval, S.; Paramasivam, P. Modelling of rectangular RC columns strengthened with FRP. Cem. Concr. Compos. 2003, 25, 263–276. [Google Scholar] [CrossRef]
- Rochette, P.; Labossière, P. Axial Testing of Rectangular Column Models Confined with Composites. J. Compos. Constr. 2000, 4, 129–136. [Google Scholar] [CrossRef]
- Wang, Y.C.; Restrepo, J.I. Investigation of concentrically loaded reinforced concrete columns confined with glass fiber-reinforced polymer jackets. ACI Struct. J. 2001, 98, 377–385. [Google Scholar]
- Rocca, S. Experimental and Analytical Evaluation of FRP-Confined Large Size Reinforced Concrete Columns. Ph.D. Thesis, University of Missouri-Rolla, Rolla, MO, USA, 2007. [Google Scholar]
- Toutanji, H.; Han, M.; Gilbert, J.; Matthys, S. Behavior of Large-Scale Rectangular Columns Confined with FRP Composites. J. Compos. Constr. 2010, 14, 62–71. [Google Scholar] [CrossRef]
- Zeng, J.; Lin, G.; Teng, J.; Li, L. Behavior of large-scale FRP-confined rectangular RC columns under axial compression. Eng. Struct. 2018, 174, 629–645. [Google Scholar] [CrossRef]
- Pham, T.M.; Hadi, M.N.S. Stress Prediction Model for FRP Confined Rectangular Concrete Columns with Rounded Corners. J. Compos. Constr. 2014, 18, 04013019. [Google Scholar] [CrossRef]
- Wu, Y.-F.; Wei, Y. General Stress-Strain Model for Steel- and FRP-Confined Concrete. J. Compos. Constr. 2015, 19, 04014069. [Google Scholar] [CrossRef]
- ACI Committee 440 440.2R-17. Guide for the Design and Construction of Externally Bonded FRP Systems for Strengthening Concrete Structures; American Concrete Institute (ACI): Farmington Hills, MI, USA, 2017. [Google Scholar]
- National Research Council, Advisory Committee on Technical Recommendations for Construction. Guide for the Design and Construction of Externally Bonded FRP Systems for Strengthening Existing Structures, CNR-DT200_R1; National Research Council (CNR): Rome, Italy, 2013. [Google Scholar]
- Concrete Society. Technical Report. Design Guidance for Strengthening Concrete Structures Using Fibre Composite Materials, 3rd ed.; TR55; Concrete Society: Camberley, UK, 2012. [Google Scholar]
- Triantafillou, T.; Matthys, S.; Audenaert, K.; Balázs, G.; Blaschko, M.; Blontrock, H.; Czaderski, C.; David, E.; Di Tomasso, A.; Duckett, W.; et al. Fib Bulletin 14. Externally Bonded FRP Reinforcement for RC Structures; 2001; Available online: https://www.fib-international.org/publications/fib-bulletins/externally-bonded-frp-reinforcement-for-rc-structures-100-detail.html (accessed on 9 July 2020).
- Wei, Y.-Y.; Wu, Y.-F. Unified stress–strain model of concrete for FRP-confined columns. Constr. Build. Mater. 2012, 26, 381–392. [Google Scholar] [CrossRef]
- Masia, M.J.; Gale, T.N.; Shrive, N.G. Size effects in axially loaded square-section concrete prisms strengthened using carbon fibre reinforced polymer wrapping. Can. J. Civ. Eng. 2004, 31, 1–13. [Google Scholar] [CrossRef]
- Martínez, S.; Gutiérrez, J.P.; de Diego, A.; Castro, V.J.; Echevarría, L.; Barroso, F.J.; López, C. Strengthening of low-strength concrete columns with fibre reinforced polymers. Full-scale tests. In Proceedings of the Rehabend 2020, 8th Euro-American Congress, Construction Pathology, Rehabilitation Technology and Heritage Management, Granada, Spain, 24–27 March 2020. [Google Scholar]
- ISO 527-4 1997. Plastics—Determination of Tensile Properties—Part 4: Test Conditions for Isotropic and Orthotropic Fibre-Reinforced Plastic Composites; British Standards Institution: London, UK, 1997. [Google Scholar]
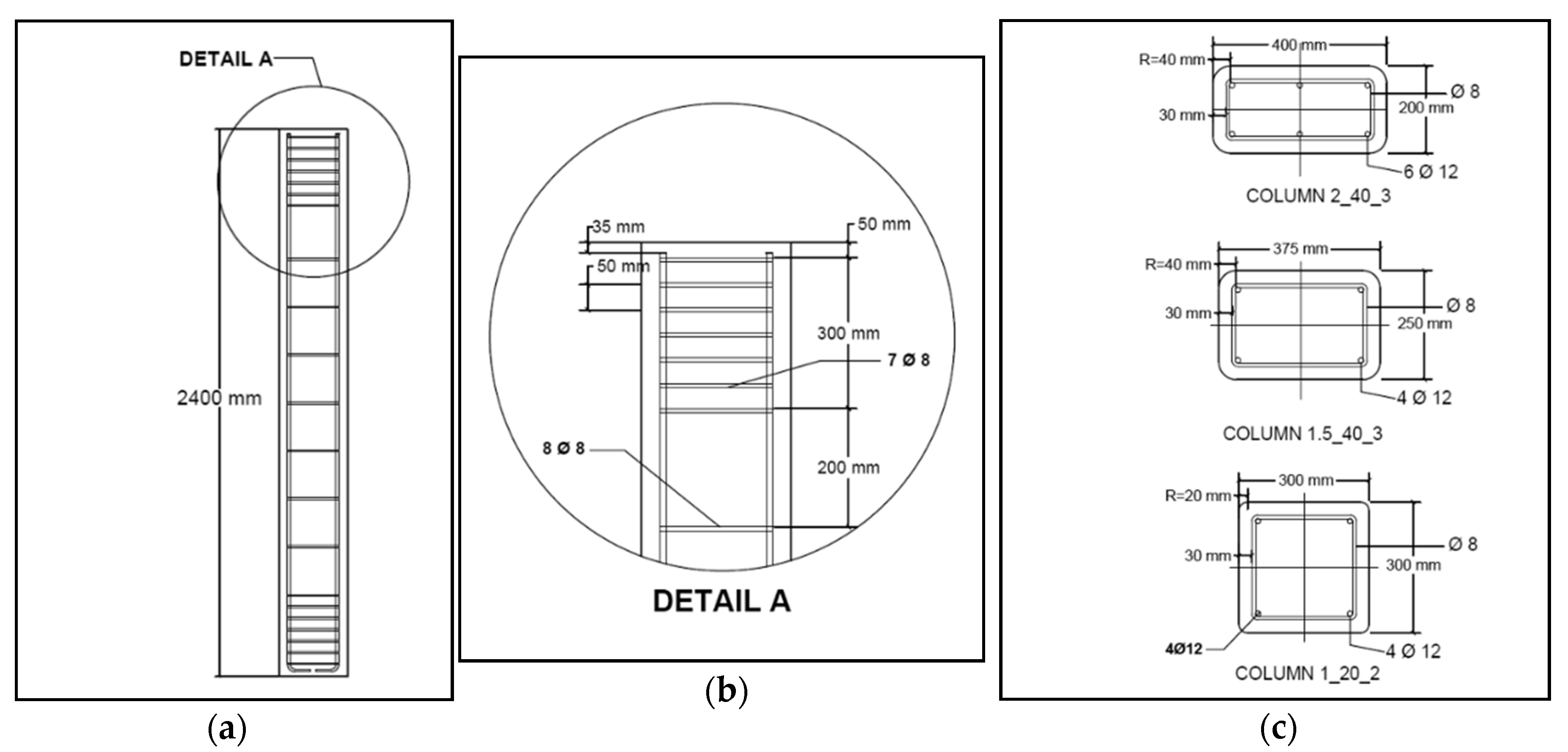
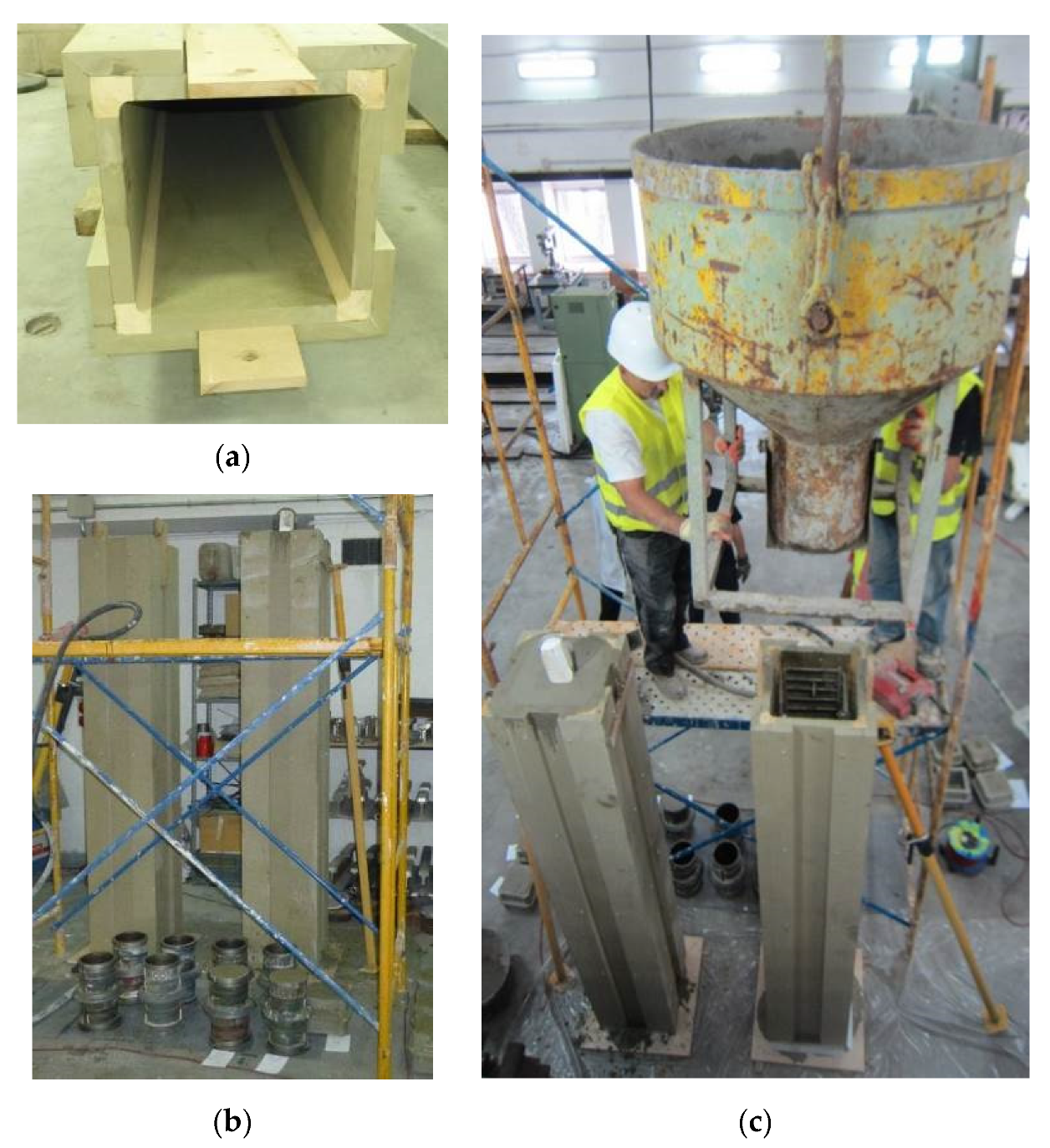






| Specimen | Section (mm2) | b/d | Rc (mm) | Number of CFRP Layers |
|---|---|---|---|---|
| Reference | 300 × 300 | 1 | - | - |
| 1_20_2 | 300 × 300 | 1 | 20 | 2 |
| 1.5_40_3 | 250 × 375 | 1.5 | 40 | 3 |
| 2_40_3 | 200 × 400 | 2 | 40 | 3 |
| Specimen | fco (N/mm2) | Qmax (kN) | fcc (N/mm2) | fcc/fco | εcc | εf,eff | εf,eff/εf | εf,max |
|---|---|---|---|---|---|---|---|---|
| Reference | 15.86 | 1660.43 | - | - | 0.00220 | - | - | - |
| 1_20_2 | 19.85 | 2623.30 | 26.87 | 1.35 | 0.00889 | 0.00906 | 0.53 | 0.01681 |
| 1.5_40_3 | 17.44 | 3115.43 | 31.43 | 1.80 | 0.01350 | 0.00838 | 0.49 | 0.01642 |
| 2_40_3 | 20.09 | 2743.80 | 30.85 | 1.54 | 0.01386 | 0.00581 | 0.34 | 0.00628 |
| Guide | Compressive Strength of Confined Concrete |
|---|---|
| ACI-440.2R (2017) | f′cc = f′c + ψf 3.3 ka fl |
| CONCRETE SOCIETY TR55 (2012) | (f′cc / f′c) = 1 + 5.25 (kε ρk − 0.01) ρε |
| CNR-DT 200 (2013) | (f′cc / f′c) = 1 + 2.6 (fl,eff /f′c) 2/3 |
| Specimen | fco (N/mm2) | fcc (N/mm2) Experimental | fcc (N/mm2) ACI-440.2R | fcc (N/mm2) TR55 | fcc (N/mm2) CNR-DT 200 |
|---|---|---|---|---|---|
| 1_20_2 | 19.85 | 26.87 | 24.19 | 25.63 | 24.48 |
| 1.5_40_3 | 17.44 | 31.43 | 20.93 | 29.08 | 22.47 |
| 2_40_3 | 20.09 | 30.85 | 22.15 | 32.79 | 23.91 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Martínez, S.; de Diego, A.; Castro, V.J.; Echevarría, L.; Barroso, F.J.; Rentero, G.; Soldado, R.P.; Gutiérrez, J.P. Strengthening of Low-Strength Concrete Columns with Fibre Reinforced Polymers. Full-Scale Tests. Infrastructures 2020, 5, 91. https://doi.org/10.3390/infrastructures5110091
Martínez S, de Diego A, Castro VJ, Echevarría L, Barroso FJ, Rentero G, Soldado RP, Gutiérrez JP. Strengthening of Low-Strength Concrete Columns with Fibre Reinforced Polymers. Full-Scale Tests. Infrastructures. 2020; 5(11):91. https://doi.org/10.3390/infrastructures5110091
Chicago/Turabian StyleMartínez, Sonia, Ana de Diego, Viviana J. Castro, Luis Echevarría, Francisco J. Barroso, Gabriel Rentero, Rafael P. Soldado, and José Pedro Gutiérrez. 2020. "Strengthening of Low-Strength Concrete Columns with Fibre Reinforced Polymers. Full-Scale Tests" Infrastructures 5, no. 11: 91. https://doi.org/10.3390/infrastructures5110091
APA StyleMartínez, S., de Diego, A., Castro, V. J., Echevarría, L., Barroso, F. J., Rentero, G., Soldado, R. P., & Gutiérrez, J. P. (2020). Strengthening of Low-Strength Concrete Columns with Fibre Reinforced Polymers. Full-Scale Tests. Infrastructures, 5(11), 91. https://doi.org/10.3390/infrastructures5110091





