Selection of Magneto-Optical Material for a Faraday Isolator Operating in High-Power Laser Radiation
Abstract
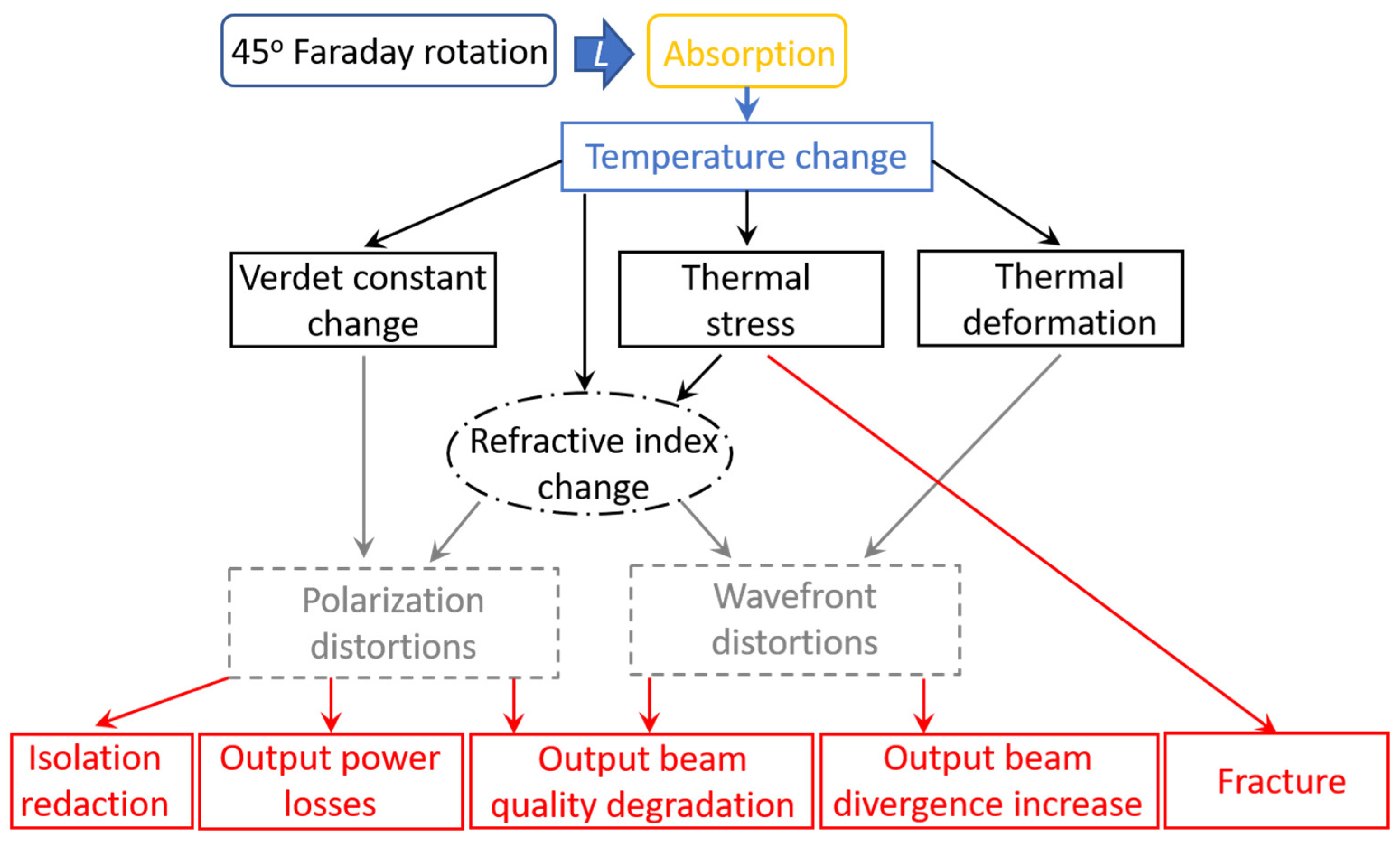
1. Introduction
2. Magneto-Optical Materials of Different Types: Advantages and Shortcomings
3. Approaches to the Creation of Faraday Isolators for High-Power Laser Radiation
4. Comparison and Ranking of Magneto-Optical Materials
5. Overview of Some Trend Magneto-Optical Ceramic Materials
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Scott, G.; Lacklison, D. Magnetooptic properties and applications of bismuth substituted iron garnets. IEEE Trans. Magn. 1976, 12, 292–311. [Google Scholar] [CrossRef]
- Mironov, E.A.; Voitovich, A.V.; Palashov, O.V. Apodizing diaphragm based on the Faraday effect. Opt. Commun. 2013, 295, 170–175. [Google Scholar] [CrossRef]
- Cooper, R.W.; Page, J.L. Magneto-optic light modulators. Radio Electron. Eng. 1970, 39, 302–304. [Google Scholar] [CrossRef]
- Dick, D.J.; Shay, T.M. Ultrahigh-noise rejection optical filter. Opt. Lett. 1991, 16, 867–869. [Google Scholar] [CrossRef] [PubMed]
- Hebner, R.E.; Malewski, R.A.; Cassidy, E.C. Optical methods of electrical measurement at high voltage levels. Proc. IEEE 1977, 65, 1524–1548. [Google Scholar] [CrossRef]
- Emmelius, M.; Pawlowski, G.; Vollmann, H.W. Materials for Optical Data Storage. Angew. Chem. Int. Edit. 1989, 28, 1445–1471. [Google Scholar] [CrossRef]
- Khazanov, E.A. Thermooptics of magnetoactive medium: Faraday isolators for high average power lasers. Phys. Usp. 2016, 59, 886–909. [Google Scholar] [CrossRef]
- Vojna, D.; Slezák, O.; Lucianetti, A.; Mocek, T. Verdet Constant of Magneto-Active Materials Developed for High-Power Faraday Devices. Appl. Sci. 2019, 9, 3160. [Google Scholar] [CrossRef]
- Palashov, O.V.; Starobor, A.V.; Perevezentsev, E.A.; Snetkov, I.L.; Mironov, E.A.; Yakovlev, A.I.; Balabanov, S.S.; Permin, D.A.; Belyaev, A.V. Thermo-Optical Studies of Laser Ceramics. Materials 2021, 14, 3944. [Google Scholar] [CrossRef]
- Ikesue, A.; Aung, Y.L. Magneto-Optic Transparent Ceramics. In Processing of Ceramics; Wiley Online Library: Hoboken, HJ, USA, 2021; pp. 143–185. [Google Scholar]
- Adhikari, R.X.; Arai, K.; Brooks, A.F.; Wipf, C.; Aguiar, O.; Altin, P.; Barr, B.; Barsotti, L.; Bassiri, R.; Bell, A.; et al. A cryogenic silicon interferometer for gravitational-wave detection. Class. Quant. Grav. 2020, 37, 165003. [Google Scholar] [CrossRef]
- Danson, C.N.; Haefner, C.; Bromage, J.; Butcher, T.; Chanteloup, J.-C.F.; Chowdhury, E.A.; Galvanauskas, A.; Gizzi, L.A.; Hein, J.; Hillier, D.I.; et al. Petawatt and exawatt class lasers worldwide. High Power Laser Sci. Eng. 2019, 7, e54. [Google Scholar] [CrossRef]
- Kuzmina, M.S.; Khazanov, E.A. Influence of cubic nonlinearity on compensation of thermally induced polarisation distortions in Faraday isolators. Quantum Electron. 2013, 43, 936. [Google Scholar] [CrossRef]
- Khazanov, E.A.; Kulagin, O.V.; Yoshida, S.; Tanner, D.; Reitze, D. Investigation of self-induced depolarization of laser radiation in terbium gallium garnet. IEEE J. Quantum Elect. 1999, 35, 1116–1122. [Google Scholar] [CrossRef]
- Mironov, E.A.; Starobor, A.V.; Snetkov, I.L.; Palashov, O.V.; Furuse, H.; Tokita, S.; Yasuhara, R. Thermo-optical and magneto-optical characteristics of CeF3 crystal. Opt. Mater. 2017, 69, 196–201. [Google Scholar] [CrossRef]
- Khazanov, E.; Andreev, N.; Palashov, O.; Poteomkin, A.; Sergeev, A.; Mehl, O.; Reitze, D. Effect of terbium gallium garnet crystal orientation on the isolation ratio of a Faraday isolator at high average power. Appl. Opt. 2002, 41, 483–492. [Google Scholar] [CrossRef]
- Snetkov, I.L. Features of thermally induced depolarization in magneto-active media with negative optical anisotropy parameter. IEEE J. Quantum Electron. 2018, 54, 1–8. [Google Scholar] [CrossRef]
- Yakovlev, A.; Snetkov, I.L. Thermal lens astigmatism induced by the photoelastic effect in m3m, 432, and 43m symmetry cubic crystals. IEEE J. Quantum Electron. 2020, 56, 6100108. [Google Scholar] [CrossRef]
- Ivanov, I.A.; Karimov, D.N.; Snetkov, I.L.; Palashov, O.V.; Kochurikhin, V.V.; Masalov, A.V.; Fedorov, V.A.; Ksenofontov, D.A.; Kabalov, Y.K. Study of the influence of Tb-Sc-Al garnet crystal composition on Verdet constant. Opt. Mater. 2017, 66, 106–109. [Google Scholar] [CrossRef]
- Popov, P.A.; Ivanov, I.A.; Karimov, D.N. Investigation of the Thermal Conductivity Terbium Gallium and Terbium Scandium Aluminum Garnet Crystals. Crystallog. Rep. 2018, 63, 451–455. [Google Scholar] [CrossRef]
- Ikesue, A.; Aung, Y.L. Ceramic laser materials. Nat. Photonics 2008, 2, 721–727. [Google Scholar] [CrossRef]
- Furuse, H.; Okabe, T.; Shirato, H.; Kato, D.; Horiuchi, N.; Morita, K.; Kim, B.-N. High-optical-quality non-cubic Yb3+-doped Ca10(PO4)6F2 (Yb:FAP) laser ceramics. Opt. Mater. Express 2021, 11, 1756–1762. [Google Scholar] [CrossRef]
- Sato, Y.; Akiyama, J.; Taira, T. Orientation control of micro-domains in anisotropic laser ceramics. Opt. Mater. Express 2013, 3, 829–841. [Google Scholar] [CrossRef]
- Sato, Y.; Arzakantsyan, M.; Akiyama, J.; Taira, T. Anisotropic Yb:FAP laser ceramics by micro-domain control. Opt. Mater. Express 2014, 4, 2006–2015. [Google Scholar] [CrossRef]
- Scott, C.; Kaliszewski, M.; Greskovich, C.; Levinson, L. Conversion of Polycrystalline Al2O3 into Single-Crystal Sapphire by Abnormal Grain Growth. J. Am. Ceram. Soc. 2002, 85, 1275–1280. [Google Scholar] [CrossRef]
- Mukhin, I.B.; Palashov, O.V.; Khazanov, E.A.; Ikesue, A.; Aung, Y.L. Experimental study of thermally induced depolarization in Nd:YAG ceramics. Opt. Express 2005, 13, 5983–5987. [Google Scholar] [CrossRef]
- Soloviev, A.A.; Snetkov, I.L.; Zelenogorsky, V.V.; Kozhevatov, I.E.; Palashov, O.V.; Khazanov, E.A. Experimental study of thermal lens features in laser ceramics. Opt. Express 2008, 16, 21012–21021. [Google Scholar] [CrossRef]
- Vyatkin, A.G.; Khazanov, E.A. Thermally induced scattering of radiation in laser ceramics with arbitrary grain size. J. Opt. Soc. Am. B 2012, 29, 3307–3316. [Google Scholar] [CrossRef]
- Snetkov, I.L.; Soloviev, A.A.; Khazanov, E.A. Study of a thermal lens in thin laser-ceramics discs. Quantum Electron. 2009, 39, 302–308. [Google Scholar] [CrossRef]
- Vyatkin, A.G.; Khazanov, E.A. Thermally Induced Beam Distortions in Sesquioxide Laser Ceramics of m3 Crystal Class-Part II. IEEE J. Quantum Electron. 2015, 51, 1700108. [Google Scholar] [CrossRef]
- Khazanov, E.A.; Maslennikov, O.V.; Ginzburg, V.N.; Kochetkov, A.A.; Nekorkin, V.I. Third-order-nonlinear effects in single crystals with arbitrary orientation and in ceramics. Opt. Express 2017, 25, 27968–27980. [Google Scholar] [CrossRef]
- Mironov, E.A.; Snetkov, I.L.; Voitovich, A.V.; Palashov, O.V. Permanent-magnet Faraday isolator with the field intensity of 25 kOe. Quantum Electron. 2013, 43, 740–743. [Google Scholar] [CrossRef]
- Mironov, E.A.; Voitovich, A.V.; Palashov, O.V. Permanent-magnet Faraday isolator with the field intensity of more than 3 tesla. Laser Phys. Lett. 2020, 17, 015001. [Google Scholar] [CrossRef]
- Snetkov, I.; Yakovlev, A. Faraday isolator based on crystalline silicon for 2-µm laser radiation. Opt. Lett. 2022, 47, 1895–1898. [Google Scholar] [CrossRef] [PubMed]
- Mironov, E.A.; Voitovich, A.V.; Starobor, A.V.; Palashov, O.V. Compensation of polarization distortions in Faraday isolators by means of magnetic field inhomogeneity. Appl. Opt. 2014, 53, 3486–3491. [Google Scholar] [CrossRef]
- Buhrer, C.F. Wideband temperature-compensated optical isolator or circulator configuration using two Faraday elements. Opt. Lett. 1989, 14, 1180–1182. [Google Scholar] [CrossRef]
- Mukhin, I.B.; Khazanov, E.A. Use of thin discs in Faraday isolators for high-average-power lasers. Quantum Electron. 2004, 34, 973–978. [Google Scholar] [CrossRef]
- Joiner, R.E.; Marburger, J.; Steier, W.H. Elimination of stress-induced birefringence effects in single-crystal high-power laser windows. Appl. Phys. Lett. 1977, 30, 485–486. [Google Scholar] [CrossRef]
- Khazanov, E.A. Compensation of thermally induced polarization distortions in Faraday isolators. Quantum Electron. 1999, 29, 59–64. [Google Scholar] [CrossRef]
- Snetkov, I.L.; Palashov, O.V.; Mukhin, I.B.; Khazanov, E.A. Compensation of thermally induced depolarization in Faraday isolators for high average power lasers. Opt. Express 2011, 19, 6366–6376. [Google Scholar] [CrossRef]
- Snetkov, I.; Palashov, O. Faraday isolator based on a TSAG single crystal with compensation of thermally induced depolarization inside magnetic field. Opt. Mater. 2015, 42, 293–297. [Google Scholar] [CrossRef]
- Starobor, A.V.; Snetkov, I.L.; Palashov, O.V. TSAG-based Faraday isolator with depolarization compensation using a counterrotation scheme. Opt. Lett. 2018, 43, 3774–3777. [Google Scholar] [CrossRef] [PubMed]
- Yakovlev, A.; Snetkov, I.; Palashov, O. The dependence of optical anisotropy parameter on dopant concentration in Yb:CaF2 and Tb:CaF2 crystals. Opt. Mater. 2018, 77, 127–131. [Google Scholar] [CrossRef]
- Starobor, A.V.; Zheleznov, D.S.; Palashov, O.V.; Khazanov, E.A. Magnetoactive media for cryogenic Faraday isolators. J. Opt. Soc. Am. B 2011, 28, 1409–1415. [Google Scholar] [CrossRef]
- Starobor, A.; Yasyhara, R.; Snetkov, I.; Mironov, E.; Palashov, O. TSAG-based cryogenic Faraday isolator. Opt. Mater. 2015, 47, 112–117. [Google Scholar] [CrossRef]
- Snetkov, I.L. Compensation for thermally induced depolarization in magneto-optical media made of materials with a negative optical anisotropy parameter. IEEE J. Quantum Electron. 2021, 57, 1–8. [Google Scholar] [CrossRef]
- Robinson, C.C. The Faraday rotation of diamagnetic glasses from 0.334 micrometer to 1.9 micrometer. Appl. Opt. 1964, 3, 1163–1166. [Google Scholar] [CrossRef]
- Padula, C.F.; Young, C.G. Optical isolators for high-power 1.06-micron glass laser systems. IEEE J. Quantum Elect. 1967, QE-3, 493–498. [Google Scholar] [CrossRef]
- Andreev, N.F.; Palashov, O.V.; Poteomkin, A.K.; Sergeev, A.M.; Khazanov, E.A.; Reitze, D.H. A 45-dB Faraday isolator for 100-W average radiation power. Quantum Electron. 2000, 30, 1107–1108. [Google Scholar] [CrossRef]
- Snetkov, I.L.; Voitovich, A.V.; Palashov, O.V.; Khazanov, E.A. Review of Faraday isolators for kilowatt average power lasers. IEEE J. Quantum Elect. 2014, 50, 434–443. [Google Scholar] [CrossRef]
- Snetkov, I.L.; Vyatkin, A.G.; Palashov, O.V.; Khazanov, E.A. Drastic reduction of thermally induced depolarization in CaF2 crystals with [111] orientation. Opt. Express 2012, 20, 13357–13367. [Google Scholar] [CrossRef]
- Mironov, E.A.; Vyatkin, A.G.; Palashov, O.V. Measurements of thermo-optical characteristics of cubic crystals using samples of arbitrary orientation. IEEE J. Quantum Electron. 2016, 40, 1–8. [Google Scholar] [CrossRef]
- Snetkov, I.L.; Balashov, V.V. Thermo-optical properties of Ho:Y2O3 ceramics. Opt. Mater. 2020, 100, 109617. [Google Scholar] [CrossRef]
- Khazanov, E.; Andreev, N.; Babin, A.; Kiselev, A.; Palashov, O.; Reitze, D. Suppression of self-induced depolarization of high-power laser radiation in glass-based Faraday isolators. J. Opt. Soc. Am. B 2000, 17, 99–102. [Google Scholar] [CrossRef]
- Yasuhara, R.; Snetkov, I.; Starobor, A.; Mironov, E.; Palashov, O. Faraday rotator based on TSAG crystal with <001> orientation. Opt. Express 2016, 24, 15486–15493. [Google Scholar] [CrossRef]
- Mironov, E.A.; Palashov, O.V.; Voitovich, A.V.; Karimov, D.N.; Ivanov, I.A. Investigation of thermo-optical characteristics of magneto-active crystal Na0.37Tb0.63F2.26. Opt. Lett. 2015, 40, 4919–4922. [Google Scholar] [CrossRef] [PubMed]
- Jalali, A.A.; Rogers, E.; Stevens, K. Characterization and extinction measurement of potassium terbium fluoride single crystal for high laser power applications. Opt. Lett. 2017, 42, 899–902. [Google Scholar] [CrossRef] [PubMed]
- Starobor, A.; Palashov, O.; Zhou, S. Thermo-optical properties of terbium-aluminum garnet ceramics doped with silicon and titanium. Opt. Lett. 2016, 41, 1510–1513. [Google Scholar] [CrossRef]
- Snetkov, I.; Starobor, A.; Palashov, O.; Balabanov, S.; Permin, D.; Rostokina, E. Thermally induced effects in a faraday isolator on terbium sesquioxide (Tb2O3) ceramics. Opt. Mater. 2021, 120, 111466. [Google Scholar] [CrossRef]
- Mironov, E.A.; Palashov, O.V.; Balabanov, S.S. High-purity CVD-ZnSe polycrystal as a magneto-active medium for a multikilowatt Faraday isolator. Opt. Lett. 2021, 46, 2119–2122. [Google Scholar] [CrossRef]
- Borrelli, N.F. Faraday Rotation in Glasses. J. Chem. Phys. 1964, 41, 3289–3293. [Google Scholar] [CrossRef]
- Berger, S.B.; Rubinstein, C.B.; Kurkjian, C.R.; Treptow, A.W. Faraday Rotation of Rare-Earth (III) Phosphate Glasses. Phys. Rev. 1964, 133, A723–A727. [Google Scholar] [CrossRef]
- Snetkov, I.L.; Permin, D.A.; Balabanov, S.S.; Palashov, O.V. Wavelength dependence of Verdet constant of Tb3+:Y2O3 ceramics. Appl. Phys. Lett. 2016, 108, 161905. [Google Scholar] [CrossRef]
- Ikesue, A.; Aung, Y.L.; Makikawa, S.; Yahagi, A. Polycrystalline (TbXY1-X)2O3 Faraday rotator. Opt. Lett. 2017, 42, 4399–4401. [Google Scholar] [CrossRef]
- Balabanov, S.S.; Permin, D.A.; Rostokina, E.Y.; Palashov, O.V.; Snetkov, I.L. Characterizations of REE:Tb2O3 magneto-optical ceramics. Phys. Status Solidi 2020, 257, 1900474. [Google Scholar] [CrossRef]
- Hu, D.; Li, X.; Zhang, L.; Snetkov, I.; Chen, P.; Dai, Z.; Balabanov, S.; Palashov, O.; Li, J. Terbium (III) Oxide (Tb2O3) Transparent Ceramics by Two-Step Sintering from Precipitated Powder. Magnetochem. 2022, 8, 73. [Google Scholar] [CrossRef]
- Morales, J.R.; Amos, N.; Khizroev, S.; Garay, J.E. Magneto-optical Faraday effect in nanocrystalline oxides. J. Appl. Phys. 2011, 109, 093110. [Google Scholar] [CrossRef]
- Snetkov, I.L.; Yakovlev, A.I.; Permin, D.A.; Balabanov, S.S.; Palashov, O.V. Magneto-optical Faraday effect in dysprosium oxide (Dy2O3) based ceramics obtained by vacuum sintering. Opt. Lett. 2018, 43, 4041–4044. [Google Scholar] [CrossRef]
- Furuse, H.; Yasuhara, R. Magneto-optical characteristics of holmium oxide (Ho2O3) ceramics. Opt. Mater. Express 2017, 7, 827–833. [Google Scholar] [CrossRef]
- Balabanov, S.; Filofeev, S.; Ivanov, M.; Kaigorodov, A.; Kuznetsov, D.; Hu, D.J.; Li, J.; Palashov, O.; Permin, D.; Rostokina, E.; et al. Fabrication and characterizations of holmium oxide based magneto-optical ceramics. Opt. Mater. 2020, 101, 109741. [Google Scholar] [CrossRef]
- Hu, D.; Li, X.; Snetkov, I.; Yakovlev, A.; Balabanov, S.; Ivanov, M.; Liu, X.; Liu, Z.; Tian, F.; Xie, T.; et al. Fabrication, microstructure and optical characterizations of holmium oxide (Ho2O3) transparent ceramics. J. Europ. Ceram. Soc. 2021, 41, 759–767. [Google Scholar] [CrossRef]
- Balabanov, S.; Filofeev, S.; Ivanov, M.; Kaigorodov, A.; Krugovykh, A.; Kuznetsov, D.; Permin, D.; Popov, P.; Rostokina, E. Fabrication and characterizations of erbium oxide based optical ceramics. Opt. Mater. 2020, 101, 109732. [Google Scholar] [CrossRef]
- Yakovlev, A.; Balabanov, S.; Permin, D.; Ivanov, M.; Snetkov, I. Faraday rotation in erbium oxide based ceramics. Opt. Mater. 2020, 101, 109750. [Google Scholar] [CrossRef]
- Permin, D.A.; Novikova, A.V.; Koshkin, V.A.; Balabanov, S.S.; Snetkov, I.L.; Palashov, O.V.; Smetanina, K.E. Fabrication and magneto-optical properties of Yb2O3 based ceramics. Magnetochem. 2020, 6, 63. [Google Scholar] [CrossRef]
- Zhang, C.; Wang, X.; Liang, L.; Yuan, H.; Qi, J.; Liao, Z.; Lu, T. Vacuum sintering of Yb2O3 transparent ceramics: Effect of ZrO2 concentration on structural and optical properties. J. Alloys Compd. 2022, 907, 164454. [Google Scholar] [CrossRef]
- Wang, X.; Lu, B.; Feng, K.; Liu, Y. Vacuum sintering of novel transparent Tm2O3 ceramics: Effect of silica additive and thermal performance. J. Europ. Ceram. Soc. 2020, 40, 6249–6253. [Google Scholar] [CrossRef]
- Xu, X.; Zhu, L.-L.; Dai, M.-M.; Zhao, C.-C.; Zhang, G.-X.; Jian, Y.-J.; Lin, H.-T. Fabrication and characterization of highly transparent ZrO2-doped Tm2O3 ceramics. Ceram. Int. 2021, 47, 28859–28865. [Google Scholar] [CrossRef]
- Snetkov, I.; Yakovlev, A.; Starobor, A.; Balabanov, S.; Permin, D.; Rostokina, E.; Palashov, O. Thermo-optical properties of terbium sesquioxide (Tb2O3) ceramics at room temperature. Opt. Lett. 2021, 46, 3592–3595. [Google Scholar] [CrossRef]
- Yakovlev, A.; Snetkov, I.; Palashov, O. Thermo-optical properties of cryogenically cooled (Tb0.9Y0.1)2O3 ceramics. Opt. Commun. 2022, 504, 127508. [Google Scholar] [CrossRef]
- Ikesue, A.; Aung, Y.L.; Makikawa, S.; Yahagi, A. Total performance of magneto-optical ceramics with a bixbyite structure. Materials 2019, 12, 421. [Google Scholar] [CrossRef]
- Vojna, D.; Slezák, O.; Yasuhara, R.; Furuse, H.; Lucianetti, A.; Mocek, T. Faraday Rotation of Dy2O3, CeF3 and Y3Fe5O12 at the Mid-Infrared Wavelengths. Materials 2020, 13, 5324. [Google Scholar] [CrossRef]
- Balabanov, S.; Filofeev, S.; Kaygorodov, A.; Khrustov, V.; Kuznetsov, D.; Novikova, A.; Permin, D.; Popov, P.; Ivanov, M. Hot pressing of Ho2O3 and Dy2O3 based magneto-optical ceramics. Opt. Mater. X 2022, 13, 100125. [Google Scholar] [CrossRef]
- Zheleznov, D.; Starobor, A.; Palashov, O.; Chen, C.; Zhou, S. High-power Faraday isolators based on TAG ceramics. Opt. Express 2014, 22, 2578–2583. [Google Scholar] [CrossRef] [PubMed]
- Dai, J.; Snetkov, I.L.; Palashov, O.V.; Pan, Y.; Kou, H.; Li, J. Fabrication, microstructure and magneto-optical properties of Tb3Al5O12 transparent ceramics. Opt. Mater. 2016, 62, 205–210. [Google Scholar] [CrossRef]
- Snetkov, I.L.; Yasuhara, R.; Starobor, A.V.; Mironov, E.A.; Palashov, O.V. Thermo-optical and magneto-optical characteristics of terbium scandium aluminum garnet crystals. IEEE J. Quantum Electron. 2015, 51, 7000307. [Google Scholar] [CrossRef]
- Mironov, E.A.; Palashov, O.V.; Karimov, D.N. EuF2-based crystals as media for high-power mid-infrared Faraday isolators. Scripta Mater. 2019, 162, 54–57. [Google Scholar] [CrossRef]
- Mironov, E.A.; Palashov, O.V. Spectral, magneto-optical and thermo-optical properties of terbium containing cubic zirconia crystal. Appl. Phys. Lett. 2018, 113, 063504. [Google Scholar] [CrossRef]
- Geller, S.; Gilleo, M.A. Structure and ferrimagnetism of yttrium and rare-earth-iron garnets. Acta. Crystallogr. 1957, 10, 239. [Google Scholar] [CrossRef]
- Ikesue, A.; Aung, Y.L. Development of optical grade polycrystalline YIG ceramics for faraday rotator. J. Am. Ceram. Soc. 2018, 101, 5120–5126. [Google Scholar] [CrossRef]
- Ikesue, A.; Aung, Y.L.; Yasuhara, R.; Iwamoto, Y. Giant Faraday rotation in heavily ce-doped YIG bulk ceramics. J. Europ. Ceram. Soc. 2020, 40, 6073–6078. [Google Scholar] [CrossRef]
- Yang, Y.; Li, X.; Liu, Z.; Hu, D.; Liu, X.; Chen, P.; Tian, F.; Zhu, D.; Zhang, L.; Li, J. Pressureless Sintering of YIG Ceramics from Coprecipitated Nanopowders. Magnetochem. 2021, 7, 56. [Google Scholar] [CrossRef]
- Aung, Y.L.; Ikesue, A. Transparent Tb3Fe5O12 ceramics as Mid-IR isolator. J. Alloys Compd. 2019, 773, 739–742. [Google Scholar] [CrossRef]
- Mironov, E.A.; Palashov, O.V.; Snetkov, I.L.; Balabanov, S.S. ZnSe-based Faraday isolator for high-power mid-IR lasers. Laser Phys. Lett. 2020, 17, 125801. [Google Scholar] [CrossRef]
- Snetkov, I.; Bulanov, D.; Yakovlev, A.; Palashov, O.; Khazanov, E. Thermally-induced depolarization in a silicon (c-Si) single crystal. Opt. Lett. 2022, 47, 473–476. [Google Scholar] [CrossRef] [PubMed]
- Starobor, A.V.; Palashov, O.V. Thermally-induced depolarization in the optical elements of the transition configuration. Laser Phys. Lett. 2014, 11, 125003. [Google Scholar] [CrossRef]

| μ* = V/α0, rad/T | μ = Vκ/α0Q, rad·W/(T·m) | Pcr, kW | Pcr,V, kW | Pmax, kW [17] | Experiments Plas, kW; I, dB | |
|---|---|---|---|---|---|---|
| MOG04 | 210 | 1.7·108 | 0.16 | 2.1 | 0.16 | 0.4; 7 [54] * 0.4; 25 [54] |
| TGG sc | 284 | 7.5·108 | 0.7 | 10 | 0.7 | 0.8; 27 [50] * 1.5; 33 [50] |
| TSAG sc | 154 | 320·108 | 29 | 3.4 | 1.7 | 1.47; 35 [55] – |
| NTF sc | 320 | 9.1·108 | 2.3 | 2.9 | 2.9 | 1.05; 31 [56] – |
| KTF sc | 2267 | 21·108 | 1.9 | 32 | 3.0 | 0.32; 42 [57] – |
| TAG cer | – | 4·108 | 0.6 | – | 0.6 | 0.4; 33 [58] – |
| Tb2O3 cer | 60 | 4.5·108 | 0.4 | 0.7 | 0.35 | 0.09; 30 [59] – |
| ZnSe @1μm cer | – | 3.2·108 | >2.5 | ∞ | >2.5 | 1.27; 30 [60] – |
| Si @1.9μm sc | 138 | 3.7·108 | 0.53 | ∞ | 14 | 0.01; 38 [34] * 0.01; 42 [34] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Snetkov, I.; Li, J. Selection of Magneto-Optical Material for a Faraday Isolator Operating in High-Power Laser Radiation. Magnetochemistry 2022, 8, 168. https://doi.org/10.3390/magnetochemistry8120168
Snetkov I, Li J. Selection of Magneto-Optical Material for a Faraday Isolator Operating in High-Power Laser Radiation. Magnetochemistry. 2022; 8(12):168. https://doi.org/10.3390/magnetochemistry8120168
Chicago/Turabian StyleSnetkov, Ilya, and Jiang Li. 2022. "Selection of Magneto-Optical Material for a Faraday Isolator Operating in High-Power Laser Radiation" Magnetochemistry 8, no. 12: 168. https://doi.org/10.3390/magnetochemistry8120168
APA StyleSnetkov, I., & Li, J. (2022). Selection of Magneto-Optical Material for a Faraday Isolator Operating in High-Power Laser Radiation. Magnetochemistry, 8(12), 168. https://doi.org/10.3390/magnetochemistry8120168







