Counterintuitive Single-Molecule Magnet Behaviour in Two Polymorphs of One-Dimensional Compounds Involving Chiral BINOL-Derived Bisphosphate Ligands
Abstract
:1. Introduction
2. Results and Discussion
2.1. Synthesis
2.2. X-ray Structures
2.3. Magnetic Properties
2.3.1. Static Magnetic Measurements
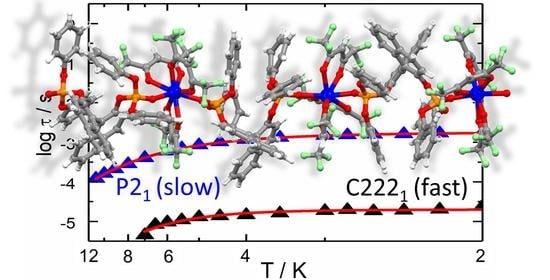
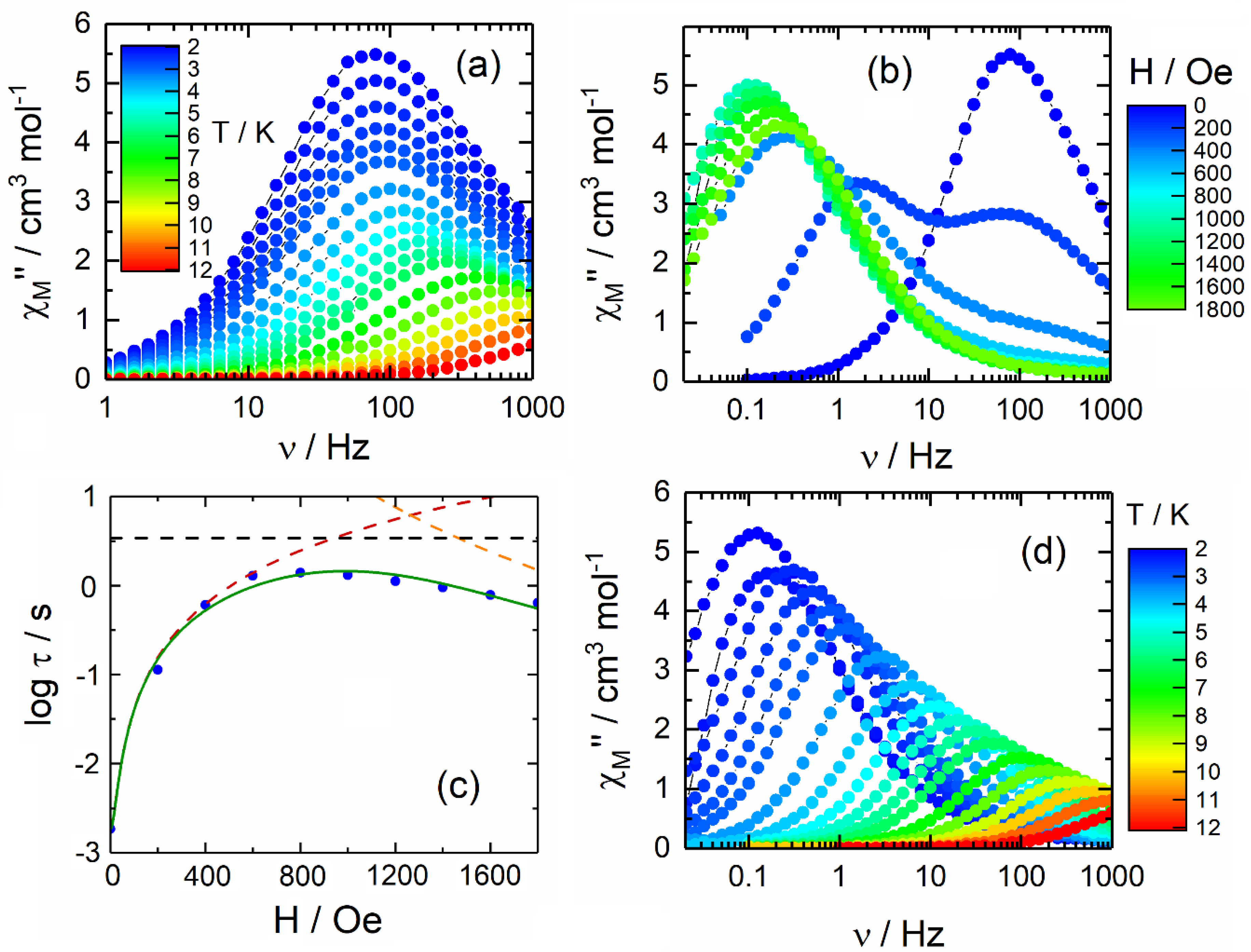
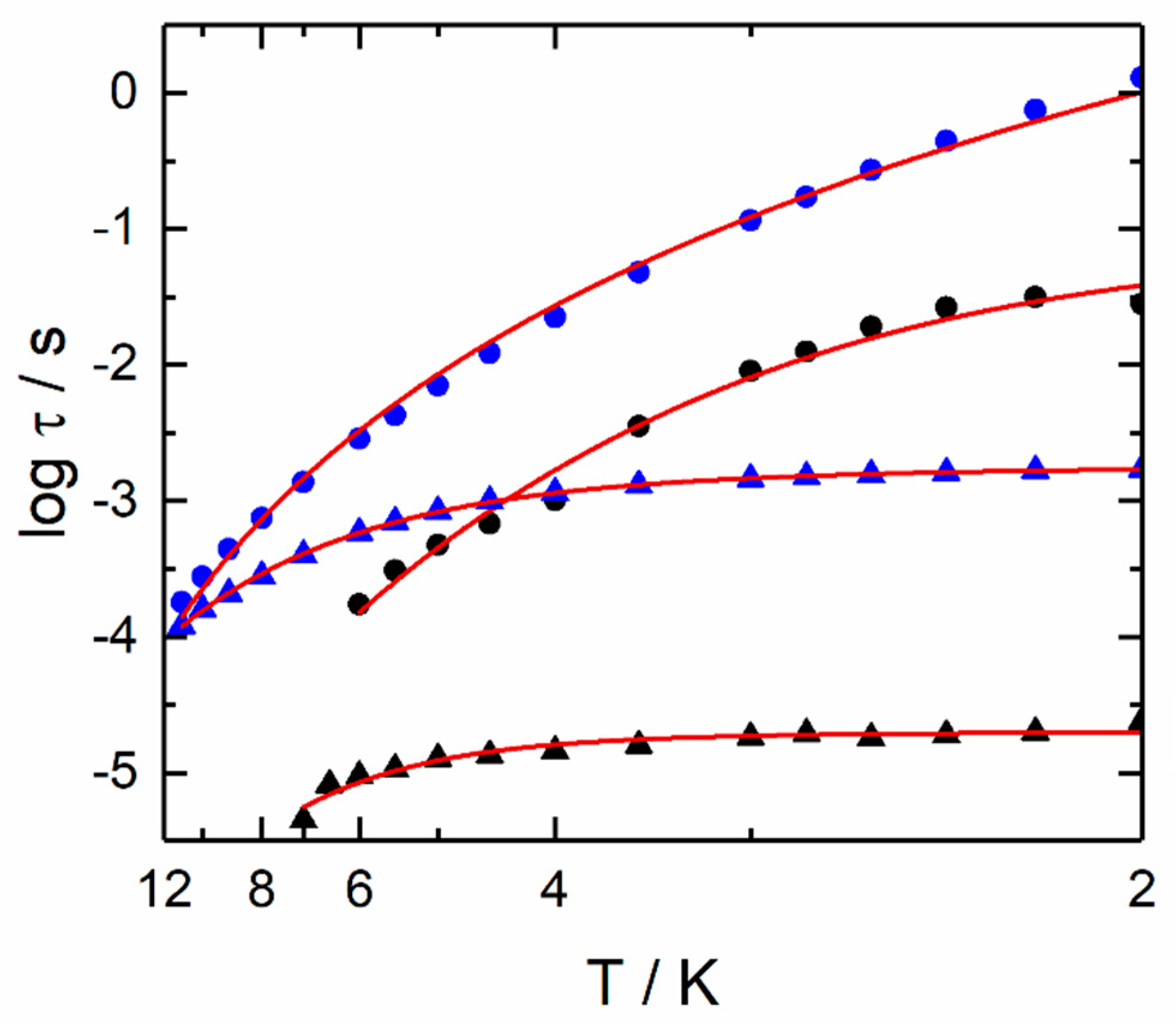
2.3.2. Dynamic Magnetic Measurements
3. Conclusions
4. Materials and Methods
4.1. Synthesis, General Procedures and Materials
4.2. Synthesis of the Ligand [8′-(Diphenoxylphosphinyl)[1,1′-binaphthalen]-8-yl]diphenoxylphosphine Oxide (L)
4.3. Synthesis of Complex [(Dy(hfac)3(L))3]n (1)
4.4. Crystallography
4.5. Physical Measurements
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| PXRD | Powder X-Ray Diffraction |
| SQUID | Superconducting Quantum Interference Device |
| SMM | Single Molecule Magnet |
| QTM | Quantum Tunneling of the Magnetisation |
| CH2Cl2 | Dichloromethane |
| hfac− | 1,1,1,5,5,5-hexafluoroacetylacetonate |
References
- Sessoli, R.; Tsai, H.L.; Schake, A.R.; Wang, S.Y.; Vincent, J.B.; Folting, K.; Gatteschi, D.; Christou, G.; Hendrickson, D.N. High-spin molecules: [Mn12O12(O2CR)16(H2O)4]. J. Am. Chem. Soc. 1993, 115, 1804–1816. [Google Scholar] [CrossRef]
- Sessoli, R.; Gatteschi, D.; Caneschi, A.; Novak, M.A. Magnetic bistability in a metal-ion cluster. Nature 1993, 365, 141–143. [Google Scholar] [CrossRef]
- Pedersen, K.S.; Ariciu, A.-M.; McAdams, S.; Weihe, H.; Bendix, J.; Tuna, F.; Piligkos, S. Toward Molecular 4f Single-Ion Magnet Qubits. J. Am. Chem. Soc. 2016, 138, 5801–5804. [Google Scholar] [CrossRef] [PubMed]
- Bogani, F.; Wernsdorfer, W. Molecular spintronics using single-molecule magnets. Nat. Mater. 2008, 7, 179–186. [Google Scholar] [CrossRef]
- Vincent, R.; Klyatskaya, S.; Ruben, M.; Wernsdorfer, W.; Balestro, F. Electronic read-out of a single nuclear spin using a molecular spin transistor. Nature 2012, 488, 357–360. [Google Scholar] [CrossRef]
- Ganzhorn, M.; Klyatskaya, S.; Ruben, M.; Wernsdorfer, W. Strong spin–phonon coupling between a single-molecule magnet and a carbon nanotube nanoelectromechanical system. Nat. Nanotechnol. 2013, 8, 165–169. [Google Scholar] [CrossRef]
- Cornia, A.; Seneor, P. The molecular way. Nat. Mater. 2017, 16, 505–506. [Google Scholar] [CrossRef]
- Thiele, S.; Balestro, F.; Ballou, R.; Klyatskaya, S.; Ruben, M.; Wernsdorfer, W. Electrically driven nuclear spin resonance in single-molecule magnets. Science 2014, 344, 1135–1138. [Google Scholar] [CrossRef]
- Leuenberger, M.N.; Loss, D. Quantum computing in molecular magnets. Nature 2001, 410, 789–793. [Google Scholar] [CrossRef] [Green Version]
- Ardavan, A.; Rival, O.; Morton, J.J.L.; Blundell, S.J.; Tyryshkin, A.M.; Timco, G.A.; Winpenny, R.E.P. Will Spin-Relaxation Times in Molecular Magnets Permit Quantum Information Processing? Phys. Rev. Lett. 2007, 98, 057201. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Stamp, P.C.E.; Gaita-Ariño, A. Spin-based quantum computers made by chemistry: Hows and whys. J. Mater. Chem. 2009, 19, 1718–1730. [Google Scholar] [CrossRef] [Green Version]
- Martínez-Pérez, M.J.; Cardona-Serra, S.; Schlegel, C.; Moro, F.; Alonso, P.J.; Prima-García, H.; Clemente-Juan, J.M.; Evangelisti, M.; Gaita-Arino, A.; Sesé, J.; et al. Gd-Based Single-Ion Magnets with Tunable Magnetic Anisotropy: Molecular Design of Spin Qubits. Phys. Rev. Lett. 2012, 108, 247213. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sessoli, R.; Boulon, M.-E.; Caneschi, A.; Mannini, M.; Poggini, L.; Wilhelm, F.; Rogalev, A. Strong magnetochiral dichroism in a paramagnetic molecular helix observed by hard X-rays. Nat. Phys. 2015, 11, 69–74. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ishikawa, N.; Sugita, M.; Ishikawa, T.; Koshihara, S.; Kaizu, Y. Lanthanide Double-Decker Complexes Functioning as Magnets at the Single-Molecular Level. J. Am. Chem. Soc. 2003, 125, 8694–8695. [Google Scholar] [CrossRef] [PubMed]
- Guo, F.-S.; Day, B.-M.; Chen, Y.-C.; Tong, M.-L.; Mansikkamäki, A.; Layfield, R.A. Magnetic hysteresis up to 80 kelvin in a dysprosium metallocene single-molecule magnet. Science 2018, 362, 1400–1403. [Google Scholar] [CrossRef] [Green Version]
- Mannini, M.; Pineider, F.; Sainctavit, P.; Danieli, C.; Otero, E.; Sciancalepore, C.; Talarico, A.M.; Arrio, M.-A.; Cornia, A.; Gatteschi, D.; et al. Magnetic memory of a single-molecule quantum magnet wired to a gold surface. Nat. Mater. 2009, 8, 194–197. [Google Scholar] [CrossRef]
- Affronte, M. Molecular nanomagnets for information technologies. J. Mater. Chem. 2009, 19, 1731–1737. [Google Scholar] [CrossRef]
- Coronado, E.; Forment-Aliaga, A.; Galán-Mascarós, J.R.; Giménez-Saiz, C.; Gómez-Garcia, C.J.; Martinéz-Ferrero, E.; Nuez, A.; Romero, F.M. Multifunctional molecular materials. Solid State Sci. 2003, 5, 917–924. [Google Scholar] [CrossRef]
- Coronado, E.; Giménez-Saiz, C.; Martí-Gastaldo, C. Crystal engineering of multifunctional molecular materials. In Engineering of Crystalline Materials Properties; Novoa, J.J., Braga, D., Addadi, L., Eds.; NATO Science for Peace and Security Series B: Physics and Biophysics; Springer: Dordrecht, The Netherlands, 2008; pp. 173–191. [Google Scholar]
- Coronado, E.; Palacio, F.; Veciana, J. Molecule–Based Magnetic Materials. Angew. Chem. Int. Ed. 2003, 42, 2570–2572. [Google Scholar] [CrossRef]
- Gómez-Romero, P.; Sánchez, C. Functional Hybrids Materials; Wiley-VCH: Weinheim, Germany, 2004. [Google Scholar]
- Fahmi, A.; Pietsch, T.; Mendoza, C.; Cheval, N. Functional hybrid materials. Mater. Today 2009, 12, 44–50. [Google Scholar] [CrossRef]
- Rocha, J.; Carlos, L.D.; Paz, F.A.A.; Ananias, D. Luminescent progress in hybrid materials science. Chem. Soc. Rev. 2011, 40, 926–940. [Google Scholar] [CrossRef]
- Sanchez, C.; Shea, K.J.; Kitagawa, S. Recent progress in hybrid materials science. Chem. Soc. Rev. 2011, 40, 471–472. [Google Scholar] [CrossRef]
- Ouahab, L. Multifunctional Molecular Materials; Taylor and Francis Group CRC Press: New York, NY, USA, 2012. [Google Scholar]
- Scott, J.F. Applications of Modern Ferroelectrics. Science 2007, 315, 954–959. [Google Scholar] [CrossRef]
- Tsymbal, E.Y.; Kohlstedt, H. Tunneling Across a Ferroelectric. Science 2006, 313, 181–183. [Google Scholar] [CrossRef]
- Cheong, S.-W.; Mostovoy, M. Multiferroics: A magnetic twist for ferroelectricity. Nat. Mater. 2007, 6, 13–20. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ramesh, R.; Spaldin, N.A. Multiferroics: Progress and prospects in thin films. Nat. Mater. 2007, 6, 21–29. [Google Scholar] [CrossRef] [PubMed]
- Rikken, G.L.J.A.; Raupach, E. Observation of magneto-chiral dichroism. Nature 1997, 390, 493–494. [Google Scholar] [CrossRef]
- Barron, L.D. Chirality, magnetism and light. Nature 2000, 405, 895–896. [Google Scholar] [CrossRef] [Green Version]
- Train, C.; Gheorghe, R.; Krstic, V.; Chamoreau, L.; Ovnesyan, N.S.; Rikken, G.L.J.A.; Gruselle, M.; Verdaguer, M. Strong magneto-chiral dichroism in enantiopure chiral ferromagnets. Nat. Mater. 2008, 7, 729–734. [Google Scholar] [CrossRef]
- Bordács, S.; Kézsmárki, I.; Szaller, D.; Demkó, L.; Kida, N.; Murakawa, H.; Onose, Y. Chirality of matter shows up via spin excitations. Nat. Phys. 2012, 8, 734–738. [Google Scholar] [CrossRef] [Green Version]
- El Rez, B.; Liu, J.; Béreau, V.; Duhayon, C.; Horino, Y.; Suzuki, T.; Coolen, L.; Sutter, J.-P. Concomitant emergence of circularly polarized luminescence and single-molecule magnet behavior in chiral-at-metal Dy complex. Inorg. Chem. Front. 2020, 7, 4527–4534. [Google Scholar] [CrossRef]
- Huizi-Rayo, U.; Zabala-Lekuona, A.; Terenzi, A.; Cruz, C.M.; Cuerva, J.M.; Rodriguez-Diéguez, A.; Garcia, J.A.; Seco, J.M.; San Sebastian, E.; Cepeda, J. Influence of thermally induced structural transformations on the magnetic and luminescence properties of tartrate-based chiral lanthanide organic-frameworks. J. Mater. Chem. C. 2020, 8, 8243–8256. [Google Scholar] [CrossRef]
- Gendron, F.; Di Pietro, S.; Abad Galan, L.; Riobé, F.; Placide, V.; Guy, L.; Zinna, F.; Di Bari, L.; Bensalah-Ledoux, A.; Guyot, Y.; et al. Luminescence, chiroptical, magnetic and ab initio crystal-field characterizations of an enantiopure helicoidal Yb(III) complex. Inorg. Chem. Front. 2021, 8, 914–926. [Google Scholar] [CrossRef]
- Lefeuvre, B.; Mattei, C.A.; Flores Gonzalez, J.; Gendron, F.; Dorcet, V.; Riobé, F.; Lalli, C.; Le Guennic, B.; Cador, O.; Maury, O.; et al. Solid-State near-Infrared Circularly Polarized Luminescence from Chiral YbIII-Single-Molecule Magnet. Chem. Eur. J. 2021, 27, 7362–7366. [Google Scholar] [CrossRef] [PubMed]
- Li, D.-P.; Wang, T.-W.; Li, C.-H.; Liu, D.-S.; Li, Y.-Z.; You, X.-Z. Single-ion magnets based on mononuclear lanthanide complexes with chiral Schiff base ligands [Ln(FTA)3L] (Ln = Sm, Eu, Gd, Tb and Dy). Chem. Commun. 2010, 46, 2929–2931. [Google Scholar] [CrossRef] [PubMed]
- Li, X.-L.; Chen, C.-L.; Gao, Y.-L.; Liu, C.-M.; Feng, X.-L.; Gui, Y.-H.; Fang, S.-M. Modulation of Homochiral DyIII Complexes: Single–Molecule Magnets with Ferroelectric Properties. Chem.–A Eur. J. 2012, 18, 14632–14637. [Google Scholar] [CrossRef] [PubMed]
- Li, X.-L.; Chen, C.-L.; Xiao, H.-P.; Wang, A.-L.; Liu, C.-M.; Zheng, X.; Gao, L.-J.; Yang, X.-G.; Fang, S.-M. Luminescent, magnetic and ferroelectric properties of noncentrosymmetric chain-like complexes composed of nine-coordinate lanthanide ions. Dalton Trans. 2013, 42, 15325–15347. [Google Scholar] [CrossRef] [PubMed]
- Long, J.; Rouquette, J.; Thibaud, J.-M.; Ferreira, R.A.S.; Carlos, L.D.; Donnadieu, B.; Vieru, V.; Chibotaru, L.F.; Konczewicz, L.; Haines, J.; et al. A High–Temperature Molecular Ferroelectric Zn/Dy Complex Exhibiting Single–Ion–Magnet Behavior and Lanthanide Luminescence. Angew. Chem. Int. Ed. 2015, 54, 2236–2240. [Google Scholar] [CrossRef]
- Li, X.-L.; Hu, M.; Yin, Z.; Zhu, C.; Liu, C.-M.; Xiao, H.-P.; Fang, S. Enhanced single-ion magnetic and ferroelectric properties of mononuclear Dy(III) enantiomeric pairs through the coordination role of chiral ligands. Chem. Commun. 2017, 53, 3998–4001. [Google Scholar] [CrossRef]
- Long, J.; Ivanov, M.S.; Khomchenko, V.A.; Mamontova, E.; Thibaud, J.-M.; Rouquette, J.; Beaudhuin, M.; Granier, D.; Ferreira, R.A.S.; Carlos, L.D.; et al. Room temperature magnetoelectric coupling in a molecular ferroelectric ytterbium(III) complex. Science 2020, 367, 671–676. [Google Scholar] [CrossRef]
- Wang, K.; Zeng, S.; Wang, H.; Dou, J.; Jiang, J. Magnetochiral dichroism in chiral mixed (phthalocyaninato)(porphyrinato) rare earth triple-decker SMMs. Inorg. Chem. Front. 2014, 1, 167–171. [Google Scholar] [CrossRef]
- Atzori, M.; Dhbaibi, K.; Douib, H.; Grasser, M.; Dorcet, V.; Breslavetz, I.; Paillot, K.; Cador, O.; Rikken, G.L.J.A.; Le Guennic, B.; et al. Helicene-Based Ligands Enable Strong Magneto-Chiral Dichroism in a Chiral Ytterbium Complex. J. Am. Chem. Soc. 2021, 143, 2671–2675. [Google Scholar] [CrossRef]
- Ou-Yang, J.-K.; Saleh, N.; Fernandez Garcia, G.; Norel, L.; Pointillart, F.; Guizouarn, T.; Cador, O.; Totti, F.; Ouahab, L.; Crassous, J.; et al. Improved slow magnetic relaxation in optically pure helicene-based DyIII single molecule magnets. Chem. Commun. 2016, 52, 14474–14477. [Google Scholar] [CrossRef] [PubMed]
- Pointillart, F.; Bernot, K.; Poneti, G.; Sessoli, R. Crystal Packing Effects on the Magnetic Slow Relaxation of Tb(III)-Nitronyl Nitroxide Radical Cyclic Dinuclear Clusters. Inorg. Chem. 2012, 51, 12218–12229. [Google Scholar] [CrossRef]
- Kishi, Y.; Pointillart, F.; Lefeuvre, B.; Riobé, F.; Le Guennic, B.; Golhen, S.; Cador, O.; Maury, O.; Fujiwara, H.; Ouahab, L. Isotopically enriched polymorphs of dysprosium single molecule magnets. Chem. Commun. 2017, 53, 3575–3578. [Google Scholar] [CrossRef]
- Mattei, C.A.; Montigaud, V.; Gendron, F.; Denis-Quanquin, S.; Dorcet, V.; Giraud, N.; Riobé, F.; Argouarch, G.; Maury, O.; Le Guennic, B.; et al. Solid-state versus solution investigation of a luminescent chiral BINOL-derived bisphosphate single-molecule magnet. Inorg. Chem. Front. 2021, 8, 947–962. [Google Scholar] [CrossRef]
- Mattei, C.A.; Montigaud, V.; Dorcet, V.; Riobé, F.; Argouarch, G.; Maury, O.; Le Guennic, B.; Cador, O.; Lalli, C.; Pointillart, F. Luminescent dysprosium single-molecule magnets made from designed chiral BINOL-derived bisphosphate ligands. Inorg. Chem. Front. 2021, 8, 963–976. [Google Scholar] [CrossRef]
- Llunell, M.; Casanova, D.; Cirera, J.; Alemany, P.; Alvarez, S. SHAPE Program for the Stereochemical Analysis of Molecular Fragments by Means of Continuous Shape Measures and Associated Tools; Departament de Quimica Fisica, Departament de Quimica Inorganica, and Institut de Quimica Teorica i Computacional–Universitat dè Barcelona: Barcelona, Spain, 2013. [Google Scholar]
- Kahn, O. Molecular Magnetism; VCH: Weinhem, Germany, 1993. [Google Scholar]
- Abragam, A.; Bleaney, B. Electron Paramagnetic Resonance of Transition Ions; Clarendon Press: Oxford, UK, 1970. [Google Scholar]
- Singh, A.; Shrivastava, K.N. Optical-acoustic two-phonon relaxation in spin systems. Phys. Status Solidi B 1979, 95, 273–277. [Google Scholar] [CrossRef]
- Shirivastava, K.N. Theory of Spin-Lattice Relaxation. Phys. Status Solidi B 1983, 177, 437. [Google Scholar] [CrossRef]
- Evans, P.; Reta, D.; Whitehead, G.F.S.; Chilton, N.F.; Mills, D.P. Bis-Monophospholyl Dysprosium Cation Showing Magnetic Hysteresis at 48 K. J. Am. Chem. Soc. 2019, 141, 19935–19940. [Google Scholar] [CrossRef] [Green Version]
- Pointillart, F.; Bernot, K.; Golhen, S.; Le Guennic, B.; Guizouarn, T.; Ouahab, L.; Cador, O. Magnetic memory in an Isotopically Enriched and Magnetically Isolated Mononuclear Dysprosium Complex. Angew. Chem. Int. Ed. 2015, 54, 1504–1507. [Google Scholar] [CrossRef]
- Pointillart, F.; Flores Gonzalez, J.; Montigaud, V.; Tesi, L.; Cherkasov, V.; Le Guennic, B.; Cador, O.; Ouahab, L.; Sessoli, R.; Kuropatov, V. Redox- and solvato-magnetic switching in a tetrathiafulvalene-based triad single-molecule magnet. Inorg. Chem. Front. 2020, 7, 2322–2334. [Google Scholar] [CrossRef]
- Car, P.-E.; Perfetti, M.; Mannini, M.; Favre, A.; Caneschi, A.; Sessoli, R. Giant field dependence of the low temperature relaxation of the magnetization in a dysprosium(iii)–DOTA complex. Chem. Commun. 2011, 47, 3751–3753. [Google Scholar] [CrossRef] [PubMed]
- Fu, D.-W.; Song, Y.-M.; Wang, G.-X.; Ye, Q.; Xiong, R.-G.; Akutagawa, T.; Nakamura, T.; Chan, P.W.H.; Huang, S.D. Dielectric Anisotropy of a Homochiral Trinuclear Nickel(II) Complex. J. Am. Chem. Soc. 2007, 129, 5346–5347. [Google Scholar] [CrossRef]
- Li, X.-L.; Chen, K.; Liu, Y.; Wang, Z.-X.; Wang, T.-W.; Zuo, J.-L.; Li, -Z.; Wang, Y.; Zhu, J.-S.; Liu, J.-M.; et al. Molecule-Based Ferroelectric Thin Films: Mononuclear Lanthanide Enantiomers Displaying Room-Temperature Ferroelectric and Dielectric Properties. Angew. Chem. Int. Ed. 2007, 46, 6820–6823. [Google Scholar] [CrossRef]
- Horiuchi, S.; Tokunaga, Y.; Giovannetti, G.; Piccozzi, S.; Itoh, H.; Shimano, R.; Kumai, R.; Tokura, Y. Above-room-temperature ferroelectricity in a single-component molecular crystal. Nature 2010, 463, 789–792. [Google Scholar] [CrossRef] [PubMed]
- Richardson, M.F.; Wagner, W.F.; Sands, D.E. Rare earth trishexafluoroacetylacetonates and related compounds. J. Inorg. Nucl. Chem. 1968, 30, 1275–1289. [Google Scholar] [CrossRef]
- Ngo Ndimba, A.; Roisnel, T.; Argouarch, G.; Lalli, C. Harvesting New Chiral Phosphotriesters by Phosphorylation of BINOL and Parent Bis-phenols. Synthesis 2019, 51, 865–873. [Google Scholar]
- Sheldrick, G.M. SHELXT—Integrated space-group and crystal-structure determination. Acta Crystallogr. Sect. A Found. Adv. 2015, 71, 3–8. [Google Scholar] [CrossRef] [Green Version]
- Sheldrick, G.M. Crystal structure refinement with SHELXL. Acta Crystallogr. Sect. C Struct. Chem. 2015, 71, 3–8. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mattei, C.A.; Lefeuvre, B.; Dorcet, V.; Argouarch, G.; Cador, O.; Lalli, C.; Pointillart, F. Counterintuitive Single-Molecule Magnet Behaviour in Two Polymorphs of One-Dimensional Compounds Involving Chiral BINOL-Derived Bisphosphate Ligands. Magnetochemistry 2021, 7, 150. https://doi.org/10.3390/magnetochemistry7110150
Mattei CA, Lefeuvre B, Dorcet V, Argouarch G, Cador O, Lalli C, Pointillart F. Counterintuitive Single-Molecule Magnet Behaviour in Two Polymorphs of One-Dimensional Compounds Involving Chiral BINOL-Derived Bisphosphate Ligands. Magnetochemistry. 2021; 7(11):150. https://doi.org/10.3390/magnetochemistry7110150
Chicago/Turabian StyleMattei, Carlo Andrea, Bertrand Lefeuvre, Vincent Dorcet, Gilles Argouarch, Olivier Cador, Claudia Lalli, and Fabrice Pointillart. 2021. "Counterintuitive Single-Molecule Magnet Behaviour in Two Polymorphs of One-Dimensional Compounds Involving Chiral BINOL-Derived Bisphosphate Ligands" Magnetochemistry 7, no. 11: 150. https://doi.org/10.3390/magnetochemistry7110150
APA StyleMattei, C. A., Lefeuvre, B., Dorcet, V., Argouarch, G., Cador, O., Lalli, C., & Pointillart, F. (2021). Counterintuitive Single-Molecule Magnet Behaviour in Two Polymorphs of One-Dimensional Compounds Involving Chiral BINOL-Derived Bisphosphate Ligands. Magnetochemistry, 7(11), 150. https://doi.org/10.3390/magnetochemistry7110150