The Asymmetrical Distribution of a Dominant Motional Electric Field within the Martian Magnetosheath
Abstract
1. Introduction
2. Model Description
3. Simulation Results
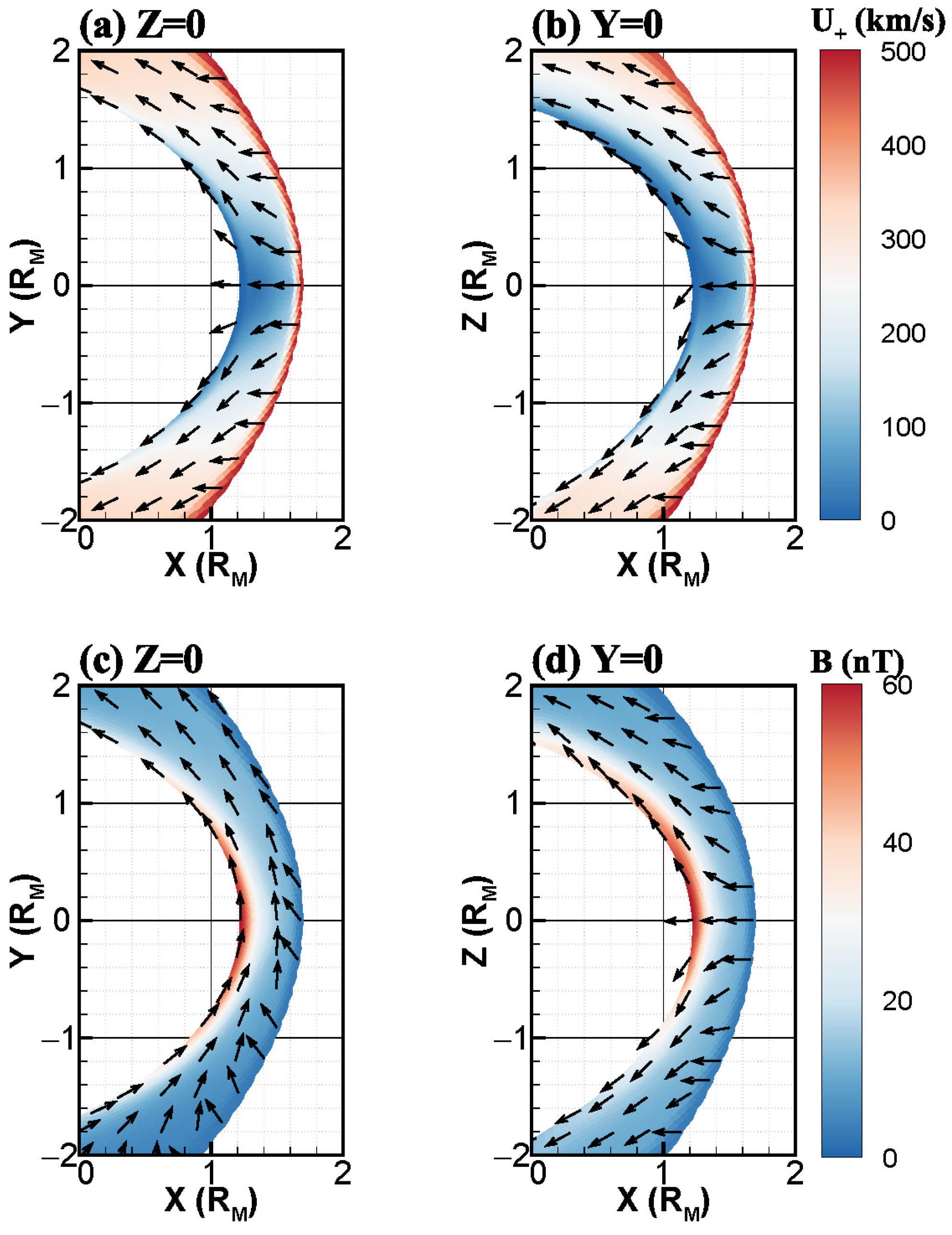
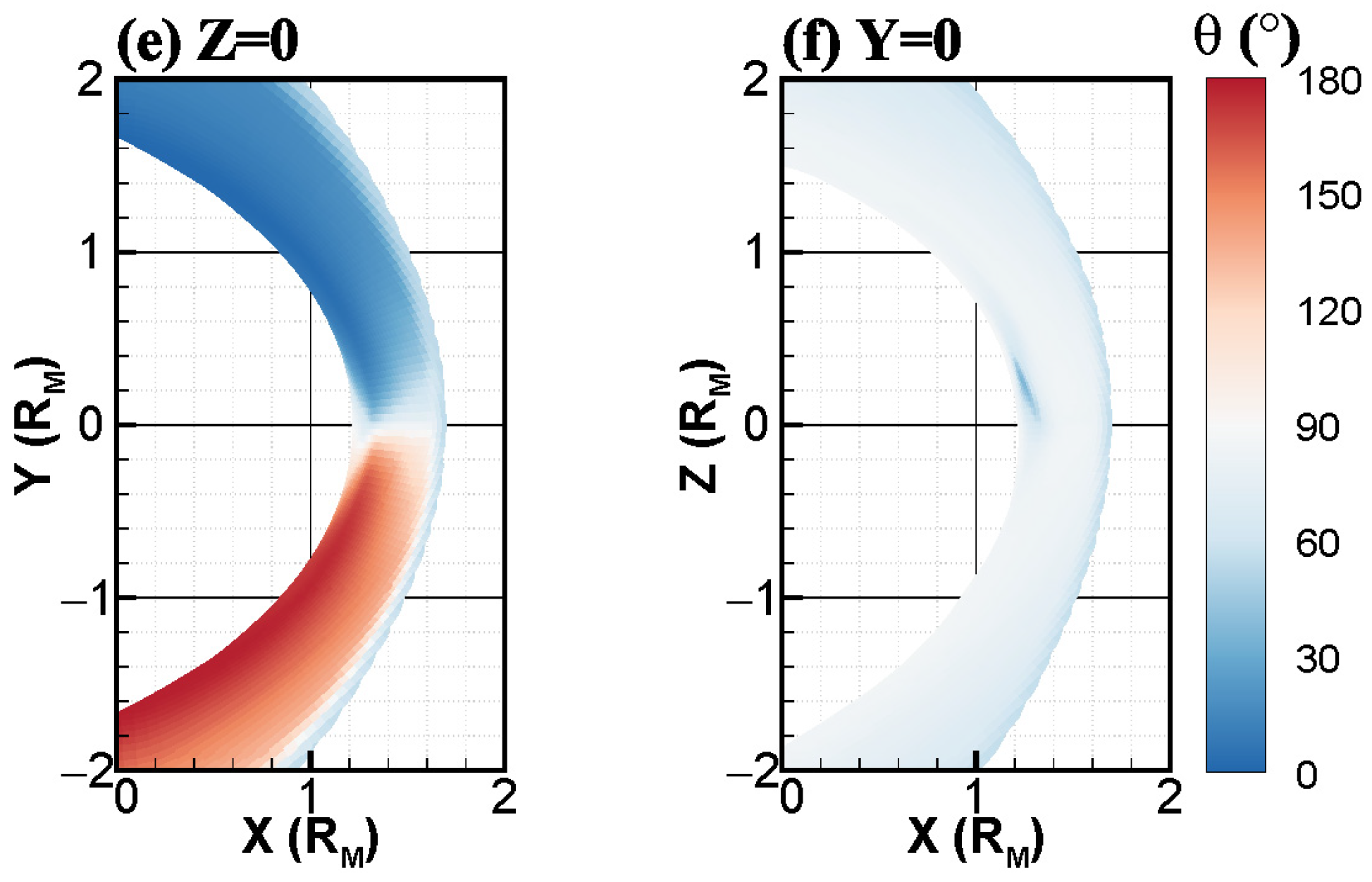
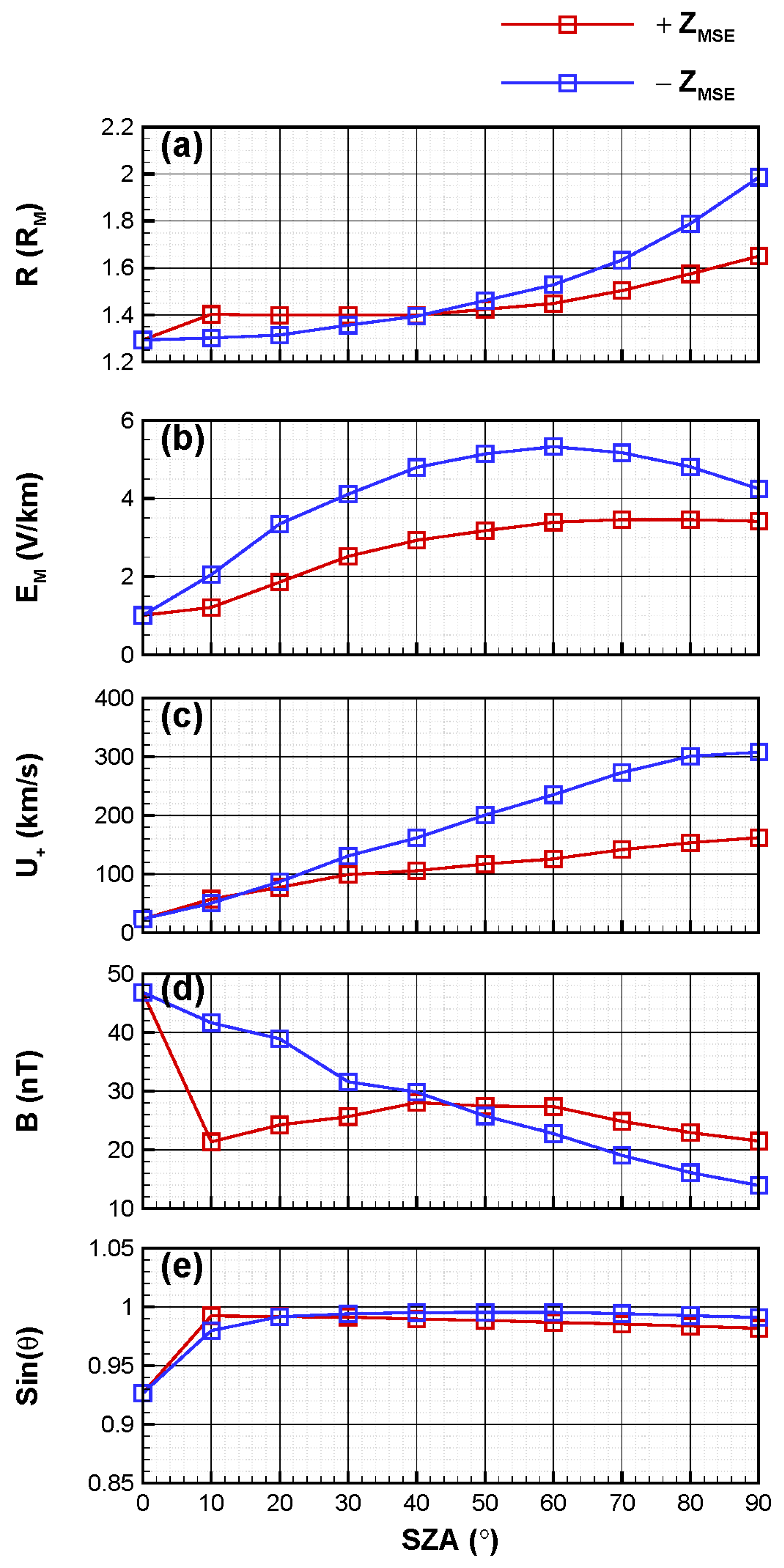
3.1. The Inherent Asymmetry Distribution of
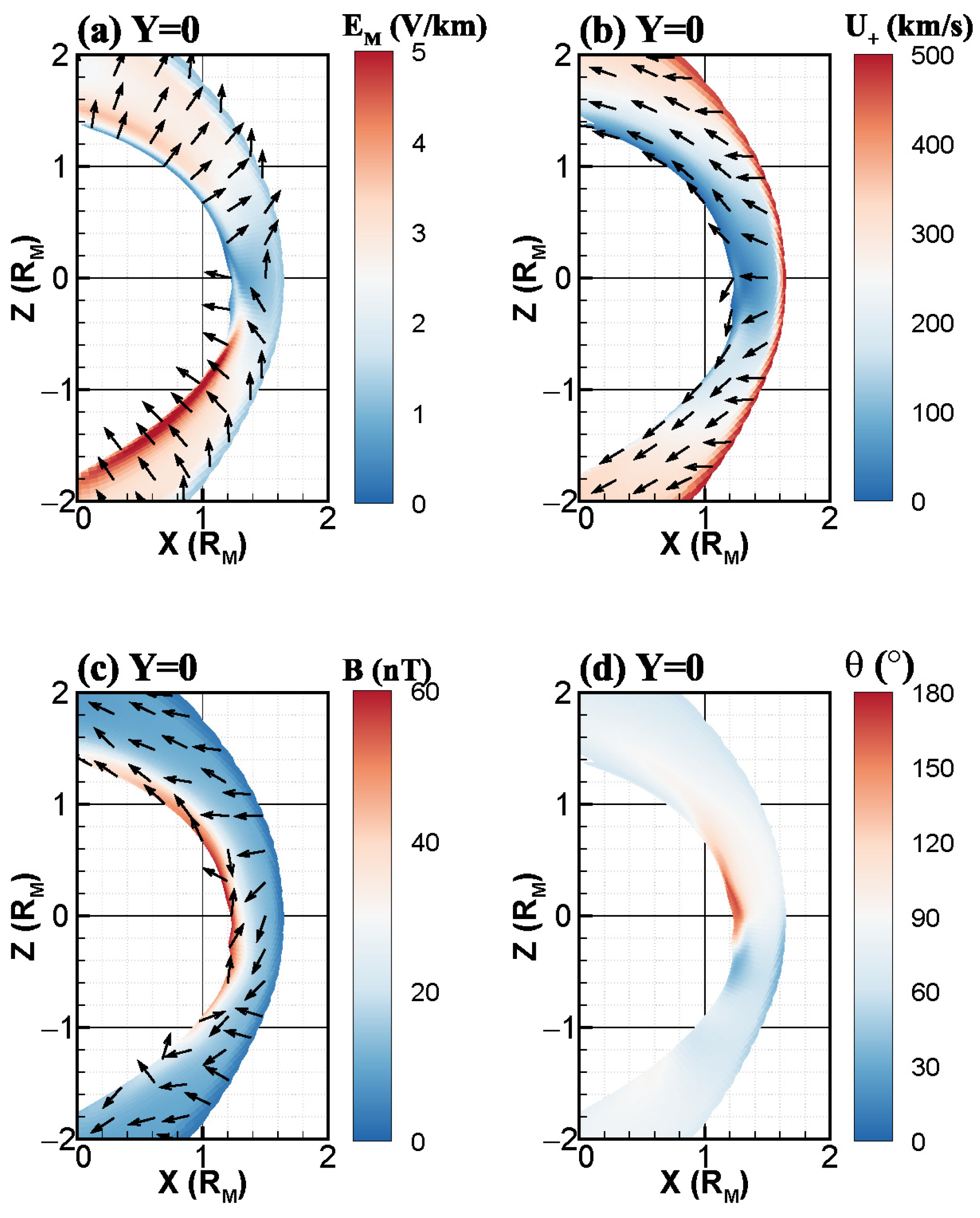
3.2. The Impact of the Crustal Field on the Asymmetry Distribution of
4. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Parker, E.N. Dynamics of the Interplanetary Gas and Magnetic Fields. Astrophys. J. 1958, 128, 664–676. [Google Scholar] [CrossRef]
- McComas, D.J.; Elliott, H.A.; Schwadron, N.A.; Gosling, J.T.; Skoug, R.M.; Goldstein, B.E. The three-dimensional solar wind around solar maximum. Geophys. Res. Lett. 2003, 30, 1517. [Google Scholar] [CrossRef]
- Scudder, J.D.; Mangeney, A.; Lacombe, C.; Harvey, C.C.; Aggson, T.L. The resolved layer of a collisionless, high β, supercritical, quasi-perpendicular shock wave, 2. Dissipative fluid electrodynamics. J. Geophys. Res. 1986, 91, 11053–11074. [Google Scholar] [CrossRef]
- Spreiter, J.R.; Summers, A.L.; Alksne, A.Y. Hydromagnetic flow around the magnetosphere. Planet. Space Sci. 1966, 14, 223–253. [Google Scholar] [CrossRef]
- Mazelle, C.; Winterhalter, D.; Sauer, K.; Trotignon, J.G.; Acuña, M.H.; Baumgärtel, K.; Bertucci, C.; Brain, D.; Brecht, S.; Delva, M.; et al. Bow shock and upstream phenomena at Mars. Space Sci. Rev. 2004, 111, 115–181. [Google Scholar] [CrossRef]
- Nagy, A.F.; Winterhalter, D.; Sauer, K.; Cravens, T.E.; Brecht, S.; Mazelle, C.; Crider, D.; Kallio, E.; Zakharov, A.; Dubinin, E.; et al. The plasma environment of Mars. Space Sci. Rev. 2004, 111, 33–114. [Google Scholar] [CrossRef]
- Moses, S.L.; Coroniti, F.V.; Scarf, F.L. Expectations for the microphysics of the Mars-solar wind interaction. Geophys. Res. Lett. 1988, 15, 429–432. [Google Scholar] [CrossRef]
- Sanchez-Cano, B.; Narvaez, C.; Lester, M.; Mendillo, M.; Mayyasi, M.; Holmstrom, M.; Halekas, J.; Andersson, L.; Fowler, C.M.; McFadden, J.P.; et al. Mars’ ionopause: A matter of pressures. J. Geophys. Res. Space Phys. 2020, 125, e2020JA028145. [Google Scholar] [CrossRef]
- Halekas, J.S.; Ruhunusiri, S.; McFadden, J.P.; Espley, J.R.; DiBraccio, G.A. Ion composition boundary layer instabilities at mars. Geophys. Res. Lett. 2019, 46, 10303–10312. [Google Scholar] [CrossRef]
- Dong, Y.; Fang, X.; Brain, D.A.; McFadden, J.P.; Halekas, J.S.; Connerney, J.E.P.; Eparvier, F.; Andersson, L.; Mitchell, D.; Jakosky, B.M. Seasonal variability of Martian ion escape through the plume and tail from MAVEN observations. J. Geophys. Res. Space Phys. 2017, 122, 4009–4022. [Google Scholar] [CrossRef]
- Shuvalov, S.; Andersson, L.; Halekas, J.S.; Fowler, C.M.; Hanley, K.G.; DiBraccio, G. Ionospheric plasma transported into the Martian magnetosheath. Geophys. Res. Lett. 2024, 51, e2023GL107953. [Google Scholar] [CrossRef]
- Qiao, F.; Li, L.; Xie, L.; Li, W.; Kong, L.; Tang, B.; Zhang, Y.; Zhang, A.; Xu, Q.; Wang, L.; et al. Acceleration of pick-up ions in the Martian magnetosheath: A Tianwen-1 case study. J. Geophys. Res. Space Phys. 2024, 129, e2024JA032461. [Google Scholar] [CrossRef]
- Wilson, L.B. Low Frequency Waves at and Upstream of Collisionless Shocks; American Geophysical Union Geophysical Monograph Series: Washington, DC, USA, 2016; Volume 216, pp. 269–291. [Google Scholar]
- Dong, Y.; Fang, X.; Brain, D.A.; McFadden, J.P.; Halekas, J.S.; Connerney, J.E.; Curry, S.M.; Harada, Y.; Luhmann, J.G.; Jakosky, B.M. Strong plume fluxes at Mars observed by MAVEN: An important planetary ion escape channel. Geophys. Res. Lett. 2015, 42, 8942–8950. [Google Scholar] [CrossRef]
- Najib, D.; Nagy, A.F.; Tóth, G.; Ma, Y. Three-dimensional, multifluid, high spatial resolution MHD model studies of the solar wind interaction with Mars. J. Geophys. Res. 2011, 116, A05204. [Google Scholar] [CrossRef]
- Li, S.B.; Lu, H.Y.; Cui, J.; Yu, Y.Q.; Mazelle, C.; Li, Y.; Cao, J.B. Effects of a dipole-like crustal field on solar wind interaction with Mars. Earth Planet. Phys. 2020, 4, 23–31. [Google Scholar] [CrossRef]
- Russell, C.T.; Mulligan, T.; Delva, M.; Zhang, T.-L.; Schwingenschuh, K. A simple test of the induced nature of the martian tail. Planet. Space Sci. 1995, 43, 875–879. [Google Scholar] [CrossRef]
- Dubinin, E.; Sauer, K.; Lundin, R.; Norberg, O.; Trotignon, J.-G.; Schwingenschuh, K.; Delva, M.; Riedler, W. Plasma characteristics of the boundary layer in the martian magnetosphere. J. Geophys. Res. 1996, 101, 27061–27075. [Google Scholar] [CrossRef]
- Dubinin, E.; Fraenz, M.; Modolo, R.; Pätzold, M.; Tellmann, S.; Vaisberg, O.; Shuvalov, S.; Zelenyi, L.; Chai, L.; Wei, Y.; et al. Induced magnetic fields and plasma motions in the inner part of the martian magnetosphere. J. Geophys. Res. Space Phys. 2021, 126, e2021JA029542. [Google Scholar] [CrossRef]
- Dubinin, E.; Fraenz, M.; Pätzold, M.; Tellmann, S.; Modolo, R.; DiBraccio, G.; McFadden, J.; Espley, J. Magnetic fields and plasma motions in a hybrid martian magnetosphere. J. Geophys. Res. Space Phys. 2023, 128, e2022JA030575. [Google Scholar] [CrossRef]
- Connerney, J.E.P.; Espley, J.R.; DiBraccio, G.A.; Gruesbeck, J.R.; Oliversen, R.J.; Mitchell, D.L.; Halekas, J.; Mazelle, C.; Brain, D.; Jakosky, B.M. First results of the MAVEN magnetic field investigation. Geophys. Res. Lett. 2015, 42, 8819–8827. [Google Scholar] [CrossRef]
- Bößwetter, A.; Bagdonat, T.; Motschmann, U.; Sauer, K. Plasma boundaries at Mars: A 3-D simulation study. Ann. Geophys. 2004, 22, 4363–4379. [Google Scholar] [CrossRef]
- Li, S.; Lu, H.; Cao, J.; Cui, J.; Ge, Y.; Zhang, X.; Rong, Z.; Li, G.; Li, Y.; Gao, J.; et al. Global electric fields at Mars inferred from Multifluid hall-MHD simulations. Astrophys. J. 2023, 949, 88. [Google Scholar] [CrossRef]
- Dubinin, E.; Fraenz, M.; Pätzold, M.; Halekas, J.S.; Mcfadden, J.; Connerney, J.E.P.; Jakosky, B.M.; Vaisberg, O.; Zelenyi, L. Solar wind deflection by mass loading in the Martian magnetosheath based on MAVEN observations. Geophys. Res. Lett. 2018, 45, 2574–2579. [Google Scholar] [CrossRef]
- Romanelli, N.; DiBraccio, G.; Halekas, J.; Dubinin, E.; Gruesbeck, J.; Espley, J.; Poh, G.; Ma, Y.; Luhmann, J.G. Variability of the solar wind flow asymmetry in the Martian magnetosheath observed by MAVEN. Geophys. Res. Lett. 2020, 47, e2020GL090793. [Google Scholar] [CrossRef]
- Dubinin, E.; Fraenz, M.; Pätzold, M.; Tellmann, S.; McFadden, J.; Halekas, J.; DiBraccio, G. Solar wind—Ionosphere interface at Mars. Ion dynamics, asymmetry, plasma jets. Geophys. Res. Lett. 2024, 51, e2023GL105073. [Google Scholar] [CrossRef]
- Zhang, C.; Rong, Z.; Klinger, L.; Nilsson, H.; Shi, Z.; He, F.; Gao, J.; Li, X.; Futaana, Y.; Ramstad, R.; et al. Three-dimensional configuration of induced magnetic fields around Mars. J. Geophys. Res. Planets 2022, 127, e2022JE007334. [Google Scholar] [CrossRef]
- Ma, Y.J.; Dong, C.F.; Toth, G.; van der Holst, B.; Nagy, A.F.; Russell, C.T.; Bougher, S.; Fang, X.; Halekas, J.S.; Espley, J.R.; et al. Importance of ambipolar electric field in driving ion loss from Mars: Results from a multifluid MHD model with the electron pressure equation included. J. Geophys. Res. Space Phys. 2019, 124, 9040–9057. [Google Scholar] [CrossRef]
- Crider, D.H.; Acuña, M.H.; Connerney, J.E.P.; Vignes, D.; Ness, N.F.; Krymskii, A.M.; Breus, T.K.; Rème, H.; Mazelle, C.; Mitchell, D.L.; et al. Observations of the latitude dependence of the location of the Martian magnetic pileup boundary. Geophys. Res. Lett. 2002, 29, 11-1–11-4. [Google Scholar] [CrossRef]
- Ma, Y.J.; Nagy, A.F.; Sokolov, I.V.; Hansen, K.C. Three-dimensional, multispecies, high spatial resolution MHD studies of the solar wind interaction with Mars. J. Geophys. Res. 2004, 109, A07211. [Google Scholar] [CrossRef]
- Harnett, E.M.; Winglee, R.M. The influence of a mini-magnetopause on the magnetic pileup boundary at Mars. Geophys. Res. Lett. 2003, 30, 2074. [Google Scholar] [CrossRef]
- Fan, K.; Fraenz, M.; Wei, Y.; Cui, J.; Rong, Z.; Chai, L.; Dubinin, E. Deflection of global ion flow by the Martian crustal magnetic fields. Astrophys. J. Lett. 2020, 898, L54. [Google Scholar] [CrossRef]
- Li, S.; Lu, H.; Cao, J.; Cui, J.; Zhou, C.; Wild, J.A.; Li, G.; Li, Y. Deflection of ion flow by magnetic fields in the Martian ionosphere. Astrophys. J. 2022, 941, 198. [Google Scholar] [CrossRef]
- Li, S.; Lu, H.; Cao, J.; Mazelle, C.; Cui, J.; Rong, Z.; Wild, J.A.; Yu, Y.; Li, X.; Li, Y.; et al. The impact and mechanism of the magnetic inclination angle on escape from Mars. Astrophys. J. 2022, 931, 30. [Google Scholar] [CrossRef]
- Song, Y.; Lu, H.; Cao, J.; Li, S.; Yu, Y.; Wang, S.; Ge, Y.; Zhang, X.; Zhou, C.; Wang, J. Effects of force in the martian plasma environment with solar wind dynamic pressure enhancement. J. Geophys. Res. Space Phys. 2023, 128, e2022JA031083. [Google Scholar] [CrossRef]
- Song, Y.; Lu, H.; Cao, J.; Wu, X.; Liu, Y.; Li, S.; Wang, S.; Wild, J.A.; Zhou, C.; Wang, J.; et al. Effects of solar wind density and velocity variations on the Martian ionosphere and plasma transport—A MHD model study. J. Geophys. Res. Space Phys. 2023, 128, e2023JA031788. [Google Scholar] [CrossRef]
- Li, S.; Lu, H.; Cao, J.; Cui, J.; Ip, W.-H.; Wild, J.A.; Zhang, X.; Chen, N.; Song, Y.; Wang, J. Asymmetrical looping magnetic fields and marsward flows on the nightside of Mars. Geophys. Res. Lett. 2024, 51, e2024GL109186. [Google Scholar] [CrossRef]
- Bougher, S.W.; Engel, S.; Hinson, D.P.; Forbes, J.M. Mars global surveyor radio science electron density profiles: Neutral atmosphere implications. Geophys. Res. Lett. 2001, 28, 3091–3094. [Google Scholar] [CrossRef]
- Huestis, D.L. Accurate evaluation of the chapman function for atmospheric attenuation. J. Quant. Spectrosc. Radiat. Transf. 2001, 69, 709–721. [Google Scholar] [CrossRef]
- Schunk, R.; Nagy, A. Ionospheres: Physics, Plasma Physics, and Chemistry; Cambridge University Press: Cambridge, UK, 2009. [Google Scholar]
- Liu, D.; Rong, Z.; Gao, J.; He, J.; Klinger, L.; Dunlop, M.W.; Yan, L.; Fan, K.; Wei, Y. Statistical properties of solar wind upstream of Mars: MAVEN observations. Astrophys. J. 2021, 911, 113. [Google Scholar] [CrossRef]
- Gao, J.W.; Rong, Z.J.; Klinger, L.; Li, X.Z.; Liu, D.; Wei, Y. A spherical harmonic Martian crustal magnetic field model combining data sets of MAVEN and MGS. Earth Space Sci. 2021, 8, e2021EA001860. [Google Scholar] [CrossRef]
- Halekas, J.S.; Brain, D.A.; Luhmann, J.G.; DiBraccio, G.A.; Ruhunusiri, S.; Harada, Y.; Fowler, C.M.; Mitchell, D.L.; Connerney, J.E.; Espley, J.R.; et al. Flows, fields, and forces in the Mars-solar wind interaction. J. Geophys. Res. Space Phys. 2017, 122, 11320–11341. [Google Scholar] [CrossRef]



| Reaction | Rate Coefficient |
|---|---|
| 1 | |
| 1 | |
| 1 | |
| Crustal Field | ||||
|---|---|---|---|---|
| Case 1 | 4 | No | ||
| Case 2 | 4 | Yes |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, S.; Lu, H.; Cao, J.; Wu, X.; Zhang, X.; Chen, N.; Song, Y.; Wang, J.; Cao, Y.; Zhao, J. The Asymmetrical Distribution of a Dominant Motional Electric Field within the Martian Magnetosheath. Magnetochemistry 2024, 10, 62. https://doi.org/10.3390/magnetochemistry10080062
Li S, Lu H, Cao J, Wu X, Zhang X, Chen N, Song Y, Wang J, Cao Y, Zhao J. The Asymmetrical Distribution of a Dominant Motional Electric Field within the Martian Magnetosheath. Magnetochemistry. 2024; 10(8):62. https://doi.org/10.3390/magnetochemistry10080062
Chicago/Turabian StyleLi, Shibang, Haoyu Lu, Jinbin Cao, Xiaoshu Wu, Xiaoxin Zhang, Nihan Chen, Yihui Song, Jianxuan Wang, Yuchen Cao, and Jianing Zhao. 2024. "The Asymmetrical Distribution of a Dominant Motional Electric Field within the Martian Magnetosheath" Magnetochemistry 10, no. 8: 62. https://doi.org/10.3390/magnetochemistry10080062
APA StyleLi, S., Lu, H., Cao, J., Wu, X., Zhang, X., Chen, N., Song, Y., Wang, J., Cao, Y., & Zhao, J. (2024). The Asymmetrical Distribution of a Dominant Motional Electric Field within the Martian Magnetosheath. Magnetochemistry, 10(8), 62. https://doi.org/10.3390/magnetochemistry10080062






