Effect of the Core–Shell Exchange Coupling on the Approach to Magnetic Saturation in a Ferrimagnetic Nanoparticle
Abstract
1. Introduction
2. The Forms of the LAMS
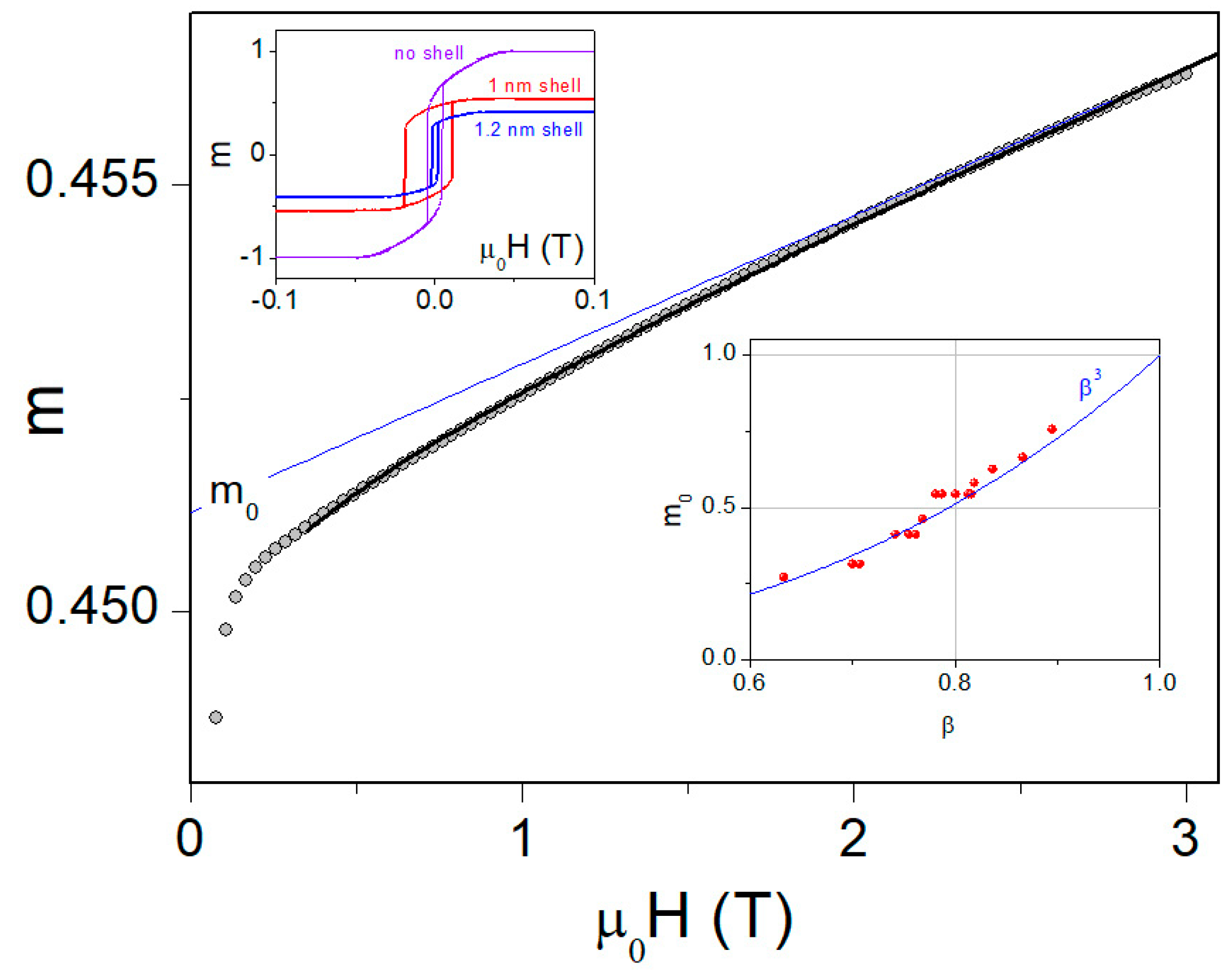
3. Micromagnetic Testing
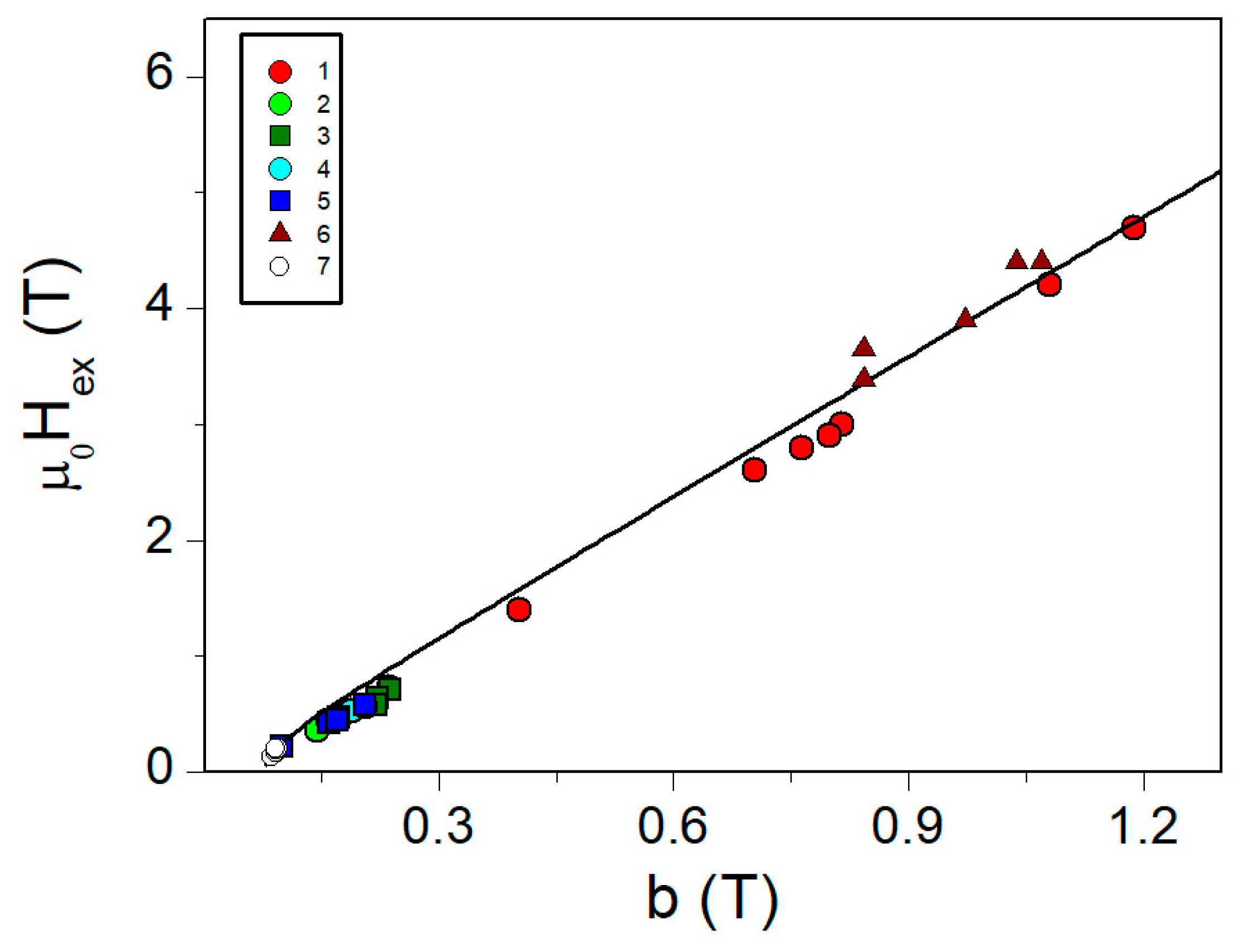
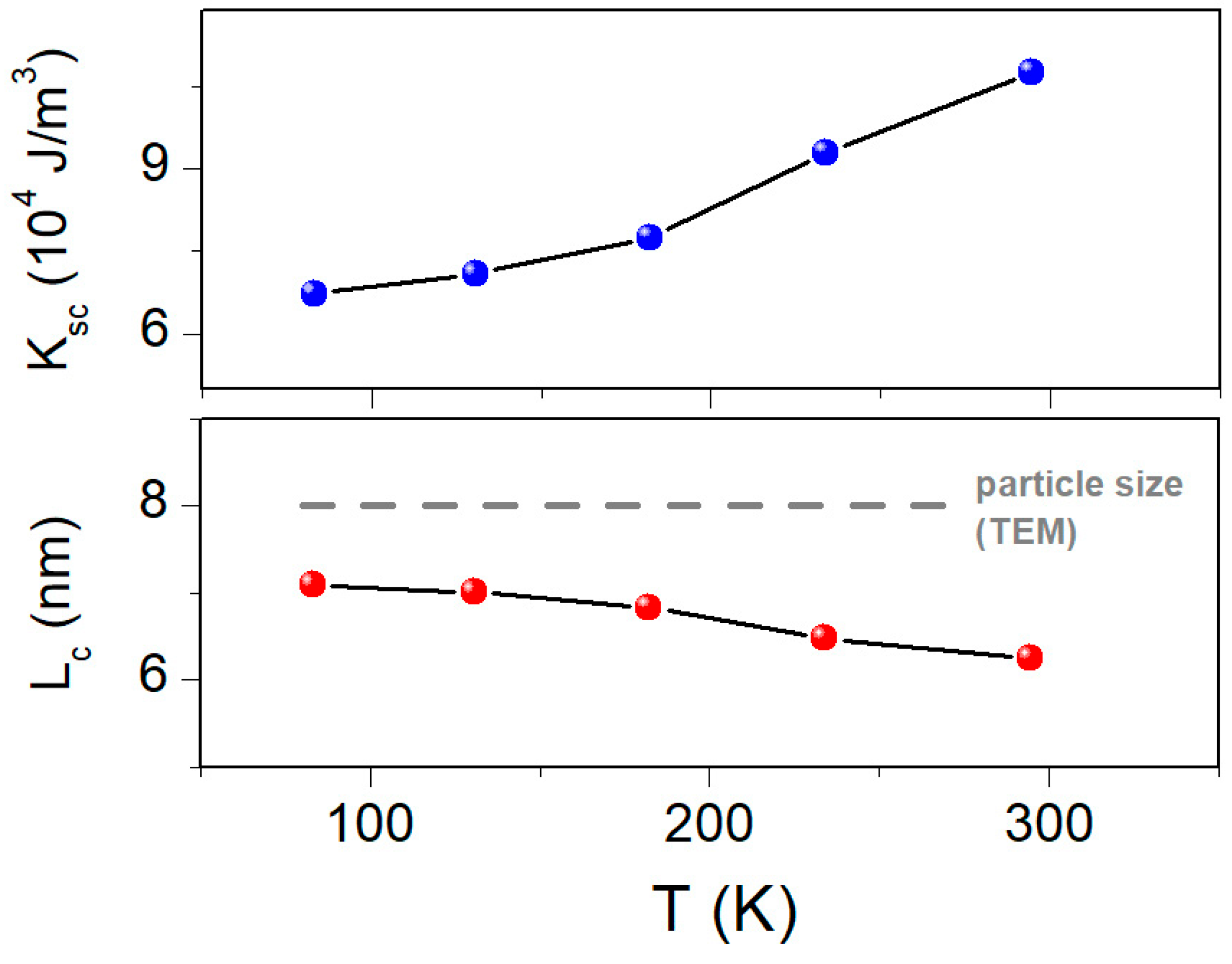
4. Experimental Testing
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Pankhurst, Q.A.; Connolly, J.; Jones, S.K.; Dobson, J. Applications of Magnetic Nanoparticles in Biomedicine. J. Phys. D Appl. Phys. 2003, 36, R167–R181. [Google Scholar] [CrossRef]
- Mohammed, L.; Gomaa, H.G.; Ragab, D.; Zhu, J. Magnetic Nanoparticles for Environmental and Biomedical Applications: A Review. Particuology 2017, 30, 1–14. [Google Scholar] [CrossRef]
- Rancourt, D.G. Magnetism of Earth, Planetary, and Environmental Nanomaterials. Rev. Mineral. Geochem. 2001, 44, 217–292. [Google Scholar] [CrossRef]
- Ma, Y.; Zou, Y.; Meng, L.; Cai, L.; Xiong, S.; Chen, G.; Dong, C.; Guan, H. Ni@C/PPy Composites Derived from Ni-MOF Materials for Efficient Microwave Absorption. Magnetochemistry 2024, 10, 24. [Google Scholar] [CrossRef]
- Ziogas, P.G.; Bourlinos, A.B.; Chatzopoulou, P.; Dimitrakopulos, G.P.; Markou, A.; Douvalis, A.P. Novel Hybrid Ferromagnetic Fe–Co/Nanodiamond Nanostructures: Influence of Carbon on Their Structural and Magnetic Properties. Magnetochemistry 2024, 10, 35. [Google Scholar] [CrossRef]
- Moacă, E.-A.; Socoliuc, V.; Stoian, D.; Watz, C.; Flondor, D.; Păcurariu, C.; Ianoș, R.; Rus, C.I.; Barbu-Tudoran, L.; Semenescu, A.; et al. Synthesis and Characterization of Bioactive Magnetic Nanoparticles from the Perspective of Hyperthermia Applications. Magnetochemistry 2022, 8, 145. [Google Scholar] [CrossRef]
- Levin, C.S.; Hofmann, C.; Ali, T.A.; Kelly, A.T.; Morosan, E.; Nordlander, P.; Whitmire, K.H.; Halas, N.J. Magnetic−Plasmonic Core−Shell Nanoparticles. ACS Nano 2009, 3, 1379–1388. [Google Scholar] [CrossRef] [PubMed]
- Rajabi-Moghaddam, H.; Naimi-Jamal, M.R.; Tajbakhsh, M. Fabrication of Copper(II)-Coated Magnetic Core-Shell Nanoparticles Fe3O4@SiO2-2-Aminobenzohydrazide and Investigation of Its Catalytic Application in the Synthesis of 1,2,3-Triazole Compounds. Sci. Rep. 2021, 11, 2073. [Google Scholar] [CrossRef] [PubMed]
- Tsamos, D.; Krestou, A.; Papagiannaki, M.; Maropoulos, S. An Overview of the Production of Magnetic Core-Shell Nanoparticles and Their Biomedical Applications. Metals 2022, 12, 605. [Google Scholar] [CrossRef]
- Mittal, A.; Roy, I.; Gandhi, S. Magnetic Nanoparticles: An Overview for Biomedical Applications. Magnetochemistry 2022, 8, 107. [Google Scholar] [CrossRef]
- Coey, J.M.D. Noncollinear Spin Arrangement in Ultrafine Ferrimagnetic Crystallites. Phys. Rev. Lett. 1971, 27, 1140–1142. [Google Scholar] [CrossRef]
- Gareev, K.G. Diversity of Iron Oxides: Mechanisms of Formation, Physical Properties and Applications. Magnetochemistry 2023, 9, 119. [Google Scholar] [CrossRef]
- Silva, N.J.O.; Amaral, V.S.; Urtizberea, A.; Bustamante, R.; Millán, A.; Palacio, F.; Kampert, E.; Zeitler, U.; de Brion, S.; Iglesias, Ò.; et al. Shifted Loops and Coercivity from Field-Imprinted High-Energy Barriers in Ferritin and Ferrihydrite Nanoparticles. Phys. Rev. B 2011, 84, 104427. [Google Scholar] [CrossRef]
- Mamiya, H.; Nakatani, I.; Furubayashi, T. Magnetic Relaxations of Antiferromagnetic Nanoparticles in Magnetic Fields. Phys. Rev. Lett. 2002, 88, 067202. [Google Scholar] [CrossRef] [PubMed]
- Kodama, R.H.; Berkowitz, A.E.; McNiff, E.J., Jr.; Foner, S. Surface Spin Disorder in NiFe2O4 Nanoparticles. Phys. Rev. Lett. 1996, 77, 394–397. [Google Scholar] [CrossRef] [PubMed]
- Kodama, R. Magnetic Nanoparticles. J. Magn. Magn. Mater. 1999, 200, 359–372. [Google Scholar] [CrossRef]
- Safronov, A.P.; Beketov, I.V.; Komogortsev, S.V.; Kurlyandskaya, G.V.; Medvedev, A.I.; Leiman, D.V.; Larrañaga, A.; Bhagat, S.M. Spherical Magnetic Nanoparticles Fabricated by Laser Target Evaporation. AIP Adv. 2013, 3, 052135. [Google Scholar] [CrossRef]
- Soler, M.A.G.; Paterno, L.G. Magnetic Nanomaterials. In Nanostructures; Elsevier: Amsterdam, The Netherlands, 2017; pp. 147–186. [Google Scholar]
- Benguettat-El Mokhtari, I.; Schmool, D.S. Ferromagnetic Resonance in Magnetic Oxide Nanoparticules: A Short Review of Theory and Experiment. Magnetochemistry 2023, 9, 191. [Google Scholar] [CrossRef]
- Kons, C.; Srikanth, H.; Phan, M.-H.; Arena, D.A.; Pereiro, M. Macrospin Model of an Assembly of Magnetically Coupled Core-Shell Nanoparticles. Phys. Rev. B 2022, 106, 104402. [Google Scholar] [CrossRef]
- Guduri, B.R.; Luyt, A.S. Structure and Mechanical Properties of Polycarbonate Modified Clay Nanocomposites. J. Nanosci. Nanotechnol. 2008, 8, 1880–1885. [Google Scholar] [CrossRef]
- Balaev, D.A.; Krasikov, A.A.; Dubrovskii, A.A.; Semenov, S.V.; Bayukov, O.A.; Stolyar, S.V.; Iskhakov, R.S.; Ladygina, V.P.; Ishchenko, L.A. Magnetic Properties and the Mechanism of Formation of the Uncompensated Magnetic Moment of Antiferromagnetic Ferrihydrite Nanoparticles of a Bacterial Origin. J. Exp. Theor. Phys. 2014, 119, 479–487. [Google Scholar] [CrossRef]
- Bedanta, S.; Kleemann, W. Supermagnetism. J. Phys. D Appl. Phys. 2009, 42, 013001. [Google Scholar] [CrossRef]
- Balaev, D.A.; Krasikov, A.A.; Dubrovskii, A.A.; Bayukov, O.A.; Stolyar, S.V.; Iskhakov, R.S.; Ladygina, V.P.; Yaroslavtsev, R.N. The Effect of Low-Temperature Heat Treatment on the Magnetic Properties of Biogenic Ferrihydrite Nanoparticles. Tech. Phys. Lett. 2015, 41, 705–709. [Google Scholar] [CrossRef]
- Balaev, D.A.; Krasikov, A.A.; Dubrovskiy, A.A.; Popkov, S.I.; Stolyar, S.V.; Bayukov, O.A.; Iskhakov, R.S.; Ladygina, V.P.; Yaroslavtsev, R.N. Magnetic Properties of Heat Treated Bacterial Ferrihydrite Nanoparticles. J. Magn. Magn. Mater. 2016, 410, 171–180. [Google Scholar] [CrossRef]
- Balaev, D.A.; Krasikov, A.A.; Dubrovskiy, A.A.; Popkov, S.I.; Stolyar, S.V.; Iskhakov, R.S.; Ladygina, V.P.; Yaroslavtsev, R.N. Exchange Bias in Nano-Ferrihydrite. J. Appl. Phys. 2016, 120, 183903. [Google Scholar] [CrossRef]
- Bruvera, I.J.; Mendoza Zélis, P.; Pilar Calatayud, M.; Goya, G.F.; Sánchez, F.H. Determination of the Blocking Temperature of Magnetic Nanoparticles: The Good, the Bad, and the Ugly. J. Appl. Phys. 2015, 118, 184304. [Google Scholar] [CrossRef]
- Komogortsev, S.V.; Patrusheva, T.N.; Balaev, D.A.; Denisova, E.A.; Ponomarenko, I.V. Cobalt Ferrite Nanoparticles in a Mesoporous Silicon Dioxide Matrix. Tech. Phys. Lett. 2009, 35, 882–884. [Google Scholar] [CrossRef]
- Poperechny, I.S.; Raikher, Y.L.; Stepanov, V.I. Dynamic Hysteresis of a Uniaxial Superparamagnet: Semi-Adiabatic Approximation. Phys. B Condens. Matter 2013, 435, 58–61. [Google Scholar] [CrossRef]
- Komogortsev, S.V.; Balaev, D.A.; Krasikov, A.A.; Stolyar, S.V.; Yaroslavtsev, R.N.; Ladygina, V.P.; Iskhakov, R.S. Magnetic Hysteresis of Blocked Ferrihydrite Nanoparticles. AIP Adv. 2021, 11, 015329. [Google Scholar] [CrossRef]
- Akulov, N.S. Uber Den Verlauf Der Magnetisierungskurve in Starken Feldern. Z. Fur Phys. Phys. 1931, 69, 822–831. [Google Scholar] [CrossRef]
- Czerlinsky, E. Über Magnetische Sättigung. Ann. Phys. 1932, 405, 80–100. [Google Scholar] [CrossRef]
- Brown, W. Theory of the Approach to Magnetic Saturation. Phys. Rev. 1940, 58, 736–743. [Google Scholar] [CrossRef]
- Devi, E.C.; Soibam, I. Law of Approach to Saturation in Mn–Zn Ferrite Nanoparticles. J. Supercond. Nov. Magn. 2018, 32, 1293–1298. [Google Scholar] [CrossRef]
- Devi, E.C.; Soibam, I. Magnetic Properties and Law of Approach to Saturation in Mn-Ni Mixed Nanoferrites. J. Alloys Compd. 2019, 772, 920–924. [Google Scholar] [CrossRef]
- Stolyar, S.V.; Komogortsev, S.V.; Gorbenko, A.S.; Knyazev, Y.V.; Yaroslavtsev, R.N.; Olkhovskiy, I.A.; Neznakhin, D.S.; Tyumentseva, A.V.; Bayukov, O.A.; Iskhakov, R.S. Maghemite Nanoparticles for DNA Extraction: Performance and Blocking Temperature. J. Supercond. Nov. Magn. 2022, 35, 1929–1936. [Google Scholar] [CrossRef]
- Iskhakov, R.S.; Komogortsev, S.V. Magnetic Microstructure of Amorphous, Nanocrystalline, and Nanophase Ferromagnets. Phys. Met. Metallogr. 2011, 112, 666–681. [Google Scholar] [CrossRef]
- Abo, G.S.; Hong, Y.-K.; Park, J.; Lee, J.; Lee, W.; Choi, B.-C. Definition of Magnetic Exchange Length. IEEE Trans. Magn. 2013, 49, 4937–4939. [Google Scholar] [CrossRef]
- Komogortsev, S.V.; Iskhakov, R.S. Law of Approach to Magnetic Saturation in Nanocrystalline and Amorphous Ferromagnets with Improved Transition Behavior between Power-Law Regimes. J. Magn. Magn. Mater. 2017, 440, 213–216. [Google Scholar] [CrossRef]
- Beketov, I.V.; Safronov, A.P.; Medvedev, A.I.; Alonso, J.; Kurlyandskaya, G.V.; Bhagat, S.M. Iron Oxide Nanoparticles Fabricated by Electric Explosion of Wire: Focus on Magnetic Nanofluids. AIP Adv. 2012, 2, 022154. [Google Scholar] [CrossRef]
- Komogortsev, S.V.; Denisova, E.A.; Iskhakov, R.S.; Balaev, A.D.; Chekanova, L.A.; Kalinin, Y.E.; Sitnikov, A. V Multilayer Nanogranular Films (Co40Fe40B20)50(SiO2)50/α-Si:H and (Co40Fe40B20)50(SiO2)50/SiO2: Magnetic Properties. J. Appl. Phys. 2013, 113, 17C105. [Google Scholar] [CrossRef]
- Komogortsev, S.V.; Iskhakov, R.S.; Zimin, A.A.; Filatov, E.Y.; Korenev, S.V.; Shubin, Y.V.; Chizhik, N.A.; Yurkin, G.Y.; Eremin, E.V. The Exchange Interaction Effects on Magnetic Properties of the Nanostructured CoPt Particles. J. Magn. Magn. Mater. 2016, 401, 236–241. [Google Scholar] [CrossRef]
- Stolyar, S.V.; Komogortsev, S.V.; Chekanova, L.A.; Yaroslavtsev, R.N.; Bayukov, O.A.; Velikanov, D.A.; Volochaev, M.N.; Cheremiskina, E.V.; Bairmani, M.S.; Eroshenko, P.E.; et al. Magnetite Nanocrystals with a High Magnetic Anisotropy Constant Due to the Particle Shape. Tech. Phys. Lett. 2019, 45, 878–881. [Google Scholar] [CrossRef]
- Devi, E.C.; Singh, S.D. Tracing the Magnetization Curves: A Review on Their Importance, Strategy, and Outcomes. J. Supercond. Nov. Magn. 2021, 34, 15–25. [Google Scholar] [CrossRef]
- Ignatchenko, V.A.; Iskhakov, R.S.; Popov, G.V. Law of Approach to Ferromagnetic Saturation in Amorphous Ferromagnets. Zh. Eksp. Teor. Fiz. 1982, 82, 1518–1531. [Google Scholar]
- Chudnovsky, E.M.; Saslow, W.M.; Serota, R.A. Ordering in Ferromagnets with Random Anisotropy. Phys. Rev. B 1986, 33, 251–261. [Google Scholar] [CrossRef] [PubMed]
- Chudnovsky, E.M. Magnetic Properties of Amorphous Ferromagnets (Invited). J. Appl. Phys. 1988, 64, 5770. [Google Scholar] [CrossRef]
- Donahue, M.J.; Porter, D.G. OOMMF User’s Guide, Version 1.0; National Institute of Standards and Technology: Gaithersburg, MD, USA, 1999. [Google Scholar]
- Newell, A.J.; Williams, W.; Dunlop, D.J. A Generalization of the Demagnetizing Tensor for Nonuniform Magnetization. J. Geophys. Res. Solid Earth 1993, 98, 9551–9555. [Google Scholar] [CrossRef]
- Aharoni, A. Demagnetizing Factors for Rectangular Ferromagnetic Prisms. J. Appl. Phys. 1998, 83, 3432–3434. [Google Scholar] [CrossRef]
- Coey, J.M.D. Magnetism and Magnetic Materials; Cambridge University Press: New York, NY, USA, 2010. [Google Scholar]
- Komogortsev, S.V.; Iskhakov, R.S.; Zimin, A.A.; Filatov, E.Y.; Korenev, S.V.; Shubin, Y.V.; Chizhik, N.A.; Yurkin, G.Y.; Eremin, E.V. Magnetic Anisotropy and Order Parameter in Nanostructured CoPt Particles. Appl. Phys. Lett. 2013, 103, 152404. [Google Scholar] [CrossRef]
- Kurlyandskaya, G.V.; Bhagat, S.M.; Safronov, A.P.; Beketov, I.V.; Larrañaga, A. Spherical Magnetic Nanoparticles Fabricated by Electric Explosion of Wire. AIP Adv. 2011, 1, 042122. [Google Scholar] [CrossRef]
- Denisova, E.A.; Komogortsev, S.V.; Iskhakov, R.S.; Chekanova, L.A.; Balaev, A.D.; Kalinin, Y.E.; Sitnikov, A.V. Magnetic Anisotropy in Multilayer Nanogranular Films (Co40Fe40B20)50(SiO2)50/α-Si:H. J. Magn. Magn. Mater. 2017, 440, 221–224. [Google Scholar] [CrossRef]
- Komogortsev, S.V.; Stolyar, S.V.; Chekanova, L.A.; Yaroslavtsev, R.N.; Bayukov, O.A.; Velikanov, D.A.; Volochaev, M.N.; Eroshenko, P.E.; Iskhakov, R.S. Square Plate Shaped Magnetite Nanocrystals. J. Magn. Magn. Mater. 2021, 527, 167730. [Google Scholar] [CrossRef]
- Usov, N.A.; Grebenshchikov, Y.B. Hysteresis Loops of an Assembly of Superparamagnetic Nanoparticles with Uniaxial Anisotropy. J. Appl. Phys. 2009, 106, 023917. [Google Scholar] [CrossRef]
- Wood, R. Exact Solution for a Stoner–Wohlfarth Particle in an Applied Field and a New Approximation for the Energy Barrier. IEEE Trans. Magn. 2009, 45, 100–103. [Google Scholar] [CrossRef]
- Yasumori, I.; Reinen, D.; Selwood, P.W. Anisotropic Behavior in Superparamagnetic Systems. J. Appl. Phys. 1963, 34, 3544–3549. [Google Scholar] [CrossRef]
- Stolyar, S.V.; Yaroslavtsev, R.N.; Tyumentseva, A.V.; Komogortsev, S.V.; Tyutrina, E.S.; Saitova, A.T.; Gerasimova, Y.V.; Velikanov, D.A.; Rautskii, M.V.; Iskhakov, R.S. Manifestation of Stoichiometry Deviation in Silica-Coated Magnetite Nanoparticles. J. Phys. Chem. C 2022, 126, 7510–7516. [Google Scholar] [CrossRef]
- Vazhenina, I.G.; Stolyar, S.V.; Tyumentseva, A.V.; Volochaev, M.N.; Iskhakov, R.S.; Komogortsev, S.V.; Pyankov, V.F.; Nikolaeva, E.D. Study of Magnetic Iron Oxide Nanoparticles Coated with Silicon Oxide by Ferromagnetic Method. Phys. Solid State 2023, 65, 884. [Google Scholar] [CrossRef]
- Pérez, N.; Guardia, P.; Roca, A.G.; Morales, M.P.; Serna, C.J.; Iglesias, O.; Bartolomé, F.; García, L.M.; Batlle, X.; Labarta, A. Surface Anisotropy Broadening of the Energy Barrier Distribution in Magnetic Nanoparticles. Nanotechnology 2008, 19, 475704. [Google Scholar] [CrossRef] [PubMed]
- Zákutná, D.; Nižňanský, D.; Barnsley, L.C.; Babcock, E.; Salhi, Z.; Feoktystov, A.; Honecker, D.; Disch, S. Field Dependence of Magnetic Disorder in Nanoparticles. Phys. Rev. X 2020, 10, 031019. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Komogortsev, S.V.; Stolyar, S.V.; Mokhov, A.A.; Fel’k, V.A.; Velikanov, D.A.; Iskhakov, R.S. Effect of the Core–Shell Exchange Coupling on the Approach to Magnetic Saturation in a Ferrimagnetic Nanoparticle. Magnetochemistry 2024, 10, 47. https://doi.org/10.3390/magnetochemistry10070047
Komogortsev SV, Stolyar SV, Mokhov AA, Fel’k VA, Velikanov DA, Iskhakov RS. Effect of the Core–Shell Exchange Coupling on the Approach to Magnetic Saturation in a Ferrimagnetic Nanoparticle. Magnetochemistry. 2024; 10(7):47. https://doi.org/10.3390/magnetochemistry10070047
Chicago/Turabian StyleKomogortsev, Sergey V., Sergey V. Stolyar, Alexey A. Mokhov, Vladimir A. Fel’k, Dmitriy A. Velikanov, and Rauf S. Iskhakov. 2024. "Effect of the Core–Shell Exchange Coupling on the Approach to Magnetic Saturation in a Ferrimagnetic Nanoparticle" Magnetochemistry 10, no. 7: 47. https://doi.org/10.3390/magnetochemistry10070047
APA StyleKomogortsev, S. V., Stolyar, S. V., Mokhov, A. A., Fel’k, V. A., Velikanov, D. A., & Iskhakov, R. S. (2024). Effect of the Core–Shell Exchange Coupling on the Approach to Magnetic Saturation in a Ferrimagnetic Nanoparticle. Magnetochemistry, 10(7), 47. https://doi.org/10.3390/magnetochemistry10070047





