Investigation and Modeling of Gas-Liquid Mass Transfer in a Sparged and Non-Sparged Continuous Stirred Tank Reactor with Potential Application in Syngas Fermentation
Abstract
1. Introduction
2. Materials and Methods
2.1. CSTR Configuration and Operating Conditions
2.2. Calculations
2.2.1. Overall Volumetric Mass Transfer Coefficient
2.2.2. Volumetric Flow Rate at Various Headspace Pressures
2.2.3. Power Consumption
2.2.4. Mass Transfer Model of a 7-L CSTR
2.2.5. Statistical Analysis
3. Results and Discussion
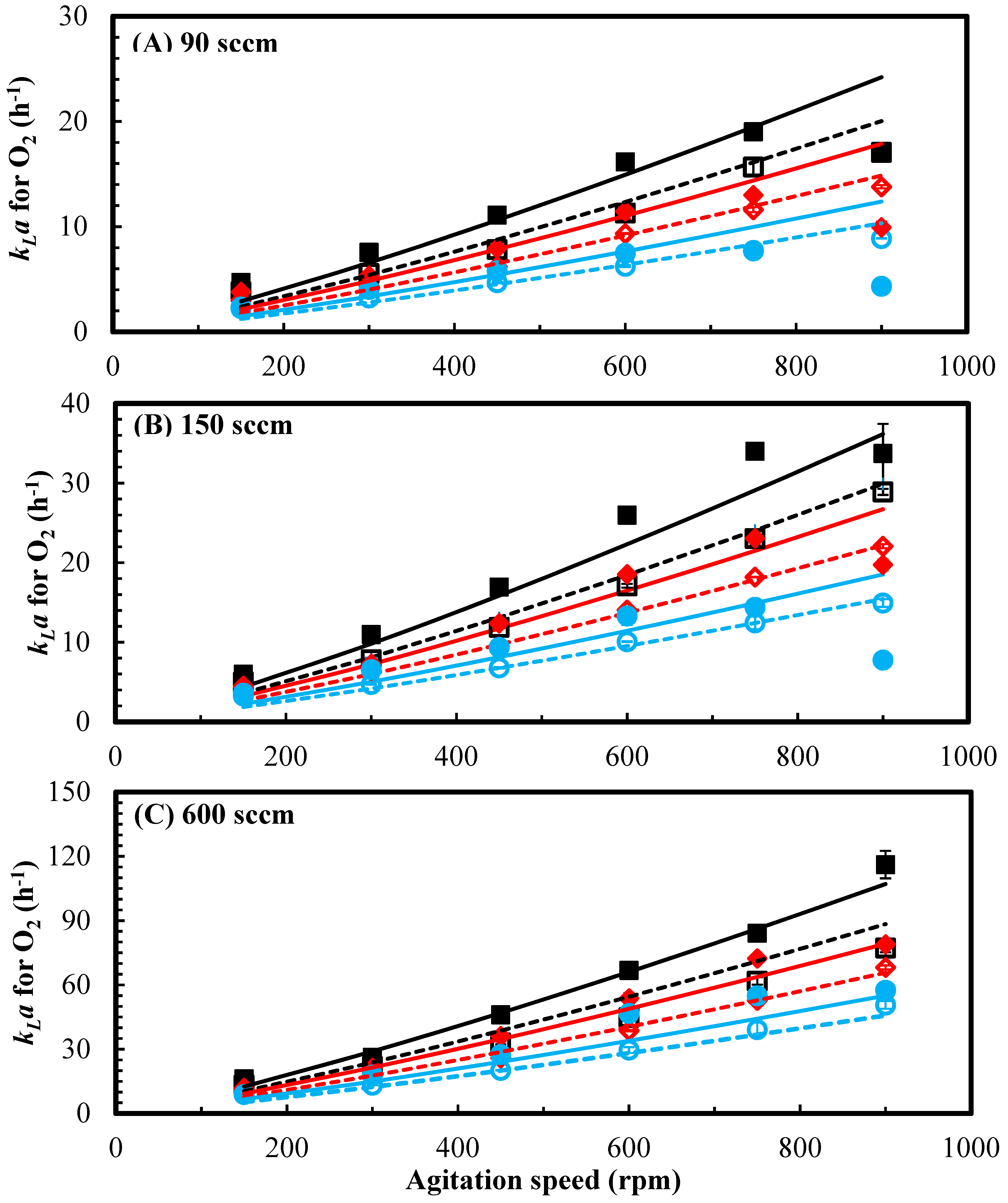
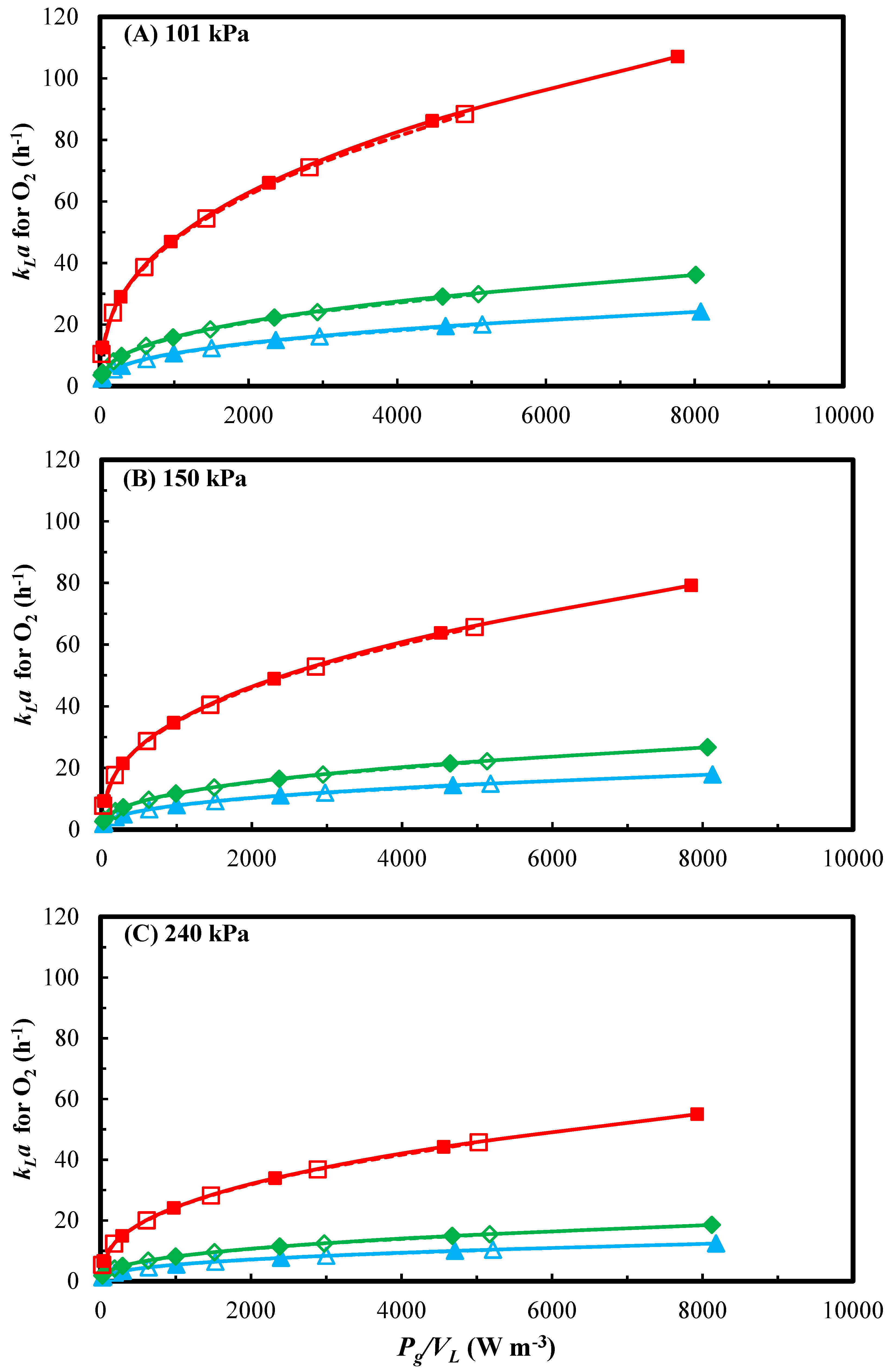
3.1. Effect of Agitation Speed, Pressure and Gas Flow Rate on kLa
3.2. Effect of Headspace Backmixing on kLa
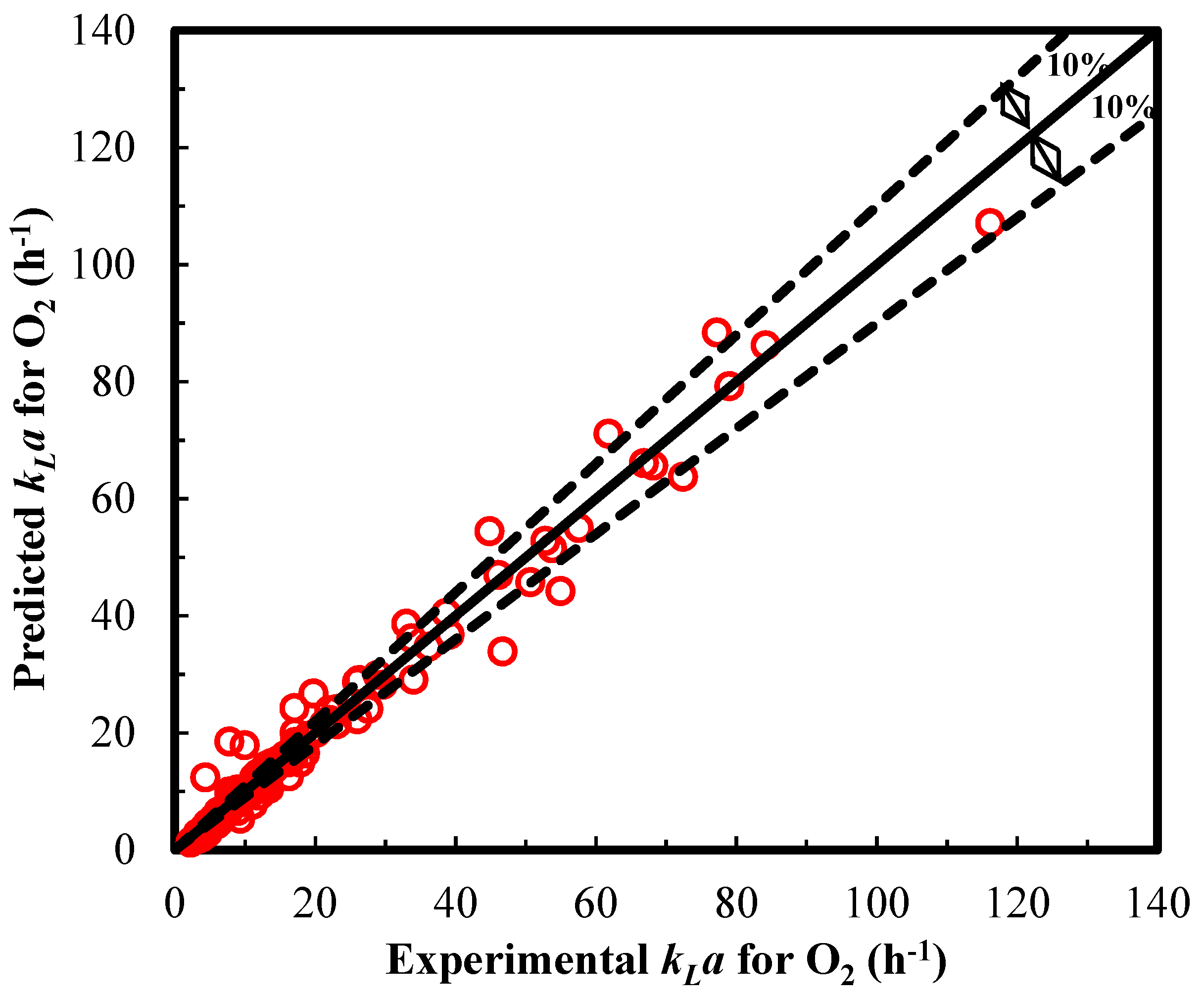
3.3. Model Predictions of kLa for O2
3.4. Model Predictions of kLa for CO, CO2 and H2
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| a | Gas-liquid interfacial area per unit volume (m−1) |
| A, B, C and E Parameters in Equations (9) and (10) | |
| CL | Bulk dissolved oxygen (DO) concentration in the liquid (mol m−3) |
| CS | Saturated dissolved oxygen (DO) concentration (mol m−3) |
| D | Impeller diameter (m) |
| Di and Dj | Diffusivities of gas species i and j in water (cm2 s−1) |
| DO | Dissolved oxygen (%) |
| g | Gravitational acceleration (m s−2) |
| h | Distance of microsparger from the surface of liquid (m) |
| kL | Liquid film mass transfer coefficient (m h−1) |
| kLa | Overall volumetric mass transfer coefficient (h−1) |
| Ma | Molecular weight of air (g mol−1) |
| MFC | Mass flow controller |
| na | Molar air flow rate (mol min−1) |
| N | Rotation speed of impeller (s−1) |
| NA | Aeration number (dimensionless) |
| NFr | Froude number (dimensionless) |
| NIST | National Institute of Science and Technology |
| Np | Power number of single Rushton impeller or marine impeller (dimensionless) |
| PHS | Headspace pressure (kPa) |
| PHP | Liquid pressure above the microsparger (kPa) |
| Ptotal | Total pressure in the CSTR (kPa) |
| Pg | Total impeller power consumption at gassed condition (W) |
| Pg,lower | Gassed power consumption of single impeller mounted directly above microsparger (W) |
| Pg,upper | Gassed power consumption for impellers not directly installed above microsparger (W) |
| Pg/VL | Power consumption per unit volume (W m−3) |
| Pu | Ungassed power consumption of single impeller (W) |
| Qg | Volumetric air flow rate at the applied pressure and 37 °C (mL min−1) |
| R | Ideal gas law constant (L kPa mol−1 K−1) |
| t | Time (h) |
| T | Tank diameter (m) |
| TNIST | NIST standard temperature of 293.15 K |
| vg | Superficial gas velocity (m s−1) |
| VL | Liquid working volume in CSTR (m3) |
| VNIST | Volumetric flow rate of air at standard NIST conditions (m3 min−1) |
| α, β and c | Model parameters in Equation (11) |
| ρa | Air density (kg m−3) |
| ρw | Water density (kg m−3) |
| µ | Dynamic viscosity of water (Pa s) |
References
- Ibrahim, M.F.; Ramli, N.; Kamal Bahrin, E.; Abd-Aziz, S. Cellulosic biobutanol by Clostridia: Challenges and improvements. Renew. Sust. Energ. Rev. 2017, 79, 1241–1254. [Google Scholar] [CrossRef]
- Devarapalli, M.; Atiyeh, H.K. A review of conversion processes for bioethanol production with a focus on syngas fermentation. Biofuel Res. J. 2015, 2, 268–280. [Google Scholar]
- Liu, K.; Atiyeh, H.K.; Pardo-Planas, O.; Ezeji, T.C.; Ujor, V.; Overton, J.C.; Berning, K.; Wilkins, M.R.; Tanner, R.S. Butanol production from hydrothermolysis-pretreated switchgrass: Quantification of inhibitors and detoxification of hydrolyzate. Bioresour. Technol. 2015, 189, 292–301. [Google Scholar] [CrossRef] [PubMed]
- Ramachandriya, K.D.; Kundiyana, D.K.; Sharma, A.M.; Kumar, A.; Atiyeh, H.K.; Huhnke, R.L.; Wilkins, M.R. Critical factors affecting the integration of biomass gasification and syngas fermentation technology. AIMS Bioeng. 2016, 3, 188–210. [Google Scholar] [CrossRef]
- Pardo-Planas, O.; Atiyeh, H.K.; Phillips, J.R.; Aichele, C.P.; Mohammad, S. Process simulation of ethanol production from biomass gasification and syngas fermentation. Bioresour. Technol. 2017, 245, 925–932. [Google Scholar] [CrossRef] [PubMed]
- Tanner, R.S. Production of ethanol from synthesis gas. In Bioenergy; Wall, J.D., Harwood, C.S., Demain, A.L., Eds.; American Society of Microbiology: Washington, DC, USA, 2008; pp. 147–151. [Google Scholar]
- Wilkins, M.R.; Atiyeh, H.K. Microbial production of ethanol from carbon monoxide. Curr. Opin. Biotechnol. 2011, 22, 326–330. [Google Scholar] [CrossRef] [PubMed]
- Liu, K.; Atiyeh, H.K.; Tanner, R.S.; Wilkins, M.R.; Huhnke, R.L. Fermentative production of ethanol from syngas using novel moderately alkaliphilic strains of Alkalibaculum bacchi. Bioresour. Technol. 2012, 104, 336–341. [Google Scholar]
- Phillips, J.R.; Atiyeh, H.K.; Tanner, R.S.; Torres, J.R.; Saxena, J.; Wilkins, M.R.; Huhnke, R.L. Butanol and hexanol production in Clostridium carboxidivorans syngas fermentation: Medium development and culture techniques. Bioresour. Technol. 2015, 190, 114–121. [Google Scholar] [CrossRef]
- Sun, X.; Atiyeh, H.K.; Kumar, A.; Zhang, H.; Tanner, R.S. Biochar enhanced ethanol and butanol production by Clostridium carboxidivorans from syngas. Bioresour. Technol. 2018, 265, 128–138. [Google Scholar] [CrossRef]
- Bredwell, M.D.; Srivastava, P.; Worden, R.M. Reactor design issues for synthesis-gas fermentations. Biotechnol. Prog. 1999, 15, 834–844. [Google Scholar]
- Klasson, K.T.; Ackerson, M.D.; Clausen, E.C.; Gaddy, J.L. Biological conversion of coal and coal-derived synthesis gas. Fuel 1993, 72, 1673–1678. [Google Scholar] [CrossRef]
- Munasinghe, P.C.; Khanal, S.K. Biomass-derived syngas fermentation into biofuels: Opportunities and challenges. Bioresour. Technol. 2010, 101, 5013–5022. [Google Scholar] [CrossRef] [PubMed]
- Vega, J.L.; Antorrena, G.M.; Clausen, E.C.; Gaddy, J.L. Study of gaseous substrate fermentations: Carbon monoxide conversion to acetate. 2. Continuous culture. Biotechnol. Bioeng. 1989, 34, 785–793. [Google Scholar] [CrossRef] [PubMed]
- Orgill, J.J.; Atiyeh, H.K.; Devarapalli, M.; Phillips, J.R.; Lewis, R.S.; Huhnke, R.L. A comparison of mass transfer coefficients between trickle-bed, hollow fiber membrane and stirred tank reactors. Bioresour. Technol. 2013, 133, 340–346. [Google Scholar] [CrossRef] [PubMed]
- Shen, Y.; Brown, R.; Wen, Z. Enhancing mass transfer and ethanol production in syngas fermentation of Clostridium carboxidivorans P7 through a monolithic biofilm reactor. Appl. Energ. 2014, 136, 68–76. [Google Scholar] [CrossRef]
- Devarapalli, M.; Atiyeh, H.K.; Phillips, J.R.; Lewis, R.S.; Huhnke, R.L. Ethanol production during semi-continuous syngas fermentation in a trickle bed reactor using Clostridium ragsdalei. Bioresour. Technol. 2016, 209, 56–65. [Google Scholar] [CrossRef] [PubMed]
- Shen, Y.; Brown, R.C.; Wen, Z. Syngas fermentation by Clostridium carboxidivorans P7 in a horizontal rotating packed bed biofilm reactor with enhanced ethanol production. Appl. Energ. 2017, 187, 585–594. [Google Scholar] [CrossRef]
- Jang, N.; Yasin, M.; Park, S.; Lovitt, R.W.; Chang, I.S. Determination of volumetric gas–liquid mass transfer coefficient of carbon monoxide in a batch cultivation system using kinetic simulations. Bioresour. Technol. 2017, 239, 387–393. [Google Scholar] [CrossRef] [PubMed]
- Yasin, M.; Park, S.; Jeong, Y.; Lee, E.Y.; Lee, J.; Chang, I.S. Effect of internal pressure and gas/liquid interface area on the CO mass transfer coefficient using hollow fibre membranes as a high mass transfer gas diffusing system for microbial syngas fermentation. Bioresour. Technol. 2014, 169, 637–643. [Google Scholar] [CrossRef]
- Lee, P.-H.; Ni, S.-Q.; Chang, S.-Y.; Sung, S.; Kim, S.-H. Enhancement of carbon monoxide mass transfer using an innovative external hollow fiber membrane (HFM) diffuser for syngas fermentation: Experimental studies and model development. Chem. Eng. J. 2012, 184, 268–277. [Google Scholar] [CrossRef]
- Orgill, J.J.; Abboud, M.C.; Atiyeh, H.K.; Devarapalli, M.; Sun, X.; Lewis, R.S. Measurement and prediction of mass transfer coefficients for syngas constituents in a hollow fiber reactor. Bioresour. Technol. 2019, 276, 1–7. [Google Scholar] [CrossRef] [PubMed]
- Liew, F.M.; Koöpke, M.; Simpson, S.D. Gas fermentation for commercial biofuels production. In Liquid, Gaseous and Solid Biofuels; Fang, Z., Ed.; InTech: London, UK, 2013; pp. 125–173. [Google Scholar]
- Shen, Y.; Brown, R.; Wen, Z. Syngas fermentation of Clostridium carboxidivoran P7 in a hollow fiber membrane biofilm reactor: Evaluating the mass transfer coefficient and ethanol production performance. Biochem. Eng. J. 2014, 85, 21–29. [Google Scholar] [CrossRef]
- Abubackar, H.N.; Veiga, M.C.; Kennes, C. Biological conversion of carbon monoxide: Rich syngas or waste gases to bioethanol. BiofuelsBioprod. Biorefin. 2011, 5, 93–114. [Google Scholar] [CrossRef]
- Yasin, M.; Jeong, Y.; Park, S.; Jeong, J.; Lee, E.Y.; Lovitt, R.W.; Kim, B.H.; Lee, J.; Chang, I.S. Microbial synthesis gas utilization and ways to resolve kinetic and mass-transfer limitations. Bioresour. Technol. 2015, 177, 361–374. [Google Scholar] [CrossRef] [PubMed]
- Asimakopoulos, K.; Gavala, H.N.; Skiadas, I.V. Reactor systems for syngas fermentation processes: A review. Chem. Eng. J. 2018, 348, 732–744. [Google Scholar] [CrossRef]
- Klasson, K.T.; Ackerson, M.D.; Clausen, E.C.; Gaddy, J.L. Bioreactors for synthesis gas fermentations. Resour. Conserv. Recy. 1991, 5, 145–165. [Google Scholar] [CrossRef]
- Younesi, H.; Najafpour, G.; Ku Ismail, K.S.; Mohamed, A.R.; Kamaruddin, A.H. Biohydrogen production in a continuous stirred tank bioreactor from synthesis gas by anaerobic photosynthetic bacterium: Rhodopirillum rubrum. Bioresour. Technol. 2008, 99, 2612–2619. [Google Scholar] [CrossRef]
- Lopes, M.; Belo, I.; Mota, M. Over-pressurized bioreactors: Application to microbial cell cultures. Biotechnol. Prog. 2014, 30, 767–775. [Google Scholar] [CrossRef]
- Lopes, M.; Mota, M.; Belo, I. Oxygen Mass Transfer Rate in a Pressurized Lab-Scale Stirred Bioreactor. Chem. Eng. Technol. 2013, 36, 1779–1784. [Google Scholar] [CrossRef]
- Shuler, M.L.; Fikret, K. Bioprocess Engineering: Basic Concepts, 2nd ed.; Prentice Hall: Englewood Cliffs, NJ, USA, 2002; p. 576. [Google Scholar]
- Kawase, Y.; Halard, B.; Moo-Young, M. Liquid-phase mass transfer coefficients in bioreactors. Biotechnol. Bioeng. 1992, 39, 1133–1140. [Google Scholar] [CrossRef]
- McCabe, W.L.; Smith, J.C. Unit Operations of Chemcial Engineering, 7th ed.; Mcgraw-Hill Inc.: New York, NY, USA, 2005; p. 1007. [Google Scholar]
- Bakker, A.; Smith, J.; Myers, K. How to disperse gases in liquids. Chem. Eng. 1994, 101, 98–104. [Google Scholar]
- Munson, B.R.; Young, D.F.; Okiishi, T.H.; Huebsch, W.W. Fundamentals of Fluid Mechanics, 6th ed.; Willey: Hoboken, NJ, USA, 2009; p. 784. [Google Scholar]
- Lee, J.M. Biochemcial Engineering; Prentice-Hall: Englewood Cliffs, NJ, USA, 1992; p. 240. [Google Scholar]
- Blanch, H.W.; Clark, d.S. Biochemcial Engineering; Marcel Dekker, Inc: New York, NY, USA, 1997; p. 702. [Google Scholar]
- Ahmed, A.; Cateni, B.G.; Huhnke, R.L.; Lewis, R.S. Effects of biomass-generated producer gas constituents on cell growth, product distribution and hydrogenase activity of Clostridium carboxidivorans P7T. Biomass Bioenergy 2006, 30, 665–672. [Google Scholar] [CrossRef]
- Stenberg, O.; Andersson, B. Gas-liquid mass transfer in agitated vessels—II. Modelling of gas-liquid mass transfer. Chem. Eng. Sci. 1988, 43, 725–730. [Google Scholar] [CrossRef]
- Liu, K.; Atiyeh, H.K.; Stevenson, B.S.; Tanner, R.S.; Wilkins, M.R.; Huhnke, R.L. Continuous syngas fermentation for the production of ethanol, n-propanol and n-butanol. Bioresour. Technol. 2014, 151, 69–77. [Google Scholar] [CrossRef] [PubMed]
- Phillips, J.; Klasson, K.; Clausen, E.; Gaddy, J. Biological production of ethanol from coal synthesis gas. Appl. Biochem. Biotechnol. 1993, 39, 559–571. [Google Scholar] [CrossRef]
- Munasinghe, P.C.; Khanal, S.K. Syngas fermentation to biofuel: Evaluation of carbon monoxide mass transfer and analytical modeling using a composite hollow fiber (CHF) membrane bioreactor. Bioresour. Technol. 2012, 122, 130–136. [Google Scholar] [CrossRef] [PubMed]
- Jang, N.; Yasin, M.; Kang, H.; Lee, Y.; Park, G.W.; Park, S.; Chang, I.S. Bubble coalescence suppression driven carbon monoxide (CO)-water mass transfer increase by electrolyte addition in a hollow fiber membrane bioreactor (HFMBR) for microbial CO conversion to ethanol. Bioresour. Technol. 2018, 263, 375–384. [Google Scholar] [CrossRef]
- Riggs, S.S.; Heindel, T.J. Measuring Carbon Monoxide Gas—Liquid Mass Transfer in a Stirred Tank Reactor for Syngas Fermentation. Biotechnol. Prog. 2006, 22, 903–906. [Google Scholar] [CrossRef]
- Ungerman, A.J.; Heindel, T.J. Carbon Monoxide Mass Transfer for Syngas Fermentation in a Stirred Tank Reactor with Dual Impeller Configurations. Biotechnol. Prog. 2007, 23, 613–620. [Google Scholar] [CrossRef]
- Munasinghe, P.C.; Khanal, S.K. Evaluation of hydrogen and carbon monoxide mass transfer and a correlation between the myoglobin-protein bioassay and gas chromatography method for carbon monoxide determination. Rsc Adv. 2014, 4, 37575–37581. [Google Scholar] [CrossRef]
- Munasinghe, P.C.; Khanal, S.K. Syngas fermentation to biofuel: Evaluation of carbon monoxide mass transfer coefficient (kLa) in different reactor configurations. Biotechnol. Prog. 2010, 26, 1616–1621. [Google Scholar] [CrossRef] [PubMed]



| Parameters | ||||||
|---|---|---|---|---|---|---|
| Headspace pressure (kPa) | 101 | 101 | 150 | 150 | 240 | 240 |
| Agitation speed (rpm) | 150 | 900 | 150 | 900 | 150 | 900 |
| kLa for O2 (h−1) a | 0.7 ± 0.0 | 67.3 ± 1.0 † | 0.8 ± 0.0 | 92.0 ± 3.5 † | 1.3 ± 0.4 | 119.3 ± 2.1 † |
| kLa for O2 (h−1) b | — ‡ | 67.0 ± 0.2 † | — | 88.2 ± 0.3 † | — | 122.3 ± 1.4 † |
| kLa for O2 (h−1) c | 0.2 ± 0.0 | 1.5 ± 0.0 | 0.2 ± 0.0 | 1.8 ± 0.0 | 0.3 ± 0.0 | 1.8 ± 0.0 |
| Parameters | |||
|---|---|---|---|
| Headspace pressure (kPa) a | 101 | 150 | 240 |
| Volumetric flow rate at 37 °C (mL min−1) | 628 | 428 | 270 |
| kLa without flushing headspace with air (h−1) | 116.2 ± 6.4 | 79.0 ± 0.1 † | 57.7 ± 0.5 † |
| kLa with flushing headspace with aira (h−1) | 139.8 ± 5.4 | 116.8 ± 5.7 † | 82.6 ± 1.4 † |
| kLa increase due to backmixing (%) | 20.3 | 47.9 | 43.2 |
| Reactora | Physical (Working) Volumes (L) | Gas | Medium | Gas Flow (mL min−1) | Gas Pressure (kPa) | Agitation (rpm) | Liquid Flow (mL min−1) | kLa (h−1) | References |
|---|---|---|---|---|---|---|---|---|---|
| CSTR | 7.0 (3.0) | Air | Water | 600 | 101 | 900 | --- | 116 | This study |
| 600 | 101 | 900 | --- | 140b | |||||
| 600 | 240 | 900 | --- | 58 | |||||
| 600 | 240 | 900 | --- | 83b | |||||
| 0 | 240 | 150 | --- | 1 | |||||
| 0 | 240 | 900 | --- | 119 | |||||
| 7.0 (5.6) | 600 | 101 | 900 | --- | 79 | ||||
| 600 | 240 | 900 | --- | 51 | |||||
| CSTR | 14 (7.0) | CO | Water | 6000 | NA | 600 | --- | 155 | [45] |
| CSTR | 14 (7.0) | CO | Water | 5000 | NA | 400 | --- | 100 | [46] |
| 15,000 | NA | 400 | --- | 153 | |||||
| CSTR | 3 (2.5) | Air | Water | 400 | 101 | 900 | --- | 114 | [15] |
| TBR | 0.5 (0.2) | Air | Water | 106 | 101 | --- | 50 | 421 | |
| HFR | NA (0.025) | Air+21%O2 | Water | 2000 | 106 | --- | 400 | 1062 | |
| CSTR | 3.3 (1.5) | CO | Water | 375 | NA | 600 | --- | 88 | [18] |
| 1050 | NA | 600 | --- | 160 | |||||
| h-RPB | 3.3 (1.5) | CO | Water | 1500 | 115 | 100 | --- | 70 | |
| HFR | NA (3.0) | CO | Water | NA | 136 | --- | 1500 | 205 | [43] |
| NA | 308 | --- | 1500 | 947 | |||||
| HFR | 4.0 (2.0) | CO | Water | 2000 | 138 | --- | NA | 137 | [44] |
| HFR | 0.5 (0.4) | CO | Water | NA | 139c | --- | NA | 155 | [20] |
| NA | 170d | --- | NA | 92 | |||||
| NA | 195d | --- | NA | 136 | |||||
| Air-lift | NAa (3.0) | CO | Water | 5000 | 170 | --- | --- | 91 | [48] |
| Air-lift | NA (3.0) | CO | Water | 5000 | 170 | --- | 500 | 130 | [47] |
| H2 | Water | 5000 | 170 | --- | 500 | 97 | |||
| HFR cw CSTR | NA (2.5e) | CO:H2:CO2f | Water | 139 | NA | 90 | --- | 385g | [21] |
| HFR cw CSTR | NA (8.0h) | CO | Water | 5000 | 205 | 200 | 1000 | 1096 | [24] |
| MBR cw CSTR | NA (8.0 h) | CO | Water | 500 | NA | NA | 500 | 450 | [16] |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, K.; Phillips, J.R.; Sun, X.; Mohammad, S.; Huhnke, R.L.; Atiyeh, H.K. Investigation and Modeling of Gas-Liquid Mass Transfer in a Sparged and Non-Sparged Continuous Stirred Tank Reactor with Potential Application in Syngas Fermentation. Fermentation 2019, 5, 75. https://doi.org/10.3390/fermentation5030075
Liu K, Phillips JR, Sun X, Mohammad S, Huhnke RL, Atiyeh HK. Investigation and Modeling of Gas-Liquid Mass Transfer in a Sparged and Non-Sparged Continuous Stirred Tank Reactor with Potential Application in Syngas Fermentation. Fermentation. 2019; 5(3):75. https://doi.org/10.3390/fermentation5030075
Chicago/Turabian StyleLiu, Kan, John R. Phillips, Xiao Sun, Sayeed Mohammad, Raymond L. Huhnke, and Hasan K. Atiyeh. 2019. "Investigation and Modeling of Gas-Liquid Mass Transfer in a Sparged and Non-Sparged Continuous Stirred Tank Reactor with Potential Application in Syngas Fermentation" Fermentation 5, no. 3: 75. https://doi.org/10.3390/fermentation5030075
APA StyleLiu, K., Phillips, J. R., Sun, X., Mohammad, S., Huhnke, R. L., & Atiyeh, H. K. (2019). Investigation and Modeling of Gas-Liquid Mass Transfer in a Sparged and Non-Sparged Continuous Stirred Tank Reactor with Potential Application in Syngas Fermentation. Fermentation, 5(3), 75. https://doi.org/10.3390/fermentation5030075






