Transient Shallow Water Wave Interactions with a Partially Fragmented Ice Shelf
Abstract
1. Introduction
2. Mathematical Model
2.1. Preliminaries
2.2. Solution for a Finite Ice Shelf Fragment
2.3. Solution for Semi-Infinite Ice Shelf
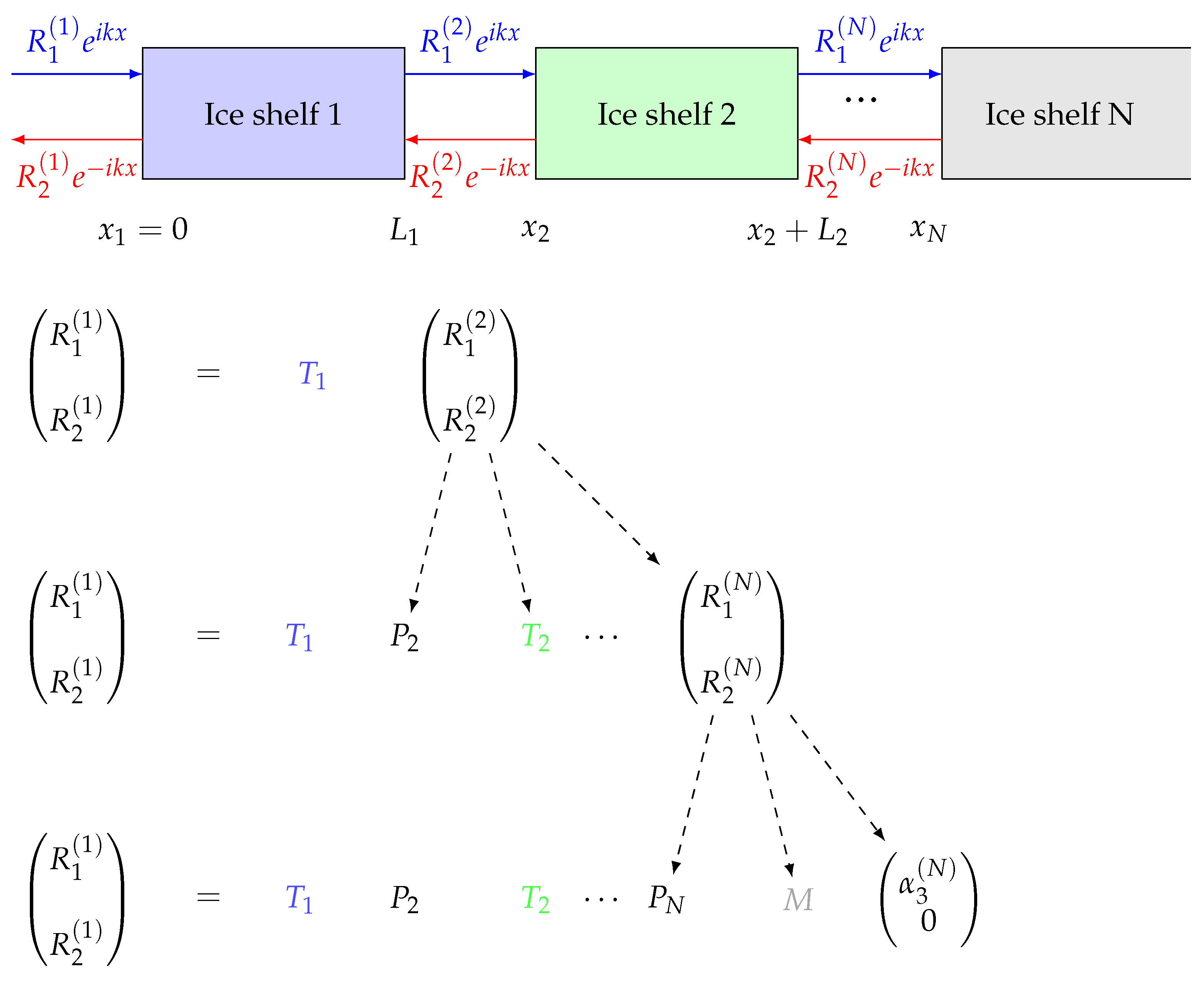
2.4. Multiple Scattering Solution
2.5. Energy Conservation
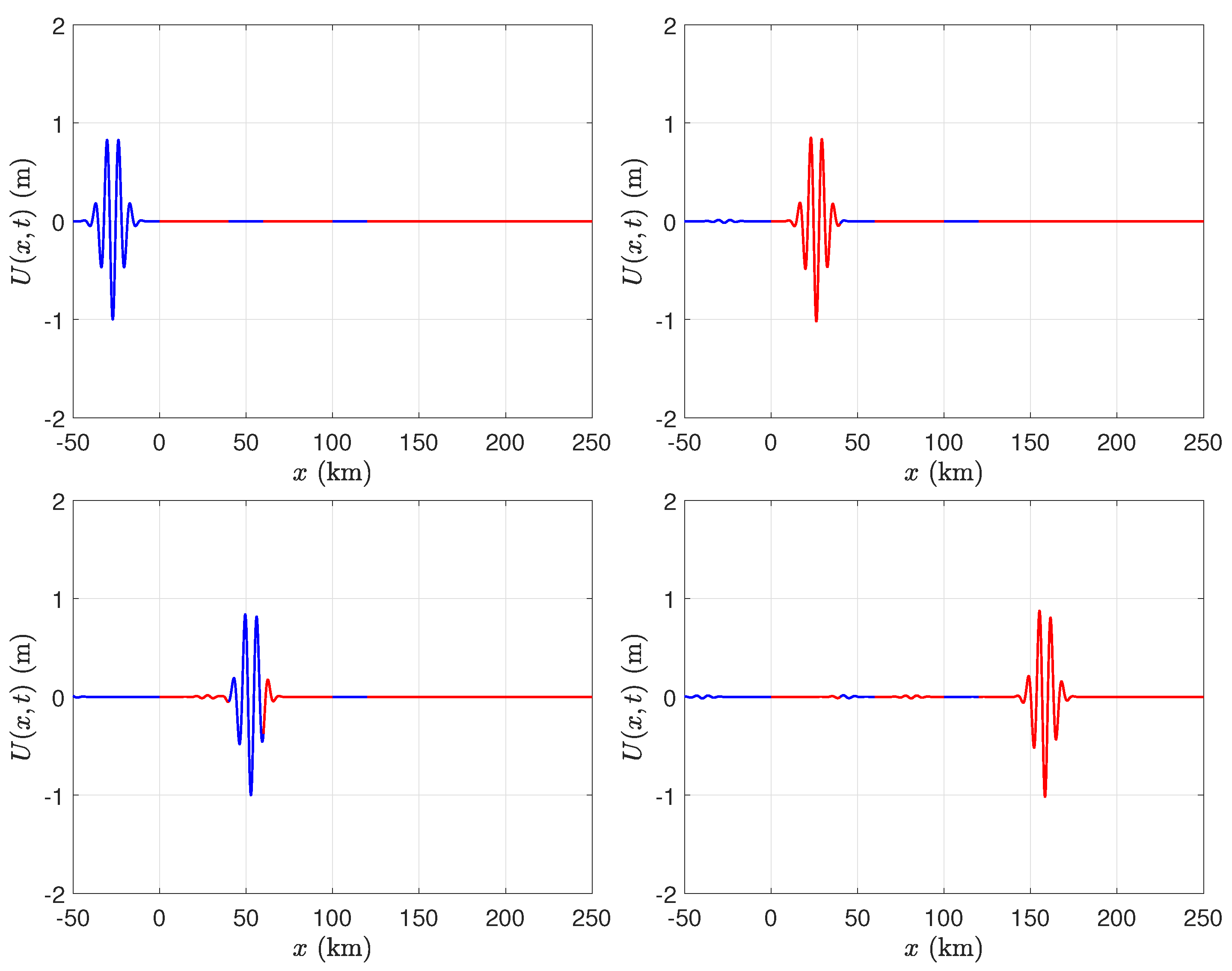
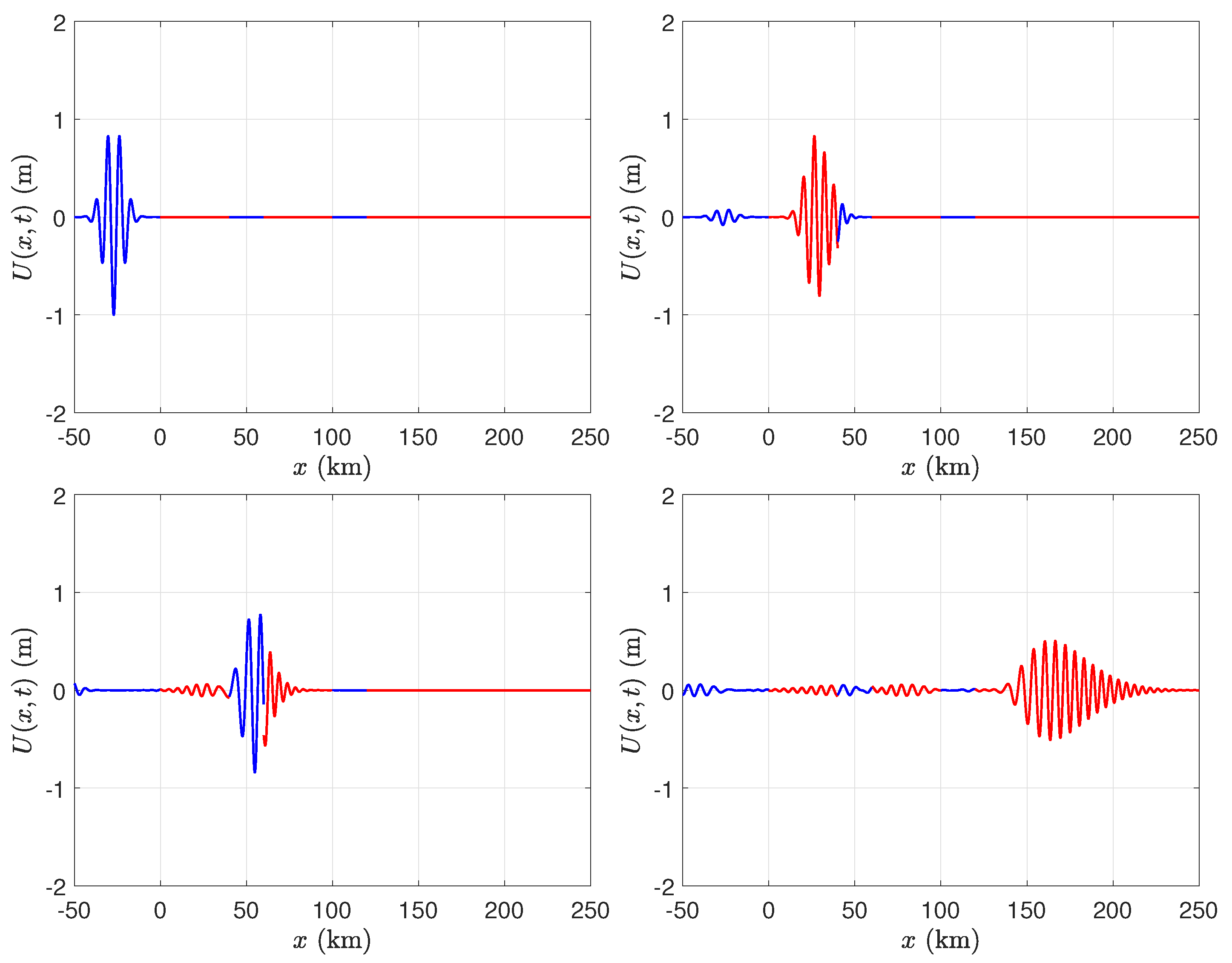
3. Time Domain Solution
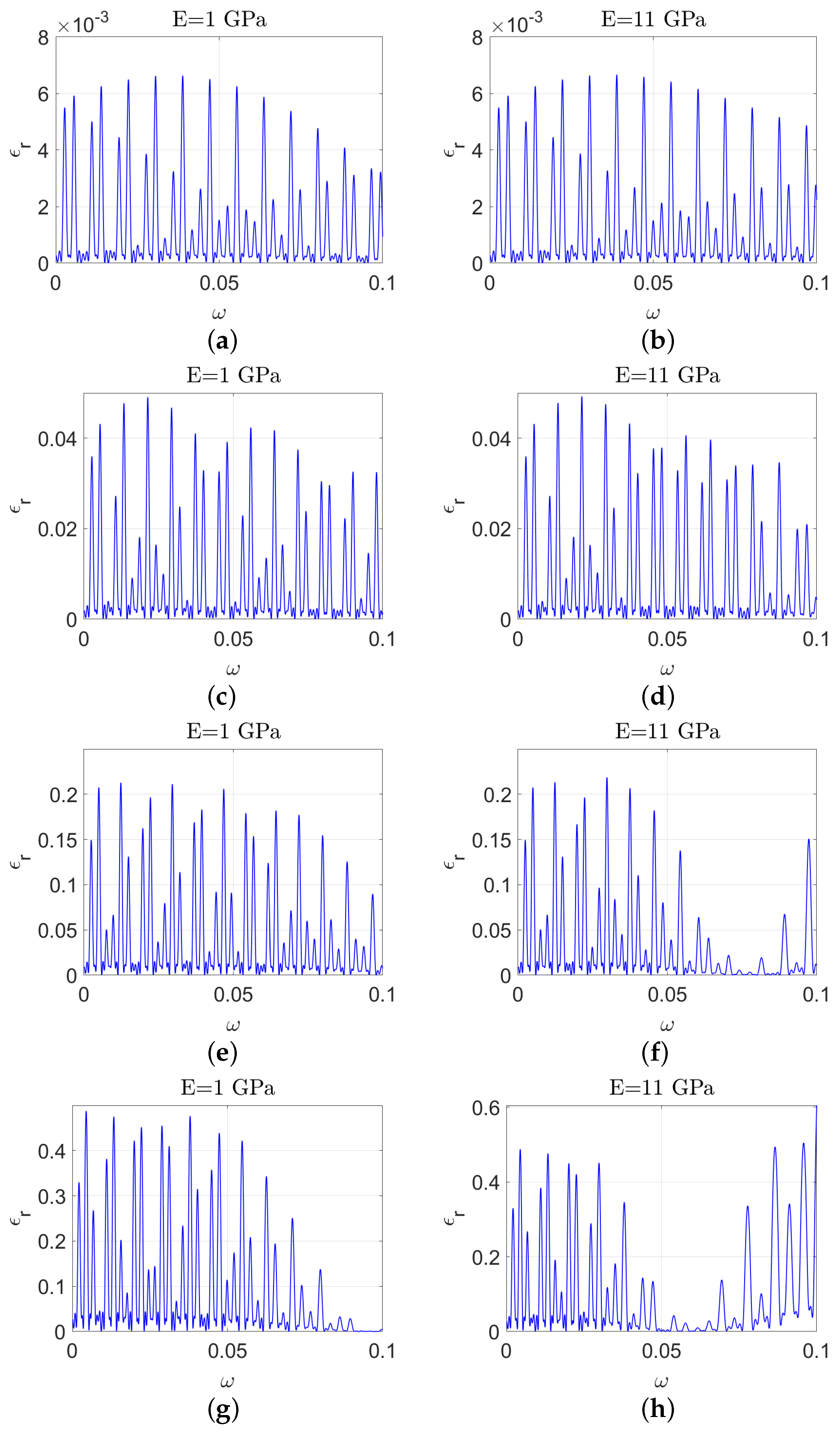
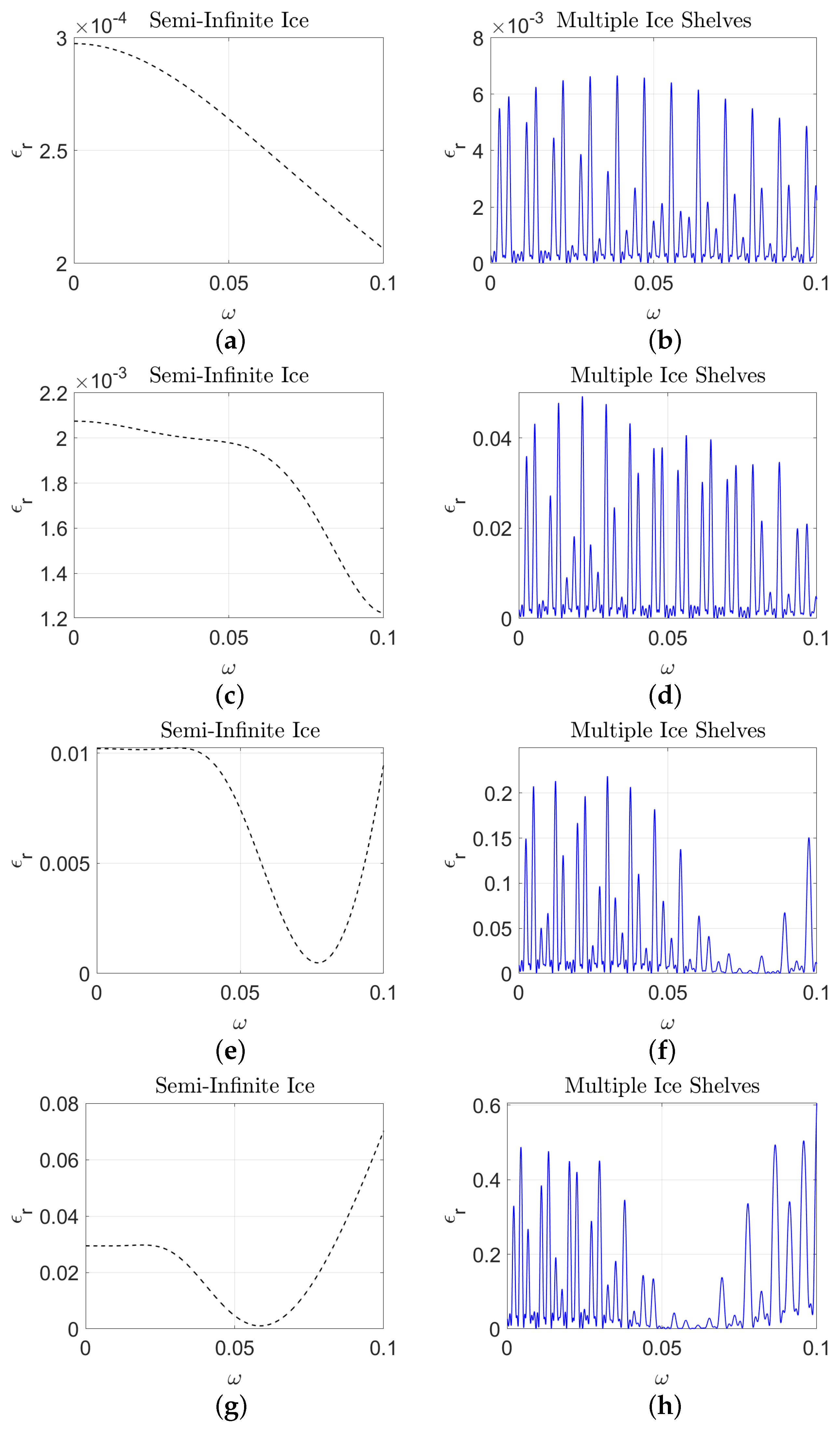
4. Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Energy Balance Calculation
References
- Frederikse, T.; Landerer, F.; Caron, L.; Adhikari, S.; Parkes, D.; Humphrey, V.W.; Dangendorf, S.; Hogarth, P.; Zanna, L.; Cheng, L.; et al. The causes of sea-level rise since 1900. Nature 2020, 584, 393–397. [Google Scholar] [CrossRef]
- Thomas, R.H. Ice shelves: A review. J. Glaciol. 1979, 24, 273–286. [Google Scholar] [CrossRef]
- Scambos, T.; Bohlander, J.; Shuman, C.; Skvarca, P. Glacier acceleration and thinning after ice shelf collapse in the Larsen B embayment, Antarctica. Geophys. Res. Lett. 2004, 31, 3–7. [Google Scholar] [CrossRef]
- Holdsworth, G.; Glynn, J.E. Iceberg calving from floating glaciers by a vibrating mechanism. J. Geophys. Res. 1978, 274, 464–466. [Google Scholar] [CrossRef]
- Holdsworth, G.; Glynn, J.E. A Mechanismfor the Formation of Large Iceberg. J. Geophys. Res. 1981, 86, 3210–3222. [Google Scholar] [CrossRef]
- Brunt, K.M.; Okal, E.A.; Macayeal, D.R. Antarctic ice-shelf calving triggered by the Honshu (Japan) earthquake and tsunami, March 2011. J. Glaciol. 2011, 57, 785–788. [Google Scholar] [CrossRef]
- Cathles Iv, L.M.; Okal, E.A.; Macayeal, D.R. Seismic observations of sea swell on the floating Ross Ice Shelf, Antarctica. J. Geophys. Res. 2009, 114, 1–11. [Google Scholar] [CrossRef]
- Bromirski, P.D.; Sergienko, O.V.; MacAyeal, D.R. Transoceanic infragravity waves impacting antarctic ice shelves. Geophys. Res. Lett. 2010, 37, 1–6. [Google Scholar] [CrossRef]
- Brunt, K.M.; King, M.A.; Fricker, H.A.; Macayeal, D.R. Flow of the Ross ice Shelf, Antarctica, is modulated by the ocean tide. J. Glaciol. 2010, 56, 157–161. [Google Scholar] [CrossRef]
- MacAyeal, D.R.; Okal, E.A.; Aster, R.C.; Bassis, J.N.; Brunt, K.M.; Cathles, L.M.; Drucker, R.; Flicker, H.A.; Kim, Y.J.; Martin, S.; et al. Transoceanic wave propagation links iceberg calving margins of Antarctica with storms in tropics and Northern Hemisphere. Geophys. Res. Lett. 2006, 33, 1–4. [Google Scholar] [CrossRef]
- Bromirski, P.D.; Stephen, R.A. Response of the Ross Ice Shelf, Antarctica, to ocean gravity-wave forcing. Ann. Glaciol. 2012, 53, 163–172. [Google Scholar] [CrossRef]
- Bromirski, P.D.; Diez, A.; Gerstoft, P.; Stephen, R.A.; Bolmer, T.; Wiens, D.A.; Aster, R.C.; Nyblade, A. Ross ice shelf vibrations. Geophys. Res. Lett. 2015, 42, 7589–7597. [Google Scholar] [CrossRef]
- DeConto, R.M.; Pollard, D. Contribution of Antarctica to past and future sea-level rise. Nature 2016, 531, 591–597. [Google Scholar] [CrossRef] [PubMed]
- Massom, R.A.; Scambos, T.A.; Bennetts, L.G.; Reid, P.; Squire, V.A.; Stammerjohn, S.E. Antarctic ice shelf disintegration triggered by sea ice loss and ocean swell. Nature 2018, 558, 383–389. [Google Scholar] [CrossRef]
- Chen, Z.; Bromirski, P.D.; Gerstoft, P.; Stephen, R.A.; Wiens, D.A.; Aster, R.C.; Nyblade, A.A. Ocean-excited plate waves in the Ross and Pine Island Glacier ice shelves. J. Glaciol. 2018, 64, 730–744. [Google Scholar] [CrossRef]
- Chen, Z.; Bromirski, P.D.; Gerstoft, P.; Stephen, R.A.; Lee, W.S.; Yun, S.; Olinger, S.D.; Aster, R.C.; Wiens, D.A.; Nyblade, A.A. Ross Ice Shelf Icequakes Associated with Ocean Gravity Wave Activity. Geophys. Res. Lett. 2019, 46, 8893–8902. [Google Scholar] [CrossRef]
- Vaughan, D.G. Tidal flexure at ice shelf margins. J. Geophys. Res. 1995, 100, 6213–6224. [Google Scholar] [CrossRef]
- Schmeltz, M.; Rignot, E.; MacAyeal, D. Tidal flexure along ice-sheet margins: Comparison of InSAR with an elastic-plate model. Ann. Glaciol. 2002, 34, 202–208. [Google Scholar] [CrossRef]
- Sergienko, O.V. Normal modes of a coupled ice-shelf/sub-ice-shelf cavity system. J. Glaciol. 2013, 59, 76–80. [Google Scholar] [CrossRef]
- Bennetts, L.G.; Meylan, M.H. Complex resonant ice shelf vibrations. Soc. Ind. Appl. Math. 2021, 81, 1483–1502. [Google Scholar] [CrossRef]
- McNeil, S.; Meylan, M.H. Time-Dependent Modelling of the Wave-Induced Vibration of Ice Shelves. J. Mar. Sci. Eng. 2023, 11, 1191. [Google Scholar] [CrossRef]
- Aljabri, R.; Meylan, M.H. Time Domain Vibration Analysis of an Ice Shelf. J. Mar. Sci. Eng. 2024, 12, 468. [Google Scholar] [CrossRef]
- Kalyanaraman, B.; Bennetts, L.G.; Lamichhane, B.; Meylan, H. On the shallow-water limit for modelling ocean-wave induced ice-shelf vibrations. Wave Motion 2019, 90, 1–16. [Google Scholar] [CrossRef]
- England, M.R.; Wagner, T.J.W.; Eisenman, I. Modeling the breakup of tabular icebergs. Sci. Adv. 2020, 6, eabd1273. [Google Scholar] [CrossRef] [PubMed]
- Mokus, N.G.A.; Montiel, F. Wave-triggered breakup in the marginal ice zone generates lognormal floe size distributions: A simulation study. Cryosphere 2022, 16, 4447–4472. [Google Scholar] [CrossRef]
- Meylan, M.H. Spectral solution of time-dependent shallow water hydroelasticity. J. Fluid Mech. 2002, 454, 387–402. [Google Scholar] [CrossRef]
- Stoker, J.J. Water Waves: The Mathematical Theory with Applications; New York Interscience Publishers: New York, NY, UDA, 1957. [Google Scholar] [CrossRef][Green Version]
- Chen, J. Nonlocal Euler—Bernoulli Beam Theories; Springer: Cham, Switzerland, 2021. [Google Scholar] [CrossRef]
- Bronson, R.; Costa, G.B. Matrix Methods: Applied Linear Algebra, 3rd ed.; Academic Press: Cambridge, MA, USA, 2009. [Google Scholar] [CrossRef]
- Martin, P.A. N masses on an infinite string and related one-dimensional scattering problems. Wave Motion 2014, 51, 296–307. [Google Scholar] [CrossRef]
- Papathanasiou, T.K.; Karperaki, A.E.; Belibassakis, K.A. On the resonant hydroelastic behaviour of ice shelves. Ocean Model. 2019, 133, 11–26. [Google Scholar] [CrossRef]
- Wilks, B.; Meylan, M.H.; Montiel, F.; Wakes, S. Generalised eigenfunction expansion and singularity expansion methods for canonical time-domain wave scattering problems. arXiv 2023. [Google Scholar] [CrossRef]
- Wilks, B.; Meylan, M.H.; Montiel, F.; Wakes, S. Generalised eigenfunction expansion and singularity expansion methods for two-dimensional acoustic time-domain wave scattering problems. arXiv 2023. [Google Scholar] [CrossRef]







Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alshahrani, F.; Meylan, M.H.; Wilks, B. Transient Shallow Water Wave Interactions with a Partially Fragmented Ice Shelf. Fluids 2024, 9, 192. https://doi.org/10.3390/fluids9080192
Alshahrani F, Meylan MH, Wilks B. Transient Shallow Water Wave Interactions with a Partially Fragmented Ice Shelf. Fluids. 2024; 9(8):192. https://doi.org/10.3390/fluids9080192
Chicago/Turabian StyleAlshahrani, Faraj, Michael H. Meylan, and Ben Wilks. 2024. "Transient Shallow Water Wave Interactions with a Partially Fragmented Ice Shelf" Fluids 9, no. 8: 192. https://doi.org/10.3390/fluids9080192
APA StyleAlshahrani, F., Meylan, M. H., & Wilks, B. (2024). Transient Shallow Water Wave Interactions with a Partially Fragmented Ice Shelf. Fluids, 9(8), 192. https://doi.org/10.3390/fluids9080192







