Oscillation of a Liquid Column in an Eccentric Annulus
Abstract
1. Introduction
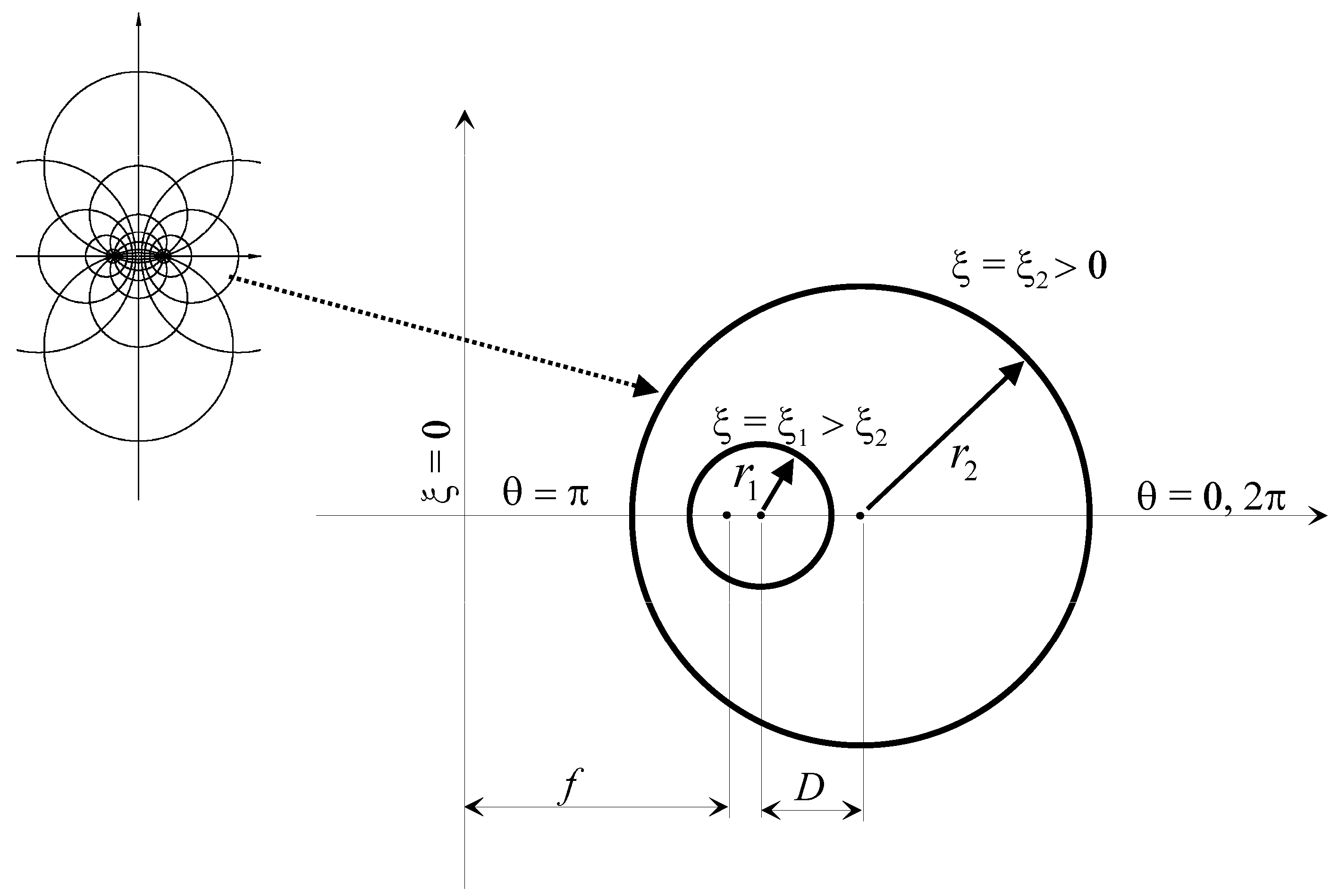
2. Velocity Profile
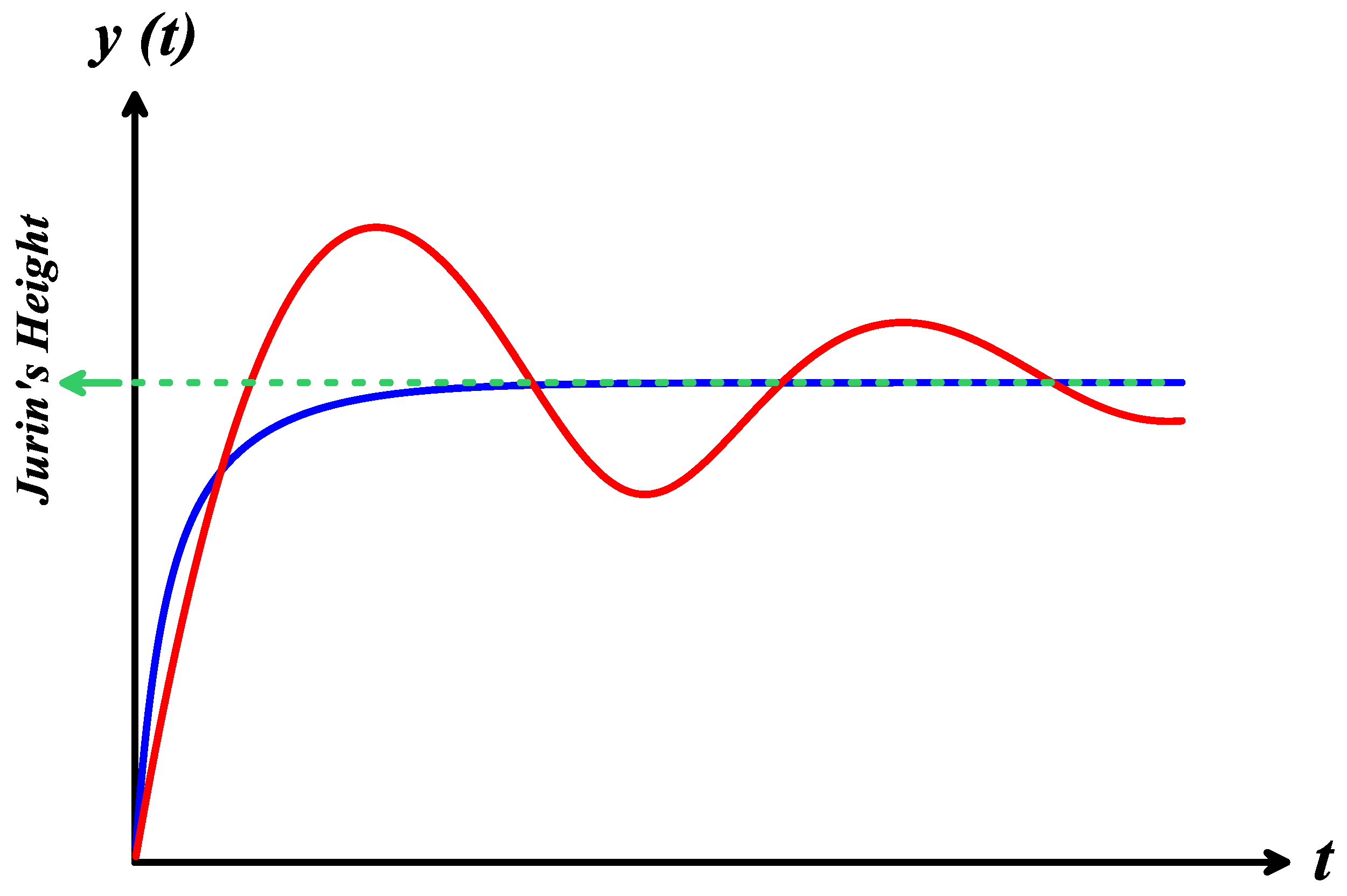
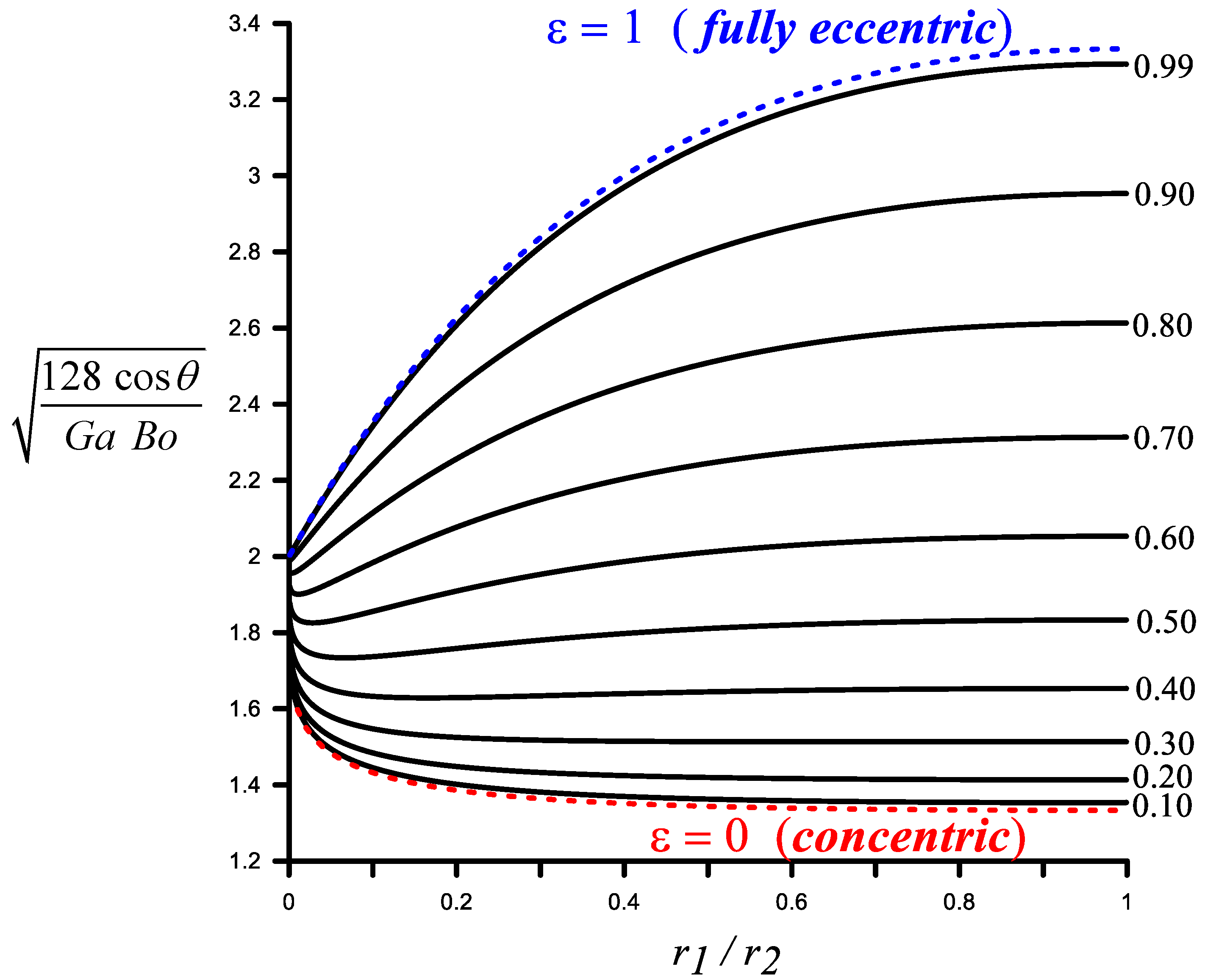
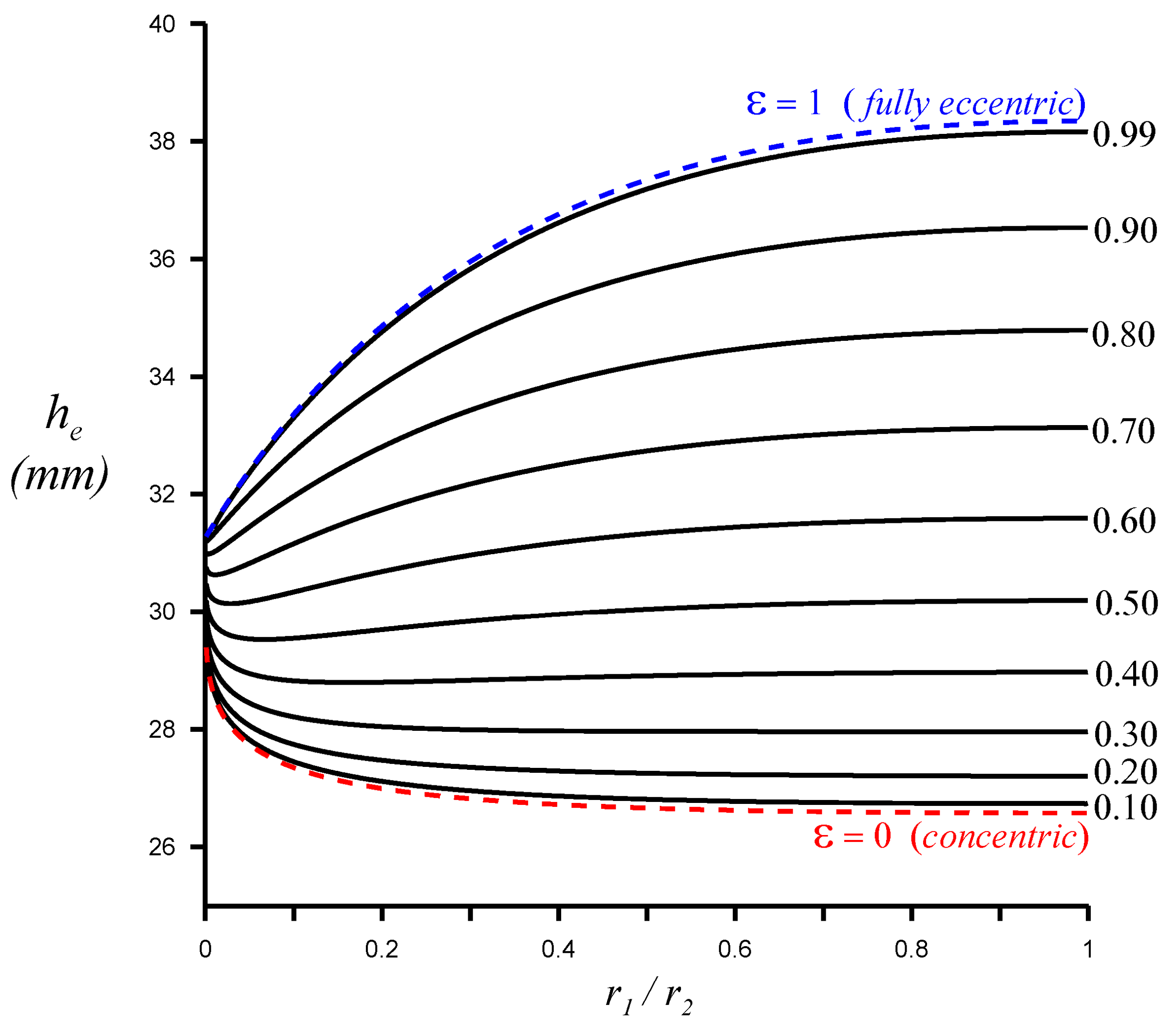
3. Capillary Rise
4. Conclusions
Supplementary Materials
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| () | Radius of the tube |
| Pressure | |
| () | Axial velocity |
| () | Axial direction |
| () | Viscosity |
| Bipolar coordinate | |
| () | Focal length |
| () | Center-to-center distance |
| Scale factors | |
| () | Average velocity |
| Dimensionless average velocity | |
| Eccentricity | |
| () | Equilibrium height |
| Density | |
| () | Perimeter |
| Cross-sectional area | |
| Height of the liquid column | |
| Surface tension | |
| Contact angle | |
| () | Column height |
| Gravitational acceleration | |
| Galileo number | |
| Bond number | |
| () | Hydraulic radius |
| Dimensionless height | |
| Dimensionless equilibrium height |
References
- De Gennes, P.G.; Brochard-Wyart, F.; Quéré, D. Capillarity and Wetting Phenomena: Drops, Bubbles, Pearls, Waves; Springer: New York, NY, USA, 2003. [Google Scholar]
- Quéré, D. Capillarity and Wetting Phenomena: Drops, Bubbles, Pearls, Waves. Annu. Rev. Fluid Mech. 1997, 31, 347–384. [Google Scholar] [CrossRef]
- Lago, M.; Araujo, M. Capillary rise in porous media. J. Colloid Interface Sci. 2001, 234, 35–43. [Google Scholar] [CrossRef] [PubMed]
- Delgado, J.M.P.Q.; Gama, C.; Silva, C.L. The Role of Capillarity in Porous Media. In Transport Processes in Porous Media; Springer: Berlin/Heidelberg, Germany, 2017; pp. 141–173. [Google Scholar]
- Green, W.H.; Ampt, G.A. Studies in Soil Physics, Part 1, the Flow of Air and Water through Soils. J. Agric. Sci. 1911, 4, 11–24. [Google Scholar]
- Lucas, R.; Kollid, Z. Ueber das Zeitgesetz des kapillaren Aufstiegs von Flüssigkeiten. Kolloid-Zeitschrift 1918, 23, 15–22. [Google Scholar] [CrossRef]
- Washburn, E.V. The Dynamics of Capillary Flow. Phys. Rev. 1921, 17, 273–283. [Google Scholar] [CrossRef]
- Masoodi, R.; Languri, E.; Ostadhossein, A. Dynamics of liquid rise in a vertical capillary tube. J. Colloid Interface Sci. 2013, 389, 268–272. [Google Scholar] [CrossRef] [PubMed]
- Szekely, J.; Neumann, A.W.; Chuang, Y.K. The rate of capillary penetration and the applicability of the Washburn equation. J. Colloid Interface Sci. 1971, 35, 273–278. [Google Scholar] [CrossRef]
- Fries, N. Capillary Transport Processes in Porous Materials—Experiment and Model; Cuvillier Verlag: Göttingen, Germany, 2010. [Google Scholar]
- Płociniczak, Ł.; Świtała, M. Monotonicity, oscillations and stability of a solution to a nonlinear equation modelling the capillary rise. Phys. D 2018, 362, 1–8. [Google Scholar] [CrossRef]
- Shah, N.A.; Ebaid, A.; Oreyeni, T.; Yook, S.-J. MHD and porous effects on free convection flow of viscous fluid between vertical parallel plates: Advance thermal analysis. Waves Random Complex Media 2023. [Google Scholar] [CrossRef]
- Zeeshan; Ahammad, N.A.; Shah, N.A.; Chung, J.D.; Attaullah; Rasheed, H.U. Analysis of Error and Stability of Nanofluid over Horizontal Channel with Heat/Mass Transfer and Nonlinear Thermal Conductivity. Mathematics 2023, 11, 690. [Google Scholar] [CrossRef]
- Souhar, K.; Kriraa, M.; Bammou, L.; Alami, S.; Bouchgl, J.; Feddaoui, M.; Aniss, S. Convecting Threshold in Nanofluid Driven by Centrifugal Forces in a Rotating Annular Hele-Shaw. Comput. Therm. Sci. Int. J. 2017, 9, 49–62. [Google Scholar] [CrossRef]
- Yagoubi, M.; Aniss, S. Effect of vertical quasi-periodic vibrations on the stability of the free surface of a fluid layer. Eur. Phys. J. Plus 2017, 132, 226. [Google Scholar] [CrossRef]
- Souhar, K.; Aniss, S. Effect of Coriolis force on the thermosolutal convection threshold in a rotating annular Hele-Shaw cell. Heat Mass Transf. 2012, 48, 175–182. [Google Scholar] [CrossRef]
- Moon, P.; Spencer, D.E. Field Theory Handbook, Including Coordinate Systems, Differential Equations and Their Solutions, 2nd ed.; Springer: New York, NY, USA, 1988; pp. 1–48. [Google Scholar]
- Alassar, R.S.; El-Gebily, M.A. Inviscid Flow Past two Cylinders. ASME J. Fluids Eng. 2009, 131, 054501. [Google Scholar] [CrossRef]
- Arfken, G. Mathematical Methods for Physicists; Academic Press: London, UK, 1970. [Google Scholar]
- Alassar, R.S. Slip Flow in Eccentric Annuli. ASME J. Fluids Eng. 2017, 139, 041201. [Google Scholar] [CrossRef]
- Fries, N.; Dreyer, M. Dimensionless scaling methods for capillary rise. J. Colloid Interface Sci. 2009, 338, 514–518. [Google Scholar] [CrossRef] [PubMed]
- MacDonald, D.A. Fully Developed Incompressible Flow between Non-Coaxial Circular Cylinders. J. Appl. Math. Phys. (ZAMP) 1982, 33, 738–751. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alassar, R.S. Oscillation of a Liquid Column in an Eccentric Annulus. Fluids 2024, 9, 189. https://doi.org/10.3390/fluids9080189
Alassar RS. Oscillation of a Liquid Column in an Eccentric Annulus. Fluids. 2024; 9(8):189. https://doi.org/10.3390/fluids9080189
Chicago/Turabian StyleAlassar, Rajai S. 2024. "Oscillation of a Liquid Column in an Eccentric Annulus" Fluids 9, no. 8: 189. https://doi.org/10.3390/fluids9080189
APA StyleAlassar, R. S. (2024). Oscillation of a Liquid Column in an Eccentric Annulus. Fluids, 9(8), 189. https://doi.org/10.3390/fluids9080189




