Vapor Bubble Deformation and Collapse near Free Surface
Abstract
1. Introduction
2. SPH Modeling
3. Validation
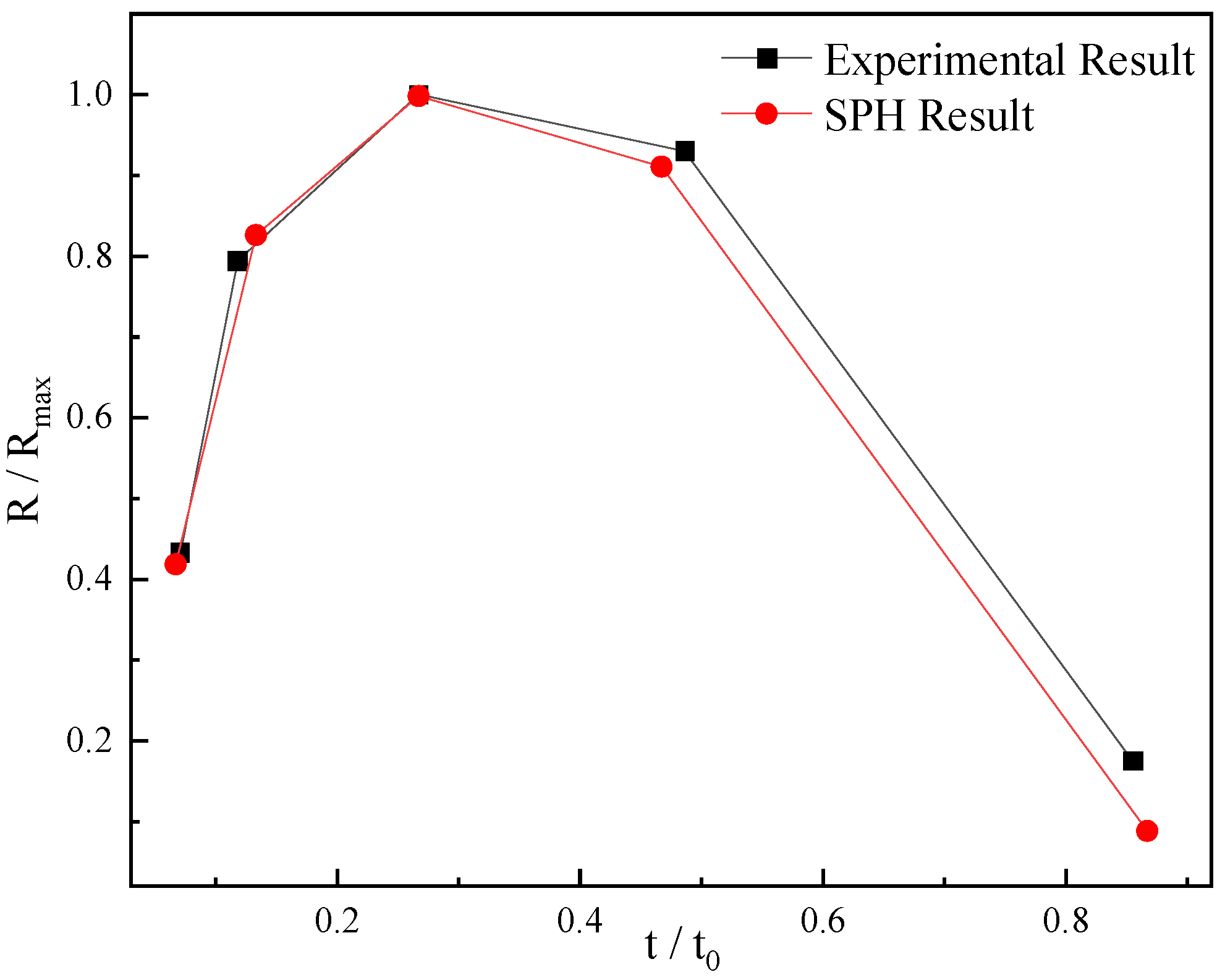
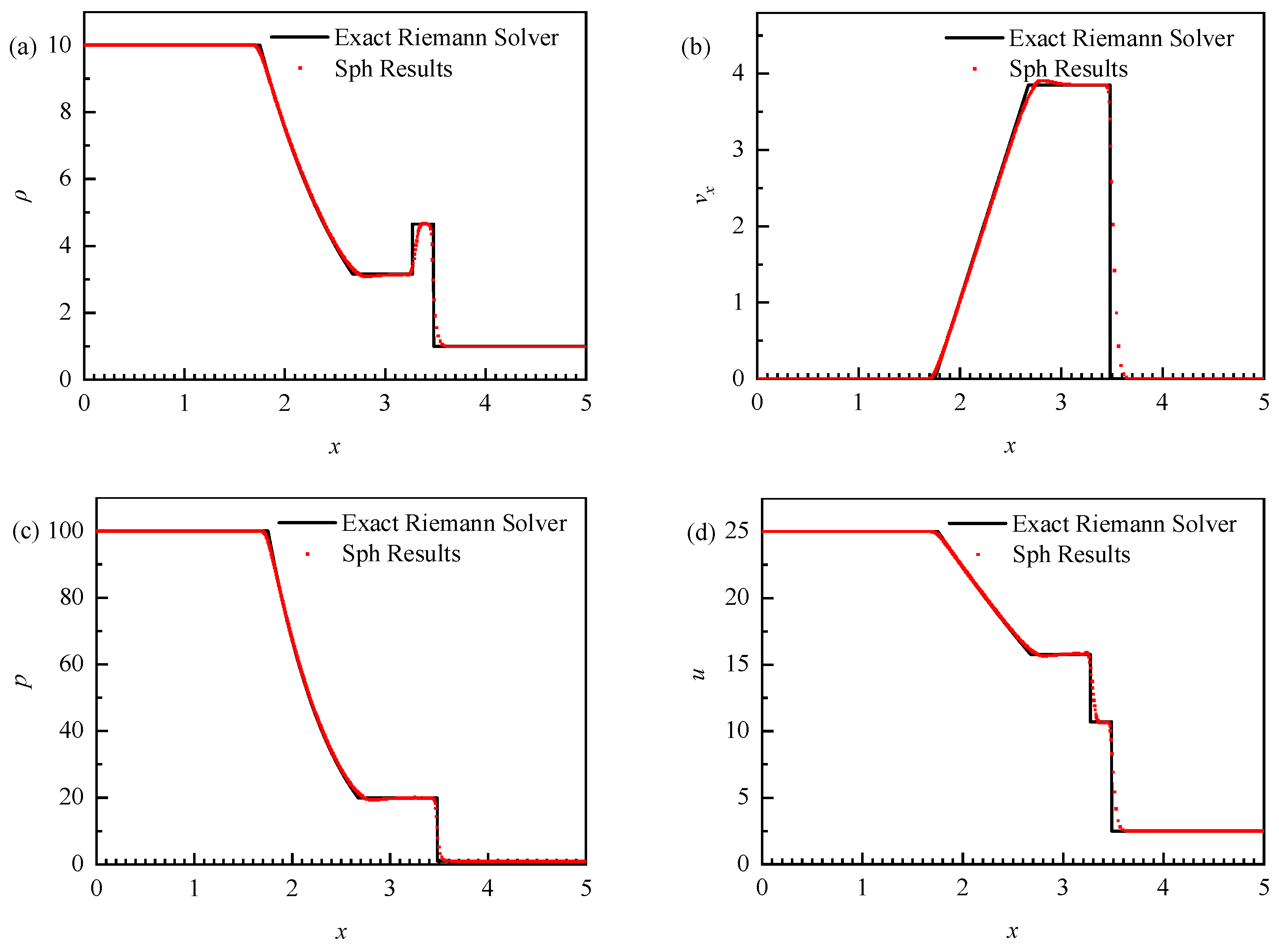
3.1. Comparison Verification
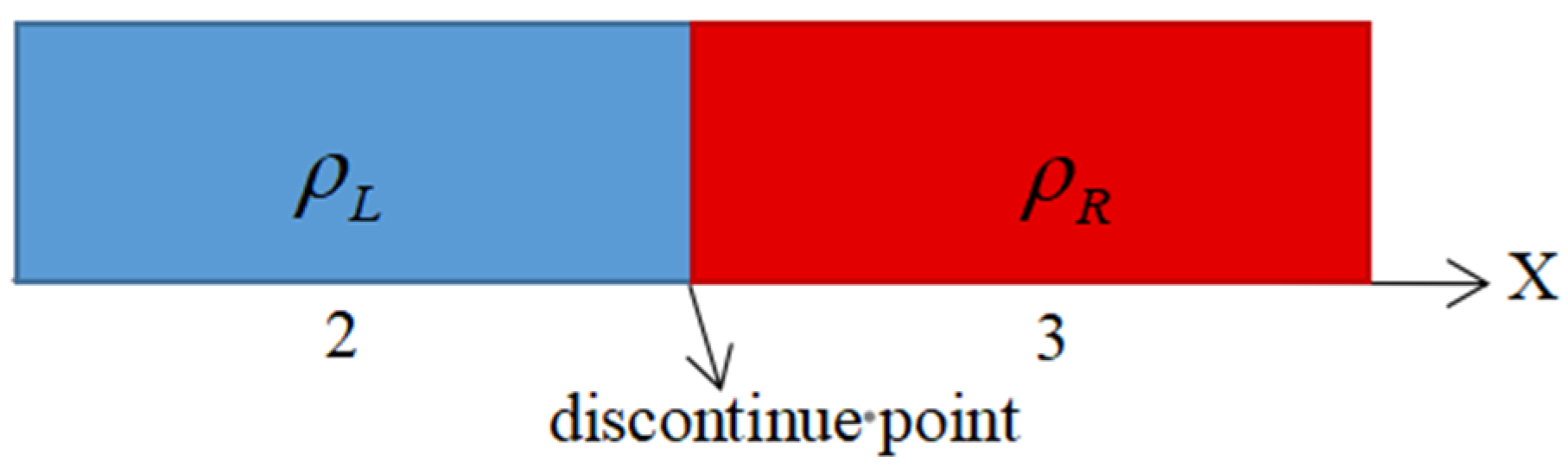
3.2. Shock Wave
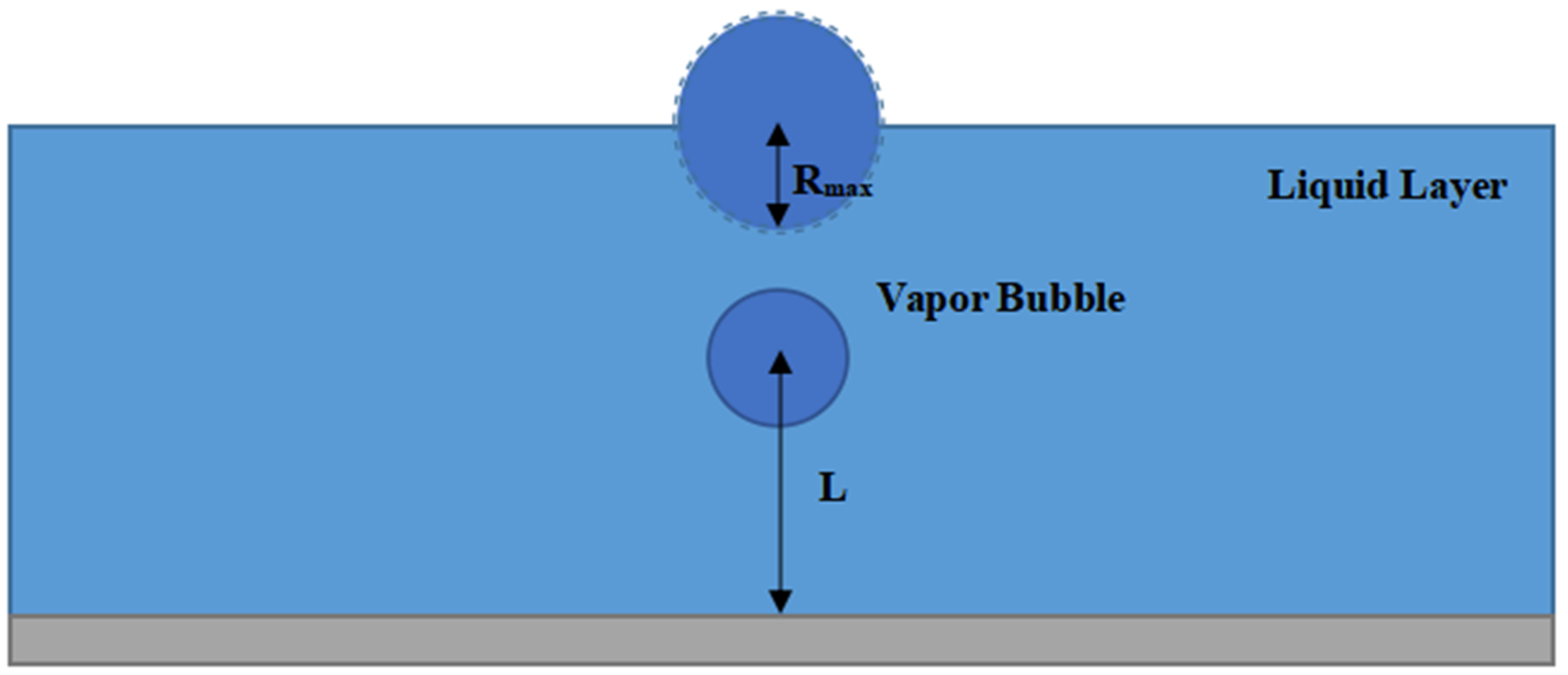
4. Numerical Settings
5. Results
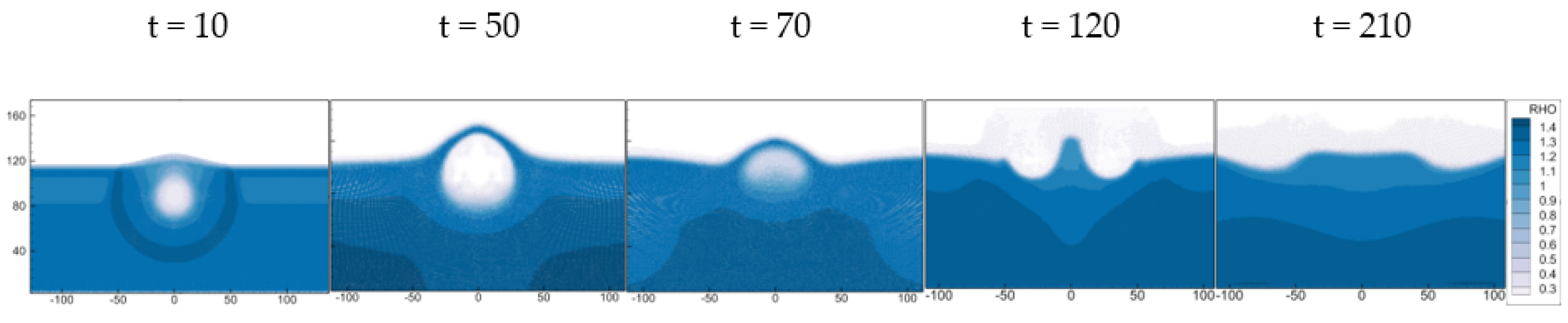
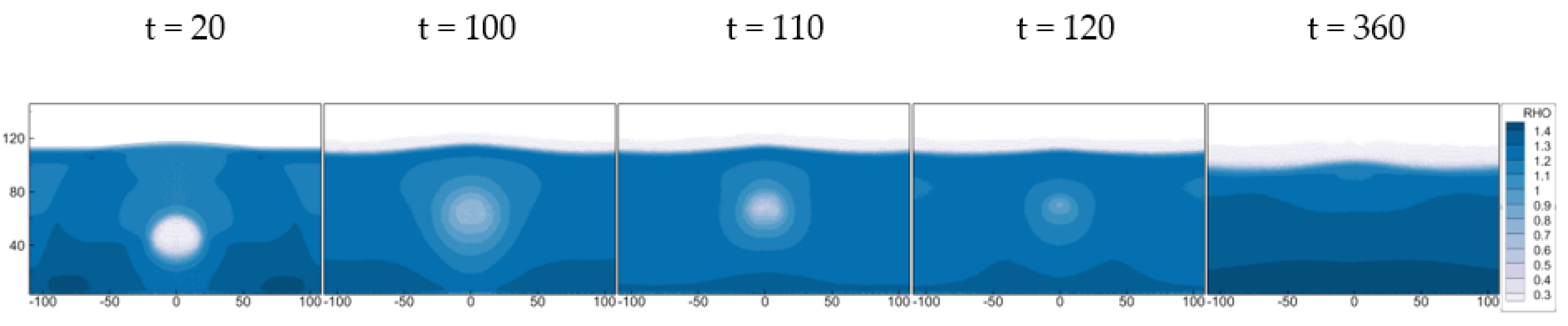
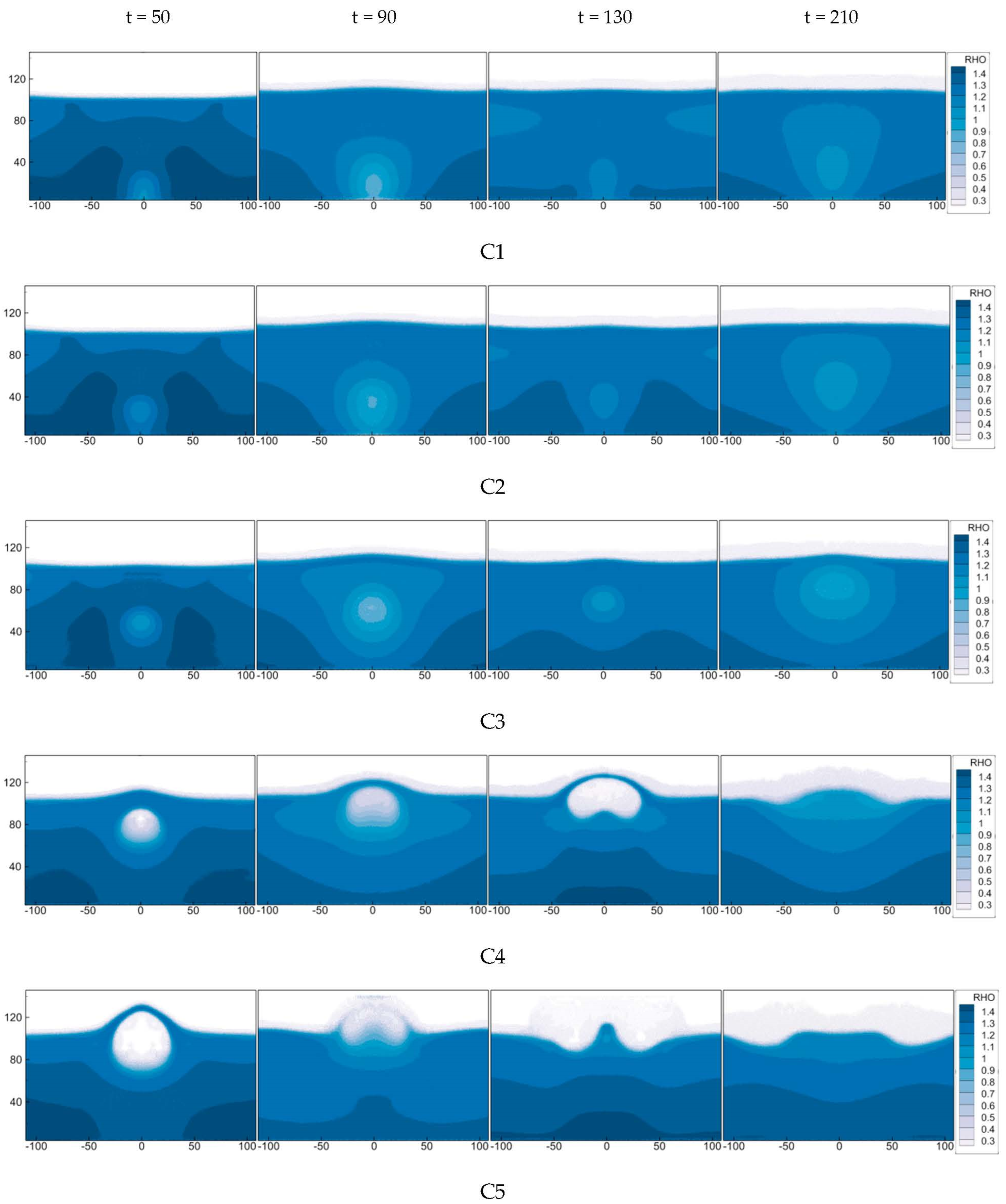
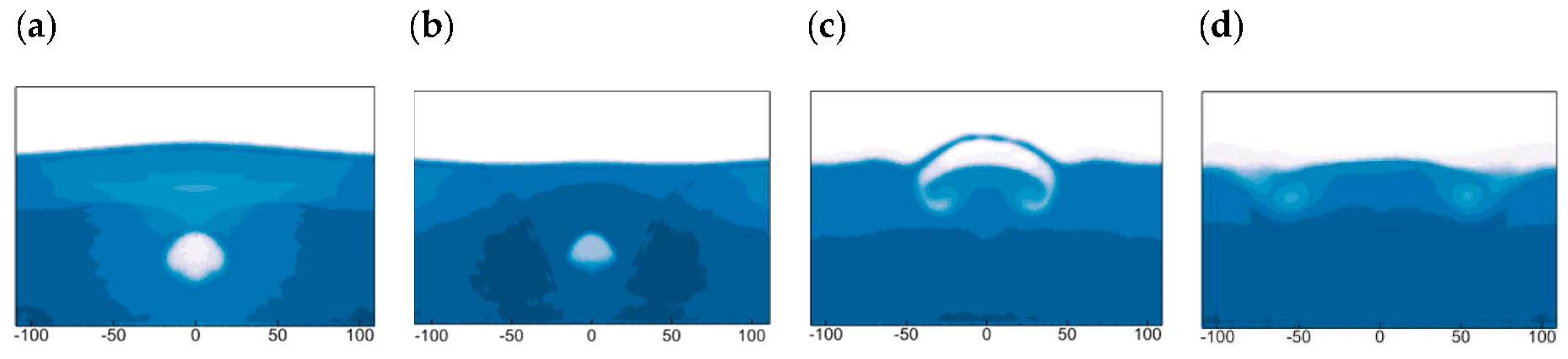
5.1. Category of Bubble Deformation
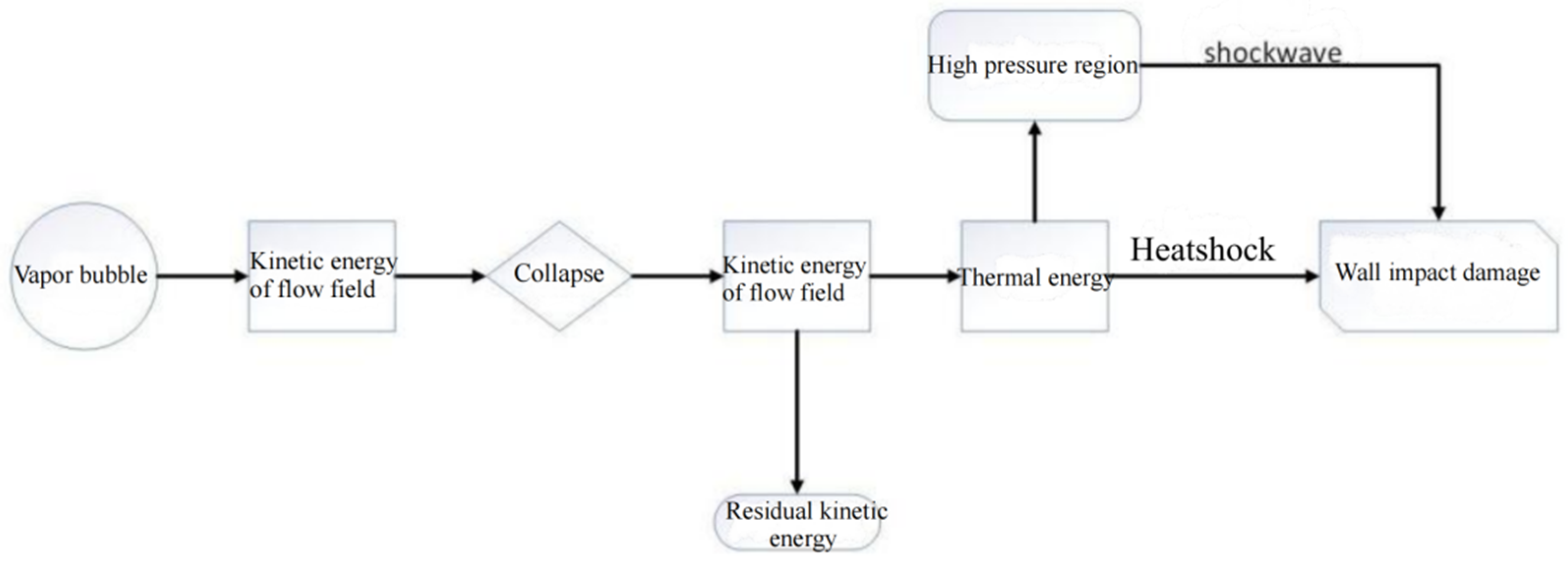
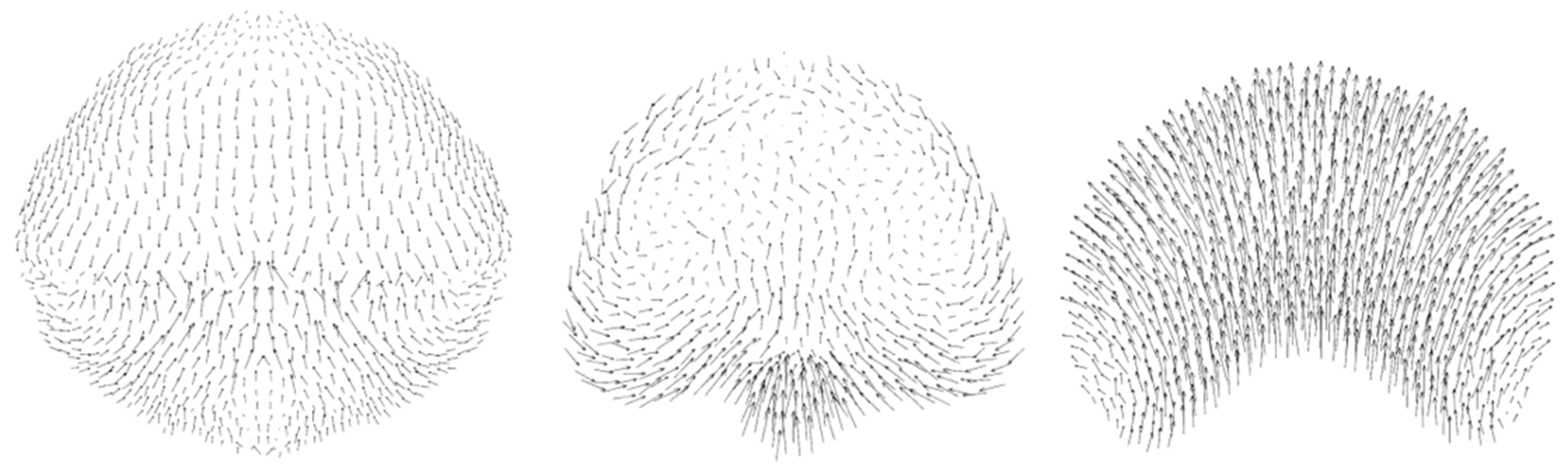
5.2. Mechanism Discussion
5.2.1. Jet Bubble
5.2.2. Spheroidal Bubble
5.2.3. Umbrella Bubble
5.2.4. Semi-Crescent Bubble
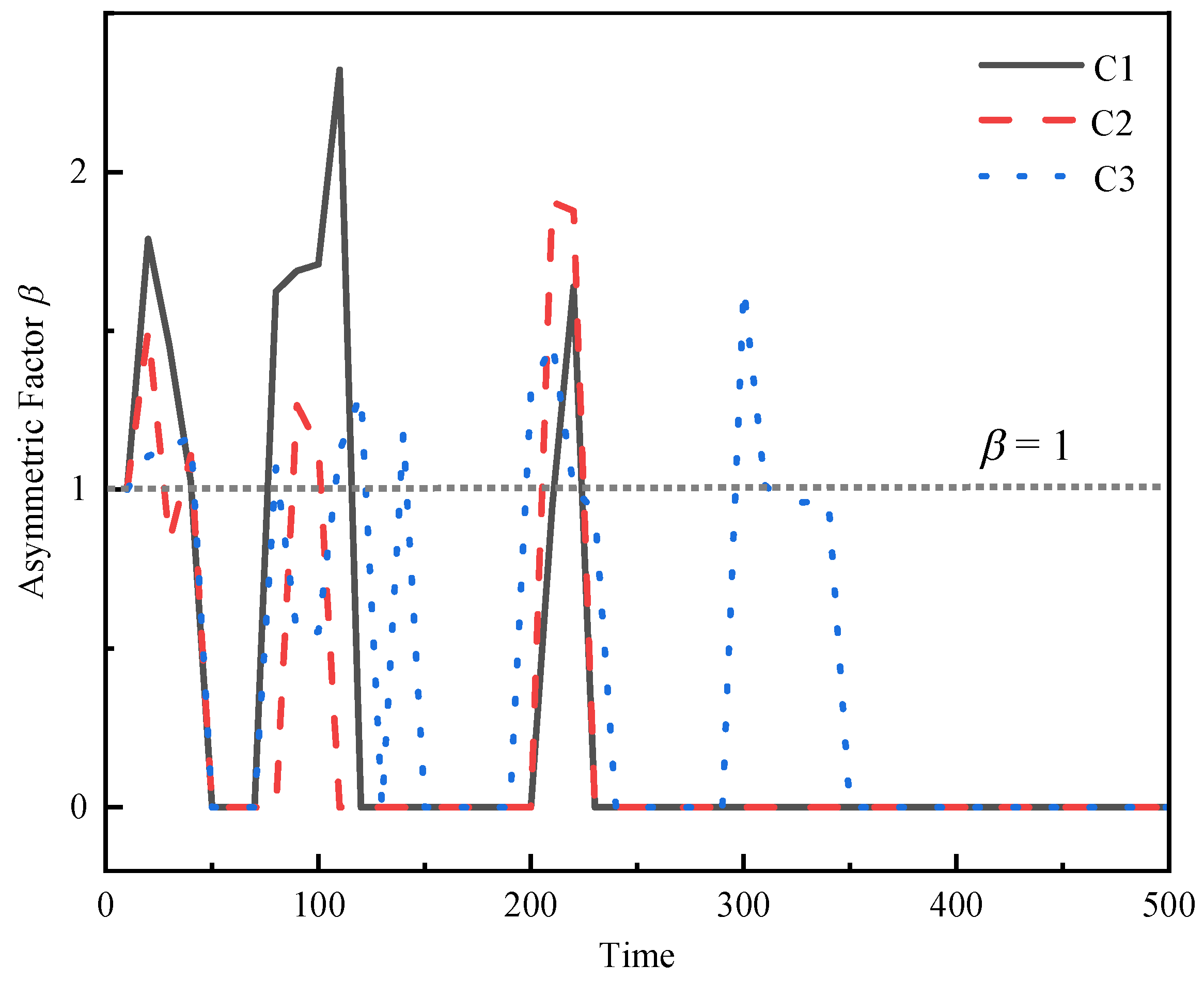
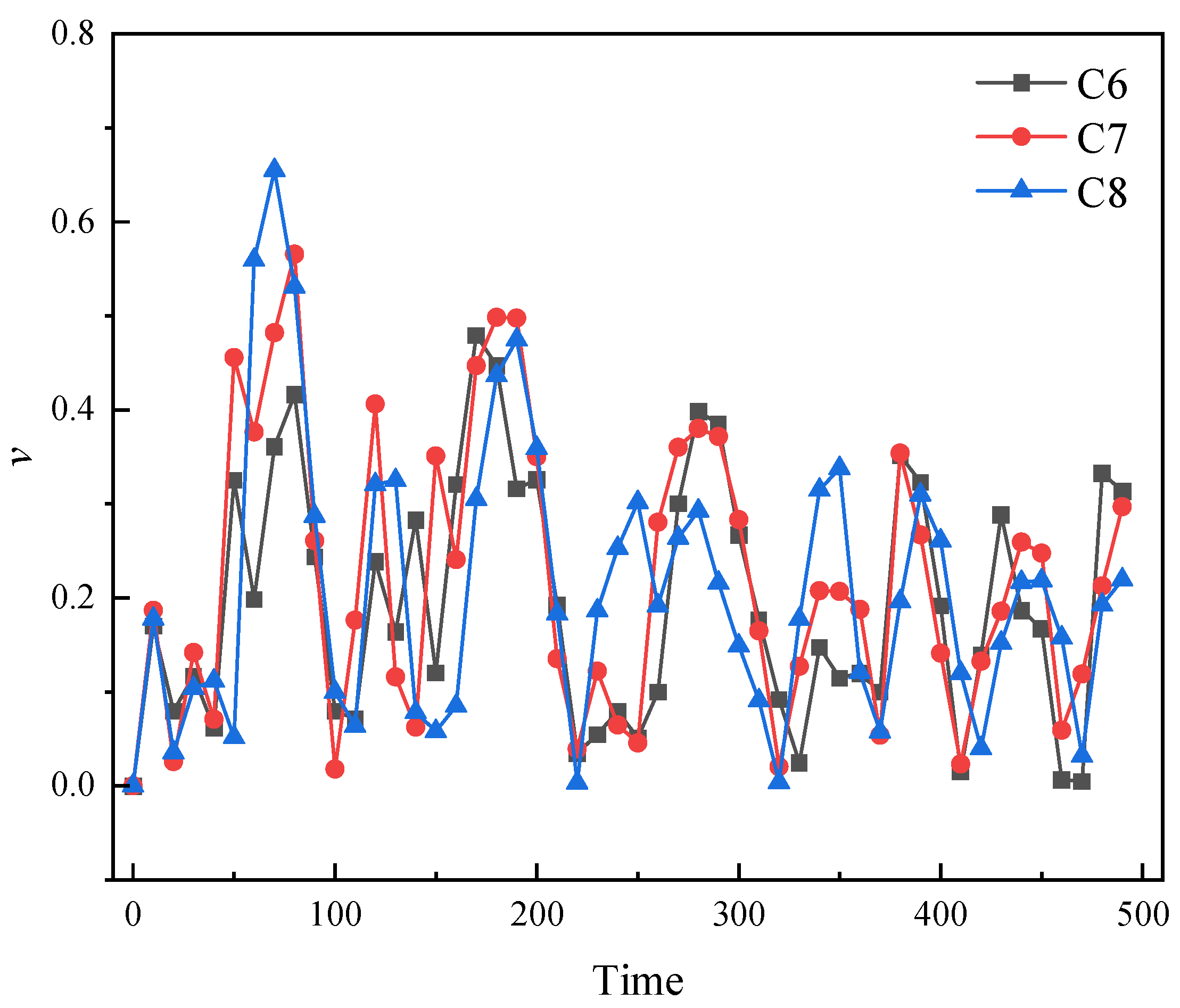
5.3. Bubble Deformation when Re < 10
5.3.1. Deformation of Near-Wall Bubbles
5.3.2. Deformation of Liquid-Surface Bubbles
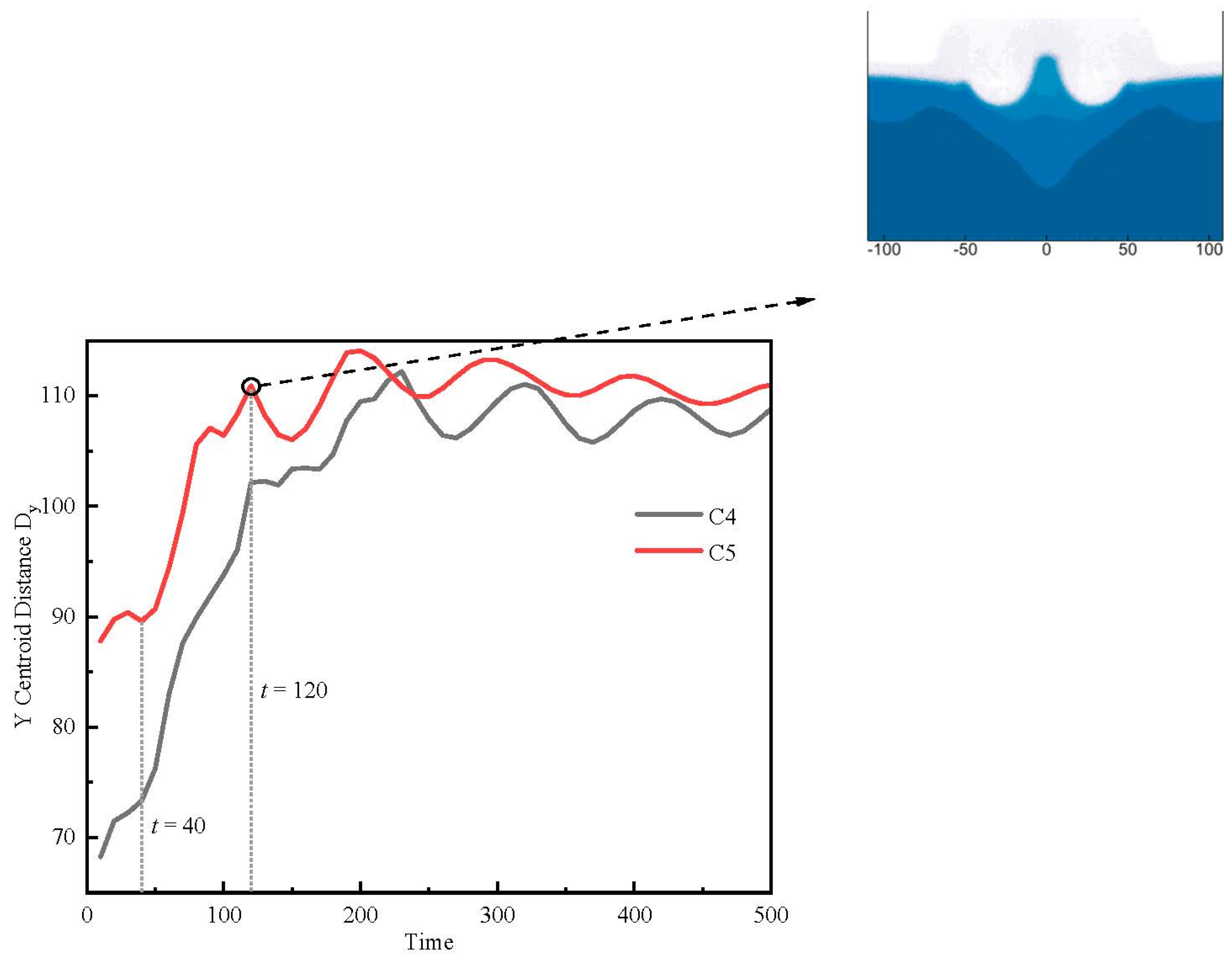
5.4. Bubble Deformation When Re ≥ 10
Deformation of Near-Wall Bubbles
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Krefting, D.; Mettin, R.; Lauterborn, W. High-speed observation of acoustic cavitation erosion in multibubble systems. Ultrason. Sonochem. 2004, 11, 119–123. [Google Scholar] [CrossRef] [PubMed]
- Shao, J.; Xuan, M.; Dai, L.; Si, T.; Li, J.; He, Q. Near-Infrared-Activated Nanocalorifiers in Microcapsules: Vapor Bubble Generation for In Vivo Enhanced Cancer Therapy. Angew. Chem. Int. Ed. 2015, 54, 12782–12787. [Google Scholar] [CrossRef] [PubMed]
- Oyarte Gálvez, L.; Fraters, A.; Offerhaus, H.L.; Versluis, M.; Hunter, I.W.; Rivas, D.F. Microfluidics control the ballistic energy of thermocavitation liquid jets for needle-free injections. J. Appl. Phys. 2020, 127, 104901. [Google Scholar] [CrossRef]
- Chudnovskii, V.M.; Yusupov, V.I.; Dydykin, A.V.; Nevozhai, V.; Kisilev, A.Y.; Zhukov, S.A.; Bagratashvili, V.N. Laser-induced boiling of biological liquids in medical technologies. Quantum Electron. 2017, 47, 361–370. [Google Scholar] [CrossRef]
- Rekhviashvili, S.S. Single-bubble sonoluminescence model. Tech. Phys. Lett. 2008, 34, 1072–1074. [Google Scholar] [CrossRef]
- Prosperetti, A. Vapor Bubbles. Annu. Rev. Fluid Mech. 2017, 49, 221–248. [Google Scholar] [CrossRef]
- Robinson, P.B.; Blake, J.R.; Kodama, T.; Shima, A.; Tomita, Y. Interaction of cavitation bubbles with a free surface. J. Appl. Phys. 2001, 89, 8225–8237. [Google Scholar] [CrossRef]
- Zhang, Y.; Qiu, X.; Zhang, X.; Tang, N. Collapsing dynamics of a laser-induced cavitation bubble near the edge of a rigid wall. Ultrason. Sonochemistry 2020, 67, 105157. [Google Scholar] [CrossRef]
- Gonzalez, S.R.; Klaseboer, E.; Khoo, B.C.; Ohl, C.-D. Cavitation bubble dynamics in a liquid gap of variable height. J. Fluid Mech. 2011, 682, 241–260. [Google Scholar] [CrossRef]
- Sun, C.; Can, E.; Dijkink, R.; Lohse, D.; Prosperetti, A. Growth and collapse of a vapour bubble in a microtube: The role of thermal effects. J. Fluid Mech. 2009, 632, 5–16. [Google Scholar] [CrossRef]
- Edel, Z.J.; Mukherjee, A. Experimental investigation of vapor bubble growth during flow boiling in a microchannel. Int. J. Multiph. Flow 2011, 37, 1257–1265. [Google Scholar] [CrossRef]
- Kangude, P.; Srivastava, A. Understanding the growth mechanism of single vapor bubble on a hydrophobic surface: Experiments under nucleate pool boiling regime. Int. J. Heat Mass Tran. 2020, 154, 119775. [Google Scholar] [CrossRef]
- Tang, J.K. Experimental and Simulation Research on the Oscillation Characteristics of Laser-Induced Cavitation Bubble Near a Hydrophobic Wall Surface. Master Thesis, Jiangsu University, Zhenjiang, China, 2020. [Google Scholar]
- Sagar, H.J.; El Moctar, O. Numerical simulation of a laser-induced cavitation bubble near a solid boundary considering phase change. Ship Technol. Res. 2018, 65, 163–179. [Google Scholar] [CrossRef]
- Krishnan, S.E.J.P. On the scaling of jetting from bubble collapse at a liquid surface. J. Fluid Mech. 2017, 822, 791–812. [Google Scholar] [CrossRef]
- Zhang, A.M.; Wang, C.; Wang, S.P. Experimental study of interaction between bubble and free surface. Acta Phys. Sinina-Chin. Ed. 2012, 61, 300–312. [Google Scholar] [CrossRef]
- Ma, Y.; Chung, J.N. A study of bubble dynamics in reduced gravity forced-convection boiling. Int. J. Heat Mass Tran. 2001, 44, 399–415. [Google Scholar] [CrossRef]
- Liu, X.M.; He, J.; Lu, J.; Ni, X.-W. Effect of liquid viscosity on the behavior of laser-induced cavitation bubbles. J. Optoelectron. Laser 2008, 157, 985–988. [Google Scholar]
- Phan, T.; Nguyen, V.; Park, W. Numerical study on strong nonlinear interactions between spark-generated underwater explosion bubbles and a free surface. Int. J. Heat Mass Tran. 2020, 163, 120506. [Google Scholar] [CrossRef]
- Wang, R.Y.; Chen, P.P.; Ban, C.Y. Keeping Volume Fraction of Fluid in Reconstructing Moving-interfaces of VOF on Rectangular Meshes. Chin. J. Comput. Phys. 2008, 122, 431–436. [Google Scholar]
- Nguyen, V.T.; Phan, T.H.; Duy, T.N.; Kim, D.H.; Park, W.G. Modeling of the bubble collapse with water jets and pressure loads using a geometrical volume of fluid based simulation method. Int. J. Multiph. Flow 2022, 152, 104103. [Google Scholar] [CrossRef]
- Arai, E.; Villafranco, D.; Grace, S.; Ryan, E. Simulating bubble dynamics in a buoyant system. Int. J. Numer. Methods Fluids 2020, 92, 169–188. [Google Scholar] [CrossRef]
- Liu, M.B.; Liu, G.R.; Li, S. Smoothed particle hydrodynamics: A meshfree method. Comput. Mech. 2004, 33, 491. [Google Scholar] [CrossRef]
- Wang, Y. Numerical Simulation and Experimental Research on Motion and Growth of Single Bubble Based on Diffuse Interface Method. Ph.D. Thesis, South China University of Technology, Guangzhou, China, 2019. [Google Scholar]
- Sigalotti, L.D.G.; Troconis, J.; Sira, E.; Peña-Polo, F.; Klapp, J. Diffuse-interface modeling of liquid-vapor coexistence in equilibrium drops using smoothed particle hydrodynamics. Phys. Rev. E 2014, 90, 013021. [Google Scholar] [CrossRef] [PubMed]
- Gallo, M.; Magaletti, F.; Casciola, C.M. Thermally activated vapor bubble nucleation: The Landau-Lifshitz–Van der Waals approach. Phys. Rev. Fluids 2018, 3, 053604. [Google Scholar] [CrossRef]
- Dong, W.; Yu, H.L.; Li, R.Y. Bubble Deformation in an electric field. J. Eng. Thermophys. 2006, 27, 265–267. [Google Scholar]
- Nugent, S.; Posch, H.A. Liquid drops and surface tension with smoothed particle applied mechanics. Phys. Rev. E 2000, 62, 4968–4975. [Google Scholar] [CrossRef]
- Yang, X.; Liu, M.; Peng, S. Smoothed particle hydrodynamics modeling of viscous liquid drop without tensile instability. Comput. Fluids 2014, 92, 199–208. [Google Scholar] [CrossRef]
- Xiong, H.B.; Zhang, C.Y.; Yu, Z.S. Multiphase SPH modeling of water boiling on hydrophilic and hydrophobic surfaces. Int. J. Heat Mass Tran. 2019, 130, 680–692. [Google Scholar] [CrossRef]








| ηs | CASE ID | L/dx |
|---|---|---|
| 1.0 | C1 | 15 |
| C2 | 30 | |
| C3 | 60 | |
| C4 | 100 | |
| C5 | 120 | |
| 0.1 | C6 | 15 |
| C7 | 30 | |
| C8 | 60 | |
| C9 | 100 | |
| C10 | 120 |
| CASE ID | H/dx | L/dx | ε = H/Rmax | λ = L/Rmax | γ = ε − λ | Nsecb |
|---|---|---|---|---|---|---|
| C1 | 160 | 15 | 4.31 | 0.32 | 3.99 | 0 |
| C2 | 160 | 30 | 3.78 | 0.57 | 3.21 | 0 |
| C3 | 160 | 60 | 3.55 | 1.06 | 2.48 | 0 |
| C4 | 160 | 100 | 2.97 | 1.48 | 1.48 | 0 |
| C5 | 160 | 120 | 2.22 | 1.43 | 0.79 | 0 |
| C6 | 160 | 15 | 2.18 | 0.16 | 2.02 | 3 |
| C7 | 160 | 30 | 2.36 | 0.35 | 2.01 | 3 |
| C8 | 160 | 60 | 2.68 | 0.80 | 1.87 | 4 |
| C9 | 160 | 100 | 2.78 | 1.39 | 1.39 | 0 |
| C10 | 160 | 120 | 2.29 | 1.37 | 0.92 | 0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, Y.; Wang, Q.; Xiong, H.; Qian, L. Vapor Bubble Deformation and Collapse near Free Surface. Fluids 2023, 8, 187. https://doi.org/10.3390/fluids8070187
Chen Y, Wang Q, Xiong H, Qian L. Vapor Bubble Deformation and Collapse near Free Surface. Fluids. 2023; 8(7):187. https://doi.org/10.3390/fluids8070187
Chicago/Turabian StyleChen, Yue, Qichao Wang, Hongbing Xiong, and Lijuan Qian. 2023. "Vapor Bubble Deformation and Collapse near Free Surface" Fluids 8, no. 7: 187. https://doi.org/10.3390/fluids8070187
APA StyleChen, Y., Wang, Q., Xiong, H., & Qian, L. (2023). Vapor Bubble Deformation and Collapse near Free Surface. Fluids, 8(7), 187. https://doi.org/10.3390/fluids8070187







