Using Computation Fluid Dynamics to Determine Oil Droplet Breakup Parameters during Emulsion Atomization with Pressure Swirl Nozzles
Abstract
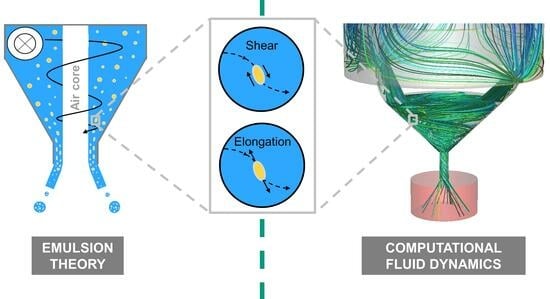
:1. Introduction
- There is a complete cylindrical air core inside of the nozzle, which is what enables stable atomization.
- Because of the air core, the liquid forms a thin lamella in the outlet channel, where the oil droplets experience the highest shear rate.
- This highest shear rate determines the maximum oil droplet size that can result from the atomization step.
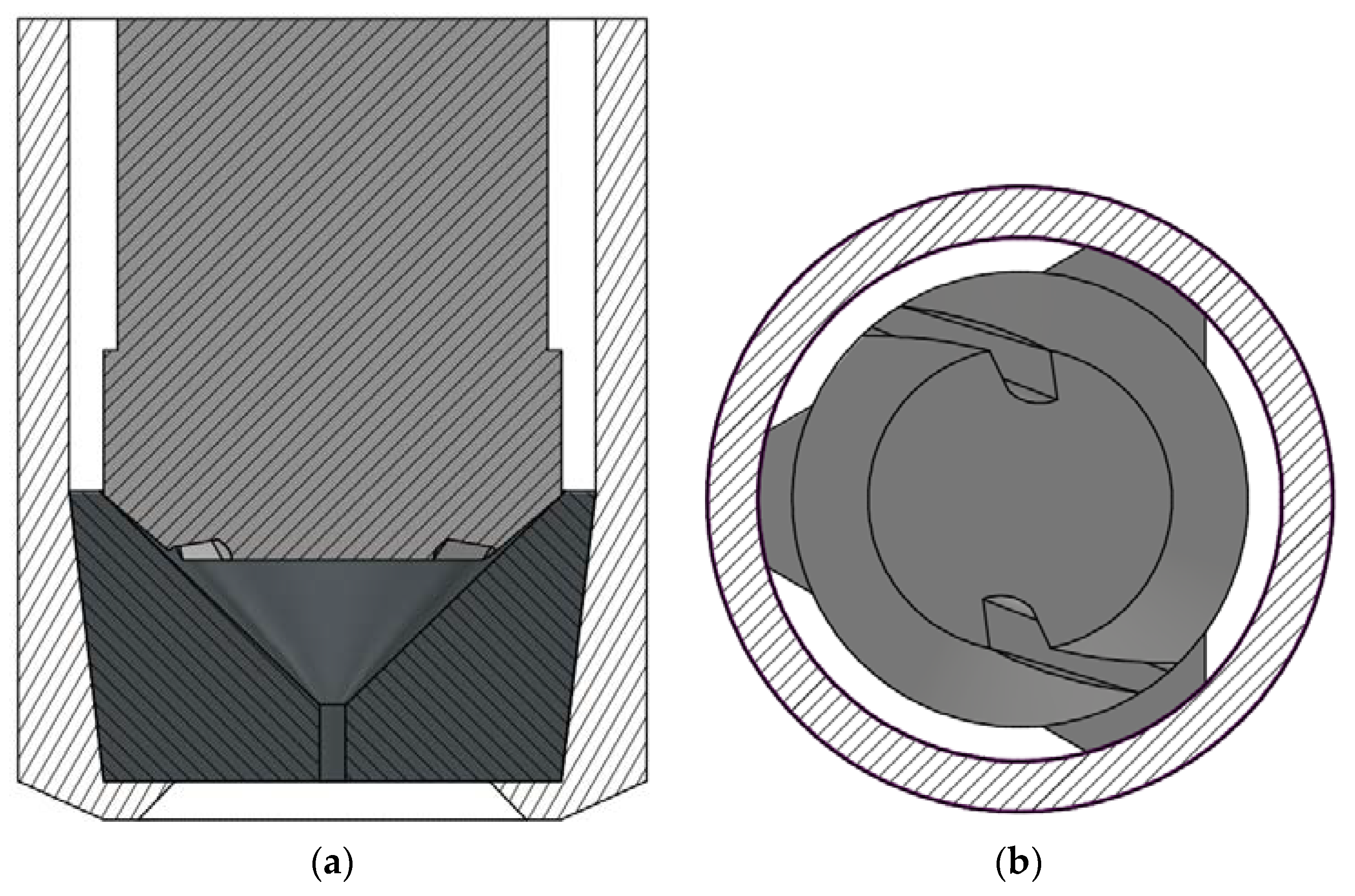
2. Nozzle Designs
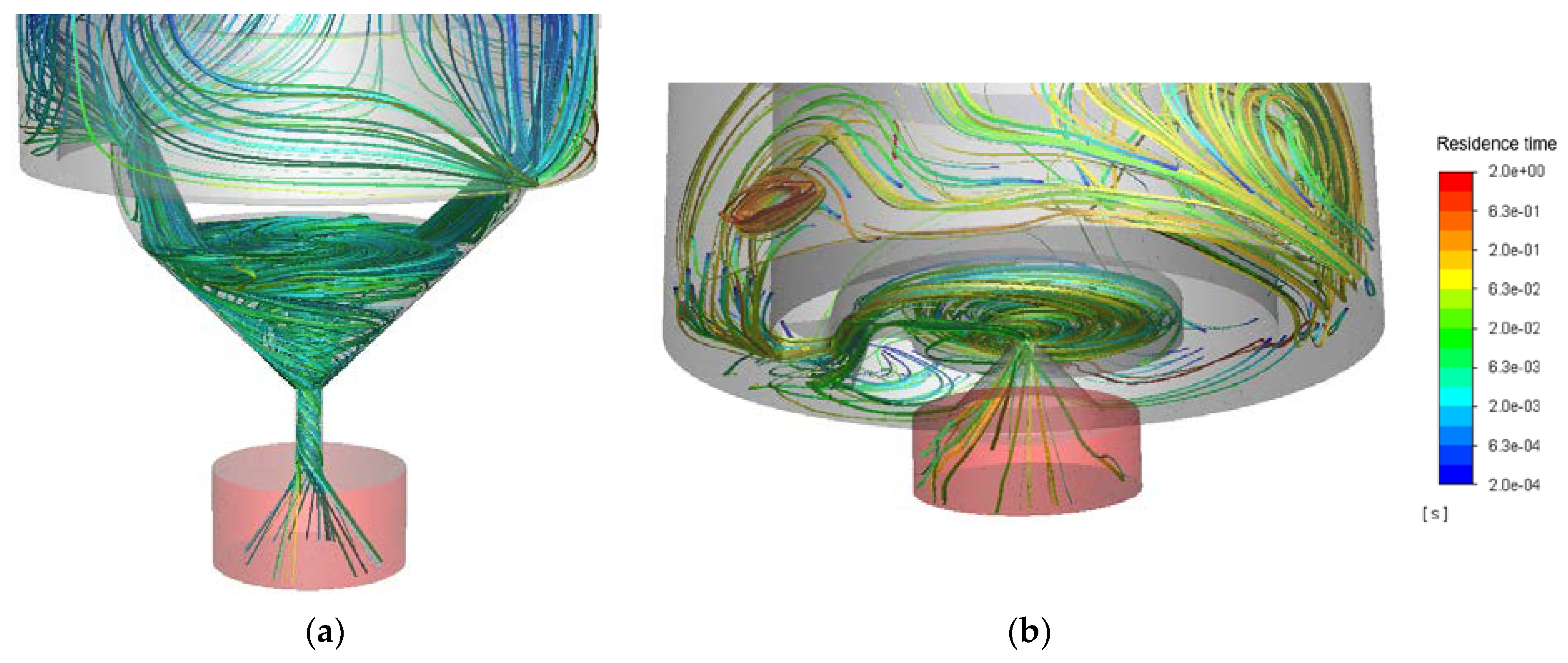
2.1. SK Nozzle
2.2. MiniSDX Nozzle
3. Experimental Setup
3.1. Atomization Rig
3.2. Model System
3.3. Spray Angle Measurement
4. Numerical Model
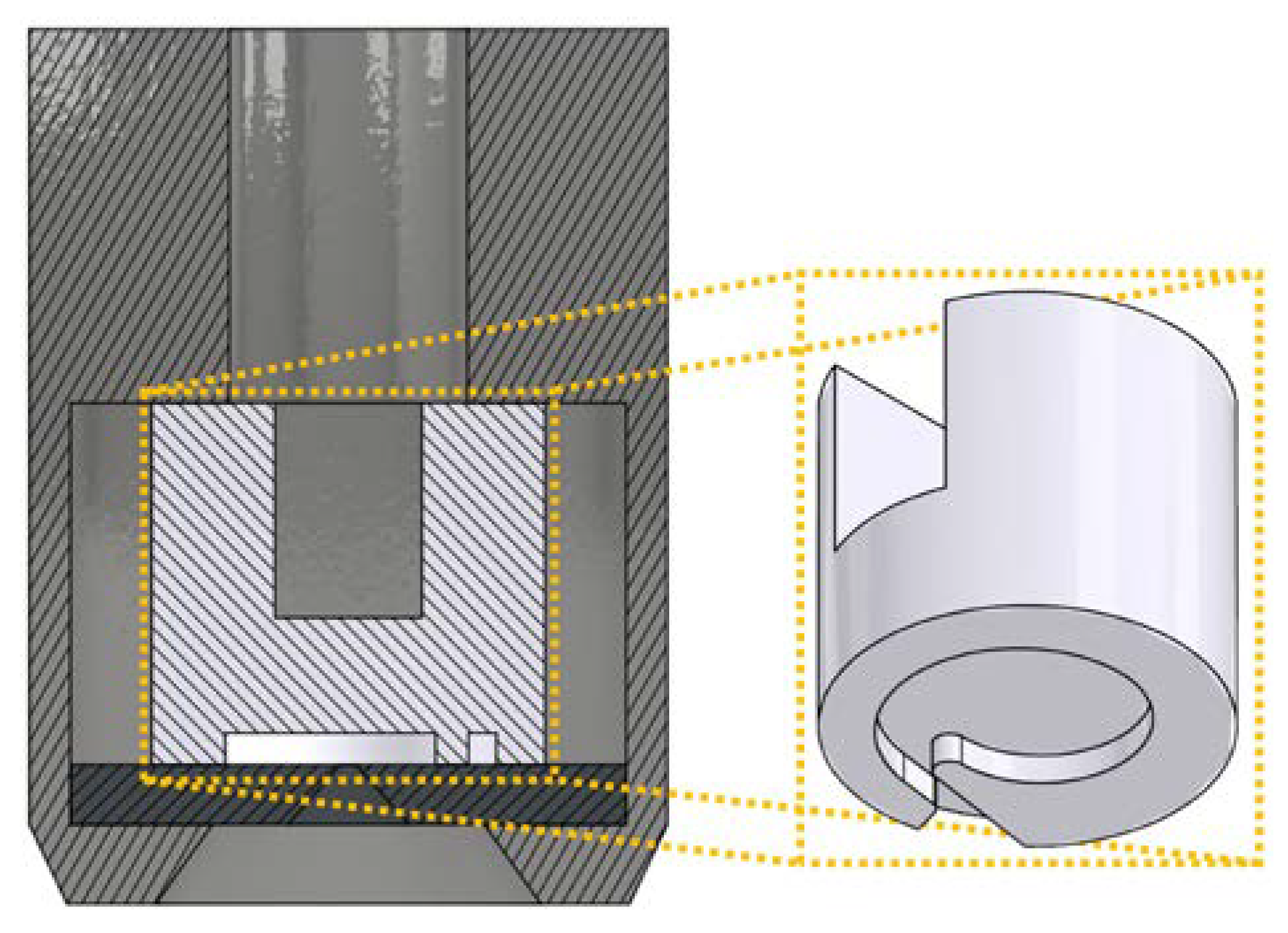
4.1. Internal Volume of the Spray Nozzles
4.2. Governing Equations and Models
4.3. Boundary and Simulation Conditions
4.4. Mesh Generation
4.5. Droplet Parameter Measurements
5. Results
5.1. Validation of Predicted Operating Conditions
5.2. Validation of Predicted Flow Behaviour for the SK Nozzle
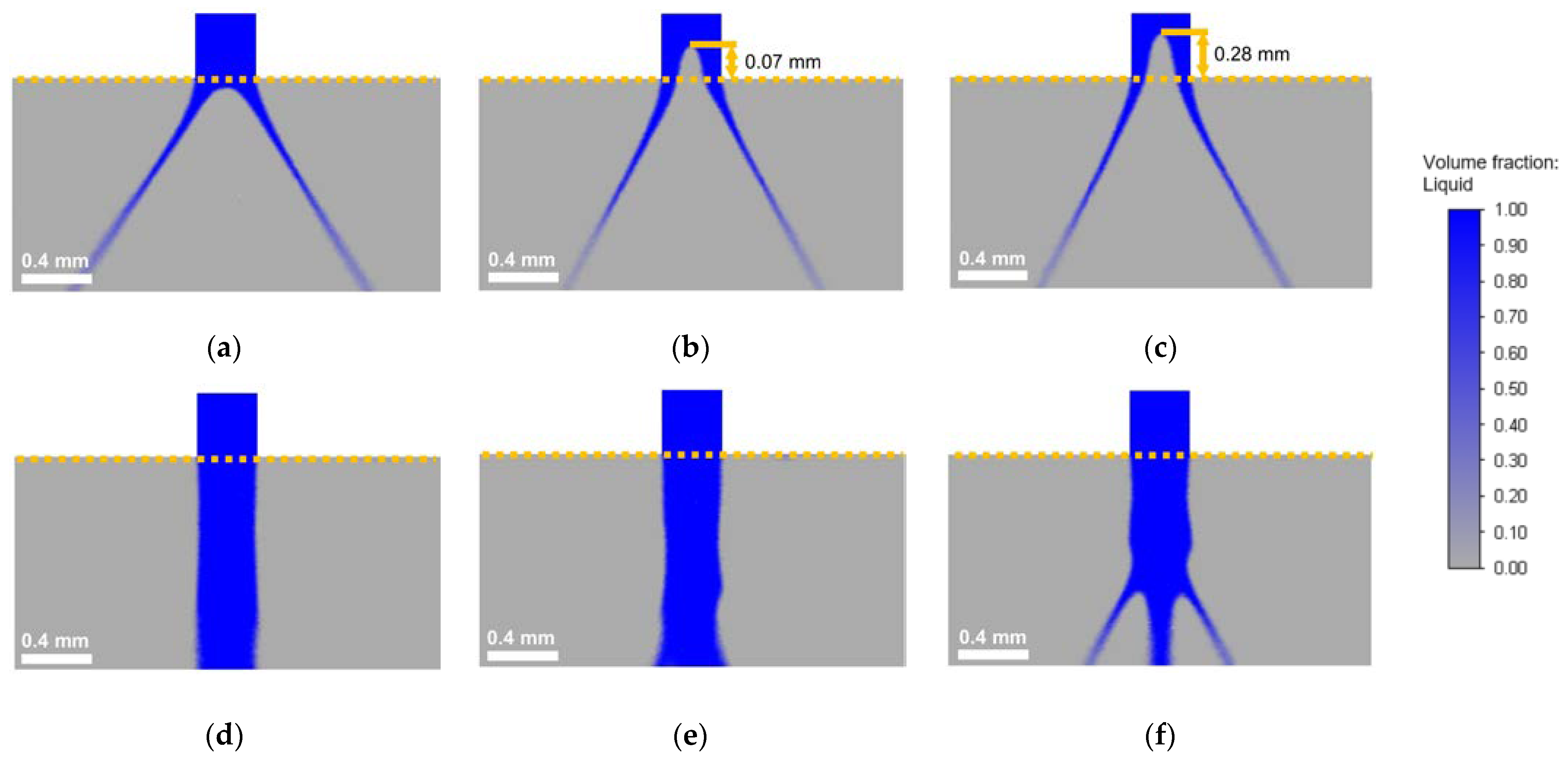
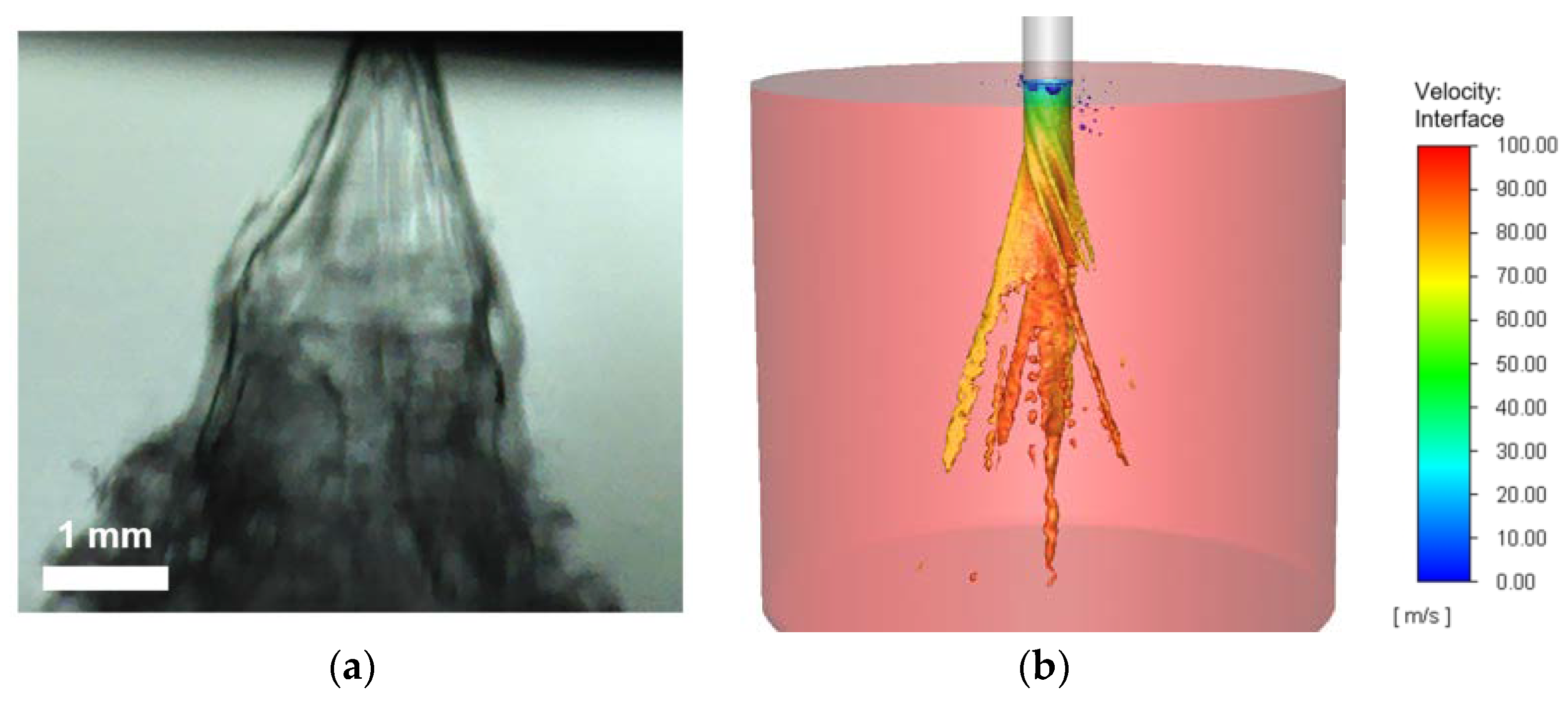
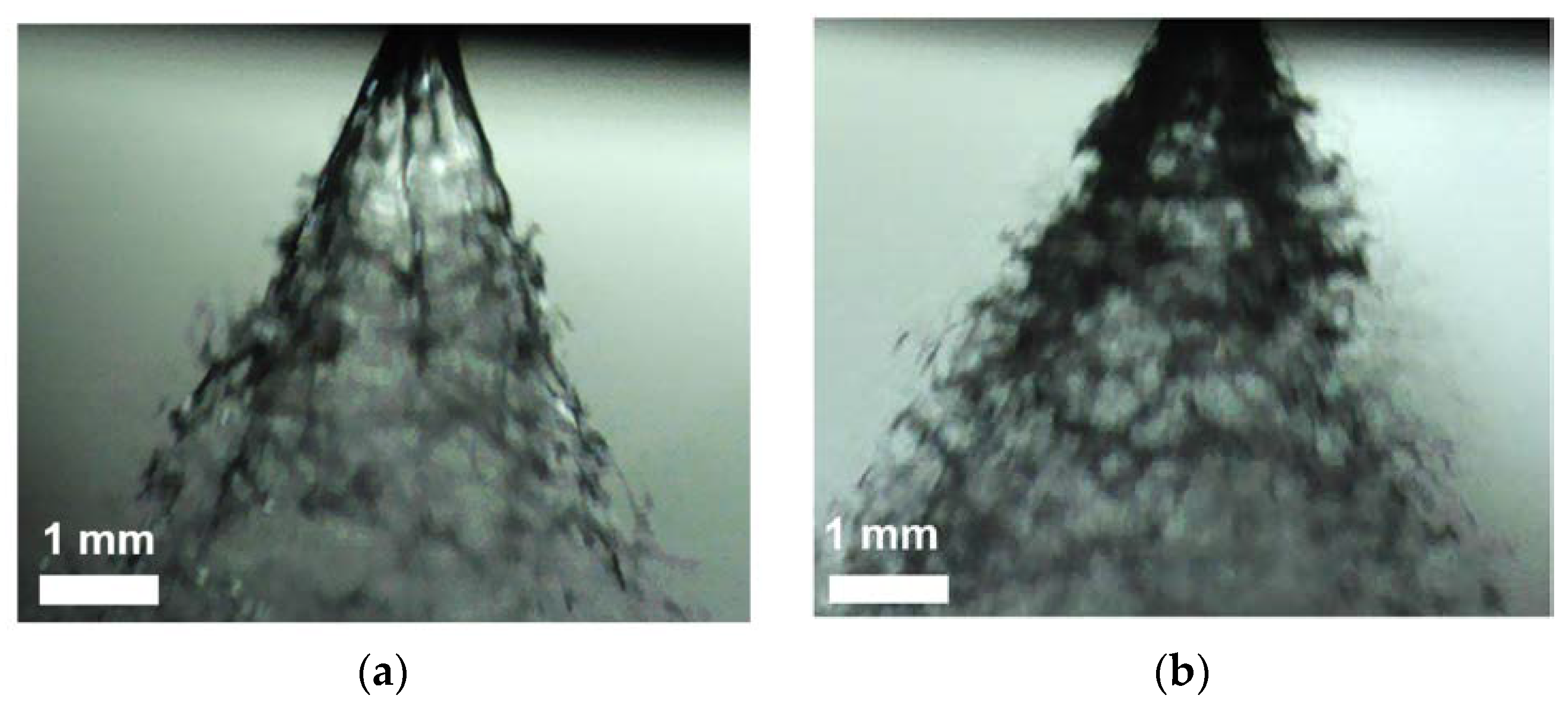
5.2.1. Spray Cone Formation
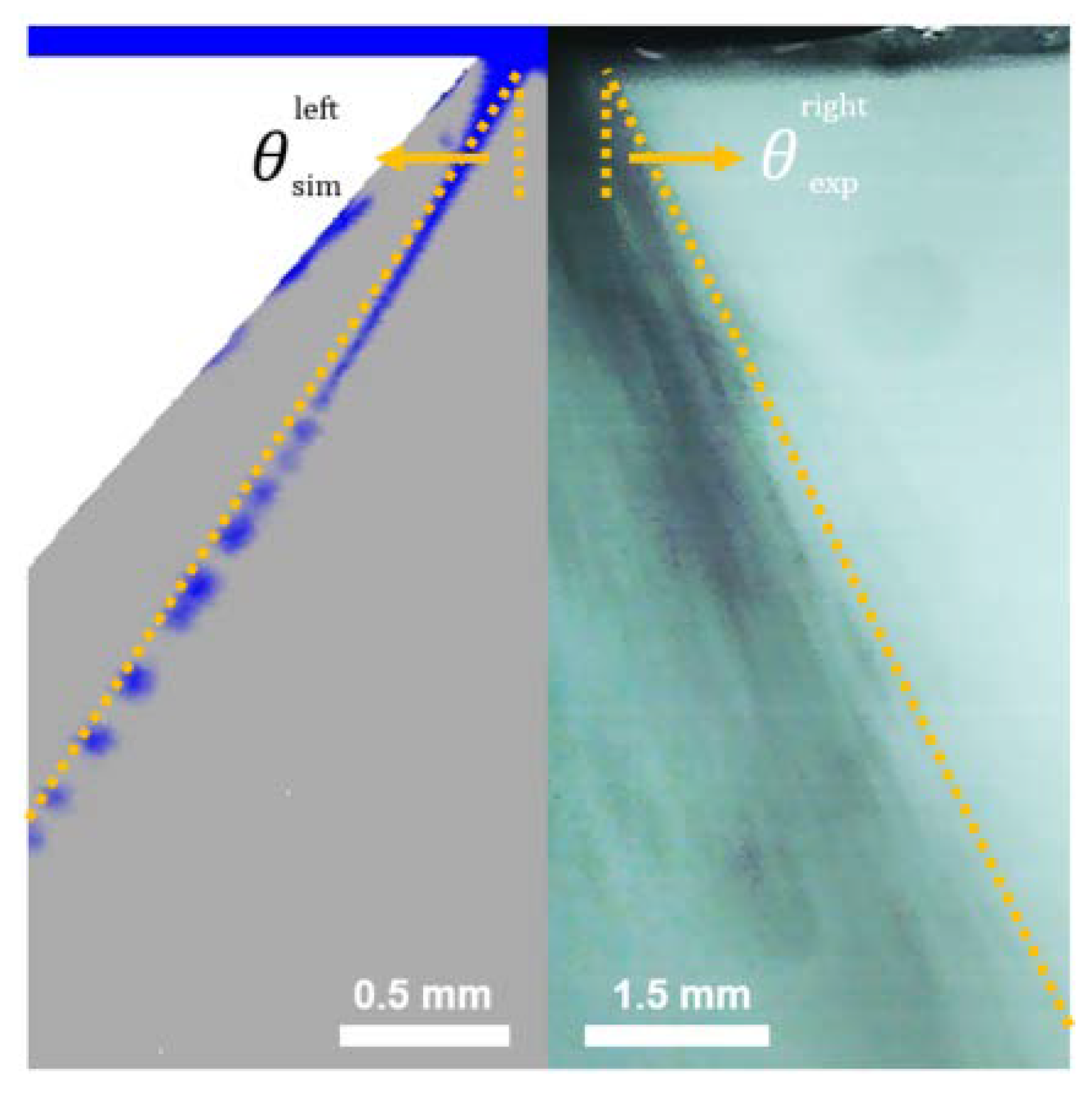
5.2.2. Spray Angle Evaluation
5.2.3. Validation of Simulated Flow Conditions
5.3. Validation of Predicted Flow Behaviour for the MiniSDX Nozzle
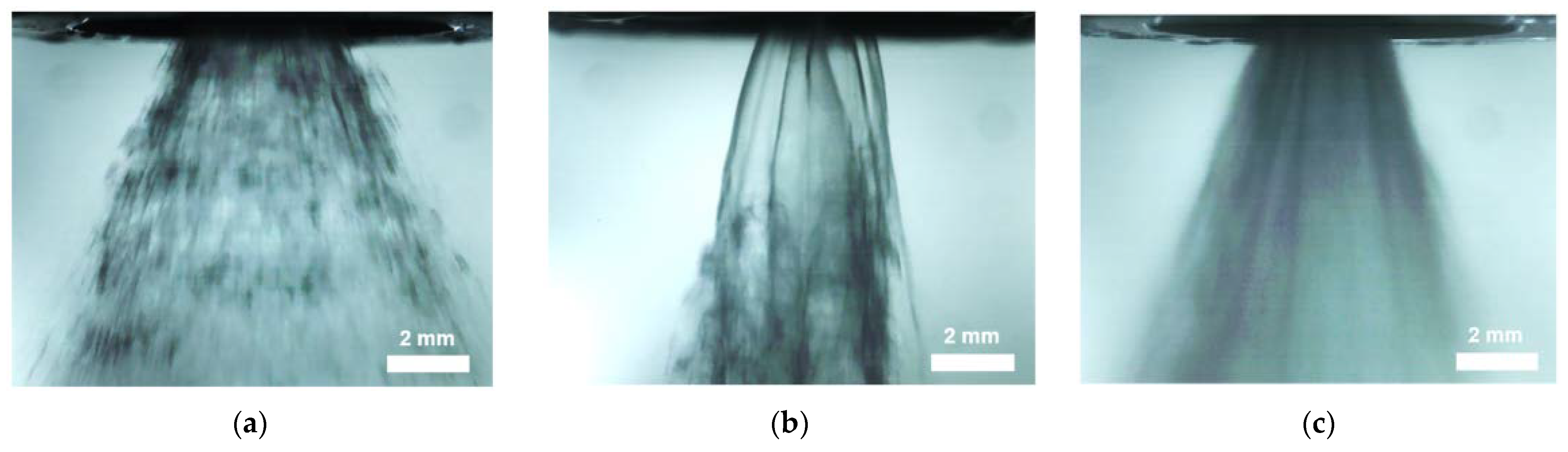
5.3.1. Spray Cone Formation
5.3.2. Spray Angle Evaluation
5.3.3. Validation of Simulated Flow Conditions
5.4. Determination of Oil Droplet Breakup Parameters
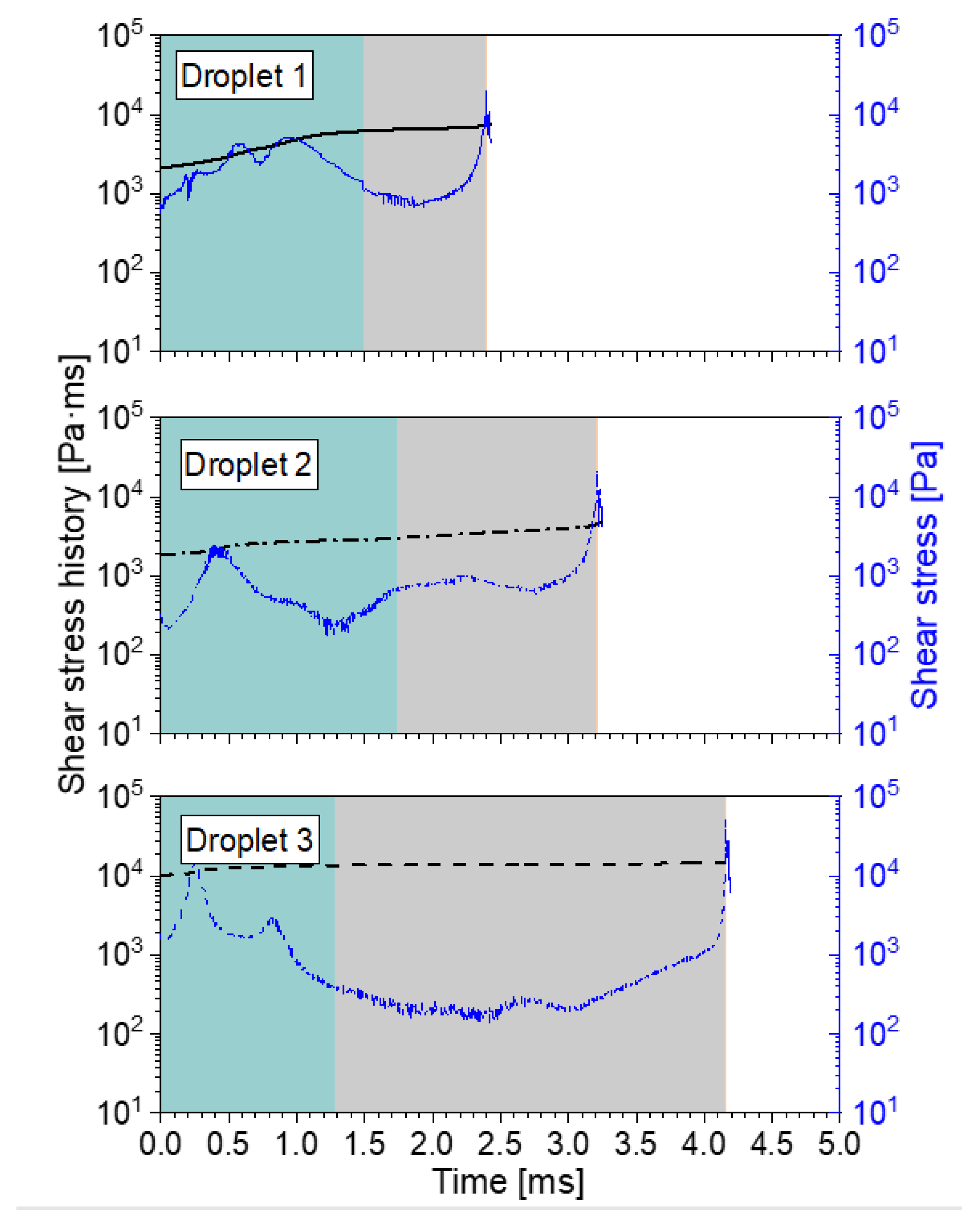
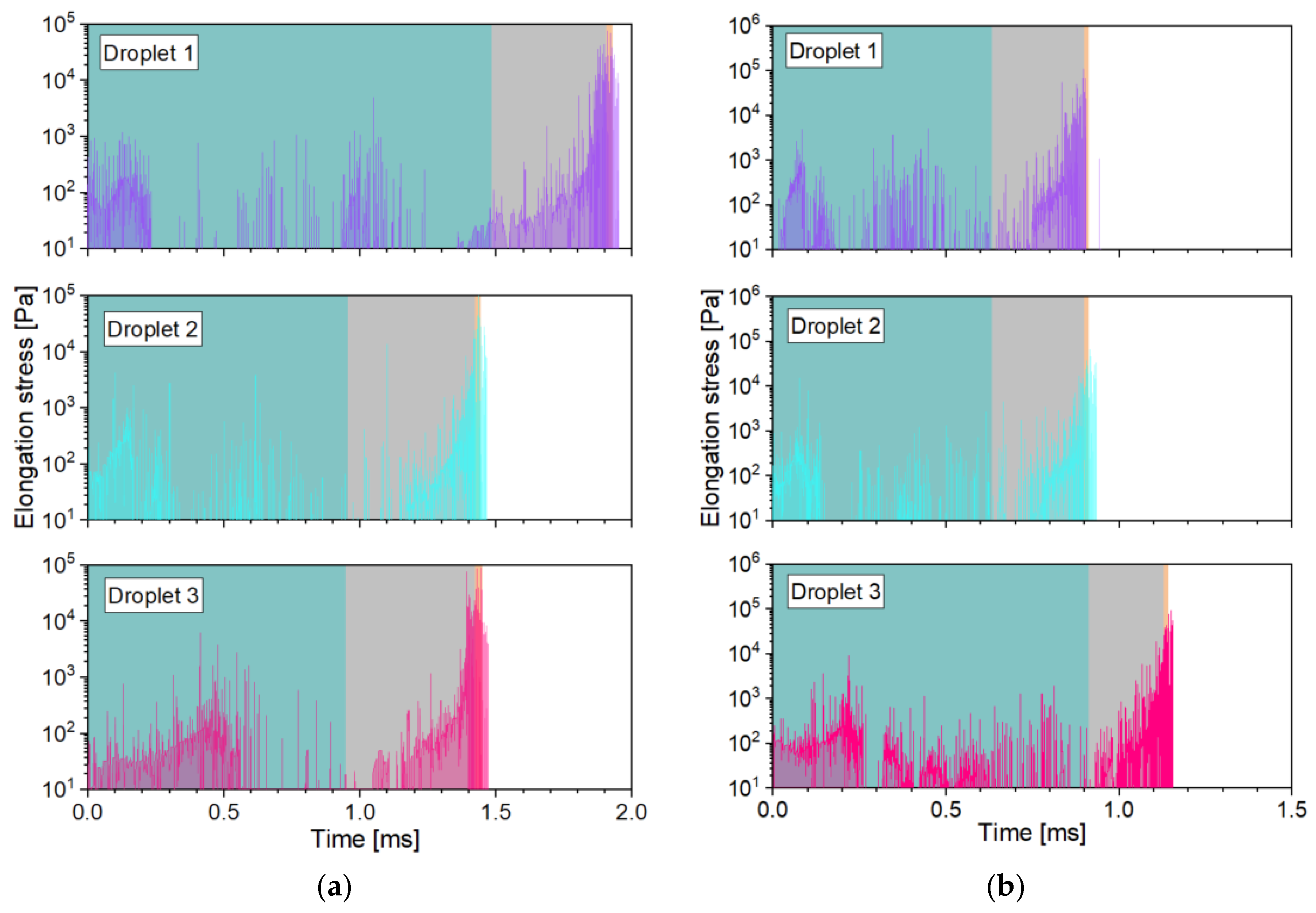
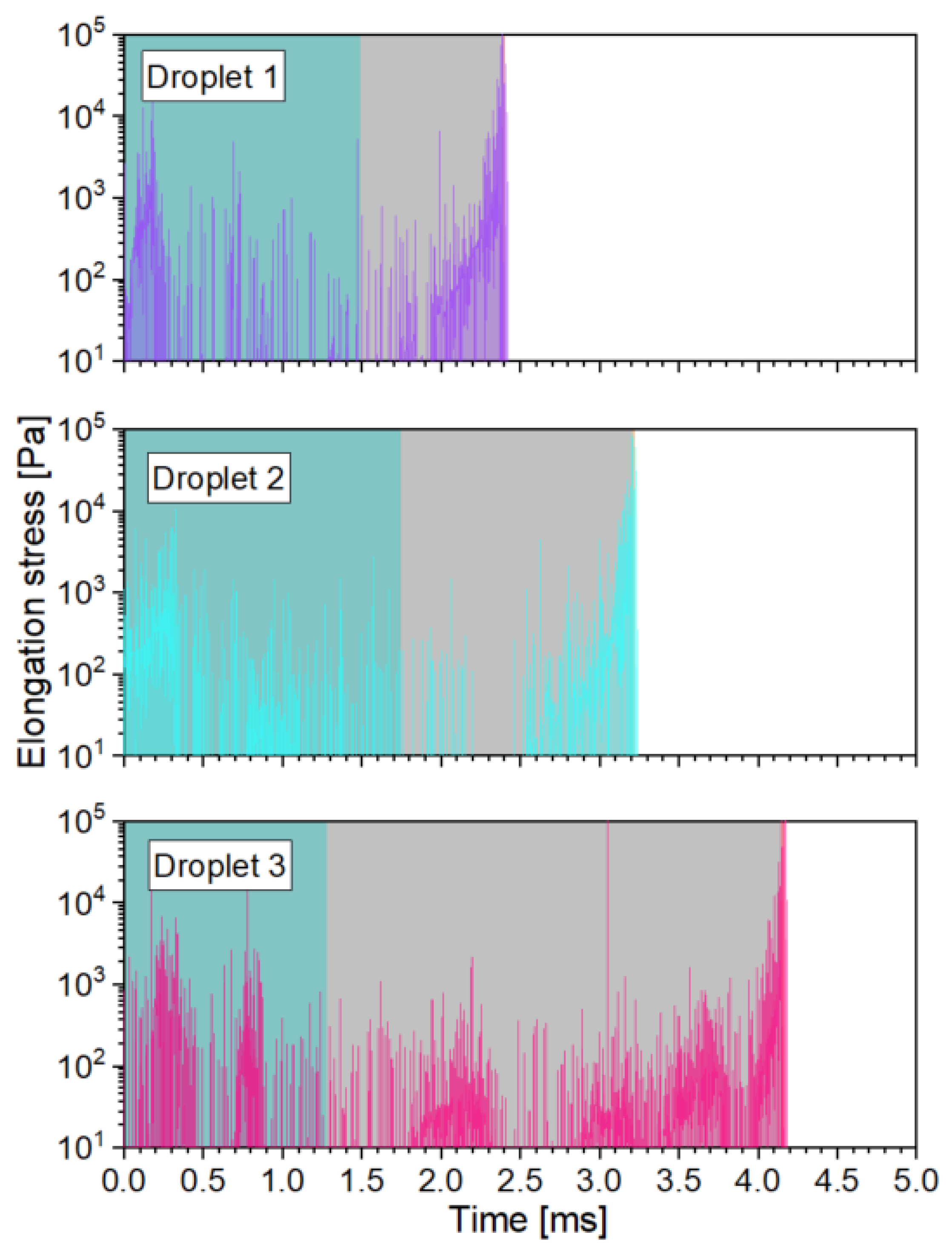
5.4.1. Deformation Stresses
5.4.2. Deformation Time
6. Evaluation of Droplet Breakup
6.1. Emulsion Theory
6.2. Two-Step Droplet Breakup Inside of the Nozzles
6.2.1. Breakup at the Inlet Ports
6.2.2. Breakup at the Outlet Channels
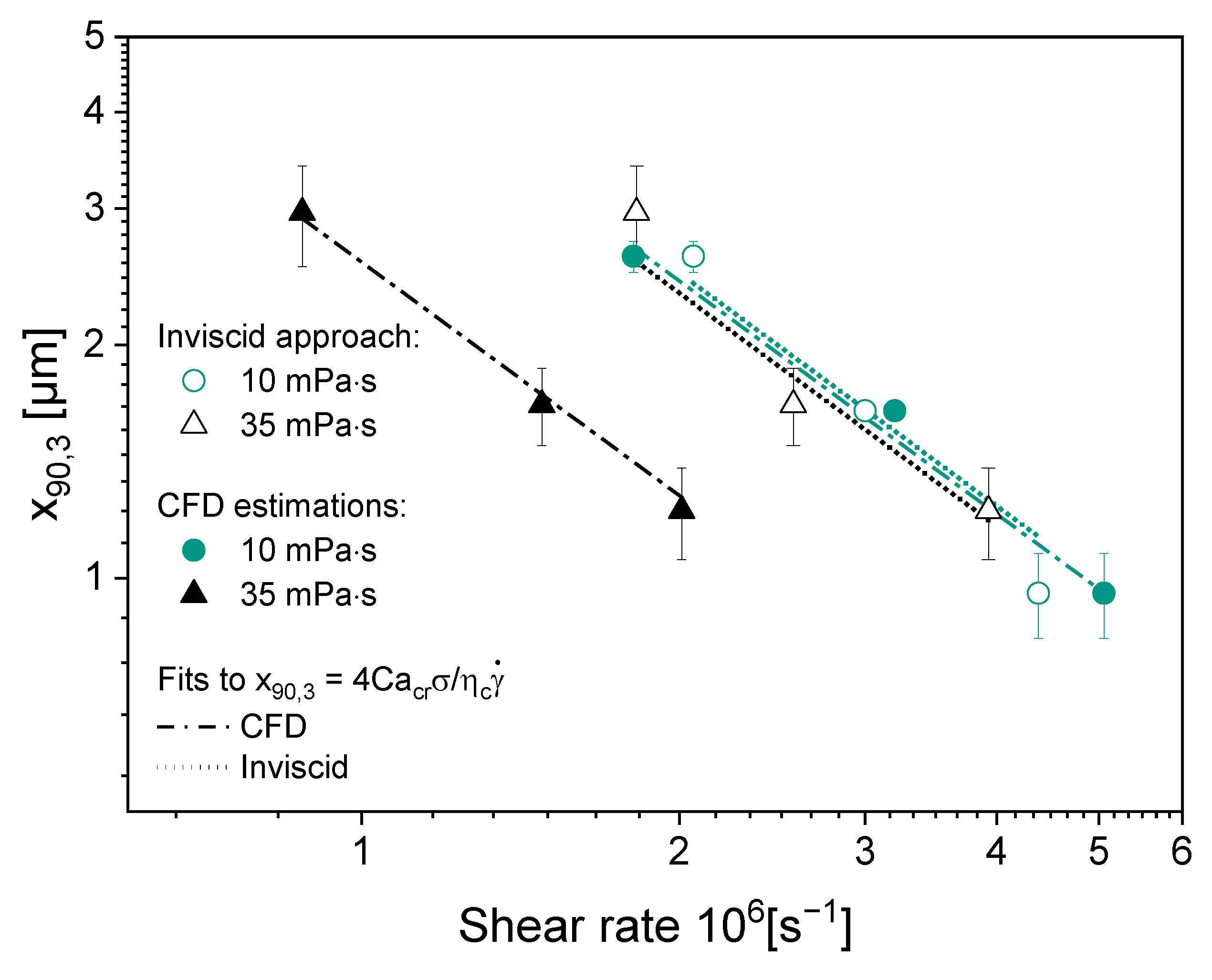
6.3. Correlation with Experimental Droplet Sizes
7. Conclusions
- A complete air core forms inside of the nozzles.
- The highest shear rate is found at the nozzle outlet channel.
- This shear stress at the nozzle outlet is correlated with the final oil droplet size.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Three-Dimensional Liquid Profiles of the Nozzle Outlets

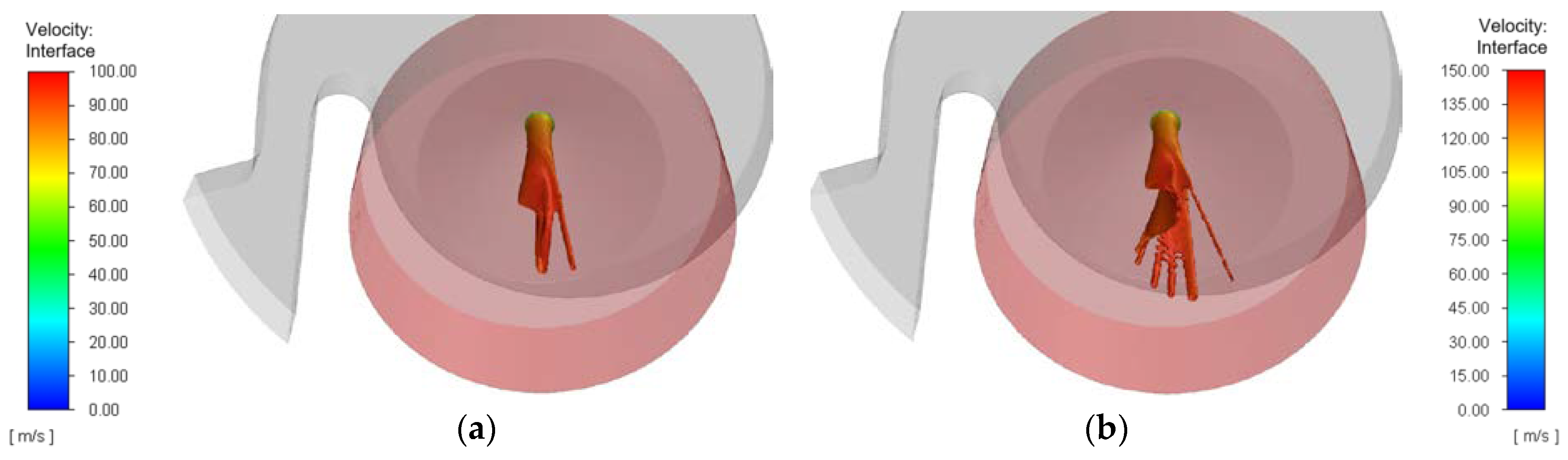
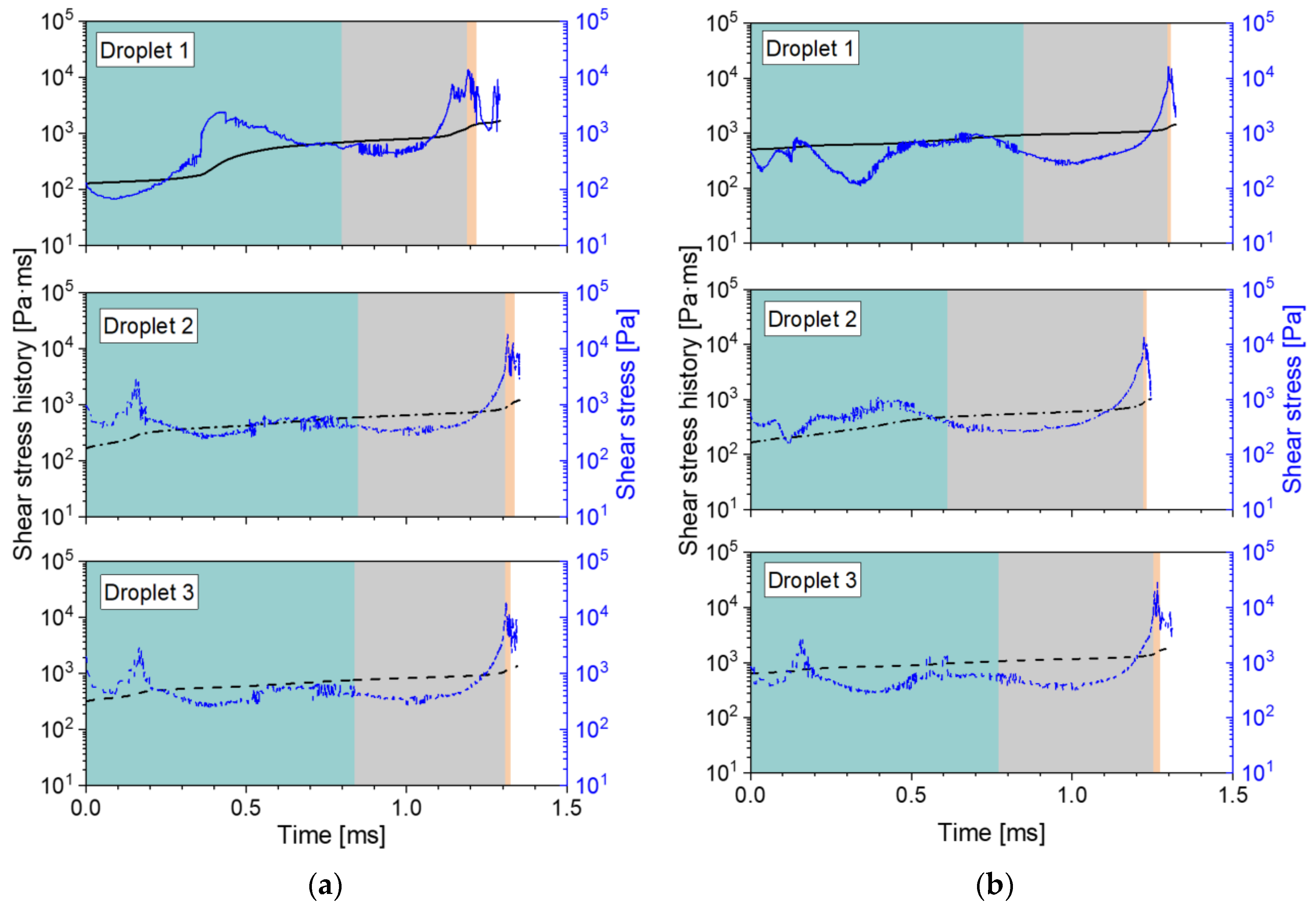
Appendix B. Shear Stress Profiles for the SK Nozzle
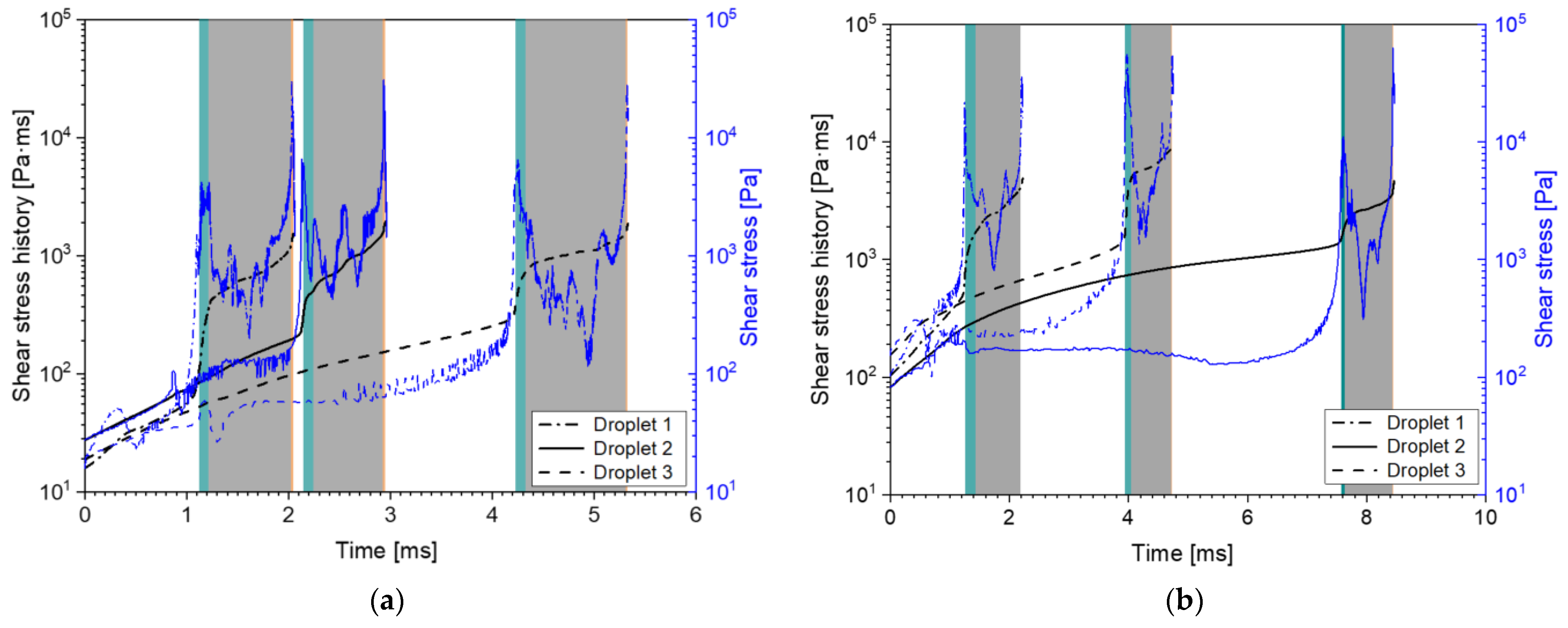

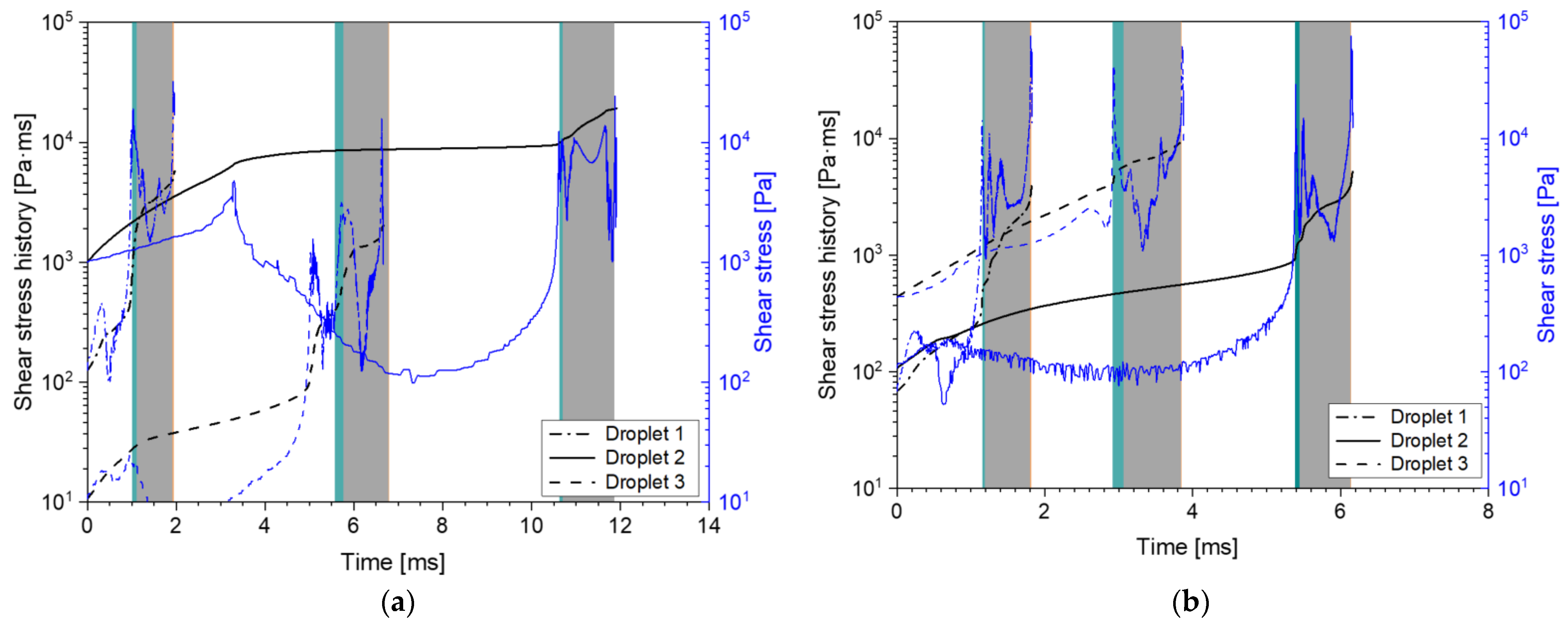
Appendix C. Elongation Stress Profiles for the SK Nozzle
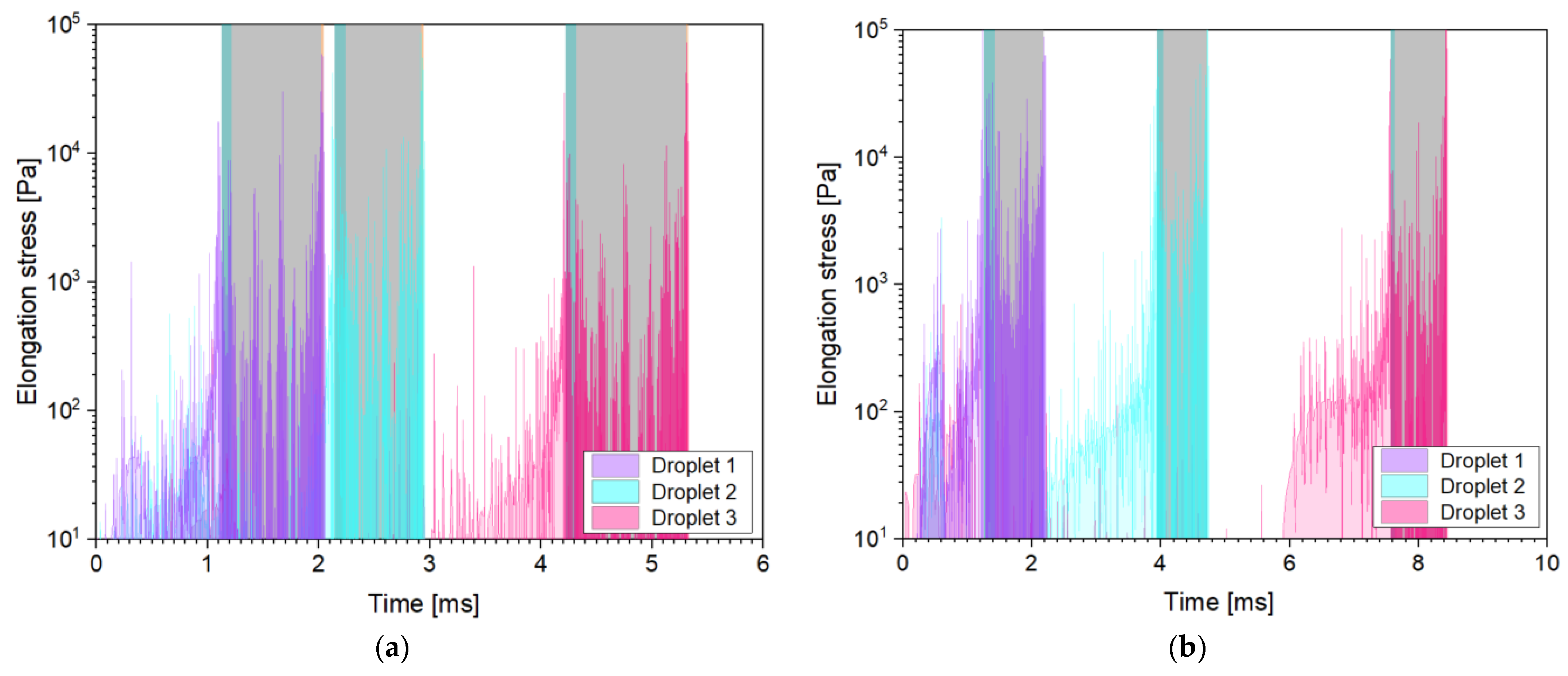
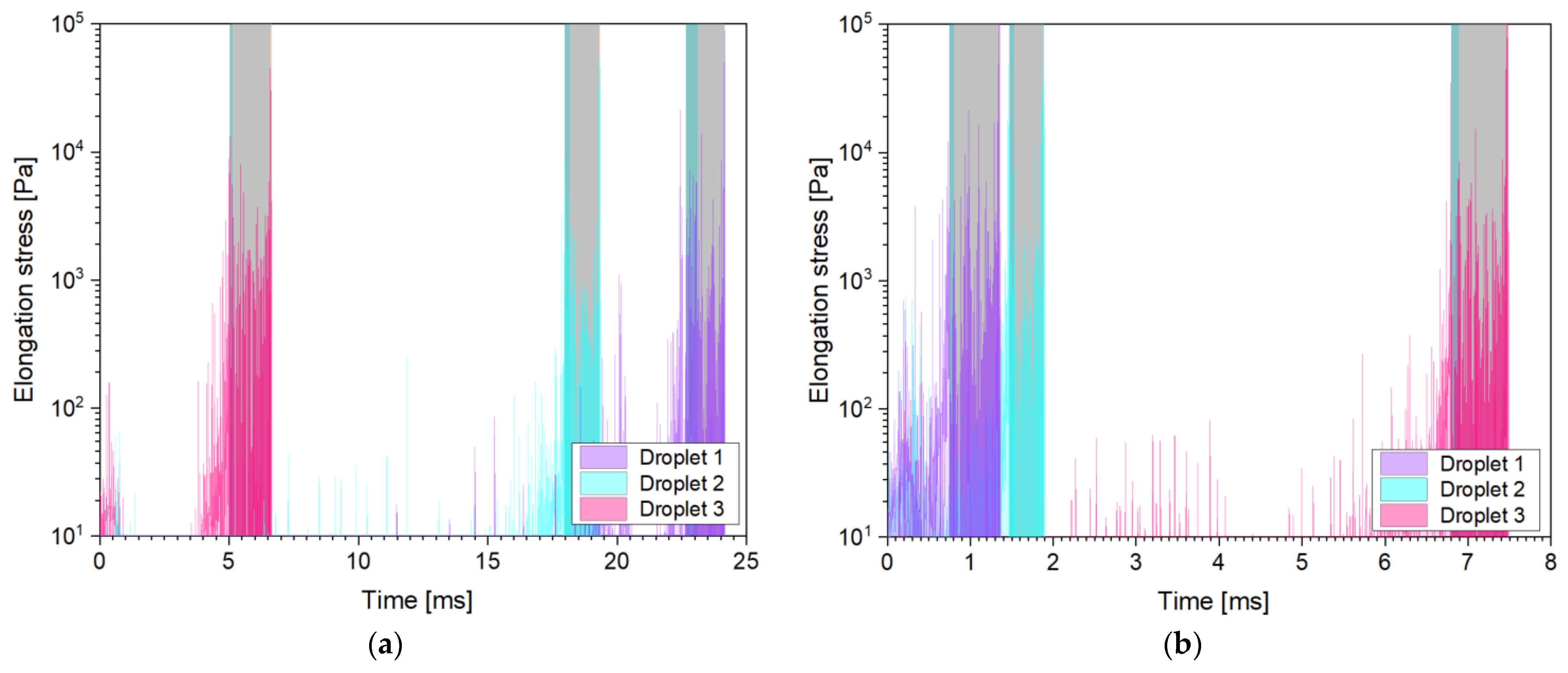
Appendix D. Shear Stress Profiles for the MiniSDX Nozzle

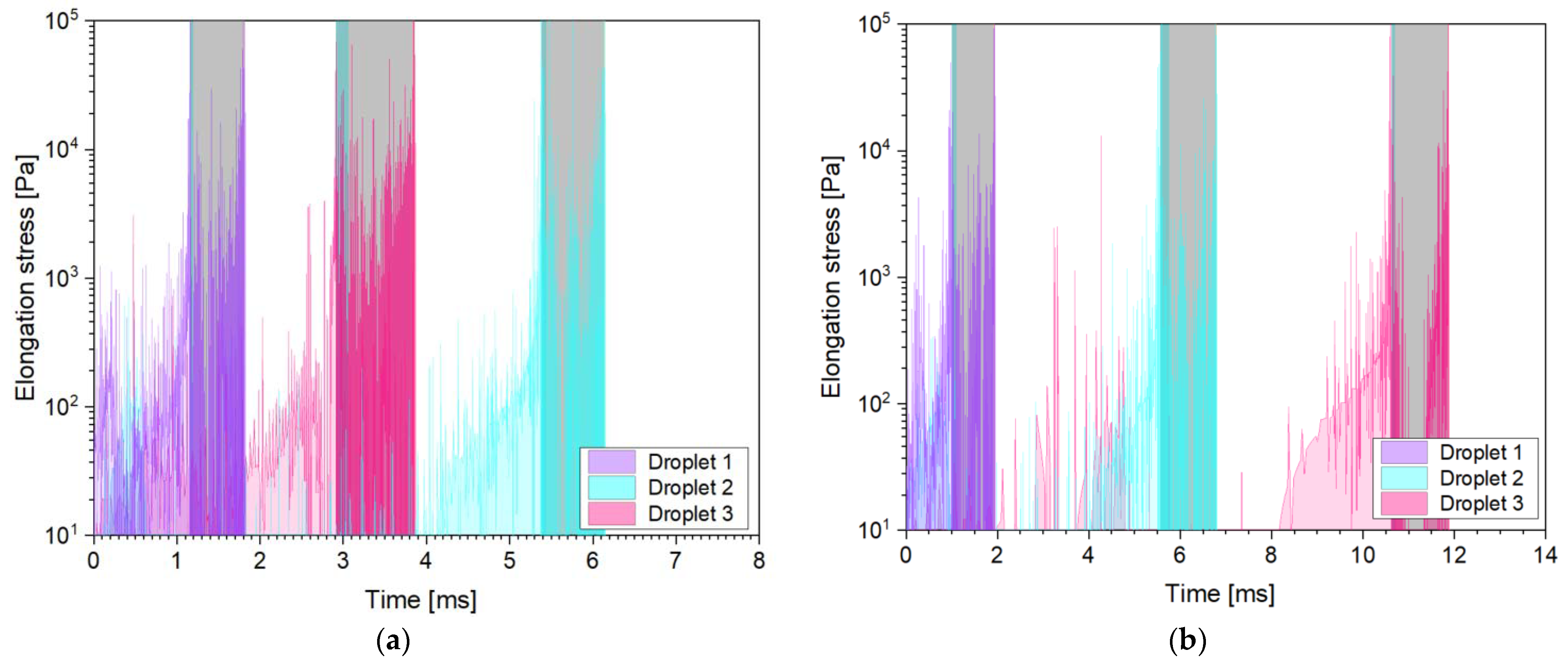
Appendix E. Elongational Stress Profiles for the MiniSDX Nozzle

References
- Reineccius, G.A. The Spray Drying of Food Flavors. Dry. Technol. 2004, 22, 1289–1324. [Google Scholar] [CrossRef]
- Vega, C.; Roos, Y.H. Invited review: Spray-dried dairy and dairy-like emulsions--compositional considerations. J. Dairy Sci. 2006, 89, 383–401. [Google Scholar] [CrossRef]
- Del Hernandez Sanchez, M.R.; Cuvelier, M.-E.; Turchiuli, C. Design of liquid emulsions to structure spray dried particles. J. Food Eng. 2015, 167, 99–105. [Google Scholar] [CrossRef]
- Taboada, M.L.; Schäfer, A.-C.; Karbstein, H.P.; Gaukel, V. Oil droplet breakup during pressure swirl atomization of food emulsions: Influence of atomization pressure and initial oil droplet size. J. Food Process Eng. 2021, 44, e13598. [Google Scholar] [CrossRef]
- Barbosa-Cánovas, G.V.; Ortega-Rivas, E.; Juliano, P.; Yan, H. Food Powders: Physical Properties, Processing, and Functionality; Springer: Boston, MA, USA, 2005; pp. 271–304. ISBN 978-0-306-47806-2. [Google Scholar]
- Lefebvre, A.H.; McDonell, V.G. Atomization and Sprays, 2nd ed.; CRC Press: Boca Raton, FL, USA; London, UK; New York, NY, USA, 2017; ISBN 9781498736268. [Google Scholar]
- Walzel, P. Spraying and Atomizing of liquids. In Ullmann’s Encyclopedia of Industrial Chemistry; Wiley-VCH Verlag GmbH & Co. KGaA: Weinheim, Germany, 2000; ISBN 3527306730. [Google Scholar]
- Bolszo, C.D.; Narvaez, A.A.; McDonell, V.; Dunn-Rankin, D.; Sirignano, W.A. Pressure swirl atomization of water-in-oil emulsions. At. Sprays 2010, 20, 1077–1099. [Google Scholar] [CrossRef]
- Taboada, M.L.; Müller, E.; Fiedler, N.; Karbstein, H.P.; Gaukel, V. Oil droplet breakup during pressure swirl atomization of emulsions: Influence of emulsion viscosity and viscosity ratio. J. Food Eng. 2022, 321, 110941. [Google Scholar] [CrossRef]
- Renze, P.; Heinen, K.; Schönherr, M. Experimental and Numerical Investigation of Pressure Swirl Atomizers. Chem. Eng. Technol. 2011, 34, 1191–1198. [Google Scholar] [CrossRef]
- Laurila, E.; Roenby, J.; Maakala, V.; Peltonen, P.; Kahila, H.; Vuorinen, V. Analysis of viscous fluid flow in a pressure-swirl atomizer using large-eddy simulation. Int. J. Multiph. Flow 2019, 113, 371–388. [Google Scholar] [CrossRef]
- Maly, M.; Jedelsky, J.; Slama, J.; Janackova, L.; Sapik, M.; Wigley, G.; Jicha, M. Internal flow and air core dynamics in Simplex and Spill-return pressure-swirl atomizers. Int. J. Heat Mass Transf. 2018, 123, 805–814. [Google Scholar] [CrossRef]
- ANSYS, Inc. Ansys Fluent Theory Guide, Release 2019 R3; ANSYS, Inc.: Canonsburg, PA, USA, 2019. [Google Scholar]
- Maly, M.; Slama, J.; Sapik, M.; Jedelsky, J. 2D and 3D numerical modelling of internal flow of Pressure-swirl atomizer. EPJ Web Conf. 2019, 213, 2055. [Google Scholar] [CrossRef]
- Anderson, J.D. Fundamentals of Aerodynamics, 6th ed.; McGraw-Hill Education: New York, NY, USA, 2017; ISBN 1259129918. [Google Scholar]
- Jabbari, M.; Bulatova, R.; Hattel, J.H.; Bahl, C. An evaluation of interface capturing methods in a VOF based model for multiphase flow of a non-Newtonian ceramic in tape casting. Appl. Math. Model. 2014, 38, 3222–3232. [Google Scholar] [CrossRef]
- Ballesteros Martínez, M.Á.; Gaukel, V. Time-Averaged Analysis and Numerical Modelling of the Behavior of the Multiphase Flow and Liquid Lamella Thickness Inside an Internal-Mixing ACLR Nozzle. Flow Turbul. Combust 2023, 110, 601–628. [Google Scholar] [CrossRef]
- Ballesteros Martínez, M.; Pereyra, E.; Ratkovich, N. CFD study and experimental validation of low liquid-loading flow assurance in oil and gas transport: Studying the effect of fluid properties and operating conditions on flow variables. Heliyon 2020, 6, e05705. [Google Scholar] [CrossRef] [PubMed]
- Zeigarnik, Y.A. Annular flow. In A-to-Z Guide to Thermodynamics, Heat and Mass Transfer, and Fluids Engineering; Begellhouse: Danbury, CT, USA, 2006. [Google Scholar]
- Ramsey, M.S. Rheology, viscosity, and fluid types. In Practical Wellbore Hydraulics and Hole Cleaning; Elsevier: Amsterdam, The Netherlands, 2019; pp. 217–237. ISBN 9780128170885. [Google Scholar]
- Shashi Menon, E. Fluid flow in pipes. In Transmission Pipeline Calculations and Simulations Manual; Elsevier: Amsterdam, The Netherlands, 2015; pp. 149–234. ISBN 9781856178303. [Google Scholar]
- Okumura, K. CFD Simulation by Automatically Generated Tetrahedral and Prismatic Cells for Engine Intake Duct and Coolant Flow in Three Days. In SAE Technical Paper Series; SAE 2000 World Congress, MAR. 06, 2000; SAE International 400 Commonwealth Drive: Warrendale, PA, USA, 2000. [Google Scholar]
- White, F.M. Fluid Mechanics, 8th ed.; McGraw-Hill: New York, NY, USA, 2016; ISBN 978-0073398273. [Google Scholar]
- Schlichting, H.; Gersten, K. Boundary-Layer Theory; Springer: Berlin/Heidelberg, Germany, 2017; ISBN 978-3-662-52917-1. [Google Scholar]
- Petrie, C.J. Extensional viscosity: A critical discussion. J. Non-Newton. Fluid Mech. 2006, 137, 15–23. [Google Scholar] [CrossRef]
- George, H.F.; Qureshi, F. Newton’s law of viscosity, newtonian and non-newtonian fluids. In Encyclopedia of Tribology; Wang, Q.J., Chung, Y.-W., Eds.; Springer: Boston, MA, USA, 2013; pp. 2416–2420. ISBN 978-0-387-92896-8. [Google Scholar]
- Grace, H.P. Dispersion phenomena in high viscosity immiscible fluid systems and application of static mixers as dispersion devices in such systems. Chem. Eng. Commun. 1982, 14, 225–277. [Google Scholar] [CrossRef]
- Spurk, J.H.; Aksel, N. Fluid Mechanics, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2008; ISBN 9783540735366. [Google Scholar]
- Preiss, F.J.; Rütten, E.; Tröster, A.; Gräf, V.; Karbstein, H.P. Influence of the droplet trajectory on the resulting droplet deformation and droplet size distribution in high-pressure homogenizer orifices. Can. J. Chem. Eng. 2022, 100, 1451–1467. [Google Scholar] [CrossRef]
- Tannehill, J.C.; Anderson, D.A.; Pletcher, R.H. Computational Fluid Mechanics and Heat Transfer, 2nd ed.; Taylor & Francis: Washington, DC, USA, 1997; ISBN 9781560320463. [Google Scholar]
- Galbiati, C.; Tonini, S.; Conti, P.; Cossali, G.E. Numerical Simulations of Internal Flow in an Aircraft Engine Pressure Swirl Atomizer. J. Propuls. Power 2016, 32, 1433–1441. [Google Scholar] [CrossRef]
- Lee, E.J.; Oh, S.Y.; Kim, H.Y.; James, S.C.; Yoon, S.S. Measuring air core characteristics of a pressure-swirl atomizer via a transparent acrylic nozzle at various Reynolds numbers. Exp. Therm. Fluid Sci. 2010, 34, 1475–1483. [Google Scholar] [CrossRef]
- Liu, Z.; Huang, Y.; Sun, L. Studies on air core size in a simplex pressure-swirl atomizer. Int. J. Hydrog. Energy 2017, 42, 18649–18657. [Google Scholar] [CrossRef]
- Brackbill, J.; Kothe, D.; Zemach, C. A continuum method for modeling surface tension. J. Comput. Phys. 1992, 100, 335–354. [Google Scholar] [CrossRef]
- Szeliga, N.; Helmrich von Elgott, L.; Bezecny, D.; Richter, S.; Hoffmann, M.; Schlüter, M. Large-Scale Experiments on the Formation of Surface Vortices with and without Vortex Suppression. Chem. Ing. Tech. 2019, 91, 1802–1811. [Google Scholar] [CrossRef]
- Köhler, K.; Schuchmann, H.P. Emulgiertechnik: Grundlagen, Verfahren und Anwendungen, 3rd ed.; Behr: Hamburg, Germany, 2012; ISBN 978-3-89947-869-3. [Google Scholar]
- Taylor, G. The formation of emulsions in definable fields of flow. Proc. R. Soc. Lond. A 1934, 146, 501–523. [Google Scholar] [CrossRef]
- Jiao, J.; Burgess, D.J. Multiple emulsion stability: Pressure balance and interfacial film strength. In Multiple Emulsions; Aserin, A., Ed.; John Wiley & Sons, Inc: Hoboken, NJ, USA, 2007; pp. 1–27. ISBN 9780470209264. [Google Scholar]
- Walstra, P.; Smulders, P.E. Emulsion formation. In Modern Aspects of Emulsion Science; Binks, B.P., Ed.; The Royal Society of Chemistry: London, UK, 1998; pp. 56–99. ISBN 978-0-85404-439-9. [Google Scholar]
- Feigl, K.; Baniabedalruhman, A.; Tanner, F.X.; Windhab, E.J. Numerical simulations of the breakup of emulsion droplets inside a spraying nozzle. Phys. Fluids 2016, 28, 123103. [Google Scholar] [CrossRef]
- Qin, K.; Tank, H.; Wilson, S.A.; Downer, B.; Liu, L. Controlling Droplet-Size Distribution Using Oil Emulsions in Agricultural Sprays. At. Sprays 2010, 20, 227–239. [Google Scholar] [CrossRef]






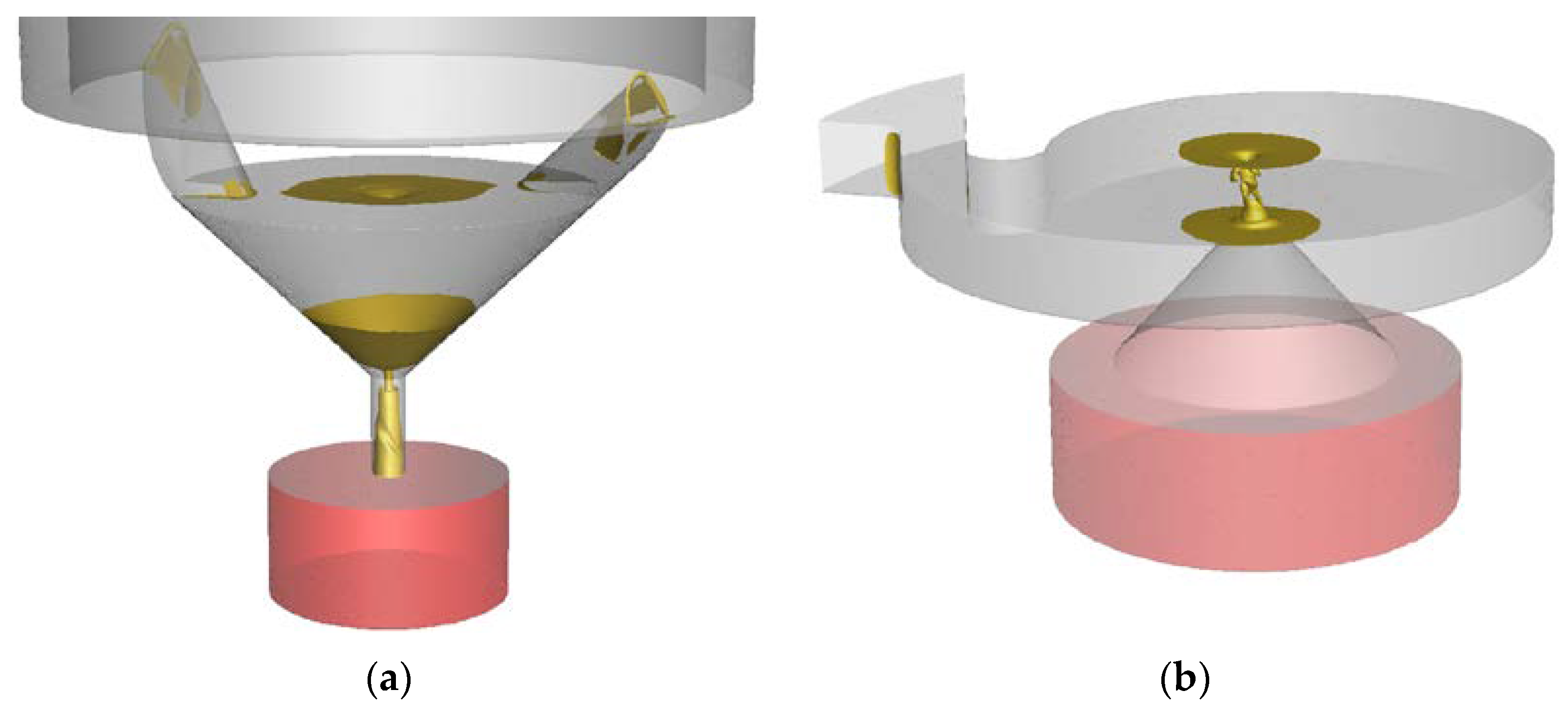
| Phase | µ (mPa∙s) | ρ (kg∙m−3) |
|---|---|---|
| Emulsion 1: 26.2 wt% MD | 10 | 1102 |
| Emulsion 2: 35.5 wt% MD | 35 | 1153 |
| Air | 0.018 | 1.15 |
| Parameter | Value | |||
|---|---|---|---|---|
| M1 | M2 | M3 | M4 | |
| Reference size (µm) | 17 | 16 | 15 | 14 |
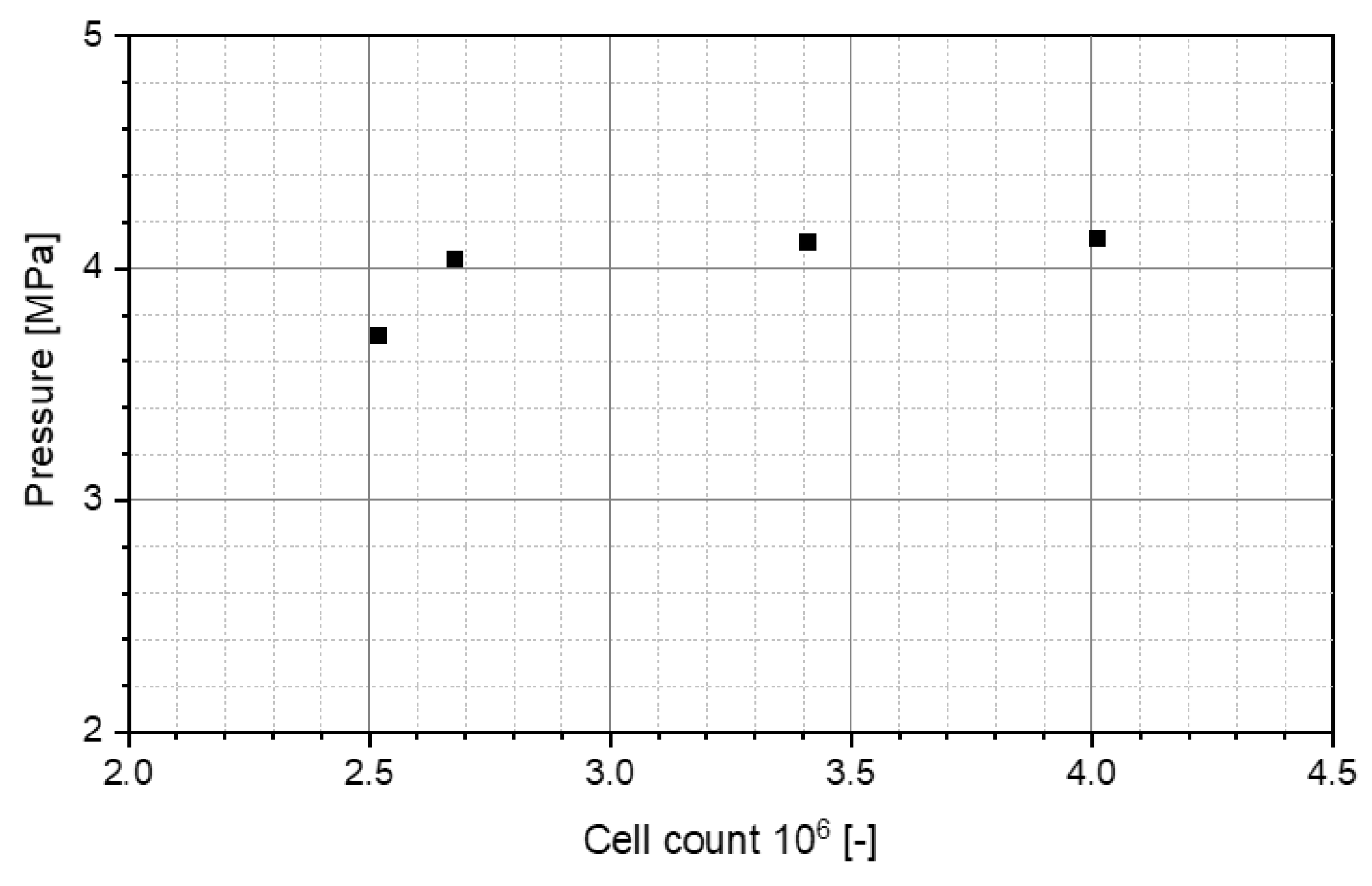
| Cell count (106) | 2.52 | 2.68 | 3.41 | 4.01 |
| Radial poly elements | 20 | 21 | 23 | 24 |
| Number of prism layers | 8 | |||
| Thickness (y+) of first layer | 0.6 µm (0.33) | |||
| Max. growth rate | 1.5 | |||
| µ (mPa·s) | pexp (MPa) | psim (MPa) | QL (L·min−1) | θsim (°) | θexp (°) 2 | uax (m/s) | urot (m/s) | S (−) |
|---|---|---|---|---|---|---|---|---|
| 10 | 5 | 4.2 | 0.26 | 66 | 48 ± 3 | 60 | 50 | 1.20 |
| 10 | 11.6 | 0.35 | 58 | 56 ± 2 | 80 | 60 | 1.33 | |
| 20 | 21.8 | 0.44 | 56 | 55 ± 2 | 120 | 80 | 1.50 | |
| 35 | 5 | 4.4 | 0.30 | - | 43 ± 1 | 80 | 25 | 3.20 |
| 10 | 8.8 | 0.44 | - | 40 ± 3 | 120 | 45 | 2.66 | |
| 20 | 13.1 | 0.53 | - | 46 ± 2 | 160 | 70 | 2.28 | |
| 20 1 | 20 | 0.65 | - | - | 190 | 100 | 1.9 |
| µ (mPa·s) | pexp (MPa) | psim (MPa) | QL (m3·s−1) | θsim (°) 1 | θexp (°) 1 | uax (m/s) | urot (m/s) | S (−) |
|---|---|---|---|---|---|---|---|---|
| 10 | 5 | 4.8 | 0.40 | 62 ± 2 | 61 ± 7 | 95 | 80 | 1.18 |
| 10 | 9.4 | 0.53 | 56 ± 6 | 51 ± 2 | 120 | 90 | 1.26 | |
| 20 | 17 | 0.66 | 55 ± 2 | 54 ± 1 | 125 | 105 | 1.25 | |
| 35 | 5 | 6 | 0.47 | - | 30 ± 3 | 100 | 40 | 2.50 |
| 10 | 13.5 | 0.70 | - | 56 ± 5 | 150 | 70 | 2.14 |
| Nozzle | µ (mPa·s) | pexp (MPa) | Inlet Ports | Outlet Channel | ||
|---|---|---|---|---|---|---|
| τmax (kPa) | ΔSSH (kPa·ms) | τmax (kPa) | ΔSSH (kPa·ms) | |||
| SK | 10 | 5 | 10.1 ± 7.7 | 0.43 ± 0.38 | 18.1 ± 2.7 | 0.20 ± 0.01 |
| 10 | 5.6 ± 1.2 | 0.29 ± 0.04 | 32.0 ± 2.2 | 0.22 ± 0.02 | ||
| 20 | 4.3 ± 3.0 | 0.08 ± 0.06 | 50.6 ± 0.1 | 0.21 ± 0.00 | ||
| 35 | 5 | 11.5 ± 8.0 | 1.79 ± 0.77 | 30.7 ± 5.6 | 0.49 ± 0.02 | |
| 10 | 27.5 ± 20.4 | 1.47 ± 0.70 | 51.8 ± 7.3 | 0.28 ± 0.07 | ||
| 20 | 27.9 ± 14.0 | 0.63 ± 0.77 | 70.4 ± 8.3 | 0.41 ± 0.10 | ||
| MiniSDX | 10 | 5 | 1.5 ± 0.7 | 0.74 ± 0.49 | 13.7 ± 0.4 | 0.16 ± 0.01 |
| 10 | 2.5 ± 0.2 | 0.48 ± 0.07 | 15.2 ± 4.1 | 0.14 ± 0.01 | ||
| 20 | 2.0 ± 0.6 | 0.40 ± 0.11 | 26.4 ± 2.4 | 0.10 ± 0.01 | ||
| 35 | 5 | 6.6 ± 5.7 | 0.32 ± 0.99 | 27.7 ± 13.7 | 0.20 ± 0.11 | |
| 10 | 5.0 ± 2.9 | 1.38 ± 0.23 | 52.5 ± 12.2 | 0.52 ± 0.24 | ||
| Nozzle | µ (mPa·s) | pexp (MPa) | Inlet Ports | Outlet Channel | ||
|---|---|---|---|---|---|---|
| δmax (kPa) | δavg (kPa) | δmax (kPa) | δavg (kPa) | |||
| SK | 10 | 5 | 8.55 ± 4.0 | 3.43 ± 2.1 | 54.0 ± 30.4 | 4.51 ± 0.8 |
| 10 | 10.5 ± 2.1 | 2.98 ± 1.1 | 39.4 ± 13.8 | 4.20 ± 0.3 | ||
| 20 | 8.28 ± 7.9 | 2.92 ± 1.9 | 152 ± 44.7 | 5.00 ± 0.3 | ||
| 35 | 5 | 32.4 ± 10.8 | 6.73 ± 2.6 | 144 ± 55.5 | 8.37 ± 1.6 | |
| 10 | 30.0 ± 18.0 | 4.59 ± 1.5 | 190 ± 156 | 9.11 ± 3.7 | ||
| 20 | 120 ± 76.5 | 13.5 ± 10.5 | 105 ± 0.1 | 9.27 ± 2.0 | ||
| MiniSDX | 10 | 5 | 4.98 ± 0.9 | 2.27 ± 0.4 | 102 ± 35.8 | 7.09 ± 1.3 |
| 10 | 31.1 ± 39.4 | 5.50 ± 2.2 | 93.1 ± 54.9 | 5.96 ± 2.3 | ||
| 20 | 9.65 ± 5.1 | 2.81 ± 0.8 | 56.6 ± 11.3 | 8.53 ± 4.0 | ||
| 35 | 5 | 14.9 ± 4.3 | 2.44 ± 0.1 | 88.5 ± 8.4 | 12.1 ± 2.9 | |
| 10 | 31.5 ± 19.5 | 3.69 ± 1.1 | 271 ± 180 | 20.1 ± 3.3 | ||
| µ (mPa·s) | pexp (MPa) | Inlet Ports | Swirl Chamber | Outlet Channel | ||||
|---|---|---|---|---|---|---|---|---|
| t10,0 (µs) | t50,0 (µs) | t90,0 (µs) | t10,0 (µs) | t50,0 (µs) | t90,0 (µs) | t50,0 (µs) | ||
| 10 | 5 | 99 | 134 | 317 | 504 | 821 | 1440 | 20 |
| 10 | 86 | 112 | 287 | 508 | 592 | 1100 | 14 | |
| 20 | 62 | 76 | 199 | 366 | 395 | 638 | 10 | |
| 35 | 5 | 83 | 124 | 626 | 569 | 1092 | 3610 | 14 |
| 10 | 60 | 83 | 379 | 432 | 599 | 1800 | 11 | |
| 20 | 47 | 63 | 137 | 457 | 560 | 866 | 10 | |
| µ (mPa·s) | pexp (MPa) | Inlet Ports | Swirl Chamber | Outlet Channel | ||||
|---|---|---|---|---|---|---|---|---|
| t10,0 (µs) | t50,0 (µs) | t90,0 (µs) | t10,0 (µs) | t50,0 (µs) | t90,0 (µs) | t50,0 (µs) | ||
| 10 | 5 | 869 | 1020 | 1390 | 404 | 533 | 717 | 22 |
| 10 | 801 | 880 | 1120 | 312 | 468 | 612 | 18 | |
| 20 | 548 | 628 | 909 | 205 | 239 | 268 | 13 | |
| 35 | 5 | 1090 | 1340 | 2150 | 817 | 1440 | 2750 | 16 |
| 10 | 689 | 859 | 1570 | 464 | 616 | 1570 | 10 | |
| Nozzle | µ (mPa·s) | pexp (MPa) | Shear Stress | Elongation Stress | Interm. xmax,ip (µm) | ||
|---|---|---|---|---|---|---|---|
| xmax (µm) | tcr (µs) | xmax (µm) | tcr (µs) | ||||
| SK | 10 | 5 | 3.3 | 3.2 | 1.7 | 25 | 1.7 E |
| 10 | 5.9 | 6.5 | 2.0 | 30 | 2.0 E | ||
| 20 | 7.7 | 9.1 | 2.0 | 31 | 2.0 E | ||
| 35 | 5 | 2.4 | 2.8 | 1.1 | 10 | 1.1 E | |
| 10 | 1.0 | 1.1 | 1.5 | 16 | 1.0 S | ||
| 20 | 1.0 | 1.1 | 0.5 | 4.7 | 0.5 E | ||
| MiniSDX | 10 | 5 | 23 | 178 | 2.6 | 49 | 2.6 E |
| 10 | 13 | 42 | 1.1 | 13 | 1.1 E | ||
| 20 | 17 | 69 | 2.1 | 33 | 2.1 E | ||
| 35 | 5 | 4.2 | 11 | 2.9 | 43 | 2.9 E | |
| 10 | 5.5 | 15 | 1.9 | 22 | 1.9 E | ||
| Nozzle | µ (mPa·s) | pexp (MPa) | Shear Stress | Elongation Stress | Final xmax,oc (µm) | Exp. x90,3 (µm) | ||||
|---|---|---|---|---|---|---|---|---|---|---|
| xmax (µm) | tcr (µs) | tadj,cr (µs) | xmax (µm) | tcr (µs) | tadj,cr (µs) | |||||
| SK | 10 | 5 | 1.8 | — 1 | 16 | 1.6 | — 1 | — 1 | 1.8 S | 2.6 |
| 10 | 1.0 | 8.8 | 3.0 | 1.7 | — 1 | — 1 | 1.0 S | 1.6 | ||
| 20 | 0.7 | 2.3 | 1.5 | 1.4 | — 1 | — 1 | 0.7 S | 1.0 | ||
| 35 | 5 | 0.9 | — 1 | 8.5 | 0.8 | — 1 | — 1 | 0.9 S | 3.0 | |
| 10 | 0.5 | 33 2 | 2.4 | 0.8 | — 1 | — 1 | 0.5 S | 1.7 | ||
| 20 | 0.4 | — 1 | 2.6 | 0.8 | — 1 | — 1 | 0.4 S | 1.2 | ||
| MiniSDX 3 | 10 | 5 | 2.4 | — 1 | 14 | 1.0 | — 1 | — 1 | 2.4 S | — |
| 10 | 2.2 | — 1 | — 1 | 1.2 | — 1 | — 1 | 1.1 E 4 | — | ||
| 20 | 1.3 | 24 2 | 4.0 | 0.8 | — 1 | — 1 | 1.3 S | — | ||
| 35 | 5 | 1.1 | 5.6 | 3.0 | 0.6 | — 1 | 14 | 0.6 E | — | |
| 10 | 0.6 | 2.2 | 1.5 | 0.4 | — 1 | 7.5 | 0.4 E | — | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ballesteros Martínez, M.Á.; Gaukel, V. Using Computation Fluid Dynamics to Determine Oil Droplet Breakup Parameters during Emulsion Atomization with Pressure Swirl Nozzles. Fluids 2023, 8, 277. https://doi.org/10.3390/fluids8100277
Ballesteros Martínez MÁ, Gaukel V. Using Computation Fluid Dynamics to Determine Oil Droplet Breakup Parameters during Emulsion Atomization with Pressure Swirl Nozzles. Fluids. 2023; 8(10):277. https://doi.org/10.3390/fluids8100277
Chicago/Turabian StyleBallesteros Martínez, Miguel Ángel, and Volker Gaukel. 2023. "Using Computation Fluid Dynamics to Determine Oil Droplet Breakup Parameters during Emulsion Atomization with Pressure Swirl Nozzles" Fluids 8, no. 10: 277. https://doi.org/10.3390/fluids8100277
APA StyleBallesteros Martínez, M. Á., & Gaukel, V. (2023). Using Computation Fluid Dynamics to Determine Oil Droplet Breakup Parameters during Emulsion Atomization with Pressure Swirl Nozzles. Fluids, 8(10), 277. https://doi.org/10.3390/fluids8100277