Impact of the Dissipation on the Nonlinear Interactions and Turbulence of Gravity-Capillary Waves
Abstract
1. Introduction
2. Theory of Surface Waves Propagating at an Air/Water Interface
2.1. Free-Surface Problem

2.2. Potential Flow Approximation
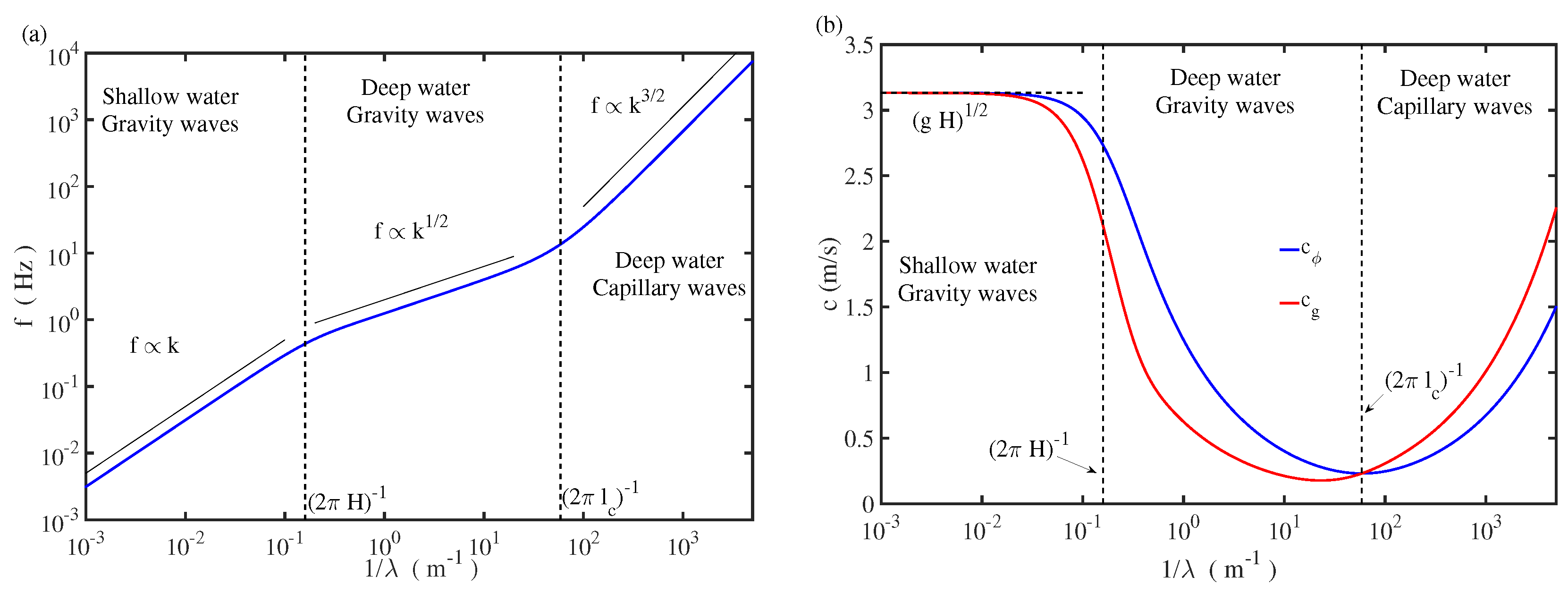
2.3. Linear Water Wave Theory
2.4. Hamiltonian Formulation of Water Waves
2.5. Nonlinearities in the Propagation of Surface Waves
- the convective acceleration in the Navier–Stokes equation, leading to the term in the dynamic boundary condition at the free surface (Equation (12));
- the square of the deformation of the free surface involved in the free-surface mean curvature ;
- the product in the kinematic boundary condition (Equation (5)), where is the horizontal gradient (along x and y). At first order,
2.6. Resonant Three-Wave Interactions
2.7. Non-Resonant Three-Wave Interactions
2.8. A Few Words on Some Other Effects of Nonlinearities
3. Linear Dissipation of Surface Waves
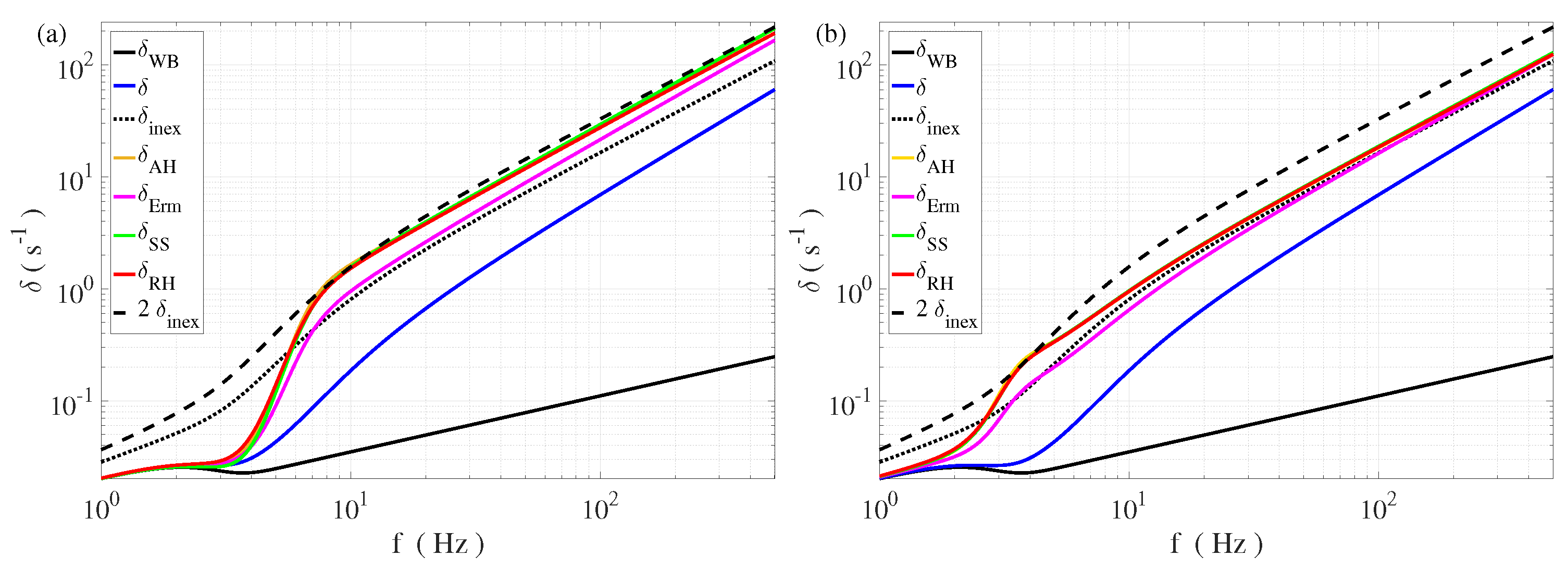
3.1. Viscous Decay Rates for a Clean Free Surface
- the linearized kinematic boundary condition, , taken at ;
- the linearized dynamic boundary condition for normal stresses taken at :
- the linearized dynamic boundary condition for tangential stresses taken at :
3.2. The Problem of the Surface Contamination

3.3. Meniscus Dissipation
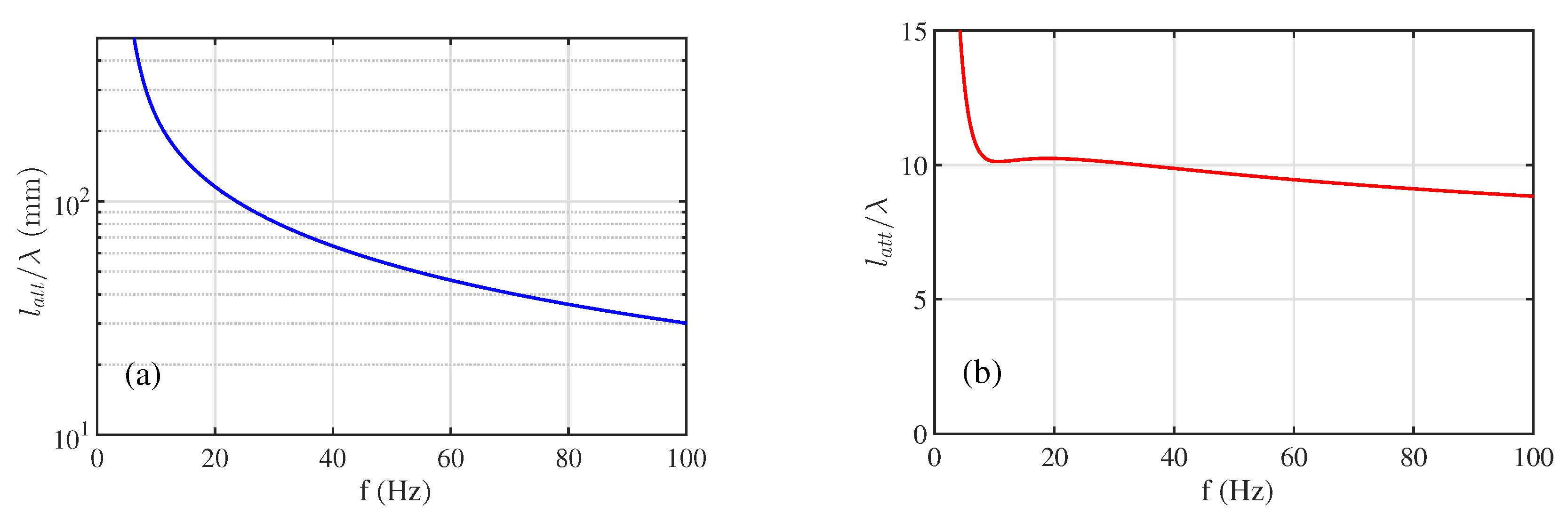
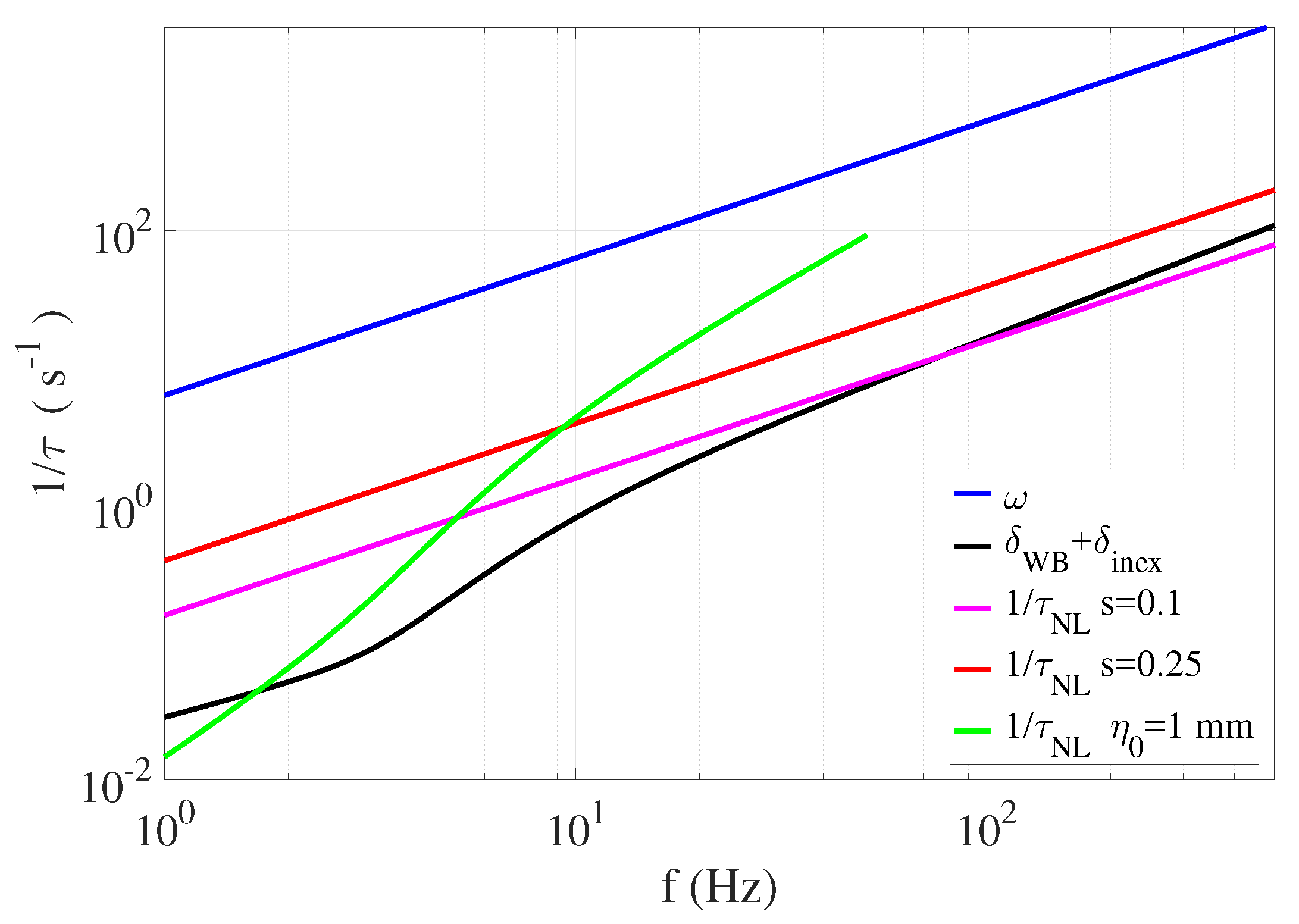
4. Orders of Magnitude of the Damping of Gravity–Capillary Waves and Consequences for Time Scale Separation
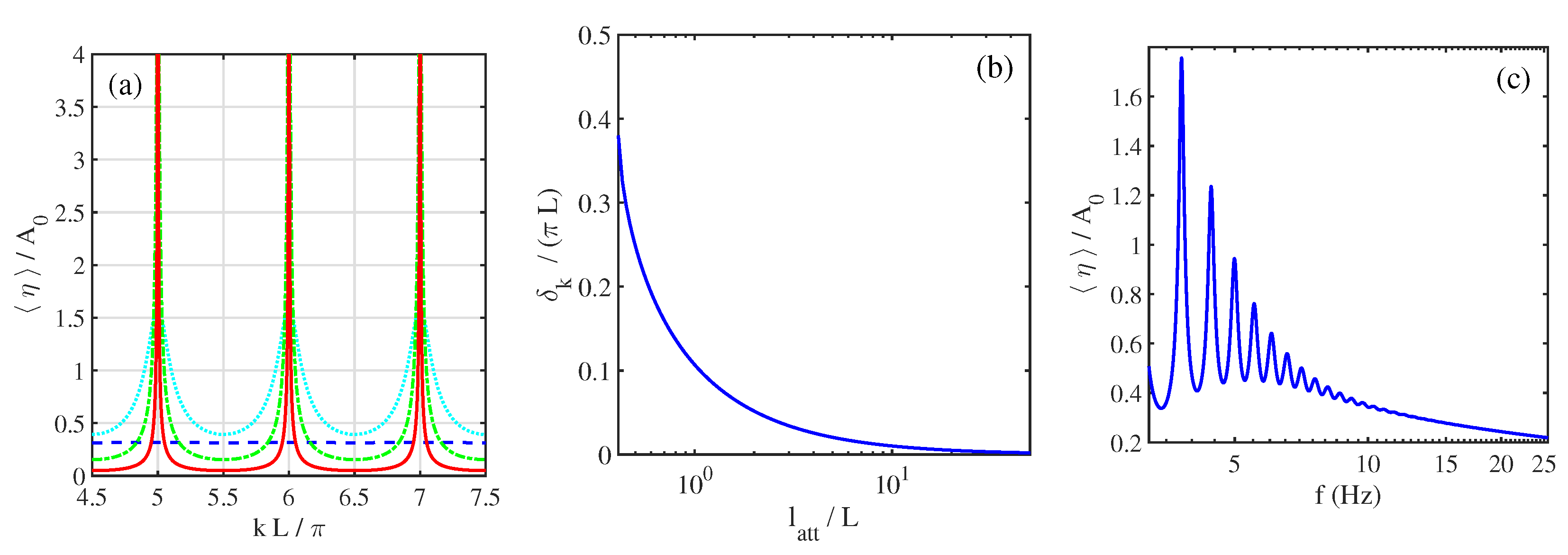
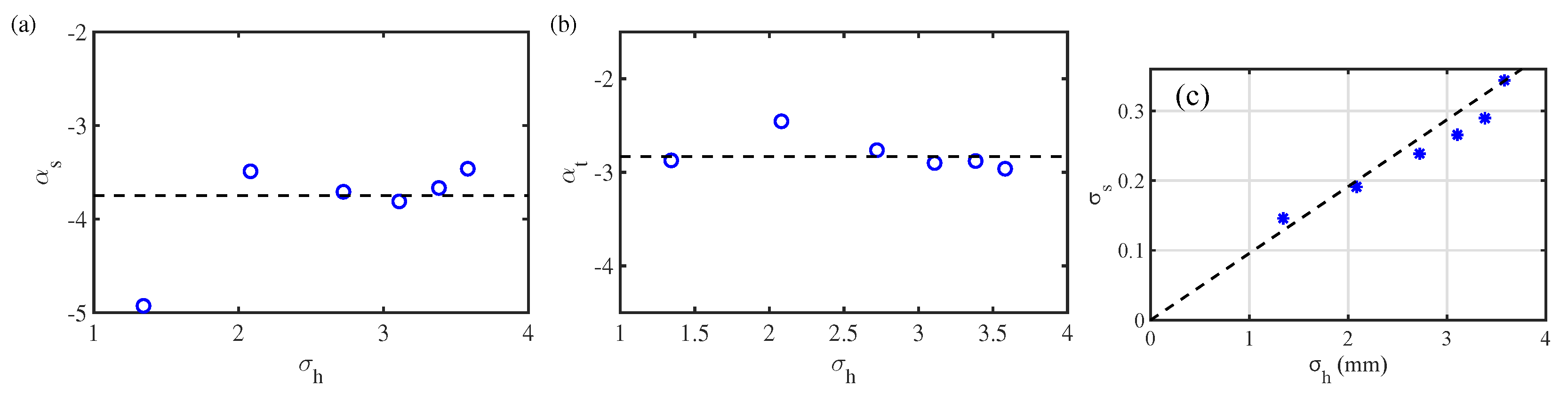
5. Consequences of Wave Dissipation on Finite Size Effects
6. Consequences of Dissipation on Three-Wave Interactions between Surface Waves
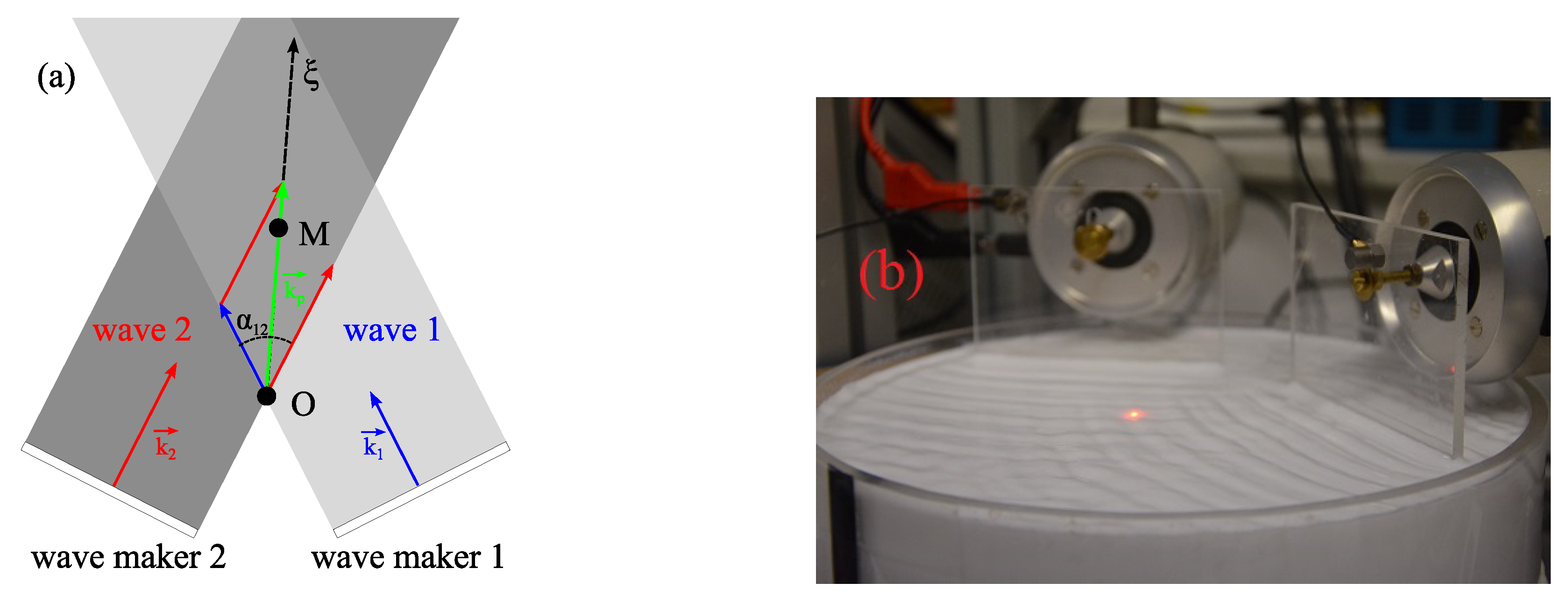
6.1. Experimental Study of Three-Wave Interactions of Waves
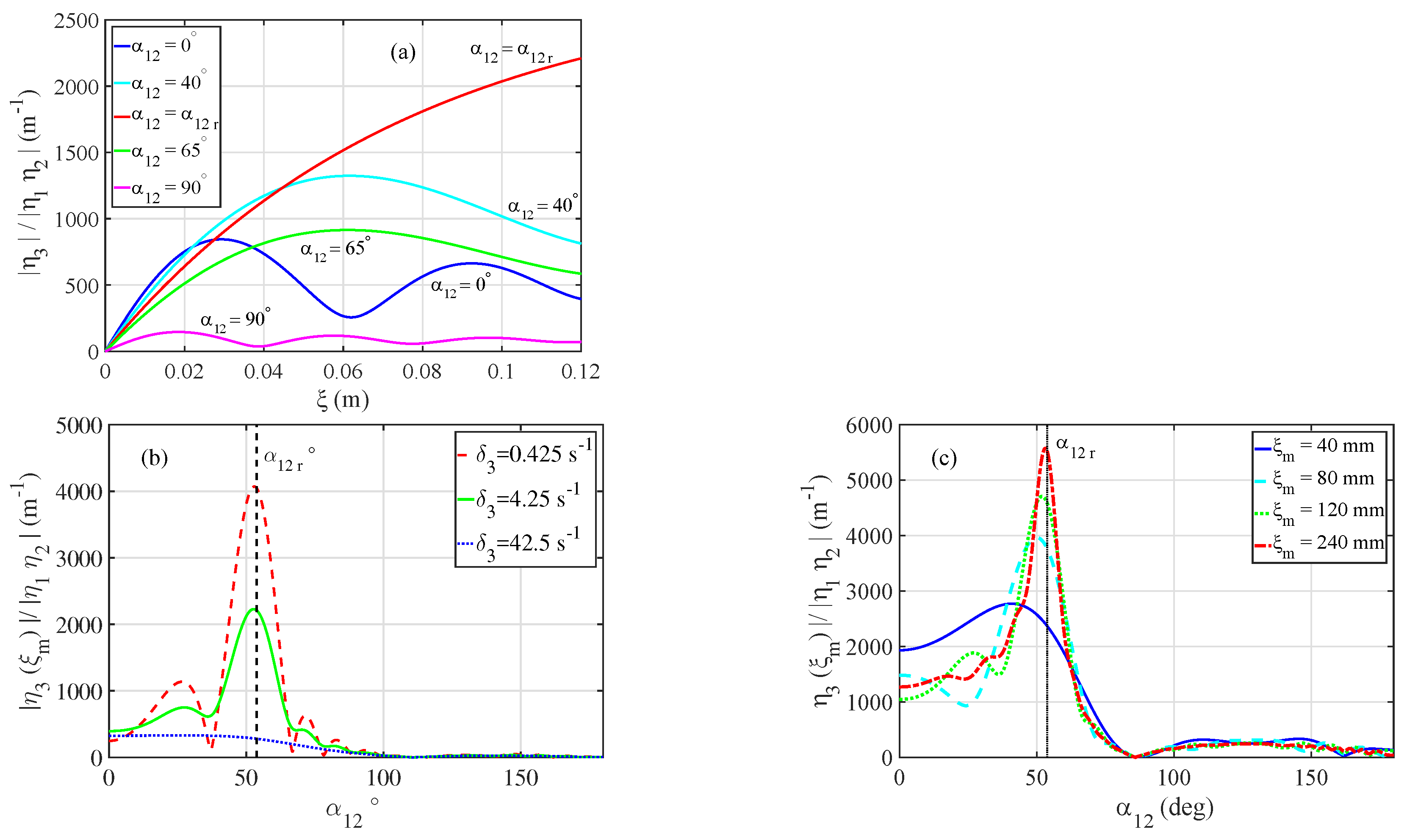
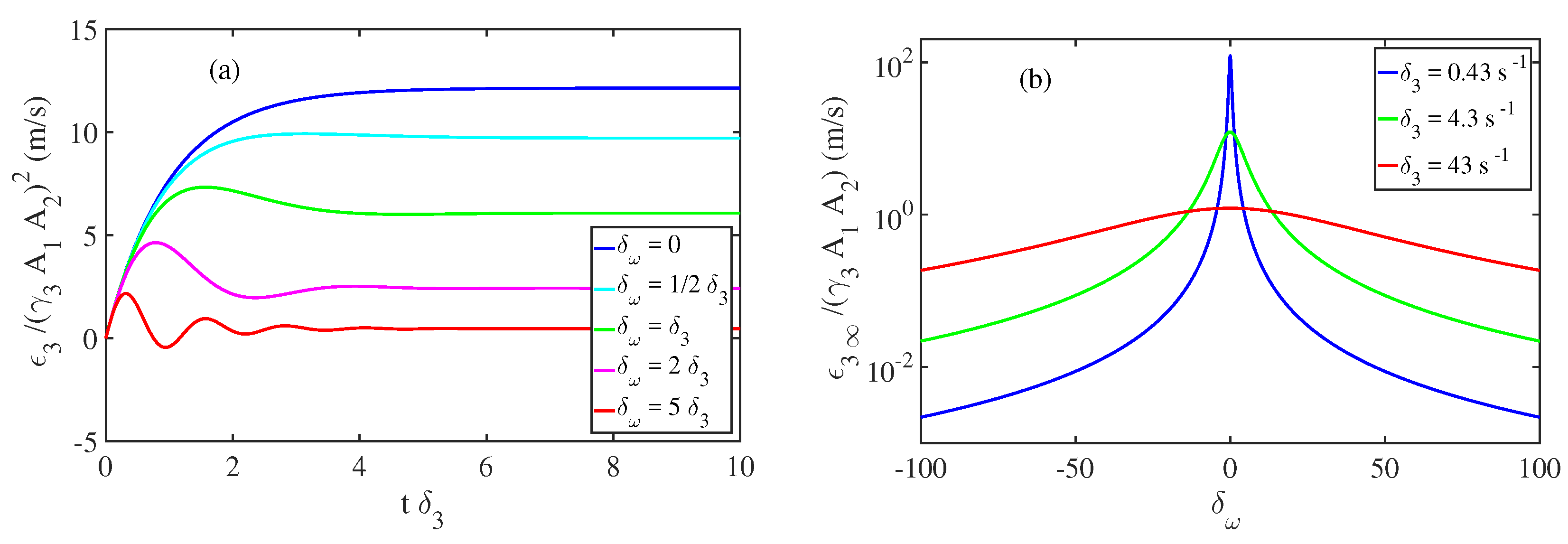
6.2. Forced Three-Wave Interactions of Gravity–Capillary Waves
6.3. Energy Flux for a Non-Resonant Three-Wave Interaction
7. Consequences of Dissipation on the Capillary Wave Turbulence
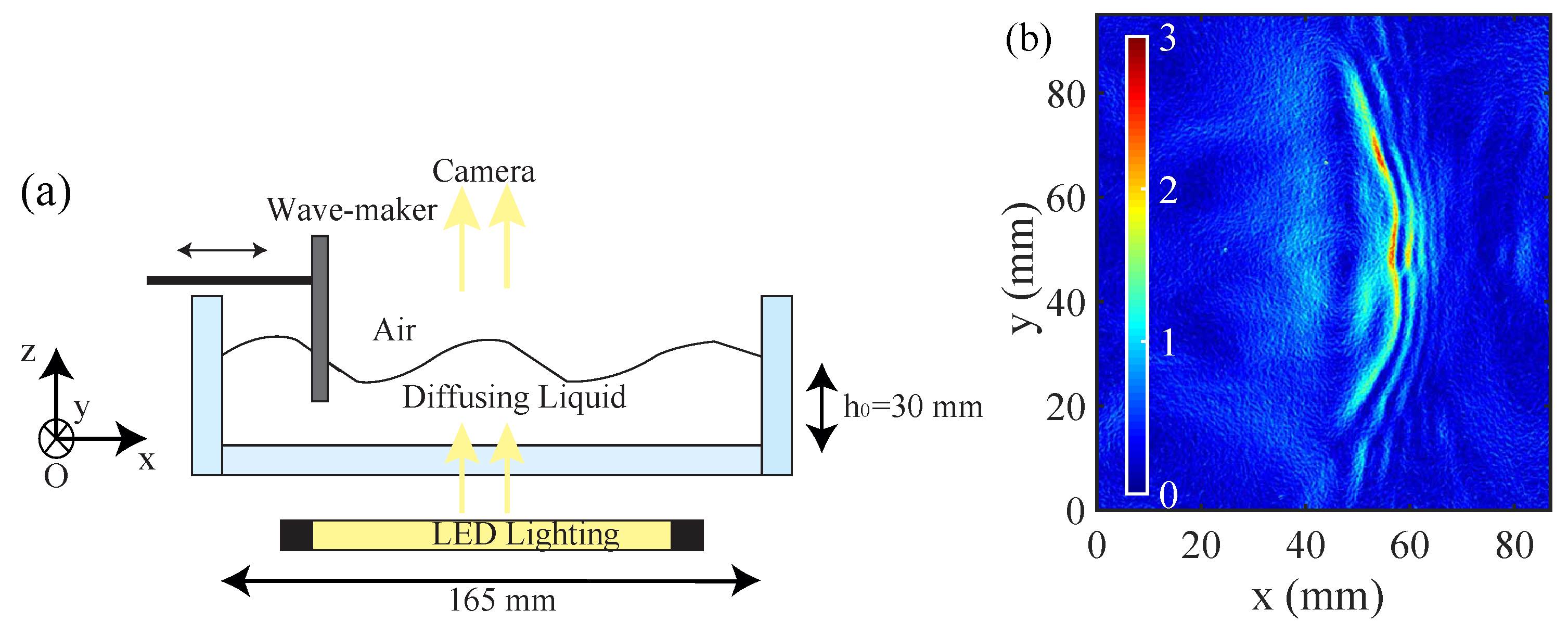
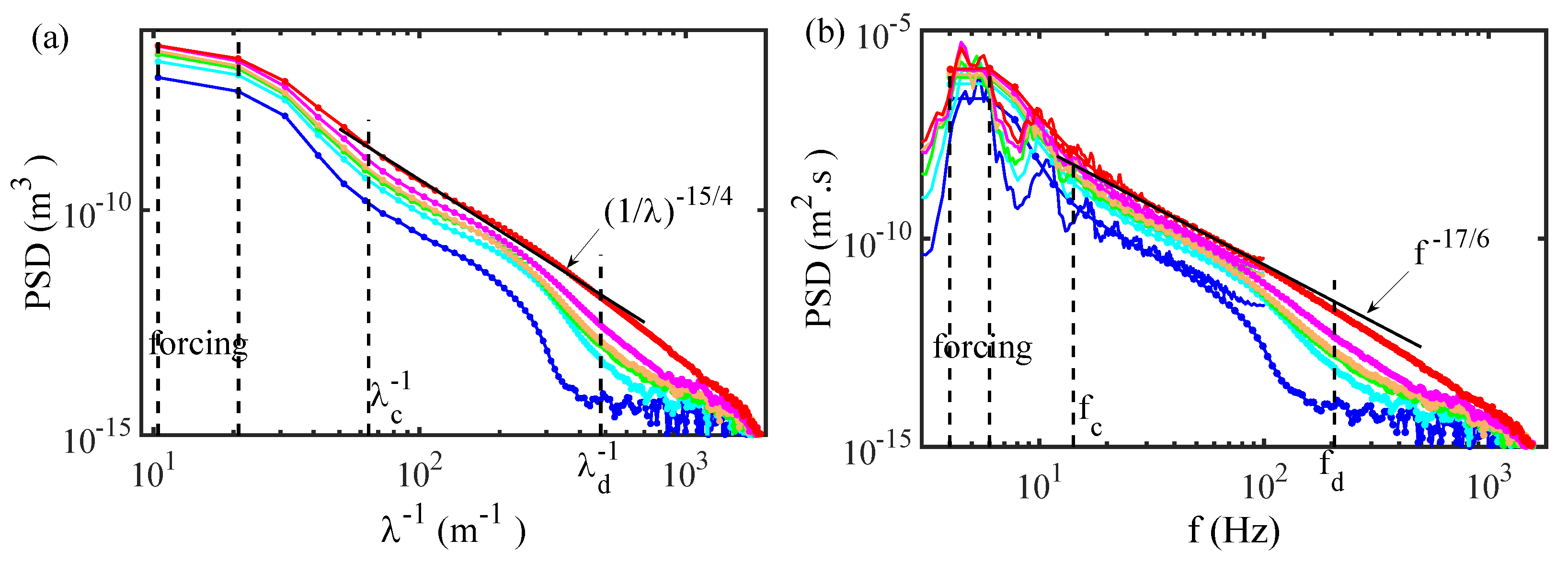
7.1. Capillary Wave Turbulence Forced by Gravity Waves
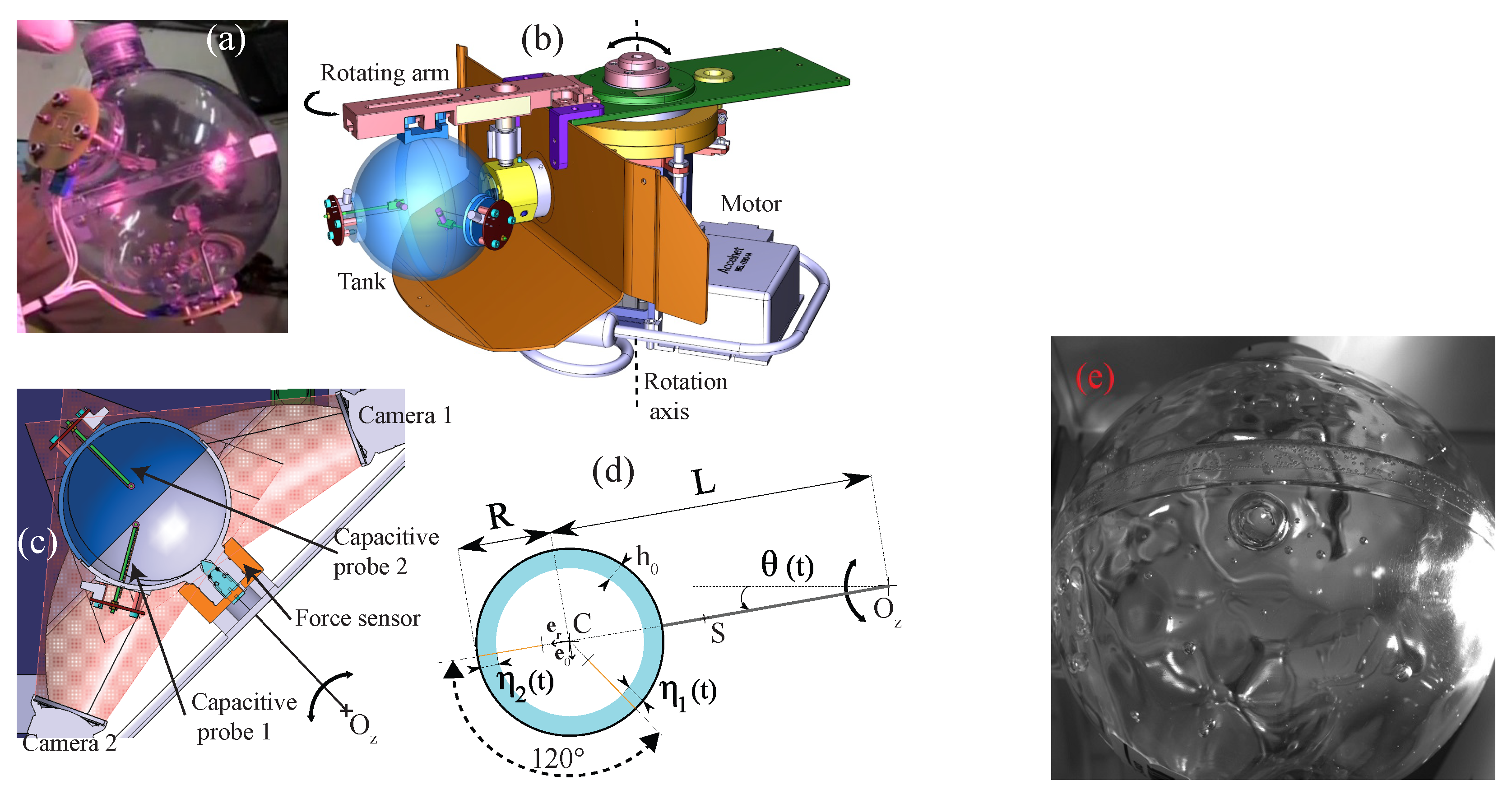
7.2. Turbulence of Pure Capillary Waves in Microgravity
7.3. Discussion
8. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Feynman, R.P.; Leighton, R.B.; Sands, M. The Feynman Lectures on Physics, Volume I: Mainly Mechanics, Radiation, and Heat; Addison-Wesley: Reading, MA, USA, 1964. [Google Scholar]
- Bühler, O. Waves and Mean Flow, 2nd ed.; Cambridge University Press: Cambridge, UK, 2014. [Google Scholar]
- Philipps, O.M. Wave interactions—The evolution of an idea. J. Fluid Mech. 1981, 106, 21–227. [Google Scholar] [CrossRef]
- Craik, A.D.D. Wave Interactions and Fluid Flows; Cambridge University Press: Cambridge, UK, 1986. [Google Scholar]
- Hammack, J.L.; Henderson, D.M. Resonant interactions among surface water waves. Annu. Rev. Fluid Mech. 1993, 25, 55–97. [Google Scholar] [CrossRef]
- Philipps, O.M. On the dynamics of unsteady gravity waves of finite amplitude. Part 1. The elementary interactions. J. Fluid Mech. 1960, 9, 193–217. [Google Scholar] [CrossRef]
- Longuet-Higgins, M.S. Resonant interactions between two trains of gravity waves. J. Fluid Mech. 1962, 12, 321–332. [Google Scholar] [CrossRef]
- McGoldrick, L.F. Resonant interactions among capillary-gravity waves. J. Fluid Mech. 1965, 21, 305–331. [Google Scholar] [CrossRef]
- Hasselmann, K. Nonlinear interactions treated by the methods of theoretical physics (with application to the generation of waves by wind). Proc. R. Soc. A 1967, 299, 77–100. [Google Scholar] [CrossRef]
- Janssen, P. The Interaction of Ocean Waves and Wind; Cambridge University Press: New York, NY, USA, 2004. [Google Scholar]
- Boyd, R.W. Nonlinear Optics; Academic Press: Berlin, Germany, 2008. [Google Scholar]
- Zakharov, V.E.; L’vov, V.; Falkovich, G. Kolmogorov Spectra of Turbulence; Springer: Berlin/Heidelberg, Germany, 1992. [Google Scholar]
- Nazarenko, S. Wave Turbulence; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- McGoldrick, L.F. An experiment on second-order capillary gravity resonant wave interactions. J. Fluid Mech. 1970, 40, 251. [Google Scholar] [CrossRef]
- Henderson, D.M.; Hammack, J.L. Experiments on ripple instabilities, Part 1. Resonant Triads. J. Fluid Mech. 1987, 184, 15–41. [Google Scholar] [CrossRef]
- Szeri, A.J. Capillary waves and air-sea gas transfer. J. Fluid Mech. 1997, 332, 341–358. [Google Scholar] [CrossRef]
- Saylor, J.R.; Handler, R.A. Gas transport across an air/water interface populated with capillary waves. Phys. Fluids 1997, 9, 2529. [Google Scholar] [CrossRef]
- Hwang, P.; Burrage, D.M.; Wang, D.W.; Wesson, J.C. Ocean Surface Roughness Spectrum in High Wind Condition for Microwave Backscatter and Emission Computations. J. Atmos. Ocean. Technol. 2013, 30, 2168–2187. [Google Scholar] [CrossRef]
- Zhang, X. Enhanced dissipation of short gravity and gravity capillary waves due to parasitic capillaries. Phys. Fluids 2002, 14, 81. [Google Scholar] [CrossRef]
- Tsai, W.T.; Hung, L.P. Enhanced energy dissipation by parasitic capillaries on short gravity-capillary waves. J. Phys. Oceanogr. 2010, 40, 2435–2450. [Google Scholar] [CrossRef]
- Caulliez, G. Dissipation regimes for short wind waves. J. Geophys. Res. Ocean. 2013, 118, 672–684. [Google Scholar] [CrossRef]
- Melville, W.K.; Fedorov, A. The equilibrium dynamics and statistics of gravity-capillary waves. J. Fluid Mech. 2015, 767, 449–466. [Google Scholar] [CrossRef]
- Deike, L.; Popinet, S.; Melville, W.K. Capillary effects on wave breaking. J. Fluid Mech. 2015, 769, 541–569. [Google Scholar] [CrossRef]
- Lamb, H. Hydrodynamics; Springer: Berlin/Heidelberg, Germany, 1932. [Google Scholar]
- Whitham, G.B. Linear and Nonlinear Waves; Wiley-Interscience: Hoboken, NJ, USA, 1999. [Google Scholar]
- Mei, C.C.; Stiassnie, M.; Yue, D.K.P. Theory and Applications of Surface Waves; World Scientific Publishing: Singapore, 2005. [Google Scholar]
- Dias, F.; Kharif, C. Nonlinear gravity and capillary-gravity waves. Annu. Rev. Fluid Mech. 1999, 31, 301–346. [Google Scholar] [CrossRef]
- Deike, L. Etudes Expérimentales et Numériques de la Turbulence D’ondes de Surface. Ph.D. Thesis, Université Paris Diderot—Paris 7, Paris, France, 2013. [Google Scholar]
- Michel, G. Parois et Ondes de Surface: Dissipation, Effet Doppler et Interactions Non Linéaires. Ph.D. Thesis, PSL Reseach University, École Normale Supérieure, Paris, France, 2017. [Google Scholar]
- Segur, H. Lecture 1: Introduction to linear and non-linear waves. In Proceedings of the Nonlinear Waves; Buhler, O., Helfrich, K., Eds.; Woods Hole Oceanographic Institution: Woods Hole, MA, USA, 2009. [Google Scholar]
- Dias, F.; Dyachenko, A.I.; Zakharov, V.E. Theory of weakly damped free-surface flows: A new formulation based on potential flow solutions. Phys. Lett. A 2008, 372, 1297–1302. [Google Scholar] [CrossRef]
- Rajan, G.K.; Henderson, D.M. Linear waves at a surfactant-contaminated interface separating two fluids: Dispersion and dissipation of capillary-gravity waves. Phys. Fluids 2018, 30, 072104. [Google Scholar] [CrossRef]
- Jamin, T.; Gordillo, L.; Ruiz-Chavarría, G.; Berhanu, M.; Falcon, E. Experiments on generation of surface waves by an underwater moving bottom. Proc. R. Soc. A 2015, 471, 245–259. [Google Scholar] [CrossRef]
- Crapper, G.D. An exact solution for progressive capillary waves of arbitrary amplitude. J. Fluid Mech. 1957, 2, 532. [Google Scholar] [CrossRef]
- Jamin, T. Interactions Entre Ondes de Surface Et Écoulements Hydrodynamiques. Ph.D. Thesis, Université Paris Diderot, Paris, France, 2016. [Google Scholar]
- Guyon, E.; Hulin, J.P.; Petit, L.; Mitescu, C.D. Physical Hydrodynamics, 2nd ed.; Oxford University Press: Oxford, UK, 2015. [Google Scholar]
- Luke, J.C. A variational principle for a fluid with a free surface. J. Fluid Mech. 1967, 27, 395–397. [Google Scholar] [CrossRef]
- Zakharov, V.E. Stability of periodic waves of finite amplitude on the surface of a deep fluid. J. Appl. Mech. Tech. Phys. 1968, 9, 190. [Google Scholar] [CrossRef]
- Luke, J.C. On Hamilton’s principle for surface waves. J. Fluid Mech. 1977, 83, 153–158. [Google Scholar] [CrossRef]
- Pushkarev, A.N.; Zakharov, V.E. Turbulence of capillary waves—Theory and numerical simulation. Phys. D 2000, 135, 98. [Google Scholar] [CrossRef]
- Case, K.M.; Chiu, S.C. Three-wave resonant interactions of gravity-capillary waves. Phys. Fluids 1977, 20, 742–745. [Google Scholar] [CrossRef]
- Cazaubiel, A.; Haudin, F.; Falcon, E.; Berhanu, M. Forced three-wave interactions of capillary-gravity surface waves. Phys. Rev. Fluids 2019, 4, 074803. [Google Scholar] [CrossRef]
- Simmons, W.F. A variational method for week resonant wave interactions. Proc. R. Soc. A 1969, 309, 551–575. [Google Scholar] [CrossRef]
- Aubourg, Q.; Mordant, N. Investigation of resonances in gravity-capillary wave turbulence. Phys. Rev. Fluids 2016, 1, 023701. [Google Scholar] [CrossRef]
- Aubourg, Q.; Campagne, A.; Peureux, C.; Ardhuin, F.; Sommeria, J.; Viboud, S.; Mordant, N. Three-wave and four-wave interactions in gravity wave turbulence. Phys. Rev. Fluids 2017, 2, 114802. [Google Scholar] [CrossRef]
- Longuet-Higgins, M.S. The Generation of capillary waves by steep gravity waves. J. Fluid Mech. 1963, 16, 138–159. [Google Scholar] [CrossRef]
- Perlin, M.; Schultz, W.W. Capillary effects on surface waves. Annu. Rev. Fluid Mech. 2000, 32, 241–274. [Google Scholar] [CrossRef]
- Fedorov, A.; Melville, W.K. Nonlinear gravity-capillary waves with forcing and dissipation. J. Fluid Mech. 1998, 354, 1–42. [Google Scholar] [CrossRef]
- Landau, L.D.; Lifshitz, E.M. Fluid Mechanics, 2nd ed.; Course of Theoretical Physics; Butterworth-Heinemann: Oxford, UK, 1987; Volume 6. [Google Scholar]
- Dore, B.D. Some effects of the air-water interface on gravity waves. Geophys. Astrophys. Fluid Dyn. 1978, 10, 213–230. [Google Scholar] [CrossRef]
- van Dorn, W.G. Boundary dissipation of oscillatory waves. J. Fluid Mech. 1966, 24, 769. [Google Scholar] [CrossRef]
- Henderson, D.M.; Lee, R.C. Laboratory generation and propagation of ripples. Phys. Fluids 1986, 29, 619–624. [Google Scholar] [CrossRef]
- Henderson, D.M.; Segur, H. The role of dissipation in the evolution of ocean swell. J. Geophys. Res. Ocean. 2013, 118, 5074. [Google Scholar] [CrossRef]
- Przadka, A.; Cabane, B.; Pagneux, V.; Maurel, A.; Petitjeans, P. Fourier transform profilometry for water waves: How to achieve clean water attenuation with diffusive reflection at the water surface ? Exp. Fluids 2012, 52, 519–527. [Google Scholar] [CrossRef]
- Campagne, A.; Hassaini, R.; Redor, I.; Sommeria, J.; Viboud, S.; Mordant, N. Impact of dissipation on the energy spectrum of experimental turbulence of gravity surface waves. Phys. Rev. Fluids 2018, 3, 044801. [Google Scholar] [CrossRef]
- Miles, J.W. Surface-wave damping in closed basins. Proc. R. Soc. London. Ser. A 1967, 297, 297–459. [Google Scholar] [CrossRef]
- Lucassen, J. Longitudinal capillary waves. Part 1.—Theory. Trans. Faraday Soc. 1968, 64, 2221–2229. [Google Scholar] [CrossRef]
- Hühnerfuss, H.; Lange, P.A.; Walter, W. Relaxation effects in monolayers and their contribution to water wave damping: II. The Marangoni phenomenon and gravity wave attenuation. J. Colloid Interface Sci. 1985, 108, 442–450. [Google Scholar] [CrossRef]
- Alpers, W.; Hühnerfuss, H. Damping of Ocean Waves by Surface Films: A New Look at an Old Problem. J. Geophys. Res. 1989, 94, 6251–6265. [Google Scholar] [CrossRef]
- Ermakov, S. Resonance damping of gravity-capillary waves on the water surface covered with a surface-active film. Izv. Atmos. Ocean. Phys. 2003, 39, 624–628. [Google Scholar]
- Sutherland, G.; Halsne, T.; Rabault, J.; Jensen, A. The attenuation of monochromatic surface waves due to the presence of an inextensible cover. Wave Motion 2017, 68, 88–96. [Google Scholar] [CrossRef]
- Mass, J.T.; Milgram, J.H. Dynamic behavior of natural sea surfactant film. J. Geophys. Res. Ocean. 1998, 103, 15695–15715. [Google Scholar] [CrossRef]
- Haudin, F.; Cazaubiel, A.; Deike, L.; Jamin, T.; Falcon, E.; Berhanu, M. Experimental study of three-wave interactions among capillary-gravity surface waves. Phys. Rev. E 2016, 93, 043110. [Google Scholar] [CrossRef]
- Henderson, D.M. Effects of surfactants on Faraday-wave dynamics. J. Fluid Mech. 1998, 365, 89–107. [Google Scholar] [CrossRef]
- Kidambi, R. Capillary damping of inviscid surface waves in a circular cylinder. J. Fluid Mech. 2009, 627, 323–340. [Google Scholar] [CrossRef]
- Viola, F.; Brun, P.T.; Gallaire, F. Capillary hysteresis in sloshing dynamics: A weakly nonlinear analysis. J. Fluid Mech. 2018, 837, 788–818. [Google Scholar] [CrossRef]
- Viola, F.; Gallaire, F. Theoretical framework to analyze the combined effect of surface tension and viscosity on the damping rate of sloshing waves. Phys. Rev. Fluids 2018, 3, 094801. [Google Scholar] [CrossRef]
- Monsalve, E.; Maurel, A.; Pagneux, V.; Petitjeans, P. Space-time-resolved measurements of the effect of pinned contact line on the dispersion relation of water waves. Phys. Rev. Fluids 2022, 7, 014802. [Google Scholar] [CrossRef]
- Michel, G.; Petrelis, F.; Fauve, S. Acoustic Measurement of Surface Wave Damping by a Meniscus. Phys. Rev. Lett. 2016, 116, 174301. [Google Scholar] [CrossRef] [PubMed]
- Douady, S. Experimental study of the Faraday instability. J. Fluid Mech. 1990, 221, 383–409. [Google Scholar] [CrossRef]
- Berhanu, M.; Falcon, E.; Deike, L. Turbulence of capillary waves forced by steep gravity waves. J. Fluid Mech. 2018, 850, 803. [Google Scholar] [CrossRef]
- Hassaini, R.; Mordant, N. Confinement effects on gravity-capillary wave turbulence. Phys. Rev. Fluids 2018, 3, 094805. [Google Scholar] [CrossRef]
- Wright, W.B.; Budakian, R.; Putterman, S.J. Diffusing Light Photography of Fully Developed Isotropic Ripple Turbulence. Phys. Rev. Lett. 1996, 76, 4528. [Google Scholar] [CrossRef]
- Zakharov, V.E. Weak turbulence in media with a decay spectrum. J. Appl. Mech. Tech. Phys. 1965, 6, 22–24. [Google Scholar] [CrossRef]
- Zakharov, V.E.; Filonenko, N.N. Weak turbulence of capillary waves. J. Appl. Mech. Tech. Phys. 1967, 8, 37. [Google Scholar] [CrossRef]
- Zakharov, V.E.; Filonenko, N.N. Energy spectrum for stochastic oscillations of the surface of a liquid. Sov. Phys. Dokl. 1967, 11, 881. [Google Scholar]
- Zakharov, V.E. On the spectrum of turbulence in plasma without magnetic field. J. Exp. Theor. Phys. 1967, 24, 455–459. [Google Scholar]
- Benney, D.J.; Saffman, P.G. Nonlinear interactions of random waves in a dispersive medium. Proc. R. Soc. A 1966, 289, 301–320. [Google Scholar] [CrossRef]
- Benney, D.J.; Newell, A.C. Random wave closures. Stud. Appl. Math. 1969, 48, 29–53. [Google Scholar] [CrossRef]
- Hasselmann, K. On the non-linear energy transfer in a gravity-wave spectrum, part 1: General theory. J. Fluid Mech. 1962, 12, 481. [Google Scholar] [CrossRef]
- Newell, A.; Rumpf, B. Wave Turbulence. Annu. Rev. Fluid Mech 2011, 43, 59. [Google Scholar] [CrossRef]
- Nazarenko, S.; Lukaschuk, S. Wave Turbulence on Water Surface. Annu. Rev. Condens. Matter Phys. 2016, 7, 61. [Google Scholar] [CrossRef]
- Falcon, E.; Mordant, N. Experiments in Surface Gravity–Capillary Wave Turbulence. Annu. Rev. Fluid Mech. 2022, 54, 1–25. [Google Scholar] [CrossRef]
- Galtier, S. Wave turbulence: The case of capillary waves. Geophys. Astrophys. Fluid Dyn. 2021, 115, 234–257. [Google Scholar] [CrossRef]
- Connaughton, C.; Nazarenko, S.; Newell, A. Dimensional analysis and weak turbulence. Phys. D 2003, 184, 86–97. [Google Scholar] [CrossRef][Green Version]
- Zakharov, V.E. Energy balance in a wind-driven sea. Phys. Scr. 2010, T142, 014052. [Google Scholar] [CrossRef]
- Pan, Y.; Yue, D.K.P. Understanding discrete capillary-wave turbulence using a quasi-resonant kinetic equation. J. Fluid Mech. 2017, 816, R1. [Google Scholar] [CrossRef]
- Michel, G.; Pétrélis, F.; Fauve, S. Observation of thermal equilibrium in capillary wave turbulence. Phys. Rev. Lett. 2017, 118, 144502. [Google Scholar] [CrossRef] [PubMed]
- Pushkarev, A.N.; Zakharov, V.E. Turbulence of Capillary Waves. Phys. Rev. Lett. 1996, 76, 3320. [Google Scholar] [CrossRef] [PubMed]
- Pan, Y.; Yue, D.K.P. Direct Numerical Investigation of Turbulence of Capillary Waves. Phys. Rev. Lett. 2014, 113, 094501. [Google Scholar] [CrossRef]
- Pan, Y.; Yue, D.K.P. Decaying capillary wave turbulence under broad-scale dissipation. J. Fluid Mech. 2015, 780, R1. [Google Scholar] [CrossRef]
- Deike, L.; Fuster, D.; Berhanu, M.; Falcon, E. Direct Numerical Simulations of Capillary Wave Turbulence. Phys. Rev. Lett. 2014, 112, 234501. [Google Scholar] [CrossRef]
- Henry, E.; Alstrom, P.; Levinsen, M.T. Prevalence of weak turbulence in strongly driven surface ripples. EPL Europhys. Lett. 2000, 52, 27. [Google Scholar] [CrossRef]
- Brazhnikov, M.Y.; Kolmakov, G.V.; Levchenko, A.A.; Mezhov-Deglin, L.P. Observation of capillary turbulence on the water surface in a wide range of frequencies. EPL Europhys. Lett. 2002, 58, 510. [Google Scholar] [CrossRef]
- Falcon, E.; Laroche, C.; Fauve, S. Observation of Gravity-Capillary Wave Turbulence. Phys. Rev. Lett. 2007, 98, 094503. [Google Scholar] [CrossRef]
- Falcón, C.; Falcon, E.; Bortolozzo, U.; Fauve, S. Capillary wave turbulence on a spherical fluid surface in low gravity. EPL Europhys. Lett. 2009, 86, 14002. [Google Scholar] [CrossRef]
- Xia, H.; Shats, M.G.; Punzmann, H. Modulation instability and capillary wave turbulence. EPL Europhys. Lett. 2010, 91, 14002. [Google Scholar] [CrossRef]
- Wright, W.B.; Budakian, R.; Pine, D.J.; Putterman, S.J. Imaging of Intermittency in Ripple-Wave Turbulence. Science 1997, 278, 1609. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Snouck, D.; Westra, M.T.; van de Water, W. Turbulent parametric surface waves. Phys. Fluids 2009, 21, 025102. [Google Scholar] [CrossRef]
- Düring, G.; Falcón, C. Symmetry Induced Four-Wave Capillary Wave Turbulence. Phys. Rev. Lett. 2009, 103, 174503. [Google Scholar] [CrossRef]
- Aubourg, Q.; Mordant, N. Nonlocal Resonances in Weak Turbulence of Gravity-Capillary Waves. Phys. Rev. Lett. 2015, 114, 144501. [Google Scholar] [CrossRef]
- Cobelli, P.; Przadka, A.; Petitjeans, P.; Lagubeau, G.; Pagneux, V.; Maurel, A. Different Regimes for Water Wave Turbulence. Phys. Rev. Lett. 2011, 107, 214503. [Google Scholar] [CrossRef]
- Deike, L.; Berhanu, M.; Falcon, E. Decay of capillary wave turbulence. Phys. Rev. E 2012, 85, 066311. [Google Scholar] [CrossRef]
- Berhanu, M.; Falcon, E. Space-time resolved capillary wave turbulence. Phys. Rev. E 2013, 87, 033003. [Google Scholar] [CrossRef]
- Deike, L.; Miquel, B.; Gutiérrez, P.; Jamin, T.; Semin, B.; Berhanu, M.; Falcon, E.; Bonnefoy, F. Role of the basin boundary conditions in gravity wave turbulence. J. Fluid Mech. 2015, 781, 196–225. [Google Scholar] [CrossRef]
- Deike, L.; Berhanu, M.; Falcon, E. Energy flux measurement from the dissipated energy in capillary wave turbulence. Phys. Rev. E 2014, 89, 023003. [Google Scholar] [CrossRef]
- Fedorov, A.; Melville, W.K.; Rozenberg, A. An experimental and numerical study of parasitic capillary waves. Phys. Fluids 1998, 10, 1315. [Google Scholar] [CrossRef]
- Watson, K.M.; Buchsbaum, S.B. Interaction of capillary waves with longer waves. Part 1. General theory and specific applications to waves in one dimension. J. Fluid Mech. 1996, 321, 87–120. [Google Scholar] [CrossRef]
- Watson, K.M. Interaction of capillary waves with longer waves. Part 2. Applications to waves in two surface dimensions and to waves in shallow water. J. Fluid Mech. 1999, 397, 99–117. [Google Scholar] [CrossRef]
- Falcon, E.; Fauve, S.; Laroche, C. Observation of intermittency in wave turbulence. Phys. Rev. Lett. 2007, 98, 154501. [Google Scholar] [CrossRef] [PubMed]
- Falcon, E.; Fauve, S.; Laroche, C. On the origin of intermittency in wave turbulence. EPL Europhys. Lett. 2010, 90, 34005. [Google Scholar] [CrossRef]
- Nazarenko, S.; Lukaschuk, S.; McLelland, S.; Denissenko, P. Statistics of surface gravity wave turbulence in the space and time domains. J. Fluid Mech. 2010, 642, 395. [Google Scholar] [CrossRef]
- Humbert, T.; Cadot, O.; Düring, G.; Josserand, C.; Rica, S.; Touzé, C. Wave turbulence in vibrating plates: The effect of damping. EPL Europhys. Lett. 2013, 102, 30002. [Google Scholar] [CrossRef][Green Version]
- Miquel, B.; Alexakis, A.; Mordant, N. Role of dissipation in flexural wave turbulence: From experimental spectrum to Kolmogorov-Zakharov spectrum. Phys. Rev. E 2014, 89, 062925. [Google Scholar] [CrossRef]
- Berhanu, M.; Falcon, E.; Michel, F.; Gissinger, C.; Fauve, S. Capillary wave turbulence experiments in microgravity. EPL Europhys. Lett. 2019, 128, 34001. [Google Scholar] [CrossRef]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Berhanu, M. Impact of the Dissipation on the Nonlinear Interactions and Turbulence of Gravity-Capillary Waves. Fluids 2022, 7, 137. https://doi.org/10.3390/fluids7040137
Berhanu M. Impact of the Dissipation on the Nonlinear Interactions and Turbulence of Gravity-Capillary Waves. Fluids. 2022; 7(4):137. https://doi.org/10.3390/fluids7040137
Chicago/Turabian StyleBerhanu, Michael. 2022. "Impact of the Dissipation on the Nonlinear Interactions and Turbulence of Gravity-Capillary Waves" Fluids 7, no. 4: 137. https://doi.org/10.3390/fluids7040137
APA StyleBerhanu, M. (2022). Impact of the Dissipation on the Nonlinear Interactions and Turbulence of Gravity-Capillary Waves. Fluids, 7(4), 137. https://doi.org/10.3390/fluids7040137