The Capillary Waves’ Contribution to Wind-Wave Generation
Abstract
1. Introduction
2. Basic Theory of Wind-Supported Waves
2.1. Introduction
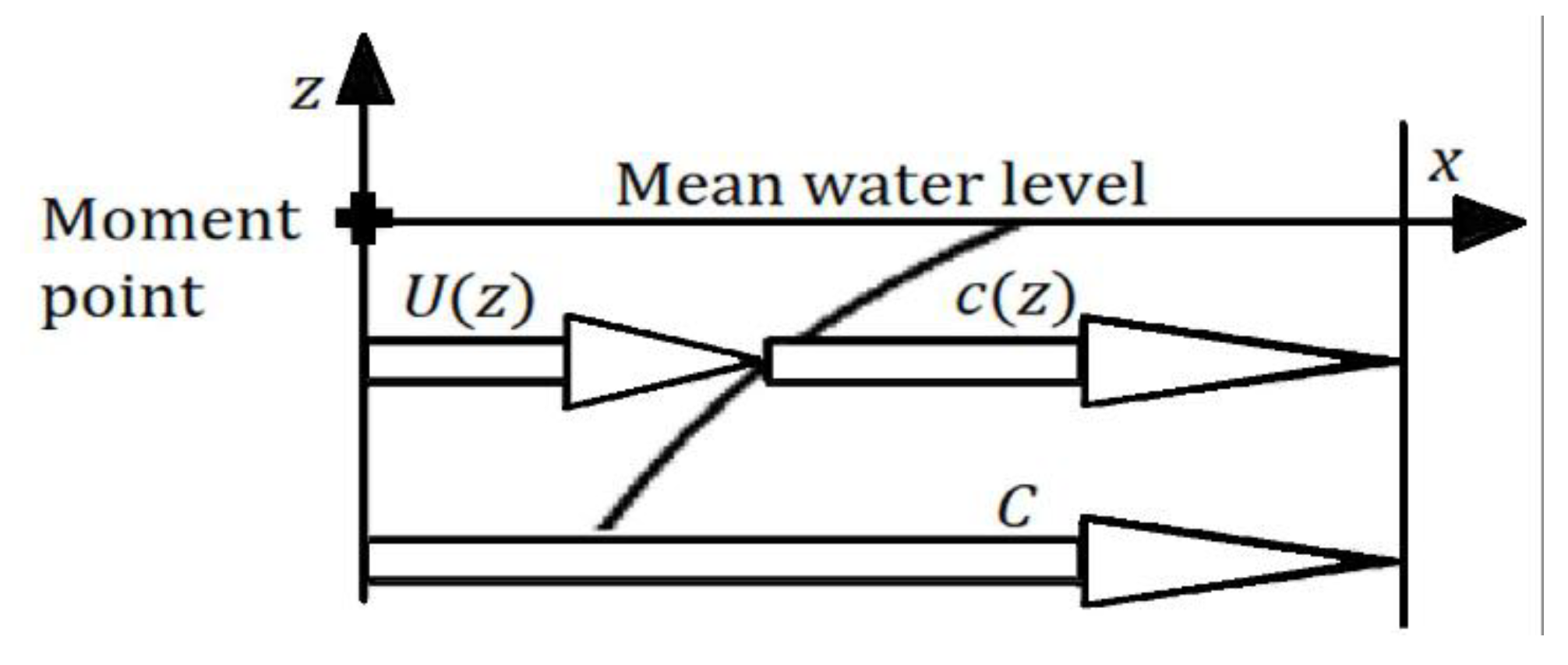
2.2. Basis
2.3. Continuity
2.4. The Air Pressure at the Surface
2.5. The Equation of Motion, Horizontal Component
2.6. Phase Speed and Frequency
2.7. The Equation of Motion, Vertical Component
2.8. Numerical Results
3. Vertical Flux of Energy through the Surface
3.1. Introduction
3.2. The Air Pressure’s Primary Contribution
3.3. The Air Pressure’s Second Contribution
3.4. The Shear Stress Contribution
3.5. Dissipation and Excess Work
4. Spin and Angular Momentum
4.1. Spin of Free Waves
4.2. Input of Angular Momentum from the Wind
4.3. Frequency Downshifting
4.4. Swells
5. Conclusions
- Energy input from the wind is provided in three different ways by and as given by (46), (49) and (51).
- A consequence of (60) is that shear stresses provide input of angular momentum from the wind to the high-frequency parts of a spectrum only.
- Another consequence of (60) is that swells cannot grow when energy and spin input from other frequencies is missing.
- Without energy input by and to wind-supported capillaries, the ocean would have been mainly flat. Merely ship waves, tsunamis, etc. would exist.
- The air pressure cannot amplify gravity waves without input of energy and spin from other frequencies through wave–wave interactions. Refs. Equations (56) and (60).
- Spin conservation implies energy transfer to waves with higher phase speeds. Ref. Equation (58).
- It follows from (39) that wind-supported waves depend on the existence of laminar surface conditions, and that and are mutually dependent on each other.
- A shear current implies that the orbits of the water of linear waves are elliptic, where the horizontal axes are longer than the vertical axes when . Ref. Equations (7), (16) and (38).
- It follows from Table 2 that the vorticity of the current determines the frequency of wind-supported waves.
- ○
- Observed wave heights of wind-supported waves hardly exceed 1 mm.
- ○
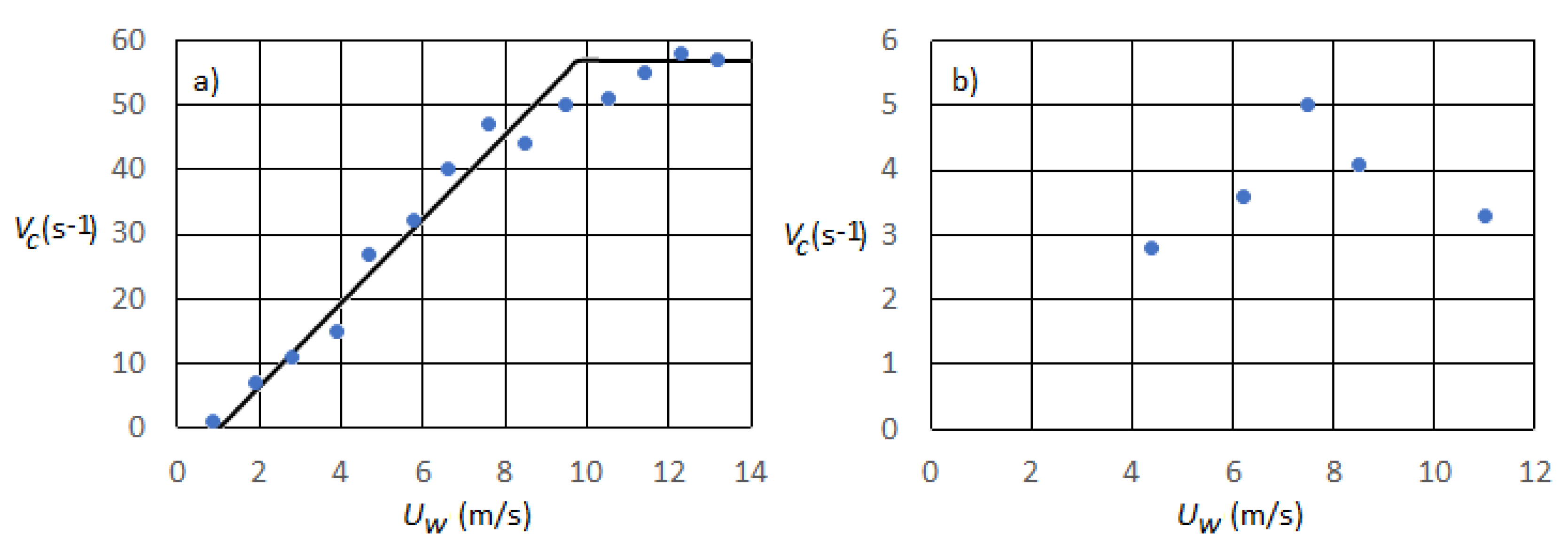
- If (1) is correct, then is not a function of the wind speed when the wind speed exceeds 10 m/s. It implies that the theory does not explain why increasing gales imply increasing wave growth.
Funding
Acknowledgments
Conflicts of Interest
Correction Statement
Appendix A. Spin Conservation of Free Waves
References
- Tulin, M.P.; Waseda, T. Laboratory observations of wave group evolution, including breaking effects. J. Fluid Mech. 1999, 378, 197–232. [Google Scholar] [CrossRef]
- Naeser, H. Why ripples become swells and waves in shallow water decay rapidly. Geophys. Astrophys. Fluid Dyn. 1979, 13, 335–345. [Google Scholar] [CrossRef]
- Longuet-Higgins, M. Spin and angular momentum in gravity waves. J. Fluid Mech. 1980, 97, 1–25. [Google Scholar] [CrossRef]
- Naeser, H. A theory for the evolution of wind-generated gravity-wave spectra due to dissipation. Geophys. Astrophys. Fluid Dyn. 1981, 18, 75–92. [Google Scholar] [CrossRef]
- Grare, L.; Peirson, W.L.; Branger, H.; Walker, J.; Giovanangeli, J.-P.; Makin, V. Growth and dissipation of wind-forced, deep-water waves. J. Fluid Mech. 2013, 722, 5–50. [Google Scholar] [CrossRef]
- Caulliez, G. Dissipation regimes for short wind waves. J. Geophys. Res. Oceans 2013, 118, 682–684. [Google Scholar] [CrossRef]
- Shemdin, O.H. Wind-generated current and phase speed of wind waves. J. Phys. Oceanogr. 1972, 2, 411–419. [Google Scholar] [CrossRef]
- McLeish, W.L.; Putland, G.E. 1975 Measurements of wind-driven flow profiles in the top millimeter of water. J. Phys. Oceanogr. 1975, 5, 516–518. [Google Scholar] [CrossRef]
- Wu, J. Wind-induced drift currents. J. Fluid Mech. 1975, 68, 49–70. [Google Scholar] [CrossRef]
- Siddiqui, M.H.K.; Loewen, M.R. Characteristics of the wind drift layer and microscale breaking waves. J. Fluid Mech. 2007, 573, 417–456. [Google Scholar] [CrossRef]
- Miles, J.W. On the generation of waves by shear flows. J. Fluid Mech. 1957, 3, 185–204. [Google Scholar] [CrossRef]
- Miles, J.W. On the generation of waves by shear flows. Part 2. J. Fluid Mech. 1959, 6, 568–582. [Google Scholar] [CrossRef]
- Airy, G.B. Tides and waves. Encycl. Metrop. 1841, 11, 192. [Google Scholar]
- Longuet-Higgins, M. Parasitic capillaries: A direct calculation. J. Fluid Mech. 1995, 301, 79–107. [Google Scholar] [CrossRef]
- Melville, W.K.; Fedorov, A.V. 2015 The equilibrium dynamics and statistics of gravity-capillaries. J. Fluid Mech. 2015, 767, 449–466. [Google Scholar] [CrossRef]
- Gibbs, J.W. On the equilibrium of heterogeneous substances. Part II. Trans. Conn. Acad. Arts Sci. 1878, 3, 343–524. [Google Scholar]
- Franklin, B.; Brownrigg, W.; Farish, M. On the stilling of waves by means of oil. R. Soc. 1774, 12, 445–459. [Google Scholar]
- Jeffreys, H. On the formation of water waves by wind. Proc. R. Soc. A 1925, 107, 189–206. [Google Scholar]
- Jeffreys, H. On the formation of water waves by wind II. Proc. R. Soc. A 1925, 110, 341–347. [Google Scholar]
- Thompson, P.D. The propagation of small surface disturbances through rotational flow. Ann. N. Y. Acad. Sci. 1949, 51, 463–474. [Google Scholar] [CrossRef]
- Abdullah, A.J. Wave motion at the surface of a current which has an exponential distribution of vorticity. Ann. N. Y. Acad. Sci. 1949, 51, 425–441. [Google Scholar] [CrossRef]
- Valenzuela, G.R. The growth of gravity-capillaries in the coupled shear flow. J. Fluid Mech. 1976, 76, 229–250. [Google Scholar] [CrossRef]
- Kawai, S. Generation of initial wavelets by instability of a coupled shear flow and their evolution to wind waves. J. Fluid Mech. 1979, 93, 661–703. [Google Scholar] [CrossRef]
- Plant, W.J.; Wright, J.W. Phase speeds of upwind and downwind travelling short gravity waves. J. Geophys. Res. 1980, 85, 3304–3310. [Google Scholar] [CrossRef]
- Van Gastel, K.; Janssen, P.A.E.M.; Komen, G.J. On phase velocity and growth rate of wind-induced gravity-capillaries. J. Fluid Mech. 1985, 161, 199–216. [Google Scholar] [CrossRef]
- Kinsman, B. Wind Waves—Their Generation and Propagation on the Ocean Surface; Prentice-Hall: Englewood Cliffs, NJ, USA, 1965. [Google Scholar]
- Lamb, H. Hydrodynamics, 6th ed.; Cambridge University Press: Cambridge, UK, 1932. [Google Scholar]
- Bachelor, G.K. An Introduction to Fluid Dynamics; Cambridge University Press: Cambridge, UK, 1974. [Google Scholar]
- Melville, W.K.; Shear, R.; Veron, F. Laboratory measurements of the generation and evolution of Langmuir circulations. J. Fluid Mech. 1998, 364, 31–58. [Google Scholar] [CrossRef]
- Cheung, T.K.; Street, R.L. The turbulent layer in the water at an air-water interface. J. Fluid Mech. 1988, 194, 133–151. [Google Scholar] [CrossRef]
- Lake, B.M.; Yuen, H.C.; Rungaldier, H.; Ferguson, W.E. Nonlinear deep-water waves: Theory and experiment. Part 2. Evolution of a continuous wave train. J. Fluid Mech. 1977, 83, 49–74. [Google Scholar] [CrossRef]
- Lake, B.M.; Yuen, H.C. Model for nonlinear wind waves: Part 1. Physical model and experimental evidence. Evolution of a continuous wave train. J. Fluid Mech. 1978, 88, 33–62. [Google Scholar] [CrossRef]
- Tulin, M.P. Breaking of ocean waves and downshifting. In Waves and Nonlinear Processes in Hydrodynamics; Grue, J., Gjevik, B., Weber, J.E., Eds.; Kluwer Academic Publishers: Dordrecht, The Netherlands; Boston, MA, USA; London, UK, 1996; pp. 177–190. [Google Scholar]
- Hara, T.; Mei, C.C. Wind effects on the nonlinear evolution of slowly varying gravity-capillary waves. J. Fluid Mech. 1994, 267, 221–250. [Google Scholar] [CrossRef]
- Bretherton, F.P.; Garret, C.J.R. Wavetrains in inhomogeneous moving media. Proc. R. Soc. Lond. A 1968, 302, 529–554. [Google Scholar]
- Coastal Engineering Research Center (US). Shore Protection Manual, 3rd ed.; U.S. Army Corps of Engineer, Coastal Engineer Research Center: Vicksburg, MS, USA, 1977. [Google Scholar]
- Snodgrass, F.E.; Growe, G.W.; Hasselmann, K.F.; Miller, G.R.; Munk, W.H.; Powers, W.H. Propagation of ocean swell across the Pacific. Philos. Trans. 1966, 259, 431–497. [Google Scholar]
- Hasselmann, K.; Barnett, T.B.; Bouws, E.; Carlson, H.; Cartwright, D.E.; Enke, K.; Ewing, J.A.; Gienapp, H.; Hasselmann, D.E.; Kruseman, P.; et al. Measurements of wind-wave growth and swell decay during the Joint North Sea Wave Project. Ergänzungsheft Zur Dtsch. Hydrogr. Z. 1973, 8, 12. [Google Scholar]
- Benjamin, T.B.; Feir, J.E. The disintegration of wave trains on deep water Part 1. Theory. J. Fluid Mech. 1967, 27, 417–430. [Google Scholar] [CrossRef]
- Naeser, H. Energy accumulation in ocean waves. Proc. 3rd Eur. Wave Energy Conf. 1998, 2, 296–303. [Google Scholar]
- Mitsuyasu, H. Measurements of high-frequency spectrum of ocean surface waves. J. Phys. Ocean. 1977, 7, 882–891. [Google Scholar] [CrossRef]
- Torsethaugen, K. A two peak wave spectrum model. OAME—II Safety and reliability. ASME 1993, 175–180. [Google Scholar]
- Naeser, H. Conservation of partial angular momentum with applications on water waves. J. Appl. Sci. 2002, 2, 682–685. [Google Scholar]





| Formula | Derived from; by Using | Equation |
|---|---|---|
| (17) | (T1) | |
| (5) | (T2) | |
| (5) | (T3) | |
| (5); (14), (19) | (T4) | |
| (3) | (T5) | |
| (18); (T5) | (T6) | |
| (27); (18), (T5) | (T7) | |
| (18), (21); (T5) | (T8) | |
| (20); (T3) | (T9) | |
| (20); (T2) | (T10) | |
| (22); (6), (T9), (T10) | (T11) | |
| (T10); (T2) | (T12) | |
| (20); (18), (T1), (T4), (T5), (T6) | (T13) | |
| (T15); (18), (T1), (T4), (T5), (T8), (T10) | (T14) | |
| (T12), (T14) | (T15) | |
| (6); (T3) | (T16) | |
| (6); (T2) | (T17) | |
| (T17); (T2) | (T18) | |
| (6); (18), (T1), (T4), (T5) | (T19) | |
| (T19); (18), (T1), (T4), (T5), (T8) | (T20) | |
| (T18), (T20) | (T21) | |
| (23); (6), (T16), (T18) | (T22) | |
| (28); (T2) | (T23) | |
| (28); (16), (T1), (T4), (T5) | (T24) |
| λ (mm) | 3.08 | 4.00 | 5.00 | 7.00 | 10.00 | 15.00 | 30.00 | 40.00 | Input | |
|---|---|---|---|---|---|---|---|---|---|---|
| κ (mm−1) | 2.040 | 1.571 | 1.257 | 0.898 | 0.628 | 0.419 | 0.209 | 0.157 | 2π/λ | |
| ω0 (s−1) ω0 (s−1) | 20 °C | 777.20 | 531.93 | 386.80 | 242.75 | 152.75 | 95.69 | 51.55 | 42.24 | (33) |
| 0 °C | 526.11 | 385.15 | 240.99 | 151.16 | 94.32 | 50.48 | 42.07 | (33) | ||
| N0·103 | 20 °C | 5.35 | 4.64 | 4.08 | 3.32 | 2.58 | 1.834 | 0.851 | 0.584 | (27) |
| N0·103 | 0 °C | 8.44 | 7.43 | 6.03 | 4.69 | 3.33 | 1.539 | 1.056 | (27) | |
| I | 20 °C | 1.073 | 1.068 | 1.064 | 1.058 | 1.051 | 1.043 | 1.029 | 1.024 | (38) |
| I | 0 °C | 1.092 | 1.086 | 1.078 | 1.069 | 1.058 | 1.040 | 1.032 | (38) | |
| V (s−1) | 20 °C | 56.87 | 36.23 | 24.71 | 13.98 | 7.77 | 4.08 | 1.504 | 1.021 | (18) |
| V (s−1) | 0 °C | 48.34 | 32.99 | 18.68 | 10.37 | 5.48 | 2.01 | 1.367 | (18) | |
| Uw (m/s) | 20 °C | ≥ 9.75 | 6.57 | 4.80 | 3.15 | 2.18 | 1.62 | 1.23 | 1.16 | (1) |
| Uw (m/s) | 0 °C | 8.44 | 6.08 | 3.87 | 2.60 | 1.84 | 1.31 | 1.21 | (1) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Naeser, H. The Capillary Waves’ Contribution to Wind-Wave Generation. Fluids 2022, 7, 73. https://doi.org/10.3390/fluids7020073
Naeser H. The Capillary Waves’ Contribution to Wind-Wave Generation. Fluids. 2022; 7(2):73. https://doi.org/10.3390/fluids7020073
Chicago/Turabian StyleNaeser, Harald. 2022. "The Capillary Waves’ Contribution to Wind-Wave Generation" Fluids 7, no. 2: 73. https://doi.org/10.3390/fluids7020073
APA StyleNaeser, H. (2022). The Capillary Waves’ Contribution to Wind-Wave Generation. Fluids, 7(2), 73. https://doi.org/10.3390/fluids7020073





