Unsteady MHD Mixed Convection Flow of Non-Newtonian Casson Hybrid Nanofluid in the Stagnation Zone of Sphere Spinning Impulsively
Abstract
1. Introduction
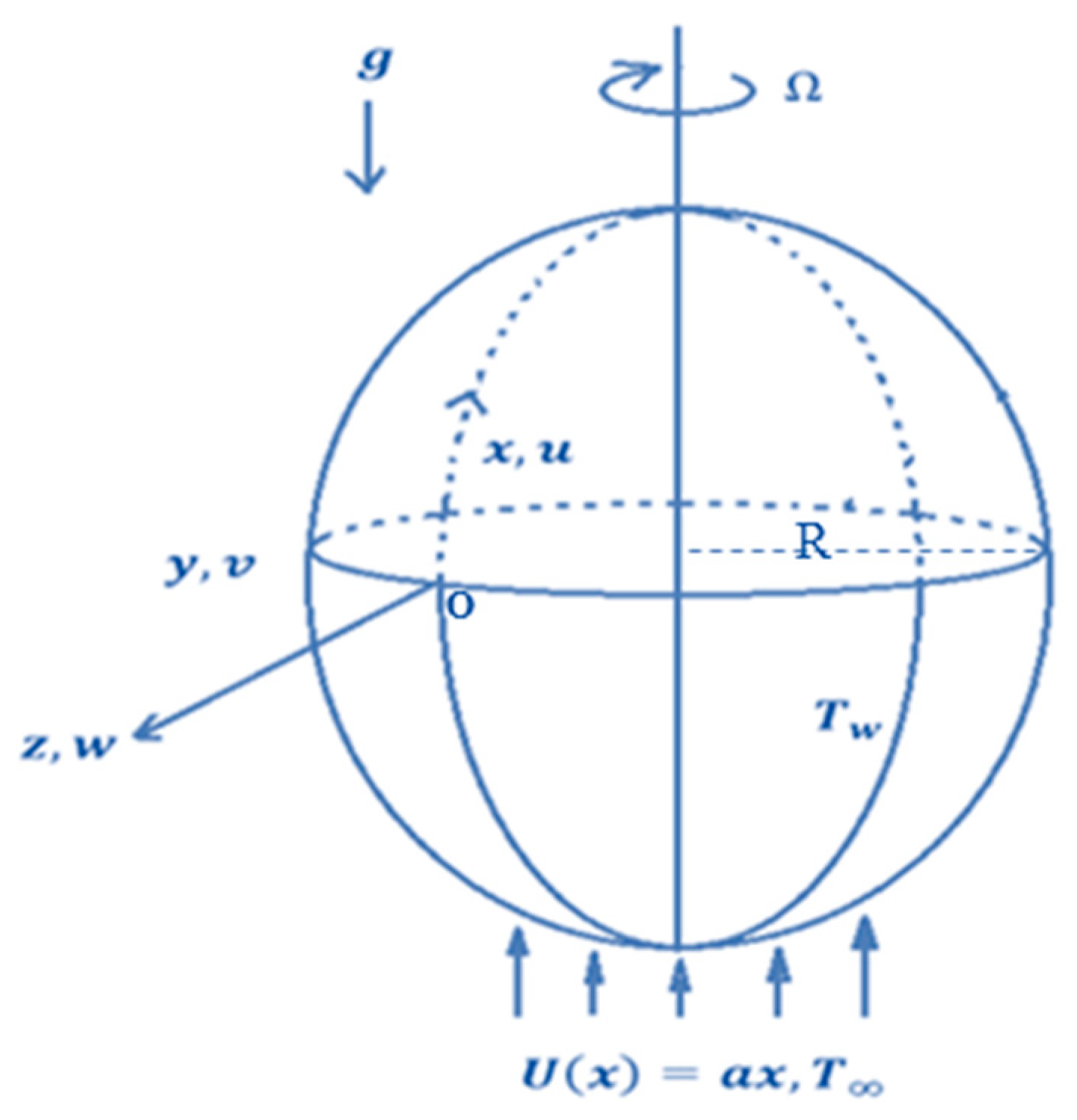
2. Problem Formulation
- The fluid is an electrical conductor in the existence of a fixed magnetic field applied in the z-direction.
- The sphere is at rest in the surrounding fluid prior to time , and the temperature of the surface is .
- At , the temperature of the surface for the sphere is unexpectedly increased to , where .
- It is assumed that the properties of the fluid are fixed, excluding the density, which is set based the Boussinesq approximation.
- The rheological equation for the non-compressible and isotropic flow state of a Casson fluid iswhere and which is the distortion rate components, µB refers to the dynamic viscosity of the non-Newtonian fluid, based on the non-Newtonian model is the critical value of , and indicates to the yield stress fluid.
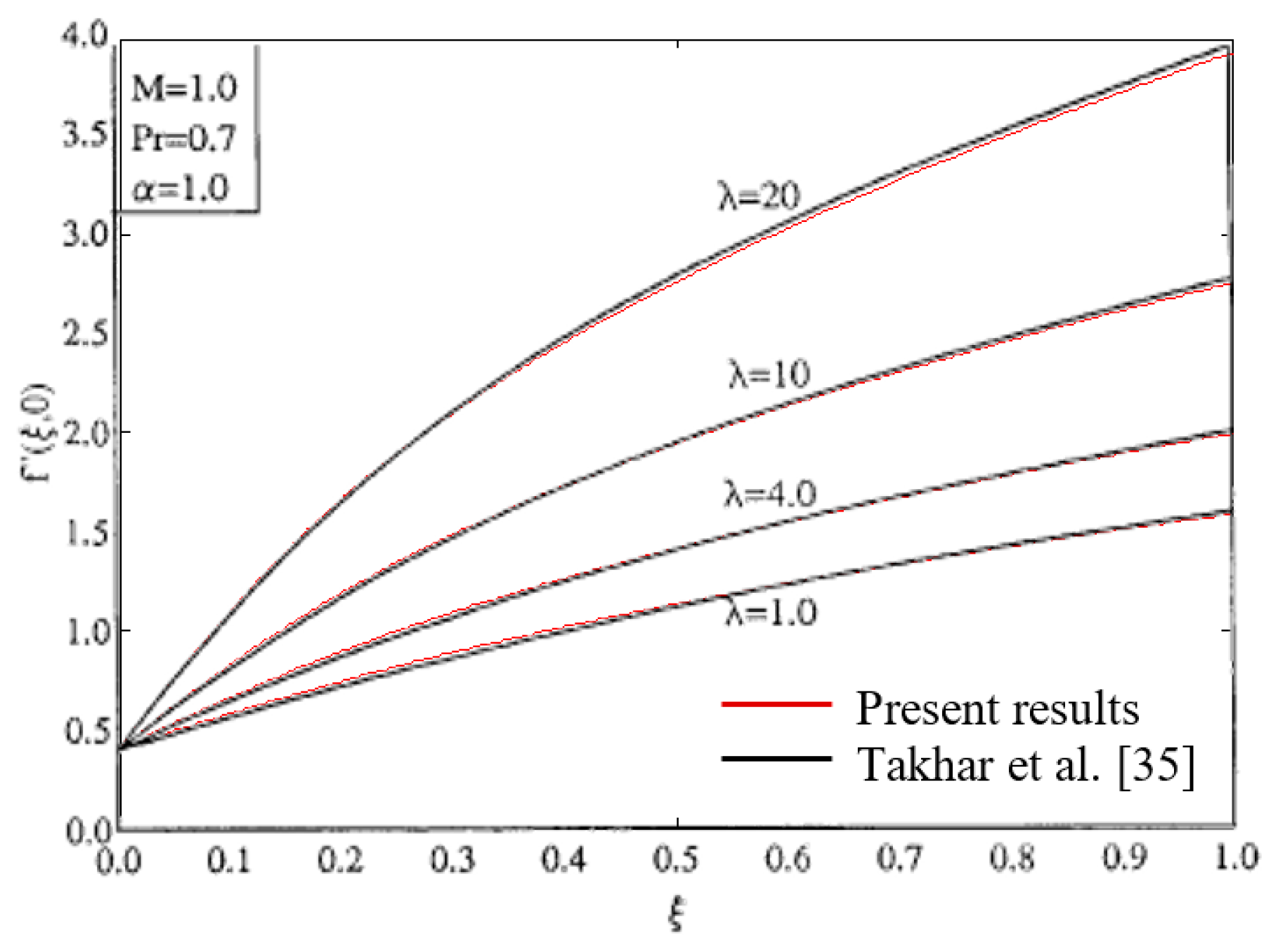
- According to these suppositions, Joule heating impacts and the viscous dissipation are ignored. As depicted in Takhar at al. [35], the governing equations can be written:
3. Results and Discussion
4. Conclusions
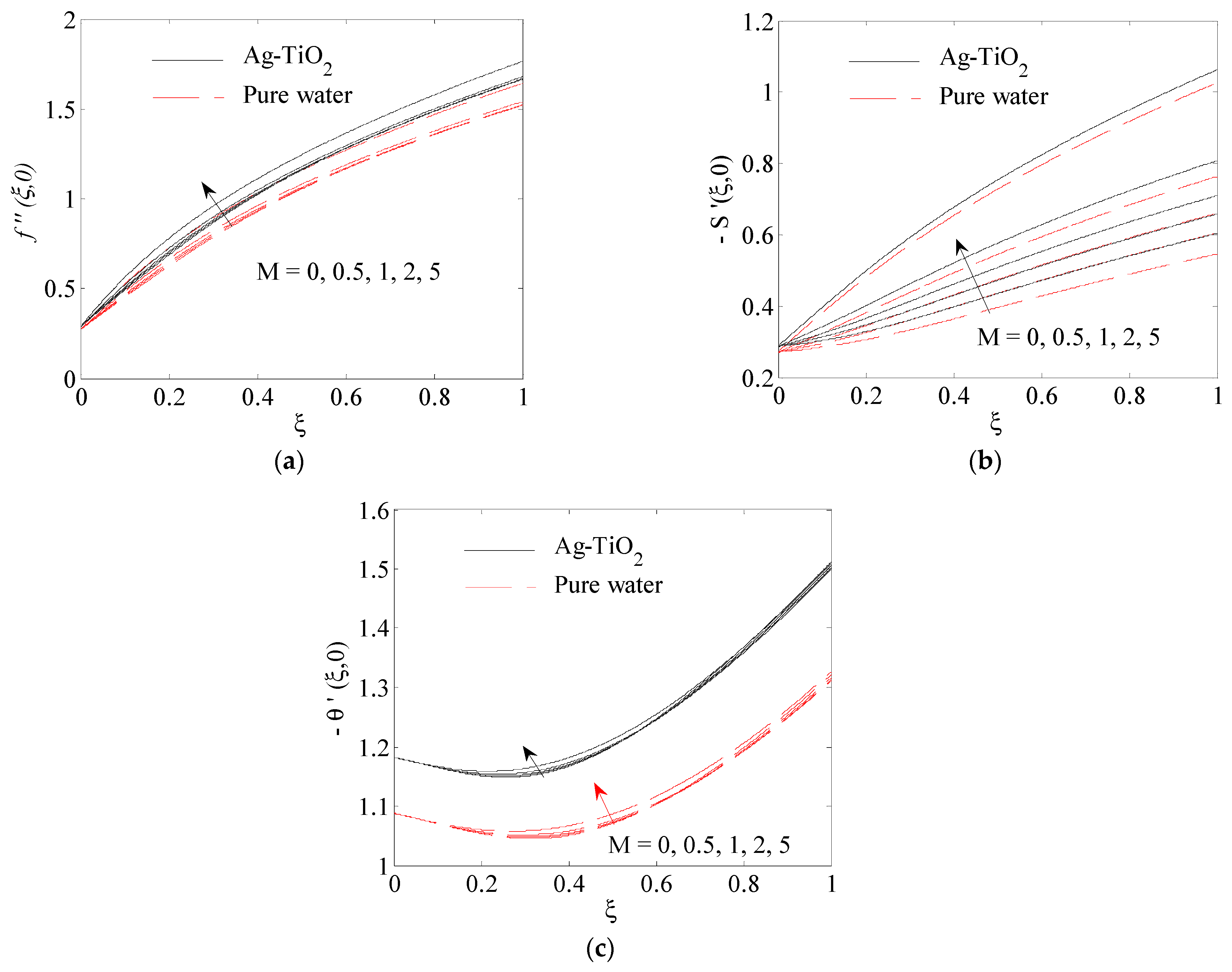
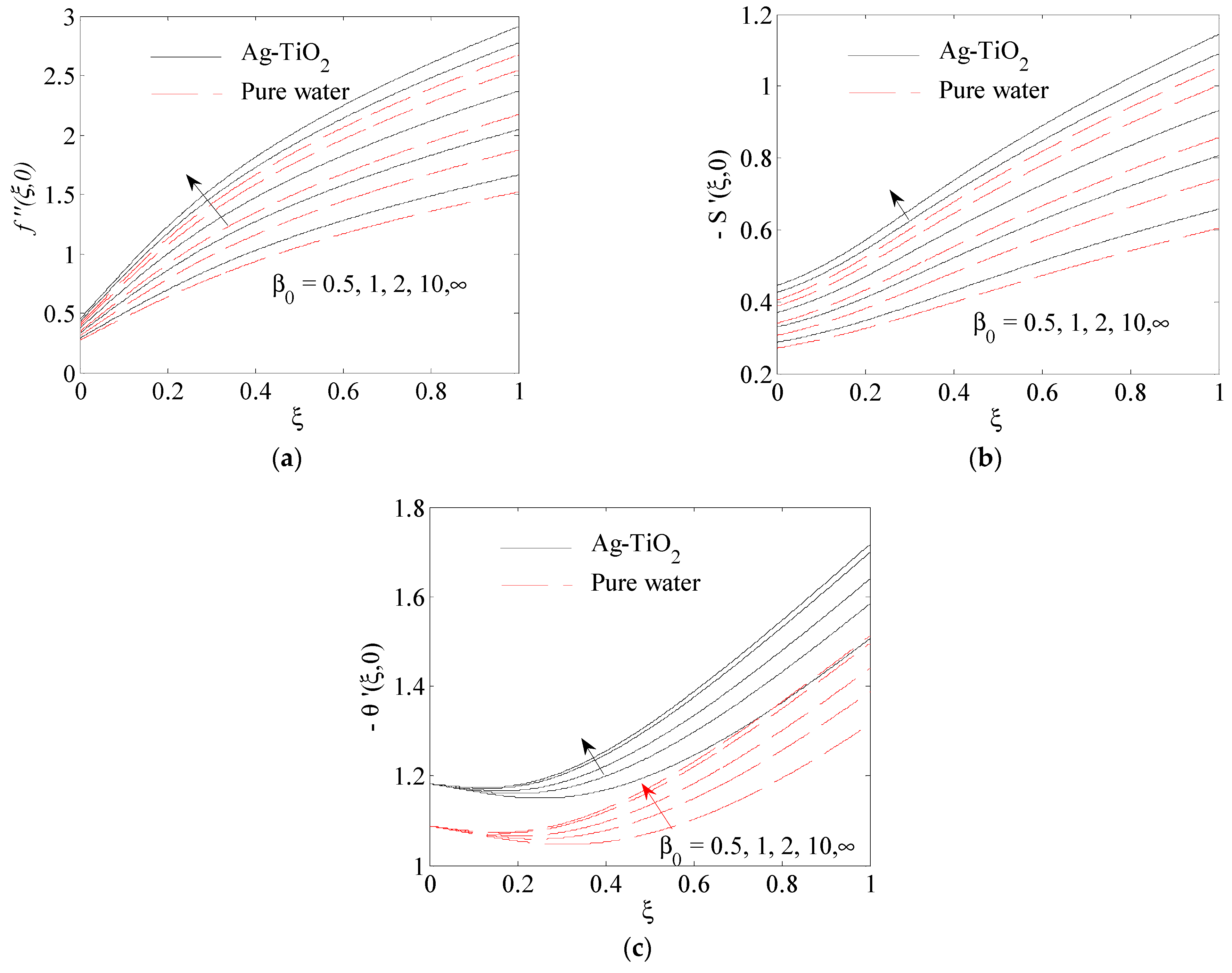
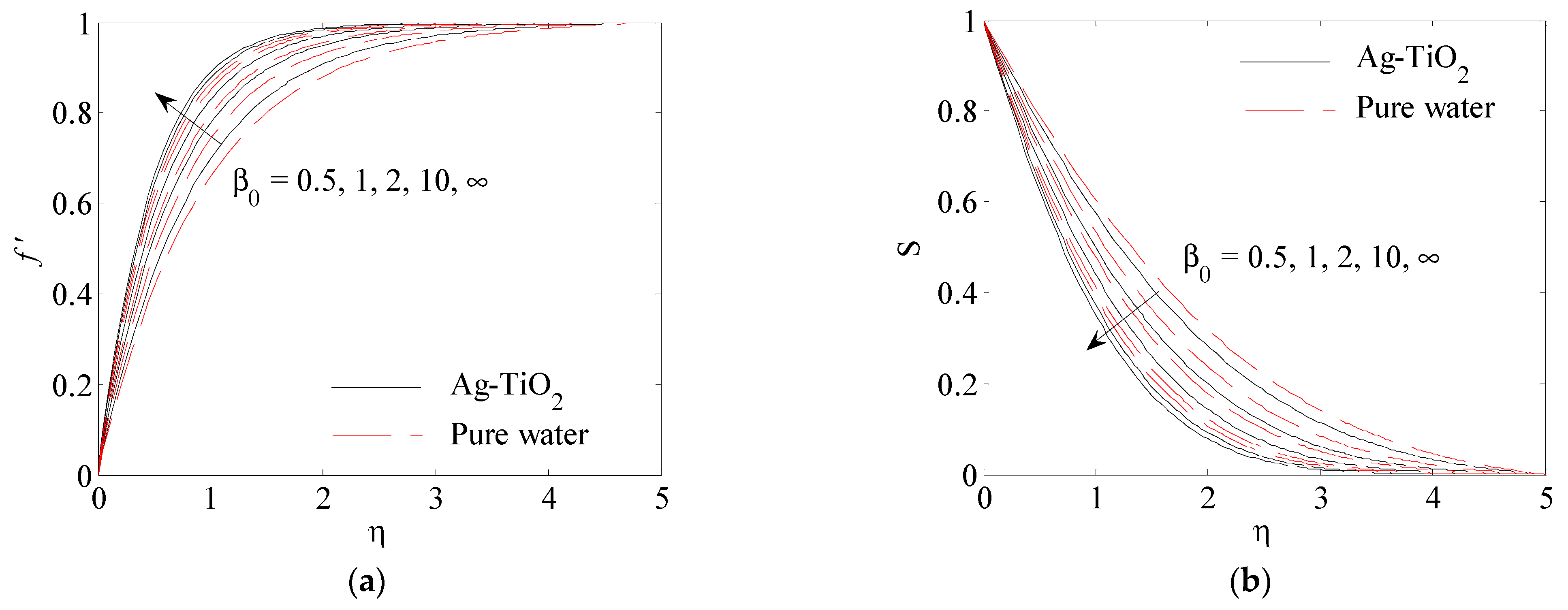
- The heat transfer rate and surface shear stress in x- and y-direction are enhanced by increasing the magnetic field, Casson, mixed convection and the sphere rotation parameters.
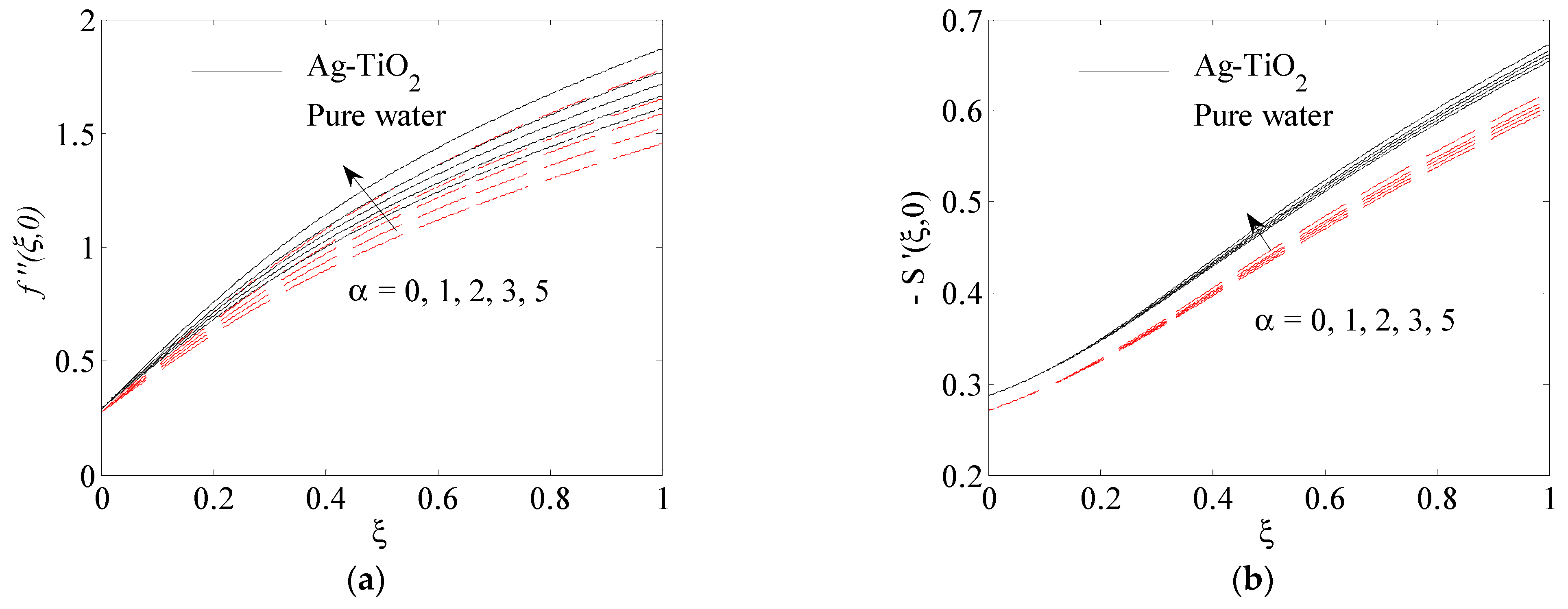
- The velocity profile in x-direction, temperature profile and surface shear stresses in x- and y-direction are enhanced by the increase in the thermal radiation, unlike the rate of heat transfer and the velocity profile in y-direction, which is decreased by boosting the thermal radiation parameter.
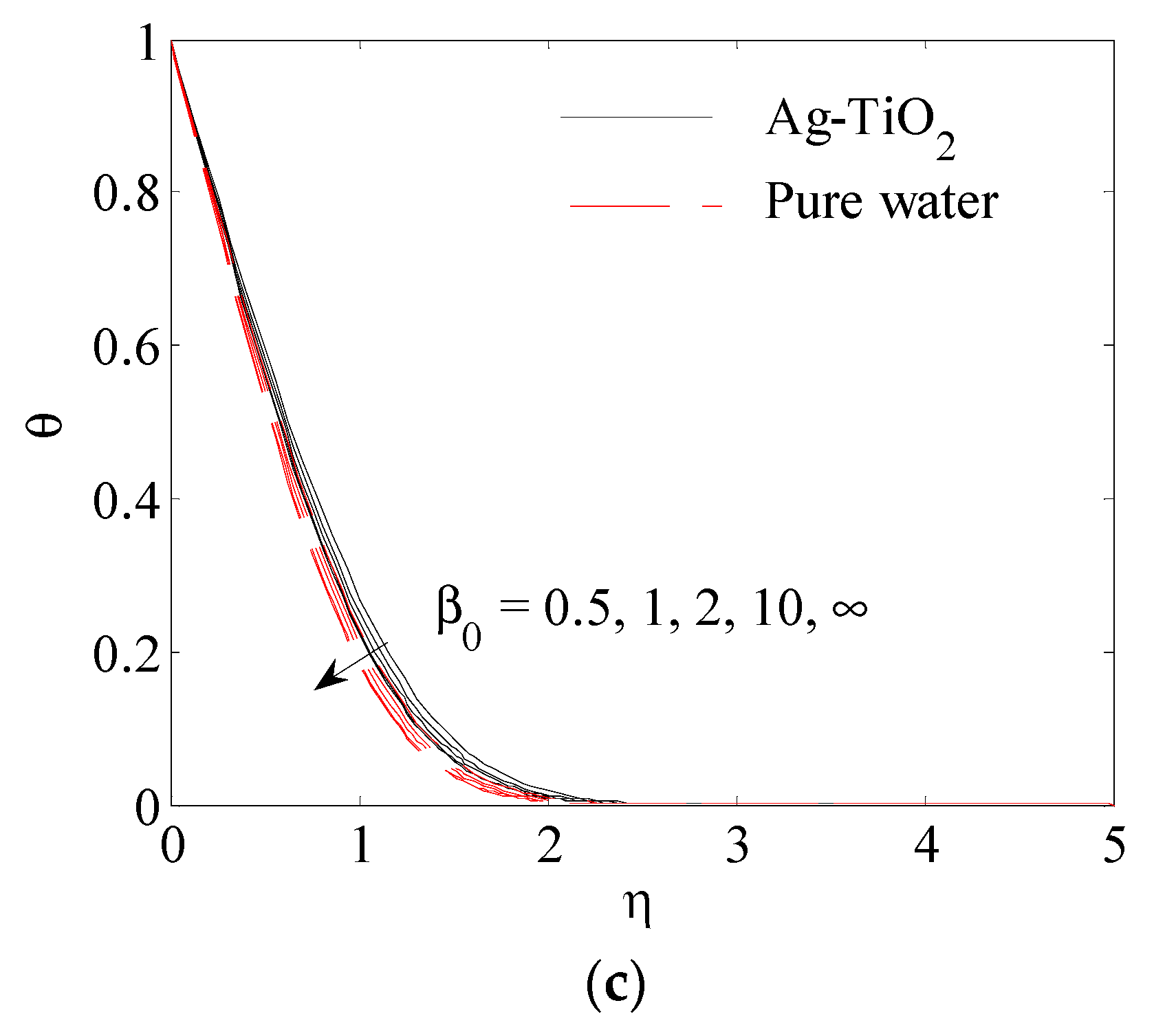
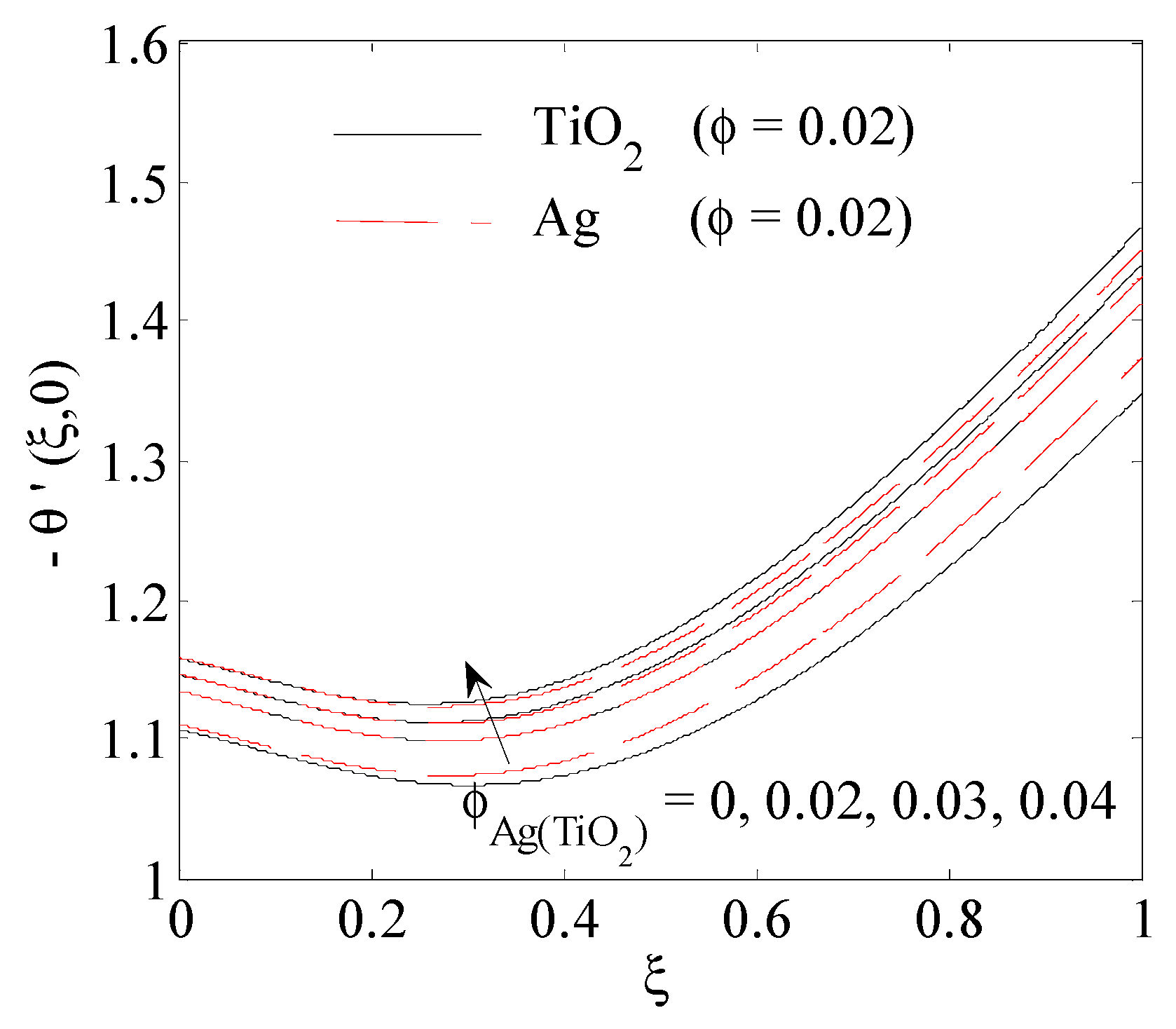
- Surface shear stresses in x- and y-direction, the velocity distribution in x-direction and temperature distribution of hybrid nanofluid (Ag-TiO2/H2O) is greater as compared to the base fluid.
- Both velocity distributions in y-direction and the temperature distribution in non-Newtonian hybrid nanofluid are greater than in Newtonian hybrid nanofluid, unlike the velocity distribution where it is noticed that the of non-Newtonian hybrid nanofluid is smaller than Newtonian fluid.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hoskin, N.E. The laminar boundary layer on a rotating sphere. In 50 Jahre Grenzschichfforschung; Vieweg Teubner Verlag: Wiesbaden, Germany, 1995; pp. 127–131. [Google Scholar]
- Sickmann, I. The calculation of the thermal laminar boundary layer on rotating sphere. Z. Angew. Math. Phys. 1962, 13, 468–482. [Google Scholar] [CrossRef]
- Chao, B.T.; Greif, R. Laminar forced convection over rotating bodies. J. Heat Transf. 1974, 96, 463–466. [Google Scholar] [CrossRef]
- Chao, B.T. An analysis of forced convection over nonisothermal surfaces via universal functions. In Recent Advances in Engineering Science; Lehigh University: Bethlehem, PA, USA, 1977; pp. 471–483. [Google Scholar]
- Lee, M.H.; Jang, D.R.; Dewitt, K.J. Laminar boundary layer heat transfer over rotating bodies in forced flow. J. Heat Transf. 1978, 100, 496–502. [Google Scholar] [CrossRef]
- Rajasekaran, R.; Palekar, M.G. Mixed convection about a rotating sphere. Int. J. Heat Mass Transf. 1985, 28, 959–968. [Google Scholar] [CrossRef]
- Hall, M.G. The boundary layer over an impulsively started flat plate. Proc. R. Soc. A 1969, 310, 401–414. [Google Scholar]
- Stewartson, K. On the impulsive motion of a flat plate in a viscous fluid. Part I. Q. J. Mech. Appl. Math. 1951, 4, 183–198. [Google Scholar] [CrossRef]
- Stewartson, K. On the impulsive motion of a flat plate in a viscous fluid. Part II. Q. J. Mech. Appl. Math. 1973, 26, 143–152. [Google Scholar] [CrossRef]
- Watkins, C.A. Heal transfer in the boundary layer over an impulsively started flat plate. J. Heat Transf. 1975, 97, 482–484. [Google Scholar] [CrossRef]
- Mustafa, M.; Hayat, T.; Pop, I.; Hendi, A. Stagnation-point flow and heat transfer of a Casson fluid towards a stretching sheet. Z. Nat. A 2012, 67, 70–76. [Google Scholar] [CrossRef]
- Mustafa, M.; Hayat, T.; Pop, I.; Aziz, A. Unsteady boundary layer flow of a Casson fluid due to an impulsively started moving flat plate. Heat Transf. 2011, 40, 563–576. [Google Scholar] [CrossRef]
- Nadeem, S.; Haq, R.U.; Akbar, N.S.; Khan, Z.H. MHD three-dimensional Casson fluid flow past a porous linearly stretching sheet. Alex. Eng. J. 2013, 52, 577–582. [Google Scholar] [CrossRef]
- Boyd, J.; Buick, J.; Green, M.S. Analysis of the Casson and Carreau-Yasuda non-Newtonian blood models in steady and oscillatory flow using the lattice Boltzmann method. Phys. Fluids 2007, 19, 93–103. [Google Scholar] [CrossRef]
- Chamkha, A.J.; Ahmed, S.E. Unsteady MHD heat and mass transfer by mixed convection flow in the forward stagnation region of a rotating sphere at different wall conditions. Chem. Eng. Commun. 2011, 199, 122–141. [Google Scholar] [CrossRef]
- Eldabe, N.T.M.; Saddeck, G.; El-Sayed, A.F. Heat transfer of MHD non-Newtonian Casson fluid flow between two rotating cylinders. Mech. Mech. Eng. 2001, 5, 237–251. [Google Scholar]
- Nadeem, S.; Haq, R.U.; Akbar, N.S. MHD three-dimensional boundary layer flow of Casson nanofluid past a linearly stretching sheet with convective boundary condition. IEEE Trans. Nanotechnol. 2014, 13, 109–115. [Google Scholar] [CrossRef]
- Andersson, H.I.; Aarseth, J.B.; Dandapat, B.S. Heat transfer in a liquid film on an unsteady stretching surface. Int. J. Heat Mass Transf. 2000, 43, 69–74. [Google Scholar] [CrossRef]
- Mukhopadhyay, S.; De Ranjan, P.; Bhattacharyya, K.; Layek, G.C. Casson fluid flow over an unsteady stretching surface. Ain Shams Eng. J. 2013, 4, 933–938. [Google Scholar] [CrossRef]
- Mahdy, A.; Ahmed, S.E. Unsteady MHD convective flow of non-Newtonian Casson fluid in the stagnation region of an impulsively rotating sphere. J. Aerosp. Eng. 2017, 30, 04017036. [Google Scholar] [CrossRef]
- Bhattacharyya, K. MHD stagnation-point flow of Casson fluid and heat transfer over a stretching sheet with thermal radiation. J. Thermodyn. 2013, 2013, 169674. [Google Scholar] [CrossRef]
- Mahdy, A. Heat transfer and flow of a Casson fluid due to a stretching cylinder with the Soret and Dufour effects. J. Eng. Phys. Thermophys. 2015, 88, 927–936. [Google Scholar] [CrossRef]
- Nandy, S.K. Analytical solution of MHD stagnation-point flow and heat transfer of Casson fluid over a stretching sheet with partial slip. Int. Sch. Res. Not. Thermodyn. 2013, 2013, 108264. [Google Scholar] [CrossRef]
- Makanda, G.; Shaw, S.; Sibanda, P. Diffusion of chemically reactive species in Casson fluid flow over an Unsteady Stretching surface in porous medium in the presence of a magnetic field. Math. Probl. Eng. 2015, 2015, 724596. [Google Scholar] [CrossRef]
- Mahdy, A.; Ahmed, S.E. Unsteady MHD double diffusive convection in the stagnation region of an impulsively rotating sphere in the presence of thermal radiation effect. J. Taiwan Inst. Chem. Eng. 2016, 58, 173–180. [Google Scholar]
- Choi, S.U.S.; Eastman, J.A. Enhancing thermal conductivity of fluids with nanoparticles. Dev. Appl. Non Newton. Flows 1995, 66, 99–105. [Google Scholar]
- Choi, S.U.S.; Zhang, Z.G.; Lockwood, F.E.; Gruike, E.A. Anomalous thermal conductivity enhancement in nanotube suspensions. Appl. Phys. Lett. 2001, 79, 2252–2254. [Google Scholar] [CrossRef]
- Raju, C.S.K.; Sandeep, N. Unsteady Casson nanofluid flow over a rotating cone in a rotating frame filled with ferrous nanoparticles: A numerical study. J. Magn. Magn. Mater. 2017, 421, 216–224. [Google Scholar] [CrossRef]
- Momin, G.G. Experimental investigation of mixed convection with water-Al2O3 & hybrid nanofluid in inclined tube for laminar flow. Int. J. Sci. Technol. Res. 2013, 2, 195–202. [Google Scholar]
- Suresh, S.; Venkitaraj, K.; Selvakumar, P.; Chandrasekar, M. Synthesis of Al2O3–Cu/water hybrid nanofluids using two step method and its thermo physical properties. Colloids Surf. A Physicochem. Eng. Asp. 2011, 388, 41–48. [Google Scholar] [CrossRef]
- Suresh, S.; Venkitaraj, K.; Selvakumar, P.; Chandrasekar, M. Effect of Al2O3–Cu/water hybrid nanofluid in heat transfer. Exp. Therm. Fluid Sci. 2012, 38, 54–60. [Google Scholar] [CrossRef]
- Suresh, S.; Venkitaraj, K.P.; Hameed, M.S.; Sarangan, J. Turbulent heat transfer and pressure drop characteristics of dilute water based Al2O3–Cu hybrid nanofluids. J. Nanosci. Nanotechnol. 2014, 14, 2563–2572. [Google Scholar] [CrossRef]
- Mahdy, A. Simultaneous impacts of MHD and variable wall temperature on transient mixed Casson nanofluid flow in the stagnation point of rotating sphere. Appl. Math. Mech. 2018, 39, 1327–1340. [Google Scholar] [CrossRef]
- EL-Zahar, E.R.; Rashad, A.M.; Seddek, L.F. The impact of sinusoidal surface temperature on the natural convective flow of a ferrofluid along a vertical plate. Mathematics 2019, 7, 1014. [Google Scholar] [CrossRef]
- Takhar, H.S.; Chamkha, A.J.; Nath, G. Unsteady laminar MHD flow and heat transfer in the stagnation region of an impulsively spinning and translating sphere in the presence of buoyancy forces. Heat Mass Transf. 2001, 37, 397–402. [Google Scholar] [CrossRef]
- Rashidi, M.M.; Vishnu Ganesh, N.; Abdul Hakeem, A.K.; Ganga, B. Buoyancy effect on MHD flow of nanofluid over a stretching sheet in the presence of thermal radiation. J. Mol. Liq. 2014, 198, 234–238. [Google Scholar] [CrossRef]
- Alwawi, F.A.; Alkasasbeh, H.T.; Rashad, A.M.; Idris, R. A Numerical Approach for the Heat Transfer Flow of Carboxymethyl Cellulose-Water Based Casson Nanofluid from a Solid Sphere Generated by Mixed Convection under the Influence of Lorentz Force. Mathematics 2020, 8, 1094. [Google Scholar] [CrossRef]

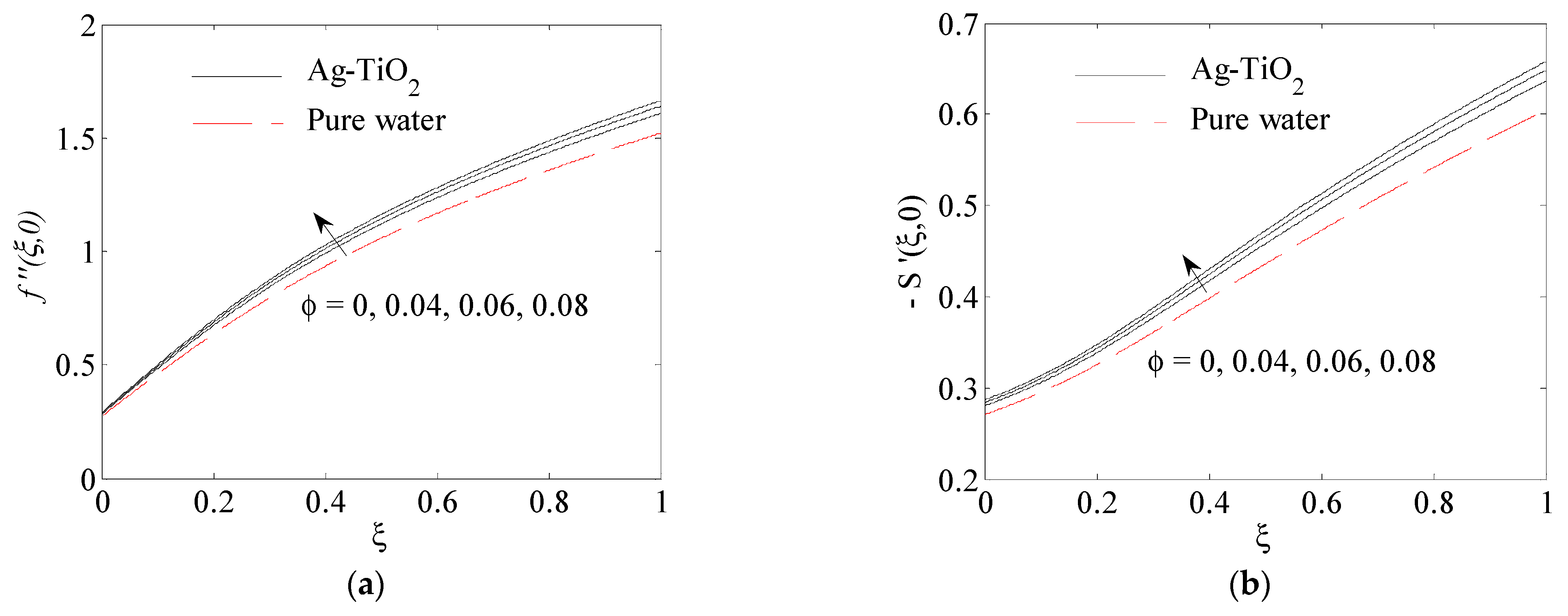



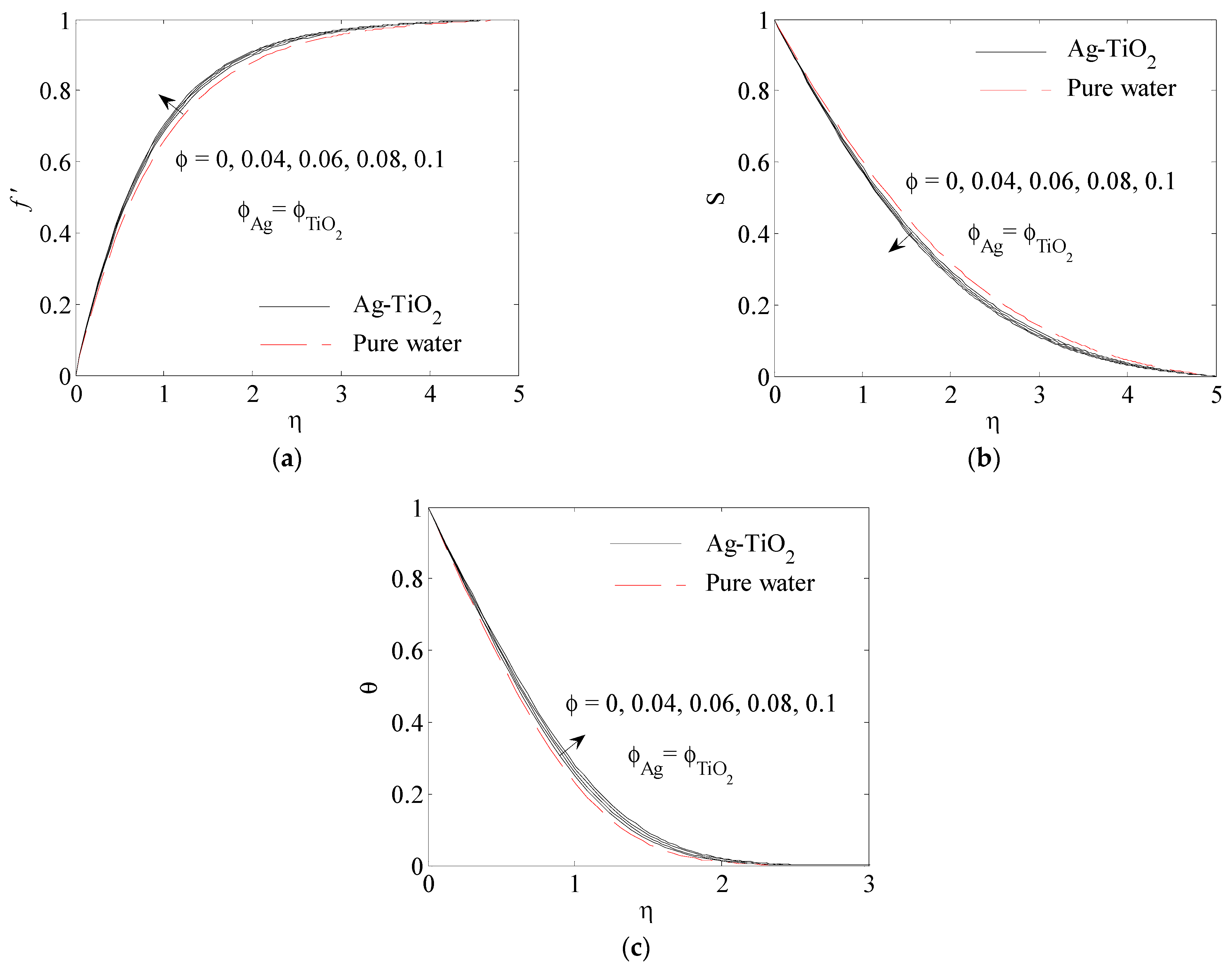

| Physical Property | Water | Ag | TiO2 |
|---|---|---|---|
| Kg m−3 | 997.1 | 10,500 | 4250 |
| J kg−1 K−1 | 4179 | 235 | 686.2 |
| W m−1 K−1 | 0.613 | 429 | 8.9538 |
| 105 K−1 | 21 | 1.89 | 0.9 |
| × 10−6 S m−1 | 5.5 × 10−12 | 63 | 2.4 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
El-Zahar, E.R.; Mahdy, A.E.N.; Rashad, A.M.; Saad, W.; Seddek, L.F. Unsteady MHD Mixed Convection Flow of Non-Newtonian Casson Hybrid Nanofluid in the Stagnation Zone of Sphere Spinning Impulsively. Fluids 2021, 6, 197. https://doi.org/10.3390/fluids6060197
El-Zahar ER, Mahdy AEN, Rashad AM, Saad W, Seddek LF. Unsteady MHD Mixed Convection Flow of Non-Newtonian Casson Hybrid Nanofluid in the Stagnation Zone of Sphere Spinning Impulsively. Fluids. 2021; 6(6):197. https://doi.org/10.3390/fluids6060197
Chicago/Turabian StyleEl-Zahar, Essam R., Abd El Nasser Mahdy, Ahmed M. Rashad, Wafaa Saad, and Laila F. Seddek. 2021. "Unsteady MHD Mixed Convection Flow of Non-Newtonian Casson Hybrid Nanofluid in the Stagnation Zone of Sphere Spinning Impulsively" Fluids 6, no. 6: 197. https://doi.org/10.3390/fluids6060197
APA StyleEl-Zahar, E. R., Mahdy, A. E. N., Rashad, A. M., Saad, W., & Seddek, L. F. (2021). Unsteady MHD Mixed Convection Flow of Non-Newtonian Casson Hybrid Nanofluid in the Stagnation Zone of Sphere Spinning Impulsively. Fluids, 6(6), 197. https://doi.org/10.3390/fluids6060197







