Experimental and Numerical Study of Gas Injection Effect on the Methane–Air Combustion inside a Coaxial Burner
Abstract
1. Introduction
2. Problem Setup
3. Numerical Methods
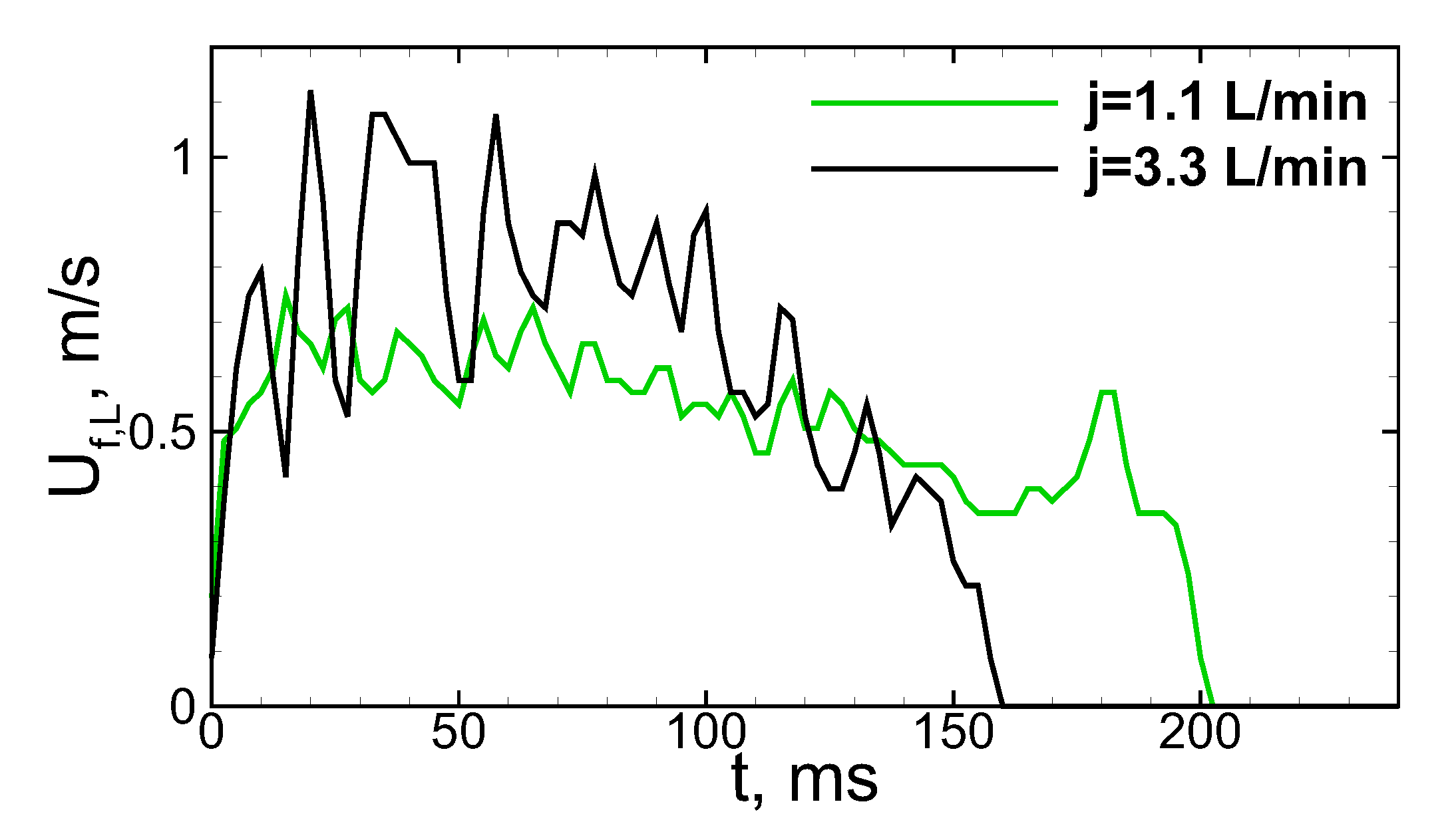
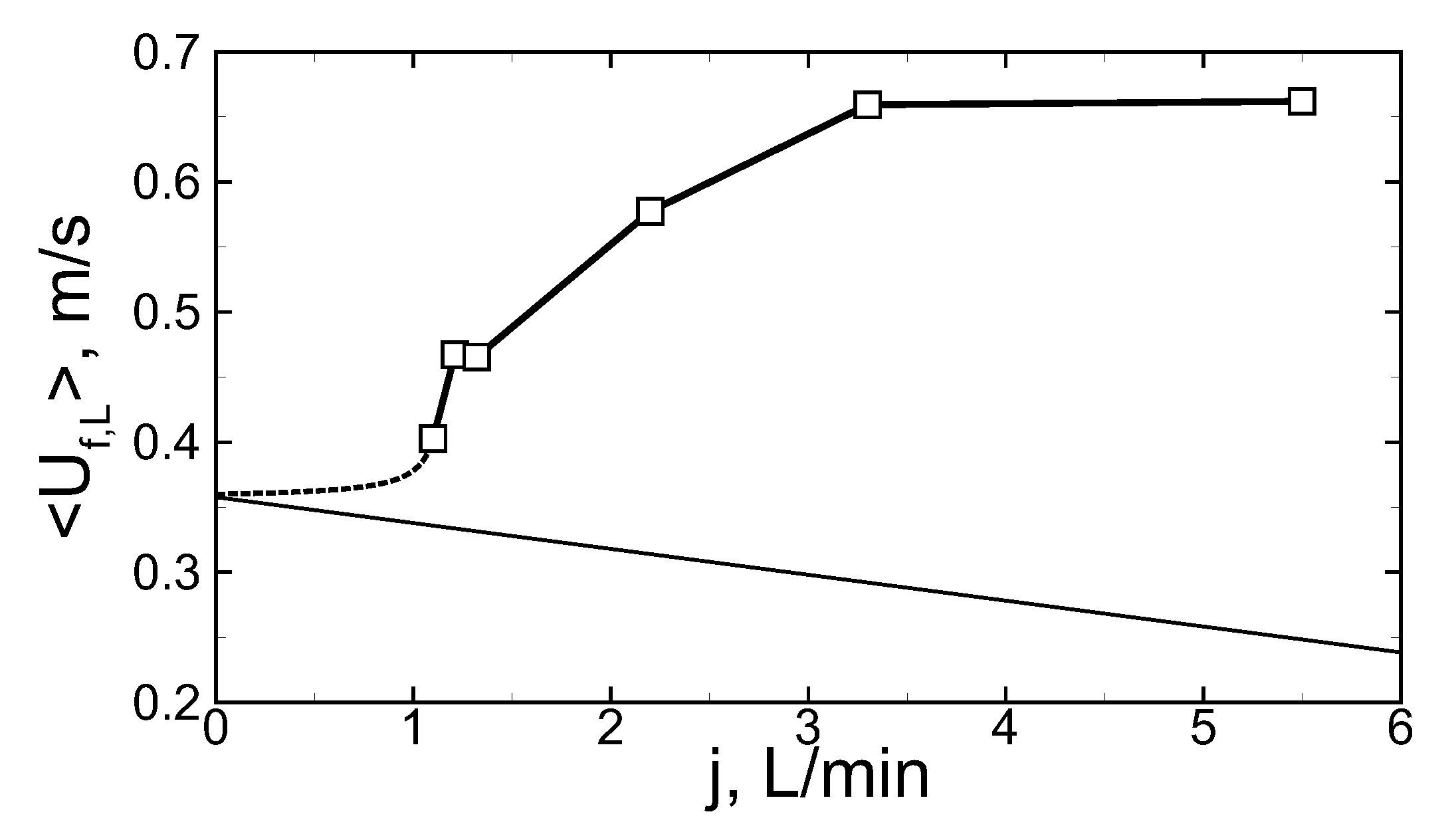
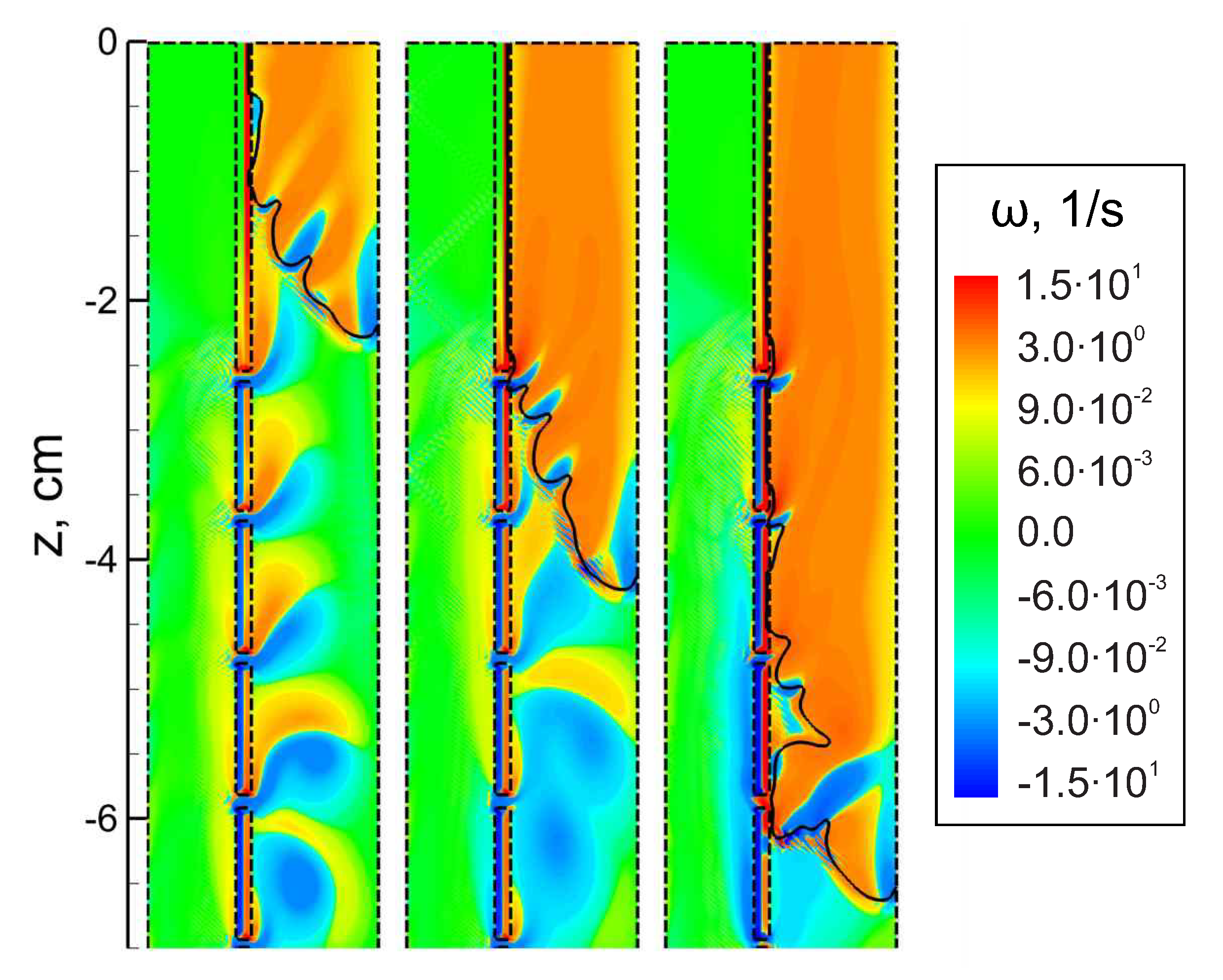
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| JANAF | Joint Army, Navy and Air Force |
References
- Senthil Kumar, M.; Ramesh, A.; Nagalingam, B. Use of hydrogen to enhance the performance of a vegetable oil fuelled compression ignition engine. Int. J. Hydrogen Energy 2003, 28, 1143–1154. [Google Scholar] [CrossRef]
- Aggarwal, S.; Awomolo, O.; Akber, K. Ignition characteristics of heptane–hydrogen and heptane–methane fuel blends at elevated pressures. Int. J. Hydrogen Energy 2011, 36, 15392–15402. [Google Scholar] [CrossRef]
- Ji, C.; Wang, S.; Zhang, B. Performance of a hybrid hydrogen–gasoline engine under various operating conditions. Appl. Energy 2012, 97, 584–589. [Google Scholar] [CrossRef]
- Oh, S.H.; Yoon, S.H.; Song, H.; Han, J.G.; Kim, J.M. Effect of hydrogen nanobubble addition on combustion characteristics of gasoline engine. Int. J. Hydrogen Energy 2013, 38, 14849–14853. [Google Scholar] [CrossRef]
- Ilbas, M.; Yilmaz, I.; Kaplan, Y. Investigations of hydrogen and hydrogen–hydrocarbon composite fuel combustion and emission characteristics in a model combustor. Int. J. Hydrogen Energy 2005, 30, 1139–1147. [Google Scholar] [CrossRef]
- Shudo, T.; Omori, K.; Hiyama, O. NOx reduction and NO2 emission characteristics in rich-lean combustion of hydrogen. Int. J. Hydrogen Energy 2008, 33, 4689–4693. [Google Scholar] [CrossRef]
- Huo, M.; Lin, S.; Liu, H.; Lee, C.F.F. Study on the spray and combustion characteristics of water–emulsified diesel. Fuel 2014, 123, 218–229. [Google Scholar] [CrossRef]
- Pelce, P.; Clavin, P. Influence of hydrodynamics and diffusion upon the stability limits of laminar premixed flames. J. Fluid Mech. 1982, 124, 219. [Google Scholar] [CrossRef]
- Ju, Y.; Maruta, K. Microscale combustion: Technology development and fundamental research. Prog. Energy Combust. Sci. 2011, 37, 669–715. [Google Scholar] [CrossRef]
- Yakovenko, I.S.; Ivanov, M.F.; Kiverin, A.D.; Melnikova, K.S. Large-scale flame structures in ultra-lean hydrogen-air mixtures. Int. J. Hydrogen Energy 2018, 43, 1894–1901. [Google Scholar] [CrossRef]
- Volodin, V.V.; Golub, V.V.; Kiverin, A.D.; Melnikova, K.S.; Mikushkin, A.Y.; Yakovenko, I.S. Large-scale Dynamics of Ultra-lean Hydrogen-air Flame Kernels in Terrestrial Gravity Conditions. Combust. Sci. Technol. 2020. [Google Scholar] [CrossRef]
- Jones, A.R.; Lloyd, S.A.; Weinberg, F.J. Combustion in heat exchangers. Proc. R. Soc. Lond. A. Math. Phys. Sci. 1978, 360, 97–115. [Google Scholar] [CrossRef]
- Ronney, P. Analysis of non-adiabatic heat-recirculating combustors. Combust. Flame 2003, 135, 421–439. [Google Scholar] [CrossRef]
- Ju, Y.; Choi, C. An analysis of sub-limit flame dynamics using opposite propagating flames in mesoscale channels. Combust. Flame 2003, 133, 483–493. [Google Scholar] [CrossRef]
- Sirignano, W.A.; Pham, T.K.; Dunn-Rankin, D. Miniature-scale liquid-fuel-film combustor. Proc. Combust. Inst. 2002, 29, 925–931. [Google Scholar] [CrossRef]
- Kichatov, B.; Korshunov, A.; Kiverin, A.; Son, E. Foamed emulsion—Fuel on the base of water-saturated oils. Fuel 2017, 203, 261–268. [Google Scholar] [CrossRef]
- Kichatov, B.; Korshunov, A.; Kiverin, A.; Son, E. Methods for Regulation of Flame Speed in the Foamed Emulsion. Combust. Sci. Technol. 2017, 189, 2095–2114. [Google Scholar] [CrossRef]
- Kichatov, B.; Korshunov, A.; Kiverin, A.; Son, E. Experimental study of foamed emulsion combustion: Influence of solid microparticles, glycerol and surfactant. Fuel Process. Technol. 2017, 166, 77–85. [Google Scholar] [CrossRef]
- Kichatov, B.; Korshunov, A.; Kiverin, A.; Ivanov, M. Effect of ultrasonic emulsification on the combustion of foamed emulsions. Fuel Process. Technol. 2018, 169, 178–190. [Google Scholar] [CrossRef]
- Kichatov, B.; Korshunov, A.; Kiverin, A.; Saveliev, A. The role of explosive boiling in the process of foamed emulsion combustion. Int. J. Heat Mass Transf. 2018, 119, 199–207. [Google Scholar] [CrossRef]
- Kichatov, B.; Korshunov, A.; Kiverin, A.; Son, E. Combustion of hydrogen–oxygen microfoam on the water base. Int. J. Hydrogen Energy 2017, 42, 16866–16876. [Google Scholar] [CrossRef]
- Leontiev, A.; Saveliev, A.; Kichatov, B.; Kiverin, A.; Korshunov, A.; Sudakov, V. Effect of gaseous coolant temperature on the transpiration cooling for porous wall in the supersonic flow. Int. J. Heat Mass Transf. 2019, 142, 118433. [Google Scholar] [CrossRef]
- Renard, P.H.; Thévenin, D.; Rolon, J.; Candel, S. Dynamics of flame/vortex interactions. Prog. Energy Combust. Sci. 2000, 26, 225–282. [Google Scholar] [CrossRef]
- Kadowaki, S.; Hasegawa, T. Numerical simulation of dynamics of premixed flames: Flame instability and vortex—Flame interaction. Prog. Energy Combust. Sci. 2005, 31, 193–241. [Google Scholar] [CrossRef]
- Searby, G.; Clavin, P. Weakly Turbulent, Wrinkled Flames in Premixed Gases. Combust. Sci. Technol. 1986, 46, 167–193. [Google Scholar] [CrossRef]
- Akkerman, V.; Bychkov, V.; Eriksson, L.E. Numerical study of turbulent flame velocity. Combust. Flame 2007, 151, 452–471. [Google Scholar] [CrossRef]
- Akkerman, V.B.; Bychkov, V.V.; Bastiaans, R.J.M.; de Goey, L.P.H.; van Oijen, J.A.; Eriksson, L.E. Flow-flame interaction in a closed chamber. Phys. Fluids 2008, 20, 055107. [Google Scholar] [CrossRef]
- Akkerman, V.; Ivanov, M.; Bychkov, V. Turbulent Flow Produced by Piston Motion in a Spark-ignition Engine. Flow Turbul. Combust. 2009, 82, 317–337. [Google Scholar] [CrossRef]
- Bychkov, V.; Petchenko, A.; Akkerman, V. Increase of the flame velocity in a rotating gas and the renormalization approach to turbulent burning. Combust. Sci. Technol. 2007, 179, 1231–1259. [Google Scholar] [CrossRef]
- Bradley, D.; Hundy, G. Burning velocities of methane–air mixtures using hot-wire anemometers in closed-vessel explosions. Symp. Int. Combust. 1971, 13, 575–583. [Google Scholar] [CrossRef]
- Kuo, K.K.; Acharya, R. Fundamentals of Turbulent and Multiphase Combustion, 1st ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2012. [Google Scholar]
- McGrattan, K.; McDermott, R.; Hostikka, S.; Floyd, J.; Vanella, M. Fire Dynamics Simulator Technical Reference Guide Volume 1: Mathematical Model. In Technical Report NIST Special Publication 1018-1; U.S. Department of Commerce, National Institute of Standards and Technology: Gaithersburg, MD, USA, 2019. [Google Scholar] [CrossRef]
- Bykov, V.; Kiverin, A.; Koksharov, A.; Yakovenko, I. Analysis of transient combustion with the use of contemporary CFD techniques. Comput. Fluids 2019, 194, 104310. [Google Scholar] [CrossRef]
- Chase, M.W. Data Reported in NIST Standard Reference Database 69, June 2005 Release: NIST Chemistry WebBook. J. Phys. Chem. Ref. Data Monogr. 1998, 9, 1–1951. [Google Scholar]
- Hirschfelder, J.O.; Curtiss, C.F.; Bird, R.B. The Molecular Theory of Gases and Liquids, Revised ed.; Wiley-Interscience: Hoboken, NJ, USA, 1964. [Google Scholar]
- Kee, R.J.; Coltrin, M.E.; Glarborg, P. Chemically Reacting Flow: Theory and Practice, 1st ed.; Wiley-Interscience: Hoboken, NJ, USA, 2003. [Google Scholar]
- Coffee, T.; Heimerl, J. Transport algorithms for premixed, laminar steady-state flames. Combust. Flame 1981, 43, 273–289. [Google Scholar] [CrossRef]
- Franzelli, B.; Riber, E.; Gicquel, L.Y.; Poinsot, T. Large Eddy Simulation of combustion instabilities in a lean partially premixed swirled flame. Combust. Flame 2012, 159, 621–637. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kiverin, A.; Kichatov, B.; Korshunov, A.; Gubernov, V.; Yakovenko, I.; Yarkov, A. Experimental and Numerical Study of Gas Injection Effect on the Methane–Air Combustion inside a Coaxial Burner. Fluids 2021, 6, 60. https://doi.org/10.3390/fluids6020060
Kiverin A, Kichatov B, Korshunov A, Gubernov V, Yakovenko I, Yarkov A. Experimental and Numerical Study of Gas Injection Effect on the Methane–Air Combustion inside a Coaxial Burner. Fluids. 2021; 6(2):60. https://doi.org/10.3390/fluids6020060
Chicago/Turabian StyleKiverin, Alexey, Boris Kichatov, Alexey Korshunov, Vladimir Gubernov, Ivan Yakovenko, and Andrey Yarkov. 2021. "Experimental and Numerical Study of Gas Injection Effect on the Methane–Air Combustion inside a Coaxial Burner" Fluids 6, no. 2: 60. https://doi.org/10.3390/fluids6020060
APA StyleKiverin, A., Kichatov, B., Korshunov, A., Gubernov, V., Yakovenko, I., & Yarkov, A. (2021). Experimental and Numerical Study of Gas Injection Effect on the Methane–Air Combustion inside a Coaxial Burner. Fluids, 6(2), 60. https://doi.org/10.3390/fluids6020060





