Effect of Vertical Vibration on the Mixing Time of a Passive Scalar in a Sparged Bubble Column Reactor
Abstract
1. Introduction
2. Experimental Methods
2.1. Vibrating Bubble Column Facility
2.2. Mixing Time Measurement
2.3. Bubble Size Measurement
2.4. Void Fraction Measurement
2.5. Test Matrix
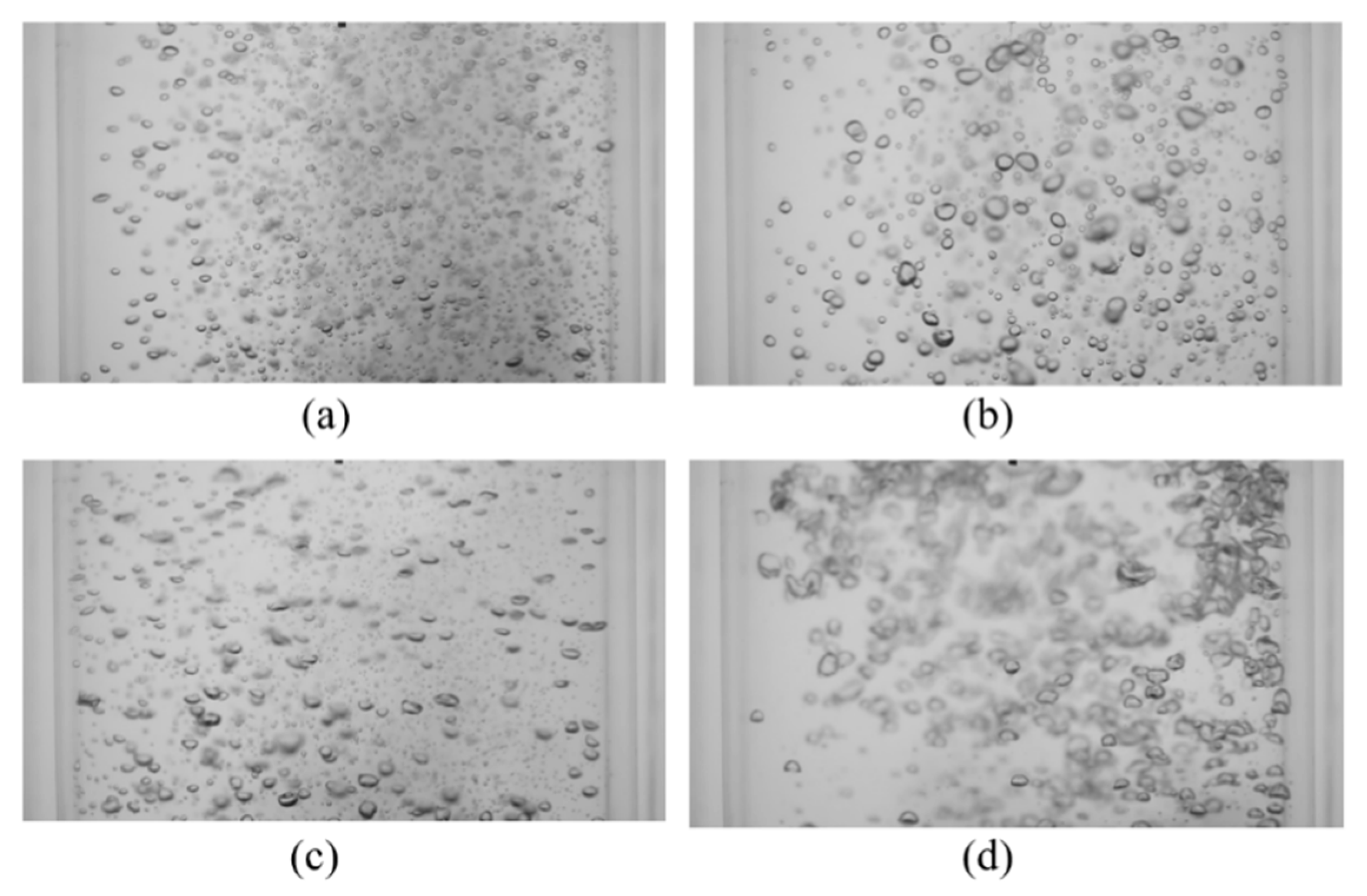
3. Bubble Induced Mixing in a Static Bubble Column
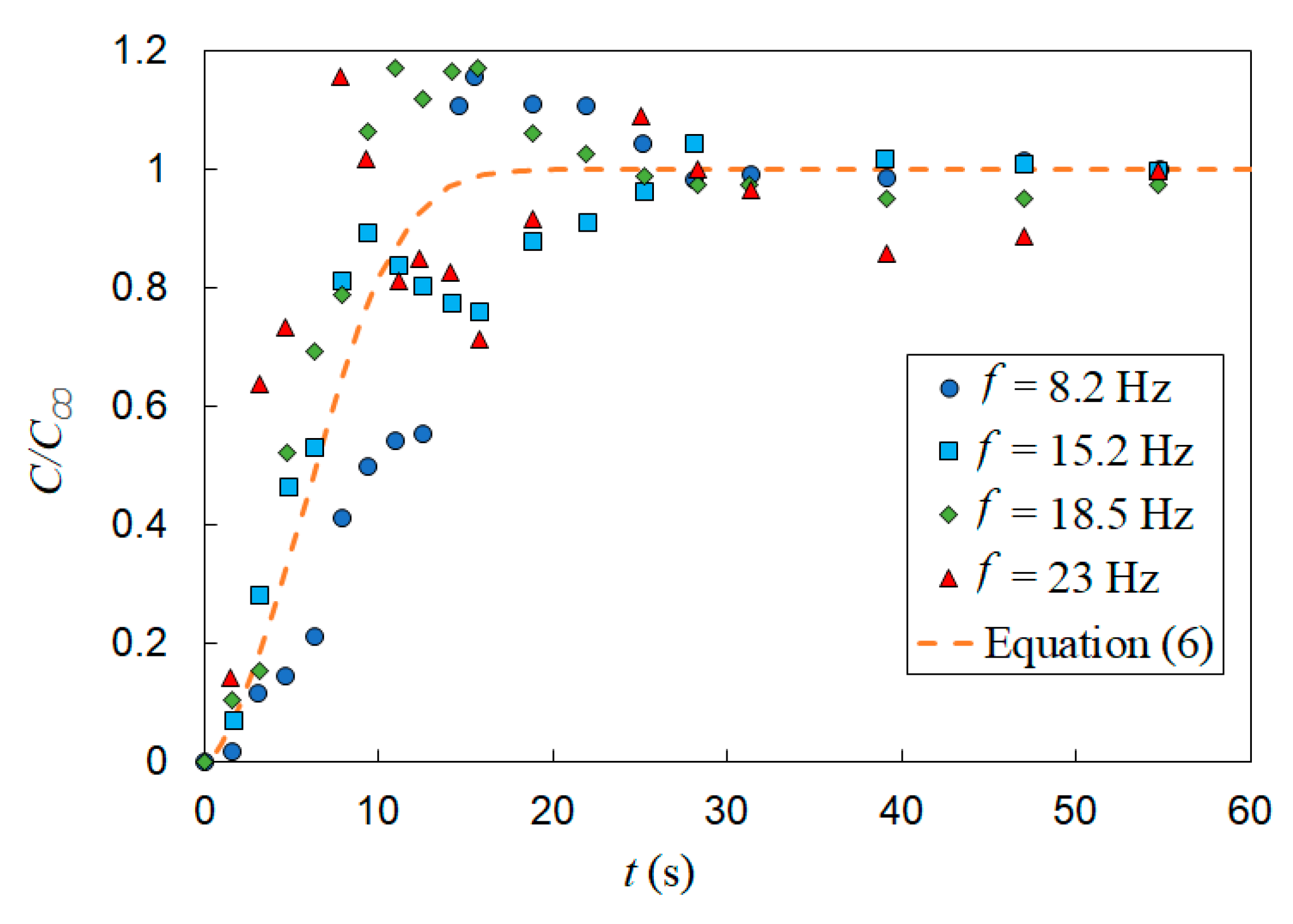
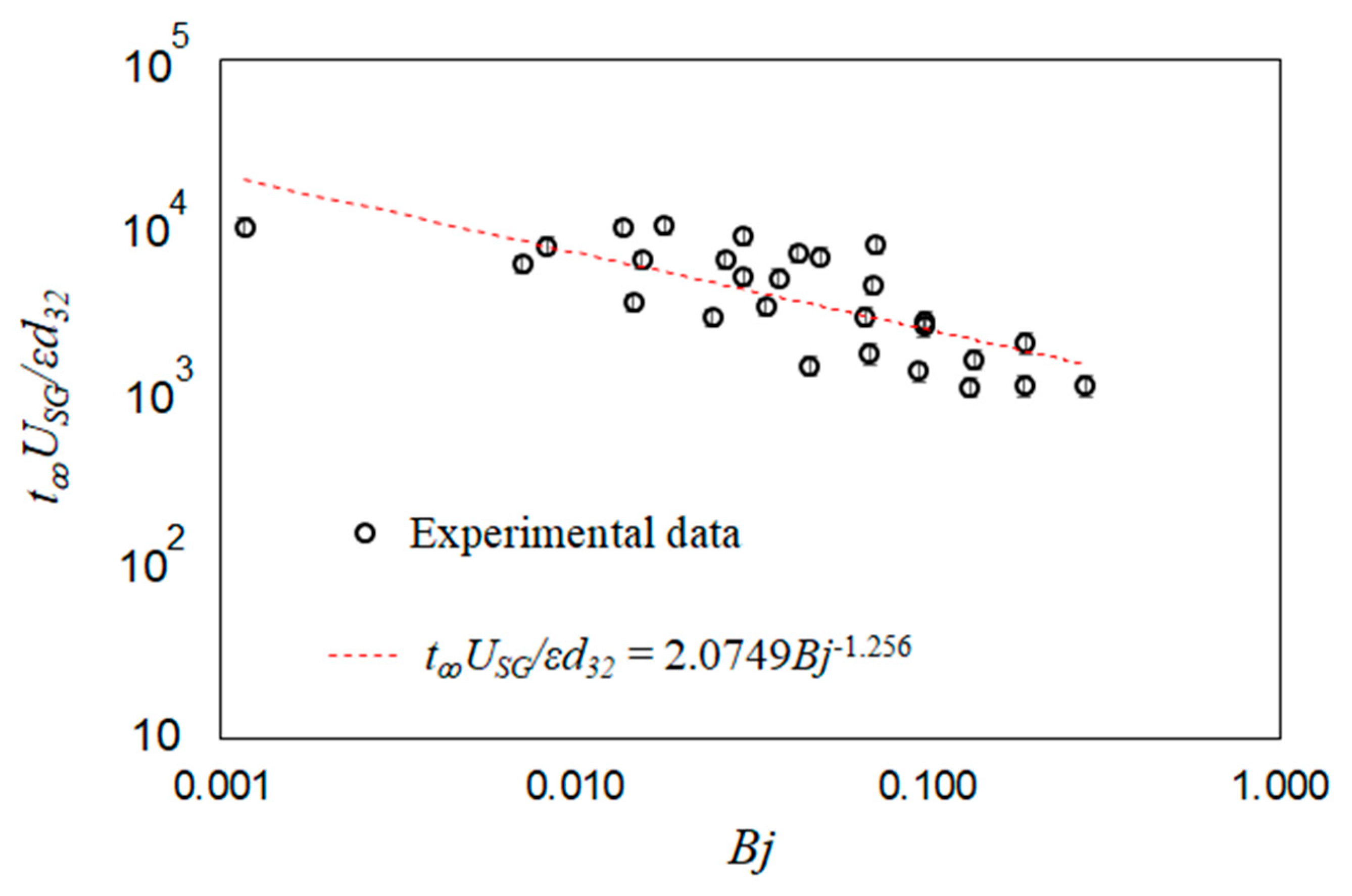
4. Effect of Vertical Vibration on Bubble-Induced Mixing
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| Symbol | Description | Unit |
| a | Phase interfacial area | [mm2] |
| A | Vibration amplitude | [mm] |
| AR | Bubble aspect ratio | [-] |
| Bj | Transient buoyancy (Bjerknes) number | [-] |
| C | Concentration of the passive scalar | [ppm] |
| d | Diameter | [mm] |
| D | Bubble column diameter | [mm] |
| f | Vibration frequency | [s−1] |
| g | Gravitational acceleration | [ms−2] |
| H | Liquid column height | [mm] |
| P | Power input | [kgm3s−3] |
| p | pressure | [kgm−1s−2] |
| Q | Volumetric flow rate | [mLmin−1] |
| r | Pore radius | [μm] |
| Re | Reynolds number | [-] |
| SG | Specific gravity | [-] |
| t | Time | [s] |
| U | Phase velocity | [mms−1] |
| Greek Letters and Symbols | ||
| ∆H | Vertical distance between two pressure taps | [m] |
| ∆h | Monometer reading | [m] |
| ∆P | Differential pressure | [kg m−1s−2] |
| α | Bubble major axis | [mm] |
| β | Bubble minor axis | [mm] |
| ε | Void fraction | [-] |
| μ | Dynamic Viscosity | [kgm−1s−1] |
| ν | Kinematic viscosity | [m2s−1] |
| ρ | Density | [kgm−3] |
| σ | Surface tension | [kgs−2] |
| Φ | scale of segregation | [-] |
| ω | Vibration angular velocity | [s−1] |
| Subscripts | ||
| inj | Injector tube | |
| ps | Passive scalar (dye) | |
| p | Characteristics of the pore sparger | |
| SG | Superficial gas | |
| L | Liquid (phase) | |
| o | Monometer working fluid | |
| m | Specific quantities | |
| 32 | Suater mean diameter | |
| 0 | Ambient properties | |
| G | Gas phase | |
| b | Bubble | |
| cap | Capillary | |
| ∞ | Steady state condition |
References
- Besnaci, C.; Roig, V.; Risso, F. Mixing induced by a random dispersion at high particulate Reynolds number. In Proceedings of the 7th International Conference on Multiphase Flow, Tampa, FL, USA, 30 May–4 June 2010. [Google Scholar]
- Lance, M.; Bataille, J. Turbulence in the liquid phase of a uniform bubbly air-water flow. J. Fluid Mech. 1991, 222, 95–118. [Google Scholar] [CrossRef]
- Martínez-Mercado, J.; Palacios-Morales, C.A.; Zenit, R. Measurement of pseudoturbulence intensity in monodispersed bubbly liquids for 10 < Re < 500. Phys. Fluids 2007, 19, 103302. [Google Scholar]
- Riboux, G.; Risso, F.; Legendre, D. Experimental characterization of the agitation generated by bubbles rising at high Reynolds number. J. Fluid Mech. 2010, 643, 509–539. [Google Scholar] [CrossRef]
- Mercado, J.M.; Gomez, D.C.; Van Gils, D.; Sun, C.; Lohse, D. On bubble clustering and energy spectra in pseudo-turbulence. J. Fluid Mech. 2010, 650, 287–306. [Google Scholar] [CrossRef]
- Mendez-Diaz, S.; Serrano-Garcia, J.C.; Zenit, R.; Hernandez-Cordero, J.A. Power spectral distributions of pseudo-turbulent bubbly flows. Phys. Fluids 2013, 25, 043303. [Google Scholar] [CrossRef]
- Alméras, E.; Risso, F.; Roig, V.; Cazin, S.; Plais, C.; Augier, F. Mixing by bubble-induced turbulence. J. Fluid Mech. 2015, 776, 458–474. [Google Scholar] [CrossRef]
- Bouche, E.; Cazin, S.; Roig, V.; Risso, F. Mixing in a swarm of bubbles rising in a confined cell measured by mean of PLIF with two different dyes. Exp. Fluids 2013, 54, 1552. [Google Scholar] [CrossRef][Green Version]
- Bouche, E.; Roig, V.; Risso, F.; Billet, A.M. Homogeneous swarm of high-Reynolds-number bubbles rising within a thin gap. Part 2. Liquid dynamics. J. Fluid Mech. 2014, 758, 508–521. [Google Scholar] [CrossRef]
- Alméras, E.; Cazin, S.; Roig, V.; Risso, F.; Augier, F.; Plais, C. Time-resolved measurement of concentration fluctuations in a confined bubbly flow by LIF. Int. J. Multiph. Flow 2016, 83, 153–161. [Google Scholar] [CrossRef]
- Alméras, E.; Plais, C.; Euzenat, F.; Risso, F.; Roig, V.; Augier, F. Scalar mixing in bubbly flows: Experimental investigation and diffusivity modelling. Chem. Eng. Sci. 2016, 140, 114–122. [Google Scholar] [CrossRef]
- Alméras, E.; Plais, C.; Roig, V.; Risso, F.; Augier, F. Mixing mechanisms in a low-sheared inhomogeneous bubble column. Chem. Eng. Sci. 2018, 186, 52–61. [Google Scholar] [CrossRef]
- Besagni, G.; Inzoli, F.; Ziegenhein, T. Two-Phase Bubble Columns: A Comprehensive Review. ChemEngineering 2018, 2, 13. [Google Scholar] [CrossRef]
- Mareuge, I.; Lance, M. Bubble-induced dispersion of a passive scalar in bubbly flows. In Proceedings of the 2nd International Conference on Multiphase Flow, Kyoto, Japan, 3–7 April 1995. PT1-3–8. [Google Scholar]
- Abbas, M.; Billet, A.M.; Roig, V. Experiments on mass transfer and mixing in a homogeneous bubbly flow. In ICHMT Digital Library Online; Begel House Inc: Danbury, CT, USA, 2009. [Google Scholar]
- Loisy, A. Direct Numerical Simulation of Bubbly Flows: Coupling with Scalar Transport and Turbulence. Ph.D. Thesis, Université de Lyon, Lyon, France, 2016. [Google Scholar]
- Wiemann, D.; Mewes, D. Prediction of backmixing and mass transfer in bubble columns using a multifluid model. Ind. Eng. Chem. Res. 2005, 44, 4959–4967. [Google Scholar] [CrossRef]
- Radl, S.; Khinast, J.G. Multiphase flow and mixing in dilute bubble swarms. AICHE J. 2010, 56, 2421–2445. [Google Scholar] [CrossRef]
- Mohagheghian, S.; Elbing, B.R. Study of bubble size and velocity in a vibrating bubble column. In Proceedings of the ASME 2016 Fluids Engineering Division Summer Meeting Collocated with the ASME 2016 Heat Transfer Summer Conference and the ASME 2016 14th International Conference on Nanochannels, Microchannels, and Minichannels, Washington, DC, USA, 10–14 July 2016; p. V01BT33A012. [Google Scholar]
- Mohagheghian, S.; Elbing, B.R. Characterization of Bubble Size Distributions within a Bubble Column. Fluids 2018, 3, 13. [Google Scholar] [CrossRef]
- Mohagheghian, S.; Still, A.; Elbing, B.; Ghajar, A. Study of bubble size, void fraction, and mass transport in a bubble column under high amplitude vibration. ChemEngineering 2018, 2, 16. [Google Scholar] [CrossRef]
- Mohagheghian, S. Study of Bubbly and Annular Flow Using Quantitative Flow Visualization. Ph.D. Thesis, Mechanical & Aerospace Engineering, Oklahoma State University, Stillwater, OK, USA, 2019. [Google Scholar]
- Besagni, G.; Inzoli, F.; Ziegenhein, T.; Lucas, D. Computational Fluid-Dynamic modeling of the pseudo-homogeneous flow regime in large-scale bubble columns. Chem. Eng. Sci. 2017, 160, 144–160. [Google Scholar] [CrossRef]
- Wilkinson, P.M.; Spek, A.P.; van Dierendonck, L.L. Design parameters estimation for scale-up of high-pressure bubble columns. AICHE J. 1992, 38, 544–554. [Google Scholar] [CrossRef]
- Still, A. Multiphase Phenomena in a Vibrating Bubble Column Reactor. Master’s Thesis, Mechanical & Aerospace Engineering, Oklahoma State University, Stillwater, OK, USA, 2010. [Google Scholar]
- Houghton, G.; McLean, A.M.; Ritchie, P.D. Mechanism of formation of gas bubble-beds. Chem. Eng. Sci. 1957, 7, 40–50. [Google Scholar] [CrossRef]
- Elbing, B.R.; Winkel, E.S.; Ceccio, S.L.; Perlin, M.; Dowling, D.R. High-reynolds-number turbulent-boundary-layer wall-pressure fluctuations with dilute polymer solutions. Phys. Fluids 2010, 22, 085104. [Google Scholar] [CrossRef]
- Abràmoff, M.D.; Magalhães, P.J.; Ram, S.J. Image processing with ImageJ. Biophotonics Int. 2004, 11, 36–42. [Google Scholar]
- Peters, R.; Rasband, W.S. ImageJ; US National Institutes of Health: Bethesda, MD, USA, 2012.
- Rasband, W.S. ImageJ. Available online: http://imagej.nih.gov/ij (accessed on 16 April 2013).
- Schneider, C.A.; Rasband, W.S.; Eliceiri, K.W. NIH Image to ImageJ: 25 years of image analysis. Nat. Methods 2012, 9, 671. [Google Scholar] [CrossRef] [PubMed]
- Knopf, F.C.; Waghmare, Y.; Ma, J.; Rice, R.G. Pulsing to improve bubble column performance: II. Jetting gas rates. AIChE J. 2006, 52, 1116–1126. [Google Scholar] [CrossRef]
- Jameson, G.J.; Davidson, J.F. The motion of a bubble in a vertically oscillating liquid: Theory for an inviscid liquid, and experimental results. Chem. Eng. Sci. 1966, 21, 29–34. [Google Scholar] [CrossRef]
- Jameson, G.J. The motion of a bubble in a vertically oscillating viscous liquid. Chem. Eng. Sci. 1966, 21, 35–48. [Google Scholar] [CrossRef]
- Houghton, G. Particle trajectories and terminal velocities in vertically oscillating fluids. Can. J. Chem. Eng. 1966, 44, 90–95. [Google Scholar]
- Rubin, E. Behavior of gas bubbles in vertically vibrating liquid columns. Can. J. Chem. Eng. 1986, 46, 145–149. [Google Scholar] [CrossRef]
- Foster, J.M.; Botts, J.A.; Barbin, A.R.; Vachon, R.I. Bubble trajectories and equilibrium levels in vibrated liquid columns. J. Basic Eng. 1986, 90, 125–132. [Google Scholar] [CrossRef]
- Marmur, A.; Rubin, E. Viscous effect on stagnation depth of bubbles in a vertically oscillating liquid column. Can. J. Chem. Eng. 1976, 54, 509–514. [Google Scholar] [CrossRef]
- Still, A.L.; Ghajar, A.J.; O’Hern, T.J. Effect of amplitude on mass transport, void fraction and bubble size in a vertically vibrating liquid-gas bubble column reactor. In Proceedings of the ASME Fluids Engineering Summer Meeting-FEDSM2013-16116, Incline Village, NV, USA, 7–11 July 2013; pp. 7–11. [Google Scholar]
- Waghmare, Y.G.; Dorao, C.A.; Jakobsen, H.A.; Knopf, F.C.; Rice, R.G. Bubble size distribution for a bubble column reactor undergoing forced oscillations. Ind. Eng. Chem. Res. 2009, 48, 1786–1796. [Google Scholar] [CrossRef]
- Waghmare, Y.G.; Knopf, F.C.; Rice, R.G. The Bjerknes effect: Explaining pulsed-flow behavior in bubble columns. AICHE J. 2007, 53, 1678–1686. [Google Scholar] [CrossRef]
- Waghmare, Y.G.; Rice, R.G.; Knopf, F.C. Mass transfer in a viscous bubble column with forced oscillations. Ind. Eng. Chem. Res. 2008, 47, 5386–5394. [Google Scholar] [CrossRef]
- Budzyński, P.; Dziubiński, M. Intensification of bubble column performance by introduction pulsation of liquid. Chem. Eng. Process. 2014, 78, 44–57. [Google Scholar] [CrossRef]
- Budzyński, P.; Gwiazda, A.; Dziubiński, M. Intensification of mass transfer in a pulsed bubble column. Chem. Eng. Process. 2017, 112, 18–30. [Google Scholar] [CrossRef]
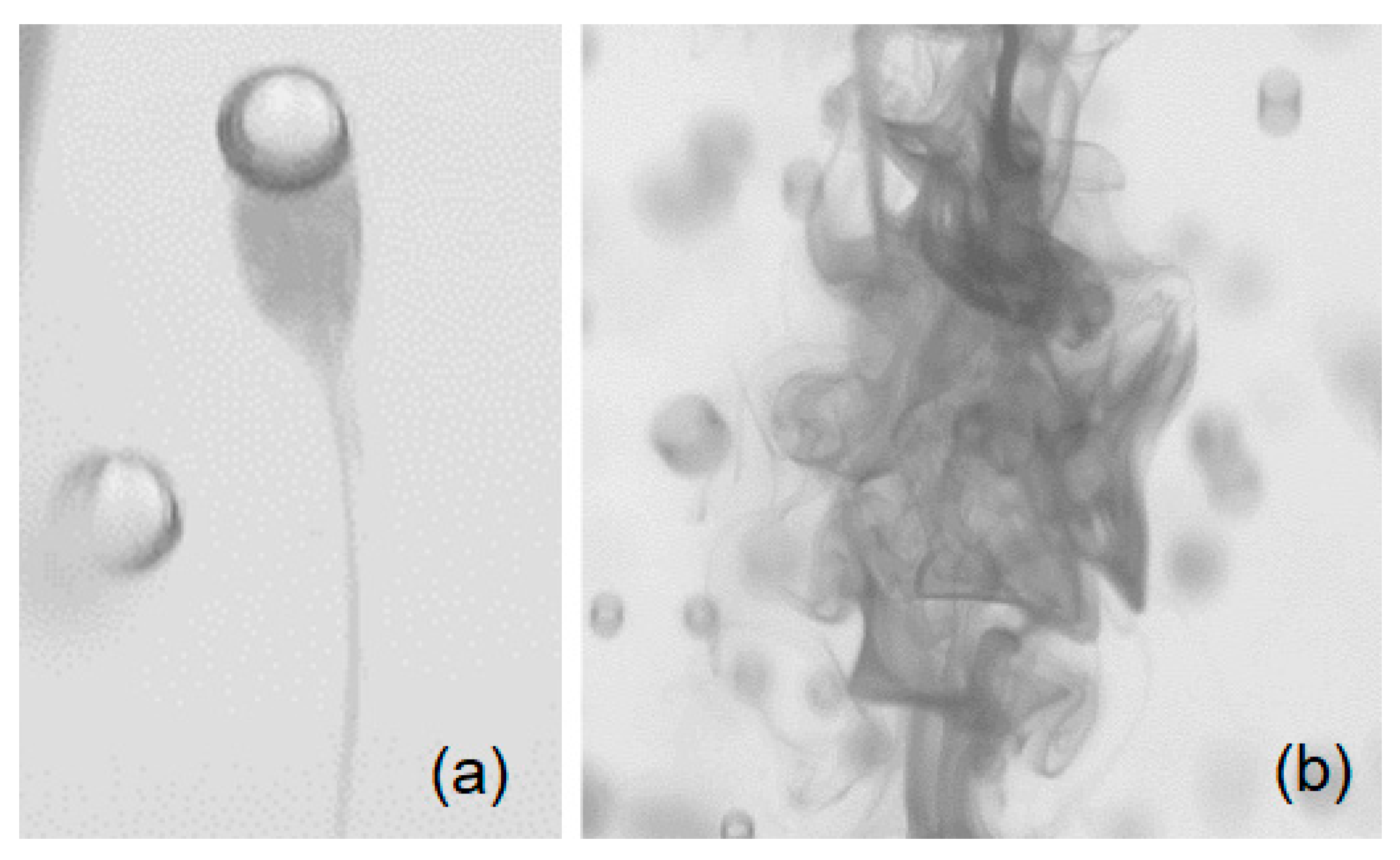
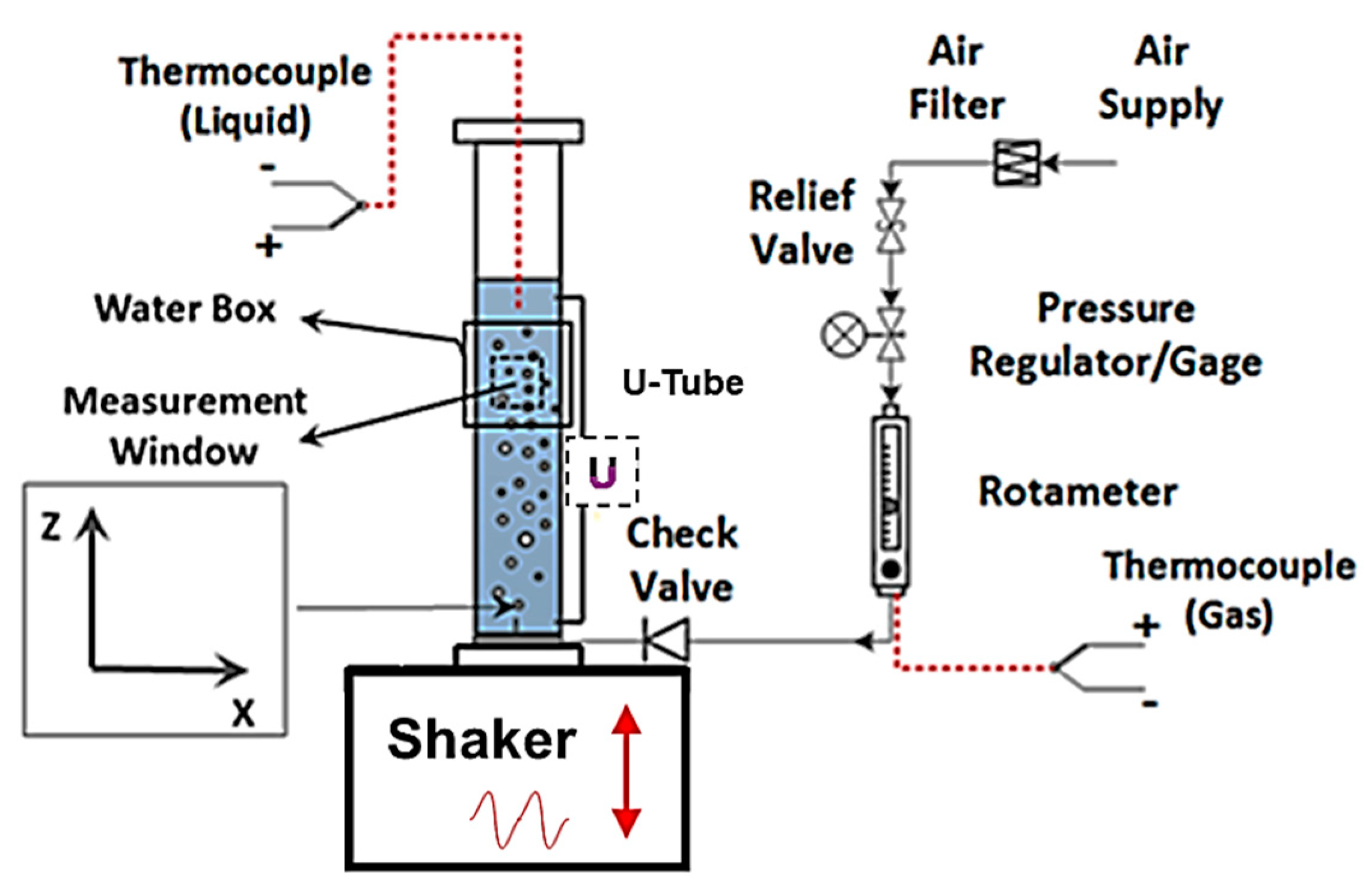
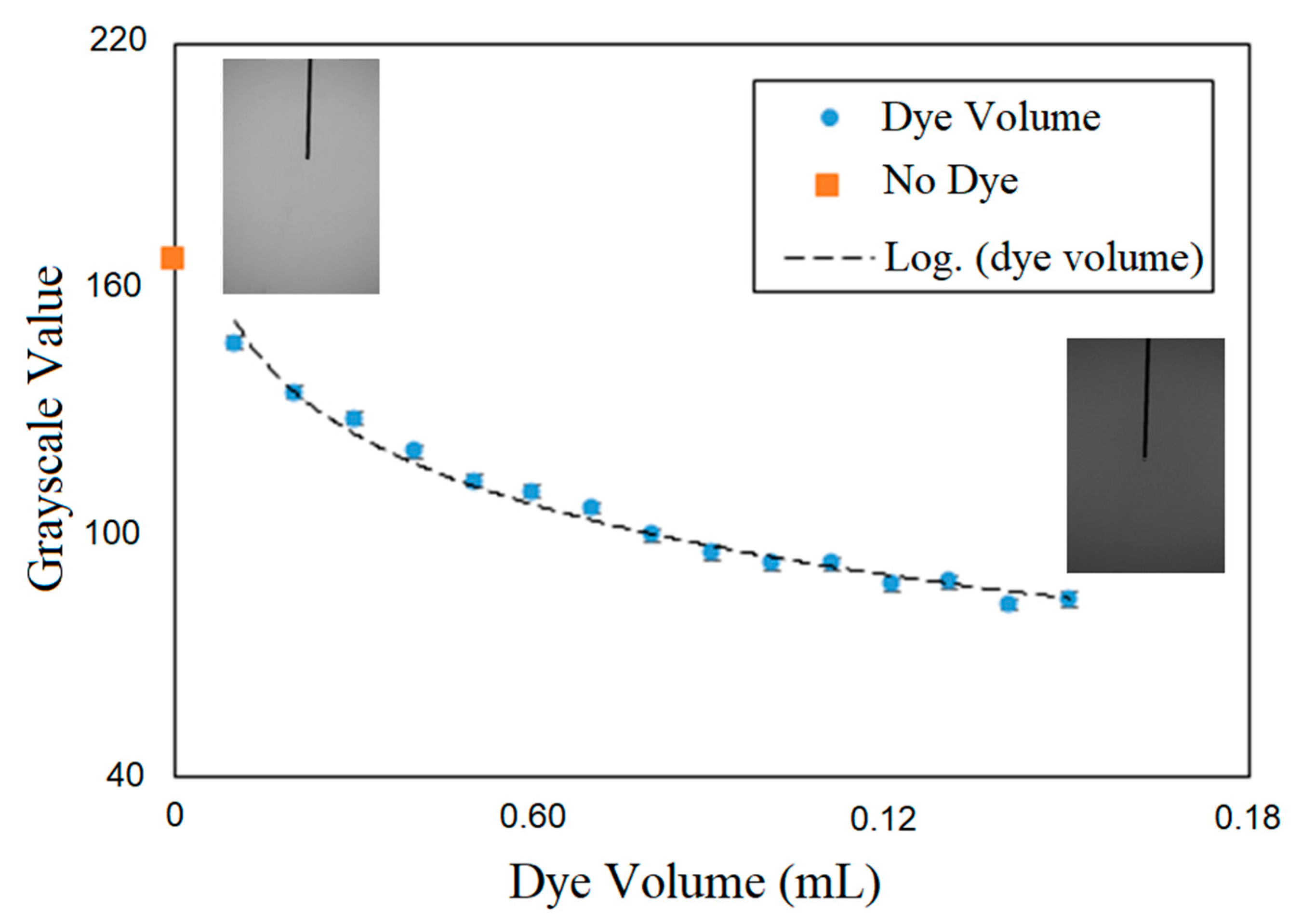

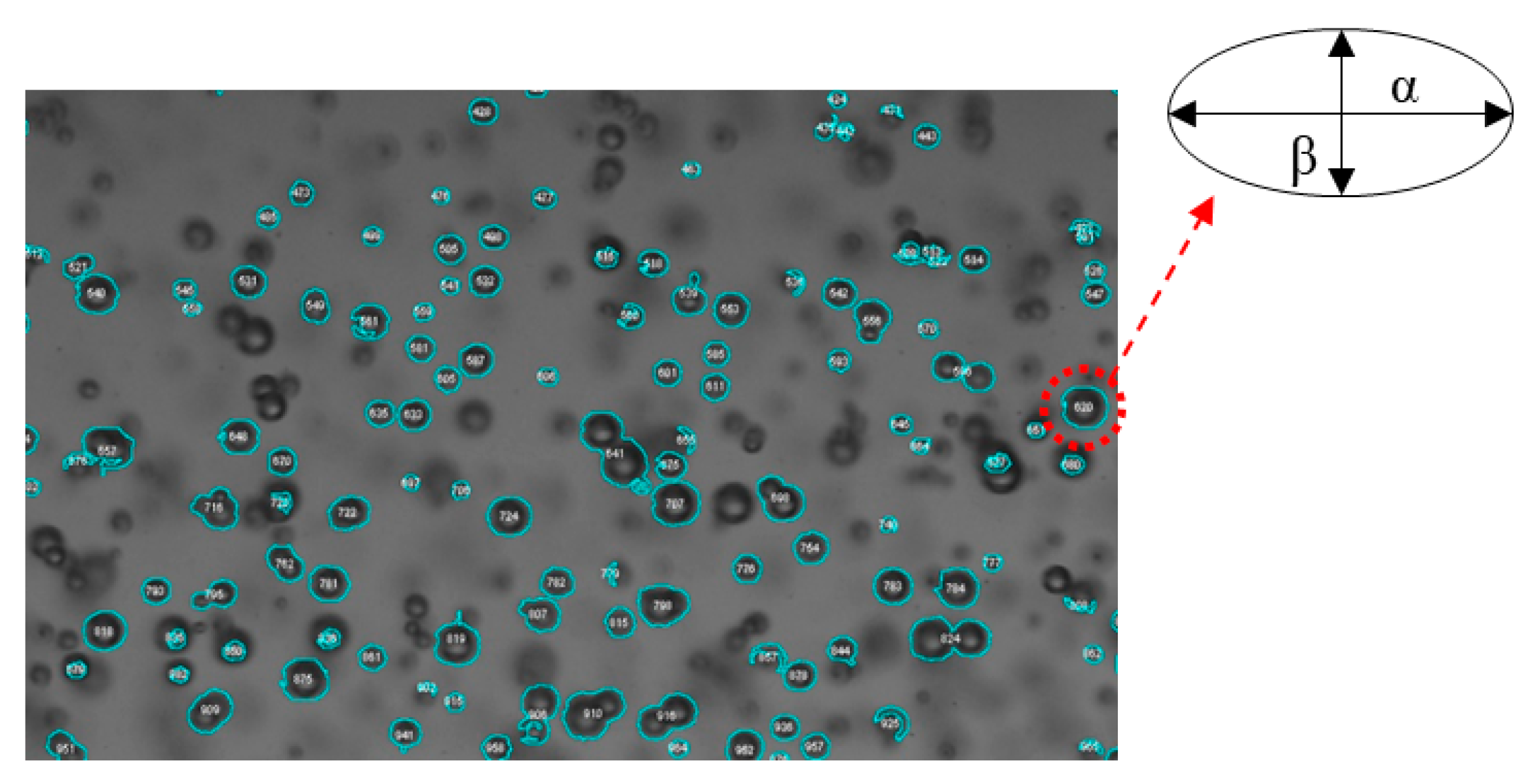

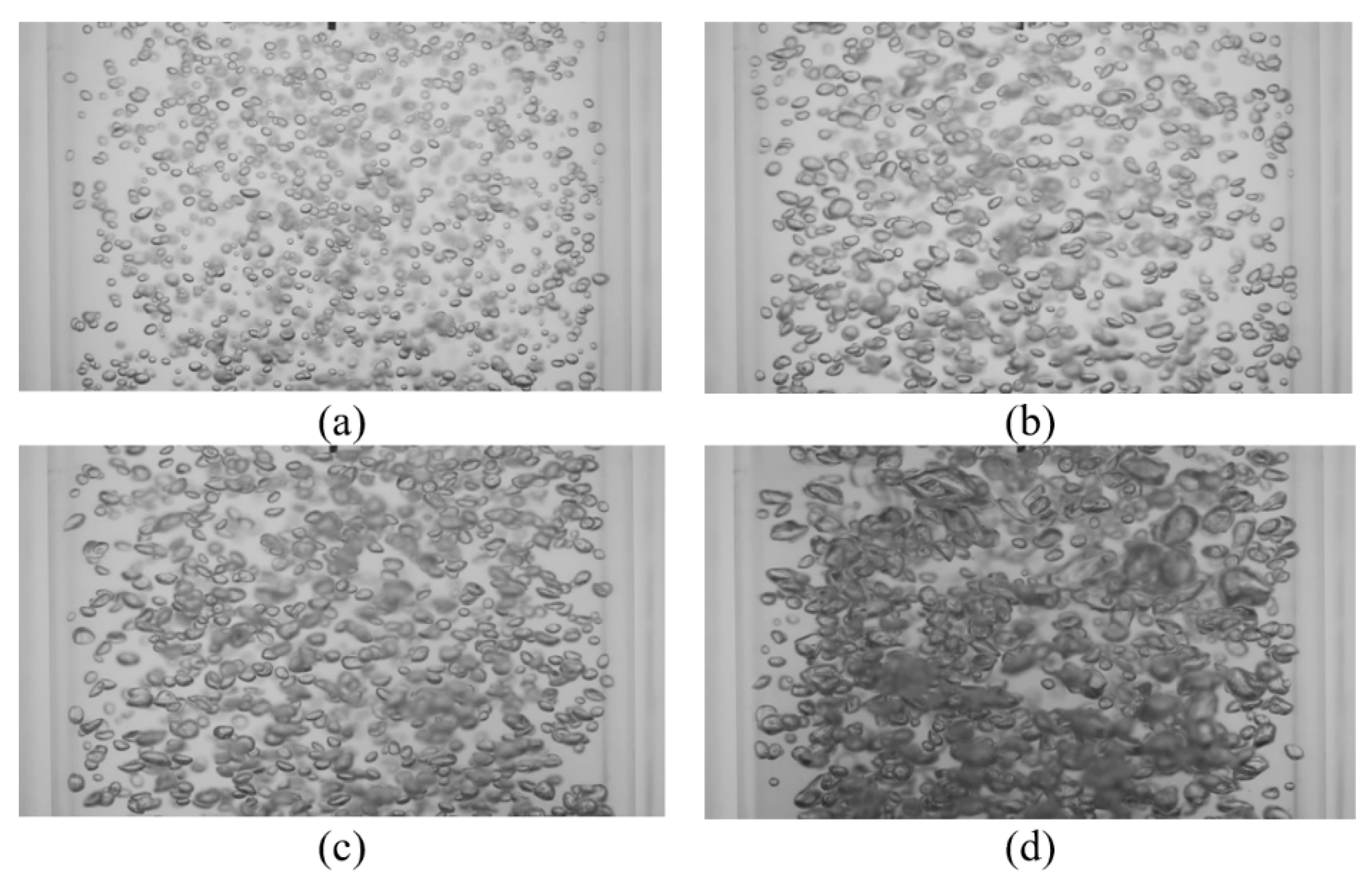
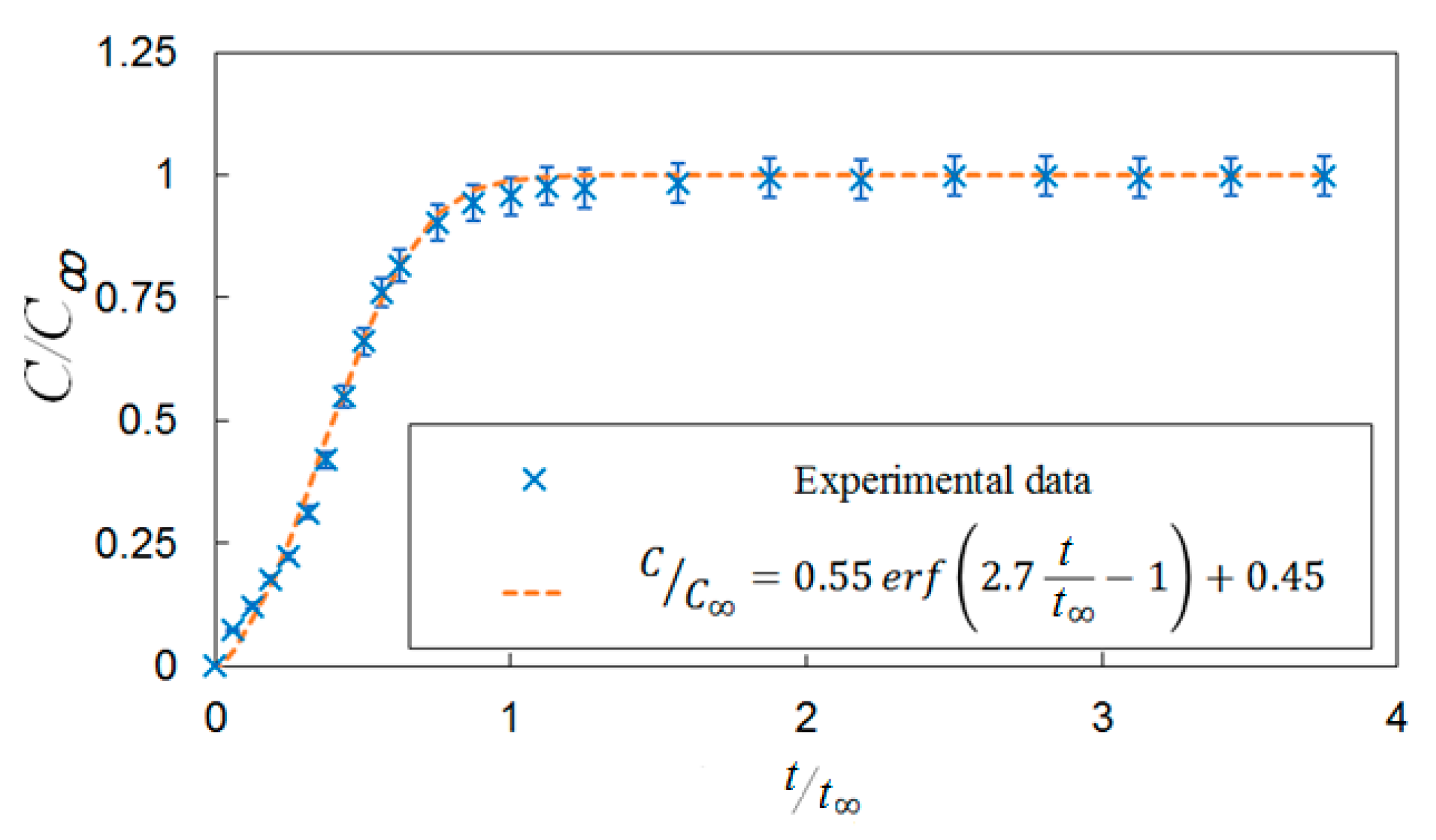

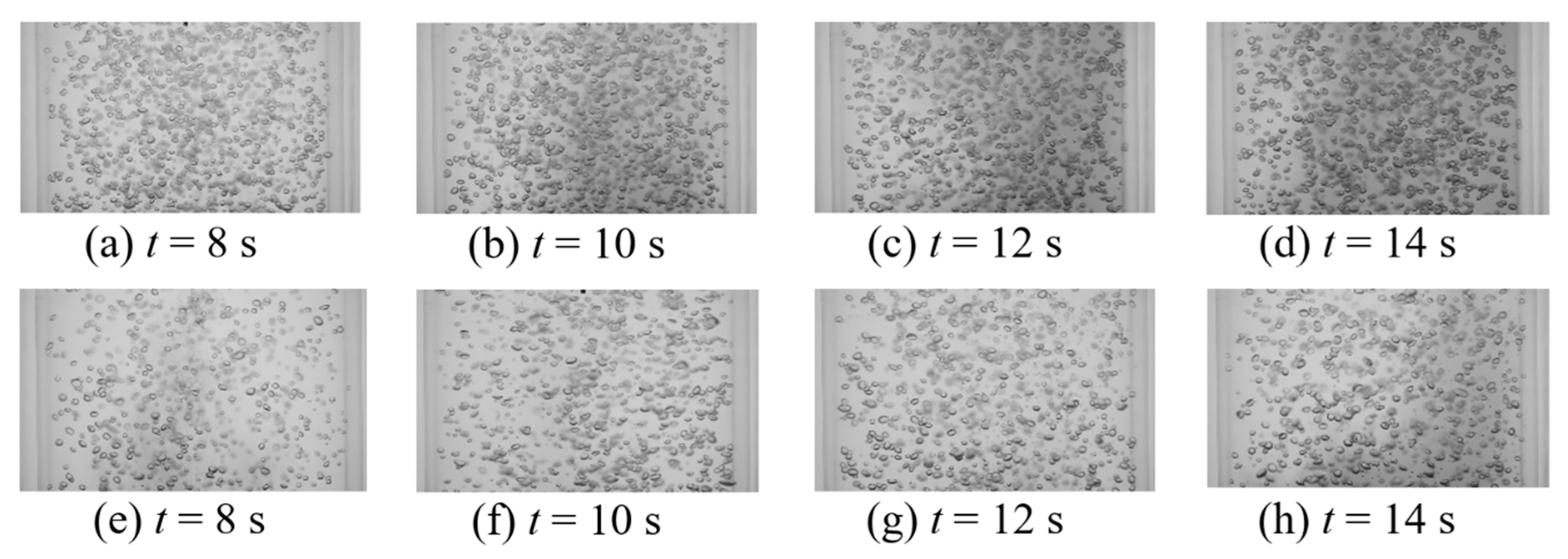
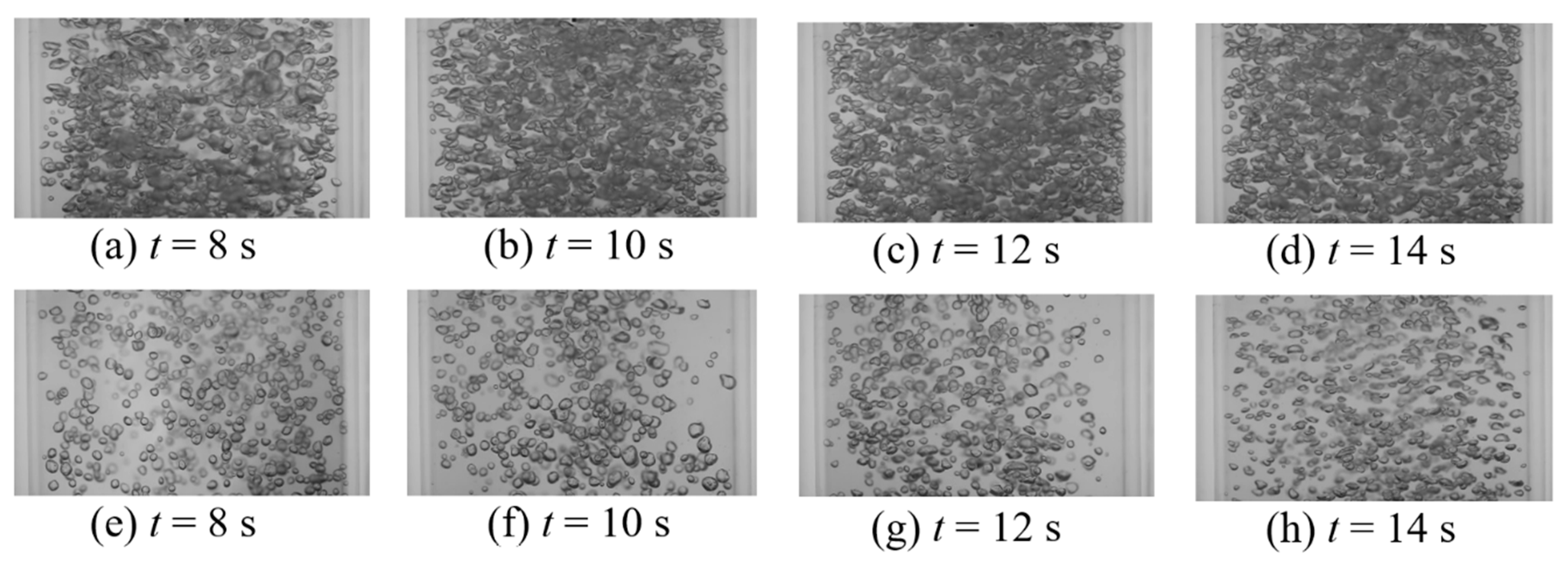


| # | USG (mm/s) | Pm (W/kg) | ε (-) | d32 (mm) | t∞ (s) |
|---|---|---|---|---|---|
| 1 | 13.8 | 0.14 | 2.6% | 2.35 | 16 |
| 2 | 27.6 | 0.27 | 3.4% | 2.51 | 16 |
| 3 | 41.4 | 0.41 | 4.2% | 2.56 | 16 |
| 4 | 55.2 | 0.54 | 5.0% | 2.69 | 16 |
| 5 | 69.0 | 0.68 | 5.9% | 2.86 | 16 |
| # | USG (mm/s) | A (mm) | f (Hz) | Pm (W/kg) | Bj (-) | ε (-) | d32 (mm) | t∞ (s) |
|---|---|---|---|---|---|---|---|---|
| 1 | 9.6 | 0.6 | 9.7 | 0.13 | 0.002 | 1.1% | 2.45 | 25 |
| 2 | 12.4 | 0.6 | 14.5 | 0.26 | 0.011 | 1.0% | 2.64 | 25 |
| 3 | 27.6 | 0.6 | 15.4 | 0.43 | 0.014 | 1.1% | 2.88 | 25 |
| 4 | 31.7 | 0.6 | 20.1 | 0.67 | 0.042 | 1.2% | 2.60 | 20 |
| 5 | 11 | 0.6 | 8 | 0.13 | 0.001 | 1.1% | 2.45 | 25 |
| 6 | 11 | 0.6 | 15 | 0.26 | 0.013 | 1.0% | 2.64 | 25 |
| 7 | 11 | 0.6 | 18.8 | 0.40 | 0.032 | 1.1% | 2.88 | 25 |
| 8 | 11 | 0.6 | 23.3 | 0.67 | 0.076 | 1.2% | 2.60 | 25 |
| 9 | 11 | 0.6 | 9.5 | 0.15 | 0.072 | 1.5% | 2.35 | 25 |
| 10 | 11 | 1.2 | 9.5 | 0.26 | 0.008 | 1.7% | 2.02 | 25 |
| 11 | 11 | 1.2 | 11.5 | 0.38 | 0.018 | 1.3% | 2.77 | 35 |
| 12 | 11 | 1.2 | 13.1 | 0.51 | 0.030 | 1.7% | 3.04 | 25 |
| 13 | 11 | 1.2 | 14.3 | 0.63 | 0.043 | 1.6% | 2.94 | 30 |
| 14 | 11 | 1.6 | 9.5 | 0.39 | 0.007 | 1.5% | 2.70 | 25 |
| 15 | 11 | 1.6 | 11 | 0.53 | 0.016 | 1.4% | 3.09 | 25 |
| 16 | 11 | 1.6 | 12 | 0.66 | 0.027 | 1.4% | 3.15 | 20 |
| 17 | 11 | 1.6 | 14 | 0.98 | 0.038 | 2.5% | 2.32 | 25 |
| 18 | 11 | 1.9 | 9.5 | 0.49 | 0.070 | 2.9% | 1.74 | 16 |
| 19 | 11 | 1.9 | 9.9 | 0.54 | 0.025 | 2.1% | 2.76 | 16 |
| 20 | 11 | 1.9 | 10.8 | 0.67 | 0.035 | 2.1% | 2.54 | 17 |
| 21 | 11 | 1.9 | 12.7 | 1.03 | 0.067 | 2.2% | 2.76 | 17 |
| 22 | 11 | 1.9 | 14 | 1.34 | 0.099 | 2.5% | 2.73 | 18 |
| 23 | 11 | 3.3 | 9.5 | 1.27 | 0.063 | 1.9% | 3.13 | 10 |
| 24 | 11 | 3.3 | 8.8 | 1.03 | 0.047 | 3.0% | 3.08 | 13 |
| 25 | 11 | 3.3 | 9.7 | 1.34 | 0.069 | 1.9% | 3.13 | 10 |
| 26 | 11 | 3.3 | 10.6 | 1.72 | 0.098 | 2.0% | 3.08 | 15 |
| 27 | 11 | 3.3 | 11.5 | 2.16 | 0.136 | 2.7% | 3.13 | 13 |
| 28 | 11 | 3.3 | 12.5 | 2.75 | 0.190 | 2.3% | 2.98 | 13 |
| 29 | 11 | 5.7 | 8 | 2.17 | 0.095 | 2.8% | 3.02 | 11 |
| 30 | 11 | 5.7 | 8.7 | 2.76 | 0.133 | 3.9% | 2.88 | 12 |
| 31 | 11 | 5.7 | 9.5 | 3.56 | 0.189 | 3.6% | 2.82 | 11 |
| 32 | 11 | 5.7 | 10.5 | 4.77 | 0.282 | 3.9% | 2.40 | 10 |
| # | USG (mm/s) | A (mm) | f (Hz) | Pm (W/kg) | t∞ (s) |
|---|---|---|---|---|---|
| 1 | 11 | 0.6 | 8 | 0.13 | 25 |
| 2 | 11 | 0.6 | 15 | 0.26 | 25 |
| 3 | 11 | 0.6 | 18.8 | 0.41 | 25 |
| 4 | 11 | 0.6 | 23.3 | 0.67 | 25 |
| # | USG (mm/s) | A (mm) | f (Hz) | Pm (W/kg) | t∞ (s) |
|---|---|---|---|---|---|
| 1 | 11 | 1.2 | 9.5 | 0.26 | 25 |
| 2 | 11 | 1.6 | 9.5 | 0.39 | 25 |
| 3 | 11 | 1.9 | 9.5 | 0.49 | 16 |
| 4 | 11 | 3.3 | 9.5 | 1.27 | 10 |
| 5 | 11 | 5.7 | 9.5 | 3.56 | 11 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mohagheghian, S.; Ghajar, A.J.; Elbing, B.R. Effect of Vertical Vibration on the Mixing Time of a Passive Scalar in a Sparged Bubble Column Reactor. Fluids 2020, 5, 6. https://doi.org/10.3390/fluids5010006
Mohagheghian S, Ghajar AJ, Elbing BR. Effect of Vertical Vibration on the Mixing Time of a Passive Scalar in a Sparged Bubble Column Reactor. Fluids. 2020; 5(1):6. https://doi.org/10.3390/fluids5010006
Chicago/Turabian StyleMohagheghian, Shahrouz, Afshin J. Ghajar, and Brian R. Elbing. 2020. "Effect of Vertical Vibration on the Mixing Time of a Passive Scalar in a Sparged Bubble Column Reactor" Fluids 5, no. 1: 6. https://doi.org/10.3390/fluids5010006
APA StyleMohagheghian, S., Ghajar, A. J., & Elbing, B. R. (2020). Effect of Vertical Vibration on the Mixing Time of a Passive Scalar in a Sparged Bubble Column Reactor. Fluids, 5(1), 6. https://doi.org/10.3390/fluids5010006






