Progress in Phenomenological Modeling of Turbulence Damping around a Two-Phase Interface
Abstract
1. Introduction
2. Egorov’s Model
3. Calculation of
3.1. Existing Methods
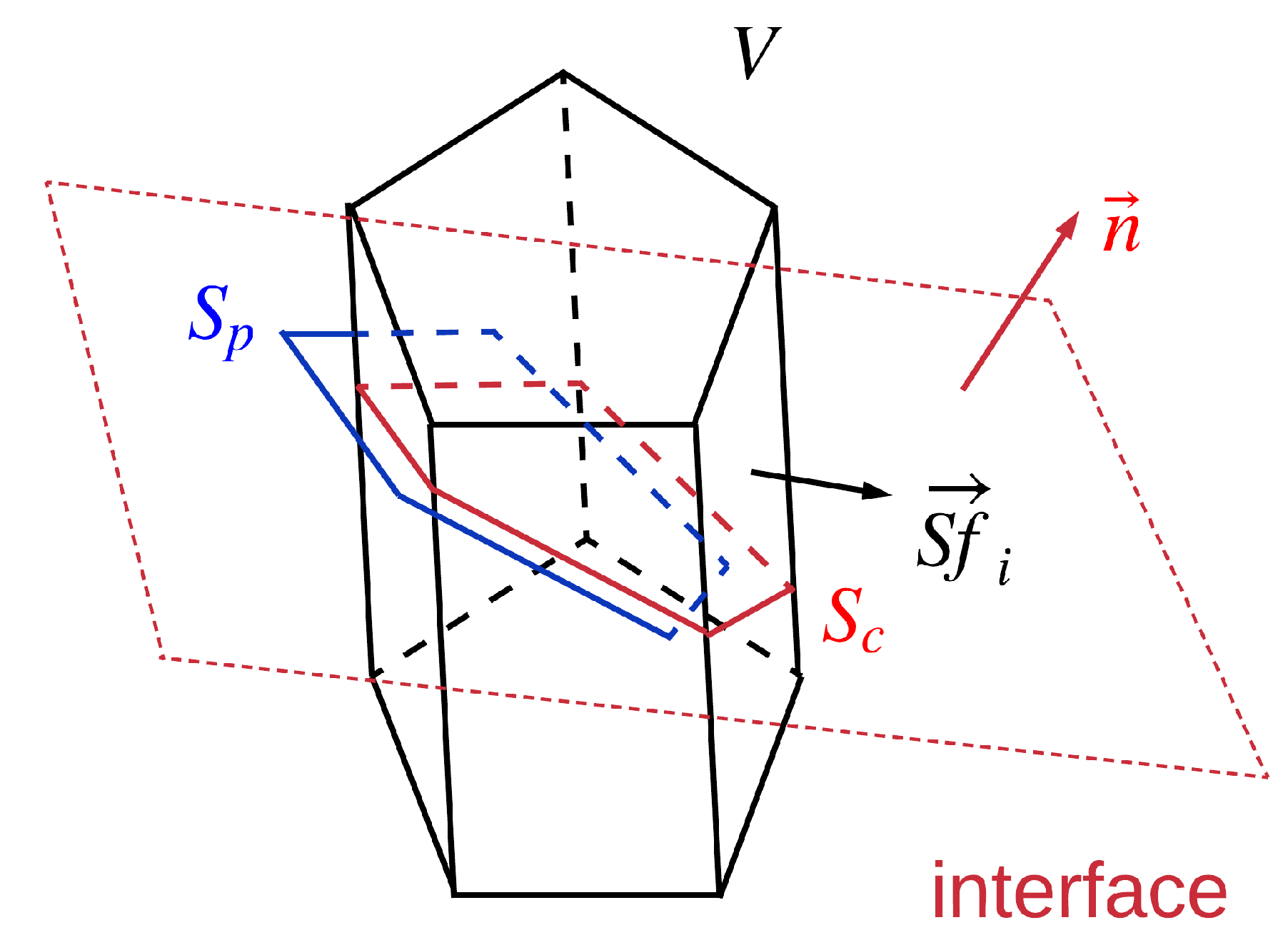
3.2. A New Length Scale
4. Numerical Setup
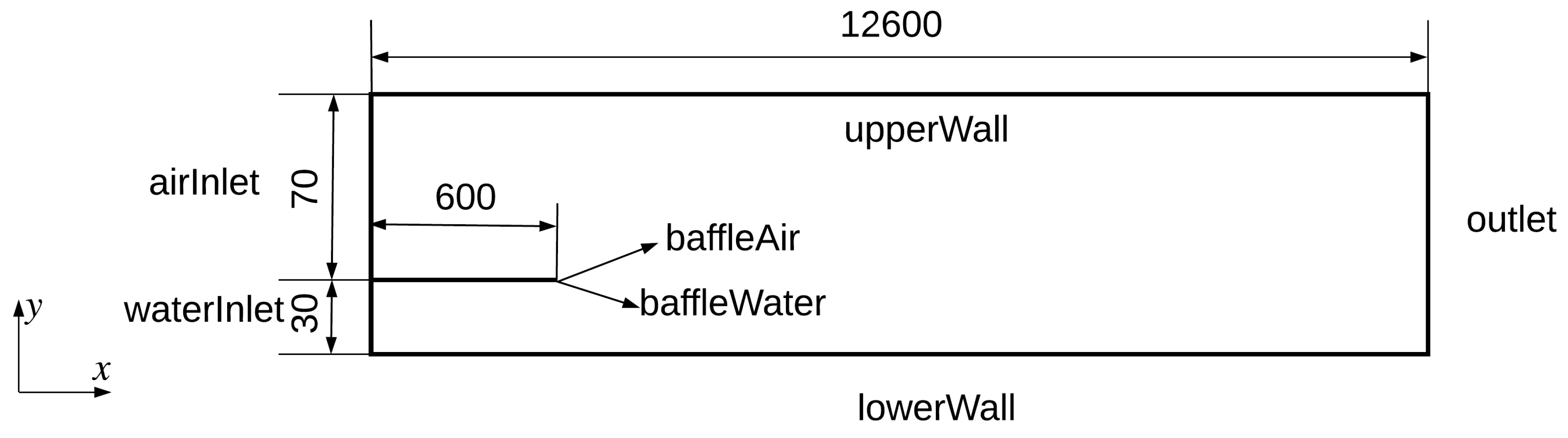
4.1. Computational Domain
4.2. Boundary Conditions
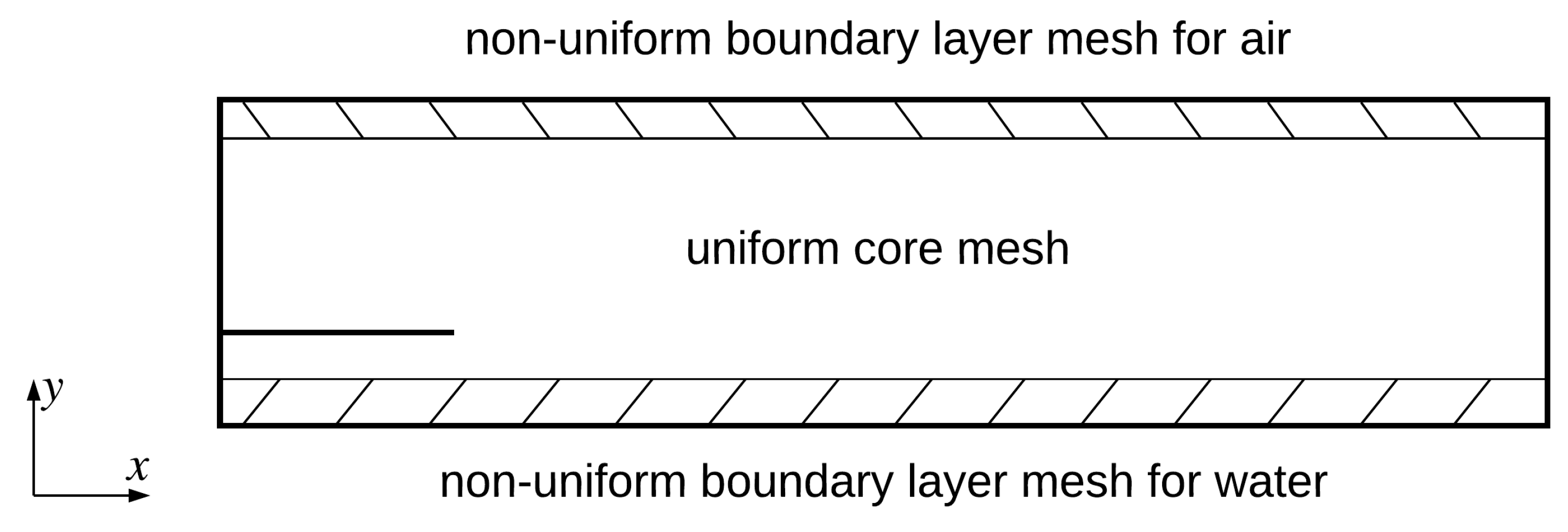
4.3. Mesh
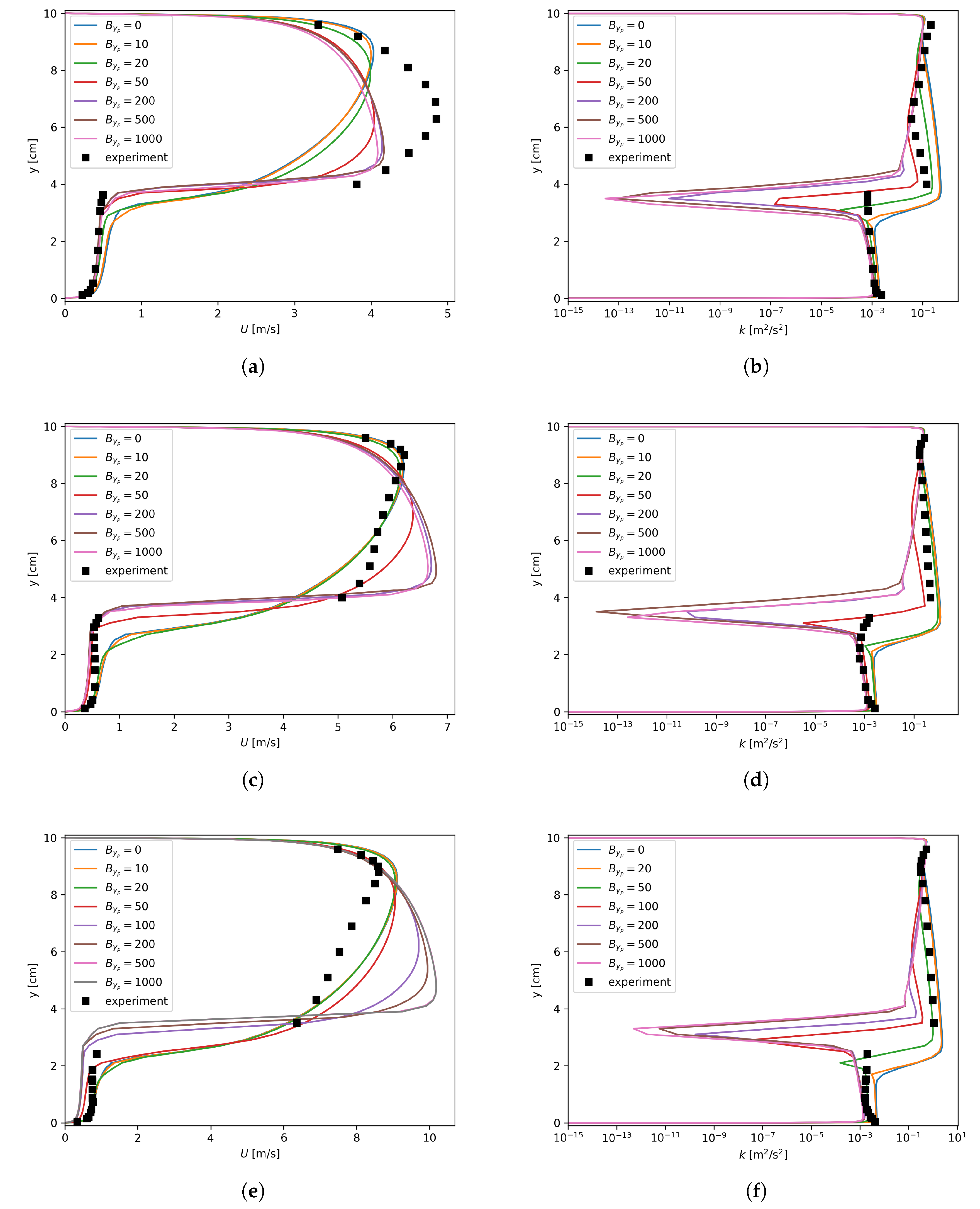
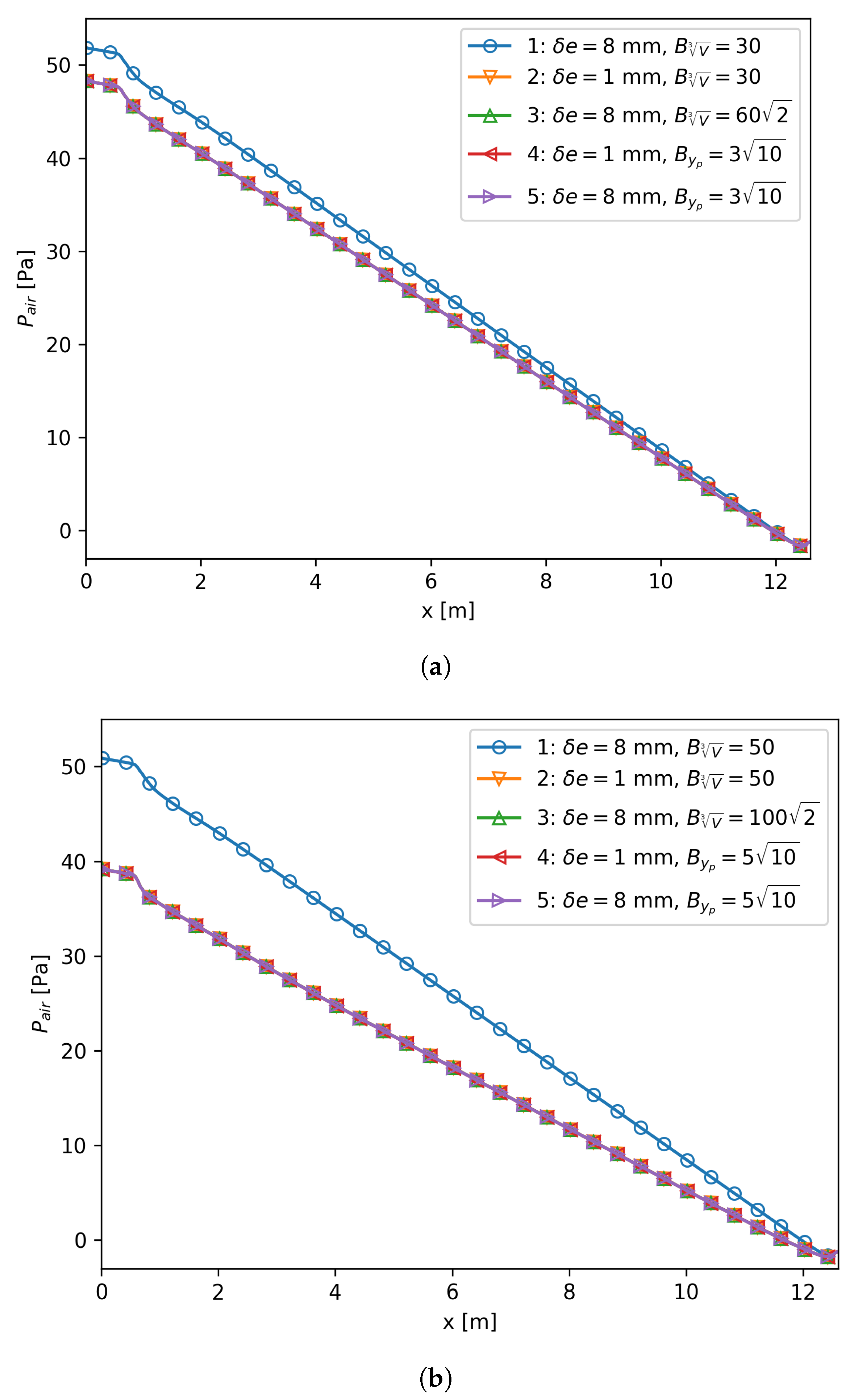
5. Advantages of over
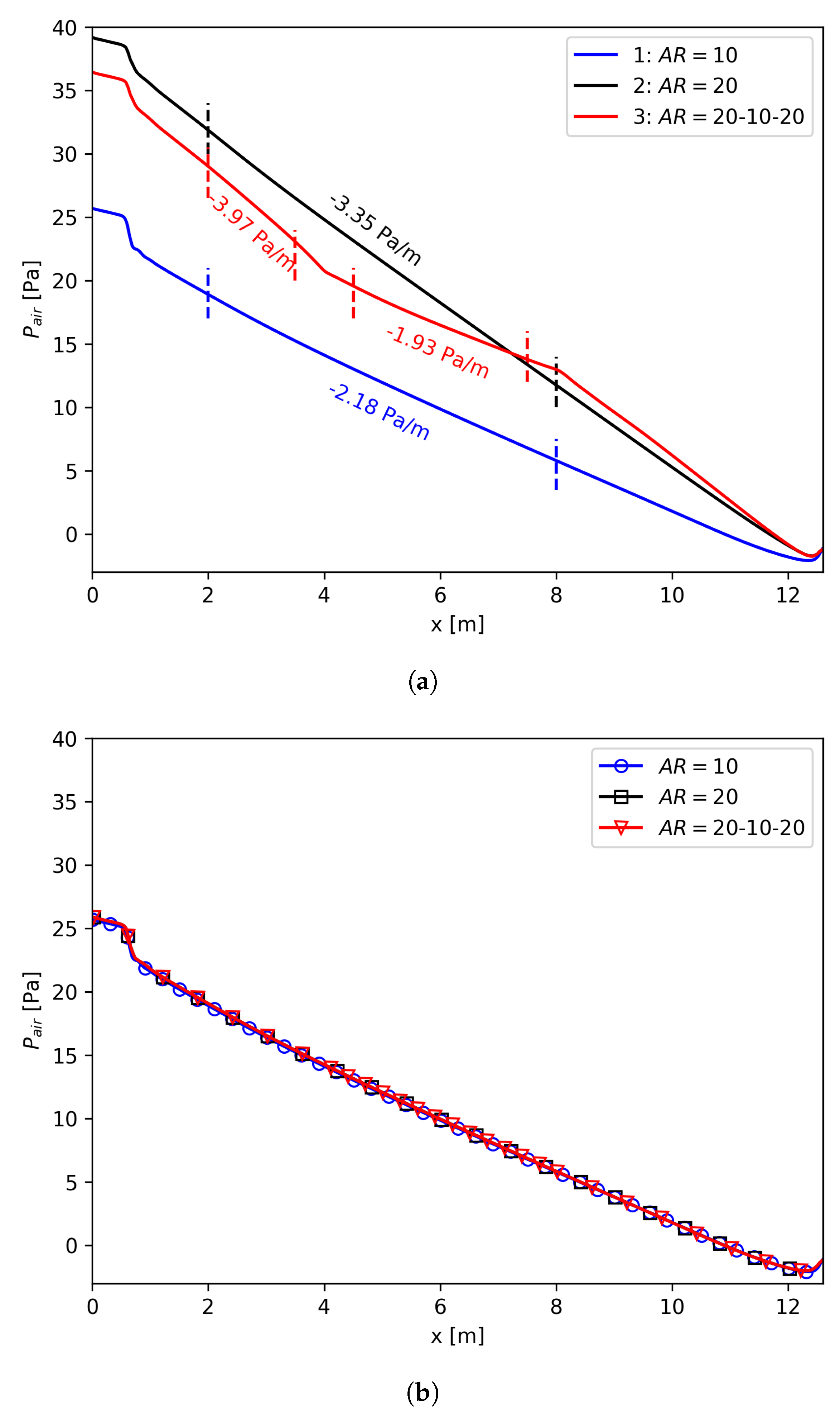
5.1. Problems with 2D Simulations
5.2. Aspect Ratio Effect
5.3. Notes on
6. Further Discussions on Egorov’s Model
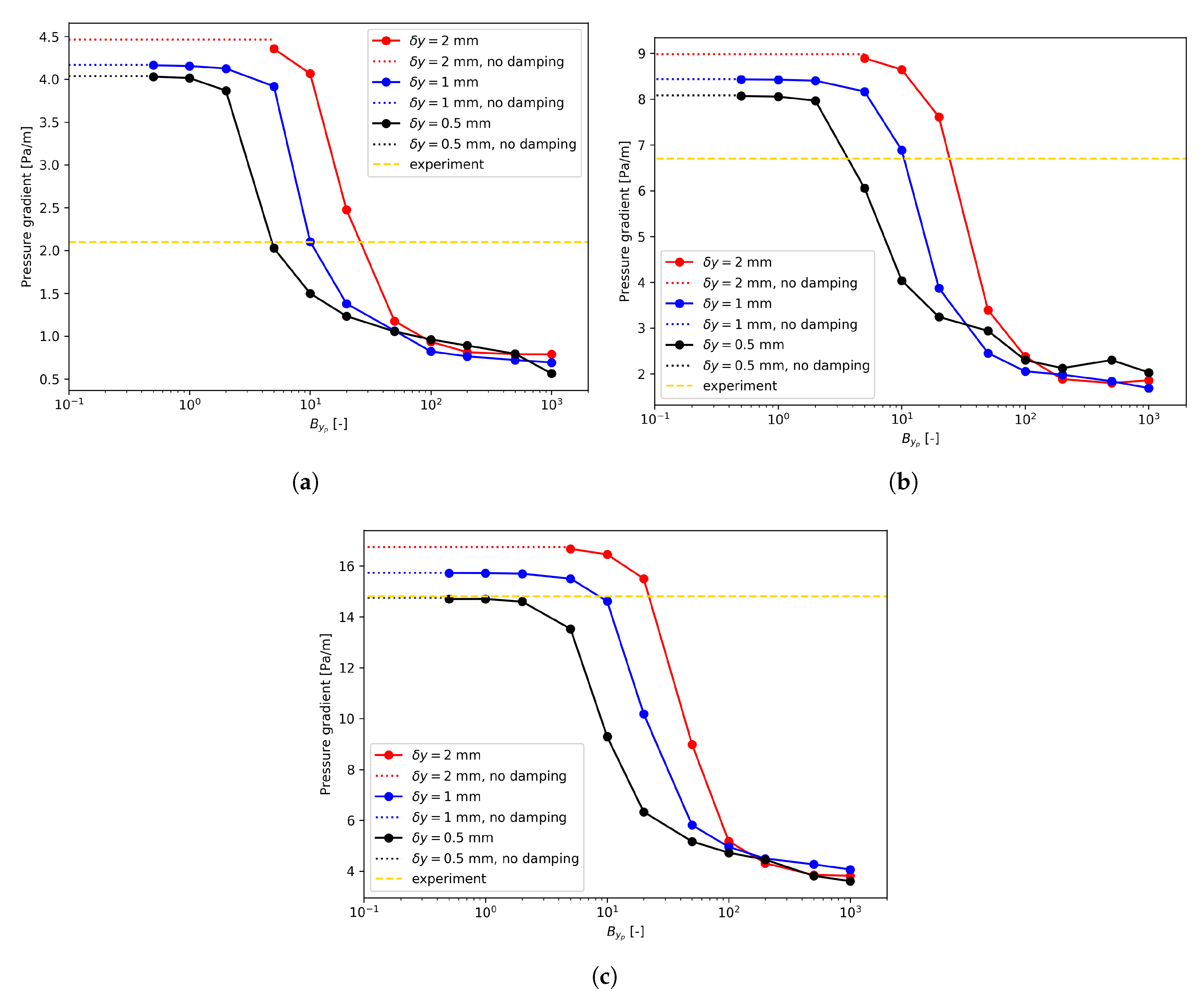
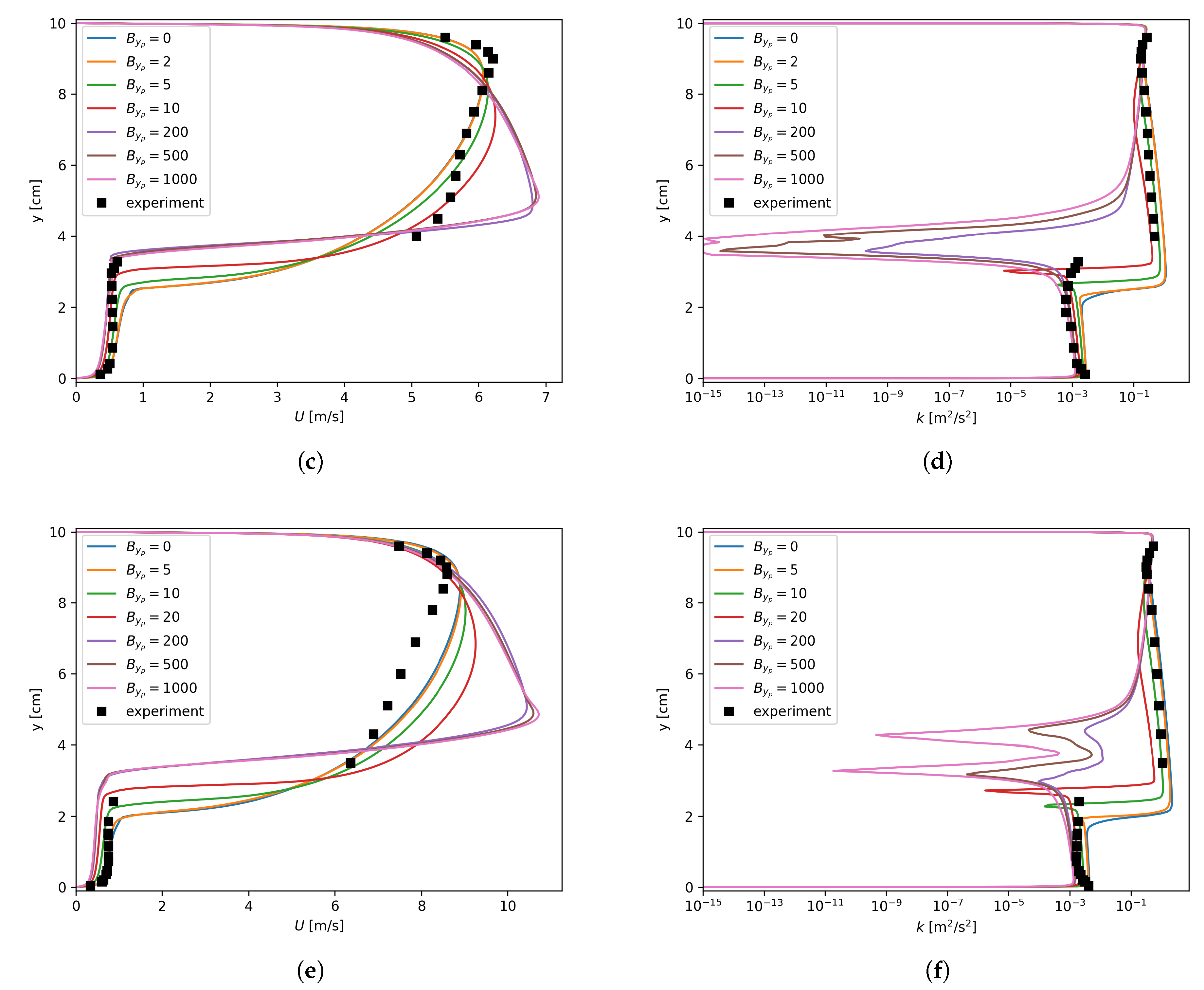
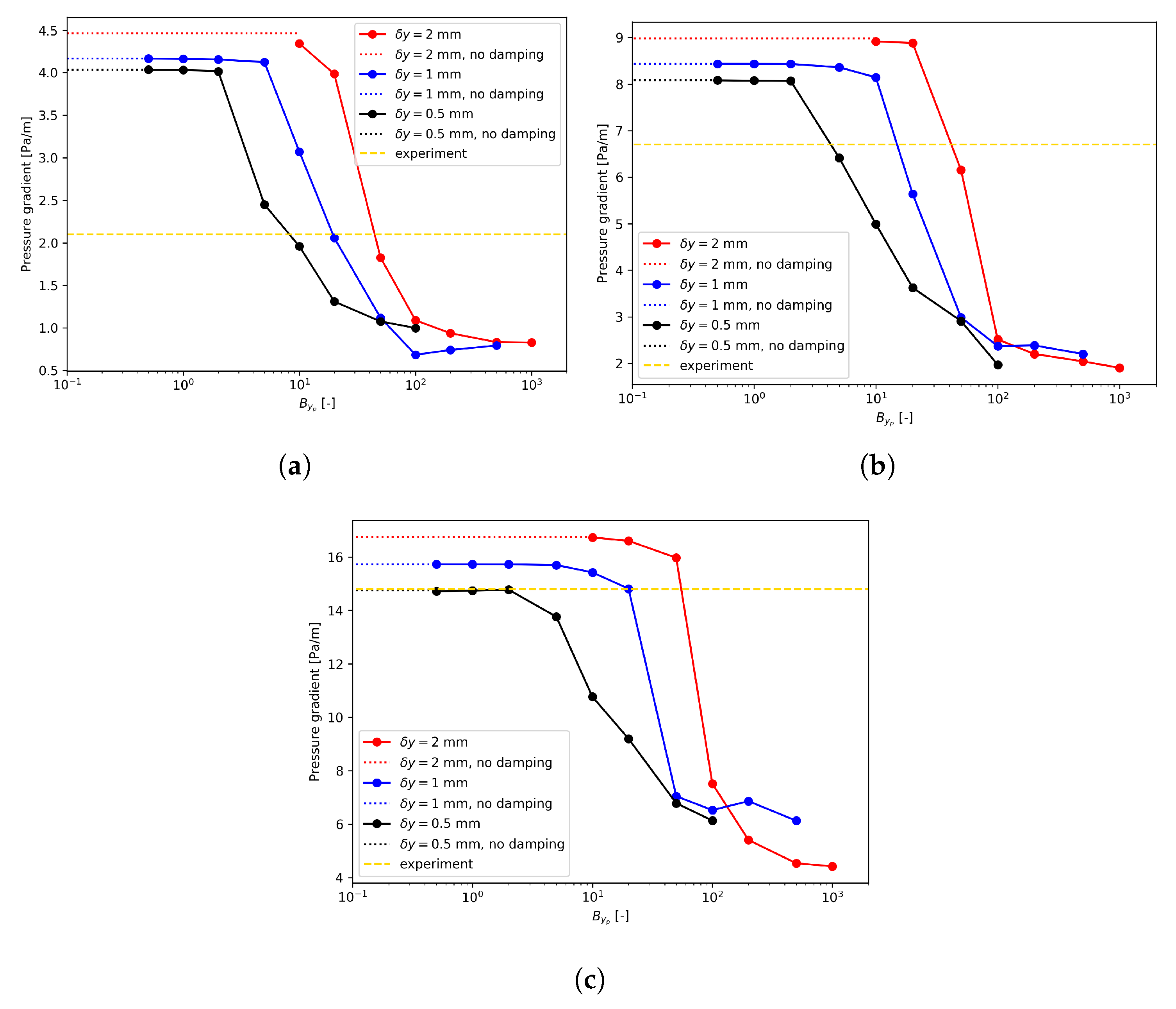
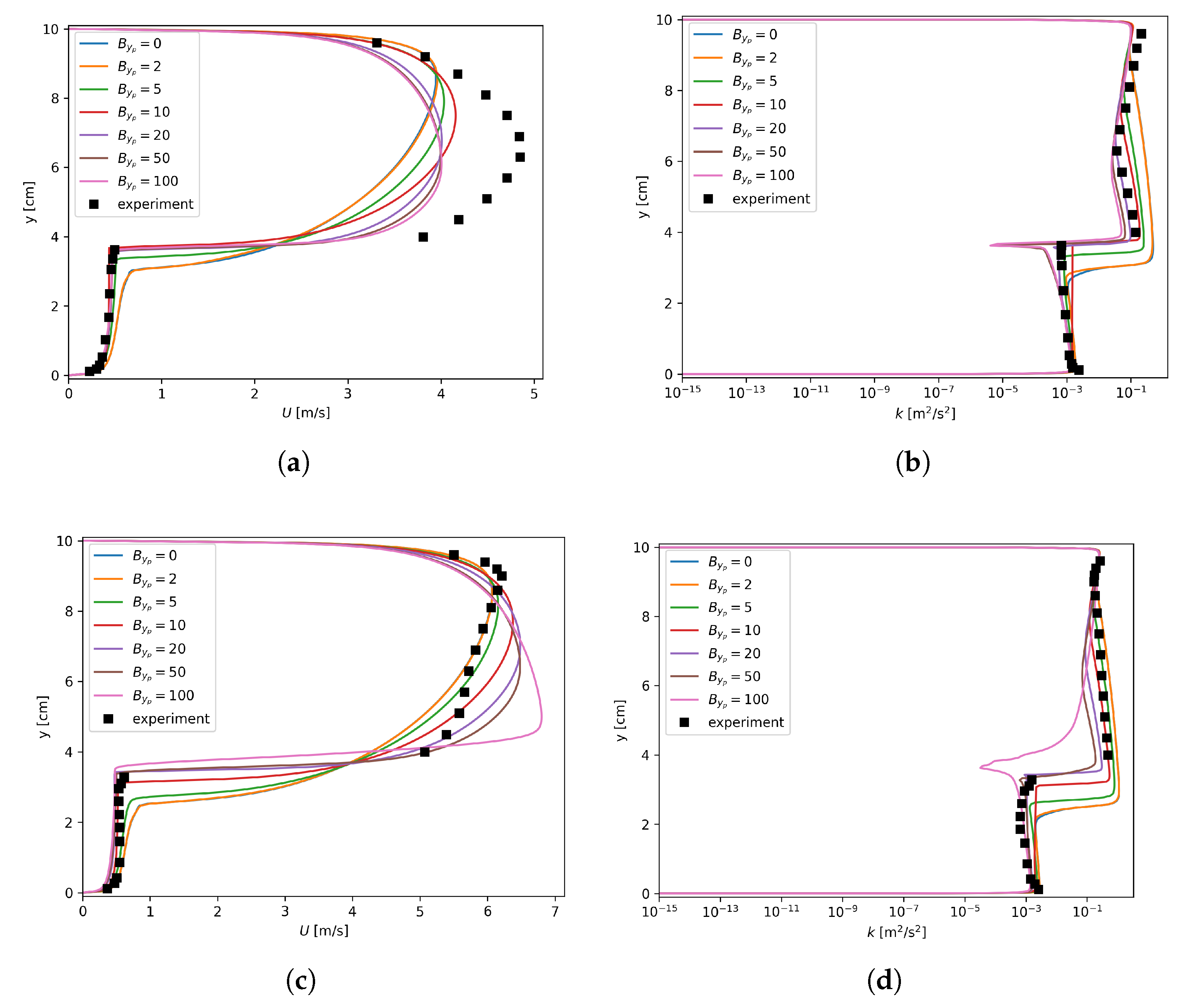
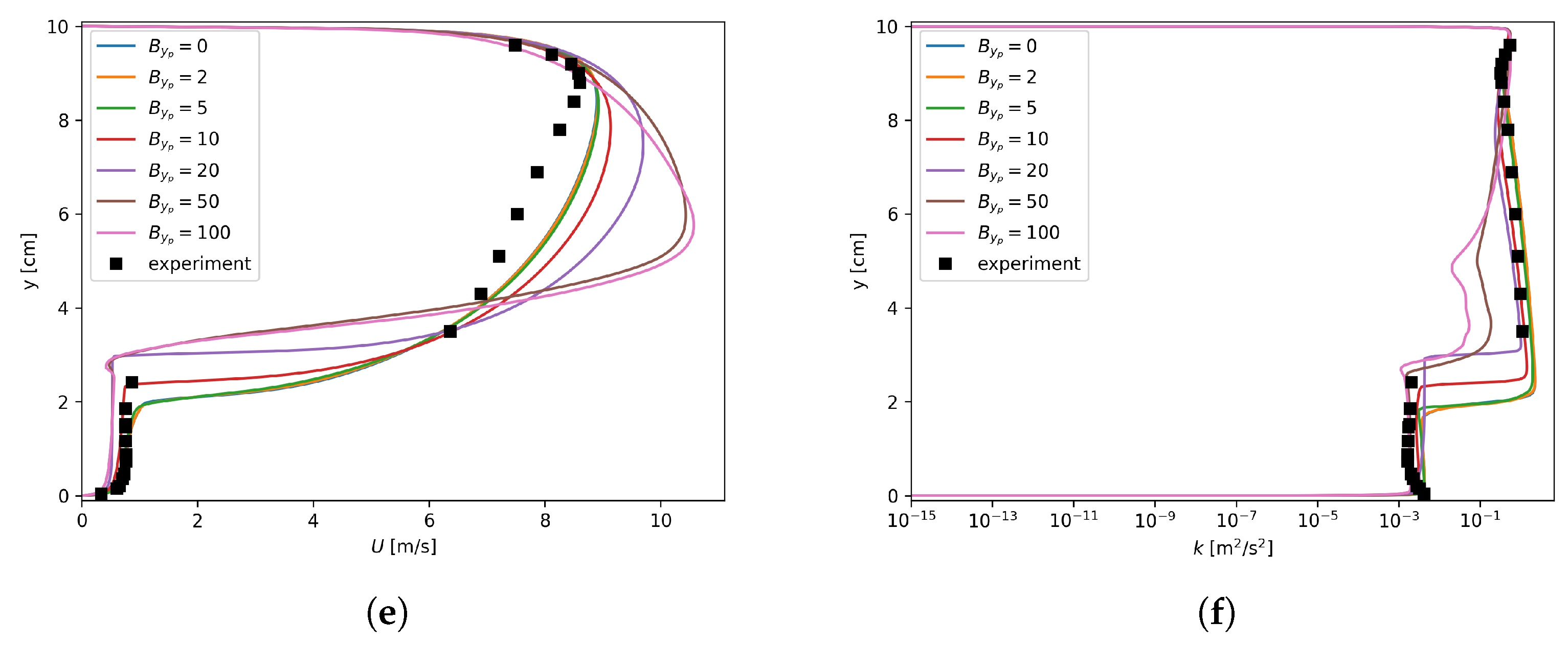
6.1. There Is No Large Enough Value for B
6.2. Symmetric Treatment for Damping Terms
7. Asymmetric Treatment for Damping Terms
8. Conclusions and Outlooks
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
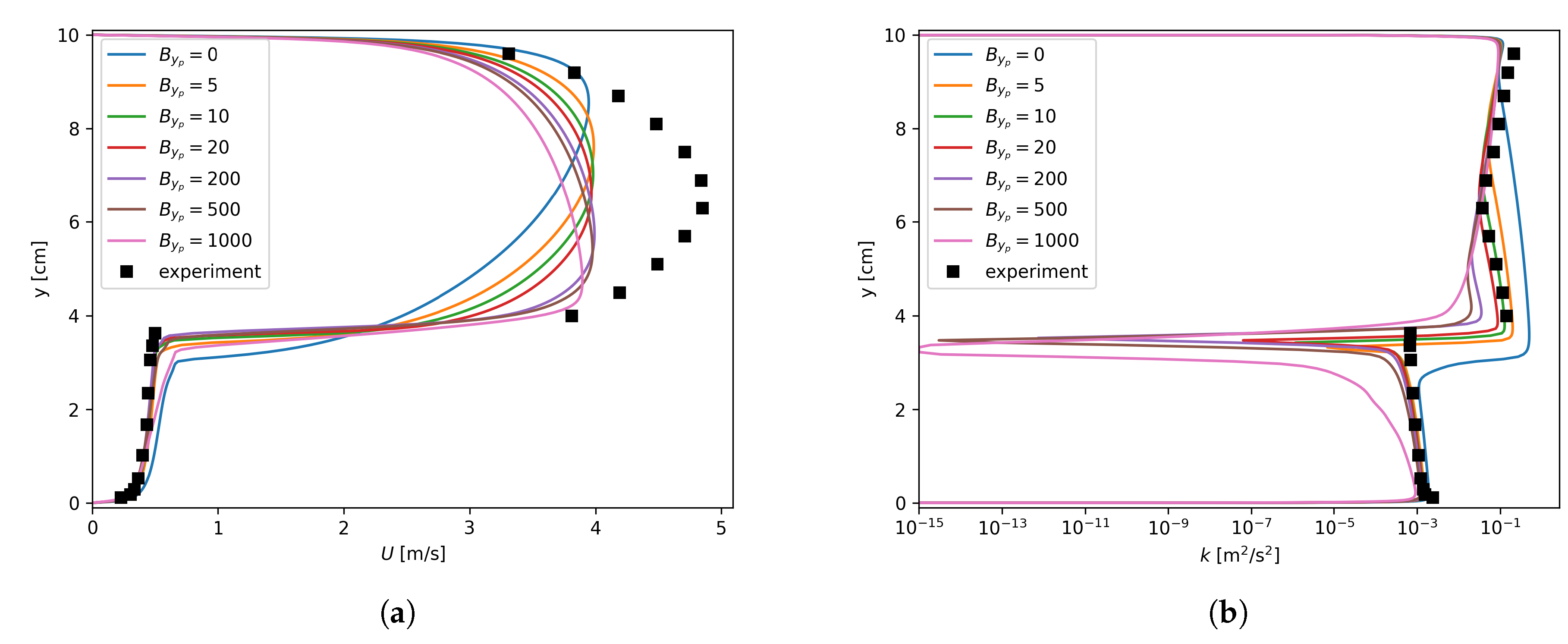
Appendix A. U and k Profiles for Meshes with δy = 2 mm and δy = 1 mm

References
- Hirt, C.; Nichols, B. Volume of fluid (VOF) method for the dynamics of free boundaries. J. Comput. Phys. 1981, 39, 201–225. [Google Scholar] [CrossRef]
- Ishii, M.; Mishima, K. Two-fluid model and hydrodynamic constitutive relations. Nucl. Eng. Des. 1984, 82, 107–126. [Google Scholar] [CrossRef]
- Sussman, M.; Smereka, P.; Osher, S. A Level Set Approach for Computing Solutions to Incompressible Two-Phase Flow. J. Comput. Phys. 1994, 114, 146–159. [Google Scholar] [CrossRef]
- Fabre, J.; Suzanne, C.; Masbernat, L. Experimental Data Set No. 7: Stratified Flow, Part I: Local Structure. Multiph. Sci. Technol. 1987, 3, 285–301. [Google Scholar] [CrossRef]
- Rashidi, M.; Banerjee, S. The effect of boundary conditions and shear rate on streak formation and breakdown in turbulent channel flows. Phys. Fluids A Fluid Dyn. 1990, 2, 1827–1838. [Google Scholar] [CrossRef]
- Lombardi, P.; De Angelis, V.; Banerjee, S. Direct numerical simulation of near-interface turbulence in coupled gas-liquid flow. Phys. Fluids 1996, 8, 1643–1665. [Google Scholar] [CrossRef]
- Fulgosi, M.; Lakehal, D.; Banerjee, S.; De Angelis, V. Direct numerical simulation of turbulence in a sheared air-water flow with a deformable interface. J. Fluid Mech. 2003, 319–345. [Google Scholar] [CrossRef]
- Egorov, Y. Validation of CFD codes with PTS-relevant test cases. In 5th Euratom Framework Programme ECORA Project; European Commission: Brussels, Belgium, 2004; pp. 91–116. [Google Scholar]
- Liovic, P.; Lakehal, D. Multi-physics treatment in the vicinity of arbitrarily deformable gas-liquid interfaces. J. Comput. Phys. 2007, 222, 504–535. [Google Scholar] [CrossRef]
- Lo, S.; Tomasello, A. Recent progress in CFD modelling of multiphase flow in horizontal and near-horizontal pipes. In Proceedings of the 7th North American Conference on Multiphase Technology, BHR Group, Banff, AB, Canada, 2–4 June 2010; pp. 209–219. [Google Scholar]
- Thompson, C.P.; Sawko, R. Interface Turbulence Treatments in RANS Models of Stratified Gas/Liquid Flows. In Proceedings of the ASME 2012 International Mechanical Engineering Congress and Exposition, Houston, TX, USA, 9–15 November 2012; pp. 621–626. [Google Scholar]
- Frederix, E.; Mathur, A.; Dovizio, D.; Geurts, B.; Komen, E. Reynolds-averaged modeling of turbulence damping near a large-scale interface in two-phase flow. Nucl. Eng. Des. 2018, 333, 122–130. [Google Scholar] [CrossRef]
- Qiu, G.; Wei, X.; Cai, W.; Jiang, Y. Development and validation of numerical model of condensation heat transfer and frictional pressure drop in a circular tube. Appl. Therm. Eng. 2018, 143, 225–235. [Google Scholar] [CrossRef]
- Bristot, A.; Morvan, H.P.; Simmons, K.; Klingsporn, M. Effect of turbulence damping in vof simulation of an aero-engine bearing chamber. In Proceedings of the ASME Turbo Expo 2017, Charlotte, NC, USA, 26–30 June 2017; Volume 2B-2017, pp. 1–10. [Google Scholar] [CrossRef]
- Elhanafi, A.; Fleming, A.; Leong, Z.; Macfarlane, G. Effect of RANS-based turbulence models on nonlinear wave generation in a two-phase numerical wave tank. Prog. Comput. Fluid Dyn. 2017, 17, 141. [Google Scholar] [CrossRef]
- Fan, W.; Li, H.; Anglart, H. Numerical investigation of spatial and temporal structure of annular flow with disturbance waves. Int. J. Multiph. Flow 2019, 110, 256–272. [Google Scholar] [CrossRef]
- Gada, V.H.; Tandon, M.P.; Elias, J.; Vikulov, R.; Lo, S. A large scale interface multi-fluid model for simulating multiphase flows. Appl. Math. Model. 2017, 44, 189–204. [Google Scholar] [CrossRef]
- Höhne, T.; Mehlhoop, J.P. Validation of closure models for interfacial drag and turbulence in numerical simulations of horizontal stratified gas-liquid flows. Int. J. Multiph. Flow 2014, 62, 1–16. [Google Scholar] [CrossRef]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Menter, F.R.; Esch, T. Elements of Industrial Heat Transfer Predictions. In Proceedings of the 16th Brazilian Congress of Mechanical Engineering, Uberlandia, Brazil, 26–30 November 2001; pp. 117–127. [Google Scholar] [CrossRef]
- ANSYS Inc. ANSYS FLUENT 18.0 Theory Guide; ANSYS Inc.: Cannonsburg, PA, USA, 2017. [Google Scholar]
- Fan, W.; Anglart, H. vFoam: A new set of volume of fluid solvers for turbulent isothermal multiphase flows. arXiv 2018, arXiv:1811.12580. [Google Scholar]
- Gada, V.; Punde, P.; Tandon, M.P.; Vikulov, R. Role of Turbulence Damping at the Gas-Liquid Interface. In Proceedings of the 9th International Conference on Multiphase Flow, Firenze, Italy, 22–27 May 2016. [Google Scholar]
- Lorencez, C.; Nasr-Esfahany, M.; Kawaji, M.; Ojha, M. Liquid turbulence structure at a sheared and wavy gas-liquid interface. Int. J. Multiph. Flow 1997, 23, 205–226. [Google Scholar] [CrossRef]
- Lakehal, D. Status and future developments of Large-Eddy Simulation of turbulent multi-fluid flows (LEIS and LESS). Int. J. Multiph. Flow 2018, 104, 322–337. [Google Scholar] [CrossRef]


| Run Reference | Air Average Velocity (m/s) | Water Average Velocity (m/s) | Pressure Gradient (Pa/m) |
|---|---|---|---|
| 250 | 3.66 | 0.395 | 2.10 |
| 400 | 5.50 | 0.476 | 6.70 |
| 600 | 7.56 | 0.698 | 14.80 |
| airInlet | waterInlet | outlet | upperWall | lowerWall | baffleAir | baffleWater | |
|---|---|---|---|---|---|---|---|
| U | mappedC | mappedC | advective | no slip | no slip | no slip | no slip |
| fixed flux | fixed flux | fixed total pressure | fixed flux | fixed flux | fixed flux | fixed flux | |
| k | mappedN | mappedN | wall function | wall function | |||
| mappedN | mappedN | wall function | wall function | wall function | wall function |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fan, W.; Anglart, H. Progress in Phenomenological Modeling of Turbulence Damping around a Two-Phase Interface. Fluids 2019, 4, 136. https://doi.org/10.3390/fluids4030136
Fan W, Anglart H. Progress in Phenomenological Modeling of Turbulence Damping around a Two-Phase Interface. Fluids. 2019; 4(3):136. https://doi.org/10.3390/fluids4030136
Chicago/Turabian StyleFan, Wenyuan, and Henryk Anglart. 2019. "Progress in Phenomenological Modeling of Turbulence Damping around a Two-Phase Interface" Fluids 4, no. 3: 136. https://doi.org/10.3390/fluids4030136
APA StyleFan, W., & Anglart, H. (2019). Progress in Phenomenological Modeling of Turbulence Damping around a Two-Phase Interface. Fluids, 4(3), 136. https://doi.org/10.3390/fluids4030136





