Cardiac Triangle Mapping: A New Systems Approach for Noninvasive Evaluation of Left Ventricular End Diastolic Pressure

Abstract

Noninvasive and practical assessment of hemodynamics is a critical unmet need in the treatment of both chronic and acute cardiovascular diseases. Particularly, the ability to monitor left ventricular end-diastolic pressure (LVEDP) noninvasively offers enormous benefit for managing patients with chronic congestive heart failure. Recently, we provided proof of concept that a new cardiac metric, intrinsic frequency (IF), derived from mathematical analysis of non-invasively captured arterial waveforms, can be used to accurately compute cardiovascular hemodynamic measures, such as left ventricle ejection fraction (LVEF), by using a smartphone. In this manuscript, we propose a new systems-based method called cardiac triangle mapping (CTM) for hemodynamics evaluation of the left ventricle. This method is based on intrinsic frequency (IF) and systolic time interval (STI) methods that allows computation of LVEDP from noninvasive measurements. Since the CTM method only requires arterial waveform and electrocardiogram (ECG), it can eventually be adopted as a simple smartphone-based device, an inexpensive hand-held device, or perhaps (with future design modifications) a wearable sensor. Such devices, combined with this method, would allow for remote monitoring of heart failure patients.

1. Introduction

Cardiovascular diseases (CVDs) are the underlying cause of approximately one in three deaths in the United States annually. Thirty-four percent of American adults suffer from CVD. The total direct medical costs of these illnesses are projected to rise to $818 billion by 2030 [1]. Certainly, these statistics indicate a critical need to develop new methods and innovative devices for diagnosing and monitoring CVDs. Ideally, these methods must increase patient comfort, be easy to use by clinicians and patients in their home environment, and finally be noninvasive to reduce complications and cost. Among CVDs, heart failure (HF) is a distressingly common problem [1] that is expected to become more prevalent as the population ages [2]. Hospital systems have consequently expended major efforts to monitor HF status in outpatients, with a focus on reducing re-admission for decompensated heart failure. An implantable pulmonary artery pressure sensor was developed that allowed home transcutaneous monitoring downloaded to an internet-based recording that could be reviewed on line by physicians. This device was compared in a randomized clinical trial and found to be superior to usual care in reducing hospitalizations for heart failure patients [3]. This proof-of-concept work showed that home hemodynamic monitoring can make a clinical impact even when the monitored value is pulmonary artery diastolic pressure, a reasonable, but flawed estimate of cardiac filling pressure.

Pressure inside the left ventricle (LV) at the end of the diastolic phase of the cardiac cycle, LV end diastolic pressure (LVEDP), is an important measure of LV function [4]. Elevated LVEDP is generally indicative of poor LV function in both heart failure with preserved ejection fraction (HFpEF) and of heart failure with reduced ejection fraction (HFrEF). HF requiring hospitalization follows a process that usually spans over several months [5]. It is generally accepted that HF rehospitalization can be reduced by early detection of elevated LVEDP [5]. Furthermore, in hospital HF management depends on clinical assessments, so congestion may not be addressed sufficiently during hospitalization [6]. Therefore, a noninvasive evaluation of LVEDP can significantly impact the diagnosis and management of HF. Such a method could reduce the discharge of inadequately treated patients and help maintain them in a compensated state after discharge.

Conventional methods for LVEDP evaluation, either directly through left heart catheterization or indirectly using pulmonary capillary wedge pressure (PCWP), are invasive. Therefore, their usage is limited to unstable patients or those with serious symptoms for whom cardiac catheterization is justifiable. In this paper, we introduce a systems fluid dynamic approach that allows for noninvasive evaluation of left ventricle end diastolic pressure (LVEDP) to improve upon HF diagnostics and monitoring.

Recently, we introduced the intrinsic frequency (IF) concept that reveals clinically relevant information about the dynamics of the left ventricle (LV), arterial system, and their interactions [7,8,9,10]. IF frequencies are operating frequencies based on the Sparse Time-Frequency Representation (STFR) [11], treating the LV combined with the aorta and the remaining peripheral arteries as a coupled dynamical system (heart + aortic tree), which is decoupled upon closure of the aortic valve [8,9,12]. Utilizing the IF method, one can extract two IF frequencies, ω₁ and ω₂ (along with other independent variables [9]), from a single arterial blood pressure waveform. One important advantage of the IF method is that the absolute magnitude of the arterial pressure wave is not required to extract the IF parameters, only the waveform morphology [8,9]. As such, the IFs can be easily acquired noninvasively, instantaneously, and inexpensively using a smartphone [7,8,13] or arterial applanation tonometry. The first IF, ω₁, describes the dynamics of the systolic phase of the cardiac cycle where the LV and aorta (vasculature) are a coupled dynamic system [7,8,9]. The second IF, ω₂, is dominated by the dynamics of the vasculature [9,10,12].

Previous studies have indicated that ω₁ is mainly determined by the LV contractility while ω₂ is mostly determined by vascular function (e.g., arterial stiffness, arterial wave dynamics, and afterload) [9,10,12,14]. In a blind clinical study [8], it was shown LV ejection fraction (LVEF, a surrogate of LV contractility) derived using IF applied to carotid waveforms extracted using an iPhone [8] were similar to those measured from cardiac magnetic resonance imaging (CMRI) (r = 0.74, p < 0.00001). Importantly, a very strong correlation between LVEF measured by CMRI and LVEF computed by IF (LVEF-IF) was observed (r = 0.94, p < 0.0001) among HF patients [7,8]. Recently, in a blind cross-sectional study of 191 childhood cancer survivors who are at risk for anthracycline-related cardiac dysfunction, LVEF-IF was compared with LVEF from 2D-echocardiography and CMRI [13]. Using the CMRI as the gold standard, the results showed that the detection of cardiac systolic dysfunction (as measured by LVEF) via the noninvasive LVEF-IF method was more accurate and sensitive than 2D echocardiography [13]. Using Framingham Heart Study data, we have recently demonstrated that central arterial stiffness (carotid-femoral pulse wave velocity (PWV)) can be computed using IF of a single noninvasively measured carotid pressure waveform (without any need for ECG measurement and/or femoral tonometry) [12]. The result showed that estimated PWV by IF method is equivalent to PWV measurements obtained by direct methods in predicting the risk for CVD [12]. These studies point to the importance of the IF method as a powerful tool for noninvasive ventricular performance (contractility) and vascular function (afterload) evaluation [8,9,12,13].

In this paper, we propose a new systems-based approach, called Cardiac Triangle Mapping (CTM), for hemodynamic evaluation of the LV. This method uses the pre-ejection period (PEP) and IF mathematics to compute LVEDP. In this study, we tested the proposed CTM method using retrospective previously published data.

2. Theory and Methods

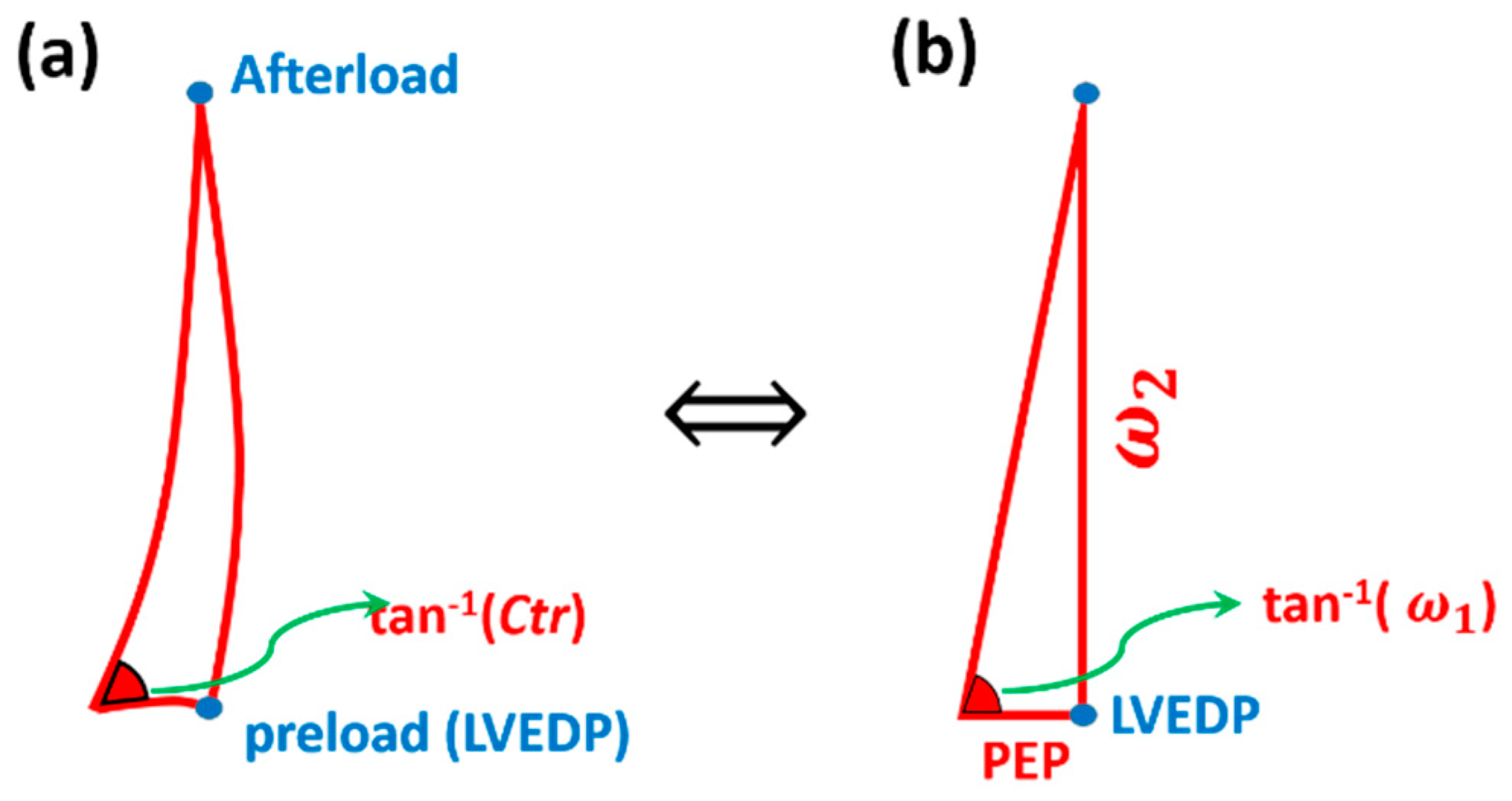
2.1. Intrinsic Frequency Method

The intrinsic frequency (IF) method models a dynamic system as an object rotating around an origin. The angular velocity of the rotation is the intrinsic frequency. In the coupled LV-aorta system, the average angular velocity of rotation (instantaneous frequency) during systole when the LV and aorta are coupled together is ω₁. The average angular velocity during diastole when the aorta gets decoupled from LV (after the closure of the aortic valve) is ω₂. Simply put, the IF method assumes that the instantaneous frequency of a coupled dynamical system is piecewise constant in time with the step that occurs at the time of decoupling. In the LV-aorta system, the decoupling time is the time of the closure of the aortic valve. It is obvious from the definition that IF frequencies are fundamentally different than Fourier harmonics or any other resonance-type frequencies [9,15]. The mathematical formulation of the IF method is as follows:

$$\mathrm{Minimize}:\Vert p\left(t\right)-\chi \left(0,T_0\right)\left[(a_1\cos \left({\omega }_{1}t\right)+b_1\sin \left({\omega }_{1}t\right)\right]-\chi \left(T_0,T\right)[(a_2\cos \left({\omega }_{2}t\right)+b_2\sin \left({\omega }_{2}t\right)]-c{\Vert }_2^2$$

(1)

This L₂ minimization is subject to the continuity at T₀ (time of the decoupling = time of the dicrotic notch) and periodicity of the waveform. Here, χ(a,b) is the indicator function (χ(a,b) = 1 if a ≤ t ≤ b and χ(a,b) = 0 otherwise) and p(t) is the arterial waveform. a₁, a₂, c, b₁, b₂, ω₁, and ω₂ are the unknowns that can be found by solving this nonconvex minimization problem. Details regarding mathematical formulation of the IF method [9], its computational procedure [9], and its convergence/accuracy [15] were provided in previous publications [9,15,16]. Figure 1 illustrates how the IF-reconstructed waveform from ω₁, and ω₂ represents the original waveform.

The IF method uses the information stored in an arterial waveform (e.g., carotid waveform) and creates a multidimensional IF space. These dimensions include the ω₁ and ω₂, duration of the cycle, and the coordinates of the decoupling point. The decoupling coordinates are represented as the relative height of decoupling at the dicrotic notch (RHDN) and the time of decoupling at the dicrotic notch (T₀). Previous studies [8,17] have indicated that LVEF (surrogate of LV contractility), LV-arterial coupling optimality, and central pulse wave velocity (a major determinant of arterial impedance and pulsatile afterload) can be evaluated as a function of IF parameters [9,10,12,14] (Figure 2). Previous results indicate that LV contractility and afterload can be approximated as a function of IFs (ω₁, ω₂, …). Therefore, without a loss of generality, we can represent LV contractility and afterload as functions of ω₁ and ω₂. In mathematical language:

$$Ctr\approx f_1\left({\omega }_1,{\omega }_2\right),$$

(2)

$$AL\approx f_2\left({\omega }_1,{\omega }_2\right),$$

(3)

Here, Ctr is the LV contractility and AL is the afterload.

2.2. Pre-Ejection Period

The systolic time interval (STI) method was first introduced in the 1960s by Weissler et al. [18]. Clinical studies have shown that LV function performances, such as contractility and preload, can be approximated under certain conditions using STI methods [19]. Two major components of the STI method are the LV ejection time (LVET) and pre-ejection period (PEP). LVET is the time interval from the opening of the aortic valve to the closure of the aortic valve marked by the dicrotic notch (note that LVET is the same as T₀ in the IF method). PEP is the time interval from the beginning of the QRS complex to the opening of the aortic valve. The QRS complex is a combination of the Q wave, R wave, and S wave from ECG where the Q wave is any downward deflection immediately after the P wave, the R wave is an upward deflection after the Q wave, and the S wave is a rapid downward deflection after the R wave. Previous studies have shown that LV preload is inversely proportional to PEP at a given LV contractility and afterload (Talley et al. [20]). Figure 3 shows how PEP is measured from simultaneous pressure waveforms and electrocardiogram (ECG).

2.3. Cardiac Triangle Mapping Hypothesis

Cardiac Triangle Mapping (CTM) is based on the fact that ventricular function is modulated by three factors: (i) The contractile state of the myocardium or contractility, (ii) the afterload that is related to vascular function and LV-arterial coupling, and (iii) the preload, which can be quantified by LVEDP.

IF parameters, such as ω₁ and ω₂, are extracted from the arterial waveforms. These waveforms are the result of interactions between LV contractility and vascular function (resistance, compliance, LV-aorta coupling); therefore, they carry little or no information about the state of the LV preload. This means that PEP provides information that cannot be retrieved from IFs (ω₁ and ω₂). It has been shown that at a fixed LV contractility and afterload, PEP changes (inversely) as preload changes [20]. Since PEP is related to LV preload while ω₁ and ω₂ are not, PEP should be orthogonal (or at the very least not parallel) to ω₁ and ω₂ (and other IF dimensions). As a result, a combination of IFs (ω₁ and ω₂) and PEP creates a complete set that can map LV dynamics, and subsequently provide information about the hemodynamics of LV. The CTM hypothesis states that ω₁ and ω₂, PEP map the global ventricular function. In mathematical form:

$$F\left[Ctr, AL, \mathrm{preload}\right]\equiv G\left[{\omega }_1, {\omega }_2, \mathrm{PEP}\right],$$

(4)

The corollary of $F\equiv G$ is that all LV-related mechanical biomarkers, such as LVEDP, can be computed as a function of IFs (e.g., ω₁, ω₂) and PEP. In mathematical language:

$$\mathrm{LVEDP}=\lambda ·f_3\left(\mathrm{IFs},\mathrm{PEP}\right),$$

(5)

Here, λ is a constant that assures the physical units on both sides are the same (e.g., pressure as mmHg).

The state of LV performance is defined by Ctr, AL, and preload; therefore, it can geometrically be represented as a triangle ($\Delta \mathrm{ABC}$ in Figure 4). For the purposes of this manuscript, we will refer to it as a cardiac triangle. Inspired by the shape of the pressure and ECG waveforms in a cardiac cycle (Figure 4), and without a loss of generality, we assume the cardiac triangle is a right triangle where PEP is one leg (AC), afterload minus LVEDP is the other leg (BC), and the slope of the AB line is Ctr (in other words, the tangent inverse of Ctr is the angle, $\angle \mathrm{A}$). This choice of Ctr is in agreement with past studies that showed LV dp/dt_max is a measure of contractility [21]) (Figure 4). In our assumption, the nonlinear curves from point A to point B and A to C are approximated as straight lines. The error associated with assuming AB and AC as straight lines can be reduced by appropriate usage of nonlinear parameters, such as ω₁. According to the triangle of Figure 4, the below relationship can be written:

$$Ctr=\frac{AL-\mathrm{LVEDP}}{\mathrm{PEP}},$$

(6)

Physiological or physical parameters, such as LV contractility, arterial diastolic pressure, LVEDP, etc., are the edges, angles, or vertices of the cardiac triangle (Figure 5). As described in the previous paragraph, the edges are not necessarily straight. The edges can be curvilinear as shown in Figure 5a. According to Equation (4), a triangle formed by ω₁, ω₂, and PEP (we call this triangle the IF-triangle) can also define the full state of LV performance, hence allowing for computation of LVEDP. This is based on a simple law of geometry: By knowing three components (e.g., three sides or two sides + one angle), the whole triangle can be solved. Without a loss of generality, the IF-triangle can be considered as a triangle with straight sides (Figure 5b) since IF parameters (e.g., ω₁ and ω₂) carry non-linearities associated with the LV-aorta system.

To derive an expression for the LVEDP form Ctr, AL, and PEP, we simply need to solve Equation (6) for LVEDP:

$$\mathrm{LVEDP}=AL-Ctr\times \mathrm{PEP},$$

(7)

Combining Equation (7) with Equations (2) and (3):

$$\mathrm{LVEDP}=f_2\left({\omega }_1,{\omega }_2\right)-f_1\left({\omega }_1,{\omega }_2\right)\times \mathrm{PEP},$$

(8)

Equation (8) is an explicit version of Equation (5) that uses IFs and PEP to compute LVEDP.

2.4. Compatibility of CTM with Hemodynamical Changes

An important step in verifying the validity of the CTM method is to show that the cardiac triangle of CTM (Figure 5) remains intact as the LV-arterial system undergoes functional changes. We used the results of Talley et al. [20] to test the validity of our proposed CTM theory. They performed a series of (dog) experiments to evaluate the effect of LV contractility, LVEDP (surrogate for preload), and AoDP (surrogate for afterload) on PEP. Talley et al. [20] used various levels of inotropic stimuli to force changes in LV contractility while keeping LVEDP and the afterload constant to impose LVEDP changes at fixed contractility and afterload, and to manipulate afterload at a constant LV contractility and LVEDP.

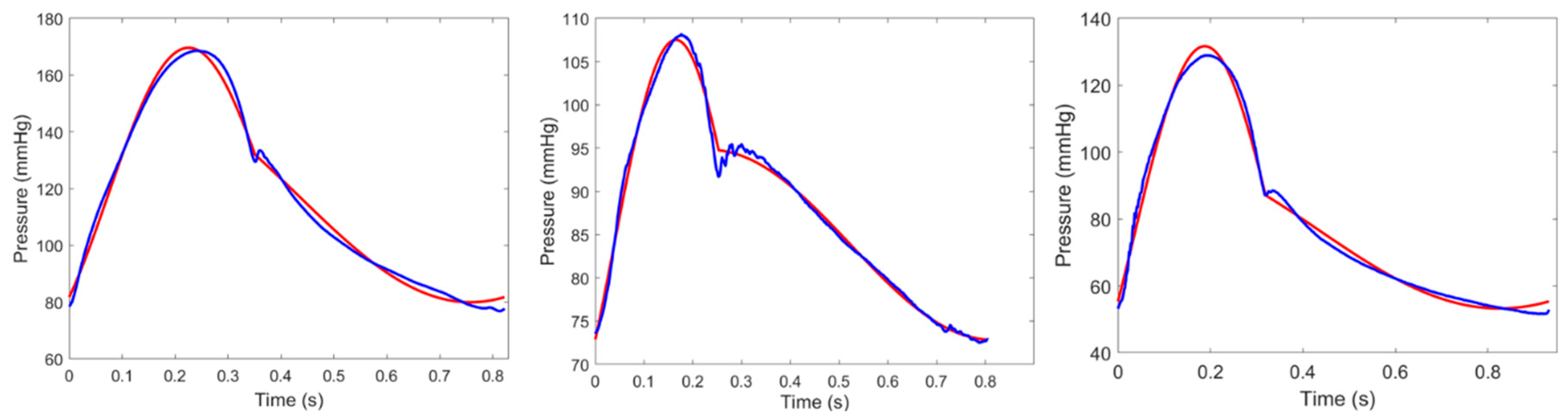
2.5. Testing CTM Using Clinical Retrospective Data

Computations of ω₁ and ω₂ (and, subsequently, f₁ and f₂) were not possible since arterial waveforms were unavailable within the retrospective dataset. Consequentially, we used a simplified version of Equation (7) (or Equation (8)) where hemodynamical surrogates for AL (or f₂) and Ctr (or f₁) were considered. Recent results have shown that ω₁ is highly correlated with various indexes of LV contractility [17]. Furthermore, the Pahlevan et al. [8] study indicated that ω₁ corrected with the LV ejection time (${\omega }_1\sqrt{T_0}$, where T₀ is the LVET) is strongly correlated with LVEF. Therefore, by introducing a minor error, we can replace f₁ (or Ctr) in Equation (8) with $\mathrm{LVEF}/\sqrt{T_0}$. Aortic diastolic pressure (AoDP) was also considered as a surrogate for AL). Hence, Equation (8) is simplified to:

$$\mathrm{LVEDP}=c_1\mathrm{AoDP}-c_2\frac{\mathrm{LVEF}\times \mathrm{PEP}}{\sqrt{T_0}},$$

(9)

Here, c₁ is a unitless constant and c₂ is a constant with a unit of $\mathrm{mmHg}/\sqrt{\sec \mathrm{ond}}$. We then used linear multiple regression to compute the best value for c₁ and c₂. To determine c₁ and c₂, the least squares method was used [22]. The advantage of this method is that it allows the residuals to be treated as continuous differentiable measures. The disadvantage is that outliers may have disproportional impacts on the computed values.

A retrospective dataset from Gerrard et al. [23] was used to test the validity of the CTM method and approximate the error of Equation (9). This dataset includes all physiological data required for Equation (9), such as LVEF, PEP, T₀, AoDP, and LVEDP.

3. Results

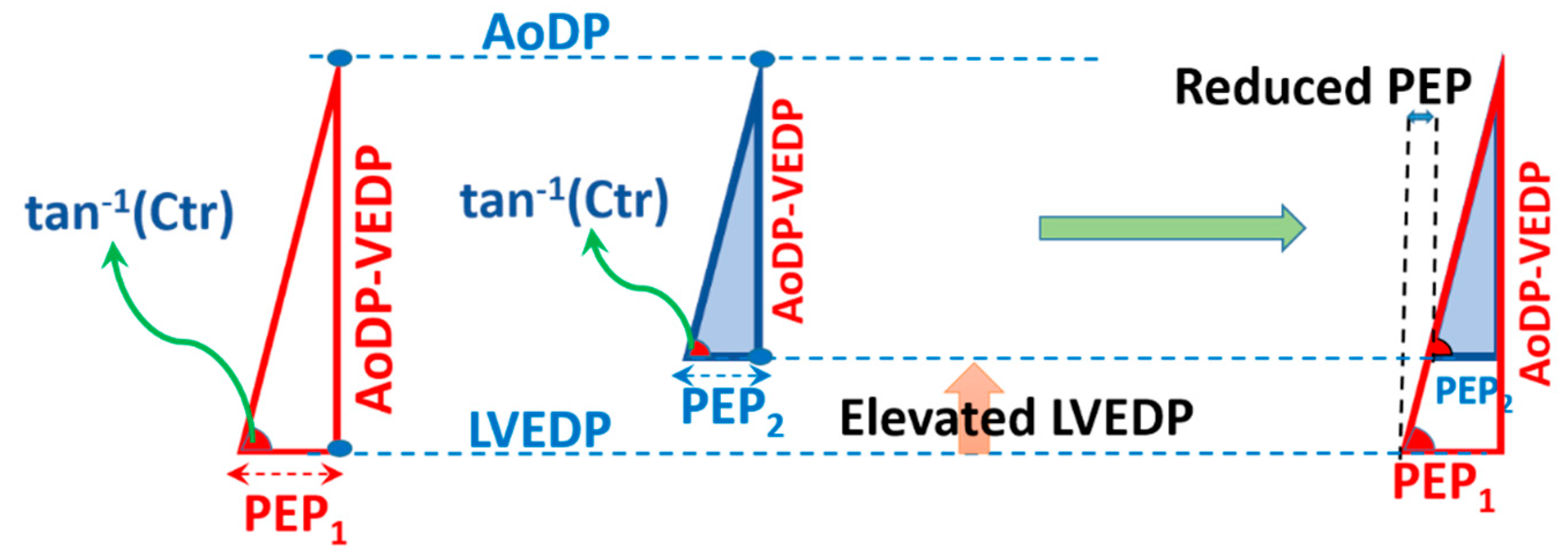
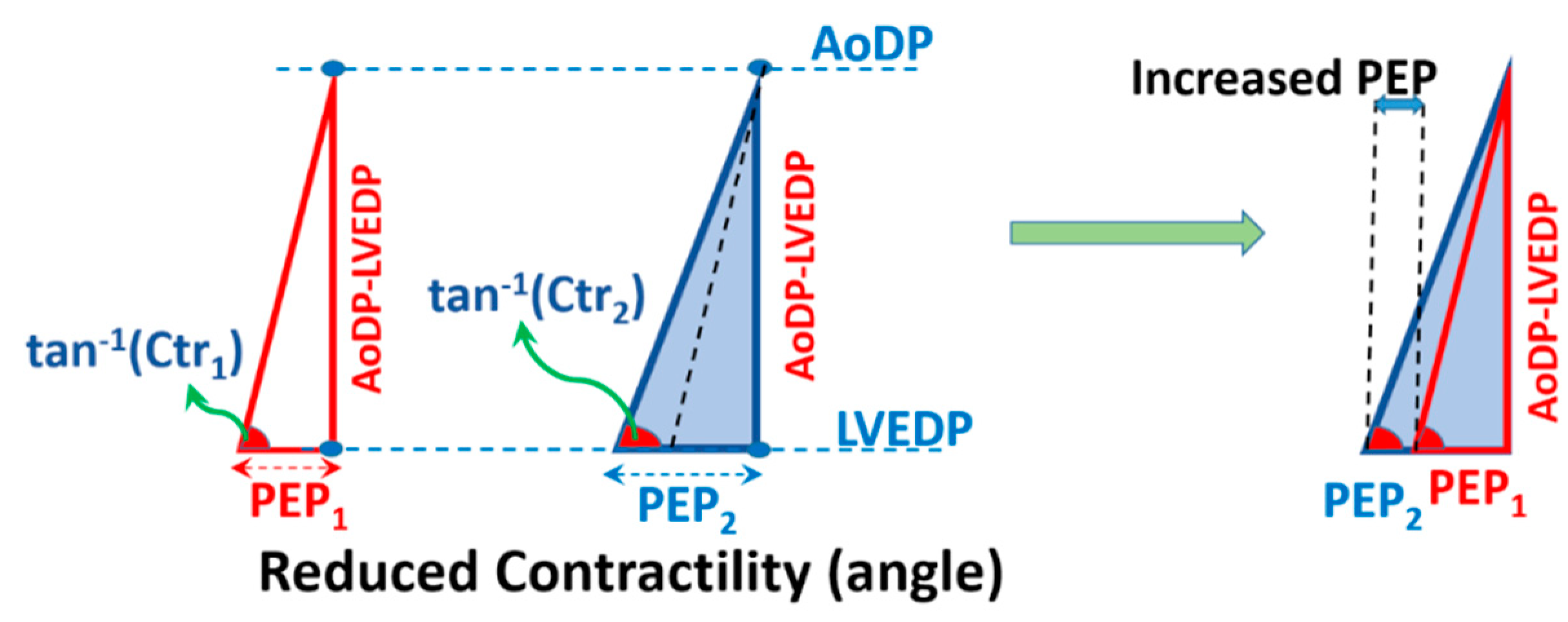
3.1. Compatibility of CTM with Hemodynamical Changes

3.1.1. Elevation of LVEDP at Constant Contractility and AoDP

As is illustrated in Figure 6, PEP decreases when LVEDP increases at fixed LV contractility and AoDP according to the CTM method. This is in agreement with the Talley et al. [20] results.

3.1.2. Changing Contractility at Constant LVEDP and AoDP

Talley et al. [20] demonstrated that PEP increases as LV contractility decreases when the LVEDP and aortic diastolic pressure are kept constant. As shown in Figure 7, the CTM cardiac triangle predicts the same conclusion.

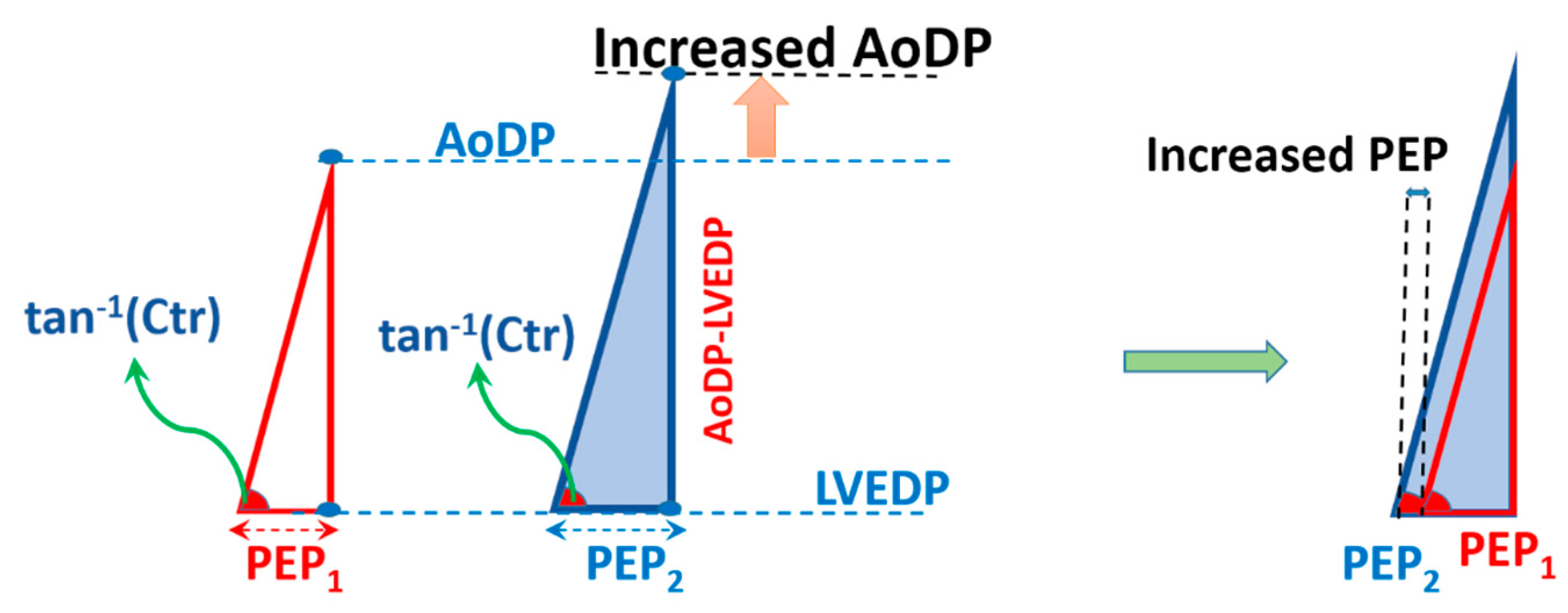
3.1.3. Elevation of AoDP at Constant Contractility and LVEDP

PEP increases when AoDP increases at constant contractility and constant LVEDP according to the CTM method (Figure 8). Talley et al. [20] also reported that increases in AoDP at constant contractility and constant LVEDP led to an increase of PEP [20].

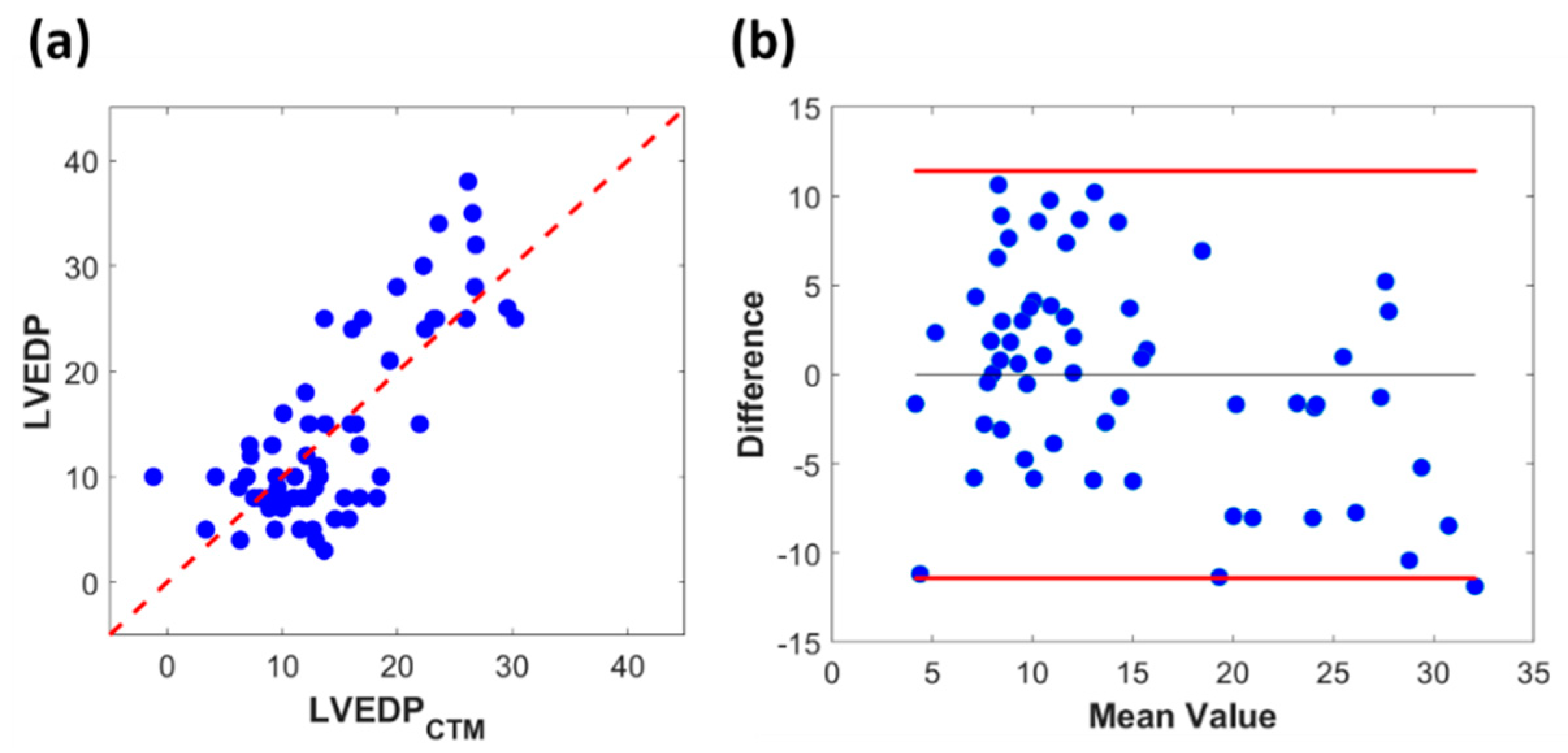
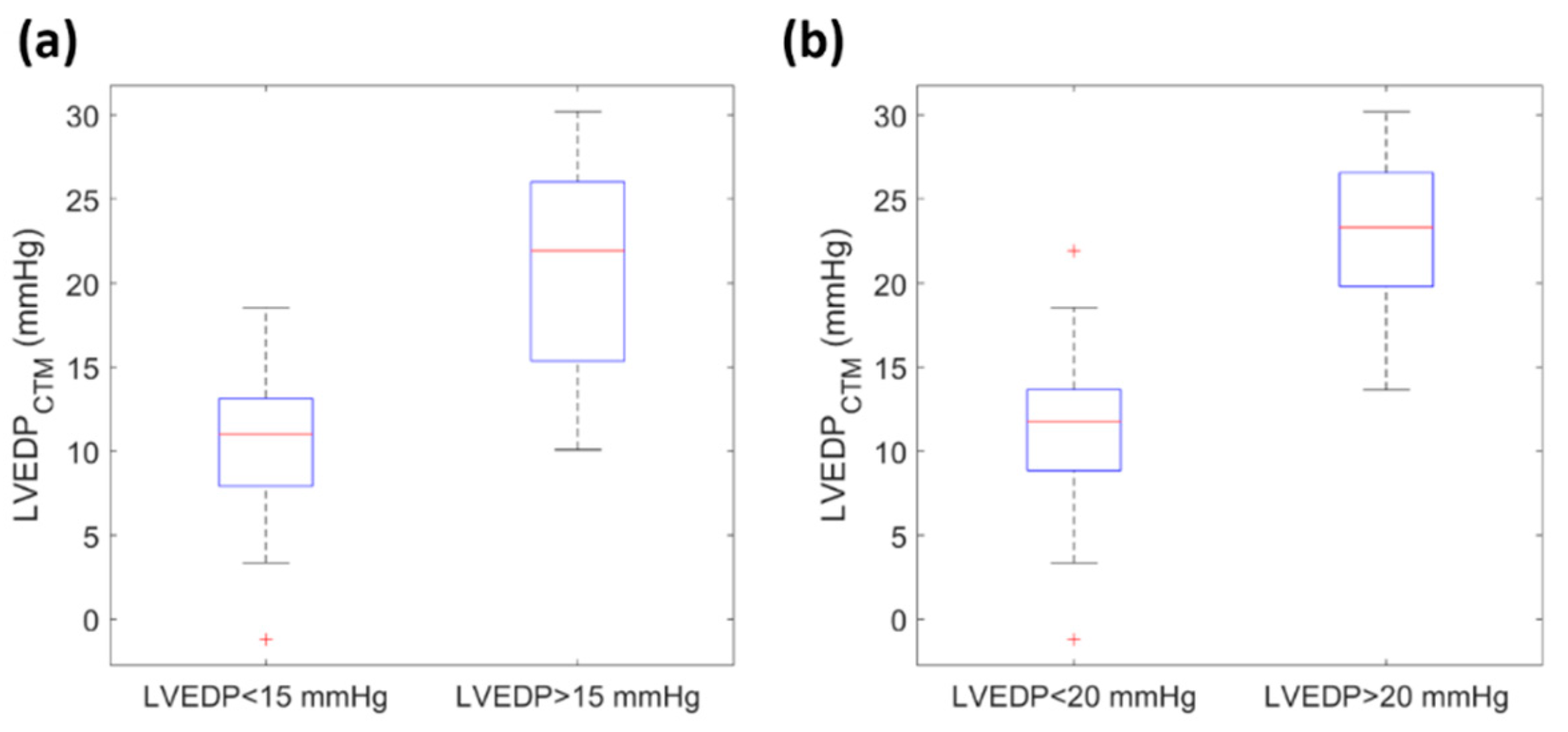
3.2. Clinical Results

Table 1. Hemodynamics and physiological values of the study population.

	Number or Mean Value (Range)	Standard Deviation
Age (years)	46 (19–65)	12
HR (bpm)	80 (55–114)	15
PEP (ms)	127 (82–171)	21.6
LVEF (%)	44 (8–84)	18
Gender (M/F)	36/26	NA
AoDP (mmHg)	72 (50–100)	10.6
T0 (ms)	241 (172–340)	38
LVEDP (mmHg)	15 (3–38)	9

HR is heart rate, PEP is pre-ejection period, LVEF is LV ejection fraction, AoDP is aortic diastolic pressure, T₀ is LV systolic ejection time, and LVEDP is LV end diastolic pressure.

shows the physiological and hemodynamical values of the study population. The dataset includes 62 subjects (36 men and 26 women), aged 19 to 65 years (mean = 46; SD = 20). The raw data of the results presented in this table, Figure 9 and Figure 10 are provided as supplementary data. It includes patients with various cardiovascular diseases, such as ischemic heart diseases, cardiomyopathy, atrial fibrillation, Mitral valve diseases, and aortic valve diseases (see Gerrard et al. [23] for more details).

LVEDP computed using the CTM method (LVEDP_CTM, Equation (9)) and invasively measured LVEDP were highly correlated, r = 0.76 (p < 0.0001) (Figure 9a). The limits of agreement (LoA) were ±11.4 mmHg (Figure 9b). The root mean square error (RMSE) was 5.9 mmHg.

Figure 10a is the boxplot for LVEDP_CTM, associated with values of greater or smaller than 15 mmHg (the threshold for diagnosis). Figure 10b is the boxplot for LVEDP_CTM, associated with values of greater or smaller than 20 mmHg (the threshold for therapeutic intervention).

4. Discussion

The results of this study provide the proof-of-concept that left ventricular end diastolic pressure (LVEDP) can be estimated noninvasively using the systems-based cardiac triangle mapping (CTM) method. The ability to monitor LVEDP noninvasively can revolutionize the diagnosis, monitoring, and treatment of HF patients. Moreover, this method provides a means to continually monitor HF patients at home, and maintain a compensated state by keeping patients’ LVEDP below a targeted value. It could also reduce rehospitalization for HF by preventing premature discharge.

Left heart catheterization is the gold standard clinical method for evaluating LVEDP. The alternative clinical practice for indirect assessment of filling pressure (LVEDP) is measuring pulmonary capillary wedge pressure (PCWP) in right-heart catheterization settings. PCWP measurement is also highly invasive, but does not require a Cathlab setting, and can be performed in intensive care units (ICUs). Recent clinical studies have indicated that PCWP is poorly calibrated to LVEDP (Bland-Altman limits of agreement (LoA) of −15.2 to 9.5 mmHg according to Halpern et al. [24] and −17.2 to 11.4 mmHg according to Bitar et al. [25]). A recent study [25] has shown a moderate correlation between PCWP and LVEDP, r = 0.57. Methods based on the Valsalva maneuver (forced expiration against a closed glottis) have been recently proposed for the indirect approximation of LV filling pressure [26,27]. These methods are based on multiple studies, which demonstrated that the Valsalva maneuver can help diagnose HF since changes in the strain phase of arterial waveforms due to the Valsalva maneuver are related to the LV filling pressure [28,29,30]. Major limitations of Valsalva-based methods include the need to maintain uniform strain during the maneuver and risk of developing ectopy during the maneuver [26]. It is noteworthy that recent studies based on these methods [31,32] have only indicated a moderate correlation with filling pressure measures, such as PCWP [32] (r = 0.58) and LVEDP [31] (r = 0.56). Some studies have proposed echocardiography-based approaches for the assessment of LV filling pressure [33,34]. Recently, Anderson et al. [35] presented an echocardiographic-based algorithm that provides an accurate estimation of LV filling pressure. However, this study did not include patients without cardiac diseases, and echo requires expert interpretation. Use of a wireless implantable system for monitoring pulmonary artery pressures in outpatients was shown to significantly reduce hospitalizations in heart failure patients [3]. However, implementation of this device requires an invasive procedure, is relatively expensive, and long-term outcomes remain to be answered. While these implantable devices are proving to be useful in more advanced heart failure patients, there is nothing available to frequently monitor the much larger group of patients with less severe disease. Additionally, there are significant patient groups where pulmonary diastolic pressure is not an accurate estimation of LVEDP. Diseased states, such as pulmonary hypertension and chronic lung disease, can lead to significant overestimation of LVEDP using pulmonary artery diastolic pressure. The proposed CTM method is a systems approach based on intrinsic frequency (IF) that provides a noninvasive, instantaneous, and practical way of measuring LVEDP in any setting, including at home.

Our first step in verifying the validity of the CTM method was to show that the triangle in CTM (Figure 4 and Figure 5) remains intact as the LV-arterial system goes through functional changes. Talley et al. [20] have performed a series of animal experiments to study LV contractility and PEP using dog models. They demonstrated that increases in LVEDP at fixed contractility (as measured by dp/dt_max) and afterload (as quantified by AoDP) result in PEP reduction. The CTM method predicts the same pattern as illustrated in Figure 6. Furthermore, the results in Talley et al. [20] also demonstrate that PEP increases as LV contractility decreases when LVEDP and AoDP are kept constant. As shown in Figure 7, CTM theory predicts the same conclusion. Additionally, the study reports that increases in AoDP at constant contractility and constant LVEDP lead to an increase of PEP [20]. Again, CTM predicts the same results (Figure 8). These observations point to the physiological validity of the proposed CTM method.

Our LVEDP results for the CTM method are based on retrospective published data [23]. Since LV and arterial pressure waveforms were not available in the dataset, the direct computations of ω₁ and ω₂ were not possible. Therefore, Equation (8) was modified to Equation (9) and instead of IFs, their functional equivalents, such as LV contractility surrogate (LVEF) and afterload surrogate (AoDP), were used. As shown in Figure 9a,b, there is a good agreement between the direct invasive measurement of LVEDP and LVEDP from the CTM method (r = 0.76, LoA = ±11.4, and RMSE = 5.9 mmHg). The clinical significance and value of this method is highlighted when these r = 0.76 and LoA = ±11.4 values are compared to recently reported r (0.56–0.58) and LoA (−15.2 to 9.5 and −17.2 to 11.4) of PCWP and other noninvasive LVEDP surrogates [31,32,33,34]. More importantly, since IFs (of ω₁ and ω₂) carry the nonlinearity of the system, the accuracy of LVEDP computation from the CTM method (using Equation (8)) might be even higher than the reported results in this manuscript. Whether the accuracy is indeed higher is yet to be determined in future prospective clinical studies when LVEDP, PEP, ω₁, and ω₂ will be measured simultaneously.

As exemplified in Figure 10a, the CTM method demonstrates a clear separation between subjects with elevated LVEDP (15 mmHg) and subjects with normal LVEDP (<15 mmHg). This divergence points to the usefulness of CTM in the diagnosis of decompensated HF patients. As demonstrated in Figure 10b, the CTM method can also distinguish reasonably well between subjects with LVEDP greater than 20 mmHg (a possible cutoff for immediate therapeutic intervention), and those with LVEDP less than 20 mmHg.

The major limitation of this study is using Equation (9), which employs LVEF, instead of Equation (8), which requires IFs. This limitation was unavoidable due to the retrospective dataset we used, where carotid waveforms were not available. However, recent studies [7,8,13] indicate that LVEF can be computed from IFs of the pressure waveforms. Our goal in this paper was to propose the CTM theory and link it to IFs. Our plan is to assess the accuracy of Equation (8) using IFs in a prospective heterogeneous cohort. An accurate PEP calculation is essential in the computation of LVEDP from the CTM method. The accuracy of the PEP calculation depends primarily on the following criteria: First, the quality of ECG measurement; second, the quality of the arterial pressure waveform measurement; third, accurate identification of the beginning of the Q-wave from the QRS complex; and lastly, accurate identification of the opening of the aortic valve from the pressure waveform. Due to the retrospective nature of the data we were not able to assess the accuracy of PEP calculations.

5. Conclusions

The results of this paper suggest that LVEDP can be approximated from IFs’ surrogates and PEP using the CTM method. Since both PEP and IFs can be measured noninvasively, the result of this study will enable care providers and patients to measure LVEDP noninvasively. Although the mathematics of IF for measuring noninvasive LVEDP is complex, the computation of all variables required for LVEDP, including ω₁ and ω₂, can be completed automatically in a fraction of a second from the carotid waveform and ECG signal input. Furthermore, since the CTM method only requires arterial waveform and ECG, and both of these can be measured by a smartphone [8,36], the CTM method can eventually be adopted as a simple smartphone-based device, an inexpensive hand-held device (similar to Armenian et al. [13]), or perhaps in future design modifications a wearable sensor. Such devices combined with this method would allow for remote monitoring of patients, maintenance of a compensated state by keeping patients’ LVEDP below a targeted value from the comfort of their own home, and immediate access to their patient data remotely by health care providers.