Fundamental Rheology of Disperse Systems Based on Single-Particle Mechanics
Abstract
:1. Introduction
- Rigid-solid spherical particles: uncharged and electrically charged
- Rigid-porous spherical particles
- Non-rigid (soft) solid particles
- Liquid droplets: without and with surfactant
- Bubbles: without and with surfactant
- Capsules
- Core-shell particles: solid core-porous shell, solid core-liquid shell, liquid core-liquid shell
- Rigid-solid non-spherical particles
- Ferromagnetic rigid-solid spherical and non-spherical particles
2. Bulk Stress and Bulk Rate of Strain in Particulate Fluids
3. Rheological Constitutive Equation for Particulate Fluids
4. Rheology of Suspensions of Rigid-Solid Spherical Particles
4.1. Electrically-Neutral Particles
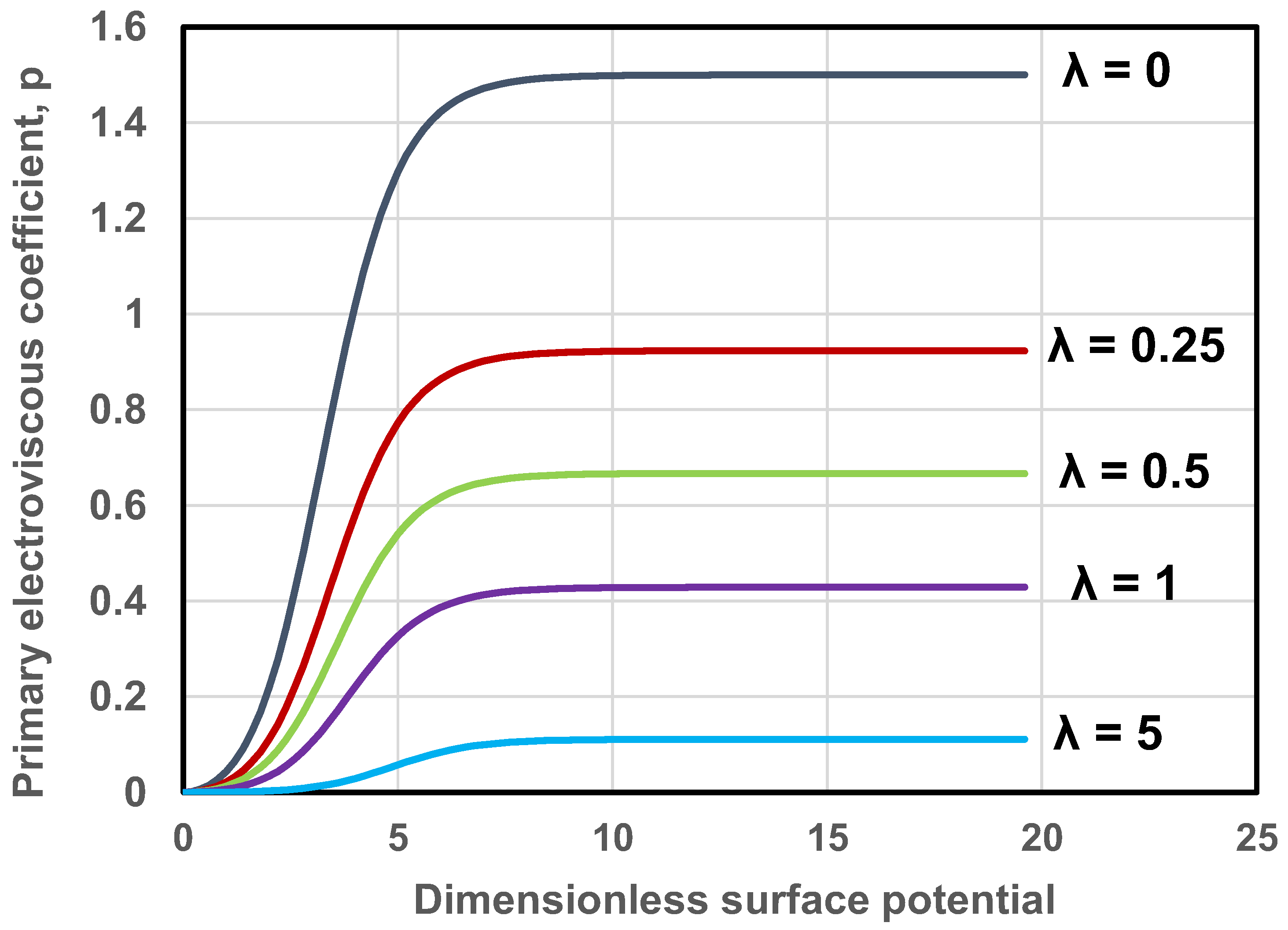
4.2. Electrically-Charged Particles
5. Rheology of Suspensions of Rigid-Porous Spherical Particles
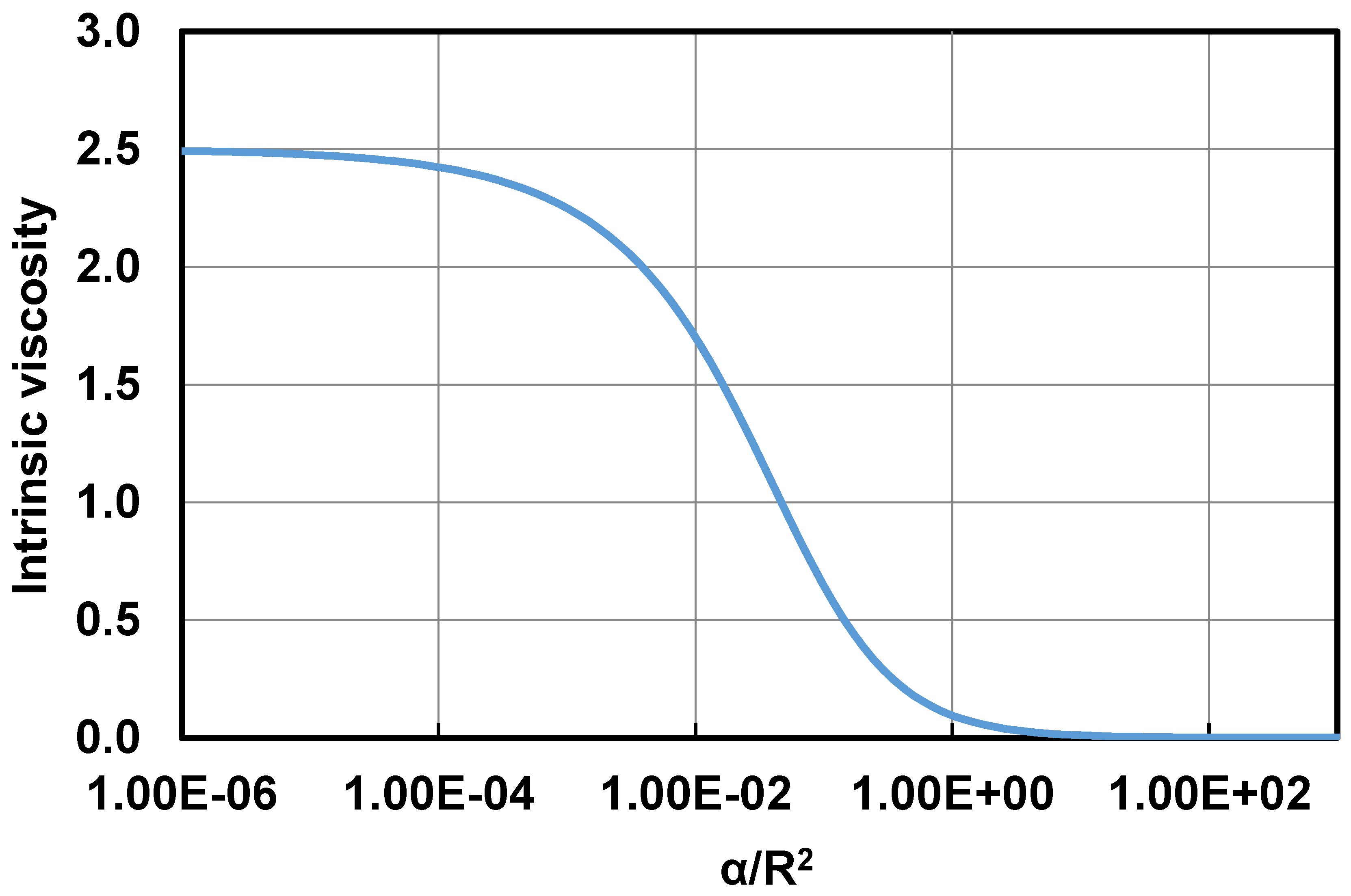
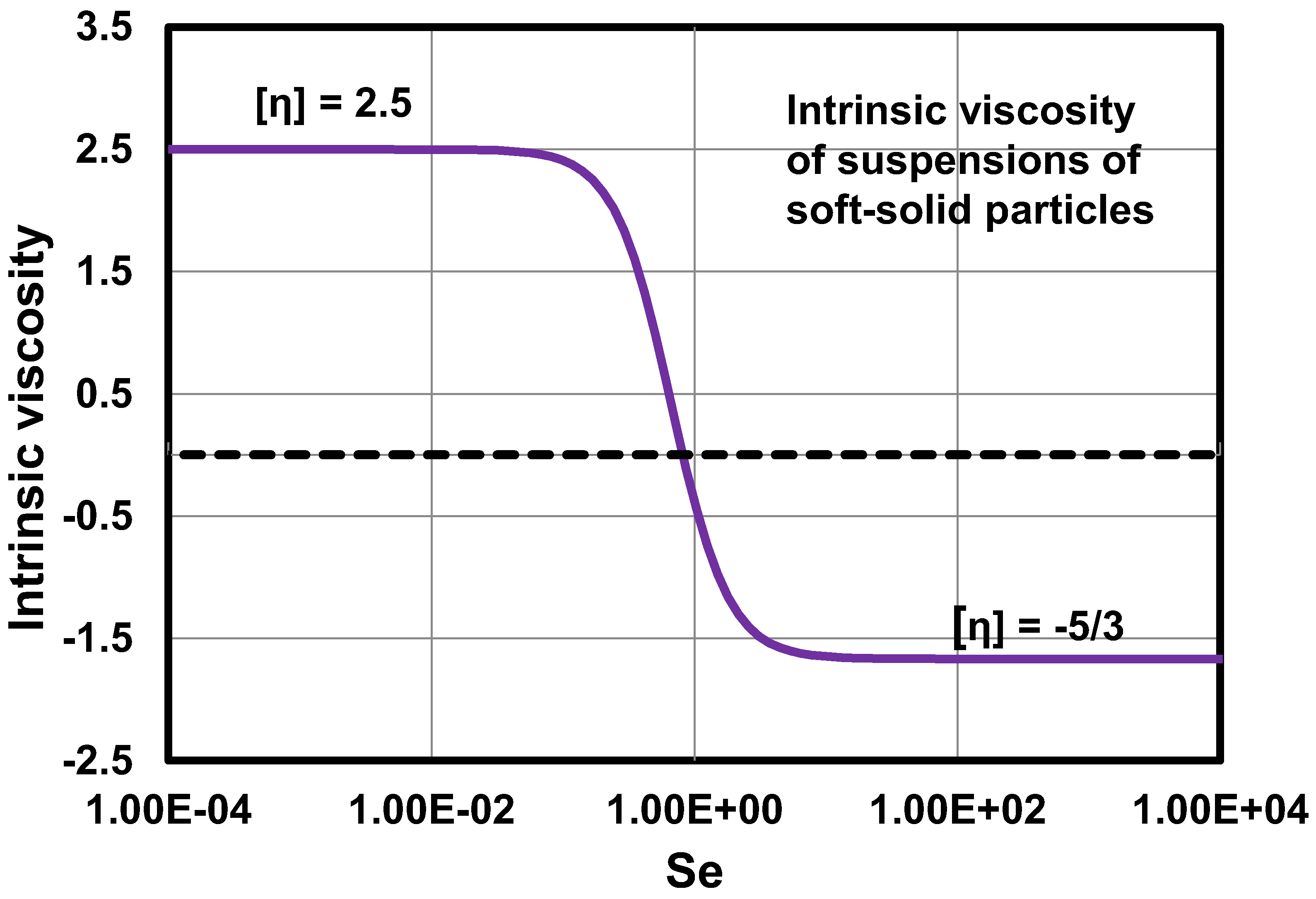
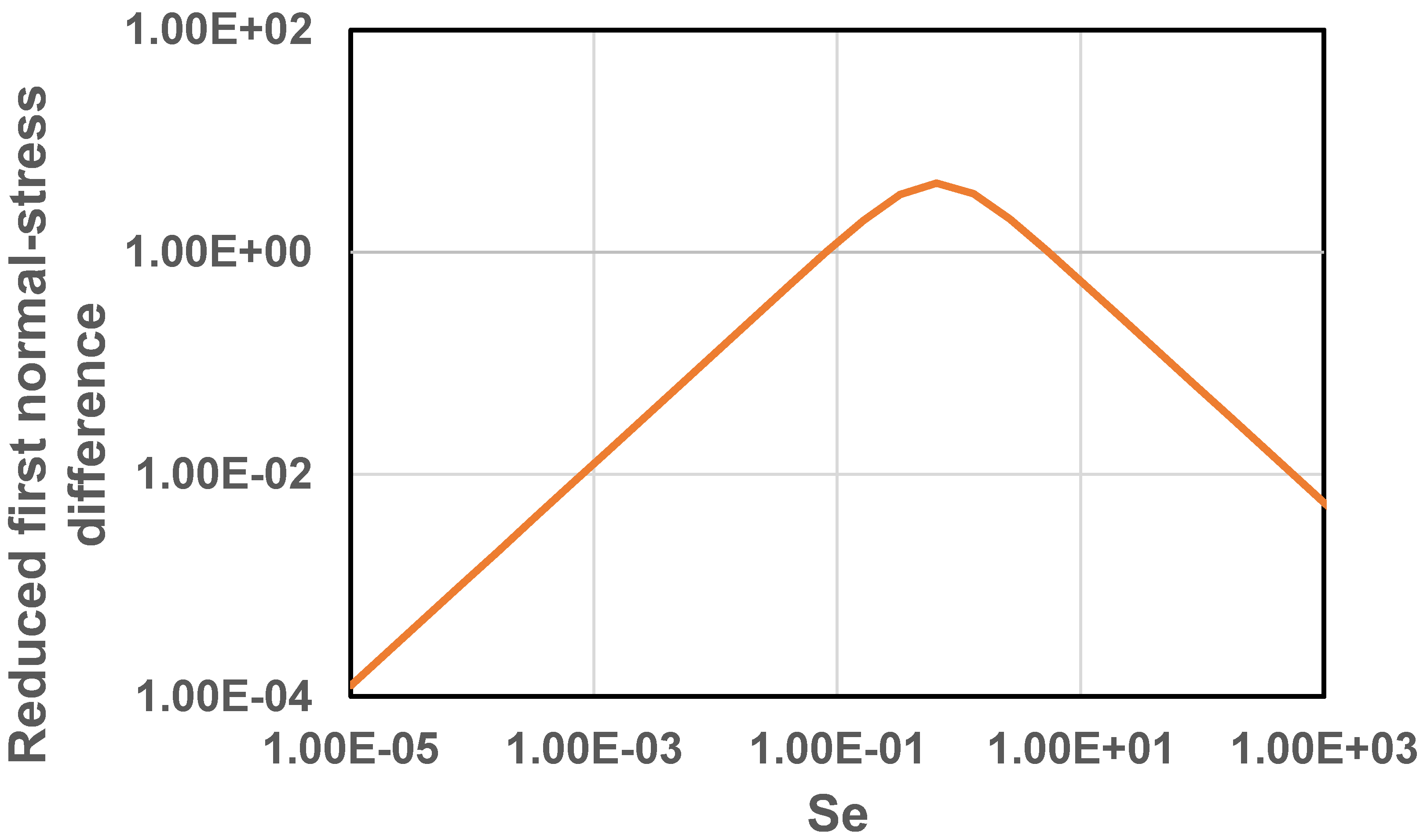
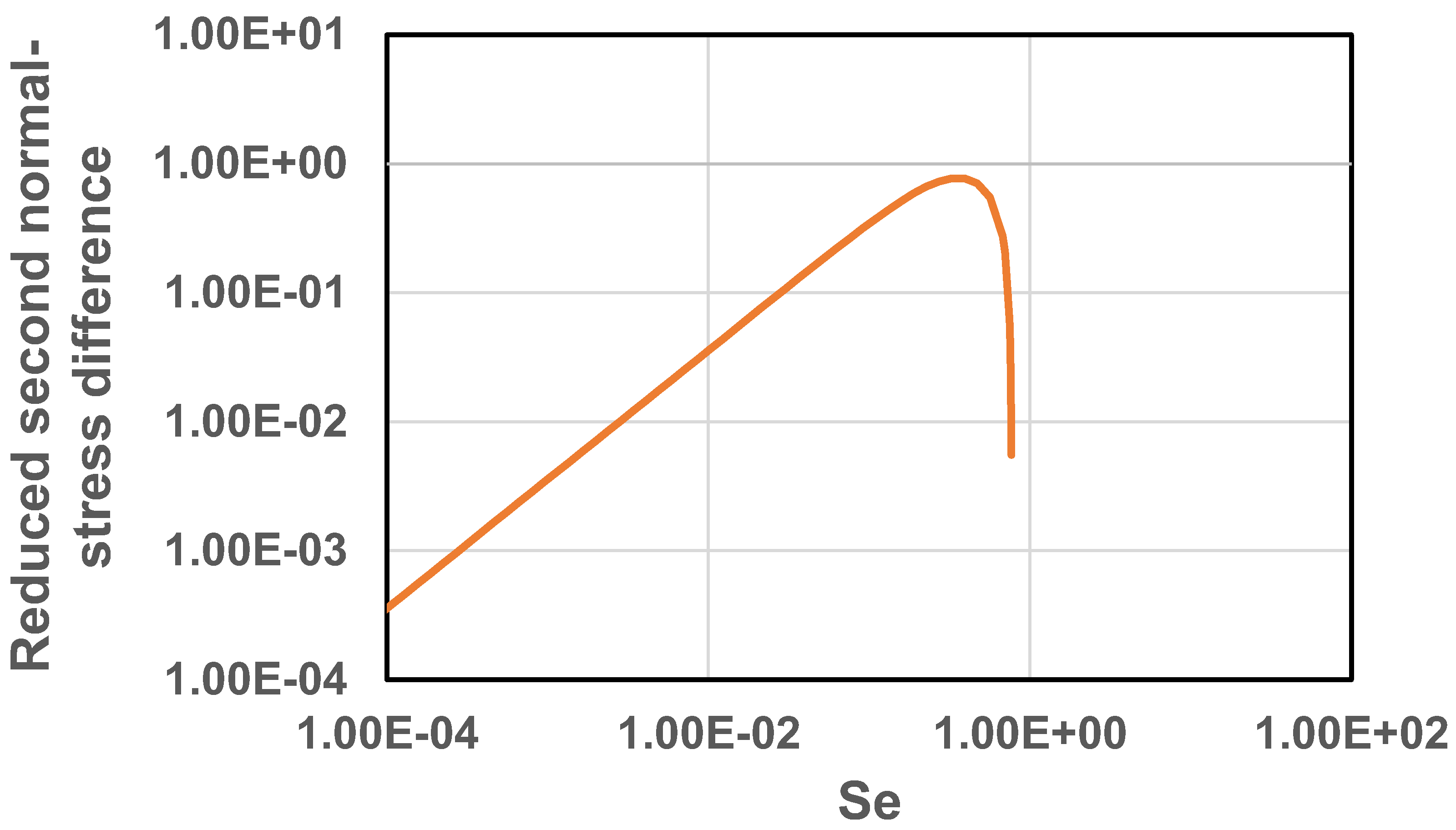
6. Rheology of Suspensions of Non-Rigid (Soft) Solid Particles
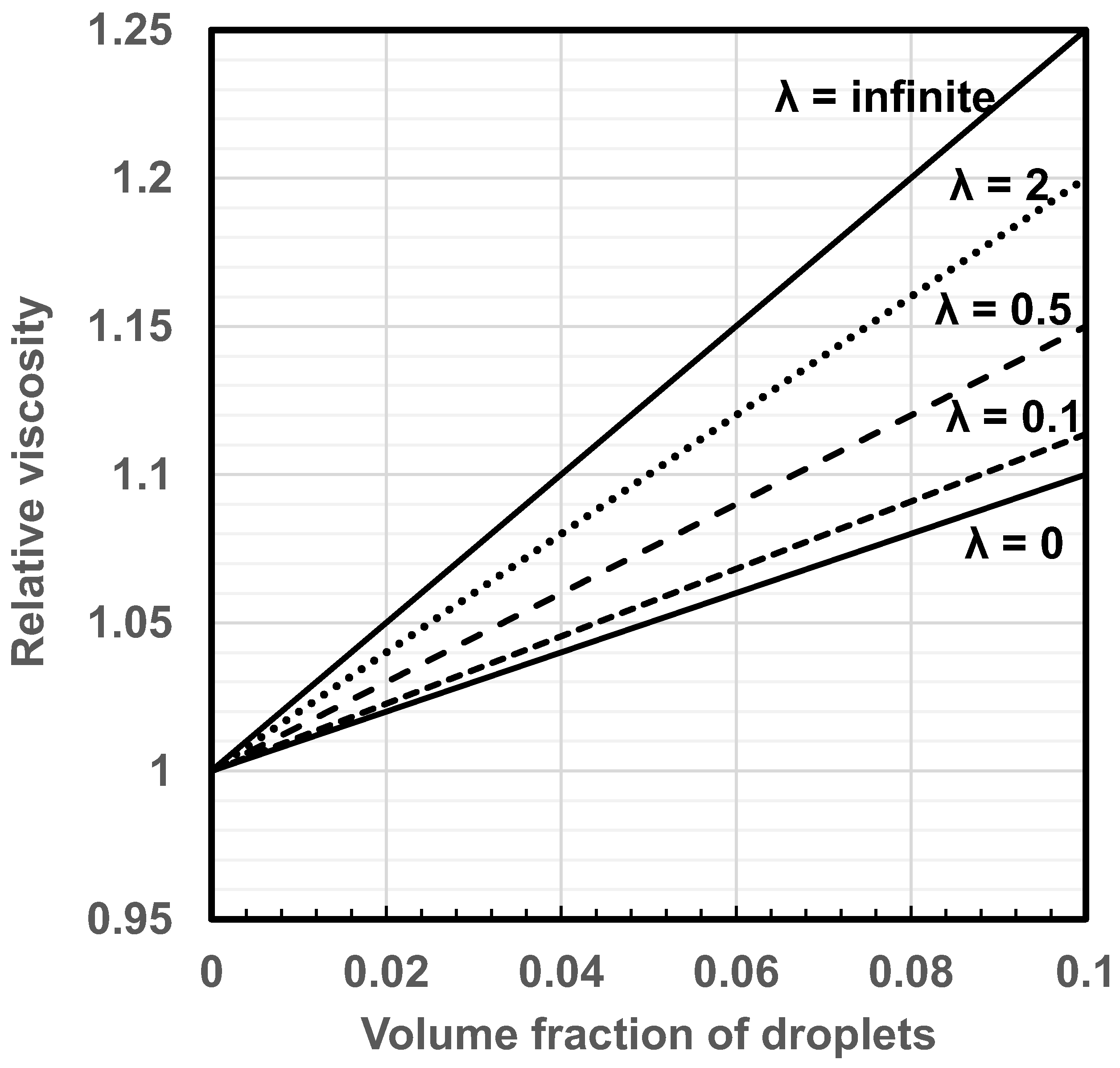
7. Rheology of Emulsions
7.1. Influence of Electric Charge Present on the Surface of Emulsion Droplets
7.2. Influence of Surfactant on Emulsion Rheology
8. Rheology of Bubbly Suspensions
8.1. Influence of Electric Charge Present on the Surface of Bubbles
8.2. Influence of Surfactant Present on the Surface of Bubbles
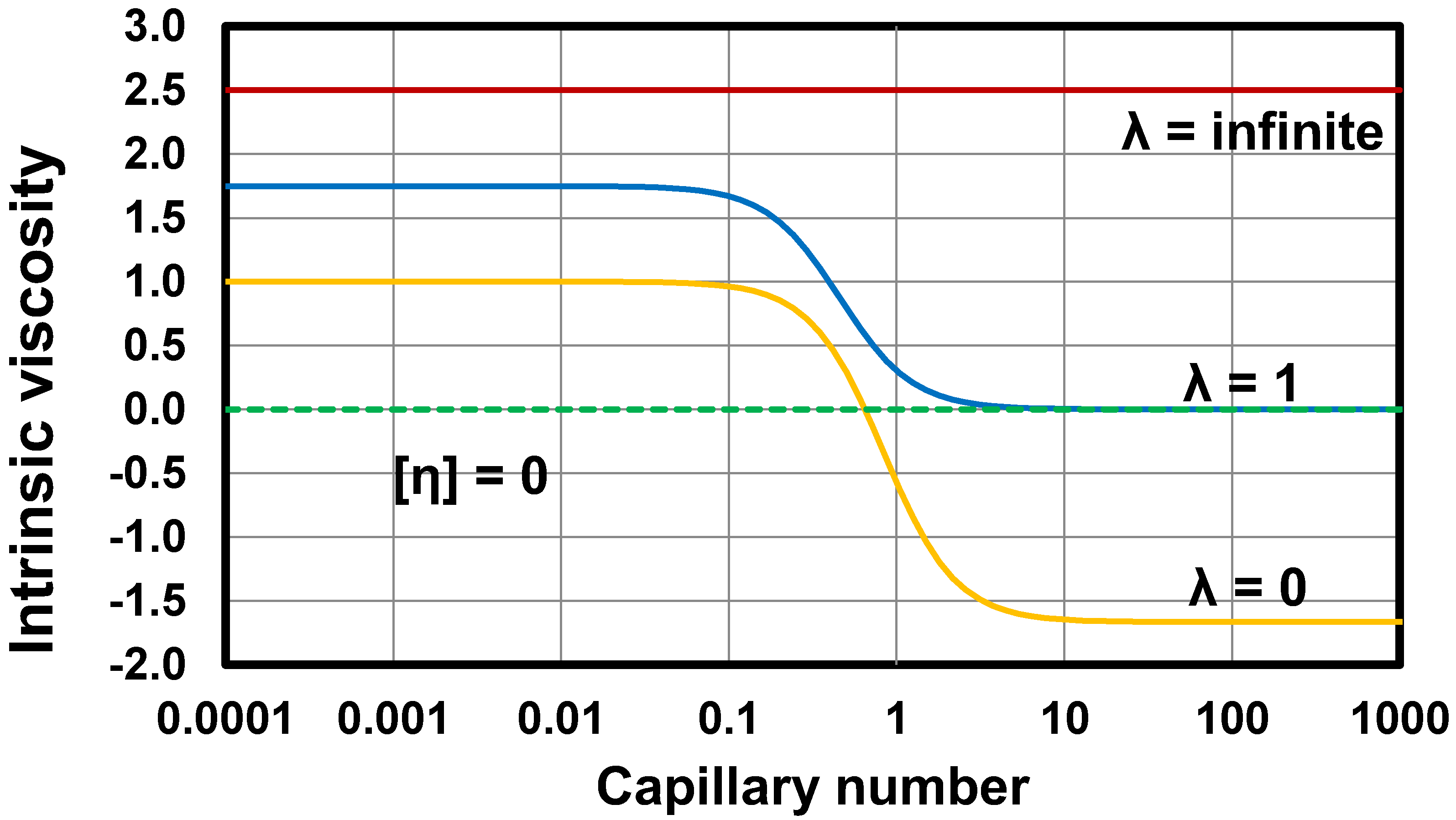
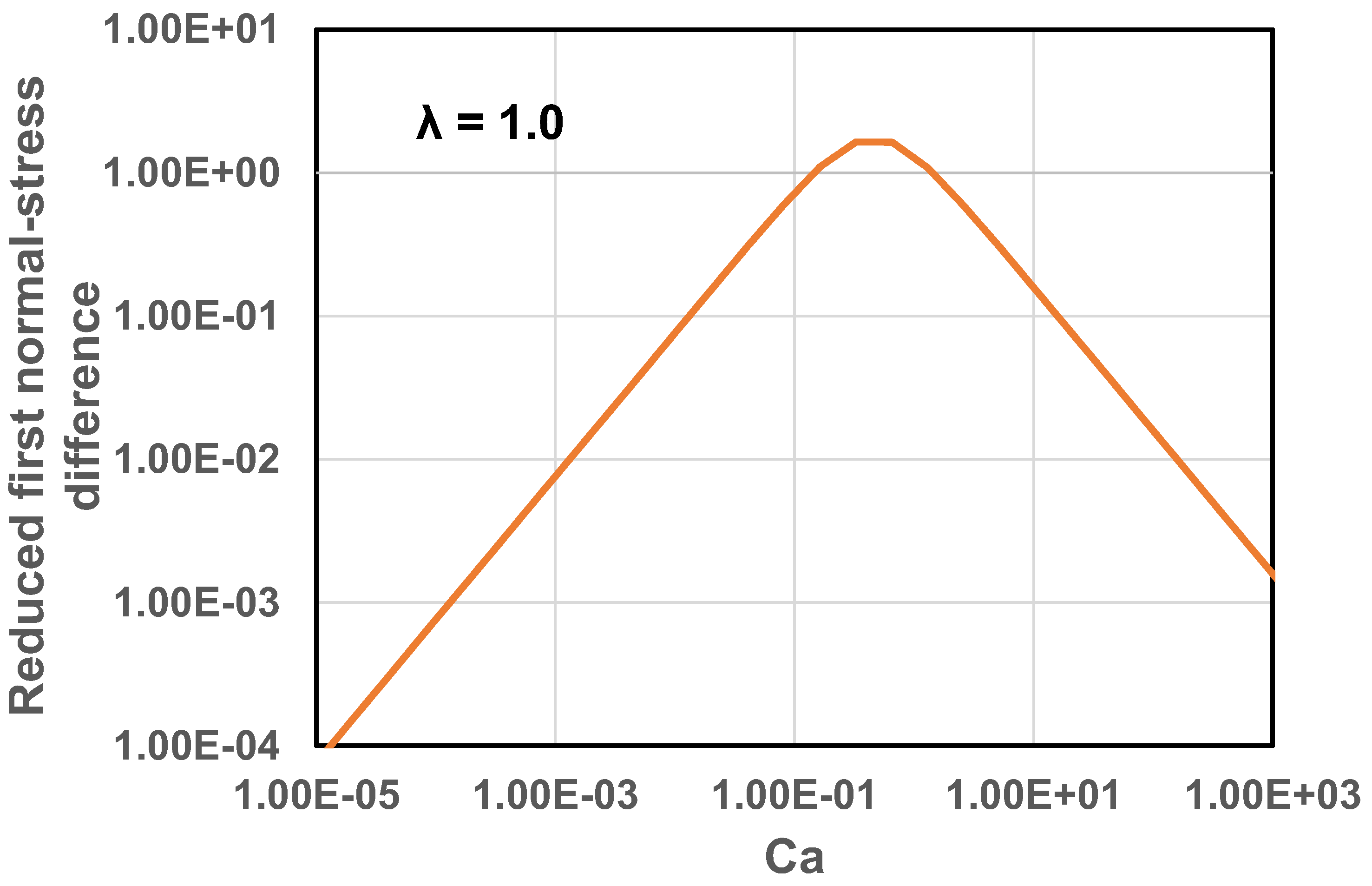
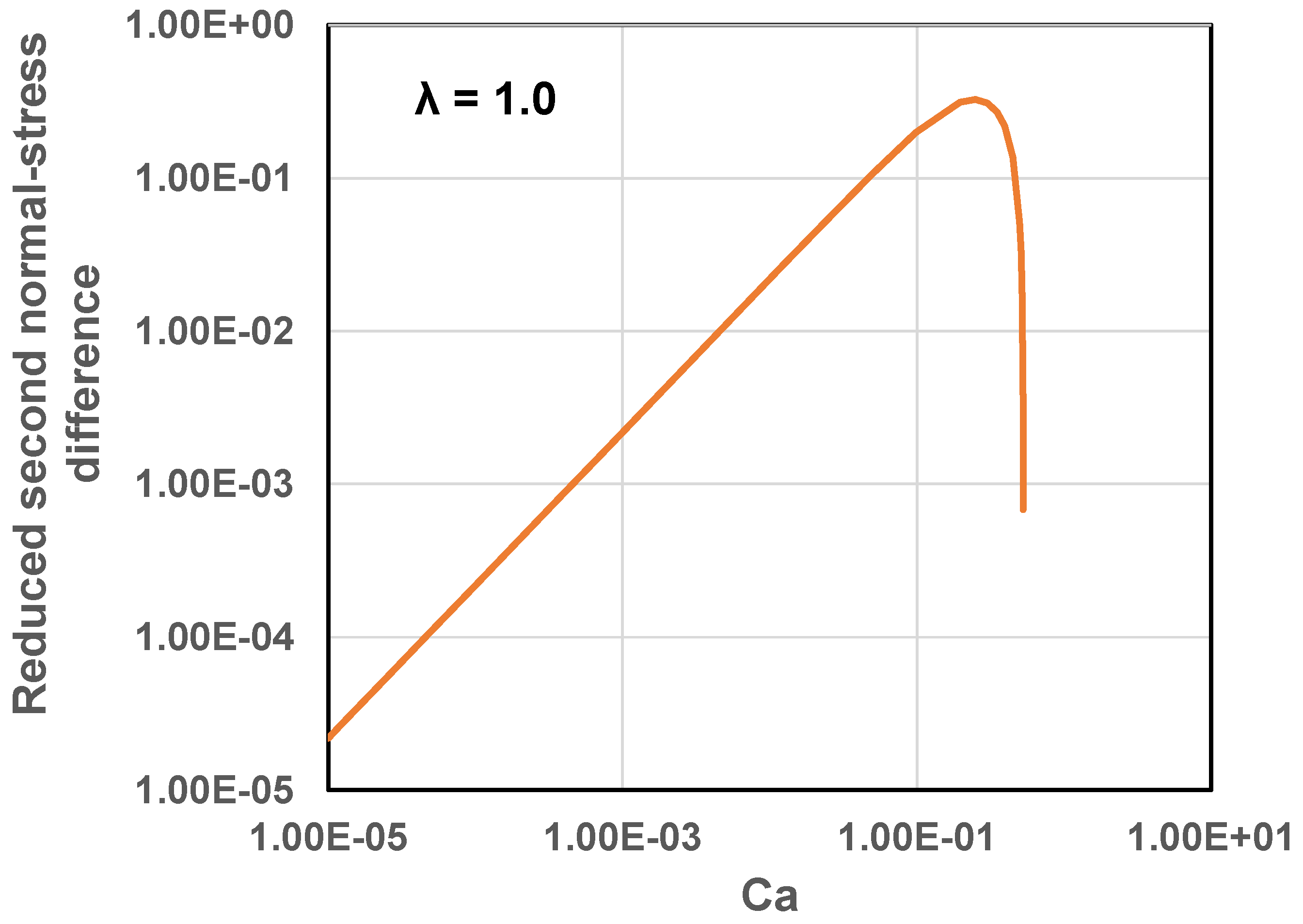
8.3. Influence of Capillary Number
9. Rheology of Suspensions of Capsules
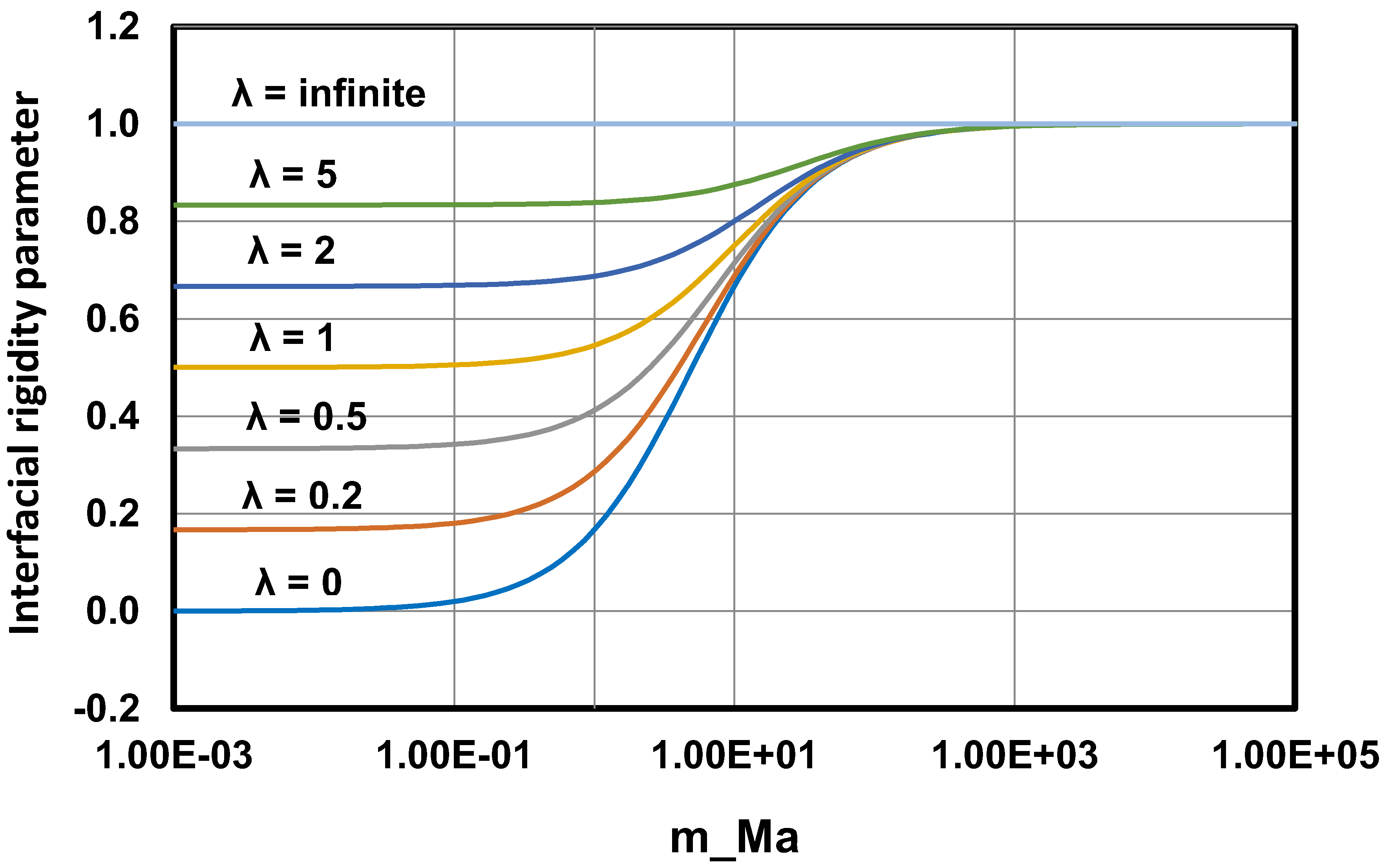
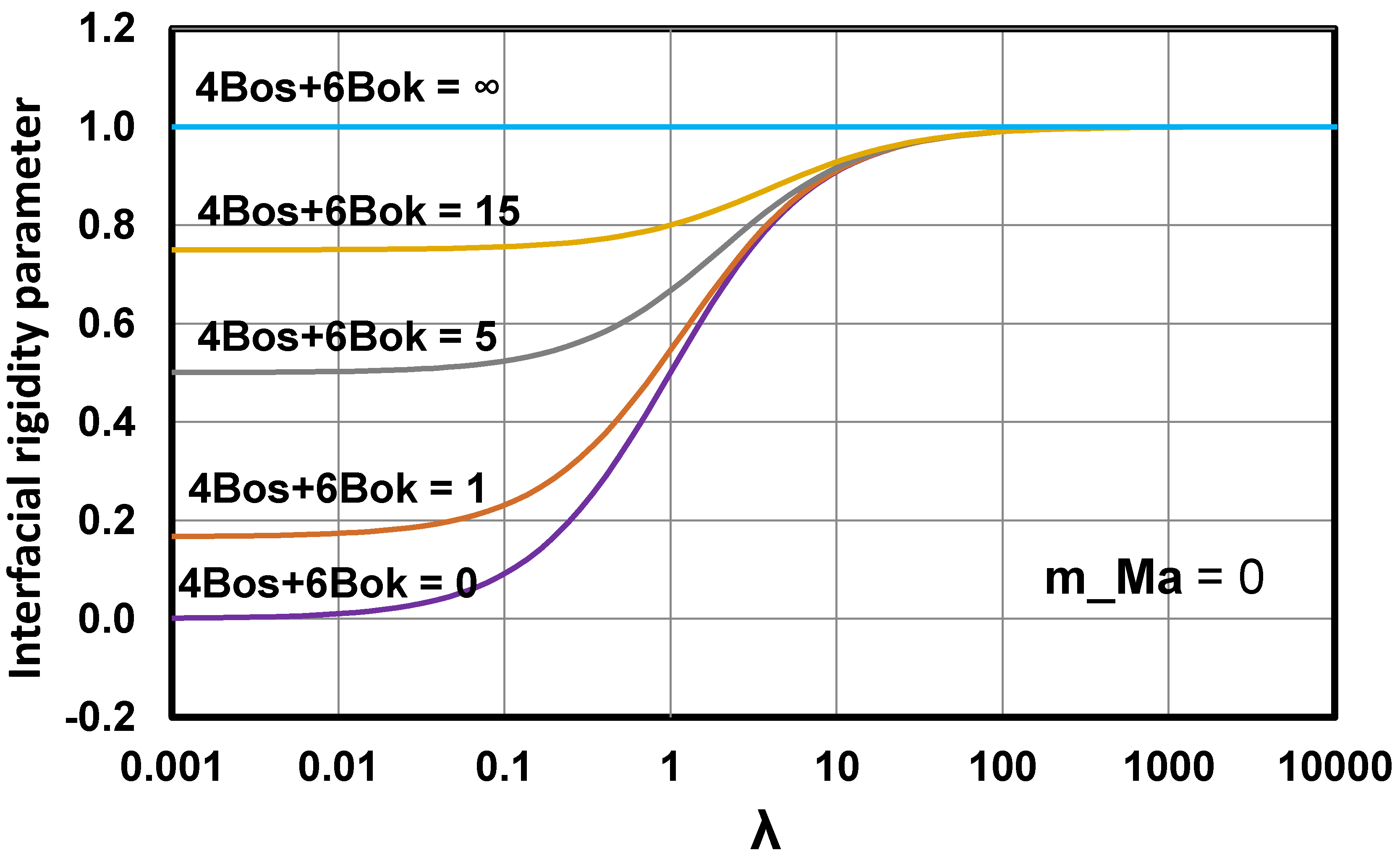
10. Rheology of Suspensions of Core-Shell Particles
10.1. Solid Core—Porous Shell
10.2. Solid Core—Liquid Shell
10.3. Liquid Core—Liquid Shell
11. Rheology of Suspensions of Rigid Non-Spherical Particles
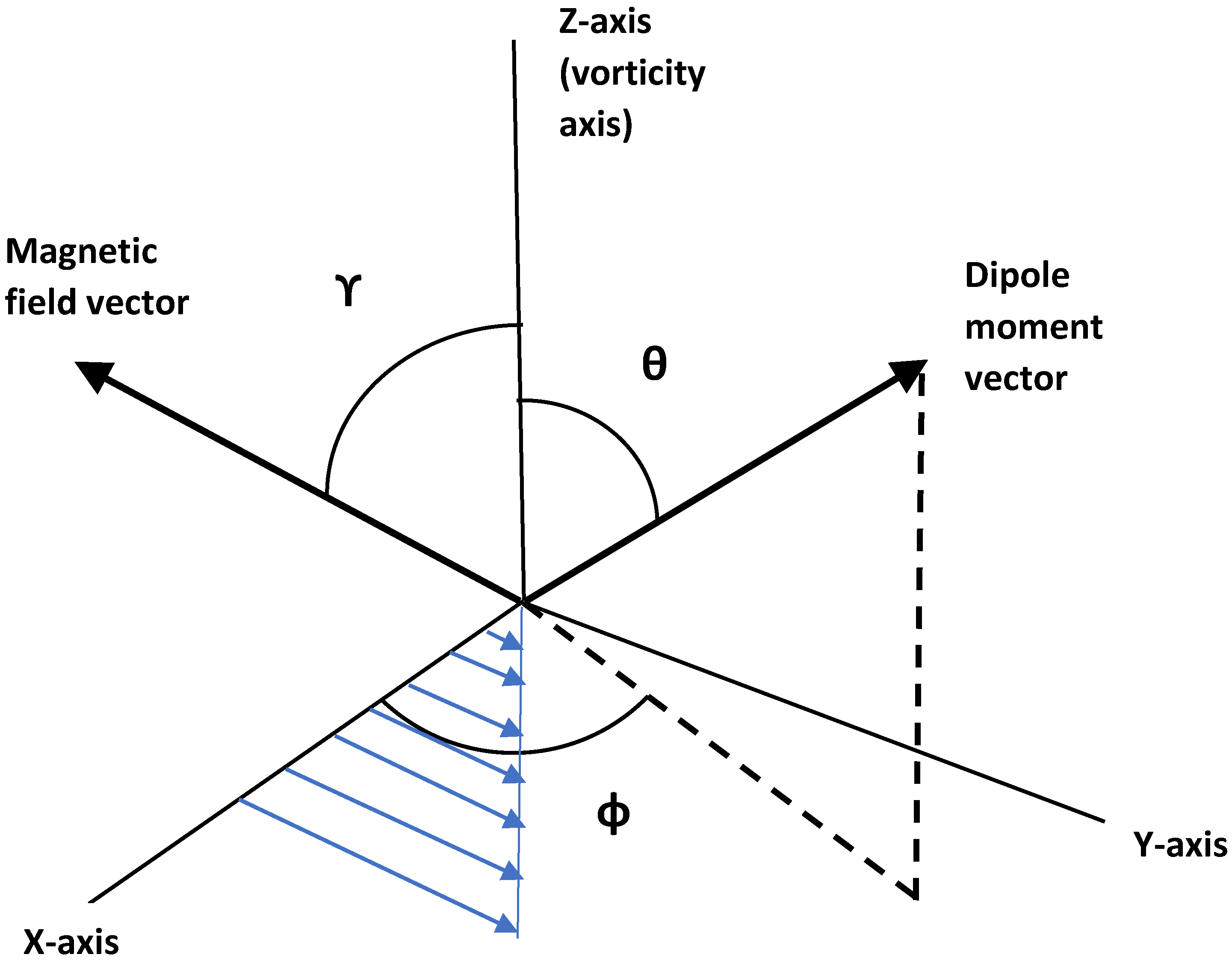
12. Rheology of Suspensions of Ferromagnetic Particles
13. Concluding Remarks
Conflicts of Interest
References
- Kissa, E. Dispersions; Marcel Dekker: New York, NY, USA, 1999. [Google Scholar]
- Conley, R.F. Practical Dispersions; VCH Publishers: New York, NY, USA, 1996. [Google Scholar]
- McKay, R.B. Technological Applications of Dispersions; Marcel Dekker: New York, NY, USA, 1994. [Google Scholar]
- Morrison, I.D.; Ross, S. Colloidal Dispersions; Wiley: New York, NY, USA, 2002. [Google Scholar]
- Wasan, D.; Nikolov, A.; Henderson, D. New vistas in dispersion science and engineering. AIChE J. 2003, 49, 550–556. [Google Scholar] [CrossRef]
- Batchelor, G.K. The stress system in a suspension of force-free particles. J. Fluid Mech. 1970, 41, 545–570. [Google Scholar] [CrossRef]
- Brenner, H. Rheology of two-phase systems. Rev. Fluid Mech. 1970, 2, 137–176. [Google Scholar] [CrossRef]
- Brenner, H. Suspension rheology. Prog. Heat Mass Transf. 1972, 5, 89–129. [Google Scholar]
- Pal, R. Rheology of Particulate Dispersions and Composites; CRC Press: Boca Raton, FL, USA, 2007. [Google Scholar]
- Batchelor, G.K. Transport properties of two-phase materials with random structure. Annu. Rev. Fluid Mech. 1974, 6, 227–255. [Google Scholar] [CrossRef]
- Einstein, A. Eine neue Bestimmung der Molekul-dimension. Ann. Phys. 1906, 19, 289–306. (In German) [Google Scholar] [CrossRef]
- Einstein, A. Berichtigung zu meiner Arbeit: Eine neue Bestimmung der Molekul-dimension. Ann. Phys. 1911, 339, 591–592. (In German) [Google Scholar] [CrossRef]
- De Kruif, C.G.; Van lersel, E.M.F.; Vrij, A.; Russel, W.B. Hard sphere colloidal dispersions: Viscosity as a function of shear rate and volume fraction. J. Chem. Phys. 1985, 83, 4717–4725. [Google Scholar] [CrossRef]
- Jones, D.A.R.; Leary, B.; Boger, D.V. The rheology of concentrated colloidal suspension of hard spheres. J. Colloid Interface Sci. 1991, 147, 479–495. [Google Scholar] [CrossRef]
- Cheng, P.Y.; Schachman, H.K. Studies on the validity of the Einstein viscosity law and Stokes’ law of sedimentation. J. Polym. Sci. 1955, 16, 19–30. [Google Scholar] [CrossRef]
- Pasquino, R.; Grizzuti, N.; Maffettone, P.L. Rheology of dilute and semidilute noncolloidal hard sphere suspensions. J. Rheol. 2008, 52, 1369–1384. [Google Scholar] [CrossRef]
- Brady, J.F.; Khair, A.S.; Swaroop, M. On the bulk viscosity of suspensions. J. Fluid. Mech. 2006, 554, 109–123. [Google Scholar] [CrossRef]
- Russel, W.B. The rheology of suspensions of charged rigid spheres. J. Fluid Mech. 1978, 85, 209–232. [Google Scholar] [CrossRef]
- Russel, W.B. Bulk stresses due to deformation of the electrical double layer around a charged sphere. J. Fluid Mech. 1978, 85, 673–683. [Google Scholar] [CrossRef]
- Von Smoluchowski, M. Studien uber Kolloidstatistik Mechanismus der Diffusion. Kolloid Z. 1916, 18, 48–54. [Google Scholar] [CrossRef]
- Booth, F. The electroviscous effect for suspensions of solid spherical particles. Proc. R. Soc. Lond. Ser. A 1950, 203, 533–551. [Google Scholar] [CrossRef]
- Lever, D.A. Large distortion of the electrical double layer around a charged particle by a shear flow. J. Fluid Mech. 1979, 92, 421–433. [Google Scholar] [CrossRef]
- Sherwood, J.D. The primary electroviscous effect in a suspension of spheres. J. Fluid Mech. 1980, 101, 609–629. [Google Scholar] [CrossRef]
- Watterson, I.G.; White, L.R. Primary electroviscous effect in suspensions of charged spherical particles. J. Chem. Soc. Faraday Trans. 2 1981, 77, 1115–1128. [Google Scholar] [CrossRef]
- Hinch, E.J.; Sherwood, J.D. The primary electroviscous effect in a suspension of spheres with thin double layers. J. Fluid Mech. 1983, 132, 337–347. [Google Scholar] [CrossRef]
- Mcdonogh, R.W.; Hunter, R.J. The primary electroviscous effect. J. Rheol. 1983, 27, 189–199. [Google Scholar] [CrossRef]
- Rubio-Hernandez, F.J.; Carrique, F.; Ruiz-Reina, E. The primary electroviscous effect in colloidal suspensions. Adv. Colloid Interface Sci. 2004, 107, 51–60. [Google Scholar] [CrossRef] [PubMed]
- Rubio-Hernandez, F.J.; Gomez-Merino, A.I.; Ruiz-Reina, E.; Garcia-Sanchez, P. An experimental test of Booth’s primary electroviscous effect theory. J. Colloid Interface Sci. 2002, 255, 208–213. [Google Scholar] [CrossRef] [PubMed]
- Ohshima, H. Primary electroviscous effect in a dilute suspension of charged mercury drops. Langmuir 2006, 22, 2863–2869. [Google Scholar] [CrossRef] [PubMed]
- Ohshima, H. Primary electroviscous effect in a moderately concentrated suspension of charged spherical particles. Langmuir 2007, 23, 12061–12066. [Google Scholar] [CrossRef] [PubMed]
- Khair, A.S.; Star, A.G. The bulk electroviscous effect. Rheol. Acta 2013, 52, 255–269. [Google Scholar] [CrossRef]
- Zackrisson, M.; Bergenholtz, J. Intrinsic viscosity of dispersions of core-shell particles. Colloids Surfaces A 2003, 225, 119–127. [Google Scholar] [CrossRef]
- Natraj, V.; Chen, S.B. Primary electroviscous effect in a suspension of charged porous spheres. J. Colloid Interface Sci. 2002, 251, 200–207. [Google Scholar] [CrossRef] [PubMed]
- Goddard, J.D.; Miller, C. Nonlinear effects in the rheology of dilute suspensions. J. Fluid Mech. 1967, 28, 657–673. [Google Scholar] [CrossRef]
- Roscoe, R. On the rheology of a suspension of viscoelastic spheres in a viscous liquid. J. Fluid Mech. 1967, 28, 273–293. [Google Scholar] [CrossRef]
- Gao, T.; Hu, H.H. Deformation of elastic particles in viscous shear flow. J. Comput. Phys. 2009, 228, 2132–2151. [Google Scholar] [CrossRef]
- Gao, T.; Hu, H.H.; Ponte Castaneda, P. Rheology of a suspension of elastic particles in a viscous shear flow. J. Fluid Mech. 2011, 687, 209–237. [Google Scholar] [CrossRef]
- Gao, T.; Hu, H.H.; Ponte Castaneda, P. Shape dynamics and rheology of soft elastic particles in a shear flow. Phys. Rev. Lett. 2012, 108, 4. [Google Scholar] [CrossRef] [PubMed]
- Wetzel, E.D.; Tucker, C.L. Droplet deformation in dispersions with unequal viscosities and zero interfacial tension. J. Fluid Mech. 2001, 426, 199–228. [Google Scholar] [CrossRef]
- Avazmohammadi, R.; Ponte Castaneda, P. The rheology of non-dilute dispersions of highly deformable viscoelastic particles in Newtonian fluids. J. Fluid Mech. 2015, 763, 386–432. [Google Scholar] [CrossRef]
- Villone, M.M.; Hulsen, M.A.; Anderson, P.D.; Maffettone, P.L. Simulations of deformable systems in fluids under shear flow using an arbitrary Lagrangian Eulerian technique. Comput. Fluids 2014, 90, 88–100. [Google Scholar] [CrossRef]
- Schowalter, W.R.; Chaffey, C.E.; Brenner, H. Rheological behavior of a dilute emulsion. J. Colloid Interface Sci. 1968, 26, 152–160. [Google Scholar] [CrossRef]
- Frankel, N.A.; Acrivos, A. The constitutive equation for a dilute emulsion. J. Fluid Mech. 1970, 44, 65–78. [Google Scholar] [CrossRef]
- Barthes-Biesel, D.; Acrivos, A. The rheology of suspensions and its relation to phenomenological theories for non-Newtonian fluids. Int. J. Multiph. Flow 1973, 1, 1–24. [Google Scholar] [CrossRef]
- Choi, S.J.; Schowalter, W.R. Rheological properties of nondilute suspensions of deformable particles. Phys. Fluids 1975, 18, 420–427. [Google Scholar] [CrossRef]
- Taylor, G.I. The viscosity of a fluid containing small drops of another fluid. Proc. Roy. Soc. Lond. A 1934, 146, 501–523. [Google Scholar] [CrossRef]
- Gabriele, M.; Pasquino, R.; Grizzuti, N. Effects of viscosity-controlled interfacial mobility on the coalescence of immiscible polymer blends. Macromol. Mater. Eng. 2011, 296, 263–269. [Google Scholar] [CrossRef]
- Hur, B.K.; Kim, C.B.; Lee, C.G. Functional relationship between volume concentration of dispersed phase and relative viscosity in a liquid-liquid dispersion and emulsion. J. Ind. Eng. Chem. 2000, 6, 318–324. [Google Scholar]
- Blawzdziewicz, J.; Vlahovska, P.; Loewenberg, M. Rheology of a dilute emulsion of surfactant-covered spherical drops. Physica A 2000, 276, 50–85. [Google Scholar] [CrossRef]
- Vlahovska, P.; Blawzdziewicz, J.; Loewenberg, M. Nonlinear rheology of a dilute emulsion of surfactant-covered spherical drops in time-dependent flows. J. Fluid Mech. 2002, 463, 1–24. [Google Scholar] [CrossRef]
- Vlahovska, P.; Blawzdziewicz, J.; Loewenberg, M. Small-deformation theory for a surfactant-covered drop in linear flows. J. Fluid Mech. 2009, 624, 293–337. [Google Scholar] [CrossRef]
- Danov, K.D. On the viscosity of dilute emulsions. J. Colloid Interface Sci. 2001, 235, 144–149. [Google Scholar] [CrossRef] [PubMed]
- Edwards, D.A.; Brenner, H.; Wasan, D.T. Interfacial Transport Processes and Rheology; Butterworth-Heinemann: Boston, MA, USA, 1991. [Google Scholar]
- Pal, R. Rheological behavior of bubble-bearing magmas. Earth Planet. Sci. Lett. 2003, 207, 165–179. [Google Scholar] [CrossRef]
- Pal, R. Rheological constitutive equation for bubbly suspensions. Ind. Eng. Chem. Res. 2004, 43, 5372–5379. [Google Scholar] [CrossRef]
- Barthes-Biesel, D. Motion of a spherical microcapsule freely suspended in a linear shear flow. J. Fluid Mech. 1980, 100, 831–853. [Google Scholar] [CrossRef]
- Barthes-Biesel, D.; Chhim, V. The constitutive equation of a dilute suspension of spherical microcapsules. Int. J. Multiph. Flow 1981, 7, 493–505. [Google Scholar] [CrossRef]
- Barthes-Biesel, D.; Rallison, J.M. The time dependent deformation of a capsule freely suspended in a linear shear flow. J. Fluid Mech. 1981, 113, 251–267. [Google Scholar] [CrossRef]
- Bredimas, M.; Veyssie, M.; Barthes-Biesel, D.; Chhim, V. Model suspensions of spherical capsules: Physical and rheological properties. J. Colloid Interface Sci. 1983, 93, 513–520. [Google Scholar] [CrossRef]
- Barthes-Biesel, D.; Sgaier, H. Role of membrane viscosity in the orientation and deformation of a capsule suspended in shear flow. J. Fluid Mech. 1985, 160, 119–135. [Google Scholar] [CrossRef]
- Drochon, A.; Barthes-Biesel, D.; Lacombe, C.; Lelievre, J.C. Determination of the red blood cell apparent membrane elastic modulus from viscometric measurements. J. Biomech. Eng. Trans. ASME 1990, 112, 241–249. [Google Scholar] [CrossRef]
- Barthes-Biesel, D. Role of interfacial properties on the motion and deformation of capsules in shear flow. Physica A 1991, 172, 103–124. [Google Scholar] [CrossRef]
- Pieper, G.; Rehage, H.; Barthes-Biesel, D. Deformation of a capsule in a spinning drop apparatus. J. Colloid Interface Sci. 1998, 202, 293–300. [Google Scholar] [CrossRef]
- Barthes-Biesel, D. Mechanics of encapsulated droplets. Prog. Colloid Polym. Sci. 1998, 111, 58–64. [Google Scholar]
- Diaz, A.; Pelekasis, N.; Barthes-Biesel, D. Transient response of a capsule subjected to varying flow conditions: Effect of internal fluid viscosity and membrane elasticity. Phys. Fluids 2000, 12, 948–957. [Google Scholar] [CrossRef]
- Diaz, A.; Barthes-Biesel, D.; Pelekasis, N. Effect of membrane viscosity on the dynamic response of an axisymmetric capsule. Phys. Fluids 2001, 13, 3835–3838. [Google Scholar] [CrossRef]
- Barthes-Biesel, D.; Diaz, A.; Dhenin, E. Effect of constitutive laws for two-dimensional membranes on flow-induced capsule deformation. J. Fluid Mech. 2002, 460, 211–222. [Google Scholar] [CrossRef]
- Drochon, A. Rheology of dilute suspensions of red blood cells: Experimental and theoretical approaches. Eur. Phys. J. Appl. Phys. 2003, 22, 155–162. [Google Scholar] [CrossRef]
- Lac, E.; Barthes-Biesel, D.; Pelekasis, N.A.; Tsamopoulos, J. Spherical capsules in three-dimensional unbounded Stokes flows: Effect of the membrane constitutive law and onset of buckling. J. Fluid Mech. 2004, 516, 303–334. [Google Scholar] [CrossRef]
- Lac, E.; Barthes-Biesel, D. Deformation of a capsule in simple shear flow: Effect of membrane pre-stress. Phys. Fluids 2005, 17, 2177–2195. [Google Scholar] [CrossRef]
- Barthes-Biesel, D. Modeling the motion of capsules in flow. Curr. Opin. Colloid Interface Sci. 2011, 16, 3–12. [Google Scholar] [CrossRef]
- Foessel, E.; Walter, J.; Salsac, A.V.; Barthes-Biesel, D. Influence of internal viscosity on deformation and buckling of a spherical capsule in a simple shear flow. J. Fluid Mech. 2011, 672, 318–347. [Google Scholar] [CrossRef]
- Walter, J.; Salsac, A.V.; Barthes-Biesel, D. Ellipsoidal capsules in simple shear flow: Prolate versus oblate initial shapes. J. Fluid Mech. 2011, 676, 477–486. [Google Scholar] [CrossRef]
- Omori, T.; Ishikawa, T.; Barthes-Biesel, D.; Salsac, A.V.; Imai, Y.; Yamaguchi, T. Tension of red blood cell membrane in simple shear flow. Phys. Rev. E 2012, 86, 056321. [Google Scholar] [CrossRef] [PubMed]
- Dupont, C.; Salsac, A.V.; Barthes-Biesel, D. Off-plane motion of a prolate capsule in shear flow. J. Fluid Mech. 2013, 721, 180–198. [Google Scholar] [CrossRef]
- Dupont, C.; Salsac, A.V.; Barthes-Biesel, D.; Vidrascu, M.; Le Tallec, P. Influence of bending resistance on the dynamics of a spherical capsule in shear flow. Phys. Fluids 2015, 27, 051902. [Google Scholar] [CrossRef]
- Ohshima, H. Primary electroviscous effect in a dilute suspension of soft particles. Langmuir 2008, 24, 6453–6461. [Google Scholar] [CrossRef] [PubMed]
- Davis, A.M.J.; Brenner, H. Emulsions containing a third solid internal phase. J. Eng. Mech. 1981, 107, 609–621. [Google Scholar]
- Stone, H.A.; Leal, L.G. Breakup of concentric double emulsion droplets in linear flows. J. Fluid Mech. 1990, 211, 123–156. [Google Scholar] [CrossRef]
- Jeffery, G.B. The motion of ellipsoidal particles immersed in a viscous fluid. Proc. R. Soc. A 1922, 102, 161–179. [Google Scholar] [CrossRef]
- Brenner, H. Rheology of a dilute suspension of axisymmetric Brownian particles. Int. J. Multiph. Flow 1974, 1, 195–341. [Google Scholar] [CrossRef]
- Leal, L.G.; Hinch, E.J. The effect of weak Brownian rotations on particles in shear flow. J. Fluid Mech. 1971, 46, 685–703. [Google Scholar] [CrossRef]
- Hinch, E.J.; Leal, L.G. The effect of Brownian motion on the rheological properties of a suspension of non-spherical particles. J. Fluid Mech. 1972, 52, 683–712. [Google Scholar] [CrossRef]
- Leal, L.G.; Hinch, E.J. The rheology of a suspension of nearly spherical particles subject to Brownian rotations. J. Fluid Mech. 1972, 55, 745–765. [Google Scholar] [CrossRef]
- Hinch, E.J.; Leal, L.G. Time-dependent shear flows of a suspension of particles with weak Brownian rotations. J. Fluid Mech. 1973, 57, 753–767. [Google Scholar] [CrossRef]
- Leal, L.G.; Hinch, E.J. Theoretical studies of a suspension of rigid particles affected by Brownian couples. Rheol. Acta 1973, 12, 127–132. [Google Scholar] [CrossRef]
- Hinch, E.J.; Leal, L.G. Constitutive equations in suspension mechanics. Part 2. Approximate forms for a suspension of rigid particles affected by Brownian rotations. J. Fluid Mech. 1976, 76, 187–208. [Google Scholar] [CrossRef]
- Batchelor, G.K. The stress generated in a non-dilute suspension of elongated particles by pure straining motion. J. Fluid Mech. 1971, 46, 813–829. [Google Scholar] [CrossRef]
- Brenner, H. Dynamics of neutrally buoyant particles in low Reynolds number flows. Prog. Heat Mass Transf. 1972, 6, 509–574. [Google Scholar]
- Petrie, C.J.S. The rheology of fibre suspensions. J. Non-Newton. Fluid Mech. 1999, 87, 369–402. [Google Scholar] [CrossRef]
- Jezek, J.; Saic, S.; Segeth, K. Numerical modeling of the movement of a rigid particle in viscous fluid. Appl. Maths 1999, 44, 469–479. [Google Scholar] [CrossRef]
- Yamamoto, S.; Matsuaka, T. Viscosity of dilute suspensions of rodlike particles: A numerical simulation method. J. Chem. Phys. 1994, 100, 3317–3324. [Google Scholar] [CrossRef]
- Barbosa, S.E.; Bibbo, M.A. Fiber motion and rheology of suspensions with uniform fiber orientation. J. Polym. Sci. Part B 2000, 38, 1788–1799. [Google Scholar] [CrossRef]
- Bibbo, M.A.; Dinh, S.M.; Armstrong, R.C. Shear flow properties of semi-concentrated fiber suspensions. J. Rheol. 1985, 29, 905–929. [Google Scholar] [CrossRef]
- Dinh, S.M.; Armstrong, R.C. A rheological equation of state for semi-concentrated fibre suspensions. J. Rheol. 1984, 28, 207–227. [Google Scholar] [CrossRef]
- Ivanov, Y.; Van de Ven, T.G.M.; Mason, S.G. Damped oscillations in the viscosity of suspensions of rigid rods. I. Monomodal suspensions. J. Rheol. 1982, 26, 213–230. [Google Scholar] [CrossRef]
- Ivanov, Y.; Van de Ven, T.G.M. Damped oscillations in the viscosity of suspensions of rigid rods. II. Bimodal and polydisperse suspensions. J. Rheol. 1982, 26, 231–244. [Google Scholar] [CrossRef]
- Anczurowski, E.; Mason, S.G. The kinetics of flowing dispersions. II. Equilibrium orientations of rods and discs (theoretical). J. Colloid Interface Sci. 1967, 23, 522–532. [Google Scholar] [CrossRef]
- Anczurowski, E.; Mason, S.G. The kinetics of flowing dispersions: III. Equilibrium orientations of rods and discs (experimental). J. Colloid Interface Sci. 1967, 23, 533–546. [Google Scholar] [CrossRef]
- Anczurowski, E.; Cox, R.G.; Mason, S.G. The kinetics of flowing dispersions. IV. Transient orientations of cylinders. J. Colloid Interface Sci. 1967, 23, 547–562. [Google Scholar] [CrossRef]
- Gauthier, F.; Goldsmith, H.L.; Mason, S.G. The kinetics of flowing dispersions. V. Orientation distributions of cylinders in Newtonian and non-Newtonian systems. Colloid Polym. Sci. 1971, 248, 1000–1015. [Google Scholar]
- Okagawa, A.; Cox, R.G.; Mason, S.G. The kinetics of flowing dispersions. VI. Transient orientation and rheological phenomena of rods and discs in shear flow. J. Colloid Interface Sci. 1973, 45, 303–329. [Google Scholar] [CrossRef]
- Okagawa, A.; Cox, R.G.; Mason, S.G. The kinetics of flowing dispersions. VII. Oscillatory behavior of rods and discs in shear flow. J. Colloid Interface Sci. 1973, 45, 330–358. [Google Scholar] [CrossRef]
- Scheraga, H.A. Non-Newtonian viscosity of solutions of ellipsoidal particles. J. Chem. Phys. 1955, 23, 1526–1532. [Google Scholar] [CrossRef]
- Leal, L.G. The slow motion of slender rod-like particles in a second-order fluid. J. Fluid Mech. 1975, 69, 305–337. [Google Scholar] [CrossRef]
- Zirnsak, M.A.; Hurr, D.U.; Boger, D.V. Normal stresses in fibre suspensions. J. Non-Newton. Fluid Mech. 1994, 54, 153–193. [Google Scholar] [CrossRef]
- Lipscombe, G.G.; Denn, M.M.; Hurr, D.U.; Boger, D.V. The flow of fiber suspensions in complex geometries. J. Non-Newton. Fluid Mech. 1988, 26, 297–325. [Google Scholar] [CrossRef]
- Manhart, M. Rheology of suspensions of rigid-rod like particles in turbulent channel flow. J. Non-Newton. Fluid Mech. 2003, 112, 269–293. [Google Scholar] [CrossRef]
- Altan, M.C.; Advani, S.G.; Guceri, S.I.; Pipes, R.S. On the description of the orientation state for fiber suspensions in homogeneous flows. J. Rheol. 1989, 33, 1129–1155. [Google Scholar] [CrossRef]
- Stover, C.A.; Koch, D.L.; Cohen, C. Observations of fiber orientation in simple shear flow of semi-dilute suspensions. J. Fluid Mech. 1992, 238, 277–296. [Google Scholar] [CrossRef]
- Shagfeh, E.S.G.; Fredrickson, G.H. The hydrodynamic stress in a suspension of rods. Phys. Fluids 1990, 2, 7–24. [Google Scholar] [CrossRef]
- Rahnama, M.; Koch, D.L.; Shagfeh, E.S.G. The effect of hydrodynamic interactions on the orientation distribution in a fiber suspension subject to simple shear flow. Phys. Fluids 1995, 7, 487–506. [Google Scholar] [CrossRef]
- Petrich, M.P.; Koch, D.L.; Cohen, C. An experimental determination of the stress-microstructure relationship in semi-concentrated fiber suspensions. J. Non-Newton. Fluid Mech. 2000, 95, 101–133. [Google Scholar] [CrossRef]
- Koch, D.L. A model for orientational diffusion in fiber suspensions. Phys. Fluids 1995, 7, 2086–2088. [Google Scholar] [CrossRef]
- Mongruel, A.; Cloitre, M. Shear viscosity of suspensions of aligned non-Brownian fibres. Rheol. Acta 1999, 38, 451–457. [Google Scholar] [CrossRef]
- Wierenga, A.M.; Philipse, A.P. Low-shear viscosity of isotropic dispersions of (Brownian) rods and fibres; a review of theory and experiments. Colloids Surfaces 1998, 137, 355–372. [Google Scholar] [CrossRef]
- Jeffrey, D.J.; Acrivos, A. The rheological properties of suspensions of rigid particles. AIChE J. 1976, 22, 417–432. [Google Scholar] [CrossRef]
- Eberle, A.P.R.; Baird, D.G.; Wapperom, P. Rheology of non-Newtonian fluids containing glass fibers: A review of experimental literature. Ind. Eng. Chem. Res. 2008, 47, 3470–3488. [Google Scholar] [CrossRef]
- Eberle, A.P.R.; Baird, D.G.; Wapperom, P.; Velez-Garcia, G.M. Using transient rheology to determine material parameters in fiber suspension theory. J. Rheol. 2009, 53, 685–705. [Google Scholar] [CrossRef]
- Ganani, E.; Powell, R.L. Suspensions of rodlike particles: Literature review and data correlations. J. Compos. Mater. 1985, 19, 194–215. [Google Scholar] [CrossRef]
- Derakhshandeh, B.; Kerekes, R.J.; Hatzikiriakos, S.G.; Bennington, C.P.J. Rheology of pulp fibre suspensions: A critical review. Chem. Eng. Sci. 2011, 66, 3460–3470. [Google Scholar] [CrossRef]
- Iso, Y.; Koch, D.L.; Cohen, C. Orientation in simple shear flow of semi-dilute fiber suspensions. 1. Weakly elastic fluids. J. Non-Newton. Fluid Mech. 1996, 62, 115–134. [Google Scholar] [CrossRef]
- Chinesta, F.; Ausias, G. Rheology of Non-Spherical Particle Suspensions; ISTE Press Ltd: London, UK, 2015. [Google Scholar]
- Phan-Thien, N.; Graham, A.L. A new constitutive model for fiber suspensions. Rheol. Acta 1991, 30, 44–57. [Google Scholar] [CrossRef]
- Powell, R.L. Rheology of suspensions of rodlike particles. J. Stat. Phys. 1990, 62, 1073–1091. [Google Scholar] [CrossRef]
- Ramazani, A.A.; Ait-Kadi, A.; Grmela, M. Rheology of fiber suspensions in viscoelastic media: Experiments and model predictions. J. Rheol. 2001, 945–962. [Google Scholar] [CrossRef]
- Sephr, M.; Ausias, G.; Carreau, P.J. Rheological properties of short fiber filled polypropylene in transient shear flow. J. Non-Newton. Fluid Mech. 2004, 123, 19–32. [Google Scholar] [CrossRef]
- Sephr, M.; Carreau, P.J.; Grmela, M.; Ausias, G.; Lafleur, P.G. Comparison of rheological properties of fiber suspensions with model predictions. J. Polym. Eng. 2004, 24, 579–610. [Google Scholar]
- Sephr, M.; Carreau, P.J.; Moan, M.; Ausias, G. Rheological properties of short fiber model suspensions. J. Rheol. 2004, 48, 1023–1048. [Google Scholar] [CrossRef]
- Rao, B.N.; Tang, L.; Altan, M.C. Rheological properties of non-Brownian spheroidal particle suspensions. J. Rheol. 1994, 38, 1335–1351. [Google Scholar] [CrossRef]
- Chen, B.; Koch, D.L. Rheology of dilute suspensions of charged fibers. Phys. Fluids 1996, 8, 2792–2807. [Google Scholar] [CrossRef]
- Odenbach, S. Ferrofluids—magnetically controlled suspensions. Colloids Surfaces A. 2003, 217, 171–178. [Google Scholar] [CrossRef]
- McTague, J.P. Magnetoviscosity of magnetic colloids. J. Chem. Phys. 1969, 51, 133–136. [Google Scholar] [CrossRef]
- Hall, W.F.; Busenberg, S.N. Viscosity of magnetic suspensions. J. Chem. Phys. 1969, 51, 137–144. [Google Scholar] [CrossRef]
- Brenner, H. Rheology of a dilute suspension of dipolar spherical particles in an external field. J. Colloid Int. Sci. 1970, 32, 141–158. [Google Scholar] [CrossRef]
- Brenner, H.; Weissman, M.H. Rheology of a dilute suspension of dipolar spherical particles in an external field: II. Effects of rotary Brownian motion. J. Colloid Int. Sci. 1972, 41, 499–531. [Google Scholar] [CrossRef]
- Hinch, E.J.; Leal, L.G. Note on the rheology of dilute suspension of dipolar spheres with weak Brownian couples. J. Fluid Mech. 1972, 56, 803–813. [Google Scholar] [CrossRef]
- Rosenzweig, R.E. Ferrohydrodynamics; Cambridge University Press: Cambridge, UK, 1985. [Google Scholar]
- Strand, S.R.; Kim, S. Dynamics and rheology of a dilute suspension of dipolar nonspherical particles in an external field: Part 1. Steady shear flows. Rheol. Acta 1992, 31, 94–117. [Google Scholar] [CrossRef]
- Raj, K.; Moskowitz, B.; Casciari, R. Advances in ferrofluid technology. J. Magn. Magn. Mater. 1995, 149, 174–180. [Google Scholar] [CrossRef]
- Almog, Y.; Frankel, I. The motion of axisymmetric dipolar particles in homogeneous shear flow. J. Fluid Mech. 1995, 289, 243–261. [Google Scholar] [CrossRef]
- Almog, Y.; Frankel, I. Rheology of dilute suspensions of Brownian dipolar axisymmetric particles. J. Fluid Mech. 1998, 366, 289–310. [Google Scholar] [CrossRef]
- Odenbach, S.; Stork, H. Shear dependence of filed-induced contributions to the viscosity of magnetic fluids at low shear rates. J. Magn. Magn. Mater. 1998, 183, 188–194. [Google Scholar] [CrossRef]
- Puyesky, I.; Frankel, I. The motion of a dipolar spherical particle in homogeneous shear and time-periodic fields. J. Fluid Mech. 1998, 369, 191–216. [Google Scholar]
- Odenbach, S.; Rylewicz, T.; Heyen, M. A rheometer dedicated for the investigation of viscoelastic effects in commercial magnetic fluids. J. Magn. Magn. Mater. 1999, 201, 155–158. [Google Scholar] [CrossRef]
- Odenbach, S. Magnetoviscous and viscoelastic effects in ferrofluids. Int. J. Mod. Phys. B. 2000, 14, 1615–1631. [Google Scholar] [CrossRef]
- Odenbach, S.; Raj, K. The influence of large particles and agglomerates on the magnetoviscous effect in ferrofluids. Magnetohydrodynamics 2000, 36, 312–319. [Google Scholar] [CrossRef]
- Felderhof, B.U. Steady state magnetoviscosity of a dilute ferrofluid. Magnetohydrodynamics 2000, 36, 329–334. [Google Scholar] [CrossRef]
- Zubarev, A.Y.; Iskakova, L.Y. On the theory of the dynamic properties of dense magnetic fluids: Polydisperse media. Colloid J. 2001, 63, 706–713. [Google Scholar] [CrossRef]
- Odenbach, S. Magnetic fluids. Adv. Colloid Interface Sci. 1993, 46, 263–282. [Google Scholar] [CrossRef]
- Odenbach, S. Investigation of the Weissenberg effect in suspensions of magnetic nanoparticles. Phys. Fluids 1999, 11, 2901–2905. [Google Scholar] [CrossRef]
- Odenbach, S. Ferrofluids: Magnetically controllable liquids. Proc. Appl. Math. Mech. 2002, 1, 28–32. [Google Scholar]
- Zubarev, A.Y.; Odenbach, S.; Fleischer, J. Rheological properties of dense ferrofluids. Effect of chain-like aggregates. J. Magn. Magn. Mater. 2002, 252, 241–243. [Google Scholar] [CrossRef]
- Melzner, K.; Odenbach, S. Investigation of the Weissenberg effect in ferrofluids under microgravity conditions. J. Magn. Magn. Mater. 2002, 252, 250–252. [Google Scholar] [CrossRef]
- Patel, R.; Upadhyay, R.V.; Mehta, R.V. Viscosity measurements of a ferrofluid: Comparison with various hydrodynamic equations. J. Colloid Int. Sci. 2003, 263, 661–664. [Google Scholar] [CrossRef]
- Charles, S.W.; Popplewell, J. Properties and application of magnetic liquids. Endeavour 1982, 6, 153–161. [Google Scholar] [CrossRef]
- Jansons, K.M. Determination of the constitutive equations for a magnetic fluid. J. Fluid Mech. 1983, 137, 187–216. [Google Scholar] [CrossRef]
- Kim, S.; Lawrence, C.J. Similarity solutions for the orientation distribution function and rheological properties of suspensions of axisymmetric particles with external couples. J. Non-Newtonian Fluid Mech. 1987, 24, 297–310. [Google Scholar] [CrossRef]
- Brenner, H. Particles in low Reynolds number flows. Progr. Heat Mass Transf. 1972, 6, 509–574. [Google Scholar]
- Karis, T.E.; Jhon, M.S. Preface. Colloids Surfaces A 1993, 80, 1993. [Google Scholar] [CrossRef]
- Kwon, T.M.; Jhon, M.S.; Choi, H.J.; Karis, T.E. Rheomagnetic properties of mixed magnetic particle suspensions. Colloids Surfaces A 1993, 80, 39–46. [Google Scholar] [CrossRef]
- Kwon, T.M.; Frattini, P.L.; Sadani, L.N.; Jhon, M.S. Rheooptical study of magnetic particle orientation under external fields. Colloids Surfaces A 1993, 80, 47–61. [Google Scholar] [CrossRef]
- Kwon, T.M.; Jhon, M.S.; Choi, H.J. Rheological study of magnetic particle suspensions. Mat. Chem. Phys. 1997, 49, 225–228. [Google Scholar] [CrossRef]
- Kwon, T.M.; Jhon, M.S.; Choi, H.J. Viscosity of magnetic particle suspension. J. Mol. Liq. 1998, 75, 115–126. [Google Scholar] [CrossRef]
- Choi, H.J.; Kim, C.A.; Kwon, T.M.; Jhon, M.S. Viscosity of magnetic particle suspensions. J. Magn. Magn. Mater. 2000, 209, 228–230. [Google Scholar] [CrossRef]
- Choi, H.J.; Kwon, T.M.; Jhon, M.S. Effects of shear rate and particle concentration on rheological properties of magnetic particle suspensions. J. Mater. Sci. 2000, 35, 889–894. [Google Scholar] [CrossRef]
- Jhon, M.S.; Kwon, T.M.; Choi, H.J.; Karis, T.E. Microrheological study of magnetic particle suspensions. Ind. Eng. Chem. Res. 1996, 35, 3027–3031. [Google Scholar] [CrossRef]
- Leal, L.G. On the effect of particle couples on the motion of a dilute suspension of spheroids. J. Fluid Mech. 1971, 46, 395–416. [Google Scholar] [CrossRef]



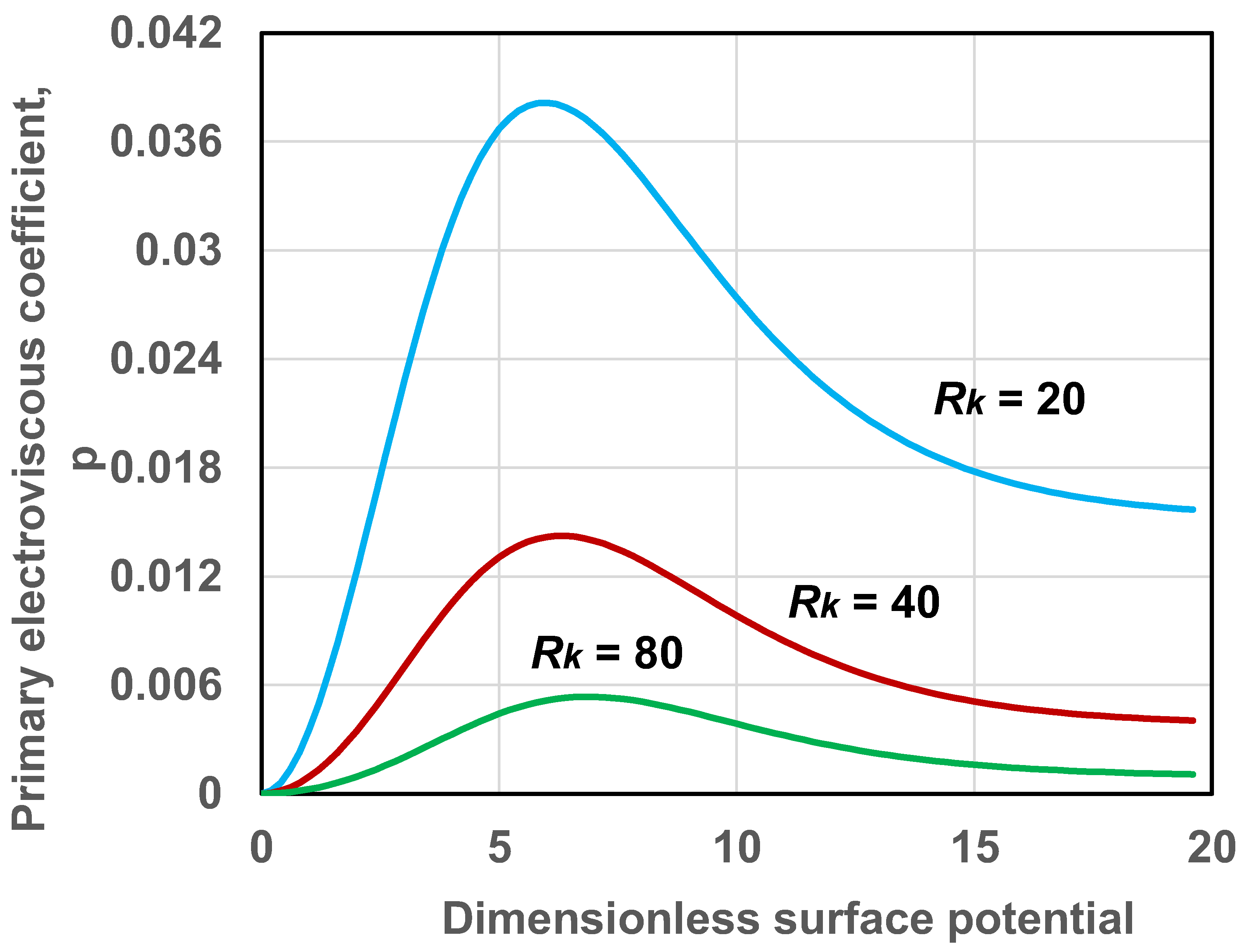
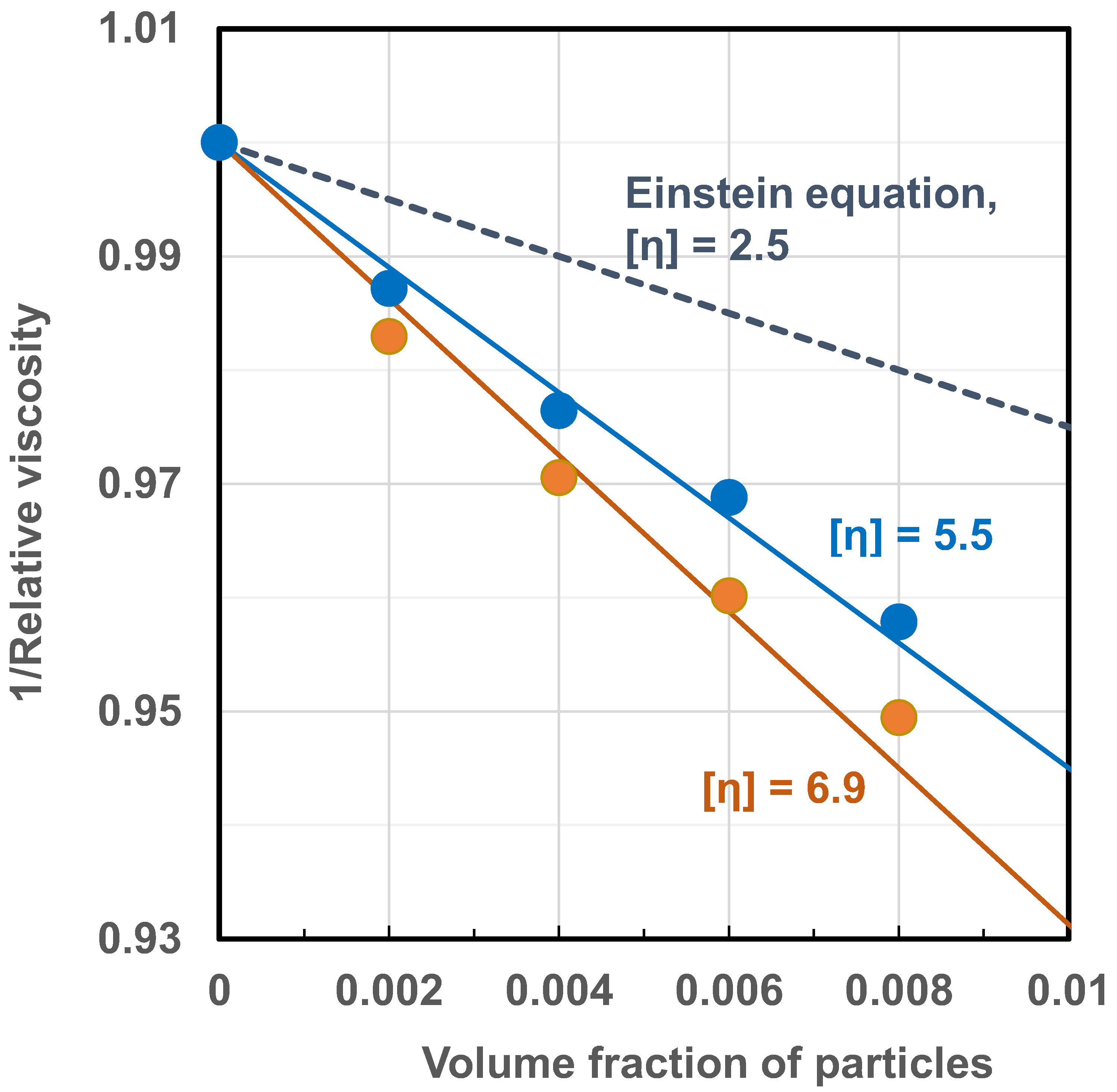
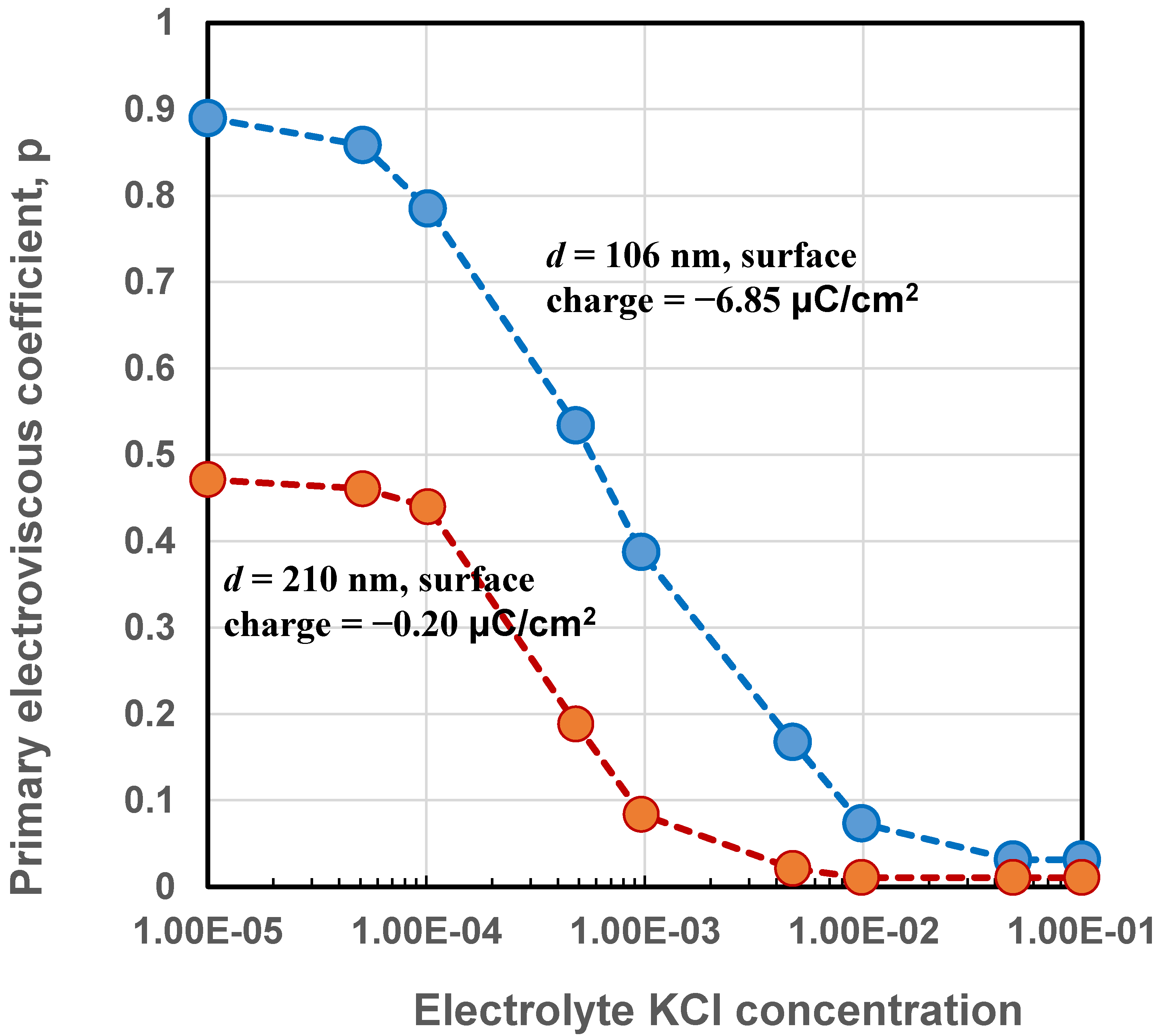
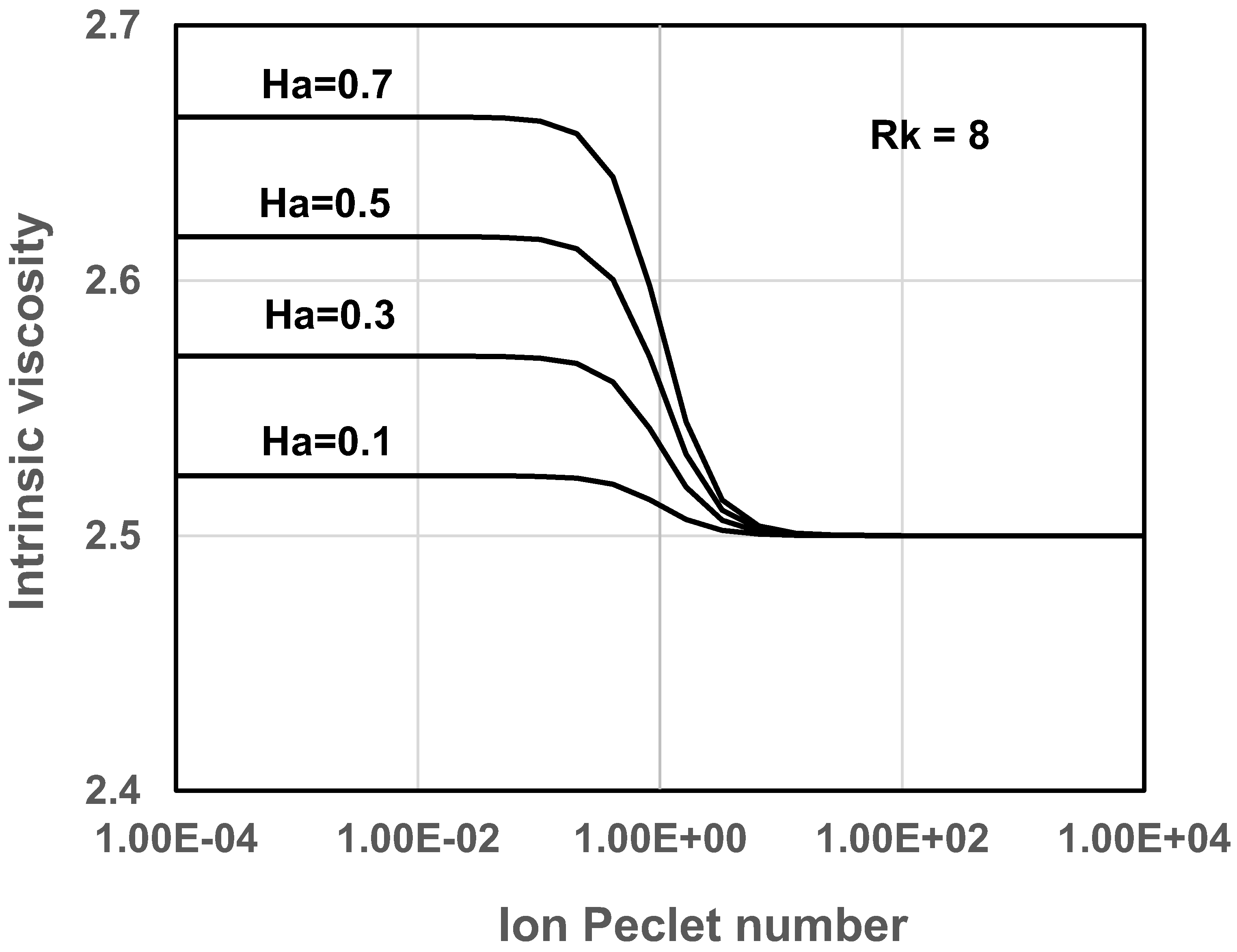
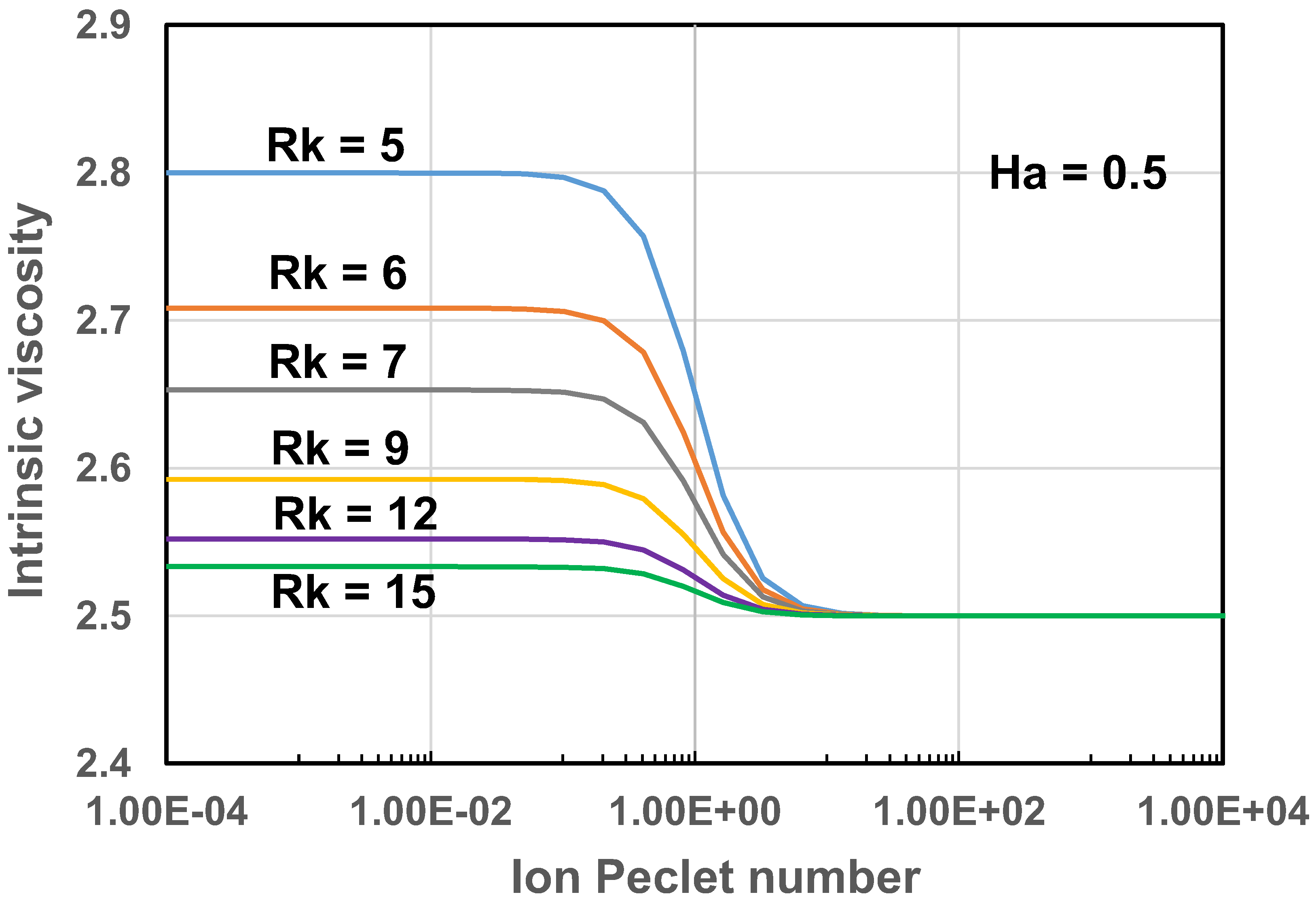
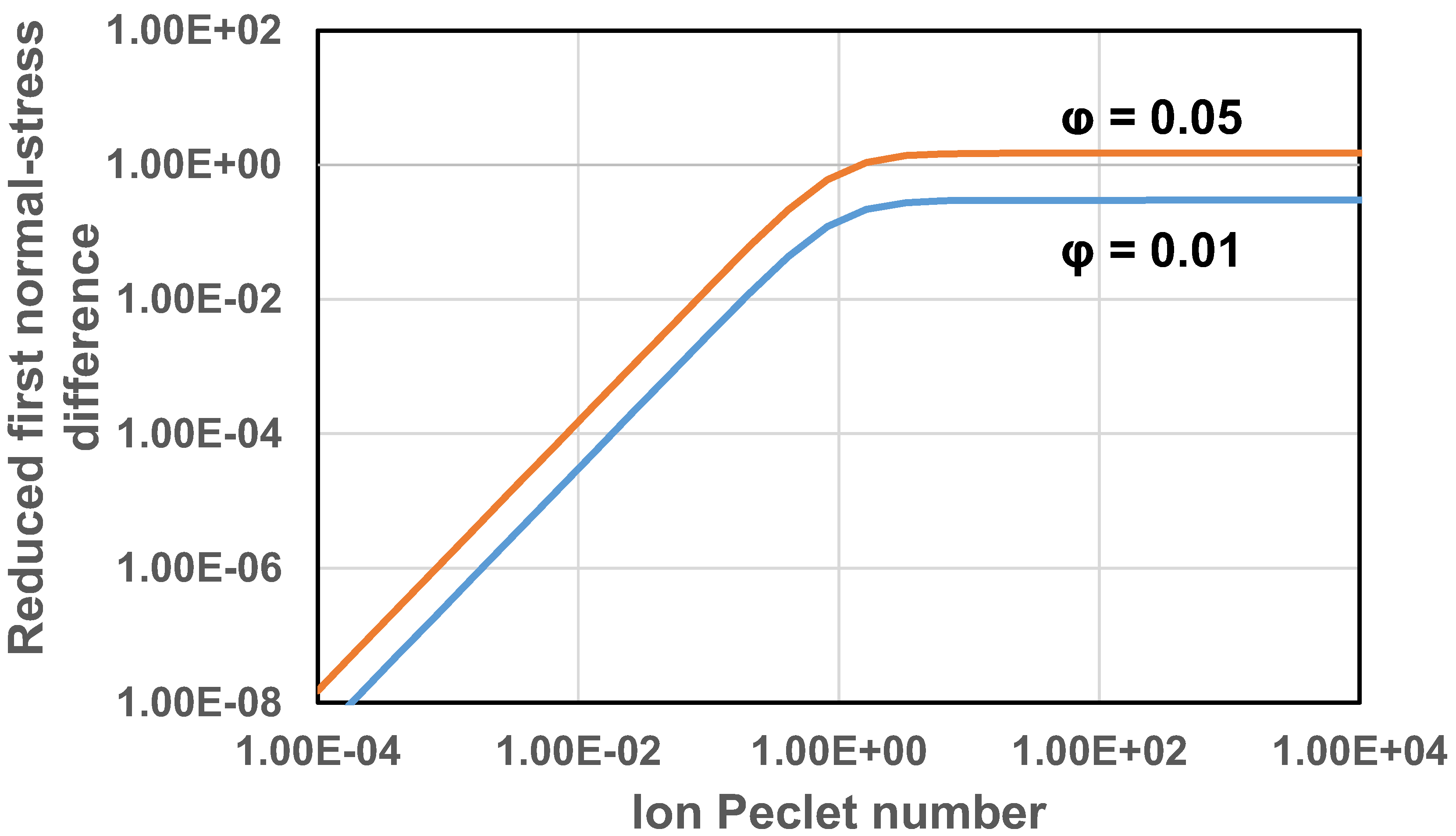
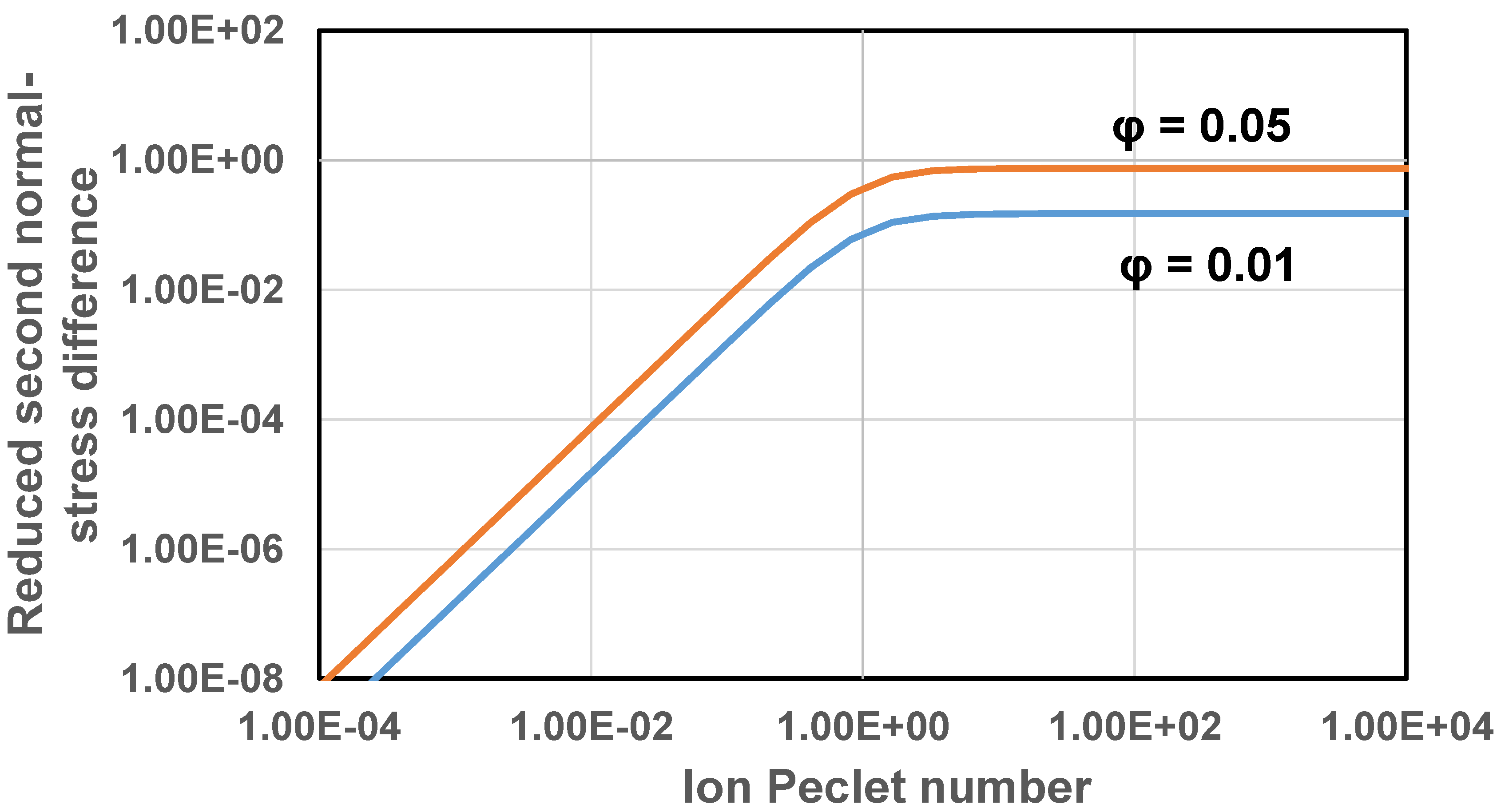
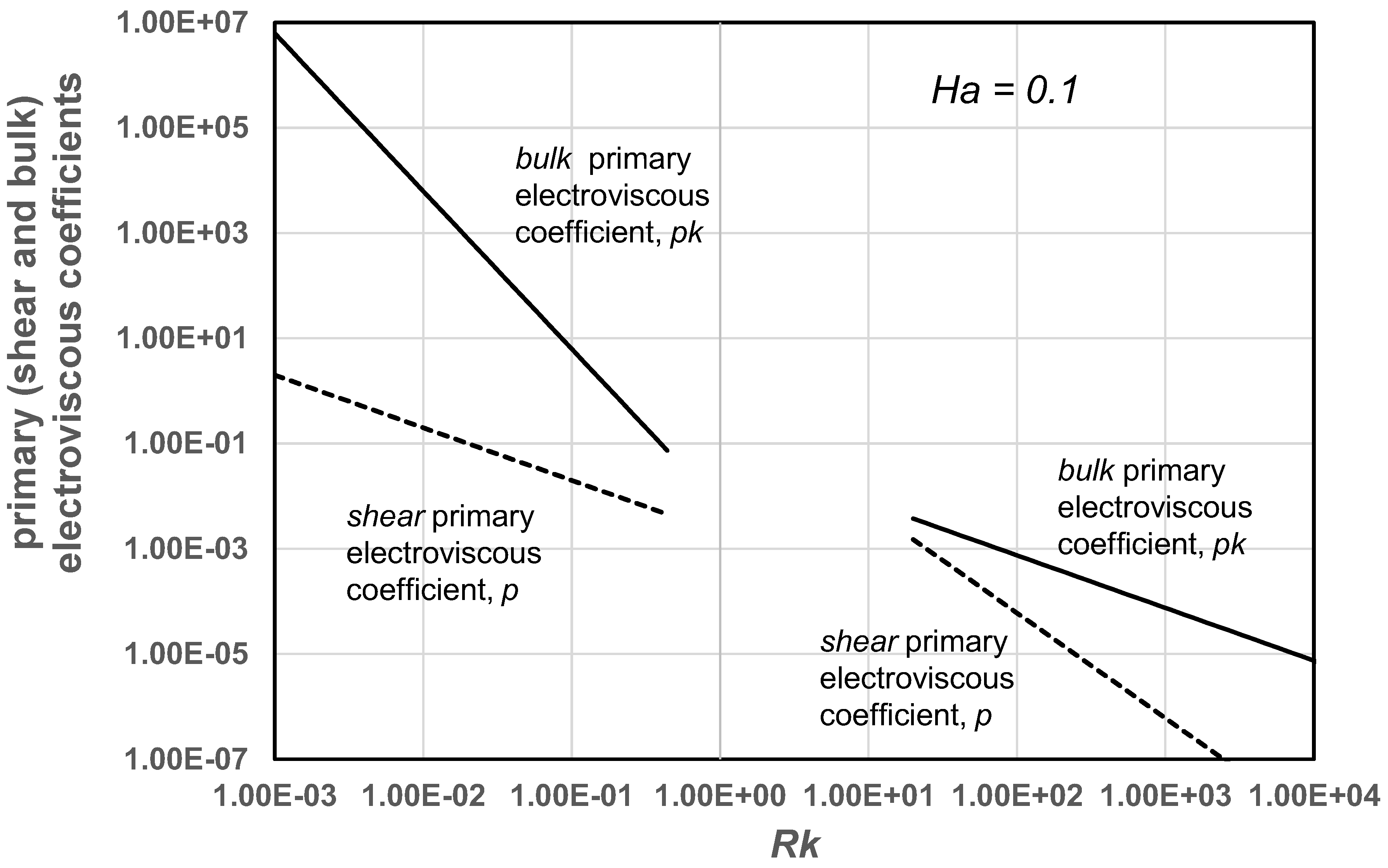




© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pal, R. Fundamental Rheology of Disperse Systems Based on Single-Particle Mechanics. Fluids 2016, 1, 40. https://doi.org/10.3390/fluids1040040
Pal R. Fundamental Rheology of Disperse Systems Based on Single-Particle Mechanics. Fluids. 2016; 1(4):40. https://doi.org/10.3390/fluids1040040
Chicago/Turabian StylePal, Rajinder. 2016. "Fundamental Rheology of Disperse Systems Based on Single-Particle Mechanics" Fluids 1, no. 4: 40. https://doi.org/10.3390/fluids1040040
APA StylePal, R. (2016). Fundamental Rheology of Disperse Systems Based on Single-Particle Mechanics. Fluids, 1(4), 40. https://doi.org/10.3390/fluids1040040




