Neutrality Versus Materiality: A Thermodynamic Theory of Neutral Surfaces
Abstract
:1. Introduction
2. A Critical Assessment of McDougall et al. (2014)
2.1. Objectives of This Section
2.2. Summary of McDougall et al. (2014)’s Arguments
- the definition of the buoyancy force acting on a single fluid parcel entering the dynamical interpretation of the neutral tangent plane Equation (1),
- the assumption that it is physically meaningful to parameterise isopycnal and diapycnal dispersion in terms of second-rank diffusion tensor as proposed by [29],
- the observation that lateral dispersion is about 7 orders of magnitude larger than quasi-vertical dispersion,
- the observed smallness of viscous dissipation in the ocean,
- the assumption that it is legitimate to regard the displacement entering the neutral tangent plane Equation (1) as an actual fluid parcel displacement.
- (i)
- involve no small-scale turbulent mixing, in which case the combined (two-step) process is adiabatic and isohaline and so is equivalent to epineutral dispersion (meaning along a neutral tangent plane), or
- (ii)
- the sinking and rising parcels would mix and entrain in a plumelike fashion with the ocean environment, and therefore experience irreversible mixing.
2.3. A Critical Discussion of McDougall et al. (2014)
2.3.1. Expanding on Point (i) of McDougall et al. (2014)
- First, a non-neutral stirring event associated with the displacement , which as it takes the parcel away from its equilibrium position, must entail a non-zero energy cost and some finite buoyancy force, implying ;
- second, a re-laminarisation process during which the fluid parcel seeks to find its closest level of neutral buoyancy, which is also associated with a displacement experiencing a nonzero buoyancy force and finite energy cost.
2.3.2. Are Neutral Trajectories Really Neutral?
2.3.3. Are Neutral Trajectories Really Adiabatic and Isohaline?
2.3.4. Do Neutral Rotated Diffusion Tensors Really Minimise Spurious Diapycnal Mixing?
3. Neutrality and the Energetics Cost of Adiabatic and Isohaline Stirring on Isopycnal Surfaces
3.1. Objectives of This Section
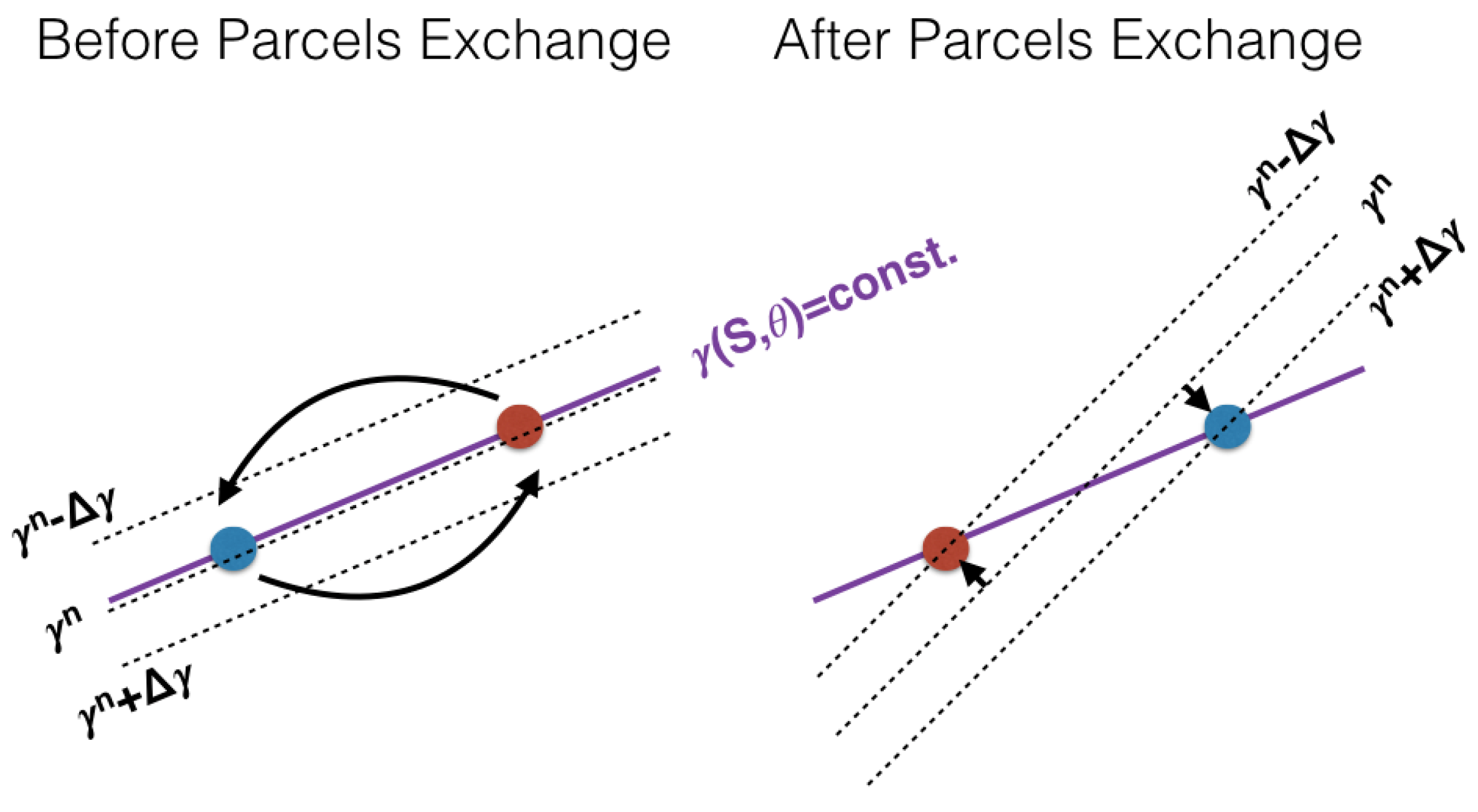
3.2. Link between the Energy Cost of Parcel Exchanges and Lateral Dispersion
3.3. Theory of the Energetics of Two-Parcel Exchanges on Material Isopycnal Surfaces
3.4. Energy-Based Definition of Global Neutral Surfaces
4. Neutrality and Energetics of Parcels Exchanges Using a Non-Material Density Variable
4.1. Objectives of This Section
4.2. Description of Adiabatic and Isohaline Stirring Using Orthobaric Density
5. A posteriori Thermodynamic Justification for Computing from the Minimisation of
5.1. Objectives of This Section
5.2. Density/Spiciness Representation of Water Masses
6. Summary and Conclusions
- Elementary but rigorous physical considerations clearly indicate that the physical processes for lateral dispersion in the ocean must in general have a non-zero buoyancy, which is confirmed by a survey of the literature on the topic. The concept of epineutral dispersion, therefore, only makes sense if viewed as the aggregate result of many individual non-neutral (i.e., having non-zero buoyancy) stirring events, so that it is only the net displacement aggregated over N individual stirring events that is approximately neutral and solution of the neutral tangent plane equation ;
- It is not true that neutral trajectories obtained as solutions of the neutral tangent plane Equation (1) can describe actual trajectories, contrary to what is usually assumed, because such trajectories implicitly require the existence of non-material sources of heat and salt. It is also not true that neutral tangent planes represent surfaces along which fluid parcels can be exchanged without experiencing (restoring or otherwise) buoyancy forces. Indeed, irreducible thermobaric forces always accompany adiabatic and isohaline parcels exchanges, and will force any pair of fluid parcels out of their original neutral tangent plane as soon as the parcel exchange takes place. If parcel exchanges on neutral tangent planes truly occurred without experiencing buoyancy forces, they would not experience thermobaric dianeutral dispersion;
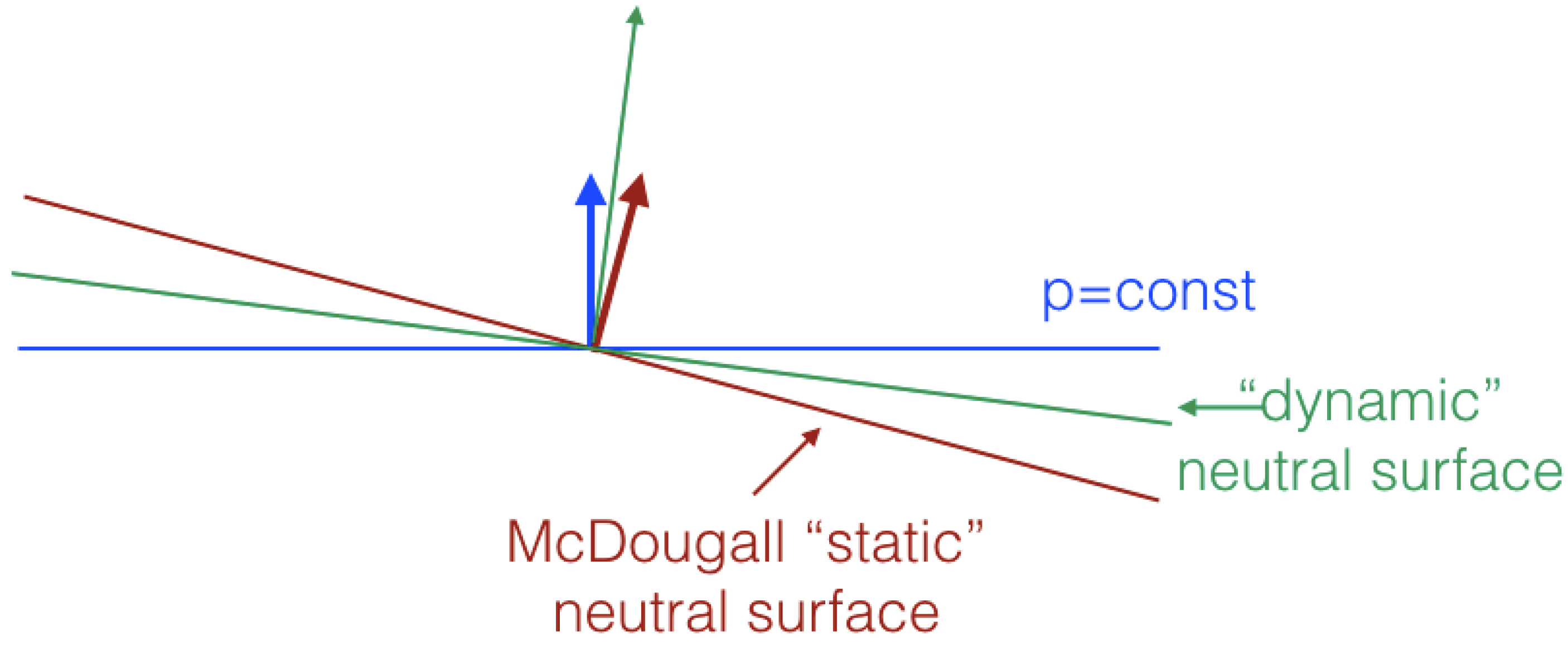
- The widespread idea that the neutral tangent plane Equation (1) can be justified in terms of momentum considerations appears to be invalid since the quantity cannot represent the full vertical force acting on a fluid parcel experiencing an adiabatic and isohaline lateral displacement owing to its lack of dependence on the horizontal pressure gradient. The new concept of ‘dynamic neutrality’ is introduced to describe lateral displacements satisfying to distinguish it from Mcdougall buoyancy-based ‘statistic neutrality’. In contrast to McDougall’s neutral displacements, ‘dynamic’ neutral displacements occur in the wedge of instability, have a non-zero buoyancy, a negative energy cost (they release available potential energy) and are necessarily transient;
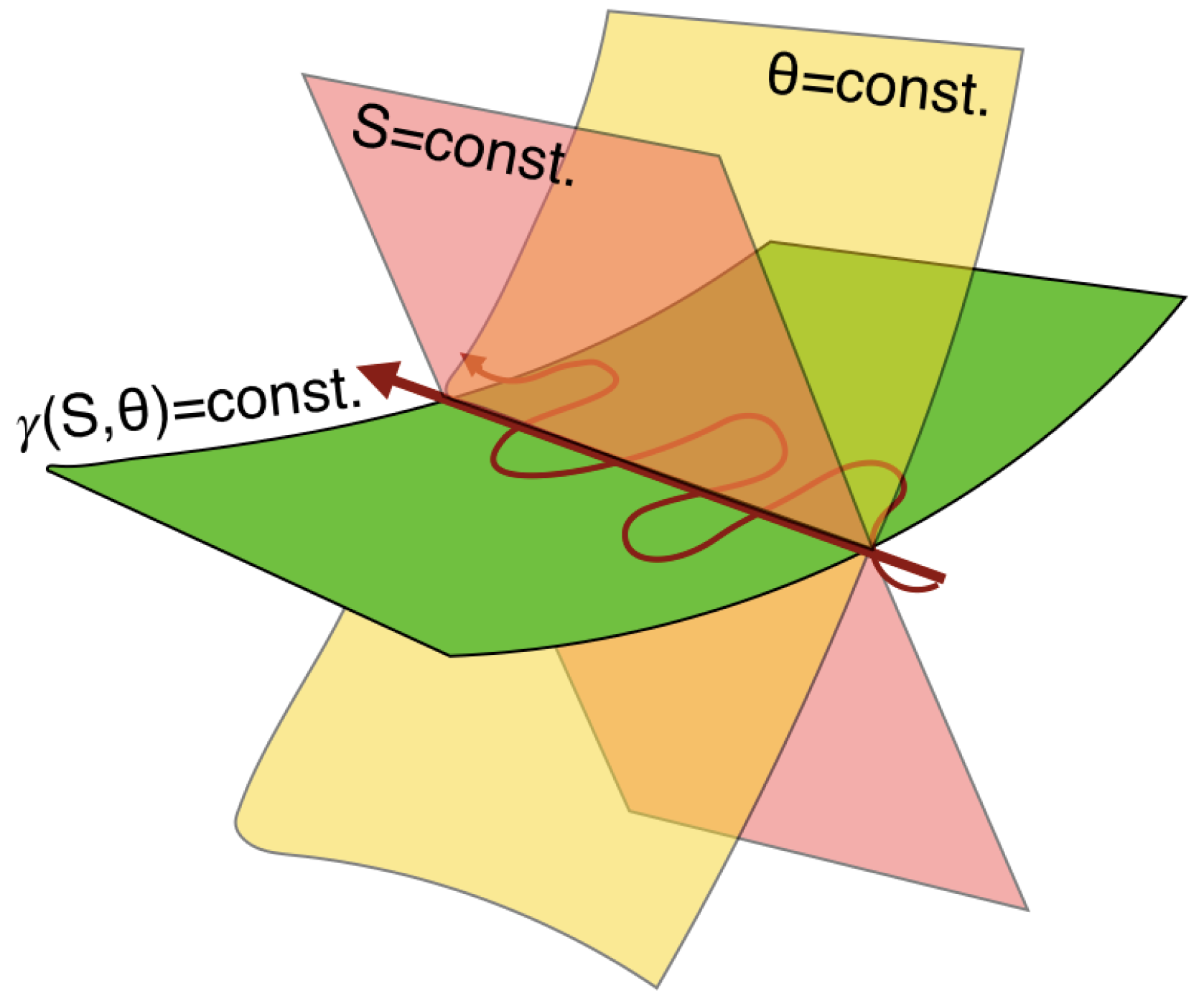
- Since the stirring events making up epineutral/isopycnal/lateral dispersion are usually individually non-neutral, it is argued that the neutral tangent plane Equation (1) can only be a valid model for epineutral dispersion if interpreted in an averaged sense; however, because traditional Eulerian averages give rise to eddy-correlation terms—absent from (1)—it is postulated that (1) can only be justified from first principles as a Lagrangian or quasi-Lagrangian average of the density Equation (5). This requires that the sought-for Lagrangian or quasi-Lagrangian density variable γ whose identification is the ultimate goal of the neutral density theory should be identified prior to the computation of the mean neutral vector appearing in (1); this suggests that γ can only be meaningfully constructed in thermodynamic space, and cast doubt on the possibility to provide a rigorous justification for density variables also varying geographically;
- We established, using both energetics and thermodynamics arguments, that the criterion measuring the degree of neutrality of a material density variable is the smallness of the absolute value of the Jacobian termPhysically, has both a dynamical and thermodynamic interpretations, as it controls the energy cost of parcel exchanges on material surfaces, as well as the dependence of in-situ density on spiciness. Minimising in thermodynamic space was shown to be equivalent to maximising the neutral character of γ in physical space.
- The present theory naturally explains why most material density variables fail to be uniformly neutral in the ocean, because they are such that in the following Taylor series expansion for :It is speculated that a material density variable constructed to be solution of might be uniformly satisfactorily neutral, a resarch topic left for further research.
- It was demonstrated that the neutral and non-neutral stirring events contributing to epineutral dispersion could be characterised in terms of their energy signature, and suggested that the events with negative energy cost —that is, releasing available potential energy—are associated with enhanced lateral dispersion.
- A new mechanism for enhanced lateral dispersion was identified whose source of energy stems from the coupling between thermobaricity and density-compensated temperature/salinity anomalies. Such a mechanism does not exist in a salt-less ocean, and is speculated to act as a physical process for the removal of density-compensated anomalies and neutral helicity in the ocean;
- It was established that the use of a neutral rotated diffusion tensor, as is the current practice in numerical ocean modelling, implies that the effective diapycnal diffusivity of all conceivable material density variables is potentially much larger than the value of dianeutral diffusivity used in such tensors, raising the issue of whether the use of such tensors avoids or causes spurious diapycnal diffusion;
- It was established that orthobaric density appears to significantly more neutral based on the present energy-based definition of neutrality than suggested by McDougall and Jackett’s [36] evaluation.
- They connect for the first time epineutral dispersion with the actual stirring events that cause it; moreover, the realisation that epineutral dispersion is actually made up of non-neutral stirring events resolves some longstanding apparent paradoxes and inconsistencies between “neutral thinking”, “turbulence thinking” and “baroclinic instability thinking” that have caused much confusion and controversy in the field;
- They clearly establish the relevance of energetics for categorising the different possible dispersion regimes in the ocean, with epineutral dispersion being associated with energy neutral and unstable processes, whereas diapycnal dispersion is associated with positive energy consumption;
- They dispel the widespread misconception, e.g., [1], that the buoyancy forces involved in parcel exchanges in potential density surfaces are necessarily restoring;
- They provide a potential unifying framework for discussing a number of widely disparate results all connected to the thermobaric instability identified here, such as the thermobaric instability sustaining solitary Rossby waves discussed by [50], the existence of a spiciness mode [46], the thermobaric production of potential vorticity [47], thermobaric numerical instabilities in isopycnic numerical ocean circulation models [51], the possibility for thermobaricity to cause a form of conditional instability akin to that at the origin of thunderstorms in the atmosphere [52], that [53] speculated might be responsible for past climate change.
- They provide for the first time concrete ways to test the validity of neutral diffusion tensors; for instance if we could establish on the basis of direct numerical simulations or observations that the diapycnal diffusivity of , , and Lorenz reference density was comparable to observed values of diapycnal mixing, it would unambiguously invalidate the idea that neutral rotated diffusion tensors are necessarily the best possible practice. Until now, to paraphrase Stommel [54], our ideas about neutral density and neutral rotated diffusion have had so far a peculiarly dreamlike quality, and it has been unclear whether any of the premises on which neutral density thinking relies are actually testable or falsifiable. The present results suggest that they might be.
Acknowledgments
Conflicts of Interest
Appendix A
Appendix B

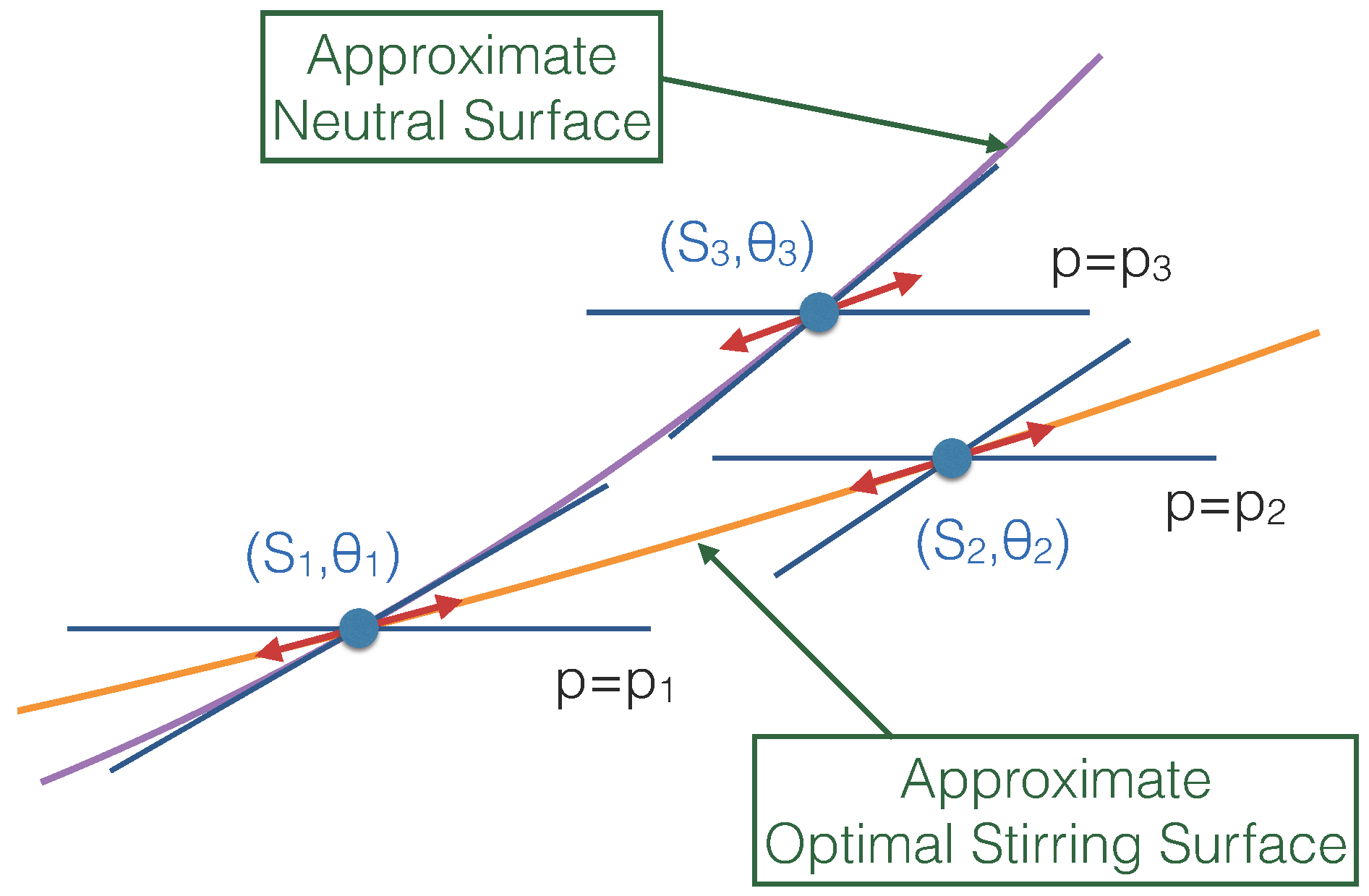
Appendix C
References
- McDougall, T.J. Neutral surfaces. J. Phys. Oceanogr. 1987, 17, 1950–1964. [Google Scholar] [CrossRef]
- Jackett, D.R.; McDougall, T.J. A neutral density variable for the world’s oceans. J. Phys. Oceanogr. 1997, 27, 237–263. [Google Scholar] [CrossRef]
- Pingree, R.D. Mixing in the deep stratified ocean. Deep Sea Res. 1972, 19, 549–561. [Google Scholar] [CrossRef]
- Ivers, W.D. The Deep Circulation in the Northern North Atlantic, with Especial Reference to the Labrador Sea. Ph.D. Thesis, Scripps Institute of Oceanography, University of California, San Diego, CA, USA, 1975. [Google Scholar]
- Montgomery, R.B. Circulation in the upper layers of the southern North Atlantic, deduced with the use of isentropic analysis. Pap. Phys. Oceanogr. Meteor 1938, 6, 55. [Google Scholar]
- McDougall, T.J.; Groeskamp, S.; Griffies, S.M. On geometrical aspects of interior ocean mixing. J. Phys. Oceanogr. 2014, 44, 2164–2175. [Google Scholar] [CrossRef]
- Thorpe, A.J.; Hoskins, B.J.; Innocenti, V. The parcel method in a baroclinic atmosphere. J. Atmos. Sci. 1989, 46, 1274–1284. [Google Scholar] [CrossRef]
- Tailleux, R. On the validity of single-parcel energetics to assess the importance of internal energy and compressibility effects in stratified fluids. J. Fluid Mech. 2015, 767, R2. [Google Scholar] [CrossRef]
- Zimmerman, J.T.F. The tidal whirlpool: A review of horizontal dispersion by tidal and residual currents. Neth. J. Sea Res. 1986, 20, 133–154. [Google Scholar] [CrossRef]
- Young, W.R.; Rhines, P.B.; Garrett, C.J.R. Shear-flow dispersion, internal waves and horizontal mixing in the ocean. J. Phys. Oceanogr. 1982, 12, 515–527. [Google Scholar] [CrossRef]
- Steinbuck, J.V.; Koseff, J.R.; Genin, A.; Stacey, M.T. Horizontal dispersion of ocean tracers in internal wave shear. J. Geophys. Res. 2011, 116. [Google Scholar] [CrossRef]
- Andrews, D.G.; McIntyre, M.E. An exact theory of nonlinear waves on a Lagrangian mean flow. J. Fluid Mech. 1978, 89, 609–646. [Google Scholar] [CrossRef]
- Andrews, D.G.; McIntyre, M.E. On wave action and its relatives. J. Fluid Mech. 1978, 89, 647–664. [Google Scholar] [CrossRef]
- Salmon, R. An alternative view of generalized Lagrangian mean theory. J. Fluid Mech. 2013, 719, 165–182. [Google Scholar] [CrossRef]
- De Szoeke, R.A.; Bennett, A.F. Microstructure fluxes across density surfaces. J. Phys. Oceanogr. 1993, 23, 2254–2264. [Google Scholar] [CrossRef]
- Young, W.R. An exact thickness-weighted average formulation of the Boussinesq equations. J. Phys. Oceanogr. 2012, 42, 692–707. [Google Scholar] [CrossRef]
- De Szoeke, R.A. Equations of motion using thermodynamic coordinates. J. Phys. Oceanogr. 2000, 30, 2184–2829. [Google Scholar] [CrossRef]
- Brankart, J.M. Impact of uncertainties in the horizontal density gradient upon low resolution global ocean modelling. Ocean Model. 2013, 66, 64–76. [Google Scholar] [CrossRef]
- De Szoeke, R.A.; Springer, S.R. Orthobaric density: A thermodynamic variable for ocean circulation studies. J. Phys. Oceanogr. 2000, 30, 2830–2852. [Google Scholar] [CrossRef]
- McDougall, T.J.; Jackett, D.R. The material derivative of neutral density. J. Mar. Res. 2005, 63, 159–185. [Google Scholar] [CrossRef]
- Eden, C.; Willebrand, J. Neutral density revisited. Deep Sea Res. Part II 1999, 46, 33–54. [Google Scholar] [CrossRef]
- Serazin, G. An Approximate Neutral Density Variable for the World’s Oceans. Master’s Thesis, Ecole Centrale Lyon, Écully, France, 2011. [Google Scholar]
- Tailleux, R. Generalised patched potential density and thermodynamic neutral density: Two new physically-based quasi-neutral density variables for ocean water masses analyses and circulation studies. J. Phys. Oceanogr. 2016, in press. [Google Scholar]
- Nycander, J. Energy conversion, mixing energy, and neutral surfaces with a nonlinear equation of state. J. Phys. Oceanogr. 2011, 41, 28–41. [Google Scholar] [CrossRef]
- Olbers, D.; Willebrand, J.; Eden, C. Ocean Dynamics; Springer: Berlin, Germany, 2012; p. 704. [Google Scholar]
- Vallis, G. Atmospheric and Oceanic Fluid Dynamics; Cambridge University Press: Cambridge, UK, 2006; p. 745. [Google Scholar]
- Haine, T.; Marshall, J. Gravitational, symmetric and baroclinic instability of the ocean mixed layer. J. Phys. Oceanogr. 1998, 28, 634–658. [Google Scholar] [CrossRef]
- Griffies, S.M. Fundamentals of Ocean Models; Princeton University Press: Princeton, NJ, USA, 2004; p. 424. [Google Scholar]
- Redi, M.H. Oceanic isopycnal mixing by coordinate rotation. J. Phys. Oceanogr. 1982, 12, 1154–1158. [Google Scholar] [CrossRef]
- Popper, K. The Logic of Scientific Discovery; Routledge: New York, NY, USA, 1959. [Google Scholar]
- Eady, E.T. Long waves and cyclone waves. Tellus 1949, 1, 33–52. [Google Scholar] [CrossRef]
- Heifetz, E.; Alpert, P.; da Silva, A. On the parcel method and the baroclinic wedge of instability. J. Atmos. Sci. 1998, 55, 788–795. [Google Scholar] [CrossRef]
- McDougall, T.J.; Church, J.A. Pitfalls with the numerical representation of isopycnal and diapycnal mixing. J. Phys. Oceanogr. 1986, 16, 196–199. [Google Scholar] [CrossRef]
- McDougall, T.J. The vertical motion of submesocale coherent vortices across neutral surfaces. J. Phys. Oceanogr. 1987, 17, 2334–2342. [Google Scholar] [CrossRef]
- Huang, R.X. Adiabatic density surface, neutral density surface, potential density surface, and mixing path. J. Trop. Oceanogr. 2014, 33, 1–19. [Google Scholar]
- McDougall, T.J.; Jackett, D.R. An assessment of orthobaric density in the global ocean. J. Phys. Oceanogr. 2005, 35, 2054–2075. [Google Scholar] [CrossRef]
- Klocker, A.; McDougall, T.J.; Jackett, D.R. A new method for forming approximately neutral surfaces. Ocean Sci. 2009, 5, 155–172. [Google Scholar] [CrossRef]
- Veronis, G. The role of models in tracer studies. In Numerical Models of Ocean Circulation; National Academy of Science: Washington, DC, USA, 2007; pp. 133–146. [Google Scholar]
- McDougall, T.J.; Jackett, D.R. The thinness of the ocean in S-Θ-p space and the implication for mean diapycnal advection. J. Phys. Oceanogr. 2007, 37, 1715–1732. [Google Scholar] [CrossRef]
- Saenz, J.A.; Tailleux, R.; Butler, E.D.; Hughes, G.O.; Oliver, K.I.C. Estimating Lorenz’s reference state in an ocean with a nonlinear equation of state for seawater. J. Phys. Oceanogr. 2015, 45, 1242–1257. [Google Scholar] [CrossRef]
- Lynn, R.J.; Reid, J.L. Characteristics and circulation of deep and abyssal waters. Deep Sea Res. 1968, 15, 577–598. [Google Scholar] [CrossRef]
- McDougall, T.J. Thermobaricity, cabelling, and water-mass conversion. J. Geophys. Res. 1987, 92, 5448–5464. [Google Scholar] [CrossRef]
- De Szoeke, R.A.; Springer, S.R. The materiality and neutrality of neutral density and orthobaric density. J. Phys. Oceanogr. 2009, 39, 1779–1799. [Google Scholar] [CrossRef]
- Pearson, H.J.; Puttock, J.S.; Hunt, J.C.R. A statistical model of fluid-element motions and vertical diffusion in a homogeneous stratified turbulent flow. J. Fluid Mech. 1983, 129, 219–249. [Google Scholar] [CrossRef]
- McDougall, T.J. The relative roles of diapycnal and isopycnal mixing on subsurface water mass convsersion. J. Phys. Oceanogr. 1984, 14, 1577–1589. [Google Scholar] [CrossRef]
- Muller, P.; Willebrand, J. Compressibility effects in the thermohaline circulation: A manifestation of the temperature-salinity mode. Deep Sea Res. 1986, 33, 559–571. [Google Scholar] [CrossRef]
- Straub, D.N. On thermobaric production of potential vorticity in the ocean. Tellus 1999, 51A, 314–325. [Google Scholar] [CrossRef]
- Tailleux, R.; Lazar, A.; Reason, C. Physics and dynamics of density-compensated temperature and salinity anomalies. Part I: Theory. J. Phys. Oceanogr. 2005, 35, 849–864. [Google Scholar] [CrossRef]
- Huang, R.X. Defining the spicity. J. Mar. Res. 2011, 69, 545–559. [Google Scholar] [CrossRef]
- De Szoeke, R.A. An effect of the thermobaric nonlinearity of the equation of state: A mechanism for sustaining solitary Rossby waves. J. Phys. Oceanogr. 2004, 34, 2042–2056. [Google Scholar] [CrossRef]
- Hallberg, R. A thermobaric instability of Lagrangian vertical coordinate ocean models. Ocean Model. 2004, 8, 279–300. [Google Scholar] [CrossRef]
- Ingersoll, A.P. Boussinesq and anelastic approximations revisited: Potential energy release during thermobaric instability. J. Phys. Oceanogr. 2005, 35, 1359–1369. [Google Scholar] [CrossRef]
- Adkins, J.F.; Ingersoll, A.P.; Pasquero, C. Rapid climate change and conditional instability of the glacial deep ocean from the thermobaric effect and geothermal heating. Quat. Sci. Rev. 2005, 24, 581–594. [Google Scholar] [CrossRef]
- Stommel, H.K. Why do our ideas about the ocean circulation have such a peculiarly dream-like quality? In Collected Works of Henry Stommel; Hogg, N.G., Huang, R.X., Eds.; American Meteorological Society: Boston, MA, USA, 1954; Volume 1, pp. 124–134. [Google Scholar]
- Tailleux, R. Available potential energy and exergy in stratified fluids. Ann. Rev. Fluid Mech. 2013, 45, 35–58. [Google Scholar] [CrossRef]


© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tailleux, R. Neutrality Versus Materiality: A Thermodynamic Theory of Neutral Surfaces. Fluids 2016, 1, 32. https://doi.org/10.3390/fluids1040032
Tailleux R. Neutrality Versus Materiality: A Thermodynamic Theory of Neutral Surfaces. Fluids. 2016; 1(4):32. https://doi.org/10.3390/fluids1040032
Chicago/Turabian StyleTailleux, Rémi. 2016. "Neutrality Versus Materiality: A Thermodynamic Theory of Neutral Surfaces" Fluids 1, no. 4: 32. https://doi.org/10.3390/fluids1040032
APA StyleTailleux, R. (2016). Neutrality Versus Materiality: A Thermodynamic Theory of Neutral Surfaces. Fluids, 1(4), 32. https://doi.org/10.3390/fluids1040032





