1. Introduction
Rectifying columns are widely used in industry to separate mixtures and make products for different purposes. The adiabatic units that are currently used have high energy consumption, which results in increased rectification costs. Rectifying columns have a height often exceeding 25 m. All this leads to high costs related to the manufacture, operation, and repair of rectifying columns.
The method used to organize refluxes in the adiabatic units does not provide optimal distribution of mixture components both on the trays and along the column height. The steam flow passes through the column and is condensed in the heat exchangers (reflux condenser). The resulting condensate enters the upper column trays with a maximum flow. This leads to several factors that reduce unit efficiency. In particular, with this reflux formation, the intensity of vapor condensation on the surface of heat exchanger pipes decreases due to the relatively low thermophysical parameters of the condensed vapors produced in the upper part of the adiabatic column (low phase transition criterion). This leads to an unjustified increase in the heat exchange surface, the dimensions of the heat exchange devices, high metal consumption, and increased consumption of heat-transfer media. Reuse of the heat-transfer medium passed through the reflux condenser requires cooling, which also results in additional energy consumption.
The heat and mass exchange processes (condensation and evaporation) on the trays along the column height are not synchronized between the mixture low-boiling and high-boiling components due to the difference in the physical properties of the draining maximum reflux flow and rising vapors. This suggests the practicability of creating a reflux on each tray, for example, by partial condensation of vapors rising along the column height.
Additionally, in the adiabatic column, we observe the supercooling of the reflux flow on the surface of multi-pass heat exchangers, despite the constant improvement of the reflux condenser design. It is obvious that a decrease in the heat-transfer medium flow rate triggers a laminar flow in the pipes, which is not permissible, and its increase leads to the pipe surface and condensate cooling. Use of different heat exchange intensifiers in multi-pass reflux condensers leads to an increase in dimensions and metal consumption. Reflux supercooling requires additional heating to the boiling point. The reflux not heated to the boiling point condenses volatile components (impurities) on the upper trays and entrains them to the bottom of the column. It is therefore not possible to completely separate and purify a distillate from impurities, which is currently in demand.
An unreasonably large flow of the reflux on the upper trays leads to an increase in the diameter of the concentrating section of the column to accommodate overall overflows on them and increase the number of contact devices.
The displacement of a large vapor flow along the column results in increased unit resistance and heat losses.
The use of conventional contact devices on trays such as fixed and movable valves or caps is justified only at low unit capacity. An increase in the liquid flow rate leads to a sharp decrease in the tray efficiency due to the flow of gas bubbles from the gas–liquid layer. Additionally, an increase in the steam load on the trays with such contact devices leads to liquid pulsations, drop entrainment, and flooding. Stagnant zones form on the trays, which leads to the formation of deposits on their surfaces.
With conventional trays, a large volume of liquid is typically placed to provide a certain height for the liquid layer, which keeps them from being used, for example, for processing explosive and thermolabile mixtures. This also keeps the unit from being quickly brought to its operating mode.
The above factors prove that new design solutions are required both in the creation of rectifying columns and in the choice of contact devices on the tray.
One method for intensifying the separation process is through managing the process in diabatic rectification and using vortex contact devices on trays.
Diabatic distillation is a successful example of a simple process intensification technology for improving separation and thermal efficiency. To convert an adiabatic column into a diabatic one, a reboiler and condenser are partially replaced with heat exchangers built into the column [
1,
2].
Replacing an adiabatic unit with a diabatic one allows reducing the temperature difference between the heater and the cooling liquid [
3]. This process uses cheaper heat-transfer fluids and coolants.
In turn in terms of their characteristics, centrifugal mass-exchange contact devices are most suitable for solving the problem of reducing equipment dimensions and increasing the productivity and efficiency of existing mass-exchange units. Centrifugal mass-exchange contact devices are able to provide up to 80% higher efficiency than traditional units [
4,
5].
The most advanced achievements in this area today are centrifugal trays such as Swirltube, ConSep Sulzer [
6,
7,
8,
9,
10], Ultra-Frac [
11,
12], CoFlo [
13,
14], and UOP SimulFlow [
15].
Different centrifugal tray designs are conventionally divided into vortex and film direct-flow vortex units. In film direct-flow vortex contact units (see
Figure 1), steam (gas) transports liquids as a film along the inner surface of pipes mounted on trays [
16,
17,
18].
They are notable for their high gas capacity. However, due to their high metal content, low liquid output, and the presence of stagnant zones on the tray deck, they are not widely used.
The designs of vortex trays with tangential swirlers in contact devices (
Figure 2, [
19,
20]) are easy to manufacture and provide high efficiency, but require structural refinement.
We conducted our first diabatic rectification studies for a column 0.1 m in diameter with 25 vortex trays (see
Figure 2a), which were fitted with dephlegmators in the form of coils [
21] (see
Figure 3a).
According to the average data obtained on the column, the tray efficiency in diabatic rectification was 0.8 and in adiabatic rectification 0.4. We obtained the dependency to determine the stage efficiency as follows [
21]
where
E is the Murphree efficiency;
m is the slope of the equilibrium curve of the mixture;
G is the vapor phase flow rate, kg/s;
L is the liquid phase flow rate, kg/s;
H is the liquid level at the stage, m;
h is the swirler channel height, m;
Re is the Reynolds number.
It has been shown that supplying the heat-transfer medium to the dephlegmators placed on the trays has a major influence on the separation process in the diabatic column.
In this regard, a promising heat exchange device for carrying out partial condensation of vapors on the diabatic column trays is a dephlegmator made of pipes vertically mounted along the column height [
22,
23] (see
Figure 3b).
In the dephlegmator made of pipes (see
Figure 3b), it is possible to intensify vapor condensation and reduce condensate (reflux) supercooling. The dephlegmator is compact, easy to manufacture, and has a low metal content. The device also does not significantly affect the hydrodynamics and mass exchange on the trays. Temperature stresses are easily eliminated; for example, by installing a lens compensator on the pipe surface.
To intensify heat removal in the pipe dephlegmator, a film flow was placed over their surface [
24]. At that, it is possible to ensure not only gravitational flow of the heat-transfer film, but also an ascending and descending film flow without significant changes in the design [
25,
26]. In this case, the heat-transfer coefficient is an order of magnitude higher than that for the single-phase flow in the heat exchanger pipes. Heat removal is carried out at a lower heat-transfer medium flow rate. Combined heat removal can be carried out in the dephlegmator pipes by evaporation from the film surface, heating, and boiling.
For example, maintaining the boiling process in the draining film allows intensifying heat transfer and maintaining the same temperature of the heat-transfer medium along the column height. The magnitude of the heat-transfer coefficient during the boiling of the gravitationally flowing water film reaches [
27] 10,000 W/(m
2 K) and more.
This paper presents work on the development of a diabatic column design employing a new approach to reflux formation on trays and the organization of methods for intensifying heat removal and separation, which allows reducing capital and operating costs due to a decrease in unit dimensions and steam and heat-transfer medium consumption.
We developed new designs of contact vortex devices on trays, which allowed lowering the volume of liquid on the tray deck, reducing sediment formation conditions and the number of stagnant zones, and increasing tray efficiency and steam and liquid productivity.
2. Materials and Methods
2.1. Designs of the Experimental Rectification Unit and Vortex Devices
For the trays of the reinforcing rectification column, a vortex contact device [
28], shown in
Figure 4a, was developed, efficiently operating at relatively low liquid loads.
For exhaustive columns where high liquid loads are required, the devices [
29] shown in
Figure 4b,c were developed.
The contact device shown in
Figure 4a consists of a cylindrical insert 1, swirler 2 equipped with bottom 3 and cover 4 where hydraulic lock cup 5, overflow device 6 with drain bar 7 and a sealing device 8 for heat exchange pipes (not shown in
Figure 4a) are located. Bottom 3 has steam passage channels 9.
The bent part of the drain bar 7 forms a curvature of the rotating gas–liquid layer surface in the device and ensures liquid flow into the overflow device 6. The liquid is supplied via the overflow device to a cavity of hydraulic lock cup 5, and then, under the influence of centrifugal force, is pressed onto the surface of cylindrical insert 1. The gas passes through the channels of swirler 2 in rotational motion and contacts the gas–liquid layer rotating on the surface of cylindrical insert 1.
The contact device (
Figure 4b,c) consists of tray deck 1 fitted with gas passage pipe connector 2, cover 3, and tangential swirler 4 with annular channels 5 and 6.
Steam enters the device via channel 2 into the swirler. It acquires rotational motion and disperses in the form of currents into the liquid located on the tray deck, thereby forming a developed interphase surface [
30].
The design parameters of the studied swirlers are as follows: channel height and width h = 0.003–0.08 m, b = 0.0015–0.010 m, channel wall slope angle α = 26°. Outer swirler radius Rout = 0.103 m. Inner swirler radius Rin = 0.054–0.092 m. Number of channels n = 8–40 pcs. The length of the channel varied from 0.004 to 0.022 m.
The experimental unit designed to study the rectification process is presented in
Figure 5.
The working volume of still 1 of the experimental unit was 0.1 m
3. Electric heaters with total power of 30 kW were fitted in the still heat exchange jacket. Column shells 2 were 0.2 m in diameter, between which three trays 3 were fitted. Above the upper tray was a condenser 15 made of a copper pipe 6 mm in diameter in the form of a coil. Vortex contact devices 4 (also shown in
Figure 4a) were mounted on the trays. A heat exchange pipe 5 in diameter 0.028 m was passed through them. The upper end of pipe 5 is equipped with heat-transfer medium (water) injection chamber 16. The lower end is connected to storage containers 9 connected by pipelines with vacuum pump 8.
Vapors of the reinforced mixture condensed in condenser 6 are returned via the hydraulic seal to still 1.
For adiabatic rectification, the heat-transfer medium was fed only to condensers 15 and 6.
In studying diabatic rectification, the heat-transfer medium was heated in heat exchanger 7 to the required temperature and fed to dephlegmator pipe 5.
On the inner surface of pipe 5, liquid distributor 14 provided a draining liquid film. A vacuum created in container 9 provided for boiling of the draining water film and evaporation of moisture from the surface. This ensured the partial condensation of rising vapors in the unit and the formation of a reflux on each tray.
2.2. Experimental Data Processing
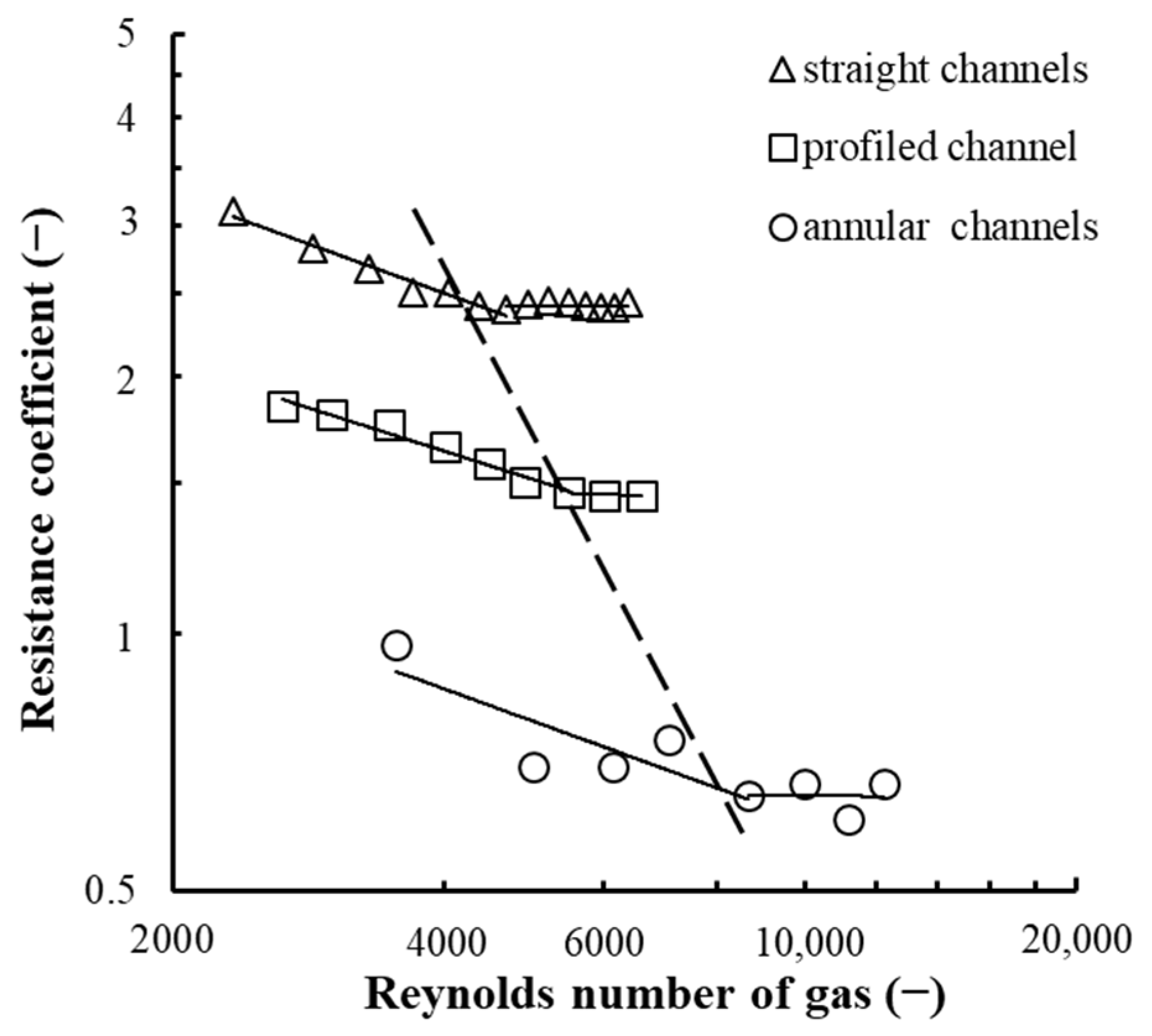
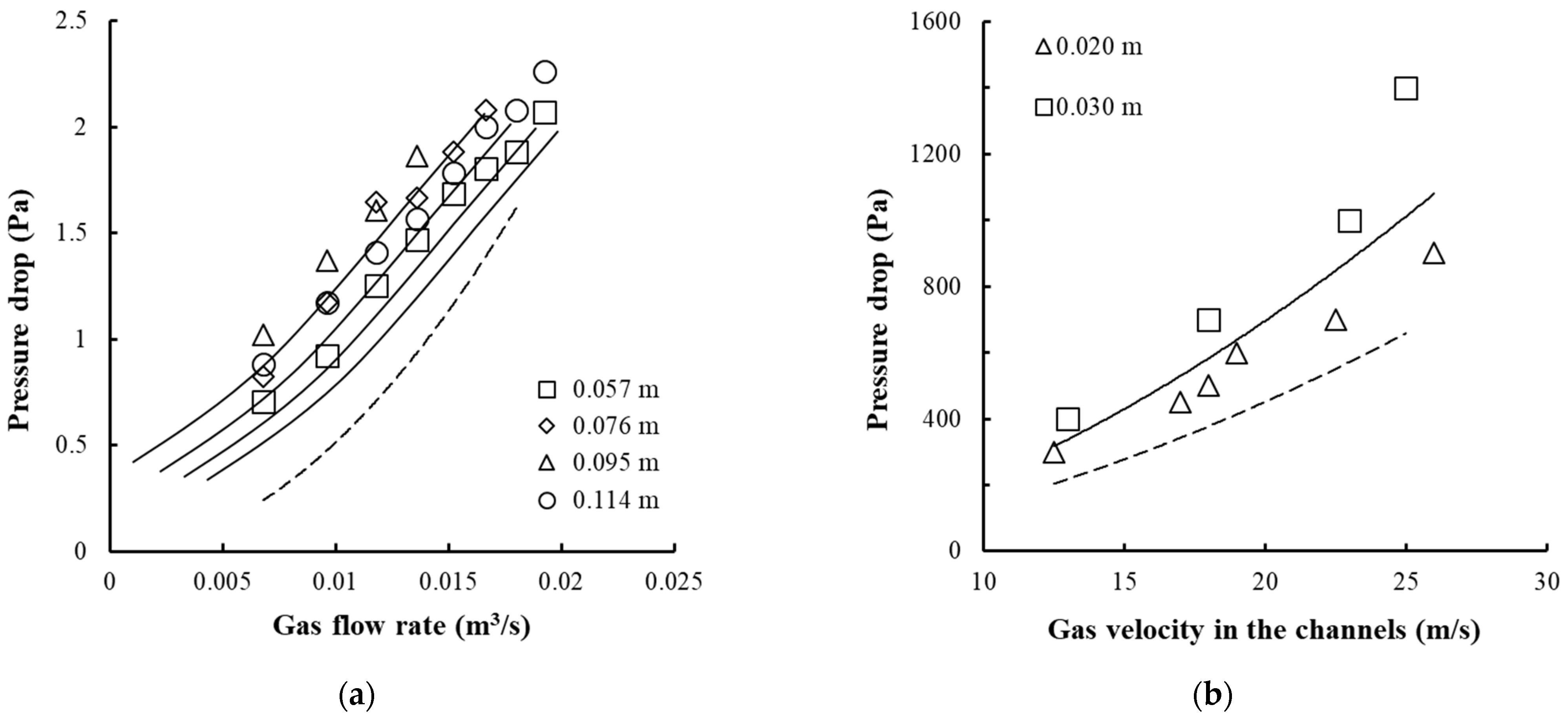
In studying the hydrodynamic parameters of the vortex devices, the flow rate of the supplied liquid was recorded using a rotameter and the gas flow rate using a diaphragm.
Differential pressure gauges were used to measure the tray pressure drop.
The resistance coefficient was determined according to
where Δ
P is the swirler pressure drop, Pa;
uG is the average gas flow rate in the swirler channels, m/s;
ρG is gas density, kg/m
3.
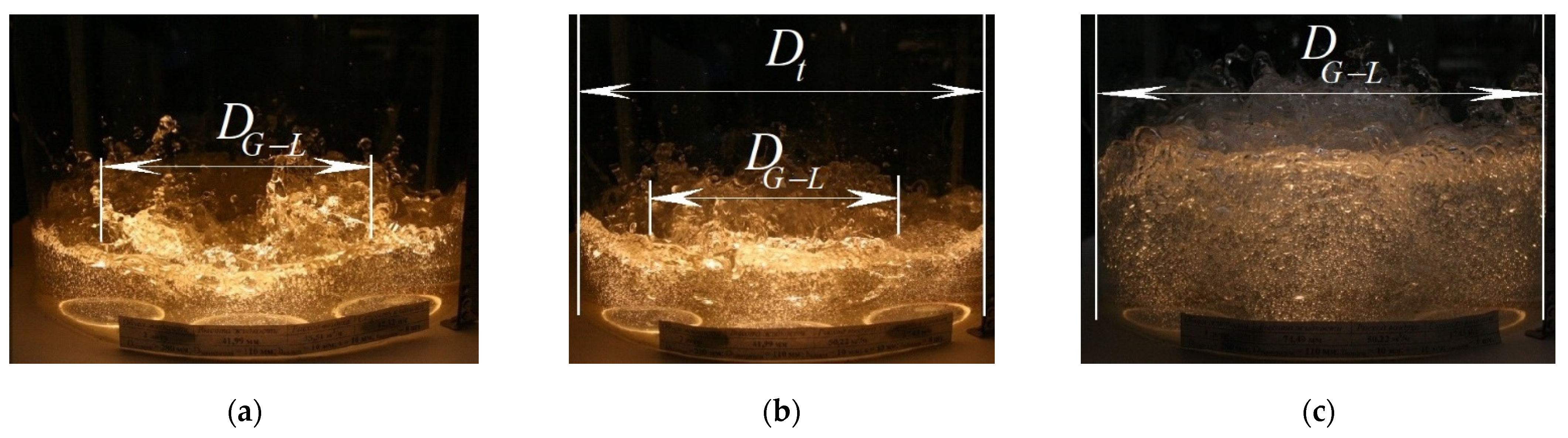
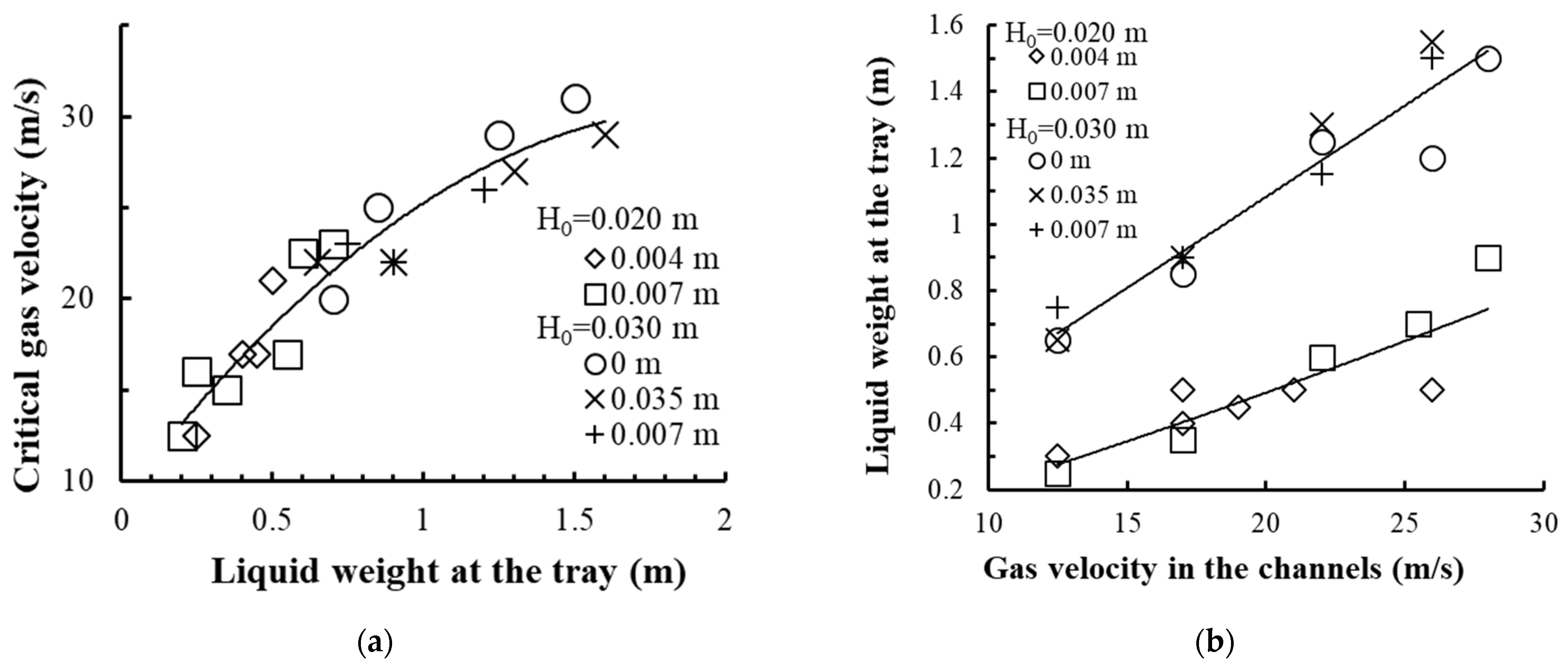
The critical gas velocity in the swirler channels and the angular velocity of the gas–liquid layer, were determined using video recording.
The magnitude of the gas content in the liquid layer was determined by the volumetric method according to
where
H0 is the height of the liquid layer on the tray, m;
HG−L is the height of the gas–liquid layer, m.
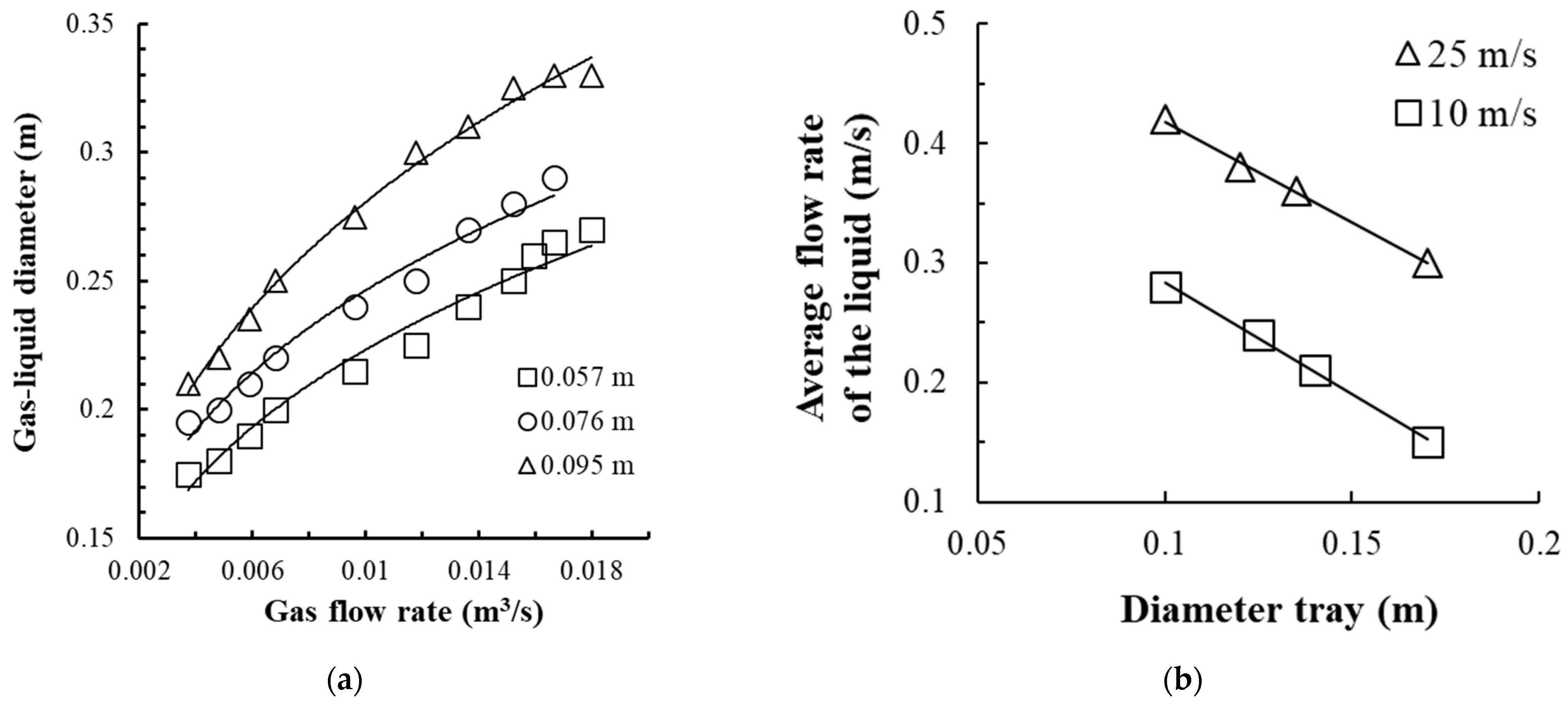
The interphase surface was determined as
where
φ is gas content;
Db is the average surface diameter of the bubble, m.
Dissipation of the gas energy
ε (W/kg) supplied into the working volume of the shell through air currents was calculated according to the formula
where
U is internal gas energy, W;
M is liquid weight, kg.
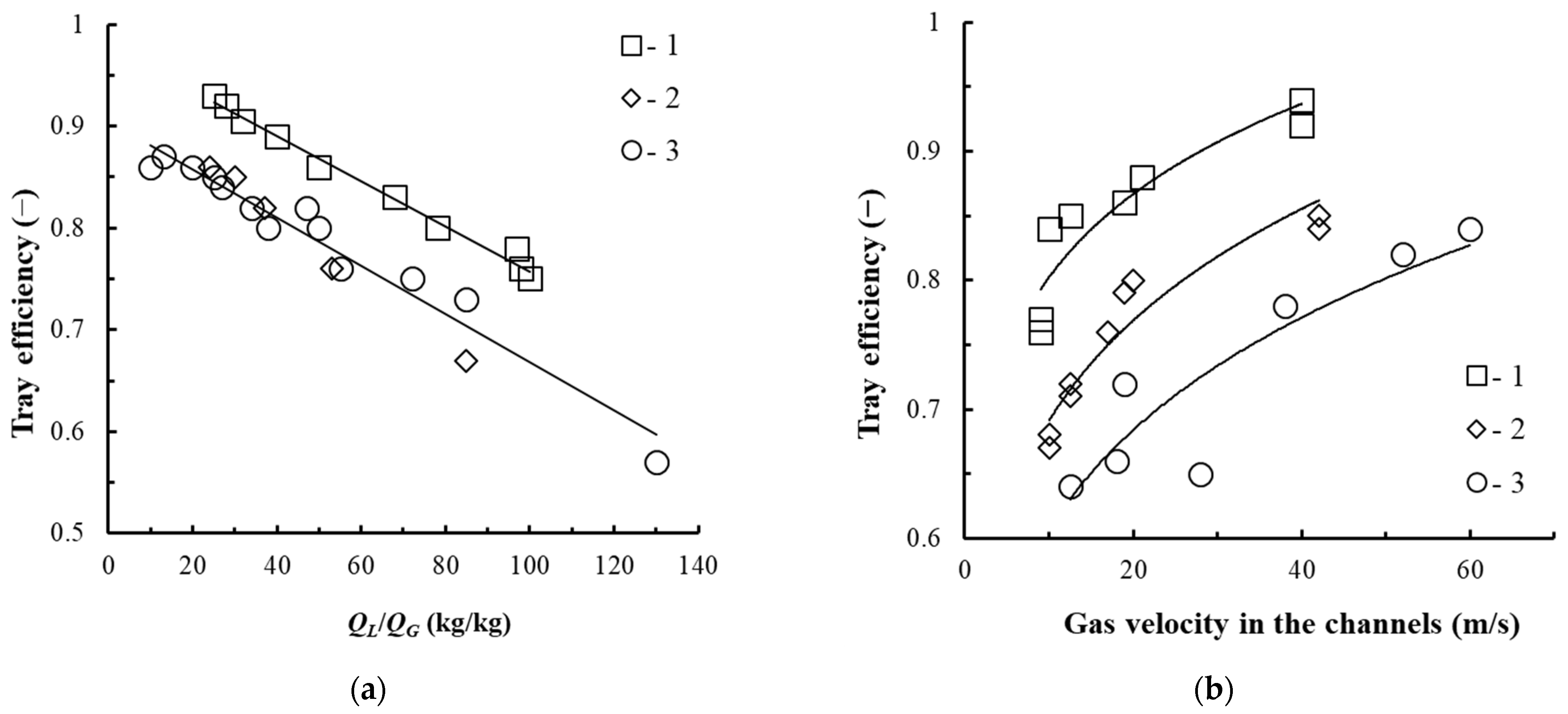
The mass transfer on the vortex contact tray shown in
Figure 4b,c was studied using the example of water absorption of air oxygen according to [
31].
The initial concentration of oxygen in the water was 0.1 × 10−3 kg/m3. A polarographic sensor was used to measure the oxygen concentration in the water.
The water flow rate with reduced oxygen content ranged from 0.1 to 1.0 m3/h.
The mass transfer intensity was determined according to the dependence:
where
QL is the flow rate of oxygen-free water supplied to the tray, m
3/s;
c0 is the concentration of oxygen in the liquid supplied to the tray, kg/m
3;
c is the concentration of oxygen in the liquid on the tray, kg/m
3;
V is the volume of liquid on the tray, m
3;
c* is the equilibrium concentration of oxygen in the liquid, kg/m
3;
βV is the volumetric mass transfer coefficient, s
−1.
The rectification and heat exchange process was studied this using the unit presented in
Figure 5.
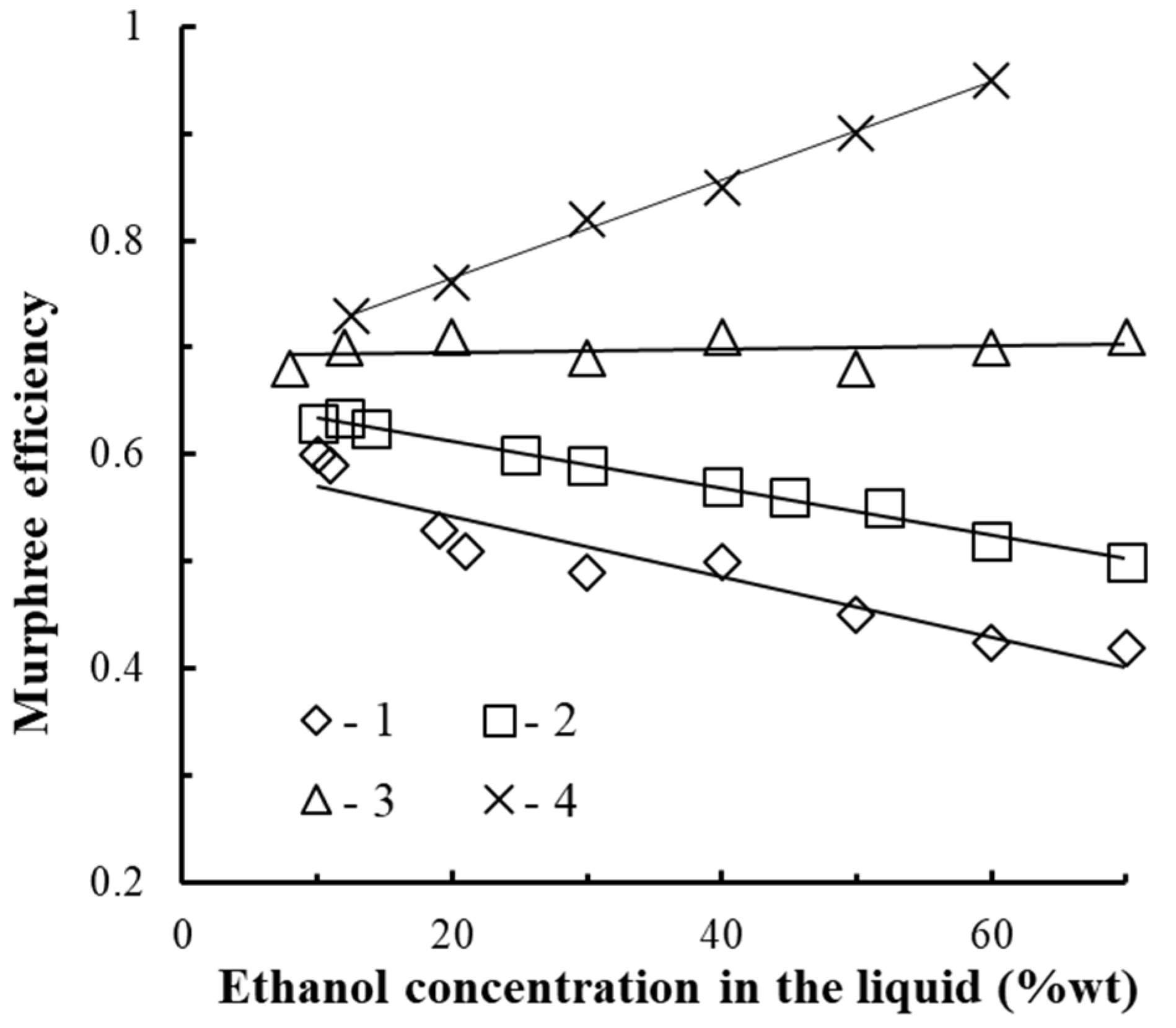
The vortex tray efficiency during rectification was determined according to the Murphree formula:
where
y* is the equilibrium concentration of vapors with the liquid on the tray, % mol;
yent is the ethanol vapor concentration at the tray inlet, % mol;
yex is the ethanol vapor concentration at the tray outlet, % mol.
In rectification, the initial concentration of the mixture in the still was (1–60)% wt. Ethanol concentration was measured in the vapor and liquid phases. The composition of ethyl alcohol was determined using a LR-3 refractometer and an alcohol meter calibrated used a YCDplus a YCDplus mass-spectrometer.
The temperature indicators in the liquid and vapor phases were determined using TSM-9418 resistance thermometers, secondary Thermodat 35TsO/GVS devices calibrated using glass thermometers with a scale division of 0.01 °C, taking into account the influence of the mixture vapor velocity on the temperature indicators at the sensor site.
The flow rate of steam and liquid in the column was calculated based on material and thermal balances.
4. Mass Transfer on the Vortex Tray
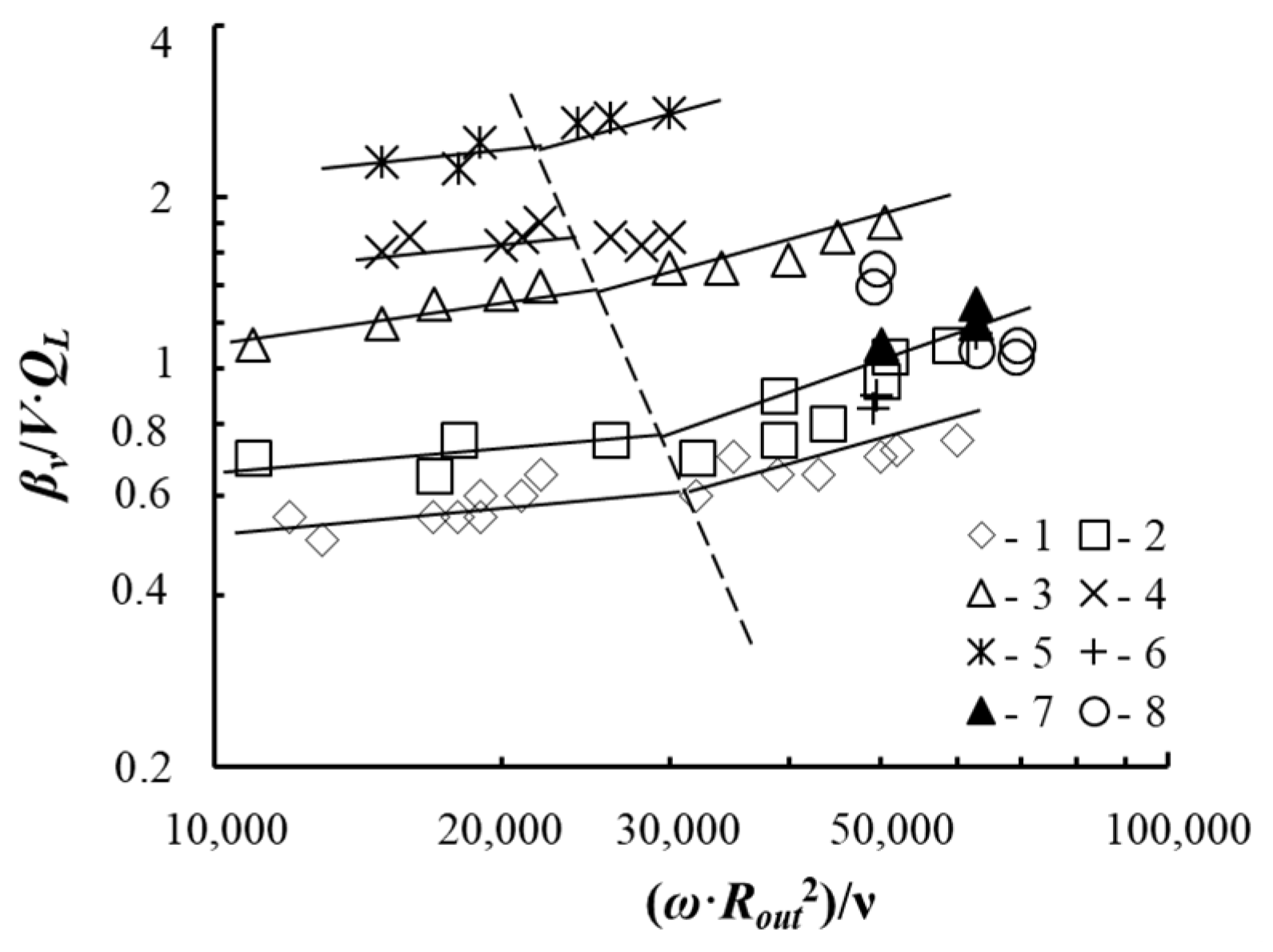
The experimental values of the mass transfer coefficient, in the context of absorption, obtained during the operation of vortex devices in the bubble-annular and annular modes are shown in
Figure 12.
The experimental data are consistent with calculated data (lines in
Figure 11) according to the dependence [
31]
where
βV—mass transfer intensity, s
−1;
V—volume of liquid in motion, m
3;
QL—flow rate of oxygen-free water supplied to the tray, m
3/s;
A = 2 × 10
−3,
p = 0.24 under bubble-annular flow mode;
A = 0.55 × 10
−3,
p = 0.38—under annular flow mode;
ω—angular velocity of liquid, s
−1;
Rout—external swirler radius, m; m;
ν—liquid kinematic viscosity coefficient, m
2/s;
HG-L—height of the gas–liquid layer, m;
h—height of the channels in the swirler, m;
Sc—Schmidt criterion.
The mass transfer coefficient (
h−1) can also be assessed according to the dependence proposed in the work [
30]:
where a is the interphase surface, m
−1;
ε is the dissipation of gas energy, W/kg.
The angular velocity of the rotating gas–liquid layer on the cylindrical insert surface of the device was 10–20 s−1.
The maximum dissipation of gas energy (up to 5 W/kg) was achieved on the vortex contact device with annular channels in the bubble-annular mode. In this case, the magnitude of the surface mass transfer coefficient was 0.5 × 10−3 m/s.
6. Diabatic Alcohol Columns
6.1. Column Design
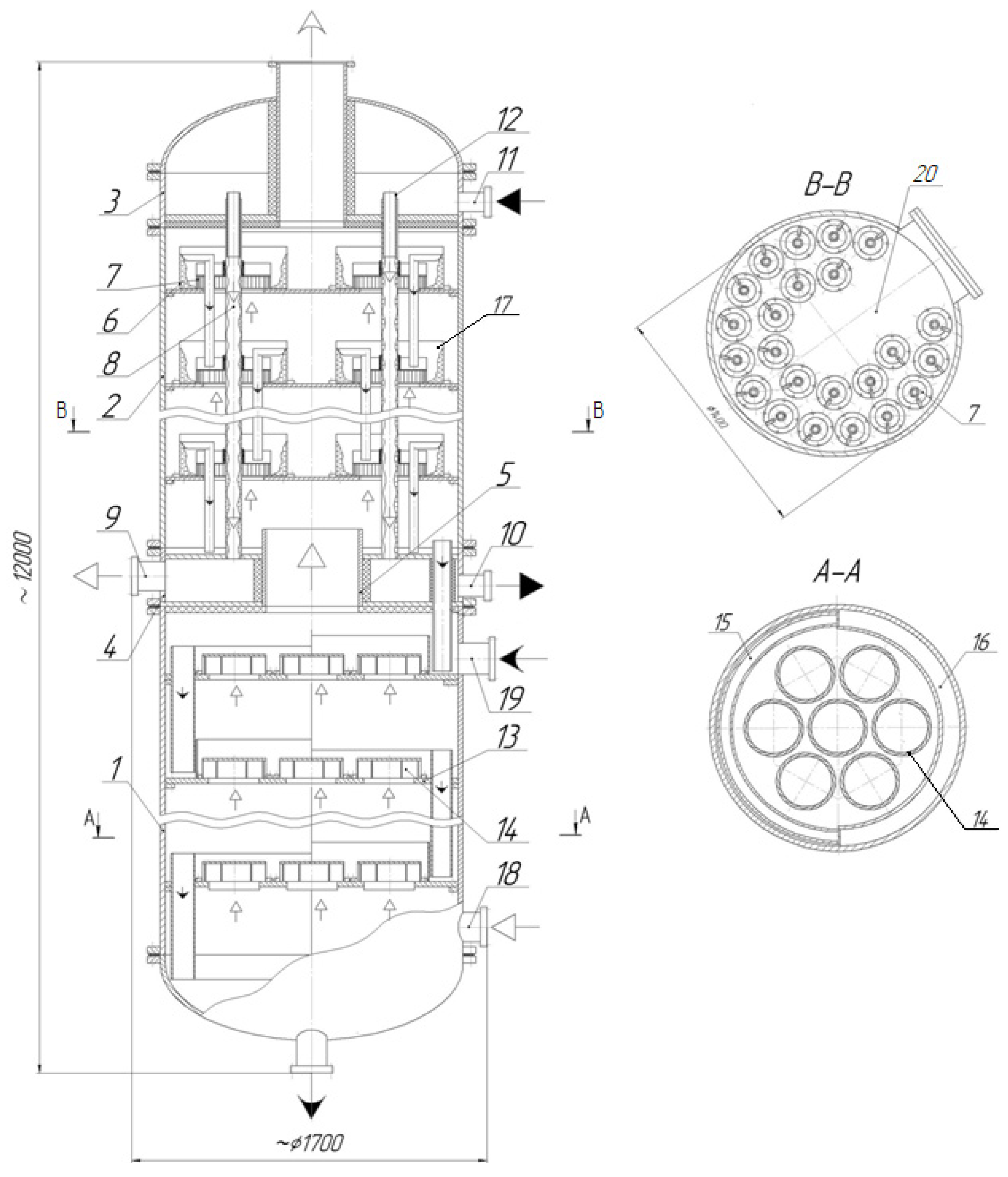
A design of an alcohol diabatic column was created based on the developed and studied vortex devices. The diagram is presented in
Figure 16.
The column consists of lower exhaustive column 1, upper concentrating column 2 equipped with chambers 3 and 4 for heat-transfer medium outlet, including steam passage pipe connectors 5.
Vortex contact devices 7 (shown in
Figure 4a) are located in the concentrating section of column 2 on trays 6. Heat exchange pipes 8 are also fitted to create a reflux in each vortex device due to partial condensation of the mixture ascending vapors on their outer surface. The lower end of the heat exchange pipes is connected to chamber 4 equipped with connecting piece 9 for venting gas into the vacuum line and connecting piece 10 for removing the heat-transfer medium. The upper end of the heat exchange pipes is connected to the cavity of chamber 3 where the heat-transfer medium is supplied through connecting piece 11 and is equipped with pipe connectors for creating liquid film 12.
In the exhaustive section of column 1 on trays 13, vortex devices 14 (shown in
Figure 4c) are fitted to operate in the bubble and bubble-annular modes.
The tray of the exhaustive column includes liquid flows 15, hydraulic lock cup 16, and vortex devices 14.
Vortex devices 7 are fastened and cylindrical inserts 17 are located on tray 6 of the concentrating column along concentric circles.
Cavity 20 allows the trays to be assembled and disassembled in the shells of column 2. The cylindrical inserts installed tightly against the tray prevents the ingress of liquid between them, which eliminates stagnant zones and reduces the volume of liquid in the device.
The column is also equipped with process pipe connectors and bosses for the process and fraction selection.
During operation of the column, vapors from the still pass through the vortex contact devices 14 located on the trays of the column exhaustive section and have contact with the liquid on it. After the exhaustive section of the column, the vapors pass through pipe connector 5 and enter vortex contact devices 7 located on trays 6 of the concentrating section of the column. There, they interact with the reflux rotating in the form of a gas–liquid layer on the inner surface of cylindrical inserts 17. The reflux forms on each tray due to condensation of the vapor on the surface of the heat exchange pipes, which then flows from one tray to another through the overflow pipe connectors. The heat-transfer medium (for example, water) is supplied to chamber 3 through connecting piece 11, which then enters the annular gap formed by pipe connector 12 and pipe 8, and there forms a film. The liquid film flows down the inner surface of heat exchange pipes 8, providing heat removal (vapor condensation). At the heat exchange pipe outlet, water flows into chamber 4 and exits the column through connecting piece 10. To ensure the boiling of the gravity-drainage water film in the cavity of pipes 8, a vacuum is provided in chambers 3 and 4 by connecting the vacuum line to connecting piece 9.
At an ethanol column capacity of 2900 L/h and a reflux ratio of four, the vapor flow rate of the column concentrating section was equal to 2.2 m3/s, while the total reflux flow rate was 2.48 kg/s. The column diameter was 1.4 m.
In this case, the number of vortex contact devices on the concentrating column trays was 23 pieces. The cylindrical insert diameter was 0.2 m, the swirler diameter was 0.17 m, the number of channels in the swirler was 40 pcs, the channel height was 0.008–0.01 m, and the channel width was 0.005–0.01 m. The diameter of heat exchange pipes was 0.03 m.
The number of vortex devices on the exhaustive column trays was 7 pieces, their diameter 0.35 m, and the internal 0.25 m. The channel height was 0.045 m, the channel width 0.011 m, and the number of channels 11 pcs.
6.2. Comparing the Alcohol Column Parameters at Ethanol Capacity 2900 L/h in Adiabatic and Diabatic Rectification
Height of column concentrating sections. With the accepted theoretical number of concentrating trays 25 pcs. [
36], the number of actual vortex trays in adiabatic rectification and an efficiency of 0.4 was 63 pcs. At an efficiency of 0.7, the number of trays in the diabatic column with vortex devices was 36 pcs. With the accepted distance between the trays of 300 mm, the height of the working section of the adiabatic concentrating column would be 19 m, and that of the diabatic concentrating column would be 11 m.
Dephlegmator surface. The magnitude of the heat transfer coefficient in the pipes of the multiway shell-and-tube dephlegmator of the adiabatic alcohol column fitted in the upper part of the unit according to the industrial data [
36] is 580 W/(m
2 K). With different designs of the dephlegmator and the varying temperature of the reflux entering the upper tray, the surface of the adiabatic column dephlegmator could be [
36] 100–185 m
2.
In the diabatic column, the dephlegmator consists of heat exchange pipes built into the column height. Heat removal in the dephlegmator pipes is carried out due to the boiling of a liquid film caused by reduced pressure in the heat exchange pipe cavity. According to the data [
26], the heat transfer coefficient during boiling in the film is 4000 W/(m
2 K). During condensation of the ethanol–water mixture vapors [
36], the heat transfer coefficient is 2000 W/(m
2 K). Then the heat exchange surface of the heat exchange pipes is 72 m
2. This is lower than that of the adiabatic column.
It is important to note that the heat transfer coefficient during vapor condensation in the adiabatic column dephlegmator is lower than that on the heat exchange pipe surface in the diabatic column. This is due to the relatively low thermophysical parameters of condensed vapors in the upper section of the adiabatic column (a small magnitude of a phase transition criterion) [
26]. This results in an increase in the heat exchange surface in the adiabatic column dephlegmator.
Heat-transfer medium consumption. In the shell-and-tube dephlegmator of the adiabatic alcohol column, based on the thermal balance, when heating water from 20 to 70 °C, the water flow rate was 60 m3/h.
Heat removal in the diabatic column is carried out in a draining water film during boiling and evaporation of vapors from its surface. Heat removal takes place mainly due to a phase transition. In this case, the flow rate of water supplied to the heat exchange pipes is only necessary to maintain a turbulent mode in the draining film. Therefore, the water flow rate is three times lower than the adiabatic column (20 m
3/h). This heat-transfer medium flow rate ensures the necessary Reynolds number to maintain the heat transfer coefficient [
24] in the film during boiling.
Energy consumption. The temperature of the condensate flowing from the multi-pass dephlegmator pipe surface onto the upper tray of the adiabatic column was 30–60 °C. Therefore, an additional heat flow of the ascending steam was spent on heating the condensate to the boiling point. On average, according to [
36], a difference between the incoming reflux temperature and the rising vapor temperature is 10 °C. Then the heat flow of vapors spent on heating the reflux on the upper tray is ~104 kW.
According to experimental data, in a diabatic alcohol column, the supercooling of condensate on the dephlegmator pipe surface will be maximum 3 °C. This results in a decrease in the flow rate of rising vapors in the column for heating the condensate.
It is important to note that in a diabatic column, the heat-transfer medium is reused to irrigate the dephlegmator pipes without a preliminary cooling procedure, for example, in a cooling tower. This also results in a decrease in energy consumption.
During rectification under pressure in an alcoholic diabatic column, it is possible to use secondary steam produced in the cavity of the heat exchange pipes during the boiling of the heat-transfer medium. This, when installing the heat pump (ejector), will save up to 30% steam.
There are possible options for reducing energy consumption when replacing the dephlegmator pipes with heat ones [
37,
38,
39].
Summing up the results presented in the article, the number of trays in the diabatic column decreased from 63 to 36 pieces and the height of the unit decreased by half compared to the adiabatic one. With a column diameter of 1.4 m, there were 7–23 vortex contact devices on the tray, whereas in the adiabatic column with the cap trays, there were 49. The surface of the reflux condenser heat exchange pipes of the diabatic column was 72 m2, while that of the adiabatic column was 100–185 m2.
The flow rate of water supplied to the reflux condenser pipes decreased by three times and amounted to 20 m3/h. The heat flow of vapors spent on heating the reflux in the diabatic column decreased three times, and the volume of liquid on the tray deck of the concentrating section of the columns decreased six times. Additional measures specified in the article, for example, the installation of a heat pump (ejector), will save up to 30% of the steam supplied to the column.
7. Conclusions
Based on experimental data obtained from studying hydrodynamics, mass transfer, and the rectification process on single-element trays, we developed a new design of an alcohol diabatic distillation column with built-in heat exchange pipes as the reflux condenser. The column is fitted with two types of vortex contact devices designed for the concentrating and exhaustive sections of the column.
The new contact devices for the column concentrating section allow reducing the volume of liquid on the tray deck, eliminating stagnant zones, evenly distributing the reflux, and decreasing the flow rate of steam and the heat-transfer medium. At that, the efficiency increases due to the partial rectification and intensification of the mass and heat flow transfer.
The new tray design for the column exhaustive section allows eliminating the fluctuation of liquid on the tray deck due to the uniform distribution of steam jets in the liquid, increasing steam energy dissipation, and evenly distributing steam bubbles and organizing their circulation in the liquid layer on the tray. The design provides an increase in the steam load and the supplied liquid, allows organizing a large interphase surface of up to 1200 m−1, and increases the mass-transfer coefficient up to (0.5–0.8) × 10−3 m/s.
The dependencies were determined and tested. They allow determining the tray operating mode, gas content, hydraulic resistance, interphase surface magnitude, angular velocity of the liquid–vapor mixture, magnitude of the mass-transfer coefficient, and efficiency.
The results show the prospects of using new vortex contact devices and trays, as well as the proposed design of the diabatic column.
 —liquid;
—liquid;  —steam.
—steam.
 —liquid;
—liquid;  —steam.
—steam.
 —liquid;
—liquid;  —steam.
—steam.
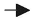 —liquid;
—liquid;  —steam.
—steam.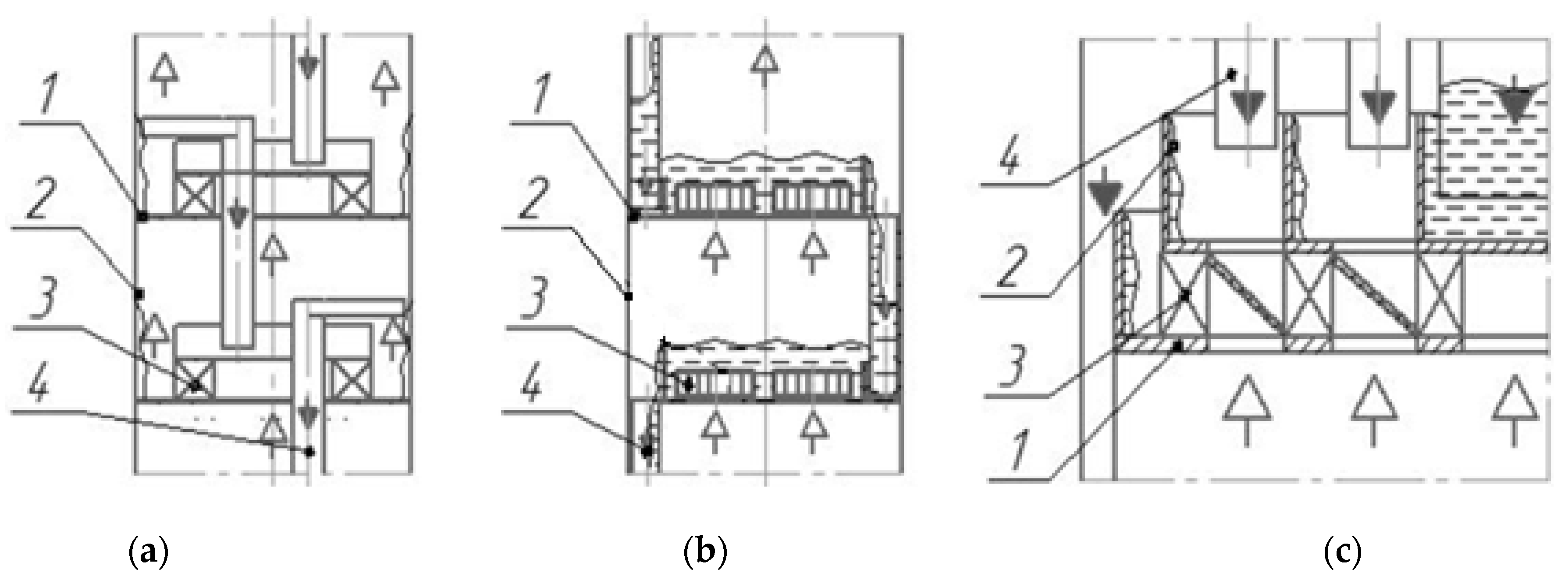
 —liquid;
—liquid;  —steam.
—steam.
 —liquid;
—liquid;  —steam.
—steam.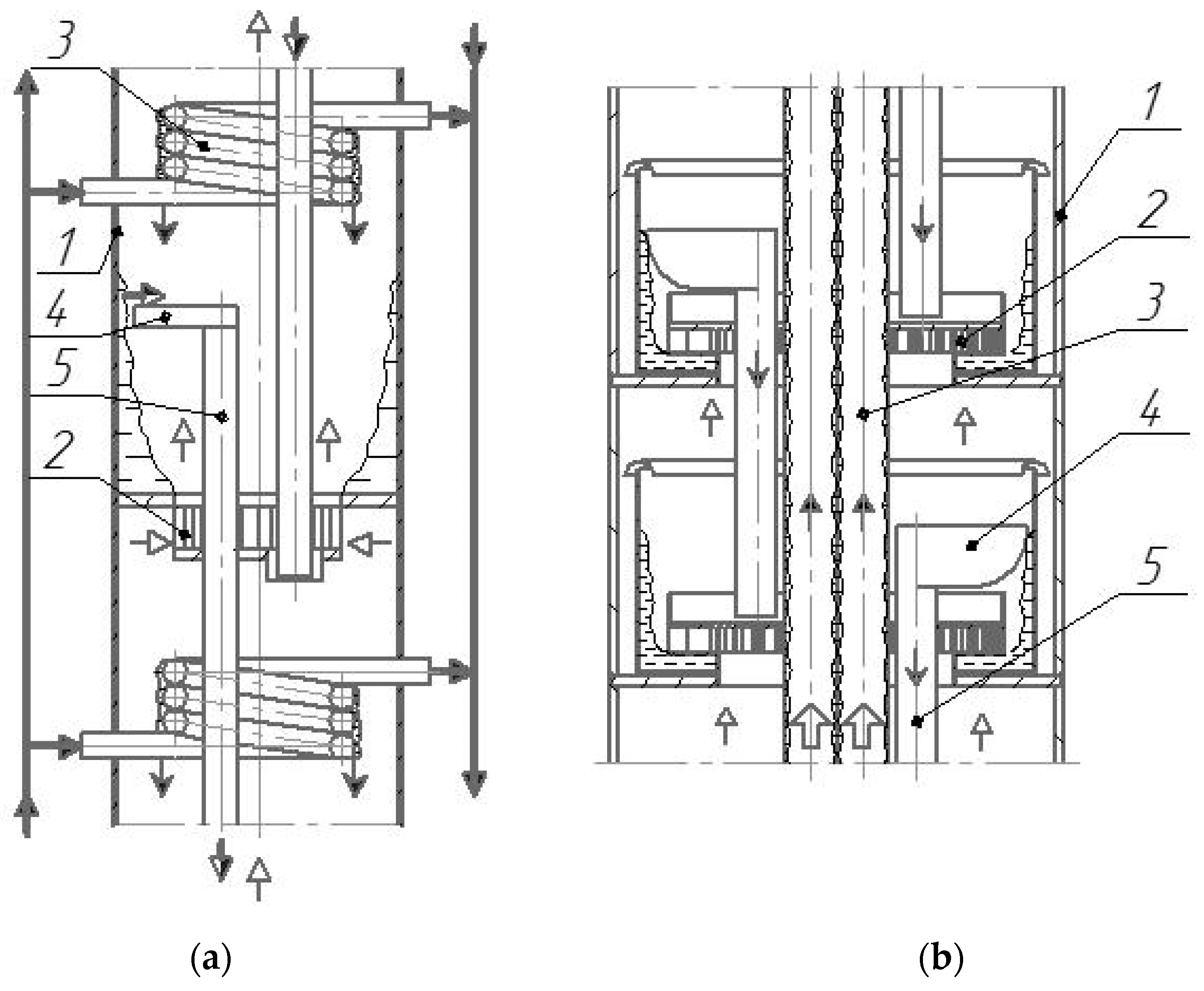
 —gas.
—gas.
 —gas.
—gas.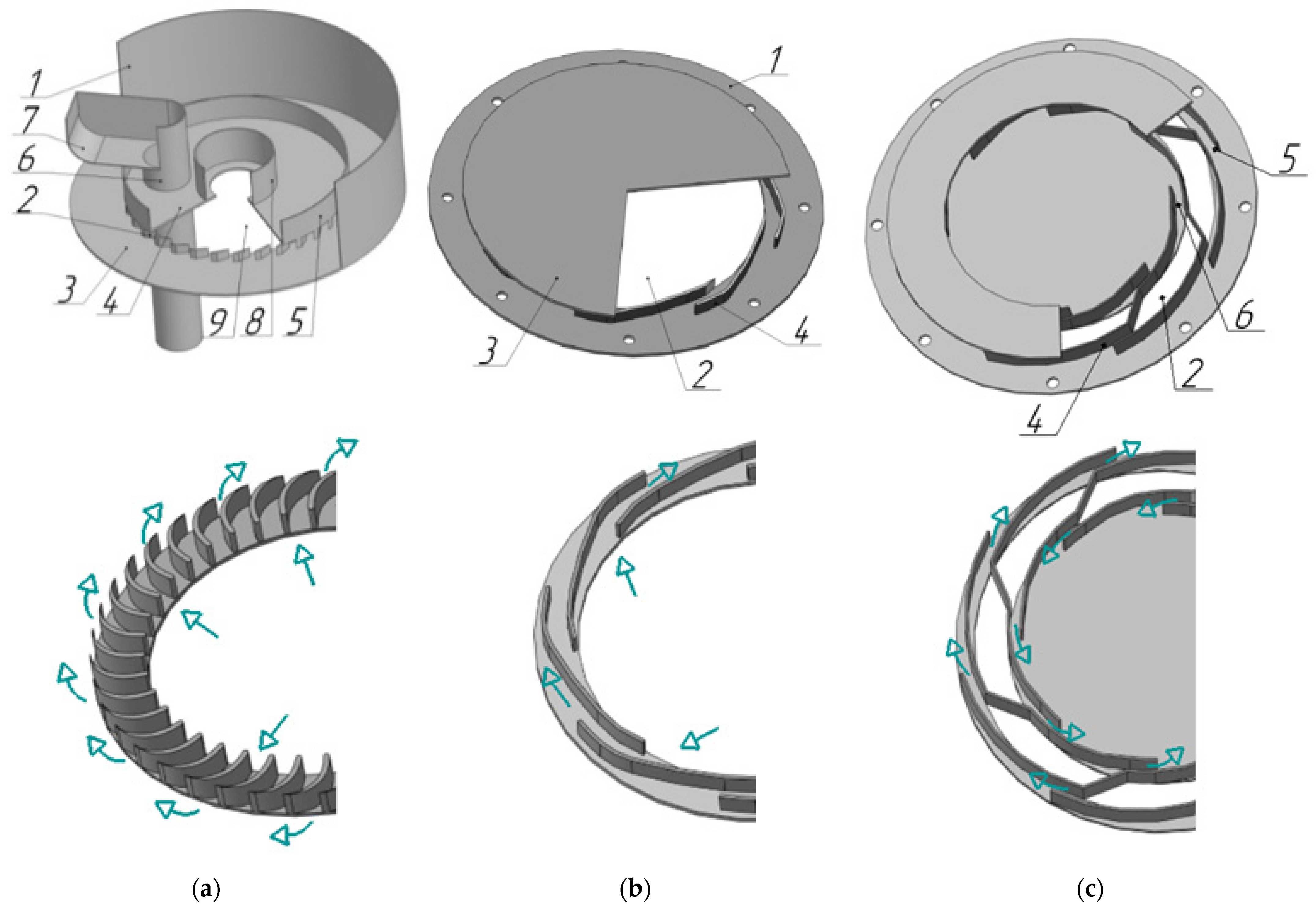
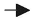 —heat-transfer medium;
—heat-transfer medium;  —steam;
—steam;  —reflux (condensate).
—reflux (condensate).
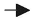 —heat-transfer medium;
—heat-transfer medium;  —steam;
—steam;  —reflux (condensate).
—reflux (condensate).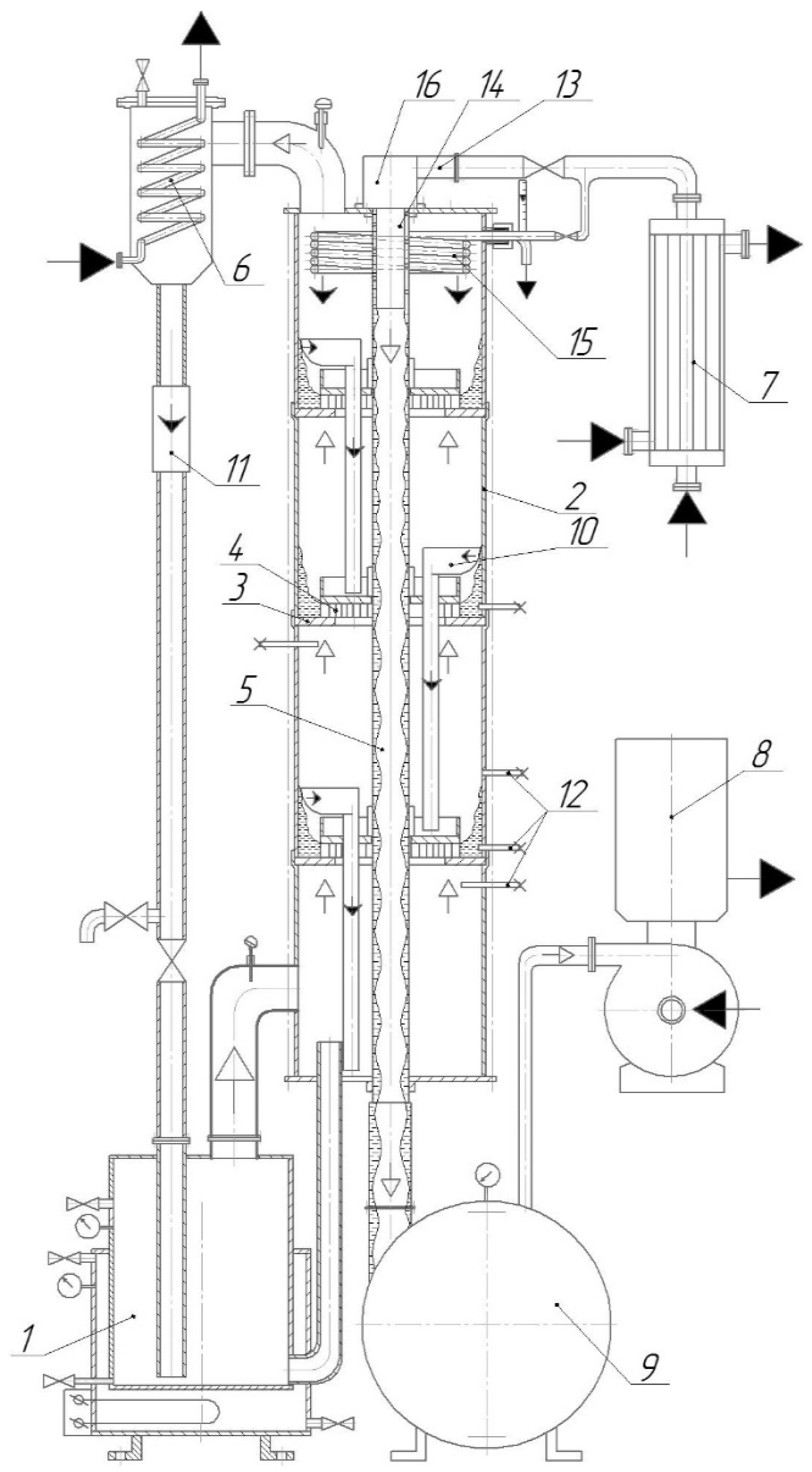

 —heat-transfer medium;
—heat-transfer medium;  —steam;
—steam;  —working mixture.
—working mixture.
 —heat-transfer medium;
—heat-transfer medium;  —steam;
—steam;  —working mixture.
—working mixture.