Thermal Decomposition Kinetic Study of Non-Recyclable Paper and Plastic Waste by Thermogravimetric Analysis
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. The Measurement of the Kinetic Parameters of the Reaction
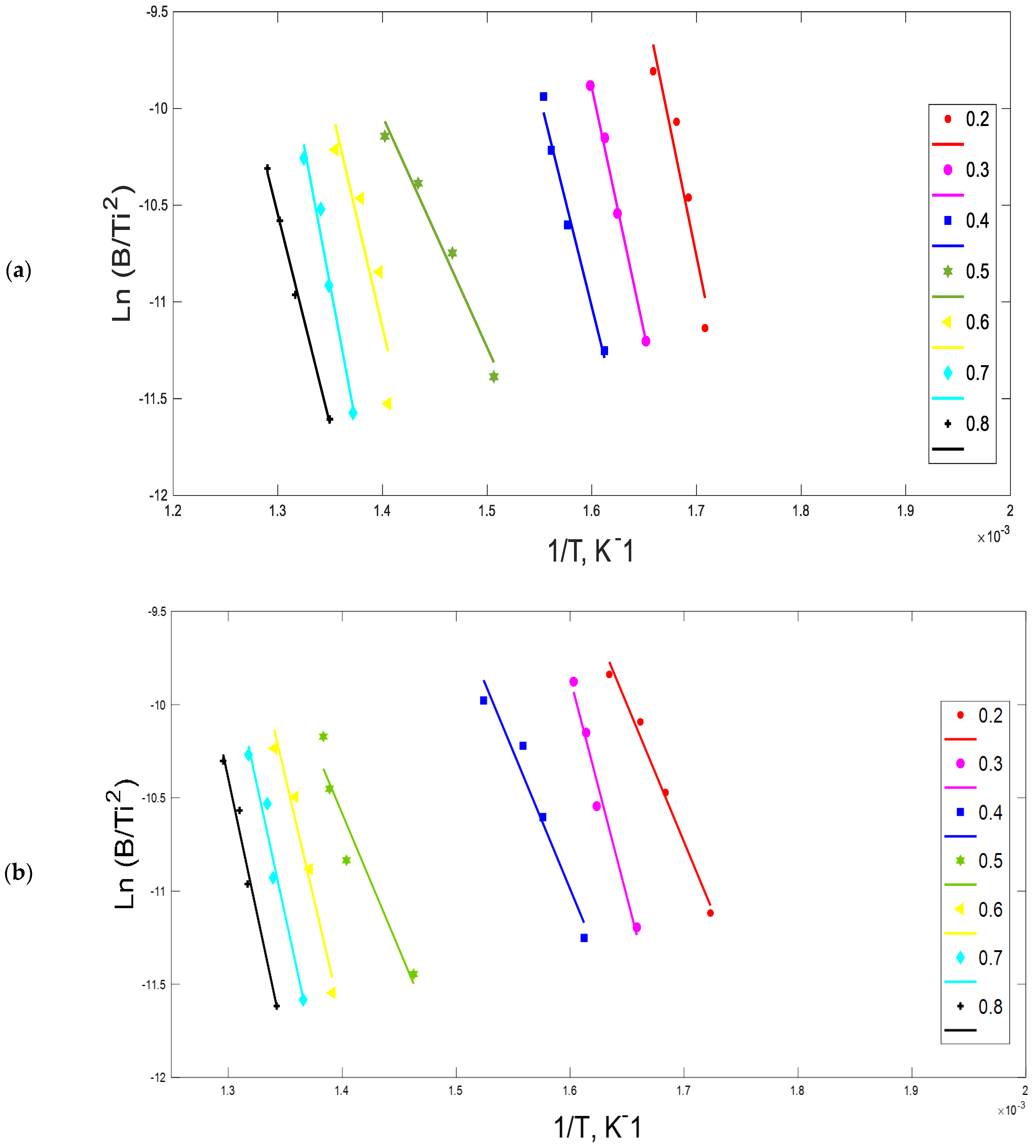
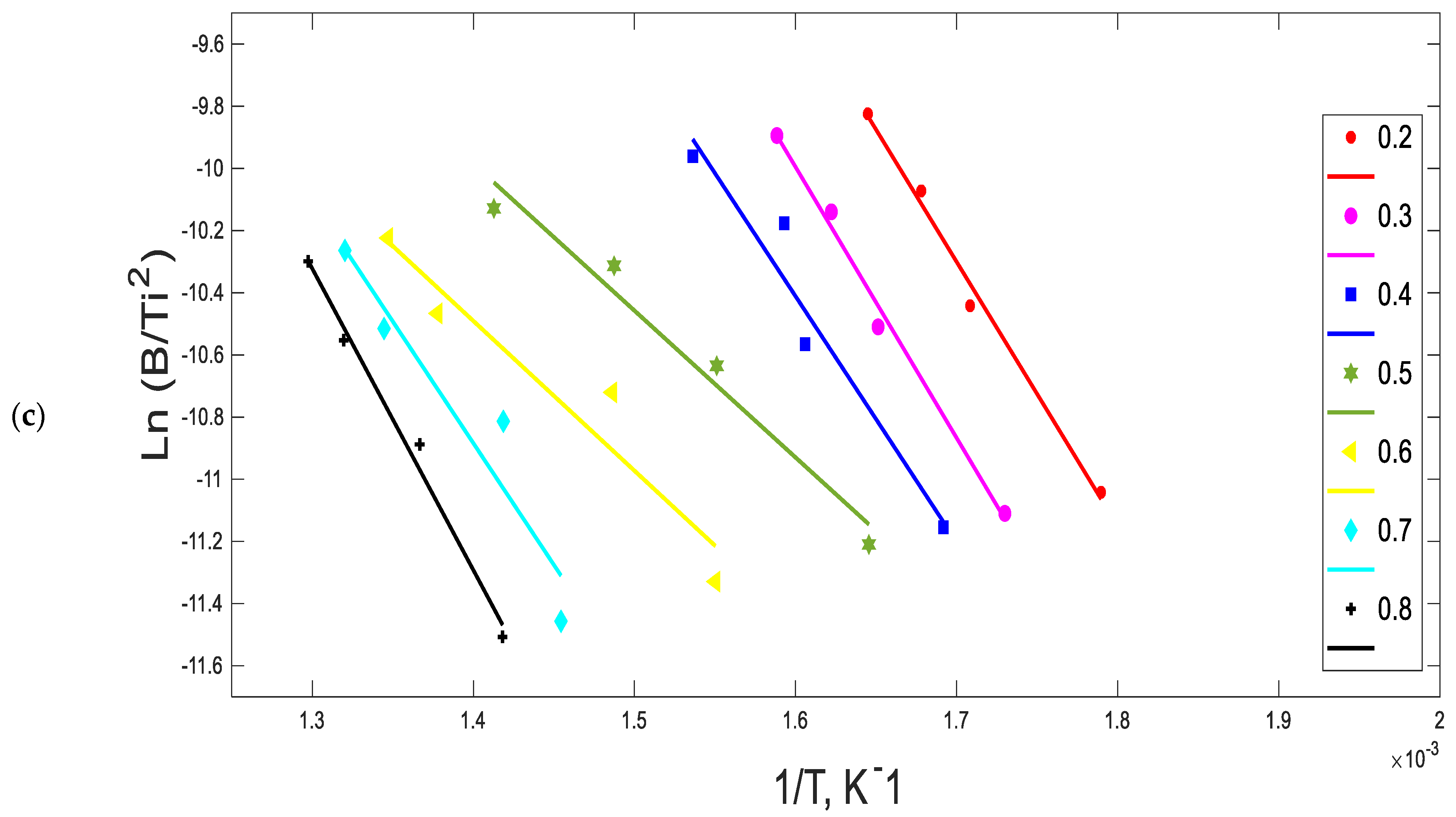
2.3. Ozawa–Flynn–Wall (OFW) Method
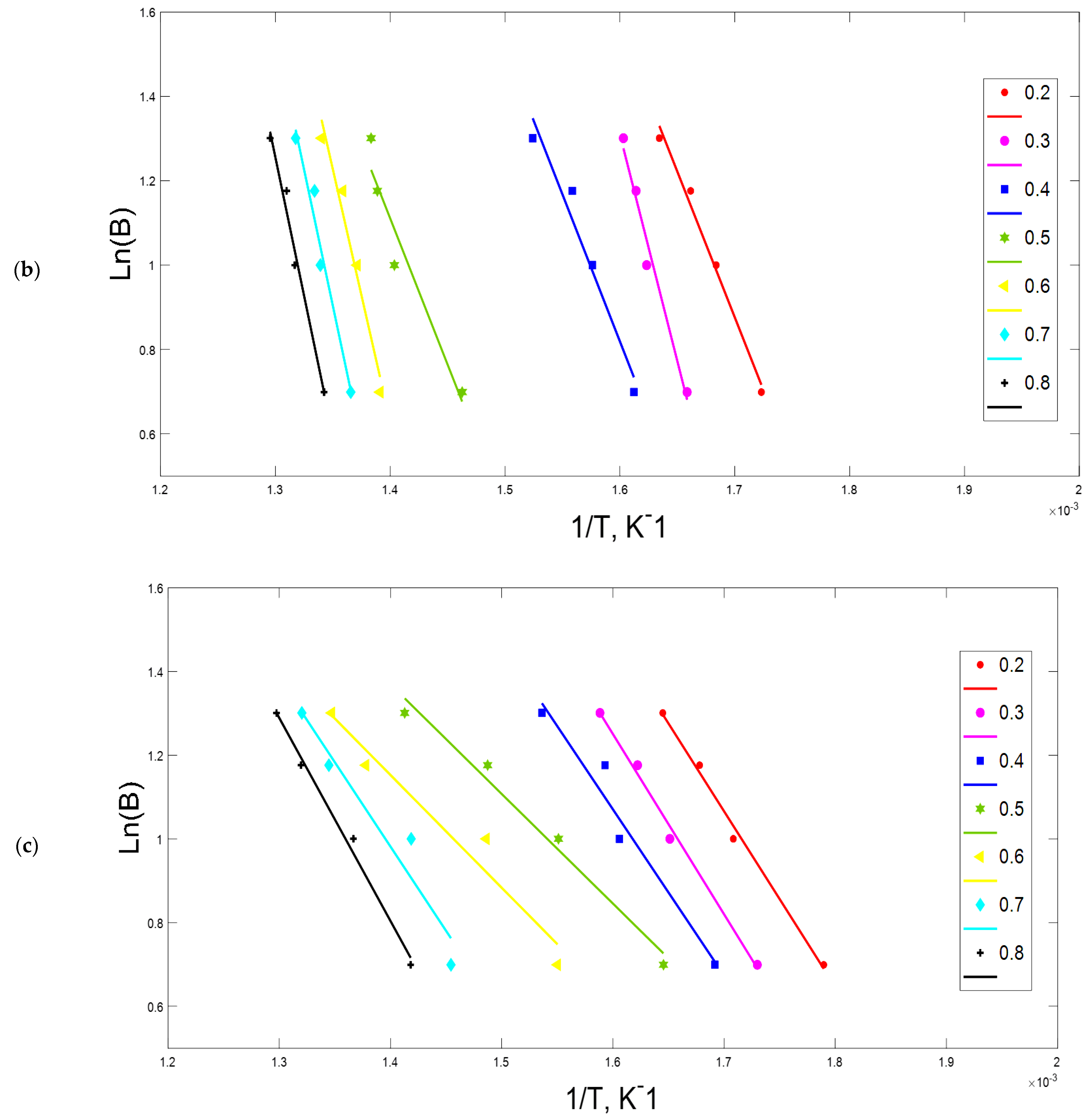
2.4. Kissinger-Akahira-Sunose (KAS)
3. Result and Discussion
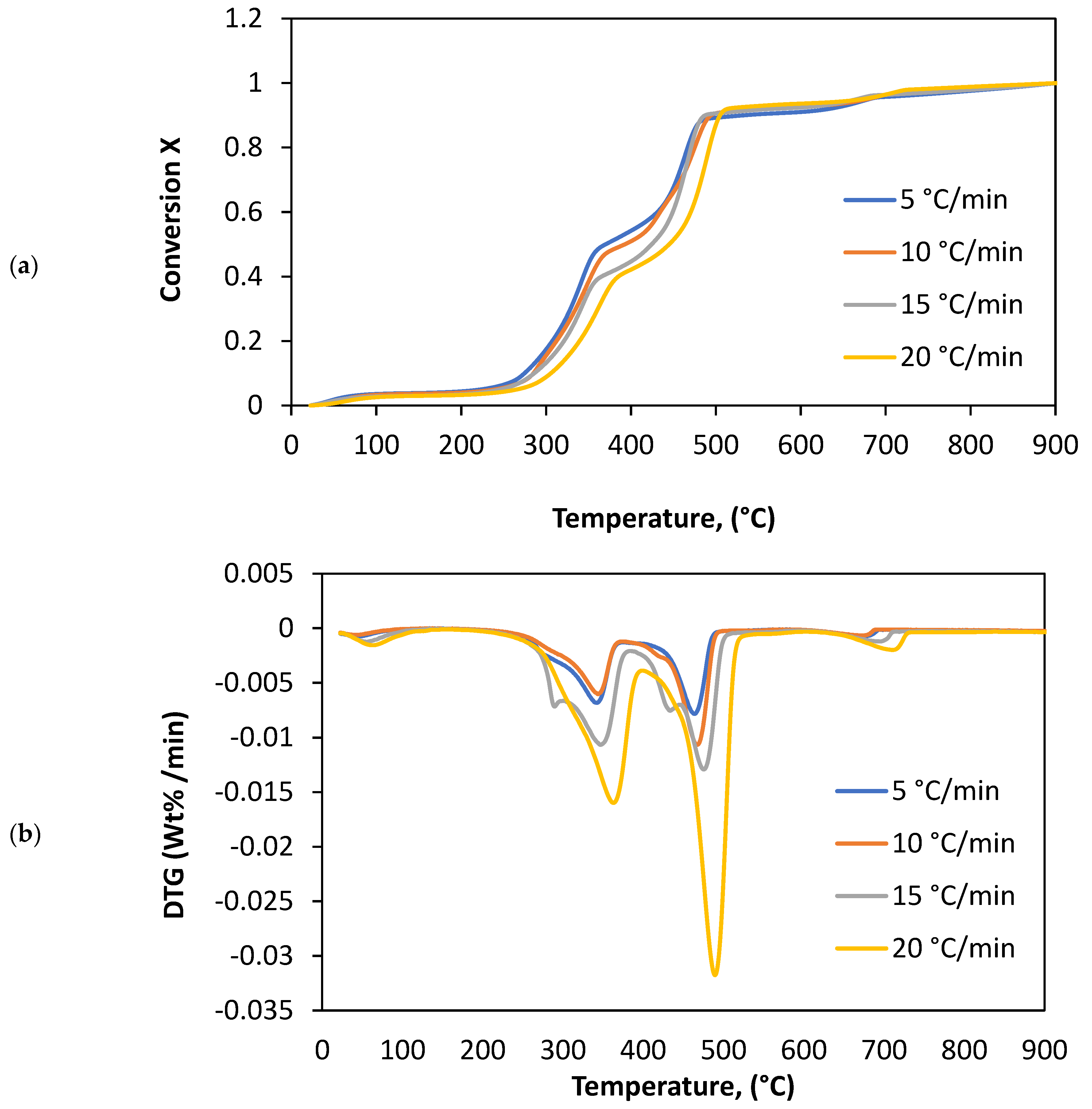
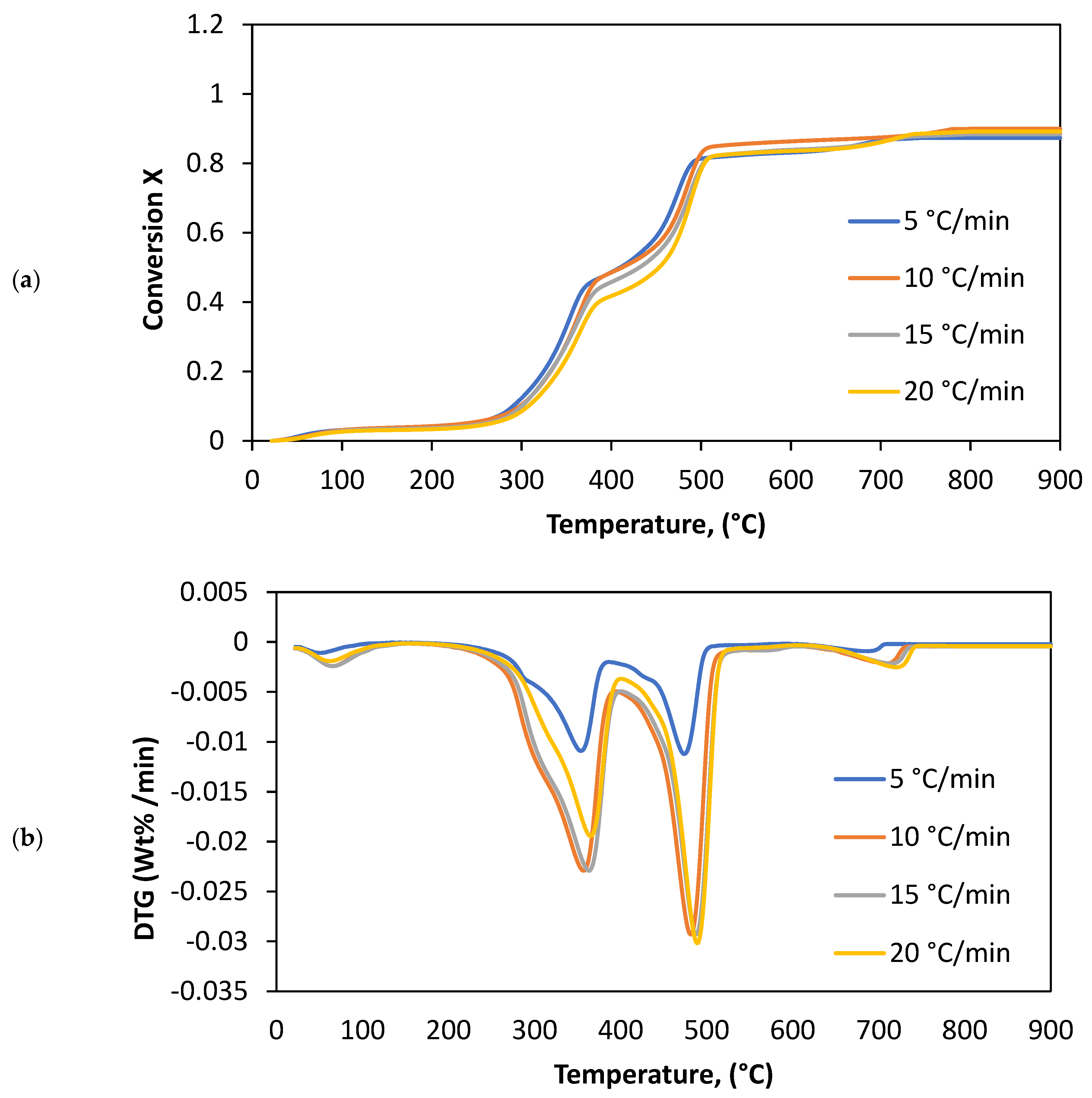
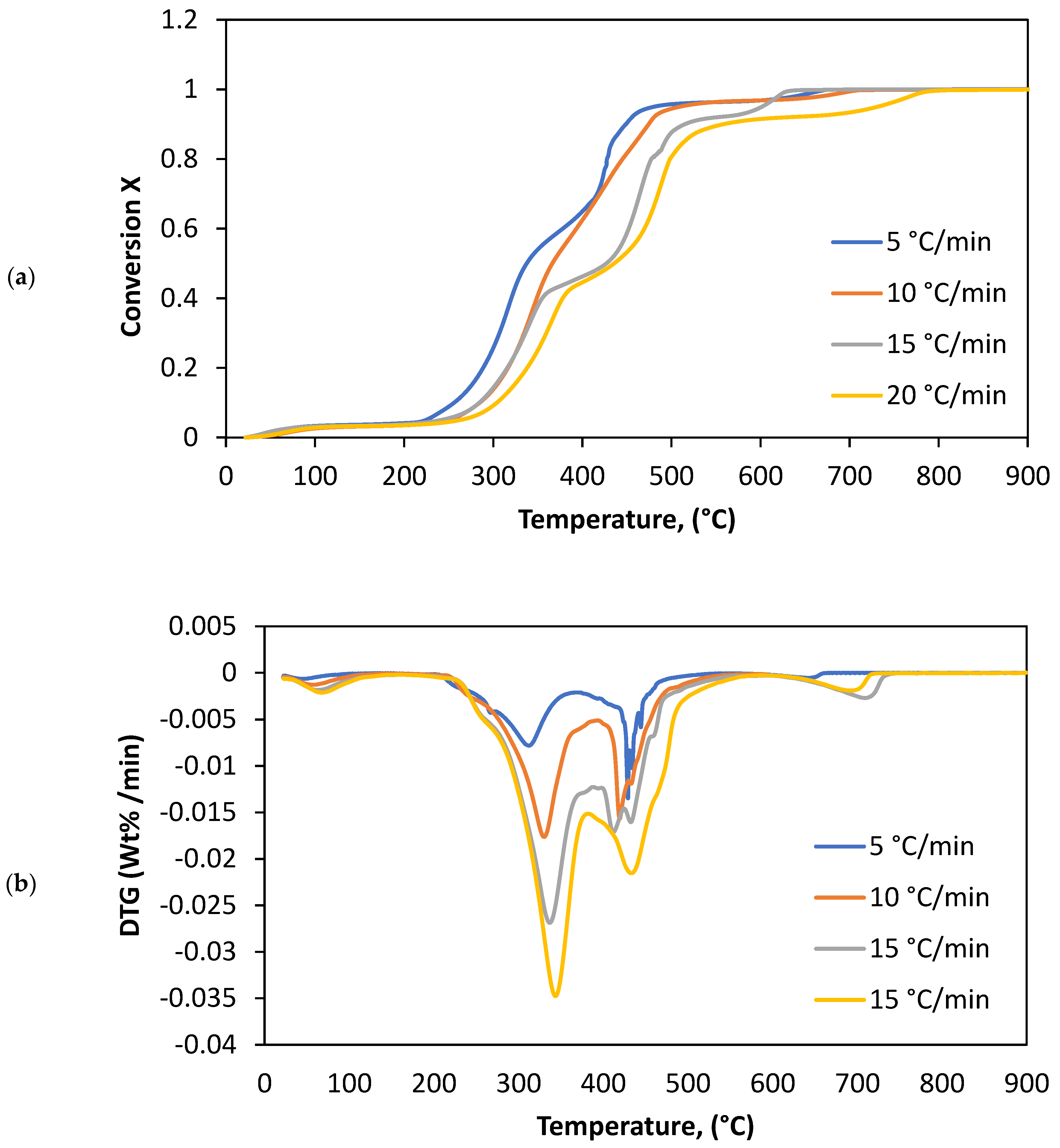
3.1. Thermal Parameters
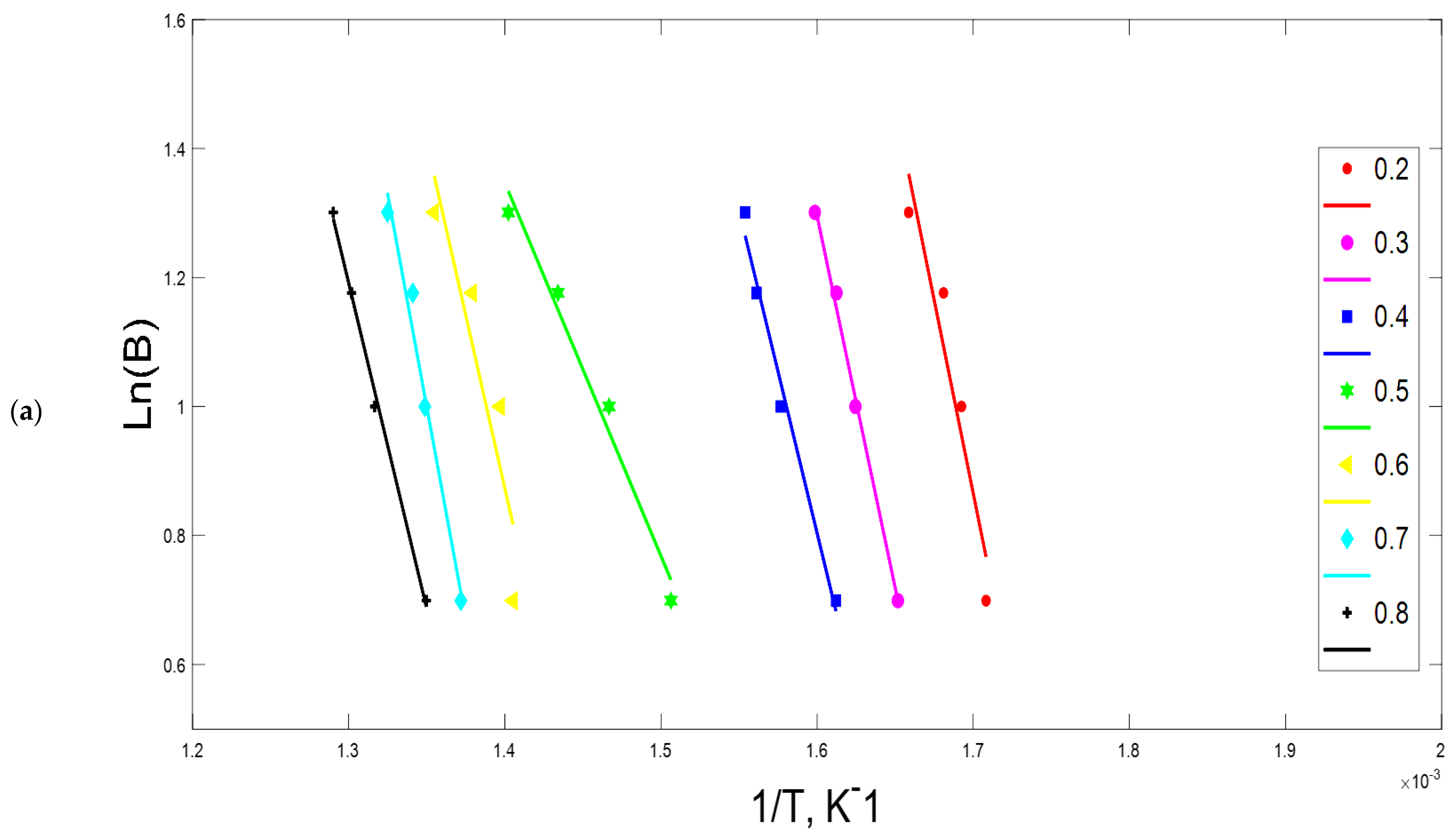
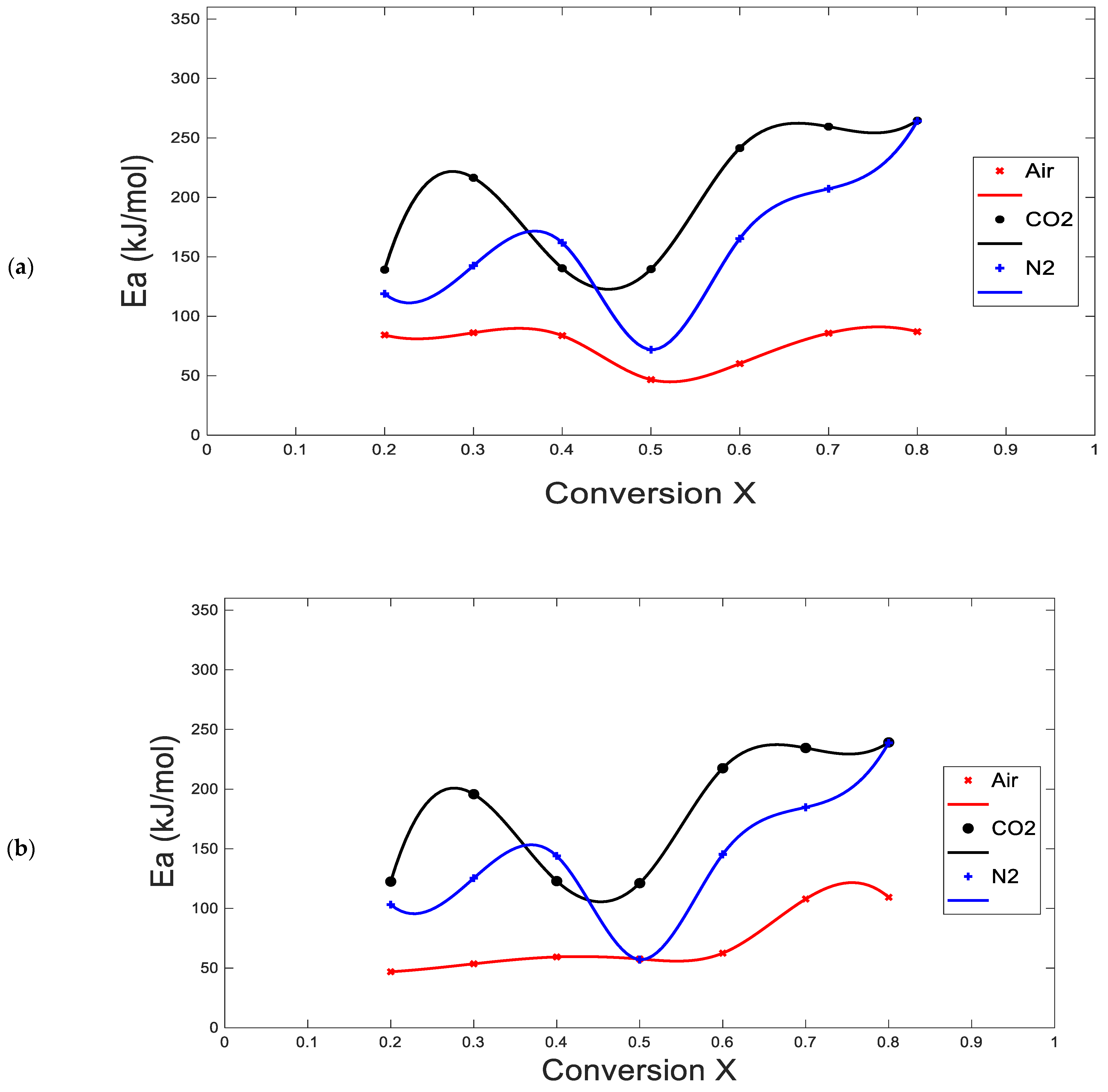
3.2. Kinetic Parameters
4. Discussion
4.1. Thermal Analysis
4.2. Kinetic Analysis
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Activation energy (kJ/mol) | |
| Pre-exponential factor (min−1) | |
| Conversion | |
| trademark | |
| Rate constant of reaction | |
| Initial mass of sample (mg) | |
| Instantaneous mass of sample (mg) | |
| Absolute temperature (K) | |
| Maximum peak temperature (°C) | |
| β | Heating rate (°C/min) |
| Universal gas constant (kJ/mol.K) | |
| Temperature (°C) | |
| Final residual mass of sample (mg) | |
| Time (min) |
References
- Kweku, D.W.; Bismark, O.; Maxwell, A.; Desmond, K.A.; Danso, K.B.; Oti-Mensah, E.A.; Quachie, A.T.; Adormaa, B.B.; Koomson, D. Greenhouse Effect: Greenhouse Gases and Their Impact on Global Warming. J. Sci. Res. Rep. 2018, 17, 1–9. [Google Scholar] [CrossRef]
- Hassanpouryouzband, A.; Joonaki, E.; Edlmann, K.; Haszeldine, R.S. Offshore Geological Storage of Hydrogen: Is This Our Best Option to Achieve Net-Zero? ACS Energy Lett. 2021, 6, 2181–2186. [Google Scholar] [CrossRef]
- Ahmad, T.; Zhang, D. A critical review of comparative global historical energy consumption and future demand: The story told so far. Energy Rep. 2020, 6, 1973–1991. [Google Scholar] [CrossRef]
- Rahman, M.; Rahman, S.; Rahman, M.; Hasan, M.; Shoaib, S.; Rushd, S. Greenhouse Gas Emissions from Solid Waste Management in Saudi Arabia—Analysis of Growth Dynamics and Mitigation Opportunities. Appl. Sci. 2021, 11, 1737. [Google Scholar] [CrossRef]
- De la Barrera, B.; Hooda, P. Greenhouse gas emissions of waste management processes and options: A case study. Waste Manag. Res. 2016, 34, 658–665. [Google Scholar] [CrossRef]
- Klass, D.L. Biomass for Renewable Energy, Fuels, and Chemicals; Academic Press: San Diego, CA, USA, 1998. [Google Scholar]
- Saidur, R.; Abdelaziz, E.; Demirbas, A.; Hossain, M.; Mekhilef, S. A review on biomass as a fuel for boilers. Renew. Sustain. Energy Rev. 2011, 15, 2262–2289. [Google Scholar] [CrossRef]
- Adhikari, S.; Nam, H.; Chakraborty, J.P. Conversion of Solid Wastes to Fuels and Chemicals Through Pyrolysis. Waste Biorefinery 2018, 239–263. [Google Scholar] [CrossRef]
- Harjanne, A.; Korhonen, J. Abandoning the concept of renewable energy. Energy Policy 2019, 127, 330–340. [Google Scholar] [CrossRef]
- Zafar, S. Municipal Solid Wastes in Qatar|Blogging Hub. 2019. Available online: https://www.cleantechloops.com/municipal-solid-waste-in-qatar/ (accessed on 20 October 2020).
- Diaz-Barriga-Fernandez, A.; Santibañez-Aguilar, J.; González-Campos, J.; Nápoles-Rivera, F.; Ponce-Ortega, J.; El-Halwagi, M. Strategic planning for managing municipal solid wastes with consideration of multiple stakeholders. Comput. Aided Chem. Eng. Elsevier. 2018, 44, 1597–1602. [Google Scholar]
- Alam, O.; Qiao, X. An in-depth review on municipal solid waste management, treatment and disposal in Bangla-desh. Sustain. Cities Soc. 2020, 52, 101775. [Google Scholar] [CrossRef]
- Syguła, E.; Świechowski, K.; Hejna, M.; Kunaszyk, I.; Białowiec, A. Municipal Solid Waste Thermal Analysis—Pyrolysis Kinetics and Decomposition Reactions. Energies 2021, 14, 4510. [Google Scholar] [CrossRef]
- Wang, Y.; Kinoshita, C. Kinetic model of biomass gasification. Sol. Energy 1993, 51, 19–25. [Google Scholar] [CrossRef]
- Arshad, M.A.; Maaroufi, A.; Benavente, R.; Pinto, G. Kinetics of the Thermal Decomposition Mechanisms of Con-ducting and Non-conducting epoxy/Al Composites. J. Mater. Environ. Sci 2014, 5, 1342–1354. [Google Scholar]
- Nakamura, S. Fundamentals of Chemical Reaction Kinetics. Sol. Chem. Energy Convers. Theory Appl. Cham Springer Int. Publ. 2016. Available online: https://link.springer.com/chapter/10.1007/978-3-319-25400-5_4 (accessed on 12 May 2021).
- Mamleev, V.; Bourbigot, S.; Le Bras, M.; Lefebvre, J. Three model-free methods for calculation of activation energy in TG. J. Therm. Anal. Calorim. 2004, 78, 1009–1027. [Google Scholar] [CrossRef]
- Slopiecka, K.; Bartocci, P.; Fantozzi, F. Thermogravimetric analysis and kinetic study of poplar wood pyrolysis. Appl. Energy 2012, 97, 491–497. [Google Scholar] [CrossRef]
- Benhacine, F.; Yahiaoui, F.; Hadj-Hamou, A. Thermal stability and kinetic study of isotactic polypropyl-ene/Algerian bentonite nanocomposites prepared via melt blending. J. Polym. 2014, 2014, 1–9. [Google Scholar] [CrossRef]
- Cui, H.W.; Jiu, J.; Sugahara, T.; Nagao, S.; Suganuma, K.; Uchida, H.; Schroder, K. Using the Friedman method to study the thermal degradation kinetics of photonically cured electrical-ly conductive adhesives. J. Therm. Anal. Calorim. 2015, 119, 425–433. [Google Scholar] [CrossRef]
- Nelson, J. Determination of Kinetic Parameters of Six Ablation Polymers by Thermogravimetric Analysis. 1967. Available online: https://apps.dtic.mil/sti/citations/ADA306600 (accessed on 12 May 2021).
- Kessler, M.R.; White, S.R. Cure kinetics of the ring-opening metathesis polymerization of dicyclopentadiene. J. Polym. Sci. Part A: Polym. Chem. 2002, 40, 2373–2383. [Google Scholar] [CrossRef]
- Li, A.; Gao, N.; Quan, C. Thermogravimetric analysis and kinetic study on large particles of printed circuit board wastes Furfural clean production View project MSW Gasification View project Thermogravimetric analysis and kinetic study on large particles of printed circuit board wastes. Waste Manag. 2009, 29, 2353–2360. [Google Scholar]
- Morais, L.C.; Maia, A.A.D.; Guandique, M.E.G.; Rosa, A.H. Pyrolysis and Combustion of Sugarcane Bagasse; Springer: Berlin/Heidelberg, Germany, 2017; Volume 129, pp. 1813–1822. [Google Scholar]
- Gerassimidou, S.; Velis, A.C.; Williams, P.T.; Komilis, D. Characterisation and composition identification of waste-derived fuels obtained from municipal solid waste using thermogravimetry: A review. Waste Manag. Res. 2020, 38, 942–965. [Google Scholar] [CrossRef]
- Guida, M.Y.; Lanaya, S.; Rbihi, Z.; Hannioui, A. Thermal degradation behaviors of sawdust wood waste: Pyrolysis kinetic and mechanism. J. Mater. Environ. Sci. 2019, 10, 742–755. [Google Scholar]
- Singh, S.; Wu, C.; Williams, P. Pyrolysis of waste materials using TGA-MS and TGA-FTIR as complementary characterisation techniques. J. Anal. Appl. Pyrolysis 2012, 94, 99–107. [Google Scholar] [CrossRef]
- Chen, S.; Meng, A.; Long, Y.; Zhou, H.; Li, Q.; Zhang, Y. TGA pyrolysis and gasification of combustible municipal solid waste. J. Energy Inst. 2015, 88, 332–343. [Google Scholar] [CrossRef]
- Azam, M.; Setoodeh Jahromy, S.; Raza, W.; Jordan, C.; Harasek, M.; Winter, F. Comparison of the combustion characteristics and kinetic study of coal, municipal solid waste, and refuse-derived fuel: Model-fitting methods. Wiley Online Libr. 2019, 7, 2646–2657. [Google Scholar] [CrossRef]
- Szűcs, T.; Szentannai, P.; Szilágyi, I.M.; Bakos, L.P. Comparing different reaction models for combustion kinetics of solid recovered fuel. J. Therm. Anal. Calorim. 2019, 139, 555–565. [Google Scholar] [CrossRef]
- Miskolczi, N.; Buyong, F. Thermogravimetric analysis and pyrolysis kinetic study of Malaysian refuse derived fuels Production of methane gas from biomass by using anaerobic digestion View project FACE Project UKM View pro-ject. Taylor Fr. 2010, 83, 125–132. [Google Scholar]
- Liu, Z.; Jiang, Z.; Fei, B.; Liu, X.E. Thermal decomposition of Chinese fir. BioResources 2013, 8, 2013. [Google Scholar] [CrossRef][Green Version]
- Zan, R.; Wang, W.; Xu, R.; Schenk, J.; Zheng, H.; Wang, H. Gasification Characteristics and Kinetics of Unburned Pulverized Coal in Blast Furnaces. Energies 2019, 12, 4324. [Google Scholar] [CrossRef]
- Jess, A.; Andresen, A.-K. Influence of mass transfer on thermogravimetric analysis of combustion and gasification reactivity of coke. Fuel 2010, 89, 1541–1548. [Google Scholar] [CrossRef]
- Jayaraman, K.; Gokalp, I.; Bonifaci, E.; Merlo, N. Kinetics of steam and CO 2 gasification of high ash coal–char produced under various heating rates. Fuel 2015, 154, 370–379. [Google Scholar] [CrossRef]
- Gu, X.; Zhou, X.; Wu, M.; Wang, X.; Chen, Y.; Cheng, K. Kinetic Study of Pine Sawdust Pyrolysis via TG/DTG Analysis. Cellulose Chem. Technol. 2017, 51, 387–394. [Google Scholar]
- Nisar, J.; Khan, M.A.; Iqbal, M.; Shah, A.; Khan, R.A.; Sayed, M.; Mahmood, T. Comparative Study of Kinetics of the Thermal Decomposition of Polypropylene Using Different Methods. Adv. Polym. Technol. 2018, 37, 1168–1175. [Google Scholar] [CrossRef]
- Chew, J.J.; Soh, M.; Sunarso, J.; Yong, S.-T.; Doshi, V.; Bhattacharya, S. Isothermal kinetic study of CO2 gasification of torrefied oil palm biomass. Biomass Bioenergy 2020, 134, 105487. [Google Scholar] [CrossRef]
- Muravyev, N.V.; Pivkina, A.N.; Koga, N. Critical Appraisal of Kinetic Calculation Methods Applied to Overlapping Multistep Reactions. Molecules 2019, 24, 2298. [Google Scholar] [CrossRef]
- Wong, F.F.; Lin, C.M.; Chen, K.-L.; Shen, Y.-H.; Huang, J.-J. Improvement of the thermal latency for epoxy-phenolic resins by novel amphiphatic imidazole catalysts. Macromol. Res. 2010, 18, 324–330. [Google Scholar] [CrossRef]
- Government Communications Office. Qatar National Vision 2030. Fourth Pillar Environ. Dev. 2020, 209–230. [Google Scholar]
| Proximate Analysis (wt, %) | Ultimate Analysis (wt, %) | ||
|---|---|---|---|
| Moisture content | 3.1 ± 0.4 | C | 45.7 ± 2.7 |
| Ash | 11.8 ± 0.5 | H | 6.4 ± 0.2 |
| Volatile matter content | 77.6 ± 1.4 | S | 0.2 ± 0.06 |
| Fixed carbon | 7.5 ± 1.4 | N | 0.6 ± 0.2 |
| Gross calorific value, (MJ/kg) | 23.1 ± 2.8 | O (by difference) | 35.3 ± 1.9 |
| Density, kg/m3 | 450 | ||
| First Stage | Second Stage | Third Stage | Fourth Stage | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| β | Start * | End ** | Weight | Start * | End ** | Weight | Start * | End ** | Weight | Start * | End ** | Weight |
| °C/min | (°C) | (°C) | Loss (%) | (°C) | (°C) | Loss (%) | (°C) | (°C) | Loss (%) | (°C) | (°C) | Loss (%) |
| 5 | 28.2 | 156.9 | 3.6 | 233.5 | 376.3 | 34.2 | 376.3 | 471.4 | 41.4 | 636.6 | 688.1 | 11.2 |
| 10 | 28.8 | 156.5 | 4.7 | 233.5 | 378.6 | 35.6 | 378.6 | 493.8 | 42.6 | 638.6 | 693.7 | 11.4 |
| 15 | 28.1 | 157.3 | 4.9 | 233.5 | 395.6 | 37.6 | 395.6 | 510.4 | 45.7 | 640.5 | 712.4 | 9.2 |
| 20 | 27.7 | 158.9 | 5.2 | 233.5 | 426.7 | 39.6 | 426.7 | 522.2 | 48.5 | 641.6 | 732.4 | 4.7 |
| Average | 28.2 | 157.4 | 4.6 | 233.5 | 394.3 | 36.8 | 394.3 | 499.5 | 44.3 | 639.3 | 706.7 | 9.1 |
| First Stage | Second Stage | Third Stage | Fourth Stage | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| β | Start * | End ** | Weight | Start * | End ** | Weight | Start * | End ** | Weight | Start * | End ** | Weight |
| °C/min | (°C) | (°C) | Loss (%) | (°C) | (°C) | Loss (%) | (°C) | (°C) | Loss (%) | (°C) | (°C) | Loss (%) |
| 5 | 28.6 | 167.6 | 3.4 | 199.2 | 380.4 | 34.2 | 380.4 | 483.4 | 51.2 | 622.2 | 658.3 | 3.8 |
| 10 | 28.5 | 166.2 | 3.6 | 199.2 | 410.3 | 34.6 | 410.3 | 489.6 | 50 | 622.2 | 692.2 | 3.9 |
| 15 | 28.4 | 164.4 | 3.8 | 199.2 | 412.2 | 37.3 | 412.2 | 550.6 | 41.6 | 622.2 | 708.9 | 4.4 |
| 20 | 28.7 | 163.8 | 3.9 | 198.3 | 428.6 | 38.5 | 428.6 | 558.4 | 42.3 | 623.4 | 792.7 | 5.5 |
| Average | 28.6 | 165.5 | 3.7 | 198.9 | 407.9 | 36.2 | 407.9 | 520.5 | 46.3 | 622.5 | 713.0 | 4.4 |
| First Stage | Second Stage | Third Stage | Fourth Stage | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Β °C/min | Start * (°C) | End ** (°C) | Weight Loss (%) | Start * (°C) | End ** (°C) | Weight Loss (%) | Start * (°C) | End ** (°C) | Weight Loss (%) | Start * (°C) | End ** (°C) | Weight Loss (%) |
| 5 | 28.4 | 140.3 | 4.3 | 214.2 | 332.5 | 33.4 | 332.5 | 478.6 | 52.7 | 615.5 | 655.3 | 3.2 |
| 10 | 28.2 | 150.4 | 4.6 | 214.2 | 347.5 | 34.3 | 347.5 | 524.6 | 52.1 | 615.5 | 690.2 | 3.7 |
| 15 | 28.6 | 150.2 | 4.4 | 214.3 | 358.3 | 35.3 | 358.3 | 534.5 | 42.3 | 616.3 | 705.9 | 4.5 |
| 20 | 28.2 | 160.4 | 5.3 | 214.5 | 385.1 | 38.9 | 385.1 | 546.2 | 42.6 | 617.5 | 745.8 | 5.4 |
| Average | 28.3 | 150.3 | 4.7 | 214.3 | 355.9 | 35.5 | 335.9 | 521 | 47.4 | 616.2 | 699.3 | 4.2 |
| N2 | CO2 | Air | ||||
|---|---|---|---|---|---|---|
| β °C/min | Tm | DTGTm | Tm | DTGTm | Tm | DTGTm |
| °C | wt %/min | °C | wt %/min | °C | wt %/min | |
| 5 | 471 ± 7.0 | −0.0094 ± 0.0014 | 466 ± 5 | −0.0097 ± 0.0014 | 317 ± 4.2 | −0.0071 ± 0.0009 |
| 10 | 481 ± 6.7 | −0.019 ± 0.0057 | 474 ± 6 | −0.019 ± 0.0079 | 335 ± 13.4 | −0.015 ± 0.0024 |
| 15 | 486 ± 4.8 | −0.021 ± 0.0056 | 482 ± 5 | −0.029 ± 0.0073 | 336 ± 2.0 | −0.025 ± 0.0027 |
| 20 | 487 ± 1.1 | −0.032 ± 0.006 | 488 ± 1.2 | −0.032 ± 0.002 | 340.3 ± 10.5 | −0.016 ± 0.0082 |
| N2 | CO2 | Air | |||||||
|---|---|---|---|---|---|---|---|---|---|
| / | (kJ/mol) | / | (min−1) | (kJ/mol) | / | (min−1) | (kJ/mol) | / | (min−1) |
| 0.2 | 118.9 | 0.802 | 2.7 × 109 | 139.2 | 0.988 | 1.5 × 1011 | 84.6 | 0.993 | 2.7 × 106 |
| 0.3 | 142.5 | 0.995 | 1.6 × 1011 | 216.5 | 0.977 | 6.4 × 1010 | 86.8 | 0.993 | 2.2 × 106 |
| 0.4 | 161.9 | 0.996 | 2.7 × 1013 | 140.4 | 0.959 | 5.1 × 1010 | 80.0 | 0.957 | 2.2 × 106 |
| 0.5 | 71.9 | 0.966 | 6.9 × 104 | 139.6 | 0.934 | 4.1 × 109 | 52.8 | 0.981 | 2.0 × 106 |
| 0.6 | 165.4 | 0.970 | 2.7 × 1011 | 241.4 | 0.968 | 1.4 × 109 | 54.2 | 0.951 | 1.0 × 106 |
| 0.7 | 207.2 | 0.857 | 2.4 × 1014 | 259.5 | 0.967 | 1.1 × 109 | 81.1 | 0.934 | 4.6 × 105 |
| 0.8 | 264.2 | 0.931 | 2.4 × 1018 | 264.6 | 0.981 | 9.5 × 108 | 97.7 | 0.991 | 3.5 × 105 |
| Average | 161.7 | 0.931 | 3.4 × 1017 | 200.2 | 0.968 | 4.0 × 1010 | 76.7 | 0.971 | 1.6 × 106 |
| 161.7 ± 24.7 | 200.2 ± 33.6 | 76.7 ± 15.4 | |||||||
| N2 | CO2 | Air | |||||||
|---|---|---|---|---|---|---|---|---|---|
| / | (kJ/mol) | / | (min−1) | (kJ/mol) | / | (min−1) | (kJ/mol) | / | (min−1) |
| 0.2 | 103.2 | 0.771 | 1.8 × 108 | 122.5 | 0.986 | 4.8 × 107 | 46.9 | 0.957 | 9.9 × 102 |
| 0.3 | 125.3 | 0.994 | 2.0 × 1010 | 195.7 | 0.974 | 1.1 × 1013 | 53.6 | 0.958 | 4.3 × 103 |
| 0.4 | 143.9 | 0.996 | 9.8 × 1011 | 122.9 | 0.952 | 5.2 × 107 | 59.3 | 0.977 | 1.5 × 104 |
| 0.5 | 57.1 | 0.951 | 9.4 × 103 | 121.1 | 0.922 | 3.8 × 107 | 57.6 | 0.918 | 1.1 × 104 |
| 0.6 | 145.3 | 0.965 | 1.3 × 1012 | 217.4 | 0.964 | 3.9 × 1014 | 62.5 | 0.914 | 3.0 × 104 |
| 0.7 | 184.7 | 0.840 | 4.9 × 1015 | 234.8 | 0.963 | 6.6 × 1015 | 107.9 | 0.856 | 5.0 × 108 |
| 0.8 | 238.8 | 0.924 | 3.7 × 1020 | 239.5 | 0.979 | 1.4 × 1016 | 109.3 | 0.927 | 6.8 × 108 |
| Average | 142.6 | 0.920 | 5.3 × 1019 | 179.6 | 0.963 | 3.1 × 1015 | 71.0 | 0.925 | 1.7 × 108 |
| 142.6 ± 23.5 | 179.0 ± 31.9 | 71.0 ± 4.4 | |||||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Al-Moftah, A.M.S.H.; Marsh, R.; Steer, J. Thermal Decomposition Kinetic Study of Non-Recyclable Paper and Plastic Waste by Thermogravimetric Analysis. ChemEngineering 2021, 5, 54. https://doi.org/10.3390/chemengineering5030054
Al-Moftah AMSH, Marsh R, Steer J. Thermal Decomposition Kinetic Study of Non-Recyclable Paper and Plastic Waste by Thermogravimetric Analysis. ChemEngineering. 2021; 5(3):54. https://doi.org/10.3390/chemengineering5030054
Chicago/Turabian StyleAl-Moftah, Ahmad Mohamed S. H., Richard Marsh, and Julian Steer. 2021. "Thermal Decomposition Kinetic Study of Non-Recyclable Paper and Plastic Waste by Thermogravimetric Analysis" ChemEngineering 5, no. 3: 54. https://doi.org/10.3390/chemengineering5030054
APA StyleAl-Moftah, A. M. S. H., Marsh, R., & Steer, J. (2021). Thermal Decomposition Kinetic Study of Non-Recyclable Paper and Plastic Waste by Thermogravimetric Analysis. ChemEngineering, 5(3), 54. https://doi.org/10.3390/chemengineering5030054






