A Semi-Empirical Method for the Estimation of the Hydration Number of Mn(II)-Complexes

Abstract

A semi-empirical equation to estimate the hydration number of Mn(II) complexes was derived from a database of 49 previously published ¹H longitudinal Nuclear Magnetic Relaxation Dispersion profiles. This equation has the longitudinal ¹H relaxivity and the molecular weight of the Mn(II) complex under consideration as parameters.

1. Introduction

During the last decades, Gd³⁺-chelates, such as Gd-DTPA (DTPA = diethylenetriamine-N,N,N’,N’’,N’’,N’’’-pentaacetate) and Gd-DOTA (DOTA = 1,4,7,10-tetraazacyclododecane-N,N’,N’’,N’’’-tetraacetate), have become indispensable tools for the contrast enhancement of magnetic resonance images [1,2,3]. These contrast agents (CAs) have proven to be generally extremely safe; only 0.03% of all administrations (about 100 million worldwide) gave rise to serious adverse effects. However, during recent years, some concerns have arisen because of (i) incidents of nephrogenic systemic fibrosis (NSF) associated with Gd³⁺-complexes of linear DTPA derivates and (ii) observation of Gd-accumulation in the brains of patients with normal renal function, after repeated administrations of DTPA-type of CAs [4,5,6,7,8]. Both effects may be ascribed to the relative kinetic instability of Gd³⁺ complexes of linear polyaminocarboxylates. The concerns about Gd-based CAs have led to an increased interest in application of Gd³⁺-free CAs. High spin d⁵ Mn²⁺ is an attractive alternative for f⁷ Gd³⁺, because it has only two less unpaired electrons, whereas a high electronic symmetry is favorable for high relaxivities. Moreover, Mn²⁺ is less toxic than Gd³⁺, as reflected in its important role as a cofactor in many enzymatic reactions, including the anti-oxidant enzyme superoxide dismutase, as well as in enzymes involved in neurotransmitter synthesis and metabolism in the brain. However, the concentration of free Mn²⁺ in organisms is very low, for instance 0.3–1 μg·L⁻¹ in human blood [9]. High concentrations are neurotoxic, and therefore, Mn²⁺ preferably needs to be sequestered for safe application as CA in humans. Due to its lower charge, complexes are usually less stable than their Gd³⁺-counterparts. It is important to assure that the dissociation of Mn²⁺-based CAs is minimized in order to avoid any neurotoxic side effects. On the other hand, the relaxation rate enhancing efficacy should be as high as possible in view of the inherently low sensitivity of magnetic resonance imaging (MRI) CAs. Finding a balance between kinetic stability and optimal sensitivity is a challenge during the design of novel Mn²⁺-based CAs.

The efficacy of a CA is usually expressed by its relaxivity, the longitudinal, or transverse relaxation rate enhancement normalized for a solution containing 1 mM of paramagnetic metal ions (r₁ and r₂, respectively). One of the most important parameters governing the longitudinal relaxivity (r₁) of paramagnetic CAs is the number of water molecules in the first coordination sphere of the metal ion (q), since the inner sphere contribution to r₁ is linearly proportional to it. Several good methods are available to evaluate q for Gd³⁺-based CAs, including measurements of lanthanide induced ¹⁷O NMR shifts (NMR = Nuclear Magnetic Resonance) of water [1,10,11], determination of the ¹⁷O scalar coupling constant (A_O/ħ) from simultaneous fitting of ¹⁷O shift and/or relaxation data and ¹H NMRD data (NMRD = Nuclear Magnetic Relaxation Dispersion), and comparison of the luminescence decay rates of Eu³⁺ or Tb³⁺ complexes in H₂O and D₂O [12]. The determination of q for Mn²⁺-complexes is more challenging. The luminescence decay method is impossible, because the complexes are not luminescent. ¹⁷O NMR methods are more ambiguous since they rely on the assumption that A_O/ħ is almost independent on the coordination environment of Mn²⁺, which appears not to be case: values in the range −(26–73) × 10⁶ rad·s⁻¹ have been evaluated from ¹⁷O NMR measurements on Mn²⁺ complexes (see below,

Table 1. Comparison of q_lit with q_ref as calculated with Equations (11) and (12) for the database of Mn²⁺ complexes. Other parameters of relevance for the relaxivity at 298 K and hyperfine coupling constants reported in the literature are included.

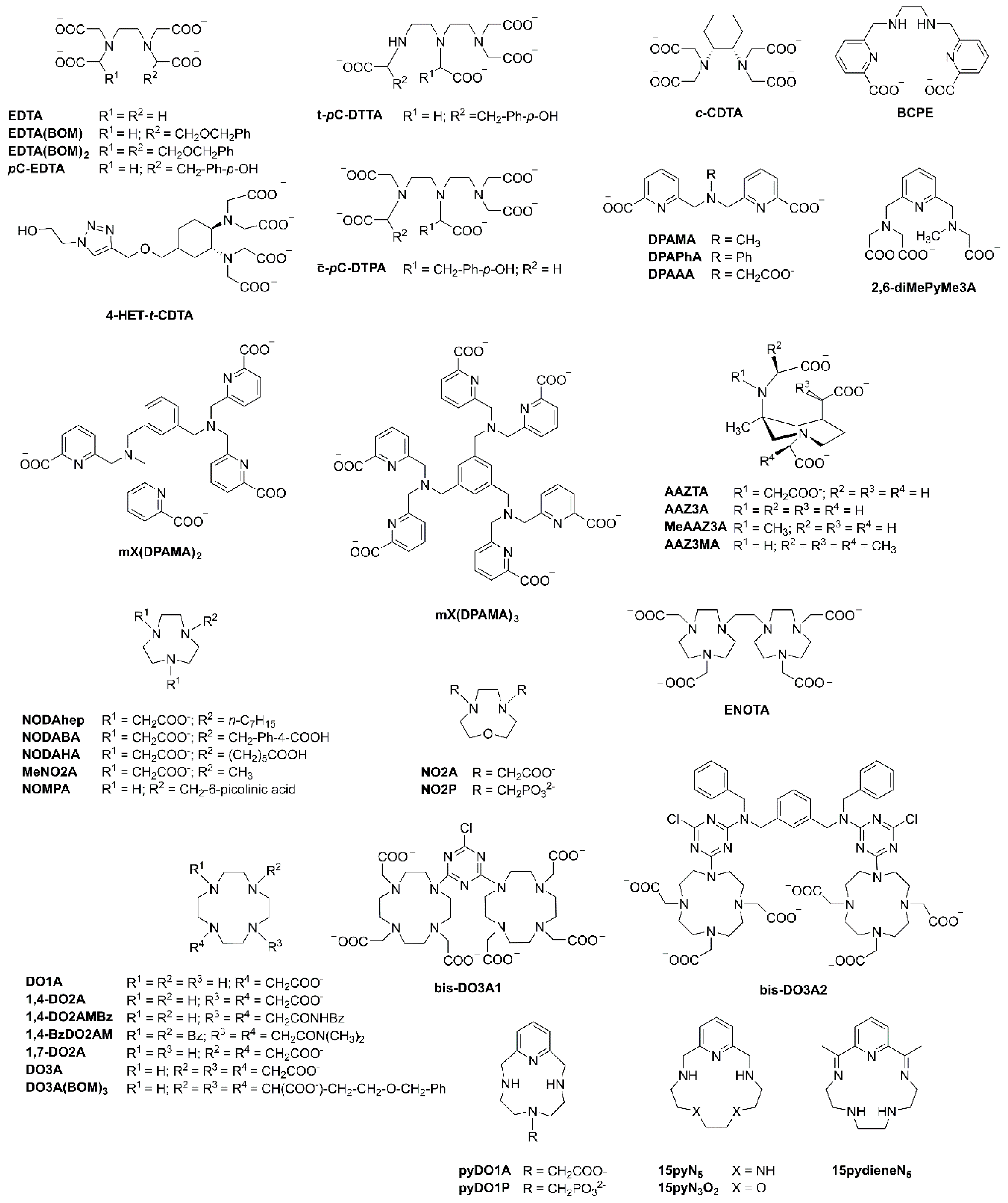
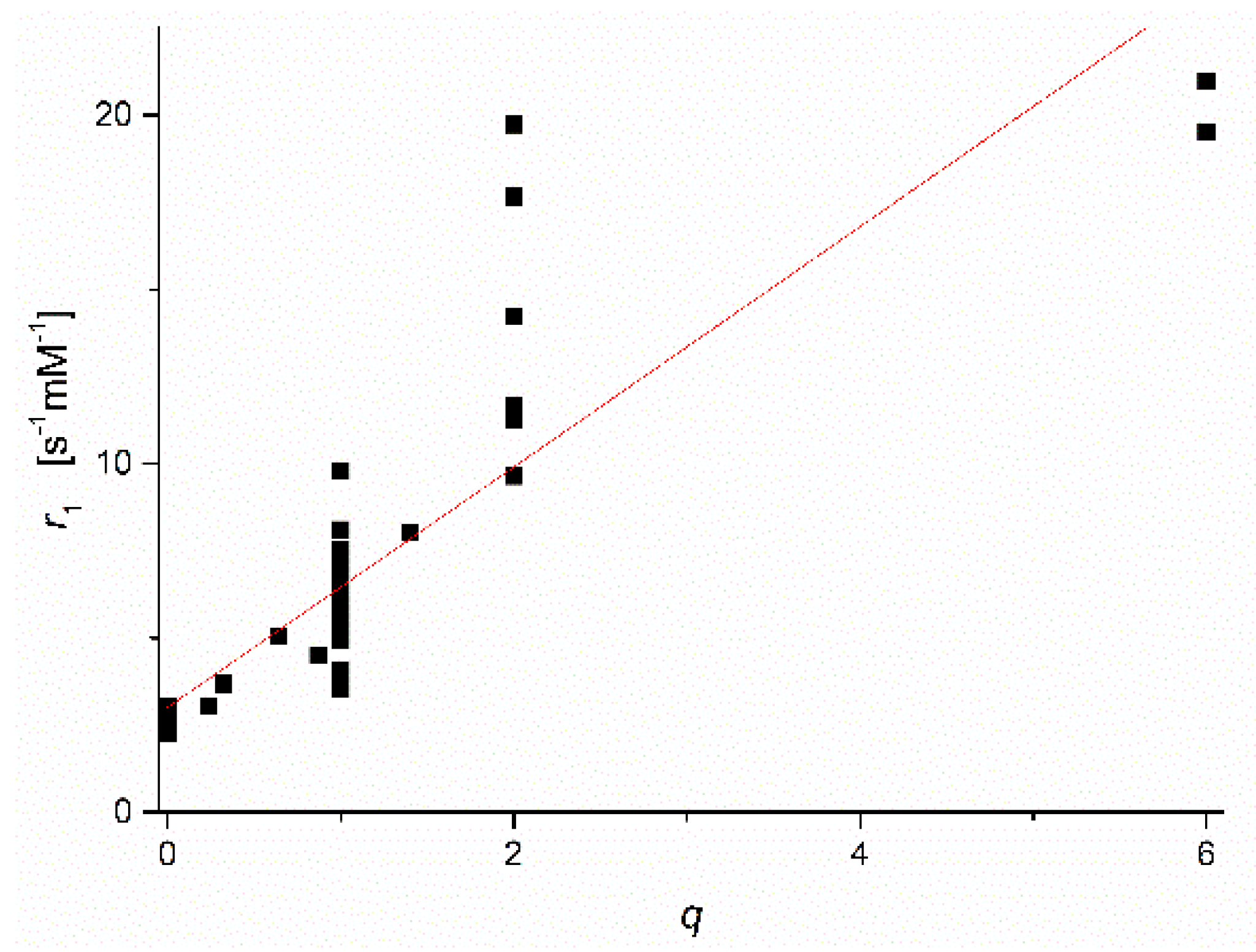
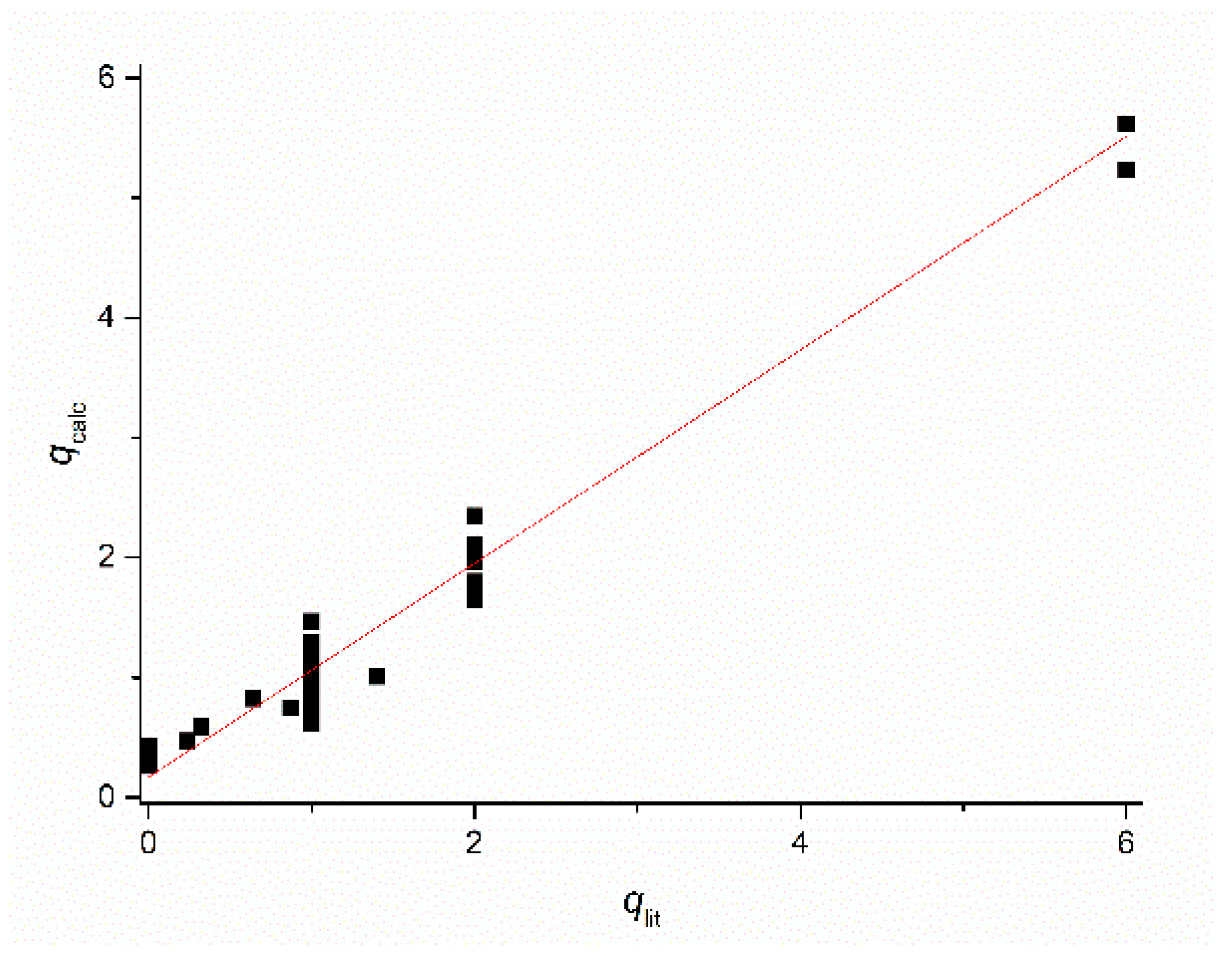
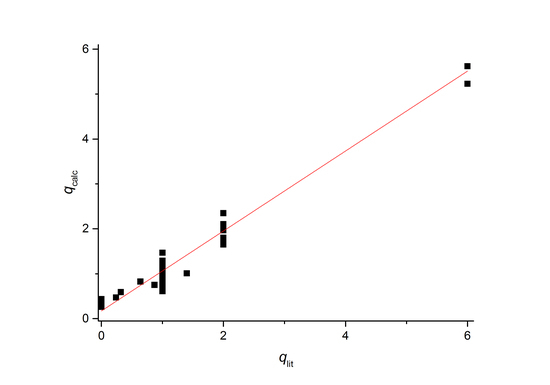
Organic Ligand	qlita	r1b (s−1·mM−1)	FW	qcalcc	τRd (ps)	τS0d (ps)	τMd (ns)	RMnHd (Å)	|AO/ħ| d (106 rad/s)	Ref.
DOTA	0.0	2.76	455.3	0.4	-	-	-	-	-	[28]
DTPA	0.0	2.40	390.2	0.4	-	-	-	-	-	[29]
DTPA	0.0	2.30	390.2	0.4	-	-	-	-	-	[30]
1,7-DO2A	0.0	2.53	341.3	0.4	-	152	-	-	-	[31]
NOTA	0.0	2.30	356.2	0.4	-	-	-	-	-	[28]
BCPE	0.0	2.23	383.3	0.4	-	87	-	-	-	[32]
DO3A	0.0	2.27	398.3	0.4	-	117	-	-	-	[31]
AAZTA	0.0	2.53	412.3	0.4	-	148	-	-	-	[33]
c-pC-DTPA	0.0	3.02	492.4	0.4	-	161	-	-	-	[34]
t-pC-DTTA	0.0	2.55	510.4	0.4	-	108	-	-	-	[34]
DO3A(BOM)3	0.0	2.55	758.7	0.3	-	135	-	-	-	[35]
bis-DO3A1	0.0	2.27	837.8	0.3	-	93	-	-	-	[36]
bis-DO3A2	0.0	2.48	1340.3	0.3	-	137	-	-	-	[36]
AAZ3MA	0.2	3.04	415.3	0.5	51.0	96	7.52	2.81	8.7	[33]
MeAAZ3A	0.3	3.68	387.3	0.6	50.0	140	7.94	2.81	7.9	[33]
AAZ3A	0.6	5.05	373.3	0.8	50.0	158	21.3	2.81	7.2	[33]
1,4-DO2A	0.9	4.50	359.3	0.7	46.0	74	88.2	2.83	43.0	[31]
DO1A	1.0	4.04	302.3	0.7	22.0	88	0.168	2.83	39.4	[31]
MeNO2A	1.0	4.96	330.2	0.9	36.0	101	1.60	2.77	46.0	[37]
pyDO1A	1.0	3.54	336.3	0.6	23.0	449	0.330	-	-	[38]
NOMPA	1.0	6.21	336.3	1.1	51.2	129	0.361	2.77	−73.3	[39]
EDTA	1.0	5.41	361.2	0.9	56.0	81	2.12	2.83	40.5	[31]
EDTA	1.0	5.41	361.2	0.9	57.0	81	2.12	2.83	40.5	[31]
EDTA	1.0	5.81	361.2	1.0	-	-	-	-	-	[30]
EDTA	1.0	5.60	361.2	0.9	-	-	-	-	-	[29]
pyDO1P	1.0	3.84	371.2	0.6	38.6	36	0.565	-	39.9	[38]
NO2P	1.0	8.03	387.1	1.3	103.0	87	83.333	2.75	33.3	[40]
2,6-diMePyMe3A	1.0	5.23	395.2	0.8	46.0	52	0.357	2.83	26.4	[41]
NODAHep	1.0	8.10 g	414.4	1.3	84.0	37	370	2.75	30.0	[42]
DPAAA	1.0	6.75	415.2	1.0	47.6	146	7.94	2.76	31.5	[43]
c-CDTA	1.0	6.12	415.3	0.9	74.0	78	4.44	2.83	42.7	[44]
NODAHA	1.0	7.51 g	429.3	1.1	80.0	32	370	2.75	30.0	[42]
NODABA	1.0	9.80 g	449.3	1.5	121.0	37	769	2.75	30.0	[42]
t-pC-EDTA	1.0	5.93	467.3	0.9	75.4	80	-	2.92	-	[34]
EDTA(BOM)	1.0	5.61 e	495.3	0.8	83.7	87	10.7	2.90	-	[35]
1,4-DO2AMBz	1.0	5.67	539.6	0.8	85.0	71	5.71	2.83	33.0	[45]
4-HET-t-CDTA	1.0	6.72	570.4	0.9	104.9	56	5.68	2.83	40.0	[46]
1,4-BzDO2AM	1.0	5.89	595.7	0.8	96.0	60	3.95	2.83	31.0	[45]
EDTA(BOM)2	1.0	7.25 e	629.5	0.9	110.8	84	7.60	2.90	-	[35]
ENOTA	1.0	6.46 f	658.5	0.8	85.0	4317	18.2	2.75	5.2	[23]
NO2A	1.4	5.67	317.2	1.0	22.0	160	0.840	2.75	33.3	[40]
15pyN5	2.0	9.64	340.3	1.7	28.3	8710	14.5	2.81	38.6	[47]
15pyN3O2	2.0	11.52	342.3	2.0	40.3	7174	263	2.81	38.6	[47]
15pydieneN5	2.0	14.20	364.3	2.3	-	-	-	-	-	[30]
DPAMA	2.0	11.25	390.3	1.8	47.8	167	3.27	2.74	-	[32]
DPAPhA	2.0	11.65	452.3	1.7	81.0	87	17.9	2.78	25.0	[43]
mX(DPAMA)2	2.0	17.65	854.6	2.1	95.8	183	32.7	2.74	-	[32]
mX(DPAMA)3	2.0	19.72	1243.8	2.2	136.0	173	32.7	2.74	-	[32]
none	6.0	19.52 h	163.0	5.6	30.0	26042	35.5	2.83	34.6	[13]
none	6.0	20.98 i	163.0	6.0	-	-	-	-	-	[30]

^a As reported in reference. ^b Measured in reported NMRD profile at Larmor frequency (LF) = 0.01 MHz, unless stated otherwise. ^c As calculated with Equations (11) and (12). ^d Reported values evaluated from analysis of Nuclear Magnetic Relaxation Dispersion (NMRD) and ¹⁷O NMRD data. ^e LF = 0.03 MHz. ^f LF =1.14 MHz. ^g LF = 0.02 MHz. ^h LF = 1.00 MHz. ^I LF = 1.35 MHz.

). Esteban-Gómez et al. have estimated by DFT calculations that variations of Mn–O distances and dihedral angles among these complexes may lead to A_O/ħ values ranging between 30 and 58 × 10⁶ rad·s⁻¹ [13]. By contrast, the range of A_O/ħ values observed for Gd³⁺-complexes observed is narrower (−(3.6–4.2) × 10⁶ rad·s⁻¹) [11], allowing reasonable accurate estimations of q using a ¹⁷O NMR shift and/or relaxation rates. Moreover, accurate Mn²⁺-induced shift measurements are difficult because of the very large line broadenings and relatively small induced shifts.

X-ray crystallography may provide structures of Mn²⁺ complexes in the solid state, but these structures are not necessarily the same in solution. Frequently dimeric or oligomeric assemblies occur in crystal structures, which will dissociate upon dissolution in water. Often, a value of q is estimated based on the denticity of the organic ligand and assuming a total Mn²⁺-coordination number of 6. However, high spin Mn²⁺-complexes have almost no ligand stabilization energy, and consequently, do not show a clear preference for a specific coordination number. Others use the magnitudes of the longitudinal relaxivities to estimate q, which is ambiguous as well, as will be demonstrated below. Recently, Póta et al. suggested that DFT calculations (at the M062X/TZVP level) may provide reliable q-values [14]; however, these calculations require excessive amounts of computing time with supercomputers.

Here, we will demonstrate that reliable q values for Mn²⁺-complexes can be obtained from experimental r₁ values at low Larmor frequencies (LF) and the formula weight (FW) of the complexes.

2. Results and Discussion

A database of 49 previously published ¹H longitudinal Nuclear Magnetic Relaxation Dispersion profiles (plots of r₁ versus LF) was constructed (see Table 1 and Figure 1). The relaxivity has an inner-sphere contribution by water molecules exchanging between the first coordination sphere of the metal ion and the bulk (r_i,IS) and an outer-sphere contribution due to water molecules in the bulk that diffuse in the surroundings of the metal ion without being bound to it (r_1,OS) (see Equation (1)). The amount of a Mn²⁺-based CA needed is always very small (in the mM range) and the Mn²⁺-induced chemical shifts of the water protons are negligible. Under those conditions, r_1,IS was related to the longitudinal relaxation time of a Mn²⁺-bound water molecule (T_1M), according to Equation (2) [15,16]:

r₁ = r_1,IS + r_1,OS

(1)

$$r_{1,\mathrm{IS}}=\frac{q}{55556\left(T_{1\mathrm{M}}+{\tau }_{\mathrm{M}}\right)}$$

(2)

Here, τ_M is the residence time of a water molecule in the first coordination sphere of Mn²⁺. Since the electronic relaxation time (T_1e) for Mn²⁺-complexes was generally larger than the rotational correlation time (τ_R), the contribution of the Curie relaxation mechanism to the longitudinal relaxation was negligible; only the dipolar and the scalar mechanisms needed to be taken into consideration. These contributions (T_1M,DD⁻¹ and T_1M,SC⁻¹, respectively) may be estimated by using the Solomon-Bloembergen-Morgan (SBM) equations (Equations (3)–(7)) [17,18]:

$$\frac{1}{T_{1\mathrm{M}}}=\frac{1}{T_{1M,DD}}+\frac{1}{T_{1M,SC}}$$

(3)

$$\frac{1}{T_{1,DD}}=\frac{2}{15}{\left(\frac{{\mu }_0}{4\pi }\right)}^2\frac{{\hslash }^2{\gamma }_{\mathrm{S}}^2{\gamma }_{\mathrm{I}}^2}{r_{\mathrm{MnH}}^6}S\left(S+1\right)\left(\frac{3{\tau }_{\mathrm{d}1}}{1+{\omega }_{\mathrm{I}}^2{\tau }_{\mathrm{d}1}^2}+\frac{7{\tau }_{\mathrm{d}2}}{1+{\omega }_{\mathrm{S}}^2{\tau }_{\mathrm{d}2}^2}\right)$$

(4)

$$\frac{1}{T_{1,SC}}=\frac{S\left(S+1\right)}{3}{\left(\frac{A_H}{ħ}\right)}^2\left(\frac{2{\tau }_{s2}}{1+{\omega }_s^2{\tau }_{s2}^2}\right)$$

(5)

Here, (μ₀/4π) is the magnetic permeability in vacuum, r_MnH is the distance between Mn²⁺ and the H-atom of a bound water molecule, S is the electron spin (S = 5/2 for Mn²⁺), γ_I is the ¹H nuclear gyromagnetic ratio, γ_S is the electron gyromagnetic ratio, and ω_I and ω_S are the Larmor frequencies of the proton and electron spin, respectively, A_H/ħ is the hyperfine coupling constant between Mn²⁺ and the bound water proton, τ_di⁻¹ = τ_M⁻¹ + τ_R⁻¹+ T_ie⁻¹, and τ_s2⁻¹ = τ_M⁻¹ + T_2e⁻¹. The electronic relaxation times are often interpreted in terms of the zero-field splitting (ZFS) interaction using Equations (6) and (7) [19]:

$$\frac{1}{T_{1\mathrm{e}}}=\frac{1}{25}{\Delta }^2{\tau }_{\mathrm{v}}\left[4S\left(S+1\right)-3\right]\left(\frac{1}{1+{\omega }_{\mathrm{s}}^2{\tau }_{\mathrm{v}}^2}+\frac{4}{1+4{\omega }_{\mathrm{s}}^2{\tau }_{\mathrm{v}}^2}\right)$$

(6)

$$\frac{1}{T_{2\mathrm{e}}}=\frac{1}{50}{\Delta }^2{\tau }_{\mathrm{v}}\left[4S\left(S+1\right)-3\right]\left(\frac{5}{1+{\omega }_{\mathrm{s}}^2{\tau }_{\mathrm{v}}^2}+\frac{2}{1+4{\omega }_{\mathrm{s}}^2{\tau }_{\mathrm{v}}^2}+3\right)$$

(7)

In these equations, Δ² represents the mean-squared fluctuation of the ZFS, and τ_v is the correlation time for the instantaneous distortion of the coordination polyhedron of Mn²⁺. From these equations, it can be derived that the low field limiting value of the longitudinal and transverse electronic relaxation rates (τ_S0⁻¹) were equal, given by Equation (8):

$$\frac{1}{{\tau }_{S0}}=\frac{1}{5}{\Delta }^2{\tau }_{\mathrm{v}}\left[4S\left(S+1\right)-3\right]$$

(8)

The outer-sphere contribution to the relaxivity (r_1,OS) is described by Equations (9) and (10) [20]:

$$r_{1,\mathrm{OS}}=\left(\frac{32\pi }{405}\right){\left(\frac{{\mu }_0}{4\pi }\right)}^2{\gamma }_{\mathrm{I}}^2{\gamma }_{\mathrm{S}}^2{\hslash }^{2}S\left(S+1\right)\frac{N_{\mathrm{A}}}{a_{\mathrm{MnH}}{D}_{\mathrm{MnH}}}\left[3J_{\mathrm{OS}}\left({\omega }_{\mathrm{I}},T_{1\mathrm{e}}\right)+7J_{\mathrm{OS}}\left({\omega }_{\mathrm{S}},T_{2\mathrm{e}}\right)\right]$$

(9)

$$J_{\mathrm{OS}}\left(\omega ,T_{\mathrm{je}}\right)=Re\left\{\frac{1+\frac{1}{4}{\left[i\omega {\tau }_{\mathrm{MnH}}+\left(\frac{{\tau }_{\mathrm{MnH}}}{T_{\mathrm{je}}}\right)\right]}^{\frac{1}{2}}}{1+{\left[i\omega {\tau }_{\mathrm{MnH}}+\left(\frac{{\tau }_{\mathrm{MnH}}}{T_{\mathrm{je}}}\right)\right]}^{\frac{1}{2}}+\frac{4}{9}\left[i\omega {\tau }_{\mathrm{MnH}}+\left(\frac{{\tau }_{\mathrm{MNH}}}{T_{\mathrm{je}}}\right)\right]+\frac{1}{9}{\left[i\omega {\tau }_{\mathrm{MnH}}+\left(\frac{{\tau }_{\mathrm{MnH}}}{T_{\mathrm{je}}}\right)\right]}^{\frac{3}{2}}}\right\}$$

(10)

Here, N_A is Avogadro’s number, a_MnH is the distance of closest approach of a diffusing water molecule to Mn²⁺, D_MnH stands for the diffusion coefficient, and J_os(ω, T_je) (j = 1, 2) are spin density functions. The diffusion correlation time (τ_MnH) is given by a_MnH²/D_MnH.

An inspection of Equations (4) and (5) indicates that in an NMRD profile, two inflection points may be expected: one originating from 1/T_1,DD when ωτ_d2 = 1 (at LF ≈ 10 MHz), and another one due to 1/T_1,SC when ωτ_S2 = 1 (at LF ≈ 0.06 MHz) [21,22]. Until now, only two Mn^II-based CAs have been observed that have NMRD profiles showing these two inflection points: [Mn^II(H₂O)₆]²⁺ [21,22] and [Mn^II₂(ENOTA)(H₂O)₂] [23]. All other reported NMRD profiles display only the high field dispersion at LF ≈ 10 MHz, indicating that for these compounds the contribution of the scalar interaction to the relaxivity is negligible.

It should be noted that the SBM equations, particularly Equations (6) and (7), only hold under certain conditions, such as (i) the Redfield condition of extreme narrowing (Δ_sτ_RH << 1, Δ_s is the static ZFS), (ii) the ZFS energy should be much larger than the Zeeman energy (E_ZFS >> E_Zeeman), (iii) the electronic decay should be mono-exponential [24,25]. Outside these limits, a proper description of the electronic relaxation requires more complicated calculations that also take the static ZFS into account. Although the conditions for applying the SBM theory for the modeling of NMRD profiles are not always valid for Mn²⁺ complexes, this theory has been applied in almost all studies on the complexes mentioned in Table 1. It may be justified if the Redfield condition applies and if only data for LF > 10 MHz were considered [26], because then the influence of the electronic relaxation can be neglected. Otherwise, the SBM model can be applied for a qualitative description of structure relaxivity relationships when the best fit parameters obtained, particularly those concerning the electronic relaxation, are considered as effective rather than physically relevant [27]. The most relevant parameters governing the relaxivity as determined by fitting of NMRD profiles with the SBM equations are included in Table 1.

For the evaluation of q, we focused our attention to the low field parts of the NMRD profiles. Below the dispersion at LF ≈ 10 MHz, the profiles always had a plateau, where r₁ was rather large and almost independent of LF, which allows an accurate measurement of r₁. At low field, r₁ was dominated by q and the electronic relaxation, whereas at higher field strengths the nuclear relaxation dominated, which in turn was dominated by q, τ_R, and τ_M. This might lead to local maxima in the profile for high molecular weight compounds and relatively low relaxivities for low molecular compounds [2].

Values of r₁ at 0.01 MHz were measured in the profiles with a single dispersion. In the profiles for [Mn(H₂O)₆]²⁺ and [Mn₂(ENOTA)(H₂O)₂], the r₁ was measured at about 1 MHz (at the plateau behind the first dispersion), where the scalar contribution may be neglected.

Figure 2 displays a plot of the collected low field r₁-values versus the reported q values for the Mn²⁺ complexes concerned (q_lit). Many of the q_lit values were obtained by applying a combination of several of the methods described above. For q = 0, the datapoints are in a narrow range of r₁-values around an average of 2.4 ± 0.2 s⁻¹·mM⁻¹. This value can therefore be applied as a good estimate of the outer sphere contribution of all Mn²⁺ complexes. For q > 0, Figure 2 clearly shows that the ranges of r₁ values for various q-values overlap. Hence r₁ ranges alone cannot be applied for the evaluation of q.

A closer inspection of the structures corresponding with datapoints at q = 1 and q = 2 indicates that r₁ increased with the molecular volume of the Mn²⁺-complexes at each q, which suggests that the r₁ at low field also depended on the rotational correlation time (τ_R). Therefore, we next considered the datapoints for q ≠ 0. For these data, r₁/q appeared not to be linearly proportional to the molecular weight (FW). Regression of r₁/q as a function of FW with the computer program CurveExpert 1.4 using all in-built models afforded a good fit with Equation (11):

$$y=9.16\left\{1-exp\left(-2.97\times FW\times {10}^{-3}\right)\right\}$$

(11)

The hydration number can be calculated with Equation (12):

$$q_{calc}=\frac{r_1}{y}$$

(12)

Figure 3 shows the good agreement between the calculated hydration numbers (q_calc) and the values mentioned in the publications from which the r₁ values were taken (q_lit). Fractional q numbers are also correctly predicted. From Figure 3, the accuracy of q_calc is estimated to be ±0.4. The good agreement between all calculated and reported q values indicates that the applied previously reported q values are correct. Moreover, it indicates that q and FW are the main parameters that determined the relaxivity; apparently, the influence of other parameters was rather small. This is surprising, since r₁ at low LF was expected to be dominated by the electronic relaxation. Even the q-values of complexes with relatively large τ_S0-values (Mn₂(ENOTA)(H₂O)₂, [Mn(15pyN₅)(H₂O)]²⁺, [Mn(15pyN₃O₂)(H₂O)₂]²⁺, and [Mn(H₂O)₆]²⁺, see Table 1) seem to be predicted correctly. To explain this, simulations of r₁ at LF = 0.01 MHz as a function of τ_M, τ_R, and τ_S0 were made using the SBM equations. Figure 4 shows, for example, the simulations for typical q = 1 Mn²⁺ complexes. Figure 4A shows that r₁ is unsensitive to variations in τ_M; however, it is strongly dependent on τ_R, and Figure 4B shows that r₁ was only very sensitive to variations of τ_S0 for complexes for which τ_R > 150 ps. The complexes in the database used to deduce Equations (11) and (12) are exclusively of low and medium molecular weight compounds (FW < 1250). The simulations in Figure 4 confirmed that for those complexes, r₁ can be expected to be insensitive to variations in electronic relaxation rates and τ_M. However, it may be expected that these Equations are no longer valid for high molecular weight Mn²⁺ complexes. However, in those cases, q can generally be estimated from low molecular analogs.

An attempt to apply a similar procedure for the evaluation of q from r₁ values at LF = 60 MHz resulted in unreasonable q_calc values that deviated substantially from q_lit.

3. Methods

The relaxivities were extracted from published NMRD profiles using the computer program WebPlotDigitizer, version 4.1 [48]. Equation (11) was selected with the computer program CurveExpert, version 1.4 [49].

4. Conclusions

An inspection of published ¹H NMRD profiles of Mn²⁺-complexes of low and medium molecular weights (FW < 1250) shows that the magnitudes of r₁ at LF ≤ 0.1 MHz is dominated by the parameters q and τ_R. Using a dataset of 49 published NMRD profiles as learning set, Equations (11) and (12) were evaluated for a quick and reliable estimation of q from r₁ values at LF ≤ 0.1 MHz and the molecular weight of the complex.