Impact-Induced Plastic Deformation in CuZr Metallic Glass and MG/Cu Composites
Abstract
1. Introduction
2. Results and Discussion
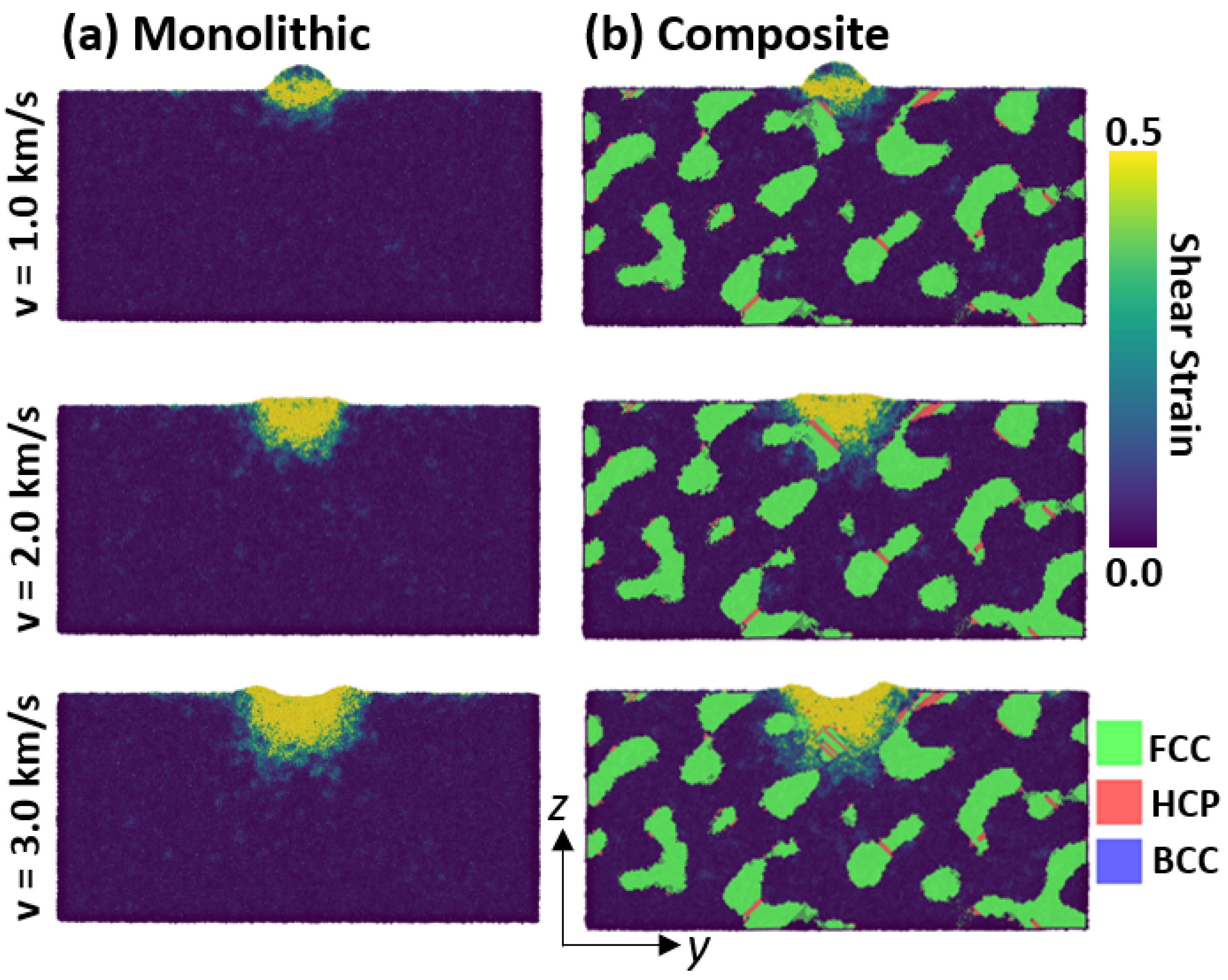
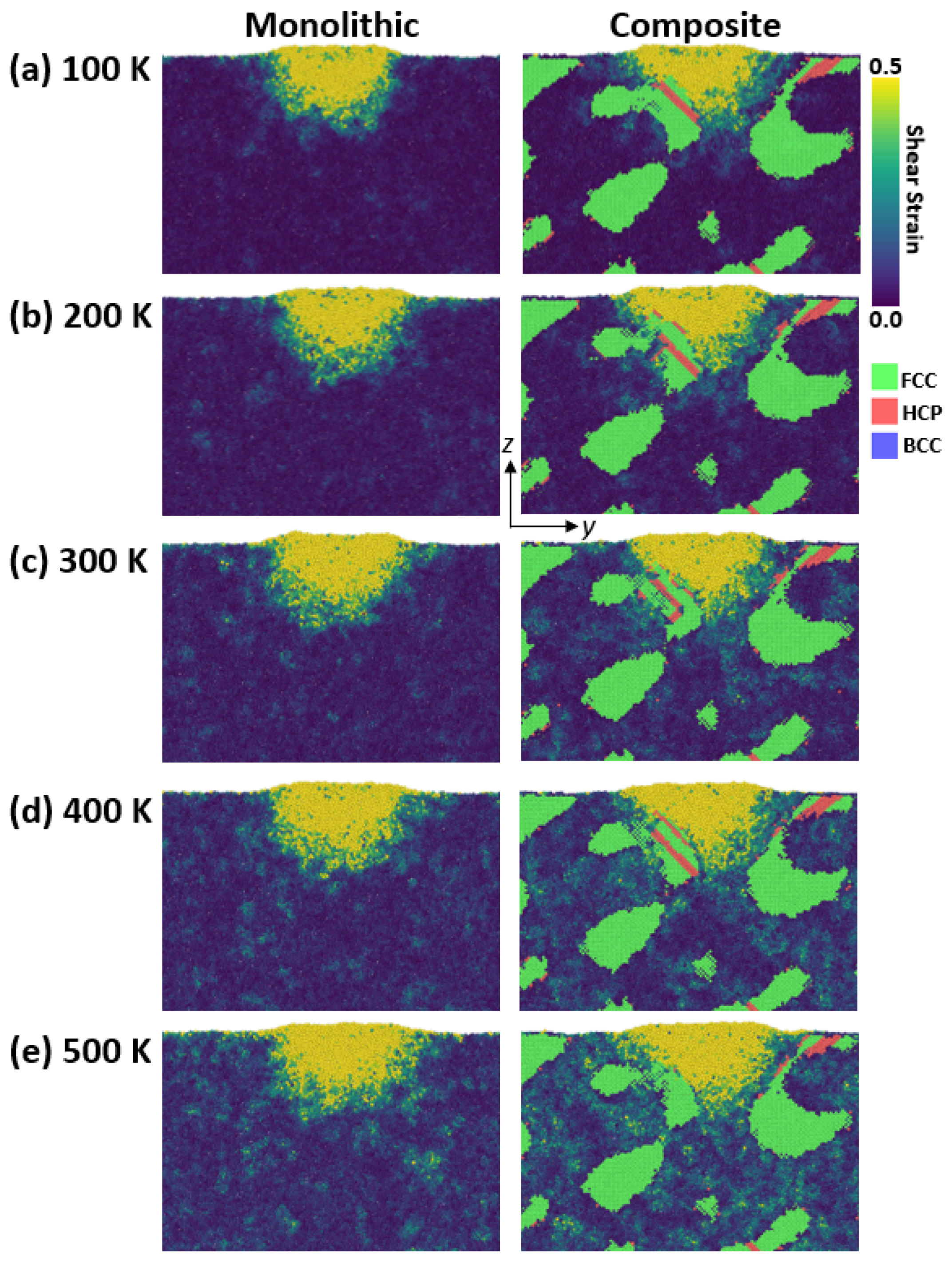
2.1. Deformation Fields
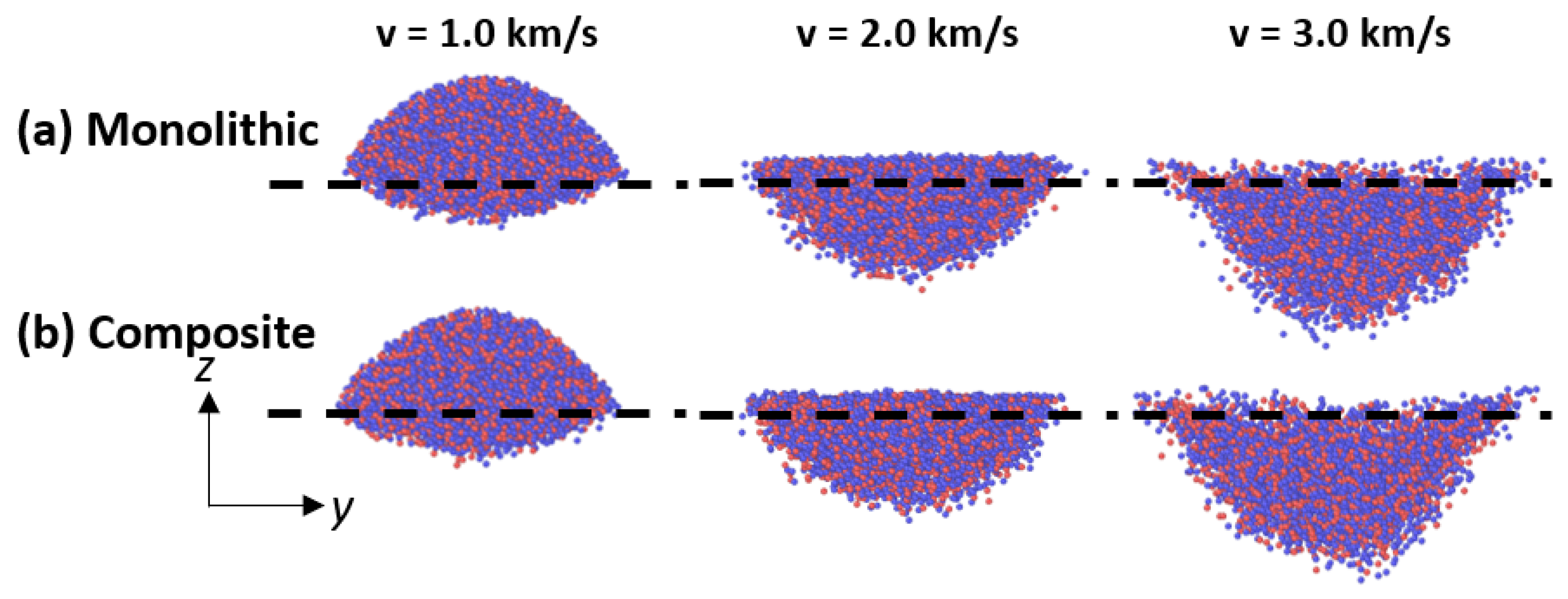
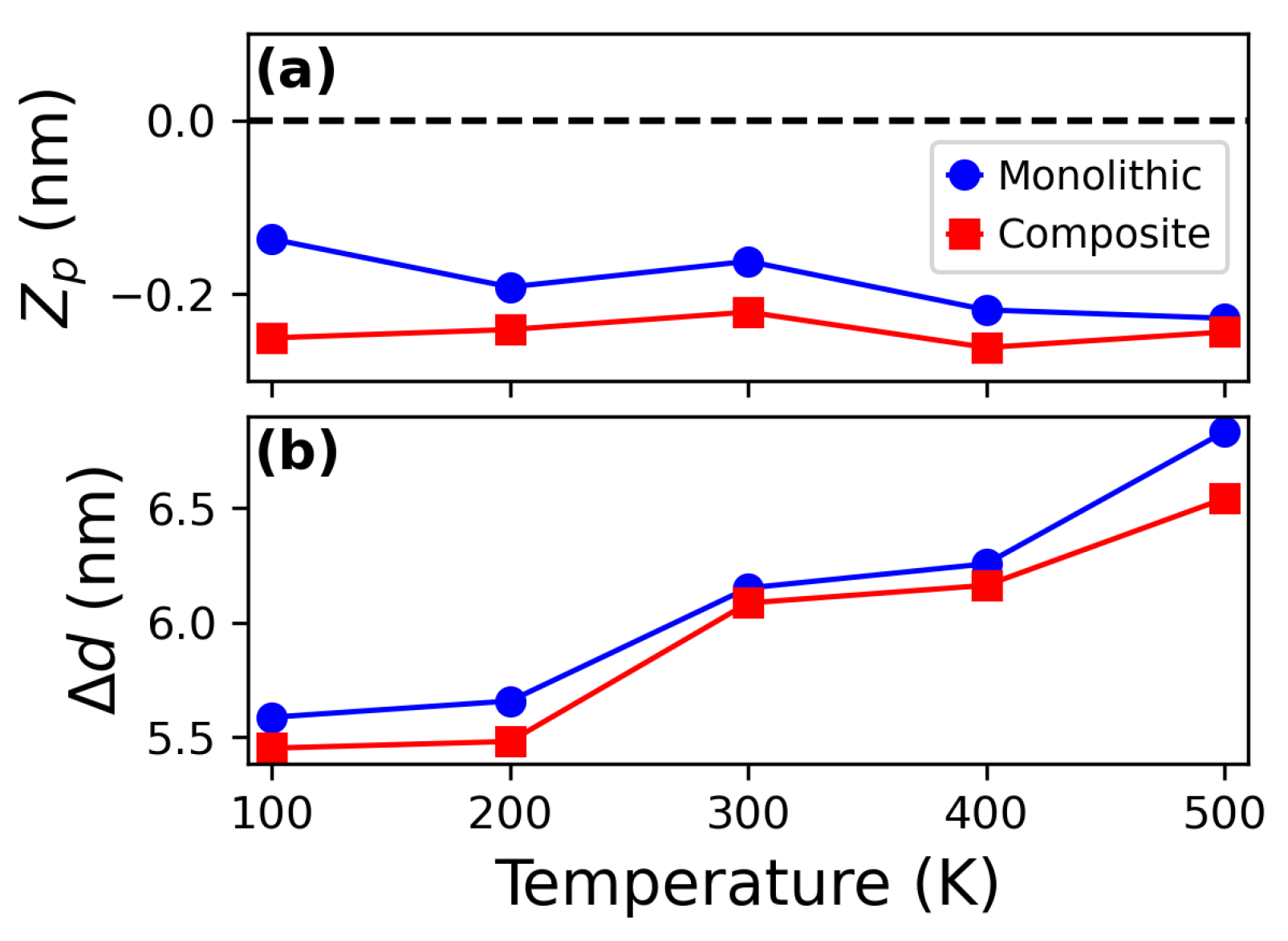
2.2. Penetration and Projectile Deformation
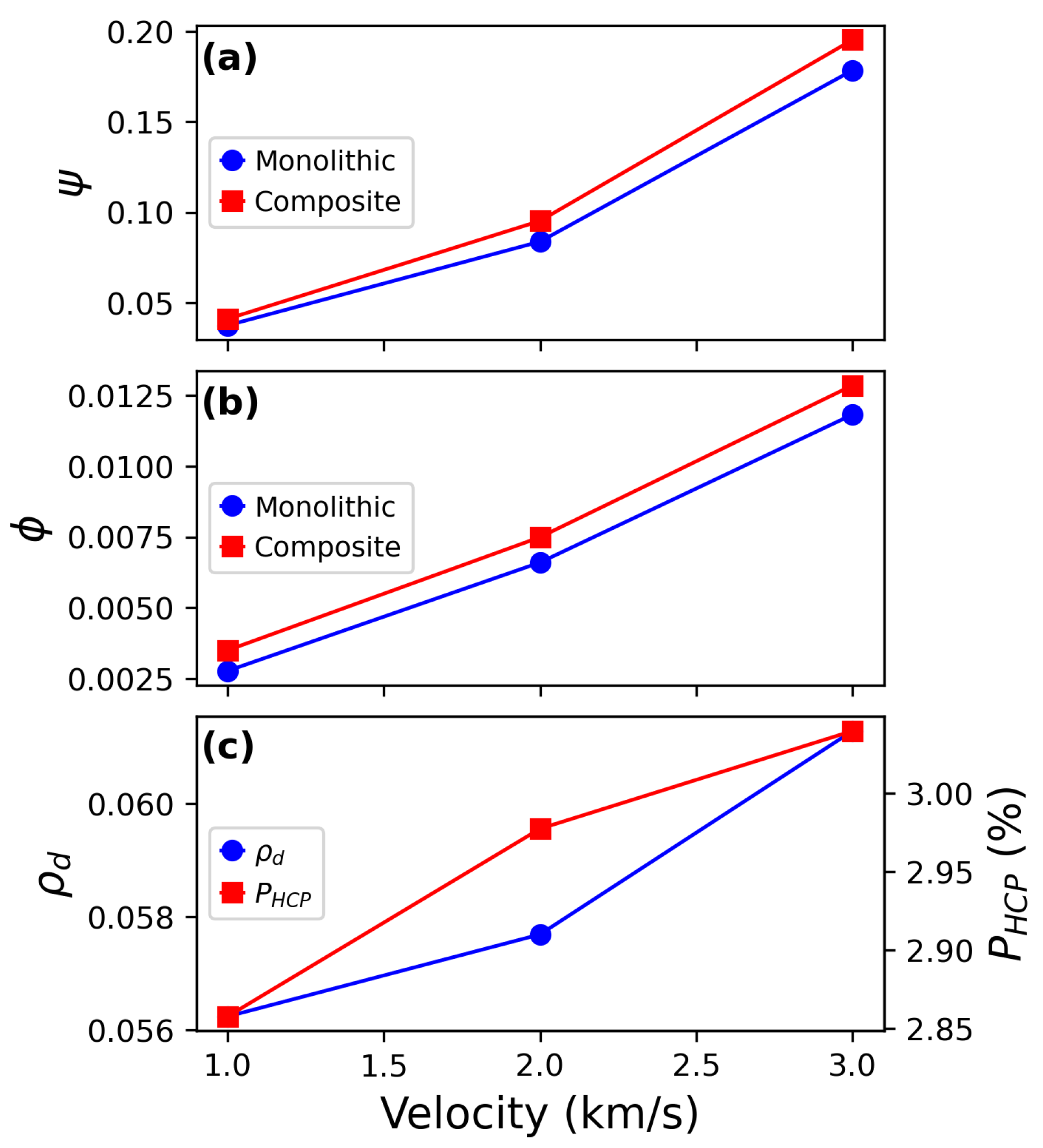
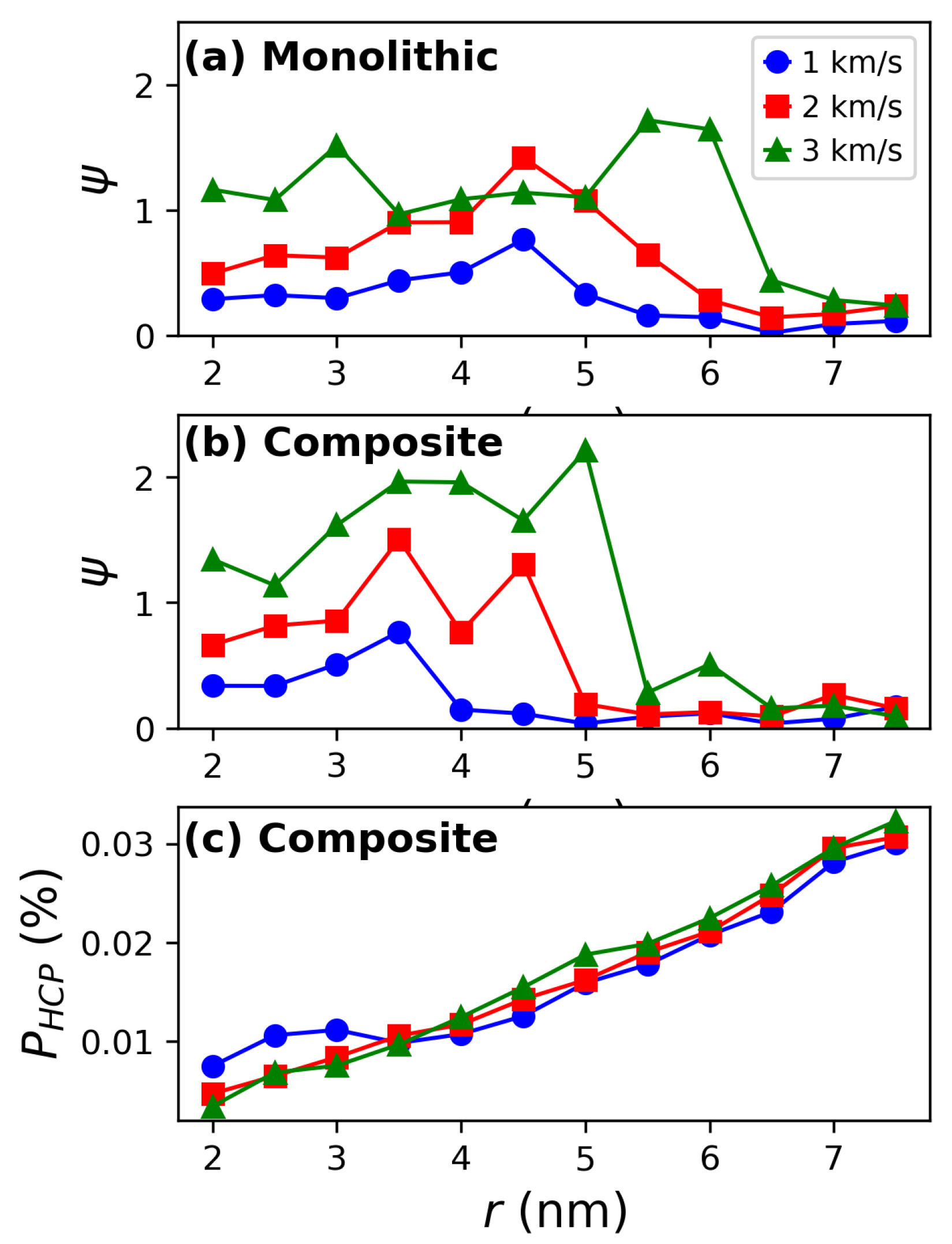
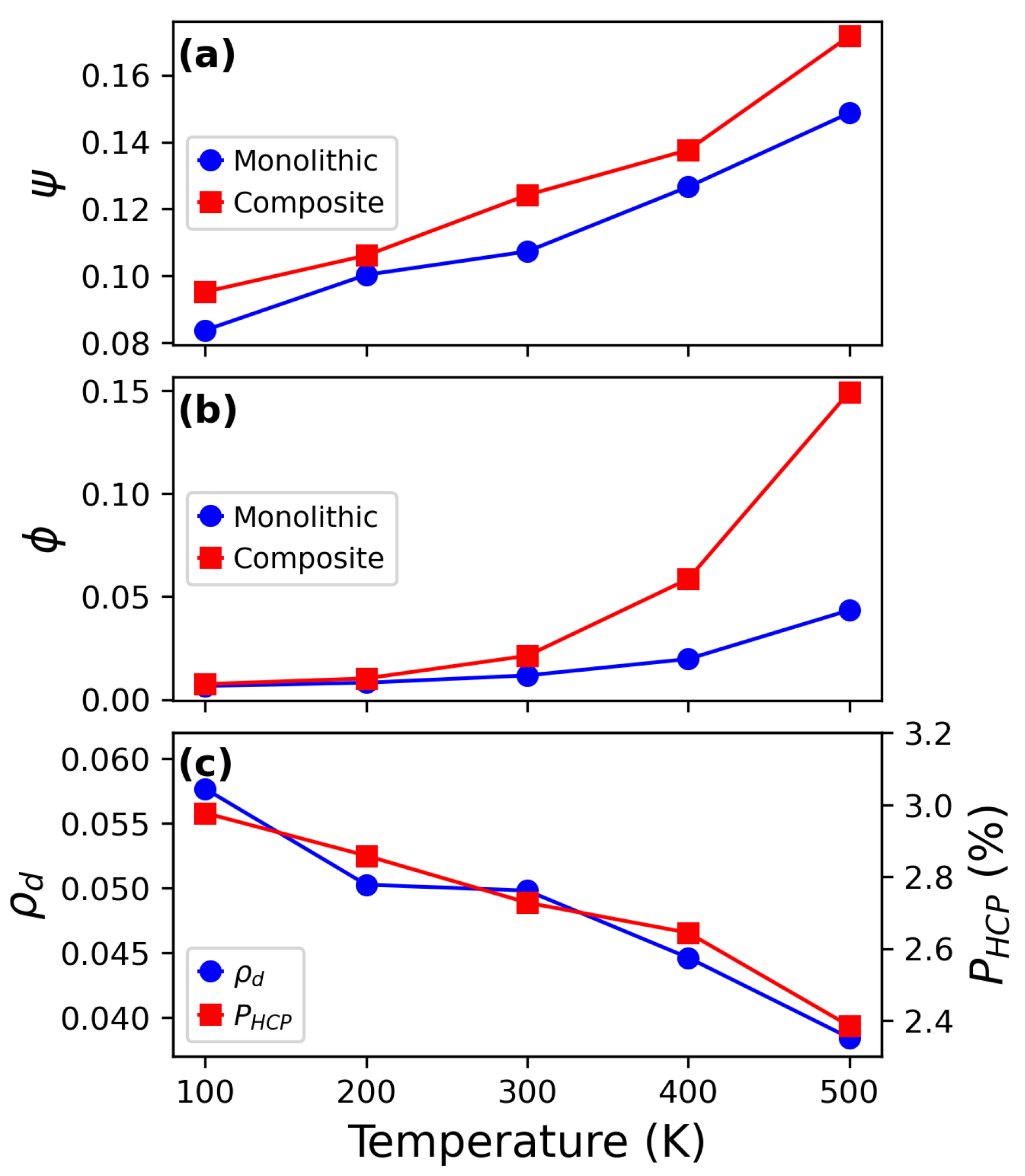
2.3. Quantification of Plastic Deformation
2.4. Temperature Effect
2.5. Relationship Between Plastic Deformation and Temperature
2.6. Implications and Future Perspectives
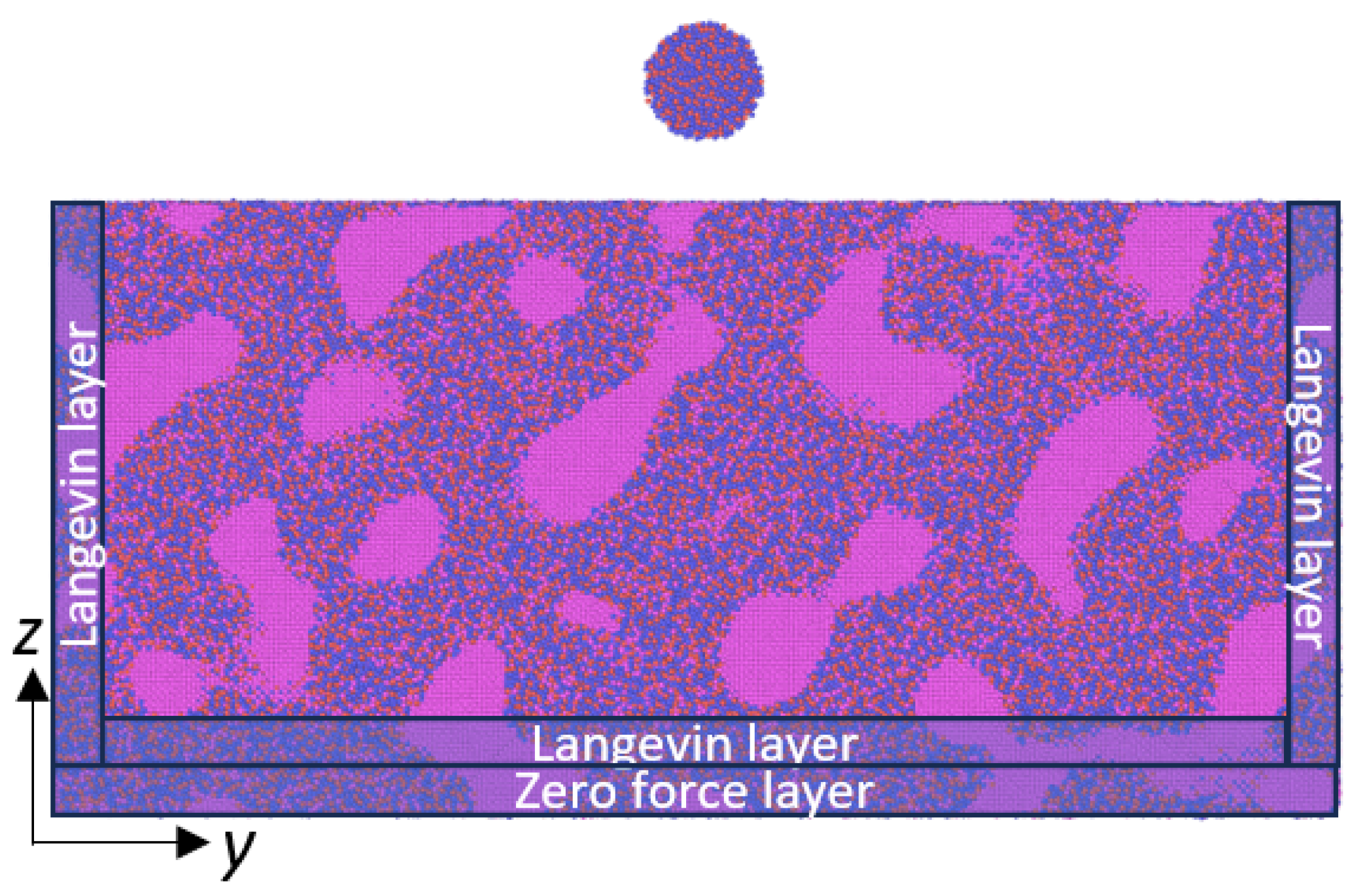
3. Materials and Methods
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Correction Statement
References
- Ryan, S.; Schaefer, F.; Destefanis, R.; Lambert, M. A ballistic limit equation for hypervelocity impacts on composite honeycomb sandwich panel satellite structures. Adv. Space Res. 2008, 41, 1152–1166. [Google Scholar] [CrossRef]
- Pieters, C.M.; Noble, S.K. Space weathering on airless bodies. J. Geophys. Res. Planets 2016, 121, 1865–1884. [Google Scholar] [CrossRef]
- Kılıc, N.; Bedir, S.; Erdik, A.; Ekici, B.; Tasdemirci, A.; Guden, M. Ballistic behavior of high hardness perforated armor plates against 7.62 mm armor piercing projectile. Mater. Des. 2014, 63, 427–438. [Google Scholar] [CrossRef]
- Cour-Palais, B.G. Hypervelocity impact in metals, glass and composites. Int. J. Impact Eng. 1987, 5, 221–237. [Google Scholar] [CrossRef]
- Safri, S.; Sultan, M.; Yidris, N.; Mustapha, F. Low velocity and high velocity impact test on composite materials–a review. Int. J. Eng. Sci 2014, 3, 50–60. [Google Scholar]
- Collins, G.S.; Melosh, H.J.; Ivanov, B.A. Modeling damage and deformation in impact simulations. Meteorit. Planet. Sci. 2004, 39, 217–231. [Google Scholar] [CrossRef]
- Seaman, L.; Curran, D.R.; Shockey, D.A. Computational models for ductile and brittle fracture. J. Appl. Phys. 1976, 47, 4814–4826. [Google Scholar] [CrossRef]
- Wensink, H.; Elwenspoek, M.C. A closer look at the ductile-brittle transition in solid particle erosion. Wear 2002, 253, 1035–1043. [Google Scholar] [CrossRef]
- Michel, Y.; Chevalier, J.M.; Durin, C.; Espinosa, C.; Malaise, F.; Barrau, J.J. Hypervelocity impacts on thin brittle targets: Experimental data and SPH simulations. Int. J. Impact Eng. 2006, 33, 441–451. [Google Scholar] [CrossRef]
- Hao, G.; Dong, X.; Du, M.; Li, Z.; Dou, Z. A comparative study of ductile and brittle materials due to single angular particle impact. Wear 2019, 428–429, 258–271. [Google Scholar] [CrossRef]
- Pham, T.M.; Hao, H. Influence of global stiffness and equivalent model on prediction of impact response of RC beams. Int. J. Impact Eng. 2018, 113, 88–97. [Google Scholar] [CrossRef]
- Hassani, M.; Veysset, D.; Nelson, K.A.; Schuh, C.A. Material hardness at strain rates beyond 106 s-1 via high velocity microparticle impact indentation. Scr. Mater. 2020, 177, 198–202. [Google Scholar] [CrossRef]
- Cai, J.; Griesbach, C.; Ahnen, S.G.; Thevamaran, R. Dynamic Hardness Evolution in Metals from Impact Induced Gradient Dislocation Density. Acta Mater. 2023, 249, 118807. [Google Scholar] [CrossRef]
- Fortov, V.; Kim, V.; Lomonosov, I.; Matveichev, A.; Ostrik, A. Numerical modeling of hypervelocity impacts. Int. J. Impact Eng. 2006, 33, 244–253. [Google Scholar] [CrossRef]
- Tan, V.; Ching, T. Computational simulation of fabric armour subjected to ballistic impacts. Int. J. Impact Eng. 2006, 32, 1737–1751. [Google Scholar] [CrossRef]
- Kilic, N.; Ekici, B.; Hartomacıoglu, S. Determination of penetration depth at high velocity impact using finite element method and artificial neural network tools. Def. Technol. 2015, 11, 110–122. [Google Scholar] [CrossRef]
- Žmindák, M.; Pelagić, Z.; Pastorek, P.; Močilan, M.; Vybošťok, M. Finite Element Modelling of High Velocity Impact on Plate Structures. Procedia Eng. 2016, 136, 162–168. [Google Scholar] [CrossRef]
- Yehia Abdel-Nasser, A.M.E.; Al-Mallah, I. Impact analysis of composite laminate using finite element method. Ships Offshore Struct. 2017, 12, 219–226. [Google Scholar] [CrossRef]
- Madhukar, A.; Ostoja-Starzewski, M. Finite Element Methods in Human Head Impact Simulations: A Review. Ann. Biomed. Eng. 2019, 47, 1832–1854. [Google Scholar] [CrossRef]
- Song, G.; Yang, H.; Hogan, C.J. Thermal energy evolution and mechanical deformation of monocrystalline yttria-stabilized zirconia nanoparticles in aerosol deposition processes. Appl. Surf. Sci. 2022, 585, 152603. [Google Scholar] [CrossRef]
- Burlison, S.; Becker, M.F.; Kovar, D. A molecular dynamics study of the effects of velocity and diameter on the impact behavior of zinc oxide nanoparticles. Model. Simul. Mater. Sci. Eng. 2023, 31, 075008. [Google Scholar] [CrossRef]
- Song, G.; Hogan, C.J. Crystal grain size effects and crystallinity dynamics during supersonic particle impacts. Int. J. Plast. 2023, 170, 103758. [Google Scholar] [CrossRef]
- Amigo, N. Deposition of droplets on metallic glass substrates: Thermophysical and structural characterization. J. Mol. Liq. 2025, 421, 126879. [Google Scholar] [CrossRef]
- Chitrakar, T.V.; Becker, M.F.; Kovar, D. Influence of Crystallographic Orientation on the Deformation of Ag Nanoparticles During High-Speed Impact. J. Therm. Spray Technol. 2023, 32, 2683–2700. [Google Scholar] [CrossRef]
- Dutta, A. Surface damage of CuZr metallic glass by hypervelocity nano-projectile: A molecular dynamics study. Comput. Mater. Sci. 2018, 141, 41–48. [Google Scholar] [CrossRef]
- Wachter, J.; Amigo, N.; Gutiérrez, G.; Zúñiga, A. Atomic scale study of the impact of metallic glass nanoparticles at high velocities. J. Non-Cryst. Solids 2024, 628, 122866. [Google Scholar] [CrossRef]
- Dwivedi, P.; Fraile, A.; Polcar, T. Tungsten wall cratering under high-velocity dust impacts: Influence of impact angle and temperature. J. Nucl. Mater. 2024, 600, 155289. [Google Scholar] [CrossRef]
- Greer, A.L. Metallic glasses…on the threshold. Mater. Today 2009, 12, 14–22. [Google Scholar] [CrossRef]
- Schroers, J. Processing of Bulk Metallic Glass. Adv. Mater. 2010, 22, 1566–1597. [Google Scholar] [CrossRef]
- Chen, M. A brief overview of bulk metallic glasses. NPG Asia Mater. 2011, 3, 82–90. [Google Scholar] [CrossRef]
- Wu, F.F.; Chan, K.C.; Jiang, S.S.; Chen, S.H.; Wang, G. Bulk metallic glass composite with good tensile ductility, high strength and large elastic strain limit. Sci. Rep. 2014, 4, 5302. [Google Scholar] [CrossRef]
- Qiao, J.; Jia, H.; Liaw, P.K. Metallic glass matrix composites. Mater. Sci. Eng. Rep. 2016, 100, 1–69. [Google Scholar] [CrossRef]
- Sha, Z.D.; Branicio, P.S.; Lee, H.P.; Tay, T.E. Strong and ductile nanolaminate composites combining metallic glasses and nanoglasses. Int. J. Plast. 2017, 90, 231–241. [Google Scholar] [CrossRef]
- Fu, X.; Wang, G.; Wu, Y.; Song, W.; Shek, C.H.; Zhang, Y.; Shen, J.; Ritchie, R.O. Compressive ductility and fracture resistance in CuZr-based shape-memory metallic-glass composites. Int. J. Plast. 2020, 128, 102687. [Google Scholar] [CrossRef]
- Yaghoobi, M.; Voyiadjis, G.Z. The effects of temperature and strain rate in fcc and bcc metals during extreme deformation rates. Acta Mater. 2018, 151, 1–10. [Google Scholar] [CrossRef]
- Amigo, N.; Careglio, C.A.; Ardiani, F.; Manelli, A.; Tramontina, D.R.; Bringa, E.M. Thermal effects on the mechanical behavior of CuZr metallic glasses. Appl. Phys. A 2024, 130, 616. [Google Scholar] [CrossRef]
- Amigo, N. Mechanical characterization of nanocrystalline Cu-Ag alloys subjected to shear deformation. Mol. Simul. 2024, 50, 1411–1419. [Google Scholar] [CrossRef]
- Reddy, K.V.; Deng, C.; Pal, S. Dynamic characterization of shock response in crystalline-metallic glass nanolaminates. Acta Mater. 2019, 164, 347–361. [Google Scholar] [CrossRef]
- Zhang, J.; Wang, X.; Zhang, M.; Deng, L.; Gong, P. The shock forming process of Cu50Zr50 metallic glasses studied via molecular dynamics simulation. J. Non-Cryst. Solids 2022, 584, 121518. [Google Scholar] [CrossRef]
- Song, W.; Yu, Y.; Guan, Y. Role of void shape on shock responses of nanoporous metallic glasses via molecular dynamics simulation. Int. J. Mech. Sci. 2022, 218, 107076. [Google Scholar] [CrossRef]
- Song, W.; Meng, Y.; Xiao, L.; Liu, S. Tuning dynamic mechanical properties of Cu50Zr50 nanoglasses/ nanopolycrystalline Cu composites investigated by molecular dynamics simulation. J. Non-Cryst. Solids 2024, 625, 122715. [Google Scholar] [CrossRef]
- Jami, H.; Jabbarzadeh, A. Molecular simulation of high-velocity deposition of titanium dioxide nanoparticles on titanium. Appl. Surf. Sci. 2021, 542, 148567. [Google Scholar] [CrossRef]
- Stukowski, A.; Albe, K.; Farkas, D. Nanotwinned fcc metals: Strengthening versus softening mechanisms. Phys. Rev. B 2010, 82, 224103. [Google Scholar] [CrossRef]
- Asadi, E.; Zaeem, M.A.; Moitra, A.; Tschopp, M.A. Effect of vacancy defects on generalized stacking fault energy of fcc metals. J. Physics: Condens. Matter 2014, 26, 115404. [Google Scholar] [CrossRef] [PubMed]
- Amigo, N. Mechanical Properties of Copper with Dendritic Silver Inclusions: Insights from Molecular Dynamics Simulations. Comput. Mater. Contin. 2024, 81, 3665–3678. [Google Scholar] [CrossRef]
- Priezjev, N.V. Aging and rejuvenation during elastostatic loading of amorphous alloys: A molecular dynamics simulation study. Comput. Mater. Sci. 2019, 168, 125–130. [Google Scholar] [CrossRef]
- Wang, M.; Liu, H.; Li, J.; Jiang, Q.; Yang, W.; Tang, C. Thermal-pressure treatment for tuning the atomic structure of metallic glass Cu-Zr. J. Non-Cryst. Solids 2020, 535, 119963. [Google Scholar] [CrossRef]
- Amigo, N. Cryogenic thermal cycling rejuvenation in metallic glasses: Structural and mechanical assessment. J. Non-Cryst. Solids 2022, 596, 121850. [Google Scholar] [CrossRef]
- Gao, Y.; Wang, H.; Zhao, J.; Sun, C.; Wang, F. Anisotropic and temperature effects on mechanical properties of copper nanowires under tensile loading. Comput. Mater. Sci. 2011, 50, 3032–3037. [Google Scholar] [CrossRef]
- Amigo, N.; Valencia, F. Mechanical and structural assessment of CuZr metallic glasses rejuvenated by thermal-pressure treatments. Comput. Mater. Sci. 2021, 198, 110681. [Google Scholar] [CrossRef]
- Heggen, M.; Spaepen, F.; Feuerbacher, M. Creation and annihilation of free volume during homogeneous flow of a metallic glass. J. Appl. Phys. 2004, 97, 033506. [Google Scholar] [CrossRef]
- Yoo, B.G.; Kim, J.Y.; Kim, Y.J.; Choi, I.C.; Shim, S.; Tsui, T.Y.; Bei, H.; Ramamurty, U.; Jang, J.I. Increased time-dependent room temperature plasticity in metallic glass nanopillars and its size-dependency. Int. J. Plast. 2012, 37, 108–118. [Google Scholar] [CrossRef]
- Qiu, Y.; Xu, C.; Fu, E.; Wang, P.; Du, J.; Hu, Z.; Yan, X.; Cao, X.; Wang, Y.; Shao, L. Mechanisms for the free volume tuning the mechanical properties of metallic glass through ion irradiation. Intermetallics 2018, 101, 173–178. [Google Scholar] [CrossRef]
- Jafary-Zadeh, M.; Tavakoli, R.; Srolovitz, D.J.; Zhang, Y.W. Thermally induced failure mechanism transition and its correlation with short-range order evolution in metallic glasses. Extrem. Mech. Lett. 2016, 9, 215–225. [Google Scholar] [CrossRef]
- Rohith, P.; Sainath, G.; Srinivasan, V. Effect of size, temperature and strain rate on dislocation density and deformation mechanisms in Cu nanowires. Phys. Condens. Matter 2019, 561, 136–140. [Google Scholar] [CrossRef]
- Saffarini, M.H.; Voyiadjis, G.Z.; Ruestes, C.J. Temperature effect on nanoporous gold under uniaxial tension and compression. Comput. Mater. Sci. 2021, 200, 110766. [Google Scholar] [CrossRef]
- Grossman, E.; Gouzman, I.; Verker, R. Debris/Micrometeoroid Impacts and Synergistic Effects on Spacecraft Materials. MRS Bull. 2010, 35, 41–47. [Google Scholar] [CrossRef]
- Schonberg, W.P. Protecting Earth-orbiting spacecraft against micro-meteoroid/orbital debris impact damage using composite structural systems and materials: An overview. Adv. Space Res. 2010, 45, 709–720. [Google Scholar] [CrossRef]
- Rakib, M.A.; Smith, S.T.; Tafsirojjaman, T. A review of shielding systems for protecting off-earth structures from micrometeoroid and orbital debris impact. Acta Astronaut. 2024, 223, 404–425. [Google Scholar] [CrossRef]
- Plimpton, S. Fast Parallel Algorithms for Short-Range Molecular Dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef]
- Thompson, A.P.; Aktulga, H.M.; Berger, R.; Bolintineanu, D.S.; Brown, W.M.; Crozier, P.S.; In’t Veld, P.J.; Kohlmeyer, A.; Moore, S.G.; Nguyen, T.D.; et al. LAMMPS–A flexible simulation tool for particle-based materials modeling at the atomic, meso, and continuum scales. Comp. Phys. Comm. 2022, 271, 108171. [Google Scholar] [CrossRef]
- Borovikov, V.; Mendelev, M.I.; King, A.H. Effects of stable and unstable stacking fault energy on dislocation nucleation in nano-crystalline metals. Model. Simul. Mater. Sci. Eng. 2016, 24, 085017. [Google Scholar] [CrossRef]
- Soyarslan, C.; Bargmann, S.; Pradas, M.; Weissmüller, J. 3D stochastic bicontinuous microstructures: Generation, topology and elasticity. Acta Mater. 2018, 149, 326–340. [Google Scholar] [CrossRef]
- Shimizu, F.; Ogata, S.; Li, J. Theory of Shear Banding in Metallic Glasses and Molecular Dynamics Calculations. Mater. Trans. 2007, 48, 2923–2927. [Google Scholar] [CrossRef]
- Cheng, Y.Q.; Cao, A.J.; Ma, E. Correlation between the elastic modulus and the intrinsic plastic behavior of metallic glasses: The roles of atomic configuration and alloy composition. Acta Mater. 2009, 57, 3253–3267. [Google Scholar] [CrossRef]
- Shi, Y.; Falk, M.L. Strain Localization and Percolation of Stable Structure in Amorphous Solids. Phys. Rev. Lett. 2005, 95, 095502. [Google Scholar] [CrossRef]
- Adibi, S.; Branicio, P.S.; Joshi, S.P. Suppression of Shear Banding and Transition to Necking and Homogeneous Flow in Nanoglass Nanopillars. Sci. Rep. 2015, 5, 15611. [Google Scholar] [CrossRef]
- Stukowski, A. Structure identification methods for atomistic simulations of crystalline materials. Model. Simul. Mater. Sci. Eng. 2012, 20, 045021. [Google Scholar] [CrossRef]
- Faken, D.; Jónsson, H. Systematic analysis of local atomic structure combined with 3D computer graphics. Comput. Mater. Sci. 1994, 2, 279–286. [Google Scholar] [CrossRef]
- Stukowski, A. Visualization and analysis of atomistic simulation data with OVITO–the Open Visualization Tool. Model. Simul. Mater. Sci. Eng. 2010, 18, 015012. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Amigo, N.; Wachter, J.; Leiva-Pavés, P. Impact-Induced Plastic Deformation in CuZr Metallic Glass and MG/Cu Composites. Inorganics 2025, 13, 141. https://doi.org/10.3390/inorganics13050141
Amigo N, Wachter J, Leiva-Pavés P. Impact-Induced Plastic Deformation in CuZr Metallic Glass and MG/Cu Composites. Inorganics. 2025; 13(5):141. https://doi.org/10.3390/inorganics13050141
Chicago/Turabian StyleAmigo, Nicolás, Javier Wachter, and Pablo Leiva-Pavés. 2025. "Impact-Induced Plastic Deformation in CuZr Metallic Glass and MG/Cu Composites" Inorganics 13, no. 5: 141. https://doi.org/10.3390/inorganics13050141
APA StyleAmigo, N., Wachter, J., & Leiva-Pavés, P. (2025). Impact-Induced Plastic Deformation in CuZr Metallic Glass and MG/Cu Composites. Inorganics, 13(5), 141. https://doi.org/10.3390/inorganics13050141








