The Synthesis and Crystal Structure of Six Quaternary Lithium-Alkaline Earth Metal Alumo-Silicides and Alumo-Germanides, A2LiAlTt2 (A = Mg, Ca, Sr, Ba; Tt = Si, Ge)
1
Charter School of Wilmington, Wilmington, DE 19807, USA
2
Departamento de Química, Facultad de Ciencias, Universidad Nacional de Colombia-Bogotá, Carrera 30 No. 45-03, Bogotá 111321, Colombia
3
Department of Chemistry and Biochemistry, University of Delaware, Newark, DE 19716, USA
*
Author to whom correspondence should be addressed.
Inorganics 2023, 11(9), 351; https://doi.org/10.3390/inorganics11090351
Submission received: 1 August 2023
/
Revised: 16 August 2023
/
Accepted: 23 August 2023
/
Published: 26 August 2023
(This article belongs to the Special Issue Modern Methods, Modern Users, and Modern Materials: Solid-State Materials Synthesis and Crystal Growth Methods)
Abstract
:Reported are the synthesis and structural characterization of a series of quaternary lithium-alkaline earth metal alumo-silicides and alumo-germanides with the base formula A2LiAlTt2 (A = Ca, Sr, Ba; Tt = Si, Ge). To synthesize each compound, a mixture of the elements with the desired stoichiometric ratio was loaded into a niobium tube, arc welded shut, enclosed in a silica tube under vacuum, and heated in a tube furnace. Each sample was analyzed by powder and single-crystal X-ray diffraction, and the crystal structure of each compound was confirmed and refined from single-crystal X-ray diffraction data. The structures, despite the identical chemical formulae, are different, largely dependent on the nature of the alkaline earth metal. The differing cation determines the structure type—the calcium compounds are part of the TiNiSi family with the Pnma space group, the strontium compounds are isostructural with Na2LiAlP2 with the Cmce space group, and the barium compounds crystallize with the PbFCl structure type in the P4/nmm space group. The anion (silicon or germanium) only impacts the size of the unit cell, with the silicides having smaller unit cell volumes than the germanides.
1. Introduction
With climate change becoming increasingly dominant, switching from fossil fuels to sustainable energy sources and reducing global inefficiencies are main priorities. Zintl phases typically contain electropositive cations (alkali, alkaline earth, or rare earth metals) and electronegative early p-block metals and semimetals from groups 13 to 15, which are the (poly)anions [1]. Given that many Zintl phases are intrinsic semiconductors, they could be the bases for materials with applications in solid-state energy conversion and/or storage [2,3,4,5,6,7,8,9,10], which further sustainability.
In recent years, the lithiation behavior of Si-, Ge-, and Sn-based clathrates, many of which can be viewed as Zintl phases [11], has become the subject of increased research interest [12,13,14,15,16,17,18,19,20,21,22]. The undertaken fundamental studies, either by us or by several other teams, have been carried out in the hopes of adapting the clathrates’ desirable characteristics for prospective high-capacity Li-ion battery applications [23,24]. In particular, over the course of our work with the type-I clathrates Ba8AlxSi46−x and Ba8AlxGe46−x (0 < x < 16) [21,22], we observed the presence of some unknown phases as reaction byproducts. The latter were presumed to be new quaternary compounds with hitherto unknown structures and compositions. These observations were the motivation to create the following research plan for the summer internship; and so, the goal of this research was the rational synthesis of new Zintl phases comprising lithium and silicon or germanium. 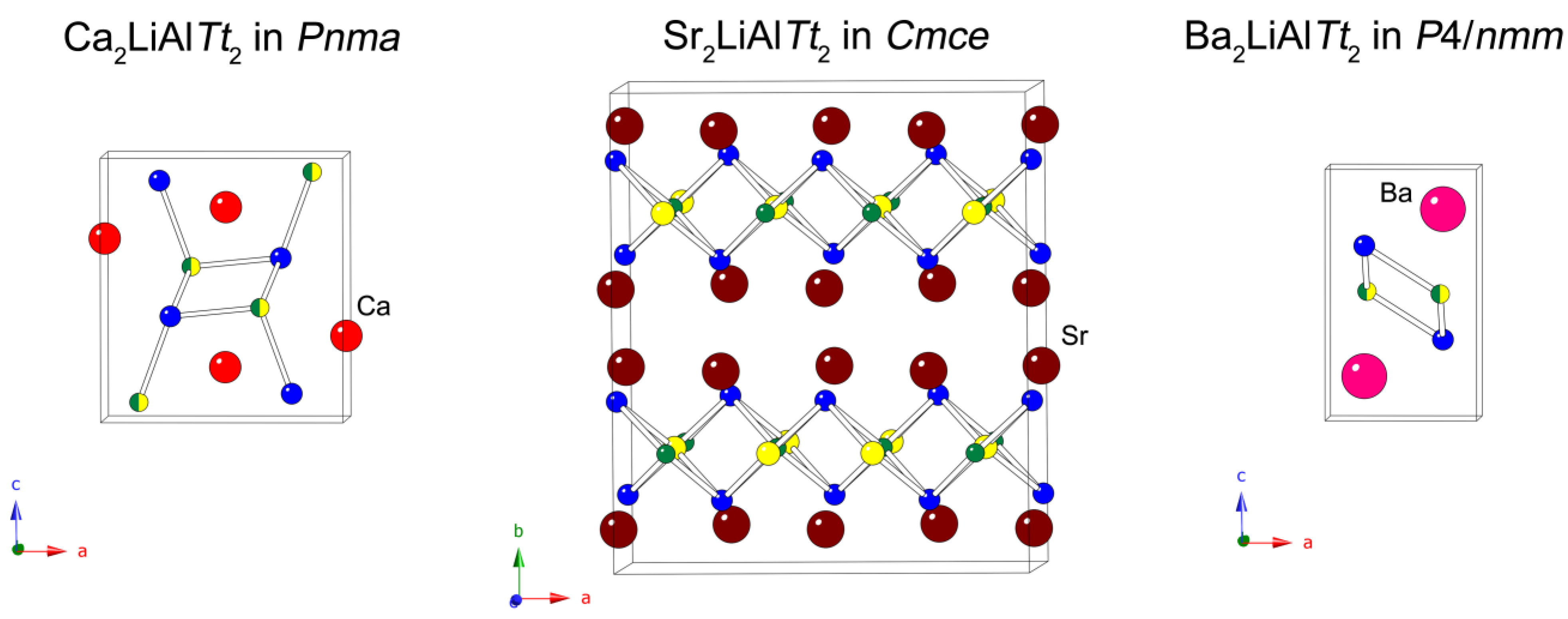Hence, we set out to explore the A–Li–Al–Tt systems (A = Mg, Ca, Sr, Ba; Tt = Si, Ge) and our starting points were the equiatomic nominal compositions. The syntheses quickly yielded several new compounds in each respective system. Below, we detail the synthetic work that led to the identification of the title compounds, as well as describe their crystal structures. At the outset, we draw attention to the fact that the structures, despite their same general chemical formula, are different (Figure 1). Each compound’s differing anion (silicon or germanium) impacts only the size of the unit cell, with the silicides having smaller unit cell volumes than the germanides. The nature of the alkaline earth metal appears to influence which structure forms, with the calcium compounds being analogs of the TiNiSi/SrMgSi family (orthorhombic Pnma space group, no. 62), the strontium compounds being a part of the Na2LiAlP2 structural family (orthorhombic Cmce space group, no. 64), and the barium compounds crystallizing with the PbFCl structure type in the tetragonal P4/nmm space group (no. 129). To this end, attempts at the synthesis of Mg2LiAlSi2 and Mg2LiAlGe2 failed (Vide infra).
Several europium and ytterbium compounds were also synthesized. The characterization work is still ongoing and the results will be detailed in a later publication. We will just note that the europium compounds appear to mirror the crystal chemistry of the barium compounds, but the ytterbium compounds have differing structures and compositions. For that reason, the synthesized rare earth metal compounds are not included here.
2. Results
Crystal data for A2LiAlTt2 (A = Ca, Sr, Ba; Tt = Si, Ge) can be found in Table 1, Table 2 and Table 3, respectively. We should note that our initial results from the system Ba–Li–Al–Ge, based on single-crystal X-ray diffraction work, showed the co-existence of Li and Al atoms on a single crystallographic site, which piqued our attention. Later on, the same result was replicated for the system Ba–Li–Al–Si. The Ca-compounds, although with a differing crystal structure, also showed Li and Al atoms on a single crystallographic site (Table 4). The refined chemical formulae in all four cases were ALixAl1–xTt (A = Ca, Ba; Tt = Si, Ge) with x ≈ ½; however, subsequent synthetic work showed that changes in the nominal Li:Al ratio do not contribute to changes in the unit cell volume and there were no peak shifts when experimental and simulated powder X-ray diffraction patterns were compared. For the Sr-based compounds, Li and Al were found to occupy individual sites and were not disordered. Therefore, in this article, we labeled all six new alumo-silicides and alumo-germanides with the base formula A2LiAlTt2 (A = Ca, Sr, Ba; Tt = Si, Ge), neglecting the arguably small possibility of trivial deviations from the stated stoichiometry.
As seen from Table 1, Table 2 and Table 3 (as well as from Table 4, where the atomic coordinates of each of the six structures are given), there are three basic structures adopted by each of the Ca, Sr, and Ba compounds, respectively. All three types of structures (Figure 1) share a common feature—tetrahedral (or rather distorted tetrahedral) coordination of Al and Li atoms by the atoms of the Tt element. In the case of the Ca and Ba compounds, there is no long-range ordering and the refined models have Li and Al atoms occupying the same crystallographic positions, while in the case of the Sr compounds, there is no randomization and the derived crystal structures of Sr2LiAlSi2 and Sr2LiAlGe2 are devoid of disorder. Apparently, the packing of the AlTt4 and LiTt4 fragments together with the increasing size of the alkaline earth metal atom (in the expected order Ca < Sr < Ba) governs the formation of each structure, as it will be discussed in the next section. In that regard, we will mention that we attempted to synthesize Mg2LiAlSi2 and Mg2LiAlGe2 using the same synthesis procedure (elements in the targeted stoichiometric ratio loaded into niobium tube, arc welded shut, enclosed in a silica tube under vacuum, and heated in tube furnaces according to the same heating profile as for the Ca, Sr, and Ba counterparts). The resultant products were not the quaternaries Mg2LiAlSi2 and Mg2LiAlGe2. Instead, three different crystals could be identified with a naked eye: (1) blue, shiny gem-like crystals; (2) atmosphere-reactive silver crystals; and (3) dark, hard, brittle crystals. The blue crystals were determined by single-crystal X-ray diffraction to be cubic Mg2Si or Mg2Ge [25]. The other crystals could not be identified reliably. We posit that Mg is either too small to form structures comparable to the Ca, Sr, and Ba compounds detailed in this paper, or may form covalent bonds with Al and the Tt element. Reactions with the heavier congener of Si and Ge, Sn, were not explored as a part of this study due to time limitations.
In summary, the reaction method detailed in this paper, with or without significant deviations, may produce more A2LiAlTt2 (A = Mg, Yb; Tt = Si, Ge, Sn) compounds, but further inquiries must be made.
3. Discussion
We begin the discussion with the structure of Ca2LiAlSi2 and Ca2LiAlGe2, which crystallize with the orthorhombic space group Pnma (no. 62). They are isotypic with TiNiSi structure type (Pearson symbol oP12) [25]; the structure has three unique positions in the asymmetric unit. As such, and as described previously, Li and Al atoms are considered to be statistically disordered.
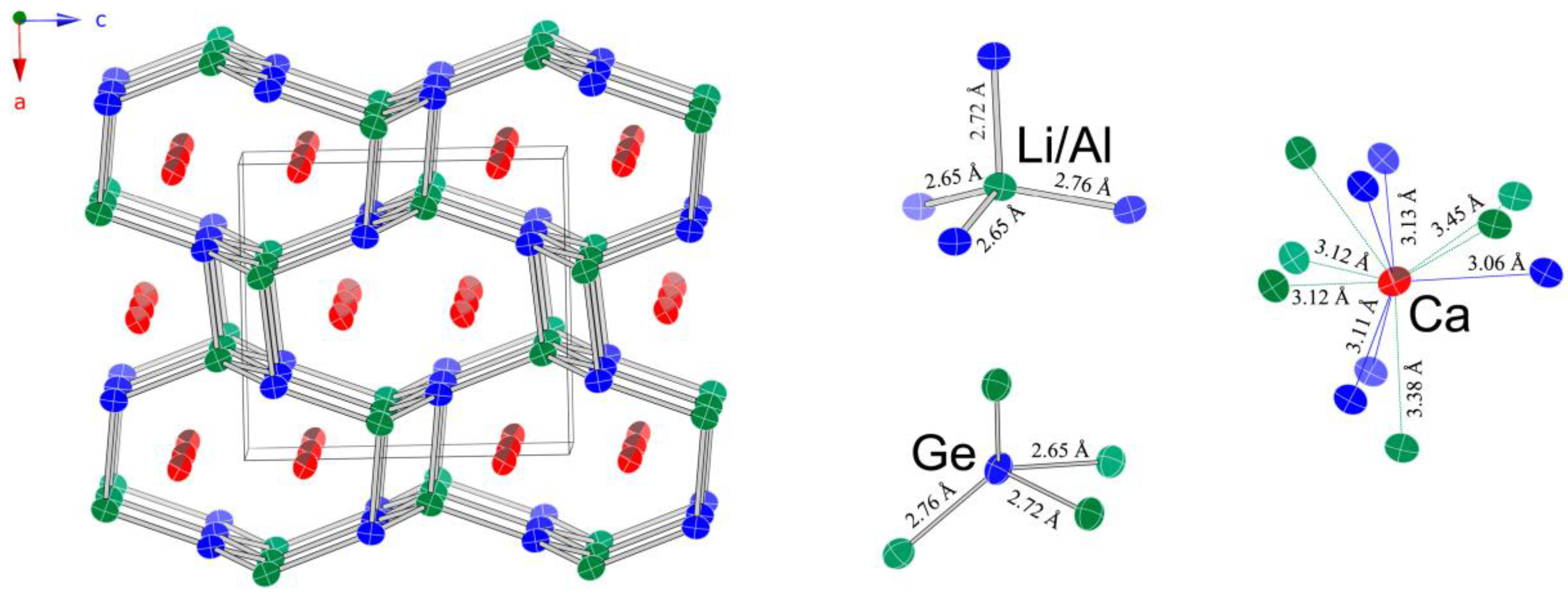
A schematic representation of the structure is given in Figure 2. The chosen view shows that the structure can be viewed as a 3D network made up of edge- and corner-shared tetrahedra (AlTt4 and LiTt4), with the Ca atoms taking the space within the created channels. The tetrahedral units are slightly distorted from the ideal geometry and have two shorter and two longer Li/Al–Tt bonds. The respective distances are tabulated in Table 5.
Four-fold coordination environments exist around the Si and Ge atoms too, but in that case, the distortions from the ideal tetrahedral angle, in particular, are more severe. Si—Li/Al and Ge—Li/Al distances are about 10% longer than the sum of the single-bonded Pauling covalent radii (rLi = 1.225 Å; rAl = 1.248 Å; rSi = 1.173 Å; rGe = 1.242 Å) [26]. These distances match the reported values in a number of alumo-silicides and alumo-germanides, such as Li8Al3Si5 [27,28], LiAlSi [28], CaAl2Si2 [29,30], SrAl2Si2 [31,32], BaAl2Si2 [33], LnAl2Si2 (Ln = Y, Sm, Tb, Dy, Yb) [34], Ln2Al3Si2 and Ln2AlSi2 (Ln = Y, Tb-Lu) [35], Ba7.5Al13Si29 [36], LiAlGe [37], CaAl2Ge2 [38,39], LnAl2Ge2 (Ln = Y, La, Nd, Gd, Tb, Lu) [40], BaAl2Ge2 [41], A3Al2Ge2 (A = Ca, Sr, Ba) [42], and LnAl2X2 (Ln = Eu, Yb; X = Si, Ge) [43], among others. Ca atoms have a total coordination number 11, with 6 nearest Li/Al and 5 nearest Tt atoms. The shortest contact is dCa—Si = 3.06 Å, which also fairly closely matches the sum of the single-bonded Pauling covalent radii of Si and Ca (rCa = 1.736 Å).
In the fully ionic approximation, the structures of Ca2LiAlSi2 and Ca2LiAlGe2 can be rationalized as (Ca2+)2(Li+)(Al3+)(Si4−)2 and (Ca2+)2(Li+)(Al3+)(Ge4−)2, i.e., the compounds should be considered intrinsic semiconductors. Applying the Zintl concept [1] also leads to the same conclusion—when treating Li as a cation and Al as a part of the polyanionic four-bonded substructure, it becomes possible to partition the valence electrons the following way: (Ca2+)2(Li+)(4b-Al1−)(4b-Si0)(0b-Si4−) and (Ca2+)2(Li+)(4b-Al1−)(4b-Ge0)(0b-Ge4−). To arrive at this formula breakdown, we are assuming covalent Si—Al and Ge—Al interactions; Si and Al are coordinated to Al atoms 50% of the time. In the remaining 50% of the time, Li takes the Al position and covalency of the Si—Li and Ge—Li is neglected, i.e., Si and Ge are treated as isolated, closed-shell anions.
Following periodic trends, the structure of Sr2LiAlSi2 and Sr2LiAlGe2 will be discussed next. These compounds are also orthorhombic, but with the base-centered space group Cmce (no. 64). They are isotypic with Na2LiAlP2 (Pearson symbol oS48) [44]. The structure has six unique positions in the asymmetric unit—two for the Sr atom, two for the Si or Ge atoms, and one each for the Li and Al atoms, Table 4. As such, and as described previously, this is the only “true 2-1-1-2” structure, given that Li and Al atoms are ordered crystallographically here.
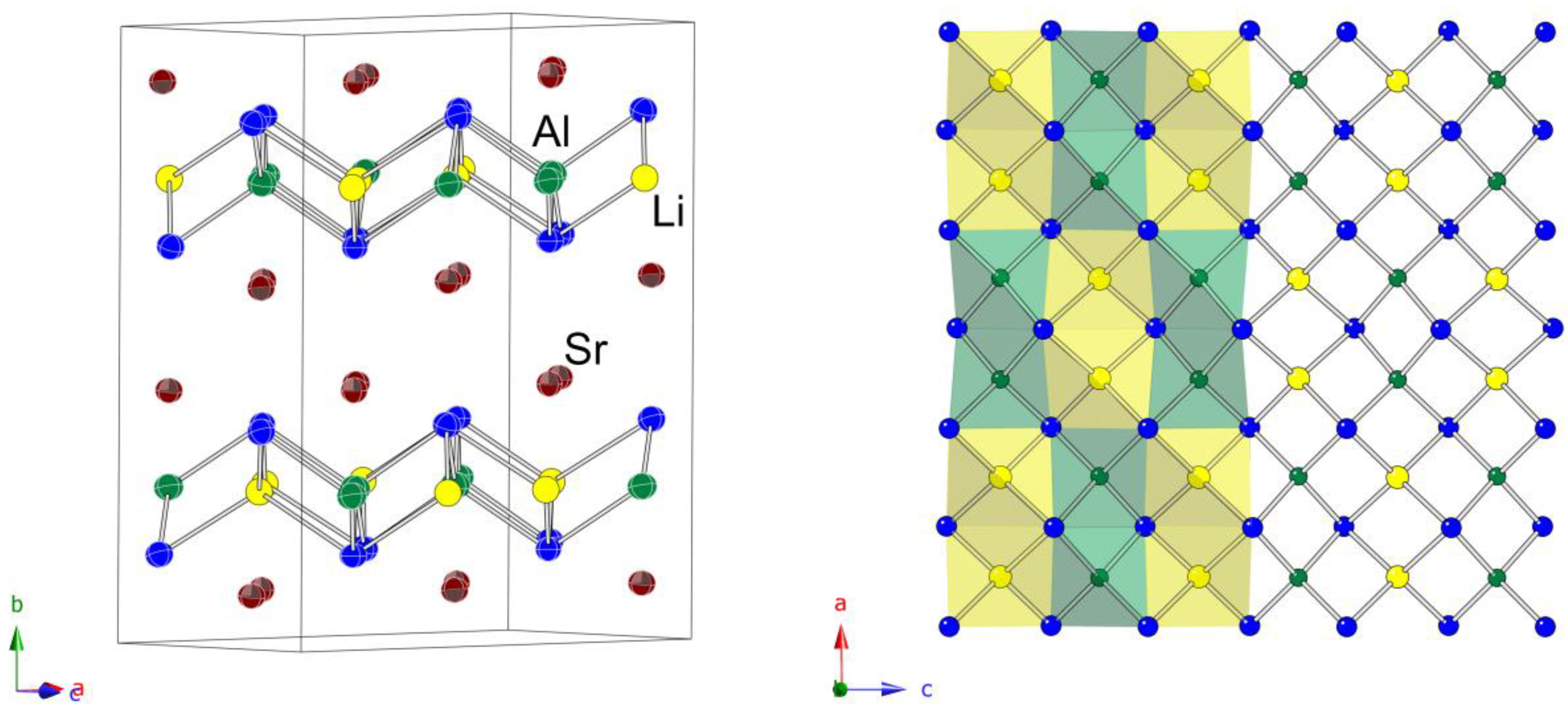
A schematic representation of the structure is given in Figure 3. The chosen view shows that the structure can be viewed as slabs made up of all edge-shared AlTt4 and LiTt4 tetrahedra parallel to the ac plane. The general topology of the 2D polyanionic substructure is reminiscent of the PbO-like layers that are frequently observed in the structures of many pnictides and chalcogenides [45,46,47], and analogous to the layers seen in the tetragonal structures of Ba2LiAlSi2 and Ba2LiAlGe2, which will be discussed next. The difference is that here, given that there is no Li/Al disorder, the exact positions of Li and Al atoms are known and the ordering pattern is checkerboard-like, with a repeating sequence of AlTt4 × 2 by LiTt4 × 2 (Figure 3, right panel).
The AlTt4 and LiTt4 tetrahedral units are ever so slightly distorted from the ideal geometry and have two shorter and two longer Li/Al–Tt bonds. The refined bond lengths match the sum of the single-bonded Pauling covalent radii of the elements well. Sr atoms are arranged in double layers between the [LiAlSi2] and [LiAlGe2] slabs. They have a total coordination number of nine, with five nearest Tt atoms, two nearest Al, and two neighboring Li atoms. All respective distances are tabulated in Table 6.
The application of the Zintl concept here [1] is more instructive than what we considered before on the examples of Ca2LiAlSi2 and Ca2LiAlGe2, given that no disorder is present and no assumptions need to be made. Hence, using the same scheme to partition the valence electrons, we can propose the following formulae breakdown: (Sr2+)2(Li+)(4b-Al1−)(2b-Si2−)2 and (Sr2+)2(Li+)(4b-Al1−)(2b-Ge2−)2. To arrive at these formulae, only Si—Al and Ge—Al interactions were treated as covalent. In a more extreme case, where Si—Li and Ge—Li bonds are also treated as covalent, all Tt atoms will be four-bonded and will bear no formal charge. Li with four covalent bonds must be considered formally as 4b-Li3−, in analogy with the Zintl clathrate Ba8Li5Ge41 [11]. In this scenario, the valence electron count can be ascribed as follows: (Sr2+)2(4b-Li3−)(4b-Al1−)(4b-Si0)2 and (Sr2+)2(4b-Li3−)(4b-Al1−)(4b-Ge0)2.
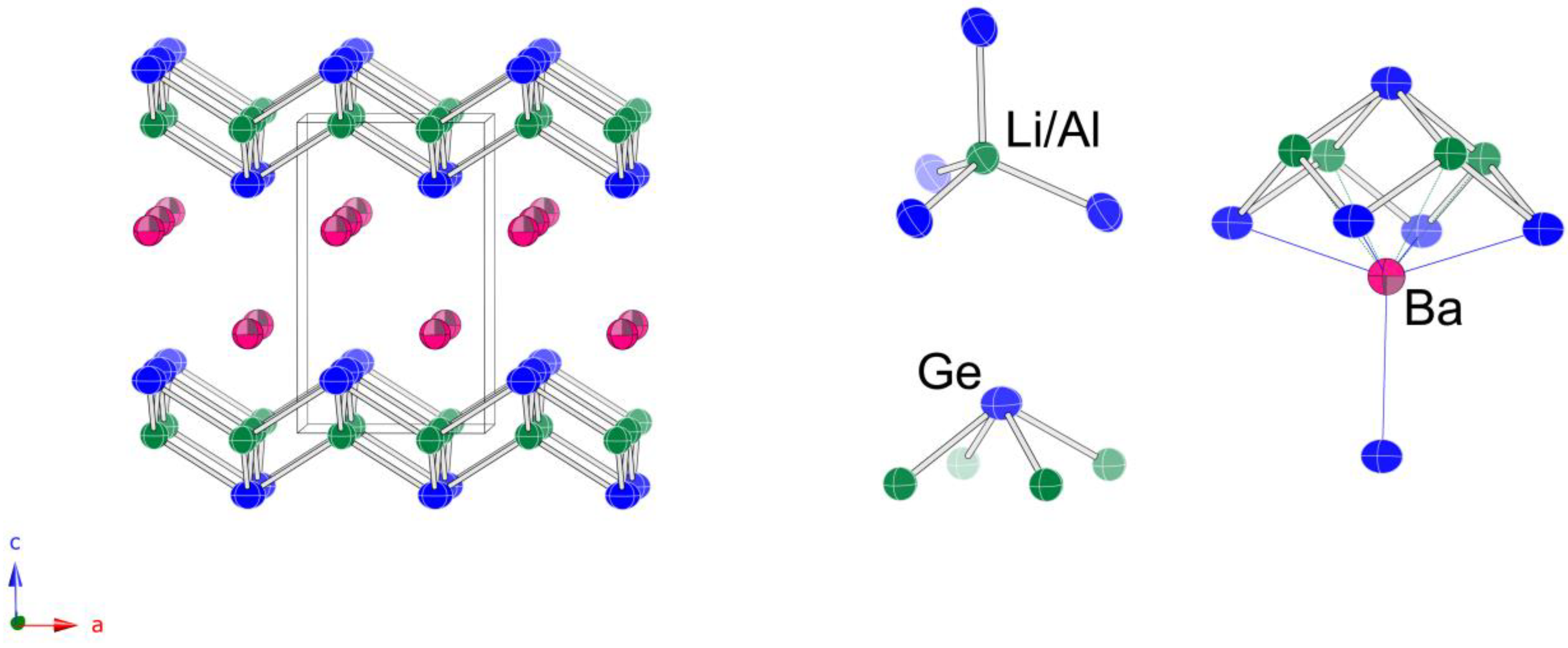
The last remaining structure to briefly discuss is that of Ba2LiAlSi2 and Ba2LiAlGe2. The latter two compounds crystallize with the tetragonal space group P4/nmm (no. 129). This is a structure type known as PbFCl (Pearson symbol tP6) [25], which is very common and well-known (Figure 4). There are three unique positions in the asymmetric unit (Table 4), meaning that Li and Al atoms are, again, statistically disordered.
From the schematic representation of the structure in Figure 4, it is evident that the overall arrangement of the atoms is nearly identical to that described above for the orthorhombic Sr2LiAlSi2 and Sr2LiAlGe2; the major difference is that the ordering of the tetrahedral AlTt4 and LiTt4 units is averaged in this symmetry. The Ba atoms take the space between the layers and have the exact same coordination environment (CN 9) as the Sr atoms in Sr2LiAlSi2 and Sr2LiAlGe2 (vide supra). Another Si atom is 3.9 Å away, i.e., is the topmost Si atom in Figure 4, for which a line denoting Si—Ba contact is not drawn.
Naturally, given that Ba is the largest element from group 2, all distances here are slightly longer compared to the Ca- and Sr-based compounds. Specifically, the average bond lengths of A–Al and A–Li (A = Ca, Sr, Ba) in Ca2LiAlTt2, Sr2LiAlTt2, and Ba2LiAlTt2 (Tt = Si, Ge) increase as the differing cation increases in size. In other words, the average A–Al and A–Li contacts in Ca2LiAlSi2 are shorter than those in Sr2LiAlSi2, which are in turn shorter than those in Ba2LiAlSi2. The same principle applies to the analogous germanides. For example, for the silicides, the average Ca–Al/Li distance is 3.14 Å, whereas the average Ba–Al/Li is 3.36 Å (recall that both structures have Al and Li atoms disordered).
Of note is also the fact that the fourfold symmetry in Ba2LiAlTt2 makes the tetrahedral units much closer to the ideal geometry, as they have four equal Li/Al–Tt bonds. Ge coordination environment is square pyramidal. All respective distances are tabulated in Table 7.
Just like with the cases of Ca2LiAlTt2 and Sr2LiAlTt2, in the fully ionic approximation, the structures of Ba2LiAlSi2 and Ba2LiAlGe2 can be rationalized as (Ba2+)2(Li+)(Al3+)(Si4−)2 and (Ba2+)2(Li+)(Al3+)(Ge4−)2.
4. Materials and Methods
4.1. Synthesis
Objective: To synthesize and establish the structure of a series of novel quaternary alkaline earth metal and rare earth metal silicides and germanides, with the base formula A2LiAlTt2 (A = Mg, Ca, Sr, Ba, Eu, Yb; Tt = Si, Ge). For this purpose, given that Li and most of the alkaline earth metals are reactive towards air and moisture, all synthetic and post-synthetic manipulations were performed inside an argon-filled glove box or under vacuum.
All metals were used as received from Alfa or Aldrich (>99.9% wt.), except for Ba and Li, whose surfaces had to be carefully cleaned with a blade each time the metal rods were cut. In a typical experiment, a mixture of elements with the desired stoichiometric ratio (total weight ca. 500 mg) was loaded into a Nb tube, which was then sealed by arc welding under an Ar atmosphere. The welded Nb tube was subsequently enclosed in a fused silica tube, which was flame-sealed under vacuum (below discharge).
To synthesize A2LiAlSi2 and A2LiAlGe2, the tubes with the reactant mixtures inside were heated in tube furnaces to 1223 K (rate of 300 K/h) and equilibrated for 8 h, followed by a cooling to 573 K at a rate of 200 K/h. The tubes were rotated in the furnaces at temperatures > 973 K. At the completion of their heat treatment, all tubes were quenched in cold water. The products of this method were not phase-pure.
Note: Many of the compounds detailed in this paper react rapidly with air and/or moisture. Corresponding with periodic trends, the germanides are more reactive than the silicide compounds. Moreover, the compounds’ reactivity can be represented as Ca2LiAlTt2 < Sr2LiAlTt2 < Ba2LiAlTt2. A2LiAlGe2 (A = Ca, Sr, Ba) samples reacted with the ambient air and turned opaquely yellow within a few minutes. The A2LiAlSi2 samples experienced no visible color changes, but were not air stable beyond one hour, even when covered under an oil film. In direct contact with deionized water, both A2LiAlSi2 and A2LiAlGe2 ignited and volatilized. Due to these compounds’ extreme sensitivity to the ambient atmosphere, further characterization besides the crystallographic work could not be effectively completed.
4.2. Crystallographic Studies
Powder X-ray diffraction (PXRD) measurements were carried out at room temperature on a Rigaku Miniflex diffractometer (filtered Cu Kα radiation, λ = 1.5418 Å). Small portions of the obtained samples were ground into powder using agate mortars and pestles inside the argon-filled glovebox. The specimens for PXRD were prepared by covering the polycrystalline material with a light film of oil and were transferred to another glovebox, which housed the diffractometer. The data were collected as quickly as possible with a typical θ–θ scan of 0.05°/step and data acquisition rate of 2 s/step. The collected powder X-ray diffraction patterns were used for phase identification of the reaction products only.
The PXRD measurements before and after exposure to air over confirmed the previously stated instability in air.
Single-crystal X-ray diffraction data for the six compounds were collected at 200 K using a Bruker SMART CCD-based diffractometer (three-circle goniometer; monochromated Mo Kα radiation (λ = 0.710 73 Å)). Firstly, several crystals from each batch were selected and checked before the best ones were chosen for intensity data collection. The experiments were managed in batch runs consisting of an appropriate number of frames to ensure 98+% coverage in angular range in 2θ of up to ca. 60° (frame width was typically 0.5–0.6° in ω and θ with a data acquisition rate of 6–8 s/frame). Data processing was performed with the Bruker supplied software. SADABS was used for semi-empirical absorption correction based on equivalents [48]. The structure factors were sorted and merged by the subprogram XPREP in the SHELXTL software package [49], which was also employed in the space group determination. The structures were solved by direct methods and refined to convergence by full-matrix least-squares methods on F2. Selected details of the data collection and relevant crystallographic parameters are given in Table 1, Table 2, Table 3 and Table 4. Refined distances are tabulated in Table 5, Table 6 and Table 7.
Author Contributions
Conceptualization, S.B.; methodology, P.K., L.C.P.-Q. and G.M.D.; data curation, P.K., L.C.P.-Q. and G.M.D.; validation, P.K., L.C.P.-Q., G.M.D. and S.B.; formal analysis, S.B.; investigation, P.K., L.C.P.-Q. and G.M.D.; resources, S.B.; writing—original draft preparation, P.K.; writing—conceptualization, P.K. and S.B.; writing—review and editing, S.B.; visualization, S.B.; supervision, S.B.; project administration, S.B.; funding acquisition, S.B. All authors have read and agreed to the published version of the manuscript.
Funding
This work was supported by the US National Science Foundation (NSF) through grant DMR-2004579.
Data Availability Statement
The corresponding crystallographic information files (CIF) have been deposited with the Cambridge Crystallographic Database Centre (CCDC) and can be obtained free of charge via http://www.ccdc.cam.ac.uk/conts/retrieving.html (or from the CCDC, 12 Union Road, Cambridge CB2 1EZ, UK; Fax: +44-1223-336033; E-mail: [email protected]) with the following depository numbers: 2285060–2285065.
Acknowledgments
The authors thank T.-S. You for early exploratory work in the Ca–Li–Al–Ge system and M. O. Ogunbunmi for help with single-crystal X-ray diffraction data. P.K. gives thanks to Jennifer Toner and Konstantinos Kontomaris for their exceptional guidance and encouragement throughout this project.
Conflicts of Interest
The authors declare no conflict of interest.
References
- Nesper, R. The Zintl-Klemm Concept—A Historical Survey. Z. Anorg. Allg. Chem. 2014, 640, 2639–2648. [Google Scholar] [CrossRef]
- Toberer, E.S.; May, A.F.; Snyder, G.J. Zintl Chemistry for Designing High Efficiency Thermoelectric Materials. Chem. Mater. 2010, 22, 624–634. [Google Scholar] [CrossRef]
- Kauzlarich, S.M.; Brown, S.R.; Snyder, G.J. Zintl Phases for Thermoelectric Devices. Dalton Trans. 2007, 21, 2099. [Google Scholar] [CrossRef]
- Yang, Z.; Trask, S.E.; Gilbert, J.A.; Li, X.; Tsai, Y.; Jansen, A.N.; Ingram, B.J.; Bloom, I. Exploring the Promise of Multifunctional “Zintl-Phase-Forming” Electrolytes for Si-Based Full Cells. ACS Appl. Mater. Interfaces 2022, 14, 53860–53871. [Google Scholar] [CrossRef]
- McDowell, M.T.; Lee, S.W.; Nix, W.D.; Cui, Y. 25th Anniversary Article: Understanding the Lithiation of Silicon and Other Alloying Anodes for Lithium-Ion Batteries. Adv. Mater. 2013, 25, 4966–4985. [Google Scholar] [CrossRef] [PubMed]
- Madsen, G.K.H. Automated Search for New Thermoelectric Materials: The Case of LiZnSb. J. Am. Chem. Soc. 2006, 128, 12140–12146. [Google Scholar] [CrossRef] [PubMed]
- Kauzlarich, S.M. Special Issue: Advances in Zintl Phases. Materials 2019, 12, 2554. [Google Scholar] [CrossRef]
- Snyder, G.J.; Toberer, E.S. Complex Thermoelectric Materials. Nat. Mater. 2008, 7, 105–114. [Google Scholar] [CrossRef]
- Böhme, B.; Minella, C.B.; Thoss, F.; Lindemann, I.; Rosenburg, M.; Pistidda, C.; Møller, K.T.; Jensen, T.R.; Giebeler, L.; Baitinger, M.; et al. B1-Mobilstor: Materials for Sustainable Energy Storage Techniques—Lithium Containing Compounds for Hydrogen and Electrochemical Energy Storage. Adv. Eng. Mater. 2014, 16, 1189–1195. [Google Scholar] [CrossRef]
- Shi, X.-L.; Zou, J.; Chen, Z.-G. Advanced Thermoelectric Design: From Materials and Structures to Devices. Chem. Rev. 2020, 120, 7399–7515. [Google Scholar] [CrossRef]
- Nolas, G.S. The Physics and Chemistry of Inorganic Clathrates; Springer Science+Business Media: Dordrecht, The Netherlands, 2014. [Google Scholar]
- Ghosh, K.; Ovchinnikov, A.; Baitinger, M.; Krnel, M.; Burkhardt, U.; Grin, Y.; Bobev, S. Lithium Metal Atoms Fill Vacancies in the Germanium Network of a Type-I Clathrate: Synthesis and Structural Characterization of Ba8Li5Ge41. Dalton Trans. 2023, 52, 10310–10322. [Google Scholar] [CrossRef] [PubMed]
- Dopilka, A.; Weller, J.M.; Ovchinnikov, A.; Childs, A.; Bobev, S.; Peng, X.; Chan, C.K. Structural Origin of Reversible Li Insertion in Guest-Free, Type-II Silicon Clathrates. Adv. Energy Sustain. Res. 2021, 2, 2000114. [Google Scholar] [CrossRef]
- Dopilka, A.; Childs, A.; Ovchinnikov, A.; Zhao, R.; Bobev, S.; Peng, X.; Chan, C.K. Structural and Electrochemical Properties of Type VIII Ba8Ga16−δSn30+δ Clathrate (δ ≈ 1) during Lithiation. ACS Appl. Mater. Interfaces 2021, 13, 42564–42578. [Google Scholar] [CrossRef] [PubMed]
- Dopilka, A.; Childs, A.; Bobev, S.; Chan, C.K. Electrochemical Lithium Alloying Behavior of Guest-Free Type II Silicon Clathrates. J. Phys. Chem. C 2021, 125, 19110–19118. [Google Scholar] [CrossRef]
- Dopilka, A.; Childs, A.; Bobev, S.; Chan, C.K. Understanding the Amorphous Lithiation Pathway of the Type I Ba8Ge43 Clathrate with Synchrotron X-ray Characterization. Chem. Mater. 2020, 32, 9444–9457. [Google Scholar] [CrossRef]
- Tarnev, T.; Wilde, P.; Dopilka, A.; Schuhmann, W.; Chan, C.K.; Ventosa, E. Surface Properties of Battery Materials Elucidated Using Scanning Electrochemical Microscopy: The Case of Type I Silicon Clathrate. ChemElectroChem 2019, 7, 665–671. [Google Scholar] [CrossRef]
- Wagner, N.A.; Raghavan, R.; Zhao, R.; Wei, Q.; Peng, X.; Chan, C.K. Electrochemical Cycling of Sodium-Filled Silicon Clathrate. ChemElectroChem 2013, 1, 347–353. [Google Scholar] [CrossRef]
- Peng, X.; Wei, Q.; Li, Y.; Chan, C.K. First-Principles Study of Lithiation of Type I Ba-Doped Silicon Clathrates. J. Phys. Chem. C 2015, 119, 28247–28257. [Google Scholar] [CrossRef]
- Dopilka, A.; Peng, X.; Chan, C.K. Ab Initio Investigation of Li and Na Migration in Guest-Free, Type I Clathrates. J. Phys. Chem. C 2019, 123, 22812–22822. [Google Scholar] [CrossRef]
- Dopilka, A.; Zhao, R.; Weller, J.M.; Bobev, S.; Peng, X.; Chan, C.K. Experimental and Computational Study of the Lithiation of Ba8AlyGe46−y Based Type I Germanium Clathrates. ACS Appl. Mater. Interfaces 2018, 10, 37981–37993. [Google Scholar] [CrossRef]
- Zhao, R.; Bobev, S.; Krishna, L.; Yang, T.; Weller, J.M.; Jing, H.; Chan, C.K. Anodes for Lithium-Ion Batteries Based on Type I Silicon Clathrate Ba8Al16Si30—Role of Processing on Surface Properties and Electrochemical Behavior. ACS Appl. Mater. Interfaces 2017, 9, 41246–41257. [Google Scholar] [CrossRef] [PubMed]
- Yang, J.; Tse, J.S. Silicon Clathrates as Anode Materials for Lithium Ion Batteries? J. Mater. Chem. A 2013, 1, 7782–7789. [Google Scholar] [CrossRef]
- Li, Y.; Raghavan, R.; Wagner, N.; Davidowski, S.K.; Baggetto, L.; Zhao, R.; Cheng, Q.; Yarger, J.L.; Veith, G.M.; Ellis-Terrell, C.; et al. Type I Clathrates as Novel Silicon Anodes: An Electrochemical and Structural Investigation. Adv. Sci. 2015, 2, 1500057. [Google Scholar] [CrossRef] [PubMed]
- Pearson, W.B. Pearson’s Handbook of Crystallographic Data for Intermetallic Phases; American Society for Metals: Metals Park, OH, USA, 1985. [Google Scholar]
- Pauling, L. The Nature of the Chemical Bond; Cornell University Press: Ithaca, NY, USA, 1960. [Google Scholar]
- Wu, H.; Zhang, H.; Cheng, X.; Cai, L. Structure and Thermodynamic Properties of Li8Al3Si5. J. Alloys Compd. 2006, 426, 57–63. [Google Scholar] [CrossRef]
- Kevorkov, D.; Gröbner, J.; Schmid-Fetzer, R. The Al–Li–Si System: 1. A New Structure Type Li8Al3Si5 and the Ternary Solid-State Phase Equilibria. J. Solid State Chem. 2001, 156, 500–505. [Google Scholar] [CrossRef]
- Zheng, C.; Hoffmann, R.; Nesper, R.; von Schnerring, H.-G. Site Preferences and Bond Length Differences in CaAl2Si2-Type Zintl Compounds. J. Am. Chem. Soc. 1986, 108, 1876–1884. [Google Scholar] [CrossRef]
- Burdett, J.K.; Miller, G.J. Fragment Formalism in Main-Group Solids: Applications to Aluminum Boride (AlB2), Calcium Aluminum Silicide (CaAl2Si2), Barium-Aluminum (BaAl4), and Related Materials. Chem. Mater. 1990, 2, 12–26. [Google Scholar] [CrossRef]
- Kauzlarich, S.M.; Condron, C.L.; Wassei, J.K.; Ikeda, T.; Snyder, G.J. Structure and High-Temperature Thermoelectric Properties of SrAl2Si2. J. Solid State Chem. 2009, 182, 240–245. [Google Scholar] [CrossRef]
- Zevalkink, A.; Bobnar, M.; Schwarz, U.; Grin, Y. Making and Breaking Bonds in Superconducting SrAl4−xSix (0 ≤ x ≤ 2). Chem. Mater. 2017, 29, 1236–1244. [Google Scholar] [CrossRef]
- Condron, C.L.; Hope, H.; Paula, P.M.B.; Schultz, A.J.; Kauzlarich, S.M. Synthesis, Structure, and Properties of BaAl2Si2. Inorg. Chem. 2007, 46, 4523–4529. [Google Scholar] [CrossRef]
- Kranenberg, C.; Johrendt, D.; Mewis, A. Untersuchungen zum Existenzgebiet des CaAl2Si2-Strukturtyps bei ternären Siliciden. Z. Anorg. Allg. Chem. 1999, 625, 1787–1793. [Google Scholar] [CrossRef]
- Kranenberg, C.; Mewis, A. Darstellung und Kristallstrukturen von Ln2Al3Si2 and Ln2AlSi2 (Ln: Y, Tb–Lu). Z. Anorg. Allg. Chem. 2000, 626, 1448–1453. [Google Scholar] [CrossRef]
- Condron, C.L.; Porter, R.; Guo, T.; Kauzlarich, S.M. Crystal Structures, Raman Spectroscopy, and Magnetic Properties of Ba7.5Al13Si29 and Eu0.27Ba7.22Al13Si29. Inorg. Chem. 2005, 44, 9185–9191. [Google Scholar] [CrossRef] [PubMed]
- Gulebaglan, S.E.; Dogan, E.K. Prediction the Structural, Electronic, Elastic and Dynamical Properties of LiAlGe and LiInGe Half-Heusler Crystals by Density Functional Theory. Mater. Today Commun. 2022, 32, 104082. [Google Scholar] [CrossRef]
- Carrillo-Cabrera, W.; Gil, R.C.; Grin, Y. Refinement of the crystal structure of monocalcium dialuminide digermanide, Ca[Al2Ge2]. Z. Krist. New Cryst. Struct. 2001, 216, 535–536. [Google Scholar] [CrossRef]
- Suen, N.-T.; Bobev, S. Structures of Three Alkaline-Earth Metal Germanides Refined from Single-Crystal X-ray Diffraction Data. Chemistry 2022, 4, 1429–1438. [Google Scholar] [CrossRef]
- Kranenberg, C.; Johrendt, D.; Mewis, A. The Stability Range of the CaAl2Si2-Type Structure in Case of LnAl2Ge2 Compounds. Solid State Sci. 2002, 4, 261–265. [Google Scholar] [CrossRef]
- Leoni, S.; Carrillo-Cabrera, W.; Schnelle, W.; Grin, Y. BaAl2Ge2: Synthesis, Crystal Structure, Magnetic and Electronic Properties, Chemical Bonding, and Atomistic Model of the α↔β Phase Transition. Solid State Sci. 2003, 5, 139–148. [Google Scholar] [CrossRef]
- Widera, A.; Eisenmann, B.; Schäfer, H.; Turban, K. Darstellung und Kristallstruktur von Ca3Al2Ge2, Sr3Al2Ge2 und Ba3Al2Ge2/Preparation and Crystal Structure of Ca3Al2Ge2, Sr3Al2Ge2 and Ba3Al2Ge2. Z. Naturforsch B 1976, 31, 1592–1595. [Google Scholar] [CrossRef]
- Kranenberg, C.; Johrendt, D.; Mewis, A.; Pöttgen, R.; Kotzyba, G.; Rosenhahn, C.; Mosel, B.D. Structure and Properties of the Compounds LnAl2X2 (Ln = Eu, Yb; X = Si, Ge). Solid State Sci. 2000, 2, 215–222. [Google Scholar] [CrossRef]
- Somer, M.; Carrillo-Cabrera, W.; Peters, E.M.; Peters, K.; von Schnering, H.G. Crystal structure of disodium lithium diphosphidoaluminate, Na2LiAlP2. Z. Krist. Cryst. Mater. 1995, 210, 778–779. [Google Scholar] [CrossRef]
- Ovchinnikov, A.; Bobev, S. Zintl Phases with Group 15 Elements and the Transition Metals: A Brief Overview of Pnictides with Diverse and Complex Structures. J. Solid State Chem. 2019, 270, 346–359. [Google Scholar] [CrossRef]
- Fernandes, R.M.; Coldea, A.I.; Ding, H.; Fisher, I.R.; Hirschfeld, P.J.; Kotliar, G. Iron Pnictides and Chalcogenides: A New Paradigm for Superconductivity. Nature 2022, 601, 35–44. [Google Scholar] [CrossRef] [PubMed]
- Mizuguchi, Y.; Takano, Y. Superconductivity in PbO-Type Fe Chalcogenides. Z. Krist. 2011, 226, 417–434. [Google Scholar] [CrossRef]
- SADABS NT, Version 2.10; Bruker Analytical X-ray Systems, Inc.: Madison, WI, USA, 2001.
- SHELXTL, Version 6.12; Bruker Analytical X-ray Systems, Inc.: Madison, WI, USA, 2001.
Figure 1.
Schematic representation of the three types of crystal structures adopted by the A2LiAlTt2 (A = Ca, Sr, Ba; Tt = Si, Ge) compounds. Green, yellow, and blue colors represent the Al, Li, and Tt atoms, respectively; mixed-occupied Al/Li positions are shown as two-tone. Ca, Sr, and Ba atoms can be distinguished by the light red, dark red, and magenta spheres, respectively. Bonds (sticks) are drawn only between neighboring Al, Li, and Tt atoms. Unit cells are outlined.
Figure 1.
Schematic representation of the three types of crystal structures adopted by the A2LiAlTt2 (A = Ca, Sr, Ba; Tt = Si, Ge) compounds. Green, yellow, and blue colors represent the Al, Li, and Tt atoms, respectively; mixed-occupied Al/Li positions are shown as two-tone. Ca, Sr, and Ba atoms can be distinguished by the light red, dark red, and magenta spheres, respectively. Bonds (sticks) are drawn only between neighboring Al, Li, and Tt atoms. Unit cells are outlined.
Figure 2.
Schematic representation of the orthorhombic crystal structure of Ca2LiAlGe2 (TiNiSi/SrMgSi structure type, space group Pnma). Green, blue, and red colors represent Al/Li, Ge, and Ca atoms, respectively. Anisotropic displacement parameters are drawn at the 95% probability level. Unit cell is outlined. Distances (rounded to fewer significant figures) are indicated. A full list of all values with their standard deviations can be found in Table 5.
Figure 2.
Schematic representation of the orthorhombic crystal structure of Ca2LiAlGe2 (TiNiSi/SrMgSi structure type, space group Pnma). Green, blue, and red colors represent Al/Li, Ge, and Ca atoms, respectively. Anisotropic displacement parameters are drawn at the 95% probability level. Unit cell is outlined. Distances (rounded to fewer significant figures) are indicated. A full list of all values with their standard deviations can be found in Table 5.
Figure 3.
(left) Schematic representation of the orthorhombic crystal structure of Sr2LiAlGe2 (Na2LiAlP2 structure type, space group Cmce). The chosen projection is to highlight the similarity to another structure, and the unit cell is outlined. Green, yellow, blue, and red colors represent the Al, Li, Ge, and Sr atoms, respectively. Anisotropic displacement parameters are drawn at the 95% probability level. (right) A projection of a single [LiAlGe2] slab, viewed down the b-axis. The checkerboard-like pattern of all edge-shared tetrahedra is emphasized.
Figure 3.
(left) Schematic representation of the orthorhombic crystal structure of Sr2LiAlGe2 (Na2LiAlP2 structure type, space group Cmce). The chosen projection is to highlight the similarity to another structure, and the unit cell is outlined. Green, yellow, blue, and red colors represent the Al, Li, Ge, and Sr atoms, respectively. Anisotropic displacement parameters are drawn at the 95% probability level. (right) A projection of a single [LiAlGe2] slab, viewed down the b-axis. The checkerboard-like pattern of all edge-shared tetrahedra is emphasized.
Figure 4.
Schematic representation of the tetragonal crystal structure of Ba2LiAlGe2 (PbFCl structure type, space group P4/nmm). Green, blue, and magenta colors represent the Al/Li, Ge, and Ba atoms, respectively. Anisotropic displacement parameters are drawn at the 95% probability level.
Figure 4.
Schematic representation of the tetragonal crystal structure of Ba2LiAlGe2 (PbFCl structure type, space group P4/nmm). Green, blue, and magenta colors represent the Al/Li, Ge, and Ba atoms, respectively. Anisotropic displacement parameters are drawn at the 95% probability level.
Table 1.
Selected crystallographic data for Ca2LiAlSi2 and Ca2LiAlGe2 (T = 200(2) K; Mo Kα, λ = 0.71073 Å, space group Pnma (no. 62)). Pearson symbol for this structure is oP12 [25].
Table 1.
Selected crystallographic data for Ca2LiAlSi2 and Ca2LiAlGe2 (T = 200(2) K; Mo Kα, λ = 0.71073 Å, space group Pnma (no. 62)). Pearson symbol for this structure is oP12 [25].
| Chemical Formula | Ca2LiAlSi2 | Ca2LiAlGe2 |
|---|---|---|
| Formula weight/g mol−1 | 170.26 | 259.26 |
| a/Å | 7.2914 (7) | 7.3255 (8) |
| b/Å | 4.3411 (4) | 4.3801 (5) |
| c/Å | 7.9511 (8) | 8.0246 (9) |
| V/Å3 | 251.67 (4) | 257.48 (5) |
| ρcalc./g cm−3 | 2.25 | 3.34 |
| μ(Mo-Kα)/cm−1 | 27.3 | 136.5 |
| Collected reflections | 1999 | 5187 |
| Independent reflections | 430 | 450 |
| Goodness-of-fit | 1.074 | 1.044 |
| R1 (I > 2σ(I)) a | 0.0189 | 0.0287 |
| wR2 (I > 2σ(I)) a | 0.0407 | 0.0535 |
| R1 (all data) a | 0.0233 | 0.0361 |
| wR2 (all data) a | 0.0417 | 0.0553 |
| Δρmax,min/e−·Å−3 | 0.38, −0.25 | 0.65, −0.76 |
a R1 = ∑||Fo| − |Fc||/∑|Fo|; wR2 = [∑[w(Fo2 − Fc2)2]/∑[w(Fo2)2]]1/2, where w = 1/[σ2Fo2 + (AP)2 + (BP)] and P = (Fo2 + 2Fc2)/3; A and B are the respective weight coefficients (see the CIFs).
Table 2.
Selected crystallographic data for Sr2LiAlSi2 and Sr2LiAlGe2 (T = 200(2) K; Mo Kα, λ = 0.71073 Å, space group Cmce (no. 64)). Pearson symbol for this structure is oS48 [25].
Table 2.
Selected crystallographic data for Sr2LiAlSi2 and Sr2LiAlGe2 (T = 200(2) K; Mo Kα, λ = 0.71073 Å, space group Cmce (no. 64)). Pearson symbol for this structure is oS48 [25].
| Chemical Formula | Sr2LiAlSi2 | Sr2LiAlGe2 |
|---|---|---|
| Formula weight/g mol−1 | 265.34 | 354.34 |
| a/Å | 12.5283 (4) | 12.616 (3) |
| b/Å | 14.4678 (7) | 14.574 (3) |
| c/Å | 6.2735 (7) | 6.3133 (3) |
| V/Å3 | 1137.1 (2) | 1160.7 (4) |
| ρcalc./g cm−3 | 3.10 | 4.06 |
| μ(Mo-Kα)/cm−1 | 191.7 | 285.3 |
| Collected reflections | 6957 | 4519 |
| Independent reflections | 908 | 848 |
| Goodness-of-fit | 1.18 | 1.10 |
| R1 (I > 2σ(I)) a | 0.0263 | 0.0334 |
| wR2 (I > 2σ(I)) a | 0.0434 | 0.0551 |
| R1 (all data) a | 0.0388 | 0.0619 |
| wR2 (all data) a | 0.0464 | 0.0617 |
| Δρmax,min/e−·Å−3 | 1.28, −0.72 | 1.27, −0.94 |
a R1 = ∑||Fo| − |Fc||/∑|Fo|; wR2 = [∑[w(Fo2 − Fc2)2]/∑[w(Fo2)2]]1/2, where w = 1/[σ2Fo2 + (AP)2 + (BP)] and P = (Fo2 + 2Fc2)/3; A and B are the respective weight coefficients (see the CIFs).
Table 3.
Selected crystallographic data for Ba2LiAlSi2 and Ba2LiAlGe2 (T = 200(2) K; Mo Kα, λ = 0.71073 Å, space group P4/nmm (no. 129)). Pearson symbol for this structure is tP6 [25].
Table 3.
Selected crystallographic data for Ba2LiAlSi2 and Ba2LiAlGe2 (T = 200(2) K; Mo Kα, λ = 0.71073 Å, space group P4/nmm (no. 129)). Pearson symbol for this structure is tP6 [25].
| Chemical Formula | Ba2LiAlSi2 | Ba2LiAlGe2 |
|---|---|---|
| Formula weight/g mol−1 | 364.78 | 453.78 |
| a/Å | 4.5574 (6) | 4.5965 (7) |
| c/Å | 7.5582 (3) | 7.5822 (7) |
| V/Å3 | 156.98 (4) | 160.19 (5) |
| ρcalc./g cm−3 | 3.86 | 4.70 |
| μ(Mo-Kα)/cm−1 | 128.4 | 214.1 |
| Collected reflections | 1309 | 1316 |
| Independent reflections | 172 | 170 |
| Goodness-of-fit | 1.108 | 1.099 |
| R1 (I > 2σ(I)) a | 0.0167 | 0.0226 |
| wR2 (I > 2σ(I)) a | 0.0339 | 0.0443 |
| R1 (all data) a | 0.0194 | 0.0286 |
| wR2 (all data) a | 0.0348 | 0.0456 |
| Δρmax,min/e−·Å−3 | 1.10, −0.71 | 0.95, −0.91 |
a R1 = ∑||Fo| − |Fc||/∑|Fo|; wR2 = [∑[w(Fo2 − Fc2)2]/∑[w(Fo2)2]]1/2, where w = 1/[σ2Fo2 + (AP)2 + (BP)] and P = (Fo2 + 2Fc2)/3; A and B are the respective weight coefficients (see the CIFs).
Table 4.
Refined atomic coordinates for A2LiAlTt2 (A = Ca, Sr, Ba; Tt = Si, Ge). In all cases, unless noted otherwise, the atoms are refined anisotropically and the values of their equivalent isotropic displacement parameters (Ueq/Å2) are given as well.
Table 4.
Refined atomic coordinates for A2LiAlTt2 (A = Ca, Sr, Ba; Tt = Si, Ge). In all cases, unless noted otherwise, the atoms are refined anisotropically and the values of their equivalent isotropic displacement parameters (Ueq/Å2) are given as well.
| Ca2LiAlSi2 | |||||
|---|---|---|---|---|---|
| Atom | Wyckoff Position | x | y | z | Ueq/Å2 |
| Ca | 4c | 0.0100 (1) | 1/4 | 0.6907 (1) | 0.013 (1) |
| Li/Al a | 4c | 0.1500 (1) | 1/4 | 0.0708 (1) | 0.012 (1) |
| Si | 4c | 0.2802 (1) | 1/4 | 0.3960 (1) | 0.016 (1) |
| Ca2LiAlGe2 | |||||
| Ca | 4c | 0.0104 (1) | 1/4 | 0.6923 (1) | 0.014 (1) |
| Li/Al a | 4c | 0.1500 (1) | 1/4 | 0.0704 (3) | 0.018 (1) |
| Ge | 4c | 0.2780 (1) | 1/4 | 0.3959 (1) | 0.015 (1) |
| Sr2LiAlSi2 | |||||
| Sr1 | 8e | 1/4 | 0.3349 (1) | 1/4 | 0.009 (1) |
| Sr2 | 8f | 0 | 0.1634 (1) | 0.2283 (1) | 0.010 (1) |
| Li | 8d | 0.1254 (7) | 0 | 0 | 0.01 b |
| Al | 8d | 0.3720 (1) | 0 | 0 | 0.012 (1) |
| Si1 | 8e | 1/4 | 0.1028 (1) | 1/4 | 0.009 (1) |
| Si2 | 8f | 0 | 0.3968 (1) | 0.2235 (2) | 0.010 (1) |
| Sr2LiAlGe2 | |||||
| Sr1 | 8e | 1/4 | 0.3352 (1) | 1/4 | 0.010 (1) |
| Sr2 | 8f | 0 | 0.1634 (1) | 0.2294 (1) | 0.011 (1) |
| Li | 8d | 0.1257 (5) | 0 | 0 | 0.01 b |
| Al | 8d | 0.3726 (1) | 0 | 0 | 0.015 (1) |
| Ge1 | 8e | 1/4 | 0.1047 (1) | 1/4 | 0.009 (1) |
| Ge2 | 8f | 0 | 0.3951 (1) | 0.2276 (1) | 0.010 (1) |
| Ba2LiAlSi2 | |||||
| Ba | 2c | 1/4 | 1/4 | 0.6732 (1) | 0.018 (1) |
| Li/Al a | 2a | 3/4 | 1/4 | 0 | 0.015 (1) |
| Si | 2c | 1/4 | 1/4 | 0.1914 (3) | 0.020 (1) |
| Ba2LiAlGe2 | |||||
| Ba | 2c | 1/4 | 1/4 | 0.6743 (1) | 0.016 (1) |
| Li/Al a | 2a | 3/4 | 1/4 | 0 | 0.016 (1) |
| Ge | 2c | 1/4 | 1/4 | 0.1953 (1) | 0.018 (1) |
a Refined occupancies were constrained to be Li:Al = 50:50. b Refined isotropically (Ueq = Uiso) and with value constrained to the average Ueq value for the heavy atoms in the structure.
Table 5.
Selected interatomic distances (Å) in Ca2LiAlSi2 and Ca2LiAlGe2.
| Ca2LiAlSi2 | Ca2LiAlGe2 | ||
|---|---|---|---|
| Atom Pair | Distance/Å | Atom Pair | Distance/Å |
| Si—Li/Al | 2.709 (1) | Ge—Li/Al | 2.736 (3) |
| Si—Li/Al | 2.754 (1) | Ge—Li/Al | 2.776 (3) |
| Si—Li/Al × 2 | 2.6272 (6) | Ge—Li/Al × 2 | 2.653 (2) |
| Ca—Li/Al | 3.190 (1) | Ca—Li/Al | 3.201 (3) |
| Ca—Li/Al × 2 | 3.1093(7) | Ca—Li/Al × 2 | 3.130 (2) |
| Ca—Li/Al | 3.349 (1) | Ca—Li/Al | 3.381 (3) |
| Ca—Li/Al × 2 | 3.4301 (7) | Ca—Li/Al × 2 | 3.458 (2) |
| Ca—Si | 3.0615 (7) | Ca—Ge | 3.082 (1) |
| Ca—Si × 2 | 3.1089(5) | Ca—Ge × 2 | 3.1242 (7) |
| Ca—Si × 2 | 3.1168 (5) | Ca—Ge × 2 | 3.1414 (8) |
| Li/Al—Si × 2 | 2.6272 (6) | Li/Al—Ge × 2 | 2.653 (2) |
| Li/Al—Si | 2.709 (1) | Li/Al—Ge | 2.735 (3) |
| Li/Al—Si | 2.754 (1) | Li/Al—Ge | 2.776 (3) |
Table 6.
Selected interatomic distances (Å) in Sr2LiAlSi2 and Sr2LiAlGe2.
| Sr2LiAlSi2 | Sr2LiAlGe2 | ||
|---|---|---|---|
| Atom Pair | Distance/Å | Atom Pair | Distance/Å |
| Si1—Al × 2 | 2.647 (1) | Ge1—Al × 2 | 2.686 (2) |
| Si1—Li × 2 | 2.666 (5) | Ge1—Li × 2 | 2.70 (1) |
| Si2—Al × 2 | 2.600 (1) | Ge2—Al × 2 | 2.643 (2) |
| Si2—Li × 2 | 2.775 (5) | Ge2—Li × 2 | 2.79 (1) |
| Sr1—Al × 2 | 3.2413 (9) | Sr1—Al × 2 | 3.264 (2) |
| Sr1—Li × 2 | 3.257 (4) | Sr1—Li × 2 | 3.275 (9) |
| Sr1—Si1 × 2 | 3.2639 (5) | Sr1—Ge1 × 2 | 3.2758 (7) |
| Sr1—Si1 | 3.358 (2) | Sr1—Ge2 | 3.360 (2) |
| Sr1—Si2 × 2 | 3.2621 (5) | Sr1—Ge2 × 2 | 3.2756 (7) |
| Sr2—Al × 2 | 3.3260 (9) | Sr2—Al × 2 | 3.343 (2) |
| Sr2—Li × 2 | 3.179 (5) | Sr2—Li × 2 | 3.206 (9) |
| Sr2—Si1 × 2 | 3.2554 (5) | Sr2—Ge1 × 2 | 3.2706 (7) |
| Sr2—Si2 | 3.226 (1) | Sr2—Ge2 | 3.2585 (6) |
| Sr2—Si2 | 3.285 (1) | Sr2—Ge2 | 3.281 (1) |
| Sr2—Si2 | 3.378 (2) | Sr2—Ge2 | 3.377 (2) |
| Al—Si1 × 2 | 2.647 (1) | Al—Ge1 × 2 | 2.686 (2) |
| Al—Si2 × 2 | 2.600 (1) | Al—Ge2 × 2 | 2.643 (2) |
| Li—Si1 × 2 | 2.666 (5) | Li—Ge1 × 2 | 2.70 (1) |
| Li—Si2 × 2 | 2.775 (5) | Li—Ge2 × 2 | 2.79 (1) |
Table 7.
Selected interatomic distances (Å) in Ba2LiAlSi2 and Ba2LiAlGe2.
| Ba2LiAlSi2 | Ba2LiAlGe2 | ||
|---|---|---|---|
| Atom Pair | Distance/Å | Atom Pair | Distance/Å |
| Ba—Li/Al × 4 | 3.3607 (5) | Ba—Li/Al × 4 | 3.3738 (7) |
| Ba—Si × 4 | 3.3812 (7) | Ba—Ge × 4 | 3.3974 (6) |
| Ba—Si | 3.641 (2) | Ba—Ge | 3.632 (2) |
| Si—Li/Al × 4 | 2.6992 (1) | Ge—Li/Al × 4 | 2.7340 (7) |
| Li/Al—Si × 4 | 2.6992 (1) | Li/Al—Ge × 4 | 2.7340 (7) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
MDPI and ACS Style
Kontomaris, P.; Darone, G.M.; Paredes-Quevedo, L.C.; Bobev, S. The Synthesis and Crystal Structure of Six Quaternary Lithium-Alkaline Earth Metal Alumo-Silicides and Alumo-Germanides, A2LiAlTt2 (A = Mg, Ca, Sr, Ba; Tt = Si, Ge). Inorganics 2023, 11, 351. https://doi.org/10.3390/inorganics11090351
AMA Style
Kontomaris P, Darone GM, Paredes-Quevedo LC, Bobev S. The Synthesis and Crystal Structure of Six Quaternary Lithium-Alkaline Earth Metal Alumo-Silicides and Alumo-Germanides, A2LiAlTt2 (A = Mg, Ca, Sr, Ba; Tt = Si, Ge). Inorganics. 2023; 11(9):351. https://doi.org/10.3390/inorganics11090351
Chicago/Turabian StyleKontomaris, Paraskevi, Gregory M. Darone, Laura C. Paredes-Quevedo, and Svilen Bobev. 2023. "The Synthesis and Crystal Structure of Six Quaternary Lithium-Alkaline Earth Metal Alumo-Silicides and Alumo-Germanides, A2LiAlTt2 (A = Mg, Ca, Sr, Ba; Tt = Si, Ge)" Inorganics 11, no. 9: 351. https://doi.org/10.3390/inorganics11090351
Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.
