Modelling and Design of Holographic Optical Elements for Beam-Coupling Applications for a Range of Incident Beam Angles
Abstract
1. Introduction
2. Modelling and Design
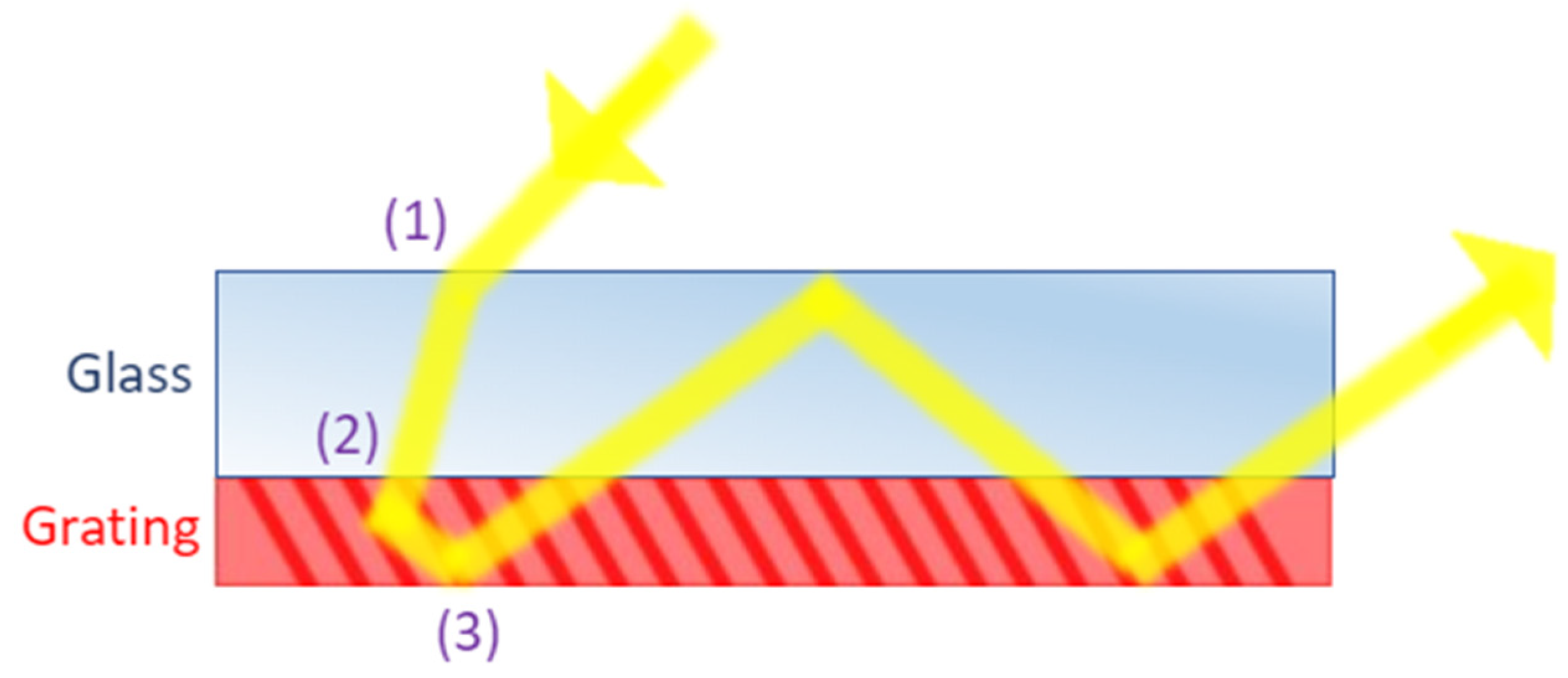
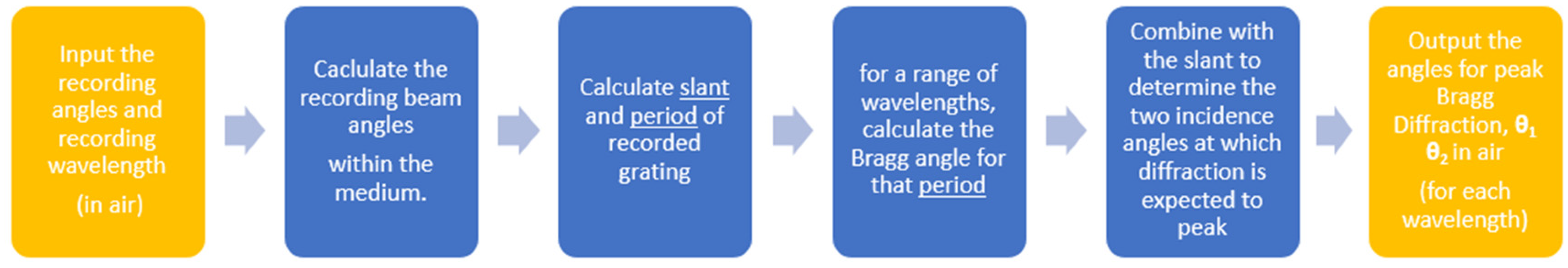
2.1. Identification of HOE Parameters for Desired Input and Output Angles
2.2. Calculation of the Appropriate Recording Beam Angles for a Range of Recording Wavelengths
2.3. Calculation of the Appropriate Recording Beam Angles for Fabrication of Couplers
2.4. Modelling the Two Incident Angles at Which Bragg Diffraction Peaks Appear for a Range of Input Beam Wavelengths
3. Fabrication of VHOEs—Materials and Method
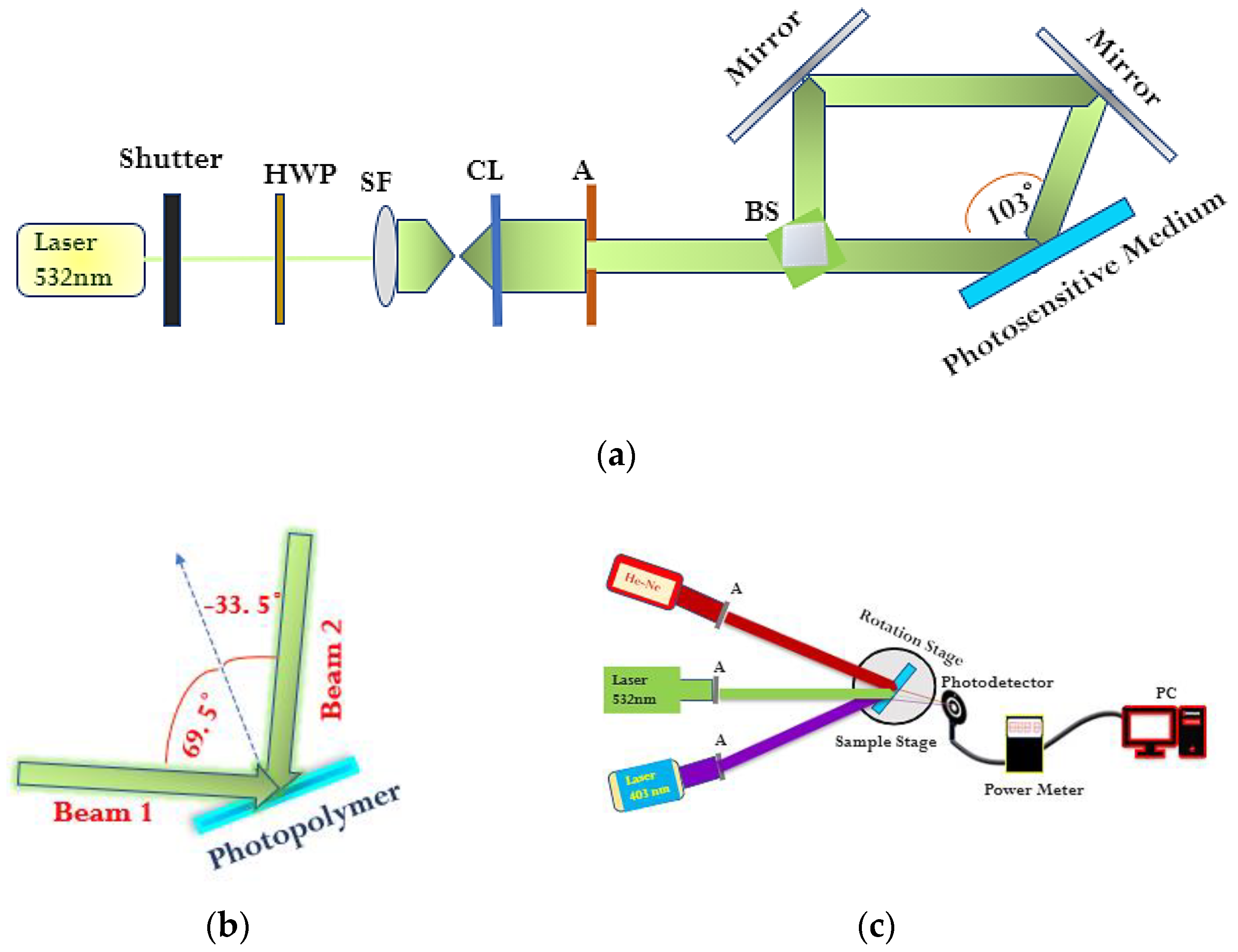
3.1. Fabrication of VHOEs
3.2. Characterisation of VHOE Performance
4. Experimental Results
4.1. Optimisation of the Holographic Recording Conditions for Couplers
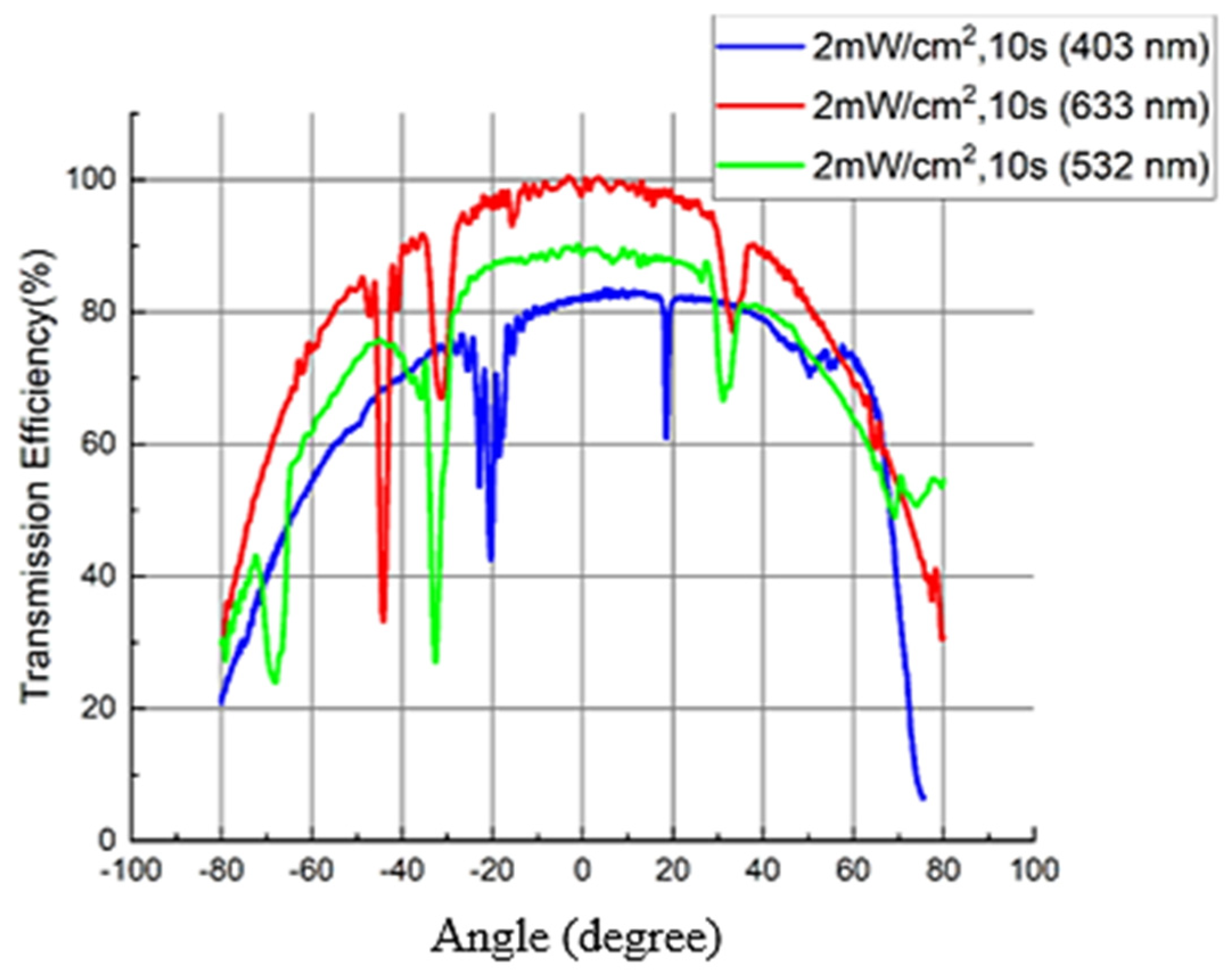
4.2. Multi-Wavelength Angular Characterisation of the Fabricated Couplers
5. Discussion
5.1. Bragg Angles for the Recorded Structure at the Three Probe Wavelengths
5.2. Spurious Gratings and Additional Diffraction Peaks
- The back reflection of the two primary recording beams interfering with one another (creates a transmission grating with the same spatial frequency as the intended coupler but with opposite slant, the beams have similar intensities, so a high-contrast pattern is recorded);
- The back reflection of each beam interfering with that beam (creates two non-slanted reflection gratings, presumably quite weak due to the poor beam ratio);
- The back reflection from each beam interfering with the opposite main recording beam (creates two slanted reflection gratings, presumably quite weak due to the poor beam ratio).
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ashley, J.; Bernal, M.-P.; Burr, G.W.; Coufal, H.; Guenther, H.; Hoffnagle, J.A.; Jefferson, C.M.; Marcus, B.; Macfarlane, R.M.; Shelby, R.M.; et al. Holographic data storage technology. IBM J. Res. Dev. 2000, 44, 341–368. [Google Scholar] [CrossRef]
- Dhar, L.; Hill, A.; Curtis, K.; Wilson, W.; Ayres, M. Holographic Data Storage: From Theory to Practical Systems; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Li, J.; Hu, P.; Jin, J.; Wang, J.; Liu, J.; Wu, J.; Lin, X.; Tan, X. Highly sensitive photopolymer for holographic data storage. Opt. Express 2022, 30, 40599–40610. [Google Scholar] [CrossRef] [PubMed]
- Miller, J.M.; de Beaucoudrey, N.; Chavel, P.; Turunen, J.; Cambril, E. Design and fabrication of binary slanted surface-relief gratings for a planar optical interconnection. Appl. Opt. 1997, 36, 5717–5727. [Google Scholar] [CrossRef] [PubMed]
- Soares, O. Holographic coupler for fiber optics. Opt. Eng. 1981, 20, 740–745. [Google Scholar] [CrossRef]
- Singh, A.K.; Yadav, A.; Khan, A.A.; Roy, S.; Yadav, H.L. Design and analysis of holographic optical elements for their use as couplers with appreciable efficiency at different optical transmission windows. Optik 2022, 261, 169184. [Google Scholar] [CrossRef]
- He, C.; Neild, A.; Helmerson, K.; Cincotta, S.; Zuk, J.; Armstrong, J. Dual-aperture hologram receiver for visible light communications. Opt. Commun. 2021, 490, 126943. [Google Scholar] [CrossRef]
- Shimizu, S.; Okamoto, A.; Mizukawa, F.; Ogawa, K.; Tomita, A.; Takahata, T.; Shinada, S.; Wada, N. Volume holographic spatial mode demultiplexer with a dual-wavelength method. Appl. Opt. 2018, 57, 146–153. [Google Scholar] [CrossRef]
- Zhang, Y.; Fan, H.; Poon, T.-C. Optical image processing using acousto-optic modulators as programmable volume holograms: A review. Chin. Opt. Lett. 2022, 20, 021101. [Google Scholar] [CrossRef]
- Alcaraz, P.E.; Nero, G.; Blanche, P.-A. Bandwidth optimization for the Advanced Volume Holographic Filter. Opt. Express 2022, 30, 576–587. [Google Scholar] [CrossRef]
- Park, J.-H.; Lee, B. Holographic techniques for augmented reality and virtual reality near-eye displays. Light Adv. Manuf. 2022, 3, 1–14. [Google Scholar] [CrossRef]
- Putilin, A.; Morozov, A.; Kopenkin, S.; Dubynin, S.; Borodin, Y.P. Holographic waveguide periscopes in augmented reality displays. Opt. Spectrosc. 2020, 128, 1828–1836. [Google Scholar] [CrossRef]
- Li, G.; Lee, D.; Jeong, Y.; Cho, J.; Lee, B. Holographic display for see-through augmented reality using mirror-lens holographic optical element. Opt. Lett. 2016, 41, 2486–2489. [Google Scholar] [CrossRef] [PubMed]
- Piao, J.-A.; Li, G.; Piao, M.-L.; Kim, N. Full Color Holographic Optical Element Fabrication for Waveguide-type Head Mounted Display Using Photopolymer. J. Opt. Soc. Korea 2013, 17, 242–248. [Google Scholar] [CrossRef]
- Wu, Z.; Liu, J.; Wang, Y. A high-efficiency holographic waveguide display system with a prism in-coupler. J. Soc. Inf. Disp. 2013, 21, 524–528. [Google Scholar] [CrossRef]
- Zhang, N.; Liu, J.; Han, J.; Li, X.; Yang, F.; Wang, X.; Hu, B.; Wang, Y. Improved holographic waveguide display system. Appl. Opt. 2015, 54, 3645–3649. [Google Scholar] [CrossRef]
- Lv, Z.; Liu, J.; Xiao, J.; Kuang, Y. Integrated holographic waveguide display system with a common optical path for visible and infrared light. Opt. Express 2018, 26, 32802–32811. [Google Scholar] [CrossRef] [PubMed]
- Gu, Y.; Weng, Y.; Wei, R.; Shen, Z.; Wang, C.; Zhang, L.; Zhang, Y. Holographic waveguide display with large field of view and high light efficiency based on polarized volume holographic grating. IEEE Photonics J. 2021, 14, 1–7. [Google Scholar] [CrossRef]
- Shen, Z.; Wang, Z.; Weng, Y.; Zhang, Y. 15.2: Design and Realization of Full-Color VHG Holographic Waveguide Display. In SID Symposium Digest of Technical Papers, Proceedings of the International Conference on Display Technology (ICDT) 2022, Fuzhou, China, 9–12 July 2022; Shen, Z., Wang, C., Weng, Y., Zhang, Y., Eds.; Wiley Online Library: Hoboken, NJ, USA, 2022. [Google Scholar]
- Collados, M.V.; Chemisana, D.; Atencia, J. Holographic solar energy systems: The role of optical elements. Renew. Sustain. Energy Rev. 2016, 59, 130–140. [Google Scholar] [CrossRef]
- Kostuk, R.K.; Rosenberg, G. Analysis and design of holographic solar concentrators. In High and Low Concentration for Solar Electric Applications III, Proceedings of the SOLAR ENERGY + APPLICATIONS, San Diego, CA, USA, 10–14 August 2008; Kostuk, R.K., Rosenberg, G., Eds.; SPIE: Bellingham, WA, USA, 2008. [Google Scholar]
- Naydenova, I.; Akbari, H.; Dalton, C.; Yahya, M.; Ilyas, S.M.; Wei, C.P.T.; Toal, V.; Martin, S. Photopolymer Holographic Optical Elements for Application in Solar Energy Concentrators. Holography-Basic Principles and Contemporary Applications; Mihaylova, E., Ed.; InTech: London, UK, 2013. [Google Scholar]
- Zhang, D.; Gordon, M.; Russo, J.M.; Vorndran, S.D.; Kostuk, R.K. Spectrum-splitting photovoltaic system using transmission holographic lenses. J. Photonics Energy 2013, 3, 034597. [Google Scholar] [CrossRef]
- Ferrara, M.A.; Bianco, G.; Borbone, F.; Centore, R.; Striano, V.; Coppola, G. Volume holographic optical elements as solar concentrators. In Holographic Materials and Optical Systems; IntechOpen: London, UK, 2017; pp. 27–50. [Google Scholar]
- Müller, H. Application of holographic optical elements in buildings for various purposes like daylighting, solar shading and photovoltaic power generation. Renew. Energy 1994, 5, 935–941. [Google Scholar] [CrossRef]
- Neipp, C.; Taleb, S.I.; Francés, J.; Fernández, R.; Puerto, D.; Calzado, E.M.; Gallego, S.; Beléndez, A. Analysis of the Imaging Characteristics of Holographic Waveguides Recorded in Photopolymers. Polymers 2020, 12, 1485. [Google Scholar] [CrossRef]
- Aspnes, E.D.; Castillo-Aguilella, J.E.; Courreges, R.D.; Hauser, P.S.; Stewart, K.R. Non-latitude and vertically mounted solar energy concentrators. U.S. Patent US20130312811A1, 28 November 2013. [Google Scholar]
- Aspnes, E.D.; Castillo-Aguilella, J.E.; Courreges, R.D.; Hauser, P.S.; Stewart, K.R. Solar energy concentrator with multiplexed diffraction gratings. U.S. Patent US20130319524A1, 5 December 2013. [Google Scholar]
- Kao, H.; Ma, J.; Wang, C.; Wu, T.; Su, P. Crosstalk-Reduced Double-Layer Half-Divided Volume Holographic Concentrator for Solar Energy Concentration. Sensors 2020, 20, 6903. [Google Scholar] [CrossRef] [PubMed]
- Huang, Q.; Ashley, P.R. Holographic Bragg grating input–output couplers for polymer waveguides at an 850-nm wavelength. Appl. Opt. 1997, 36, 1198–1203. [Google Scholar] [CrossRef]
- Wang, C.; Ma, J.; Kao, H.; Wu, T.; Su, P. Wide-Band High Concentration-Ratio Volume-Holographic Grating for Solar Concentration. Sensors 2020, 20, 6080. [Google Scholar] [CrossRef] [PubMed]
- Ludman, J.E. Holographic solar concentrator. Appl. Opt. 1982, 21, 3057–3058. [Google Scholar] [CrossRef] [PubMed]
- Bigler, C.M.; Blanche, P.-A.; Sarma, K. Holographic waveguide heads-up display for longitudinal image magnification and pupil expansion. Appl. Opt. 2018, 57, 2007–2013. [Google Scholar] [CrossRef] [PubMed]
- Stoeva, P.; Mikulchyk, T.; Rogers, B.; Oubaha, M.; Martin, S.; Ferrara, M.A.; Coppola, G.; Naydenova, I. Development of volume holographic optical element for application in wound healing monitoring. In Proceedings of the Photonics Ireland, Virtual Conference, 14–16 June 2021. [Google Scholar]
- Toal, V.; Whelan, M.; Volcan, A.; Naydenova, I.; Martin, S. Replay at optical communications wavelengths of holographic gratings recorded in the visible. In Proceedings of the SPIE, Varna, Bulgaria, 21–25 May 2005. [Google Scholar]
- Fernández, R.; Bleda, S.; Gallego, S.; Neipp, C.; Márquez, A.; Tomita, Y.; Pascual, I.; Beléndez, A. Holographic waveguides in photopolymers. Opt. Express 2019, 27, 827–840. [Google Scholar] [CrossRef]
- Kogelnik, H. Coupled wave theory for thick hologram gratings. Bell Syst. Tech. J. 1969, 48, 2909–2947. [Google Scholar] [CrossRef]
- Moharam, M.; Gaylord, T. Rigorous coupled-wave analysis of planar-grating diffraction. JOSA 1981, 71, 811–818. [Google Scholar] [CrossRef]
- Gaylord, T.K.; Moharam, M. Analysis and applications of optical diffraction by gratings. Proc. IEEE 1985, 73, 894–937. [Google Scholar] [CrossRef]
- Syms, R.; Solymar, L. Planar volume phase holograms formed in bleached photographic emulsions. Appl. Opt. 1983, 22, 1479–1496. [Google Scholar] [CrossRef] [PubMed]
- Vojtsek, P.; Kveton, M.; Richter, I. Complex method for angular-spectral analysis of volume phase diffraction gratings recorded in photopolymers. J. Eur. Opt. Soc. 2016, 11, 16009. [Google Scholar] [CrossRef]
- Mackey, D.; O’Reilly, P.; Naydenova, I. Theoretical modeling of the effect of polymer chain immobilization rates on holographic recording in photopolymers. JOSA A 2016, 33, 920–929. [Google Scholar] [CrossRef] [PubMed]
- Zhao, G.; Mouroulis, P. Diffusion model of hologram formation in dry photopolymer materials. J. Mod. Opt. 1994, 41, 1929–1939. [Google Scholar] [CrossRef]
- Sheridan, J.T.; Lawrence, J.R. Nonlocal-response diffusion model of holographic recording in photopolymer. JOSA A 2000, 17, 1108–1114. [Google Scholar] [CrossRef] [PubMed]
- Babeva, T.; Naydenova, I.; Mackey, D.; Martin, S.; Toal, V. Two-way diffusion model for short-exposure holographic grating formation in acrylamide-based photopolymer. JOSA B 2010, 27, 197–203. [Google Scholar] [CrossRef]
- Heifetz, A.; Shen, J.T.; Tsang, S.C.; Pati, G.S.; Lee, J.-K.; Shahriar, M. Angular directivity of diffracted wave in Bragg-mismatched readout of volume holographic gratings. In Proceedings of the Frontiers in Optics, San Jose, CA, USA, 16–20 September 2007; Optica Publishing Group: Washington, DC, USA, 2007. [Google Scholar]
- Bañares-Palacios, P.; Álvarez-Álvarez, S.; Marín-Sáez, J.; Collados, M.-V.; Chemisana, D.; Atencia, J. Broadband behavior of transmission volume holographic optical elements for solar concentration. Opt. Express 2015, 23, A671–A681. [Google Scholar] [CrossRef]
- Cody, D.; Naydenova, I. Theoretical modeling and deign of photonic structures in zeolite nanocomposites for gas sensing. Part I: Surface relief gratings. JOSA A 2017, 34, 2110–2119. [Google Scholar] [CrossRef]
- Guo, J.; Tu, Y.; Yang, L.; Wang, L.; Wang, B. Design of a multiplexing grating for color holographic waveguide. Opt. Eng. 2015, 54, 125105. [Google Scholar] [CrossRef]
- Close, D. Holographic optical elements. Opt. Eng. 1975, 14, 408–419. [Google Scholar] [CrossRef]
- Latta, J.N. Computer-based analysis of hologram imagery and aberrations. I. Hologram types and their nonchromatic aberrations. Appl. Opt. 1971, 10, 599–608. [Google Scholar] [CrossRef] [PubMed]
- Bruder, F.-K.; Fäcke, T.; Rölle, T. The chemistry and physics of Bayfol® HX film holographic photopolymer. Polymers 2017, 9, 472. [Google Scholar] [CrossRef] [PubMed]
- Kogelnik, H. Coupled wave theory for thick hologram gratings. In Landmark Papers On Photorefractive Nonlinear Optics; World Scientific: Singapore, 1995; pp. 133–171. [Google Scholar]








| Angle of Recording Beam 1 (in Air) | Angle of Recording Beam 2 (in Air) | Total Intensity Inside the Medium (mW/cm2) | Required Intensity Outside the Medium for Beam 1 (mW/cm2) | Required Intensity Outside the Medium for Beam 2 (mW/cm2) |
|---|---|---|---|---|
| −33.5° | 69.5° | 2 | 1.33 | 6.23 |
| 3 | 2.00 | 9.34 | ||
| 4 | 2.67 | 11.03 |
| Total Intensity (Inside Medium) (mW/cm²) | Exposure Time (sec) | 403 nm | 532 nm | 633 nm | |||
|---|---|---|---|---|---|---|---|
| Expected Angle (Degree) (Air) | Measured Angle (Degree) (Air) | Expected Angle (Degree) (Air) | Measured Angle (Degree) (Air) | Expected Angle (Degree) (Air) | Measured Angle (Degree) (Air) | ||
| 2 | 10 | −20.9 50.3 | −20.1 49.9 | −33.5 69.5 | −32.7 69.4 | −45.0 N/A | −44.2 N/A |
| 3 | 20 | −20.9 50.3 | −20.3 49.8 | −33.5 69.5 | −32.7 68.4 | −45.0 N/A | −44 N/A |
| 4 | 6 | −20.9 50.3 | −21.7 49.3 | −33.5 69.5 | −34 69.6 | −45.0 N/A | −45.6 N/A |
| Angle of Recording Beam 1 (Degree) (Air) | Angle of Recording Beam 2 (Degree) (Air) | 403 nm | 532 nm | 633 nm | |||
|---|---|---|---|---|---|---|---|
| Expected Spurious Grating Peak Position (Degree) (Air) | Measured Spurious Grating Peak Position (Degree) (Air) | Expected Spurious Grating Peak Position (Degree) (Air) | Measured Spurious Grating Peak Position (Degree) (Air) | Expected Spurious Grating Peak Position (Degree) (Air) | Measured Spurious Grating Peak Position (Degree) (Air) | ||
| 33.5 | −69.5 | −50.3 20.9 | −49.5 18.7 | −69.5 33.5 | −69.3 32.4 | NA 45 | NA Not Observed |
| 33.5 | −33.5 | NA NA | NA NA | −33.5 33.5 | −32.3 32.4 | NA NA | NA NA |
| 69.5 | −69.5 | NA NA | NA NA | −69.5 69.5 | −69.3 69.6 | −33.5 33.5 | −31.8 33.4 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chakraborty, D.; Georgiev, R.; Aspell, S.; Toal, V.; Naydenova, I.; Cody, D.; Martin, S. Modelling and Design of Holographic Optical Elements for Beam-Coupling Applications for a Range of Incident Beam Angles. Photonics 2022, 9, 936. https://doi.org/10.3390/photonics9120936
Chakraborty D, Georgiev R, Aspell S, Toal V, Naydenova I, Cody D, Martin S. Modelling and Design of Holographic Optical Elements for Beam-Coupling Applications for a Range of Incident Beam Angles. Photonics. 2022; 9(12):936. https://doi.org/10.3390/photonics9120936
Chicago/Turabian StyleChakraborty, Dipanjan, Rosen Georgiev, Sinead Aspell, Vincent Toal, Izabela Naydenova, Dervil Cody, and Suzanne Martin. 2022. "Modelling and Design of Holographic Optical Elements for Beam-Coupling Applications for a Range of Incident Beam Angles" Photonics 9, no. 12: 936. https://doi.org/10.3390/photonics9120936
APA StyleChakraborty, D., Georgiev, R., Aspell, S., Toal, V., Naydenova, I., Cody, D., & Martin, S. (2022). Modelling and Design of Holographic Optical Elements for Beam-Coupling Applications for a Range of Incident Beam Angles. Photonics, 9(12), 936. https://doi.org/10.3390/photonics9120936







