Thickness Measurement for Glass Slides Based on Chromatic Confocal Microscopy with Inclined Illumination
Abstract
1. Introduction
2. System Principle and Optical Path Design
2.1. Proposal of Chromatic Confocal Measurement with Inclined Illumination
2.1.1. Principle of Chromatic Confocal Measurement
2.1.2. Principle of Chromatic Confocal Measurement with Inclined Illumination
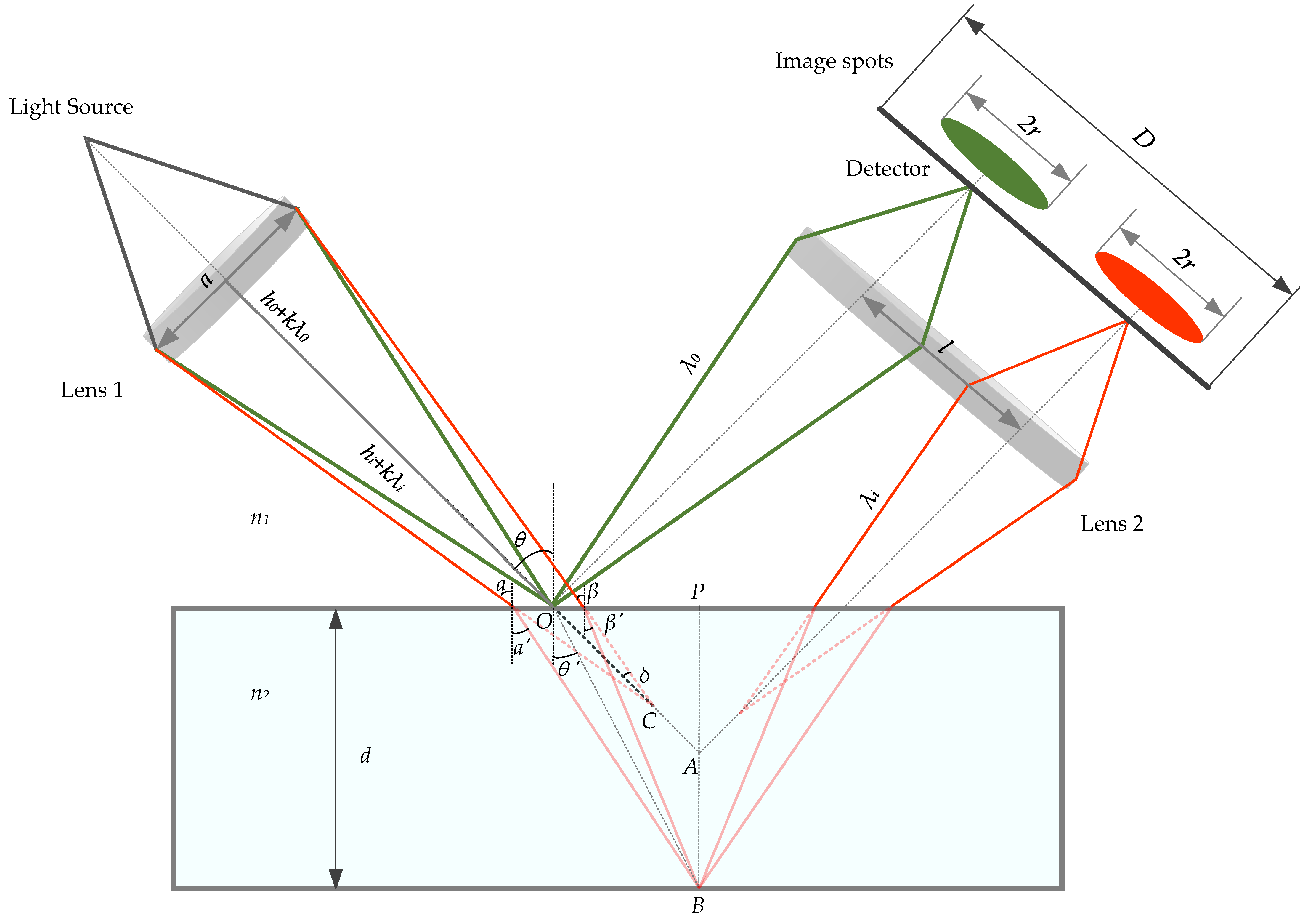
2.2. Theory of Chromatic Confocal Measurement with Inclined Illumination
2.2.1. Theoretical Analysis
2.2.2. Thickness Calculation Model in the Chromatic Confocal System with Inclined Illumination
2.2.3. Analysis for the Influence of θ on Measurement Range of Thickness
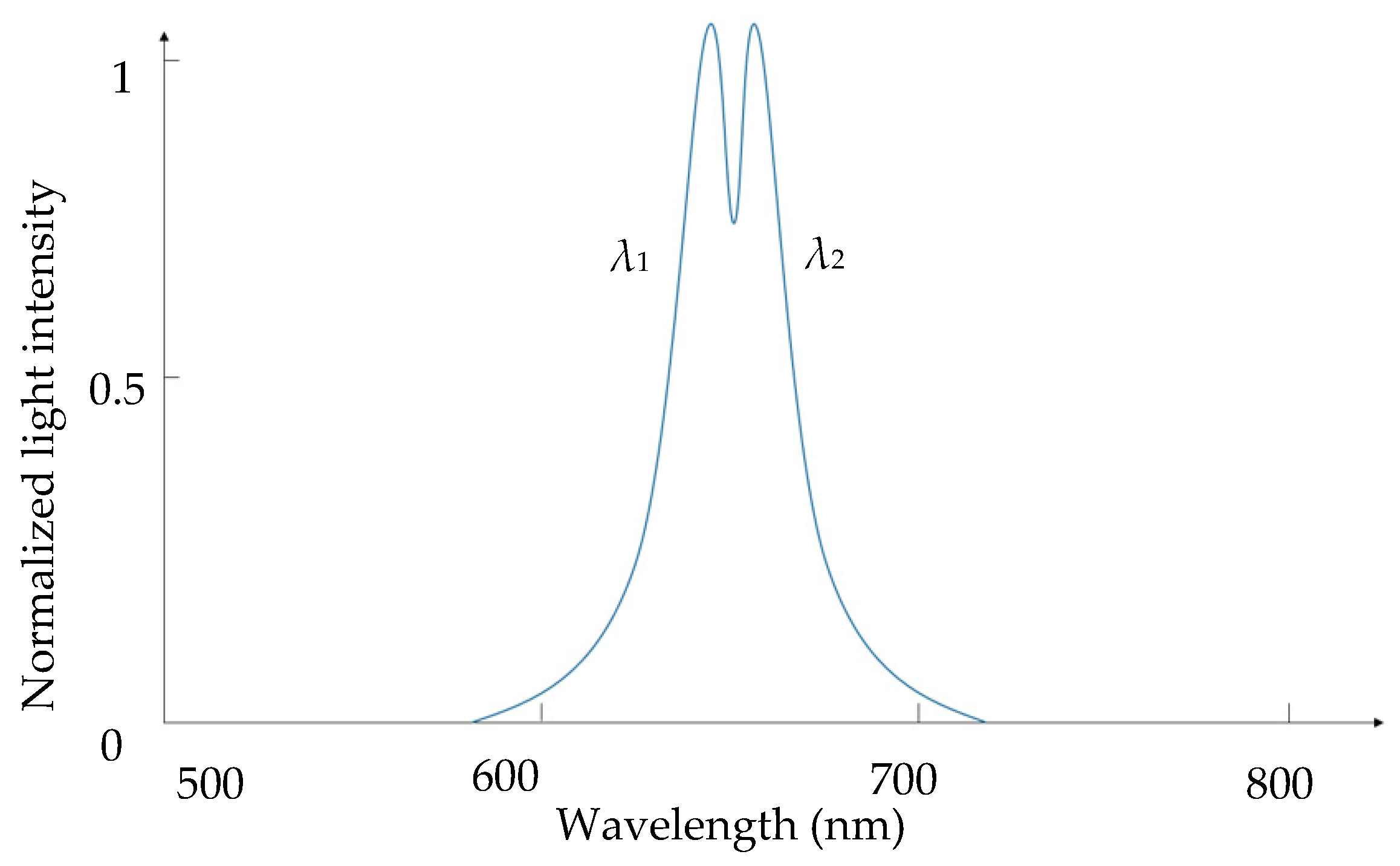
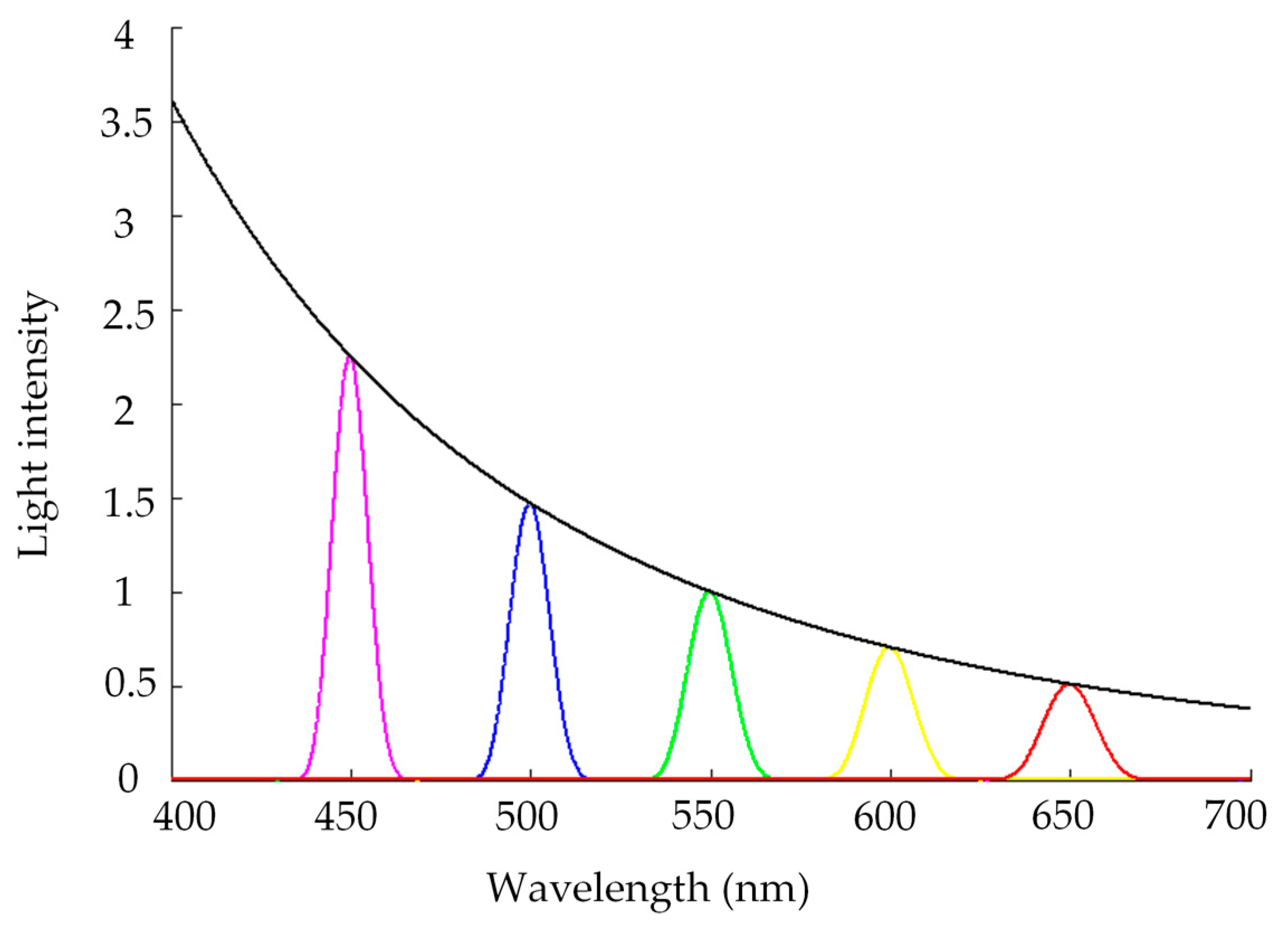
2.2.4. Color Conversion Algorithm
3. Experimental Analysis
3.1. Setup and Calibration Experiment of the System
3.1.1. The Selection of Angle θ
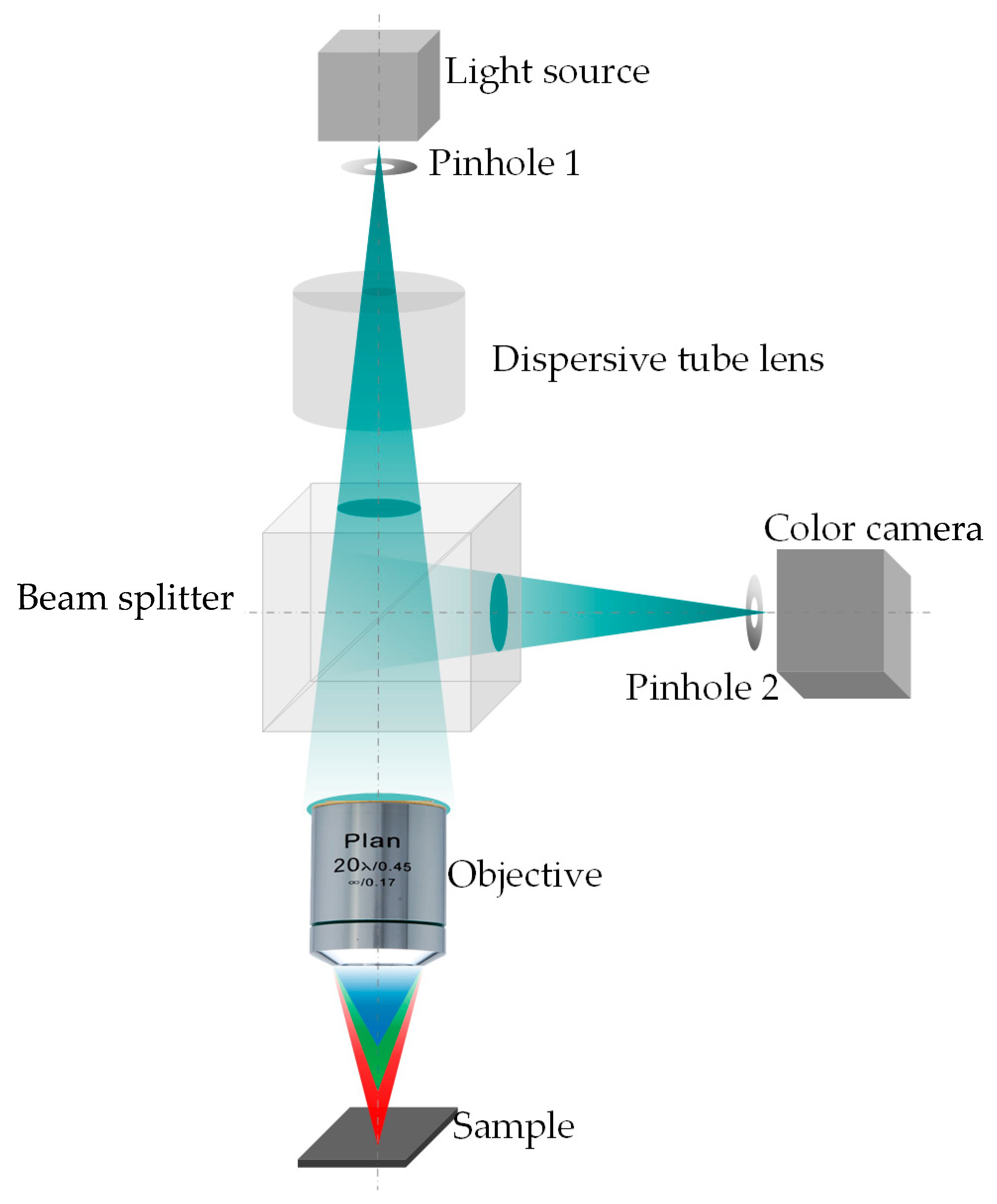
3.1.2. Setup of the System
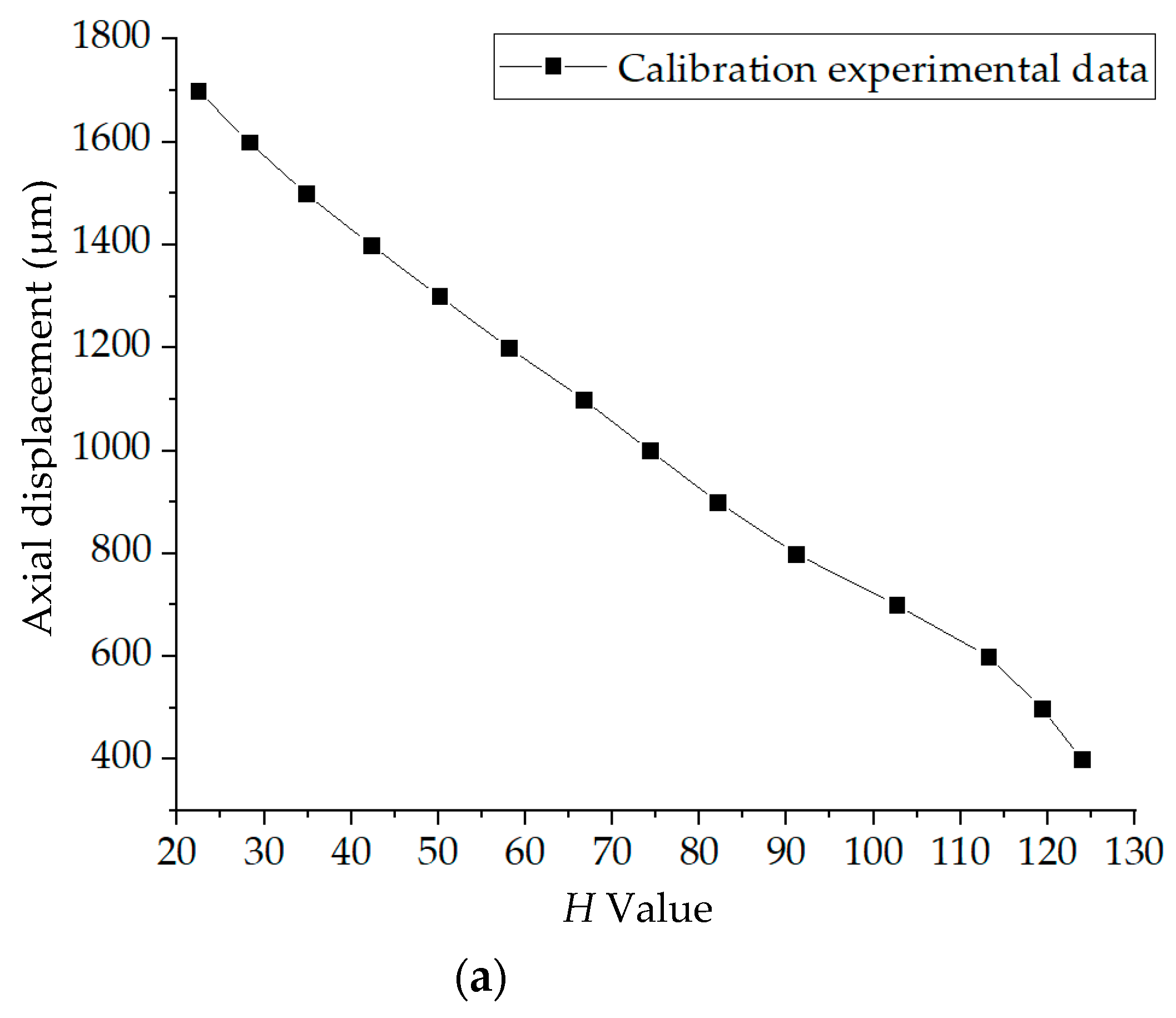
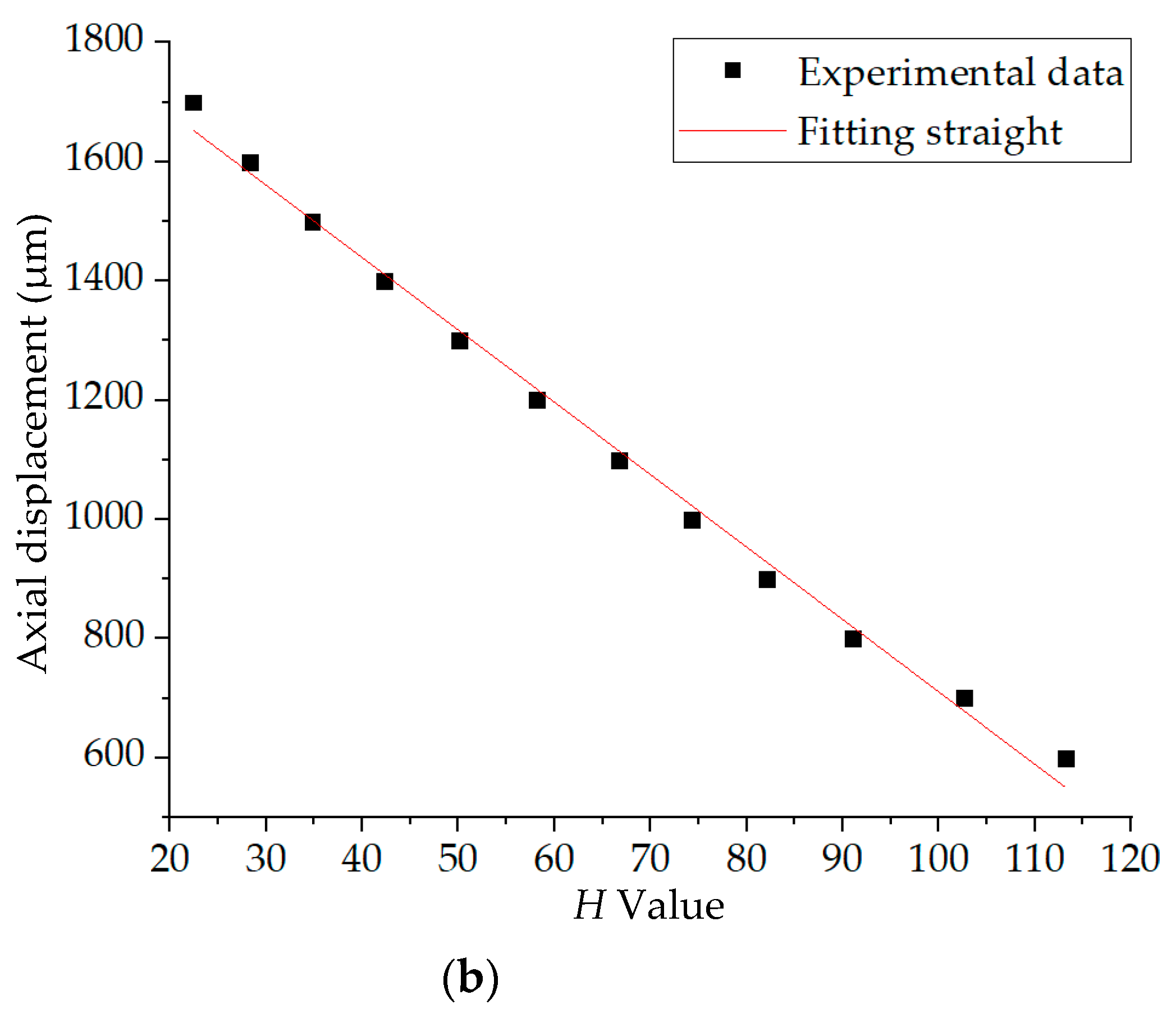
3.1.3. Calibration Experiment of the System
3.2. Thickness Measurement for Transparent Specimen
- (a)
- Slide 1: thicker transparent specimen
- (b)
- Slide 2: thinner transparent specimen
4. Discussions
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wang, Y.; Xi, M.; Liu, H.; Ding, Z.; Du, W.; Meng, X.; Sui, Y.; Li, J.; Jia, Z. On-machine noncontact scanning of high-gradient freeform surface using chromatic confocal probe on diamond turning machine. Opt. Laser Technol. 2021, 134, 106569. [Google Scholar] [CrossRef]
- Hillenbrand, M.; Lorenz, L.; Kleindienst, R.; Grewe, A.; Sinzinger, S. Spectrally multiplexed chromatic confocal multipoint sensing. Opt. Lett. 2013, 38, 4694–4697. [Google Scholar] [CrossRef]
- Chun, B.; Kim, K.; Gweon, D. Three-dimensional surface profile measurement using a beam scanning chromatic confocal microscope. Rev. Sci. Instrum. 2009, 80, 073706. [Google Scholar] [CrossRef]
- Du, H.; Zhang, W.; Ju, B.; Sun, Z.; Sun, A. A new method for detecting surface defects on curved reflective optics using normalized reflectivity. Rev. Sci. Instrum. 2020, 91, 036103. [Google Scholar] [CrossRef]
- Zou, J.; Yu, Q.; Cheng, F. Differential chromatic confocal roughness evaluation system and experimental research. Chin. Opt. 2020, 13, 1103–1114. [Google Scholar]
- Fu, S.; Kor, W.S.; Cheng, F.; Seah, L.K. In-situ measurement of surface roughness using chromatic confocal sensor. Procedia CIRP 2020, 94, 780–784. [Google Scholar] [CrossRef]
- Berkovic, G.; Zilberman, S.; Shafir, E.; Rubin, D. Chromatic confocal displacement sensing at oblique incidence angles. Appl. Opt. 2020, 59, 3183–3186. [Google Scholar] [CrossRef] [PubMed]
- Bai, J.; Li, X.; Wang, X.; Zhou, Q.; Ni, K. Chromatic Confocal Displacement Sensor with Optimized Dispersion Probe and Modified Centroid Peak Extraction Algorithm. Sensors 2019, 19, 3592. [Google Scholar] [CrossRef] [PubMed]
- Chen, C.; Wang, J.; Liu, X.; Lu, W.; Zhu, H.; Jiang, X. Influence of sample surface height for evaluation of peak extraction algorithms in confocal microscopy. Appl. Opt. 2018, 57, 6516–6526. [Google Scholar] [CrossRef] [PubMed]
- Sun, H.; Wang, S.; Bai, J.; Zhang, J.; Huang, J.; Zhou, X.; Liu, D.; Liu, C. Confocal laser scanning and 3D reconstruction methods for the subsurface damage of polished optics. Opt. Lasers Eng. 2021, 136, 106315. [Google Scholar] [CrossRef]
- Hillenbrand, M.; Weiss, R.; Endrody, C.; Grewe, A.; Hoffmann, M.; Sinzinger, S. Chromatic confocal matrix sensor with actuated pinhole arrays. Appl. Opt. 2015, 54, 4927–4936. [Google Scholar] [CrossRef] [PubMed]
- Luo, D.; Taphanel, M.; Claus, D.; Boettcher, T.; Osten, W.; Längle, T.; Beyerer, J. Area scanning method for 3D surface profilometry based on an adaptive confocal microscope. Opt. Lasers Eng. 2020, 124, 105819. [Google Scholar] [CrossRef]
- Li, S.; Liang, R. DMD-based three-dimensional chromatic confocal microscopy. Appl. Opt. 2020, 59, 4349–4356. [Google Scholar] [CrossRef] [PubMed]
- Hillenbrand, M.; Mitschunas, B.; Brill, F.; Grewe, A.; Sinzinger, S. Spectral characteristics of chromatic confocal imaging systems. Appl. Opt. 2014, 53, 7634–7642. [Google Scholar] [CrossRef] [PubMed]
- Lu, W.; Chen, C.; Wang, J.; Richard, L.; Zhang, C. Characterization of the displacement response in chromatic confocal microscopy with a hybrid radial basis function network. Opt. Express 2019, 27, 22737–22752. [Google Scholar] [CrossRef] [PubMed]
- Bai, J.; Li, X.; Wang, X.; Wang, J.; Ni, K.; Zhou, Q. Self-reference dispersion correction for chromatic confocal displacement measurement. Opt. Lasers Eng. 2021, 140, 106540. [Google Scholar] [CrossRef]
- Miks, A.; Novak, J.; Novak, P. Analysis of method for measuring thickness of plane-parallel plates and lenses using chromatic confocal sensor. Appl. Opt. 2010, 49, 3259–3264. [Google Scholar] [CrossRef]
- Boettcher, T.; Gronle, M.; Osten, W. Single-shot multilayer measurement by chromatic confocal coherence tomography. Opt. Metrol. 2017, 10329, 103290K. [Google Scholar]
- Yu, Q.; Zhang, K.; Cui, C.; Zhou, R.; Cheng, F.; Ye, R.; Zhang, Y. Method of thickness measurement for transparent specimens with chromatic confocal microscopy. Appl. Opt. 2018, 57, 9722–9728. [Google Scholar] [CrossRef]
- Li, J.; Zhao, Y.; Du, H.; Zhu, X.; Wang, K.; Zhao, M. Adaptive modal decomposition based overlapping-peaks extraction for thickness measurement in chromatic confocal microscopy. Opt. Express 2020, 28, 36176–36187. [Google Scholar] [CrossRef]
- Taphanel, M.; Beyerer, J. Fast 3D In-line Sensor for Specular and Diffuse Surfaces Combining the Chromatic Confocal and Triangulation Principle. In Proceedings of the Conference Record IEEE Instrumentation & Measurement Technology Conference, Graz, Austria, 13–16 May 2012. [Google Scholar]
- Taphanel, M.; Zink, R.; Längle, T.; Beyerer, J. Multiplex acquisition approach for high speed 3D measurements with a chromatic confocal microscope. SPIE Opt. Metrol. 2015, 9525, 95250Y. [Google Scholar]
- Seung, W.L.; Garam, C.; Sin, Y.L.; Yeongchan, C.; Heui, J.P. Coaxial spectroscopic imaging ellipsometry for volumetric thickness measurement. Appl. Opt. 2021, 60, 67–74. [Google Scholar]








| Objective Parameters | Value |
|---|---|
| magnification | 10× |
| N.A. value | 0.2 |
| Axial Displacement (μm) | H Value |
|---|---|
| 1700.00 | 22.41 |
| 1600.00 | 28.26 |
| 1500.00 | 34.78 |
| 1400.00 | 42.24 |
| 1300.00 | 50.11 |
| 1200.00 | 58.11 |
| 1100.00 | 66.66 |
| 1000.00 | 74.32 |
| 900.00 | 82.11 |
| 800.00 | 91.04 |
| 700.00 | 102.65 |
| 600.00 | 113.16 |
| 500.00 | 119.32 |
| 400.00 | 123.91 |
| Parameter | Value |
|---|---|
| α | 58.10° |
| β | 19.90° |
| α′ | 34.50° |
| β′ | 13.10° |
| θ | 39.00° |
| Piece Number | H Value of Upper Surface | H Value of Lower Surface | Value of Difference | Piece Number | H Value of Upper Surface | H Value of Lower Surface | Value of Difference |
|---|---|---|---|---|---|---|---|
| 1 | 24.45 | 63.02 | 38.57 | 21 | 24.45 | 63.11 | 38.66 |
| 2 | 24.49 | 62.75 | 38.25 | 22 | 24.49 | 63.37 | 38.89 |
| 3 | 24.46 | 63.13 | 38.67 | 23 | 24.06 | 63.10 | 39.04 |
| 4 | 24.49 | 63.10 | 38.60 | 24 | 24.49 | 63.14 | 38.64 |
| 5 | 24.47 | 63.13 | 38.66 | 25 | 24.07 | 63.16 | 39.09 |
| 6 | 24.50 | 63.01 | 38.52 | 26 | 24.45 | 63.11 | 38.66 |
| 7 | 24.46 | 63.11 | 38.66 | 27 | 24.49 | 63.06 | 38.57 |
| 8 | 24.49 | 63.12 | 38.63 | 28 | 24.45 | 63.08 | 38.63 |
| 9 | 24.48 | 63.08 | 38.59 | 29 | 24.49 | 63.11 | 38.62 |
| 10 | 24.46 | 63.16 | 38.70 | 30 | 24.46 | 63.10 | 38.63 |
| 11 | 24.49 | 62.94 | 38.45 | 31 | 24.49 | 63.05 | 38.56 |
| 12 | 24.49 | 63.17 | 38.69 | 32 | 24.49 | 63.40 | 38.91 |
| 13 | 24.46 | 63.01 | 38.55 | 33 | 24.45 | 63.12 | 38.67 |
| 14 | 24.48 | 63.13 | 38.65 | 34 | 24.48 | 63.13 | 38.65 |
| 15 | 24.49 | 63.01 | 38.51 | 35 | 24.49 | 63.37 | 38.87 |
| 16 | 24.45 | 63.18 | 38.73 | 36 | 24.50 | 63.07 | 38.57 |
| 17 | 24.49 | 62.96 | 38.47 | 37 | 24.45 | 63.09 | 38.64 |
| 18 | 24.07 | 63.15 | 39.09 | 38 | 24.49 | 63.12 | 38.63 |
| 19 | 24.49 | 62.96 | 38.47 | 39 | 24.48 | 63.09 | 38.61 |
| 20 | 24.07 | 63.20 | 39.12 | 40 | 24.46 | 63.14 | 38.68 |
| The average value of the difference | 38.67 | Relative error (σ) | 0.18 | ||||
| Piece Number | H Value of Upper Surface | H Value of Lower Surface | Value of Difference | Piece Number | H Value of Upper Surface | H Value of Lower Surface | Value of Difference |
|---|---|---|---|---|---|---|---|
| 1 | 52.07 | 59.49 | 7.42 | 21 | 52.03 | 59.48 | 7.45 |
| 2 | 52.02 | 58.91 | 6.89 | 22 | 52.08 | 59.44 | 7.36 |
| 3 | 52.09 | 59.51 | 7.42 | 23 | 52.03 | 59.36 | 7.32 |
| 4 | 52.05 | 58.90 | 6.85 | 24 | 52.09 | 59.45 | 7.35 |
| 5 | 52.05 | 59.40 | 7.35 | 25 | 52.12 | 59.40 | 7.29 |
| 6 | 52.06 | 58.57 | 6.51 | 26 | 52.09 | 59.40 | 7.32 |
| 7 | 52.08 | 59.51 | 7.43 | 27 | 52.04 | 59.48 | 7.44 |
| 8 | 52.04 | 58.88 | 6.84 | 28 | 52.06 | 59.50 | 7.43 |
| 9 | 52.06 | 59.44 | 7.38 | 29 | 52.05 | 58.98 | 6.93 |
| 10 | 52.06 | 59.40 | 7.34 | 30 | 52.07 | 59.52 | 7.45 |
| 11 | 52.07 | 59.47 | 7.41 | 31 | 52.06 | 59.44 | 7.37 |
| 12 | 52.08 | 58.95 | 6.87 | 32 | 52.07 | 59.48 | 7.41 |
| 13 | 52.05 | 59.50 | 7.44 | 33 | 52.10 | 59.45 | 7.34 |
| 14 | 52.01 | 58.94 | 6.93 | 34 | 52.07 | 59.45 | 7.38 |
| 15 | 52.07 | 59.41 | 7.34 | 35 | 52.05 | 58.91 | 6.86 |
| 16 | 52.07 | 58.90 | 6.83 | 36 | 52.12 | 59.49 | 7.37 |
| 17 | 52.09 | 59.46 | 7.37 | 37 | 52.08 | 59.43 | 7.35 |
| 18 | 52.10 | 59.47 | 7.37 | 38 | 52.06 | 59.46 | 7.40 |
| 19 | 52.07 | 59.45 | 7.37 | 39 | 52.04 | 58.95 | 6.91 |
| 20 | 52.04 | 58.96 | 6.91 | 40 | 52.09 | 59.50 | 7.41 |
| The average value of the difference | 7.24 | Relative error (σ) | 0.25 | ||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, Q.; Zhang, Y.; Shang, W.; Dong, S.; Wang, C.; Wang, Y.; Liu, T.; Cheng, F. Thickness Measurement for Glass Slides Based on Chromatic Confocal Microscopy with Inclined Illumination. Photonics 2021, 8, 170. https://doi.org/10.3390/photonics8050170
Yu Q, Zhang Y, Shang W, Dong S, Wang C, Wang Y, Liu T, Cheng F. Thickness Measurement for Glass Slides Based on Chromatic Confocal Microscopy with Inclined Illumination. Photonics. 2021; 8(5):170. https://doi.org/10.3390/photonics8050170
Chicago/Turabian StyleYu, Qing, Yali Zhang, Wenjian Shang, Shengchao Dong, Chong Wang, Yin Wang, Ting Liu, and Fang Cheng. 2021. "Thickness Measurement for Glass Slides Based on Chromatic Confocal Microscopy with Inclined Illumination" Photonics 8, no. 5: 170. https://doi.org/10.3390/photonics8050170
APA StyleYu, Q., Zhang, Y., Shang, W., Dong, S., Wang, C., Wang, Y., Liu, T., & Cheng, F. (2021). Thickness Measurement for Glass Slides Based on Chromatic Confocal Microscopy with Inclined Illumination. Photonics, 8(5), 170. https://doi.org/10.3390/photonics8050170







