Investigation on Speckle-Free Imaging at the Output of a Multimode Fiber under Various Mode Excitation Conditions
Abstract
1. Introduction
2. Theoretical Analysis
2.1. Various Modal Power Distribution by Varying the Tilt Angle of the Gaussian
2.2. Calculation of the Speckle Contrast at the Output of a Multimode Fiber
3. Simulation Results and Discussion
3.1. Various Modal Power Distribution by Varying the Tilt Angle of the Gaussian Beam
3.2. Speckle-Free Imaging under the Various Modal Power Distributions
LP02: U = 4.7652, W = 438.3741, τ02(s) = 156.685 × 10−12(s·m−1) × L(m);
LP12: U = 6.2146, W = 438.3559, τ12(s) = 156.698 × 10−12(s·m−1) × L(m);
LP21: U = 4.4569, W = 438.3773, τ21(s) = 156.683 × 10−12(s·m−1) × L(m);
LP01: U = 1.8893, W = 438.3959, τ01(s) = 156.670 × 10−12(s·m−1) × L(m).
T3 = |τ11 − τ21| = 0.8 × 10−14(s·m−1) × L(m); T4 = |τ11 − τ01| = 0.5 × 10−14(s·m−1) × L(m);
T5 = |τ02 − τ12| = 1.3 × 10−14(s·m−1) × L(m); T6 = |τ02 − τ21| = 0.2 × 10−14(s·m−1) × L(m);
T7 = |τ02 − τ01| = 1.5 × 10−14(s·m−1) × L(m); T8 = |τ12 − τ21| = 1.5 × 10−14(s·m−1) × L(m);
T9 = |τ12 − τ01| = 2.8 × 10−14(s·m−1) × L(m); T10 = |τ21 − τ01| = 1.3 × 10−14(s·m−1) × L(m).
Tmin(s) = T6 = 0.2 × 10−14(s·m−1) × L(m).
- Tmax < tc: Interference occurs among all modes, and hence, the speckle contrast at the output of a multimode fiber is not significantly reduced. The optical fiber length L must be within 16.7 m.
- Tmax > tc > Tmin: Interference occurs among some modes, and hence, the speckle contrast at the output of a multimode fiber is significantly reduced. The optical fiber length L must be in the following range: 16.7 m < L < 234.1 m.
- Tmin > tc: Interference among all modes ceases, and hence, the speckle contrast at the output of a multimode fiber is equal to 0. The optical fiber length L must exceed 234.1 m.
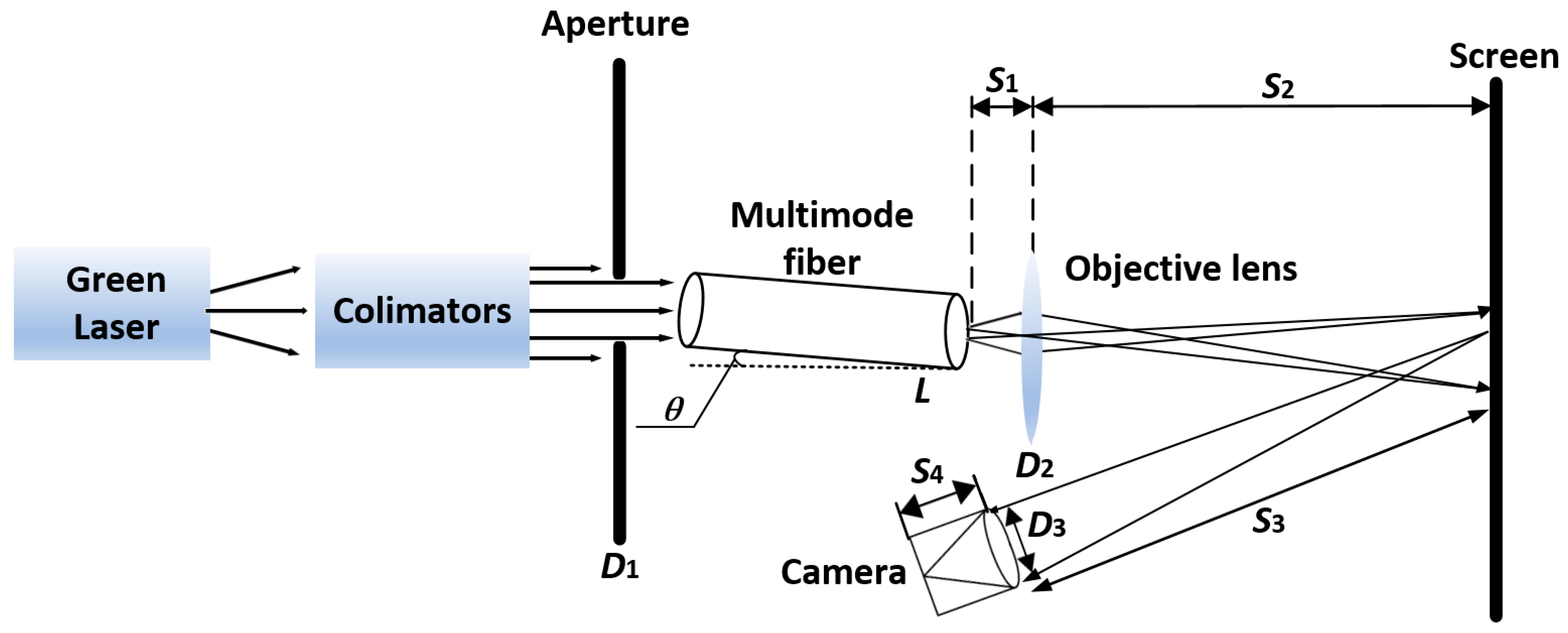
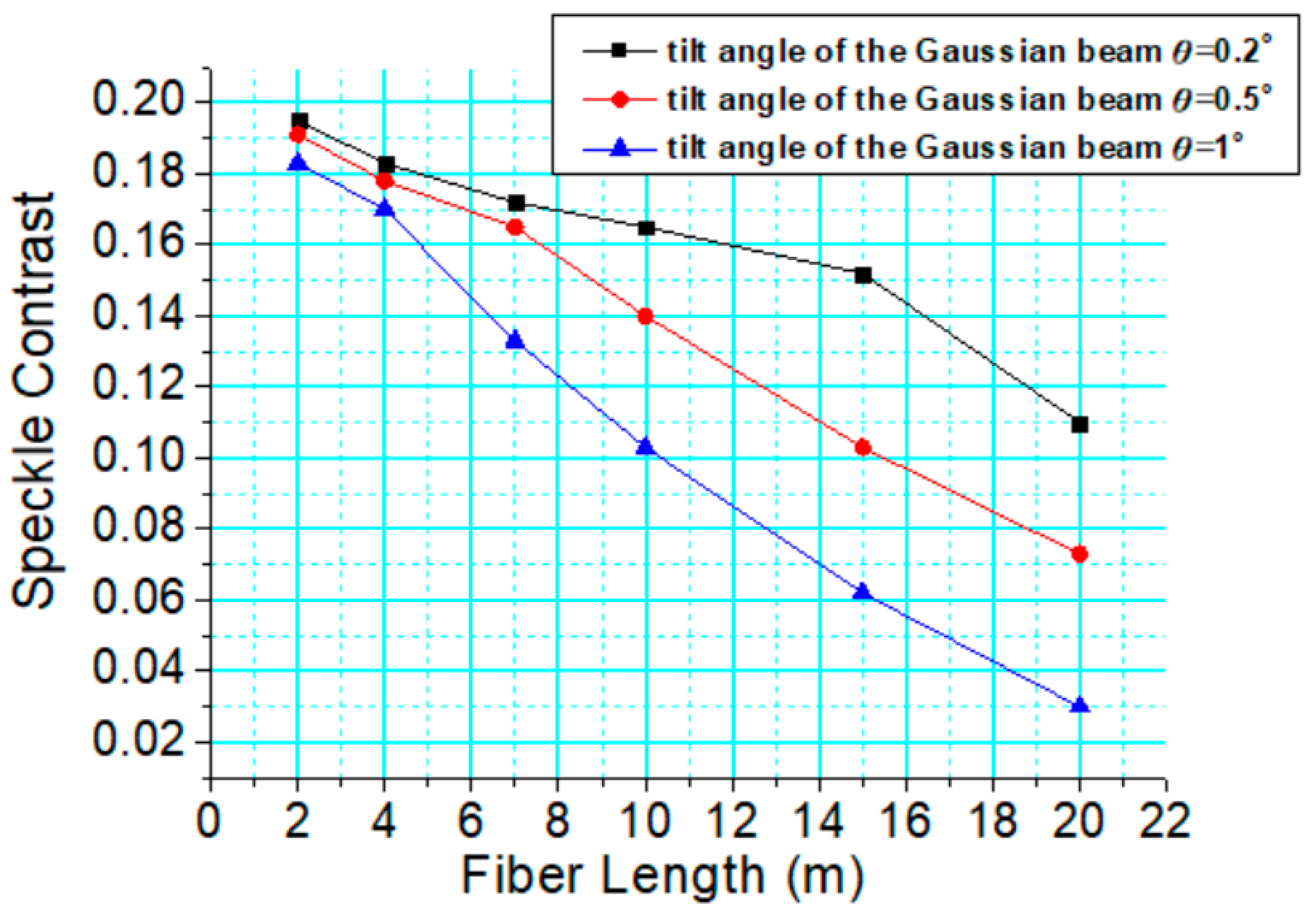
4. Experimental Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Murayama, M.; Nakayama, Y.; Yamazaki, K.; Hoshina, Y.; Watanabe, H.; Fuutagawa, N.; Kawanishi, H.; Yemura, T.; Narui, H. Watt-class green (530 nm) and blue (465 nm) laser diodes. Phys. Status Solidi A 2018, 215, 1700513. [Google Scholar] [CrossRef]
- Chellappan, K.V.; Erden, E.; Urey, H. Laser-based displays: A review. Appl. Opt. 2010, 49, F79–F98. [Google Scholar] [CrossRef]
- Humeau-Heurtier, A.; Mahe, G.; Durand, S.; Abraham, P. Multiscale entropy study of medical laser speckle contrast images. IEEE Trans. Biomed. Eng. 2012, 60, 872–879. [Google Scholar] [CrossRef]
- Feng, P.; Yang, L.; Wen, X. Coherent noise reduction in digital holographic microscopy by averaging multiple holograms recorded with a multimode laser. Opt. Express 2017, 25, 21815–21825. [Google Scholar]
- Chen, H.; Pan, J.; Yang, Z. Speckle reduction using deformable mirrors with diffusers in a laser pico-projector. Opt. Express 2017, 25, 18140–18151. [Google Scholar] [CrossRef]
- Ma, Y.; Chen, X.; Zhu, W.; Cheng, X.; Xiang, D.; Shi, F. Speckle noise reduction in optical coherence tomography images based on edge-sensitive cGAN. Biomed. Opt. Express 2018, 9, 5129–5146. [Google Scholar] [CrossRef]
- Ai, J.; Liu, R.; Tang, B.; Jia, L.; Zhao, J.; Zhou, F. A refined bilateral filtering algorithm based on adaptively-trimmed-statistics for speckle reduction in SAR imagery. Biomed. IEEE Access 2019, 7, 103443–103455. [Google Scholar] [CrossRef]
- Kubota, S.; Goodman, J.W. Very efficient speckle contrast reduction realized by moving diffuser device. Appl. Opt. 2010, 49, 4385–4391. [Google Scholar] [CrossRef] [PubMed]
- Shin, S.C.; Yoo, S.S.; Lee, S.Y.; Park, C.; Park, S.; Kwon, J.W.; Lee, S. Removal of hot spot speckle on laser projection screen using both the running screen and the rotating diffuser. J. Disp. Technol. 2006, 27, 91–96. [Google Scholar] [CrossRef]
- Sun, M.; Lu, Z. Speckle suppression with a rotating light pipe. Opt. Eng. 2010, 49, 024202. [Google Scholar] [CrossRef]
- Lapchuk, A.; Kryuchyn, A.; Petrov, V.; Yurlov, V.; Klymenko, V. Full speckle suppression in laser projectors using two Barker code-type optical diffractive elements. J. Opt. Soc. Am. A 2013, 30, 22–31. [Google Scholar] [CrossRef] [PubMed]
- Lapchuk, A.; Gorbov, I.; Le, Z.; Xiong, Q.; Lu, Z.; Prygun, O.; Pankratova, A. Experimental demonstration of a flexible DOE loop with wideband speckle suppression for laser pico-projectors. Opt. Express 2018, 26, 26188–26195. [Google Scholar] [CrossRef]
- Ma, Q.; Xu, C.Q.; Kitai, A.; Stadler, D. Speckle reduction by optimized multimode fiber combined with dielectric elastomer actuator and light pipe homogenizer. J. Disp. Technol. 2016, 12, 1162–1167. [Google Scholar] [CrossRef]
- Prygun, A.V.; Morozov, Y.M.; Kliuieva, T.Y.; Borodin, Y.A.; Le, Z. Completely passive method of speckle reduction utilizing static multimode fibre and two-dimensional diffractive optical element. J. Mod. Opt. 2019, 66, 1688–1694. [Google Scholar] [CrossRef]
- Le, Z.; Lapchuk, A.; Guo, Y.; Dai, Y.; Gorbov, I. Investigation of speckle suppression beyond human eye sensitivity by using a passive multimode fiber and a multimode fiber bundle. Opt. Express 2020, 28, 6820–6834. [Google Scholar]
- Imai, M.; Ohtsuka, Y. Speckle-pattern contrast of semiconductor laser propagating in a multimode optical fiber. Opt. Commun. 1980, 33, 4–8. [Google Scholar] [CrossRef]
- Efimov, A. Spatial coherence at the output of multimode optical fibers. Opt. Express 2014, 22, 15577–15588. [Google Scholar] [CrossRef]
- Efimov, A. Coherence and speckle contrast at the output of a stationary multimode optical fiber. Opt. Lett. 2018, 43, 4767–4770. [Google Scholar] [CrossRef]
- Halpaap, D.; Tiana-Alsina, J.; Vilaseca, M.; Masoller, C. Experimental characterization of the speckle pattern at the output of a multimode optical fiber. Opt. Express 2019, 27, 27737–27744. [Google Scholar] [CrossRef]
- Dandliker, R.; Bertholds, A.; Maystre, F. How modal noise in multimode fibers depends on source spectrum and fiber dispersion. J. Lightwave Technol. 1985, 3, 7–12. [Google Scholar] [CrossRef]
- Hlubina, P. Spectral and dispersion analysis of laser sources and multimode fibers via the statistics of the intensity pattern. J. Mod. Opt. 1994, 41, 1001–1014. [Google Scholar] [CrossRef]
- Manni, J.G.; Goodman, J.W. Versatile method for achieving 1% speckle contrast in large-venue laser projection displays using a stationary multimode optical fiber. Opt. Express 2012, 20, 11288–11315. [Google Scholar] [CrossRef] [PubMed]
- Crosignani, B.; DiPorto, P. Coherence of an electromagnetic field propagating in a weakly guiding fiber. J. Appl. Phys. 1973, 44, 4616–4617. [Google Scholar] [CrossRef]
- Gloge, D. Weakly guiding fibers. Appl. Opt. 1971, 10, 2252–2258. [Google Scholar] [CrossRef] [PubMed]
- Snyder, A.W.; Young, W.R. Modes of optical waveguides. J. Opt. Soc. Am. 1978, 68, 297–309. [Google Scholar] [CrossRef]
- Snyder, A.W.; Love, J.D. Optical Waveguide Theory; Chapman & Hall: New York, NY, USA, 1983. [Google Scholar]
- Born, M.; Wolf, E. Principles of Optics, 7th ed.; Cambridge University Press: Cambridge, UK, 1999. [Google Scholar]
- Goodman, J.W. Speckle Phenomena in Optics: Theory and Applications; Viva Books: New Delhi, India, 2008. [Google Scholar]
- Li, J.; Wang, J.; Ding, Y.; Xu, D.; Lin, H.; Jing, F. Effect of fiber coiling mode on modes excitation. Acta Opt. Sin. 2011, 31, s100204. [Google Scholar]
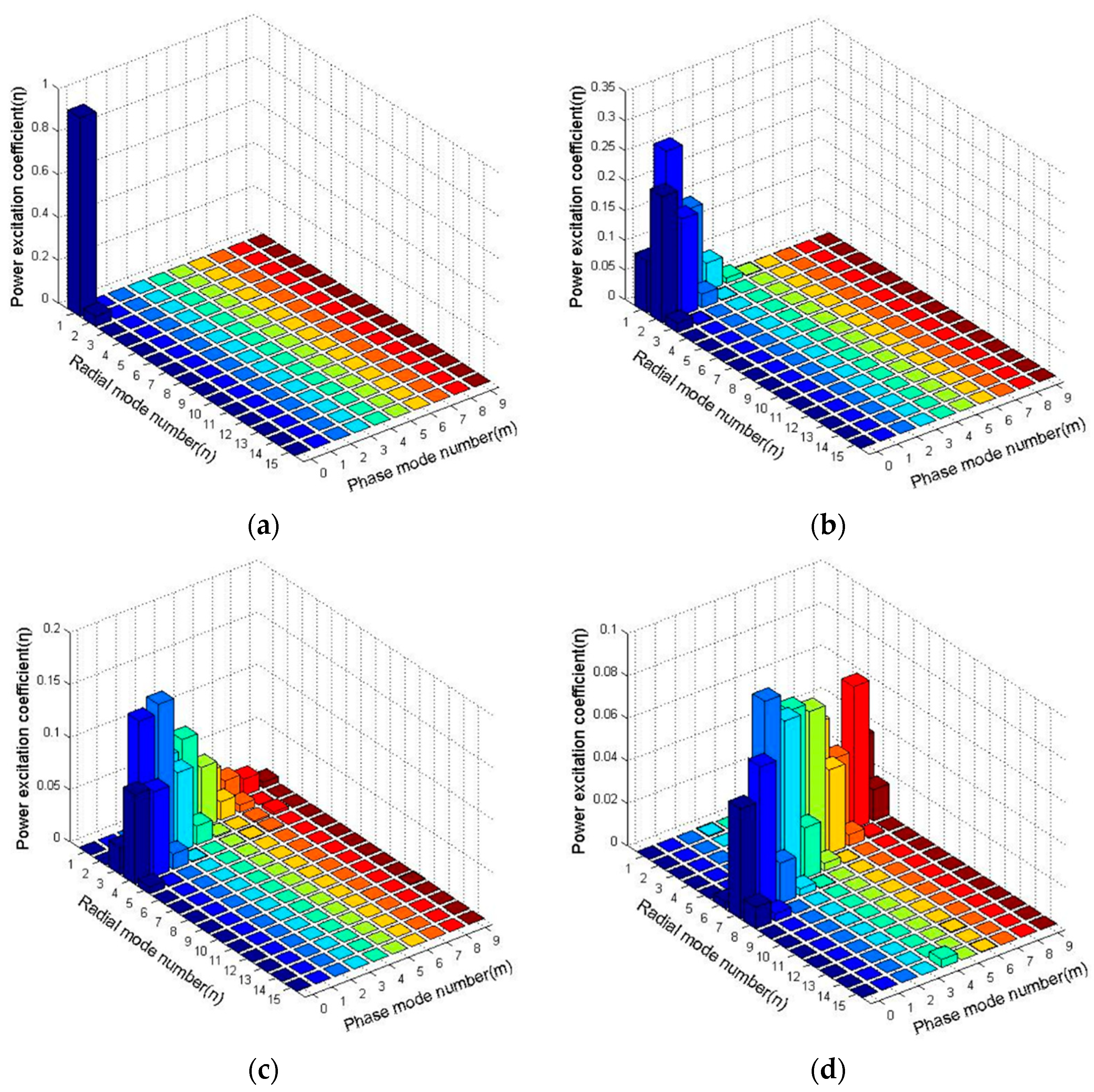


| Tilt Angle of the Gaussian Beam | Excitation Modes | Power Excitation Coefficient |
|---|---|---|
| θ = 0° | LP01 | η01 = 0.926 |
| θ = 0.2° | LP11 | η11 = 0.259 |
| LP02 | η02 = 0.211 | |
| LP12 | η12 = 0.161 | |
| LP21 | η21 = 0.148 | |
| LP01 | η01 = 0.087 | |
| θ = 0.5° | LP23 | η23 = 0.148 |
| LP13 | η13 = 0.139 | |
| LP42 | η42 = 0.091 | |
| LP04 | η04 = 0.085 | |
| LP32 | η32 = 0.081 | |
| LP14 | η14 = 0.081 | |
| LP33 | η33 = 0.076 | |
| θ = 1° | LP26 | η26 = 0.090 |
| LP36 | η36 = 0.078 | |
| LP45 | η45 = 0.074 | |
| LP55 | η55 = 0.070 | |
| LP17 | η17 = 0.068 | |
| LP84 | η84 = 0.067 | |
| LP64 | η64 = 0.056 | |
| LP07 | η07 = 0.052 |
| Tilt Angle of the Gaussian Beam | Group Delay Time (s) of the Excitation Modes | Maximum Delay Difference Tmax (s) and Minimum Delay Difference Tmin (s) | The Degree of Speckle Reduction Corresponds to the Required Length of Multimode Fiber |
|---|---|---|---|
| θ = 0.5° | τ23 = 156.760 × 10−12L τ13 = 156.736 × 10−12L τ42 = 156.751 × 10−12L τ04 = 156.762 × 10−12L τ32 = 156.731 × 10−12L τ14 = 156.790 × 10−12L τ33 = 156.785 × 10−12L | Tmax = 5.9 × 10−14L Tmin = 0.2 × 10−14L | No significant reduction in speckle contrast: L < 7.9 m Significant reduction in speckle contrast: 7.9 m < L < 234.1 m Speckle-free imaging: L > 234.1 m |
| θ = 1° | τ26 = 156.993 × 10−12L τ36 = 157.042 × 10−12L τ45 = 156.984 × 10−12L τ55 = 157.030 × 10−12L τ17 = 157.048 × 10−12L τ84 = 157.057 × 10−12L τ64 = 156.969 × 10−12L τ07 = 156.995 × 10−12L | Tmax = 8.8 × 10−14L Tmin = 0.2 × 10−14L | No significant reduction in speckle contrast: L < 5.3 m Significant reduction in speckle contrast: 5.3 m < L < 234.1 m Speckle-free imaging: L > 234.1 m |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, J.; Le, Z.; Guo, Y.; Liu, Z.; Xu, Q.; Dai, Y.; Deng, J.; Li, J.; Cai, D. Investigation on Speckle-Free Imaging at the Output of a Multimode Fiber under Various Mode Excitation Conditions. Photonics 2021, 8, 171. https://doi.org/10.3390/photonics8050171
Zhou J, Le Z, Guo Y, Liu Z, Xu Q, Dai Y, Deng J, Li J, Cai D. Investigation on Speckle-Free Imaging at the Output of a Multimode Fiber under Various Mode Excitation Conditions. Photonics. 2021; 8(5):171. https://doi.org/10.3390/photonics8050171
Chicago/Turabian StyleZhou, Jun, Zichun Le, Yanyu Guo, Zongshen Liu, Qiyong Xu, Yanxin Dai, Jiayu Deng, Jiapo Li, and Di Cai. 2021. "Investigation on Speckle-Free Imaging at the Output of a Multimode Fiber under Various Mode Excitation Conditions" Photonics 8, no. 5: 171. https://doi.org/10.3390/photonics8050171
APA StyleZhou, J., Le, Z., Guo, Y., Liu, Z., Xu, Q., Dai, Y., Deng, J., Li, J., & Cai, D. (2021). Investigation on Speckle-Free Imaging at the Output of a Multimode Fiber under Various Mode Excitation Conditions. Photonics, 8(5), 171. https://doi.org/10.3390/photonics8050171





