Chalcogenide Glass Microfibers for Mid-Infrared Optics
Abstract
:1. Introduction
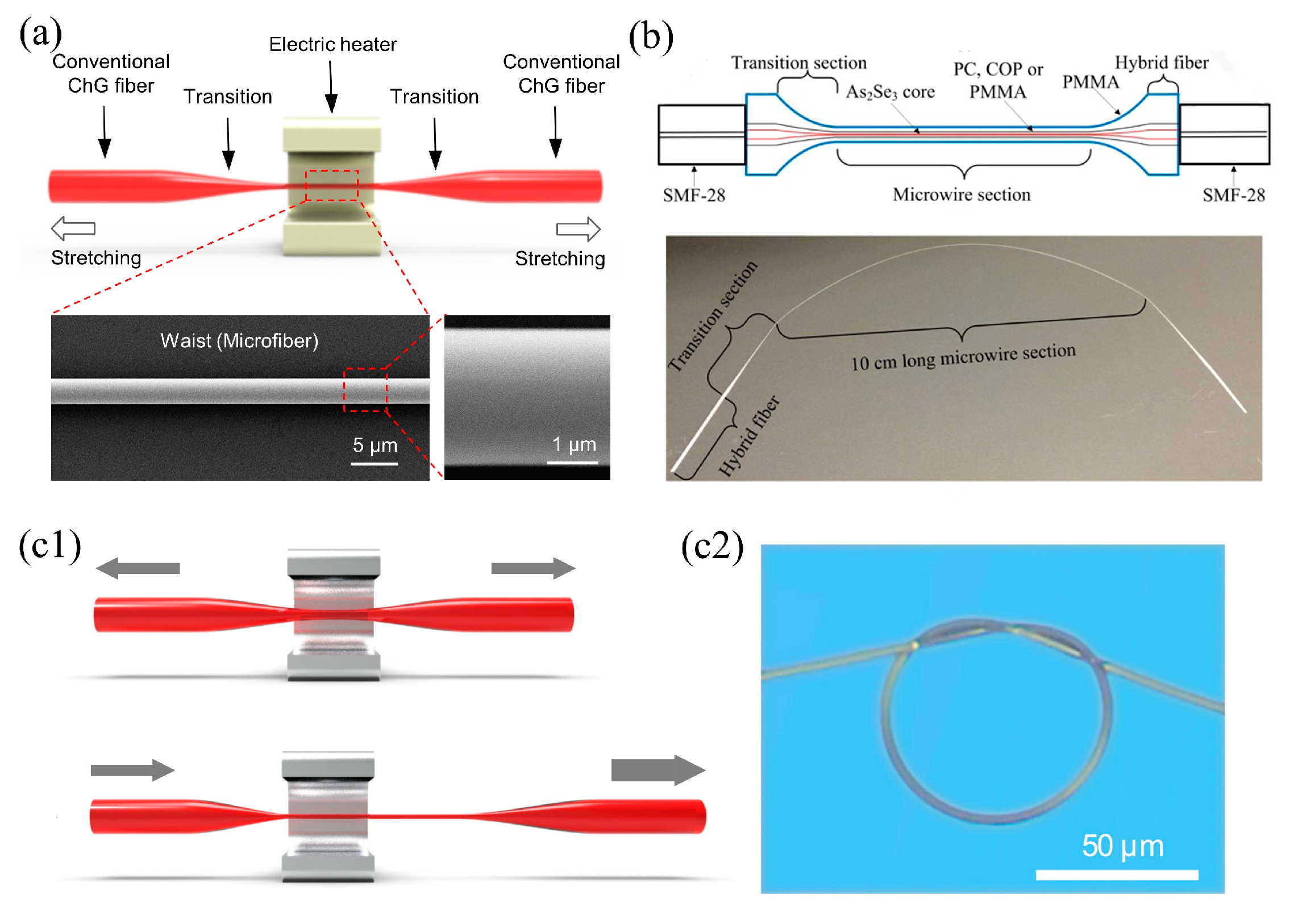
2. Fabrication
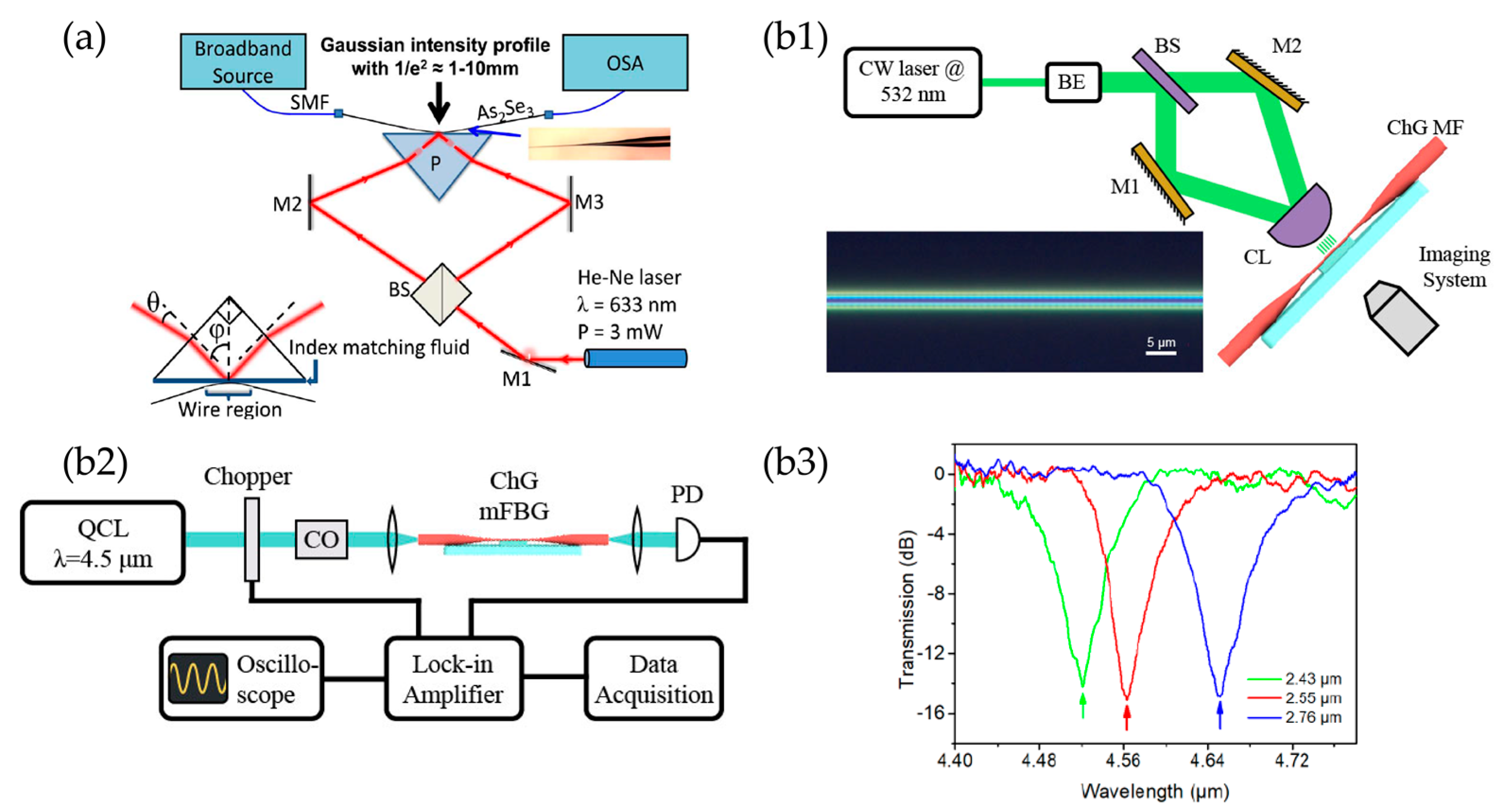
3. Optical Waveguiding Properties
4. Photonic Applications
4.1. Near-Field Optical Couplers
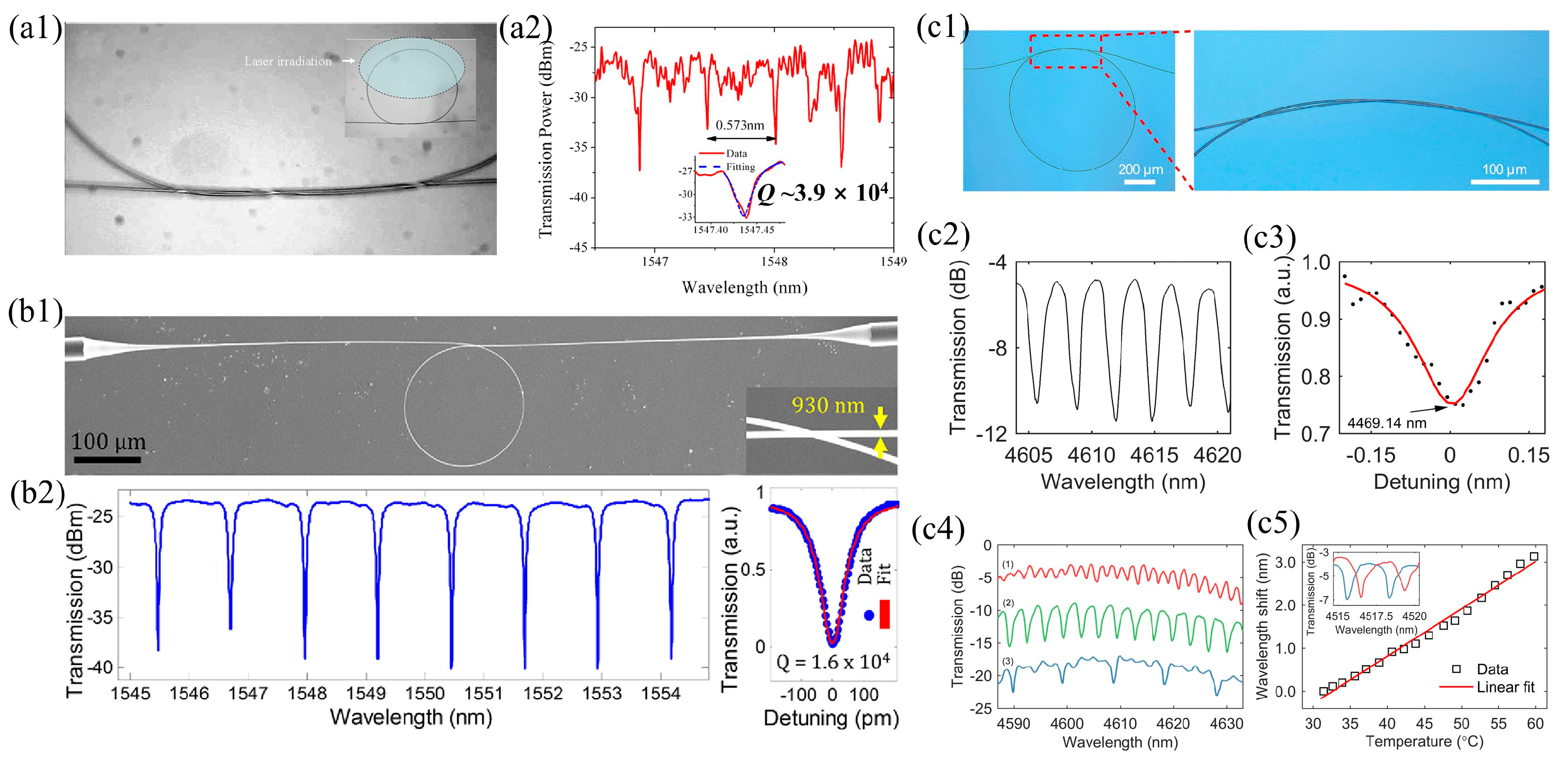
4.2. MF-Based Resonators
4.3. MF Gratings
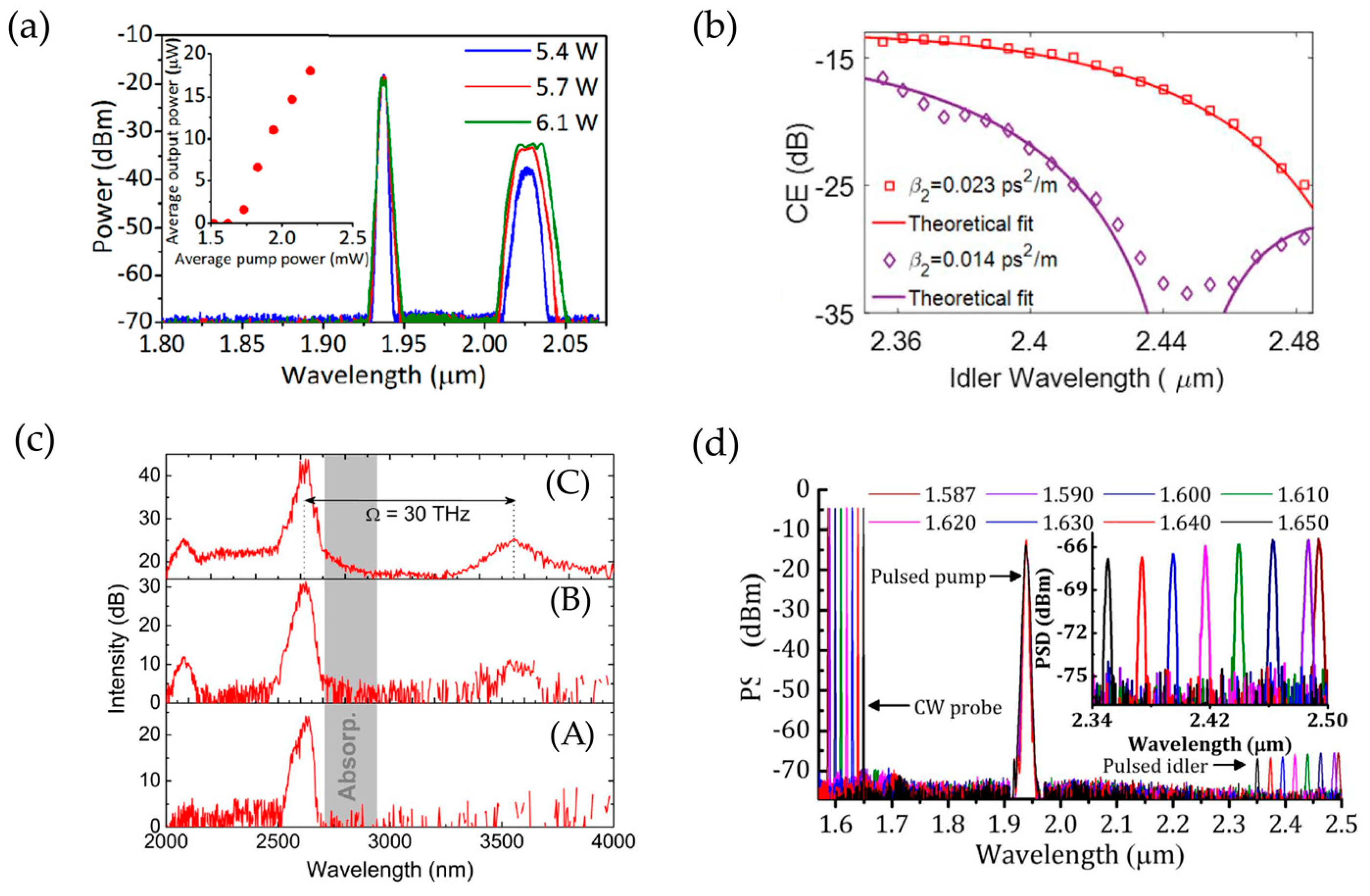
4.4. Raman Lasers
4.5. Frequency Conversion
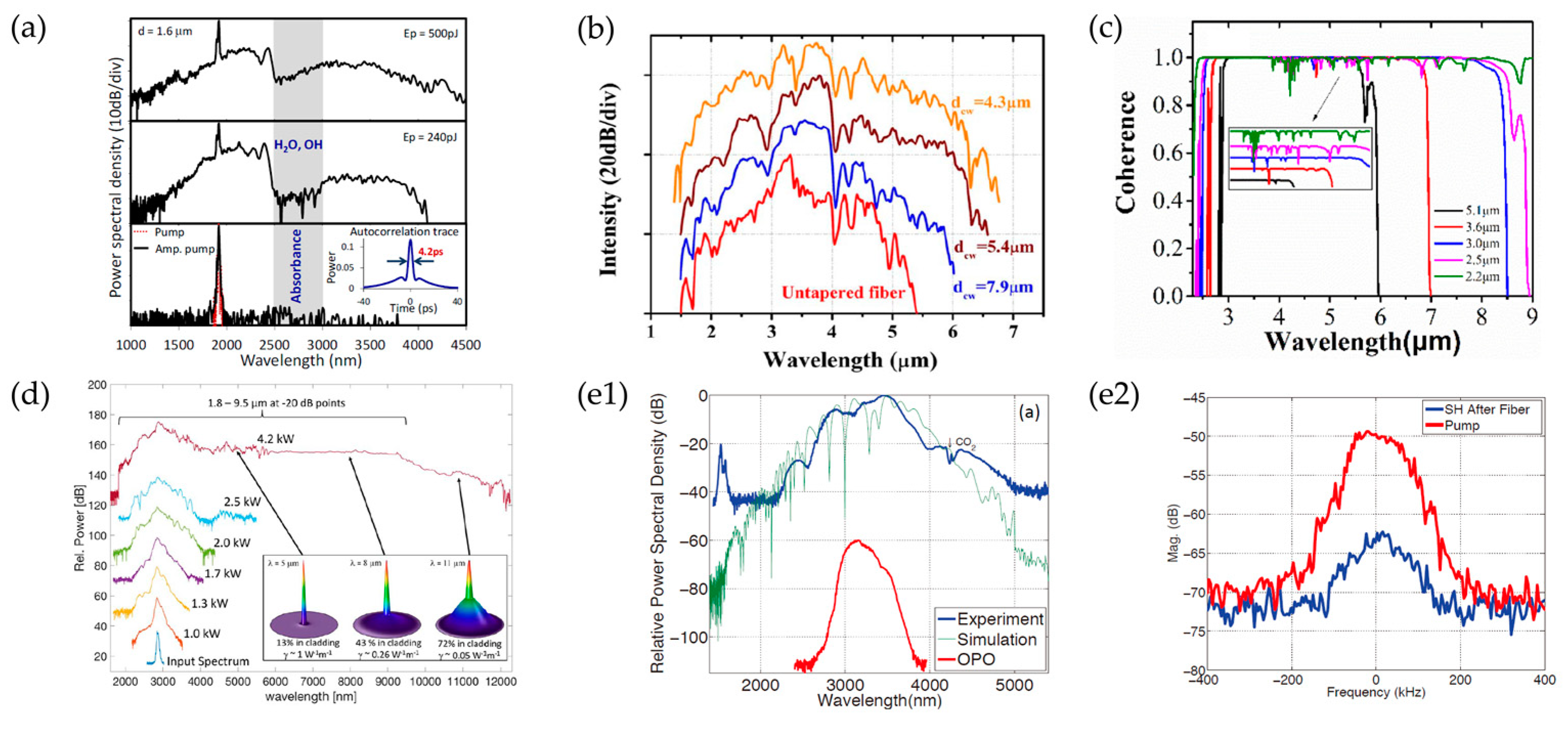
4.6. Supercontinuum Generation
4.7. Frequency Combs
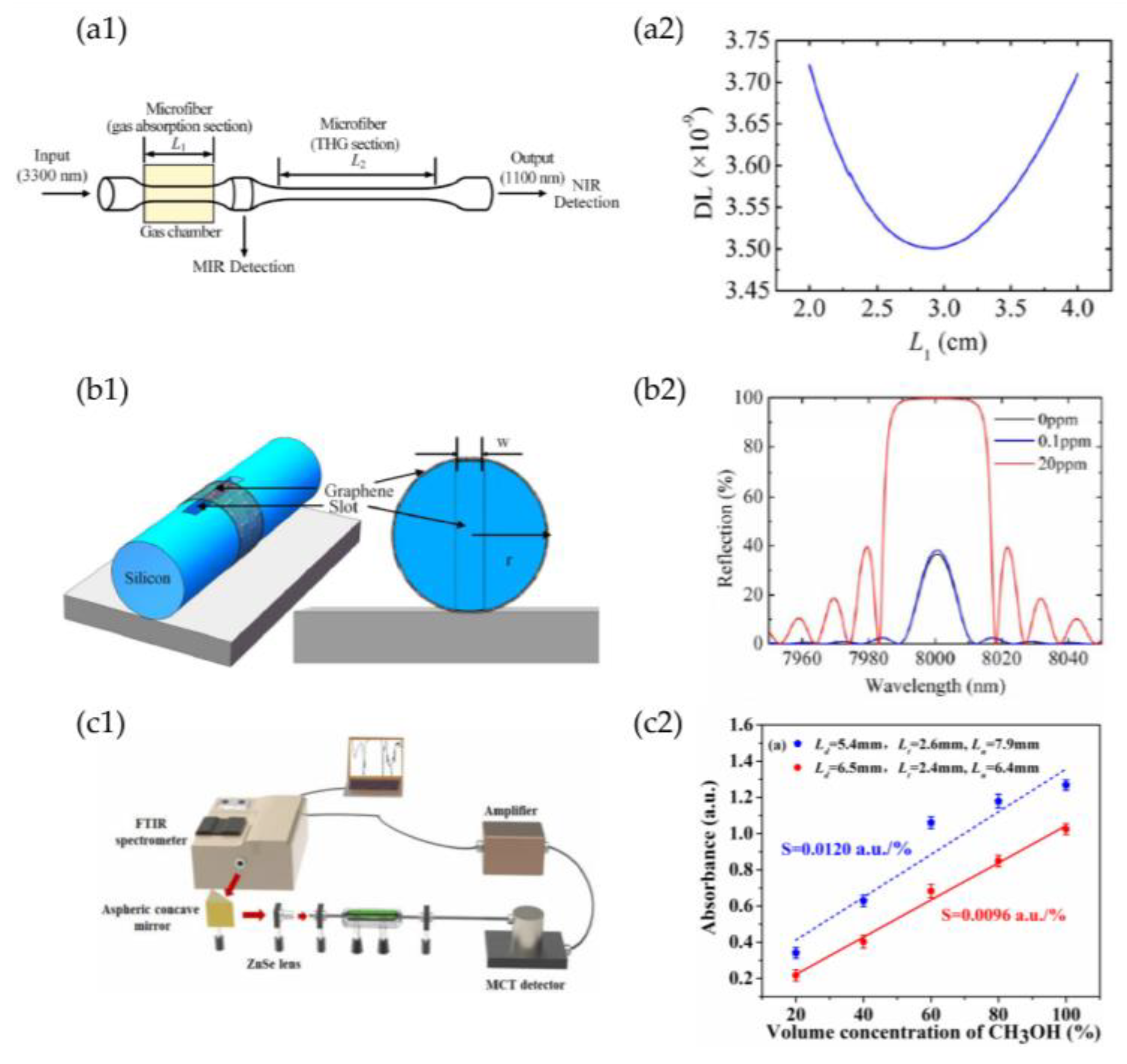
4.8. MF-Based Sensors
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Tong, L.M.; Gattass, R.R.; Ashcom, J.B.; He, S.L.; Lou, J.Y.; Shen, M.Y.; Maxwell, I.; Mazur, E. Subwavelength-diameter silica wires for low-loss optical wave guiding. Nature 2003, 426, 816–819. [Google Scholar] [CrossRef] [PubMed]
- Brambilla, G.; Xu, F.; Horak, P.; Jung, Y.M.; Koizumi, F.; Sessions, N.P.; Koukharenko, E.; Feng, X.; Murugan, G.S.; Wilkinson, J.S.; et al. Optical fiber nanowires and microwires: Fabrication and applications. Adv. Opt. Photonics 2009, 1, 107–161. [Google Scholar] [CrossRef]
- Tong, L.M.; Zi, F.; Guo, X.; Lou, J.Y. Optical microfibers and nanofibers: A tutorial. Opt. Commun. 2012, 285, 4641–4647. [Google Scholar] [CrossRef]
- Wu, X.Q.; Tong, L.M. Optical microfibers and nanofibers. Nanophotonics 2013, 2, 407–428. [Google Scholar] [CrossRef]
- Ismaeel, R.; Lee, T.; Ding, M.; Belal, M.; Brambilla, G. Optical microfiber passive components. Laser Photonics Rev. 2013, 7, 350–384. [Google Scholar] [CrossRef] [Green Version]
- Ward, J.M.; O’Shea, D.G.; Shortt, B.J.; Morrissey, M.J.; Deasy, K.; Nic Chormaic, S.G. Heat-and-pull rig for fiber taper fabrication. Rev. Sci. Instrum. 2006, 77, 083105. [Google Scholar] [CrossRef] [Green Version]
- Xu, Y.X.; Fang, W.; Tong, L.M. Real-time control of micro/nanofiber waist diameter with ultrahigh accuracy and precision. Opt. Express 2017, 25, 10434–10440. [Google Scholar] [CrossRef] [PubMed]
- Tong, L.M.; Lou, J.Y.; Mazur, E. Single-mode guiding properties of subwavelength-diameter silica and silicon wire waveguides. Opt. Express 2004, 12, 1025–1035. [Google Scholar] [CrossRef] [PubMed]
- Foster, M.A.; Moll, K.D.; Gaeta, A.L. Optimal waveguide dimensions for nonlinear interactions. Opt. Express 2004, 12, 2880–2887. [Google Scholar] [CrossRef] [Green Version]
- Foster, M.A.; Turner, A.C.; Lipson, M.; Gaeta, A.L. Nonlinear optics in photonic nanowires. Opt. Express 2008, 16, 1300–1320. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Leon-Saval, S.G.; Birks, T.A.; Wadsworth, W.J.; Russell, P.S.J.; Mason, M.W. Supercontinuum generation in submicron fibre waveguides. Opt. Express 2004, 12, 2864–2869. [Google Scholar] [CrossRef]
- Balykin, V.I.; Hakuta, K.; Le Kien, F.; Liang, J.Q.; Morinaga, M. Atom trapping and guiding with a subwavelength-diameter optical fiber. Phys. Rev. A 2004, 70, 011401. [Google Scholar] [CrossRef] [Green Version]
- Le Kien, F.; Balykin, V.I.; Hakuta, K. Atom trap and waveguide using a two-color evanescent light field around a subwavelength-diameter optical fiber. Phys. Rev. A 2004, 70, 063403. [Google Scholar] [CrossRef] [Green Version]
- Sague, G.; Vetsch, E.; Alt, W.; Meschede, D.; Rauschenbeutel, A. Cold-atom physics using ultrathin optical fibers: Light-induced dipole forces and surface interactions. Phys. Rev. Lett. 2007, 99, 163602. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Guo, X.; Ying, Y.B.; Tong, L.M. Photonic nanowires: From subwavelength waveguides to optical sensors. Acc. Chem. Res. 2014, 47, 656–666. [Google Scholar] [CrossRef] [PubMed]
- Lou, J.Y.; Wang, Y.P.; Tong, L.M. Microfiber optical sensors: A review. Sensors 2014, 14, 5823–5844. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhang, L.; Tang, Y.; Tong, L.M. Micro-/nanofiber optics: Merging photonics and material science on nanoscale for advanced sensing technology. IScience 2020, 23, 100810. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yang, Q.; Jiang, X.S.; Guo, X.; Chen, Y.; Tong, L.M. Hybrid structure laser based on semiconductor nanowires and a silica microfiber knot cavity. Appl. Phys. Lett. 2009, 94, 101108. [Google Scholar]
- Jiang, X.S.; Song, Q.H.; Xu, L.; Fu, J.; Tong, L.M. Microfiber knot dye laser based on the evanescent-wave-coupled gain. Appl. Phys. Lett. 2007, 90, 233501. [Google Scholar] [CrossRef]
- Jiang, X.S.; Yang, Q.; Vienne, G.; Li, Y.H.; Tong, L.M.; Zhang, J.J.; Hu, L.L. Demonstration of microfiber knot laser. Appl. Phys. Lett. 2006, 89, 143513. [Google Scholar] [CrossRef]
- Nagel, S.R.; MacChesney, J.B.; Walker, K.L. Optical Fiber Communications, 1st ed.; Elsevier: New York, NY, USA, 1985; pp. 1–64. [Google Scholar]
- Dai, S.X.; Wang, Y.Y.; Peng, X.F.; Zhang, P.Q.; Wang, X.S.; Xu, Y.S. A review of mid-Infrared supercontinuum generation in chalcogenide glass fibers. Appl. Sci. 2018, 8, 707. [Google Scholar] [CrossRef] [Green Version]
- Gao, S.; Bao, X.Y. Chalcogenide taper and its nonlinear effects and sensing applications. IScience 2020, 23, 100802. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Anashkina, E.A.; Andrianov, A.V.; Kim, A.V. Conversion of ultrashort laser pulses to wavelengths above 3 mm in tapered germanate fibres. Quantum Electron. 2015, 45, 437–442. [Google Scholar] [CrossRef]
- Li, Z.R.; Yao, C.F.; Jia, Z.X.; Wang, F.; Qin, G.S.; Ohishi, Y.; Qin, W.P. Broadband supercontinuum generation from 600 to 5400 nm in a tapered fluorotellurite fiber pumped by a 2010 nm femtosecond fiber laser. Appl. Phys. Lett. 2019, 115, 091103. [Google Scholar] [CrossRef]
- Saini, T.S.; Hoa, N.P.T.; Tuan, T.H.; Luo, X.; Suzuki, T.; Nish, Y. Tapered tellurite step-index optical fiber for coherent near-to-mid-IR supercontinuum generation: Experiment and modeling. Appl. Opt. 2019, 58, 415–421. [Google Scholar] [CrossRef] [PubMed]
- Kubat, I.; Agger, C.S.; Moselund, P.M.; Bang, O. Mid-infrared supercontinuum generation to 45 μm in uniform and tapered ZBLAN step-index fibers by direct pumping at 1064 or 1550 nm. J. Opt. Soc. Am. B 2013, 30, 2743–2757. [Google Scholar] [CrossRef]
- Chen, Z.G.; Taylor, A.J.; Efimov, A. Coherent mid-infrared broadband continuum generation in non-uniform ZBLAN fiber taper. Opt. Express 2009, 17, 5852–5860. [Google Scholar] [CrossRef] [PubMed]
- Zakery, A.; Elliott, S.R. Optical properties and applications of chalcogenide glasses: A review. J. Non-Cryst. Solids 2003, 330, 1–12. [Google Scholar] [CrossRef]
- Eggleton, B.J.; Luther-Davies, B.; Richardson, K. Chalcogenide photonics. Nat. Photonics 2011, 5, 141–148. [Google Scholar] [CrossRef]
- Baker, C.; Rochette, M. Highly nonlinear hybrid AsSe-PMMA microtapers. Opt. Express 2010, 18, 12391–12398. [Google Scholar] [CrossRef]
- Xin, C.G.; Wu, H.; Xie, Y.; Yu, S.L.; Zhou, N.; Shi, Z.X.; Guo, X.; Tong, L.M. CdTe microwires as mid-infrared optical waveguides. Opt. Express 2018, 26, 10944–10952. [Google Scholar] [CrossRef] [PubMed]
- Ren, H.; Shen, L.; Wu, D.; Aktas, O.; Hawkins, T.; Ballato, J.; Gibson, U.J.; Peacock, A.C. Nonlinear optical properties of polycrystalline silicon core fibers from telecom wavelengths into the mid-infrared spectral region. Opt. Mater. Express 2019, 9, 1271–1279. [Google Scholar] [CrossRef]
- Shiryaev, V.S.; Churbanov, M.F. Recent advances in preparation of high-purity chalcogenide glasses for mid-IR photonics. J. Non-Cryst. Solids 2017, 475, 1–9. [Google Scholar] [CrossRef]
- Tao, G.M.; Ebendorff-Heidepriem, H.; Stolyarov, A.M.; Danto, S.; Badding, J.V.; Fink, Y.; Ballato, J.; Abouraddy, A.F. Infrared fibers. Adv. Opt. Photonics 2015, 7, 379–458. [Google Scholar] [CrossRef]
- Churbanov, M.F.; Snopatin, G.E.; Shiryaev, V.S.; Plotnichenko, V.G.; Dianov, E.M. Recent advances in preparation of high-purity glasses based on arsenic chalcogenides for fiber optics. J. Non-Cryst. Solids 2011, 357, 2352–2357. [Google Scholar] [CrossRef]
- Aktas, O. Chalcogenide microresonators tailored to distinct morphologies by the shaping of glasses on silica tapers. Opt. Lett. 2017, 42, 907–910. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Q.M.; Li, M.; Hao, Q.; Deng, D.H.; Zhou, H.; Zeng, H.P.; Zhan, L.; Wu, X.; Liu, L.Y.; Xu, L. Fabrication and characterization of on-chip optical nonlinear chalcogenide nanofiber devices. Opt. Lett. 2010, 35, 3829–3831. [Google Scholar] [CrossRef] [PubMed]
- Ahmad, R.; Rochette, M. Photosensitivity at 1550 nm and Bragg grating inscription in As2Se3 chalcogenide microwires. Appl. Phys. Lett. 2011, 99, 061109. [Google Scholar] [CrossRef]
- Ahmad, R.; Rochette, M.; Baker, C. Fabrication of Bragg gratings in subwavelength diameter As2Se3 chalcogenide wires. Opt. Lett. 2011, 36, 2886–2888. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Florea, C.; Sanghera, J.S.; Shaw, B.; Aggarwal, I.D. Fiber Bragg gratings in As2S3 fibers obtained using a 0/−1 phase mask. Opt. Mater. 2009, 31, 942–944. [Google Scholar] [CrossRef]
- Gao, S.; Baker, C.; Cai, W.; Chen, L.; Bao, X.Y. 10 kHz-34 MHz ultrasound detection based on a dual-core hybrid taper. APL Photonics 2019, 4, 110805. [Google Scholar] [CrossRef] [Green Version]
- Fan, H.B.; Chen, L.; Bao, X.Y. Chalcogenide microfiber-assisted silica microfiber for ultrasound detection. Opt. Lett. 2020, 45, 1128–1131. [Google Scholar] [CrossRef] [PubMed]
- Yeom, D.I.; Magi, E.C.; Lamont, M.R.; Roelens, M.A.; Fu, L.B.; Eggleton, B.J. Low-threshold supercontinuum generation in highly nonlinear chalcogenide nanowires. Opt. Lett. 2008, 33, 660–662. [Google Scholar] [CrossRef]
- Xie, Y.; Cai, D.W.; Wu, H.; Pan, J.; Zhou, N.; Xin, C.G.; Yu, S.L.; Wang, P.; Jiang, X.S.; Qiu, J.R.; et al. Mid-infrared chalcogenide microfiber knot resonators. Photonics Res. 2020, 8, 616–621. [Google Scholar] [CrossRef]
- Cai, D.W.; Xie, Y.; Wang, P.; Zhang, L.; Guo, X.; Tong, L.M. Mid-infrared microfiber Bragg gratings. Opt. Lett. 2020, 45, 6114–6117. [Google Scholar] [CrossRef] [PubMed]
- Tong, L.M.; Lou, J.Y.; Ye, Z.Z.; Svacha, G.T.; Mazur, E. Self-modulated taper drawing of silica nanowires. Nanotechnology 2005, 16, 1445–1448. [Google Scholar] [CrossRef]
- Tong, L.M.; Hu, L.L.; Zhang, J.J.; Qiu, J.R.; Yang, Q.; Lou, J.Y.; Shen, Y.H.; He, J.L.; Ye, Z.Z. Photonic nanowires directly drawn from bulk glasses. Opt. Express 2006, 14, 82–87. [Google Scholar] [CrossRef]
- Li, L.Z.; Al-Kadry, A.; Abdukerim, N.; Rochette, M. Design, fabrication and characterization of PC, COP and PMMA-cladded As2Se3 microwires. Opt. Mater. Express 2016, 6, 912–921. [Google Scholar] [CrossRef]
- Baker, C.; Rochette, M. A generalized heat-brush approach for precise control of the waist profile in fiber tapers. Opt. Mater. Express 2011, 1, 1065–1076. [Google Scholar] [CrossRef]
- Rodney, W.S.; Malitson, I.H.; King, T.A. Refractive index of arsenic trisulfide. J. Opt. Soc. Am. 1958, 48, 633–636. [Google Scholar] [CrossRef]
- Van Popta, A.C.; DeCorby, R.G.; Haugen, C.J.; Robinson, T.; McMullin, J.N.; Tonchev, D.; Kasap, S.O. Photoinduced refractive index change in As2Se3 by 633nm illumination. Opt. Express 2002, 10, 639–644. [Google Scholar] [CrossRef] [PubMed]
- Huang, K.J.; Yang, S.Y.; Tong, L.M. Modeling of evanescent coupling between two parallel optical nanowires. Appl. Opt. 2007, 46, 1429–1434. [Google Scholar] [CrossRef]
- Singh, V.; Lin, P.T.; Patel, N.; Lin, H.; Li, L.; Zou, Y.; Deng, F.; Ni, C.; Hu, J.; Giammarco, J.; et al. Mid-infrared materials and devices on a Si platform for optical sensing. Sci. Technol. Adv. Mater. 2014, 15, 014603. [Google Scholar] [CrossRef]
- Hudson, D.D.; Dekker, S.A.; Maegi, E.C.; Judge, A.C.; Jackson, S.D.; Li, E.B.; Sanghera, J.S.; Shaw, L.B.; Aggarwal, I.D.; Eggleton, B.J. Octave spanning supercontinuum in an As2S3 taper using ultralow pump pulse energy. Opt. Lett. 2011, 36, 1122–1124. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Guo, X.; Qiu, M.; Bao, J.M.; Wiley, B.J.; Yang, Q.; Zhang, X.N.; Ma, Y.G.; Yu, H.K.; Tong, L.M. Direct coupling of plasmonic and photonic nanowires for hybrid nanophotonic components and circuits. Nano Lett. 2009, 9, 4515–4519. [Google Scholar] [CrossRef] [PubMed]
- Ding, Z.X.; Huang, Z.N.; Chen, Y.; Mou, C.B.; Lu, Y.Q.; Xu, F. All-fiber ultrafast laser generating gigahertz-rate pulses based on a hybrid plasmonic microfiber resonator. Adv. Photon. 2020, 2, 026002. [Google Scholar] [CrossRef] [Green Version]
- Ma, Y.Q.; Farrell, G.; Semenova, Y.; Li, B.H.; Yuan, J.H.; Sang, X.Z.; Yan, B.B.; Yu, C.X.; Guo, T.; Wu, Q. Optical microfiber-loaded surface plasmonic TE-pass polarizer. Opt. Laser Technol. 2016, 78, 101–105. [Google Scholar] [CrossRef]
- Vahala, K.J. Optical microcavities. Nature 2003, 424, 839–846. [Google Scholar] [CrossRef] [PubMed]
- He, L.N.; Ozdemir, K.; Zhu, J.G.; Kim, W.; Yang, L. Detecting single viruses and nanoparticles using whispering gallery microlasers. Nat. Nanotechnol. 2011, 6, 428–432. [Google Scholar] [CrossRef] [Green Version]
- Choi, J.; Noh, H. Enhanced absorption by coherent control in a photonic crystal resonator coupled with a microfiber. Opt. Lett. 2018, 43, 5532–5534. [Google Scholar] [CrossRef] [PubMed]
- Kim, M.K.; Hwang, I.K.; Kim, S.H.; Chang, H.J.; Lee, Y.H. All-optical bistable switching in curved microfiber-coupled photonic crystal resonators. Appl. Phys. Lett. 2007, 90, 161118. [Google Scholar] [CrossRef] [Green Version]
- Hwang, I.K.; Kim, S.K.; Yang, J.K.; Kim, S.H.; Lee, S.H.; Lee, Y.H. Curved-microfiber photon coupling for photonic crystal light emitter. Appl. Phys. Lett. 2005, 87, 131107. [Google Scholar] [CrossRef] [Green Version]
- Lecaplain, C.; Javerzac-Galy, C.; Gorodetsky, M.L.; Kippenberg, T.J. Mid-infrared ultra-high-Q resonators based on fluoride crystalline materials. Nat. Commun. 2016, 7, 13383. [Google Scholar] [CrossRef] [PubMed]
- Xie, Y.; Cai, D.W.; Pan, J.; Zhou, N.; Gao, Y.X.; Jin, Y.Y.; Jiang, X.S.; Qiu, J.R.; Wang, P.; Guo, X.; et al. Batch fabrication of high-quality infrared chalcogenide microsphere resonators. Small 2021, 17, 2100140. [Google Scholar] [CrossRef] [PubMed]
- Heylman, K.D.; Knapper, K.A.; Horak, E.H.; Rea, M.T.; Vanga, S.K.; Goldsmith, R.H. Optical microresonators for sensing and transduction: A materials perspective. Adv. Mater. 2017, 29, 1700037. [Google Scholar] [CrossRef]
- Sumetsky, M.; Dulashko, Y.; Fini, J.M.; Hale, A.; DiGiovanni, D.J. The microfiber loop resonator: Theory, experiment, and application. J. Lightwave Technol. 2006, 24, 242–250. [Google Scholar] [CrossRef]
- Guo, X.; Li, Y.H.; Jiang, X.S.; Tong, L.M. Demonstration of critical coupling in microfiber loops wrapped around a copper rod. Appl. Phys. Lett. 2007, 91, 073512. [Google Scholar] [CrossRef]
- Jiang, X.S.; Tong, L.M.; Vienne, G.; Guo, X.; Tsao, A.; Yang, Q.; Yang, D.R. Demonstration of optical microfiber knot resonators. Appl. Phys. Lett. 2006, 88, 223501. [Google Scholar] [CrossRef]
- Wang, P.; Zhang, L.; Yang, Z.Y.; Gu, F.X.; Wang, S.S.; Yang, Q.; Tong, L.M. Fusion spliced microfiber closed-loop resonators. IEEE Photonics Technol. Lett. 2010, 22, 1075–1077. [Google Scholar] [CrossRef]
- Li, W.; Wang, P.; Hu, Z.F.; Tong, L.M. Fusion splicing soft glass microfibers for photonic devices. IEEE Photonics Technol. Lett. 2011, 23, 831–833. [Google Scholar] [CrossRef]
- Zhang, B.; Zeng, P.Y.; Yang, Z.L.; Xia, D.; Zhao, J.X.; Sun, Y.D.; Huang, Y.F.; Song, J.C.; Pan, J.S.; Cheng, H.J.; et al. On-chip chalcogenide microresonators with low-threshold parametric oscillation. Photonics Res. 2021, 9, 1272–1279. [Google Scholar] [CrossRef]
- Sumetsky, M. Optical fiber microcoil resonators. Opt. Express 2004, 12, 2303–2316. [Google Scholar] [CrossRef]
- Scheuer, J.; Sumetsky, M. Optical-fiber microcoil waveguides and resonators and their applications for interferometry and sensing. Laser Photon. Rev. 2011, 5, 465–478. [Google Scholar] [CrossRef]
- Xu, Z.L.; Sun, Q.Z.; Li, B.R.; Luo, Y.Y.; Lu, W.G.; Liu, D.M.; Shum, P.P.; Zhang, L. Highly sensitive refractive index sensor based on cascaded microfiber knots with Vernier effect. Opt. Express 2015, 23, 6662–6672. [Google Scholar] [CrossRef] [PubMed]
- Liu, M.; Tang, R.; Luo, A.P.; Xu, W.C.; Luo, Z.C. Graphene-decorated microfiber knot as a broadband resonator for ultrahigh-repetition-rate pulse fiber lasers. Photonics Res. 2018, 6, C1–C7. [Google Scholar] [CrossRef]
- Luan, F.; Magi, E.; Gong, T.X.; Kabakova, I.; Eggleton, B.J. Photoinduced whispering gallery mode microcavity resonator in a chalcogenide microfiber. Opt. Lett. 2011, 36, 4761–4763. [Google Scholar] [CrossRef] [PubMed]
- Ma, P.; Choi, D.Y.; Yu, Y.; Yang, Z.Y.; Vu, K.; Nguyen, T.; Mitchell, A.; Luther-Davies, B.; Madden, S. High Q factor chalcogenide ring resonators for cavity-enhanced MIR spectroscopic sensing. Opt. Express 2015, 23, 19969–19979. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Grobnic, D.; Mihailov, S.J.; Ding, H.M.; Smelser, C.W. Bragg grating evanescent field sensor made in biconical tapered fiber with femtosecond IR radiation. IEEE Photonics Technol. Lett. 2006, 18, 160–162. [Google Scholar] [CrossRef]
- Fang, X.; Liao, C.R.; Wang, D.N. Femtosecond laser fabricated fiber Bragg grating in microfiber for refractive index sensing. Opt. Lett. 2010, 35, 1007–1009. [Google Scholar] [CrossRef] [Green Version]
- Ran, Y.; Jin, L.; Tan, Y.N.; Sun, L.P.; Li, J.; Guan, B.O. High-efficiency ultraviolet inscription of Bragg gratings in microfibers. IEEE Photonics J. 2012, 4, 181–186. [Google Scholar] [CrossRef]
- Kou, J.L.; Qiu, S.J.; Xu, F.; Lu, Y.Q. Demonstration of a compact temperature sensor based on first-order Bragg grating in a tapered fiber probe. Opt. Express 2011, 19, 18452–18457. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.X.; Meng, C.; Zhang, A.P.; Xiao, Y.; Yu, H.K.; Tong, L.M. Compact microfiber Bragg gratings with high-index contrast. Opt. Lett. 2011, 36, 3115–3117. [Google Scholar] [CrossRef] [PubMed]
- Nayak, K.P.; Le Kien, F.; Kawai, Y.; Hakuta, K.; Nakajima, K.; Miyazaki, H.T.; Sugimoto, Y. Cavity formation on an optical nanofiber using focused ion beam milling technique. Opt. Express 2011, 19, 14040–14050. [Google Scholar] [CrossRef] [PubMed]
- Guan, B.O.; Li, J.; Jin, L.; Ran, Y. Fiber Bragg gratings in optical microfibers. Opt. Fiber Technol. 2013, 19, 793–801. [Google Scholar] [CrossRef]
- Gao, S.; Jin, L.; Ran, Y.; Sun, L.P.; Li, J.; Guan, B.O. Temperature compensated microfiber Bragg gratings. Opt. Express 2012, 20, 18281–18286. [Google Scholar] [CrossRef] [PubMed]
- Luo, W.; Kou, J.L.; Chen, Y.; Xu, F.; Lu, Y.Q. Ultra-highly sensitive surface-corrugated microfiber Bragg grating force sensor. Appl. Phys. Lett. 2012, 101, 133502. [Google Scholar] [CrossRef]
- Bernier, M.; El-Amraoui, M.; Couillard, J.F.; Messaddeq, Y.; Vallee, R. Writing of Bragg gratings through the polymer jacket of low-loss As2S3 fibers using femtosecond pulses at 800 nm. Opt. Lett. 2012, 37, 3900–3902. [Google Scholar] [CrossRef] [PubMed]
- Baker, C.; Gao, S.; Chen, L.; Bao, X.Y. Self-inscribed antisymmetric long-period grating in a dual-core As2Se3-PMMA fiber. Opt. Express 2017, 25, 12409–12414. [Google Scholar] [CrossRef]
- Wang, L.L.; Ma, W.Q.; Zhang, P.Q.; Yang, D.D.; Zhu, L.; Wang, X.S.; Dai, S.X. Design and analysis of long-period fiber gratings in tapered multimode chalcogenide glass fiber for temperature measurement. J. Opt. Soc. Am. B 2019, 36, 1792–1798. [Google Scholar] [CrossRef]
- Supradeepa, V.R.; Feng, Y.; Nicholson, J.W. Raman fiber lasers. J. Opt. 2017, 19, 023001. [Google Scholar] [CrossRef]
- Pierce, M.C.; Jackson, S.D.; Dickinson, M.R.; King, T.A.; Sloan, P. Laser-tissue interaction with a continuous wave 3-μm fibre laser: Preliminary studies with soft tissue. Lasers Surg. Med. 2000, 26, 491–495. [Google Scholar] [CrossRef]
- Jackson, S.D. Towards high-power mid-infrared emission from a fibre laser. Nat. Photonics 2012, 6, 423–431. [Google Scholar] [CrossRef]
- White, R.T.; Monro, T.M. Cascaded Raman shifting of high-peak-power nanosecond pulses in As2S3 and As2Se3 optical fibers. Opt. Lett. 2011, 36, 2351–2353. [Google Scholar] [CrossRef] [PubMed]
- Abdukerim, N.; Li, L.Z.; El Amraoui, M.; Messaddeq, Y.; Rochette, M. 2 μm Raman fiber laser based on a multimaterial chalcogenide microwire. Appl. Phys. Lett. 2017, 110, 161103. [Google Scholar] [CrossRef]
- Guo, X.H.; Li, Z.R.; Jiao, Y.D.; Zhao, Z.P.; Yao, C.F.; Jia, Z.X.; Dong, K.G.; Zhang, H.Y.; Lin, H.H.; Qin, W.P.; et al. A shower of mid-infrared Raman solitons at designed wavelength of similar to 3 μm from a tapered fluorotellurite fiber. Laser Phys. 2021, 31, 095103. [Google Scholar] [CrossRef]
- Alamgir, I.; Abdukerim, N.; Rochette, M. In situ fabrication of far-detuned optical fiber wavelength converters. Opt. Lett. 2019, 44, 4467–4470. [Google Scholar] [CrossRef] [PubMed]
- Godin, T.; Combes, Y.; Ahmad, R.; Rochette, M.; Sylvestre, T.; Dudley, J.M. Far-detuned mid-infrared frequency conversion via normal dispersion modulation instability in chalcogenide microwires. Opt. Lett. 2014, 39, 1885–1888. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Li, L.Z.; Abdukerim, N.; Rochette, M. Mid-infrared wavelength conversion from As2Se3 microwires. Opt. Lett. 2017, 42, 639–642. [Google Scholar] [CrossRef]
- Liang, F.; Kang, L.; Lin, Z.S.; Wu, Y.C. Mid-infrared nonlinear optical materials based on metal chalcogenides: Structure-property relationship. Cryst. Growth Des. 2017, 17, 2254–2289. [Google Scholar] [CrossRef]
- Abdukerim, N.; Li, L.Z.; Rochette, M. Chalcogenide-based optical parametric oscillator at 2 μm. Opt. Lett. 2016, 41, 4364–4367. [Google Scholar] [CrossRef]
- Al-Kadry, A.; El Amraoui, M.; Messaddeq, Y.; Rochette, M. Two octaves mid-infrared supercontinuum generation in As2Se3 microwires. Opt. Express 2014, 22, 31131–31137. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, Y.Y.; Dai, S.X.; Li, G.T.; Xu, D.; You, C.Y.; Han, X.; Zhang, P.Q.; Wang, X.S.; Xu, P.P. 1.4–7.2 μm broadband supercontinuum generation in an As-S chalcogenide tapered fiber pumped in the normal dispersion regime. Opt. Lett. 2017, 42, 3458–3461. [Google Scholar] [CrossRef] [PubMed]
- Li, G.T.; Peng, X.F.; Dai, S.X.; Wang, Y.Y.; Xie, M.; Yang, L.; Yang, C.F.; Wei, W.Y.; Zhang, P.Q. Highly coherent 1.5–8.3 μm broadband supercontinuum generation in tapered As-S chalcogenide fibers. J. Lightwave Technol. 2019, 37, 1847–1852. [Google Scholar] [CrossRef]
- Hudson, D.D.; Antipov, S.; Li, L.Z.; Alamgir, I.; Hu, T.; Amraoui, M.; Messaddeq, Y.; Rochette, M.; Jackson, S.D.; Fuerbach, A. Toward all-fiber supercontinuum spanning the mid-infrared. Optica 2017, 4, 1163–1166. [Google Scholar] [CrossRef]
- Marandi, A.; Rudy, C.W.; Plotnichenko, V.G.; Dianov, E.M.; Vodopyanov, K.L.; Byer, R.L. Mid-infrared supercontinuum generation in tapered chalcogenide fiber for producing octave-spanning frequency comb around 3 μm. Opt. Express 2012, 20, 24218–24225. [Google Scholar] [CrossRef] [PubMed]
- Dudley, J.M.; Genty, G.; Coen, S. Supercontinuum generation in photonic crystal fiber. Rev. Mod. Phys. 2006, 78, 1135–1184. [Google Scholar] [CrossRef]
- Al-kadry, A.; Baker, C.; El Amraoui, M.; Messaddeq, Y.; Rochette, M. Broadband supercontinuum generation in As2Se3 chalcogenide wires by avoiding the two-photon absorption effects. Opt. Lett. 2013, 38, 1185–1187. [Google Scholar] [CrossRef]
- Rudy, C.W.; Marandi, A.; Vodopyanov, K.L.; Byer, R.L. Octave-spanning supercontinuum generation in in situ tapered As2S3 fiber pumped by a thulium-doped fiber laser. Opt. Lett. 2013, 38, 2865–2868. [Google Scholar] [CrossRef] [Green Version]
- Sun, Y.A.; Dai, S.X.; Zhang, P.Q.; Wang, X.S.; Xu, Y.S.; Liu, Z.J.; Chen, F.F.; Wu, Y.A.; Zhang, Y.J.; Wang, R.P.; et al. Fabrication and characterization of multimaterial chalcogenide glass fiber tapers with high numerical apertures. Opt. Express 2015, 23, 23472–23483. [Google Scholar] [CrossRef]
- Anashkina, E.A.; Shiryaev, V.S.; Koptev, M.Y.; Stepanov, B.S.; Muravyev, S.V. Development of of As-Se tapered suspended-core fibers for ultra-broadband mid-IR wavelength conversion. J. Non-Cryst. Solids 2018, 480, 43–50. [Google Scholar] [CrossRef] [Green Version]
- Leonov, S.O.; Wang, Y.C.; Shiryaev, V.S.; Snopatin, G.E.; Stepanov, B.S.; Plotnichenko, V.G.; Vicentini, E.; Gambetta, A.; Coluccelli, N.; Svelto, C.; et al. Coherent mid-infrared supercontinuum generation in tapered suspended-core As39Se61 fibers pumped by a few-optical-cycle Cr:ZnSe laser. Opt. Lett. 2020, 45, 1346–1349. [Google Scholar] [CrossRef]
- Leindecker, N.; Marandi, A.; Byer, R.L.; Vodopyanov, K.L. Broadband degenerate OPO for mid-infrared frequency comb generation. Opt. Express 2011, 19, 6304–6310. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Reid, D.T.; Gale, B.J.S.; Sun, J. Frequency comb generation and carrier-envelope phase control in femtosecond optical parametric oscillators. Laser Phys. 2008, 18, 87–103. [Google Scholar] [CrossRef]
- Keilmann, F.; Gohle, C.; Holzwarth, R. Time-domain mid-infrared frequency-comb spectrometer. Opt. Lett. 2004, 29, 1542–1544. [Google Scholar] [CrossRef] [Green Version]
- Erny, C.; Moutzouris, K.; Biegert, J.; Kuhlke, D.; Adler, F.; Leitenstorfer, A.; Keller, U. Mid-infrared difference-frequency generation of ultrashort pulses tunable between 3.2 and 4.8 μm from a compact fiber source. Opt. Lett. 2007, 32, 1138–1140. [Google Scholar] [CrossRef] [Green Version]
- Del’Haye, P.; Herr, T.; Gavartin, E.; Gorodetsky, M.L.; Holzwarth, R.; Kippenberg, T.J. Octave spanning tunable frequency comb from a microresonator. Phys. Rev. Lett. 2011, 107, 063901. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Del’Haye, P.; Schliesser, A.; Arcizet, O.; Wilken, T.; Holzwarth, R.; Kippenberg, T.J. Optical frequency comb generation from a monolithic microresonator. Nature 2007, 450, 1214–1217. [Google Scholar] [CrossRef] [Green Version]
- Bernhardt, B.; Sorokin, E.; Jacquet, P.; Thon, R.; Becker, T.; Sorokina, I.T.; Picque, N.; Hansch, T.W. Mid-infrared dual-comb spectroscopy with 2.4 μm Cr2+:ZnSe femtosecond lasers. Appl. Phys. B 2010, 100, 3–8. [Google Scholar] [CrossRef] [Green Version]
- Adler, F.; Cossel, K.C.; Thorpe, M.J.; Hartl, I.; Fermann, M.E.; Ye, J. Phase-stabilized, 1.5 W frequency comb at 2.8–4.8 μm. Opt. Lett. 2009, 34, 1330–1332. [Google Scholar] [CrossRef] [PubMed]
- Lee, K.F.; Granzow, N.; Schmidt, M.A.; Chang, W.; Wang, L.; Coulombier, Q.; Troles, J.; Leindecker, N.; Vodopyanov, K.L.; Schunemann, P.G.; et al. Midinfrared frequency combs from coherent supercontinuum in chalcogenide and optical parametric oscillation. Opt. Lett. 2014, 39, 2056–2059. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sanghera, J.S.; Shaw, L.B.; Busse, L.E.; Talley, D.; Aggarwal, I.D. Infrared transmitting fiber optics for biomedical applications. In Proceedings of the BiOS ’99 International Biomedical Optics Symposium, San Jose, CA, USA, 23–29 January 1999; Volume 3596, pp. 178–187. [Google Scholar]
- Sanghera, J.S.; Kung, F.H.; Busse, L.E.; Pureza, P.C.; Aggarwal, I.D. Infrared evanescent absorption-spectroscopy of toxic-chemicals using chalcogenide class fibers. J. Am. Ceram. Soc. 1995, 78, 2198–2202. [Google Scholar] [CrossRef]
- Michel, K.; Bureau, B.; Pouvreau, C.; Sangleboeuf, J.C.; Boussard-Pledel, C.; Jouan, T.; Rouxel, T.; Adam, J.L.; Staubmann, K.; Steinner, H.; et al. Development of a chalcogenide glass fiber device for in situ pollutant detection. J. Non-Cryst. Solids 2003, 326, 434–438. [Google Scholar] [CrossRef]
- Huang, P.; Huang, T.Y.; Zeng, S.W.; Pan, J.X.; Wu, X.; Zhao, X.; Wu, Y.H.; Ping, P.S.; Brambilla, G. Nonlinear gas sensing based on third-harmonic generation in cascaded chalcogenide microfibers. J. Opt. Soc. Am. B 2019, 36, 300–305. [Google Scholar] [CrossRef]
- Wang, J.Q.; Chen, Y.Z.; Geng, Y.F.; Hong, X.M.; Li, X.J. Theoretical design of mid-infrared graphene optical gas sensor based on slot Si core fiber. IEEE Photonics Technol. Lett. 2019, 31, 1096–1099. [Google Scholar] [CrossRef]
- Wang, M.; Yang, F.; Dai, S.X.; Cao, Z.F.; Su, J.X.; Ding, S.J.; Zhang, P.Q. Effect of the geometries of Ge-Sb-Se chalcogenide glass tapered fiber on the sensitivity of evanescent wave sensors. J. Lightwave Technol. 2021, 39, 4828–4836. [Google Scholar] [CrossRef]
- Michel, K.; Bureau, B.; Boussard-Pledel, C.; Jouan, T.; Adam, J.L.; Staubmann, K.; Baumann, T. Monitoring of pollutant in waste water by infrared spectroscopy using chalcogenide glass optical fibers. Sens. Actuators B Chem. 2004, 101, 252–259. [Google Scholar] [CrossRef]
- Keirsse, J.; Boussard-Pledel, C.; Loreal, O.; Sire, O.; Bureau, B.; Turlin, B.; Leroyer, P.; Lucas, J. Chalcogenide glass fibers used as biosensors. J. Non-Cryst. Solids 2003, 326, 430–433. [Google Scholar] [CrossRef]
- Le Coq, D.; Michel, K.; Keirsse, J.; Boussard-Pledel, C.; Fonteneaua, G.; Bureau, B.; Le Quere, J.M.; Sire, O.; Lucas, J. Infrared glass fibers for in-situ sensing, chemical and biochemical reactions. Comptes Rendus Chim. 2002, 5, 907–913. [Google Scholar] [CrossRef]
- Hocde, S.; Loreal, O.; Sire, O.; Boussard-Pledel, C.; Bureau, B.; Turlin, B.; Keirsse, J.; Leroyer, P.; Lucas, J. Metabolic imaging of tissues by infrared fiber-optic spectroscopy: An efficient tool for medical diagnosis. J. Biomed. Opt. 2004, 9, 404–407. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gutierrez-Arroyo, A.; Baudet, E.; Bodiou, L.; Nazabal, V.; Rinnert, E.; Michel, K.; Bureau, B.; Colas, F.; Charrier, J. Theoretical study of an evanescent optical integrated sensor for multipurpose detection of gases and liquids in the mid-infrared. Sens. Actuators B Chem. 2017, 242, 842–848. [Google Scholar] [CrossRef]
- Wang, X.M.; Su, J.X.; Wang, Y.Y.; Yang, C.F.; Dai, S.X.; Zhang, P.Q. High-sensitivity sensing in bare Ge-Sb-Se chalcogenide tapered fiber with optimal structure parameters. J. Non-Cryst. Solids 2021, 559, 120686. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cai, D.; Xie, Y.; Guo, X.; Wang, P.; Tong, L. Chalcogenide Glass Microfibers for Mid-Infrared Optics. Photonics 2021, 8, 497. https://doi.org/10.3390/photonics8110497
Cai D, Xie Y, Guo X, Wang P, Tong L. Chalcogenide Glass Microfibers for Mid-Infrared Optics. Photonics. 2021; 8(11):497. https://doi.org/10.3390/photonics8110497
Chicago/Turabian StyleCai, Dawei, Yu Xie, Xin Guo, Pan Wang, and Limin Tong. 2021. "Chalcogenide Glass Microfibers for Mid-Infrared Optics" Photonics 8, no. 11: 497. https://doi.org/10.3390/photonics8110497
APA StyleCai, D., Xie, Y., Guo, X., Wang, P., & Tong, L. (2021). Chalcogenide Glass Microfibers for Mid-Infrared Optics. Photonics, 8(11), 497. https://doi.org/10.3390/photonics8110497





