Wavelength-Dependent Features of Photoelectron Spectra from Nanotip Photoemission
Abstract
1. Introduction
2. Theoretical Methods
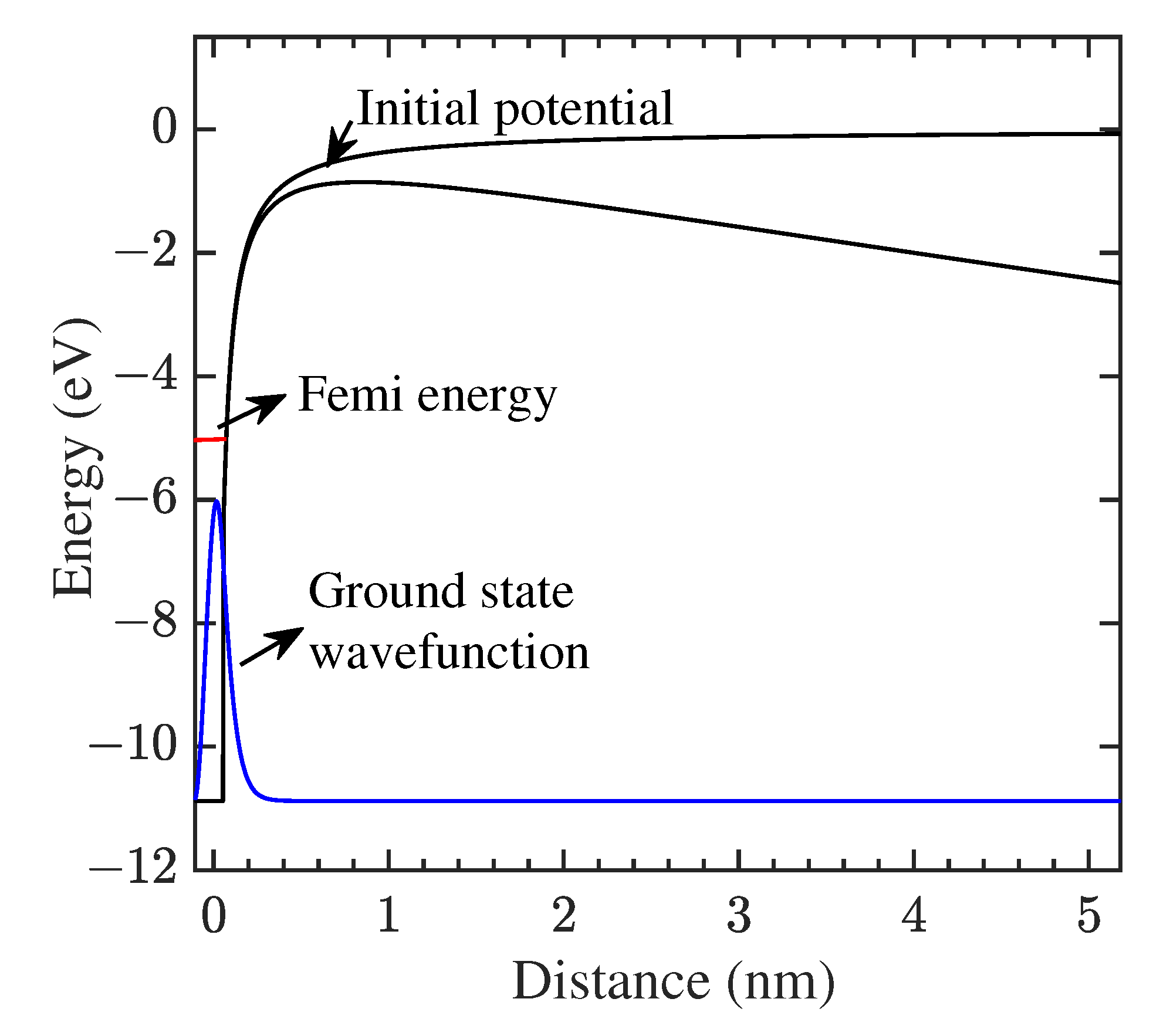
2.1. Time-Dependent Schrödinger Equation Method
2.2. Classical Trajectory Monte Carlo Method
3. Results and Discussion
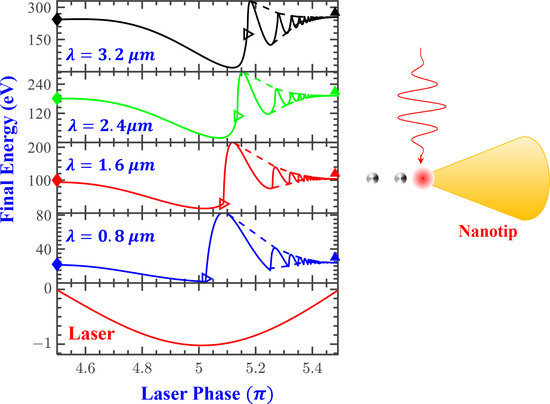
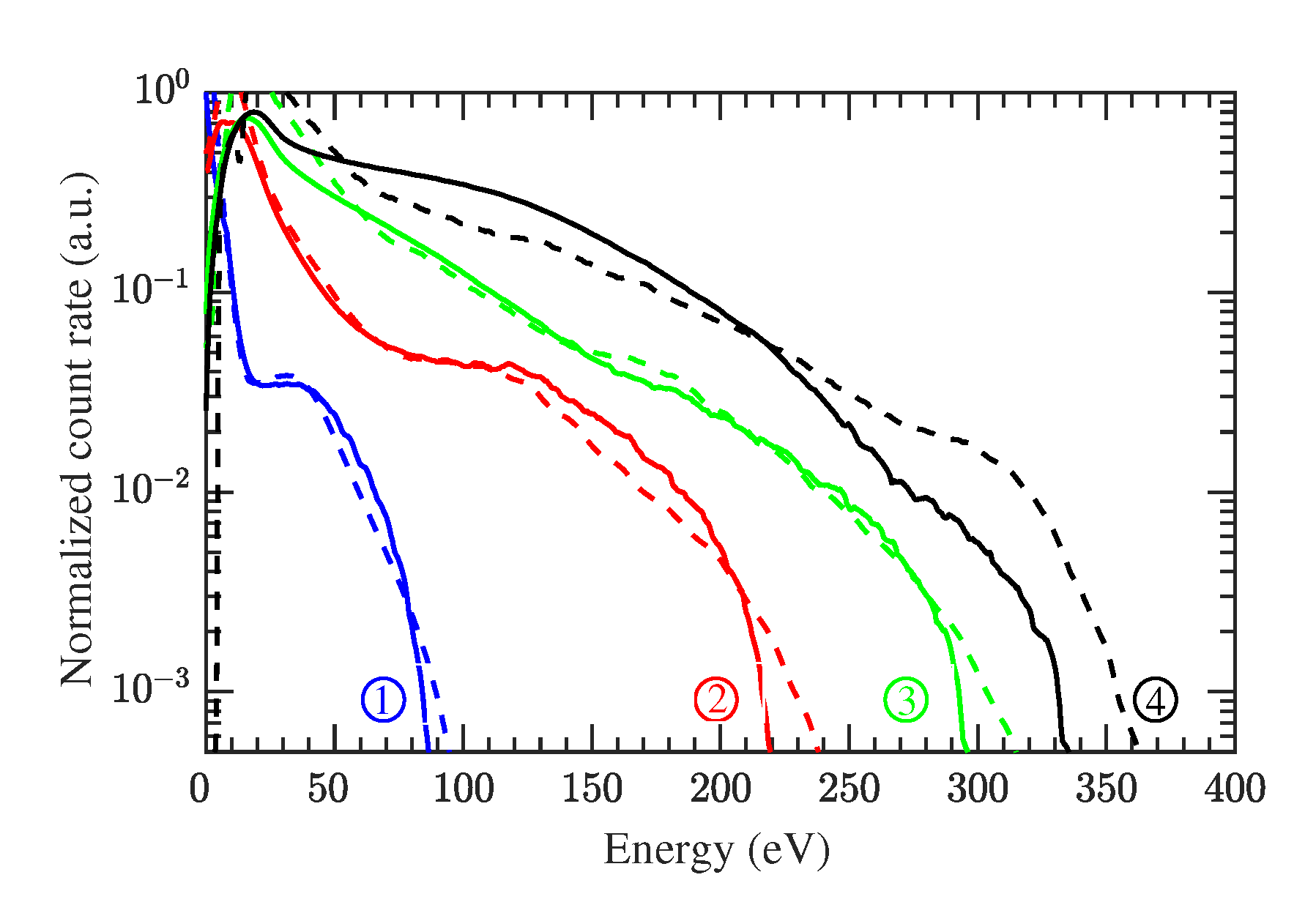
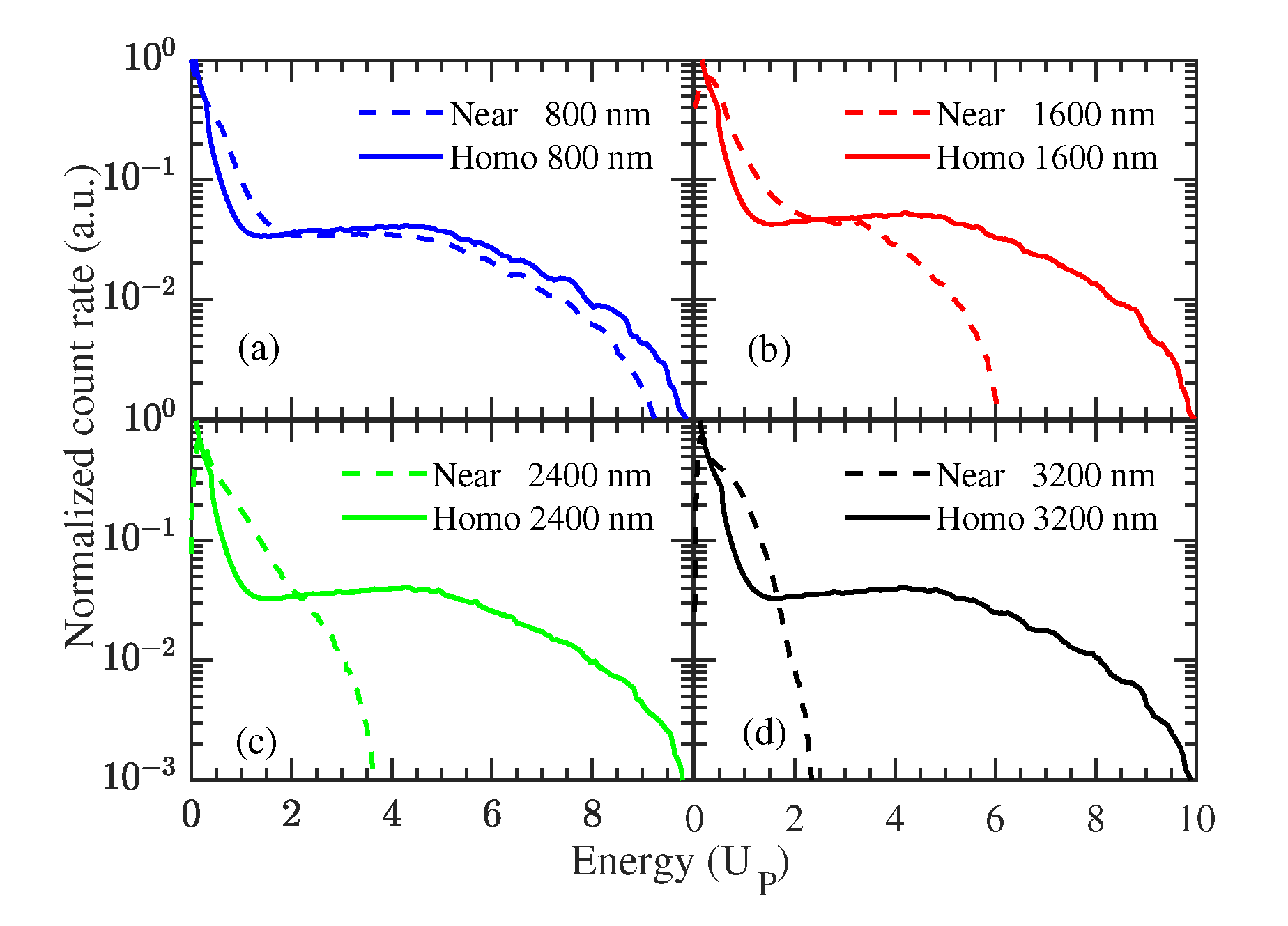
3.1. The Wavelength-Dependent Photoelectron Spectra
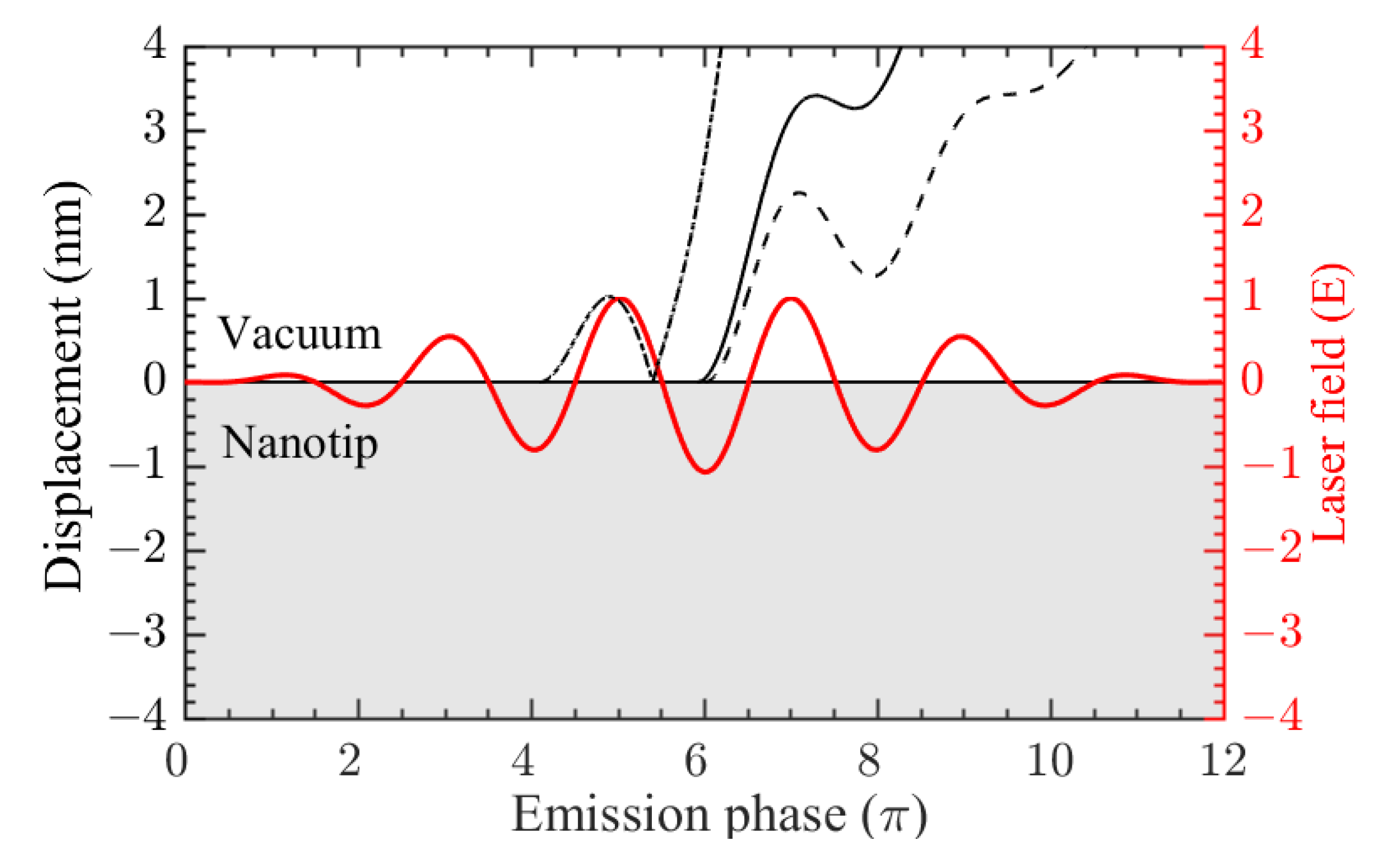
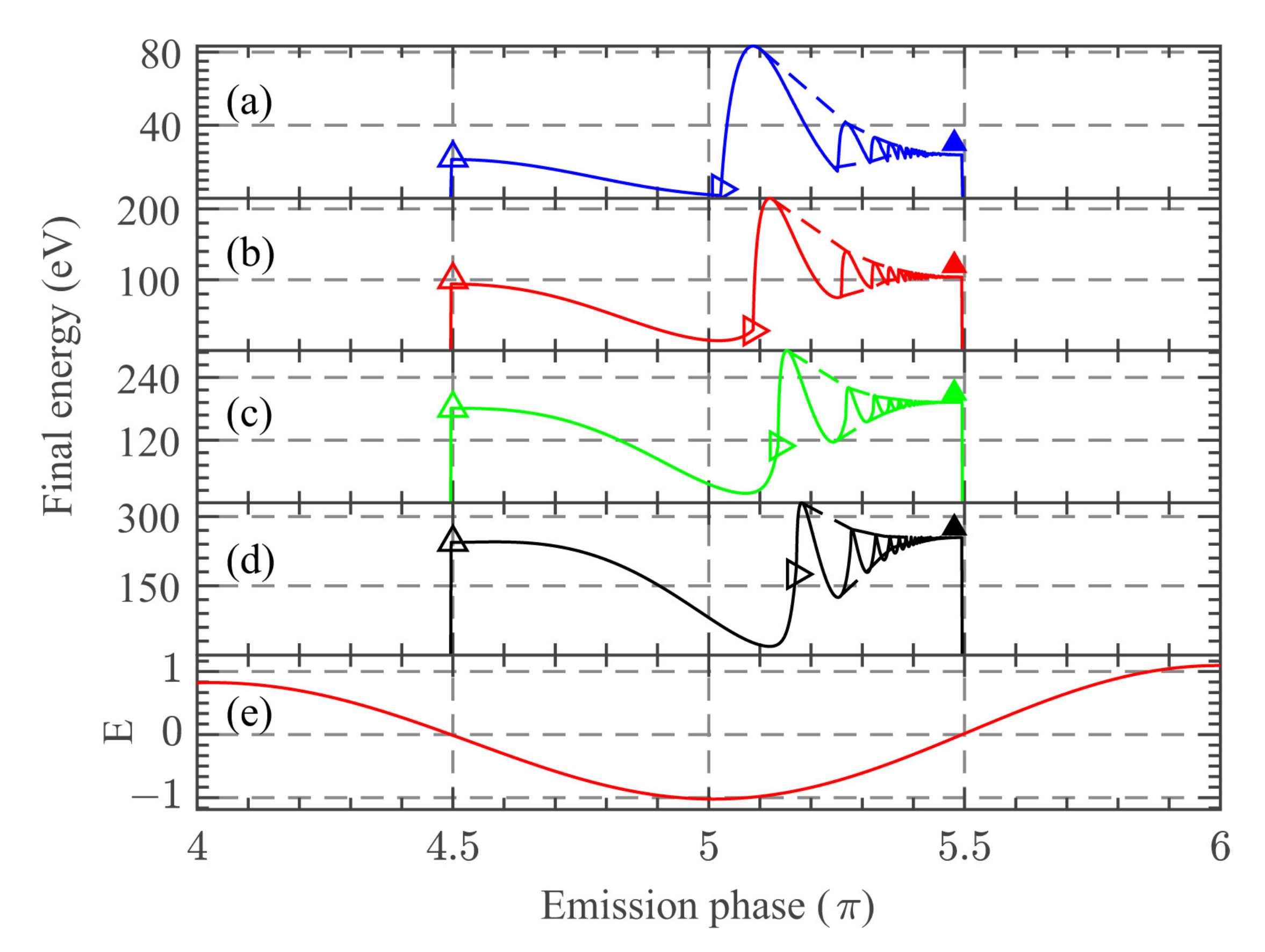
3.2. Regimes of Photoelectron Spectra from Nanotip Photoemission
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Keldysh, L. Ionization in the field of a strong electromagnetic wave. Sov. Phys. JETP 1965, 20, 1307–1314. [Google Scholar]
- Corkum, P.B. Plasma perspective on strong field multiphoton ionization. Phys. Rev. Lett. 1993, 71, 1994–1997. [Google Scholar] [CrossRef]
- Paulus, G.; Grasbon, F.; Walther, H.; Villoresi, P.; Nisoli, M.; Stagira, S.; Priori, E.; De Silvestri, S. Absolute-phase phenomena in photoionization with few-cycle laser pulses. Nature 2001, 414, 182. [Google Scholar] [CrossRef]
- Krause, J.L.; Schafer, K.J.; Kulander, K.C. High-order harmonic generation from atoms and ions in the high intensity regime. Phys. Rev. Lett. 1992, 68, 3535–3538. [Google Scholar] [CrossRef] [PubMed]
- Agostini, P.; Fabre, F.; Mainfray, G.; Petite, G.; Rahman, N.K. Free-Free Transitions Following Six-Photon Ionization of Xenon Atoms. Phys. Rev. Lett. 1979, 42, 1127–1130. [Google Scholar] [CrossRef]
- Eberly, J.H.; Javanainen, J.; Rzazewski, K. Above-threshold ionization. Phys. Rep. 1991, 204, 331–383. [Google Scholar] [CrossRef]
- Milošević, D.; Paulus, G.; Bauer, D.; Becker, W. Above-threshold ionization by few-cycle pulses. J. Phys. B 2006, 39, R203. [Google Scholar] [CrossRef]
- Walker, B.; Sheehy, B.; DiMauro, L.F.; Agostini, P.; Schafer, K.J.; Kulander, K.C. Precision Measurement of Strong Field Double Ionization of Helium. Phys. Rev. Lett. 1994, 73, 1227–1230. [Google Scholar] [CrossRef]
- Bergues, B.; Kübel, M.; Kling, N.G.; Burger, C.; Kling, M.F. Single-cycle non-sequential double ionization. IEEE J. Sel. Top. Quantum Electron. 2015, 21, 1–9. [Google Scholar] [CrossRef]
- Irvine, S.; Dechant, A.; Elezzabi, A. Generation of 0.4-keV femtosecond electron pulses using impulsively excited surface plasmons. Phys. Rev. Lett. 2004, 93, 184801. [Google Scholar] [CrossRef]
- Rácz, P.; Irvine, S.; Lenner, M.; Mitrofanov, A.; Baltuška, A.; Elezzabi, A.; Dombi, P. Strong-field plasmonic electron acceleration with few-cycle, phase-stabilized laser pulses. Appl. Phys. Lett. 2011, 98, 111116. [Google Scholar] [CrossRef]
- Bormann, R.; Gulde, M.; Weismann, A.; Yalunin, S.; Ropers, C. Tip-enhanced strong-field photoemission. Phys. Rev. Lett. 2010, 105, 147601. [Google Scholar] [CrossRef] [PubMed]
- Krüger, M.; Schenk, M.; Hommelhoff, P. Attosecond control of electrons emitted from a nanoscale metal tip. Nature 2011, 475, 78. [Google Scholar] [CrossRef] [PubMed]
- Schenk, M.; Krüger, M.; Hommelhoff, P. Strong-field above-threshold photoemission from sharp metal tips. Phys. Rev. Lett. 2010, 105, 257601. [Google Scholar] [CrossRef] [PubMed]
- Zherebtsov, S.; Fennel, T.; Plenge, J.; Antonsson, E.; Znakovskaya, I.; Wirth, A.; Herrwerth, O.; Süßmann, F.; Peltz, C.; Ahmad, I.; et al. Controlled near-field enhanced electron acceleration from dielectric nanospheres with intense few-cycle laser fields. Nat. Phys. 2011, 7, 656. [Google Scholar] [CrossRef]
- Kim, S.; Jin, J.; Kim, Y.J.; Park, I.Y.; Kim, Y.; Kim, S.W. High-harmonic generation by resonant plasmon field enhancement. Nature 2008, 453, 757. [Google Scholar] [CrossRef]
- Sivis, M.; Duwe, M.; Abel, B.; Ropers, C. Nanostructure-enhanced atomic line emission. Nature 2012, 485, E1. [Google Scholar] [CrossRef]
- Herink, G.; Solli, D.R.; Gulde, M.; Ropers, C. Field-driven photoemission from nanostructures quenches the quiver motion. Nature 2012, 483, 190–193. [Google Scholar] [CrossRef]
- Ciappina, M.F.; Pérez-Hernández, J.A.; Landsman, A.S.; Okell, W.A.; Zherebtsov, S.; Förg, B.; Schötz, J.; Seiffert, L.; Fennel, T.; Shaaran, T.; et al. Attosecond physics at the nanoscale. Rep. Prog. Phys. 2017, 80, 054401. [Google Scholar] [CrossRef]
- Krüger, M.; Schenk, M.; Förster, M.; Hommelhoff, P. Attosecond physics in photoemission from a metal nanotip. J. Phys. B 2012, 45, 074006. [Google Scholar] [CrossRef]
- Krüger, M.; Lemell, C.; Wachter, G.; Burgdörfer, J.; Hommelhoff, P. Attosecond physics phenomena at nanometric tips. J. Phys. B 2018, 51, 172001. [Google Scholar] [CrossRef]
- Wood, R.W. A new form of cathode discharge and the production of X-rays, together with some notes on diffraction. Preliminary communication. Phys. Rev. 1897, 5, 1. [Google Scholar] [CrossRef]
- Novotny, L.; Hecht, B. Principles of Nano-Optics; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar]
- Novotny, L.; Van Hulst, N. Antennas for light. Nat. Photonics 2011, 5, 83. [Google Scholar] [CrossRef]
- Martin, Y.C.; Hamann, H.F.; Wickramasinghe, H.K. Strength of the electric field in apertureless near-field optical microscopy. J. Appl. Phys. 2001, 89, 5774–5778. [Google Scholar] [CrossRef]
- Thomas, S.; Krüger, M.; Förster, M.; Schenk, M.; Hommelhoff, P. Probing of optical near-fields by electron rescattering on the 1 nm scale. Nano Lett. 2013, 13, 4790–4794. [Google Scholar] [CrossRef] [PubMed]
- Sarid, D.; Challener, W.A. Modern Introduction to Surface Plasmons: Theory, Mathematica Modeling, and Applications; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Paulus, G.G.; Lindner, F.; Walther, H.; Baltuška, A.; Goulielmakis, E.; Lezius, M.; Krausz, F. Measurement of the phase of few-cycle laser pulses. Phys. Rev. Lett. 2003, 91, 253004. [Google Scholar] [CrossRef] [PubMed]
- Haworth, C.; Chipperfield, L.; Robinson, J.; Knight, P.; Marangos, J.; Tisch, J. Half-cycle cutoffs in harmonic spectra and robust carrier-envelope phase retrieval. Nat. Phys. 2007, 3, 52. [Google Scholar] [CrossRef]
- Ishii, N.; Kaneshima, K.; Kitano, K.; Kanai, T.; Watanabe, S.; Itatani, J. Carrier-envelope phase-dependent high harmonic generation in the water window using few-cycle infrared pulses. Nat. Commun. 2014, 5, 3331. [Google Scholar] [CrossRef]
- Xiong, W.H.; Geng, J.W.; Gong, Q.; Peng, L.Y. Half-cycle cutoff in near-threshold harmonic generation. New J. Phys. 2015, 17, 123020. [Google Scholar] [CrossRef]
- Piglosiewicz, B.; Schmidt, S.; Park, D.J.; Vogelsang, J.; Groß, P.; Manzoni, C.; Farinello, P.; Cerullo, G.; Lienau, C. Carrier-envelope phase effects on the strong-field photoemission of electrons from metallic nanostructures. Nat. Photonics 2014, 8, 37. [Google Scholar] [CrossRef]
- Echternkamp, K.; Herink, G.; Yalunin, S.V.; Rademann, K.; Schäfer, S.; Ropers, C. Strong-field photoemission in nanotip near-fields: From quiver to sub-cycle electron dynamics. Appl. Phys. B 2016, 122, 80. [Google Scholar] [CrossRef]
- Wachter, G.; Lemell, C.; Burgdörfer, J.; Schenk, M.; Krüger, M.; Hommelhoff, P. Electron rescattering at metal nanotips induced by ultrashort laser pulses. Phys. Rev. B 2012, 86, 035402. [Google Scholar] [CrossRef]
- Paulus, G.G.; Becker, W.; Nicklich, W.; Walther, H. Rescattering effects in above-threshold ionization: A classical model. J. Phys. B At. Mol. Opt. Phys. 1994, 27, L703. [Google Scholar] [CrossRef]
- Krausz, F.; Ivanov, M. Attosecond physics. Rev. Mod. Phys. 2009, 81, 163. [Google Scholar] [CrossRef]
- Jackson, J.D. Classical Electrodynamics; John Wiley & Sons Inc.: New York, NY, USA, 1999; Volume 13. [Google Scholar]
- Süßmann, F.; Seiffert, L.; Zherebtsov, S.; Mondes, V.; Stierle, J.; Arbeiter, M.; Plenge, J.; Rupp, P.; Peltz, C.; Kessel, A.; et al. Field propagation-induced directionality of carrier-envelope phase-controlled photoemission from nanospheres. Nat. Commun. 2015, 6, 1–9. [Google Scholar] [CrossRef]
- Yanagisawa, H.; Schnepp, S.; Hafner, C.; Hengsberger, M.; Kim, D.E.; Kling, M.F.; Landsman, A.; Gallmann, L.; Osterwalder, J. Delayed electron emission in strong-field driven tunnelling from a metallic nanotip in the multi-electron regime. Sci. Rep. 2016, 6, 35877. [Google Scholar] [CrossRef]
- Hommelhoff, P.; Kealhofer, C.; Kasevich, M.A. Ultrafast electron pulses from a tungsten tip triggered by low-power femtosecond laser pulses. Phys. Rev. Lett. 2006, 97, 247402. [Google Scholar] [CrossRef]
- Lang, N.D.; Kohn, W. Theory of Metal Surfaces: Induced Surface Charge and Image Potential. Phys. Rev. B 1973, 7, 3541–3550. [Google Scholar] [CrossRef]
- Kang, D.; Won, E. Precise numerical solutions of potential problems using the Crank–Nicolson method. J. Comput. Phys. 2008, 227, 2970–2976. [Google Scholar] [CrossRef]
- Bauer, D.; Koval, P. Qprop: A Schrödinger-solver for intense laser–atom interaction. Comput. Phys. Commun. 2006, 174, 396–421. [Google Scholar] [CrossRef]
- Kuchiev, M.Y. Atomic antenna. JETP Lett. 1987, 45, 404–406. [Google Scholar]
- Gallagher, T.F. Above-Threshold Ionization in Low-Frequency Limit. Phys. Rev. Lett. 1988, 61, 2304–2307. [Google Scholar] [CrossRef] [PubMed]
- Ammosov, M.; Delone, N.; Krainov, V.; Perelomov, A.; Popov, V.; Terent’ev, M.; Yudin, G.L.; Ivanov, M.Y. Tunnel ionization of complex atoms and of atomic ions in an alternating electric field. Sov. Phys. JETP 1986, 64, 26. [Google Scholar]
- Fowler, R.H.; Nordheim, L. Electron emission in intense electric fields. Proc. R. Soc. Lond. A 1928, 119, 173–181. [Google Scholar]
- Forbes, R.G. Simple good approximations for the special elliptic functions in standard Fowler-Nordheim tunneling theory for a Schottky-Nordheim barrier. Appl. Phys. Lett. 2006, 89, 113122. [Google Scholar] [CrossRef]
- Babar, S.; Weaver, J. Optical constants of Cu, Ag, and Au revisited. Appl. Opt. 2015, 54, 477–481. [Google Scholar] [CrossRef]
- Gibson, G.; Freeman, R.; McIlrath, T.; Muller, H. Excitation and ionization dynamics in short-pulse multiphoton ionization. Phys. Rev. A 1994, 49, 3870. [Google Scholar] [CrossRef]
- Hansch, P.; Walker, M.; Van Woerkom, L. Spatially dependent multiphoton multiple ionization. Phys. Rev. A 1996, 54, R2559. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, X.-Y.; Liang, H.; Ciappina, M.F.; Peng, L.-Y. Wavelength-Dependent Features of Photoelectron Spectra from Nanotip Photoemission. Photonics 2020, 7, 129. https://doi.org/10.3390/photonics7040129
Wu X-Y, Liang H, Ciappina MF, Peng L-Y. Wavelength-Dependent Features of Photoelectron Spectra from Nanotip Photoemission. Photonics. 2020; 7(4):129. https://doi.org/10.3390/photonics7040129
Chicago/Turabian StyleWu, Xiao-Yuan, Hao Liang, Marcelo F. Ciappina, and Liang-You Peng. 2020. "Wavelength-Dependent Features of Photoelectron Spectra from Nanotip Photoemission" Photonics 7, no. 4: 129. https://doi.org/10.3390/photonics7040129
APA StyleWu, X.-Y., Liang, H., Ciappina, M. F., & Peng, L.-Y. (2020). Wavelength-Dependent Features of Photoelectron Spectra from Nanotip Photoemission. Photonics, 7(4), 129. https://doi.org/10.3390/photonics7040129