Subnanometer-Resolution Nanoparticle Sensing through the Strong Coupling between Surface Plasmon Polariton Whispering Gallery Resonances and Localized Surface Plasmon
Abstract
1. Introduction
2. Methods and Theory
3. Results and Discussion
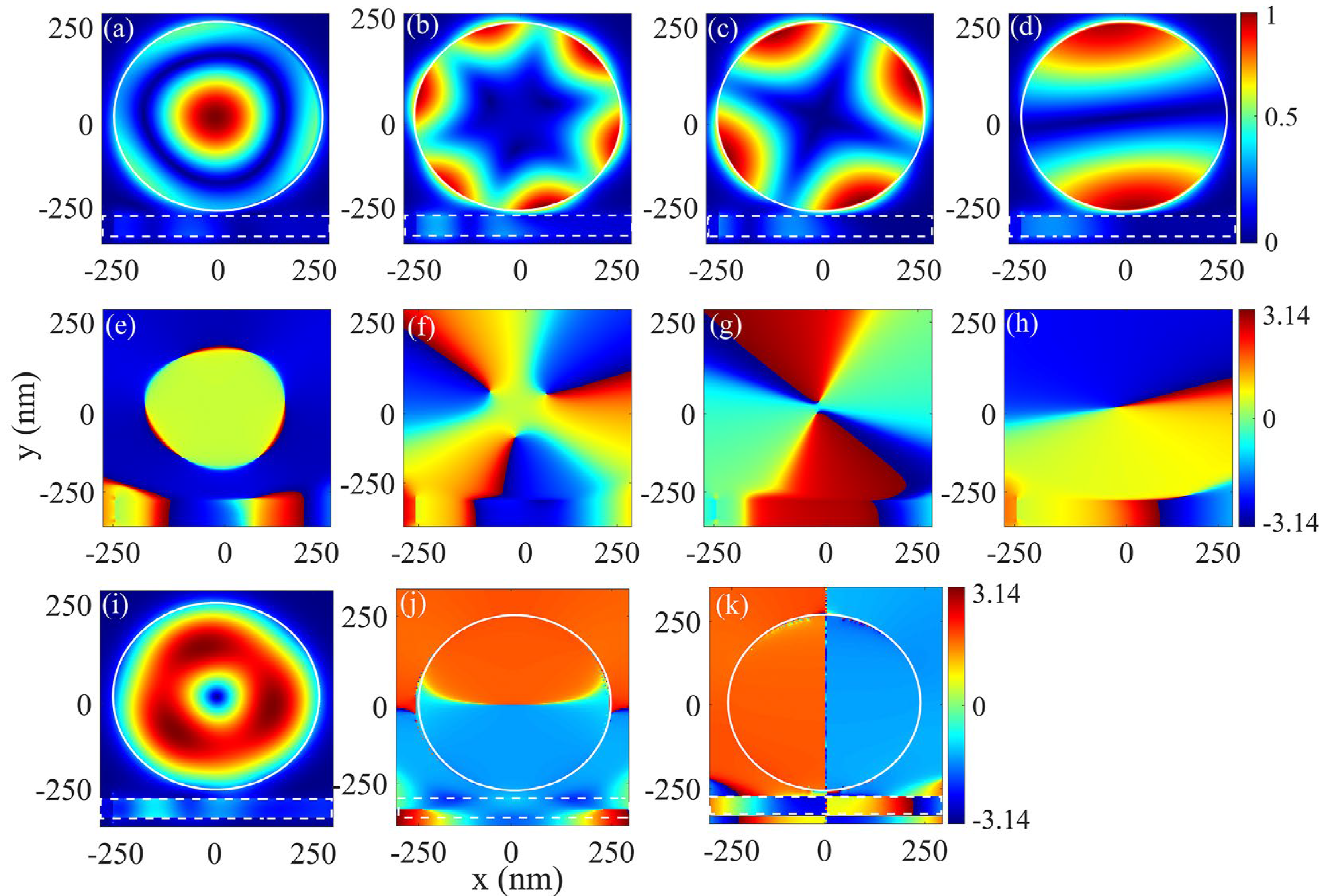
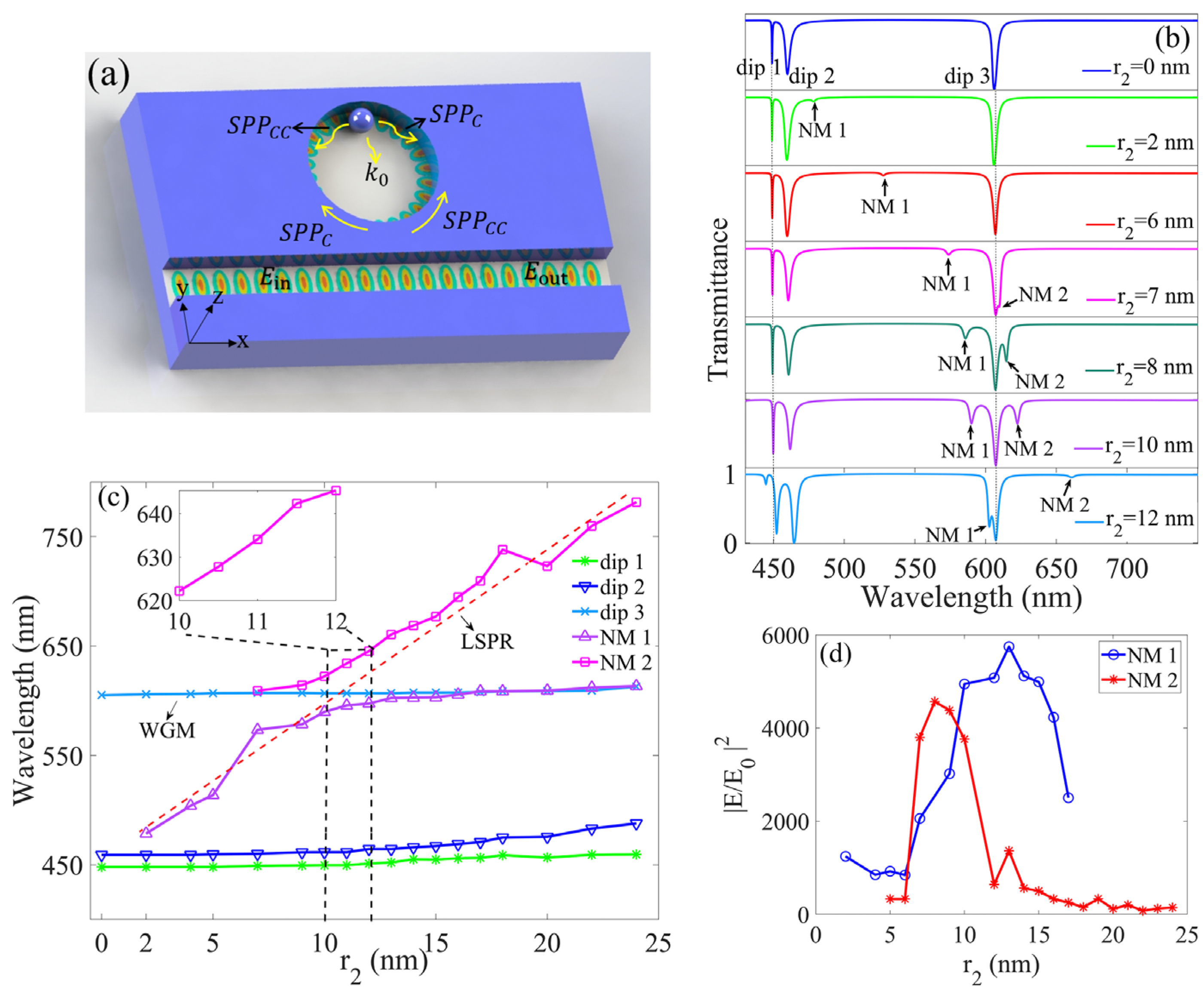
3.1. Strong Coupling between SPP WGM and LSPR
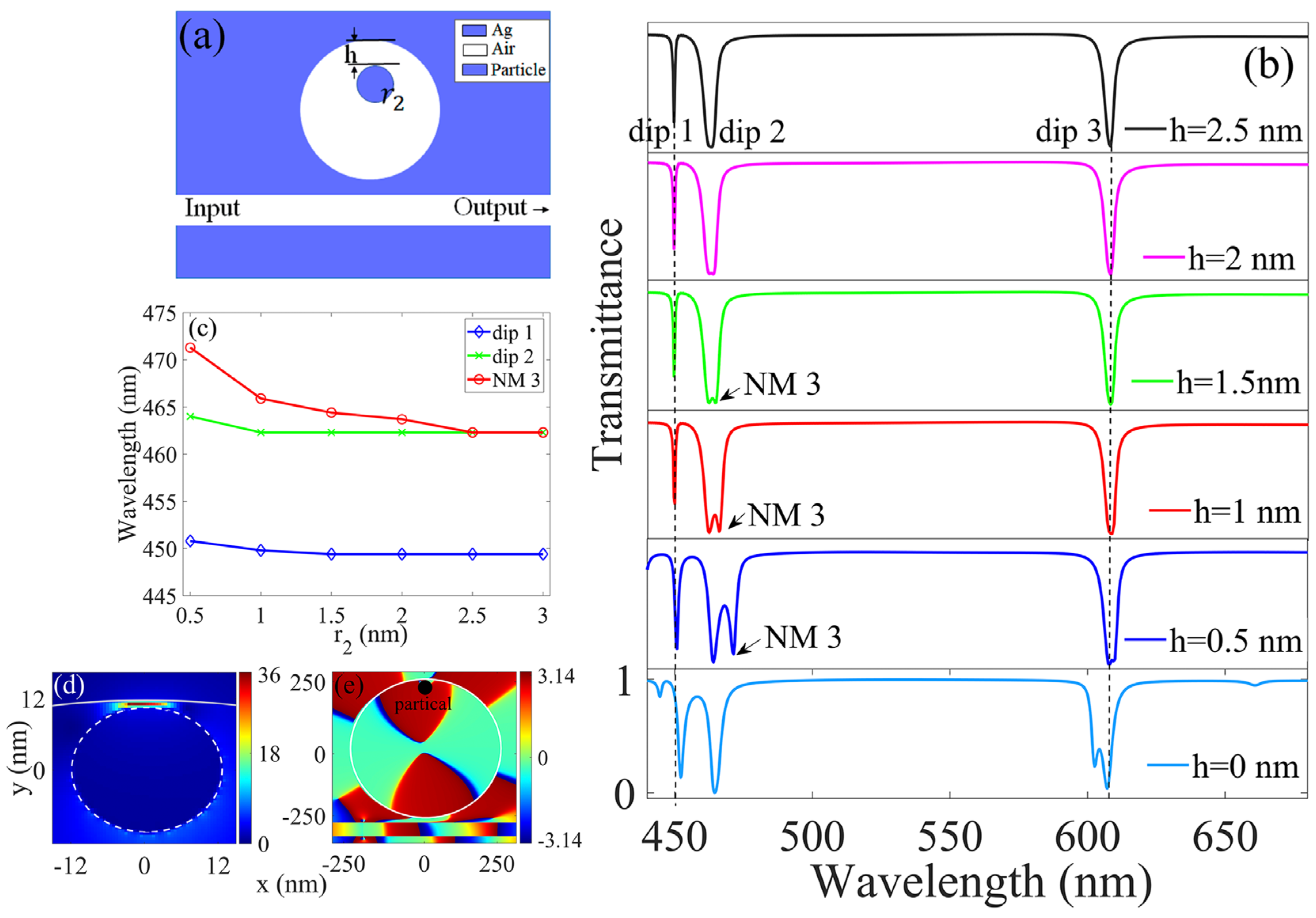
3.2. Sensing Properties of the Coupling between LSPR and SPP WGM
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Oraevsky, N. Whispering-gallery waves. Quantum Electron. 2002, 32, 377–400. [Google Scholar] [CrossRef]
- Vahala, K.J. Optical microcavities. Nature 2003, 424, 839–846. [Google Scholar] [CrossRef] [PubMed]
- Schweiger, G.; Weigel, T.; Ostendorf, A. Geometrical-optics analysis of whispering-gallery modes in the layer of a coated spherical resonator. Phys. Rev. A 2020, 102, 053506. [Google Scholar] [CrossRef]
- Fang, Z.; Haque, S.; Farajollahi, S.; Luo, H.; Lin, J.; Wu, R.; Zhang, J.; Wang, Z.; Wang, M.; Cheng, Y.; et al. Polygon coherent modes in a weakly perturbed whispering gallery microresonator for efficient second harmonic, optomechanical, and frequency comb generations. Phys. Rev. Lett. 2020, 125, 173901. [Google Scholar] [CrossRef]
- Xie, R.R.; Qin, G.Q.; Zhang, H.; Wang, M.; Li, G.Q.; Ruan, D.; Long, G.L. Phase-controlled dual-wavelength resonance in a self-coupling whispering-gallery-mode microcavity. Opt. Express 2021, 46, 773–776. [Google Scholar] [CrossRef]
- Yi, X.; Xiao, Y.F.; Liu, Y.C.; Li, B.B.; Chen, Y.L.; Li, Y.; Gong, Q. Multiple-rayleigh-scatterer-induced mode splitting in a high-Q whispering-gallery-mode microresonator. Phys. Rev. A 2011, 83, 23803. [Google Scholar] [CrossRef]
- Ghalanos, G.N.; Silver, J.M.; Bino, L.D.; Moroney, N.; Zhang, S.; Woodley, M.T.M.; Svela, A.; Del’Haye, P. Kerr-nonlinearity-induced mode-splitting in optical microresonators. Phys. Rev. Lett. 2020, 124, 223901. [Google Scholar] [CrossRef]
- Zheng, Y.; Fang, Z.; Liu, S.; Cheng, Y.; Chen, X. High-Q exterior whispering-gallery dodes in a double-layer crystalline microdisk resonator. Phys. Rev. Lett. 2019, 122, 253902. [Google Scholar] [CrossRef]
- Zhi, Y.; Yu, X.C.; Gong, Q.; Yang, L.; Xiao, Y.F. Single nanoparticle detection using optical microcavities. Adv. Mater. 2017, 29, 1604920. [Google Scholar] [CrossRef]
- Zhu, J.; Ozdemir, S.K.; Xiao, Y.F.; Li, L.; He, L.; Chen, D.R.; Yang, L. On-chip single nanoparticle detection and sizing by mode splitting in an ultrahigh-Q microresonator. Nat. Photonics 2010, 4, 46. [Google Scholar] [CrossRef]
- Cai, H.; Poon, A.W. Optical manipulation of microparticles using whispering-gallery modes in a silicon nitride microdisk resonator. Opt. Lett. 2011, 36, 4257–4259. [Google Scholar] [CrossRef]
- Kippenberg, T.J.; Spillane, S.M.; Vahala, K.J. Modal coupling in traveling-wave resonators. Opt. Lett. 2002, 27, 1669–1671. [Google Scholar] [CrossRef]
- Ge, K.; Ruan, J.; Cui, L.B.; Guo, D.; Tong, J.H.; Zhai, T.R. Dynamic manipulation of WGM lasing by tailoring the coupling strength. Opt. Express 2022, 30, 28752–28761. [Google Scholar] [CrossRef] [PubMed]
- Guendelman, G.; Lovsky, Y.; Yacoby, E.; Mor, O.E.; Kaplan-Ashiri, I.; Goldbart, O.; Dayan, B. Three-dimensional sensing of arbitrarily shaped nanoparticles by whispering gallery mode resonators. Opt. Express 2020, 28, 31297–31315. [Google Scholar] [CrossRef]
- Shangguan, Q.; Chen, Z.; Yang, H.; Cheng, S.; Yang, W.; Yi, Z.; Wu, X.; Wang, S.; Yi, Y.; Wu, P. Design of Ultra-Narrow Band Graphene Refractive Index Sensor. Sensors 2022, 22, 6483. [Google Scholar] [CrossRef]
- Saglamyurek, E.; Hrushevskyi, T.; Rastogi, A.; Heshami, K.; Leblanc, L.J. Coherent storage and manipulation of broadband photons via dynamically controlled Autler-Townes splitting. Nat. Photonics 2018, 12, 774. [Google Scholar] [CrossRef]
- Daugey, T.; Billet, C.; Dudley, J.; Merolla, J.M.; Chembo, Y.K. Kerr optical frequency comb generation using whispering-gallery-mode resonators in the pulsed-pump regime. Phys. Rev. A 2021, 103, 023521. [Google Scholar] [CrossRef]
- Kippenberg, T.J.; Spillane, S.M.; Vahala, K.J. Kerr-nonlinearity optical parametric oscillation in an ultrahigh-Q toroid microcavity. Phys. Rev. Lett. 2004, 93, 083904. [Google Scholar] [CrossRef] [PubMed]
- Zakowicz, W. Whispering-Gallery-Mode Resonances: A New Way to Accelerate Charged Particles. Phys. Rev. Lett. 2005, 95, 114801. [Google Scholar] [CrossRef]
- Kuttge, M.; García de Abajo, F.J.; Polman, A. Ultrasmall mode volume plasmonic nanodisk resonators. Nano Lett. 2010, 10, 1537–1541. [Google Scholar] [CrossRef]
- Kwon, S.H. Deep subwavelength plasmonic whispering gallery-mode cavity. Opt. Express 2012, 20, 24918–24924. [Google Scholar] [CrossRef]
- Lou, F.; Yan, M.; Thylen, L.; Qiu, M.; Wosinski, L. Whispering gallery mode nanodisk resonator based on layered metal-dielectric waveguide. Opt. Express 2014, 22, 8490–8502. [Google Scholar] [CrossRef]
- Zhao, J.; Liu, X.; Qiu, W.; Ma, Y.; Huang, Y.; Wang, J.X.; Qiang, K.; Pan, J.Q. Surface-plasmon-polariton whispering-gallery mode analysis of the graphene monolayer coated InGaAs nanowire cavity. Opt. Express 2014, 22, 5754–5761. [Google Scholar] [CrossRef]
- Min, B.; Ostby, E.; Sorger, V.; Ulin-Avila, E.; Lan, Y.; Zhang, X.; Vahala, K. High-Q surface-plasmon-polariton whispering-gallery microcavity. Nature 2009, 457, 455–458. [Google Scholar] [CrossRef]
- Li, B.B.; Clements, W.R.; Yu, X.C.; Shi, K.; Gong, Q.; Xiao, Y.F. Single nanoparticle detection using split-mode microcavity Raman lasers. Proc. Natl. Acad. Sci. USA 2014, 111, 14657–14662. [Google Scholar] [CrossRef]
- Özdemir, Ş.K.; Zhu, J.; Yang, X.; Peng, B.; Yilmaz, H.; He, L.; Faraz, M.; Huang, S.H.; Long, L.L.; Yang, L. Highly sensitive detection of nanoparticles with a self-referenced and self-heterodyned whispering-gallery Raman microlaser. Proc. Natl. Acad. Sci. USA 2014, 111, 3836–3844. [Google Scholar] [CrossRef] [PubMed]
- Özdemir, Ş.K.; He, L.; Zhu, J.; Monifi, F.; Kim, W.; Kenechukwu, O.; Yilmaz, H.; Huang, S.H.; Yang, L. On-chip whispering-gallery-mode microlasers and their applications for nanoparticle sensing. Integr. Opt. Devices Mater. Technol. XVII SPIE 2013, 8627, 132–141. [Google Scholar]
- Miroshnichenko, A.E.; Flach, S.; Kivshar, Y. Fano resonances in nanoscale structures. Rev. Mod. Phys. 2010, 82, 2257. [Google Scholar] [CrossRef]
- Fang, Y.H.; Wen, K.H.; Li, Z.F.; Wu, B.Y.; Chen, L.; Zhou, J.Y.; Zhou, D.Y. Multiple Fano resonances based on end-coupled semi-ring rectangular resonator. IEEE Photonics J. 2019, 11, 4801308. [Google Scholar] [CrossRef]
- Khan, A.D.; Miano, G. Plasmonic Fano resonances in single-layer gold conical nanoshells. Plasmonics 2013, 8, 1429–1437. [Google Scholar] [CrossRef]
- Liu, H.X.; Chen, Y.G. Localized surface plasmon induced transparency and spatial resolution enhancement of nanosensors. Phys. Scr. 2021, 96, 125622. [Google Scholar] [CrossRef]
- Zhu, Z.H.; Wang, B.Y.; Yan, X.; Liu, Y.; Zeng, Q.D.; Wang, T.; Yu, H.Q. Dynamically tunable multiband plasmon-induced transparency effect based on graphene nanoribbon waveguide coupled with rectangle cavities system. Chin. Phys. B 2022, 31, 84210. [Google Scholar] [CrossRef]
- Chen, H.; Zhang, Z.; Zhang, X.; Han, Y.; Zhou, Z.; Yang, J. Multifunctional plasmon-induced transparency devices based on hybrid metamaterial-waveguide systems. Nanomaterials 2022, 12, 3273. [Google Scholar] [CrossRef]
- Zhou, T.; Gou, X.Y.; Xu, W.; Li, Y.; Zhai, X.; Li, H.J.; Wang, L.L. Dynamically tunable plasmon-induced transparency in a T-shaped cavity waveguide based on bulk Dirac semimetals. Plasmonics 2021, 16, 323–332. [Google Scholar] [CrossRef]
- Wu, X.; Zheng, Y.; Luo, Y.; Zhang, J.; Yi, Z.; Wu, X.; Cheng, S.; Yang, W.; Yu, Y.; Wu, P. A four-band and polarization-independent BDS-based tunable absorber with high refractive index sensitivity. Phys. Chem. Chem. Phys. 2021, 23, 26864–26873. [Google Scholar] [CrossRef] [PubMed]
- Du, X.J.; Yang, Z.J.; Hu, M.L.; Ma, L.; He, J. Ultrahigh electric and magnetic near field enhancement based on high-Q whispering gallery modes in subwavelength all-dielectric resonators. Appl. Phys. Express 2021, 14, 082004. [Google Scholar] [CrossRef]
- Shi, Y.; Dong, Y.; Sun, D.; Li, G. Significant Near-Field Enhancement over Large Volumes around Metal Nanorods via Strong Coupling of Surface Lattice Resonances and Fabry–Pérot Resonance. Materials 2022, 15, 1523. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, H.; Chen, Y.-G. Subnanometer-Resolution Nanoparticle Sensing through the Strong Coupling between Surface Plasmon Polariton Whispering Gallery Resonances and Localized Surface Plasmon. Photonics 2023, 10, 212. https://doi.org/10.3390/photonics10020212
Yang H, Chen Y-G. Subnanometer-Resolution Nanoparticle Sensing through the Strong Coupling between Surface Plasmon Polariton Whispering Gallery Resonances and Localized Surface Plasmon. Photonics. 2023; 10(2):212. https://doi.org/10.3390/photonics10020212
Chicago/Turabian StyleYang, Han, and Yue-Gang Chen. 2023. "Subnanometer-Resolution Nanoparticle Sensing through the Strong Coupling between Surface Plasmon Polariton Whispering Gallery Resonances and Localized Surface Plasmon" Photonics 10, no. 2: 212. https://doi.org/10.3390/photonics10020212
APA StyleYang, H., & Chen, Y.-G. (2023). Subnanometer-Resolution Nanoparticle Sensing through the Strong Coupling between Surface Plasmon Polariton Whispering Gallery Resonances and Localized Surface Plasmon. Photonics, 10(2), 212. https://doi.org/10.3390/photonics10020212



