Individual Tuning of Directional Emission and Luminance of a Quantum Emitter in a Composite Plasmonic Antenna
Abstract
1. Introduction
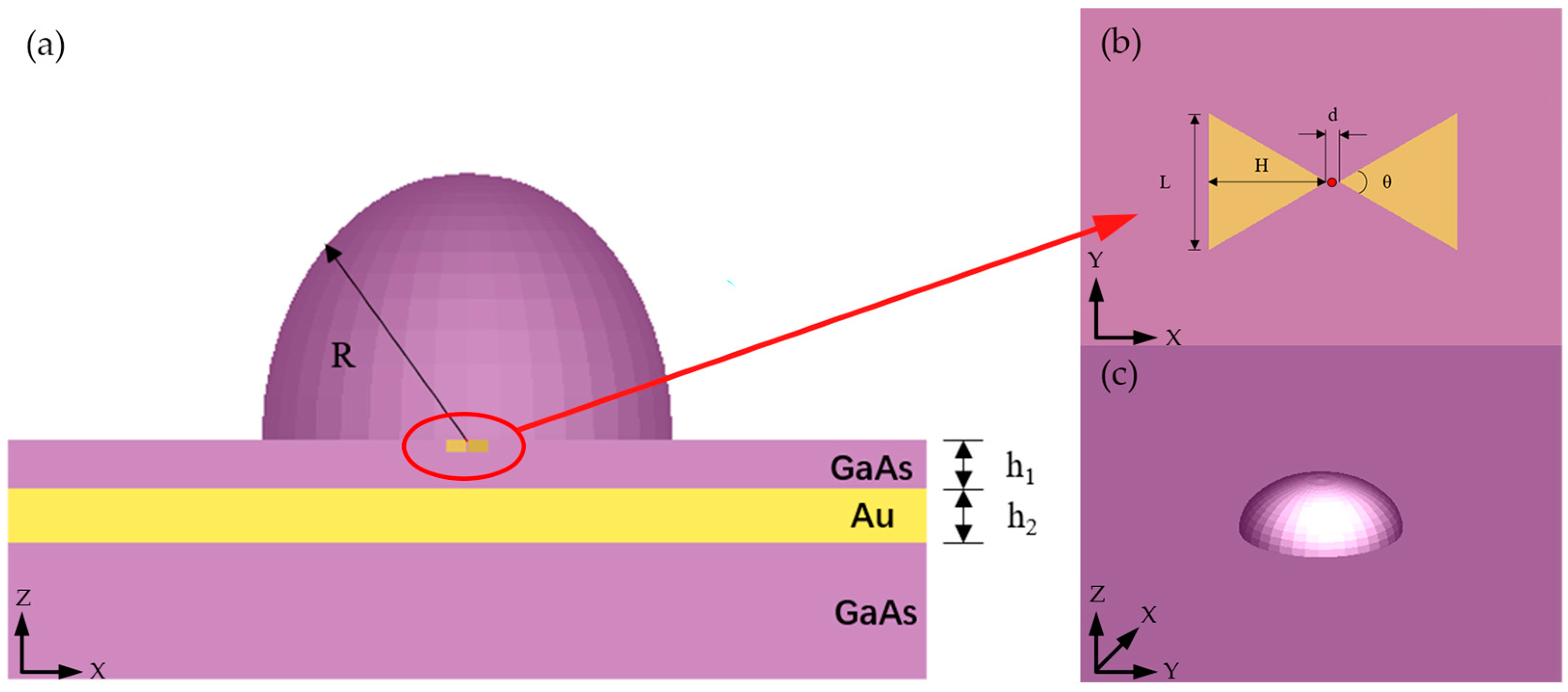
2. Structure Description
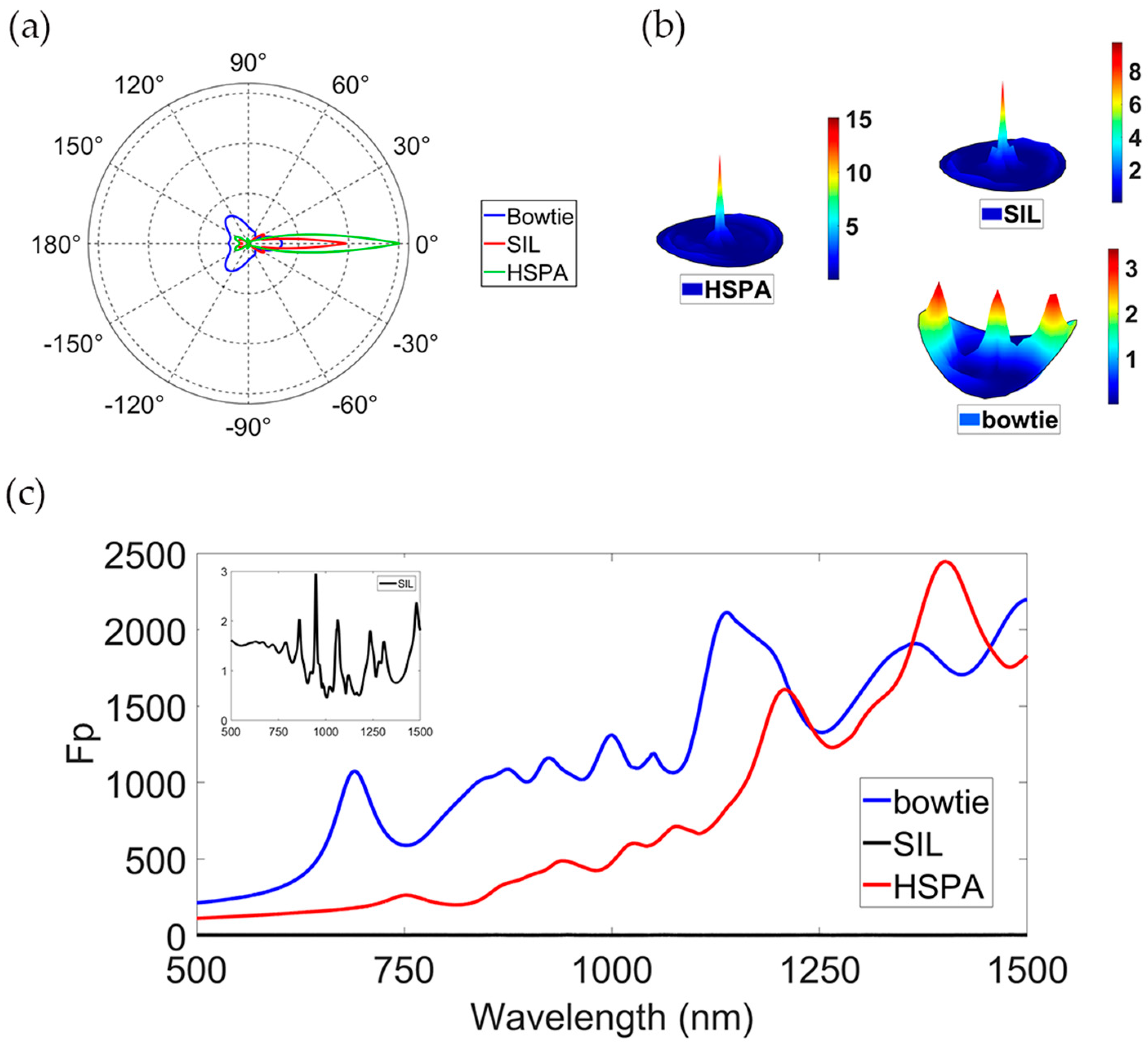
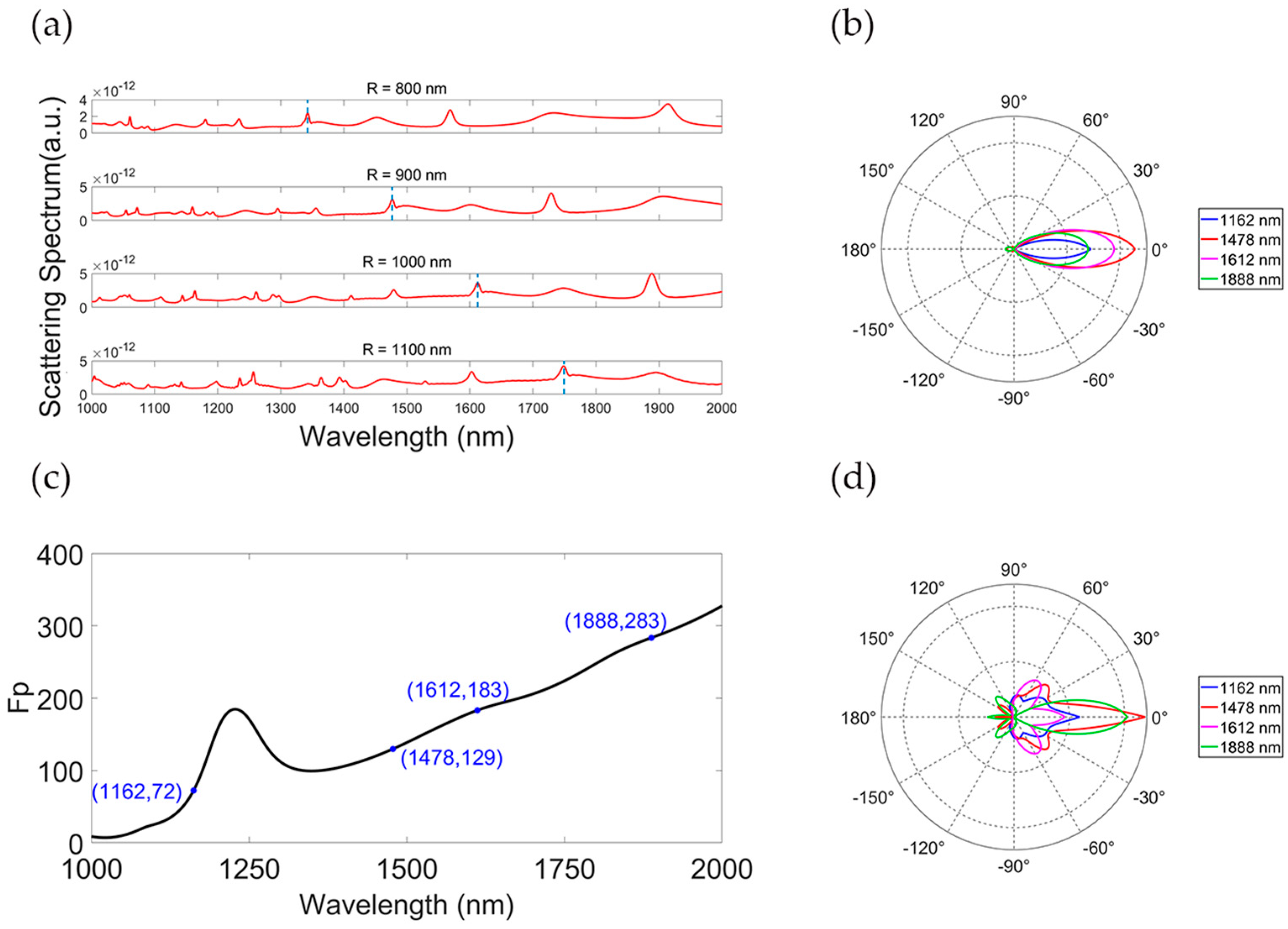
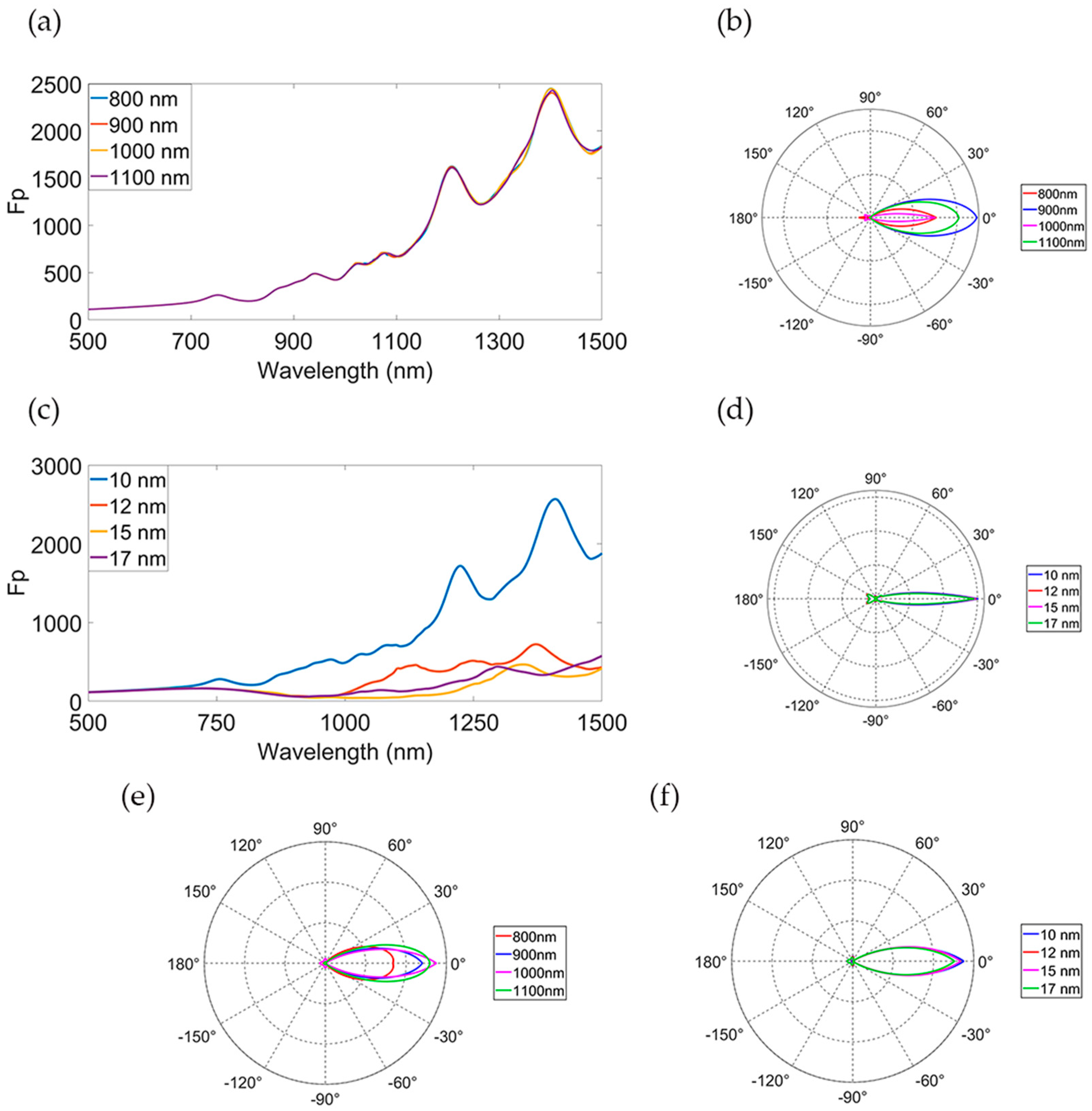
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Romeira, B.; Figueiredo, J.M.; Javaloyes, J. NanoLEDs for energy-efficient and gigahertz-speed spike-based sub-λ neuromorphic nanophotonic computing. Nanophotonics 2020, 9, 4149–4162. [Google Scholar] [CrossRef]
- Ali, A.; Qasem, Z.A.; Li, Y.; Li, Q.; Fu, H.Y. All-inorganic liquid phase quantum dots and blue laser diode-based white-light source for simultaneous high-speed visible light communication and high-efficiency solid-state lighting. Opt. Express 2022, 30, 35112–35124. [Google Scholar] [CrossRef] [PubMed]
- Ren, A.; Wang, H.; Zhang, W.; Wu, J.; Wang, Z.; Penty, R.V.; White, I.H. Emerging light-emitting diodes for next-generation data communications. Nat. Electron. 2021, 4, 559–572. [Google Scholar] [CrossRef]
- Zeng, H.Z.; Ngyuen, M.A.; Ai, X.; Bennet, A.; Solntsev, A.S.; Laucht, A.; Al-Juboori, A.; Toth, M.; Mildren, R.P.; Malaney, R.; et al. Integrated room temperature single-photon source for quantum key distribution. Opt. Lett. 2022, 47, 1673–1676. [Google Scholar] [CrossRef] [PubMed]
- Somaschi, N.; Giesz, V.; De Santis, L.; Loredo, J.C.; Almeida, M.P.; Hornecker, G.; Portalupi, S.L.; Grange, T.; Anton, C.; Demory, J.; et al. Near-optimal single-photon sources in the solid state. Nat. Photonics 2016, 10, 340–345. [Google Scholar] [CrossRef]
- Zhong, H.S.; Wang, H.; Deng, Y.H.; Chen, M.C.; Peng, L.C.; Luo, Y.H.; Qin, J.; Wu, D.; Ding, X.; Hu, Y.; et al. Quantum computational advantage using photons. Science 2020, 370, 1460–1463. [Google Scholar] [CrossRef]
- Russell, K.J.; Liu, T.L.; Cui, S.; Hu, E.L. Large spontaneous emission enhancement in plasmonic nanocavities. Nat. Photonics 2012, 6, 459–462. [Google Scholar] [CrossRef]
- Hochrainer, A.; Lahiri, M.; Erhard, M.; Krenn, M.; Zeilinger, A. Quantum indistinguishability by path identity and with undetected photons. Rev. Mod. Phys. 2022, 94, 025007. [Google Scholar] [CrossRef]
- Yang, S.; Wang, Y.; Sun, H. Advances and prospects for whispering gallery mode microcavities. Adv. Opt. Mater. 2015, 3, 1136–1162. [Google Scholar] [CrossRef]
- Chiasera, A.; Dumeige, Y.; Feron, P.; Ferrari, M.; Jestin, Y.; Nunzi Conti, G.; Pelli, S.; Soria, S.; Righini, G.C. Spherical whispering-gallery-mode microresonators. Laser Photonics Rev. 2010, 4, 457–482. [Google Scholar] [CrossRef]
- Cai, Z.; Li, Z.; Ravaine, S.; He, M.; Song, Y.; Yin, Y.; Zheng, H.; Teng, J.; Zhang, A.O. From colloidal particles to photonic crystals: Advances in self-assembly and their emerging applications. Chem. Soc. Rev. 2021, 50, 5898–5951. [Google Scholar] [CrossRef] [PubMed]
- Jiang, Q.; Roy, P.; Claude, J.B.; Wenger, J. Single photon source from a nanoantenna-trapped single quantum dot. Nano Lett. 2021, 21, 7030–7036. [Google Scholar] [CrossRef] [PubMed]
- Abudayyeh, H.; Lubotzky, B.; Blake, A.; Wang, J.; Majumder, S.; Hu, Z.; Kim, Y.; Htoon, H.; Bose, R.; Malko, A.V.; et al. Single photon sources with near unity collection efficiencies by deterministic placement of quantum dots in nanoantennas. APL Photonics 2021, 6, 036109. [Google Scholar] [CrossRef]
- Shen, L.; Lin, X.; Shalaginov, M.Y.; Low, T.; Zhang, X.; Zhang, B.; Chen, H. Broadband enhancement of on-chip single-photon extraction via tilted hyperbolic metamaterials. Appl. Phys. Rev. 2020, 7, 021403. [Google Scholar] [CrossRef]
- Bai, Y.; Liu, S. A novel dual-beam terahertz leaky-wave antenna based on spoof surface plasmon waveguide. Optoelectron. Lett. 2022, 7, 404–407. [Google Scholar] [CrossRef]
- Roy, P.; Zhu, S.; Claude, J.B.; Liu, J.; Wenger, J. Ultraviolet Resonant Nanogap Antennas with Rhodium Nanocube Dimers for Enhancing Protein Intrinsic Autofluorescence. ACS Nano 2023, 17, 22418–22429. [Google Scholar] [CrossRef] [PubMed]
- Yang, G.; Shen, Q.; Niu, Y.; Wei, H.; Bai, B.; Mikkelsen, M.H.; Sun, H.B. Unidirectional, ultrafast, and bright spontaneous emission source enabled by a hybrid plasmonic nanoantenna. Laser Photonics Rev. 2020, 14, 1900213. [Google Scholar] [CrossRef]
- Yang, J.; Kong, F.; Li, K.; Zhao, J. Optimizing the bowtie nano-antenna for enhanced purcell factor and electric field. Prog. Electromagn. Res. Lett. 2014, 44, 93–99. [Google Scholar] [CrossRef]
- Carlson, C.; Hughes, S. Dissipative modes, Purcell factors, and directional beta factors in gold bowtie nanoantenna structures. Phys. Rev. B 2020, 102, 155301. [Google Scholar] [CrossRef]
- Qian, Z.; Li, Z.; Hao, H.; Shan, L.; Zhang, Q.; Dong, J.; Gong, Q.; Gu, Y. Absorption reduction of large purcell enhancement enabled by topological state-led mode coupling. Phys. Rev. Lett. 2021, 126, 023901. [Google Scholar] [CrossRef]
- Kountouris, G.; Mørk, J.; Denning, E.V.; Kristensen, P.T. Modal properties of dielectric bowtie cavities with deep sub-wavelength confinement. Opt. Express 2022, 30, 40367–40378. [Google Scholar] [CrossRef] [PubMed]
- Weisman, D.; Carmesin, C.M.; Rozenman, G.G.; Efremov, M.A.; Shemer, L.; Schleich, W.P.; Arie, A. Diffractive guiding of waves by a periodic array of slits. Phys. Rev. Lett. 2021, 127, 014303. [Google Scholar] [CrossRef] [PubMed]
- Sayed, M.; Yu, J.; Liu, G.; Jaroniec, M. Non-noble plasmonic metal-based photocatalysts. Chem. Rev. 2022, 122, 10484–10537. [Google Scholar] [CrossRef]
- Zain, H.A.; Batumalay, M.; Rahim, H.R.; Harun, S.W. Numerical analysis of a Kretschmann surface plasmon resonance sensor with silver/TiO2/BaTiO3/silver/graphene for refractive index sensing. Optoelectron. Lett. 2023, 19, 583–586. [Google Scholar] [CrossRef]
- Kullock, R.; Ochs, M.; Grimm, P.; Emmerling, M.; Hecht, B. Electrically-driven Yagi-Uda antennas for light. Nat. Commun. 2020, 11, 115. [Google Scholar] [CrossRef] [PubMed]
- Hao, J.; Ren, J.; Du, X.; Mikkelsen, J.H.; Shen, M.; Yin, Y.Z. Pattern-reconfigurable Yagi–Uda antenna based on liquid metal. IEEE Antennas Wirel. Propag. Lett. 2021, 20, 587–591. [Google Scholar] [CrossRef]
- Chen, T.; Zhang, D.; Huang, F.; Li, Z.; Hu, F. Design of a terahertz metamaterial sensor based on split ring resonator nested square ring resonator. Mater. Res. Express 2020, 7, 095802. [Google Scholar] [CrossRef]
- Rahbany, N.; Geng, W.; Bachelot, R.; Couteau, C. Plasmon–emitter interaction using integrated ring grating–nanoantenna structures. Nanotechnology 2017, 28, 185201. [Google Scholar] [CrossRef] [PubMed]
- Jeon, W.B.; Moon, J.S.; Kim, K.Y.; Ko, Y.H.; Richardson, C.J.; Waks, E.; Kim, J.H. Plug-and-Play Single-Photon Devices with Efficient Fiber-Quantum Dot Interface. Adv. Quantum Technol. 2022, 5, 2200022. [Google Scholar] [CrossRef]
- Ma, Y.; Ballesteros, G.; Zajac, J.M.; Sun, J.; Gerardot, B.D. Highly directional emission from a quantum emitter embedded in a hemispherical cavity. Opt. Lett. 2015, 40, 2373–2376. [Google Scholar] [CrossRef]
- Chen, Y.; Zopf, M.; Keil, R.; Ding, F.; Schmidt, O.G. Highly-efficient extraction of entangled photons from quantum dots using a broadband optical antenna. Nat. Commun. 2018, 9, 2994. [Google Scholar] [CrossRef]
- Ahn, D.H.; Jang, Y.D.; Baek, J.S.; Park, S.I.; Song, J.D.; Lee, D. A broadband high-brightness quantum-dot double solid immersion lens single photon source. APL Photonics 2023, 1, 8. [Google Scholar]
- Dong, Z.; Gorelik, S.; Paniagua-Dominguez, R.; Yik, J.; Ho, J.; Tjiptoharsono, F.; Lassalle, E.; Rezaei, S.D.; Neo, D.C.; Bai, P.; et al. Silicon nanoantenna mix arrays for a trifecta of quantum emitter enhancements. Nano Lett. 2021, 21, 4853–4860. [Google Scholar] [CrossRef]
- Abudayyeh, H.; Mildner, A.; Liran, D.; Lubotzky, B.; Luder, L.; Fleischer, M.; Rapaport, R. Overcoming the rate-directionality trade-off: A room-temperature ultrabright quantum light source. Acs Nano 2021, 15, 17384–17391. [Google Scholar] [CrossRef]
- Barreda, A.; Hell, S.; Weissflog, M.A.; Minovich, A.; Pertsch, T.; Staude, I. Metal, dielectric and hybrid nanoantennas for enhancing the emission of single quantum dots: A comparative study. J. Quant. Spectrosc. Radiat. Transf. 2021, 276, 107900. [Google Scholar] [CrossRef]
- Schraml, K.; Spiegl, M.; Kammerlocher, M.; Bracher, G.; Bartl, J.; Campbell, T.; Finley, J.J.; Kaniber, M. Optical properties and interparticle coupling of plasmonic bowtie nanoantennas on a semiconducting substrate. Phys. Rev. B 2014, 90, 035435. [Google Scholar] [CrossRef]
- Santhosh, K.; Bitton, O.; Chuntonov, L.; Haran, G. Vacuum Rabi splitting in a plasmonic cavity at the single quantum emitter limit. Nat. Commun. 2016, 7, ncomms11823. [Google Scholar] [CrossRef] [PubMed]
- Kabiri, A.; Girgis, E.; Capasso, F. Buried nanoantenna arrays: Versatile antireflection coating. Nano Lett. 2013, 13, 6040–6047. [Google Scholar] [CrossRef] [PubMed]
- Morshed, M.; Li, Z.; Olbricht, B.C.; Fu, L.; Haque, A.; Li, L.; Rifat, A.A.; Rahmani, M.; Miroshnichenko, A.E.; Hattori, H.T. High fluence chromium and tungsten bowtie nano-antennas. Sci. Rep. 2019, 9, 13023. [Google Scholar] [CrossRef] [PubMed]
- Huang, J.S.; Callegari, V.; Geisler, P.; Brüning, C.; Kern, J.; Prangsma, J.C.; Wu, X.; Feichtner, T.; Ziegler, J.; Weinmann, P.; et al. Atomically flat single-crystalline gold nanostructures for plasmonic nanocircuitry. Nat. Commun. 2010, 1, 150. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xin, C.; Huang, Y.; Li, R.; Ma, Y. Individual Tuning of Directional Emission and Luminance of a Quantum Emitter in a Composite Plasmonic Antenna. Photonics 2024, 11, 444. https://doi.org/10.3390/photonics11050444
Xin C, Huang Y, Li R, Ma Y. Individual Tuning of Directional Emission and Luminance of a Quantum Emitter in a Composite Plasmonic Antenna. Photonics. 2024; 11(5):444. https://doi.org/10.3390/photonics11050444
Chicago/Turabian StyleXin, Chaonuo, Yuming Huang, Renpu Li, and Yong Ma. 2024. "Individual Tuning of Directional Emission and Luminance of a Quantum Emitter in a Composite Plasmonic Antenna" Photonics 11, no. 5: 444. https://doi.org/10.3390/photonics11050444
APA StyleXin, C., Huang, Y., Li, R., & Ma, Y. (2024). Individual Tuning of Directional Emission and Luminance of a Quantum Emitter in a Composite Plasmonic Antenna. Photonics, 11(5), 444. https://doi.org/10.3390/photonics11050444



