Amplified Nonreciprocal Reflection in a Uniform Atomic Medium with the Help of Spontaneous Emissions
Abstract
1. Introduction
2. Theoretical Model and Equations
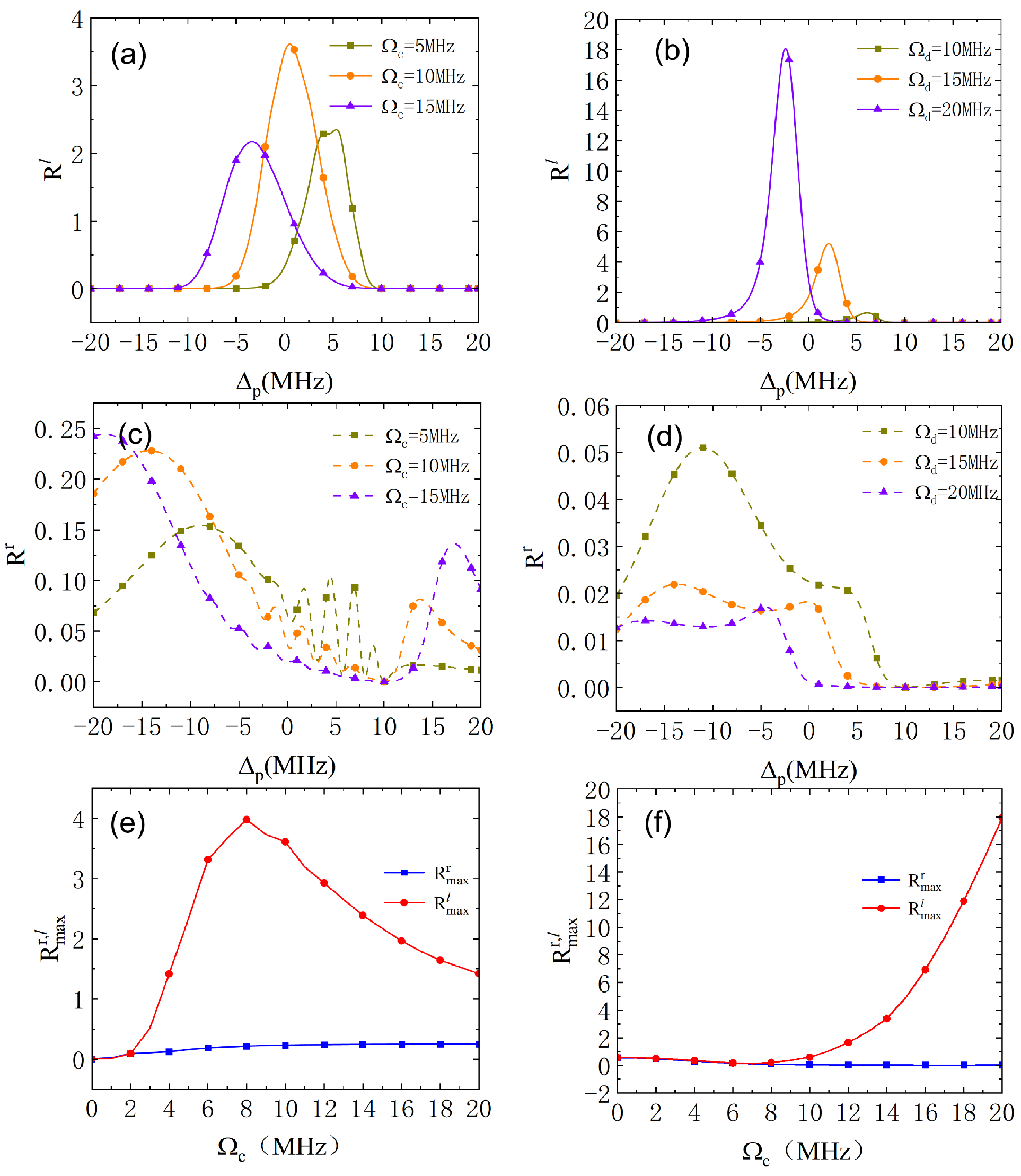
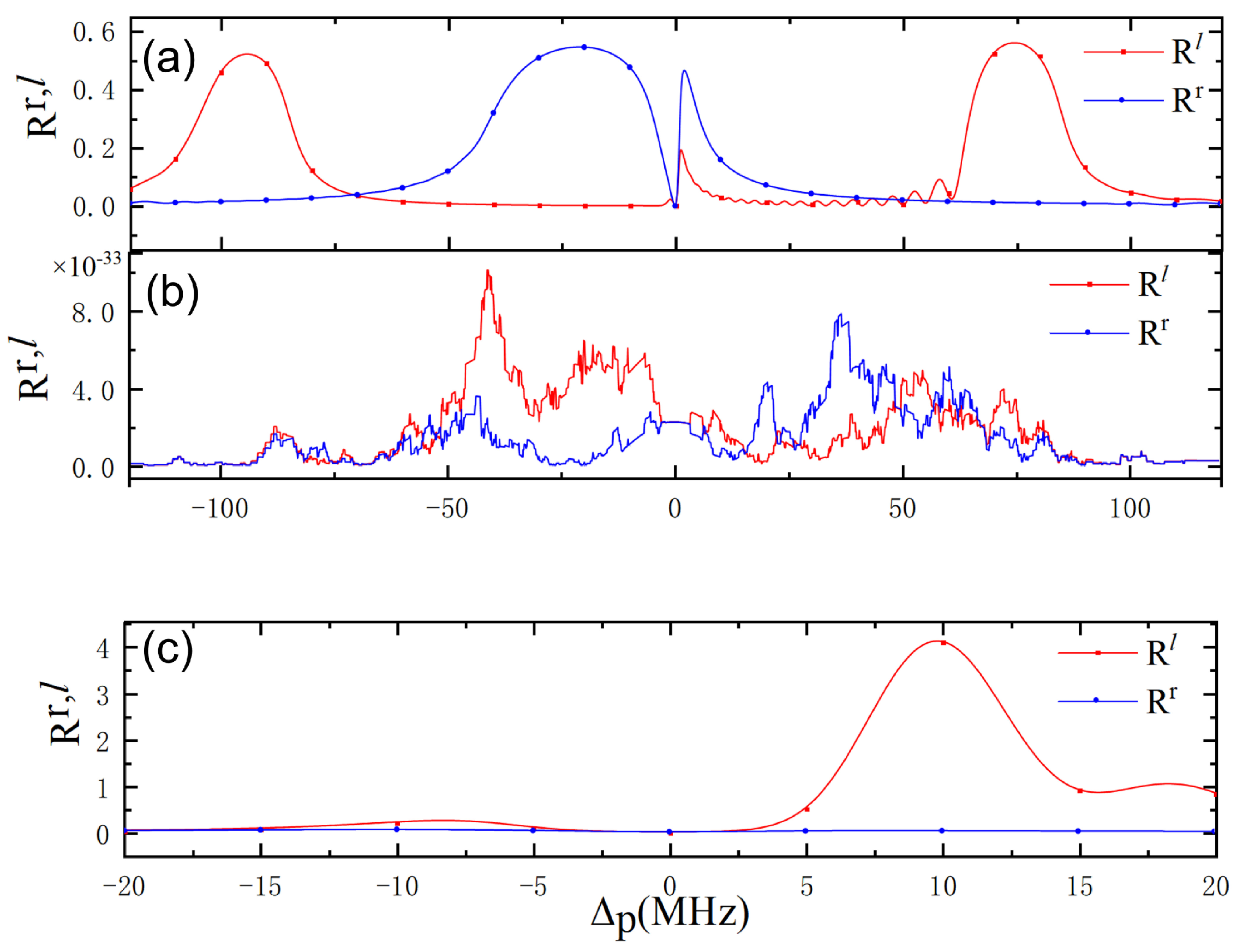
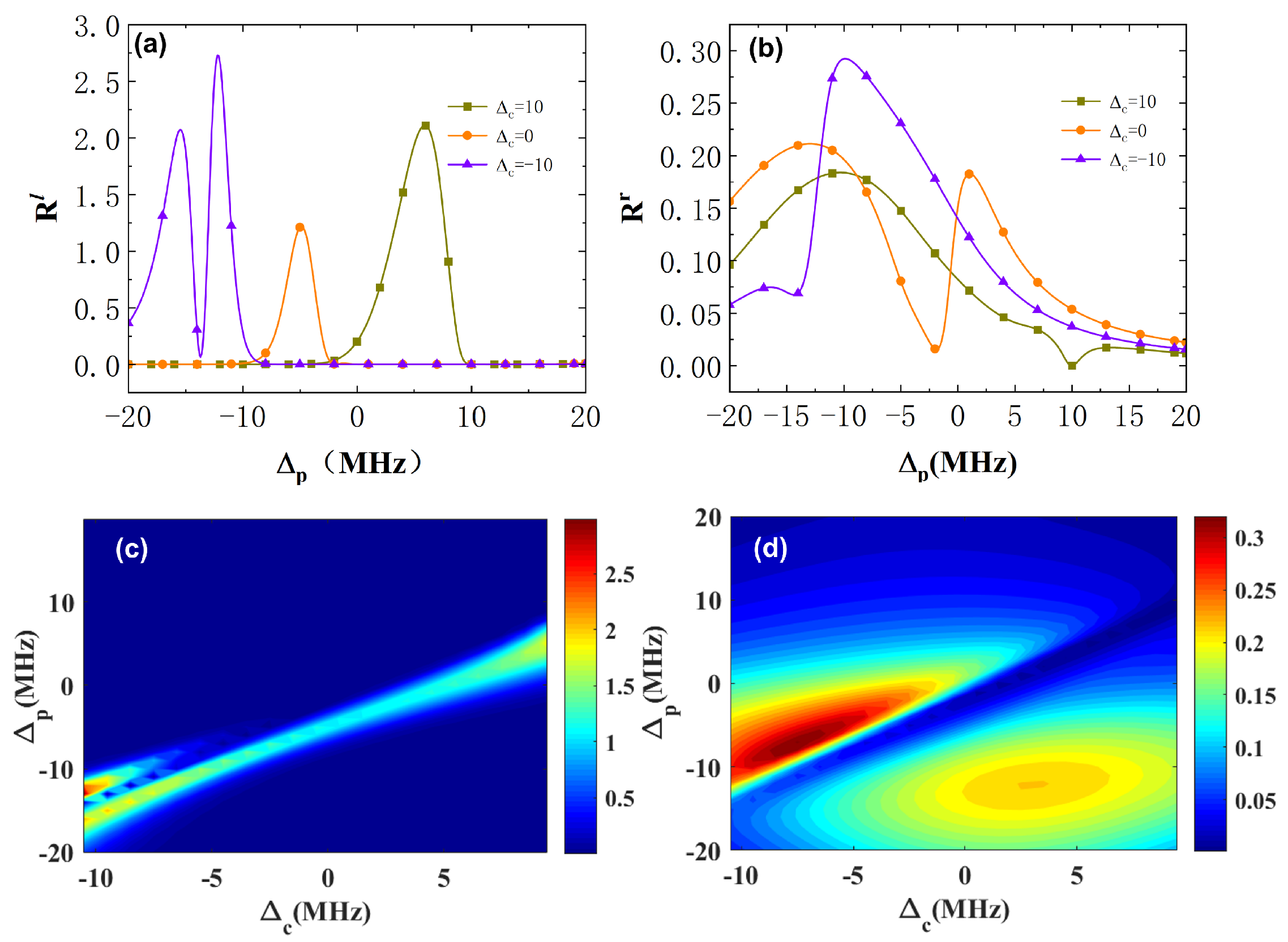
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Hu, Y.-Q.; Qi, Y.-H.; You, Y.; Zhang, S.-C.; Lin, G.-W.; Li, X.-L.; Gong, J.-B.; Gong, S.-Q.; Niu, Y.-P. Passive nonlinear optical isolators by passing dynamic reciprocity. Phys. Rev. A 2021, 16, 014046. [Google Scholar]
- KIttlaus, E.A.; Otterstrom, N.T.; Kharel, P.; Gertler, S.; Rakich, P.T. Non-reciprocal interband Brillouin modulation. Nat. Photon. 2018, 12, 613. [Google Scholar]
- Fan, S.; Shi, Y.; Lin, Q. Nonreciprocal Photonics without Magneto-Optics. IEEE Antennas Wirel. Propag. Lett. 2018, 17, 1948. [Google Scholar]
- Sounas, D.L.; Alu, A. Non-reciprocal photonics based on time modulation. Nat. Photon. 2017, 11, 774. [Google Scholar]
- Xia, K.-Y.; Lu, G.-W.; Lin, G.-W.; Cheng, Y.-Q.; Niu, Y.-P.; Gong, S.-Q.; Twamley, J. Reversible nonmagnetic single-photon isolation using unbalanced quantum coupling. Phys. Rev. A 2014, 90, 043802. [Google Scholar]
- Hu, Y.-Q.; Zhang, S.-C.; Qi, Y.-H.; Lin, G.-W.; Niu, Y.-P.; Gong, S.-Q. Multiwavelength magnetic-free optical isolator by optical pumping in warm atoms. Phys. Rev. Lett. 2019, 12, 054004. [Google Scholar]
- Shi, Y.; Yu, Z.-F.; Fan, S.-H. Limitations of nonlinear optical isolators due to dynamic reciprocity. Nat. Photon. 2015, 9, 388. [Google Scholar]
- Mailis, S. On-chip non-magnetic optical isolator. Nat. Photon. 2021, 5, 794. [Google Scholar]
- Xue, M.; Tong, H.; Dong, H.; Wang, M. Saturated Gain-Induced Non-Reciprocal Transmission and Broadband On-Chip Optical Isolator. Photonics 2024, 11, 261. [Google Scholar] [CrossRef]
- Jalas, D.; Petrov, A.; Eich, M.; Freude, W.; Fan, S.; Yu, Z.; Baets, R.; Popović, M.; Melloni, A.; Joannopoulos, J.D.; et al. What is- and what is not- an optical isolator. Nat. Photon. 2013, 7, 579. [Google Scholar]
- Shen, H.-Z.; Wang, Q.; Wang, J.; Yi, X.-X. Nonreciprocal unconventional photon blockade in a driven dissipative cavity with parametric amplification. Phys. Rev. A 2020, 101, 013826. [Google Scholar] [CrossRef]
- Zhang, S.-C.; Hu, Y.-Q.; Lin, G.-W.; Niu, Y.-P.; Xia, K.-Y.; Gong, J.-B.; Gong, S.-Q. Thermal-motion-induced non-reciprocal quantum optical system. Nat. Photon. 2018, 12, 744. [Google Scholar]
- Dong, M.-X.; Xia, K.-Y.; Zhang, W.-H.; Yu, Y.-C.; Ye, Y.-H.; Li, E.-Z.; Zeng, L.; Ding, D.-S.; Shi, B.-S.; Guo, G.-C.; et al. All-optical reversible single-photon isolation at room temperature. Sci. Adv. 2021, 7, eabe8924. [Google Scholar] [PubMed]
- Yang, H.; Qin, G.-Q.; Zhang, H.; Mao, X.; Wang, M.; Long, G.-L. Multimode interference induced optical nonreciprocity and routing in an optical microcavity. Ann. Der Phys. 2021, 533, 2000506. [Google Scholar]
- Yang, P.-F.; Xia, X.-W.; He, H.; Li, S.-K.; Han, X.; Zhang, P.; Li, G.; Zhang, P.-F.; Xu, J.-P.; Yang, Y.-P.; et al. Realization of nonlinear optical nonreciprocity on a few-photon level based on atoms strongly coupled to an asymmetric cavity. Phys. Rev. Lett. 2019, 123, 233604. [Google Scholar] [PubMed]
- Huang, R.; Miranowicz, A.; Liao, J.Q.; Nori, F.; Jing, H. Nonreciprocal photon blockade. Phys. Rev. Lett. 2018, 121, 153601. [Google Scholar] [PubMed]
- Shen, Z.; Zhang, Y.-L.; Chen, Y.; Zou, C.-L.; Xiao, Y.-F.; Zou, X.-B.; Sun, F.-W.; Guo, G.-C.; Dong, C.-H. Experimental realization of optomechanically induced non-reciprocity. Nat. Photon. 2016, 10, 657. [Google Scholar]
- Tang, L.; Tang, J.-S.; Chen, M.-Y.; Nori, F.; Xiao, M.; Xia, K.-Y. Quantum squeezing induced optical nonreciprocity. Phys. Rev. Lett. 2022, 128, 083604. [Google Scholar] [PubMed]
- Tang, J.-S.; Nie, W.; Tang, L.; Chen, M.-Y.; Su, X.; Lu, Y.-Q.; Nori, F.; Xia, K.-Y. Nonreciprocal Single-Photon Band Structure. Phys. Rev. Lett. 2022, 128, 203602. [Google Scholar]
- Ji, X.; Pan, P.; Huang, S.; Chen, A. Optical Nonreciprocity in Double Optomechanical Systems with Quadratic Coupling. Photonics 2022, 9, 728. [Google Scholar] [CrossRef]
- Wang, D.-W.; Zhou, H.-T.; Guo, M.-J.; Zhang, J.-X.; Evers, J.; Zhu, S.-Y. Optical Diode Made from a Moving Photonic Crystal. Phys. Rev. Lett. 2013, 110, 093901. [Google Scholar] [PubMed]
- Horsley, S.-A.-R.; Wu, J.-H.; Artoni, M.; La Rocca, G.-C. Dynamically induced two-color nonreciprocity in a tripod system of a moving atomic lattice. Phys. Rev. Lett. 2013, 110, 223602. [Google Scholar] [CrossRef] [PubMed]
- Cao, Q.-T.; Wang, H.-M.; Dong, C.-H.; Jing, H.; Liu, R.-S.; Chen, X.; Ge, L.; Gong, Q.-H.; Xiao, Y.-F. Experimental demonstration of spontaneous chirality in a nonlinear microresonator. Phys. Rev. Lett. 2017, 118, 033901. [Google Scholar] [PubMed]
- Wang, Q.; Xu, F.; Yu, Z.; Qian, X.; Hu, X.; Lu, Y.; Wang, H.-T. A bidirectional tunable optical diode based on periodically poled LiNbO3. Opt. Express 2010, 18, 7340–7346. [Google Scholar] [CrossRef] [PubMed]
- Miroshnichenko, A.E.; Brasselet, E.; Kivshar, Y.S. Reversible optical nonreciprocity in periodic structures with liquid crystals. Appl. Phys. Lett. 2010, 96, 063302. [Google Scholar]
- Wu, J.-H.; Artoni, M.; La Rocca, G.-C. Perfect absorption and no reflection in disordered photonic crystals. Phys. Rev. A 2017, 95, 053862. [Google Scholar]
- Tian, S.-C.; Wan, R.-G.; Wang, L.-J.; Shu, S.-L.; Lu, H.; Zhang, X.; Tong, C.-Z.; Feng, J.-L.; Xiao, M.; Wang, L.-J. Asymmetric light diffraction of two-dimensional electromagnetically induced grating with PT symmetry in asymmetric double quantum wells. Opt. Express 2018, 26, 32918. [Google Scholar] [PubMed]
- Wu, J.-H.; Artoni, M.; La Rocca, G.-C. Non-Hermitian degeneracies and unidirectional reflectionless atomic lattices. Phys. Rev. Lett. 2014, 113, 123004. [Google Scholar] [CrossRef] [PubMed]
- Horsley, S.-A.-R.; Longhi, S. Spatiotemporal deforma-tions of reflectionless potentials. Phys. Rev. A 2017, 96, 023841. [Google Scholar]
- Horsley, S.-A.-R.; Artoni, M.; La Rocca, G.-C. Spatial Kramers–Kronig relations and the reflection of waves. Nat. Photon. 2015, 9, 436. [Google Scholar]
- Baek, Y.; Park, Y. Intensity-based holographic imaging via space-domain Kramers-Kronig relations. Nat. Photon. 2021, 15, 354–360. [Google Scholar]
- Lee, C.; Baek, Y.; Hugonnet, H.; Park, Y. Single-shot wide-field topography measurement using spectrally multiplexed reflection intensity holography via space-domain Kramers-Kronig relations. Opt. Lett. 2022, 47, 1025–1208. [Google Scholar] [PubMed]
- Li, Q.; Luo, Y.; Liu, D.; Gao, Y.; Zhang, J.; Ran, L.; Ye, D.-X. A miniaturized anechoic chamber: Omnidirectional impedance matching based on truncated spatial Kramers-Kronig medium. Adv. Opt. Mater. 2022, 10, 2200381. [Google Scholar]
- Jiang, W.; Ma, Y.-G.; Yuan, J.; Yin, G.; Wu, W.-H.; He, S.-L. Deformable broadband metamaterial absorbers engineered with an analytical spatial Kramers-Kronig permittivity profile. Laser Photonics Rev. 2017, 11, 1600253. [Google Scholar]
- Zheng, D.-D.; Zhang, Y.; Liu, Y.-M.; Zhang, X.-J.; Wu, J.-H. Spatial Kramers-Kronig relation and unidirectional light reflection induced by Rydberg interactions. Phys. Rev. A 2023, 107, 013704. [Google Scholar]
- Zhang, Y.; Wu, J.-H.; Artoni, M.; La Rocca, G.-C. Controlled unidirectional reflection in cold atoms via the spatial kramers-kronig relation. Opt. Express 2021, 29, 5890–5900. [Google Scholar] [PubMed]
- Pei, X.-S.; Zhang, H.-X.; Pan, M.-M.; Geng, Y.; Li, T.-M.; Yang, H. Two-color unidirectional reflections by modulating the spatial susceptibility in a homogeneous atomic medium. Opt. Express 2023, 31, 14694. [Google Scholar] [PubMed]
- Lin, G.-W.; Zhang, S.-C.; Hu, Y.-Q.; Niu, Y.-P.; Gong, J.-B.; Gong, S.-Q. Nonreciprocal Amplification with Four-Level Hot Atoms. Phys. Rev. Lett. 2019, 123, 033902. [Google Scholar]
- Fang, K.; Luo, J.; Metelmann, A.; Matheny, M.H.; Marquardt, F.; Clerk, A.A.; Painter, O. Generalized non-reciprocity in an optomechanical circuit via synthetic magnetism and reservoir engineering. Nat. Phys. 2017, 13, 465. [Google Scholar] [CrossRef]
- Shen, Z.; Zhang, Y.-L.; Chen, Y.; Sun, F.-W.; Zou, X.-B.; Guo, G.-C.; Zou, C.-L.; Dong, C.-H. Reconfigurable optomechanical circulator and directional amplifier. Nat. Commun. 2018, 9, 1797. [Google Scholar]
- Song, L.-N.; Zheng, Q.; Xu, X.-W.; Jiang, C.; Li, Y. Optimal unidirectional amplification induced by optical gain in optomechanical systems. Phys. Rev. A 2019, 100, 043835. [Google Scholar]
- Peng, R.; Zhang, W.-Z.; Chao, S.-L.; Zhao, C.-G.; Yang, Z.; Yang, J.-Y.; Zhou, L. Unidirectional amplification in optomechanical system coupling with a structured bath. Opt. Express 2022, 30, 21649–21663. [Google Scholar] [PubMed]
- Abdo, B.; Sliwa, K.; Frunzio, L.; Devoret, M. Directional amplification with a Josephson circuit. Phys. Rev. X 2013, 3, 031001. [Google Scholar]
- de las Heras, A.M.; Carusotto, I. Unidirectional lasing in nonlinear Taiji micro-ring resonators. Phys. Rev. A 2021, 104, 043501. [Google Scholar]
- Jiang, Y.; Maayani, S.; Carmon, T.; Nori, F.; Jing, H. Nonreciprocal Phonon Laser. Phys. Rev. Appl. 2018, 10, 064037. [Google Scholar] [CrossRef]
- Jin, L. Asymmetric lasing at spectral singularities. Phys. Rev. A 2018, 97, 033840. [Google Scholar]
- Geng, Y.; Pei, X.-S.; Li, G.-R.; Lin, X.-Y.; Zhang, H.-X.; Yan, D.; Yang, H. Spatial susceptibility modulation and controlled unidirectional reflection amplification via four-wave mixing. Opt. Express 2023, 31, 38228–38239. [Google Scholar]
- Artoni, M.; La Rocca, G.-C.; Bassani, F. Resonantly absorbing one-dimensional photonic crystals. Phys. Rev. E 2005, 72, 046604. [Google Scholar]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, X.; Zheng, X.; Geng, Y.; Li, G.; Xu, Q.; Wu, J.; Yan, D.; Yang, H. Amplified Nonreciprocal Reflection in a Uniform Atomic Medium with the Help of Spontaneous Emissions. Photonics 2024, 11, 389. https://doi.org/10.3390/photonics11040389
Lin X, Zheng X, Geng Y, Li G, Xu Q, Wu J, Yan D, Yang H. Amplified Nonreciprocal Reflection in a Uniform Atomic Medium with the Help of Spontaneous Emissions. Photonics. 2024; 11(4):389. https://doi.org/10.3390/photonics11040389
Chicago/Turabian StyleLin, Xinyu, Xinfu Zheng, Yue Geng, Guanrong Li, Qiongyi Xu, Jinhui Wu, Dong Yan, and Hong Yang. 2024. "Amplified Nonreciprocal Reflection in a Uniform Atomic Medium with the Help of Spontaneous Emissions" Photonics 11, no. 4: 389. https://doi.org/10.3390/photonics11040389
APA StyleLin, X., Zheng, X., Geng, Y., Li, G., Xu, Q., Wu, J., Yan, D., & Yang, H. (2024). Amplified Nonreciprocal Reflection in a Uniform Atomic Medium with the Help of Spontaneous Emissions. Photonics, 11(4), 389. https://doi.org/10.3390/photonics11040389





