High-Power Mid-Infrared Quantum Cascade Laser with Large Emitter Width
Abstract
1. Introduction
2. Structure Analysis and Device Fabrication
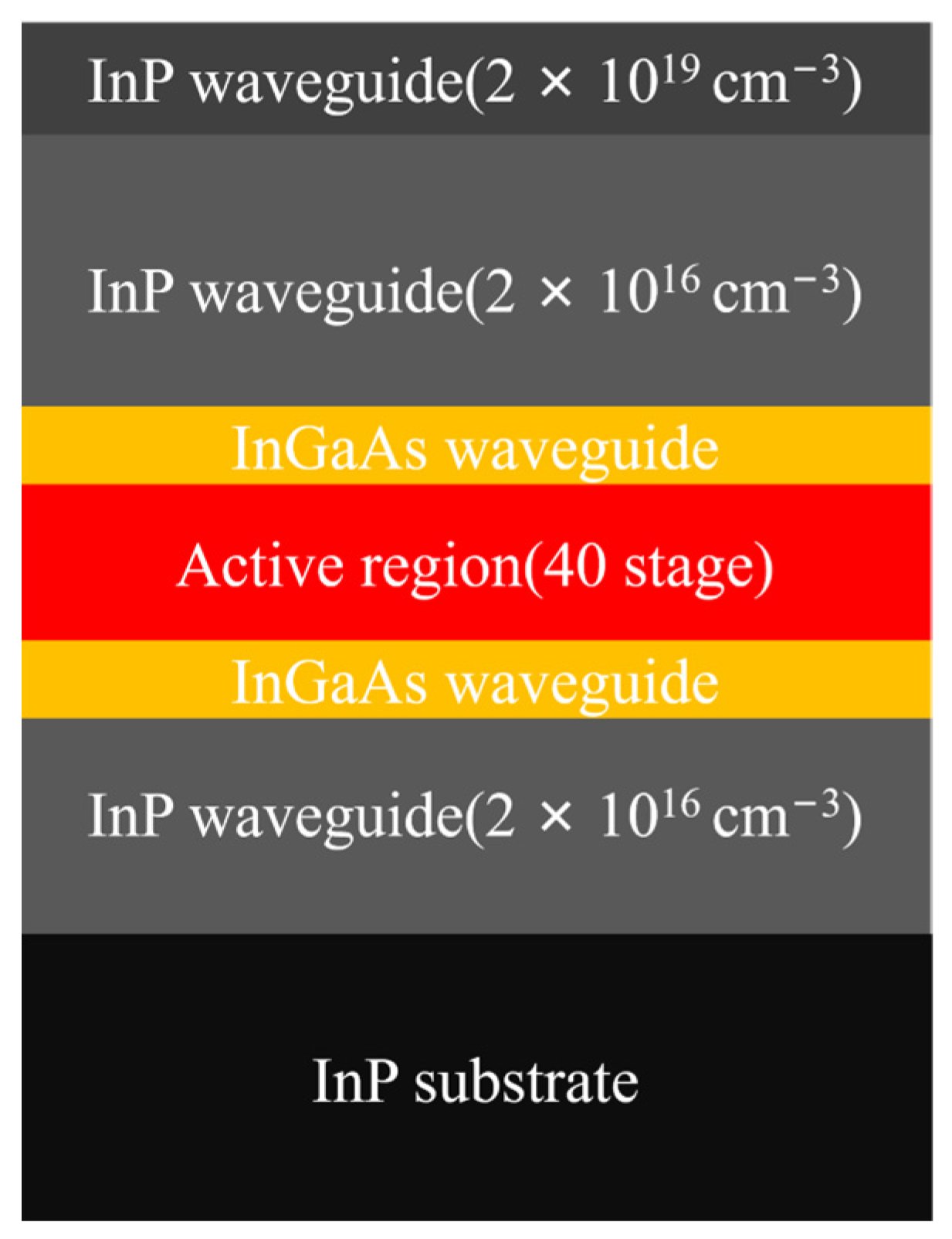
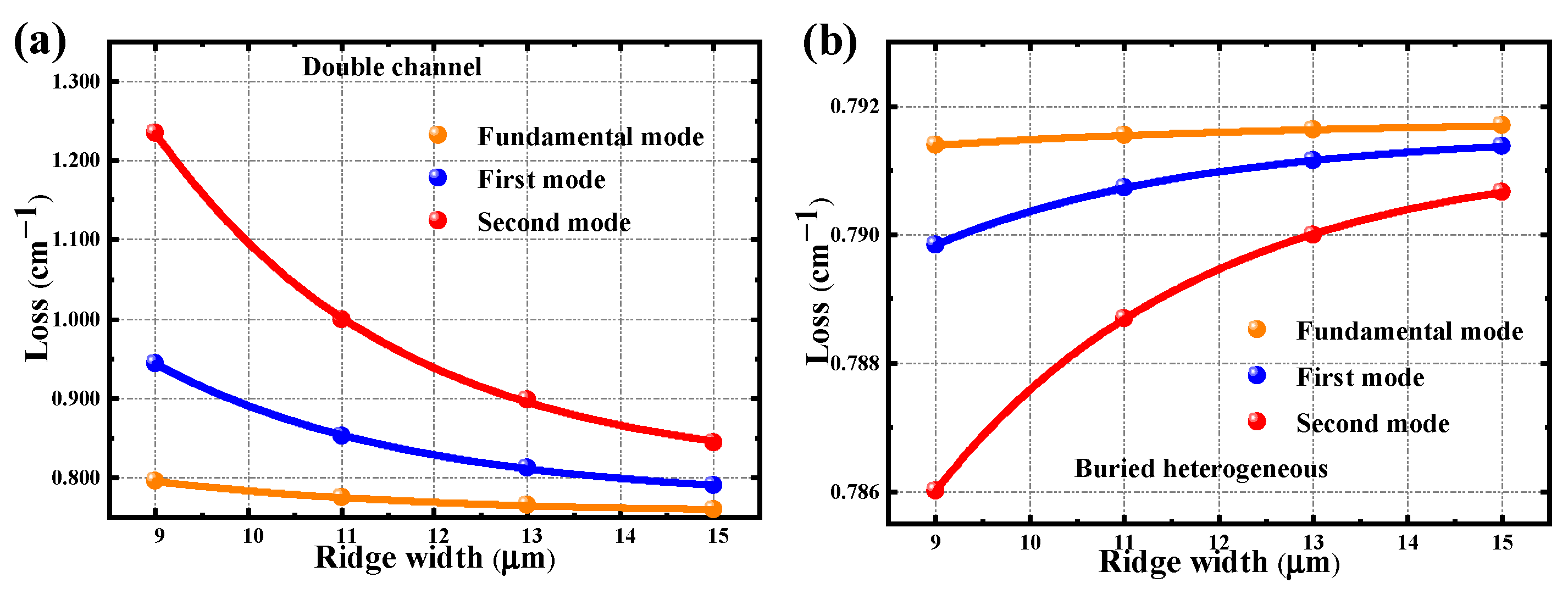
2.1. Structure Analysis
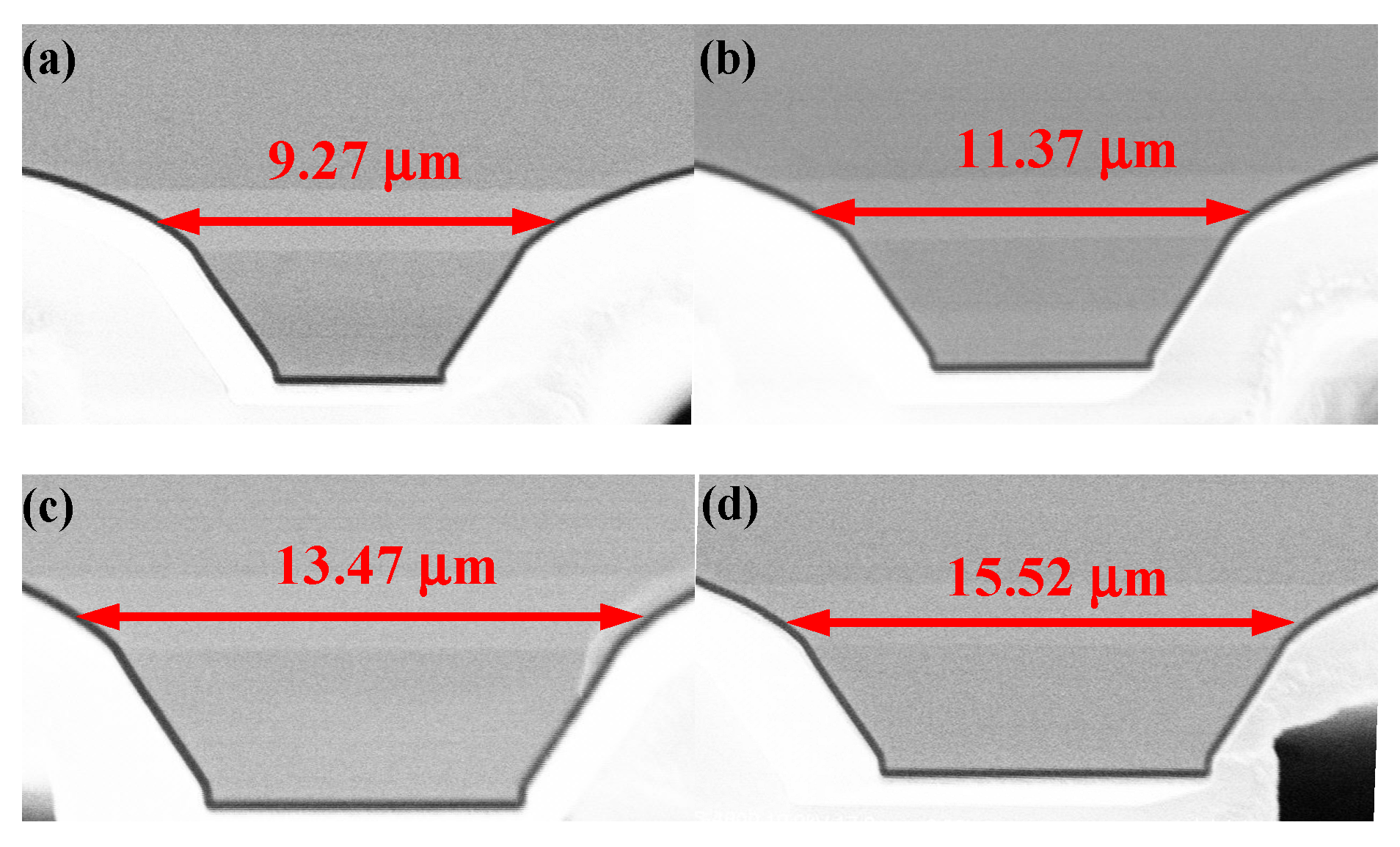
2.2. Device Fabrication
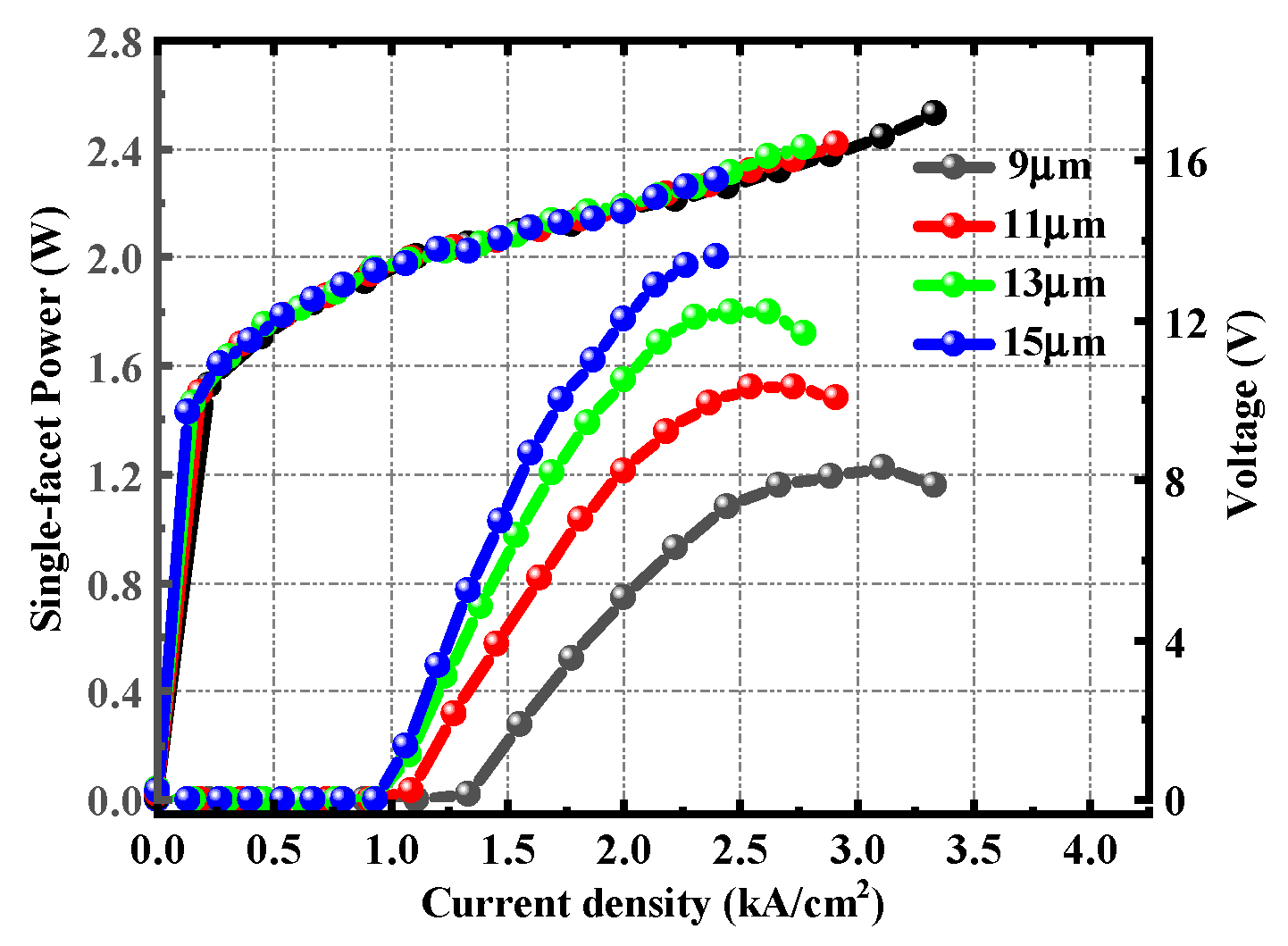
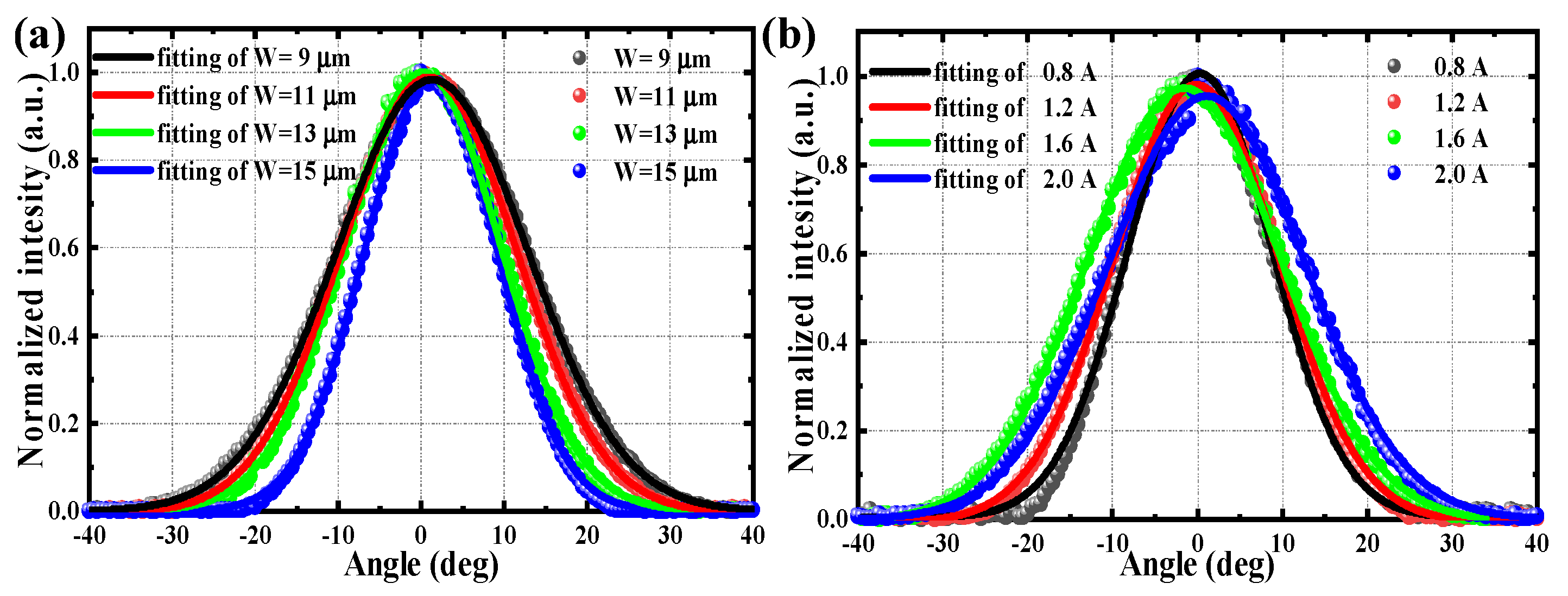
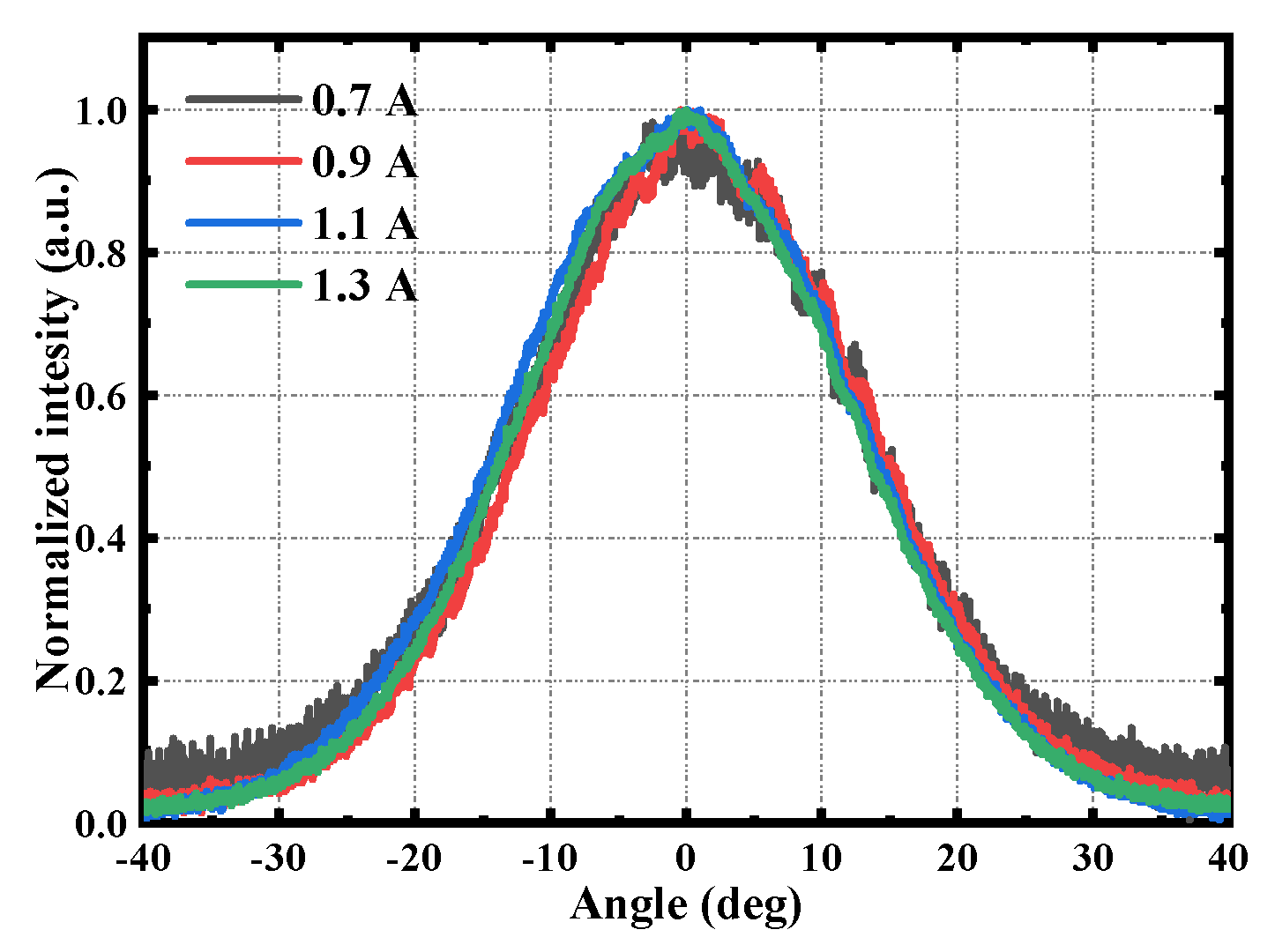
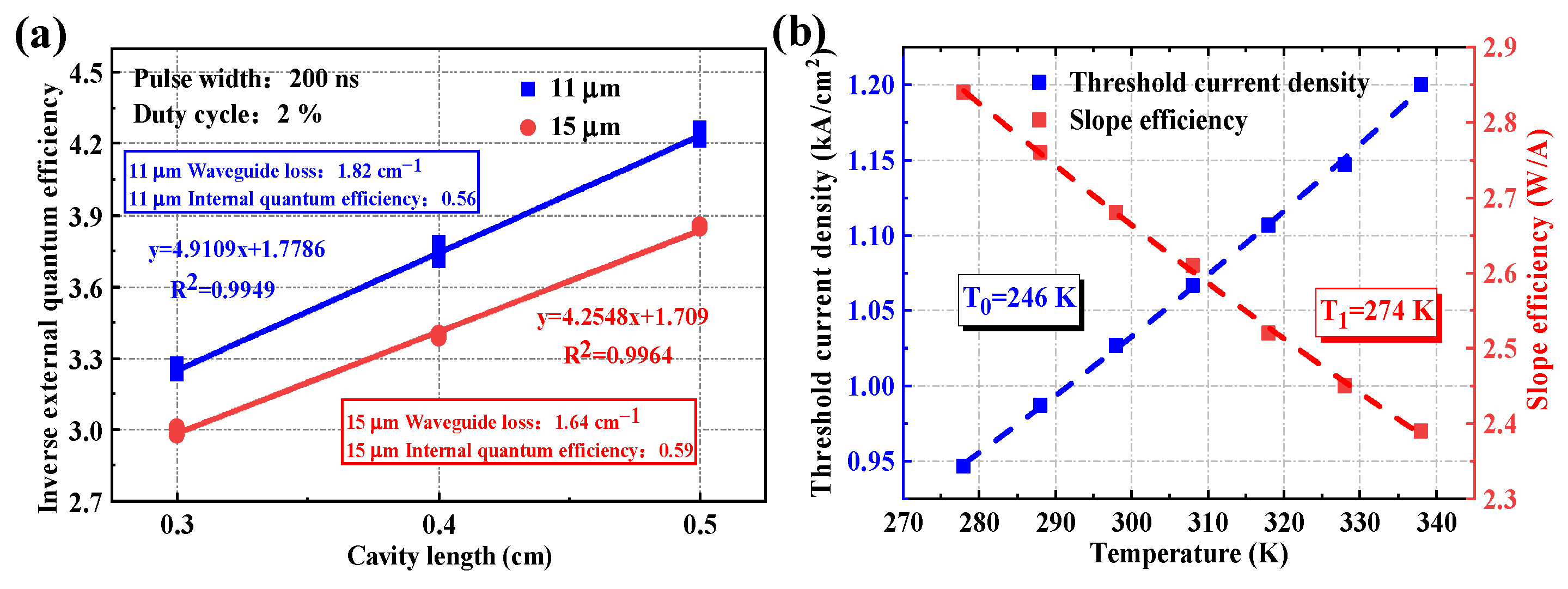
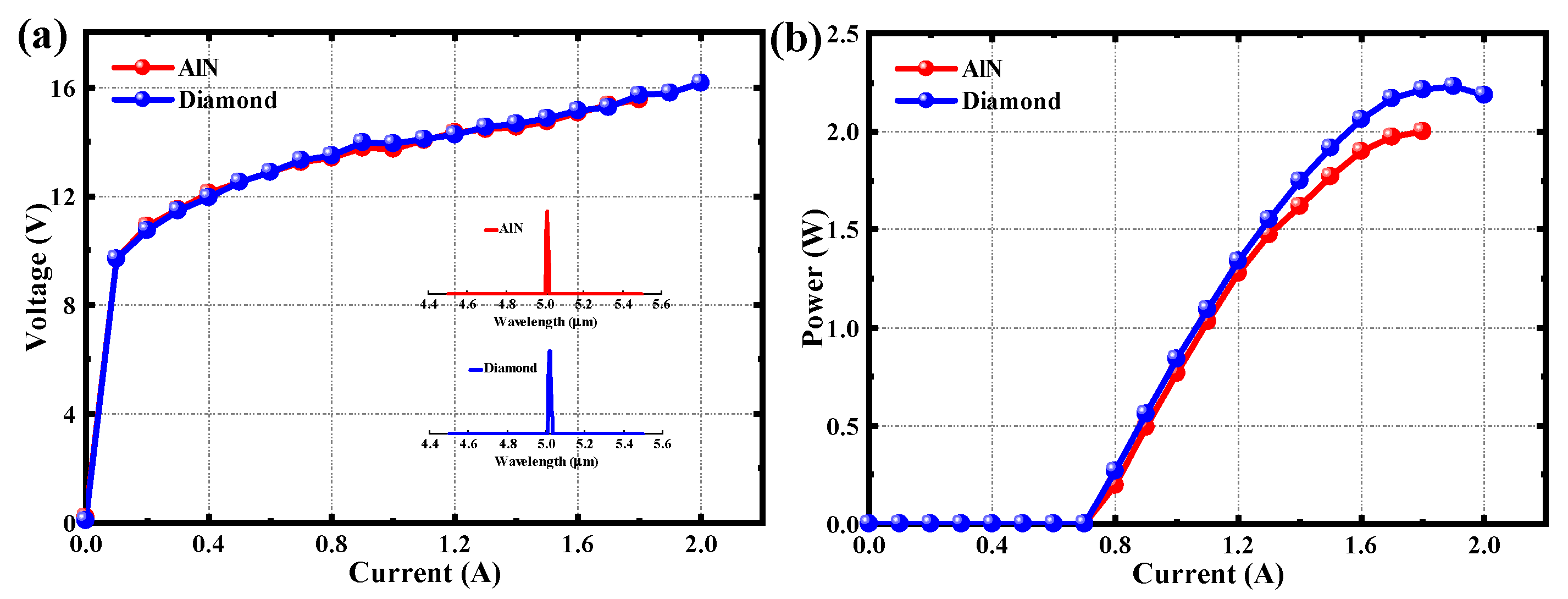
3. Device Performance and Testing
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Faist, J.; Capasso, F.; Sivco, D.L.; Sirtori, C.; Hutchinson, A.L.; Cho, A.Y. Quantum cascade laser. Science 1994, 264, 553–556. [Google Scholar] [CrossRef] [PubMed]
- Vitiello, M.S.; Scalari, G.; Williams, B.; De Natale, P. Quantum cascade lasers: 20 years of challenges. Opt. Express 2015, 23, 5167–5182. [Google Scholar] [CrossRef] [PubMed]
- Fathololoumi, S.; Dupont, E.; Chan, C.W.I.; Wasilewski, Z.R.; Laframboise, S.R.; Ban, D.; Mátyás, A.; Jirauschek, C.; Hu, Q.; Liu, H.C. Terahertz quantum cascade lasers operating up to ∼200 K with optimized oscillator strength and improved injection tunneling. Opt. Express 2012, 20, 3866–3876. [Google Scholar] [CrossRef] [PubMed]
- Li, L.; Chen, L.; Zhu, J.; Freeman, J.; Dean, P.; Valavanis, A.; Davies, A.G.; Linfield, E.H. Terahertz quantum cascade lasers with >1 W output powers. Electron. Lett. 2014, 50, 309–311. [Google Scholar] [CrossRef]
- Spitz, O.; Didier, P.; Durupt, L.; Diaz-Thomas, D.A.; Baranov, A.N.; Cerutti, L.; Grillot, F. Free-space communication with directly modulated mid-infrared quantum cascade devices. IEEE J. Sel. Top. Quantum Electron. 2021, 28, 1200109. [Google Scholar] [CrossRef]
- Dely, H.; Bonazzi, T.; Spitz, O.; Rodriguez, E.; Gacemi, D.; Todorov, Y.; Pantzas, K.; Beaudoin, G.; Sagnes, I.; Li, L.; et al. 10 Gbit s−1 free space data transmission at 9 µm wavelength with unipolar quantum optoelectronics. Laser Photonics Rev. 2022, 16, 2100414. [Google Scholar] [CrossRef]
- Cheng, F.; Zhang, J.; Guan, Y.; Yang, P.; Zhuo, N.; Zhai, S.; Liu, J.; Wang, L.; Liu, S.; Liu, F.; et al. Ultralow power consumption of a quantum cascade laser operating in continuous-wave mode at room temperature. Opt. Express 2020, 28, 36497–36504. [Google Scholar] [CrossRef]
- Wang, Z.; Kapsalidis, F.; Wang, R.; Beck, M.; Faist, J. Ultra-low threshold lasing through phase front engineering via a metallic circular aperture. Nat. Commun. 2022, 13, 230. [Google Scholar] [CrossRef]
- Cheng, F.; Zhang, J.; Sun, Y.; Zhuo, N.; Zhai, S.; Liu, J.; Wang, L.; Liu, S.; Liu, F. High performance distributed feedback quantum cascade laser emitting at λ∼6.12 um. Opt. Express 2022, 30, 5848–5854. [Google Scholar] [CrossRef]
- Fei, T.; Zhai, S.; Zhang, J.; Zhuo, N.; Liu, J.; Wang, L.; Liu, S.; Jia, Z.; Li, K.; Sun, Y.; et al. High power λ~ 8.5 μm quantum cascade laser grown by MOCVD operating continuous-wave up to 408 K. J. Semicond. 2021, 42, 112301. [Google Scholar] [CrossRef]
- Wang, H.; Zhang, J.; Cheng, F.; Zhuo, N.; Zhai, S.; Liu, J.; Wang, L.; Liu, S.; Liu, F.; Wang, Z. Watt-level, high wall plug efficiency, continuous-wave room temperature quantum cascade laser emitting at 7.7 µm. Opt. Express 2020, 28, 40155–40163. [Google Scholar] [CrossRef]
- Zhou, W.; Lu, Q.Y.; Wu, D.H.; Slivken, S.; Razeghi, M. High-power, continuous-wave, phase-locked quantum cascade laser arrays emitting at 8 µm. Opt. Express 2019, 27, 15776–15785. [Google Scholar] [CrossRef]
- Beck, M.; Hofstetter, D.; Aellen, T.; Faist, J.; Oesterle, U.; Ilegems, M.; Gini, E.; Melchior, H. Continuous wave operation of a mid-infrared semiconductor laser at room temperature. Science 2002, 295, 301–305. [Google Scholar] [CrossRef] [PubMed]
- Bai, Y.; Darvish, S.R.; Slivken, S.; Zhang, W.; Evans, A.; Nguyen, J.; Razeghi, M. Room temperature continuous wave operation of quantum cascade lasers with watt-level optical power. Appl. Phys. Lett. 2008, 92, 101105. [Google Scholar] [CrossRef]
- Lyakh, A.; Maulini, R.; Tsekoun, A.; Go, R.; Pflügl, C.; Diehl, L.; Wang, Q.J.; Capasso, F.; Patel, C.K.N. 3 W continuous-wave room temperature single-facet emission from quantum cascade lasers based on nonresonant extraction design approach. Appl. Phys. Lett. 2009, 95, 141113. [Google Scholar] [CrossRef]
- Wang, F.; Slivken, S.; Wu, D.H.; Lu, Q.Y.; Razeghi, M. Continuous wave quantum cascade lasers with 5.6 W output power at room temperature and 41% wall-plug efficiency in cryogenic operation. AIP Adv. 2020, 10, 055120. [Google Scholar] [CrossRef]
- Patel, C.K.N.; Troccoli, M.; Barron-Jimenez, R.; Titterton, D.H.; Grasso, R.J.; Richardson, M.A. Quantum cascade lasers: 25 years after the first demonstration. In Technologies for Optical Countermeasures XVI; SPIE: Bellingham, WA, USA, 2019. [Google Scholar]
- Diehl, L.; Bour, D.; Corzine, S.; Zhu, J.; Höfler, G.; Lončar, M.; Troccoli, M.; Capasso, F. High-temperature continuous wave operation of strain-balanced quantum cascade lasers grown by metal organic vapor-phase epitaxy. Appl. Phys. Lett. 2006, 89, 081101. [Google Scholar] [CrossRef]
- Diehl, L.; Bour, D.; Corzine, S.; Zhu, J.; Höfler, G.; Lončar, M.; Troccoli, M.; Capasso, F. High-power quantum cascade lasers grown by low-pressure metal organic vapor-phase epitaxy operating in continuous wave above 400 K. Appl. Phys. Lett. 2006, 88, 201115. [Google Scholar] [CrossRef]
- Troccoli, M.; Diehl, L.; Bour, D.P.; Corzine, S.W.; Yu, N.; Wang, C.Y.; Belkin, M.A.; Höfler, G.; Lewicki, R.; Wysocki, G.; et al. High-performance quantum cascade lasers grown by metal-organic vapor phase epitaxy and their applications to trace gas sensing. J. Light. Technol. 2008, 26, 3534–3555. [Google Scholar] [CrossRef]
- Wang, C.A.; Huang, R.K.; Sanchez-Rubio, A.; Goyal, A.; Donnelly, J.P.; Calawa, D.R.; Cann, S.G.; O’Donnell, F.; Plant, J.J.; Missaggia, L.J.; et al. OMVPE growth of highly strain-balanced GaInAs/AlInAs/InP for quantum cascade lasers. J. Cryst. Growth 2008, 310, 5191–5197. [Google Scholar] [CrossRef]
- Roberts, J.S.; Green, R.P.; Wilson, L.R.; Zibik, E.A.; Revin, D.G.; Cockburn, J.W.; Airey, R.J. Quantum cascade lasers grown by metalorganic vapor phase epitaxy. Appl. Phys. Lett. 2003, 82, 4221–4223. [Google Scholar] [CrossRef]
- Botez, D.; Kirch, J.D.; Boyle, C.; Oresick, K.M.; Sigler, C.; Kim, H.; Knipfer, B.B.; Ryu, J.H.; Lindberg, D.; Earles, T.; et al. High-efficiency, high-power mid-infrared quantum cascade lasers. Opt. Mater. Express 2018, 8, 1378–1398. [Google Scholar] [CrossRef]
- Fei, T.; Zhai, S.; Zhang, J.; Lu, Q.; Zhuo, N.; Liu, J.; Wang, L.; Liu, S.; Jia, Z.; Li, K.; et al. 3 W Continuous-Wave Room Temperature Quantum Cascade Laser Grown by Metal-Organic Chemical Vapor Deposition. Photonics 2023, 10, 47. [Google Scholar] [CrossRef]
- Bouzi, P.M. High Performance Quantum Cascade Lasers: Loss, Beam Stability, and Gain Engineering. Ph.D. Thesis, Princeton University, Princeton, NJ, USA, 2015; p. 142. [Google Scholar]
- Bouzi, P.M.; Liu, P.Q.; Aung, N.; Wang, X.; Fan, J.-Y.; Troccoli, M.; Gmachl, C.F. Suppression of pointing instability in quantum cascade lasers by transverse mode control. Appl. Phys. Lett. 2013, 102, 122105. [Google Scholar] [CrossRef]
- Lu, Q.Y.; Bai, Y.; Bandyopadhyay, N.; Slivken, S.; Razeghi, M. Room-temperature continuous wave operation of distributed feedback quantum cascade lasers with watt-level power output. Appl. Phys. Lett. 2010, 97, 231119. [Google Scholar] [CrossRef]
- Lu, Q.Y.; Bai, Y.; Bandyopadhyay, N.; Slivken, S.; Razeghi, M. 2.4 W room temperature continuous wave operation of distributed feedback quantum cascade. Appl. Phys. Lett. 2011, 98, 181106. [Google Scholar] [CrossRef]
- Bai, Y.; Bandyopadhyay, N.; Tsao, S.; Selcuk, E.; Slivken, S.; Razeghi, M. Highly temperature insensitive quantum cascade lasers. Appl. Phys. Lett. 2010, 97, 251104. [Google Scholar] [CrossRef]
- Lei, P.; Cheng, Y.; Zhao, W.; Tan, S.; Guo, Y.; Li, B.; Wang, J.; Zhou, D. Mid-infrared quantum cascade laser grown by MOCVD at 4.6 µm. Infrared Laser Eng. 2022, 51, 20210980. [Google Scholar]
- Ryu, J.H.; Kirch, J.D.; Knipfer, B.; Liu, Z.; Turville-Heitz, M.; Earles, T.; Marsland, R.A.; Strömberg, A.; Omanakuttan, G.; Sun, Y.-T.; et al. Beam stability of buried-heterostructure quantum cascade lasers employing HVPE regrowth. Opt. Express 2021, 29, 2819–2826. [Google Scholar] [CrossRef]
- Evans, A.J. GasMBE Growth and Characterization of Strained Layer Indium Phosphide-Gallium Indium Arsenide-Aluminum Indium Arsenide Quantum Cascade Lasers. Ph.D. Thesis, Northwestern University, Evanston, IL, USA, 2008; p. 326. [Google Scholar]
- Bewley, W.W.; Lindle, J.R.; Kim, C.S.; Vurgaftman, I.; Meyer, J.R.; Evans, A.J.; Yu, J.S.; Slivken, S.; Razeghi, M. Beam steering in high-power CW quantum-cascade lasers. IEEE J. Quantum Electron. 2005, 41, 833–841. [Google Scholar] [CrossRef]
- Bai, Y. High Wall Plug Efficiency Quantum Cascade Lasers. Ph.D. Thesis, Northwestern University, Evanston, IL, USA, 2011; p. 275. [Google Scholar]
- Lyakh, A.; Suttinger, M.; Go, R.; Figueiredo, P.; Todi, A. 5.6 μm quantum cascade lasers based on a two-material active region composition with a room temperature wall-plug efficiency exceeding 28%. Appl. Phys. Lett. 2016, 109, 121109. [Google Scholar] [CrossRef]
- Sun, Y.; Yin, R.; Zhang, J.; Liu, J.; Fei, T.; Li, K.; Guo, K.; Jia, Z.; Liu, S.; Lu, Q.; et al. High-performance quantum cascade lasers at λ∼9 µm grown by MOCVD. Opt. Express 2022, 30, 37272–37280. [Google Scholar] [CrossRef] [PubMed]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Zhang, Y.; Wang, J.; Cheng, Y.; Zhao, W.; Wei, Z.; Zhou, D. High-Power Mid-Infrared Quantum Cascade Laser with Large Emitter Width. Photonics 2024, 11, 214. https://doi.org/10.3390/photonics11030214
Wang Y, Zhang Y, Wang J, Cheng Y, Zhao W, Wei Z, Zhou D. High-Power Mid-Infrared Quantum Cascade Laser with Large Emitter Width. Photonics. 2024; 11(3):214. https://doi.org/10.3390/photonics11030214
Chicago/Turabian StyleWang, Yupei, Yuhang Zhang, Jun Wang, Yang Cheng, Wu Zhao, Zhixiang Wei, and Dayong Zhou. 2024. "High-Power Mid-Infrared Quantum Cascade Laser with Large Emitter Width" Photonics 11, no. 3: 214. https://doi.org/10.3390/photonics11030214
APA StyleWang, Y., Zhang, Y., Wang, J., Cheng, Y., Zhao, W., Wei, Z., & Zhou, D. (2024). High-Power Mid-Infrared Quantum Cascade Laser with Large Emitter Width. Photonics, 11(3), 214. https://doi.org/10.3390/photonics11030214




