High-Directionality Silicon Nitride Antenna Based on Distributed Bragg Reflector for Optical Phased Array
Abstract
1. Introduction
2. Structure and Principle
3. Simulation and Optimizing
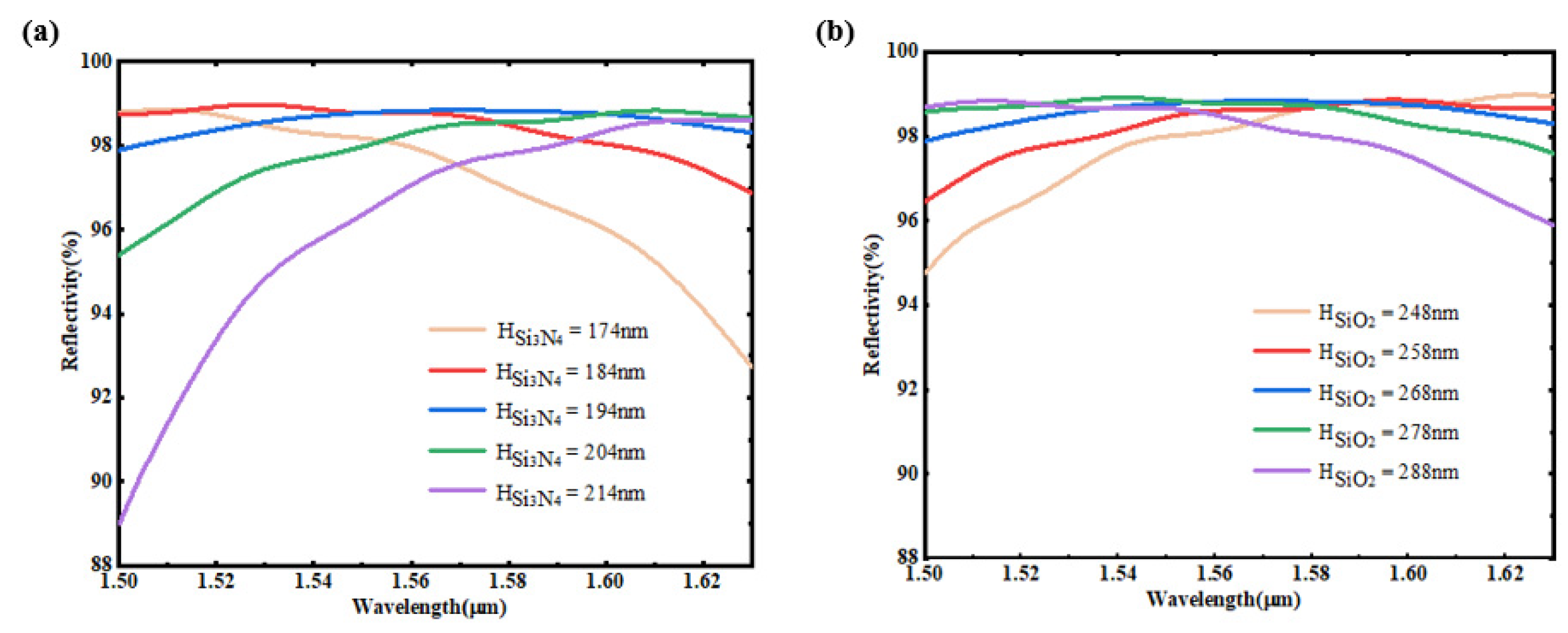
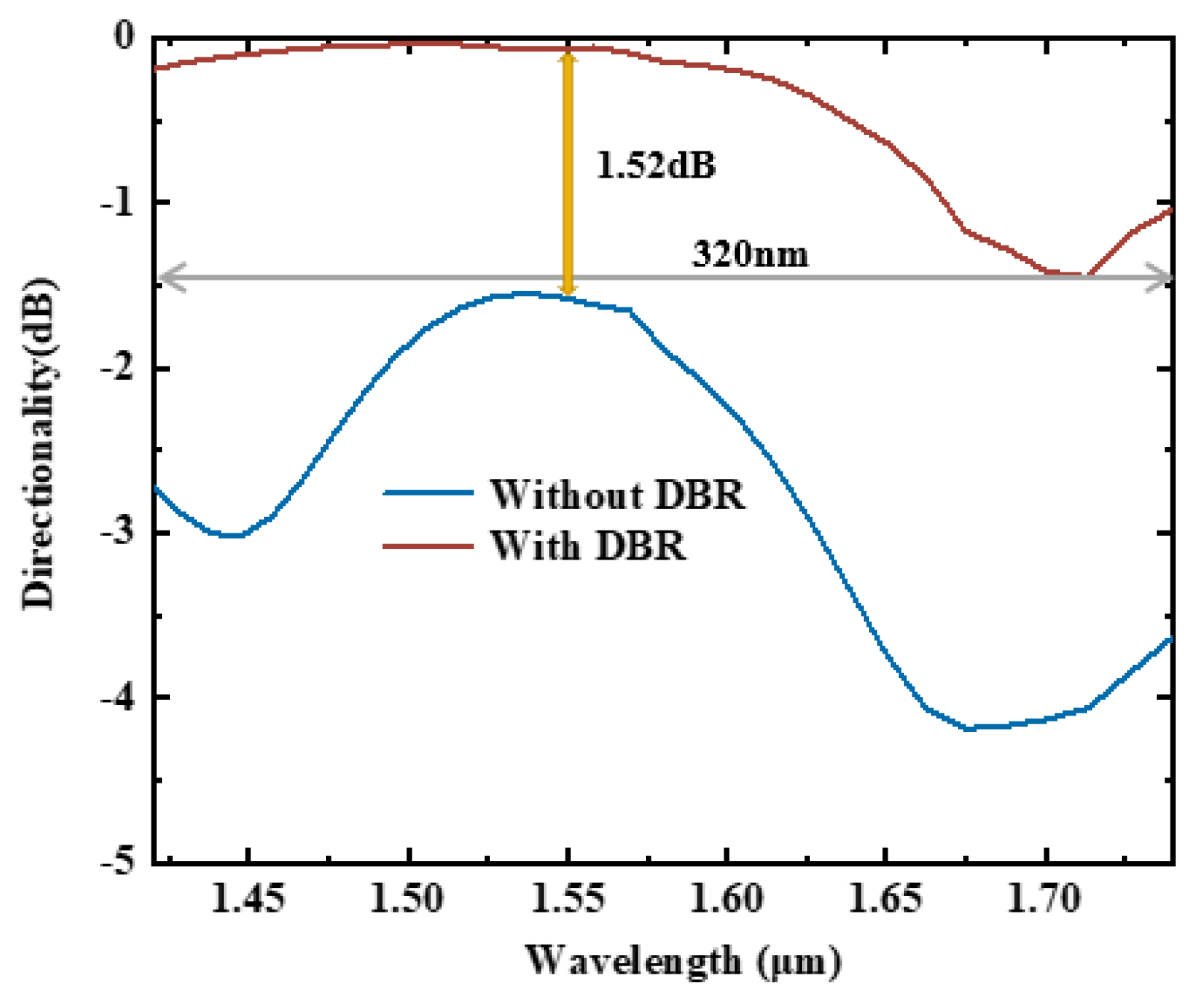
3.1. High Directionality Design
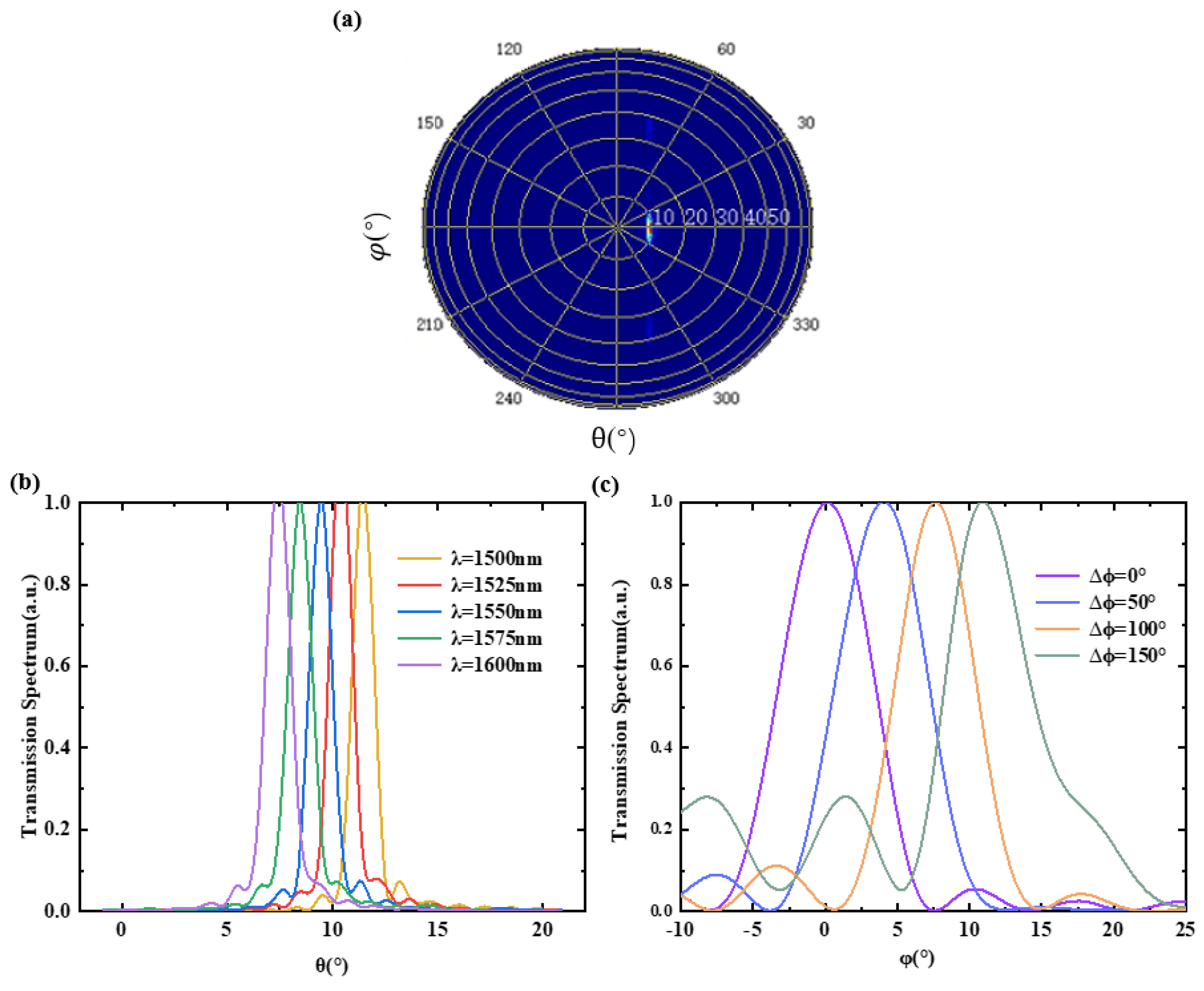
3.2. Array Sweep Characteristics
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Miller, S.A.; Chang, Y.C.; Phare, C.T.; Shin, M.C.; Zadka, M.; Roberts, S.P.; Stern, B.; Ji, X.; Mohanty, A.; Gordillo, O.A.J.; et al. Large-scale optical phased array using a low-power multi-pass silicon photonic platform. Optica 2020, 7, 3–6. [Google Scholar] [CrossRef]
- Chung, S.W.; Abediasl, H.; Hashemi, H. Monolithically integrated large-scale optical phased array in silicon-on-insulator CMOS. IEEE J. Solid-State Circuits 2018, 53, 275–296. [Google Scholar] [CrossRef]
- Hulme, J.C.; Doylend, J.K.; Heck, M.J.R.; Peters, J.D.; Davenport, M.L.; Bovington, J.T.; Coldren, L.A.; Bowers, J.E. Fully integrated hybrid silicon two dimensional beam scanner. Opt. Express 2015, 23, 5861–5874. [Google Scholar] [CrossRef]
- Xie, W.; Komljenovic, T.; Huang, J.; Tran, M.; Davenport, M.; Torres, A.; Pintus, P.; Bowers, J. Heterogeneous silicon photonics sensing for autonomous cars. Opt. Express 2019, 27, 3642–3663. [Google Scholar] [CrossRef] [PubMed]
- Hsu, C.P.; Li, B.; Solano Rivas, B.; Gohil, A.R.; Chan, P.H.; Moore, A.D.; Donzella, V. A review and perspective on optical phased array for automotive LiDAR. IEEE J. Sel. Top. Quantum Electron. 2021, 27, 8300416. [Google Scholar] [CrossRef]
- Poulton, C.V.; Byrd, M.J.; Russo, P.; Timurdogan, E.; Khandaker, M.; Vermeulen, D.; Watts, M.R. Long-range LiDAR and free-space data communication with high-performance optical phased arrays. IEEE J. Sel. Top. Quantum Electron. 2019, 25, 7700108. [Google Scholar] [CrossRef]
- Kohno, Y.; Komatsu, K.; Tang, R.; Ozeki, Y.; Nakano, Y.; Tanemura, T. Ghost imaging using a large-scale silicon photonic phased array chip. Opt. Express 2019, 27, 3817–3823. [Google Scholar] [CrossRef]
- Clevenson, H.A.; Spector, S.J.; Benney, L.; Moebius, M.G.; Brown, J.; Hare, A.; Huang, A.; Mlynarczyk, J.; Poulton, C.V.; Hosseini, E.; et al. Incoherent light imaging using an optical phased array. Appl. Phys. Lett. 2020, 116, 031105. [Google Scholar] [CrossRef]
- Sacher, W.D.; Chen, F.D.; Moradi-Chameh, H.; Liu, X.; Almog, I.F.; Lordello, T.; Chang, M.; Naderian, A.; Fowler, T.M.; Segev, E.; et al. Optical phased array neural probes for beam-steering in brain tissue. Opt. Lett. 2022, 47, 1073–1076. [Google Scholar] [CrossRef]
- Bharadwaj, P.; Deutsch, B.; Novotny, L. Optical antennas. Adv. Opt. Photonics 2009, 1, 438–483. [Google Scholar] [CrossRef]
- Guo, Y.; Guo, Y.; Li, C.; Zhang, H.; Zhou, X.; Zhang, L. Integrated optical phased arrays for beam forming and steering. Appl. Sci. 2021, 11, 4017–4058. [Google Scholar] [CrossRef]
- Kutila, M.; Pyykönen, P.; Holzhüter, H.; Colomb, M.; Duthon, P. Automotive LiDAR performance verification in fog and rain. In Proceedings of the 2018 IEEE International Conference on Intelligent Transportation Systems (ITSC), Maui, HI, USA, 4–7 November 2018; pp. 1695–1701. [Google Scholar]
- Marchetti, R.; Lacava, C.; Carroll, L.; Gradkowski, K.; Minzioni, P. Coupling strategies for silicon photonics integrated chips. Photonics Res. 2019, 7, 201–239. [Google Scholar] [CrossRef]
- Zhang, L.; Li, Y.; Hou, Y.; Wang, Y.; Tao, M.; Chen, B.; Na, Q.; Li, Y.; Zhi, Z.; Liu, X.; et al. Investigation and demonstration of a high-power handling and large-range steering optical phased array chip. Opt. Express. 2021, 29, 29755–29765. [Google Scholar] [CrossRef] [PubMed]
- Poulton, C.V.; Byrd, M.J.; Raval, M.; Su, Z.; Li, N.; Timurdogan, E.; Coolbaugh, D.; Vermeulen, D.; Watts, M.R. Large-scale silicon nitride nanophotonic phased arrays at infrared and visible wavelengths. Opt. Lett. 2017, 42, 21–24. [Google Scholar] [CrossRef] [PubMed]
- Im, C.S.; Bhandari, B.; Lee, K.P.; Kim, S.M.; Oh, M.C.; Lee, S.S. Silicon nitride optical phased array based on a grating antenna enabling wavelength-tuned beam steering. Opt. Express 2020, 28, 3270–3279. [Google Scholar] [CrossRef]
- Yu, L.; Liao, W.; Wang, P.; Ma, P.; Luo, G.; Cui, L.; Lv, C.; Zhang, Y.; Pan, J. Highly directional Si antenna based on dual-layer gratings for optical phased array. In Nanophotonics, Micro/Nano Optics, and Plasmonics VIII.; SPIE: Washington, DC, USA, 2023; pp. 1–6. [Google Scholar]
- Zaoui, W.S.; Rosa, M.F.; Vogel, W.; Berroth, M.; Butschke, J.; Letzkus, F. Cost-effective CMOS-compatible grating couplers with backside metal mirror and 69% coupling efficiency. Opt. Express 2012, 20, B238–B243. [Google Scholar] [CrossRef] [PubMed]
- Zou, J.; Yu, Y.; Ye, M.; Liu, L.; Deng, S.; Zhang, X. Ultra efficient silicon nitride grating coupler with bottom grating reflector. Opt. Express 2015, 23, 26305–26312. [Google Scholar] [CrossRef]
- Zhu, L.; Yang, W.; Chang-Hasnain, C. Very high efficiency optical coupler for silicon nanophotonic waveguide and single mode optical fiber. Opt. Express 2017, 25, 18462–18473. [Google Scholar] [CrossRef]
- Acoleyen, K.V.; Bogaerts, W.; Jana, J.; Thomas, N.L.; Romuald, H.; Baets, R. Off-chip beam steering with a one-dimensional optical phased array on silicon-on-insulator. Opt. Lett. 2009, 34, 1477–1479. [Google Scholar] [CrossRef]
- Hong, J.; Spring, A.M.; Qiu, F.; Yokoyama, S. A high efficiency silicon nitride waveguide grating coupler with a multilayer bottom reflector. Sci. Rep. 2019, 9, 12988. [Google Scholar] [CrossRef]
- Ma, P.; Luo, G.; Wang, P.; Ma, J.; Wang, R.; Yang, Z.; Zhou, X.; Zhang, Y.; Pan, J. Unidirectional SiN antenna based on dual-layer gratings for LiDAR with optical phased array. Opt. Commun. 2021, 501, 127361. [Google Scholar] [CrossRef]
- Wang, Q.; Wang, S.; Zeng, Y.; Wang, W.; Gai, Y.; Tu, Z.; Yue, W.; Wang, X.; Fang, Q.; Yu, M. Dual-layer waveguide grating antenna with high directionality for optical phased arrays. Appl. Opt. 2019, 58, 5807–5811. [Google Scholar] [CrossRef] [PubMed]
- Manan, R.; Poulton, C.V.; Watts, M.R. Unidirectional waveguide grating antennas with uniform emission for optical phased arrays. Opt. Lett. 2017, 42, 2563–2567. [Google Scholar]



| Ref. | Method | Highest Directionality | Wavelength Range |
| [23] | Dual-layer gratings antenna | 96.7% | 875–1100 nm |
| [24] | Dual-layer waveguide grating antenna | 90% | 1500–1600 nm |
| [25] | Two silicon nitride layers | 93% | 1550 nm |
| This work | Distributed Bragg reflector | 97.6% | 1420–1740 nm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wei, J.; Li, Y.; Zhang, Y.; Dai, T.; Li, X.; Zhang, X.; Wang, Y. High-Directionality Silicon Nitride Antenna Based on Distributed Bragg Reflector for Optical Phased Array. Photonics 2023, 10, 871. https://doi.org/10.3390/photonics10080871
Wei J, Li Y, Zhang Y, Dai T, Li X, Zhang X, Wang Y. High-Directionality Silicon Nitride Antenna Based on Distributed Bragg Reflector for Optical Phased Array. Photonics. 2023; 10(8):871. https://doi.org/10.3390/photonics10080871
Chicago/Turabian StyleWei, Jinmei, Yan Li, Yuejun Zhang, Tingge Dai, Xiangyu Li, Xiaowei Zhang, and Ying Wang. 2023. "High-Directionality Silicon Nitride Antenna Based on Distributed Bragg Reflector for Optical Phased Array" Photonics 10, no. 8: 871. https://doi.org/10.3390/photonics10080871
APA StyleWei, J., Li, Y., Zhang, Y., Dai, T., Li, X., Zhang, X., & Wang, Y. (2023). High-Directionality Silicon Nitride Antenna Based on Distributed Bragg Reflector for Optical Phased Array. Photonics, 10(8), 871. https://doi.org/10.3390/photonics10080871





