Generation of Photon Orbital Angular Momentum and Its Application in Space Division Multiplexing
Abstract
1. Introduction
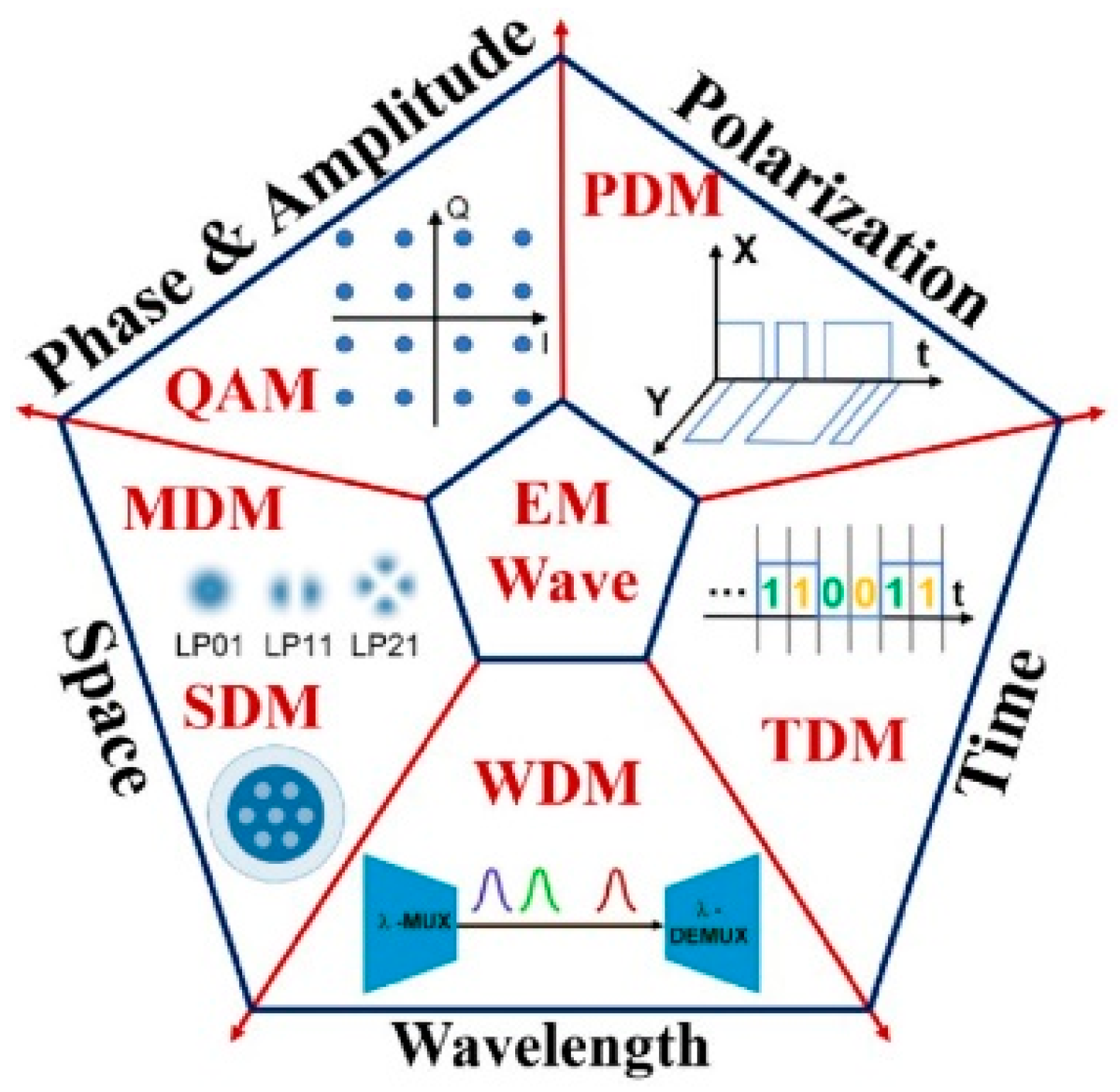
Roadmap of the Review
2. Background of OAM
2.1. OAM in High-Order Transmission
2.2. The Total Angular Momentum of a Photon with OAM
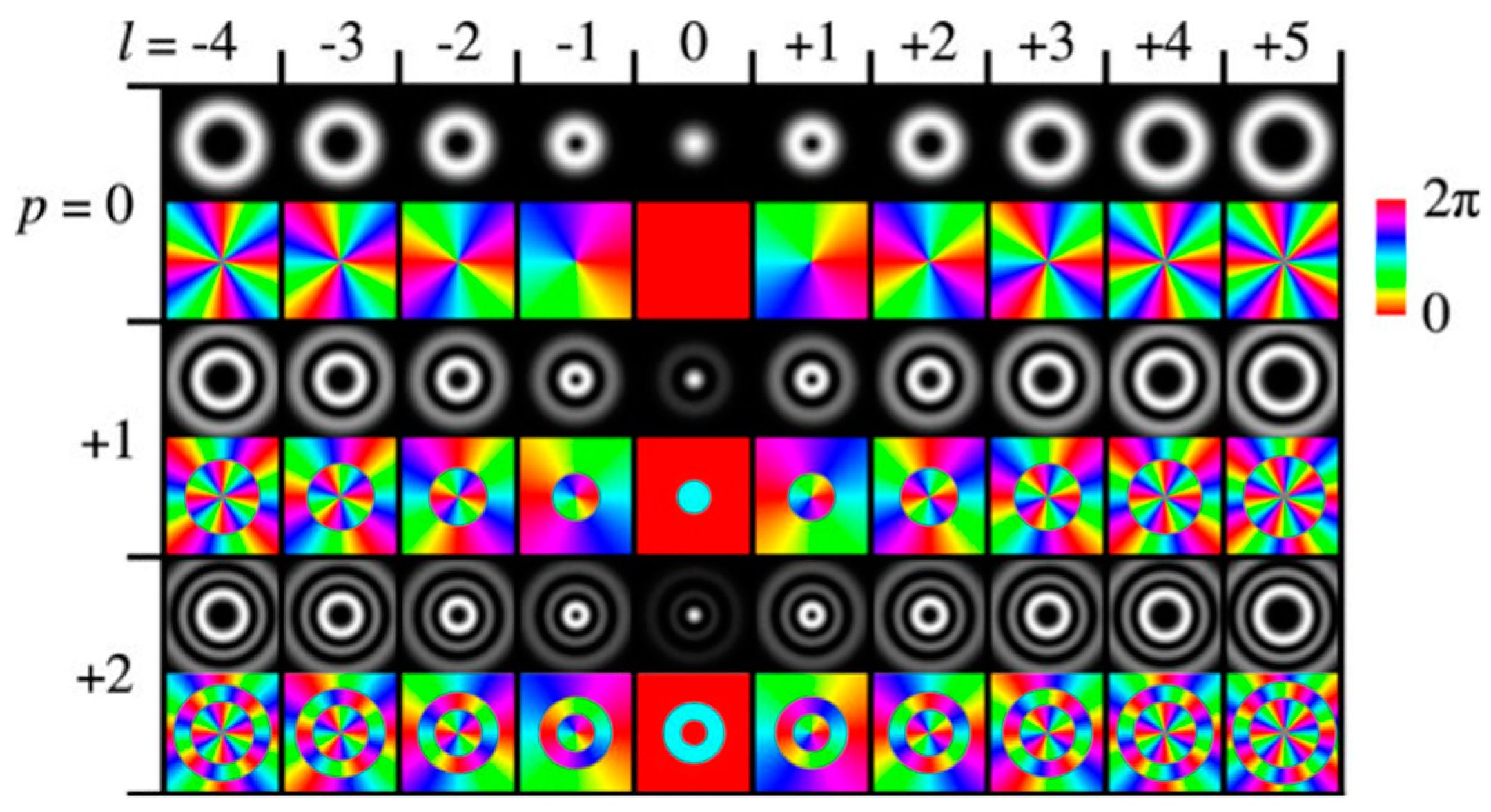
2.3. Laguerre-Gaussian Modes
3. Generation of OAM Beams
3.1. Spatial-Generating Methods
3.2. Fiber-Generating Methods
- (a)
- (b)
- Mode-selective couplers [132] used in both SMF and FMF;
- (c)
- (d)

3.3. Photonic Crystal Fibers (PCF)
- A circular PCF (C-PCF) supporting 26 OAM modes [149];
- A C-PCF with square air holes in the cladding that supports 46 OAM [150];
- A unique PCF with square and circular air holes (SC-PCF) that support 86 OAM modes [151];
- A pure-silica-based PCF with a central round air hole that supports 114 OAM modes was also designed showing higher effective refractive index difference, lower confinement loss, and non-linear coefficient [152];
- A PCF with an ssk2 dense crown glass ring with optimized central air hole radius and annular region thickness stably transmitted 394 OAM modes [153];
3.4. Measurement of OAM Modes
4. Multiplexing of Information-Carrying OAM Beams
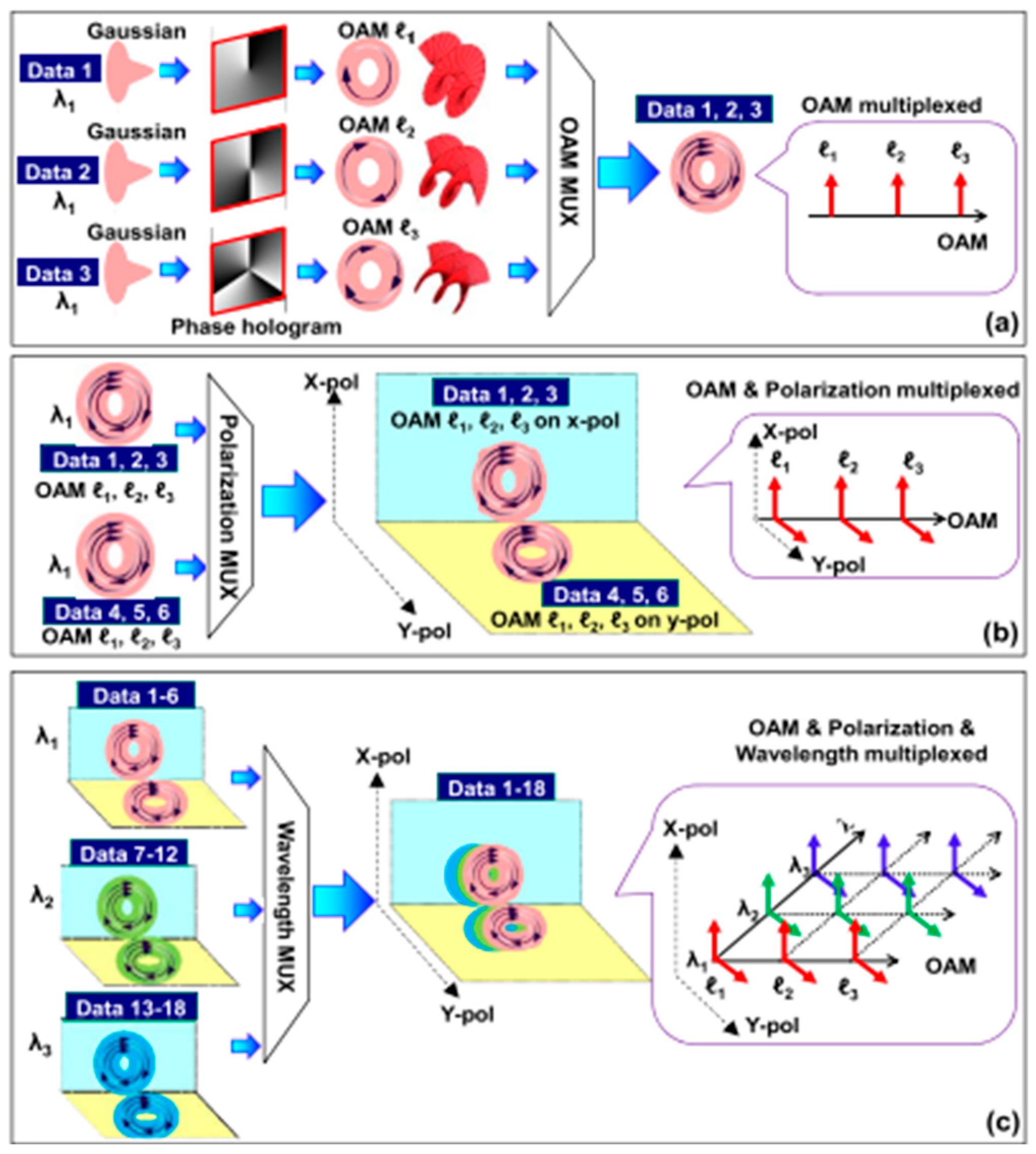
4.1. OAM in Space-Division Multiplexing
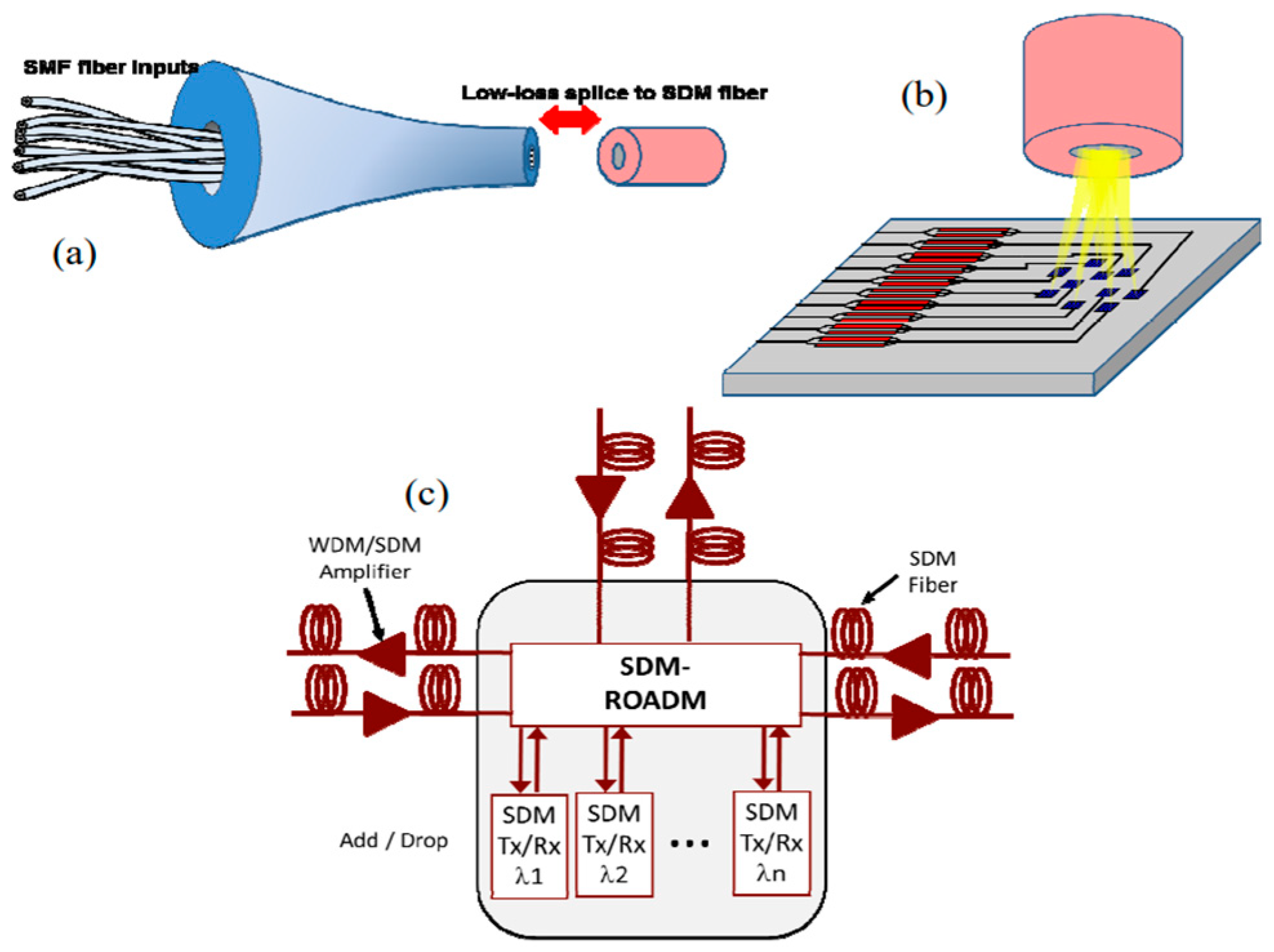
4.2. OAM-Compatible Infrastructures and Devices for Scaling
5. Summary and Outlook
5.1. Summary
5.2. Outlook and Future Perspectives
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Statista. Internet and Social Media Users in the World 2022. Available online: https://www.statista.com/statistics/617136/digital-population-worldwide/ (accessed on 20 December 2022).
- Cisco Annual Internet Report. White Paper Cisco Public. 2018. Available online: https://www.cisco.com/c/en/us/solutions/collateral/executive-perspectives/annual-internet-report/white-paper-c11-741490.html (accessed on 6 January 2023).
- Cisco Meraki Blog. 5 Apps That Hog Bandwidth on Your Network. Available online: https://meraki.cisco.com/blog/2011/08/5-apps-that-hog-bandwidth-on-your-network/ (accessed on 6 January 2023).
- Shannon, C.E. A Mathematical Theory of Communication. Bell Syst. Tech. J. 1948, 27, 379–423. [Google Scholar] [CrossRef]
- Ellis, A.D.; Zhao, J.; Cotter, D. Approaching the Non-Linear Shannon Limit. J. Lightwave Technol. 2010, 28, 423–433. [Google Scholar] [CrossRef]
- Essiambre, R.-J.; Kramer, G.; Winzer, P.J.; Foschini, G.J.; Goebel, B. Capacity Limits of Optical Fiber Networks. J. Lightwave Technol. 2010, 28, 662–701. [Google Scholar] [CrossRef]
- Kao, K.; Hockham, G. Dielectric-fibre surface waveguides for optical frequencies. Proc. Inst. Electr. Eng. 1966, 113, 1151–1158. [Google Scholar] [CrossRef]
- Ellis, D.; Suibhne, N.M.; Saad, D.; Payne, D.N. Communication networks beyond the capacity crunch. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2016, 374, 2062. [Google Scholar] [CrossRef]
- Winzer, P.J.; Neilson, D.T. From Scaling Disparities to Integrated Parallelism: A Decathlon for a Decade. J. Lightwave Technol. 2017, 35, 1099–1115. [Google Scholar] [CrossRef]
- Allen, L.; Beijersbergen, M.W.; Spreeuw, R.J.C.; Woerdman, J.P. Orbital angular momentum of light and the transformation of Laguerre-Gaussian laser modes. Phys. Rev. A 1992, 45, 8185–8189. [Google Scholar] [CrossRef]
- Padgett, M.; Bowman, R. Tweezers with a twist. Nat. Photonics 2011, 5, 343–348. [Google Scholar] [CrossRef]
- Dholakia, K.; Čižmár, T. Shaping the future of manipulation. Nat. Photonics 2011, 5, 335–342. [Google Scholar] [CrossRef]
- Moretti, D.; Felinto, D.; Tabosa, J.W.R. Storage and manipulation of orbital angular momentum of light in a cold atomic ensemble. In Proceedings of the CLEO/Europe-EQEC 2009-European Conference on Lasers and Electro-Optics and the European Quantum Electronics Conference, Munich, Germany, 14–19 June 2009. [Google Scholar] [CrossRef]
- Liu, X.J.; Liu, X.; Kwek, L.C.; Oh, C.H. Manipulating atomic states via optical orbital angular-momentum. Front. Phys. China 2008, 3, 113–125. [Google Scholar] [CrossRef]
- Toyoda, K.; Miyamoto, K.; Aoki, N.; Morita, R.; Omatsu, T. Using optical vortex to control the chirality of twisted metal nanostructures. Nano Lett. 2012, 12, 3645–3649. [Google Scholar] [CrossRef] [PubMed]
- Tamburini, F.; Anzolin, G.; Umbriaco, G.; Bianchini, A.; Barbieri, C. Overcoming the Rayleigh criterion limit with optical vortices. Phys. Rev. Lett. 2006, 97, 163903. [Google Scholar] [CrossRef]
- Ritsch-Marte, M.; Fürhapter, S.; Jesacher, A.; Bernet, S. Spiral interferometry. Opt. Lett. 2005, 30, 1953–1955. [Google Scholar] [CrossRef]
- Grillo, V.; Harvey, T.R.; Venturi, F.; Pierce, J.S.; Balboni, R.; Bouchard, F.; Gazzadi, G.C.; Frabboni, S.; Tavabi, A.H.; Li, Z.-A.; et al. Observation of nanoscale magnetic fields using twisted electron beams. Nat. Commun. 2017, 8, 689. Available online: https://sci-hub.ru/https://www.nature.com/articles/s41467-017-00829-5 (accessed on 26 January 2023). [CrossRef] [PubMed]
- Noguchi, Y.; Nakayama, S.; Ishida, T.; Saitoh, K.; Uchida, M. Efficient Measurement of the Orbital-Angular-Momentum Spectrum of an Electron Beam via a Dammann Vortex Grating. Phys. Rev. Appl. 2019, 12, 064062. [Google Scholar] [CrossRef]
- Noor, S.K.; Yasin, M.N.M.; Ismail, A.M.; Osman, M.N.; Soh, P.J.; Ramli, N.; Rambe, A.H. A Review of Orbital Angular Momentum Vortex Waves for the Next Generation Wireless Communications. IEEE Access 2022, 10, 89465–89484. [Google Scholar] [CrossRef]
- Lamilla, E.; Sacarelo, C.; Alvarez-Alvarado, M.S.; Pazmino, A.; Iza, P. Optical Encoding Model Based on Orbital Angular Momentum Powered by Machine Learning. Sensors 2023, 23, 2755. [Google Scholar] [CrossRef] [PubMed]
- Nagali, E.; Sciarrino, F. Manipulation of Photonic Orbital Angular Momentum for Quantum Information Processing. In Advanced Photonic Sciences; InTech: London, UK, 2012. [Google Scholar] [CrossRef]
- Zhu, J.; Wang, L.; Zhao, S. Orbital Angular Momentum Multiplexing Holography for Data Storage. IEEE Photonics Technol. Lett. 2023, 35, 179–182. [Google Scholar] [CrossRef]
- Ding, D.S.; Zhang, W.; Zhou, Z.-Y.; Shi, S.; Xiang, G.-Y.; Wang, X.-S.; Jiang, Y.-K.; Shi, B.-S.; Guo, G.-C. Quantum storage of orbital angular momentum entanglement in an atomic ensemble. Phys. Rev. Lett. 2015, 114, 050502. [Google Scholar] [CrossRef]
- Mcmanamon, P.; Vedadi, A.; Willner, A.E.; Choudhary, D.; Montifiore, N.; Harlev, O. High capacity and access rate, data storage using laser communications. Opt. Eng. 2021, 60, 015105. [Google Scholar] [CrossRef]
- Winzer, P.J.; Neilson, D.T.; Chraplyvy, A.R. Fiber-optic transmission and networking: The previous 20 and the next 20 years [Invited]. Opt. Express 2018, 26, 24190. [Google Scholar] [CrossRef]
- Zhang, Z.; Xiao, Y.; Ma, Z.; Xiao, M.; Ding, Z.; Lei, X.; Karagiannidis, G.K.; Fan, P. 6G Wireless Networks: Vision, Requirements, Architecture, and Key Technologies. IEEE Veh. Technol. Mag. 2019, 14, 28–41. [Google Scholar] [CrossRef]
- Akyildiz, F.I.; Kak, A.; Nie, S. 6G and Beyond: The Future of Wireless Communications Systems. IEEE Access 2020, 8, 133995–134030. [Google Scholar] [CrossRef]
- Larsson, E. Communication Systems. 1975. Available online: https://sci-hub.ru/https://commsys.isy.liu.se/MCSY-information-2022.pdf (accessed on 26 May 2023).
- Al-Amri, M.D.; El-Gomati, M.M.; Zubairy, M.S. Optics in Our Time; Springer International Publishing: Cham, Switzerland, 2016. [Google Scholar] [CrossRef]
- Schwartz, M. The Origins of Carrier Multiplexing: Major George Owen Squier and AT&T. IEEE Commun. Mag. 2008, 46, 20–24. [Google Scholar] [CrossRef]
- Squier, G.O. Multiplex Telephony and Telegraphy by Means of Electric Waves Guided by Wires. Proc. Am. Inst. Electr. Eng. 1911, 30, 857–905. [Google Scholar] [CrossRef]
- Hanzo, L.; Webb, W.T.; Keller, T. Single-and Multi-Carrier Quadrature Amplitude Modulation: Principles and Applications for Personal Communications, WLANs and Broadcasting; Wiley: Chichester, UK, 2000. [Google Scholar]
- Modern Quadrature Amplitude Modulation: Principles and Applications for Fixed and Wireless Channels. Available online: https://www.researchgate.net/publication/37530662_Modern_Quadrature_Amplitude_Modulation_Principles_and_applications_for_fixed_and_wireless_channels (accessed on 5 January 2023).
- Tucker, R.S.; Eisenstein, G.; Korotky, S.K. Optical time-division multiplexing for very high bit-rate transmission. J. Lightwave Technol. 1988, 6, 1737–1749. [Google Scholar] [CrossRef]
- Spirit, D.M.; Ellis, A.D.; Barnsley, P.E. Optical Time Division Multiplexing: Systems and Networks. IEEE Commun. Mag. 1994, 32, 56–62. [Google Scholar] [CrossRef]
- Chen, Z.Y.; Yan, L.S.; Pan, Y.; Jiang, L.; Yi, A.L.; Pan, W.; Luo, B. Use of polarization freedom beyond polarizationdivision multiplexing to support high-speed and spectral-efficient data transmission. Light. Sci. Appl. 2017, 6, e16207. [Google Scholar] [CrossRef] [PubMed]
- Brackett, C.A. Dense Wavelength Division Multiplexing Networks: Principles and Applications. IEEE J. Sel. Areas Commun. 1990, 8, 948–964. [Google Scholar] [CrossRef]
- Grobe, K.; Eiselt, M. Wavelength Division Multiplexing: A Practical Engineering Guide. Available online: https://www.perlego.com/book/1001511/wavelength-division-multiplexing-a-practical-engineering-guide-pdf (accessed on 5 January 2023).
- Li, G.; Bai, N.; Zhao, N.; Xia, C. Space-division multiplexing: The next frontier in optical communication. Adv. Opt. Photonics 2014, 6, 413. [Google Scholar] [CrossRef]
- Rademacher, G.; Luís, R.S.; Puttnam, B.J. Space-division multiplexing for optical fiber communications. Optica 2021, 8, 1186–1203. [Google Scholar] [CrossRef]
- Proakis, G.; Salehi, M. Digital Communications; McGraw-Hill: New York, NY, USA, 2008. [Google Scholar]
- Li, S.A.; Huang, H.; Pan, Z.; Yin, R.; Wang, Y.; Fang, Y.; Zhang, Y.; Bao, C.; Ren, Y.; Li, Z.; et al. Enabling Technology in High-Baud-Rate Coherent Optical Communication Systems. IEEE Access 2020, 8, 111318–111329. [Google Scholar] [CrossRef]
- Poynting, J.H. XV. On the transfer of energy in the electromagnetic field. Philos. Trans. R. Soc. Lond. 1884, 175, 343–361. [Google Scholar] [CrossRef]
- Beth, R.A. Mechanical Detection and Measurement of the Angular Momentum of Light. Phys. Rev. 1936, 50, 115–125. [Google Scholar] [CrossRef]
- Poynting, J.H. The wave motion of a revolving shaft, and a suggestion as to the angular momentum in a beam of circularly polarised light. Proc. R. Soc. Lond. Ser. A 1909, 82, 560–567. [Google Scholar] [CrossRef]
- Bangham, D.H.; Fakhoury, N. Notes on the theory of radiation. Proc. R. Soc. Lond. Ser. A 1932, 136, 36–52. [Google Scholar] [CrossRef]
- Kragh, H. Niels Bohr and the Quantum Atom; Oxford University Press: Oxford, UK, 2012. [Google Scholar] [CrossRef]
- Cohen-Tannoudji, C.; Dupont-roc, J.; Grynberg, G. Photons and Atoms: Introduction to Quantum Electrodynamics. Choice Rev. Online 1989, 27, 5805. [Google Scholar] [CrossRef]
- Nishijima. Quantum electrodynamics. J. Franklin Inst. 1965, 280, 451–452. [Google Scholar] [CrossRef]
- Zhang, F. An Evanescent Light Wave Can-not Possess a Transverse Spin. J. Mod. Phys. 2019, 10, 459–465. [Google Scholar] [CrossRef]
- van Enk, S.J.; Nienhuis, G. Spin and Orbital Angular Momentum of Photons. Europhys. Lett. (EPL) 1994, 25, 497–501. [Google Scholar] [CrossRef]
- Willner, E.; Song, H.; Zou, K.; Zhou, H.; Su, X. Orbital Angular Momentum Beams for High-Capacity Communications. J. Lightwave Technol. 2023, 41, 1918–1933. [Google Scholar] [CrossRef]
- Cao, J.; Chen, Q.; Guo, H. Creation of a controllable three dimensional optical chain by TEM01 mode radially polarized Laguerre–Gaussian beam. Optik 2013, 124, 2033–2036. [Google Scholar] [CrossRef]
- Nicolas, A.; Veissier, L.; Giacobino, E.; Maxein, D.; Laurat, J. Quantum state tomography of orbital angular momentum photonic qubits via a projection-based technique. New J. Phys. 2015, 17, 033037. [Google Scholar] [CrossRef]
- Yao, M.; Padgett, M.J. Orbital angular momentum: Origins, behavior and applications. Adv. Opt. Photonics 2011, 3, 161. [Google Scholar] [CrossRef]
- Sjöholm, J.; Palmer, K. Angular Momentum of Electromagnetic Radiation. Fundamental Physics Applied to the Radio Domain for Innovative Studies of Space and Development of New Concepts in Wireless Communications. May 2009. Available online: http://arxiv.org/abs/0905.0190 (accessed on 29 January 2023).
- Bai, X.; Jin, R.; Liu, L.; Geng, J.; Liang, X. Generation of OAM Radio Waves with Three Polarizations Using Circular Horn Antenna Array. Int. J. Antennas Propag. 2015, 2015, 132549. [Google Scholar] [CrossRef]
- Ajiki, H.; Ando, T. Aharonov-Bohm effect in carbon nanotubes. Phys. B Condens. Matter 1994, 201, 349–352. [Google Scholar] [CrossRef]
- García-Merino, J.A.; Mercado-Zúñiga, C.; Hernández-Acosta, M.A.; Aguilar-Pérez, L.A.; Villanueva-Fierro, I.; Hevia, S.A.; Torres-Torres, C. Magnetic frequency identification by quantum interference in magnetoplasmonic carbon/metal nanostructures. Mater. Sci. Eng. B 2021, 266, 115048. [Google Scholar] [CrossRef]
- Hurtado-Aviles, E.A.; Vila, M.; Vilatela, J.J.; Martines-Arano, H.; Bornacelli, J.; García-Merino, J.A.; Cervantes-Sodi, F.; Torres-Torres, C. Structured light using carbon nanostructures driven by Kerr nonlinearities and a magnetic field. Phys. Chem. Chem. Phys. 2022, 24, 1081–1090. [Google Scholar] [CrossRef]
- Kotlyar, V.V.; Kovalev, A.A.; Porfirev, A.P.; Kozlova, E.S. Three different types of astigmatic Hermite-Gaussian beams with orbital angular momentum. J. Opt. 2019, 21, 115601. [Google Scholar] [CrossRef]
- Volyar, A.; Bretsko, M.; Akimova, Y.; Egorov, Y. Measurement of the vortex and orbital angular momentum spectra with a single cylindrical lens. Appl. Opt. 2019, 58, 5748. [Google Scholar] [CrossRef]
- Pan, J.; Shen, Y.; Wan, Z.; Fu, X.; Zhang, H.; Liu, Q. Index-Tunable Structured-Light Beams from a Laser with an Intracavity Astigmatic Mode Converter. Phys. Rev. Appl. 2020, 14, 044048. [Google Scholar] [CrossRef]
- Lu, J.; Cao, C.; Zhu, Z.; Gu, B. Flexible measurement of high-order optical orbital angular momentum with a variable cylindrical lens pair. Appl. Phys. Lett. 2020, 116, 201105. [Google Scholar] [CrossRef]
- Zhou, J.; Zhang, W.; Chen, L. Experimental detection of high-order or fractional orbital angular momentum of light based on a robust mode converter. Appl. Phys. Lett. 2016, 108, 111108. [Google Scholar] [CrossRef]
- Massari, M.; Ruffato, G.; Gintoli, M.; Ricci, F.; Romanato, F. Fabrication and characterization of high-quality spiral phase plates for optical applications. Appl. Opt. 2015, 54, 4077. [Google Scholar] [CrossRef]
- Wei, W.; Mahdjoubi, K.; Brousseau, C.; Emile, O. Horn antennas for generating radio waves bearing orbital angular momentum by using spiral phase plate. IET Microw. Antennas Propag. 2016, 10, 1420–1427. [Google Scholar] [CrossRef]
- Stegenburgs, E.; Bertoncini, A.; Trichili, A.; Alias, M.S.; Ng, T.K.; Alouini, M.-S.; Liberale, C.; Ooi, B.S. Near-Infrared OAM Communication Using 3D-Printed Microscale Spiral Phase Plates. IEEE Commun. Mag. 2019, 57, 65–69. [Google Scholar] [CrossRef]
- Hui, X.; Zheng, S.; Hu, Y.; Xu, C.; Jin, X.; Chi, H.; Zhang, X. Ultralow Reflectivity Spiral Phase Plate for Generation of Millimeter-wave OAM Beam. IEEE Antennas Wirel. Propag. Lett. 2015, 14, 966–969. [Google Scholar] [CrossRef]
- Cheng, L.; Hong, W.; Hao, Z. Design and implementation of planar reflection spiral phase plate for beams with orbital angular momentum. Wiley Online Libr. 2017, 11, 260–264. [Google Scholar] [CrossRef]
- Chen, Y.; Zheng, S.; Li, Y.; Hui, X.; Jin, X.; Chi, H.; Zhang, X. A Flat-Lensed Spiral Phase Plate Based on Phase-Shifting Surface for Generation of Millimeter-Wave OAM Beam. IEEE Antennas Wirel. Propag. Lett. 2016, 15, 1156–1158. [Google Scholar] [CrossRef]
- Ruffato, G.; Massari, M.; Carli, M.; Romanato, F. Spiral phase plates with radial discontinuities for the generation of multiring orbital angular momentum beams: Fabrication, characterization, and application. Opt. Eng. 2015, 54, 111307. [Google Scholar] [CrossRef]
- Khonina, S.N.; Ustinov, A.V.; Logachev, V.I.; Porfirev, A.P. Properties of vortex light fields generated by generalized spiral phase plates. Phys. Rev. A 2020, 101, 043829. [Google Scholar] [CrossRef]
- Sabino, J. Orbital Angular Momentum of Photons: A Tool to Transmit Information, Technico Universidade de Lisboa, Lisboa, Portugal. 2016. Available online: https://fenix.tecnico.ulisboa.pt/downloadFile/844820067125161/Resumo.pdf (accessed on 1 May 2023).
- Fang, X.; Ren, H.; Gu, M. Orbital angular momentum holography for high-security encryption. Nat. Photonics 2019, 14, 102–108. [Google Scholar] [CrossRef]
- Ren, H.; Briere, G.; Fang, X.; Ni, P.; Sawant, R.; Héron, S.; Chenot, S.; Vézian, S.; Damilano, B.; Brändli, V.; et al. Metasurface orbital angular momentum holography. Nat. Commun. 2019, 10, 2986. [Google Scholar] [CrossRef]
- Zambale, G.; Doblado, N.H. OAM Beams from Incomplete Computer Generated Holograms. arXiv 2016, arXiv:1609.01791. [Google Scholar] [CrossRef]
- Zhong-Yi, G.; Shi-Liang, Q.; Zheng-He, S.; Shu-Tian, L. Superposition of orbital angular momentum of photons by a combined computer-generated hologram fabricated in silica glass with femtosecond laser pulses. Chin. Phys. B 2008, 17, 4199–4203. [Google Scholar] [CrossRef]
- Shikder, A.; Nishchal, N.K. Computer Generated Hologram Encryption Using Array of Vortex Beams. In Digital Holography and 3-D Imaging 2022; Optica Publishing Group: Washington, DC, USA, 2022; p. W2A.13. [Google Scholar] [CrossRef]
- Ruffato, G.; Rossi, R.; Massari, M.; Mafakheri, E.; Capaldo, P.; Romanato, F. Design, fabrication and characterization of Computer Generated Holograms for anti-counterfeiting applications using OAM beams as light decoders. Sci. Rep. 2017, 7, 18011. [Google Scholar] [CrossRef]
- Forbes, A.; Dudley, A.; McLaren, M. Creation and detection of optical modes with spatial light modulators. Adv. Opt. Photonics 2016, 8, 200. [Google Scholar] [CrossRef]
- Lian, Y.; Qi, X.; Wang, Y.; Bai, Z.; Wang, Y.; Lu, Z. OAM beam generation in space and its applications: A review. Opt. Lasers Eng. 2022, 151, 106923. [Google Scholar] [CrossRef]
- Thomas, G.M.; Minassian, A.; Damzen, M.J.; Rosales-Guzmán, C.; Ndagano, B.; Forbes, A.; Wu, J.; Liu, Y.; Zhang, D.; Pinnell, J.; et al. Probing the limits of orbital angular momentum generation and detection with spatial light modulators. J. Opt. 2021, 23, 15602–15611. [Google Scholar] [CrossRef]
- Cunha, A.; Figueira, G.; André, P. Enabling the study of photons orbital angular momentum for optical communications: Orbital angular momentum carrying beam modulation and demodulation using twsited nematic liquid crystal spatial light modulators. Opt. Quantum Electron. 2016, 48, 425. [Google Scholar] [CrossRef]
- Khonina, S.N.; Karpeev, S.V.; Butt, M.A. Spatial-light-modulator-based multichannel data transmission by vortex beams of various orders. Sensors 2021, 21, 2988. [Google Scholar] [CrossRef] [PubMed]
- Andersen, M.; Alperin, S.N.; Voitiv, A.A.; Holtzmann, W.G.; Gopinath, J.T.; Siemens, M.E. Characterizing vortex beams from a spatial light modulator with collinear phase-shifting holography. Appl. Opt. 2019, 58, 404. [Google Scholar] [CrossRef]
- Zhu, L.; Wang, J. Arbitrary manipulation of spatial amplitude and phase using phase-only spatial light modulators. Sci. Rep. 2014, 4, 7441. [Google Scholar] [CrossRef] [PubMed]
- Gregg, P.; Mirhosseini, M.; Rubano, A.; Marrucci, L.; Karimi, E.; Boyd, R.; Ramachandran, S. Q-Plates for Switchable Excitation of Fiber OAM Modes. In CLEO: 2015; OSA: Washington, DC, USA, 2015; p. SW4M.3. [Google Scholar] [CrossRef]
- Slussarenko, S.; Murauski, A.; Du, T.; Chigrinov, V.; Marrucci, L.; Santamato, E. Tunable liquid crystal q-plates with arbitrary topological charge. Opt. Express 2011, 19, 4085. [Google Scholar] [CrossRef] [PubMed]
- Marrucci, L. The q-plate and its future. J. Nanophotonics 2013, 7, 078598. Available online: https://sci-hub.ru/https://www.spiedigitallibrary.org/journals/journal-of-nanophotonics/volume-7/issue-1/078598/The-q-plate-and-its-future/10.1117/1.JNP.7.078598.short (accessed on 26 January 2023). [CrossRef]
- Karimi, E.; Piccirillo, B.; Nagali, E.; Marrucci, L.; Santamato, E. Efficient generation and sorting of orbital angular momentum eigenmodes of light by thermally tuned q-plates. Appl. Phys. Lett. 2009, 94, 231124. [Google Scholar] [CrossRef]
- Beijersbergen, M.W.; Allen, L.; van der Veen, H.E.L.O.; Woerdman, J.P. Astigmatic laser mode converters and transfer of orbital angular momentum. Opt. Commun. 1993, 96, 123–132. [Google Scholar] [CrossRef]
- Dong, M.; Yang, Y. Coherent Vortices Properties of Partially Coherent Elegant Laguerre-Gaussian Beams in the Free Space. Opt. Photonics J. 2020, 10, 159–166. [Google Scholar] [CrossRef]
- Ni, J.; Huang, C.; Zhou, L.M.; Gu, M.; Song, Q.; Kivshar, Y.; Qiu, C.W. Multidimensional phase singularities in nanophotonics. Science 2021, 374, eabj0039. [Google Scholar] [CrossRef]
- Li, C.-h.; Maier, S.A. REN Hao-ran Optical vortices in nanophotonics. Chin. Opt. 2021, 14, 792–811. [Google Scholar] [CrossRef]
- Kim, G.; Kim, S.; Kim, H.; Lee, J.; Badloe, T.; Rho, J. Metasurface-empowered spectral and spatial light modulation for disruptive holographic displays. Nanoscale 2022, 14, 4380. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.; Rusch, L.A.; Shi, W. Silicon Photonic Integrated Circuits for OAM Generation and Multiplexing; CRC Press: Boca Raton, FL, USA, 2021; Available online: https://sci-hub.ru/https://www.taylorfrancis.com/chapters/edit/10.1201/9780429292033-14/silicon-photonic-integrated-circuits-oam-generation-multiplexing-yuxuan-chen-leslie-rusch-wei-shi (accessed on 26 January 2023).
- Rui, G.; Gu, B.; Cui, Y.; Zhan, Q. Detection of orbital angular momentum using a photonic integrated circuit. Sci. Rep. 2016, 6, 28262. [Google Scholar] [CrossRef] [PubMed]
- Kutluyarov, R.; Fatkhiev, D.; Stepanov, I.; Grakhova, E.; Lyubopytov, V.; Sultanov, A. Design and modeling of a photonic integrated device for optical vortex generation in a silicon waveguide. Comput. Opt. 2021, 45, 324–330. [Google Scholar] [CrossRef]
- Song, H.; Zhou, H.; Zou, K.; Zhang, R.; Pang, K.; Song, H.; Su, X.; Minoofar, A.; Hu, N.; Liu, C.; et al. Demonstration of Recovering Orbital-Angular-Momentum Multiplexed Channels Using a Tunable, Broadband Pixel-Array-Based Photonic-Integrated-Circuit Receiver. J. Lightwave Technol. 2022, 40, 1346–1352. Available online: https://sci-hub.ru/https://opg.optica.org/abstract.cfm?uri=jlt-40-5-1346 (accessed on 26 January 2023). [CrossRef]
- Guan, B.; Scott, R.P.; Qin, C.; Fontaine, N.K.; Su, T.; Ferrari, C.; Cappuzzo, M.; Klemens, F.; Keller, B.; Earnshaw, M.; et al. Free-space coherent optical communication with orbital angular, momentum multiplexing/demultiplexing using a hybrid 3D photonic integrated circuit. Opt. Express 2014, 22, 145. [Google Scholar] [CrossRef]
- Alam, M.S.; Gnawali, R.; Hendrickson, J.R.; Beamer, D.; Payne, T.E.; Volk, A.; Agha, I. Photonic Integrated Circuit for Rapidly Tunable Orbital Angular Momentum Generation Using Sb2Se3 Ultra-Low-Loss Phase Change Material. Adv. Opt. Mater. 2022, 10, 2200098. [Google Scholar] [CrossRef]
- Li, S.; Klitis, C.; Nong, Z.; Gao, S.; Sorel, M.; Yu, S.; Cai, X. Orbital Angular Momentum Mode Multiplexer Based on Multimode Micro-Ring Resonator with Angular Gratings. In Proceedings of the 2016 Conference on Lasers and Electro-Optics (CLEO), Washington, DC, USA, 5–10 June 2016; p. STh3E.5. [Google Scholar] [CrossRef]
- Fatkhiev, M.; Lyubopytov, V.S.; Kutluyarov, R.V.; Sultanov, A.K. OAM signal emitter based on a ring resonator. In Optical Technologies for Telecommunications 2019; Burdin, V.A., Andreev, V.A., Morozov, O.G., Bourdine, A.V., Sultanov, A.H., Eds.; SPIE: Washington, DC, USA, 2020; p. 71. [Google Scholar] [CrossRef]
- Lin, W.; Ota, Y.; Arakawa, Y.; Iwamoto, S. An On-chip Full Poincaré Beam Emitter Based on an Optical Micro-ring Cavity. In Proceedings of the Conference on Lasers and Electro-Optics, San Jose, CA, USA, 5–10 May 2019; OSA: Washington, DC, USA, 2019. Paper SW4J.4. [Google Scholar] [CrossRef]
- Zhou, H.; Song, H.; Zhao, Z.; Zhang, R.; Song, H.; Pang, K.; Zou, K.; Liu, C.; Su, X.; Hu, N.; et al. Modal properties of a beam carrying OAM generated by a circular array of multiple ring-resonator emitters. Opt. Lett. 2021, 46, 4722. [Google Scholar] [CrossRef]
- Yuan, G.; Cao, S.; Zhang, P.; Peng, F.; Peng, Z.; Wang, Z. Achieving wide-range photonics applications based on a compact grating-assisted silicon micro-ring resonator. Optik 2019, 183, 887–896. [Google Scholar] [CrossRef]
- Li, S.; Ding, Y.; Guan, X.; Tan, H.; Nong, Z.; Wang, L.; Liu, L.; Zhou, L.; Yang, C.; Yvind, K.; et al. Compact high-efficiency vortex beam emitter based on a silicon photonics micro-ring. Opt. Lett. 2018, 43, 1319. [Google Scholar] [CrossRef]
- Li, S.; Nong, Z.; Wu, X.; Yu, W.; He, M.; Klitis, C.; Zhu, Y.; Gao, S.; Liu, J.; Li, Z.; et al. Orbital angular momentum vector modes (de)multiplexer based on multimode micro-ring. Opt. Express 2018, 26, 29895. [Google Scholar] [CrossRef]
- Stepanov, I.V.; Fatkhiev, D.M.; Lyubopytov, V.S.; Kutluyarov, R.V.; Grakhova, E.P.; Neumann, N.; Khonina, S.N.; Sultanov, A.K. Wavelength-tunable vortex beam emitter based on silicon micro-ring with pn depletion diode. Sensors 2022, 22, 929. [Google Scholar] [CrossRef] [PubMed]
- Sun, Z.; Yan, M.; Xu, B. Formation of orbital angular momentum and focused beams based on chiral double-helical metamaterials. Opt. Mater. 2020, 107, 109962. [Google Scholar] [CrossRef]
- Ma, Q.; Cui, T.J. Information Metamaterials: Bridging the physical world and digital world. PhotoniX 2020, 1, 1. [Google Scholar] [CrossRef]
- Jin, J.; Pu, M.; Wang, Y.; Li, X.; Ma, X.; Luo, J.; Zhao, Z.; Gao, P.; Luo, X. Multi-Channel Vortex Beam Generation by Simultaneous Amplitude and Phase Modulation with Two-Dimensional Metamaterial. Adv. Mater. Technol. 2017, 2, 1600201. [Google Scholar] [CrossRef]
- Chen, R.; Zhou, H.; Moretti, M.; Wang, X.; Li, J. Orbital Angular Momentum Waves: Generation, Detection, and Emerging Applications. IEEE Commun. Surv. Tutor. 2020, 22, 840–868. [Google Scholar] [CrossRef]
- Meng, Z.K.; Shi, Y.; Wei, W.Y.; Zhang, Y.; Li, L. Graphene-based metamaterial transmitarray antenna design for the generation of tunable orbital angular momentum vortex electromagnetic waves. Opt. Mater. Express 2019, 9, 3709. [Google Scholar] [CrossRef]
- Sun, Z.C.; Yan, M.Y.; Xu, B.J. Generation of orbital angular momentum and focused beams with tri-layer medium metamaterial. Chin. Phys. B 2020, 29, 104101. [Google Scholar] [CrossRef]
- Shi, Y.; Zhang, Y. Generation of Wideband Tunable Orbital Angular Momentum Vortex Waves Using Graphene Metamaterial Reflectarray. IEEE Access 2018, 6, 5341–5347. [Google Scholar] [CrossRef]
- Zhao, Z.; Wang, J.; Li, S.; Willner, A.E. Metamaterials-based broadband generation of orbital angular momentum carrying vector beams. Opt. Lett. 2013, 38, 932. [Google Scholar] [CrossRef]
- Zhao, Z.; Ren, Y.; Xie, G.; Yan, Y.; Li, L.; Huang, H.; Bao, C.; Ahmed, N.; Lavery, M.P.; Zhang, C.; et al. Experimental demonstration of 16-Gbit/s millimeter-wave communications link using thin metamaterial plates to generate data-carrying orbital-angular-momentum beams. In Proceedings of the 2015 IEEE International Conference on Communications (ICC), London, UK, 8–12 June 2015; pp. 1392–1397. [Google Scholar] [CrossRef]
- Spektor, G.; Kilbane, D.; Mahro, A.K.; Frank, B.; Ristok, S.; Gal, L.; Kahl, P.; Podbiel, D.; Mathias, S.; Giessen, H.; et al. Revealing the subfemtosecond dynamics of orbital angular momentum in nanoplasmonic vortices. Science 2017, 355, 1187–1191. [Google Scholar] [CrossRef]
- Genevet, P.; Lin, J.; Kats, M.A.; Capasso, F. Holographic Detection of the Orbital Angular Momentum of Light with Plasmonic Photodiodes. Nat. Commun. 2012, 3, 1278. Available online: https://sci-hub.ru/https://www.nature.com/articles/ncomms2293 (accessed on 17 May 2023). [CrossRef] [PubMed]
- Prinz, E.; Hartelt, M.; Spektor, G.; Orenstein, M.; Aeschlimann, M. Orbital Angular Momentum in Nanoplasmonic Vortices. ACS Photonics 2023, 10, 340–367. [Google Scholar] [CrossRef]
- Kim, H.; Park, J.; Cho, S.W.; Lee, S.Y.; Kang, M.; Lee, B. Synthesis and dynamic switching of surface plasmon vortices with plasmonic vortex lens. Nano Lett. 2010, 10, 529–536. [Google Scholar] [CrossRef] [PubMed]
- Chen, M.; Jiang, L.; Sha, W. Orbital Angular Momentum Generation and Detection by Geometric-Phase Based Metasurfaces. Appl. Sci. 2018, 8, 362. [Google Scholar] [CrossRef]
- Qin, F.; Wan, L.; Li, L.; Zhang, H.; Wei, G.; Gao, S. A Transmission Metasurface for Generating OAM Beams. IEEE Antennas Wirel. Propag. Lett. 2018, 17, 1793–1796. [Google Scholar] [CrossRef]
- Bai, X.; Liang, X.-L.; Jiao, S.; Jin, R.; Kong, F.; Sun, Y.; Wang, G.; Qian, J.; Li, X.; Cao, A.; et al. High-efficiency transmissive programmable metasurface for multimode OAM generation. Adv. Opt. Mater. 2020, 8, 2000570. [Google Scholar] [CrossRef]
- Ahmed, H.; Kim, H.; Zhang, Y.; Intaravanne, Y.; Jang, J.; Rho, J.; Chen, S.; Chen, X. Optical metasurfaces for generating and manipulating optical vortex beams. Nanophotonics 2022, 11, 941–956. [Google Scholar] [CrossRef]
- Ma, M.; Lian, Y.; Wang, Y.; Lu, Z. Generation, Transmission and Application of Orbital Angular Momentum in Optical Fiber: A Review. Front. Phys. 2021, 9, 773505. [Google Scholar] [CrossRef]
- Han, Y.; Liu, Y.G.; Wang, Z.; Huang, W.; Chen, L.; Zhang, H.W.; Yang, K. Controllable all-fiber generation/conversion of circularly polarized orbital angular momentum beams using long period fiber gratings. Nanophotonics 2018, 7, 287–293. [Google Scholar] [CrossRef]
- Yao, S.; Ren, G.; Shen, Y.; Jiang, Y.; Zhu, B.; Jian, S. Tunable Orbital Angular Momentum Generation Using All-Fiber Fused Coupler. IEEE Photonics Technol. Lett. 2018, 30, 99–102. [Google Scholar] [CrossRef]
- Pidishety, S.; Pachava, S.; Gregg, P.; Ramachandran, S.; Brambilla, G.; Srinivasan, B. Orbital angular momentum beam excitation using an all-fiber weakly fused mode selective coupler. Opt. Lett. 2017, 42, 4347. [Google Scholar] [CrossRef] [PubMed]
- Eznaveh, Z.S.; Pachava, S.; Gregg, P.; Ramachandran, S.; Brambilla, G.; Srinivasan, B. Annular Core Photonic Lantern OAM Mode Multiplexer. In Proceedings of the Optical Fiber Communication Conference, Los Angeles, CA, USA, 19–23 March 2017; OSA: Washington, DC, USA, 2017. Paper Tu3J.3. [Google Scholar] [CrossRef]
- Eznaveh, Z.S.; Zacarias, J.C.A.; Lopez, J.E.A.; Shi, K.; Milione, G.; Jung, Y.; Thomsen, B.C.; Richardson, D.J.; Fontaine, N.; Leon-Saval, S.G.; et al. Photonic lantern broadband orbital angular momentum mode multiplexer. Opt. Express 2018, 26, 30042. [Google Scholar] [CrossRef] [PubMed]
- Wei, W.; An, N.; Zhang, Z.; Tang, L.; Ding, L.; Li, Y. Design of a microstructure optical fiber supporting 52 vortex beams. Opt. Commun. 2021, 490, 126657. [Google Scholar] [CrossRef]
- Huang, W.; Liu, Y.; Wang, Z.; Zhang, W.; Luo, M.; Liu, X.; Guo, J.; Liu, B.; Lin, L. Generation and excitation of different orbital angular momentum states in a tunable microstructure optical fiber. Opt. Express 2015, 23, 33741. [Google Scholar] [CrossRef] [PubMed]
- Xu, M.; Zhou, G.; Chen, C.; Zhou, G.; Sheng, Z.; Hou, Z.; Xia, C.A. A novel micro-structured fiber for OAM mode and LP mode simultaneous transmission. J. Opt. 2018, 47, 428–436. [Google Scholar] [CrossRef]
- Chang, W.; Feng, M.; Mao, B.; Wang, P.; Wang, Z.; Liu, Y.-G. All-Fiber Fourth-Order OAM Mode Generation Employing a Long Period Fiber Grating Written By Preset Twist. J. Lightwave Technol. 2022, 40, 4804–4811. [Google Scholar] [CrossRef]
- Wang, T.; Wang, F.; Shi, F.; Pang, F.; Huang, S.; Wang, T.; Zeng, X. Generation of Femtosecond Optical Vortex Beams in All-Fiber Mode-Locked Fiber Laser Using Mode Selective Coupler. J. Lightwave Technol. 2017, 35, 2161–2166. [Google Scholar] [CrossRef]
- Lu, Y.; Jiang, Z.; Liu, W.; Liu, Q.; Jiang, M.; Jiang, K. Orbital angular momentum mode generation system based on photonic lantern. J. Opt. 2019, 21, 125702. [Google Scholar] [CrossRef]
- Arman, H.; Olyaee, S.; Mohebzadeh-Bahabady, A. Gas sensor based on large hollow-core photonic bandgap fiber. IJOP 2015, 9, 99–106. Available online: https://sci-hub.ru/https://ijop.ir/article-1-209-en.html (accessed on 31 March 2023).
- Birks, A.; Witkowska, A.; Leon-Saval, S.G.; Pham, A. All-fiber LP11 mode convertors. Opt. Lett. 2008, 33, 306–308. [Google Scholar] [CrossRef]
- Wong, G.K.L.; Xi, X.M.; Kang, M.S.; Lee, H.W.; Russell, P.S.J. Quantization of orbital angular momentum in helically twisted photonic crystal fiber. In Proceedings of the Frontiers in Optics 2012/Laser Science XXVIII (2012), Rochester, NY, USA, 14–18 October 2012. Paper FTh3C.3. [Google Scholar] [CrossRef]
- Lian, Y.; Yu, Y.; Han, S.; Luan, N.; Wang, Y.; Lu, Z. OAM Beams Generation Technology in Optical Fiber: A Review. IEEE Sens. J. 2022, 22, 3828–3843. [Google Scholar] [CrossRef]
- Russel, P.S.J. Photonic-Crystal Fibers. J. Lightwave Technol. 2006, 24, 4729–4749. [Google Scholar] [CrossRef]
- Russell, P. Photonic Crystal Fibers. Science 2003, 299, 358–362. [Google Scholar] [CrossRef] [PubMed]
- Bhattacharya, R. Generation of phase singular optical beams in microstructure optical fibers. Opt. Commun. 2018, 428, 15–21. [Google Scholar] [CrossRef]
- Al-Zahrani, F.A.; Kabir, A. Ring-core photonic crystal fiber of terahertz orbital angular momentum modes with excellence guiding properties in optical fiber communication. Photonics 2021, 8, 122. [Google Scholar] [CrossRef]
- Tian, W.; Zhang, H.; Zhang, X.; Xi, L.; Zhang, W.; Tang, X.A. circular photonic crystal fiber supporting 26 OAM modes. Opt. Fiber Technol. 2016, 30, 184–189. [Google Scholar] [CrossRef]
- Bai, X.; Chen, H.; Yang, H. Design of a circular photonic crystal fiber with square air-holes for orbital angular momentum modes transmission. Optik 2018, 158, 1266–1274. [Google Scholar] [CrossRef]
- Yu, Y.; Lian, Y.; Hu, Q.; Xie, L.; Ding, J.; Wang, Y.; Lu, Z. Design of PCF Supporting 86 OAM Modes with High Mode Quality and Low Nonlinear Coefficient. Photonics 2022, 9, 266. [Google Scholar] [CrossRef]
- Liu, Q.; Tai, S.; Lu, W.; Sun, J.; Lv, T.; Liu, C.; Sun, Y.; Lv, J.; Liu, W.; Sun, T.; et al. Design of pure silica-based photonic crystal fiber for supporting 114 OAM modes transmission. J. Opt. 2021, 23, 095701. [Google Scholar] [CrossRef]
- Fu, H.; Zhu, M.; Liu, C.; Yi, Z.; Lv, J.; Yang, L.; Wang, F.; Liu, Q.; Su, W.; Li, X.; et al. Photonic crystal fiber supporting 394 orbital angular momentum modes with flat dispersion, low nonlinear coefficient, and high mode quality. Opt. Eng. 2022, 61, 026111. [Google Scholar] [CrossRef]
- Zhao, L.; Zhao, H.; Xu, Z.; Liang, R. A design of novel photonic crystal fiber with low and flattened dispersion for supporting 84 orbital angular momentum modes. Commun. Theor. Phys. 2021, 73, 085501. [Google Scholar] [CrossRef]
- Zhang, L.; Zhang, K.; Peng, J.; Deng, J.; Yang, Y.; Ma, J. Circular photonic crystal fiber supporting 110 OAM modes. Opt. Commun. 2018, 429, 189–193. [Google Scholar] [CrossRef]
- Liu, Q.; Tai, S.; Sun, Y.; Han, M.; Wang, J.; Liu, C.; Lv, J.; Wang, S.; Sun, G.; Chu, P.K. Simple ring-structured photonic crystal fiber with low nonlinear coefficients and flat dispersion supporting 166 OAM modes. Opt. Eng. 2022, 61, 076108. [Google Scholar] [CrossRef]
- Wang, W.; Wang, N.; Li, K.; Geng, Z.; Jia, H. A novel dual guided modes regions photonic crystal fiber with low crosstalk supporting 56 OAM modes and 4 LP modes. Opt. Fiber Technol. 2020, 57, 102213. [Google Scholar] [CrossRef]
- Harris, M.; Hill, C.A.; Tapster, P.R.; Vaughan, J.M. Laser modes with helical wave fronts. Phys. Rev. A 1994, 49, 3119–3122. [Google Scholar] [CrossRef] [PubMed]
- Lv, F.; Li, X.; Tai, Y.; Zhang, L.; Nie, Z.; Chen, Q. High-order topological charges measurement of LG vortex beams with a modified Mach–Zehnder interferometer. Optik 2015, 126, 4378–4381. [Google Scholar] [CrossRef]
- Dai, K.; Gao, C.; Zhong, L.; Na, Q.; Wang, Q. Measuring OAM states of light beams with gradually-changing-period gratings. Opt. Lett. 2015, 40, 562–565. [Google Scholar] [CrossRef] [PubMed]
- Leach, J.; Padgett, M.J.; Barnett, S.M.; Franke-Arnold, S.; Courtial, J. Measuring the Orbital Angular Momentum of a Single Photon. Phys. Rev. Lett. 2002, 88, 4. [Google Scholar] [CrossRef] [PubMed]
- Zheng, S.; Wang, J. Measuring Orbital Angular Momentum (OAM) States of Vortex Beams with Annular Gratings. Sci. Rep. 2017, 7, 40781. [Google Scholar] [CrossRef] [PubMed]
- Liu, K.; Li, X.; Cheng, Y.; Gao, Y.; Fan, B.; Jiang, Y. OAM-Based Multitarget Detection: From Theory to Experiment. IEEE Microw. Wirel. Compon. Lett. 2017, 27, 760–762. [Google Scholar] [CrossRef]
- Yan, Y.; Xie, G.; Lavery, M.P.J.; Huang, H.; Ahmed, N.; Bao, C.; Ren, Y.; Cao, Y.; Li, L.; Zhao, Z. High-capacity millimetre-wave communications with orbital angular momentum multiplexing. Nat. Commun. 2014, 5, 4876. Available online: https://www.nature.com/articles/ncomms5876 (accessed on 26 May 2023). [CrossRef] [PubMed]
- Berkhout, G.C.G.; Lavery, M.P.J.; Courtial, J.; Beijersbergen, M.W.; Padgett, M.J. Efficient sorting of orbital angular momentum states of light. Phys. Rev. 2010, 105, 153601. [Google Scholar] [CrossRef]
- Ren, Y.; Wang, Z.; Liao, P.; Li, L.; Xie, G.; Huang, H.; Zhao, Z.; Yan, Y.; Ahmed, N.; Willner, A.; et al. Experimental characterization of a 400 Gbit/s orbital angular momentum multiplexed free-space optical link over 120 m. Opt. Lett. 2015, 41, 622–625. [Google Scholar] [CrossRef] [PubMed]
- Krenn, M.; Handsteiner, J.; Fink, M.; Fickler, R.; Ursin, R.; Malik, M.; Zeilinger, A. Twisted light transmission over 143 km. Proc. Natl. Acad. Sci. USA 2016, 113, 13648–13653. [Google Scholar] [CrossRef] [PubMed]
- Sakaguchi, J.; Awaji, Y.; Wada, N.; Kanno, A.; Kawanishi, T.; Hayashi, T.; Taru, T.; Kobayashi, T.; Watanabe, M. Space Division Multiplexed Transmission of 109-Tb/s Data Signals Using Homogeneous Seven-Core Fiber. J. Lightwave Technol. 2012, 30, 658–665. [Google Scholar] [CrossRef]
- Sakaguchi, J.; Puttnam, B.J.; Klaus, W.; Awaji, Y.; Wada, N.; Kanno, A.; Kawanishi, T.; Imamura, K.; Inaba, H.; Mukasa, K.; et al. 305 Tb/s Space Division Multiplexed Transmission Using Homogeneous 19-Core Fiber. J. Lightwave Technol. 2013, 31, 554–562. [Google Scholar] [CrossRef]
- Nejabati, R.; Shu, Y.; Puttnam, B.J.; Klaus, W.; Wada, N.; Awaji, Y.; Channegowda, M.; Amaya, N.; Harai, H.; Ou, Y.; et al. First Demonstration of Software Defined Networking (SDN) over Space Division Multiplexing (SDM) Optical Networks. In Proceedings of the 39th European Conference and Exhibition on Optical Communication (ECOC 2013), London, UK, 22–26 September 2013; pp. 1263–1265. [Google Scholar] [CrossRef]
- Sakaguchi, J.; Puttnam, B.J.; Klaus, W.; Awaji, Y.; Wada, N.; Kanno, A.; Kawanishi, T.; Imamura, K.; Inaba, H.; Mukasa, K.; et al. 19-core fiber transmission of 19×100×172-Gb/s SDM-WDM-PDM-QPSK signals at 305Tb/s. In Proceedings of the Optical Fiber Communication Conference, Los Angeles, CA, USA, 4–8 March 2012; OSA: Washington, DC, USA, 2012. paper PDP5C.1. [Google Scholar] [CrossRef]
- Puttnam, B.J.; Luis, R.S.; Klaus, W.; Sakaguchi, J.; Delgado Mendinueta, J.-M.; Awaji, Y.; Wada, N.; Tamura, Y.; Hayashi, T.; Hirano, M.; et al. 2.15 Pb/s transmission using a 22 core homogeneous single-mode multi-core fiber and wideband optical comb. In Proceedings of the 2015 European Conference on Optical Communication (ECOC), Valencia, Spain, 27 September–1 October 2015; pp. 1–3. [Google Scholar] [CrossRef]
- Jin, X.Q.; Li, R.; O’Brien, D.C.; Payne, F.P. Linearly polarized mode division multiplexed transmission over ring-index multimode fibres. In Proceedings of the 2013 IEEE Photonics Society Summer Topical Meeting Series, PSSTMS 2013, Waikoloa, HI, USA, 8–10 July 2013; pp. 113–114. [Google Scholar] [CrossRef]
- Karelin, N.; Louchet, H.; Kroushkov, D.; Uvarov, A.; Mingaleev, S.; Koltchanov, I.; Richter, A. Modeling and design framework for SDM transmission systems. In Proceedings of the International Conference on Transparent Optical Networks, Budapest, Hungary, 5–9 July 2015. [Google Scholar] [CrossRef]
- Ryf, R.; Randel, S.; Gnauck, A.H.; Bolle, C.; Essiambre, R.J.; Winzer, P.J.; Peckham, D.W.; McCurdy, A.; Lingle, J. Space-division multiplexing over 10 km of three-mode fiber using coherent 6 × 6 MIMO processing. In Proceedings of the Optical Fiber Communication Conference/National Fiber Optic Engineers Conference 2011, Los Angeles, CA, USA, 6–10 March 2011. Paper PDPB10. [Google Scholar] [CrossRef]
- Rjeb, A.; Fathallah, H.; Machhout, M. OAM Modes in Optical Fibers for Next Generation Space Division Multiplexing (SDM) Systems. In Fiber Optics-Technology and Applications; IntechOpen: London, UK, 2021. [Google Scholar] [CrossRef]
- Saridis, G.M.; Alexandropoulos, D.; Zervas, G.; Simeonidou, D. Survey and evaluation of space division multiplexing: From technologies to optical networks. IEEE Commun. Surv. Tutor. 2015, 17, 2136–2156. [Google Scholar] [CrossRef]
- Fontaine, K. Integrated photonics and components for space-division multiplexing systems. In Proceedings of the 2014 13th International Conference on Optical Communications and Networks, ICOCN 2014, Suzhou, China, 9–10 November 2014. [Google Scholar] [CrossRef]
- Bozinovic, N.; Yue, Y.; Ren, Y.; Tur, M.; Kristensen, P.; Huang, H.; Willner, A.E.; Ramachandran, S. Terabit-scale orbital angular momentum mode division multiplexing in fibers. Science 2013, 340, 1545–1548. [Google Scholar] [CrossRef]
- Willner, E.; Zhao, Z.; Liu, C.; Zhang, R.; Song, H.; Pang, K.; Manukyan, K.; Song, H.; Su, X.; Xie, G.; et al. Perspectives on advances in high-capacity, free-space communications using multiplexing of orbital-angular-momentum beams. APL Photonics 2021, 6, 030901. [Google Scholar] [CrossRef]
- Wang, J.; Yang, J.-Y.; Fazal, I.M.; Ahmed, N.; Yan, Y.; Huang, H.; Ren, Y.; Yue, Y.; Dolinar, S.; Tur, M.; et al. Terabit free-space data transmission employing orbital angular momentum multiplexing. Nat. Photonics 2012, 6, 488–496. [Google Scholar] [CrossRef]
- Huang, H.; Xie, G.; Yan, Y.; Ahmed, N.; Ren, Y.; Yue, Y.; Rogawski, D.; Willner, M.J.; Erkmen, B.I.; Birnbaum, K.M.; et al. 100 Tbit/s Free-Space Data Link Enabled by Three-Dimensional Multiplexing of Orbital Angular Momentum, Polarization, and Wavelength. Opt. Lett. 2014, 39, 197. [Google Scholar] [CrossRef] [PubMed]
- Klaus, W.; Sakaguchi, J.; Puttnam, B.J.; Awaji, Y.; Wada, N.; Kobayashi, T.; Watanabe, M. Free-Space Coupling Optics for Multicore Fibers. IEEE Photonics Technol. Lett. 2012, 24, 1902–1905. [Google Scholar] [CrossRef]
- Weng, Y.; He, X.; Pan, Z. Space division multiplexing optical communication using few-mode fibers. Opt. Fiber Technol. 2017, 36, 155–180. [Google Scholar] [CrossRef]
- Matsuo, S.; Sasaki, Y.; Ishida, I.; Takenaga, K.; Saitoh, K.; Koshiba, M. Recent Progress on Multi-Core Fiber and Few-Mode Fiber. In Proceedings of the Optical Fiber Communication Conference/National Fiber Optic Engineers Conference 2013, Anaheim, CA, USA, 17–21 March 2013; OSA: Washington, DC, USA, 2013; p. 3. [Google Scholar] [CrossRef]
- Winzer, J. Energy-Efficient Optical Transport Capacity Scaling Through Spatial Multiplexing. IEEE Photonics Technol. Lett. 2011, 23, 851–853. [Google Scholar] [CrossRef]
- Zhao, Z.; Ren, Y.; Xie, G.; Ahmed, N.; Ashrafi, N.; Lavery, M.P.J.; Ramachandran, S.; Ashrafi, S.; Willner, A.E.; Molisch, A.F.; et al. Optical communications using orbital angular momentum beams. Adv. Opt. Photonics 2015, 7, 66–106. [Google Scholar] [CrossRef]
- Wu, G.; Wu, G.; Wu, X.; Wu, X.; Gao, S.; Gao, S.; Tu, J.; Tu, J.; Zhou, J.; Sui, Q.; et al. Multi-channel higher-order OAM generation and switching based on a mode selective interferometer. Opt. Express 2022, 30, 25093–25102. [Google Scholar] [CrossRef]
- Erhard, M.; Fickler, R.; Krenn, M.; Zeilinger, A. Twisted photons: New quantum perspectives in high dimensions. Light Sci. Appl. 2017, 7, 17146. [Google Scholar] [CrossRef]
- Molisch, F.; Win, M.Z.; Choi, Y.S.; Winters, J.H. Capacity of MIMO systems with antenna selection. IEEE Trans. Wirel. Commun. 2005, 4, 1759–1771. [Google Scholar] [CrossRef]
- Goldsmith, A.; Jafar, S.; Jindal, N.; Vishwanath, S. Capacity limits of MIMO channels. IEEE J. Sel. Areas Commun. 2003, 21, 684–702. [Google Scholar] [CrossRef]
- Ke, X.; Ning, C.; Wang, J. Crosstalk analysis of orbital angular momentum-multiplexed state under atmospheric turbulence. Infrared Laser Eng. 2018, 47, 1122002. [Google Scholar] [CrossRef]
- Li, S.; Wang, J. Multi-Orbital-Angular-Momentum Multi-Ring Fiber for High-Density Space-Division Multiplexing. IEEE Photonics J. 2013, 5, 7101007. [Google Scholar] [CrossRef]
- Winzer, P.J. Making spatial multiplexing a reality. Nat. Photonics 2014, 8, 345–348. [Google Scholar] [CrossRef]
- Li, W.; Zhao, S. Orbital angular momentum filter based on multiple-beam interference. Opt. Commun. 2019, 430, 98–103. [Google Scholar] [CrossRef]
- Wang, J. Twisted optical communications using orbital angular momentum. Sci. China-Phys. Mech. Astron. 2019, 62, 34201. [Google Scholar] [CrossRef]
- Kareem, F.Q.; Zeebaree, S.R.M.; Dino, H.I.; M Sadeeq, M.A.; Rashid, Z.N.; Hasan, D.A.; Sharif, K.H.A. A Survey of Optical Fiber Communications: Challenges and Processing Time Influences. Asian J. Res. Comput. Sci. 2021, 7, 48–58. [Google Scholar] [CrossRef]
- Richardson, D.J.; Fini, J.M.; Nelson, L.E. Space-division multiplexing in optical fibres. Nat. Photonics 2013, 7, 354–362. [Google Scholar] [CrossRef]
- PROTEUS. Cailabs: Spatial Mode Multiplexer for Use in SDM. Available online: https://www.cailabs.com/en/products/proteus/?hsCtaTracking=fa8c0b4e-2124-464f-b5d4-43f48c442b16%7C7edc995b-d3e2-475c-96de-dc2a40a7dda4 (accessed on 30 March 2023).
- Sasaki, H.; Yagi, Y.; Yamada, T.; Lee, D. Field Experimental Demonstration on OAM-MIMO Wireless Transmission on 28 GHz Band. In Proceedings of the 2019 IEEE Globecom Workshops (GC Wkshps), Waikoloa, HI, USA, 9–13 December 2019; pp. 1–4. [Google Scholar] [CrossRef]
- NEC. NEC Successfully Demonstrates New Digital OAM Mode Multiplexing Transmission: Press Releases. Available online: https://www.nec.com/en/press/201812/global_20181219_02.html (accessed on 18 May 2023).
- Sasaki, H.; Yagi, Y.; Yamada, T.; Semoto, T.; Lee, D. An Experimental Demonstration of over 100 Gbit/s OAM Multiplexing Transmission at a Distance of 100 m on 40 GHz Band. In Proceedings of the 2020 IEEE International Conference on Communications Workshops, Dublin, Ireland, 7–11 June 2020. [Google Scholar] [CrossRef]
- Brunet, C.; Rusch, L.A. Optical fibers for the transmission of orbital angular momentum modes. Opt. Fiber Technol. 2017, 35, 2–7. [Google Scholar] [CrossRef]
- Shen, Y.; Meng, Y.; Fu, X.; Gong, M. Wavelength-tunable Hermite–Gaussian modes and an orbital-angular-momentum-tunable vortex beam in a dual-off-axis pumped Yb: CALGO laser. Opt. Lett. 2018, 43, 291–294. [Google Scholar] [CrossRef]
- Lin, D.; Feng, Y.; Ren, Z.; Richardson, D.J. The generation of femtosecond optical vortex beams with megawatt powers directly from a fiber based Mamyshev oscillator. Nanophotonics 2022, 11, 847–854. [Google Scholar] [CrossRef]
- Forbes, A. Advances in Orbital Angular Momentum Lasers. J. Lightwave Technol. 2022, 41, 2079–2086. [Google Scholar] [CrossRef]
- Geberbauer, J.W.T.; Kerridge-Johns, W.R.; Damzen, M.J. >30 W vortex LG01 or HG10 laser using a mode transforming output coupler. Opt. Express 2021, 29, 29082. [Google Scholar] [CrossRef] [PubMed]
- Shukla, M.; Gupta, S. Joint WDM and OAM Mode Group Multiplexed Transmission Over Conventional Multimode Fiber. IEEE Photonics J. 2023, 15, 7201109. [Google Scholar] [CrossRef]
- Liu, Z.; Gao, S.; Lai, Z.; Li, Y.; Ao, Z.; Li, J.; Tu, J.; Wu, Y.; Liu, W.; Li, Z. Broadband, Low-Crosstalk, and Massive-Channels OAM Modes De/Multiplexing Based on Optical Diffraction Neural Network. Laser Photonics Rev. 2023, 17, 2200536. [Google Scholar] [CrossRef]
- Jian, Y.-H.; Chow, C.-W. Design and analysis of a compact micro-ring resonator signal emitter to reduce the uniformity-induced phase distortion and crosstalk in orbital angular momentum (OAM) division multiplexing. Opt. Express 2023, 31, 810. [Google Scholar] [CrossRef] [PubMed]




| Angular Momentum | SAM | OAM |
|---|---|---|
| Wavefront polarization | Circularly polarized waves | Helically phased waves |
| Angular momentum per photon | Σ lħ | |
| Photon state number |
| Spatial Generation Methods | Cylindrical Lens | Spiral Phase Plate | Phase Hologram | Spatial Light Modulator | Q-Plates | Metamaterials |
|---|---|---|---|---|---|---|
| Cost | Normal | low | Low | High | high | Low |
| OAM modes generated | Single | Single | Single | Single/Multiple | Single | Single |
| Flexibility | Low | Low | Low | High | High | Low |
| Transmission distance | Short | Short | Short | Short | Short | Short |
| Is it passive? | Yes | Yes | Yes | Yes | Yes | Yes |
| Can it withstand high power? | Yes | Yes | No | No | Yes | No |
| Processing difficulty | Low | Low | High | High | Low | High |
| Does it enable space division multiplexing? | No | No | No | No | No | No |
| Fiber-Generating Method | Advantages | Disadvantages |
|---|---|---|
| Fiber gratings | Compatibility with existing fiber structure; highly stable and robust, low loss | Limited OAM mode selection due to design and fabrication limitations; reduced mode purity in high-order modes; limited bandwidth |
| Mode selective couplers | High mode purity; design flexibility to generate different OAM modes for various applications; wide bandwidth | Complexity in fabrication, leading to high cost and limited scalability; sensitive to misalignment |
| Photonic lanterns | Compatibility with existing fiber structure; efficient mode conversion; mode flexibility | Complexity in fabrication, leading to high cost and limited scalability; coupling losses can cause reduced OAM purity |
| Photonic crystal fibers (PCF) | High mode purity; supports multiple modes across a wide bandwidth; compact | Complex design and fabrication; high losses due to complex waveguide design |
| Method of Generation | Advantages | Disadvantages | Application |
|---|---|---|---|
| Spatial-generation methods | Beams can be shaped and manipulated with great versatility; advanced beam steering capabilities and control over beam direction; a wide range of applications | Vulnerability to environmental influences such as atmospheric turbulence, scattering, and absorption results in beam variations, effectiveness, and distortions. Alignment challenges between transmitting and receiving systems | Free-space optical communication; imaging; sensing; quantum information processing; interferometry; micromanipulations |
| Fiber-generation methods | Compatible with the existing fiber optic communication networks; robust and stable with minimal beam distortions; high data capability due to scalability in transmitting multiple OAM beams | Complexity in design and fabrication can result in high cost; inefficient coupling impacts mode purity and results in transmission loss; high modal crosstalk | Optical fiber communications; optical imaging and sensing; fiber laser |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Olaleye, T.M.; Ribeiro, P.A.; Raposo, M. Generation of Photon Orbital Angular Momentum and Its Application in Space Division Multiplexing. Photonics 2023, 10, 664. https://doi.org/10.3390/photonics10060664
Olaleye TM, Ribeiro PA, Raposo M. Generation of Photon Orbital Angular Momentum and Its Application in Space Division Multiplexing. Photonics. 2023; 10(6):664. https://doi.org/10.3390/photonics10060664
Chicago/Turabian StyleOlaleye, Temitope M., Paulo A. Ribeiro, and Maria Raposo. 2023. "Generation of Photon Orbital Angular Momentum and Its Application in Space Division Multiplexing" Photonics 10, no. 6: 664. https://doi.org/10.3390/photonics10060664
APA StyleOlaleye, T. M., Ribeiro, P. A., & Raposo, M. (2023). Generation of Photon Orbital Angular Momentum and Its Application in Space Division Multiplexing. Photonics, 10(6), 664. https://doi.org/10.3390/photonics10060664







