Fiber-Based Techniques to Suppress Stimulated Brillouin Scattering
Abstract
1. Introduction
2. Theoretical Background and Design Rationale
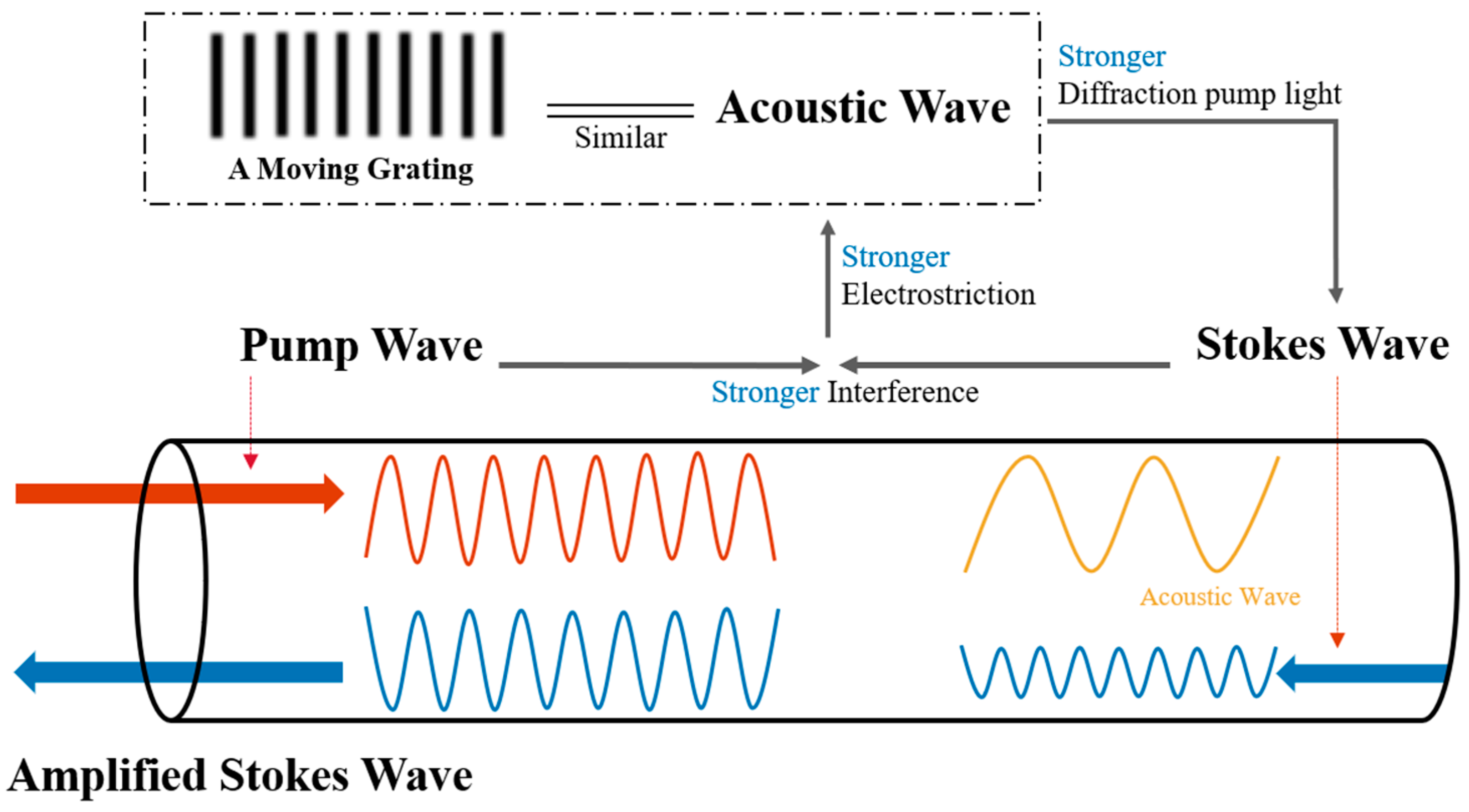
2.1. Theoretical Background of Brillouin Scattering in Optical Fibers
2.2. Fiber-Based Technique of SBS Suppression Rationale
3. Fiber-Based Techniques for SBS Suppression
3.1. Transverse Optical Fiber Design
3.1.1. Large Mode Area Fiber
3.1.2. Acoustic Tailoring
3.2. Longitudinal Variant Fiber Design
3.2.1. Concatenated Optical Fibers
3.2.2. Tapered Optical Fibers
3.3. External Perturbations (Temperature and Strain)
3.3.1. Temperature Distribution on Optical Fibers
3.3.2. Strain Distribution on Optical Fibers
3.4. Combined Techniques
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gross, E. Change of wave-length of light due to elastic heat waves at scattering in liquids. Nature 1930, 126, 201–202. [Google Scholar] [CrossRef]
- Raman, C.V. A change of wave-length in light scattering. Nature 1928, 121, 619. [Google Scholar] [CrossRef]
- McClung, F.J.; Hellwarth, R.W. Giant optical pulsations from ruby. Appl. Opt. 1962, 1, 103–105. [Google Scholar] [CrossRef]
- Woodbury, E.J.; Ng, W.K.; Co, H.A.; Calif, C.C. Ruby Laser Opeartion in the Near IR. Proc. IRE 1962, 50, 2367. [Google Scholar]
- Chiao, R.Y.; Townes, C.H.; Stoicheff, B.P. Stimulated Brillouin scattering and coherent generation of intense hypersonic waves. Phys. Rev. Lett. 1964, 12, 592. [Google Scholar] [CrossRef]
- Sundar, V.; Newnham, R.E. Electrostriction. In The Electrical Engineering Handbook, 2nd ed.; Dorf, R.C., Ed.; CRC Press: Boca Raton, FL, USA, 1997; pp. 1193–1200. [Google Scholar]
- Ippen, E.P.; Stolen, R.H. Stimulated Brillouin scattering in optical fibers. Appl. Phys. Lett. 1972, 21, 539–541. [Google Scholar] [CrossRef]
- Smith, R.G. Optical power handling capacity of low loss optical fibers as determined by stimulated Raman and Brillouin scattering. Appl. Opt. 1972, 11, 2489–2494. [Google Scholar] [CrossRef]
- Buckland, E.L.; Boyd, R.W. Electrostrictive contribution to the intensity-dependent refractive index of optical fibers. Opt. Lett. 1996, 21, 1117–1119. [Google Scholar] [CrossRef]
- Buckland, E.L. Mode-profile dependence of the electrostrictive response in fibers. Opt. Lett. 1999, 24, 872–874. [Google Scholar] [CrossRef]
- Dianov, E.M.; Sukharev, M.E.; Biriukov, A.S. Electrostrictive response in single-mode ring-index-profile fibers. Opt. Lett. 2000, 25, 390–392. [Google Scholar] [CrossRef]
- Townsend, P.D.; Poustie, A.J.; Hardman, P.J.; Blow, K.J. Measurement of the refractive-index modulation generated by electrostriction-induced acoustic waves in optical fibers. Opt. Lett. 1996, 21, 333–335. [Google Scholar] [CrossRef] [PubMed]
- Buckland, E.L.; Boyd, R.W. Measurement of the frequency response of the electrostrictive nonlinearity in optical fibers. Opt. Lett. 1997, 22, 676–678. [Google Scholar] [CrossRef] [PubMed]
- Melloni, A.; Frasca, M.; Garavaglia, A.; Tonini, A.; Martinelli, M. Direct measurement of electrostriction in optical fibers. Opt. Lett. 1998, 23, 691–693. [Google Scholar] [CrossRef] [PubMed]
- Biryukov, A.S.; Sukharev, M.E.; Dianov, E.M. Excitation of sound waves upon propagation of laser pulses in optical fibers. Quantum Electron. 2002, 32, 765. [Google Scholar] [CrossRef]
- Biryukov, A.S.; Erokhin, S.V.; Kushchenko, S.V.; Dianov, E.M. Electrostriction temporal shift of laser pulses in optical fibers. Quantum Electron. 2004, 34, 1047. [Google Scholar] [CrossRef]
- Van Deventer, M.O.; Boot, A.J. Polarization properties of stimulated Brillouin scattering in single-mode fibers. J. Light. Technol. 1994, 12, 585–590. [Google Scholar] [CrossRef]
- Engan, H.E. Analysis of polarization-mode coupling by acoustic torsional waves in optical fibers. JOSA A 1996, 13, 112–118. [Google Scholar] [CrossRef]
- Imai, Y.; Yoshida, M. Polarization characteristics of fiber-optic SBS phase conjugation. Opt. Fiber Technol. 2000, 6, 42–48. [Google Scholar] [CrossRef]
- Ferreira, M.F.; Rocha, J.F.; Pinto, J.L. Analysis of the gain and noise characteristics of fibre Brillouin amplifiers. Opt. Quant. Electron. 1994, 26, 35–44. [Google Scholar] [CrossRef]
- Schneider, T.; Hannover, D.; Junker, M. Investigation of Brillouin scattering in optical fibers for the generation of millimeter waves. J. Light. Technol. 2006, 24, 295. [Google Scholar] [CrossRef]
- Hill, K.O.; Kawasaki, B.S.; Johnson, D.C. CW Brillouin laser. Appl. Phys. Lett. 1976, 28, 608–609. [Google Scholar] [CrossRef]
- Stokes, L.F.; Chodorow, M.; Shaw, H.J. All-fiber stimulated Brillouin ring laser with submilliwatt pump threshold. Opt. Lett. 1982, 7, 509–511. [Google Scholar] [CrossRef] [PubMed]
- Smith, S.P.; Zarinetchi, F.; Ezekiel, S. Narrow-linewidth stimulated Brillouin fiber laser and applications. Opt. Lett. 1991, 16, 393–395. [Google Scholar] [CrossRef] [PubMed]
- Bayvel, P.; Giles, I.P. Linewidth narrowing in semiconductor laser pumped all-fibre Brillouin ring laser. Electron. Lett. 1989, 4, 260–262. [Google Scholar] [CrossRef]
- Grudinin, I.S.; Matsko, A.B.; Maleki, L. Brillouin lasing with a CaF2 whispering gallery mode resonator. Phys. Rev. Lett. 2009, 102, 043902. [Google Scholar] [CrossRef]
- Pant, R.; Poulton, C.; Choi, D.-Y.; McFarlane, H.; Hile, S.; Li, E.; Thevenaz, L.; Luther-Davies, B.; Madden, S.J.; Eggleton, B.J. On-chip stimulated Brillouin scattering. Opt. Express 2011, 19, 8285–8290. [Google Scholar] [CrossRef]
- Li, J.; Lee, H.; Chen, T.; Vahala, K.J. Characterization of a high coherence, Brillouin microcavity laser on silicon. Opt. Express 2012, 20, 20170–20180. [Google Scholar] [CrossRef]
- Carr, I.D.; Hanna, D.C. Performance of a Nd: YAG oscillator/ampflifier with phase-conjugation via stimulated Brillouin scattering. Appl. Phys. B 1985, 36, 83–92. [Google Scholar] [CrossRef]
- Andreev, N.F.; Khazanov, E.A.; Pasmanik, G.A. Applications of Brillouin cells to high repetition rate solid-state lasers. IEEE J. Quantum Electron. 1992, 28, 330–341. [Google Scholar] [CrossRef]
- Dane, C.B.; Zapata, L.E.; Neuman, W.A.; Norton, M.A.; Hackel, L.A. Design and operation of a 150 W near diffraction-limited laser amplifier with SBS wavefront correction. IEEE J. Quantum Electron. 1995, 31, 148–163. [Google Scholar] [CrossRef]
- Sumida, D.S.; Jones, D.C.; Rockwell, D.A. An 8.2 J phase-conjugate solid-state laser coherently combining eight parallel amplifiers. IEEE J. Quantum Electron. 1994, 30, 2617–2627. [Google Scholar] [CrossRef]
- Kong, H.J.; Lee, J.Y.; Shin, Y.S.; Byun, J.O.; Park, H.S.; Kim, H. Beam Recombination Characteristics in Array Laser Amplification Using Stimulated Brillouin Scattering Phase Conjugation. Opt. Rev. 1997, 4, 277–283. [Google Scholar] [CrossRef]
- Omatsu, T.; Kong, H.J.; Park, S.; Cha, S.; Tsubakimoto, K.; Fujita, N.; Miyanaga, N.; Nakatsuka, M.; Wang, Y.; Lu, Z.; et al. The current trends in SBS and phase conjugation. Laser Part Beams 2012, 30, 117–174. [Google Scholar] [CrossRef]
- Hon, D.T. Pulse compression by stimulated Brillouin scattering. Opt. Lett. 1980, 5, 516–518. [Google Scholar] [CrossRef]
- Xu, X.; Feng, C.; Diels, J.C. Optimizing sub-ns pulse compression for high energy application. Opt. Express 2014, 22, 13904–13915. [Google Scholar] [CrossRef]
- Song, K.Y.; Herráez, M.G.; Thévenaz, L. Observation of pulse delaying and advancement in optical fibers using stimulated Brillouin scattering. Opt. Express 2005, 13, 82–88. [Google Scholar] [CrossRef]
- Herráez, M.G.; Song, K.Y.; Thévenaz, L. Arbitrary-bandwidth Brillouin slow light in optical fibers. Opt. Express 2006, 14, 1395–1400. [Google Scholar] [CrossRef]
- Okawachi, Y.; Bigelow, M.S.; Sharping, J.E.; Zhu, Z.; Schweinsberg, A.; Gauthier, D.J.; Boyd, R.W.; Gaeta, A.L. Tunable all-optical delays via Brillouin slow light in an optical fiber. Phys. Rev. Lett. 2005, 94, 153902. [Google Scholar] [CrossRef] [PubMed]
- Luo, Y.; Zhou, X.; Effenberger, F.; Yan, X.; Peng, G.; Qian, Y.; Ma, Y. Time-and wavelength-division multiplexed passive optical network (TWDM-PON) for next-generation PON stage 2 (NG-PON2). J. Light. Technol. 2012, 31, 587–593. [Google Scholar] [CrossRef]
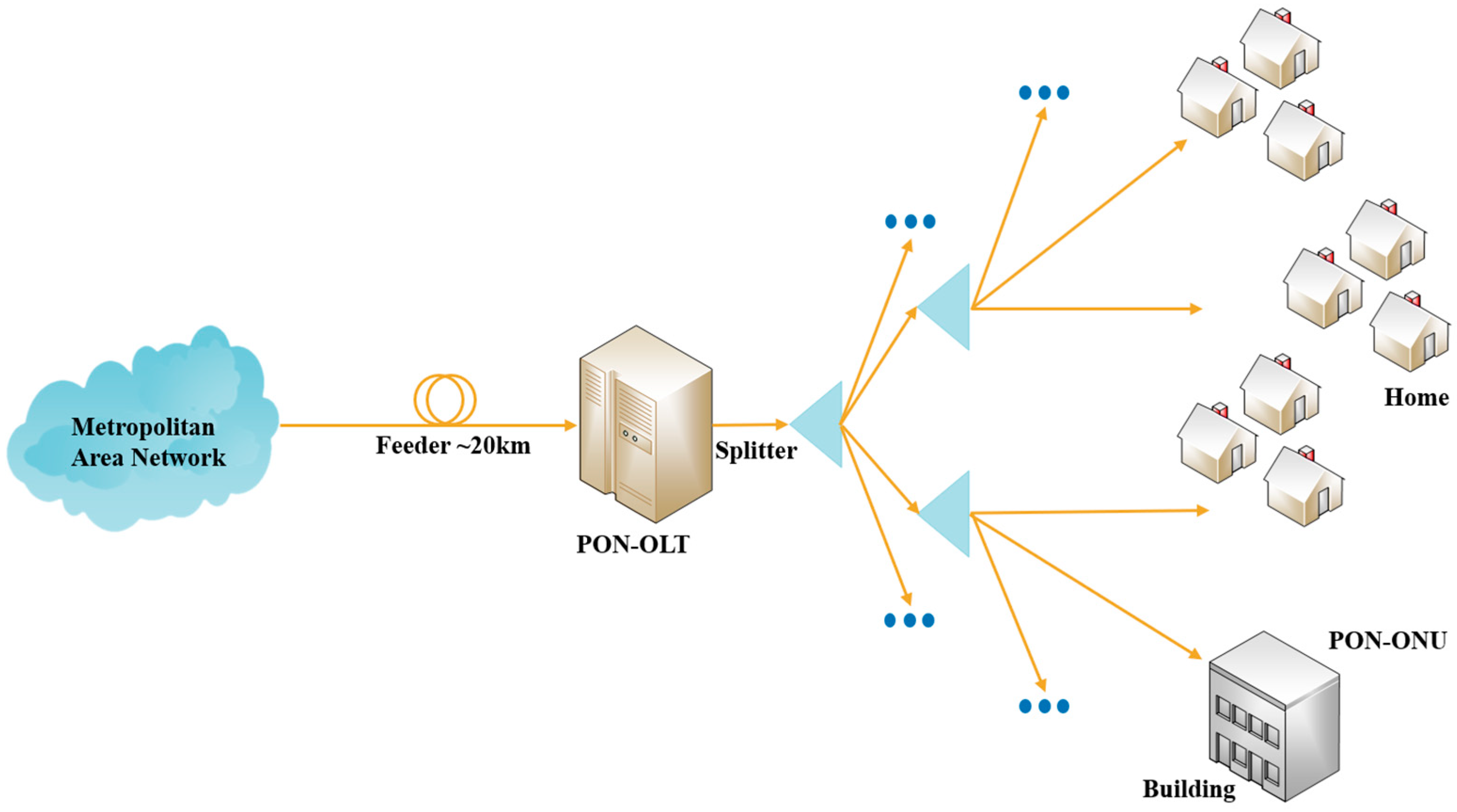
- Ellis, R.B.; Weiss, F.; Anton, O.M. HFC and PON-FTTH networks using higher SBS threshold singlemode optical fibre. Electron. Lett. 2007, 43, 1–2. [Google Scholar] [CrossRef]
- Tan, A.H.H. Super PON-a fiber to the home cable network for CATV and POTS/ISDN/VOD as economical as a coaxial cable network. J. Light. Technol. 1997, 15, 213–218. [Google Scholar] [CrossRef]
- Kovalev, V.I.; Harrison, R.G. Suppression of stimulated Brillouin scattering in high-power single-frequency fiber amplifiers. Opt. Lett. 2006, 31, 161–163. [Google Scholar] [CrossRef] [PubMed]
- Zervas, M.N.; Codemard, C.A. High power fiber lasers: A review. IEEE J. Quantum Electron. 2014, 20, 219–241. [Google Scholar] [CrossRef]
- Richardson, D.J.; Nilsson, J.; Clarkson, W.A. High power fiber lasers: Current status and future perspectives. JOSA B 2010, 27, B63–B92. [Google Scholar] [CrossRef]
- Fu, S.; Shi, W.; Feng, Y.; Zhang, L.; Yang, Z.; Xu, S.; Zhu, X.; Norwood, R.A.; Peyghambarian, N. Review of recent progress on single-frequency fiber lasers. JOSA B 2017, 34, A49–A62. [Google Scholar] [CrossRef]
- Willems, F.W.; Muys, W.; Leong, J.S. Simultaneous suppression of stimulated Brillouin scattering and interferometric noise in externally modulated lightwave AM-SCM systems. IEEE Photon. Technol. Lett. 1994, 6, 1476–1478. [Google Scholar] [CrossRef]
- Downie, J.D.; Hurley, J. Experimental study of SBS mitigation and transmission improvement from cross-phase modulation in 10.7 Gb/s unrepeatered systems. Opt. Express 2007, 15, 9527–9534. [Google Scholar] [CrossRef]
- Coles, J.B.; Kuo, B.P.P.; Alic, N.; Moro, S.; Bres, C.S.; Boggio, J.C.; Andrekson, P.A.; Karlsson, M.; Radic, S. Bandwidth-efficient phase modulation techniques for stimulated Brillouin scattering suppression in fiber optic parametric amplifiers. Opt. Express 2010, 18, 18138–18150. [Google Scholar] [CrossRef]
- Wu, P.Y.; Lu, H.H.; Ying, C.L.; Li, C.Y.; Su, H.S. An upconverted phase-modulated fiber optical CATV transport system. J. Light. Technol. 2011, 29, 2422–2427. [Google Scholar] [CrossRef]
- Anderson, B.; Robin, C.; Flores, A.; Dajani, I. Experimental study of SBS suppression via white noise phase modulation. SPIE 2014, 8961, 362–368. [Google Scholar]
- Flores, A.; Robin, C.; Lanari, A.; Dajani, I. Pseudo-random binary sequence phase modulation for narrow linewidth, kilowatt, monolithic fiber amplifiers. Opt. Express 2014, 22, 17735–17744. [Google Scholar] [CrossRef] [PubMed]
- Harish, A.V.; Nilsson, J. Optimization of phase modulation formats for suppression of stimulated Brillouin scattering in optical fibers. IEEE J Quantum Electron. 2017, 24, 5100110. [Google Scholar] [CrossRef]
- Kim, D.; Kim, B.G.; Bo, T.; Kim, H. Dither-frequency tuning technique for RSOA-based coherent WDM PON. IEEE Photon. Technol. Lett. 2018, 31, 7–10. [Google Scholar] [CrossRef]
- Yang, Y.; Li, B.; Liu, M.; Huang, X.; Feng, Y.; Cheng, D.; He, B.; Zhou, J.; Nilsson, J. Optimization and visualization of phase modulation with filtered and amplified maximal-length sequence for SBS suppression in a short fiber system: A theoretical treatment. Opt. Express 2021, 29, 16781–16803. [Google Scholar] [CrossRef] [PubMed]
- Lee, H.; Agrawal, G.P. Suppression of stimulated Brillouin scattering in optical fibers using fiber Bragg gratings. Opt. Express 2003, 11, 3467–3472. [Google Scholar] [CrossRef]
- Takushima, Y.; Okoshi, T. Suppression of stimulated Brillouin scattering using optical isolators. Electron. Lett. 1992, 12, 1155–1157. [Google Scholar] [CrossRef]
- Weßels, P.; Adel, P.; Auerbach, M.; Wandt, D.; Fallnich, C. Novel suppression scheme for Brillouin scattering. Opt. Express 2004, 12, 4443–4448. [Google Scholar] [CrossRef]
- Garmire, E. Perspectives on stimulated Brillouin scattering. NJP 2017, 19, 011003. [Google Scholar] [CrossRef]
- Garmire, E. Stimulated Brillouin review: Invented 50 years ago and applied today. Int. J. Opt. 2018, 2018, 17. [Google Scholar] [CrossRef]
- Kobyakov, A.; Sauer, M.; Chowdhury, D. Stimulated Brillouin scattering in optical fibers. Adv. Opt. Photon. 2010, 2, 1–59. [Google Scholar] [CrossRef]
- Dasgupta, S.; Poletti, F.; Liu, S.; Petropoulos, P.; Richardson, D.J.; Gruner-Nielsen, L.; Herstrom, S. Modeling Brillouin gain spectrum of solid and microstructured optical fibers using a finite element method. J. Light. Technol. 2011, 29, 22–30. [Google Scholar] [CrossRef]
- Dong, L. Formulation of a complex mode solver for arbitrary circular acoustic waveguides. J. Light. Technol. 2010, 28, 3162–3175. [Google Scholar]
- Koyamada, Y.; Sato, S.; Nakamura, S.; Sotobayashi, H.; Chujo, W. Simulating and designing Brillouin gain spectrum in single-mode fibers. J. Light. Technol. 2004, 22, 631. [Google Scholar] [CrossRef]
- Kobyakov, A.; Kumar, S.; Chowdhury, D.Q.; Ruffin, A.B.; Sauer, M.; Bickham, S.R.; Mishra, R. Design concept for optical fibers with enhanced SBS threshold. Opt. Express 2005, 13, 5338–5346. [Google Scholar] [CrossRef] [PubMed]
- Supradeepa, V.R. Stimulated Brillouin scattering thresholds in optical fibers for lasers linewidth broadened with noise. Opt. Express 2013, 21, 4677–4687. [Google Scholar] [CrossRef]
- Mao, X.P.; Tkach, R.W.; Chraplyvy, A.R.; Jopson, R.M.; Derosier, R.M. Stimulated Brillouin threshold dependence on fiber type and uniformity. IEEE Photon. Technol. Lett. 1992, 4, 66–69. [Google Scholar] [CrossRef]
- Shiraki, K.; Ohashi, M.; Tateda, M. Suppression of stimulated Brillouin scattering in a fibre by changing the core radius. Electron. Lett. 1995, 31, 668–669. [Google Scholar] [CrossRef]
- Shibata, N.; Waarts, R.G.; Braun, R.P. Brillouin-gain spectra for single-mode fibers having pure-silica, GeO2-doped, and P2O5-doped cores. Opt. Lett. 1987, 12, 269–271. [Google Scholar] [CrossRef]
- Nikles, M.; Thevenaz, L.; Robert, P.A. Brillouin gain spectrum characterization in single-mode optical fibers. J. Light. Technol. 1997, 15, 1842–1851. [Google Scholar] [CrossRef]
- Lagakos, N.; Bucaro, J.A.; Hughes, R. Acoustic sensitivity predictions of single-mode optical fibers using Brillouin scattering. Appl. Opt. 1980, 19, 3668–3670. [Google Scholar] [CrossRef]
- Shiraki, K.; Ohashi, M. Sound velocity measurement based on guided acoustic-wave Brillouin scattering. IEEE Photon. Technol. Lett. 1992, 4, 1177–1180. [Google Scholar] [CrossRef]
- Broderick, N.G.R.; Offerhaus, H.L.; Richardson, D.J.; Sammut, R.A.; Caplen, J.; Dong, L. Large mode area fibers for high power applications. Opt. Fiber Technol. 1999, 5, 185–196. [Google Scholar] [CrossRef]
- Limpert, J.; Deguil-Robin, N.; Manek-Hönninger, I.; Salin, F.; Röser, F.; Liem, A.; Schreiber, T.; Nolte, S.; Zellmer, A.; Tunnermann, A.; et al. High-power rod-type photonic crystal fiber laser. Opt. Express 2005, 13, 1055–1058. [Google Scholar] [CrossRef] [PubMed]
- Knight, J.C.; Birks, T.A.; Russell, P.S.J.; Atkin, D.M. All-silica single-mode optical fiber with photonic crystal cladding. Opt. Lett. 1996, 21, 1547–1549. [Google Scholar] [CrossRef]
- Wadsworth, W.J.; Knight, J.C.; Reeves, W.H.; Russell, P.S.J.; Arriaga, J. Yb3+-doped photonic crystal fiber laser. Electron. Lett. 2000, 36, 17. [Google Scholar] [CrossRef]
- Wadsworth, W.J.; Knight, J.C.; Russell, P.S.J. Large mode area photonic crystal fiber laser. In Proceedings of the Conference on Lasers and Electro-Optics, Baltimore, MD, USA, 11 May 2001. [Google Scholar]
- Wadsworth, W.J.; Percival, R.M.; Bouwmans, G.; Knight, J.C.; Russell, P.S.J. High power air-clad photonic crystal fiber laser. Opt. Express 2003, 11, 48–53. [Google Scholar] [CrossRef]
- El Hamzaoui, H.; Bouwmans, G.; Cassez, A.; Bigot, L.; Capoen, B.; Bouazaoui, M.; Vanvincq, O.; Douay, M. F/Yb-codoped sol-gel silica glasses: Toward tailoring the refractive index for the achievement of high-power fiber lasers. Opt. Lett. 2017, 42, 1408–1411. [Google Scholar] [CrossRef]
- Golojuch, G.; Urbańczyk, W. Large mode area photonic crystal fibers with high geometrical birefringence. SPIE 2008, 7141, 433–437. [Google Scholar]
- Chen, M.Y. Polarization and leakage properties of large-mode-area microstructured-core optical fibers. Opt. Express 2007, 15, 12498–12507. [Google Scholar] [CrossRef]
- Christensen, S.L.; Papior, S.R.; Johansen, M.M.; Hauge, J.M.; Weirich, J.; Jakobsen, C.; Michieletto, M.; Bondu, M.; Triches, M.; Alkeskjold, T.T.; et al. Photonic crystal fiber technology for monolithic single-mode large-mode-area all-solid amplifier. SPIE 2019, 10897, 255–261. [Google Scholar]
- Kim, Y.G.; Ryu, J.W.; Cha, Y.H.; Park, H.M.; Lim, G.; Han, J.M.; Ko, K.H.; Kim, T.S.; Jeong, D.Y. Maintaining the polarization, temperature and amplification characteristics of a ytterbium-doped rod-type photonic crystal fiber (PCF) amplifier. J. Korean Phys. Soc. 2011, 59, 3182–3187. [Google Scholar] [CrossRef]
- Alkeskjold, T.T.; Laurila, M.; Weirich, J.; Johansen, M.M.; Olausson, C.B.; Lumholt, O.; Noordegraaf, D.; Maack, M.D.; Jakobsen, C. Photonic crystal fiber amplifiers for high power ultrafast fiber lasers. Nanophotonics 2013, 2, 369–381. [Google Scholar] [CrossRef]
- Mamdem, Y.S.; Phéron, X.; Taillade, F.; Jaouën, Y.; Gabet, R.; Lanticq, V.; Moreau, G.; Boukenter, A.; Ouerdane, Y.; Lesoille, S.; et al. Two-dimensional FEM analysis of Brillouin gain spectra in acoustic guiding and antiguiding single mode optical fibers. In Proceedings of the COMSOL Conference 2010, Paris, France, 17 November 2010. [Google Scholar]
- Li, M.J.; Chen, X.; Wang, J.; Gray, S.; Liu, A.; Demeritt, J.A.; Ruffin, A.B.; Crowley, A.M.; Walton, D.T.; Zenteno, L.A. Al/Ge co-doped large mode area fiber with high SBS threshold. Opt. Express 2007, 15, 8290–8299. [Google Scholar] [CrossRef] [PubMed]
- Robin, C.; Dajani, I. Acoustically segmented photonic crystal fiber for single-frequency high-power laser applications. Opt. Lett. 2011, 36, 2641–2643. [Google Scholar] [CrossRef] [PubMed]
- Dragic, P.D. Brillouin suppression by fiber design. In Proceedings of the IEEE Photonics Society Summer Topicals 2010, Playa del Carmen, Mexico, 19–21 July 2010. [Google Scholar]
- Dragic, P.D. Ultra-flat Brillouin gain spectrum via linear combination of two acoustically anti-guiding optical fibres. Electron. Lett. 2012, 48, 1492–1493. [Google Scholar] [CrossRef]
- Dragic, P.D.; Liu, C.H.; Papen, G.C.; Galvanauskas, A. Optical fiber with an acoustic guiding layer for stimulated Brillouin scattering suppression. In Proceedings of the Conference on Lasers and Electro-Optics, Baltimore, MD, USA, 22–27 May 2005; Volume 3, pp. 1984–1986. [Google Scholar]
- Rae, S.; Bennion, I.; Cardwell, M.J. New numerical model of stimulated Brillouin scattering in optical fibers with nonuniformity. Opt. Commun. 1996, 123, 611–616. [Google Scholar] [CrossRef]
- Sugie, T.; Ohkawa, N.; Imai, T.; Ito, T. A 2.5 Gb/s, 364 km CPFSK repeaterless transmission experiment employing an Er-doped fiber amplifier and SBS suppression optical link. In Optical Amplifiers and Their Applications; Optica Publishing Group: Monterey, CA, USA, 1990. [Google Scholar]
- De Oliveira, C.A.S.; Jen, C.K.; Shang, A.; Saravanos, C. Stimulated Brillouin scattering in cascaded fibers of different Brillouin frequency shifts. JOSA B 1993, 10, 969–972. [Google Scholar] [CrossRef]
- Kobyakov, A.; Sauer, M.; Hurley, J.E. SBS threshold of segmented fibers. In Optical Fiber Communication Conference; Optica Publishing Group: Anaheim, CA, USA, 2005. [Google Scholar]
- Patokoski, K.; Rissanen, J.; Noronen, T.; Gumenyuk, R.; Chamorovskii, Y.; Filippov, V.; Toivonen, J. Single-frequency 100 ns/0.5 mJ laser pulses from all-fiber double clad ytterbium doped tapered fiber amplifier. Opt. Express 2019, 27, 31532–31541. [Google Scholar] [CrossRef]
- Lai, W.; Ma, P.; Liu, W.; Huang, L.; Li, C.; Ma, Y.; Zhou, P. 550 W single frequency fiber amplifiers emitting at 1030 nm based on a tapered Yb-doped fiber. Opt. Express 2020, 28, 20908–20919. [Google Scholar] [CrossRef]
- Jiang, W.; Yang, C.; Zhao, Q.; Gu, Q.; Huang, J.; Jiang, K.; Zhou, K.; Feng, Z.; Yang, Z.; Xu, S. 650 W All-Fiber Single-Frequency Polarization-Maintaining Fiber Amplifier Based on Hybrid Wavelength Pumping and Tapered Yb-Doped Fibers. Photonics 2022, 9, 518. [Google Scholar] [CrossRef]
- Huang, L.; Ma, P.; Su, R.; Lai, W.; Ma, Y.; Zhou, P. Comprehensive investigation on the power scaling of a tapered Yb-doped fiber-based monolithic linearly polarized high-peak-power near-transform-limited nanosecond fiber laser. Opt. Express 2021, 29, 761–782. [Google Scholar] [CrossRef] [PubMed]
- Kerttula, J.; Filippov, V.; Chamorovskii, Y.; Golant, K.; Okhotnikov, O.G. Actively Q-switched 1.6-mJ tapered double-clad ytterbium-doped fiber laser. Opt. Express 2010, 18, 18543–18549. [Google Scholar] [CrossRef] [PubMed]
- Kerttula, J.; Filippov, V.; Chamorovskii, Y.; Ustimchik, V.; Golant, K.; Okhotnikov, O.G. Tapered fiber amplifier with high gain and output power. Laser Phys. 2012, 22, 1734–1738. [Google Scholar] [CrossRef]
- Liu, A. Suppressing stimulated Brillouin scattering in fiber amplifiers using nonuniform fiber and temperature gradient. Opt. Express 2007, 15, 977–984. [Google Scholar] [CrossRef]
- Filippov, V.; Chamorovskii, Y.; Kerttula, J.; Golant, K.; Pessa, M.A.; Okhotnikov, O.G. Double clad tapered fiber for high power applications. Opt. Express 2008, 16, 1929–1944. [Google Scholar] [CrossRef]
- Galindez-Jamioy, C.A.; Lopez-Higuera, J.M. Brillouin distributed fiber sensors: An overview and applications. J. Sens. 2012, 2012, 204121. [Google Scholar] [CrossRef]
- Horiguchi, T.; Shimizu, K.; Kurashima, T.; Tateda, M.; Koyamada, Y. Development of a distributed sensing technique using Brillouin scattering. J. Light. Technol. 1995, 13, 1296–1302. [Google Scholar] [CrossRef]
- Bao, X.; Chen, L. Recent Progress in Brillouin Scattering Based Fiber Sensors. Sensors 2011, 11, 4152–4187. [Google Scholar] [CrossRef]
- Soto, M.A.; Thévenaz, L. Modeling and evaluating the performance of Brillouin distributed optical fiber sensors. Opt. Express 2013, 21, 31347–31366. [Google Scholar] [CrossRef]
- Grattan, K.T.V.; Sun, T. Fiber optic sensor technology: An overview. Sens. Actuator A Phys. 2000, 82, 40–61. [Google Scholar] [CrossRef]
- Imai, Y.; Shimada, N. Dependence of stimulated Brillouin scattering on temperature distribution in polarization-maintaining fibers. IEEE Photon. Technol. Lett. 1993, 5, 1335–1337. [Google Scholar] [CrossRef]
- Kurashima, T.; Horiguchi, T.; Tateda, M. Thermal effects of Brillouin gain spectra in single-mode fibers. IEEE Photon. Technol. Lett. 1990, 2, 718–720. [Google Scholar] [CrossRef]
- Kurashima, T.; Horiguchi, T.; Tateda, M. Thermal effects on the Brillouin frequency shift in jacketed optical silica fibers. Appl. Opt. 1990, 29, 2219–2222. [Google Scholar] [CrossRef] [PubMed]
- Hildebrandt, M.; Büsche, S.; Weßels, P.; Frede, M.; Kracht, D. Brillouin scattering spectra in high-power single frequency ytterbium doped fiber amplifiers. Opt. Express 2008, 16, 15970–15979. [Google Scholar] [CrossRef] [PubMed]
- Hansryd, J.; Dross, F.; Westlund, M.; Andrekson, P.A.; Knudsen, S.N. Increase of the SBS threshold in a short highly nonlinear fiber by applying a temperature distribution. J. Light. Technol. 2001, 19, 1691. [Google Scholar] [CrossRef]
- Theeg, T.; Ottenhues, C.; Sayinc, H.; Neumann, J.; Kracht, D. Core-pumped single-frequency fiber amplifier with an output power of 158 W. Opt. Lett. 2016, 41, 9–12. [Google Scholar] [CrossRef] [PubMed]
- Gray, S.; Liu, A.; Walton, D.T.; Wang, J.; Li, M.; Chen, X.; Ruffin, A.B. 502 Watt, single transverse mode, narrow linewidth, bidirectionally pumped Yb-doped fiber amplifier. Opt. Express 2007, 15, 17044–17050. [Google Scholar] [CrossRef]
- Li, W.; Yan, Z.; Ren, S.; Deng, Y.; Chen, Y.; Ma, P.; Liu, W.; Huang, L.; Pan, Z.; Zhou, P.; et al. Confined-doped active fiber enabled all-fiber high-power single-frequency laser. Opt. Lett. 2022, 47, 5024–5027. [Google Scholar] [CrossRef]
- Boggio, J.M.C.; Marconi, J.D.; Fragnito, H.L. Experimental and numerical investigation of the SBS-threshold increase in an optical fiber by applying strain distributions. J. Light. Technol. 2005, 23, 3808–3814. [Google Scholar] [CrossRef]
- Boggio, J.M.C.; Marconi, J.D.; Fragnito, H.L. 8 dB increase of the SBS threshold in an optical fiber by applying a stair ramp strain distribution. In Conference on Lasers and Electro-Optics; Optica Publishing Group: San Francisco, CA, USA, 2004. [Google Scholar]
- Marconi, J.D.; Boggio, J.M.C.; Fragnito, H.L. 7.3 dB increase of the SBS threshold in an optical fiber by applying a strain distribution. In Optical Fiber Communication Conference; Optica Publishing Group: Los Angeles, CA, USA, 2004. [Google Scholar]
- Engelbrecht, R. Analysis of SBS gain shaping and threshold increase by arbitrary strain distributions. J. Light. Technol. 2014, 32, 1689–1700. [Google Scholar] [CrossRef]
- Zhang, L.; Cui, S.; Liu, C.; Zhou, J.; Feng, Y. 170 W, single-frequency, single-mode, linearly-polarized, Yb-doped all-fiber amplifier. Opt. Express 2013, 21, 5456–5462. [Google Scholar] [CrossRef]
- Engelbrecht, R.; Hagen, J.; Schmidt, M. SBS-suppression in variably strained fibers for fiber-amplifiers and fiber-lasers with a high spectral power density. SPIE 2005, 5777, 795–798. [Google Scholar]
- Ma, P.; Tao, R.; Su, R.; Wang, X.; Zhou, P.; Liu, Z. 1.89 kW all-fiberized and polarization-maintained amplifiers with narrow linewidth and near-diffraction-limited beam quality. Opt. Express 2016, 24, 4187–4195. [Google Scholar] [CrossRef] [PubMed]
- Yoshizawa, N.; Horiguchi, T.; Kurashima, T. Proposal for stimulated Brillouin scattering suppression by fibre cabling. Electron. Lett. 1991, 27, 1100–1101. [Google Scholar] [CrossRef]
- Yoshizawa, N. Nine-dB expansion of Brillouin gain bandwidth by applying ±0.35% strain distribution to fiber by cabling. In Optical Fiber Communication Conference; Optica Publishing Group: San Jose, CA, USA, 1992. [Google Scholar]
- Yoshizawa, N.; Imai, T. Stimulated Brillouin scattering suppression by means of applying strain distribution to fiber with cabling. J. Light. Technol. 1993, 11, 1518–1522. [Google Scholar] [CrossRef]
- Khudyakov, M.M.; Tsvetkov, S.V.; Kosolapov, A.F.; Bubnov, M.M.; Lobanov, A.S.; Lipatov, D.S.; Guryanov, A.N.; Likhachev, M.E. Combined Method for SBS Suppression in High Numerical Aperture Single-Mode Optical Fibers. IEEE Photon. Technol. Lett. 2022, 34, 1069–1072. [Google Scholar] [CrossRef]









| GeO2 | P2O3 | TiO2 | B2O3 | F2 | Al2O3 | |
|---|---|---|---|---|---|---|
| Optical refractive index | ||||||
| Acoustic refractive index |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, B.; Wang, J.; Shao, X. Fiber-Based Techniques to Suppress Stimulated Brillouin Scattering. Photonics 2023, 10, 282. https://doi.org/10.3390/photonics10030282
Huang B, Wang J, Shao X. Fiber-Based Techniques to Suppress Stimulated Brillouin Scattering. Photonics. 2023; 10(3):282. https://doi.org/10.3390/photonics10030282
Chicago/Turabian StyleHuang, Bin, Jiaqi Wang, and Xiaopeng Shao. 2023. "Fiber-Based Techniques to Suppress Stimulated Brillouin Scattering" Photonics 10, no. 3: 282. https://doi.org/10.3390/photonics10030282
APA StyleHuang, B., Wang, J., & Shao, X. (2023). Fiber-Based Techniques to Suppress Stimulated Brillouin Scattering. Photonics, 10(3), 282. https://doi.org/10.3390/photonics10030282





