Micropolar Nanofluid Flow in a Stagnation Region of a Shrinking Sheet with Fe3O4 Nanoparticles
Abstract
:1. Introduction
2. Mathematical Formulation
3. Stability Analysis
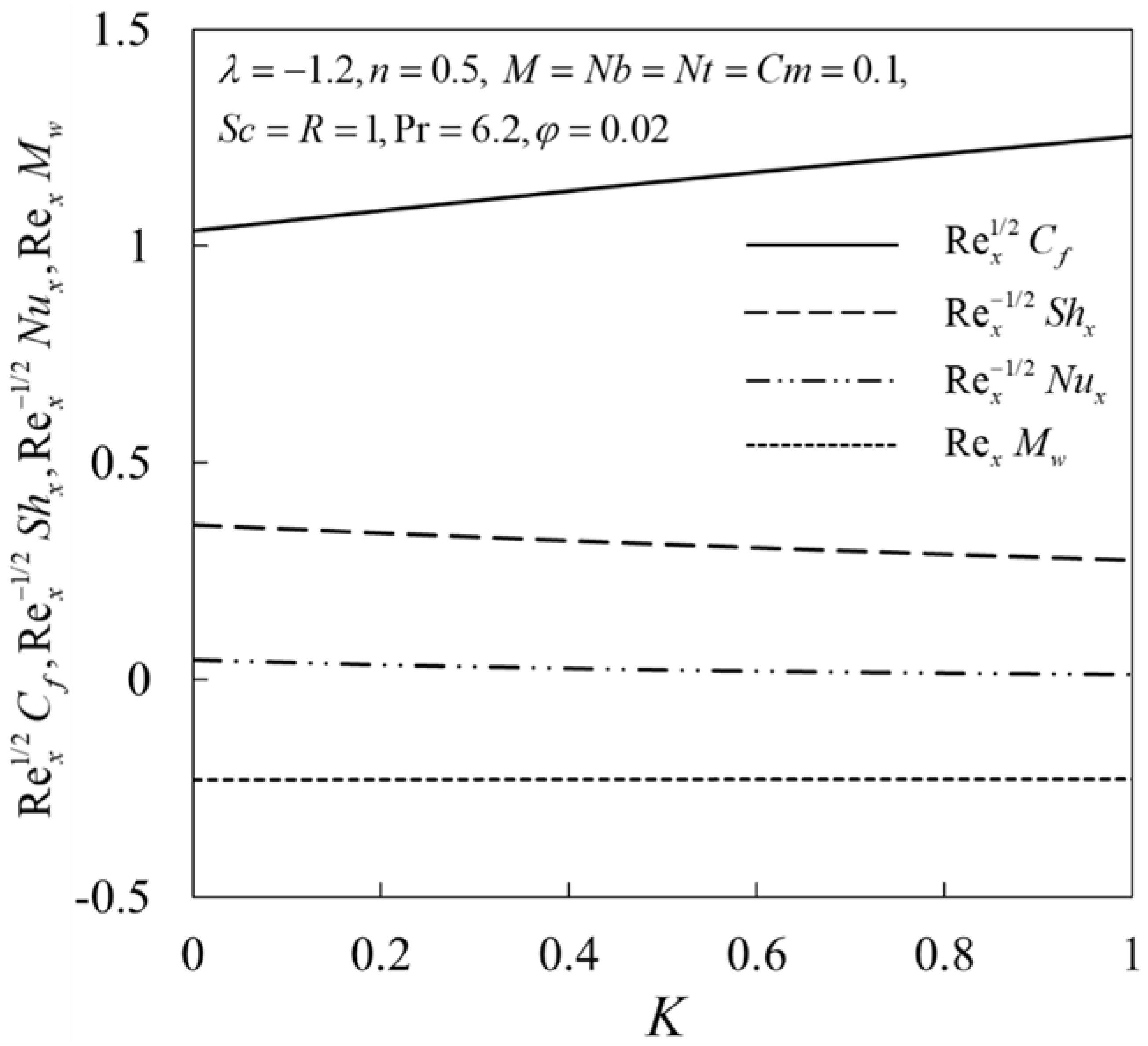
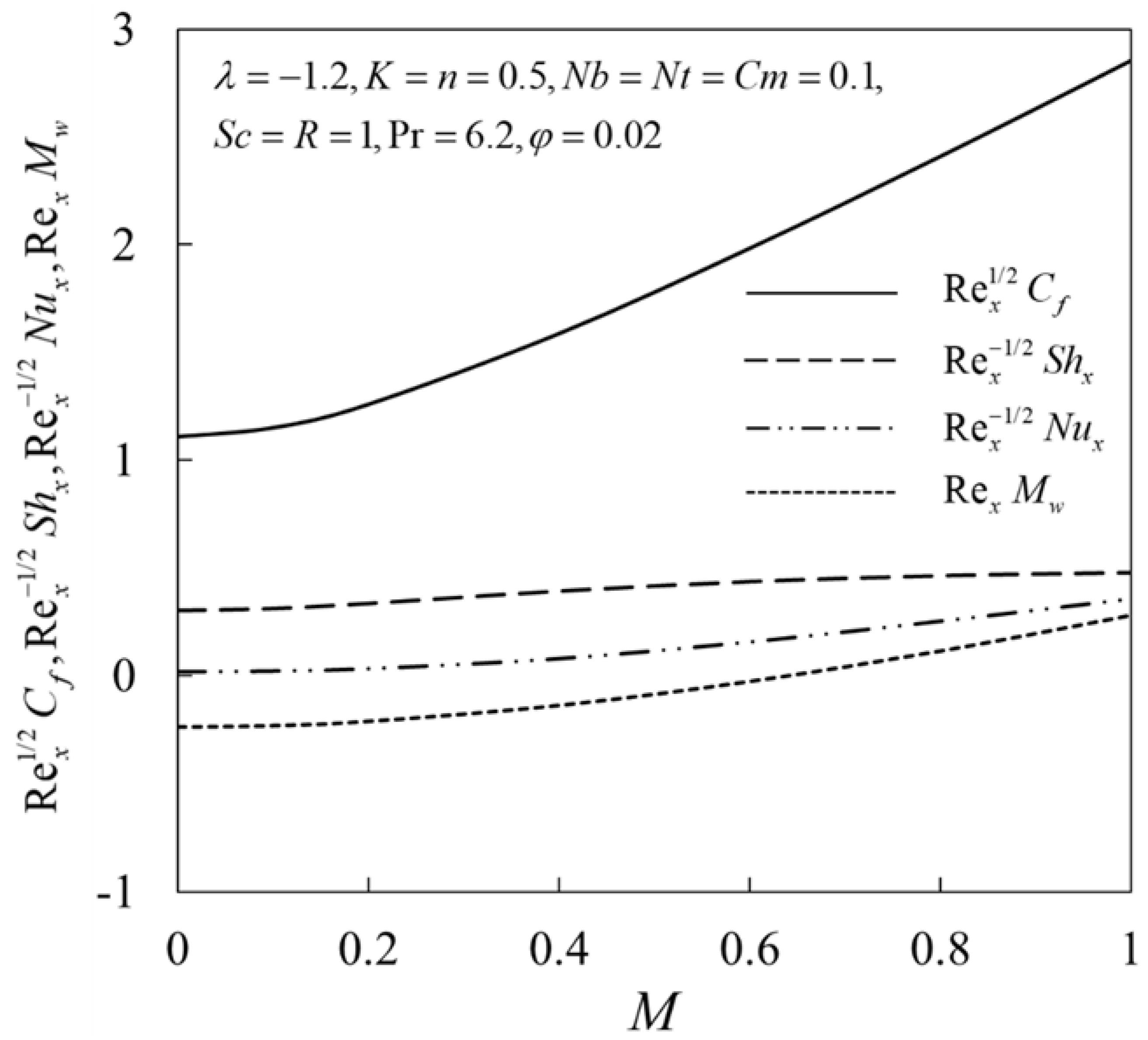
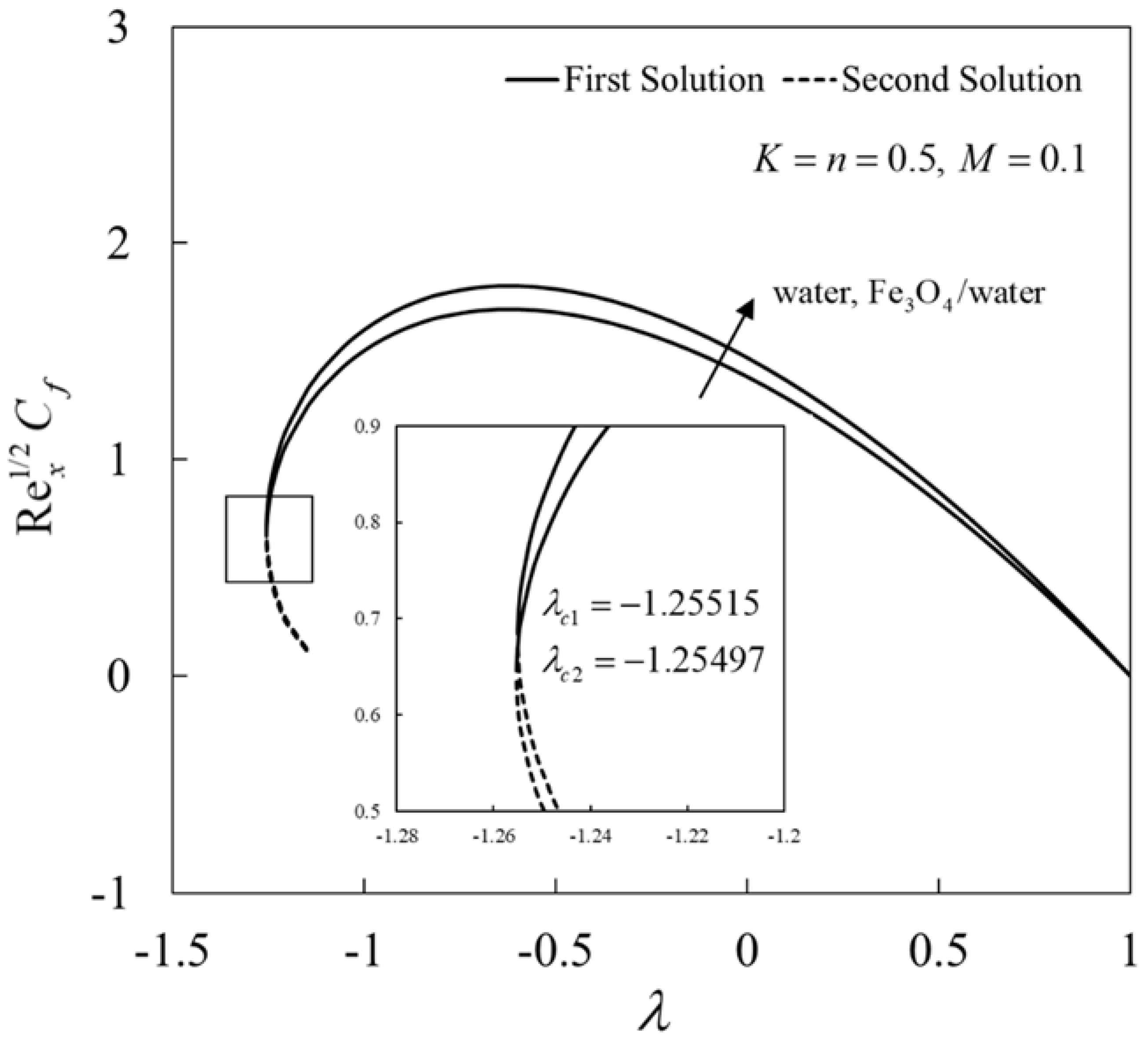
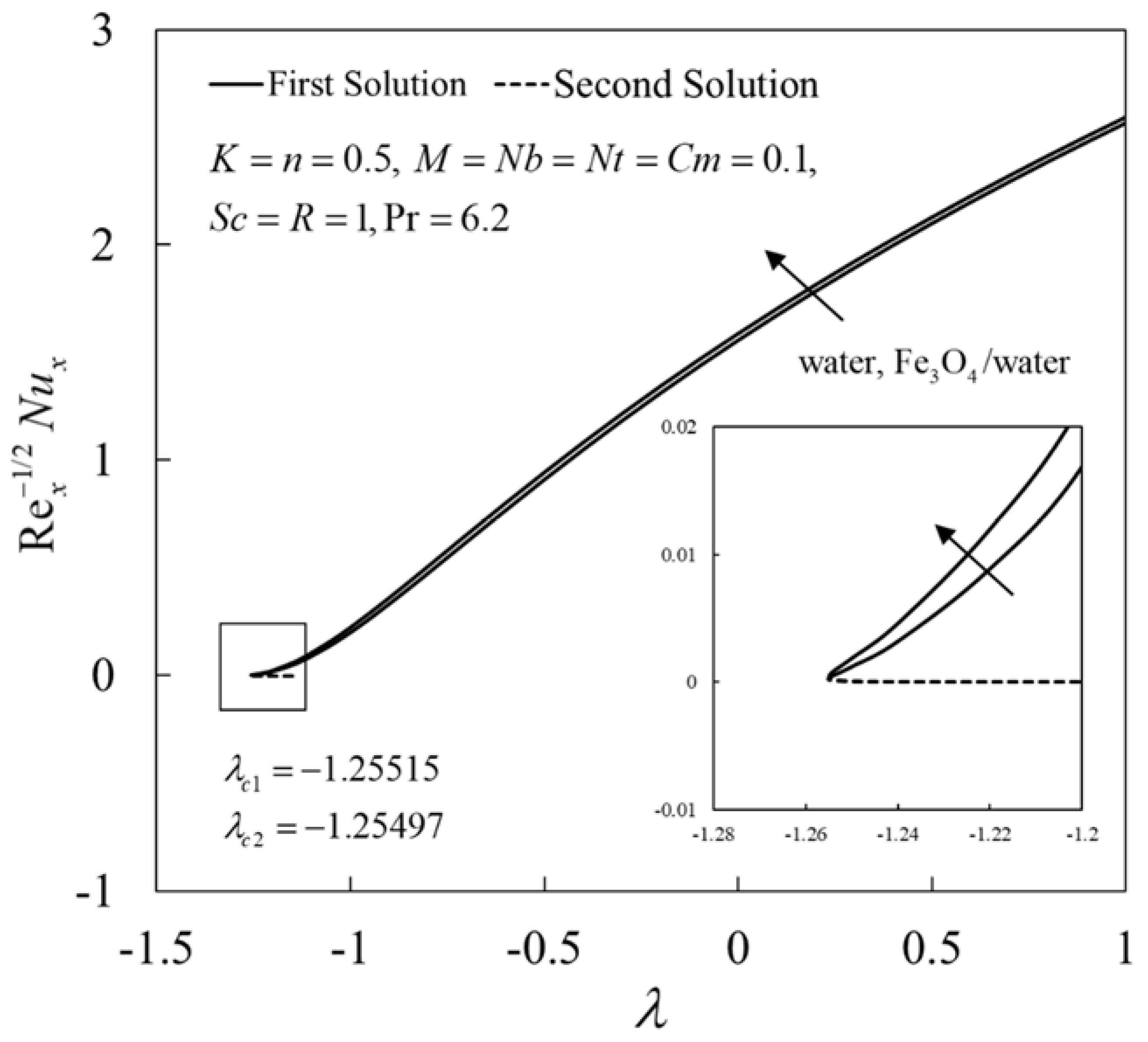
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| a, b | constants |
| B0 | strength of magnetic field (T) |
| C | concentration (mol m−3) |
| Cf | skin friction coefficient |
| Cm | chemical reaction parameter |
| cp | specific heat at constant pressure (J kg−1 K−1) |
| concentration at the surface (mol m−3) | |
| Brownian motion coefficient (m2 s−1) | |
| thermophoretic diffusion coefficient (mol m−1 s−1) | |
| dimensionless stream function | |
| dimensionless microrotation velocity | |
| microinertia coefficient (m2) | |
| material parameter | |
| mean absorption coefficient (m−1) | |
| thermal conductivity of the fluid (Wm−1 K−1) | |
| magnetic parameter | |
| local couple stress | |
| n | the micro-gyration parameter |
| N | microrotation velocity (s−1) |
| Nb | Brownian motion parameter |
| Nt | thermophoresis parameter |
| local Nusselt number | |
| Pr | Prandtl number |
| R | radiation parameter |
| Sc | Schmidt number |
| local Sherwood number | |
| T | fluid temperature (K) |
| Tw | surface temperature (K) |
| ambient temperature (K) | |
| u,v | components of velocity (m s−1) |
| x,y | Cartesian coordinates (m) |
| Greek symbols | |
| 𝛽 | first-order chemical reaction rate (s−1) |
| effective heat capacity ratio (m3 mol−1) | |
| η | similarity variable |
| θ | dimensionless temperature |
| vortex viscosity (kg m−1 s−1) | |
| λ | stretching/shrinking parameter |
| μ | dynamic viscosity (kg m−1 s−1) |
| ν | kinematic viscosity of the fluid (m2 s−1) |
| ρ | fluid density (kg m−3) |
| σ | electrical conductivity (S m−1) |
| σ∗ | Stefan–Boltzmann constant (W m−2 K−4) |
| nanoparticles volume fraction | |
| dimensionless concentration | |
| stream function (m2 s−1) | |
| ω | microinertia coefficient (kg m s−1) |
| Subscripts | |
| f | fluid |
| n | solid |
| nf | nanofluid |
| w | condition at the surface |
| Superscript | |
| ’ | differentiation with respect to η |
References
- Choi, S.U.S.; Eastman, J.A. Enhancing thermal conductivity of fluids with nanoparticles. In Proceedings of the 1995 International Mechanical Engineering Congress and Exhibition, San Francisco, CA, USA, 12–17 November 1995. [Google Scholar]
- Khanafer, K.; Vafai, K.; Lightstone, M. Buoyancy-driven heat transfer enhancement in a two-dimensional enclosure utilizing nanofluids. Int. J. Heat Mass Transf. 2003, 46, 3639–3653. [Google Scholar] [CrossRef]
- Tiwari, R.K.; Das, M.K. Heat transfer augmentation in a two-sided lid-driven differentially heated square cavity utilizing nanofluids. Int. J. Heat Mass Transf. 2007, 50, 2002–2018. [Google Scholar] [CrossRef]
- Oztop, H.F.; Abu-Nada, E. Numerical study of natural convection in partially heated rectangular enclosures filled with nanofluids. Int. J. Heat Fluid Flow 2008, 29, 1326–1336. [Google Scholar] [CrossRef]
- Jana, S.; Salehi-Khojin, A.; Zhong, W.H. Enhancement of fluid thermal conductivity by the addition of single and hybrid nano-additives. Thermochim. Acta 2007, 462, 45–55. [Google Scholar] [CrossRef]
- Suresh, S.; Venkitaraj, K.P.; Selvakumar, P.; Chandrasekar, M. Synthesis of Al2O3-Cu/water hybrid nanofluids using two step method and its thermo physical properties. Colloids Surf. A Physicochem. Eng. Asp. 2011, 388, 41–48. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Squeezed hybrid nanofluid flow over a permeable sensor surface. Mathematics 2020, 8, 898. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Mixed convection flow over an exponentially stretching/shrinking vertical surface in a hybrid nanofluid. Alex. Eng. J. 2020, 59, 1881–1891. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Dufour and soret effects on al2o3-water nanofluid flow over a moving thin needle: Tiwari and das model. Int. J. Numer. Methods Heat Fluid Flow 2021, 31, 766–782. [Google Scholar] [CrossRef]
- Khashi’ie, N.S.; Waini, I.; Zainal, N.A.; Hamzah, K. Hybrid nanofluid flow past a shrinking cylinder with prescribed surface heat flux. Symmetry 2020, 12, 1493. [Google Scholar] [CrossRef]
- Eringen, A. Theory of micropolar fluids. J. Math. Mech. 1966, 16, 1–18. [Google Scholar] [CrossRef]
- Eringen, A.C. Theory of thermomicrofluids. J. Math. Anal. Appl. 1972, 38, 480–496. [Google Scholar] [CrossRef]
- Ishak, A.; Lok, Y.Y.; Pop, I. Stagnation-point flow over a shrinking sheet in a micropolar fluid. Chem. Eng. Commun. 2010, 197, 1417–1427. [Google Scholar] [CrossRef]
- Yacob, N.A.; Ishak, A. Stagnation point flow towards a stretching/shrinking sheet in a micropolar fluid with a convective surface boundary condition. Can. J. Chem. Eng. 2012, 90, 621–626. [Google Scholar] [CrossRef]
- Soid, S.K.; Ishak, A.; Pop, I. MHD stagnation-point flow over a stretching/shrinking sheet in a micropolar fluid with a slip boundary. Sains Malays. 2018, 47, 2907–2916. [Google Scholar] [CrossRef]
- Lok, Y.Y.; Amin, N.; Campean, D.; Pop, I. Steady mixed convection flow of a micropolar fluid near the stagnation point on a vertical surface. Int. J. Numer. Methods Heat Fluid Flow 2005, 15, 654–670. [Google Scholar] [CrossRef]
- Lok, Y.Y.; Pop, I.; Ingham, D.B.; Amin, N. Mixed convection flow of a micropolar fluid near a non-orthogonal stagnation-point on a stretching vertical sheet. Int. J. Numer. Methods Heat Fluid Flow 2009, 19, 459–483. [Google Scholar] [CrossRef]
- El-Aziz, M.A. Viscous dissipation effect on mixed convection flow of a micropolar fluid over an exponentially stretching sheet. Can. J. Phys. 2009, 87, 359–368. [Google Scholar] [CrossRef]
- Zaimi, W.M.K.A.W.; Ishak, A. Stagnation flow of a micropolar fluid towards a vertical permeable surface with prescribed heat flux. Sains Malays. 2012, 41, 1263–1270. [Google Scholar]
- Turkyilmazoglu, M. Mixed convection flow of magnetohydrodynamic micropolar fluid due to a porous heated/cooled deformable plate: Exact solutions. Int. J. Heat Mass Transf. 2017, 106, 127–134. [Google Scholar] [CrossRef]
- Khashi’ie, N.S.; Arifin, N.M.; Nazar, R.; Hafidzuddin, E.H.; Wahi, N.; Pop, I. Mixed convective flow and heat transfer of a dual stratified micropolar fluid induced by a permeable stretching/shrinking sheet. Entropy 2019, 21, 1162. [Google Scholar] [CrossRef]
- Ramadevi, B.; Anantha Kumar, K.; Sugunamma, V.; Ramana Reddy, J.V.; Sandeep, N. Magnetohydrodynamic mixed convective flow of micropolar fluid past a stretching surface using modified fourier’s heat flux model. J. Therm. Anal. Calorim. 2020, 139, 1379–1393. [Google Scholar] [CrossRef]
- Buongiorno, J. Convective transport in nanofluids. J. Heat Transf. 2006, 128, 240–250. [Google Scholar] [CrossRef]
- Hsiao, K.L. Micropolar nanofluid flow with MHD and viscous dissipation effects towards a stretching sheet with multimedia feature. Int. J. Heat Mass Transf. 2017, 112, 983–990. [Google Scholar] [CrossRef]
- Anwar, M.I.; Shafie, S.; Hayat, T.; Shehzad, S.A.; Salleh, M.Z. Numerical study for MHD stagnation-point flow of a micropolar nanofluid towards a stretching sheet. J. Braz. Soc. Mech. Sci. Eng. 2017, 39, 89–100. [Google Scholar] [CrossRef]
- Hayat, T.; Khan, M.I.; Waqas, M.; Alsaedi, A.; Khan, M.I. Radiative flow of micropolar nanofluid accounting thermophoresis and brownian moment. Int. J. Hydrogen Energy 2017, 42, 16821–16833. [Google Scholar] [CrossRef]
- Ibrahim, W. Passive control of nanoparticle of micropolar fluid past a stretching sheet with nanoparticles, convective boundary condition and second-order slip. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 2017, 231, 704–719. [Google Scholar] [CrossRef]
- Siddiq, M.K.; Rauf, A.; Shehzad, S.A.; Abbasi, F.M.; Meraj, M.A. Thermally and solutally convective radiation in mhd stagnation point flow of micropolar nanofluid over a shrinking sheet. Alex. Eng. 2018, 57, 963–971. [Google Scholar] [CrossRef]
- Kumar, B.; Seth, G.S.; Nandkeolyar, R. Regression model and successive linearization approach to analyse stagnation point micropolar nanofluid flow over a stretching sheet in a porous medium with nonlinear thermal radiation. Phys. Scr. 2019, 94, 115211. [Google Scholar] [CrossRef]
- Patel, H.R.; Singh, R. Thermophoresis, brownian motion and non-linear thermal radiation effects on mixed convection mhd micropolar fluid flow due to nonlinear stretched sheet in porous medium with viscous dissipation, joule heating and convective boundary condition. Int. Commun. Heat Mass Transf. 2019, 107, 68–92. [Google Scholar] [CrossRef]
- Zaib, A.; Khan, U.; Shah, Z.; Kumam, P.; Thounthong, P. Optimization of entropy generation in flow of micropolar mixed convective magnetite (Fe3O4) ferroparticle over a vertical plate. Alex. Eng. J. 2019, 58, 1461–1470. [Google Scholar] [CrossRef]
- Ghadikolaei, S.S.; Hosseinzadeh, K.; Hatami, M.; Ganji, D.D. MHD boundary layer analysis for micropolar dusty fluid containing hybrid nanoparticles (Cu-Al2O3) over a porous medium. J. Mol. Liq. 2018, 268, 813–823. [Google Scholar] [CrossRef]
- Subhani, M.; Nadeem, S. Numerical analysis of micropolar hybrid nanofluid. Appl. Nanosci. 2019, 9, 447–459. [Google Scholar] [CrossRef]
- Subhani, M.; Nadeem, S. Numerical investigation into unsteady magnetohydrodynamics flow of micropolar hybrid nanofluid in porous medium. Phys. Scr. 2019, 94, 105220. [Google Scholar] [CrossRef]
- Al-Hanaya, A.M.; Sajid, F.; Abbas, N.; Nadeem, S. Effect of SWCNT and MWCNT on the flow of micropolar hybrid nanofluid over a curved stretching surface with induced magnetic field. Sci. Rep. 2020, 10, 8488. [Google Scholar] [CrossRef]
- Hosseinzadeh, K.; Roghani, S.; Asadi, A.; Mogharrebi, A.; Ganji, D.D. Investigation of micropolar hybrid ferro fluid flow over a vertical plate by considering various base fluid and nanoparticle shape factor. Int. J. Numer. Methods Heat Fluid Flow 2020, 31, 402–417. [Google Scholar] [CrossRef]
- Uddin, M.J.; Kabir, M.N.; Alginahi, Y.M. Lie group analysis and numerical solution of magnetohydrodynamic free convective slip flow of micropolar fluid over a moving plate with heat transfer. Comput. Math. Appl. 2015, 70, 846–856. [Google Scholar] [CrossRef]
- Zohra, F.T.; Uddin, M.J.; Ismail, A.I.M. Magnetohydrodynamic bio-nanoconvective naiver slip flow of micropolar fluid in a stretchable horizontal channel. Heat Transf. Asian Res. 2019, 48, 3636–3656. [Google Scholar] [CrossRef]
- Abdul Latiff, N.A.; Uddin, M.J.; Bég, O.A.; Ismail, A.I. Unsteady forced bioconvection slip flow of a micropolar nanofluid from a stretching/shrinking sheet. Proc. Inst. Mech. Eng. Part N J. Nanomater. Nanoeng. Nanosyst. 2016, 230, 177–187. [Google Scholar] [CrossRef]
- Uddin, M.J.; Bég, O.A.; Ismail, A.I. Radiative convective nanofluid flow past a stretching/shrinking sheet with slip effects. J. Thermophys. Heat Transf. 2015, 29, 513–523. [Google Scholar] [CrossRef]
- Rana, B.M.J.; Arifuzzaman, S.M.; Islam, S.; Reza-E-Rabbi, S.; Hossain, K.E.; Ahmmed, S.F.; Khan, M.S. Swimming of microbes in entropy optimized nano-bioconvective flow of prandtl–erying fluid. Heat Transf. 2022, 51, 5497–5531. [Google Scholar] [CrossRef]
- Khan, W.A.; Ali, M.; Waqas, M.; Shahzad, M.; Sultan, F.; Irfan, M. Importance of convective heat transfer in flow of non-newtonian nanofluid featuring brownian and thermophoretic diffusions. Int. J. Numer. Methods Heat Fluid Flow 2019, 29, 4624–4641. [Google Scholar] [CrossRef]
- Khan, W.A.; Farooq, S.; Kadry, S.; Hanif, M.; Iftikhar, F.J.; Abbas, S.Z. Variable characteristics of viscosity and thermal conductivity in peristalsis of magneto-carreau nanoliquid with heat transfer irreversibilities. Comput. Methods Programs Biomed. 2020, 190, 105355. [Google Scholar] [CrossRef]
- Khan, W.A.; Waqas, M.; Chammam, W.; Asghar, Z.; Nisar, U.A.; Abbas, S.Z. Evaluating the characteristics of magnetic dipole for shear-thinning williamson nanofluid with thermal radiation. Comput. Methods Programs Biomed. 2020, 191, 105396. [Google Scholar] [CrossRef] [PubMed]
- Khan, W.A.; Ali, M.; Irfan, M.; Khan, M.; Shahzad, M.; Sultan, F. A rheological analysis of nanofluid subjected to melting heat transport characteristics. Appl. Nanosci. 2020, 10, 3161–3170. [Google Scholar] [CrossRef]
- Patel, H.R.; Mittal, A.S.; Darji, R.R. MHD flow of micropolar nanofluid over a stretching/shrinking sheet considering radiation. Int. Commun. Heat Mass Transf. 2019, 108, 104322. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Hybrid nanofluid flow past a permeable moving thin needle. Mathematics 2020, 8, 612. [Google Scholar] [CrossRef]
- Yahaya, R.I.; Arifin, N.M.; Nazar, R.; Pop, I. Flow and heat transfer past a permeable stretching/shrinking sheet in Cu−Al2O3/water hybrid nanofluid. Int. J. Numer. Methods Heat Fluid Flow 2020, 30, 1197–1222. [Google Scholar] [CrossRef]
- Sulochana, C.; Aparna, S.R. Unsteady magnetohydrodynamic radiative liquid thin film flow of hybrid nanofluid with thermophoresis and brownian motion. Multidiscip. Modeling Mater. Struct. 2020, 16, 811–834. [Google Scholar] [CrossRef]
- Rana, P.; Shukla, N.; Bég, O.A.; Bhardwaj, A. lie group analysis of nanofluid slip flow with stefan blowing effect via modified buongiorno’s model: Entropy generation analysis. Differ. Equ. Dyn. Syst. 2021, 29, 193–210. [Google Scholar] [CrossRef]
- Mahanthesh, B.; Shehzad, S.A.; Mackolil, J.; Shashikumar, N.S. Heat transfer optimization of hybrid nanomaterial using modified buongiorno model: A sensitivity analysis. Int. J. Heat Mass Transf. 2021, 171, 121081. [Google Scholar] [CrossRef]
- Clancy, L.J. Aerodynamics; Pitman Publishing: London, UK, 1975. [Google Scholar]
- Borrelli, A.; Giantesio, G.; Patria, M.C. An exact solution for the 3D MHD stagnation-point flow of a micropolar fluid. Commun. Nonlinear Sci. Numer. Simul. 2015, 20, 121–135. [Google Scholar] [CrossRef]
- Pritchard, P.J.; Leylegian, J.C. Fox and McDonald’s Introduction to Fluid Mechanics; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2011; ISBN 9780470547557. [Google Scholar]
- Wang, C.Y. Stagnation flow towards a shrinking sheet. Int. J. Non-Linear Mech. 2008, 43, 377–382. [Google Scholar] [CrossRef]
- Hartmann, J. Hg-dynamics I. Theory of the laminar conductive liquid in a homogeneous magnetic field. Det Kgl. Dan. Vidensk. Selskab. Math. Fys. Medd. 1937, 15, 1–28. [Google Scholar]
- Pavlov, K.B. Magnetohydrodynamics flow of an incompressible viscous liquid caused by deformation of plane surface. Magnetohydrodynamics 1974, 10, 507–510. [Google Scholar]
- Ibrahim, W.; Shankar, B.; Nandeppanavar, M.M. MHD stagnation point flow and heat transfer due to nanofluid towards a stretching sheet. Int. J. Heat Mass Transf. 2013, 56, 1–9. [Google Scholar] [CrossRef]
- Ajith Krishnan, R.; Jinshah, B.S. Magnetohydrodynamic power generation. Int. J. Sci. Res. Publ. 2013, 3, 1–11. [Google Scholar] [CrossRef]
- Modest, M.F. Radiative Heat Transfer, 3rd ed.; Academic Press: Oxford, UK, 2013. [Google Scholar]
- Rosseland, S. Astrophysik und Atom-Theoretische Grundlagen; Springer: Berlin/Heidelberg, Germany, 1931; ISBN 9783662245330. [Google Scholar]
- Howell, J.R.; Siegel, R.; Mengüç, M.P. Thermal Radiation Heat Transfer, 5th ed.; CRC Press, Taylor & Fracis Group: Boca Raton, FL, USA, 2010; ISBN 9781466593275. [Google Scholar]
- Muthtamilselvan, M.; Ramya, E.; Doh, D.H. Inclined lorentz force effects on 3d micropolar fluid flow due to a stretchable rotating disks with higher order chemical reaction. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2019, 233, 323–335. [Google Scholar] [CrossRef]
- Rawat, S.K.; Upreti, H.; Kumar, M. Comparative study of mixed convective MHD cu-water nanofluid flow over a cone and wedge using modified buongiorno’s model in presence of thermal radiation and chemical reaction via cattaneo-christov double diffusion model. J. Appl. Comput. Mech. 2021, 7, 1383–1402. [Google Scholar] [CrossRef]
- Singh, K.; Pandey, A.K.; Kumar, M. Numerical solution of micropolar fluid flow via stretchable surface with chemical reaction and melting heat transfer using keller-box method. Propuls. Power Res. 2021, 10, 194–207. [Google Scholar] [CrossRef]
- Xiong, P.Y.; Chu, Y.M.; Ijaz Khan, M.; Khan, S.A.; Abbas, S.Z. Entropy optimized darcy-forchheimer flow of reiner-philippoff fluid with chemical reaction. Comput. Theor. Chem. 2021, 1200, 113222. [Google Scholar] [CrossRef]
- Merkin, J.H. On dual solutions occurring in mixed convection in a porous medium. J. Eng. Math. 1986, 20, 171–179. [Google Scholar] [CrossRef]
- Weidman, P.D.; Kubitschek, D.G.; Davis, A.M.J. The effect of transpiration on self-similar boundary layer flow over moving surfaces. Int. J. Eng. Sci. 2006, 44, 730–737. [Google Scholar] [CrossRef]
- Harris, S.D.; Ingham, D.B.; Pop, I. Mixed convection boundary-layer flow near the stagnation point on a vertical surface in a porous medium: Brinkman model with slip. Transp. Porous Media 2009, 77, 267–285. [Google Scholar] [CrossRef]
- Shampine, L.F.; Gladwell, I.; Thompson, S. Solving ODEs with MATLAB; Cambridge University Press: Cambridge, UK, 2003; ISBN 9780521824040. [Google Scholar]
- Pantokratoras, A. A common error made in investigation of boundary layer flows. Appl. Math. Model. 2009, 33, 413–422. [Google Scholar] [CrossRef]


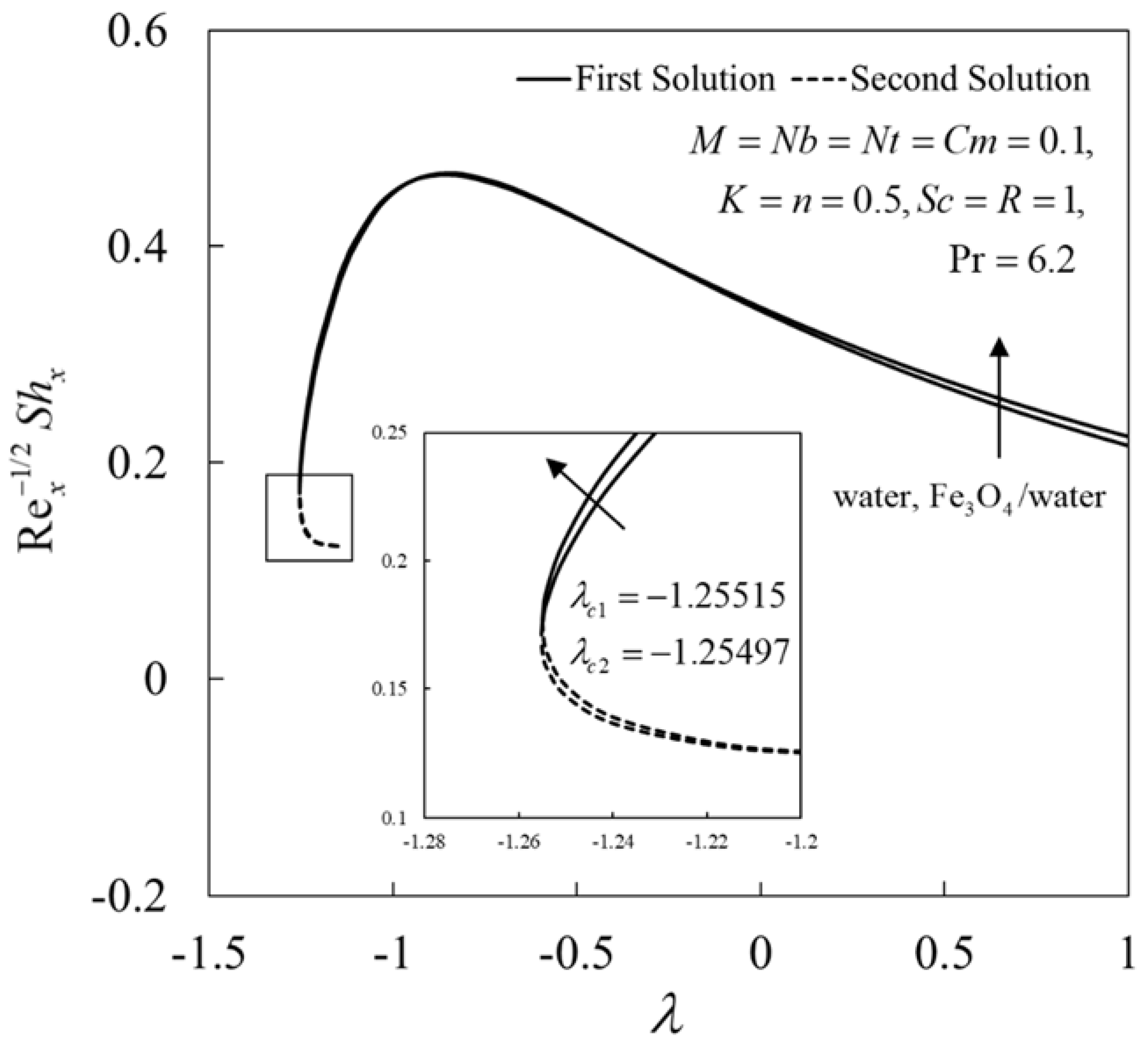
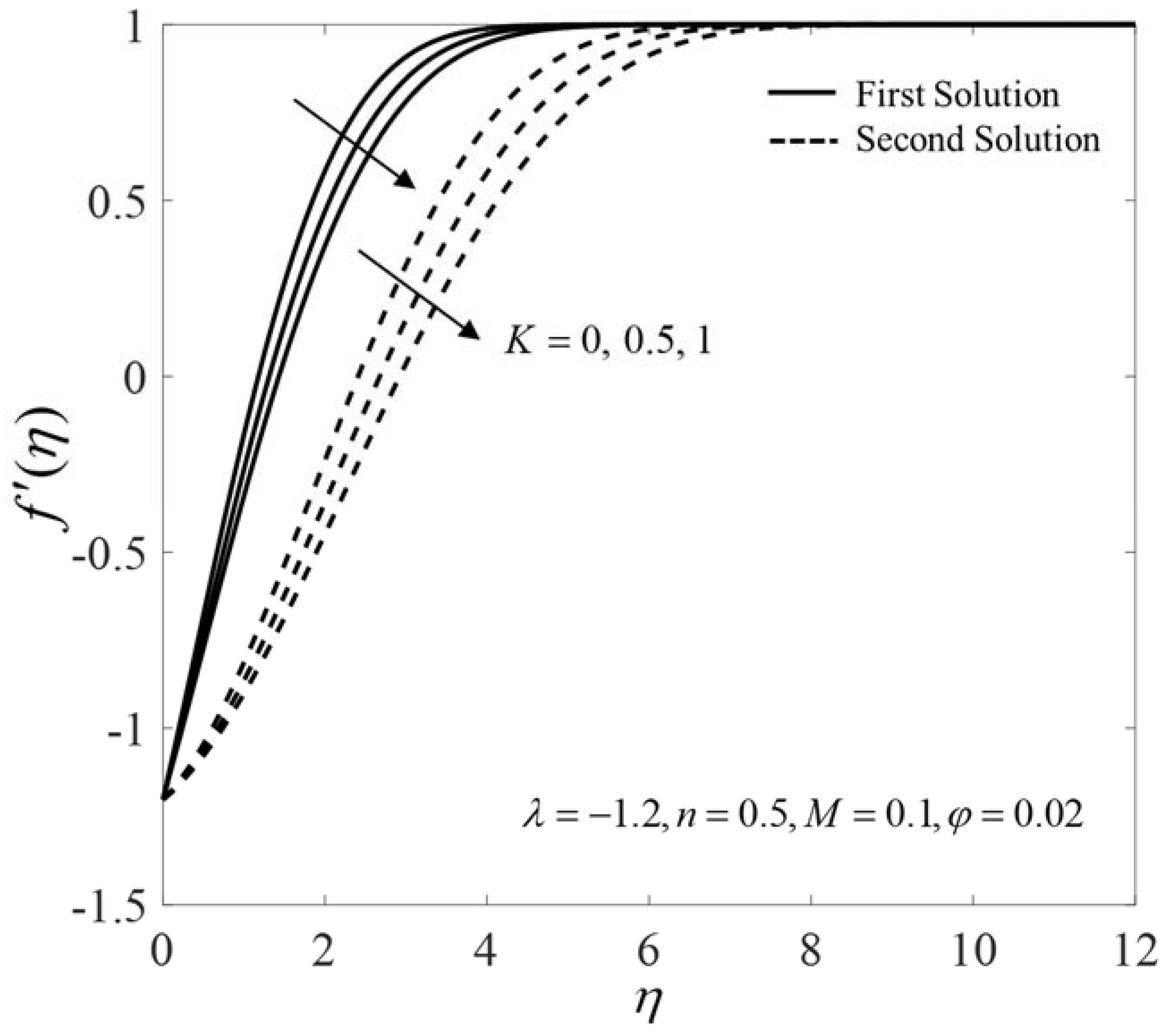

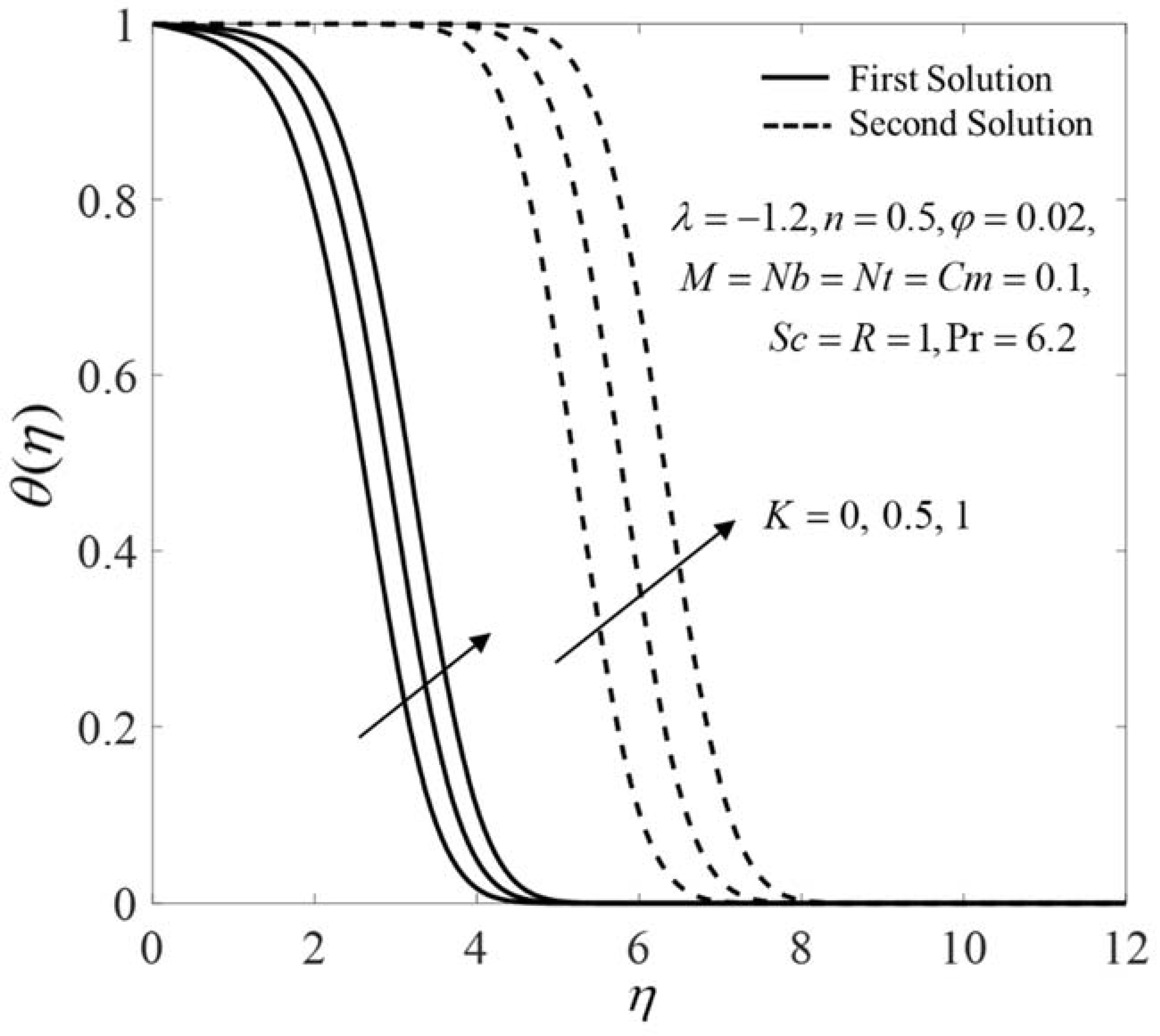

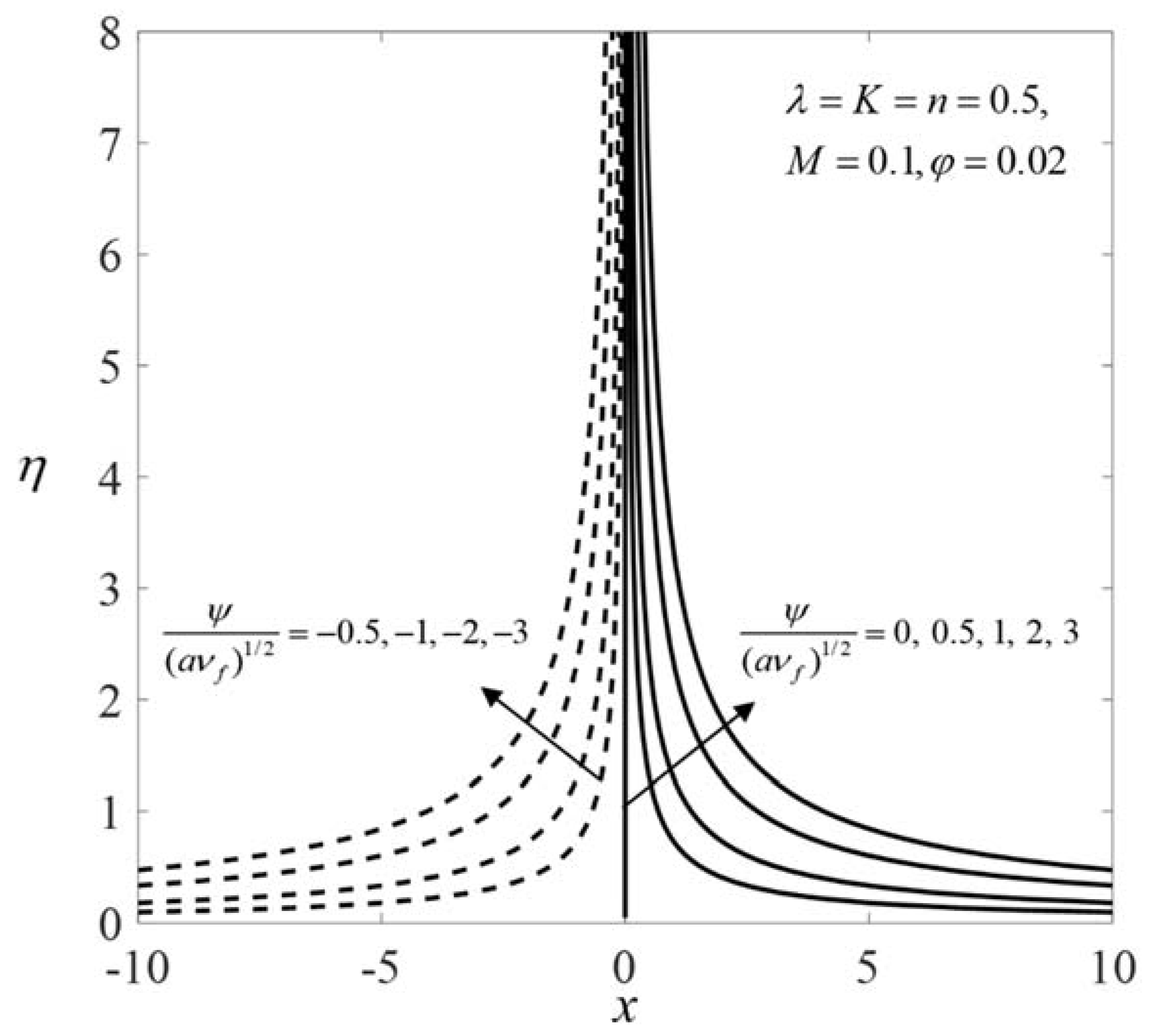
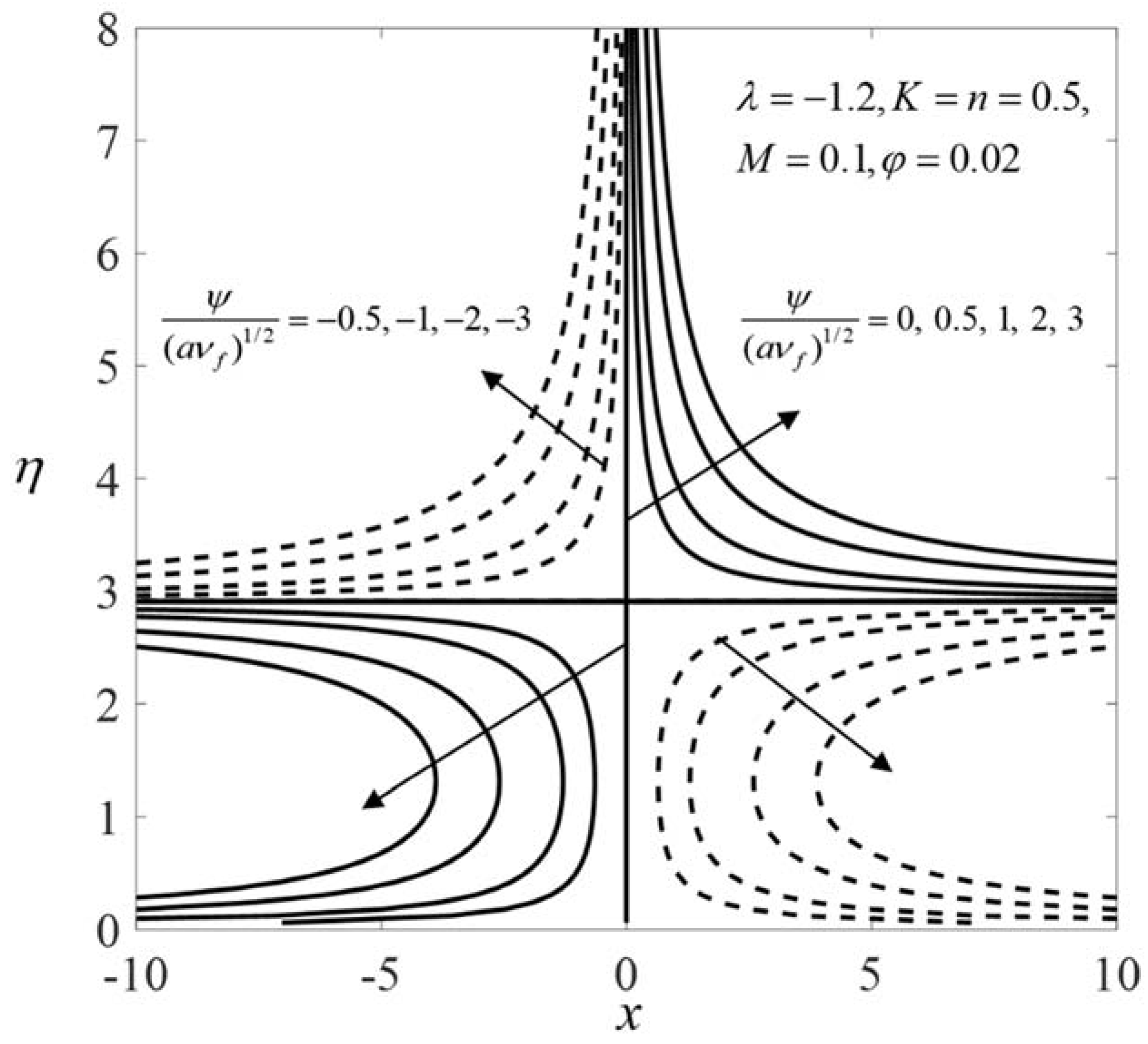
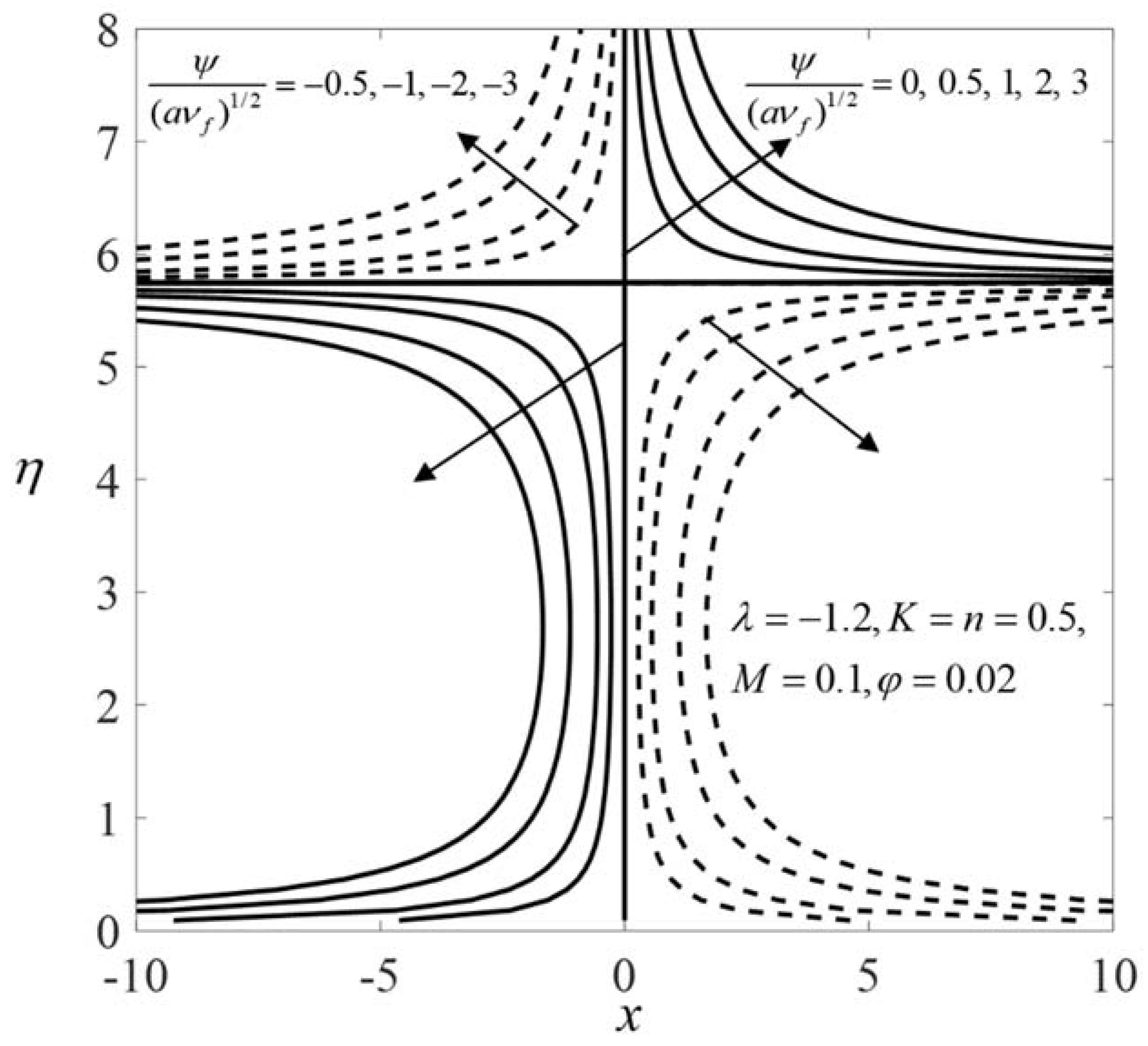
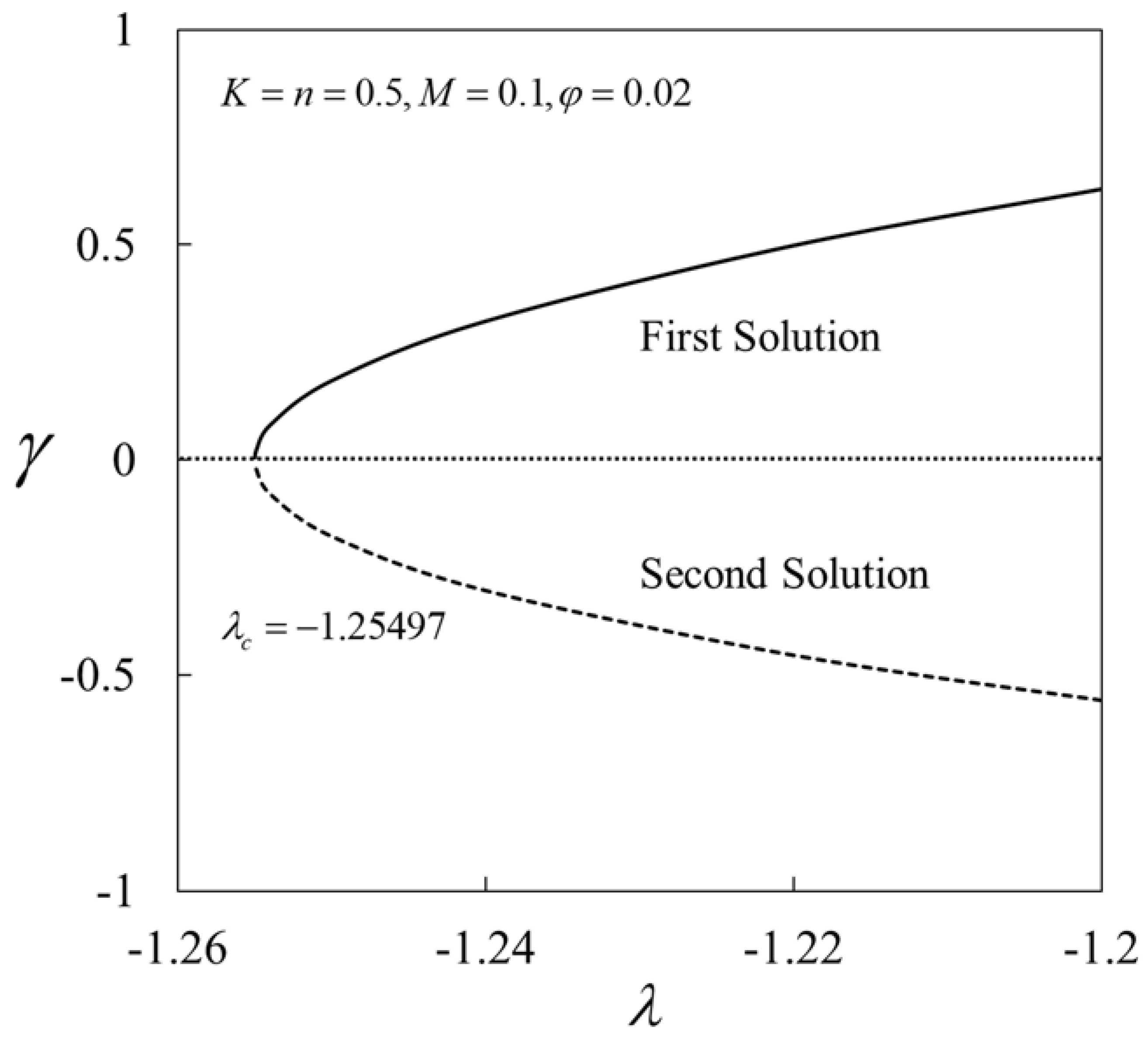
| Properties | Fe3O4 | Water |
|---|---|---|
| 5200 | 997.1 | |
| 670 | 4179 | |
| 6 | 0.613 | |
| 25,000 | 0.05 |
| Properties | Correlations |
|---|---|
| Dynamic viscosity | |
| Density | |
| Heat capacity | |
| Thermal conductivity | |
| Electrical conductivity |
| Wang [55] | Ishak et al. [13] | Present Results | Ishak et al. [13] | Present Results | |
|---|---|---|---|---|---|
| 0 | 1.232588 | 1.232588 | 1.232588 | 1.006404 | 1.006404 |
| 0.1 | 1.146560 | 1.146561 | 1.146561 | 0.936163 | 0.936163 |
| 0.2 | 1.051130 | 1.051130 | 1.051130 | 0.858244 | 0.858244 |
| 0.5 | 0.713300 | 0.713295 | 0.713295 | 0.582403 | 0.582403 |
| 1 | 0 | 0 | 0 | 0 | 0 |
| 2 | −1.887310 | −1.887307 | −1.887307 | −1.540979 | −1.540979 |
| 5 | −10.264750 | −10.264749 | −10.264749 | −8.381133 | −8.381133 |
| 0 | 0.5 | 0.1 | 0.1 | 0.1 | 1 | 0.1 | 1.082171 | −0.213464 | 0.016944 | 0.303031 |
| 0.01 | 1.115482 | −0.222586 | 0.019374 | 0.307476 | ||||||
| 0.02 | 1.149114 | −0.231704 | 0.021913 | 0.311477 | ||||||
| 0.02 | 0 | 1.034033 | −0.233661 | 0.045570 | 0.355461 | |||||
| 0.2 | 1.081306 | −0.232588 | 0.033971 | 0.337046 | ||||||
| 1 | 1.254442 | −0.230912 | 0.010571 | 0.274514 | ||||||
| 0.5 | 0 | 1.107859 | −0.238547 | 0.018468 | 0.302171 | |||||
| 0.5 | 1.779708 | −0.086577 | 0.114729 | 0.415243 | ||||||
| 1 | 2.855578 | 0.278692 | 0.356704 | 0.475854 | ||||||
| 0.1 | 0.15 | 1.149114 | −0.231704 | 0.020172 | 0.276710 | |||||
| 0.2 | 1.149114 | −0.231704 | 0.018555 | 0.259458 | ||||||
| 0.3 | 1.149114 | −0.231704 | 0.015666 | 0.242458 | ||||||
| 0.1 | 0.05 | 1.149114 | −0.231704 | 0.023474 | 0.260632 | |||||
| 0.15 | 1.149114 | −0.231704 | 0.020467 | 0.361070 | ||||||
| 0.2 | 1.149114 | −0.231704 | 0.019129 | 0.409408 | ||||||
| 0.1 | 0 | 1.149114 | −0.231704 | 0.000078 | 0.299632 | |||||
| 1.5 | 1.149114 | −0.231704 | 0.060479 | 0.309752 | ||||||
| 2 | 1.149114 | −0.231704 | 0.118147 | 0.304931 | ||||||
| 1 | 0 | 1.149114 | −0.231704 | 0.022498 | 0.180335 | |||||
| 0.15 | 1.149114 | −0.231704 | 0.021626 | 0.377014 | ||||||
| 0.2 | 1.149114 | −0.231704 | 0.021343 | 0.442529 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Waini, I.; Ishak, A.; Lok, Y.Y.; Pop, I. Micropolar Nanofluid Flow in a Stagnation Region of a Shrinking Sheet with Fe3O4 Nanoparticles. Mathematics 2022, 10, 3184. https://doi.org/10.3390/math10173184
Waini I, Ishak A, Lok YY, Pop I. Micropolar Nanofluid Flow in a Stagnation Region of a Shrinking Sheet with Fe3O4 Nanoparticles. Mathematics. 2022; 10(17):3184. https://doi.org/10.3390/math10173184
Chicago/Turabian StyleWaini, Iskandar, Anuar Ishak, Yian Yian Lok, and Ioan Pop. 2022. "Micropolar Nanofluid Flow in a Stagnation Region of a Shrinking Sheet with Fe3O4 Nanoparticles" Mathematics 10, no. 17: 3184. https://doi.org/10.3390/math10173184
APA StyleWaini, I., Ishak, A., Lok, Y. Y., & Pop, I. (2022). Micropolar Nanofluid Flow in a Stagnation Region of a Shrinking Sheet with Fe3O4 Nanoparticles. Mathematics, 10(17), 3184. https://doi.org/10.3390/math10173184







