Study on a Strong and Weak n-Connected Total Perfect k-Dominating set in Fuzzy Graphs
Abstract
:1. Introduction
2. Preliminaries
3. Main Results
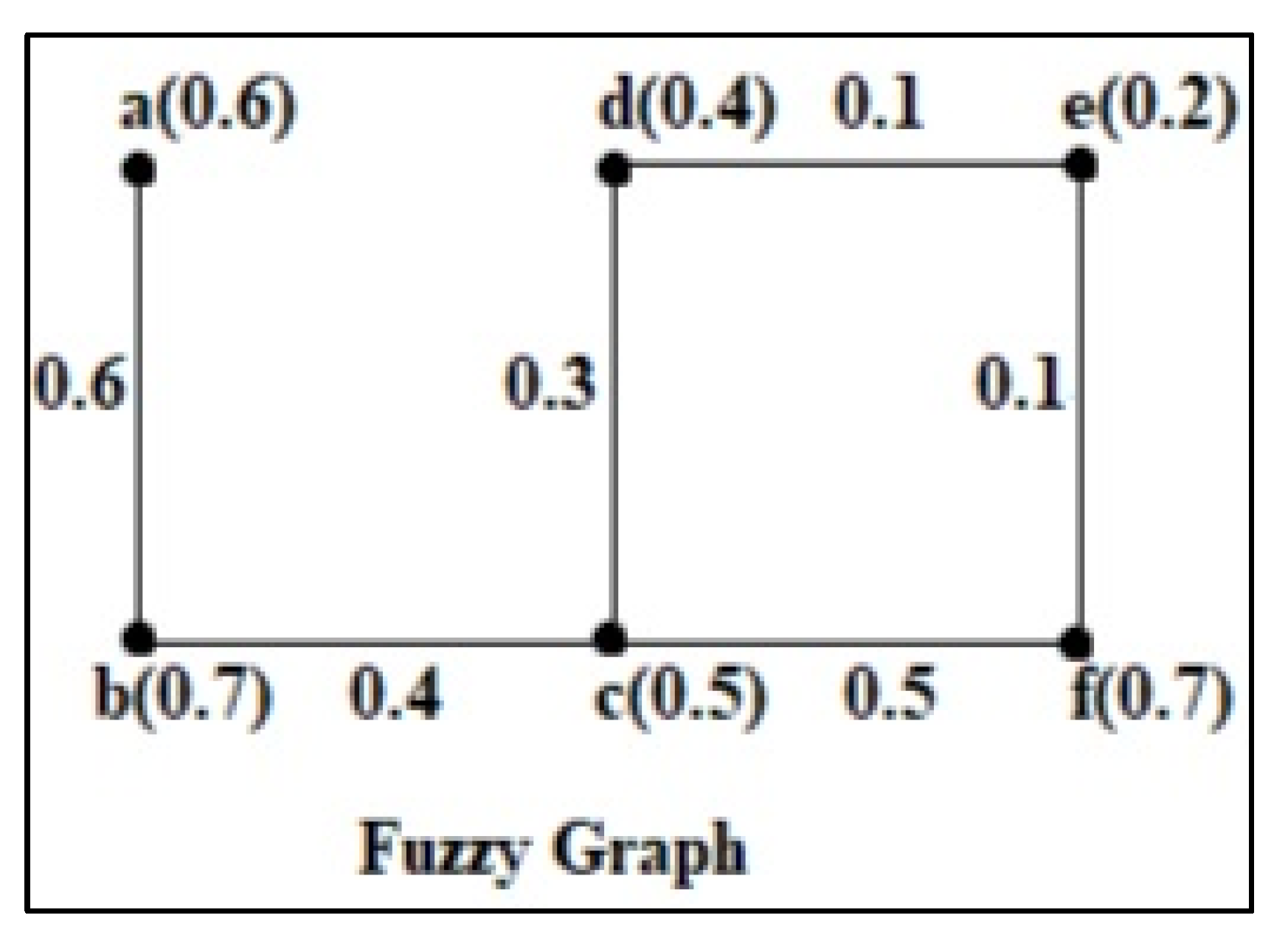
3.1. Strong -Connected Total Perfect -Domination
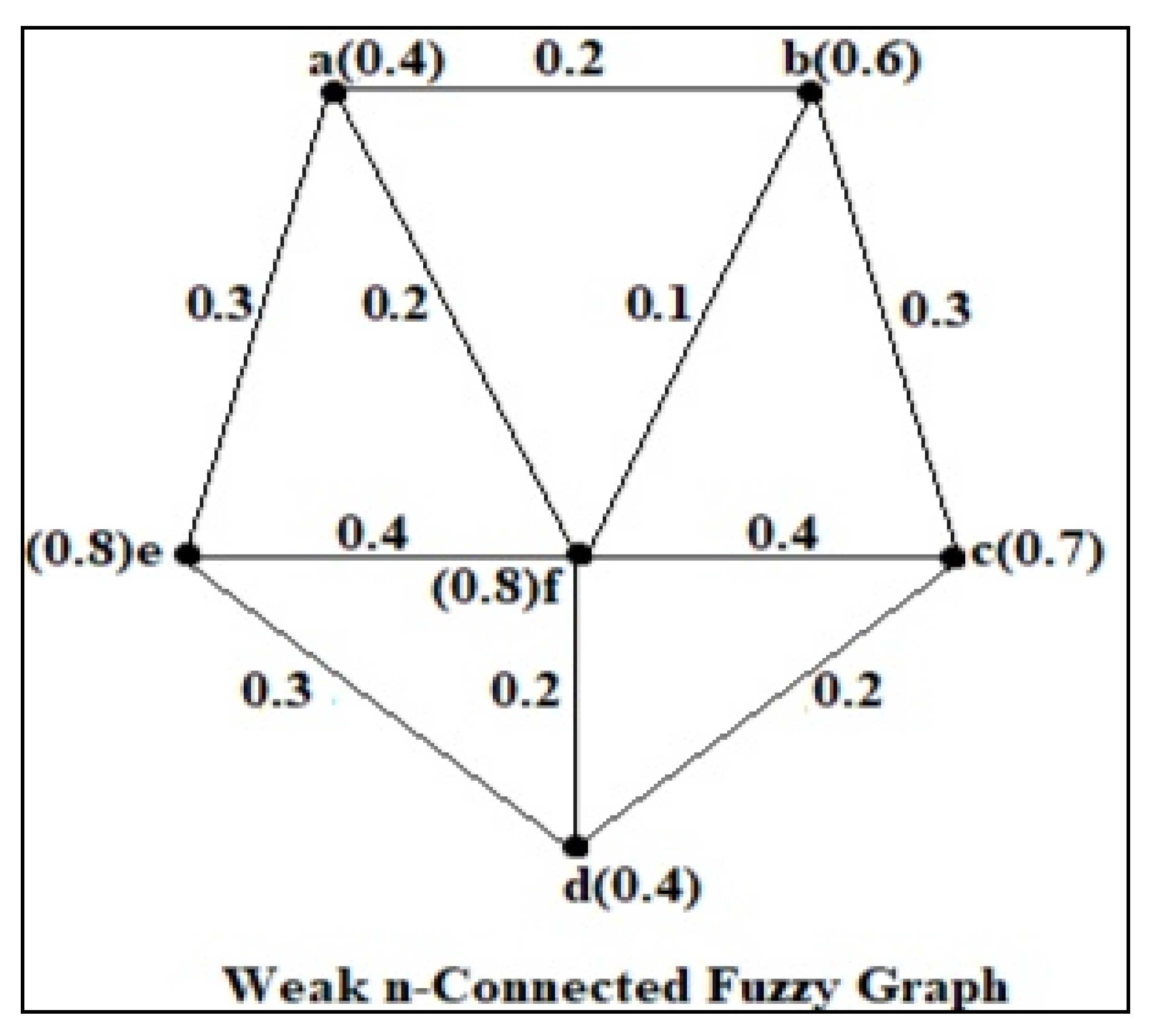
3.2. Weak -Connected Total Perfect -Domination
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zadeh, L.A. Fuzzy sets. Inf. Control. 1965, 8, 338–353. [Google Scholar] [CrossRef]
- Shivakoti, I.; Pradhan, B.B.; Diyaley, S.; Ghadai, R.K.; Kalita, K. TOPSIS-based selection of laser beam micro-marking process parameters. Arab. J. Sci. Eng. 2017, 42, 4825–4831. [Google Scholar] [CrossRef]
- Bansod, A.V.; Patil, A.P.; Kalita, K.; Deshmukh, B.D.; Khobragade, N. Fuzzy multicriteria decision-making-based optimal Zn–Al alloy selection in corrosive environment. Int. J. Mater. Res. 2020, 111, 953–963. [Google Scholar] [CrossRef]
- Ghosh, G.; Roy, S.; Merdji, A. A proposed health monitoring system using fuzzy inference system. Proc. Inst. Mech. Eng. Part H J. Eng. Med. 2020, 234, 562–569. [Google Scholar] [CrossRef] [PubMed]
- Kauffman, A. Introduction a la Theorie des Sousemsembles Flous; Masson et Cie Editeurs: Paris, UK, 1973. [Google Scholar]
- Rosenfeld, A. Fuzzy Graphs, Fuzzy Sets and Their Applications to Cognitive and Decision Processes; Academic Press: New York, NY, USA, 1975; pp. 77–95. [Google Scholar] [CrossRef]
- Mordeson, J.N.; Nair, P.S. Fuzzy Graphs and Fuzzy Hypergraphs; Springer: Berlin/Heidelberg, Germany, 2000. [Google Scholar] [CrossRef]
- Sunitha, M.S.; Kumar, A.V. Complement of a fuzzy graph. Indian J. Pure Appl. Math. 2002, 33, 1451–1464. [Google Scholar]
- Revathi, S.; Harinarayanan, C.V.R.; Muthuraj, R. Strong and Weak perfect domination in fuzzy graph. Int. J. Math. Sci. 2016, 36, 1841–1847. [Google Scholar]
- Mathew, S.; Sunitha, M.S. Types of arcs in a fuzzy graph. Inf. Sci. 2009, 179, 1760–1768. [Google Scholar] [CrossRef]
- Haynes, T.W.; Hedetniemi, S.T.; Slater, P.J. Fundamentals of Domination in Graphs; Marcel Dekker: New York, NY, USA, 1998. [Google Scholar]
- Jäntschi, L.; Bolboacă, S.D. Informational entropy of B-ary trees after a vertex cut. Entropy 2008, 10, 576–588. [Google Scholar] [CrossRef]
- Somasundaram, A.; Somasundaram, S. Domination in Fuzzy Graphs-I. Pattern Recognit. Lett. 1998, 19, 787–791. [Google Scholar] [CrossRef]
- Somasundaram, A. Domination in fuzzy graphs-II. J. Fuzzy Math. 2005, 13, 281–288. [Google Scholar] [CrossRef]
- Manjusha, O.T.; Sunitha, M.S. Total Domination in Fuzzy Graphs Using Strong Arcs. Ann. Pure Appl. Math. 2014, 9, 23–33. [Google Scholar] [CrossRef]
- Manjusha, O.T.; Sunitha, M.S. Strong domination in fuzzy graphs. Fuzzy Inf. Eng. 2015, 7, 369–377. [Google Scholar] [CrossRef]
- Nagoor Gani, A.; Basher Ahamed, M. Order and size in Fuzzy Graphs. Bull. Pure Appl. Sci. 2003, 22E, 145–148. [Google Scholar]
- Nagoor Gani, A.; Chandrasekaran, V.T. Domination in Fuzzy Graphs. Adv. Fuzzy Sets Syst. 2006, 1, 17–26. [Google Scholar]
- Nagoor Gani, A.; Basheer Ahamed, M. Strong and Weak domination in fuzzy graphs. East Asian Math. J. 2007, 23, 1–8. [Google Scholar]
- Bhutani, K.R.; Rosenfeld, A. Strong arcs in Fuzzy graphs. Inf. Sci. 2003, 152, 319–322. [Google Scholar] [CrossRef]
- Senthilraj, S. A Study on Double, Triple and N—Tuple Domination of Fuzzy Graphs. Int. J. Comput. Appl. 2014, 107, 8887. [Google Scholar] [CrossRef]
- Shanga, W.; Wanb, P.; Yaoc, F.; Hua, X. Algorithms for minimum m-connected k-tuple dominating set problem. Theor. Comput. Sci. 2007, 381, 241–247. [Google Scholar] [CrossRef]
- Nagoorgani, A.; Vasantha Gowri, S. A Study on Fuzzy K-Domination Using Strong Arc. Int. J. Fuzzy Math. Arch. 2015, 7, 203–211. [Google Scholar]
- Bharathi, P. A Note on K-Domination in Fuzzy Graphs. Int. J. Fuzzy Math. Syst. 2014, 4, 121–124. [Google Scholar]
- Chaluvaraju, B.; Chellali, M.; Vidya, K.A. Perfect k-domination in graphs. Australas. J. Comb. 2010, 48, 175–184. [Google Scholar]
- Elavarasan, K.; Gunasekar, T. A study on triple connected total perfect domination in fuzzy graphs. Adv. Math. Sci. J. 2020, 9, 93–100. [Google Scholar] [CrossRef]
- Gunasekar, T.; Elavarasan, K. A study on n-connected total perfect k-domination in fuzzy graphs with Application. Int. J. Mech. Eng. 2022, 7, 109–113. [Google Scholar]
- Natrajan, C.; Ayyaswamy, S.K. on strong (weak) domination in fuzzy graph. World Acad. Sci. Eng. Technol. 2010, 43, 526–528. [Google Scholar]
- Revathi, S.; Harinarayanan, C.V.R.; Jayalakshmi, P.J. Perfect Dominating Sets in Fuzzy Graphs. IOSR J. Math. 2013, 8, 43–47. [Google Scholar] [CrossRef]
- Revathi, S.; Harinarayanan, C.V.R.; Muthuraj, R. Connected perfect domination in fuzzy graph. Gold. Res. Thoughts 2015, 5, 1–5. [Google Scholar]
- Revathi, S.; Harinarayanan, C.V.R.; Muthuraj, R. Strong (Weak) Triple Connected Perfect Domination Number of a Fuzzy Graph. Int. J. Eng. Technol. Sci. Res. 2017, 4, 1432–1437. [Google Scholar]
- Sarala, N.; Kavitha, T. Strong (Weak) Triple Connected Domination Number of a Fuzzy Graph. Int. J. Comput. Eng. Res. 2015, 5, 18–22. [Google Scholar]
- Sarala, N.; Kavitha, T. Triple connected domination number of fuzzy graph. Int. J. Appl. Eng. Res. 2015, 10, 914–917. [Google Scholar]
- Kathavarayan, P.; Gunasekar, T.; Elavarasan, K. Some standard results on triple connected total perfect domination of fuzzy graph. Int. J. Future Gener. Commun. Netw. 2020, 13, 952–958. [Google Scholar]
- Porcuna-Enguix, L.; Bustos-Contell, E.; Serrano-Madrid, J.; Labatut-Serer, G. Constructing the Audit Risk Assessment by the Audit Team Leader When Planning: Using Fuzzy Theory. Mathematics 2021, 9, 3065. [Google Scholar] [CrossRef]
- Tomescu, M.A.; Jäntschi, L.; Rotaru, D.I. Figures of graph partitioning by counting, sequence and layer matrices. Mathematics 2021, 9, 1419. [Google Scholar] [CrossRef]
- Shoaib, M.; Mahmood, W.; Xin, Q.; Tchier, F. Certain operations on picture fuzzy graph with application. Symmetry 2021, 13, 2400. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Elavarasan, K.; Gunasekar, T.; Cepova, L.; Cep, R. Study on a Strong and Weak n-Connected Total Perfect k-Dominating set in Fuzzy Graphs. Mathematics 2022, 10, 3178. https://doi.org/10.3390/math10173178
Elavarasan K, Gunasekar T, Cepova L, Cep R. Study on a Strong and Weak n-Connected Total Perfect k-Dominating set in Fuzzy Graphs. Mathematics. 2022; 10(17):3178. https://doi.org/10.3390/math10173178
Chicago/Turabian StyleElavarasan, Krishnasamy, Tharmalingam Gunasekar, Lenka Cepova, and Robert Cep. 2022. "Study on a Strong and Weak n-Connected Total Perfect k-Dominating set in Fuzzy Graphs" Mathematics 10, no. 17: 3178. https://doi.org/10.3390/math10173178
APA StyleElavarasan, K., Gunasekar, T., Cepova, L., & Cep, R. (2022). Study on a Strong and Weak n-Connected Total Perfect k-Dominating set in Fuzzy Graphs. Mathematics, 10(17), 3178. https://doi.org/10.3390/math10173178










