A Review of Non-Destructive Evaluation (NDE) Techniques for Residual Stress Profiling of Metallic Components in Aircraft Engines
Abstract
1. Introduction
2. Diffraction Techniques
2.1. X-ray Diffraction
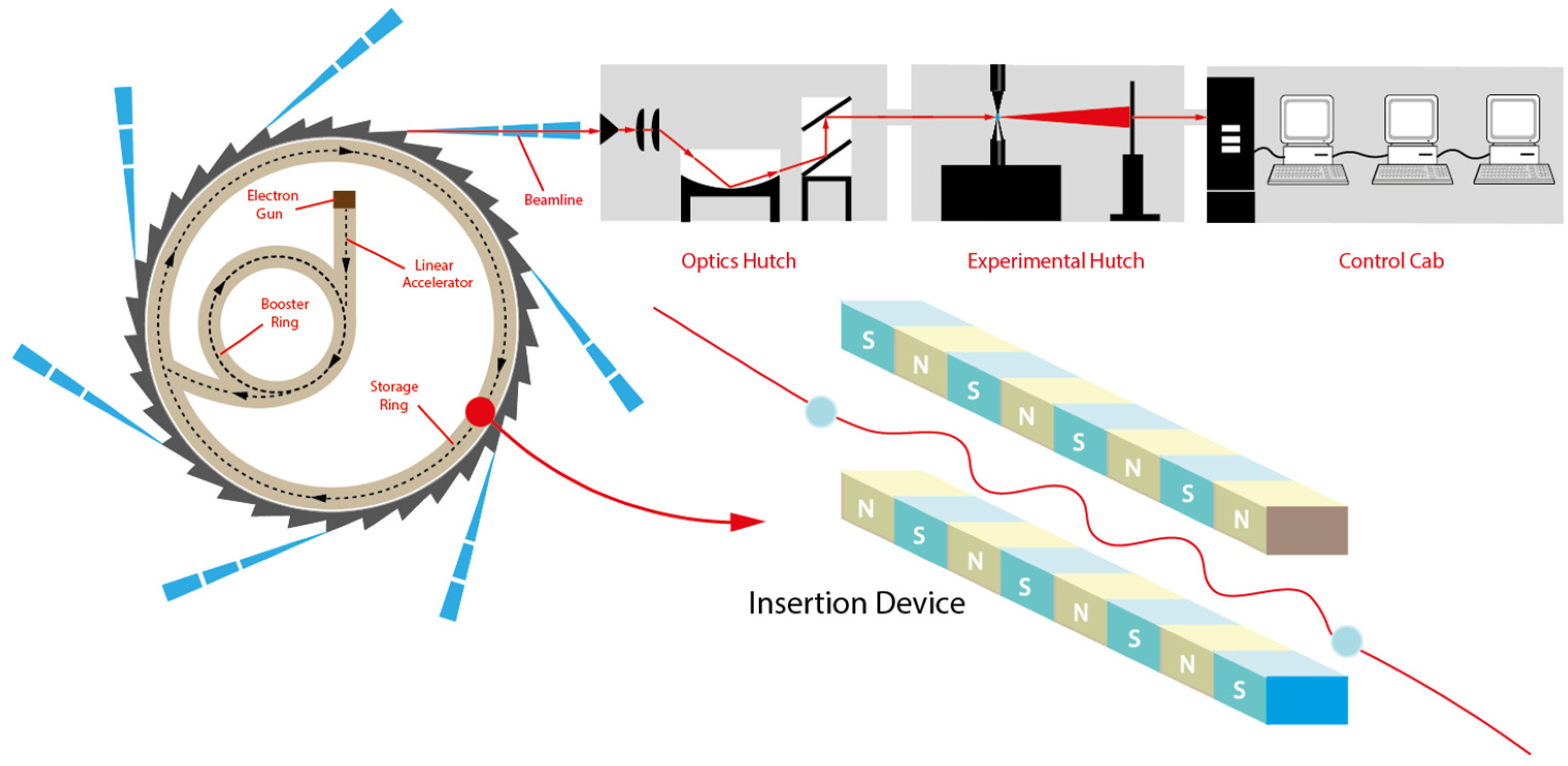
2.2. Synchrotron X-ray Diffraction
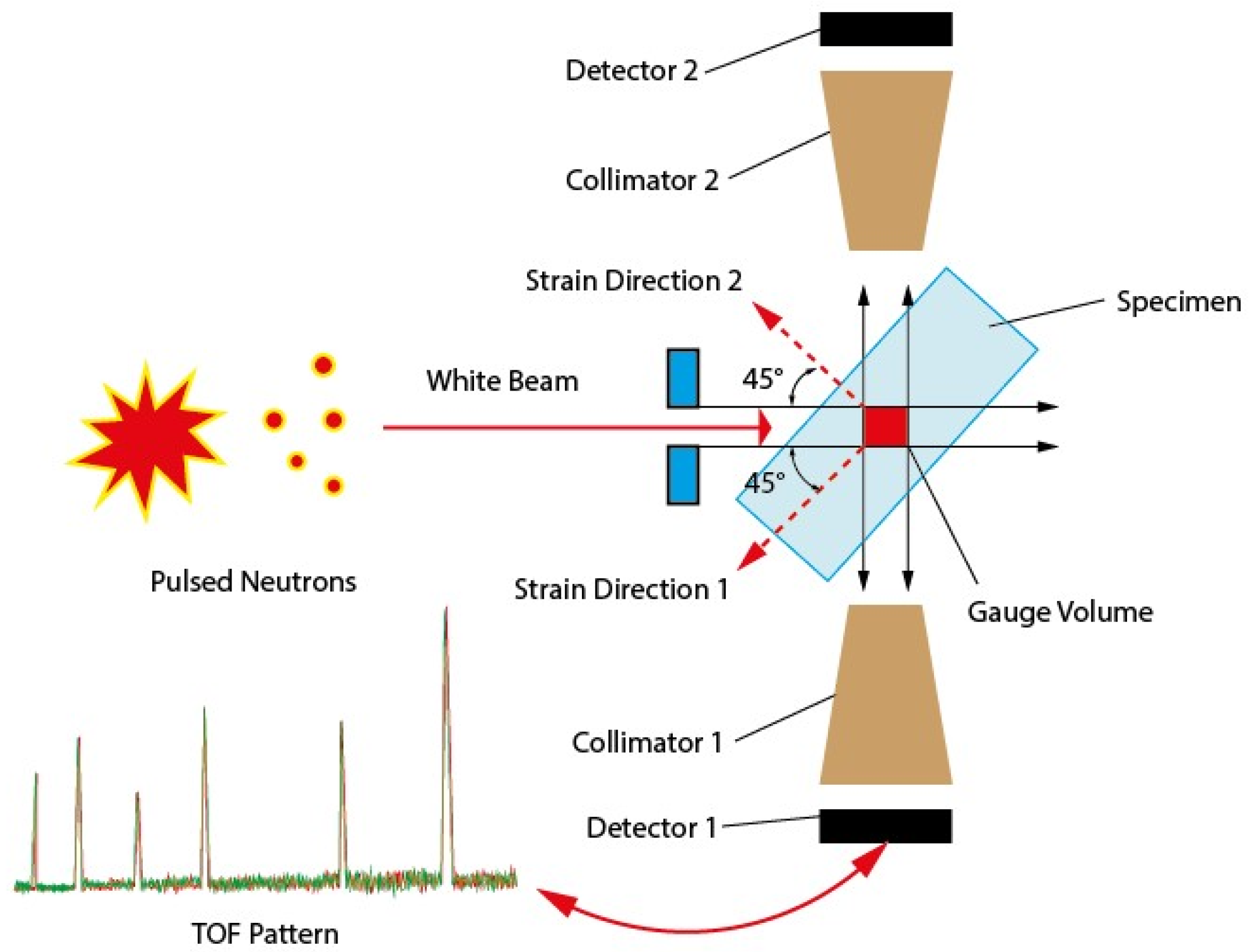
2.3. Neutron Diffraction
3. Ultrasonic Techniques
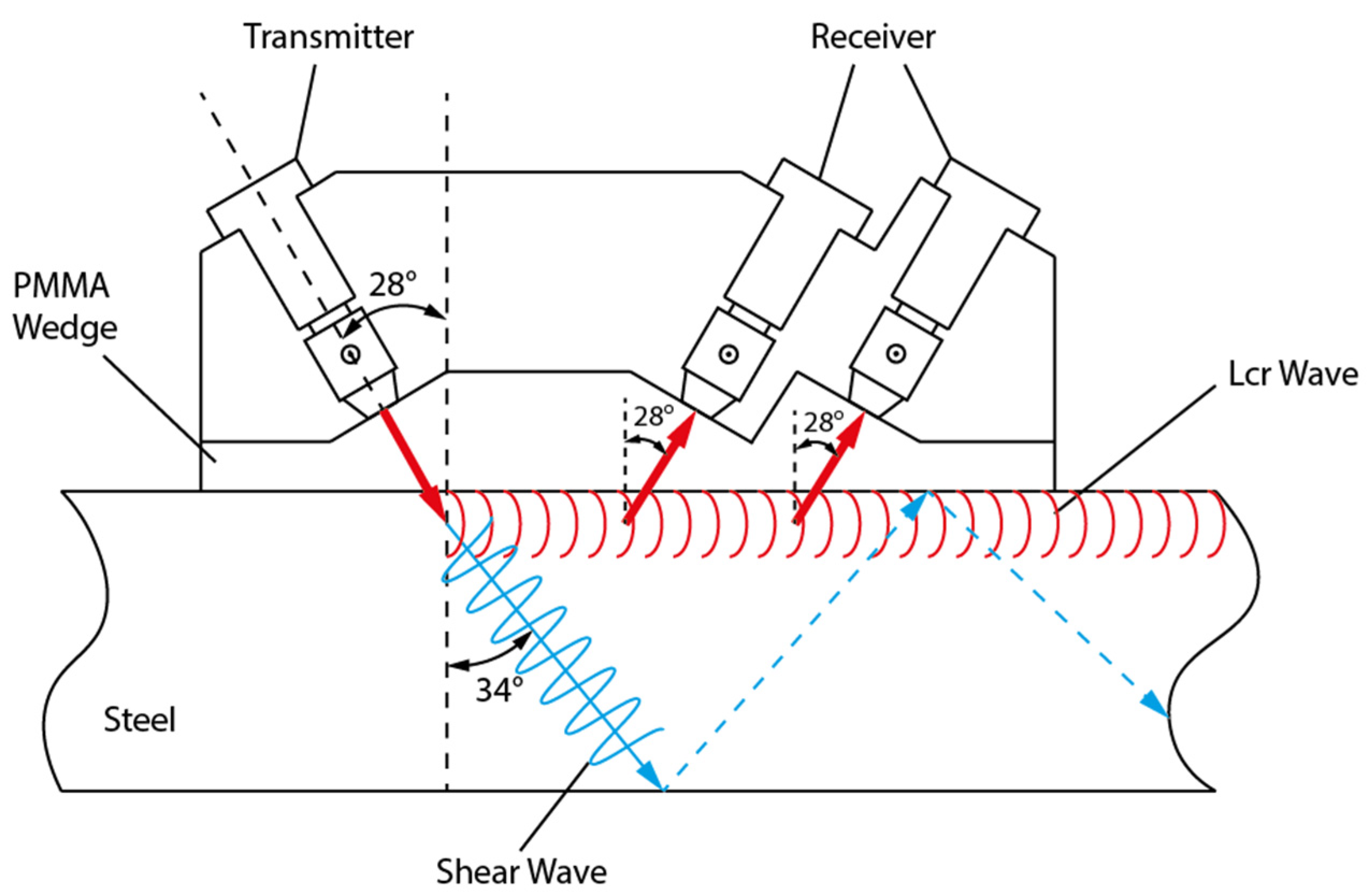
3.1. Critically Refracted Longitudinal () Wave
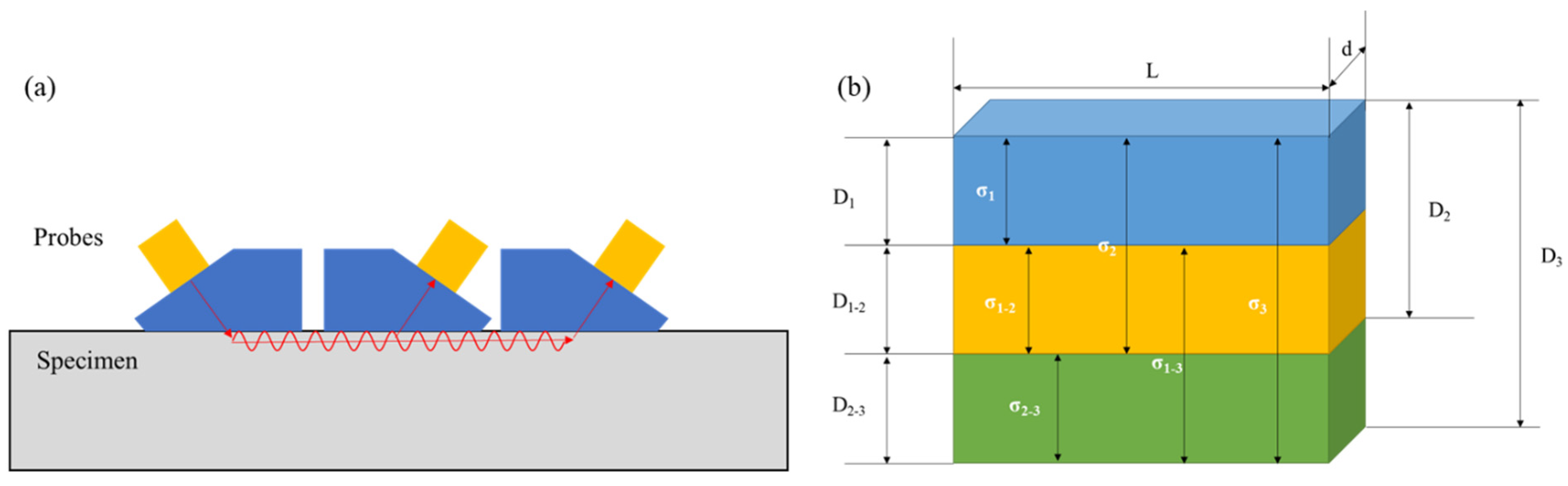
3.2. Rayleigh Wave
4. Eddy Current
4.1. Influence of Surface Roughness
4.2. Influence of Cold Work
4.3. Influence of Hardness and Microstructure
5. Hall Coefficient
6. Future Trends of Residual Stress Profiling
6.1. Stress Measurement via Data Fusion of Different NDE Techniques
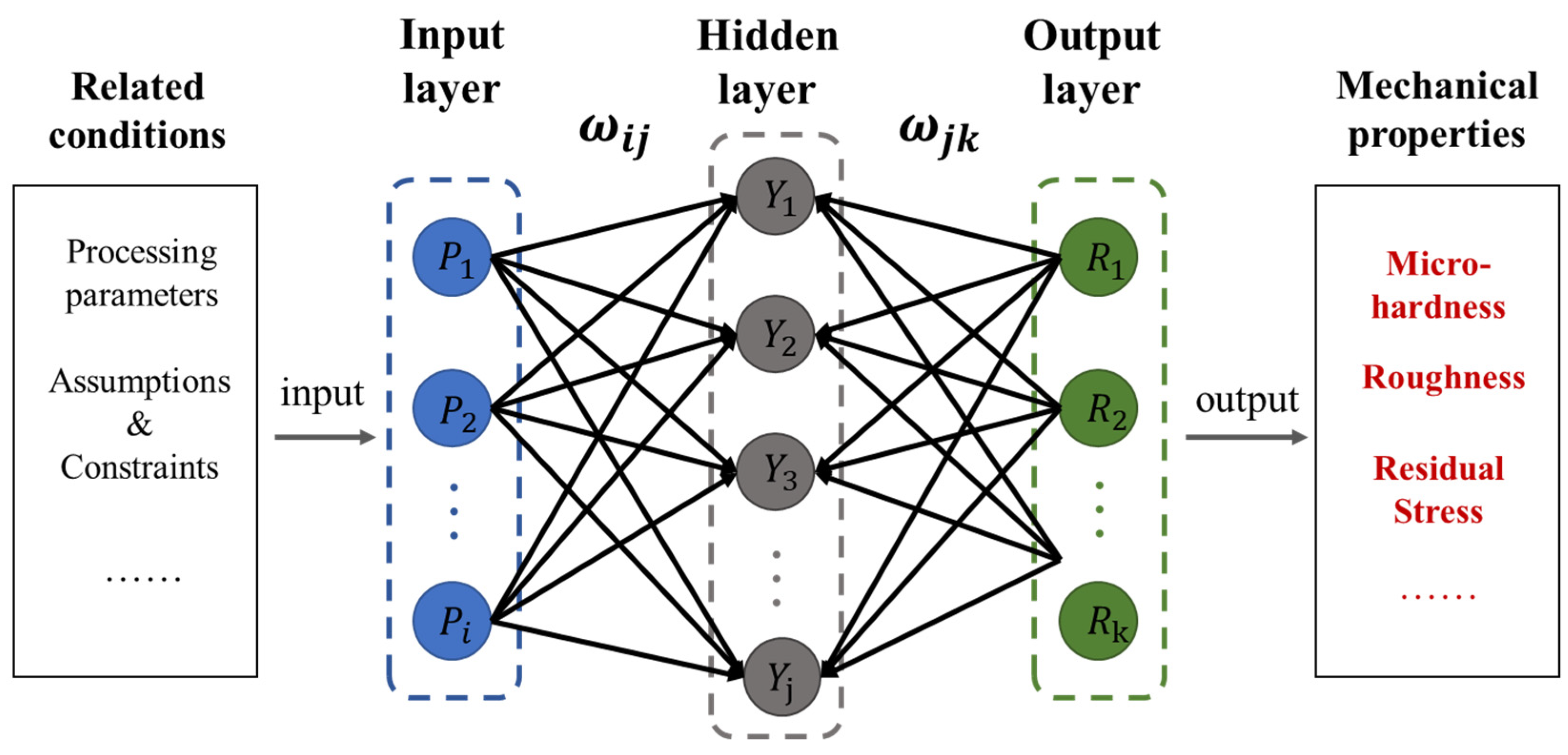
6.2. Strengthened Stress Prediction Models Based on Machine Learning
6.3. Potential SHM Strategy for Residual Stresses
7. Discussions
8. Conclusions
- The diffraction techniques are now mature and well-established for residual stress measurement with widespread applications and usually act as a calibration tool to validate the results obtained by other techniques. Synchrotron and neutron experiments can be performed in either reflection or transmission configuration with a monochromatic or white beam (full spectrum) source [65,205]. Synchrotron X-ray diffraction and neutron diffraction methods are capable of 3D mapping of residual stresses thanks to their perfect penetration depth. However, the very high cost and limited access to the facilities restrict these two methods as being only applicable to a few components of interest.
- Critically refracted longitudinal wave, Raleigh wave, eddy current, and Hall coefficient all exhibit frequency-dependent penetration depth and, therefore, are suitable for depth-dependent residual stress measurement. A common challenge for these techniques is the competing effects other than residual stress.
- Ultrasonic testing methods using or Rayleigh waves are sensitive to residual stress. Ultrasonic methods can measure the average stress volumetrically and obtain the residual stress at varied depths through inversion schemes. It is worth noting that crystallographic texture, surface roughness, and cold work will also influence the ultrasonic velocity measurements, which remains a challenge for accurately characterising residual stresses via ultrasonics-based techniques.
- Eddy current technique has been demonstrated to be successful for residual stress profiling in certain nickel-based superalloys, such as IN100 and Waspaloy, in the past two decades. However, it has also been found that in other aircraft engine materials such as IN718 and Ti64, the residual stress influence on the conductivity is obscured or even overshadowed by cold work.
- The Hall coefficient technique has been proposed recently, and it is anticipated that it can be applied to more materials than eddy current because the cold work influence on Hall coefficient is in the same direction as residual stress in the recently studied aircraft engine materials. The high-frequency inductive sensing for Hall coefficient measurement has been validated very recently and can potentially be exploited for sub-surface residual stress profiling. The dual-mode Hall impedance and eddy current conductivity measurements enable the feasibility of separating the competing residual stress and cold work contributions in surface-enhanced aero-engine materials. The Hall coefficient technique has great potential, yet still requires more research efforts.
- The separation of residual stress information requires a quantitative understanding of the coupling factors such as cold work, surface roughness, microstructure, etc. Due to the different sensitivities of these competing factors by the reviewed techniques, it is worth establishing inspection protocols that employ a combination of non-destructive techniques to obtain a more accurate and reliable residual stress profile.
- The selectivity rather than sensitivity is a more important consideration for non-destructive residual stress profiling techniques. An exclusively stress-related parameter will be extremely valuable for the development of a new non-destructive technique for residual stress profiling of aero-engine components.
- Three categories of future research trends are proposed, including data fusion of different NDE technique, strengthened prediction models based on machine learning, and potential SHM strategy, aiming at improving the accuracy, efficiency, and reliability of residual stress profiling. Nevertheless, more dedicated research efforts are required.
Funding
Conflicts of Interest
Nomenclature
| Lattice spacing of the material under X-ray diffraction test | |
| Diffraction angle | |
| X-rays’ wavelength | |
| Elastic strain | |
| Deviation of lattice spacing under residual stress | |
| Deviation of diffraction angle | |
| Azimuth angle of the measurement system | |
| Stress-free lattice spacing | |
| Planck constant | |
| Speed of light in vacuum | |
| Energy of the photon | |
| Neutron momentum | |
| Neutron mass | |
| Travelling speed of the neutron | |
| The time of flight of the neutron | |
| Flight path of the neutron | |
| Density | |
| Lamé constants | |
| Murnaghan constants | |
| Elastic Strain Components. | |
| Velocity of ultrasonic wave | |
| Stress variation | |
| Young’s modulus | |
| Acoustoelastic constant | |
| Travel time at the stress-free state | |
| Travel time change due to the temperature change | |
| Averaged stress in each layer | |
| Varied stresses | |
| Varied penetration depths of the incident wave | |
| Acoustoelastic coefficients with the superscripts denote the loading directions | |
| Acoustic velocity of SAWs | |
| Generated SAW frequencies | |
| Grating space | |
| Nonlinearity parameter of Rayleigh wave | |
| Driving fundamental frequency of Rayleigh wave | |
| Penetration depth of eddy current | |
| Frequency of eddy current | |
| Permeability of eddy current | |
| Conductivity of eddy current | |
| Isotropic plane stress | |
| Parallel and normal electro-elastic coefficients | |
| Phase and precipitates of nickel-based superalloys | |
| Hall coefficient | |
| Hall voltage | |
| Current passing through the sample | |
| Normal magnetic flux density | |
| Sample thickness | |
| Carrier density | |
| Carrier type | |
| Input layer neuron | |
| Different neurons | |
| Weights used in the ANN | |
| Output |
References
- Schajer, G.S. Practical Residual Stress Measurement Methods; Wiley: Chichester, UK, 2013. [Google Scholar]
- Fitzpatrick, M.E.; Lodini, A. Analysis of Residual Stress by Diffraction Using Neutron and Synchrotron Radiation; Taylor & Francis: London, UK, 2003. [Google Scholar]
- Withers, P.; Bhadeshia, H.K.D.H. Residual stress. Part 1: Measurement techniques. Mater. Sci. Technol. 2001, 17, 355–365. [Google Scholar] [CrossRef]
- Xu, S.; Huang, S.; Meng, X.; Sheng, J.; Zhang, H.; Zhou, J. Thermal evolution of residual stress in IN718 alloy subjected to laser peening. Opt. Lasers Eng. 2017, 94, 70–75. [Google Scholar] [CrossRef]
- Tolga Bozdana, A. On the mechanical surface enhancement techniques in aerospace industry—A review of technology. Aircr. Eng. Aerosp. Technol. 2005, 77, 279–292. [Google Scholar] [CrossRef]
- Wang, Z.-M.; Jia, Y.-F.; Zhang, X.-C.; Fu, Y.; Zhang, C.-C.; Tu, S.-T. Effects of Different Mechanical Surface Enhancement Techniques on Surface Integrity and Fatigue Properties of Ti-6Al-4V: A Review. Crit. Rev. Solid State Mater. Sci. 2019, 44, 445–469. [Google Scholar] [CrossRef]
- Youtsos, A.G. Residual Stress and Its Effects on Fatigue and Fracture; Springer: Utrecht, The Netherlands, 2006. [Google Scholar]
- Withers, P.J. Residual stress and its role in failure. Rep. Prog. Phys. 2007, 70, 2211–2264. [Google Scholar] [CrossRef]
- James, M.N. Residual stress influences on structural reliability. Eng. Fail. Anal. 2011, 18, 1909–1920. [Google Scholar] [CrossRef]
- Li, H.Y.; Sun, H.L.; Bowen, P.; Knott, J.F. Effects of compressive residual stress on short fatigue crack growth in a nickel-based superalloy. Int. J. Fatigue 2018, 108, 53–61. [Google Scholar] [CrossRef]
- Nagy, P.B. Opportunities and Challenges for Nondestructive Residual Stress Assessment. Aip Conf. Proc. 2005, 820, 22–40. [Google Scholar]
- Withers, P.J. Residual Stress: Measurement by Diffraction. In Reference Module in Materials Science and Materials Engineering; Elsevier: Amsterdam, The Netherlands, 2016. [Google Scholar]
- Ridsdale, L. Essentials of dental radiography and radiology, 5th ed. Br. Dent. J. 2013, 215, 595–596. [Google Scholar] [CrossRef]
- Noyan, I.C.; Cohen, J.B. Residual Stress: Measurement by Diffraction and Interpretation; Springer: New York, NY, USA, 1987. [Google Scholar]
- James, M.R.; Buck, O. Quantitative nondestructive measurements of residual stresses. Crit. Rev. Solid State Mater. Sci. 1980, 9, 61–105. [Google Scholar] [CrossRef]
- Kumar, D.; Idapalapati, S.; Wang, W.; Child, D.J.; Haubold, T.; Wong, C.C. Microstructure-mechanical property correlation in shot peened and vibro-peened Ni-based superalloy. J. Mater. Processing Technol. 2019, 267, 215–229. [Google Scholar] [CrossRef]
- Wong, C.C.; Hartawan, A.; Teo, W.K. Deep Cold Rolling of Features on Aero-Engine Components. Procedia CIRP 2014, 13, 350–354. [Google Scholar] [CrossRef]
- Chin, K.S.; Idapalapati, S.; Ardi, D.T. Thermal stress relaxation in shot peened and laser peened nickel-based superalloy. J. Mater. Sci. Technol. 2020, 59, 100–106. [Google Scholar] [CrossRef]
- Maleki, E.; Unal, O.; Guagliano, M.; Bagherifard, S. The effects of shot peening, laser shock peening and ultrasonic nanocrystal surface modification on the fatigue strength of Inconel 718. Mater. Sci. Eng. A 2021, 810, 141029. [Google Scholar] [CrossRef]
- Prevéy, P.S. The Effect of Cold Work on the Thermal Stability of Residual Compression in Surface Enhanced IN718. In Proceedings of the 20th ASM Materials Solutions Conference & Exposition, St. Louis, MO, USA, 10–12 October 2000. [Google Scholar]
- Ruud, C.O.; Farmer, G.D., Jr. Residual Stress Measurement by X-Rays: Errors, Limitations and Applications. In Nondestructive Evaluation of Materials; Springer: Boston, MA, USA, 1979; pp. 101–115. [Google Scholar]
- Almer, J.; Winholtz, R. X-Ray Stress Analysis. In Springer Handbook of Experimental Solid Mechanics; Sharpe, W.N., Jr., Ed.; Springer: Boston, MA, USA, 2008; pp. 801–820. [Google Scholar]
- Kocks, U.F.; Wenk, H.-R.; Tomé, C.N. Texture and Anisotropy: Preferred Orientations in Polycrystals and Their Effect on Materials Properties; Cambridge University Press: Cambridge, UK, 1998. [Google Scholar]
- Matthies, S.; Priesmeyer, H.G.; Daymond, M.R. On the diffractive determination of single-crystal elastic constants using polycrystalline samples. J. Appl. Crystallogr. 2001, 34, 585–601. [Google Scholar] [CrossRef]
- Bernier, J.V.; Miller, M.P. A direct method for the determination of the mean orientationdependent elastic strains and stresses in polycrystalline materials from strain pole figures. J. Appl. Crystallogr. 2006, 39, 358–368. [Google Scholar] [CrossRef]
- Prevéy, P.S. Problems with non-destructive surface X-ray diffraction residual stress measurement. Shot Peener 1992, 5, 5–11. [Google Scholar]
- Prevéy, P.S. Current applications of X-ray diffraction residual stress measurement. Dev. Mater. Charact. Technol. 1996, pp. 103–110. Available online: https://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.333.8402&rep=rep1&type=pdf (accessed on 10 August 2022).
- Reimers, W.; Broda, M.; Brusch, G.; Dantz, D.; Liss, K.D.; Pyzalla, A.; Schmackers, T.; Tschentscher, T. Evaluation of residual stresses in the bulk of materials by high energy synchrotron diffraction. J. Nondestruct. Eval. 1998, 17, 129–140. [Google Scholar] [CrossRef]
- Liss, K.-D.; Bartels, A.; Schreyer, A.; Clemens, H. High-energy X-rays: A tool for advanced bulk investigations in materials science and physics. Textures Microstruct. 2003, 35, 219–252. [Google Scholar] [CrossRef]
- Willmott, P. An Introduction to Synchrotron Radiation: Techniques and Applications; Wiley: Chichester, UK, 2011. [Google Scholar]
- Duke, P. Synchrotron Radiation: Production and Properties; OUP Oxford: Oxford, UK, 2008. [Google Scholar]
- Ide-Ektessabi, A. Applications of Synchrotron Radiation: Micro Beams in Cell Micro Biology and Medicine; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Beaurepaire, E.; Bulou, H.; Scheurer, F.; Jean-Paul, K. Magnetism and Synchrotron Radiation: New Trends; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Korsunsky, A.M.; Wells, K.E.; Withers, P.J. Mapping Two-Dimensional State of Strain Using Synchroton X-Ray Diffraction. Scr. Mater. 1998, 39, 1705–1712. [Google Scholar] [CrossRef]
- Gill, A.S.; Zhou, Z.; Lienert, U.; Almer, J.; Lahrman, D.F.; Mannava, S.R.; Qian, D.; Vasudevan, V.K. High spatial resolution, high energy synchrotron x-ray diffraction characterization of residual strains and stresses in laser shock peened Inconel 718SPF alloy. J. Appl. Phys. 2012, 111, 084904. [Google Scholar] [CrossRef]
- Leoni, M.; Ortolani, M.; Bertoldi, M.; Sglavo, V.M.; Scardi, P. Nondestructive Measurement of the Residual Stress Profile in Ceramic Laminates. J. Am. Ceram. Soc. 2008, 91, 1218–1225. [Google Scholar] [CrossRef]
- Fultz, B.; Howe, J. Diffraction and the X-Ray Powder Diffractometer. In Transmission Electron Microscopy and Diffractometry of Materials; Springer: Berlin/Heidelberg, Germany, 2001; pp. 1–61. [Google Scholar]
- Fiori, F.; Girardin, E.; Giuliani, A.; Manescu, A.; Rustichelli, F. Neutron and Synchrotron Non-Destructive Methods for Residual Stress Determination in Materials for Industrial Applications. In Metallic Materials with High Structural Efficiency; Senkov, O., Miracle, D., Firstov, S., Eds.; NATO Science Series II: Mathematics, Physics and Chemistry; Springer: Utrecht, The Netherlands, 2004; Volume 146, pp. 425–432. [Google Scholar]
- Skrzypek, J.J.; Egner, H.; Ganczarski, A.W.; Rustichelli, F. Advanced Materials and Structures for Extreme Operating Conditions; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Steuwer, A.; Santisteban, J.R.; Turski, M.; Withers, P.J.; Buslaps, T. High-resolution strain mapping in bulk samples using full-profile analysis of energy-dispersive synchrotron X-ray diffraction data. J. Appl. Crystallogr. 2004, 37, 883–889. [Google Scholar] [CrossRef]
- de Oliveira, U.; Ocelík, V.; De Hosson, J.T.M. Residual stress analysis in Co-based laser clad layers by laboratory X-rays and synchrotron diffraction techniques. Surf. Coat. Technol. 2006, 201, 533–542. [Google Scholar] [CrossRef]
- Pyzalla, A. Methods and Feasibility of Residual Stress Analysis by High-Energy Synchrotron Radiation in Transmission Geometry Using a White Beam. J. Nondestruct. Eval. 2000, 19, 21–31. [Google Scholar] [CrossRef]
- Xu, L.; Zhang, S.-Y.; Sun, W.; McCartney, D.G.; Hyde, T.H.; James, J.; Drakopoulos, M. Residual stress distribution in a Ti–6Al–4V T-joint weld measured using synchrotron X-ray diffraction. J. Strain Anal. Eng. Des. 2015, 50, 445–454. [Google Scholar] [CrossRef]
- Salvati, E.; Lunt, A.J.G.; Ying, S.; Sui, T.; Zhang, H.J.; Heason, C.; Baxter, G.; Korsunsky, A.M. Eigenstrain reconstruction of residual strains in an additively manufactured and shot peened nickel superalloy compressor blade. Comput. Methods Appl. Mech. Eng. 2017, 320, 335–351. [Google Scholar] [CrossRef]
- James, M.N.; Newby, M.; Hattingh, D.G.; Steuwer, A. Shot-peening of steam turbine blades: Residual stresses and their modification by fatigue cycling. Procedia Eng. 2010, 2, 441–451. [Google Scholar] [CrossRef]
- Umapathi, A.; Swaroop, S. Measurement of residual stresses in titanium alloys using synchrotron radiation. Measurement 2019, 140, 518–525. [Google Scholar] [CrossRef]
- Korsunsky, A.M.; Liu, J.; Golshan, M.; Dini, D.; Zhang, S.Y.; Vorster, W.J. Measurement of Residual Elastic Strains in a Titanium Alloy Using High Energy Synchrotron X-Ray Diffraction. Exp. Mech. 2006, 46, 519–529. [Google Scholar] [CrossRef]
- Poulsen, H.F.; Garbe, S.; Lorentzen, T.; Juul Jensen, D.; Poulsen, F.W.; Andersen, N.H.; Frello, T.; Feidenhans’l, R.; Graafsma, H. Applications of High-Energy Synchrotron Radiation for Structural Studies of Polycrystalline Materials. J. Synchrotron Radiat. 1997, 4, 147–154. [Google Scholar] [CrossRef] [PubMed]
- Lorentzen, T.; Clarke, A.P.; Poulsen, H.F.; Garbe, S.; Graafsma, H. Local strain contours around inclusions in wire-drawn CuW composites. Compos. Part A Appl. Sci. Manuf. 1997, 28, 667–674. [Google Scholar] [CrossRef]
- Royer, A.; Bastie, P. A non-destructive technique for the microstructural investigation of nickel base single crystal superalloys: High resolution diffraction of high energy synchrotron radiation. Scr. Mater. 1997, 1151, S1359–S6462. [Google Scholar] [CrossRef]
- Lee, M.; Xiao, Y.; Wittmer, D.E.; Graber, T.J.; Mini, S.M. Residual stresses in particle-reinforced ceramic composites using synchrotron radiation. J. Mater. Sci. 2002, 37, 4437–4443. [Google Scholar] [CrossRef]
- Rotundo, F.; Korsunsky, A.M. Synchrotron XRD study of residual stress in a shot peened Al/SiCp composite. Procedia Eng. 2009, 1, 221–224. [Google Scholar] [CrossRef][Green Version]
- Bordas, J.; Munro, I.H.; Glazer, A.M. Small-angle scattering experiments on biological materials using synchrotron radiation. Nature 1976, 262, 541–545. [Google Scholar] [CrossRef]
- Buras, B.; Staun Olsen, J.; Gerward, L. X-ray energy-dispersive powder diffractometry using synchrotron radiation. Nucl. Instrum. Methods 1976, 135, 193–195. [Google Scholar] [CrossRef]
- Cousins, C.S.G.; Gerward, L.; Staun Olsen, J.; Selsmark, B.; Sheldon, B.J. Determination of internal strain tensors by energy-dispersive X-ray diffraction: Results for Si using the 006 forbidden reflection. J. Appl. Crystallogr. 1982, 15, 154–159. [Google Scholar] [CrossRef]
- Buras, B.; Olsen, J.S.; Gerward, L.; Will, G.; Hinze, E. X-ray energy-dispersive diffractometry using synchrotron radiation. J. Appl. Crystallogr. 1977, 10, 431–438. [Google Scholar] [CrossRef]
- Black, D.R.; Bechtoldt, C.J.; Placious, R.C.; Kuriyama, M. Three dimensional strain measurements with x-ray energy dispersive spectroscopy. J. Nondestruct. Eval. 1985, 5, 21–25. [Google Scholar] [CrossRef]
- Croft, M.; Zakharchenko, I.; Zhong, Z.; Gurlak, Y.; Hastings, J.; Hu, J.; Holtz, R.; DaSilva, M.; Tsakalakos, T. Strain field and scattered intensity profiling with energy dispersive x-ray scattering. J. Appl. Phys. 2002, 92, 578. [Google Scholar] [CrossRef]
- Tschentscher, T.; Suortti, P. Experiments with Very High Energy Synchrotron Radiation. J. Synchrotron Radiat. 1998, 5, 286–292. [Google Scholar] [CrossRef] [PubMed]
- Korsunsky, A.M.; Collins, S.P.; Owen, R.A.; Daymond, M.R.; Achtioui, S.; James, K.E. Fast residual stress mapping using energy-dispersive synchrotron X-ray diffraction on station 16.3 at the SRS. J. Synchrotron Radiat. 2002, 9, 77–81. [Google Scholar] [CrossRef] [PubMed]
- Reimers, W.; Pyzalla, A.; Broda, M.; Brusch, G.; Dantz, D.; Schmackers, T.; Liss, K.D.; Tschentscher, T. The Use of High-Energy Synchrotron Diffraction for Residual Stress Analyses. J. Mater. Sci. Lett. 1999, 18, 581–583. [Google Scholar] [CrossRef]
- Hutchings, M.T.; Krawitz, A.D. Measurement of Residual and Applied Stress Using Neutron Diffraction; Springer Science & Business Media: Berlin/Heidelberg, Germany, 1992. [Google Scholar]
- Microstructural Analysis and Residual Stress Determination based on Scattering of Neutrons and X-ray Synchrotron Radiation. In Advanced Materials and Structures for Extreme Operating Conditions; Springer: Berlin/Heidelberg, Germany, 2008; pp. 151–210.
- Hutchings, M.T. Introduction to the Characterization of Residual Stress by Neutron Diffraction; Taylor & Francis: Boca Raton, FL, USA, 2005. [Google Scholar]
- Withers, P.J. Mapping residual and internal stress in materials by neutron diffraction. Comptes Rendus Phys. 2007, 8, 806–820. [Google Scholar] [CrossRef]
- Pierret, S.; Evans, A.; Paradowska, A.M.; Kaestner, A.; James, J.; Etter, T.; Van Swygenhoven, H. Combining neutron diffraction and imaging for residual strain measurements in a single crystal turbine blade. NDT E Int. 2012, 45, 39–45. [Google Scholar] [CrossRef]
- Liu, X.; Luzin, V.; Qin, H.; Bi, Z.; Li, M.; Liu, Y.; Sun, K.; Chen, D. Mapping of three-dimensional residual stresses by neutron diffraction in nickel-based superalloy discs prepared under different quenching conditions. Mater. Today Commun. 2022, 32, 103876. [Google Scholar] [CrossRef]
- Cihak, U.; Staron, P.; Clemens, H.; Homeyer, J.; Stockinger, M.; Tockner, J. Characterization of residual stresses in turbine discs by neutron and high-energy X-ray diffraction and comparison to finite element modeling. Mater. Sci. Eng. A 2006, 437, 75–82. [Google Scholar] [CrossRef]
- Zhang, Z.; Feng, Y.; Tan, Q.; Zou, J.; Li, J.; Zhou, X.; Sun, G.; Wang, Y. Residual stress distribution in Ni-based superalloy turbine discs during fabrication evaluated by neutron/X-ray diffraction measurement and thermomechanical simulation. Mater. Des. 2019, 166, 107603. [Google Scholar] [CrossRef]
- Zhang, Z.; Tan, Q.; Cui, Y.; Tian, Y.; Wang, Y.; Qin, H.; Bi, Z.; Wang, Y. Experimental validation of residual stress thermomechanical simulation in as-quenched superalloy discs by using diffraction and incremental hole-drilling methods. Mater. Today Commun. 2021, 27, 102229. [Google Scholar] [CrossRef]
- Xu, X.-Y.; Ma, X.-D.; Wang, H.; Ye, Z.; Chang, J.-W.; Xu, Y.; Sun, G.-A.; LÜ, W.-J.; Gao, Y.-K. Characterization of residual stresses and microstructural features in an Inconel 718 forged compressor disc. Trans. Nonferrous Met. Soc. China 2019, 29, 569–578. [Google Scholar] [CrossRef]
- Pant, P.; Proper, S.; Luzin, V.; Sjöström, S.; Simonsson, K.; Moverare, J.; Hosseini, S.; Pacheco, V.; Peng, R.L. Mapping of residual stresses in as-built Inconel 718 fabricated by laser powder bed fusion: A neutron diffraction study of build orientation influence on residual stresses. Addit. Manuf. 2020, 36, 101501. [Google Scholar] [CrossRef]
- Goel, S.; Neikter, M.; Capek, J.; Polatidis, E.; Colliander, M.H.; Joshi, S.; Pederson, R. Residual stress determination by neutron diffraction in powder bed fusion-built Alloy 718: Influence of process parameters and post-treatment. Mater. Des. 2020, 195, 109045. [Google Scholar] [CrossRef]
- Smith, M.; Levesque, J.B.; Bichler, L.; Sediako, D.; Gholipour, J.; Wanjara, P. Residual stress analysis in linear friction welded in-service Inconel 718 superalloy via neutron diffraction and contour method approaches. Mater. Sci. Eng. A 2017, 691, 168–179. [Google Scholar] [CrossRef]
- Serrano-Munoz, I.; Ulbricht, A.; Fritsch, T.; Mishurova, T.; Kromm, A.; Hofmann, M.; Wimpory, R.C.; Evans, A.; Bruno, G. Scanning Manufacturing Parameters Determining the Residual Stress State in LPBF IN718 Small Parts. Adv. Eng. Mater. 2021, 23, 2100158. [Google Scholar] [CrossRef]
- Wang, Z.; Stoica, A.D.; Ma, D.; Beese, A.M. Stress relaxation in a nickel-base superalloy at elevated temperatures with in situ neutron diffraction characterization: Application to additive manufacturing. Mater. Sci. Eng. A 2018, 714, 75–83. [Google Scholar] [CrossRef]
- Mo, F.; Sun, G.; Yang, Z.; Zhang, C.; Zhang, J.; Wang, S.; Peng, S. Elastic tension induced lattice distortions in DD10 single crystal nickel-based superalloy at 500 °C/760 MPa using in situ neutron diffraction. Mater. Sci. Eng. A 2019, 743, 504–511. [Google Scholar] [CrossRef]
- Duan, H.; Qin, L.; Pei, Y.; Li, S.; Zhao, L.-D.; Ji, V.; Gong, S. Investigations into the Surface Strain/Stress State in a Single-Crystal Superalloy via XRD Characterization. Metals 2018, 8, 376. [Google Scholar] [CrossRef]
- Mo, F.; Wu, E.; Zhang, C.; Wang, H.; Zhong, Z.; Zhang, J.; Chen, B.; Hofmann, M.; Gan, W.; Sun, G. Correlation Between the Microstructural Defects and Residual Stress in a Single Crystal Nickel-Based Superalloy During Different Creep Stages. Met. Mater. Int. 2018, 24, 1002–1011. [Google Scholar] [CrossRef]
- Syed, A.K.; Ahmad, B.; Guo, H.; Machry, T.; Eatock, D.; Meyer, J.; Fitzpatrick, M.E.; Zhang, X. An experimental study of residual stress and direction-dependence of fatigue crack growth behaviour in as-built and stress-relieved selective-laser-melted Ti6Al4V. Mater. Sci. Eng. A 2019, 755, 246–257. [Google Scholar] [CrossRef]
- Wang, Z.; Stoica, A.D.; Ma, D.; Beese, A.M. Stress relaxation behavior and mechanisms in Ti-6Al-4V determined via in situ neutron diffraction: Application to additive manufacturing. Mater. Sci. Eng. A 2017, 707, 585–592. [Google Scholar] [CrossRef]
- Boruah, D.; Ahmad, B.; Lee, T.L.; Kabra, S.; Syed, A.K.; McNutt, P.; Doré, M.; Zhang, X. Evaluation of residual stresses induced by cold spraying of Ti-6Al-4V on Ti-6Al-4V substrates. Surf. Coat. Technol. 2019, 374, 591–602. [Google Scholar] [CrossRef]
- Gloaguen, D.; Girault, B.; Courant, B.; Dubos, P.-A.; Moya, M.-J.; Edy, F.; Rebelo Kornmeier, J. Study of Residual Stresses in Additively Manufactured Ti-6Al-4V by Neutron Diffraction Measurements. Metall. Mater. Trans. A 2020, 51, 951–961. [Google Scholar] [CrossRef]
- Bray, D.E.; Stanley, R.K. Nondestructive Evaluation: A Tool in Design, Manufacturing, and Service; CRC Press: Boca Raton, FL, USA, 1997. [Google Scholar]
- Egle, D.M.; Bray, D.E. Measurement of acoustoelastic and third-order elastic constants for rail steel. J. Acoust. Soc. Am. 1976, 60, 741–744. [Google Scholar] [CrossRef]
- Crecraft, D.I. The measurement of applied and residual stresses in metals using ultrasonic waves. J. Sound Vib. 1967, 5, 173–192. [Google Scholar] [CrossRef]
- Murnaghan, F.D. Finite Deformations of an Elastic Solid. Am. J. Math. 1937, 59, 235–260. [Google Scholar] [CrossRef]
- Hughes, D.S.; Kelly, J.L. Second-Order Elastic Deformation of Solids. Phys. Rev. 1953, 92, 1145–1149. [Google Scholar] [CrossRef]
- Lu, J. Handbook of Measurement of Residual Stresses; Distributed by Prentice Hall PTR; Fairmont Press: Lilburn, GA, USA, 1996. [Google Scholar]
- Pereira, P.; Santos, A.A. Influence of Anisotropy Generated by Rolling on the Stress Measurement by Ultrasound in 7050 T7451 Aluminum. Exp. Mech. 2013, 53, 415–425. [Google Scholar] [CrossRef]
- Javadi, Y.; Akhlaghi, M.; Najafabadi, M.A. Using finite element and ultrasonic method to evaluate welding longitudinal residual stress through the thickness in austenitic stainless steel plates. Mater. Des. 2013, 45, 628–642. [Google Scholar] [CrossRef]
- Sadeghi, S.; Najafabadi, M.A.; Javadi, Y.; Mohammadisefat, M. Using ultrasonic waves and finite element method to evaluate through-thickness residual stresses distribution in the friction stir welding of aluminum plates. Mater. Des. 2013, 52, 870–880. [Google Scholar] [CrossRef]
- Belahcene, F.; Lu, J. Determination of residual stress using critically refracted longitudinal waves and immersion mode. J. Strain Anal. Eng. Des. 2002, 37, 13–20. [Google Scholar] [CrossRef]
- Bray, D.E.; Tang, W. Subsurface stress evaluation in steel plates and bars using the LCR ultrasonic wave. Nucl. Eng. Des. 2001, 207, 231–240. [Google Scholar] [CrossRef]
- Leon-Salamanca, T.; Bray, D.F. Residual stress measurement in steel plates and welds using critically refracted longitudinal (LCR) waves. Res. Nondestruct. Eval. 1996, 7, 169–184. [Google Scholar] [CrossRef]
- Junghans, P.G.; Bray, D. Beam characteristics of high angle longitudinal wave probes. In Proceedings of the Pressure Vessels and Piping Conference, San Diego, CA, USA, 23–27 June 1991; pp. 39–44. [Google Scholar]
- Javadi, Y.; Najafabadi, M.A. Comparison between contact and immersion ultrasonic method to evaluate welding residual stresses of dissimilar joints. Mater. Des. 2013, 47, 473–482. [Google Scholar] [CrossRef]
- Javadi, Y.; Najafabadi, M.A.; Akhlaghi, M. Comparison between contact and immersion method in ultrasonic stress measurement of welded stainless steel plates. J. Test. Eval. 2013, 41, 788–797. [Google Scholar] [CrossRef]
- Pei, N.; Zhao, B.; Zhao, X.; Liu, Z.; Bond, L.J. Analysis of the directivity of Longitudinal Critically Refracted (LCR) waves. Ultrasonics 2021, 113, 106359. [Google Scholar] [CrossRef]
- Sajauskas, S. Longitudinal Surface Acoustic Waves (Creeping Waves); Technologija: Kaunas, Lithuania, 2004. [Google Scholar]
- Song, W.; Xu, C.; Pan, Q.; Song, J. Nondestructive testing and characterization of residual stress field using an ultrasonic method. Chin. J. Mech. Eng. 2016, 29, 365–371. [Google Scholar] [CrossRef]
- Jassby, K.; Kishoni, D. Experimental technique for measurement of stress-acoustic coefficients of Rayleigh waves. Exp. Mech. 1983, 23, 74. [Google Scholar] [CrossRef]
- Duquennoy, M.; Ouaftouh, M.; Ourak, M.; Jenot, F. Theoretical determination of Rayleigh wave acoustoelastic coefficients: Comparison with experimental values. Ultrasonics 2002, 39, 575–583. [Google Scholar] [CrossRef]
- Hubel, S.; Dillhöfer, A.; Rieder, H.; Spies, M.; Bamberg, J.; Götz, J.; Hessert, R.; Preikszas, C. Ultrasonic evaluation of residual stresses in aero engine materials using bulk and Rayleigh surface waves. AIP Conf. Proc. 2014, 1581, 607–614. [Google Scholar] [CrossRef]
- Rjelka, M.; Barth, M.; Reinert, S.; Koehler, B.; Bamberg, J.; Baron, H.U. Third order elastic constants and Rayleigh wave dispersion of shot-peened aero-engine materials. AIP Conf. Proc. 2012, 1430, 1430–1436. [Google Scholar] [CrossRef]
- Duquennoy, M.; Ouaftouh, M.; Ourak, M.; Xu, W.-J. Influence of natural and initial acoustoelastic coefficients on residual stress evaluation: Theory and experiment. J. Appl. Phys. 1999, 86, 2490–2498. [Google Scholar] [CrossRef]
- Husson, D. A perturbation theory for the acoustoelastic effect of surface waves. J. Appl. Phys. 1985, 57, 1562. [Google Scholar] [CrossRef]
- Duqennoy, M.; Ouaftouh, M.; Ourak, M. Determination of stresses in aluminium alloy using optical detection of Rayleigh waves. Ultrasonics 1999, 37, 365–372. [Google Scholar] [CrossRef]
- Duquennoy, M.; Ouaftouh, M.; Qian, M.L.; Jenot, F.; Ourak, M. Ultrasonic characterization of residual stresses in steel rods using a laser line source and piezoelectric transducers. NDT E Int. 2001, 34, 355–362. [Google Scholar] [CrossRef]
- Köhler, B.; Barth, M.; Schubert, F.; Bamberg, J.; Baron, H.U. Characterization Of Surface Treated Aero Engine Alloys by Rayleigh Wave Velocity Dispersion. AIP Conf. Proc. 2010, 1211, 253–260. [Google Scholar] [CrossRef]
- Smith, R.J.; Li, W.; Coulson, J.; Clark, M.; Somekh, M.G.; Sharples, S.D. Spatially resolved acoustic spectroscopy for rapid imaging of material microstructure and grain orientation. Meas. Sci. Technol. 2014, 25, 055902. [Google Scholar] [CrossRef]
- Sharples, S.D.; Clark, M.; Somekh, M.G. Spatially resolved acoustic spectroscopy for fast noncontact imaging of material microstructure. Opt. Express 2006, 14, 10435–10440. [Google Scholar] [CrossRef]
- Mark, A.F.; Li, W.; Sharples, S.; Withers, P.J. Comparison of grain to grain orientation and stiffness mapping by spatially resolved acoustic spectroscopy and EBSD. J. Microsc. 2017, 267, 89–97. [Google Scholar] [CrossRef]
- Junge, M.; Qu, J.; Jacobs, L.J. Relationship between Rayleigh wave polarization and state of stress. Ultrasonics 2006, 44, 233–237. [Google Scholar] [CrossRef]
- Walker, S.V.; Kim, J.-Y.; Qu, J.; Jacobs, L.J. Fatigue damage evaluation in A36 steel using nonlinear Rayleigh surface waves. NDT E Int. 2012, 48, 10–15. [Google Scholar] [CrossRef]
- Herrmann, J.; Kim, J.-Y.; Jacobs, L.J.; Jianmin, Q.; Littles, J.W.; Savage, M.F. Assessment of material damage in a nickel-base superalloy using nonlinear Rayleigh surface waves. J. Appl. Phys. 2006, 99, 124913. [Google Scholar] [CrossRef]
- Liu, M.; Kim, J.-Y.; Jacobs, L.; Qu, J. Experimental study of nonlinear Rayleigh wave propagation in shot-peened aluminum plates—Feasibility of measuring residual stress. NDT E Int. 2011, 44, 67–74. [Google Scholar] [CrossRef]
- Barth, M.; Küttner, M.; Köhler, B.; Bamberg, J.; Baron, H.U. Universal ultrasonic goniometer for Rayleigh and surface skimming longitudinal wave dispersion measurements. AIP Conf. Proc. 2012, 1430, 1873–1880. [Google Scholar] [CrossRef]
- Hu, E.; He, Y.; Chen, Y. Experimental study on the surface stress measurement with Rayleigh wave detection technique. Appl. Acoust. 2009, 70, 356–360. [Google Scholar] [CrossRef]
- Duquennoy, M.; Ouaftouh, M.; Ourak, M. Ultrasonic evaluation of stresses in orthotropic materials using Rayleigh waves. NDT E Int. 1999, 32, 189–199. [Google Scholar] [CrossRef]
- Ditri, J.J.; Hongerholt, D. Stress distribution determination in isotropic materials via inversion of ultrasonic Rayleigh wave dispersion data. Int. J. Solids Struct. 1996, 33, 2437–2451. [Google Scholar] [CrossRef]
- Ruiz, A.; Nagy, P.B. Laser-ultrasonic surface wave dispersion measurements on surface-treated metals. Ultrasonics 2004, 42, 665–669. [Google Scholar] [CrossRef]
- Alberto, R.; Peter, B. SAW Dispersion Measurements for Ultrasonic Characterization of Surface-Treated Metals. Instrum. Mes. Métrologie 2003, 3, 59–85. [Google Scholar]
- Lavrentyev, A.I.; Veronesi, W.A. Ultrasonic characterization of near-surface material properties in shot peened Waspaloy. AIP Conf. Proc. 2002, 615, 1659–1666. [Google Scholar] [CrossRef]
- Lavrentyev, A.I.; Stucky, P.A.; Veronesi, W.A. Feasibility of ultrasonic and eddy current methods for measurement of residual stress in shot peened metals. AIP Conf. Proc. 2000, 509, 1621–1628. [Google Scholar] [CrossRef]
- Ledbetter, H.M.; Austin, M.W. Deformed-polycrystalline-copper elastic constants. Phys. Status Solidi 1987, 104, 203–212. [Google Scholar] [CrossRef]
- Maradudin, A.A.; Mills, D.L. The attenuation of rayleigh surface waves by surface roughness. Ann. Phys. 1976, 100, 262–309. [Google Scholar] [CrossRef]
- Sayers, C.M. Texture independent determination of residual stress in polycrystalline aggregates using Rayleigh waves. J. Phys. D Appl. Phys. 1984, 17, 179–184. [Google Scholar] [CrossRef]
- Sayers, C.M.; Allen, D.R.; Haines, G.E.; Proudfoot, G.G.; Wadley, H.N.G.; Thompson, R.B.; Almond, E. Texture and Stress Determination in Metals by Using Ultrasonic Rayleigh Waves and Neutron Diffraction. Philos. Trans. R. Soc. Lond. Ser. A Math. Phys. Sci. 1986, 320, 187–200. [Google Scholar]
- Blodgett, M.; Nagy, P. On the Feasibility of Eddy Current Characterization of the Near-Surface Residual Stress Distribution in Nickel-Base Superalloys. AIP Conf. Proc. 2004, 700, 1216–1223. [Google Scholar] [CrossRef]
- Yu, F.; Blodgett, M.; Nagy, P. Eddy Current Assessment of Near-Surface Residual Stress in Shot-Peened Inhomogeneous Nickel-Base Superalloys. J. Nondestruct. Eval. 2006, 25, 16–27. [Google Scholar] [CrossRef][Green Version]
- Abu-Nabah, B.A.; Nagy, P.B. High-frequency eddy current conductivity spectroscopy for residual stress profiling in surface-treated nickel-base superalloys. NDT E Int. 2007, 40, 405–418. [Google Scholar] [CrossRef]
- Nakagawa, N.; Lee, C.; Shen, Y. A High-Frequency Eddy Current Inspection System and Its Application to the Residual Stress Characterization. AIP Conf. Proc. 2006, 820, 1418–1425. [Google Scholar] [CrossRef]
- Shen, Y.; Lo, C.C.H.; Nakagawa, N.; Frishman, A.M. Residual Stress Profile Assessment by Eddy Current for Shot Peened Nickel Superalloy. J. Nondestruct. Eval. 2010, 29, 1–13. [Google Scholar] [CrossRef]
- Lee, C.; Shen, Y.; Lo, C.C.H.; Nakagawa, N. Validation of a Residual Stress Measurement Method by Swept High-Frequency Eddy Currents. AIP Conf. Proc. 2007, 894, 1213–1220. [Google Scholar] [CrossRef]
- Annamalai, S.; Fan, Z.; Castagne, S. Residual Stress Profiling of Sub-surface Treated Nickel-Based Superalloy using Electromagnetic NDE Method. In Proceedings of the Indian National Seminar & Exhibition on Non-Destructive Evaluation NDE 2016, Thiruvananthapuram, India, 15–18 December 2016. [Google Scholar]
- Rao, B.P.C. Practical Eddy Current Testing; Alpha Science International Ltd: Oxford, UK, 2007. [Google Scholar]
- Abu-Nabah, B.A.; Feng, Y.; Hassan, W.T.; Blodgett, M.P.; Nagy, P.B. Eddy current residual stress profiling in surface-treated engine alloys. Nondestruct. Test. Eval. 2009, 24, 209–232. [Google Scholar] [CrossRef]
- Nakagawa, N.; Shen, Y.; Frishman, A.M. A Study of Correlation Between Conductivity Measurement and Surface Residual Stress. AIP Conf. Proc. 2005, 760, 1363–1370. [Google Scholar] [CrossRef]
- Yu, F.; Nagy, P.B. Dynamic Piezoresistivity Calibration for Eddy Current Nondestructive Residual Stress Measurements. J. Nondestruct. Eval. 2005, 24, 143. [Google Scholar] [CrossRef]
- Blodgett, M.; Nagy, P. Eddy Current Assessment of Near-Surface Residual Stress in Shot-Peened Nickel-Base Superalloys. J. Nondestruct. Eval. 2004, 23, 107–123. [Google Scholar] [CrossRef]
- Feng, Y.; Nagy, P.B. Simple analytical approximations for eddy current profiling of the near-surface residual stress in shot-peened metals. J. Appl. Phys. 2004, 96, 1257–1266. [Google Scholar]
- Nagy, P. Inversion Procedure for Eddy Current Profiling of the Near-Surface Residual Stress in Shot-Peened Metals. AIP Conf. Proc. 2005, 760, 1355–1362. [Google Scholar] [CrossRef]
- Abu-Nabah, B.A.; Nagy, P.B. Iterative inversion method for eddy current profiling of near-surface residual stress in surface-treated metals. NDT E Int. 2006, 39, 641–651. [Google Scholar] [CrossRef]
- Blodgett, M.P.; Ukpabi, C.V.; Nagy, P.B. Surface roughness influence on eddy current electrical conductivity measurements. Mater. Eval. 2003, 61, 765–772. [Google Scholar]
- Kalyanasundaram, K.; Nagy, P.B. A simple numerical model of the apparent loss of eddy current conductivity due to surface roughness. NDT E Int. 2004, 37, 47–56. [Google Scholar] [CrossRef]
- Kalyanasundaram, K.; Nagy, P.B. On the Apparent Loss of Eddy Current Conductivity Due to Surface Roughness. AIP Conf. Proc. 2004, 700, 360–367. [Google Scholar] [CrossRef]
- Yu, F.; Nagy, P.B. Numerical method for calculating the apparent eddy current conductivity loss on randomly rough surfaces. J. Appl. Phys. 2004, 95, 8340–8351. [Google Scholar] [CrossRef]
- Johnson, M.; Nakagawa, N.; Wendt, S.; Hentscher, S.; Nelson, D.; Buhr, K.; Kilbugh, B.; Raithel, D. Studies into the Effects of Surface Roughness on Spatial Eddy-Current Data from Nickel-Based Engine Alloys. AIP Conf. Proc. 2005, 760, 425–431. [Google Scholar] [CrossRef]
- Hillmann, S.; Heuer, H.; Baron, H.U.; Bamberg, J.; Yashan, A.; Meyendorf, N. Near-surface residual stress profiling with high frequency eddy current conductivity measurement. AIP Conf. Proc. 2009, 1096, 1349–1355. [Google Scholar] [CrossRef]
- Lesthaeghe, T.J.; Larson, B.F.; Chandrasekar, R.; Frishman, A.M.; Lo, C.C.H.; Nakagawa, N. Effects of cold work on near-surface conductivity profiles in laser shock peened and shot peened nickel-base superalloy. AIP Conf. Proc. 2013, 1511, 1219–1226. [Google Scholar] [CrossRef]
- Yu, F.; Nagy, P. On the Influence of Cold Work on Eddy Current Characterization of Near-Surface Residual Stress in Shot-Peened Nickel-Base Superalloys. J. Nondestruct. Eval. 2006, 25, 107–122. [Google Scholar] [CrossRef]
- Yu, F.; Nagy, P.B. The Role of Cold Work in Eddy Current Residual Stress Measurements in Shot-Peened Nickel-Base Superalloys. AIP Conf. Proc. 2006, 820, 1402–1409. [Google Scholar] [CrossRef]
- Hillmann, S.; Heuer, H.; Robbert, A.; Baron, H.U.; Bamberg, J.; Meyendorf, N. Experimental-based discussion for the separation of residual stress and cold work in shot peened IN718 using high frequency eddy current spectroscopy. AIP Conf. Proc. 2010, 1211, 1349–1356. [Google Scholar] [CrossRef]
- Abu-Nabah, B.A.; Hassan, W.T.; Blodgett, M.P.; Nagy, P.B. Limitations of eddy current residual stress profiling in surface-treated engine alloys of various hardness levels. AIP Conf. Proc. 2010, 1211, 1357–1364. [Google Scholar]
- Abu-Nabah, B.A.; Hassan, W.T.; Ryan, D.; Blodgett, M.P.; Nagy, P.B. The Effect of Hardness on Eddy Current Residual Stress Profiling in Shot-Peened Nickel Alloys. J. Nondestruct. Eval. 2010, 29, 143–153. [Google Scholar] [CrossRef]
- Chandrasekar, R.; Frishman, A.M.; Larson, B.F.; Lo, C.C.H.; Nakagawa, N. Effects of Microstructure on Eddy Current Residual Stress Characterization of Shot-Peened Inconel 718. JOM 2012, 64, 257–264. [Google Scholar] [CrossRef]
- Chandrasekar, R.; Lo, C.; Frishman, A.; Larson, B.; Nakagawa, N. Quantification of precipitates and their effects on the response of nickel-base superalloy to shot peening. AIP Conf. Proc. 2012, 1430, 1437–1444. [Google Scholar] [CrossRef]
- Pereira, D.; Clarke, T.; Menezes, R.; Hirsch, T. Effect of microstructure on electrical conductivity of Inconel 718 alloys. Mater. Sci. Technol. 2015, 31, 669–676. [Google Scholar] [CrossRef]
- Nagarajan, B.; Castagne, S.; Annamalai, S.; Fan, Z.; Chan, W.L. Effect of Microstructure on Electrical Conductivity of Nickel-Base Superalloys. Metall. Mater. Trans. A 2017, 48, 3745–3757. [Google Scholar] [CrossRef]
- Nagy, P.B. Hall coefficient measurement for nondestructive materials characterization. AIP Conf. Proc. 2013, 1511, 1482–1489. [Google Scholar] [CrossRef]
- Kosaka, D.; Frishman, A.; Nakagawa, N. Stress dependence of the Hall coefficient of a nickel-base superalloy. AIP Conf. Proc. 2016, 1706, 090023. [Google Scholar] [CrossRef]
- Shao, Z.; Fan, Z.; Castagne, S. Stress dependence of Hall coefficient in a nickel-base superalloy. AIP Conf. Proc. 2018, 1949, 020013. [Google Scholar] [CrossRef]
- Ramsden, E. Hall-Effect Sensors: Theory and Applications, 2nd ed.; Newnes: Boston, MA, USA, 2006. [Google Scholar]
- Pauw, L.J.v.d. A Method of Measuring Specific Resistivity and Hall Effect of Discs of Arbitrary Shape. Philips Res. Rep. 1958, 13, 1–9. [Google Scholar]
- Pauw, L.J.v.d. A Method of Measuring the Resistivity and Hall Coefficient on Lamellae of Arbitrary Shape. Philips Tech. Rev. 1958, 20, 220–224. [Google Scholar]
- Kosaka, D.; Frishman, A.; Nakagawa, N. Effect of Tensile Stress on Hall Coefficients of Nickel-Base Superalloys. J. Nondestruct. Eval. 2016, 35, 38. [Google Scholar] [CrossRef]
- Velicheti, D.; Nagy, P.B.; Hassan, W. Hall Coefficient Measurement for Residual Stress Assessment in Precipitation Hardened IN718 Nickel-base Superalloy. AIP Conf. Proc. 2017, 1806, 20012. [Google Scholar] [CrossRef]
- Velicheti, D.; Nagy, P.B.; Hassan, W. Stress Assessment in Precipitation Hardened IN718 Nickel-Base Superalloy Based on Hall Coefficient Measurements. J. Nondestruct. Eval. 2017, 36, 13. [Google Scholar] [CrossRef]
- Velicheti, D.; Nagy, P.B.; Hassan, W. Nondestructive hall coefficient measurements using ACPD techniques. AIP Conf. Proc. 2018, 1949, 160006. [Google Scholar] [CrossRef]
- Velicheti, D.; Nagy, P.B.; Hassan, W. Nondestructive Measurement of Hall Coefficient for Materials Characterization. J. Nondestruct. Eval. 2017, 36, 49. [Google Scholar] [CrossRef]
- Velicheti, D.; Nagy, P.B.; Hassan, W. High-frequency Hall coefficient measurement using inductive sensing for nondestructive materials characterization. NDT E Int. 2018, 94, 109–119. [Google Scholar] [CrossRef]
- Adams, E.P. The Hall and Corbino Effects. Proc. Am. Philos. Soc. 1915, 54, 47–51. [Google Scholar]
- Velicheti, D.; Nagy, P.; Hassan, W. Inversion Procedure for Dual-Mode Electromagnetic Nondestructive Characterization of Shot-Peened IN718. NDT E Int. 2018, 101, 17–28. [Google Scholar] [CrossRef]
- Velicheti, D.; Nagy, P.B.; Hassan, W. Residual stress and cold work assessment in shot-peened IN718 using a dual-mode electromagnetic technique. NDT E Int. 2021, 121, 102463. [Google Scholar] [CrossRef]
- Shao, Z.Y.; Fan, Z. Effect of Stress and Temperature on Hall Coefficient in Two Nickel-base Superalloys. In Proceedings of the 2020 IEEE Far East NDT New Technology & Application Forum (FENDT), Nanjing, China, 20–22 November 2020; pp. 36–40. [Google Scholar]
- Gros, X.E. NDT Data Fusion; Wiley & Sons: London, UK, 1997. [Google Scholar]
- White, F.E. Data Fusion Lexicon; Joint Directors of Labs: Washington, DC, USA, 1991. [Google Scholar]
- Hall, D.L.; Llinas, J. An introduction to multisensor data fusion. Proc. IEEE 1997, 85, 6–23. [Google Scholar] [CrossRef]
- Brierley, N.; Tippetts, T.; Cawley, P. Data fusion for automated non-destructive inspection. Proc. R. Soc. A Math. Phys. Eng. Sci. 2014, 470, 20140167. [Google Scholar] [CrossRef]
- Liu, Z.; Forsyth, D.S.; Komorowski, J.P.; Hanasaki, K.; Kirubarajan, T. Survey: State of the art in NDE data fusion techniques. IEEE Trans. Instrum. Meas. 2007, 56, 2435–2451. [Google Scholar] [CrossRef]
- Habibalahi, A.; Safizadeh, M.S. Pulsed eddy current and ultrasonic data fusion applied to stress measurement. Meas. Sci. Technol. 2014, 25, 055601. [Google Scholar] [CrossRef]
- Habibalahi, A.; Moghari, M.D.; Samadian, K.; Mousavi, S.S.; Safizadeh, M.S. Improving pulse eddy current and ultrasonic testing stress measurement accuracy using neural network data fusion. Iet Sci. Meas. Technol. 2015, 9, 514–521. [Google Scholar] [CrossRef]
- Segreto, T.; Karam, S.; Simeone, A.; Teti, R. Residual stress assessment in Inconel 718 machining through wavelet sensor signal analysis and sensor fusion pattern recognition. In Proceedings of the 2nd CIRP Global Web Conference on Beyond Modern Manufacturing—Technology for the Factories of the Future (CIRPe), Electrical Network, Online, 11–12 June 2013; pp. 103–108. [Google Scholar]
- Khaleghi, B.; Khamis, A.; Karray, F.O.; Razavi, S.N. Multisensor data fusion: A review of the state-of-the-art. Inf. Fusion 2013, 14, 28–44. [Google Scholar] [CrossRef]
- Wu, R.T.; Jahanshahi, M.R. Data fusion approaches for structural health monitoring and system identification: Past, present, and future. Struct. Health Monit. Int. J. 2020, 19, 552–586. [Google Scholar] [CrossRef]
- Butler, K.T.; Davies, D.W.; Cartwright, H.; Isayev, O.; Walsh, A. Machine learning for molecular and materials science. Nature 2018, 559, 547–555. [Google Scholar] [CrossRef]
- LeCun, Y.; Bengio, Y.; Hinton, G. Deep learning. Nature 2015, 521, 436–444. [Google Scholar] [CrossRef]
- Hughes, T.W.; Williamson, I.A.D.; Minkov, M.; Fan, S. Wave physics as an analog recurrent neural network. Sci. Adv. 2019, 5, eaay6946. [Google Scholar] [CrossRef]
- Qin, Z.; Yu, Q.; Buehler, M.J. Machine learning model for fast prediction of the natural frequencies of protein molecules. RSC Adv. 2020, 10, 16607–16615. [Google Scholar] [CrossRef]
- Jensen, Z.; Kim, E.; Kwon, S.; Gani, T.Z.H.; Roman-Leshkov, Y.; Moliner, M.; Corma, A.; Olivetti, E. A Machine Learning Approach to Zeolite Synthesis Enabled by Automatic Literature Data Extraction. ACS Cent. Sci 2019, 5, 892–899. [Google Scholar] [CrossRef]
- Aykol, M.; Hegde, V.I.; Hung, L.; Suram, S.; Herring, P.; Wolverton, C.; Hummelshoj, J.S. Network analysis of synthesizable materials discovery. Nat. Commun. 2019, 10, 2018. [Google Scholar] [CrossRef] [PubMed]
- Maleki, E.; Unal, O. Shot Peening Process Effects on Metallurgical and Mechanical Properties of 316 L Steel via: Experimental and Neural Network Modeling. Met. Mater. Int. 2019, 27, 262–276. [Google Scholar] [CrossRef]
- Shen, X.; Shukla, P.; Subramaniyan, A.K.; Zammit, A.; Swanson, P.; Lawrence, J.; Fitzpatrick, M.E. Residual stresses induced by laser shock peening in orthopaedic Ti-6Al-7Nb alloy. Opt. Laser Technol. 2020, 131, 106446. [Google Scholar] [CrossRef]
- Yang, Z.; Yu, C.-H.; Buehler, M.J. Deep learning model to predict complex stress and strain fields in hierarchical composites. Sci. Adv. 2021, 7, eabd7416. [Google Scholar] [CrossRef]
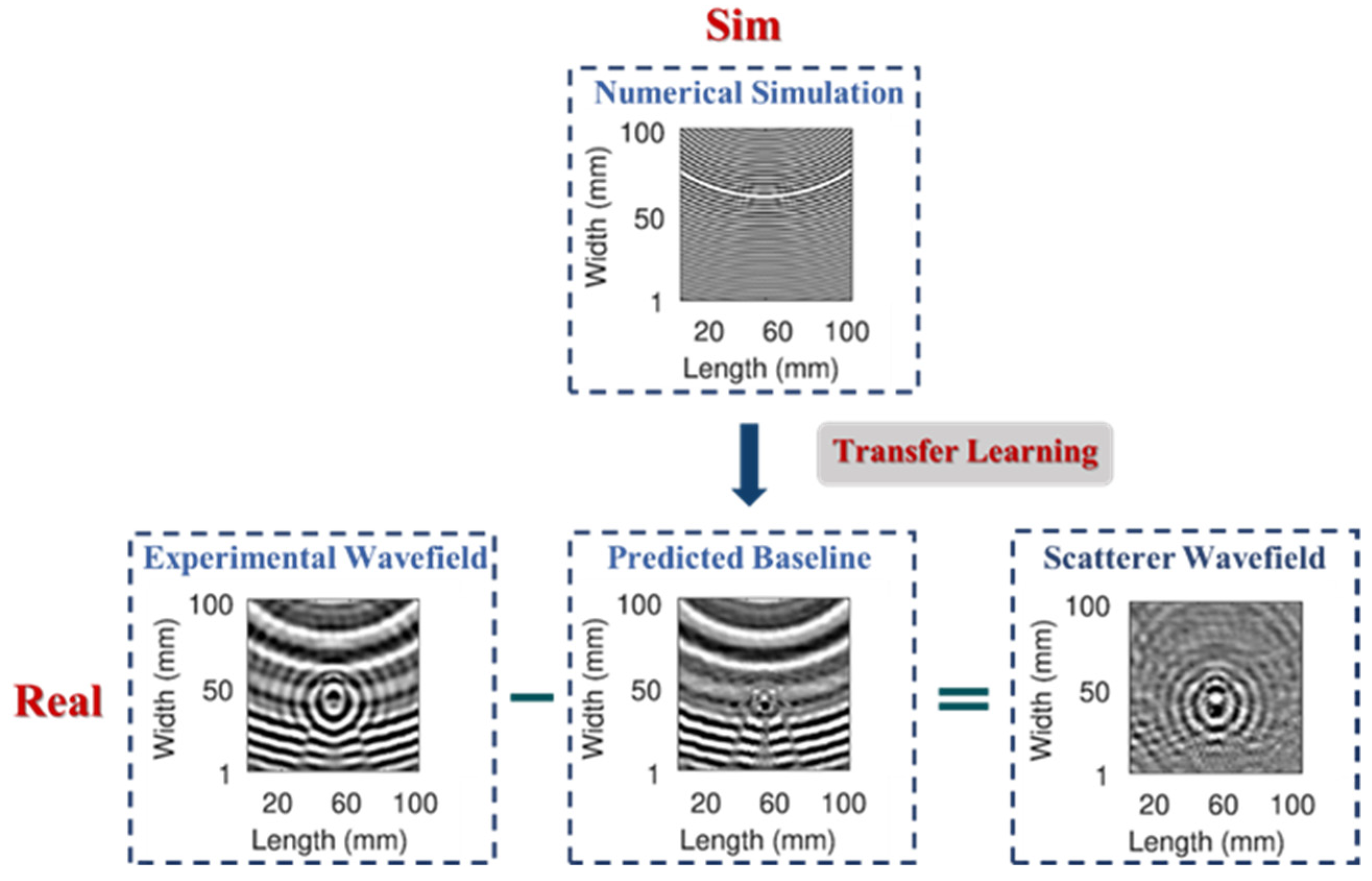
- Alguri, K.S.; Chia, C.C.; Harley, J.B. Sim-to-Real: Employing ultrasonic guided wave digital surrogates and transfer learning for damage visualization. Ultrasonics 2021, 111, 106338. [Google Scholar] [CrossRef]
- Cawley, P. Structural health monitoring: Closing the gap between research and industrial deployment. Struct. Health Monit. Int. J. 2018, 17, 147592171775004. [Google Scholar] [CrossRef]
- Croxford, A.J.; Wilcox, P.D.; Drinkwater, B.W.; Konstantinidis, G. Strategies for guided-wave structural health monitoring. Proc. R. Soc. A Math. Phys. Eng. Sci. 2007, 463, 2961–2981. [Google Scholar] [CrossRef]
- Worden, K.; Farrar, C.R.; Manson, G.; Park, G. The fundamental axioms of structural health monitoring. Proc. R. Soc. A Math. Phys. Eng. Sci. 2007, 463, 1639–1664. [Google Scholar] [CrossRef]
- Kande. On-line flaw growth monitoring in high temperature plant; 2010. Available online: http://www.kande.net/pdf/Baltica%20VIII%20 (accessed on 10 August 2022).
- Cegla, F.B.; Jarvis, A.J.C.; Davies, J.O. High temperature ultrasonic crack monitoring using SH waves. NDT E Int. 2011, 44, 669–679. [Google Scholar] [CrossRef]
- He, Y.; Pan, M.; Luo, F.; Chen, D.; Hu, X. Support vector machine and optimised feature extraction in integrated eddy current instrument. Measurement 2013, 46, 764–774. [Google Scholar] [CrossRef]
- Goulet, J.A.; Smith, I.F.C. Predicting the Usefulness of Monitoring for Identifying the Behavior of Structures. J. Struct. Eng. 2013, 139, 1716–1727. [Google Scholar] [CrossRef]
- CSIC. Available online: http://www-smartinfrastructure.eng.cam.ac.uk/files/the-smart-infrastructure-paper (accessed on 10 August 2022).
- Fultz, B.; Howe, J. Transmission Electron. Microscopy and Diffractometry of Materials; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]




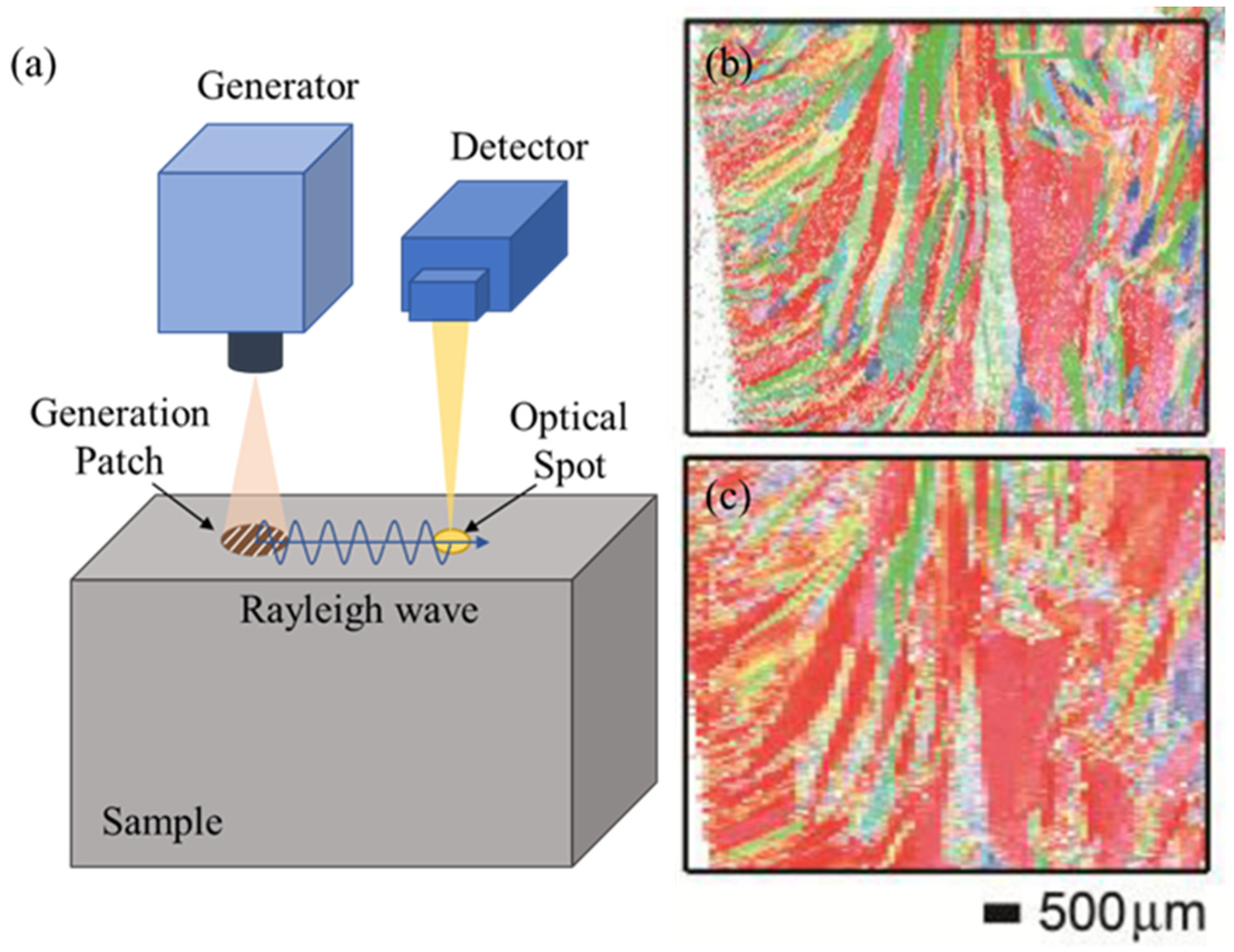
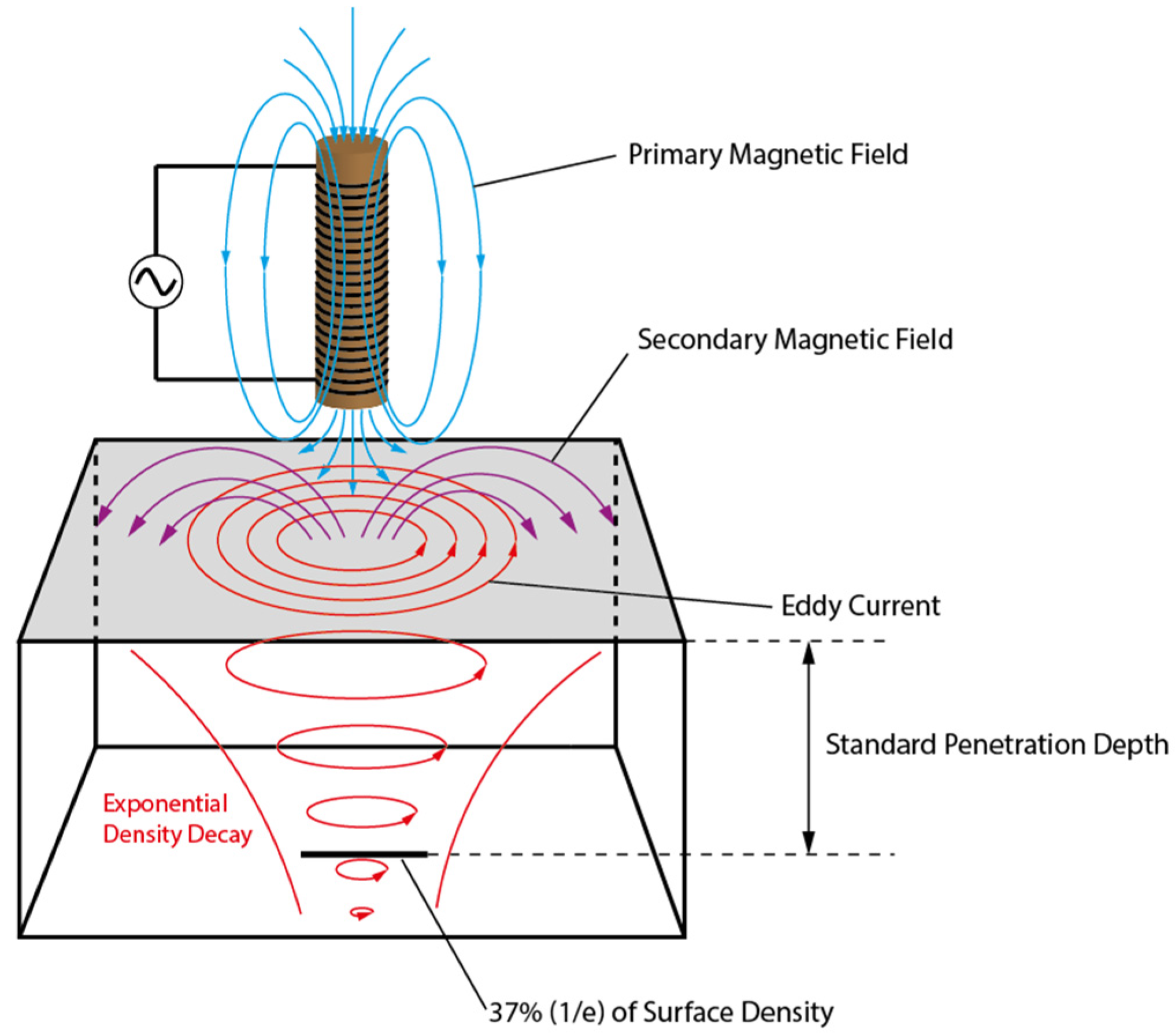
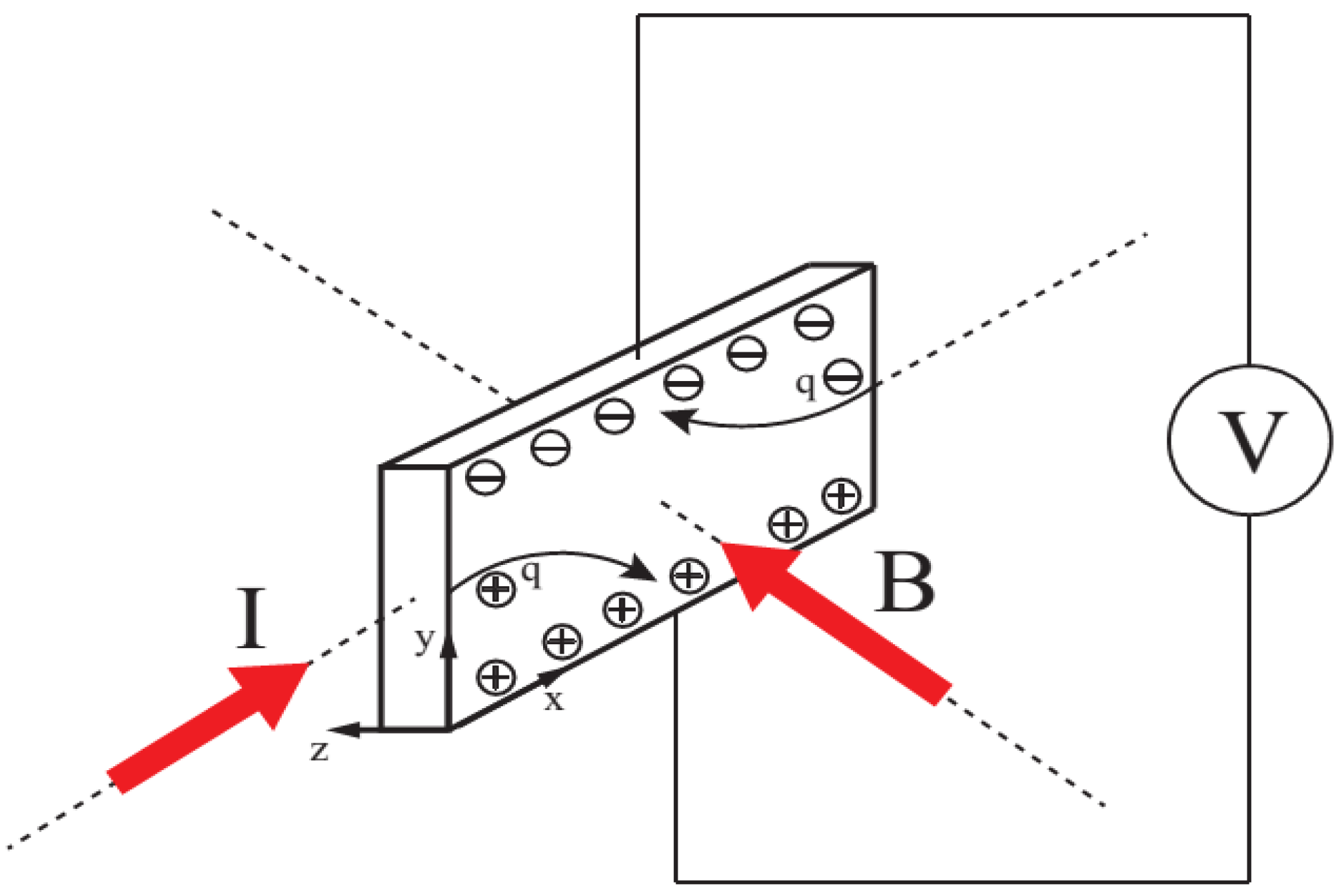
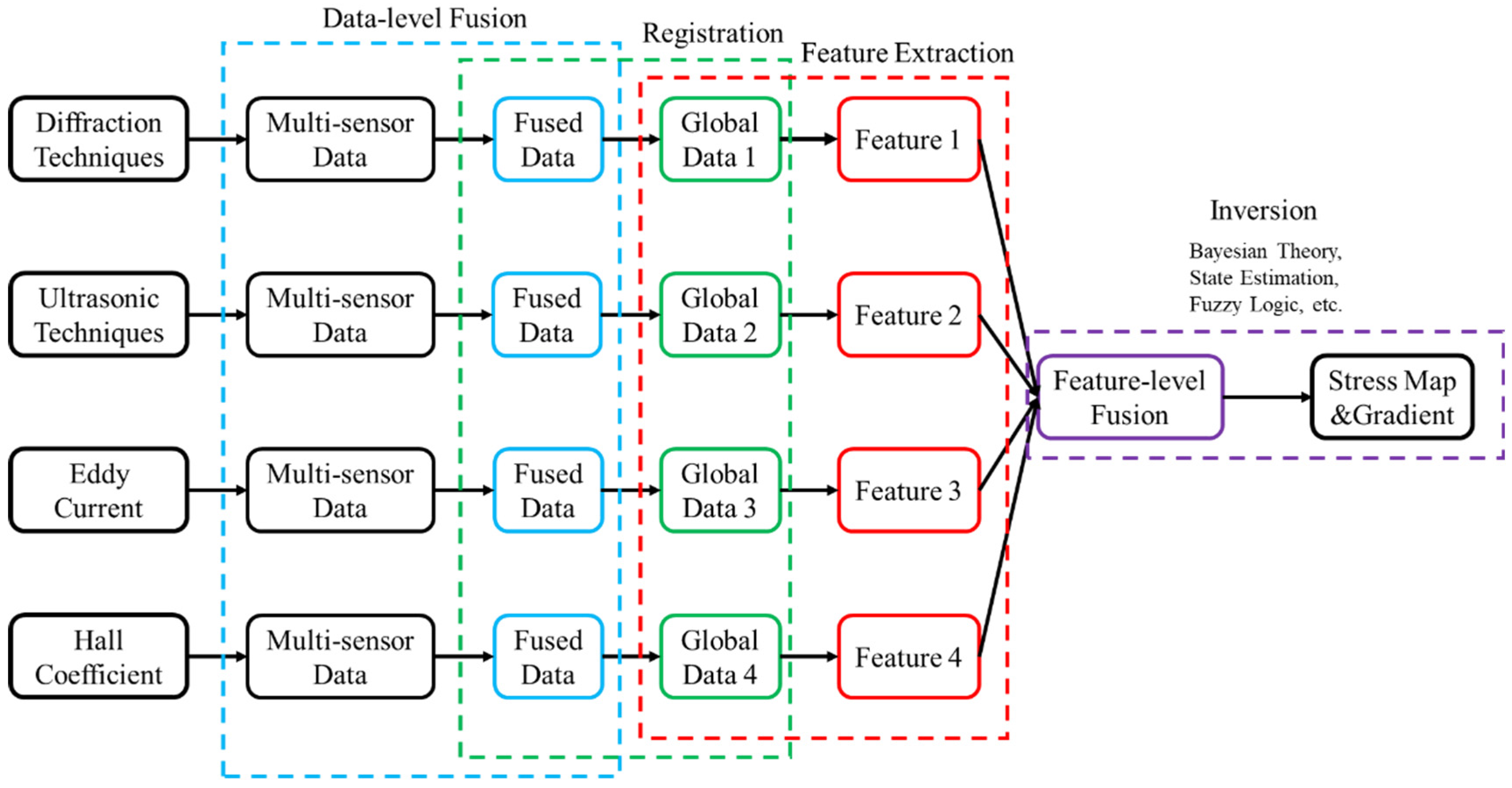

| Technique | Material Type | Portability | Advantages | Limitations |
|---|---|---|---|---|
| X-ray Diffraction | Crystalline | No | Small gauge volume Bi-axial measurements Widely available | Limited penetration depth Accuracy seriously affected by grain size and texture Semi-destructive for bulk measurement Surface preparation required |
| Synchrotron X-ray Diffraction | Crystalline | No | Good penetration depths Tri-axial residual stress measurements Small gauge volume (typically < 1 mm3) Applicable to complex shapes Indifferent to surface finish | Elongated gauge volume Only applicable to polycrystalline materials Accuracy affected by grain size and texture Very long lead time |
| Neutron Diffraction | Crystalline | No | Good penetration depths Tri-axial residual stress measurements Applicable to complex shapes Indifferent to surface finish | Only applicable to polycrystalline materials Accuracy affected by grain size and texture Very long lead time Not suitable for surface measurements |
| Critically refracted longitudinal wave | Solid | Yes | Quick measurement Greatest sensitivity to residual stress Frequency-dependent penetration depth | Dramatically influence by microstructure |
| Rayleigh wave | Solid | Yes | Quick measurement Frequency-dependent penetration depth | Dramatically influence by microstructure |
| Eddy current | Conductor | Yes | Quick measurement Frequency-dependent penetration depth | Selectivity to residual stress |
| Hall coefficient | Conductor | Yes | Quick measurement Frequency-dependent penetration depth | Selectivity to residual stress |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shao, Z.; Zhang, C.; Li, Y.; Shen, H.; Zhang, D.; Yu, X.; Zhang, Y. A Review of Non-Destructive Evaluation (NDE) Techniques for Residual Stress Profiling of Metallic Components in Aircraft Engines. Aerospace 2022, 9, 534. https://doi.org/10.3390/aerospace9100534
Shao Z, Zhang C, Li Y, Shen H, Zhang D, Yu X, Zhang Y. A Review of Non-Destructive Evaluation (NDE) Techniques for Residual Stress Profiling of Metallic Components in Aircraft Engines. Aerospace. 2022; 9(10):534. https://doi.org/10.3390/aerospace9100534
Chicago/Turabian StyleShao, Zhaoyu, Chengcheng Zhang, Yankai Li, Hai Shen, Dehan Zhang, Xudong Yu, and Ying Zhang. 2022. "A Review of Non-Destructive Evaluation (NDE) Techniques for Residual Stress Profiling of Metallic Components in Aircraft Engines" Aerospace 9, no. 10: 534. https://doi.org/10.3390/aerospace9100534
APA StyleShao, Z., Zhang, C., Li, Y., Shen, H., Zhang, D., Yu, X., & Zhang, Y. (2022). A Review of Non-Destructive Evaluation (NDE) Techniques for Residual Stress Profiling of Metallic Components in Aircraft Engines. Aerospace, 9(10), 534. https://doi.org/10.3390/aerospace9100534







