Görtler Vortices in the Shock Wave/Boundary-Layer Interaction Induced by Curved Swept Compression Ramp
Abstract
1. Introduction
2. Methodology
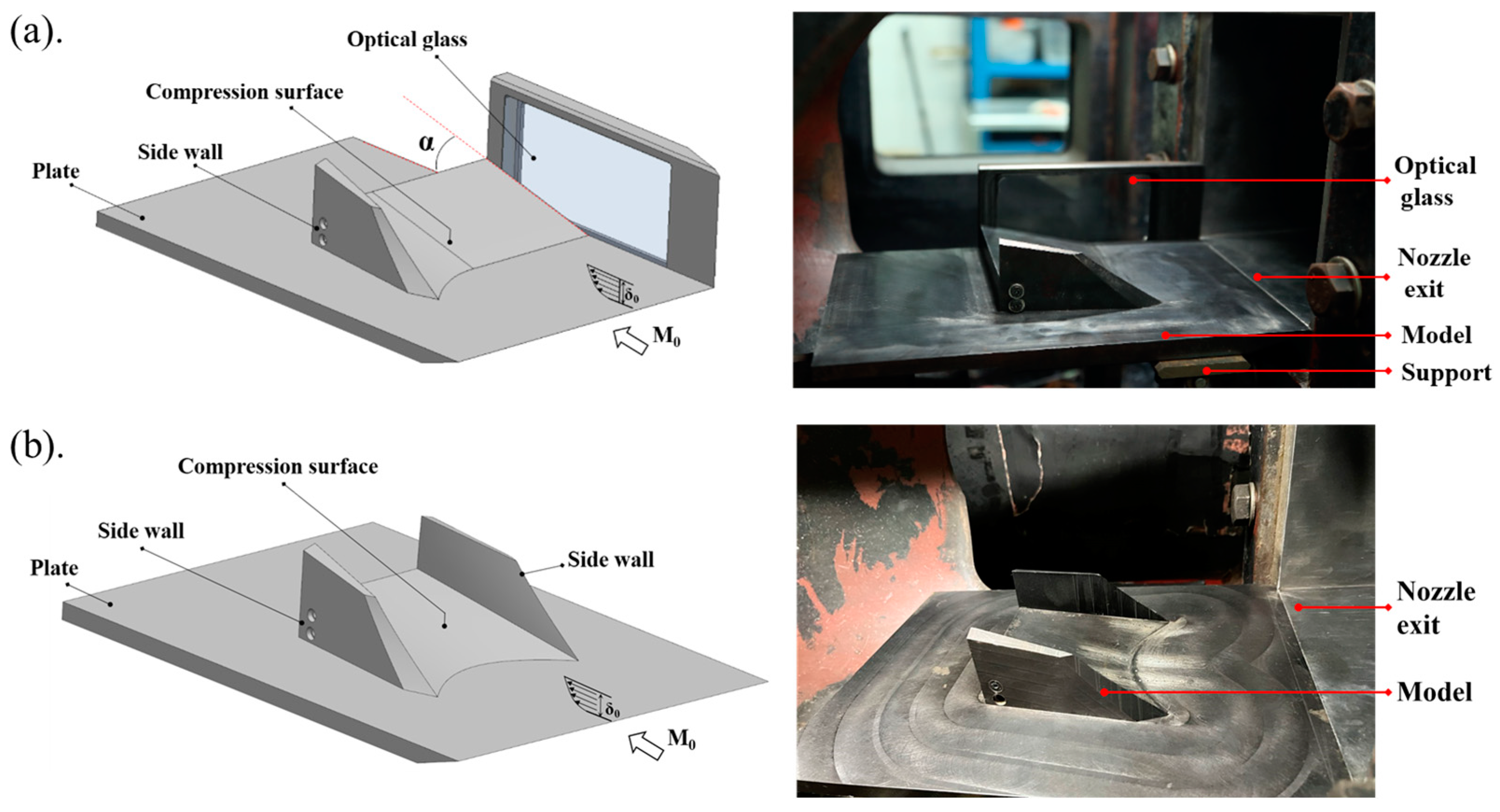
2.1. Description of the Test Model
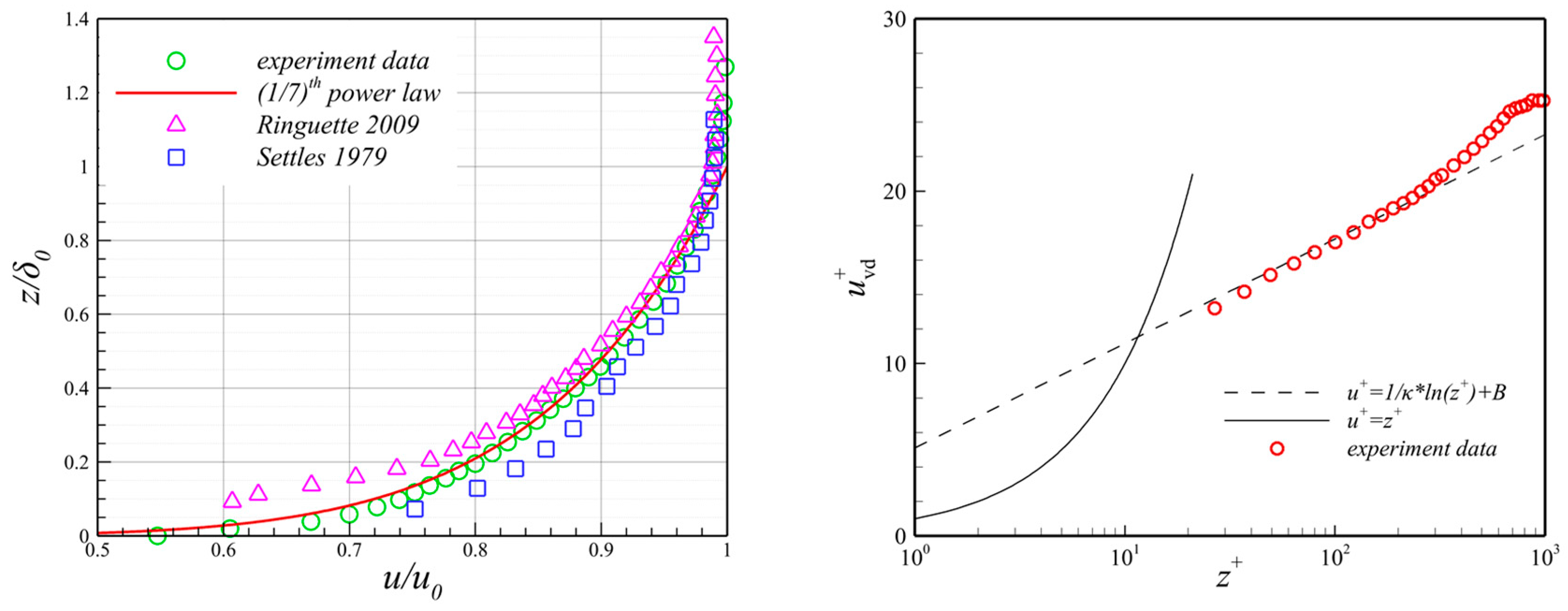
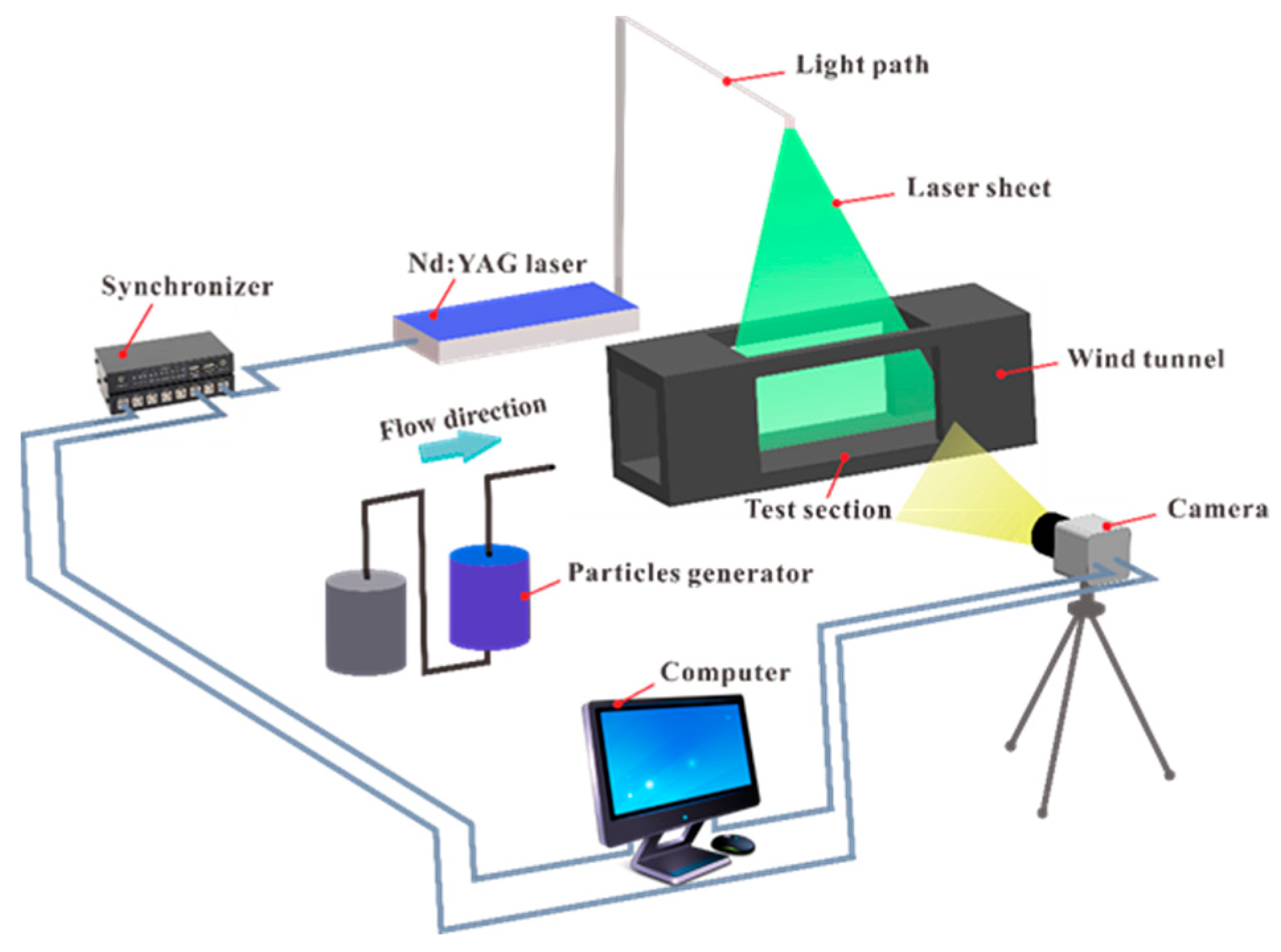
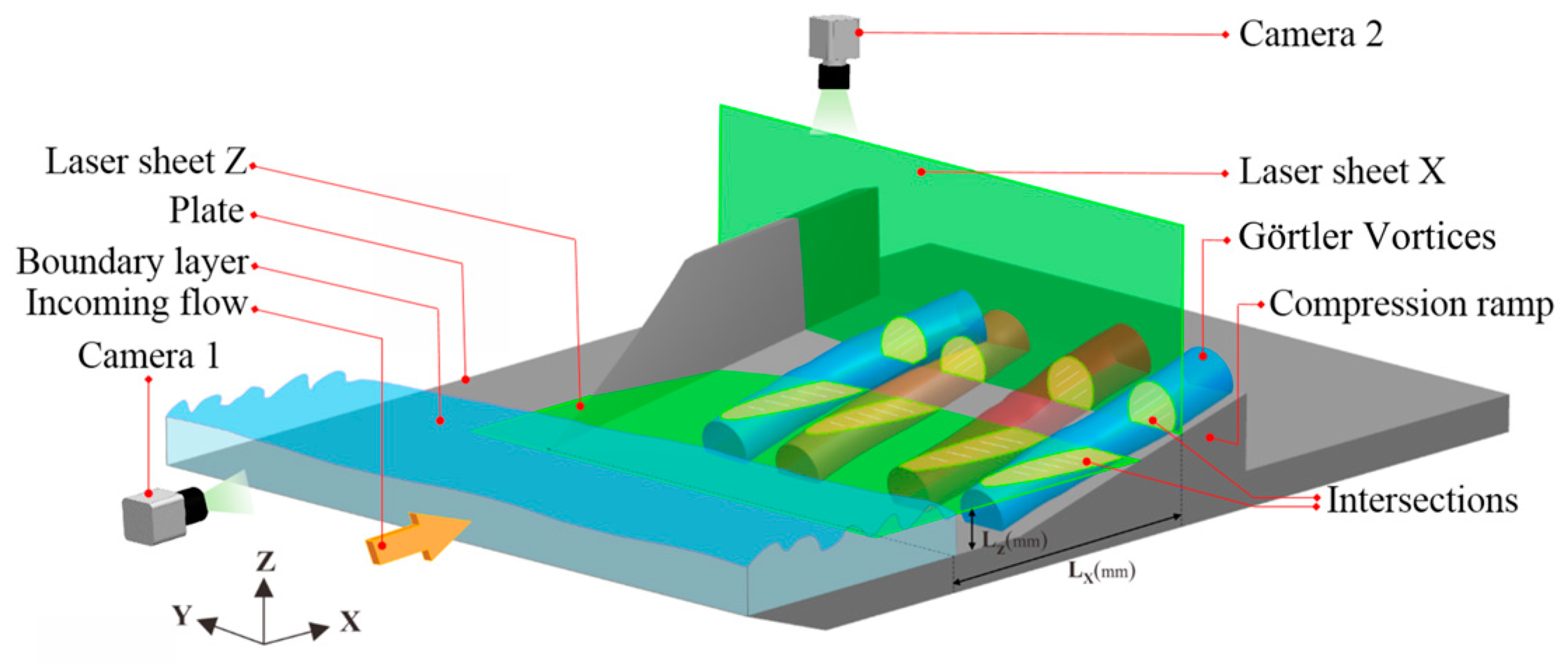
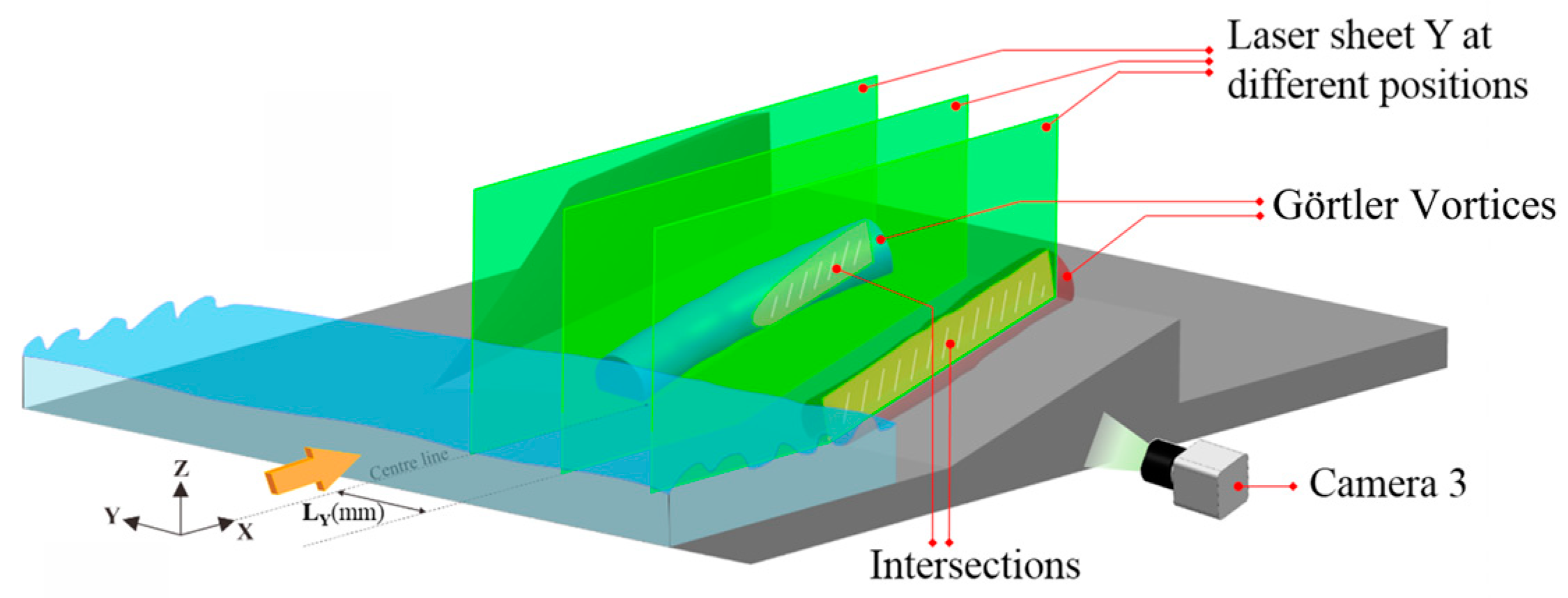
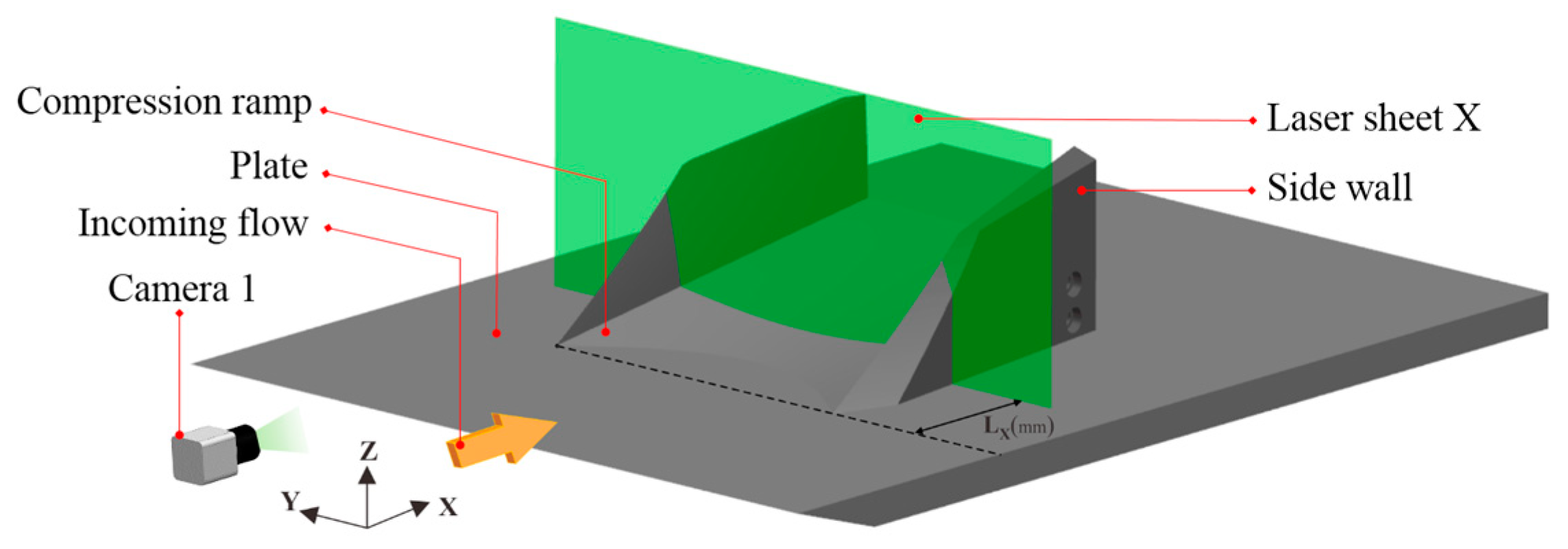
2.2. Experimental Arrangement
3. Results and Discussion
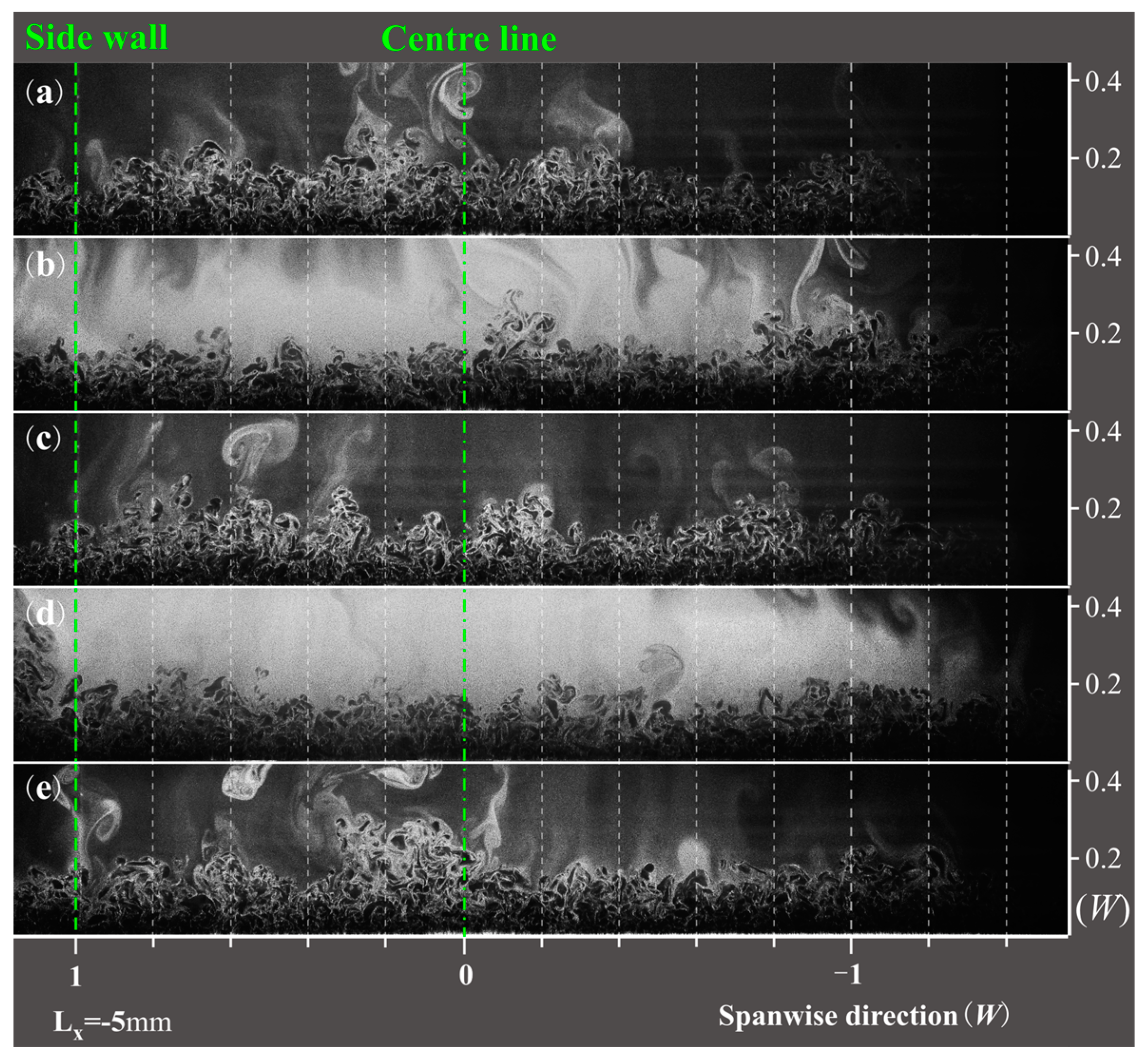
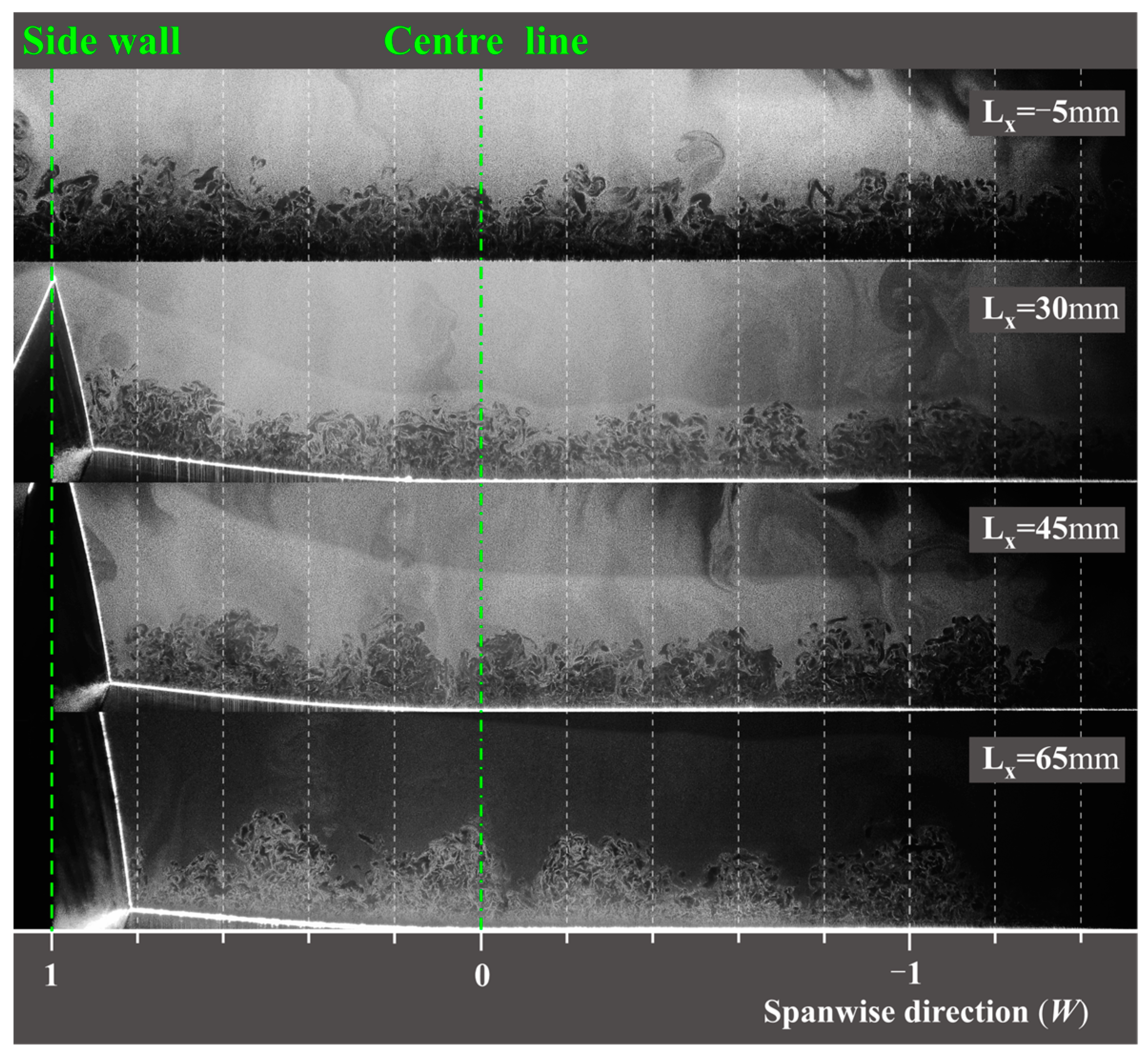
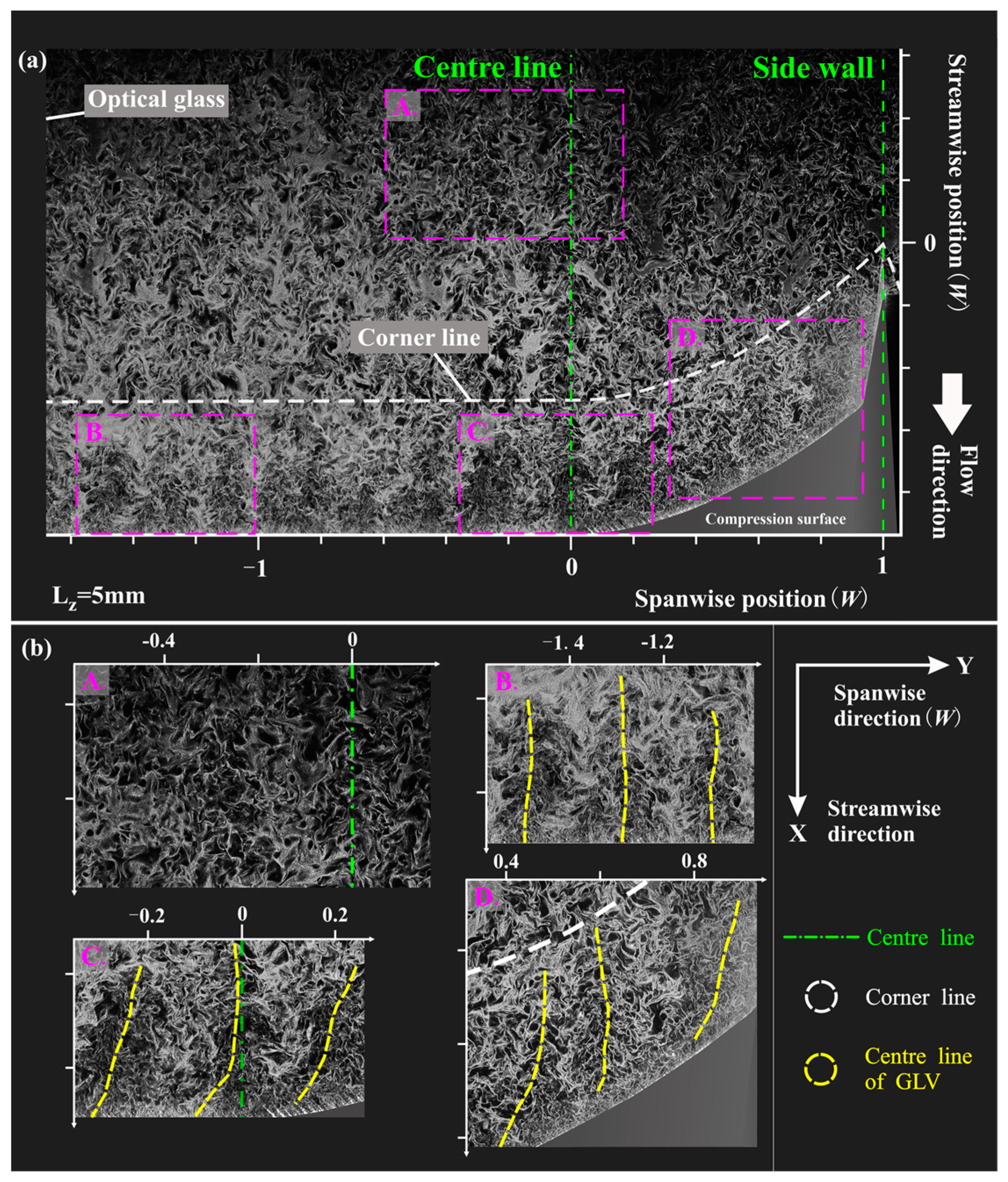
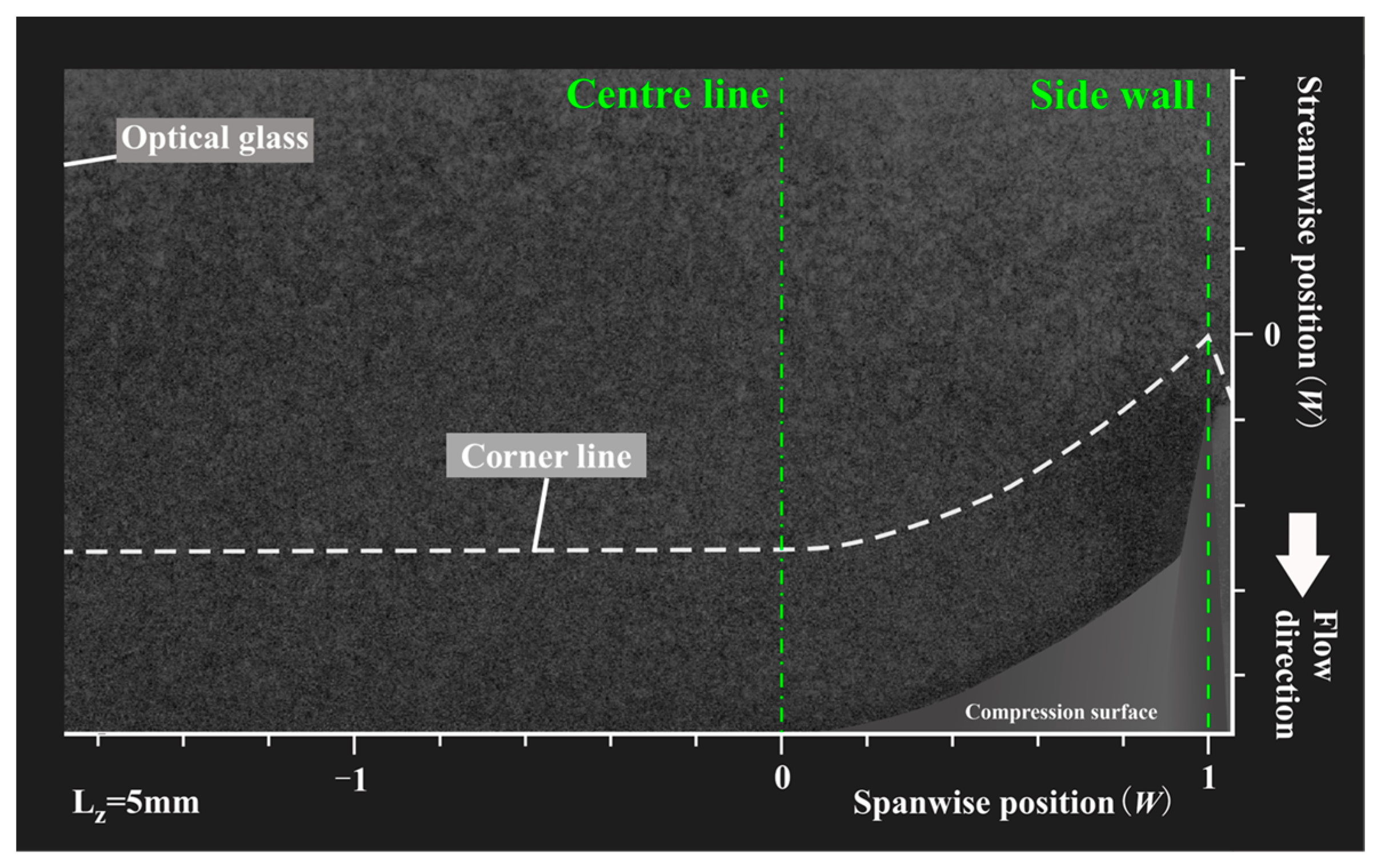
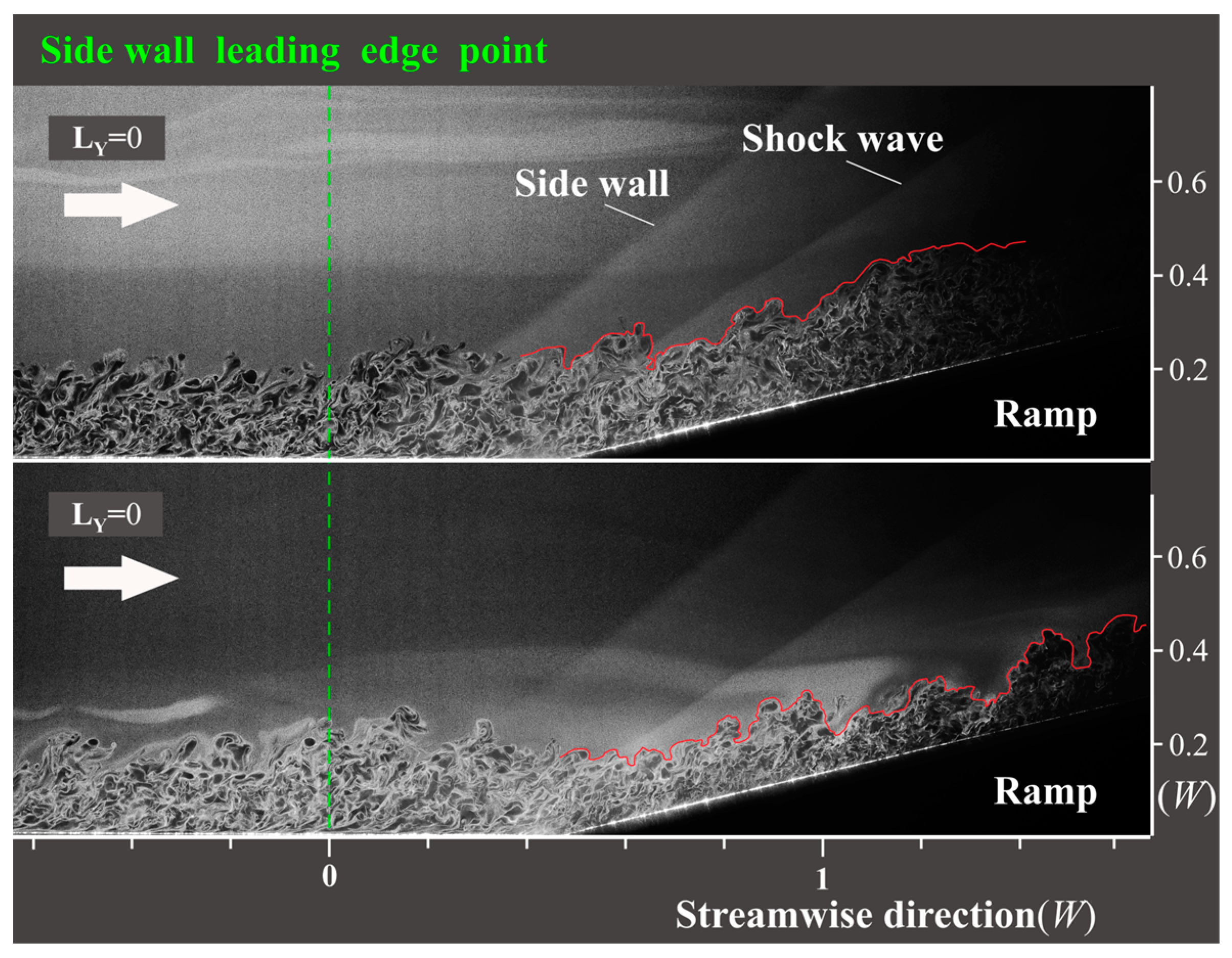
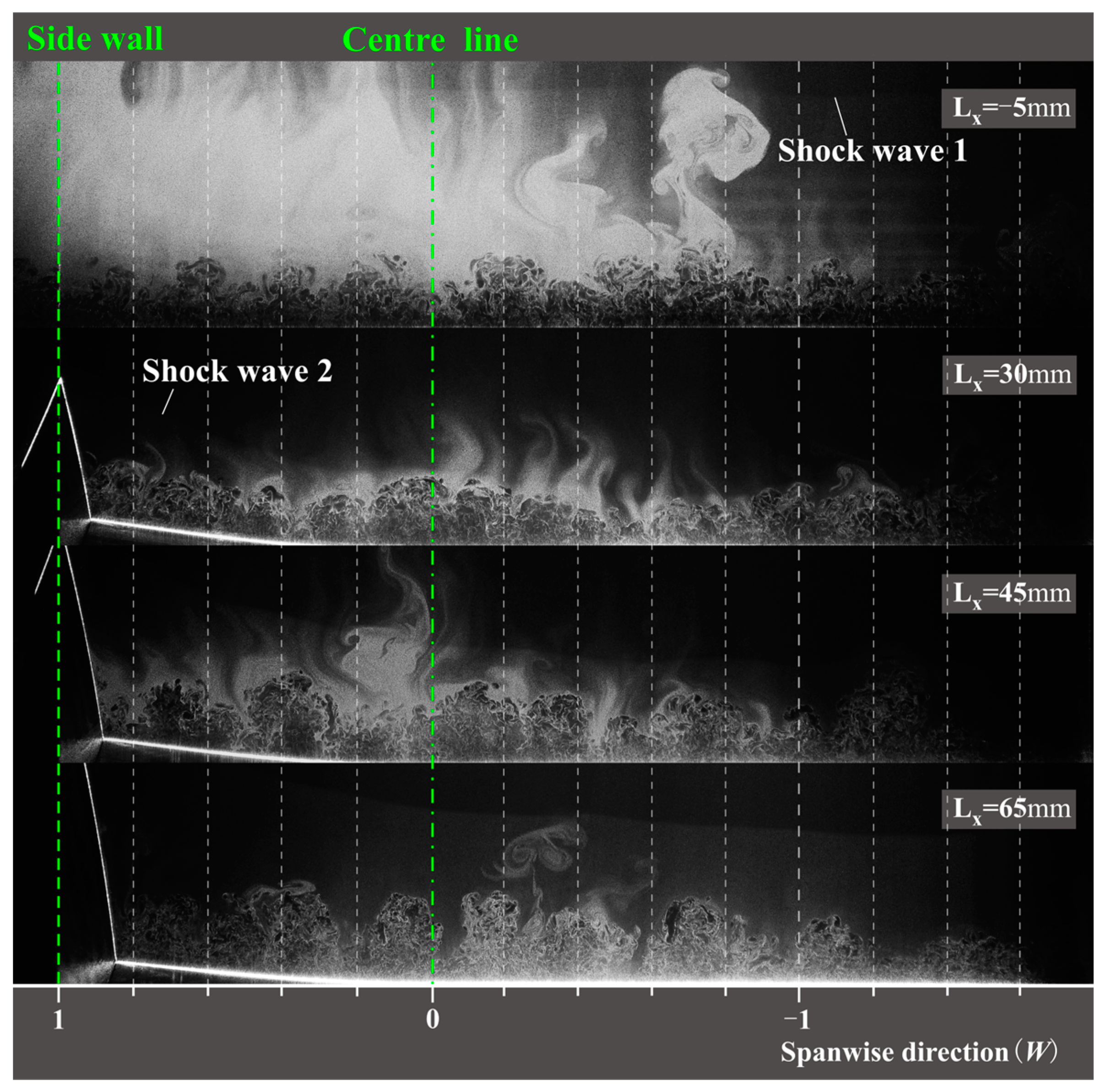
3.1. GV Structures Induced by CSCR-SWTBLI in Single-Sided Model
3.2. GV Structures Induced by CSCR-SWTBLI in Single-Sided Model at M0 = 2.66
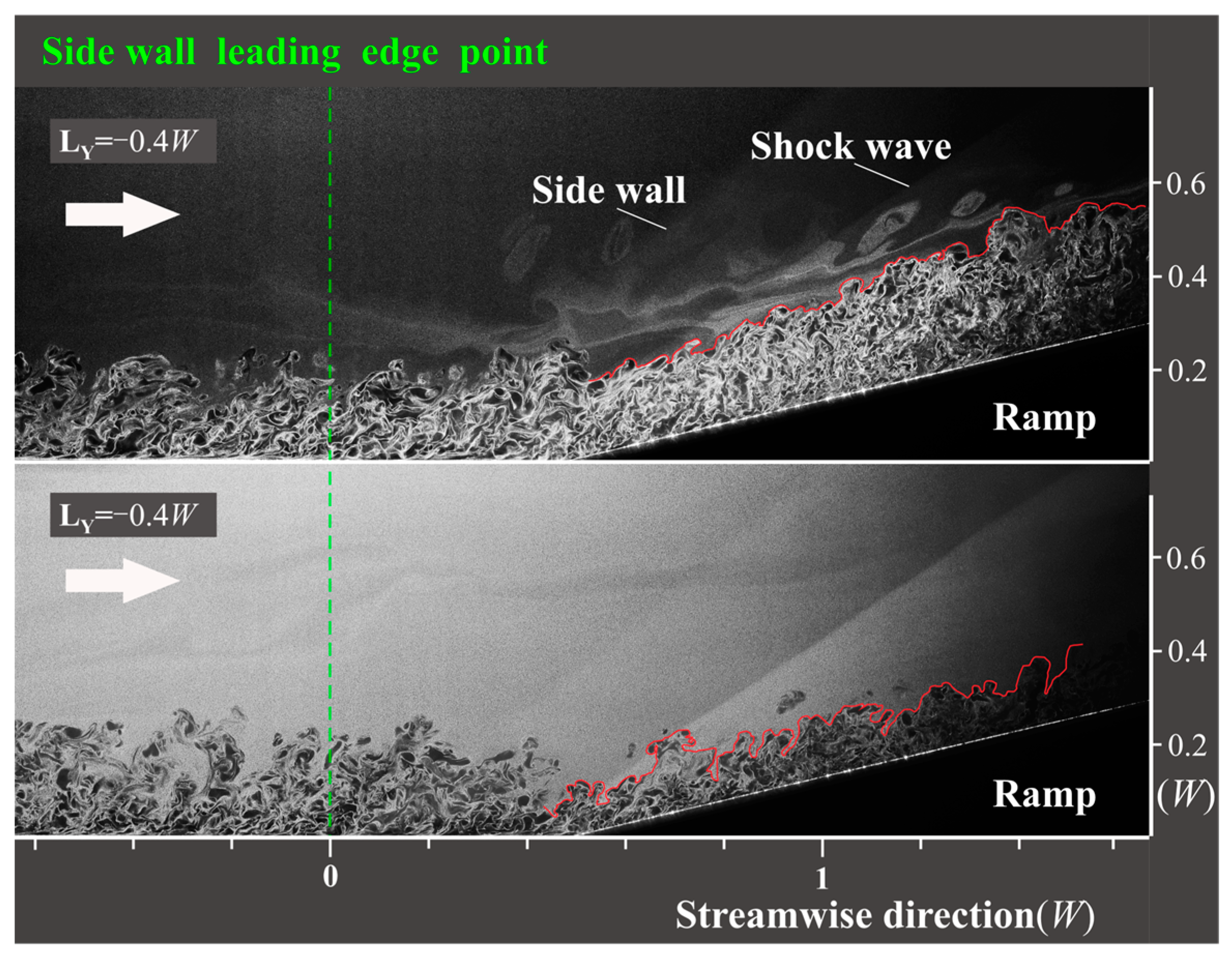
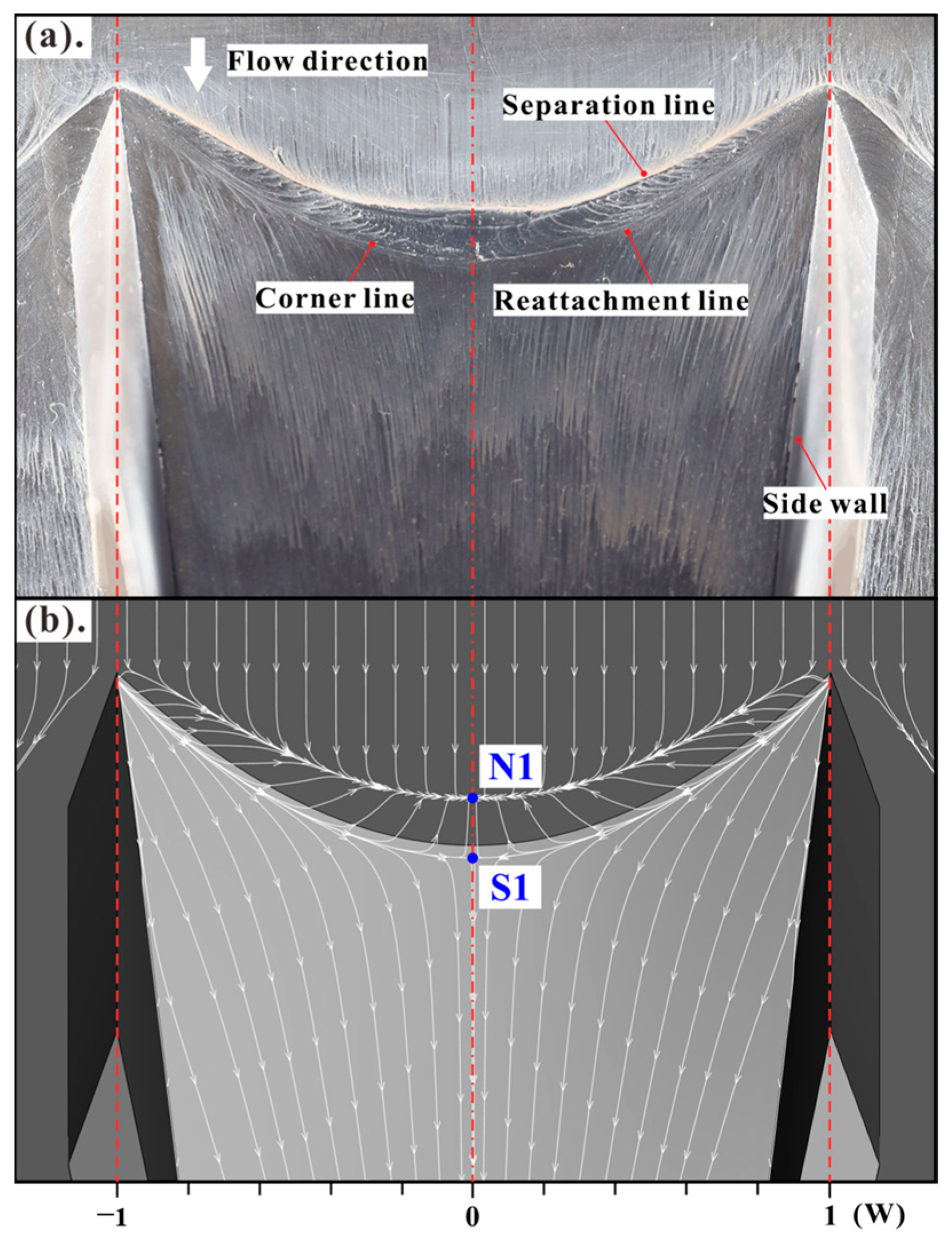
3.3. GV Structures Induced by CSCR-SWTBLI in Double-Sided Model
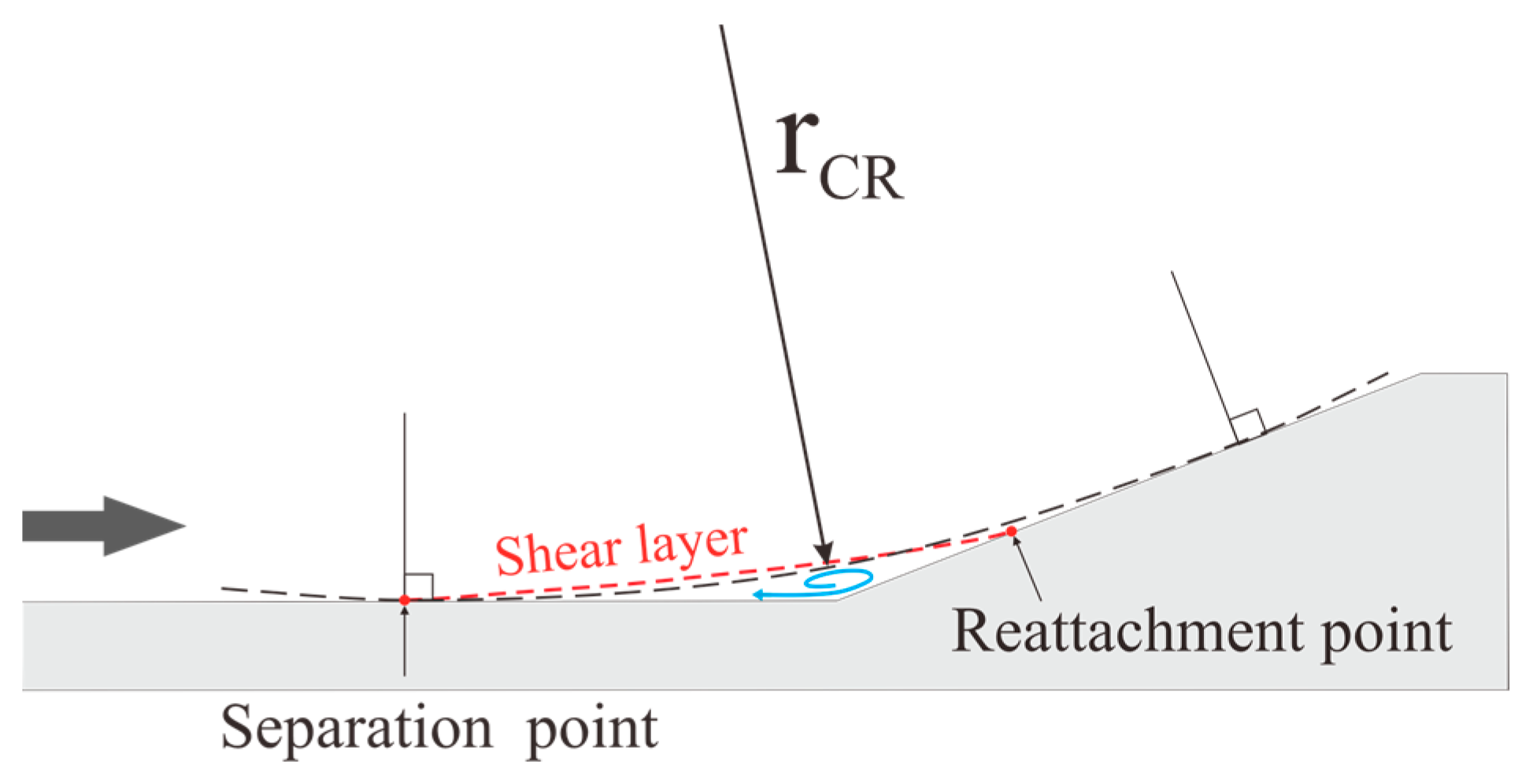
3.4. Verification of the Existence of GV Structures Induced by CSCR-SWBLI
4. Conclusions
- The quantity and spanwise position of GVs exhibit strong intermittency and are not fixed. The cross-section scale is consistently equivalent to the boundary layer thickness.
- Comparing the flow field at a +4° angle of attack reveals minimal changes in overall flow field characteristics, except for an increase in boundary layer density.
- The double-sided model demonstrates a stable, downstream-developing, large-scale vortex in the symmetry plane as a result of coupling effects. Notably, GV structures always appear in odd numbers. More importantly, the mixing effect of GVs on the boundary layer and the main stream within the inlet was found to improve the uniformity of the internal flow field and reduce distortion, and this provides a new research idea for the inlet designers to study the development and control of the boundary layer of the inward turning inlet.
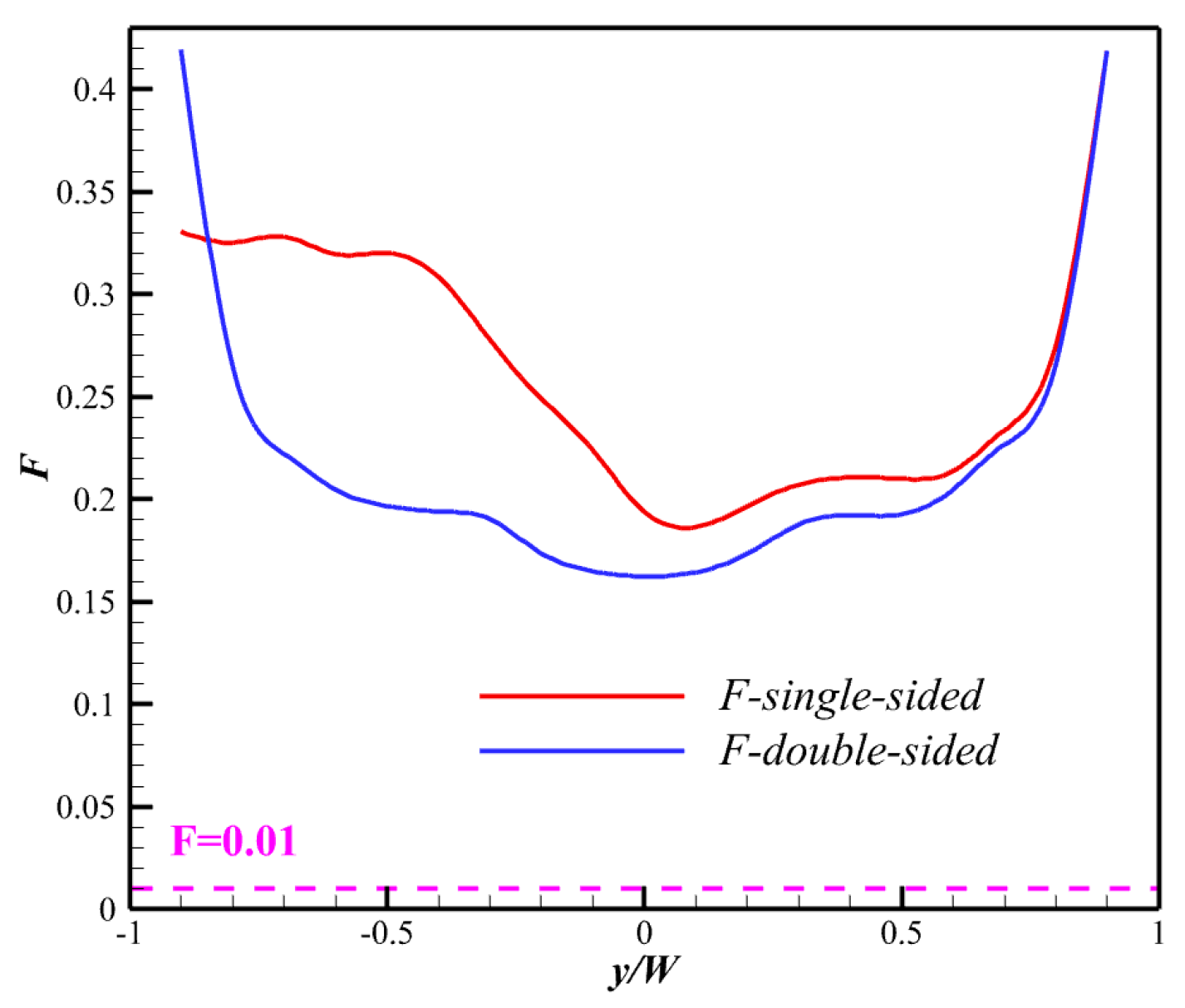
- Calculation of the GT number and F number for both single-sided and double-sided CSCR-SWBLI enables the determination of spanwise variation patterns. Both values significantly exceed the critical threshold, providing further evidence for the existence of GVs in CSCR-SWBLI.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Smart, M.K. How much compression should a scramjet inlet do? AIAA J. 2012, 50, 610–619. [Google Scholar] [CrossRef]
- Mölder, S.; Szpiro, E.J. Busemann inlet for hypersonic speeds. J. Spacecr. Rocket. 1966, 3, 1303–1304. [Google Scholar] [CrossRef]
- You, Y.C.; Liang, D.W.; Huang, G.P. Cross section controllable hypersonic inlet design using streamline-tracing and osculating axisymmetric concepts. In Proceedings of the 43rd AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Cincinnati, OH, USA, 8–11 July 2007. [Google Scholar]
- Billig, F.S.; Kothari, A.P. Streamline tracing: Technique for designing hypersonic vehicles. J. Propuls. Power 2000, 16, 465–471. [Google Scholar] [CrossRef]
- Rowan, J.G.; Michael, K.S. Design of modular shape-transition inlets for a conical hypersonic vehicle. J. Propuls. Power 2013, 29, 832–838. [Google Scholar]
- Samuel, E.O.; Charles, J.T.; John, W.S. Inward-turning streamline-traced inlet design method low-boom low-drag applications. J. Power Propuls. 2016, 32, 1178–1189. [Google Scholar]
- Li, Y.; Zheng, X.; Shi, C.; You, Y. Integration of inward-turning inlet with airframe based on dual-wave rider concept. Aerosp. Sci. Technol. 2020, 107, 106266. [Google Scholar] [CrossRef]
- Malo, F.J.; Gaitonde, D.V.; Ruffin, S. Analysis of an innovative inward turning inlet using an Air-JP8 combustion mixture at Mach 7. In Proceedings of the 36th AIAA Fluid Dynamics Conference and Exhibit, San Francisco, CA, USA, 5–8 June 2006. [Google Scholar]
- Babinsky, H.; Harvey, J.K. Shock Wave-Boundary-Layer Interactions; Cambridge University Press: Cambridge, UK, 2011; p. 32. [Google Scholar]
- Delery, J.; Marvin, G.J. Shock Wave–Boundary Layer Interactions; AGARD: Neuilly, France, 1986. [Google Scholar]
- Settles, G.S.; Vas, I.E.; Bogdonoff, S.M. Details of a shock-separated turbulent boundary layer at a compression corner. AIAA J. 1976, 14, 1709–1715. [Google Scholar] [CrossRef]
- Settles, G.S.; Fitzpatrick, T.J.; Bogdonoff, S.M. Detailed study of attached and separated compression corner flowfields in high Reynolds number supersonic flow. AIAA J. 1979, 17, 579–585. [Google Scholar] [CrossRef]
- Smits, A.J.; Muck, K.C. Experimental study of three shock wave/turbulent boundary layer interactions. J. Fluid Mech. 1987, 182, 291–314. [Google Scholar] [CrossRef]
- Elfstrom, G.M. Turbulent hypersonic flow at a wedge-compression corner. J. Fluid Mech. 1972, 53, 113–127. [Google Scholar] [CrossRef]
- Dolling, D.S.; Or, C.T. Unsteadiness of the shock wave structure in attached and separated compression ramp flows. Exp. Fluids 1985, 3, 24–32. [Google Scholar] [CrossRef]
- Loginov, M.S.; Adams, N.A.; Zheltovodov, A.A. Large-eddy simulation of shock-wave/turbulent-boundary-layer interaction. J. Fluid Mech. 2006, 565, 135–169. [Google Scholar] [CrossRef]
- Grilli, M.; Hickel, S.; Adams, N.A. Large eddy simulation of a supersonic turbulent boundary layer over a compression–expansion ramp. Int. J. Heat Fluid Flow 2013, 42, 79–93. [Google Scholar] [CrossRef]
- Zheltovodov, A.A. Some advances in research of shock wave-turbulent boundary. In Proceedings of the 44th AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 9–12 January 2006. [Google Scholar]
- Zheltovodov, A.A. Advances and problems in modeling of shock wave turbulent boundary layer interactions. In Proceedings of the International Conference on the Methods of Aero Physical Research, Novosibirsk, Russia, 1–5 July 2024. [Google Scholar]
- Zheltovodov, A.A.; Maksimov, A.; Shilein, E. Development of turbulent separated flows in the vicinity of swept shock waves. In The Interactions of Complex; Springer: Berlin/Heidelberg, Germany, 1987; pp. 67–91. [Google Scholar]
- Settles, G.S.; Teng, H. Cylindrical and conical flow regimes of three-dimensional shock/boundary layer interactions. AIAA J. 1984, 22, 194–200. [Google Scholar] [CrossRef]
- Settles, G.S.; Perkins, J.J.; Bogdonoff, S.M. Investigation of three-dimensional shock/boundary layer interaction at swept compression corners. AIAA J. 1980, 18, 779–785. [Google Scholar] [CrossRef]
- Knight, D.D.; Horstman, C.; Bogdonoff, S.M. Structure of supersonic turbulent flow past a swept compression corner. AIAA J. 1992, 30, 890–896. [Google Scholar] [CrossRef]
- Görtler, H. Instabilität laminarer Grenzschichten an konkaven Wänden gegenüber gewissen dreidimensionalen Störungen. ZAMM-J. Appl. Math. Mech./Z. Angew. Math. Mech. 1941, 21, 250–252. [Google Scholar] [CrossRef]
- Tong, F.; Li, X.; Duan, Y.; Yu, C. Direct numerical simulation of supersonic turbulent boundary layer subjected to a curved compression ramp. Phys. Fluids 2017, 29, 125101. [Google Scholar] [CrossRef]
- Wang, Q.; Wang, Z.; Zhao, Y. An experimental investigation of the supersonic turbulent boundary layer subjected to concave curvature. Phys. Fluids 2016, 28, 96–104. [Google Scholar] [CrossRef]
- Chen, X.; Zhu, Y.; Lee, C. Interactions between second mode and low-frequency waves in a hypersonic boundary layer. J. Fluid Mech. 2017, 820, 693–735. [Google Scholar] [CrossRef]
- Loginov, M.S. Large-Eddy Simulation of Shock Wave/Turbulent Boundary Layer Interaction; Technische Universität München: Munich, Germany, 2006. [Google Scholar]
- Tokura, Y.; Maekwa, H. Direct numerical simulation of impinging shock wave/transitional boundary layer interaction with separation flow. J. Fluid Sci. Technol. 2011, 6, 765–779. [Google Scholar] [CrossRef][Green Version]
- Pasquariello, V.; Hickel, S.; Adams, N.A. Unsteady effects of strong shock-wave/boundary-layer interaction at high Reynolds number. J. Fluid Mech. 2017, 823, 617–657. [Google Scholar] [CrossRef]
- Zhuang, Y.; Tan, H.J.; Li, X.; Sheng, F.J.; Zhang, Y.C. Görtler-like vortices in an impinging shock wave/turbulent boundary layer interaction flow. Phys. Fluids 2018, 30, 061702. [Google Scholar] [CrossRef]
- Li, X.; Zhang, Y.; Yu, H.; Lin, Z.K.; Tan, H.J.; Sun, S. Görtler vortices behavior and prediction in dual-incident shock-wave/turbulent-boundary-layer interactions. Phys. Fluids 2022, 34, 106103. [Google Scholar] [CrossRef]
- Ciolkosz, L.; Spina, E. An experimental study of Görtler vortices in compressible flow. In Proceedings of the 42nd AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Sacramento, CA, USA, 9–12 July 2006; p. 4512. [Google Scholar]
- Swearingen, J.D.; Blackwelder, R.F. The growth and breakdown of streamwise vortices in the presence of a wall. J. Fluid Mech. 1987, 182, 255–290. [Google Scholar] [CrossRef]
- Sun, M.; Sandham, N.D.; Hu, Z. Turbulence structures and statistics of a supersonic turbulent boundary layer subjected to concave surface curvature. J. Fluid Mech. 2019, 865, 60–99. [Google Scholar] [CrossRef]
- Ren, J.; Fu, S. Nonlinear development of the multiple Görtler modes in hypersonic boundary layer flows. In Proceedings of the 43rd AIAA Fluid Dynamics Conference, San Diego, CA, USA, 24–27 June 2013; p. 2467. [Google Scholar]
- Ren, J.; Fu, S. Secondary instabilities of Görtler vortices in high-speed boundary layer flows. J. Fluid Mech. 2015, 781, 388–421. [Google Scholar] [CrossRef]
- Roghelia, A.; Olivier, H.; Egorov, I.; Chuvakhov, P. Experimental investigation of Görtler vortices in hypersonic ramp flows. Exp. Fluids 2017, 58, 1–15. [Google Scholar] [CrossRef]
- Chen, L.; Zhang, Y.; Xue, H.; Chuvakhov, P. Experimental and numerical investigation of shock wave/boundary layer interactions induced by curved back-swept compression ramp. Aerosp. Sci. Technol. 2023, 142, 108639. [Google Scholar] [CrossRef]
- Zhang, Y.; Chen, L.; Tan, H.J.; Wang, C.; Cheng, F.; Li, C. Visualization of curved swept shock wave/turbulent boundary layer interaction in supersonic flow. J. Vis. 2020, 24, 1–7. [Google Scholar] [CrossRef]
- Ringuette, M.J.; Bookey, P.; Wyckham, C.; Smits, A.J. Experimental Study of a Mach 3 Compression Ramp Interaction at Re{theta} = 2400. AIAA J. 2009, 47, 373–385. [Google Scholar] [CrossRef]
- Anthony, K.; Manoochehr, K. A Method for Estimating Wall Friction in Turbulent Wall-Bounded Flows. Exp. Fluids 2008, 55, 773–780. [Google Scholar]
- Görtler, H. On the Three-Dimensional Instability of Laminar Boundary Layers on Concave Walls; National Advisory Commitee for Aeronautics Technical Memorandum: Hampton, VA, USA, 1954; p. 1375. [Google Scholar]
- Floryan, J.M. On the Görtler instability of boundary layers. Prog. Aerosp. Sci. 1991, 28, 235–271. [Google Scholar] [CrossRef]
- Priebe, S.; Tu, J.H.; Rowley, C.W.; Martín, M.P. Low-frequency dynamics in a shock-induced separated flow. J. Fluid Mech. 2016, 807, 441–477. [Google Scholar] [CrossRef]
- Duan, J.Y.; Li, X.; Li, X.L.; Liu, H. Direct numerical simulation of a supersonic turbulent boundary layer over a compression-decompression corner. Phys. Fluids 2021, 33, 065111. [Google Scholar] [CrossRef]
- Zhuang, Y.; Tan, H.J.; Liu, Y.Z.; Zhang, Y.C.; Ling, Y. High-Resolution Visualization of Görtler-Like Vortices in Supersonic Compression Ramp Flow. J. Vis. 2017, 20, 505–508. [Google Scholar] [CrossRef]
- Smits, A.J.; Dussauge, J.P. Turbulent Shear Layers in Supersonic Flow; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Navarro-Martinez, S.; Tutty, O.R. Numerical simulation of Görtler vortices in hypersonic compression ramps. Comput. Fluids 2005, 34, 225–247. [Google Scholar] [CrossRef]

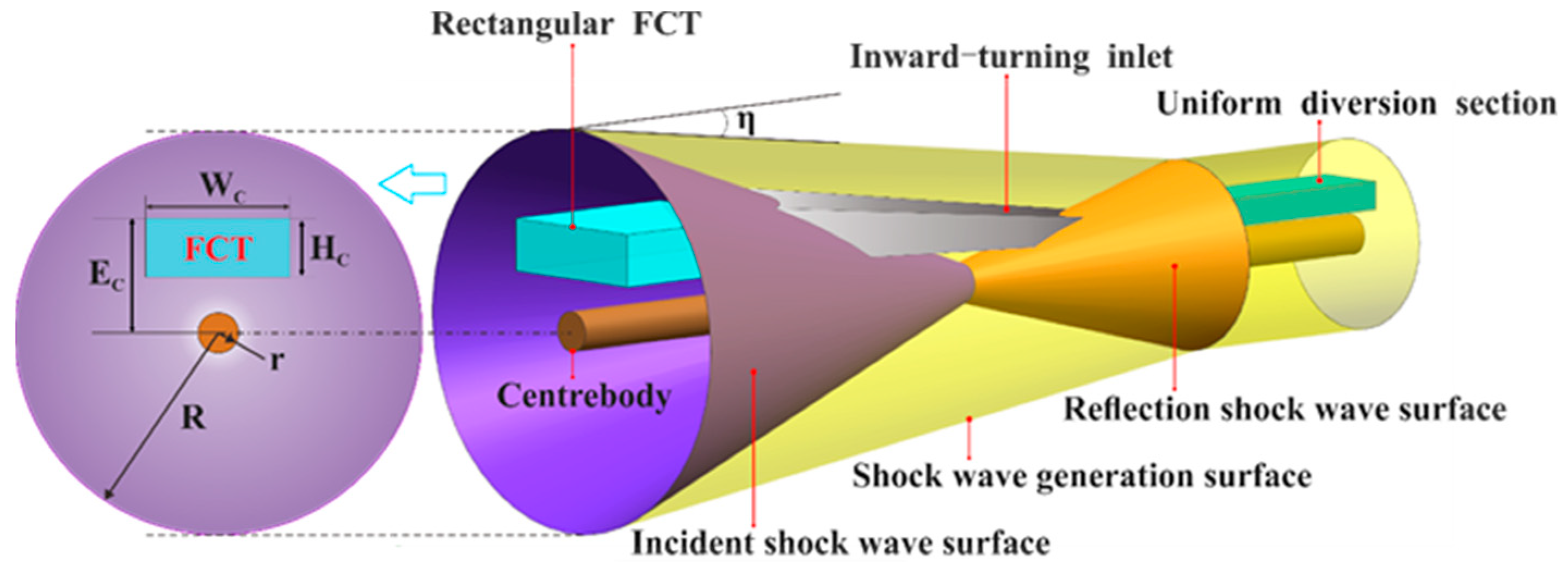






| Parameters | Value |
|---|---|
| R, mm | 150 |
| r, mm | 25 |
| Wc, mm | 100 |
| Hc, mm | 40 |
| Ec, mm | 80 |
| η, deg | 11 |
| Parameters | Value |
|---|---|
| Nominal Mach number | 3.0 |
| Actual Mach number | 2.85 ± 0.01 |
| Total temperature, K | 298.2 |
| Total pressure, kPa | 100.91 |
| Usable run time, s | >15 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, L.; Zhang, Y.; Wang, J.; Xue, H.; Xu, Y.; Wang, Z.; Tan, H. Görtler Vortices in the Shock Wave/Boundary-Layer Interaction Induced by Curved Swept Compression Ramp. Aerospace 2024, 11, 760. https://doi.org/10.3390/aerospace11090760
Chen L, Zhang Y, Wang J, Xue H, Xu Y, Wang Z, Tan H. Görtler Vortices in the Shock Wave/Boundary-Layer Interaction Induced by Curved Swept Compression Ramp. Aerospace. 2024; 11(9):760. https://doi.org/10.3390/aerospace11090760
Chicago/Turabian StyleChen, Liang, Yue Zhang, Juanjuan Wang, Hongchao Xue, Yixuan Xu, Ziyun Wang, and Huijun Tan. 2024. "Görtler Vortices in the Shock Wave/Boundary-Layer Interaction Induced by Curved Swept Compression Ramp" Aerospace 11, no. 9: 760. https://doi.org/10.3390/aerospace11090760
APA StyleChen, L., Zhang, Y., Wang, J., Xue, H., Xu, Y., Wang, Z., & Tan, H. (2024). Görtler Vortices in the Shock Wave/Boundary-Layer Interaction Induced by Curved Swept Compression Ramp. Aerospace, 11(9), 760. https://doi.org/10.3390/aerospace11090760