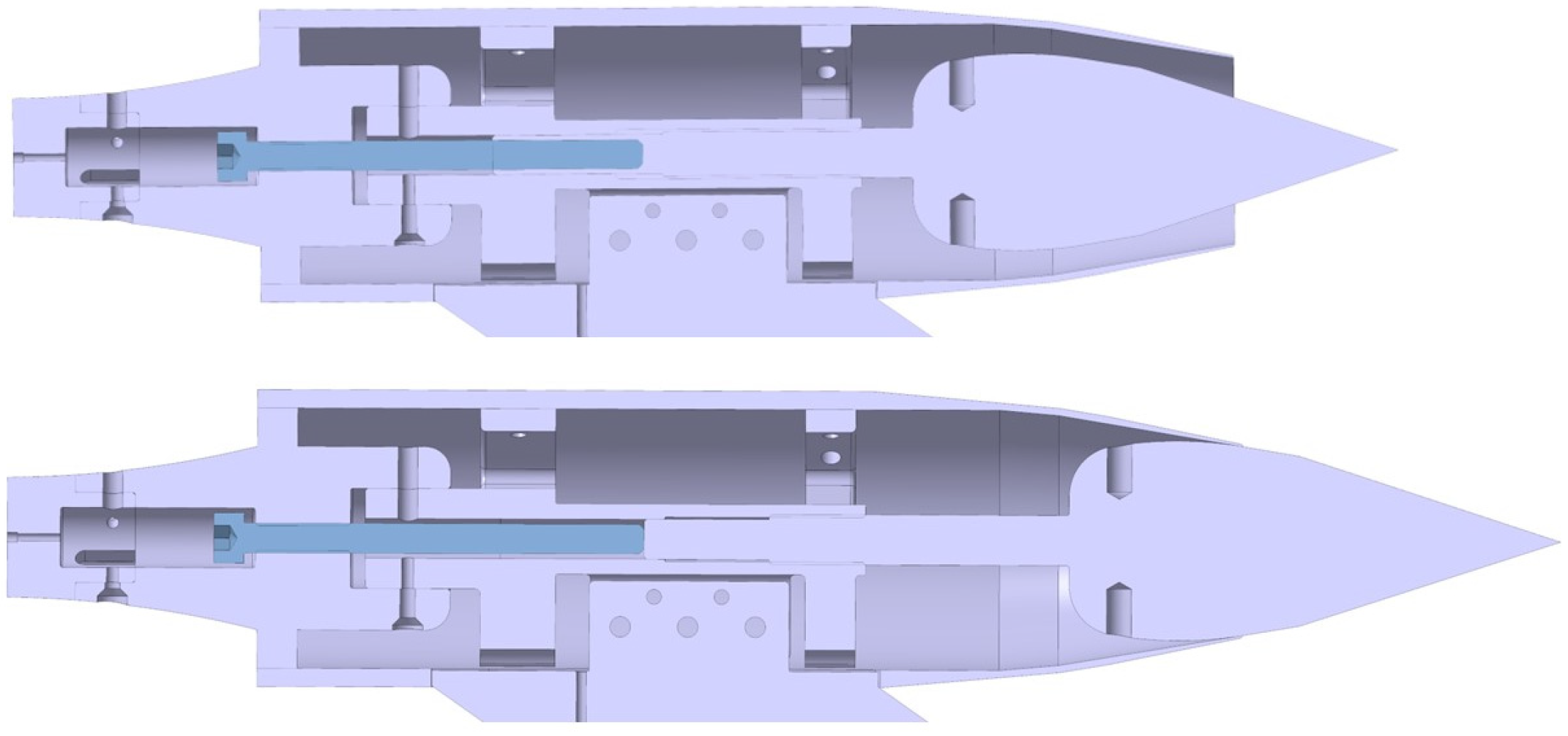
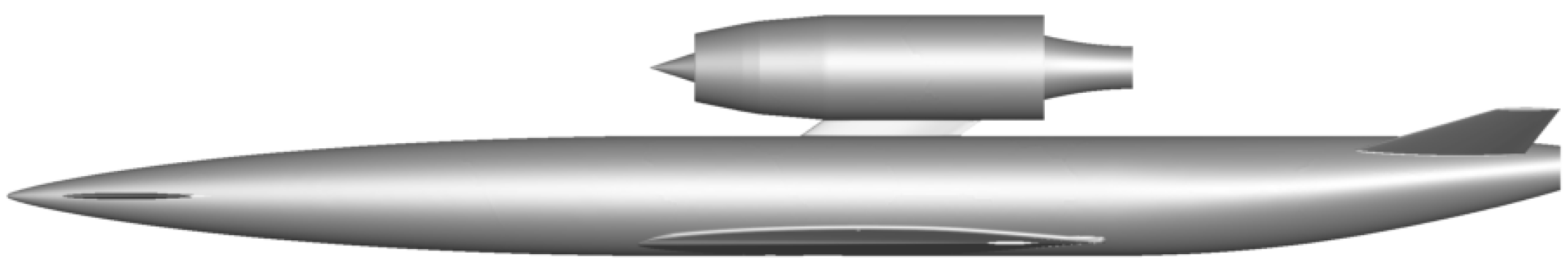
Figure 1.
Straight (top) and bent (bottom) sting for the wind tunnel model.
Figure 1.
Straight (top) and bent (bottom) sting for the wind tunnel model.
Figure 2.
Internal structure of the model nacelle in the flow-through configuration (top) and closed configuration (bottom).
Figure 2.
Internal structure of the model nacelle in the flow-through configuration (top) and closed configuration (bottom).
Figure 3.
The Trisonic Wind Tunnel at INCAS.
Figure 3.
The Trisonic Wind Tunnel at INCAS.
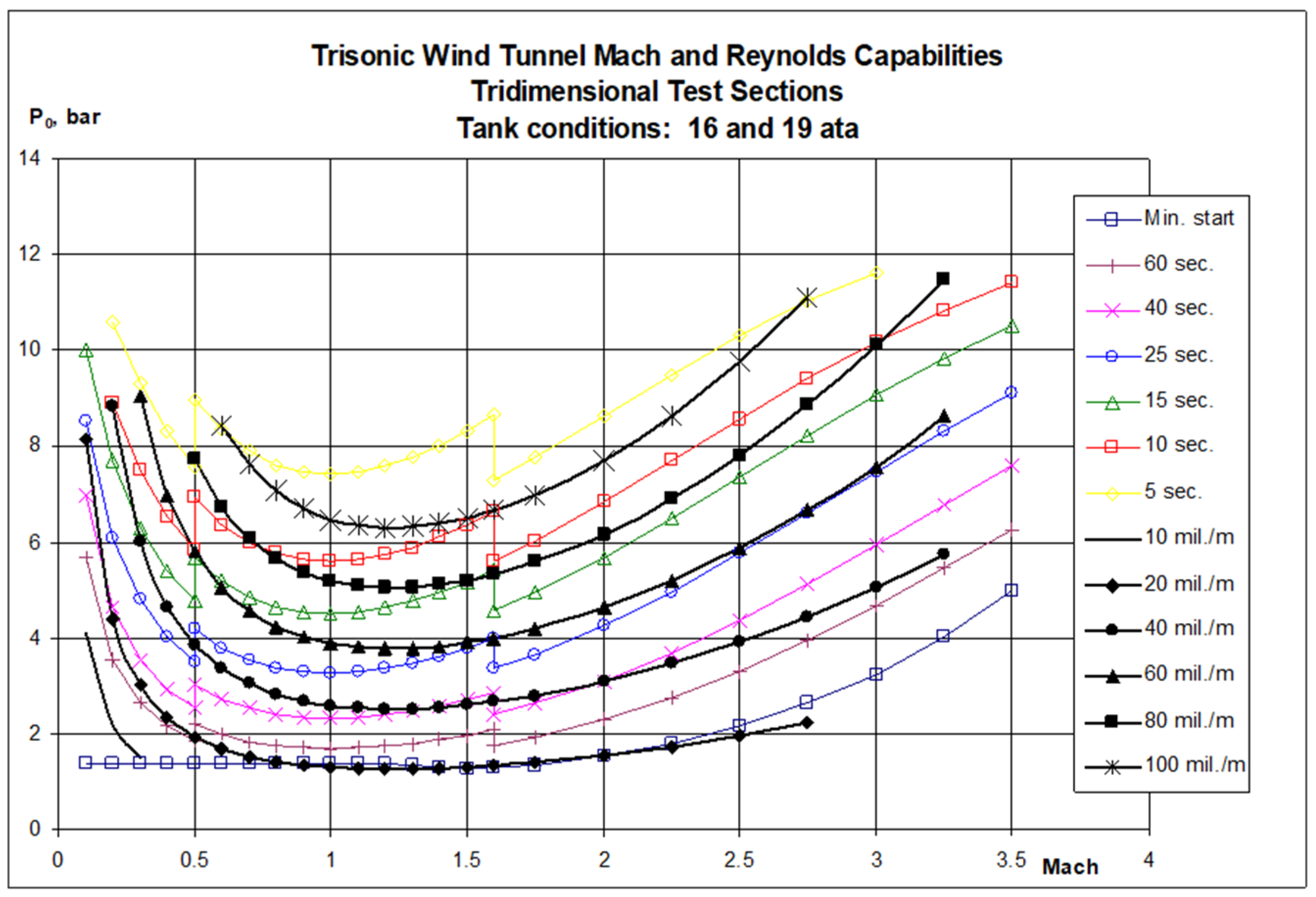
Figure 4.
The Trisonic Wind Tunnel stagnation pressure vs. Mach capability.
Figure 4.
The Trisonic Wind Tunnel stagnation pressure vs. Mach capability.
Figure 5.
CS-2 wind tunnel model with transition strips.
Figure 5.
CS-2 wind tunnel model with transition strips.
Figure 6.
Experimental results for the AoS = 0° cases: (a) drag coefficient vs. AoA; (b) lift coefficient vs. AoA; (c) pitching moment coefficient vs. AoA.
Figure 6.
Experimental results for the AoS = 0° cases: (a) drag coefficient vs. AoA; (b) lift coefficient vs. AoA; (c) pitching moment coefficient vs. AoA.
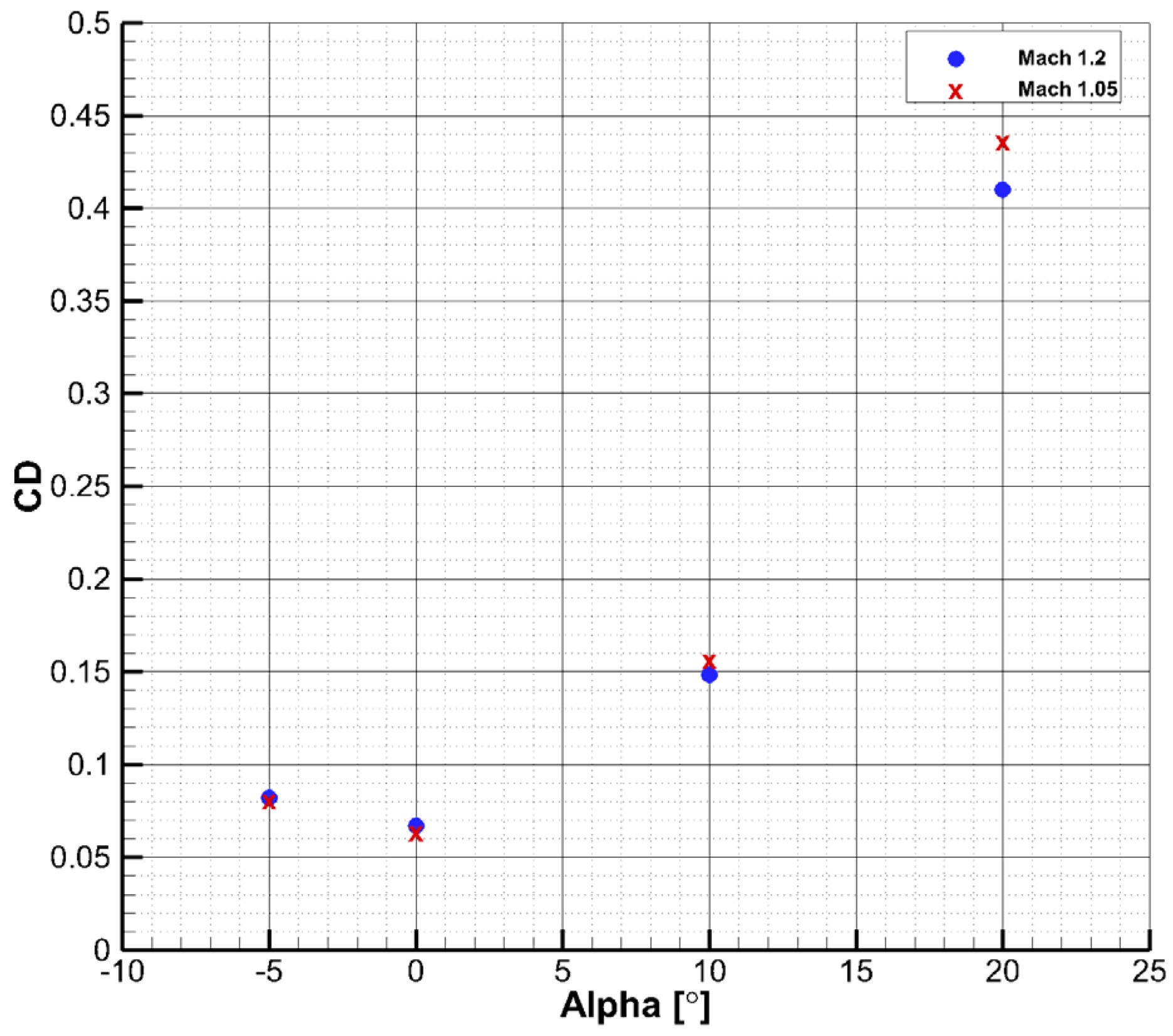
Figure 7.
CFD results for drag vs. AoA at M = 1.05 and M = 1.2 (wind tunnel configuration).
Figure 7.
CFD results for drag vs. AoA at M = 1.05 and M = 1.2 (wind tunnel configuration).
Figure 8.
Example of uncertainties for the experimental data at M = 1.05: (a) drag coefficient vs. AoA; (b) lift coefficient vs. AoA; (c) pitching moment coefficient vs. AoA.
Figure 8.
Example of uncertainties for the experimental data at M = 1.05: (a) drag coefficient vs. AoA; (b) lift coefficient vs. AoA; (c) pitching moment coefficient vs. AoA.
Figure 9.
CFD model of the wind tunnel configuration.
Figure 9.
CFD model of the wind tunnel configuration.
Figure 10.
CFD model for the configuration with a flow-through nacelle and no sting or cavity.
Figure 10.
CFD model for the configuration with a flow-through nacelle and no sting or cavity.
Figure 11.
Fluctuations of the aerodynamic forces and moment at M = 1.2 and AoA = 20°.
Figure 11.
Fluctuations of the aerodynamic forces and moment at M = 1.2 and AoA = 20°.
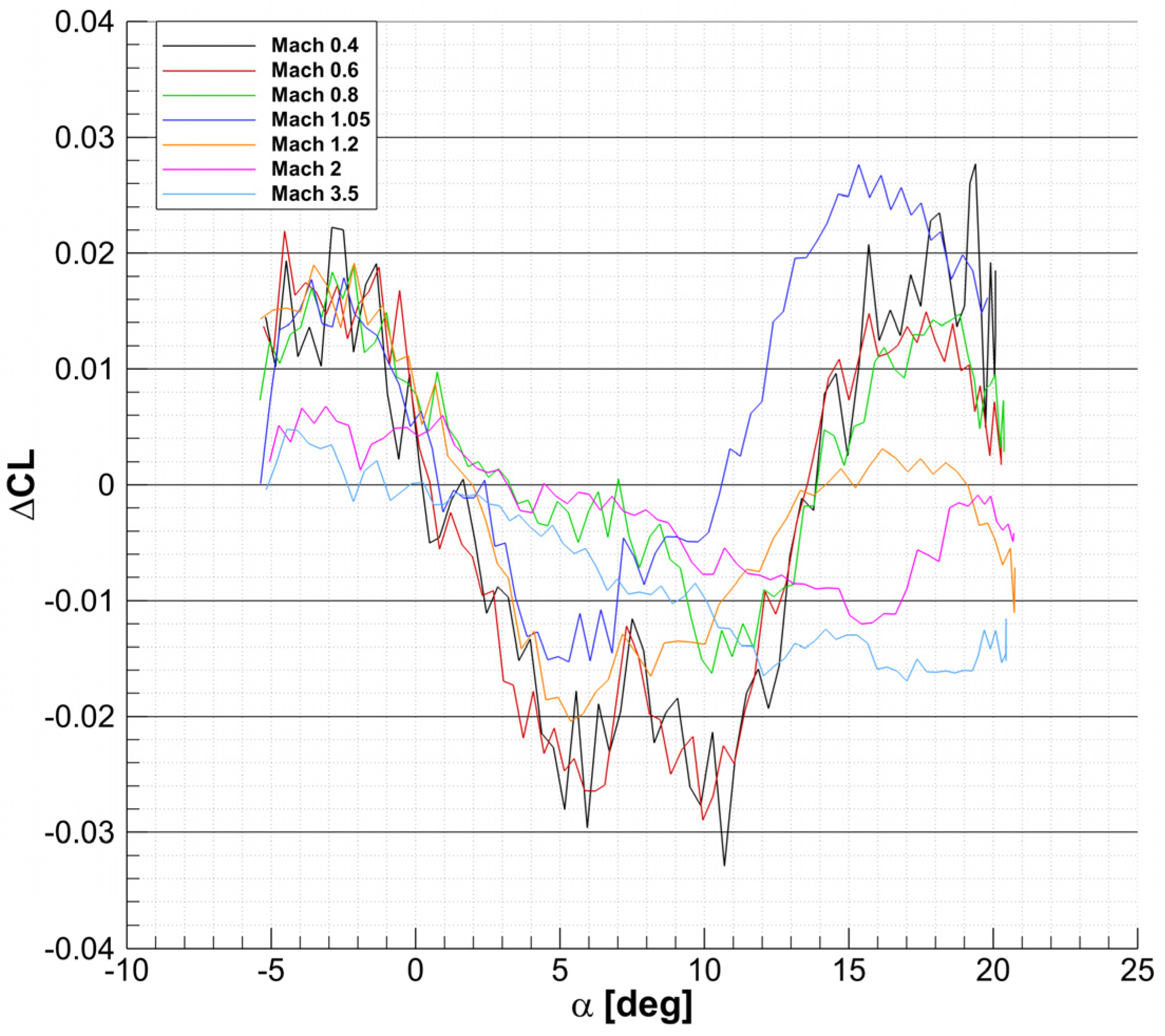
Figure 12.
Difference in lift coefficient between experimental data and CFD results for the wind tunnel configuration.
Figure 12.
Difference in lift coefficient between experimental data and CFD results for the wind tunnel configuration.
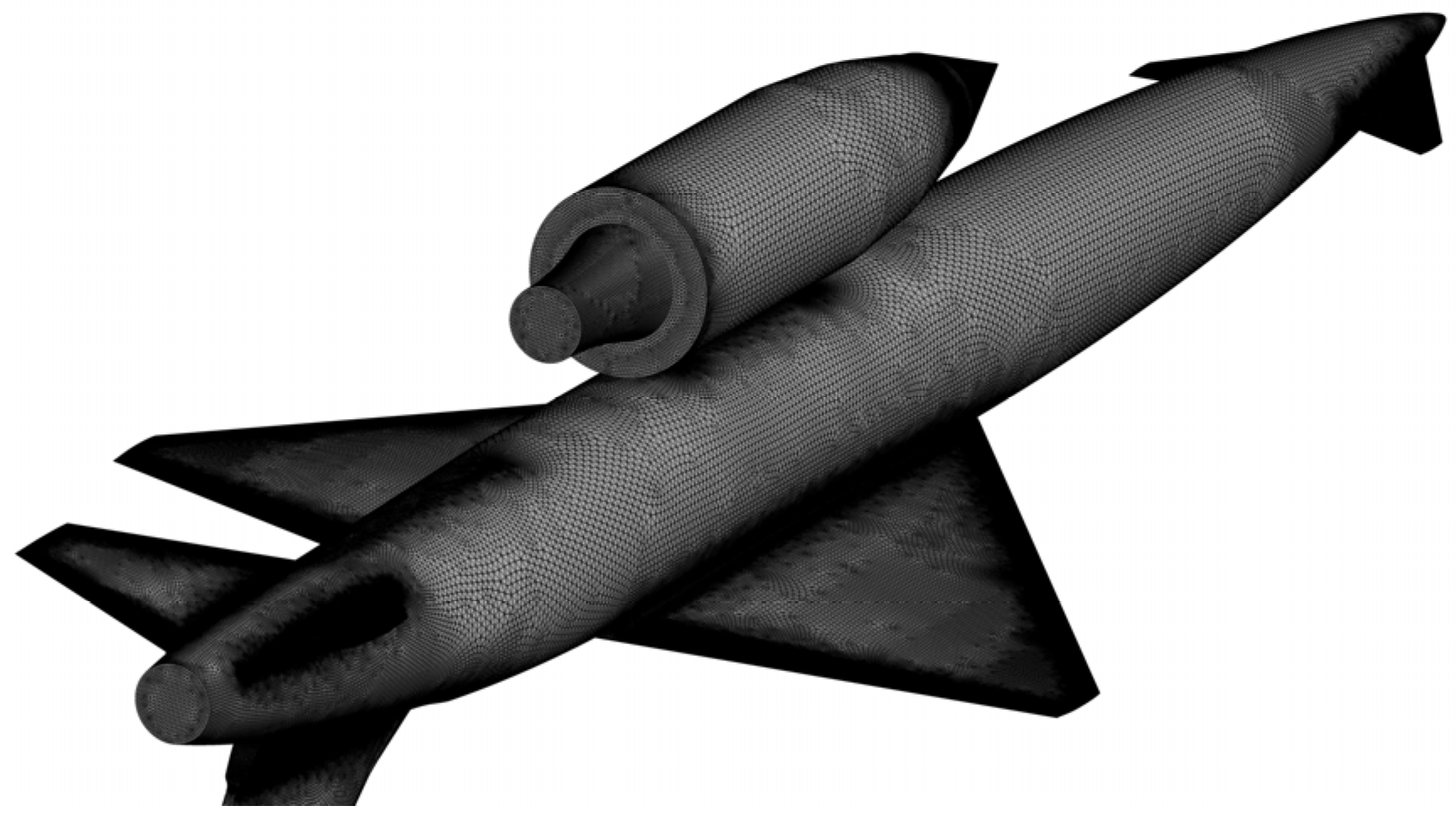
Figure 13.
Surface grid details on the wind tunnel CFD model.
Figure 13.
Surface grid details on the wind tunnel CFD model.
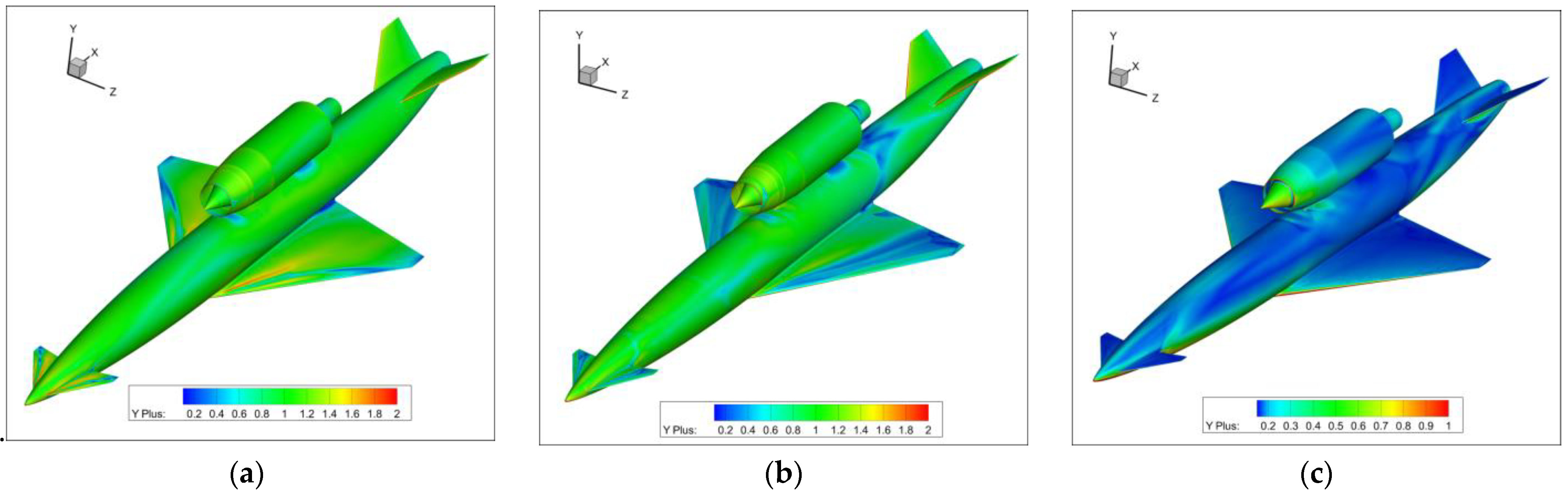
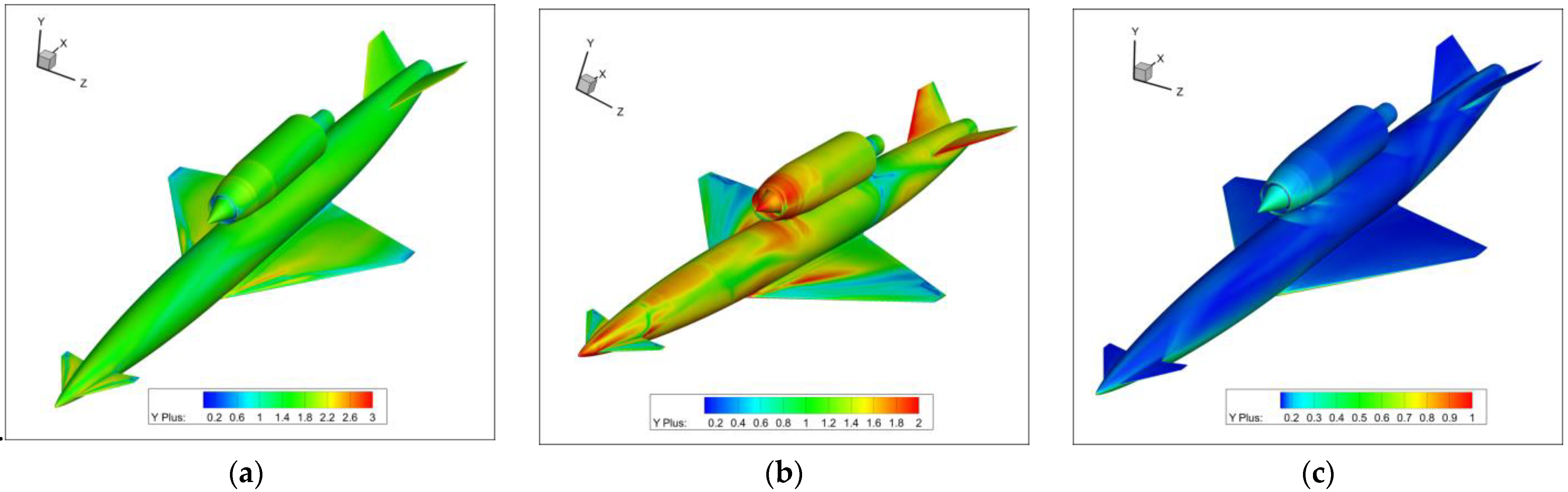
Figure 14.
distribution on the CFD wind tunnel model at Mach 0.6 (a), Mach 1.05 (b), and Mach 3.5 (c).
Figure 14.
distribution on the CFD wind tunnel model at Mach 0.6 (a), Mach 1.05 (b), and Mach 3.5 (c).
Figure 15.
distribution on the CFD wind tunnel model w/o sting and w/flow-through nacelle at Mach 0.6 (a), Mach 1.05 (b), and Mach 3.5 (c).
Figure 15.
distribution on the CFD wind tunnel model w/o sting and w/flow-through nacelle at Mach 0.6 (a), Mach 1.05 (b), and Mach 3.5 (c).
Figure 16.
distribution on the CFD model for the flight conditions at Mach 0.6 (a), Mach 1.05 (b), and Mach 3.5 (c).
Figure 16.
distribution on the CFD model for the flight conditions at Mach 0.6 (a), Mach 1.05 (b), and Mach 3.5 (c).
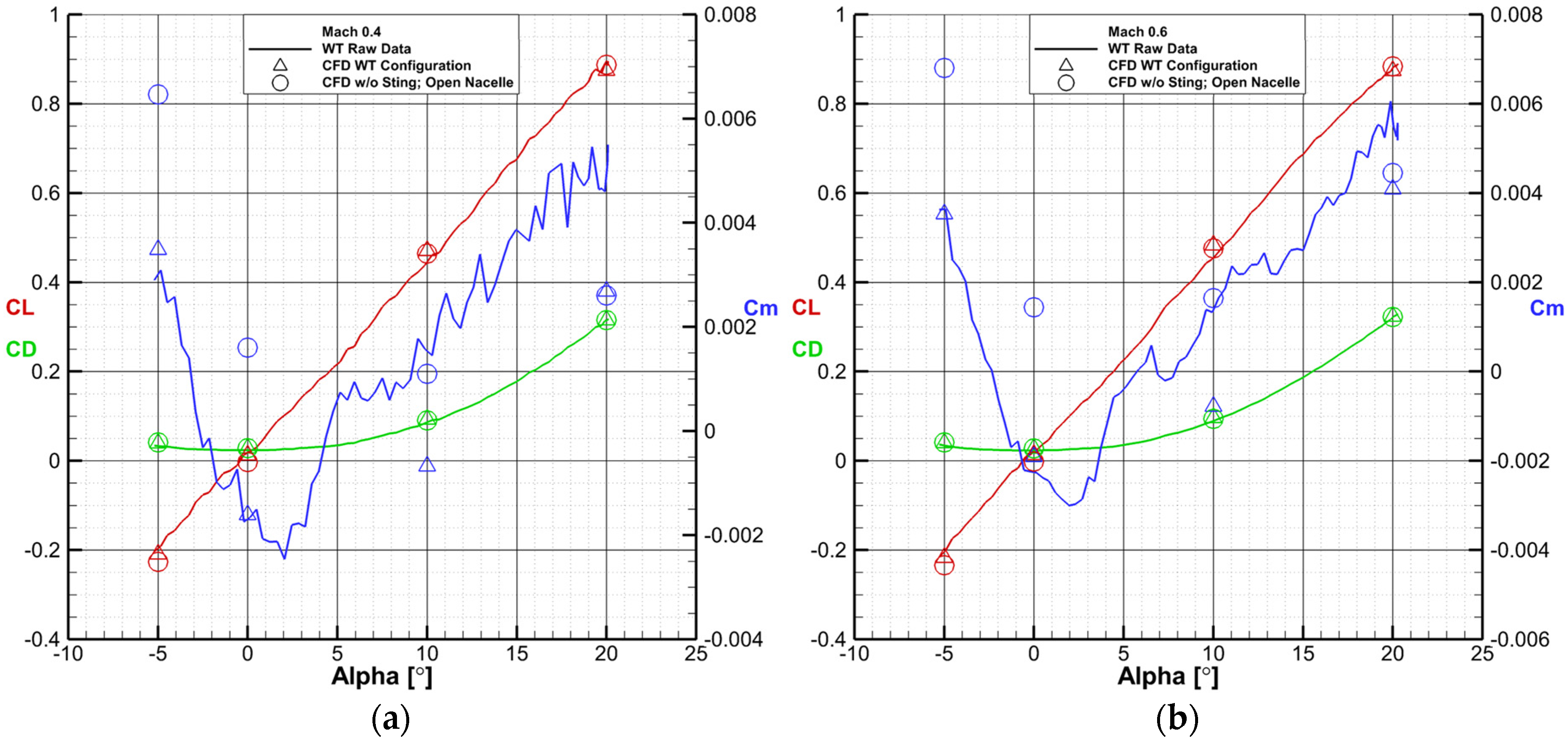
Figure 17.
Comparison between wind tunnel raw data, CFD simulations of the wind tunnel configuration, CFD simulations of the flow-through nacelle and no sting configuration at Mach 0.4 (a) and Mach 0.6 (b).
Figure 17.
Comparison between wind tunnel raw data, CFD simulations of the wind tunnel configuration, CFD simulations of the flow-through nacelle and no sting configuration at Mach 0.4 (a) and Mach 0.6 (b).
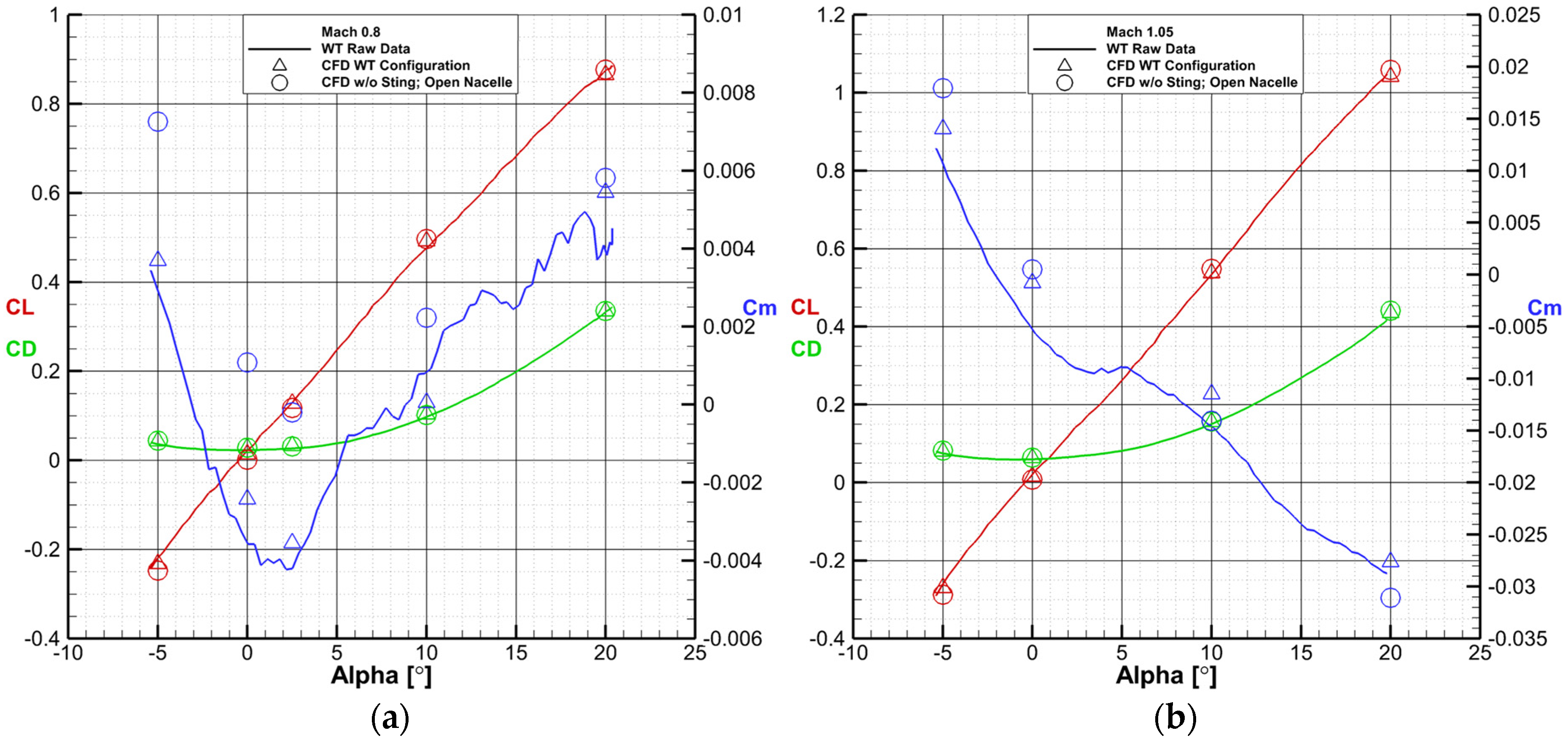
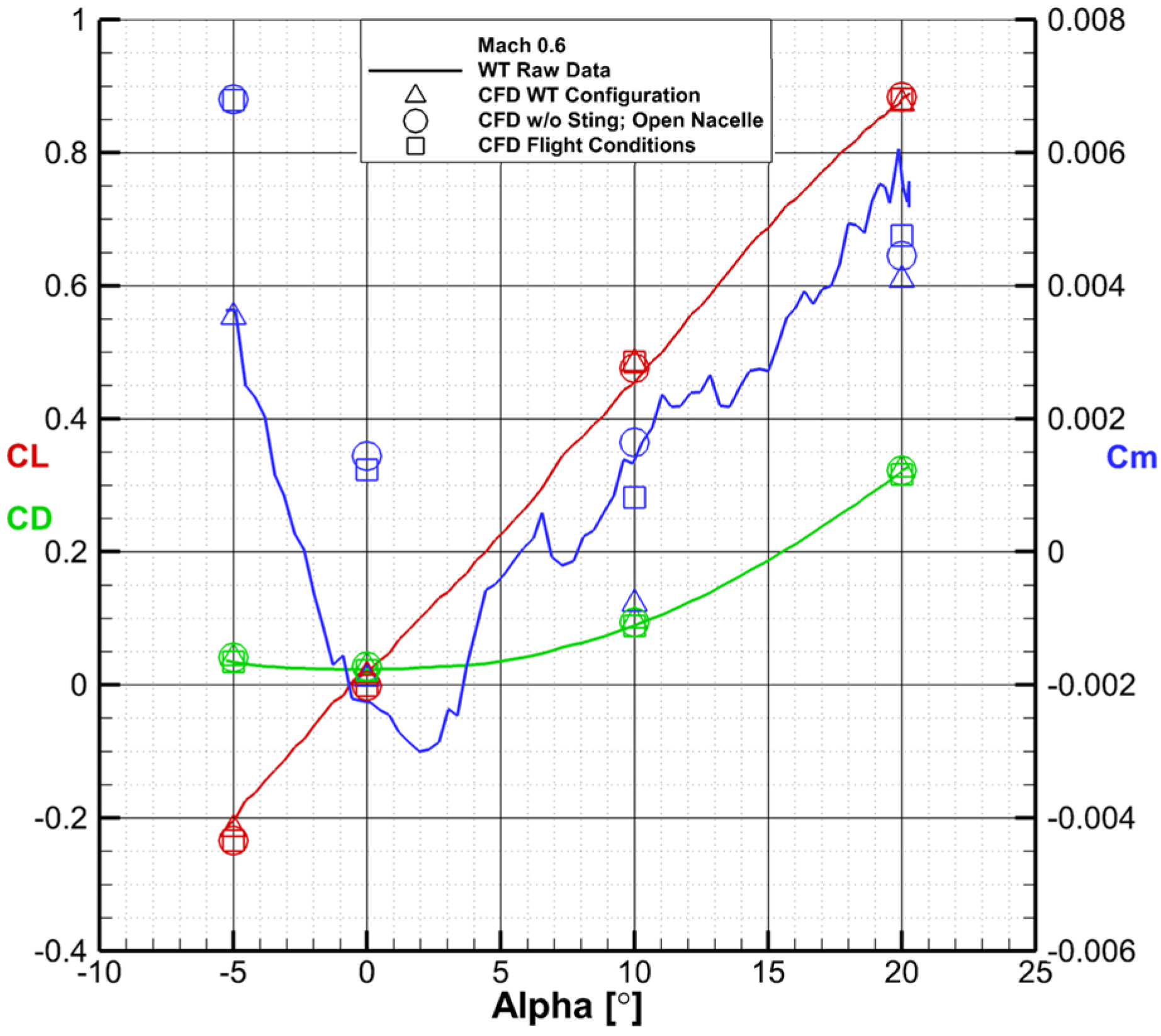
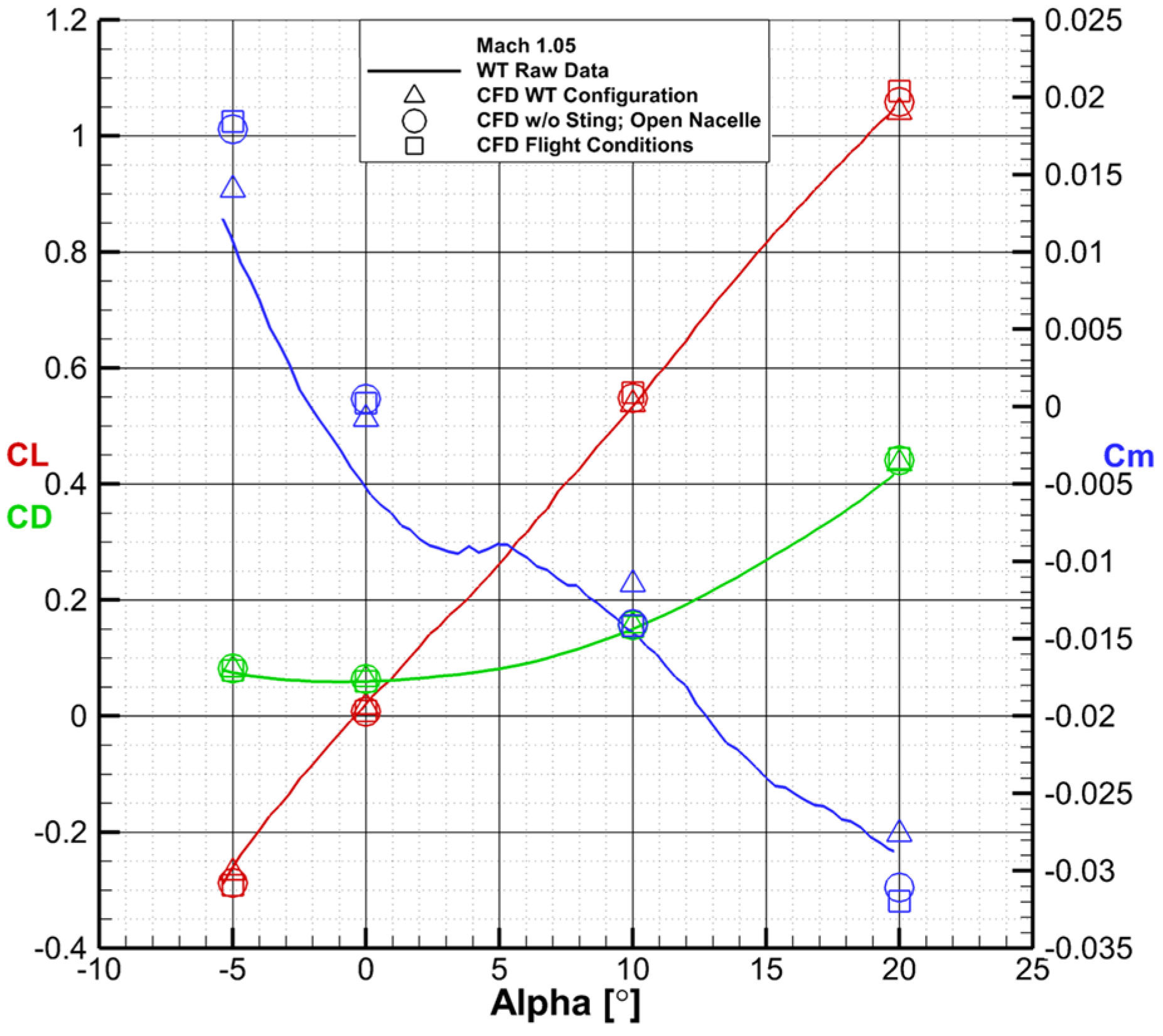
Figure 18.
Comparison between wind tunnel raw data, CFD simulations of the wind tunnel configuration, CFD simulations of the flow-through nacelle and no sting configuration at Mach 0.8 (a) and Mach 1.05 (b).
Figure 18.
Comparison between wind tunnel raw data, CFD simulations of the wind tunnel configuration, CFD simulations of the flow-through nacelle and no sting configuration at Mach 0.8 (a) and Mach 1.05 (b).
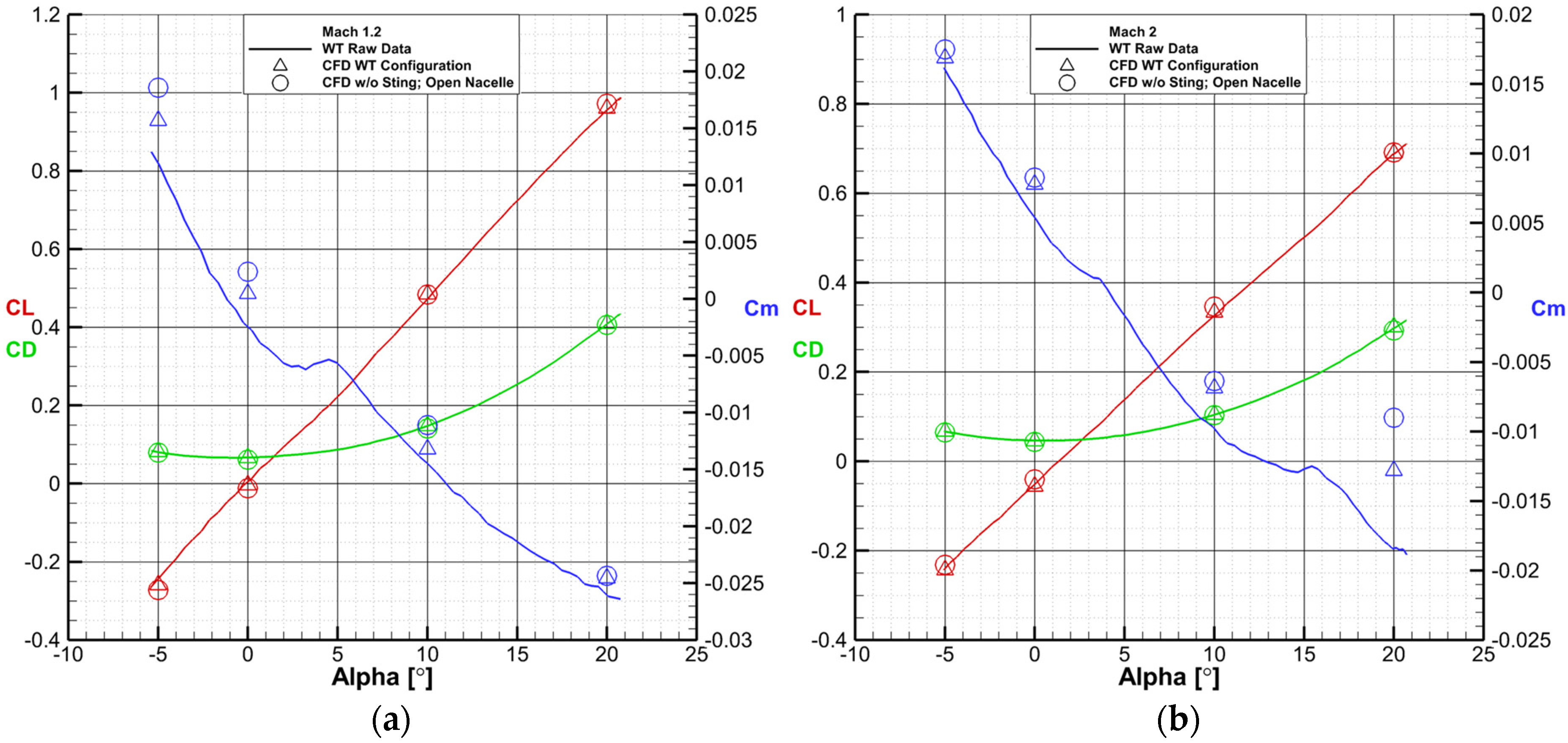
Figure 19.
Comparison between wind tunnel raw data, CFD simulations of the wind tunnel configuration, CFD simulations of the flow-through nacelle and no sting configuration at Mach 1.2 (a) and Mach 2 (b).
Figure 19.
Comparison between wind tunnel raw data, CFD simulations of the wind tunnel configuration, CFD simulations of the flow-through nacelle and no sting configuration at Mach 1.2 (a) and Mach 2 (b).
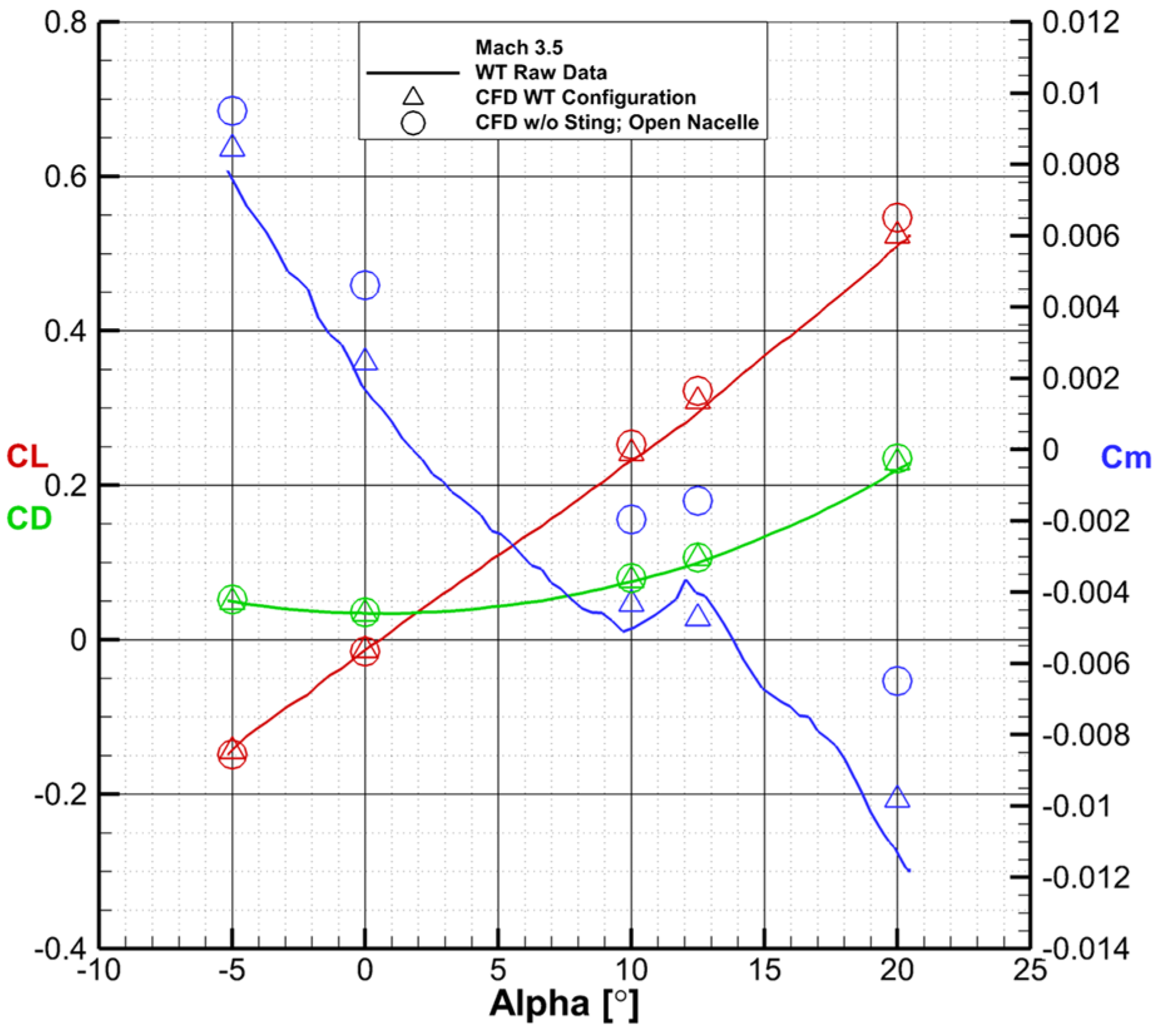
Figure 20.
Comparison between wind tunnel raw data, CFD simulations of the wind tunnel configuration, CFD simulations of the flow-through nacelle and no sting configuration at Mach 3.5.
Figure 20.
Comparison between wind tunnel raw data, CFD simulations of the wind tunnel configuration, CFD simulations of the flow-through nacelle and no sting configuration at Mach 3.5.
Figure 21.
Contours of pressure distribution differences on the model surface obtained by subtracting CFD with sting and closed nacelle from CFD without sting and flow-through nacelle.
Figure 21.
Contours of pressure distribution differences on the model surface obtained by subtracting CFD with sting and closed nacelle from CFD without sting and flow-through nacelle.
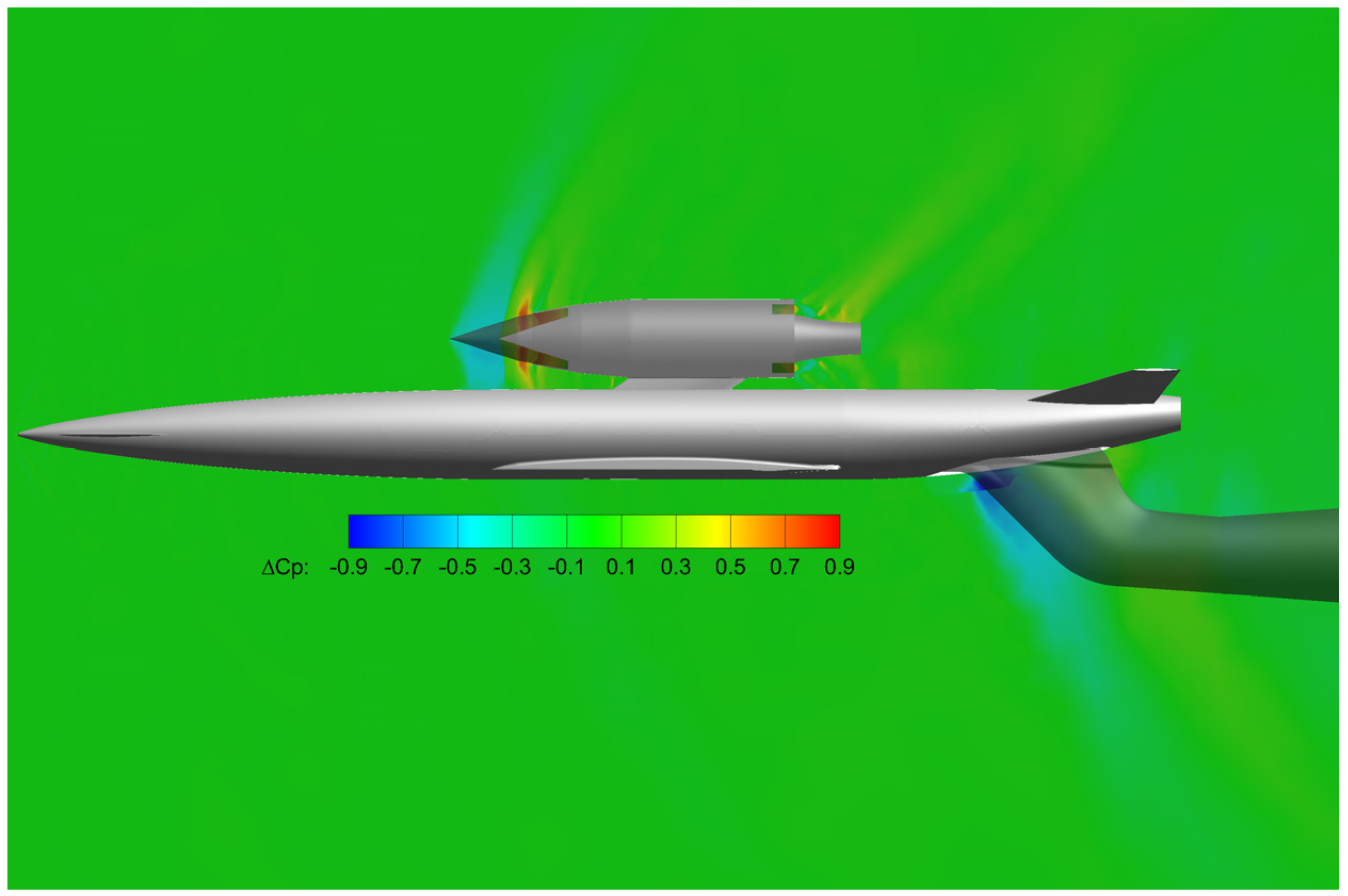
Figure 22.
Contours of pressure distribution differences on the symmetry place obtained by subtracting CFD with sting and closed nacelle from CFD without sting and flow-through nacelle.
Figure 22.
Contours of pressure distribution differences on the symmetry place obtained by subtracting CFD with sting and closed nacelle from CFD without sting and flow-through nacelle.
Figure 23.
Comparison between wind tunnel raw data, CFD simulations of the wind tunnel configuration, CFD simulations of the flow-through nacelle and no sting configuration and CFD simulations of the 1:1 scale configuration at Mach 0.6.
Figure 23.
Comparison between wind tunnel raw data, CFD simulations of the wind tunnel configuration, CFD simulations of the flow-through nacelle and no sting configuration and CFD simulations of the 1:1 scale configuration at Mach 0.6.
Figure 24.
Comparison between wind tunnel raw data, CFD simulations of the wind tunnel configuration, CFD simulations of the flow-through nacelle and no sting configuration and CFD simulations of the 1:1 scale configuration at Mach 1.05.
Figure 24.
Comparison between wind tunnel raw data, CFD simulations of the wind tunnel configuration, CFD simulations of the flow-through nacelle and no sting configuration and CFD simulations of the 1:1 scale configuration at Mach 1.05.
Figure 25.
Comparison between wind tunnel raw data, CFD simulations of the wind tunnel configuration, CFD simulations of the flow-through nacelle and no sting configuration and CFD simulations of the 1:1 scale configuration at Mach 3.5.
Figure 25.
Comparison between wind tunnel raw data, CFD simulations of the wind tunnel configuration, CFD simulations of the flow-through nacelle and no sting configuration and CFD simulations of the 1:1 scale configuration at Mach 3.5.
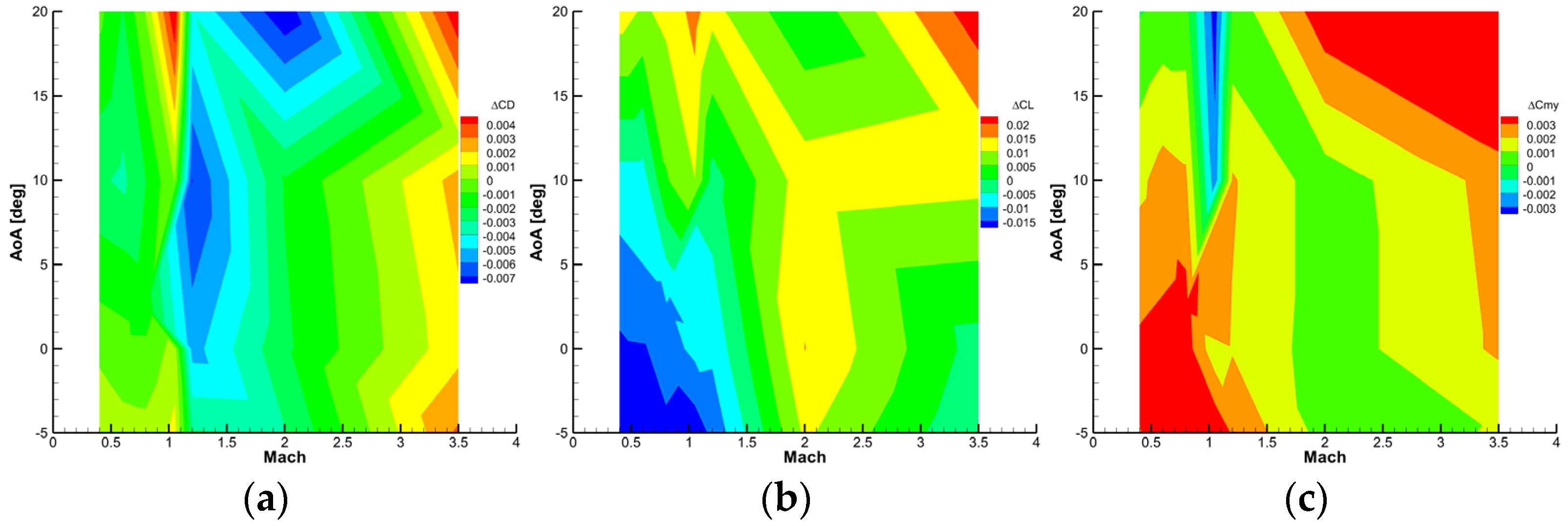
Figure 26.
Sting and open nacelle effect on drag (a), lift (b), pitching moment, (c)—CFD results.
Figure 26.
Sting and open nacelle effect on drag (a), lift (b), pitching moment, (c)—CFD results.
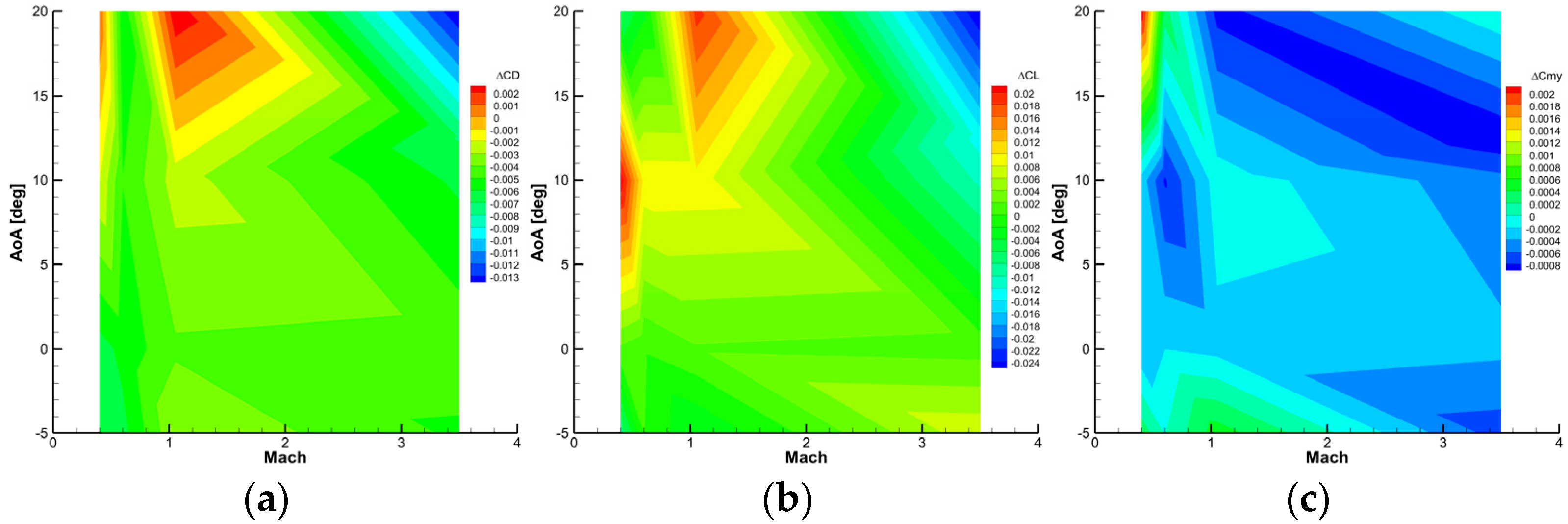
Figure 27.
Reynolds effect on drag (a), lift (b), pitching moment, (c)—CFD results.
Figure 27.
Reynolds effect on drag (a), lift (b), pitching moment, (c)—CFD results.
Table 1.
Geometrical characteristics for the full-size vehicle and the wind tunnel model.
Table 1.
Geometrical characteristics for the full-size vehicle and the wind tunnel model.
| CS-2 | Full Scale | Scale 1:28 |
|---|
| Length [m] | 24.53 | 0.876 |
| Width [m] | 8.88 | 0.317 |
| 40.77 | 0.052 |
| Reference point | - | 0.438 [50% model length] |
Table 2.
Experimental test matrix for AoS = 0°.
Table 2.
Experimental test matrix for AoS = 0°.
| M | |
|---|
| 0.4 | 11.4 |
| 0.6 | 15.7 |
| 0.8 | 18.7 |
| 0.95 | 20.1 |
| 1.05 | 20.7 |
| 1.1 | 20.8 |
| 1.2 | 21.0 |
| 1.4 | 20.7 |
| 1.6 | 19.8 |
| 2.0 | 18.2 |
| 2.5 | 25.2 |
| 3.5 | 32.7 |
Table 3.
Reference trajectory of the vehicle.
Table 3.
Reference trajectory of the vehicle.
| M | |
|---|
| 0.6 | 315.0 |
| 1.05 | 403.6 |
| 3.5 | 169.2 |
Table 4.
Characteristics of the coarse, medium, and fine grids for the wind tunnel configuration.
Table 4.
Characteristics of the coarse, medium, and fine grids for the wind tunnel configuration.
| | First Cell Height [mm] | Growth Ratio in the Prism Layers | Number of Prism Layers | |
|---|
| Coarse | 0.004 | 1.25 | 31 | 25.9 |
| Medium | 0.003 | 1.2 | 38 | 40.2 |
| Fine | 0.002 | 1.15 | 50 | 64.8 |
Table 5.
Grid independence study results for the wind tunnel configuration.
Table 5.
Grid independence study results for the wind tunnel configuration.
| | Grid | AoA | | | |
|---|
| | Coarse | 20° | 0.33912 | 0.8719 | 0.006385 |
| M 0.6 | Medium | 0.32424 | 0.87456 | 0.004092 |
| | Fine | 0.32547 | 0.87682 | 0.004161 |
| | Coarse | 0.43863 | 1.04754 | −0.02899 |
| M 1.05 | Medium | 0.43622 | 1.04176 | −0.02761 |
| | Fine | 0.43649 | 1.04349 | −0.02805 |
| | Coarse | 0.31464 | 0.68459 | −0.01321 |
| M 2 | Medium | 0.3009 | 0.69031 | −0.0128 |
| | Fine | 0.30146 | 0.69201 | −0.01293 |
Table 6.
Characteristics of the coarse, medium, and fine grids for full-size configuration.
Table 6.
Characteristics of the coarse, medium, and fine grids for full-size configuration.
| | First Cell Height [mm] | Growth Ratio in the Prism Layers | Number of Prism Layers | |
|---|
| Coarse | 0.01 | 1.25 | 42 | 34.6 |
| Medium | 0.008 | 1.2 | 52 | 51.0 |
| Fine | 0.006 | 1.15 | 68 | 82.5 |
Table 7.
Grid independence study results for the full-size configuration.
Table 7.
Grid independence study results for the full-size configuration.
| | Grid | AoA | | | |
|---|
| | Coarse | 20° | 0.444138 | 1.07688 | −0.03165 |
| M 1.05 | Medium | 0.43995 | 1.0775 | −0.03195 |
| | Fine | 0.444445 | 1.0784 | −0.03199 |
Table 8.
Grid characteristics for the three simulation cases.
Table 8.
Grid characteristics for the three simulation cases.
| Configuration | First Cell Height [mm] | Growth Ratio in the Prism Layers | Number of Prism Layers | Number of Elements [Million] |
|---|
| WT model | 0.003 | 1.2 | 38 | 40.2 |
| WT model w/o sting and open nacelle | 0.003 | 1.2 | 38 | 36.8 |
| Flight conditions model | 0.008 | 1.2 | 52 | 51 |
Table 9.
Maximum errors between experimental data and CFD.
Table 9.
Maximum errors between experimental data and CFD.
| | | | |
|---|
| Difference [counts] | 141 | 33 | 56 |
| Mach | 1.05 | 0.4 | 1.05 |
| AoA | 20° | ~10.7° | ~2.4° |
Table 10.
Maximum differences on lift, drag, and pitching moment caused by the open nacelle and sting.
Table 10.
Maximum differences on lift, drag, and pitching moment caused by the open nacelle and sting.
| | | | |
|---|
| Difference [counts] | 79 | 23 | 39 |
| Mach | 2 | 3.5 | 1.05 |
| AoA | 20° | 20° | −5° |
Table 11.
Maximum differences on lift, drag, and pitching moment caused by the Reynolds number.
Table 11.
Maximum differences on lift, drag, and pitching moment caused by the Reynolds number.
| | | | |
|---|
| Difference [counts] | 139 | 26 | 9 |
| Mach | 3.5 | 3.5 | 3.5 |
| AoA | 20° | 20° | 12.5° |