Experimental Pressure Gain Analysis of Pulsed Detonation Engine
Abstract
1. Introduction
2. Experimental Approach
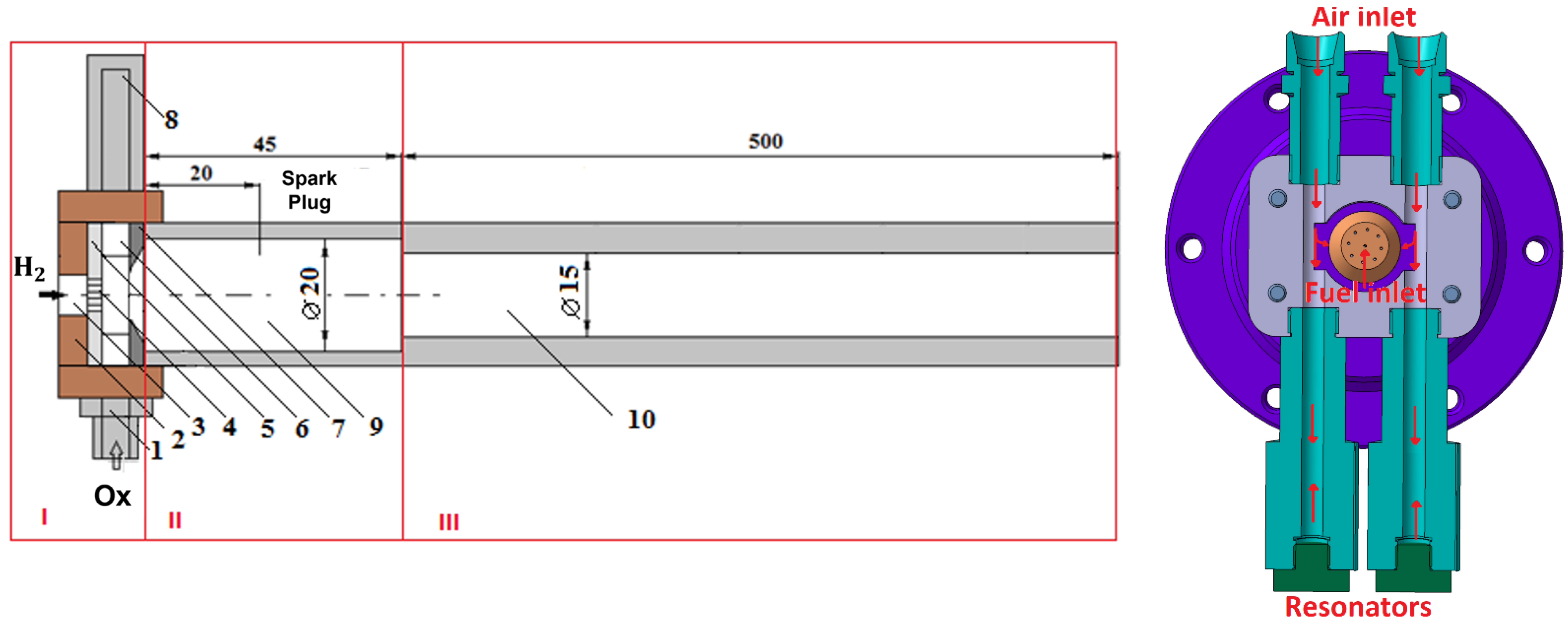
2.1. Experimental Model
2.2. Experimental Setup
2.3. Instrumentation and Test Matrix
- 0.
- Exhaust pipe length—L;
- 1.
- Spark plug operating frequency—;
- 2.
- Fuel feed line pressure—;
- 3.
- Oxidizer feed line pressure—.
2.4. Post-Processing Algorithm
3. Results and Discussion
3.1. Hydrogen/Air Mixture
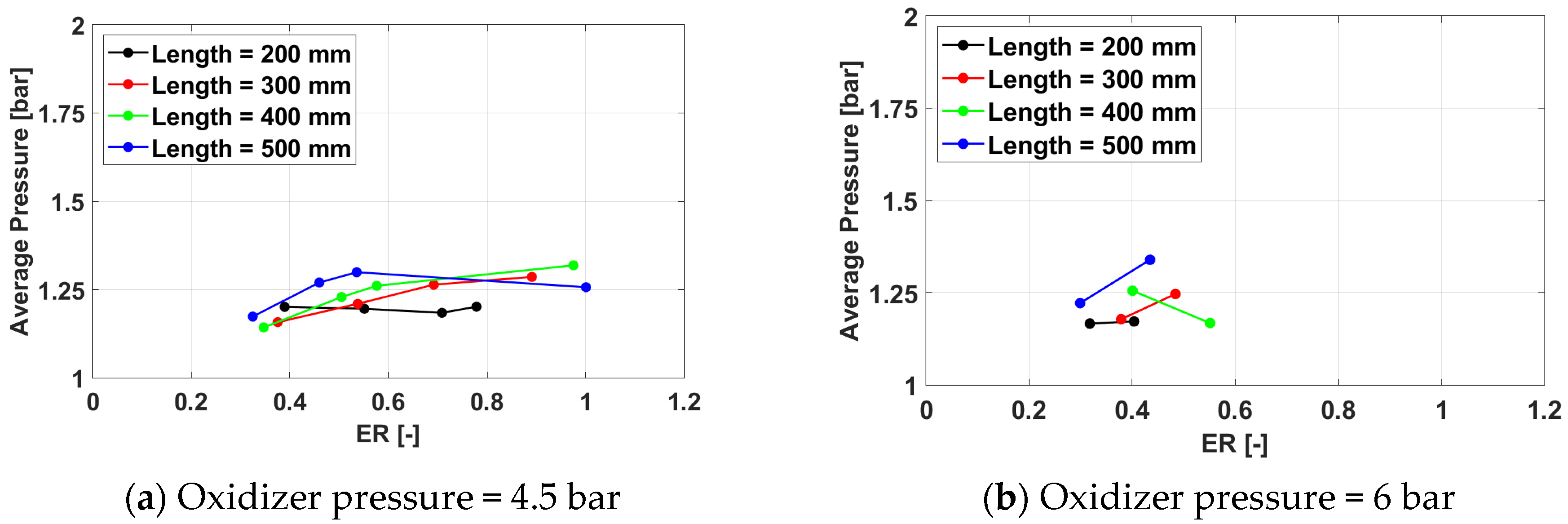
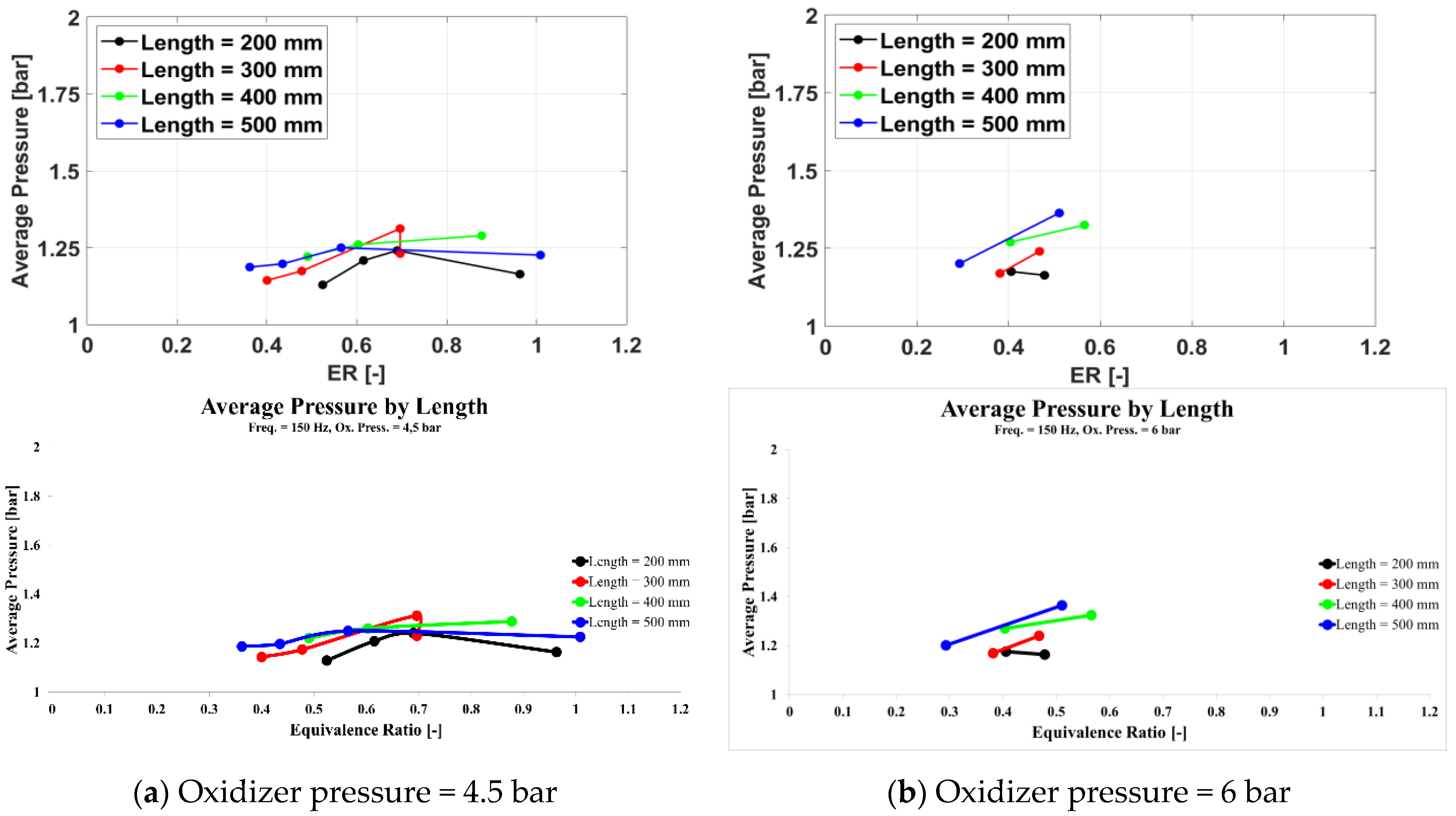
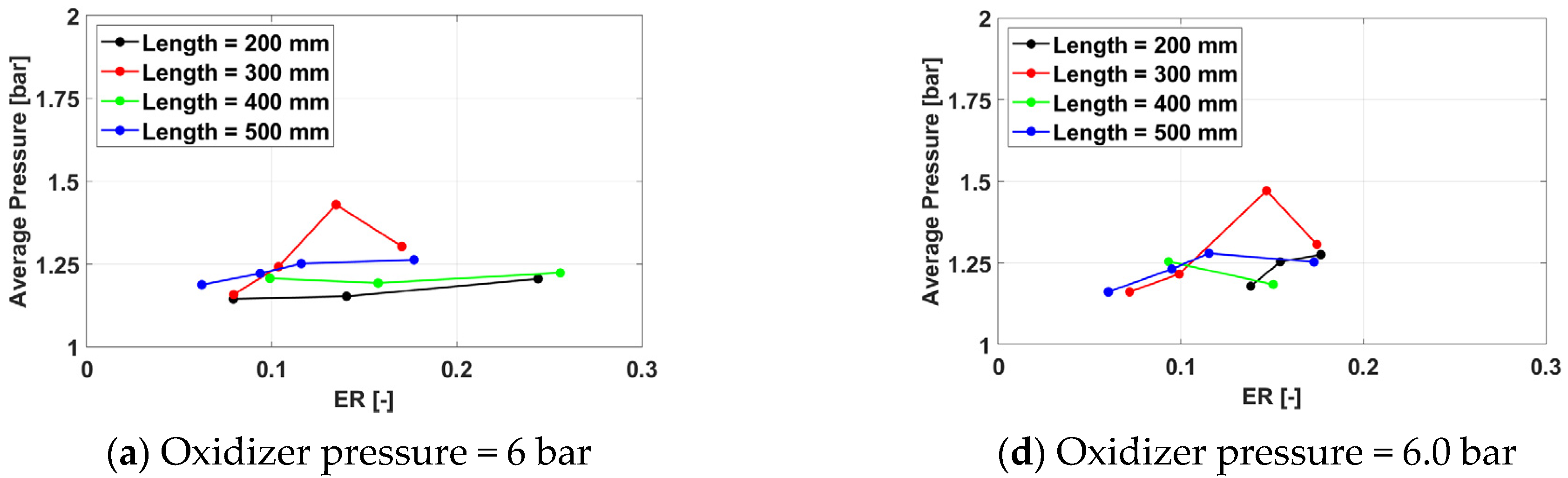
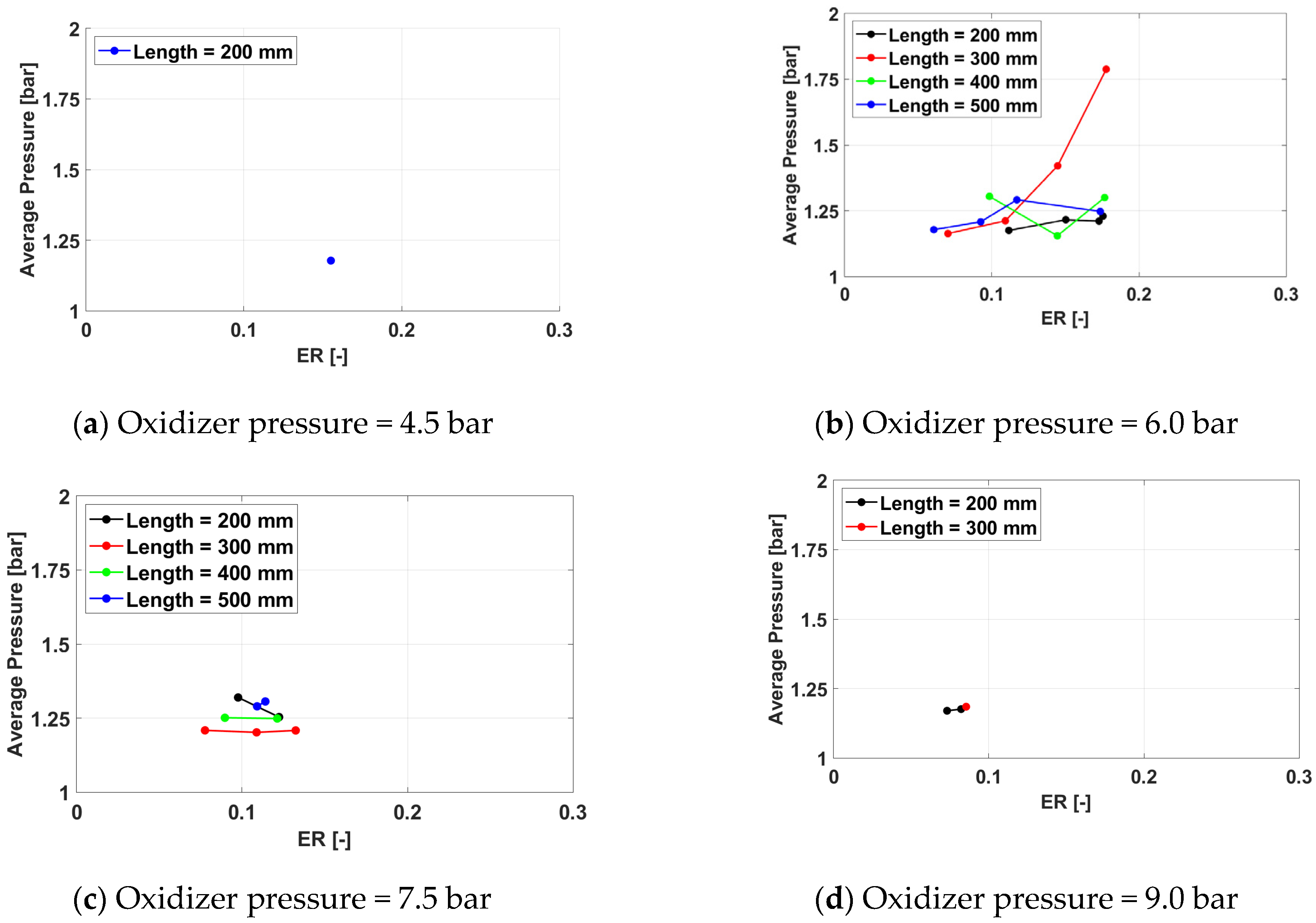
3.1.1. Average Cycle Pressure
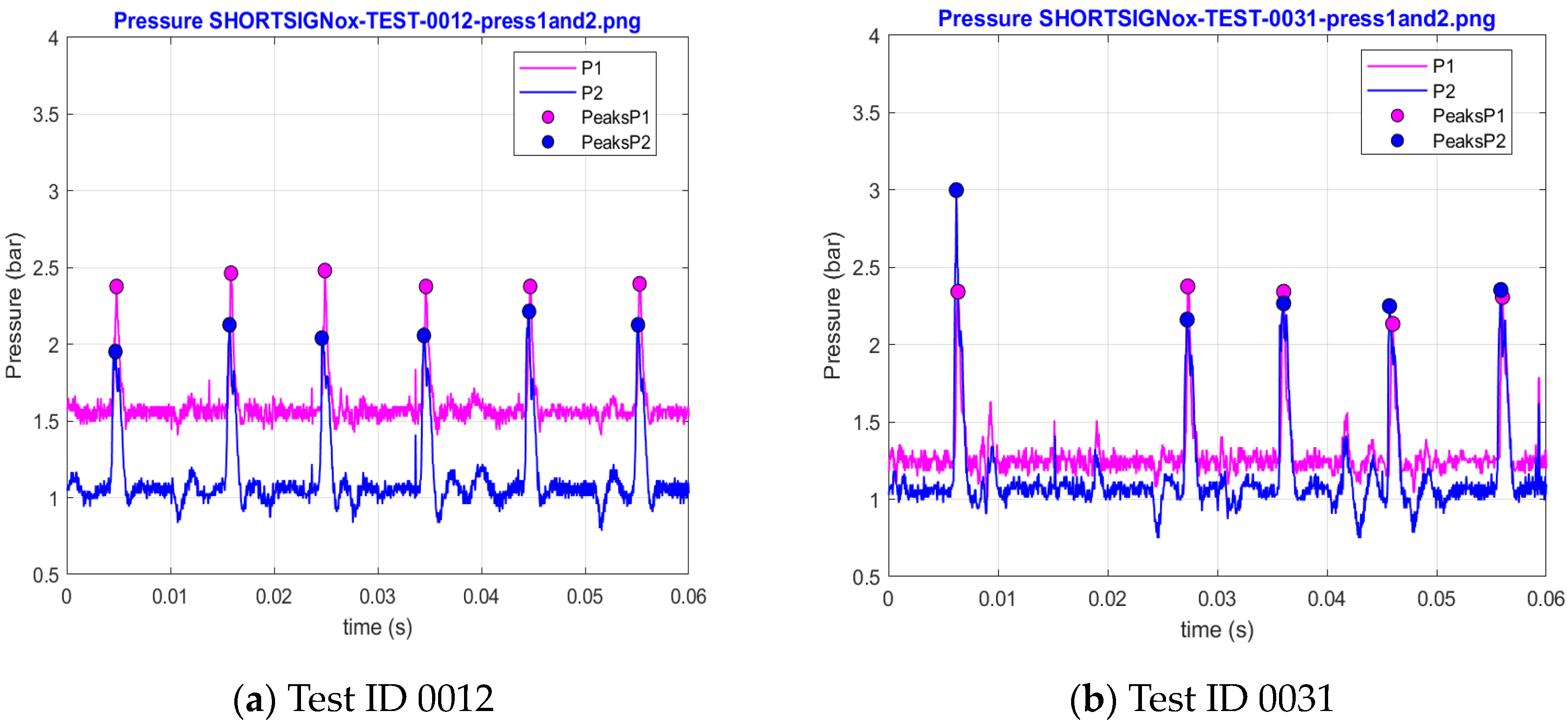
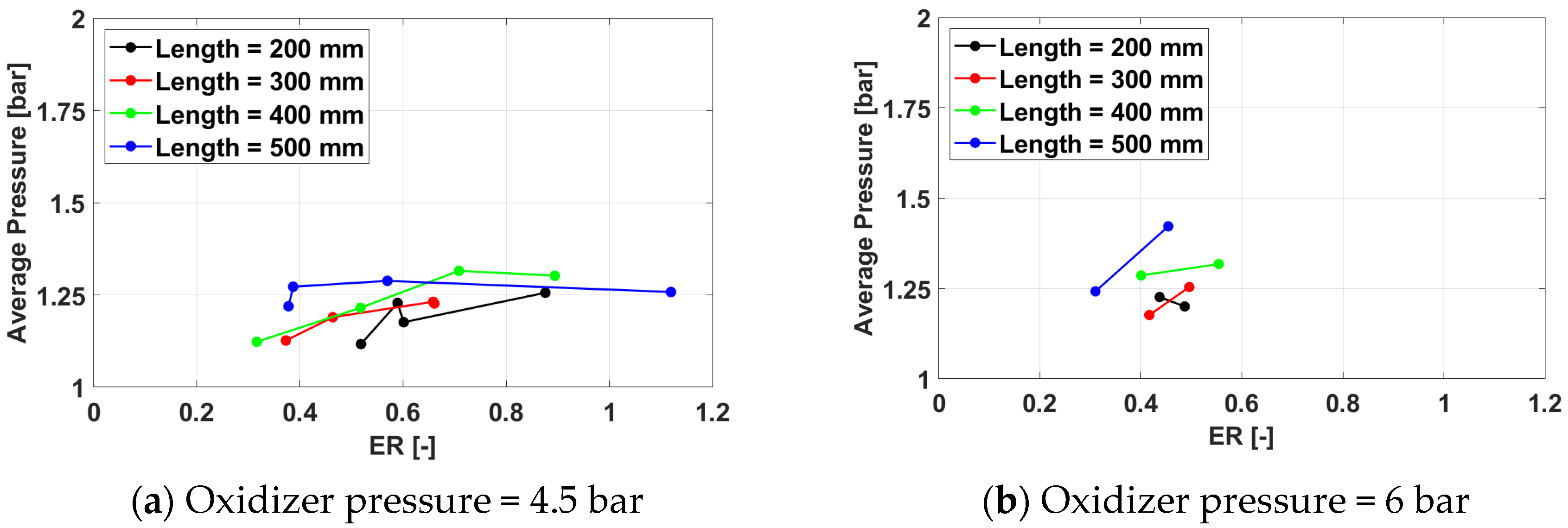
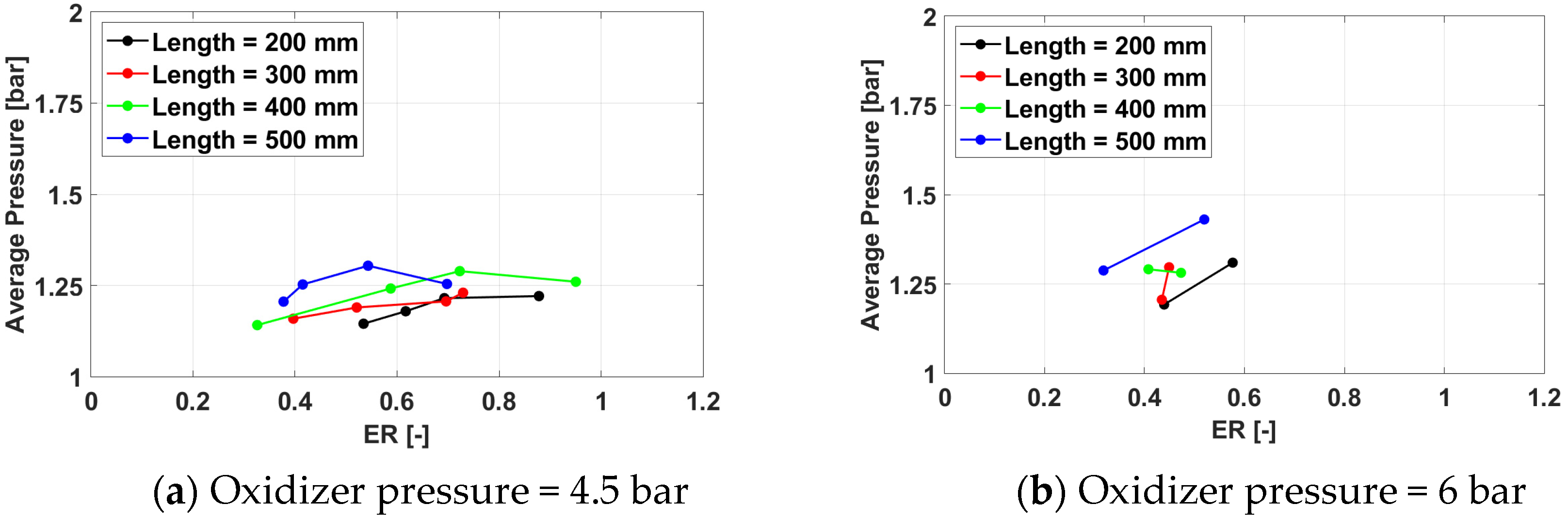
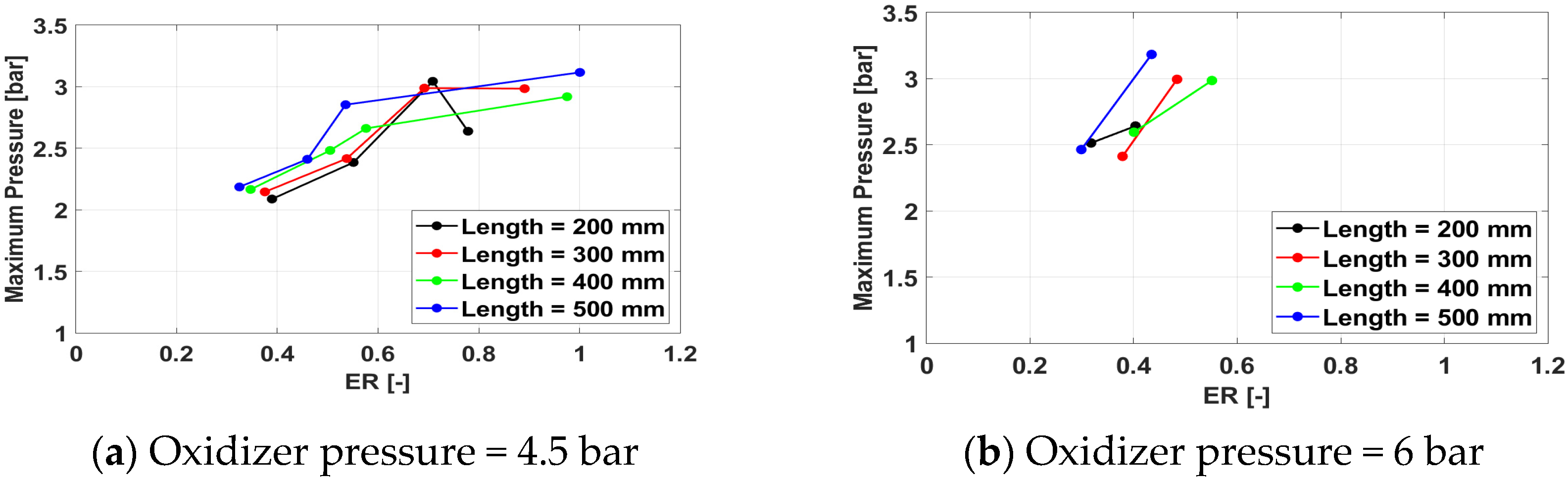
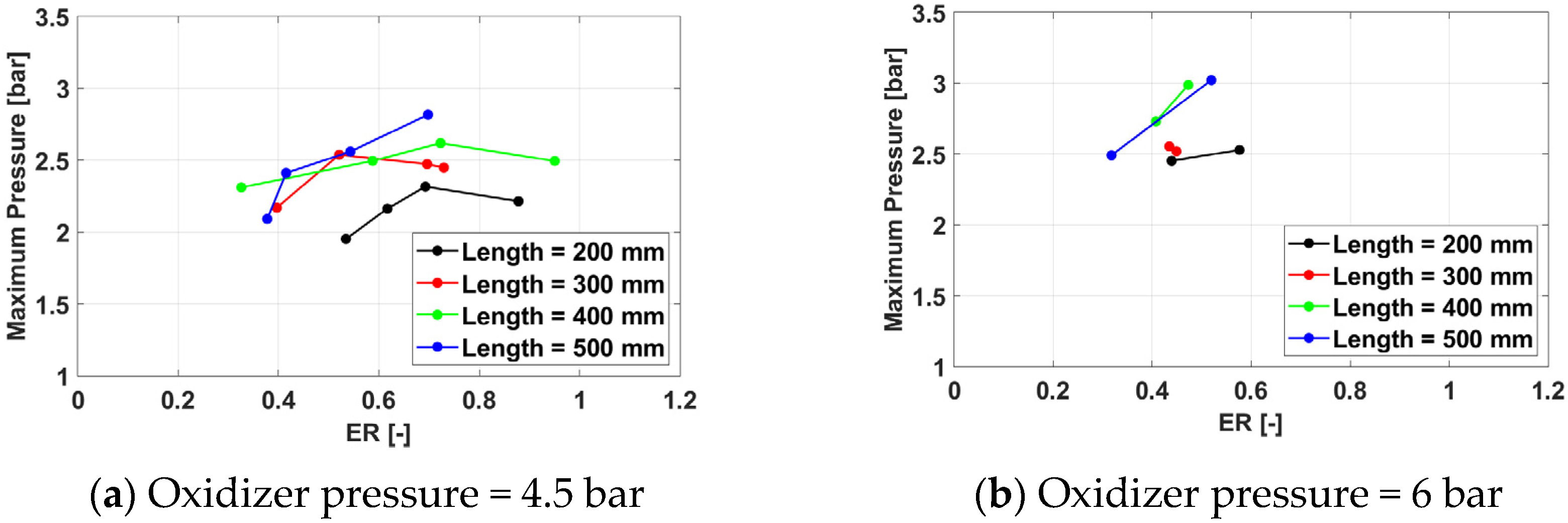
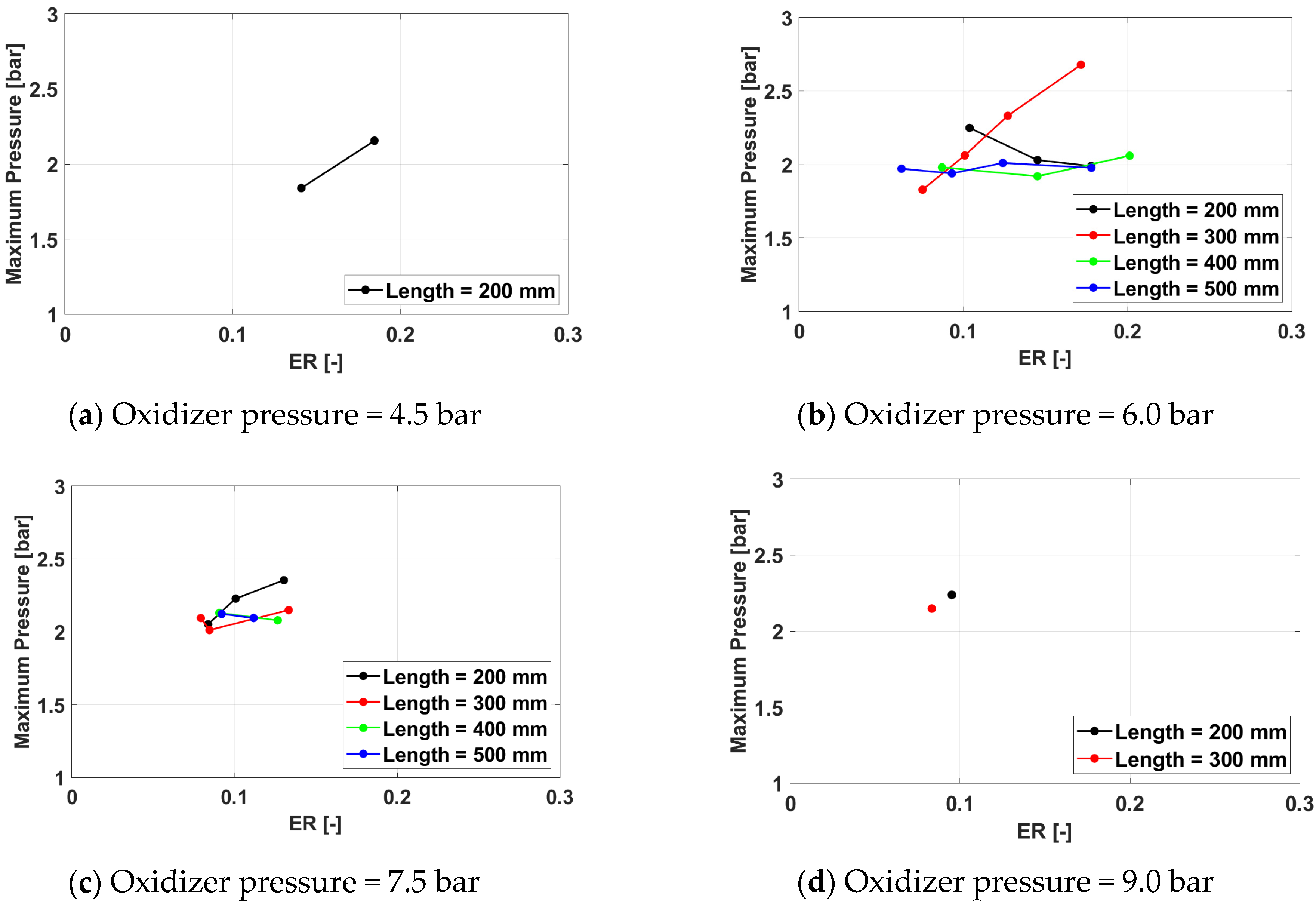
3.1.2. Maximum Cycle Pressure
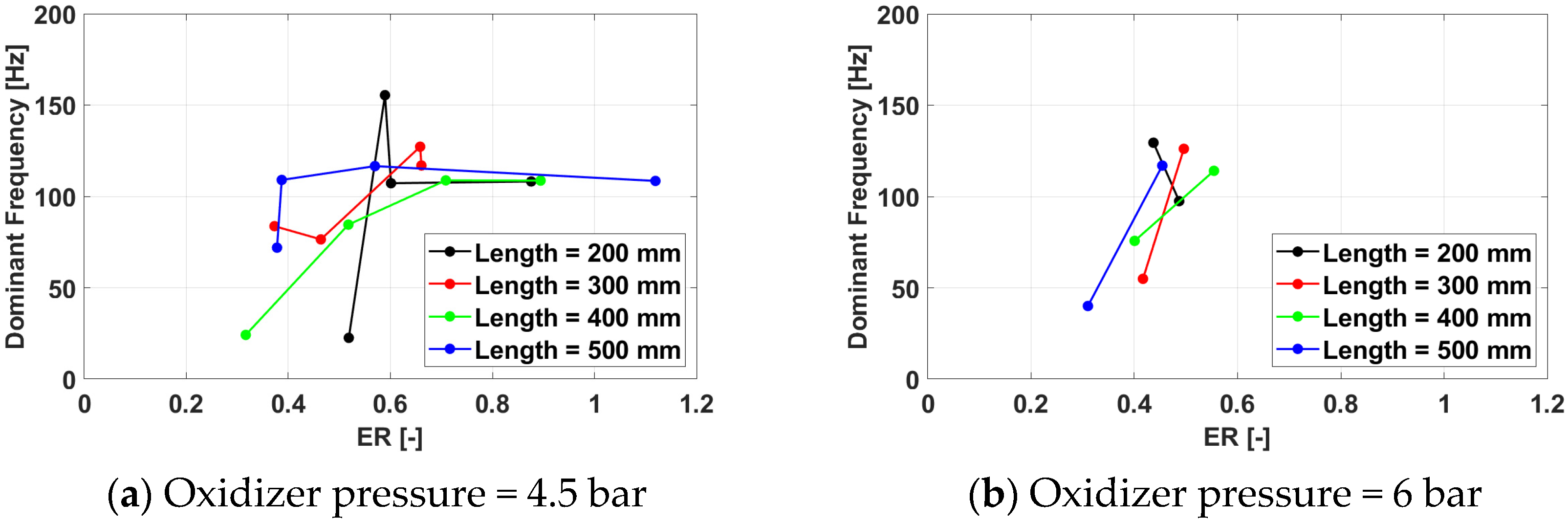
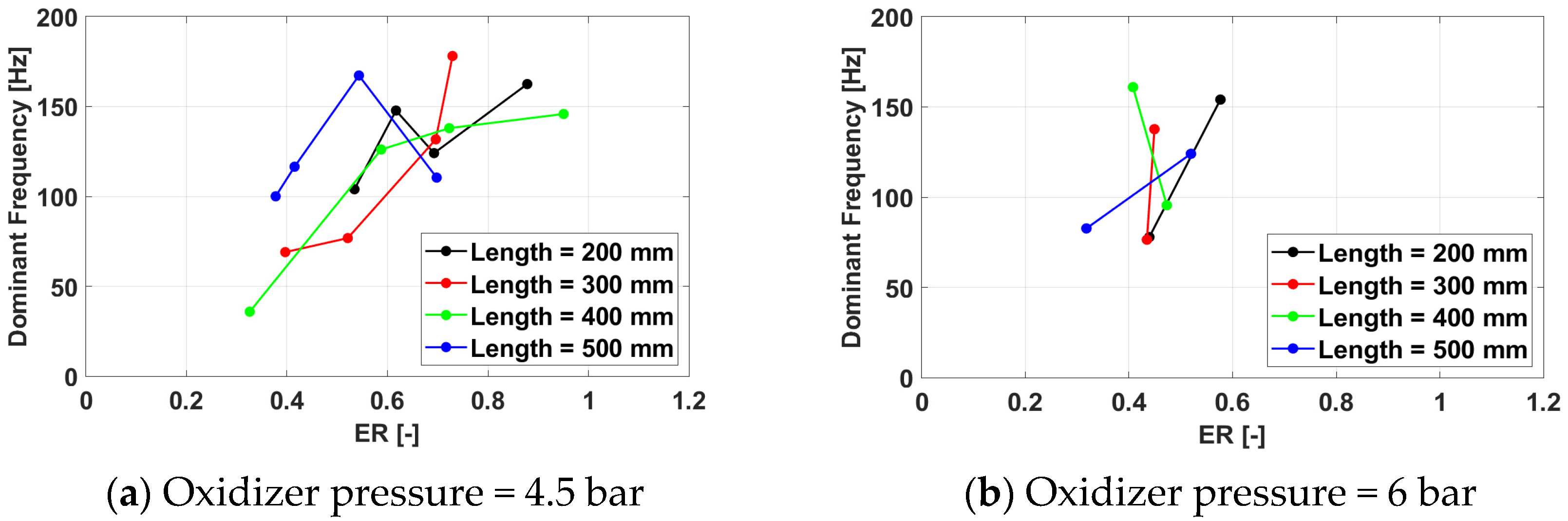
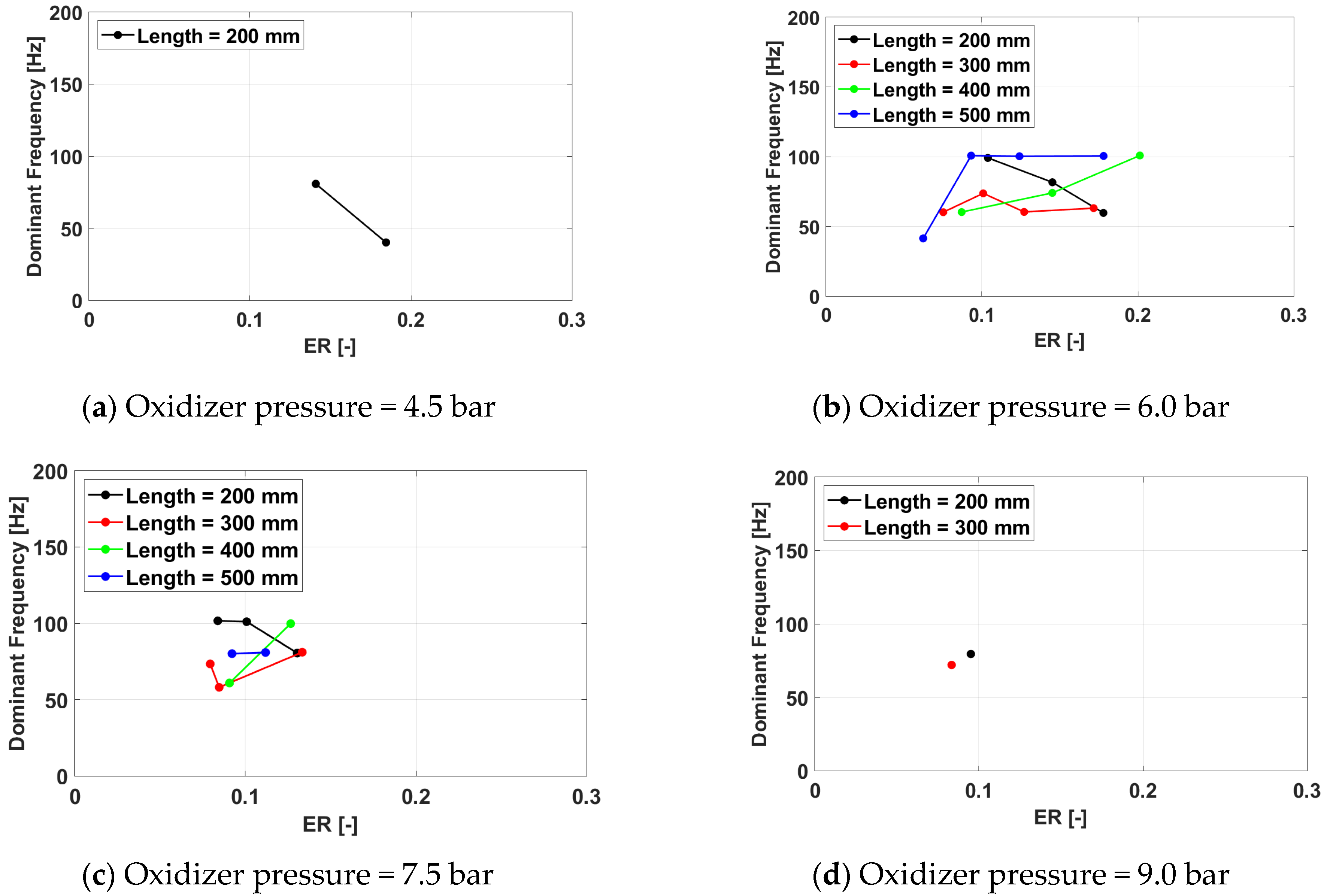
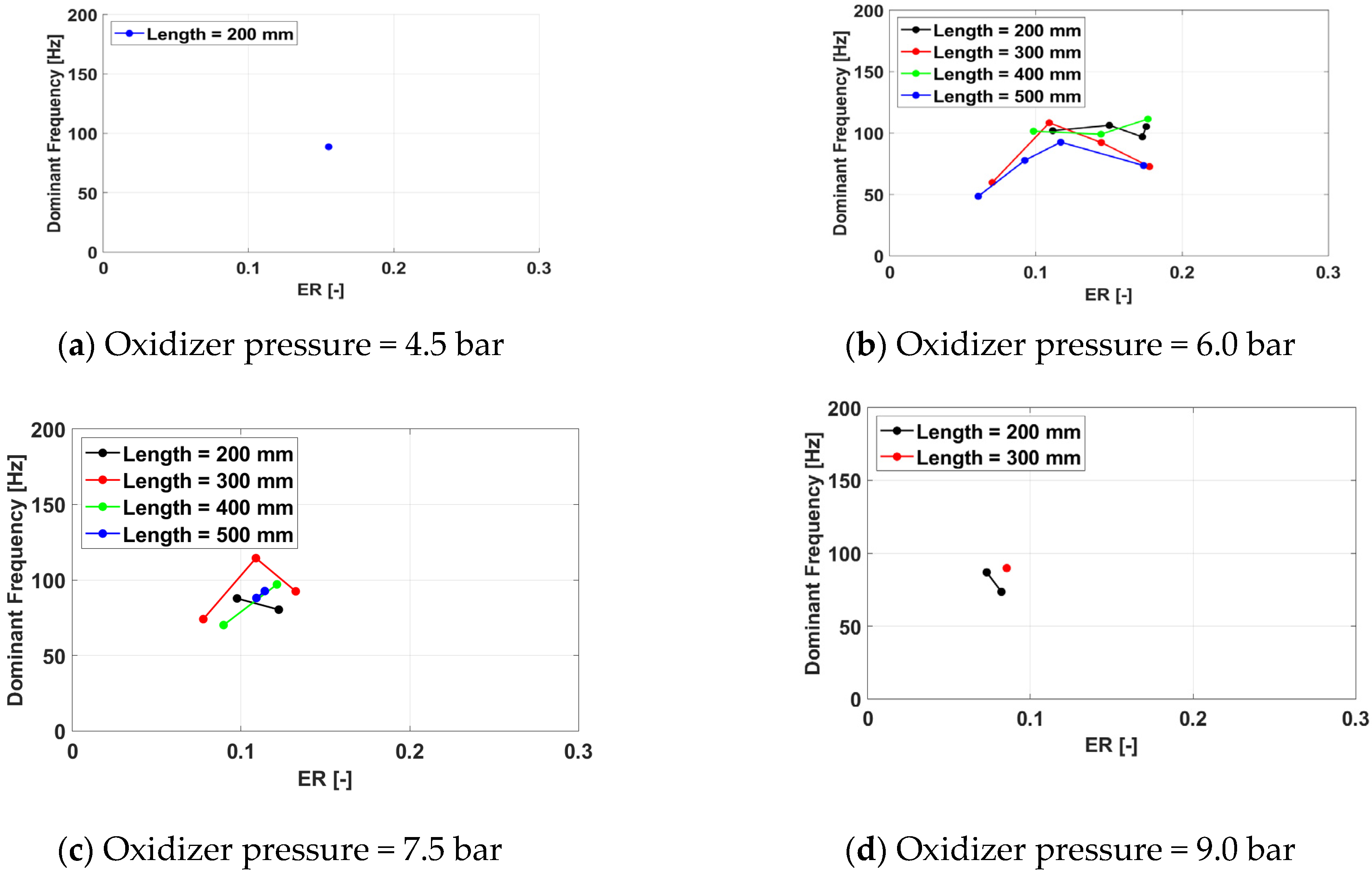
3.1.3. Operating PDC Frequency
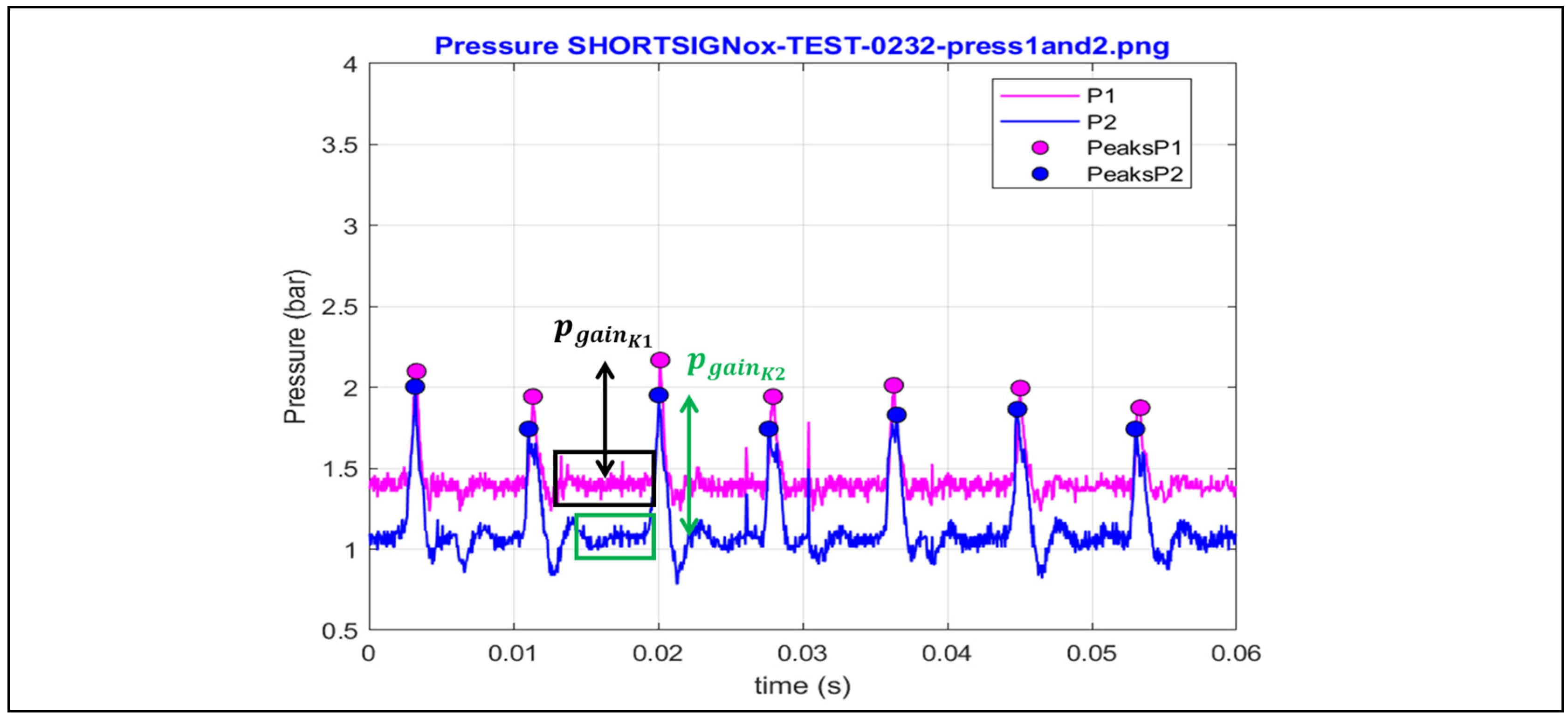
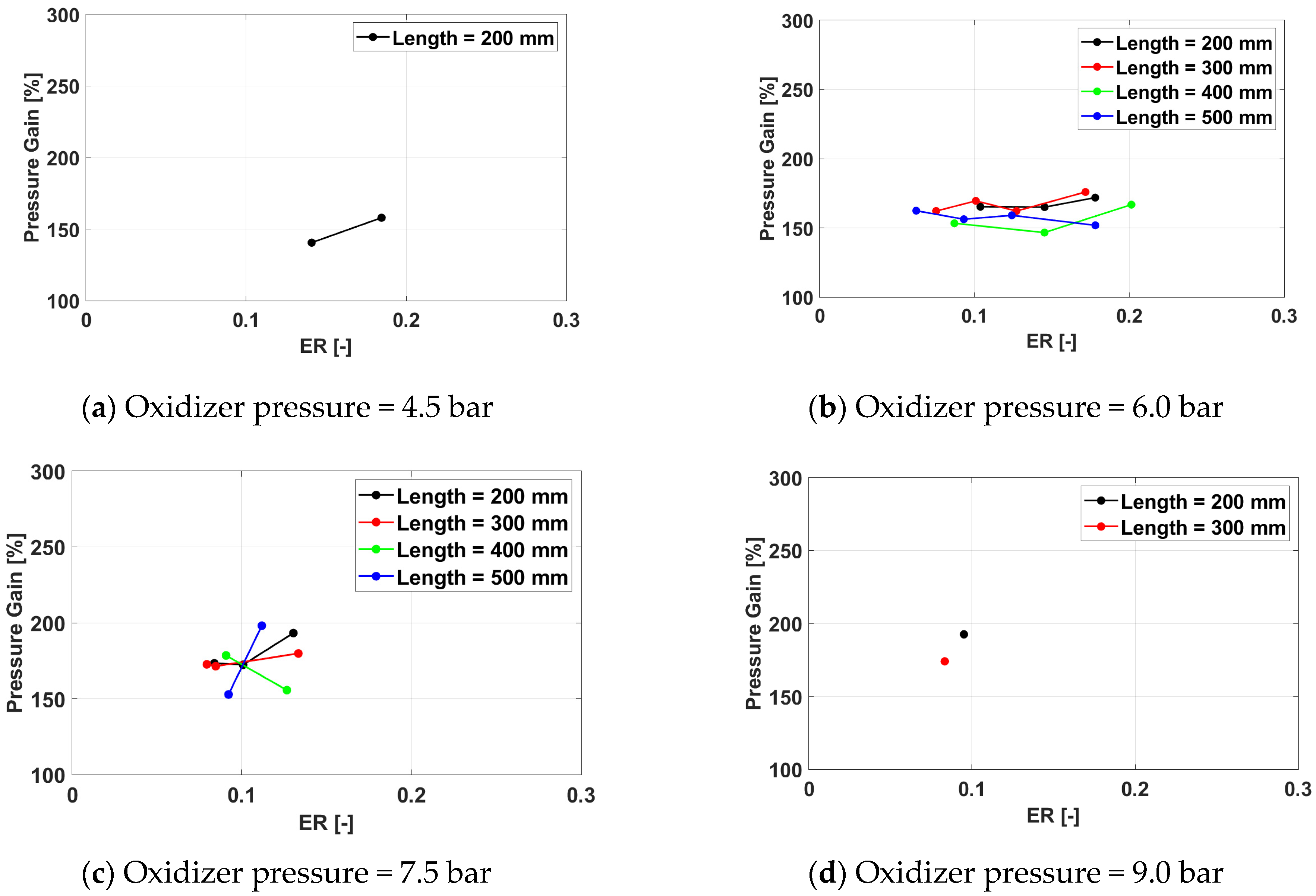
3.1.4. Pressure Gain
3.2. Hydrogen/Oxygen Operations
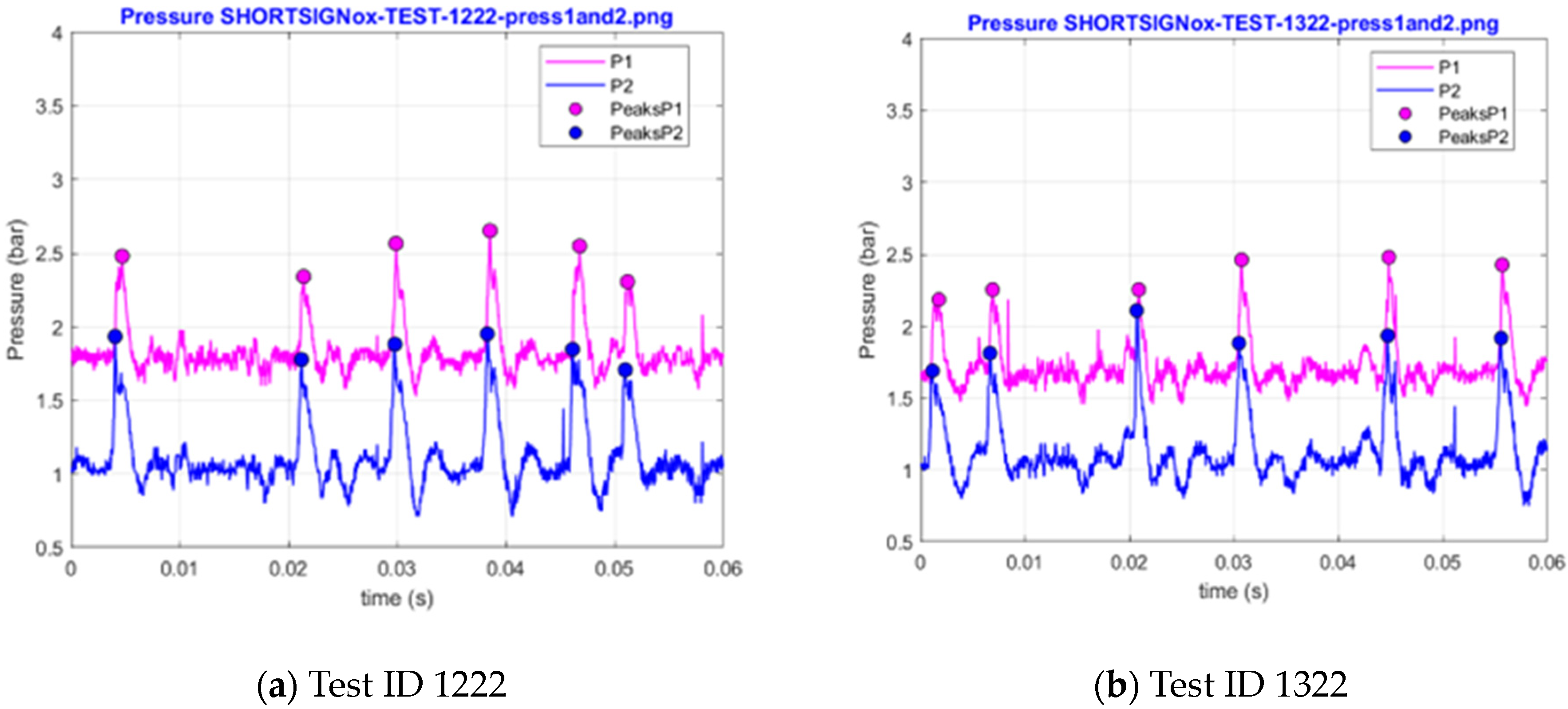
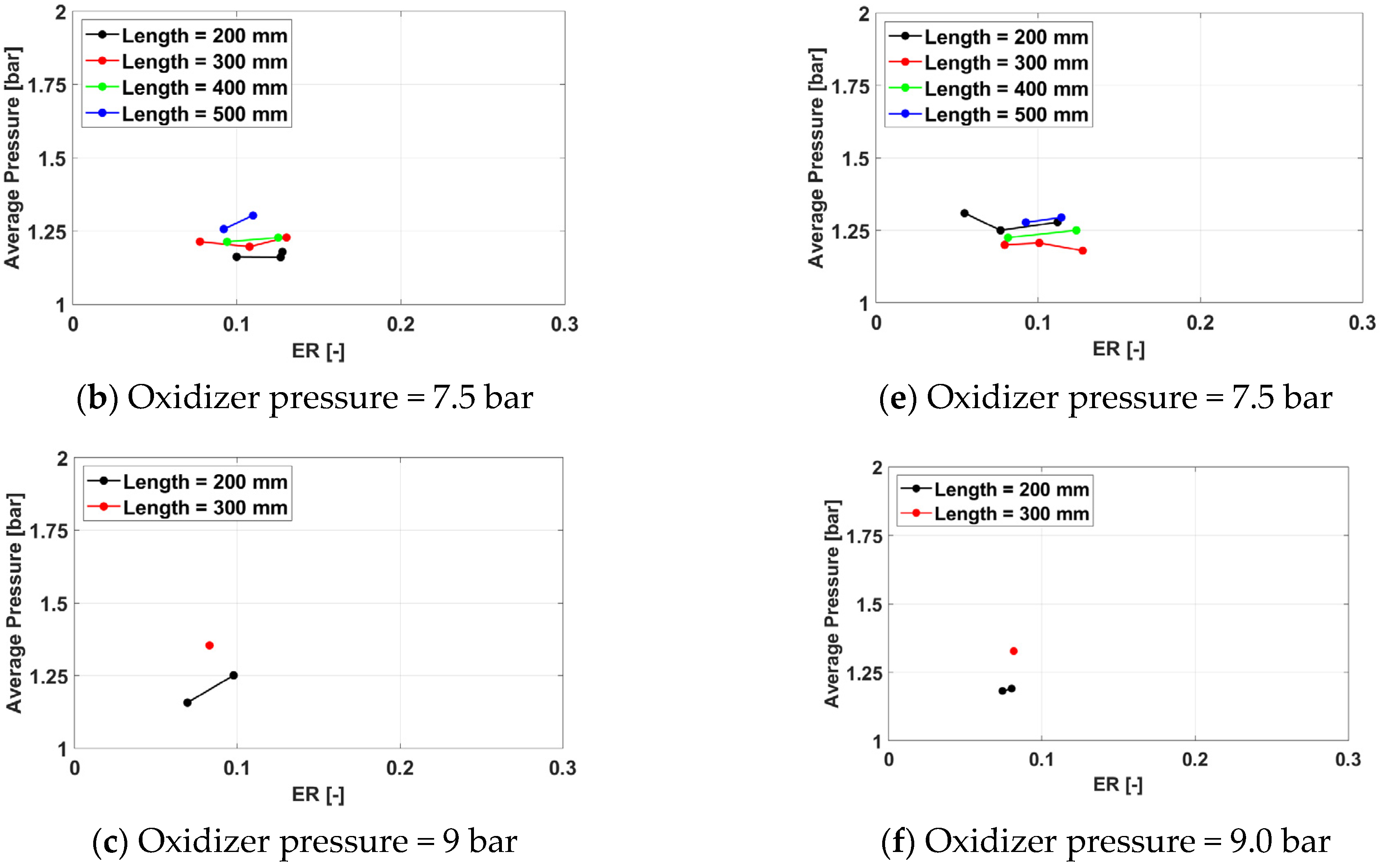
3.2.1. Average Cycle Pressure
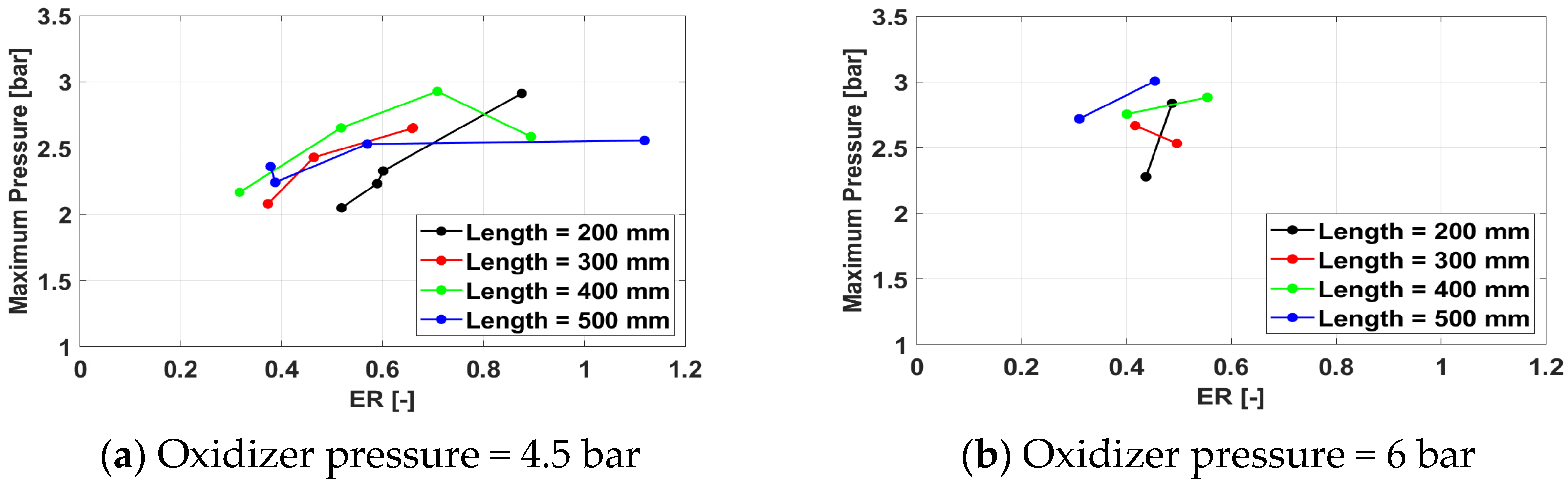
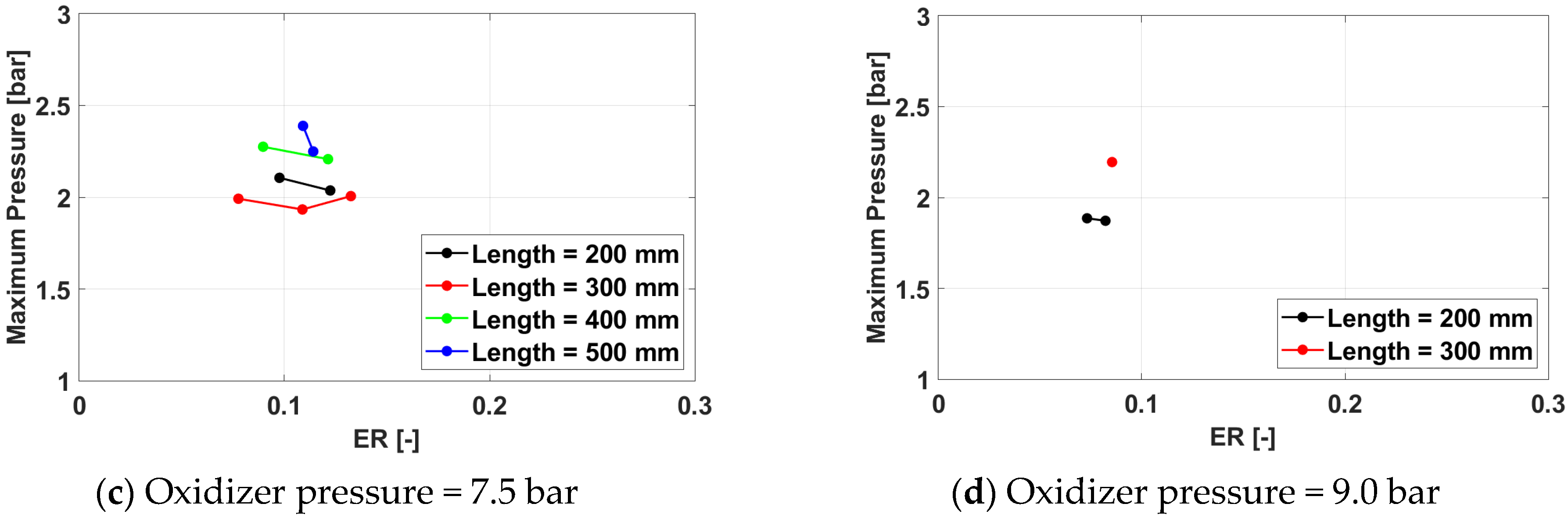
3.2.2. Maximum Cycle Pressure
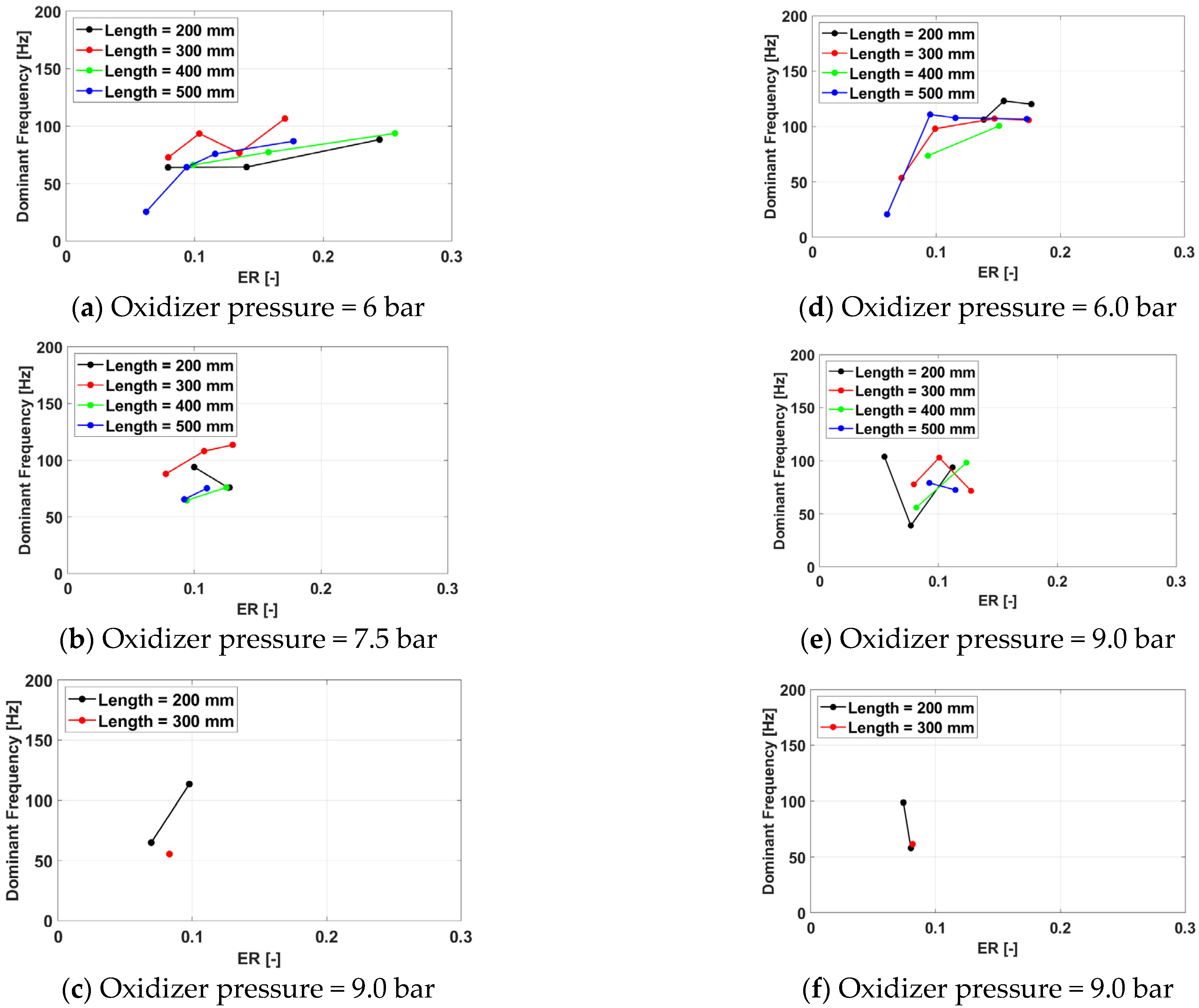
3.2.3. Operating Cycle Frequency
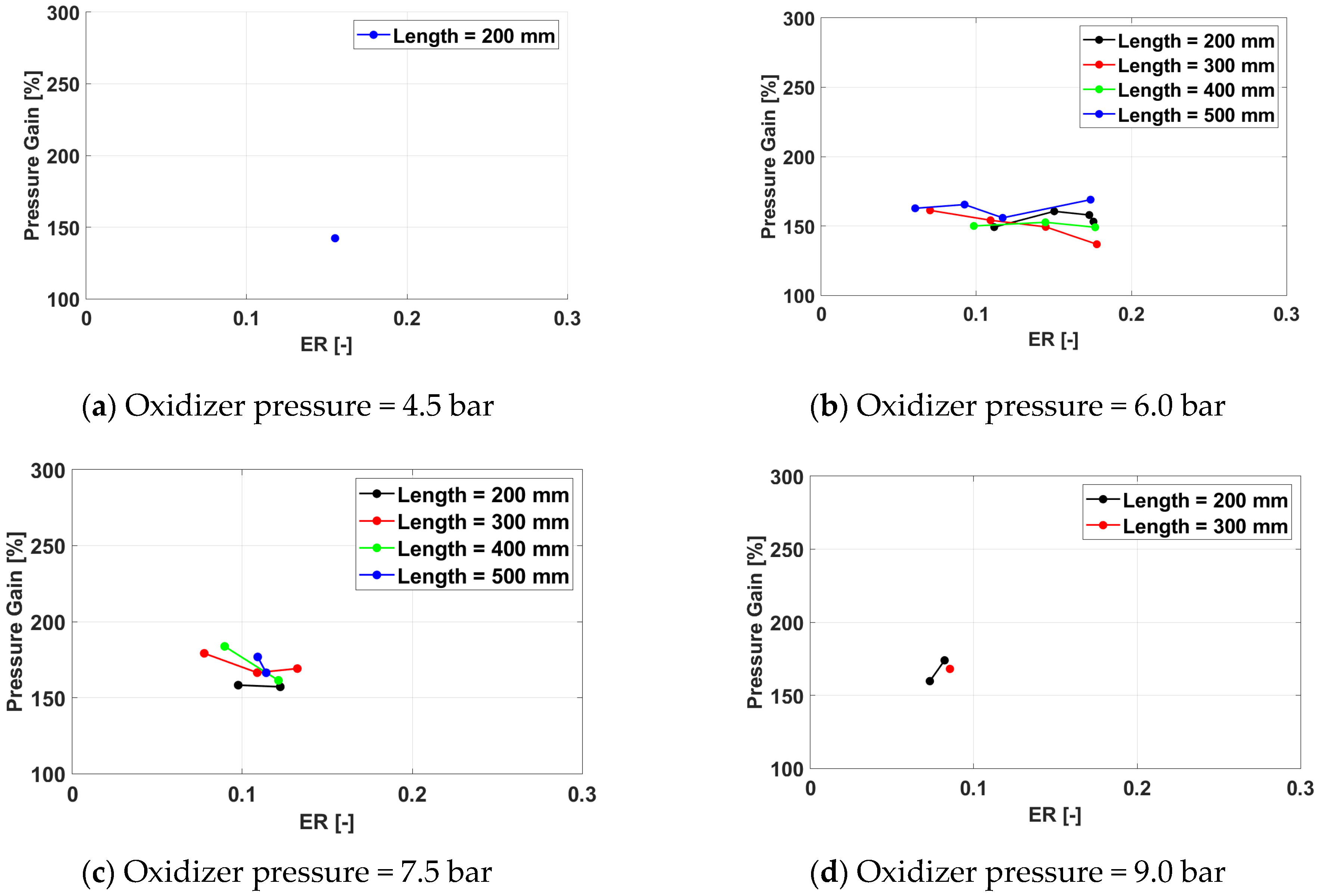
3.2.4. Pressure Gain
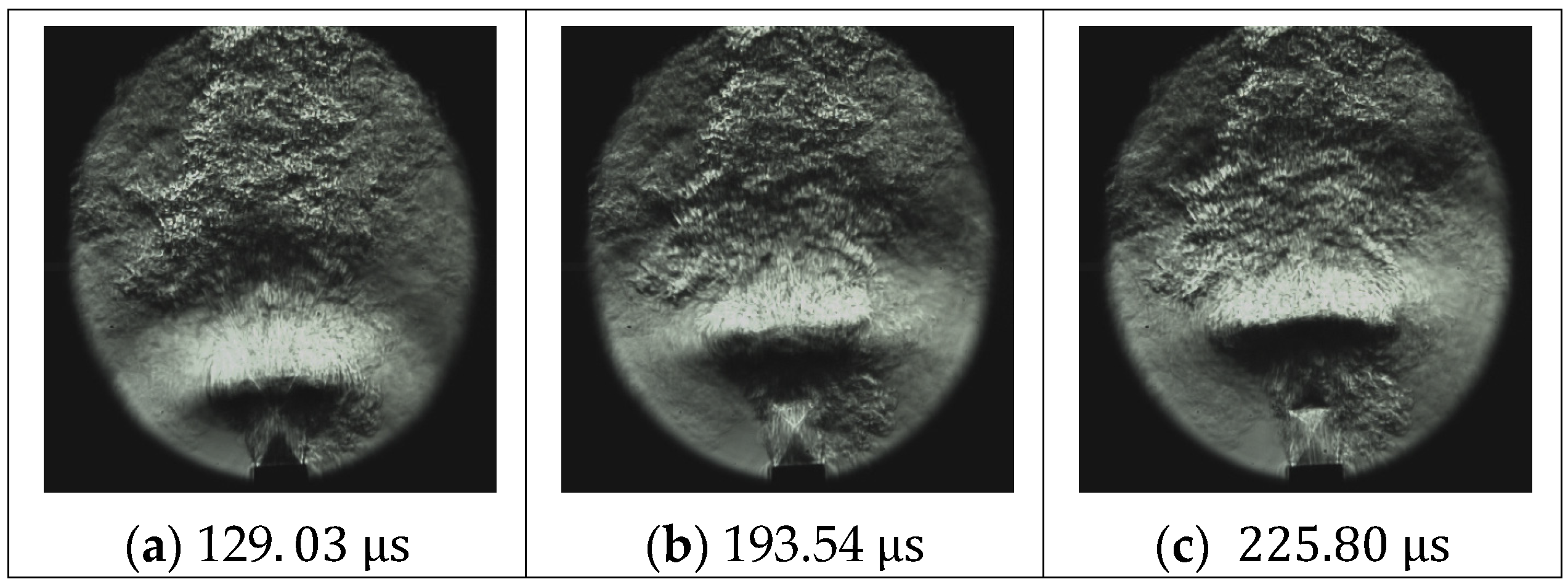
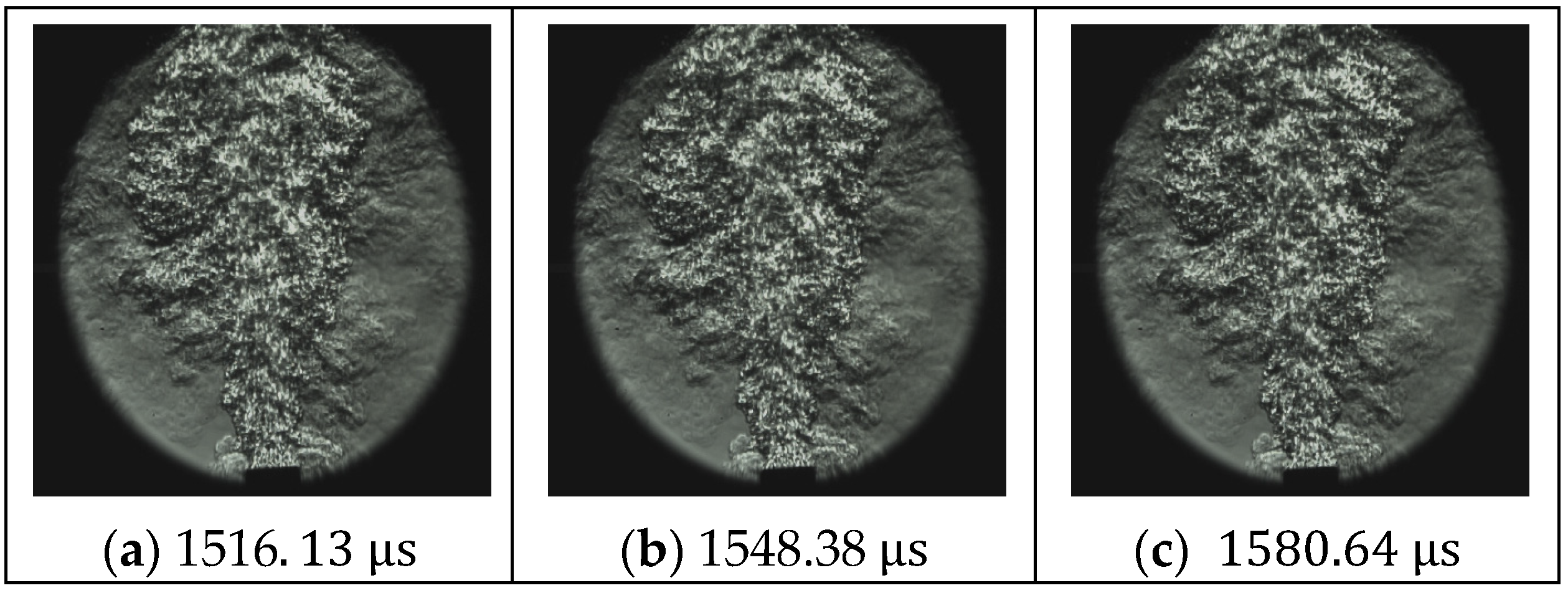
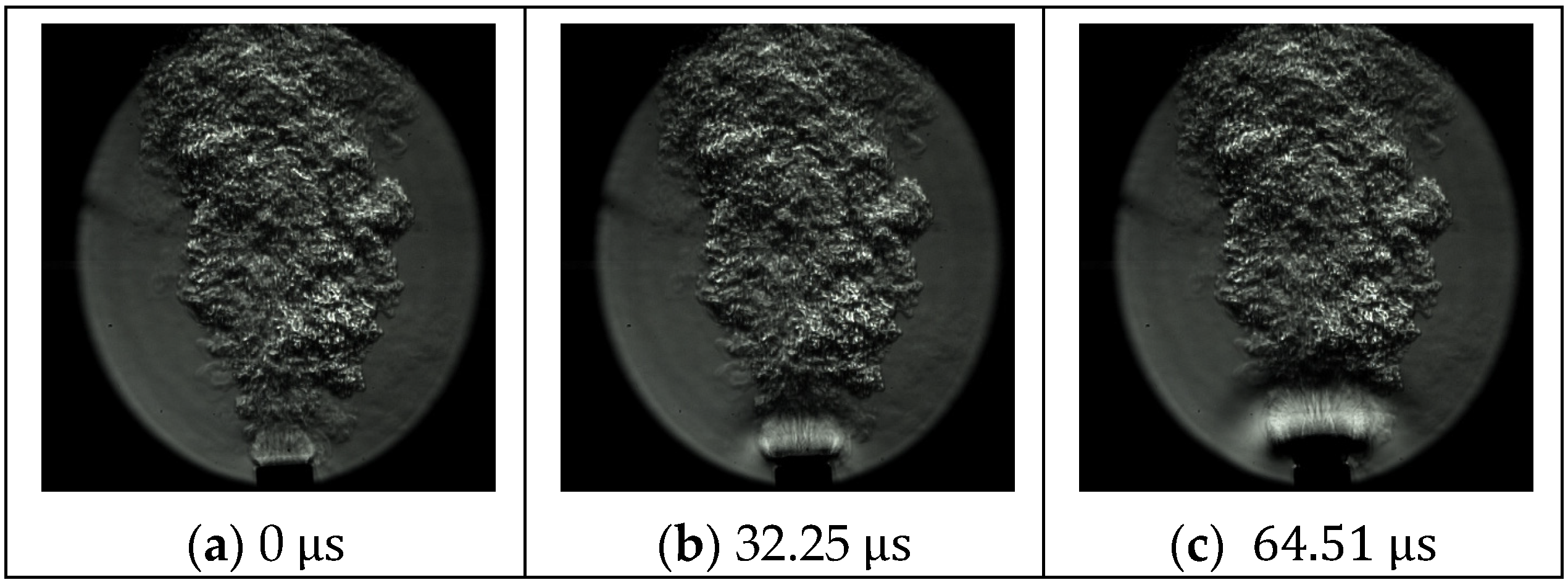
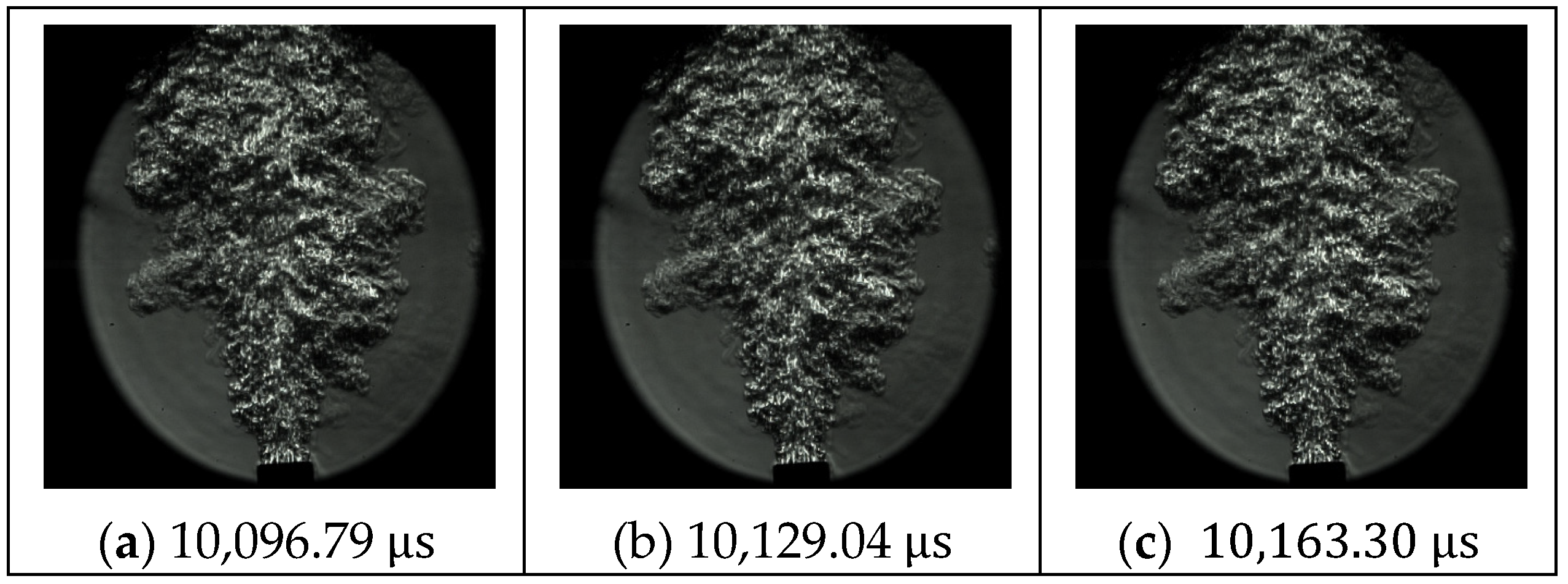
3.2.5. Schlieren Visualization
Hydrogen/Air
Hydrogen/Oxygen
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Cuciumita, C.F.; Cuciuc, T.; Porumbel, I. Evaluation of the Cycle Avearged Performances of PDE Based on Thermodynamic Cycle Computation. In Proceedings of the ASME Turbo Expo 2016, Seoul, Republic of Korea, 13–17 June 2016. GT2016-57310. [Google Scholar]
- Wintenberger, E.; Shepherd, J.E. Thermodynamic Cycle Analysis for Propagating Detonations. J. Propuls. Power 2006, 22, 694–697. [Google Scholar] [CrossRef]
- Lu, F.K.; Braun, E.M. Rotating Detonation Wave Propulsion: Experimental Challenges, Modeling, and Engine Concepts. J. Propuls. Power 2014, 30, 1125–1142. [Google Scholar] [CrossRef]
- Kailasanath, K. Review of Propulsion Applications of Detonation Waves. AIAA J. 2000, 38, 1698–1708. [Google Scholar] [CrossRef]
- Caldwell, N.; Glaser, A.; Gutmark, E. A review of pulse detonation engine research at the University of Cincinnati. In Proceedings of the 43rd AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Cincinnati, OH, USA, 8–11 July 2007; p. 5697. [Google Scholar]
- Braun, E.M.; Lu, F.K.; Wilson, D.R.; Camberos, J.A. Air breathing rotating detonation wave engine cycle analysis. Aerosp. Sci. Technol. 2013, 27, 201–208. [Google Scholar] [CrossRef]
- Pegg, R.J.; Couch, B.D.; Hunter, L.G. Pulse detonation engine air induction system analysis. In Proceedings of the 32nd Joint Propulsion Conference and Exhibit, Lake Buena Vista, FL, USA, 1–3 July 1996. AIAA Paper 96-2918. [Google Scholar]
- Cutler, A.D. Parametric Study of High Frequency Pulse Detonation Tubes. In Proceedings of the 44th AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Hartford, CT, USA, 21–23 July 2008. AIAA Paper 2008-4691. [Google Scholar]
- Matsuoka, K.; Muto, K.; Kasahara, J.; Endo, T. Development of High-Frequency Pulse Detonation Combustor without Purging Material. J. Propuls. Power 2017, 33, 43–50. [Google Scholar] [CrossRef]
- Schwinn, K.; Gejji, R.; Kan, B.; Sardeshmukh, S.; Heister, S.; Slabaugh, C.D. Self-sustained, high-frequency detonation wave generation in a semi-bounded channel. Combust. Flame 2018, 193, 384–396. [Google Scholar] [CrossRef]
- Zeldovich, Y.B. On the theory of the propagation of detonation in gaseous systems. J. Exp. Theor. Phys. 1940, 10, 542–568. (In Russian) [Google Scholar]
- Hoffmann, N. Reaction Propulsion by Intermittent Detonative Combustion. Ger. Minist. Supply 1940, AI152365, 161–167, Volkenrode Translation. [Google Scholar]
- Walker, S.H.; Shell, D.; Schena, R.; Bergmann, J.F.; Gladbach, J. The DARPA/AF Falcon Program: The Hypersonic Technology Vehicle #2 (HTV-2) Flight Demonstration Phase. In Proceedings of the 15th AIAA International Space Planes and Hypersonic Systems and Technologies Conference, Dayton, OH, USA, 28 April 2008–1 May 2008. AIAA 2008-2539. [Google Scholar]
- Gulen, S. Pressure gain combustion advantage in land-based electric power generation. J. Glob. Power Propuls. Soc. 2017, 1, 288–302. [Google Scholar] [CrossRef]
- Sakurai, T.; Sekiguchi, T.; Inoue, S. Evaluation of Pressure Gain and Turbine Inlet Conditions in a Pulse Combustion Gas Turbine. In Turbo Expo: Power for Land, Sea, and Air; American Society of Mechanical Engineers: New York, NY, USA, 2022; Volume 86007, p. V03BT04A053. [Google Scholar]
- Baptista, M.; Rasheed, A.; Badding, B.; Velagandula, O.; Dean, A. Mechanical response in a multi-tube pulsed detonation combustor-turbine hybrid system. In Proceedings of the 44th AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 9–12 January 2006; p. 1234. [Google Scholar]
- Frolov, S.M. Liquid-fueled, air-breathing pulse detonation engine demonstrator: Operation principles and performance. J. Propuls. Power 2006, 22, 1162–1169. [Google Scholar] [CrossRef]
- Frolov, S.M. Natural-gas-fueled pulse-detonation combustor. J. Propuls. Power 2014, 30, 41–46. [Google Scholar] [CrossRef]
- Wang, K.; Fan, W.; Lu, W.; Chen, F.; Zhang, Q.; Yan, C. Study on a liquid-fueled and valveless pulse detonation rocket engine without the purge process. Energy 2014, 71, 605–614. [Google Scholar] [CrossRef]
- Card, J.; Rival, D.; Ciccarelli, G. DDT in fuel–air mixtures at elevated temperatures and pressures. Shock Waves 2005, 14, 167–173. [Google Scholar] [CrossRef]
- Warimani, M.; Azami, M.H.; Khan, S.A.; Ismail, A.F.; Saharin, S.; Ariffin, A.K. Internal flow dynamics and performance of pulse detonation engine with alternative fuels. Energy 2021, 237, 121719. [Google Scholar] [CrossRef]
- Soni, S.K.; Singh, A.; Sandhu, M.; Goel, A.; Sharma, R.K. Numerical simulation to investigate the effect of obstacle on detonation wave propagation in a pulse detonation engine combustor. Int. J. Emerg. Technol. Adv. Eng. 2013, 3, 458–464. [Google Scholar]
- Falempin, F.; Bouchaud, D.; Daniau, E. Pulsed detonation engine—Towards a tactical missile application. In Proceedings of the 36th AIAA/ASME/SAE/ASEE Joint Propulsion Conference and Exhibit, Las Vegas, NV, USA, 24–28 July 2000; p. 3473. [Google Scholar]
- Teasley, T.W.; Fedotowsky, T.M.; Gradl, P.R.; Austin, B.L.; Heister, S.D. Current State of NASA Continuously Rotating Detonation Cycle Engine Development. In Proceedings of the AIAA SCITECH 2023 Forum, National Harbor, MD, USA, 23–27 January 2023. AIAA 2023-1873. [Google Scholar]
- Goto, K.; Matsuoka, K.; Matsuyama, K.; Kawasaki, A.; Watanabe, H.; Itouyama, N.; Kazuki Ishihara, K.; Buyakofu, V.; Noda, T.; Kasahara, J.; et al. Space Flight Demonstration of Rotating Detonation Engine Using Sounding Rocket S-520-31. J. Spacecr. Rocket. 2023, 60, 273–285. [Google Scholar] [CrossRef]
- Trevithick, J. GE’s Breakthrough in ‘Detonating’ Hypersonic Propulsion Is a Big Deal. The War Zone. 2023. Available online: https://www.thedrive.com/the-war-zone/ges-breakthrough-in-detonating-hypersonic-propulsion-is-a-big-deal (accessed on 14 December 2023).
- New, T.H.; Panicker, P.K.; Lu, F.K.; Tsai, H.M. Experimental Investigations on DDT Enhancements by Schelkin Spirals in a PDE. In Proceedings of the 44th AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 9–12 January 2006. [Google Scholar]
- Schultz, E.; Wintenberger, E.; Shepherd, J. Investigation of Deflagration to Detonation Transition for Application to Pulse Detonation Engine Ignition Systems. In Proceedings of the 16th JANNAF Propulsion Symposium, Pasadena, CA, USA, 6–9 May 1999. [Google Scholar]
- Sorin, R.; Zitoun, R.; Desbordes, D. Optimization of the deflagration to detonation transition: Reduction of length and time of transition. Shock. Waves 2006, 15, 137–145. [Google Scholar] [CrossRef]
- Veser, A.; Breitung, W.; Dorofeev, S.B. Run-up distances to supersonic flames in obstacle-laden tubes. J. Phys. IV Fr. 2002, 12, 333–340. [Google Scholar] [CrossRef]
- Wilson, J.; Wernet, M.P.; Paxson, D.E. Vortex Rings Generated by a Shrouded Hartmann–Sprenger Tube. AIAA J. 2006, 44, 2706–2718. [Google Scholar] [CrossRef]
- Wu, Y.; Han, Q.; Yang, G. Effects of an acoustic atomizer upon liquid-fueled detonation initiations in a detonation tube. Exp. Therm. Fluid Sci. 2019, 109, 109863. [Google Scholar] [CrossRef]
- Frolov, S.; Smetanyuk, V.; Aksenov, V.; Koval, A. Deflagration-to-detonation transition in crossedflow fast jets of propellant components. In Doklady Physical Chemistry; Springer: Berlin/Heidelberg, Germany, 2017; Volume 476, pp. 153–156. [Google Scholar]
- Cojocea, A.V.; Cuciuc, T.; Porumbel, I.; Gall, M.; Gherman, B.; Crunţeanu, D.E. Experimental Investigations of Hydrogen Fuelled Pulsed Detonation Combustor. In Turbo Expo: Power for Land, Sea, and Air; American Society of Mechanical Engineers: New York, NY, USA, 2022; Volume 86007, p. V03BT04A020. [Google Scholar]
- Shepherd, J. Detonation Database. 2002. Available online: https://shepherd.caltech.edu/detn_db/html/db.html (accessed on 12 May 2024).
- Peraldi, O.; Knystautas, R.; Lee, J.H. Criteria for transition to detonation in tubes. In Symposium (International) on Combustion; Elsevier: Amsterdam, The Netherlands, 1988; Volume 21, pp. 1629–1637, No. 1. [Google Scholar]
- Chan, C.K.; Greig, D.R. The structures of fast deflagrations and quasi-detonations. In Symposium (International) on Combustion; Elsevier: Amsterdam, The Netherlands, 1989; Volume 22, pp. 1733–1739, No. 1. [Google Scholar]
- Teodorczyk, A.; Lee, J.H.S.; Knystautas, R. Propagation mechanism of quasi-detonations. In Symposium (International) on Combustion; Elsevier: Amsterdam, The Netherlands, 1989; Volume 22, pp. 1723–1731, No. 1. [Google Scholar]






















| Line | Gas | Pressure (bar) | Temperature (K) | Mass Flow Rate (kg/s) |
|---|---|---|---|---|
| 1 | Air | 10 | 750 | 1 |
| 2 | Air, | 20 | 300 | 0.2 |
| 3 | , | 20 | 300 | 0.05 |
| Pos. | Component | Remarks |
|---|---|---|
| 1 | Compressor | Air, max 11 bar |
| 2 | Compressor tank | 500 L |
| 3 | Pressure gauge | Measures pressure in the compressor tank |
| 4 | Relief vane | Opens at 11 bar |
| 5 | Atmospheric exhaust | |
| 6 | Vane | Ball, opens the line from the compressor |
| 7 | 3-way vane | Feeds air to tank or by-passes it |
| 8 | Small air tank | 1000 L |
| 9 | Relief vane | Opens at 11 bar |
| 10 | Atmospheric exhaust | |
| 11 | Pressure gauge | Measures pressure in the small air tank |
| 12 | One-way vane | Prevents by-passed air to return to tank system |
| 13 | Control vane | Butterfly, manual, open line towards the experimental zone |
| 14 | Pressure gauge | Measures pressure downstream of the control vane |
| 15 | Flow meter | CS INSTRUMENTS, VA 500, CS INSTRUMENTS GmbH & Co KG, Tannheim, Germany |
| 16 | 3-way vane | Feeds air to the heater or by-passes it |
| 17 | Thermocouple | Measures temperature upstream of the heater |
| 18 | Heater | Electric, OSRAM SYLVANIA, SFI-8D-192-38, OSRAM SYLVANIA, Hillsborough, NH, USA |
| 19 | Thermocouple | Measures temperature downstream of the heater |
| 20 | One-way vane | Prevents by-passed air to return to heater |
| 21 | 3-way vane | Electrical allows by-pass of air during heating to protect long exposure of downstream equipment |
| 22 | Atmospheric exhaust | |
| 23 | Calibration vane | Butterfly, electrical, sets flow towards the experimental zone |
| 24 | Pressure gauge | Measures pressure downstream of calibration vane |
| 25 | Safety vane | Opens at 11 bar |
| 26 | One-way vane | Prevents backflow from the experimental zone |
| 27 | Line 1 output | Provides oxidizer to the experimental zone |
| 28 | Vane | Ball, opens the line to the large air tank |
| 29 | Small air tank | 5000 L |
| 30 | Pressure gauge | Measures pressure in the large air tank |
| 31 | Relief vane | Opens at 11 bar |
| 32 | Atmospheric exhaust | |
| 33 | Vane | Ball, connects tank system to Line 2 |
| 34 | Cylinder | Oxygen or air at 50 L, 200 bar |
| 35 | Pressure gauge | Measures pressure in the cylinder |
| 36 | Pressure regulator | Manual, Oxygen or air |
| 37 | Pressure gauge | Measures pressure downstream of the regulator |
| 38 | 3-way vane | Connects either tank system or cylinder to Line 2 |
| 39 | One-way vane | Prevents backflow from the experimental zone |
| 40 | Calibration vane | Ball, manual, set flow towards the experimental zone |
| 41 | Flow meter | ALICAT MQ-2000SLPM-D, ALICAT Scientific Inc., Tucson, AZ, USA |
| 42 | Thermocouple | Measures temperature on Line 2. Integrated in flow meter |
| 43 | Pressure gauge | Measures pressure downstream of calibration vane |
| 44 | STOP vane | Electrical, open Line 2 towards the experimental zone or buffer |
| 45 | 3-way vane | Directs flow towards buffer tank or experimental zone |
| 46 | Buffer tank | 10 l. Creates buffer for fast flow release to experimental zone |
| 47 | Relief vane | Opens at 11 bar |
| 48 | Atmospheric exhaust | |
| 49 | Pressure gauge | Measures pressure in the buffer tank |
| 50 | STOP vane | Electrical, open buffer tank towards the experimental zone |
| 51 | Line 2 output | Provides oxidizer to the experimental zone |
| 52 | Cylinder | Hydrogen or methane at 50 L, 200 bar |
| 53 | Pressure gauge | Measures pressure in the cylinder |
| 54 | Pressure regulator | Manual, Oxygen or air |
| 55 | Pressure gauge | Measures pressure downstream of the regulator |
| 56 | One-way vane | Prevents backflow from the experimental zone |
| 57 | Calibration vane | Needle, manual, set flow towards the experimental zone |
| 58 | Flow meter | ALICAT MQ-250SLPM-D, ALICAT Scientific Inc., Tucson, AZ, USA |
| 59 | Thermocouple | Measures temperature on Line 2. Integrated in flow meter |
| 60 | Pressure gauge | Measures pressure downstream of calibration vane |
| 61 | STOP vane | Electrical, open Line 2 towards the experimental zone or buffer |
| 62 | Flame arrestor | Prevents flashback |
| 63 | Line 3 output | Provides fuel to the experimental zone |
| Factor | Level 0 | Level 1 | Level 2 | Level 3 |
|---|---|---|---|---|
| L [mm] | 200 | 300 | 400 | 500 |
| [Hz] | 100 | 150 | 233 | 350 |
| [bar] | 5.5 | 7.0 | 8.5 | 10.0 |
| [bar] | 9.0 | 7.5 | 6.0 | 4.5 |
| Frequency [Hz] | Expected Number of Cycles | [s] | Temporal Domain Checked for Duplicate Peaks [s] |
|---|---|---|---|
| 100 | 6 | 0.01 | |
| 150 | 9 | 0.0067 | |
| 233 | 14 | 0.0043 | |
| 350 | 21 | 0.0029 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bogoi, A.; Cuciuc, T.; Cojocea, A.V.; Gall, M.; Porumbel, I.; Hrițcu, C.E. Experimental Pressure Gain Analysis of Pulsed Detonation Engine. Aerospace 2024, 11, 465. https://doi.org/10.3390/aerospace11060465
Bogoi A, Cuciuc T, Cojocea AV, Gall M, Porumbel I, Hrițcu CE. Experimental Pressure Gain Analysis of Pulsed Detonation Engine. Aerospace. 2024; 11(6):465. https://doi.org/10.3390/aerospace11060465
Chicago/Turabian StyleBogoi, Alina, Tudor Cuciuc, Andrei Vlad Cojocea, Mihnea Gall, Ionuț Porumbel, and Constantin Eusebiu Hrițcu. 2024. "Experimental Pressure Gain Analysis of Pulsed Detonation Engine" Aerospace 11, no. 6: 465. https://doi.org/10.3390/aerospace11060465
APA StyleBogoi, A., Cuciuc, T., Cojocea, A. V., Gall, M., Porumbel, I., & Hrițcu, C. E. (2024). Experimental Pressure Gain Analysis of Pulsed Detonation Engine. Aerospace, 11(6), 465. https://doi.org/10.3390/aerospace11060465







