Numerical Simulation on Aerodynamic Characteristics of Transition Section of Tilt-Wing Aircraft
Abstract
1. Introduction
2. Methodological Description
2.1. Computational Model
2.2. Numerical Simulation Methods
2.3. Numerical Method Validation
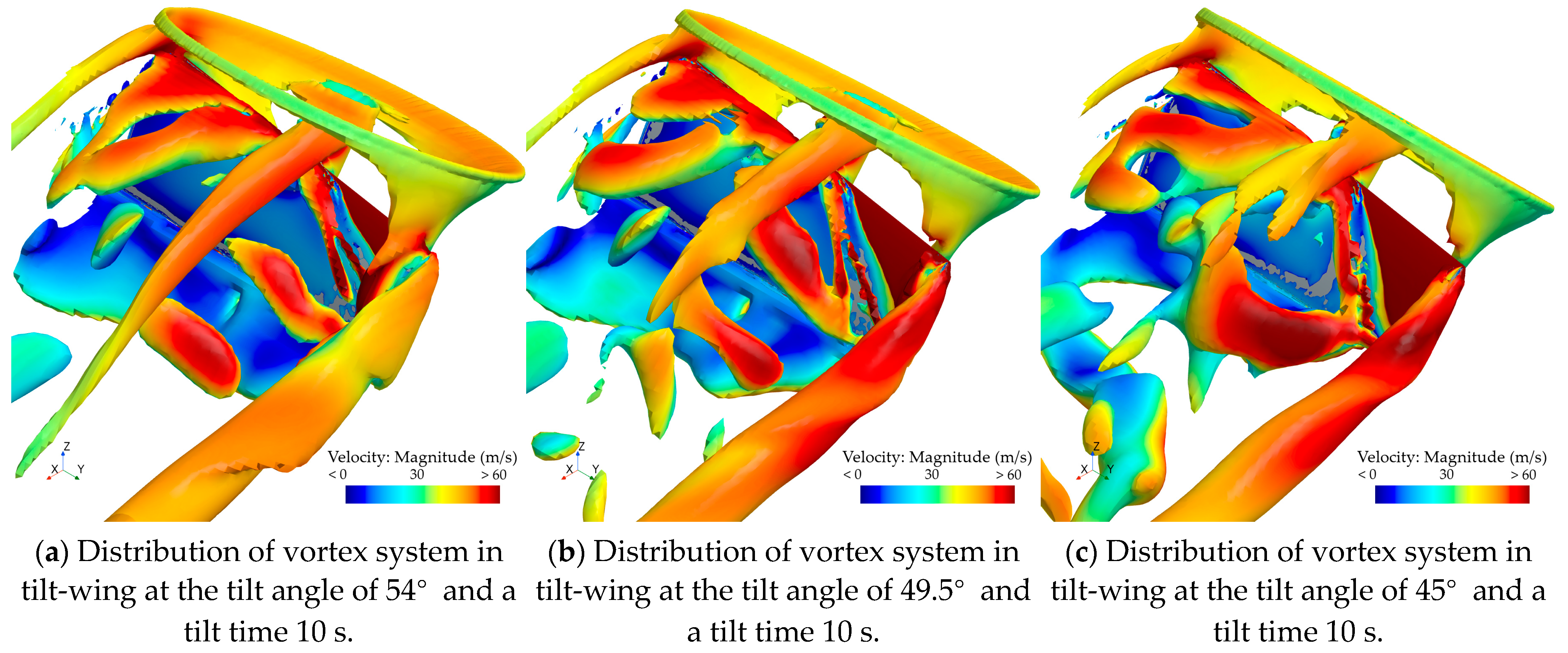
3. Results and Analyses
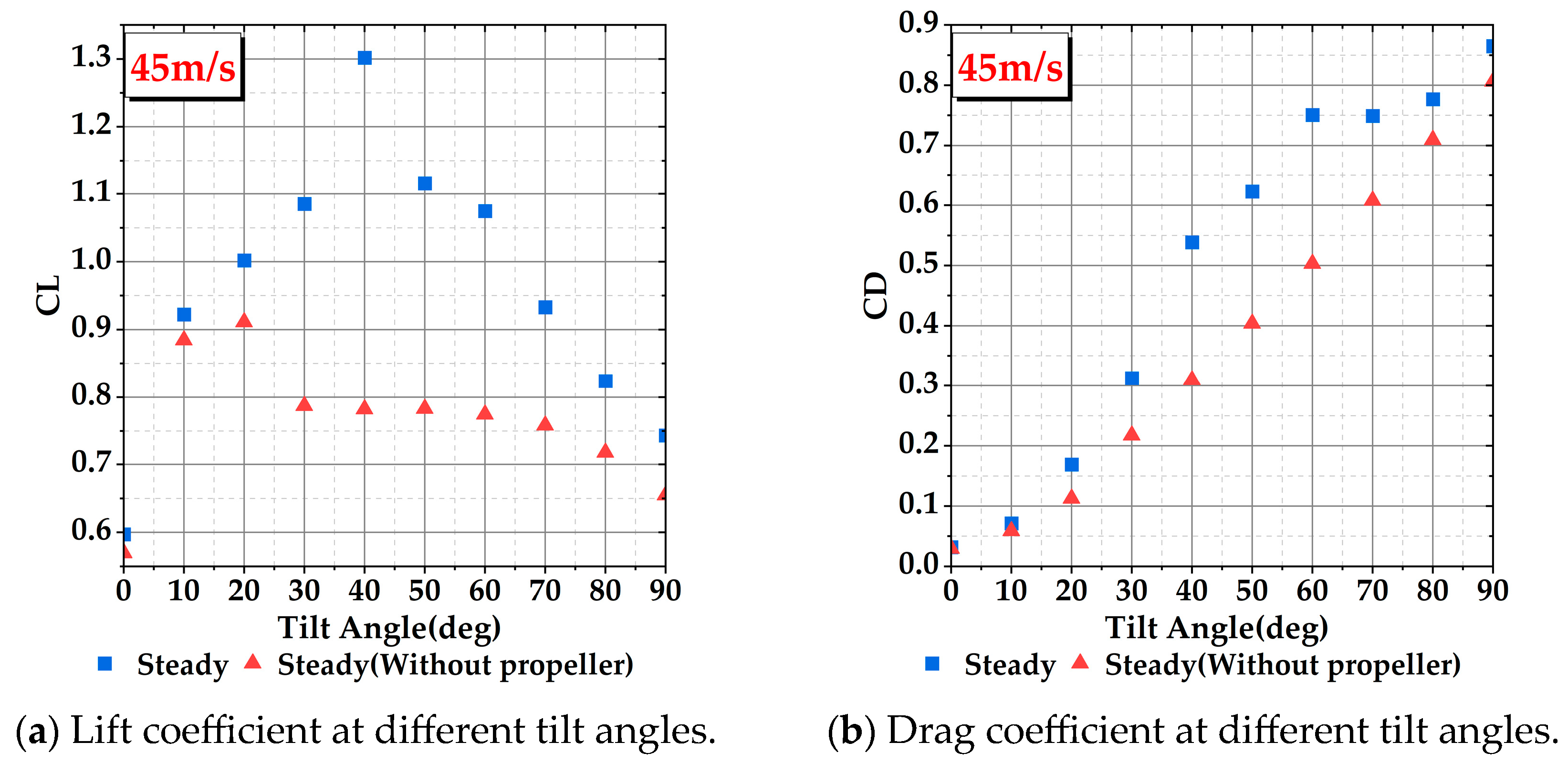
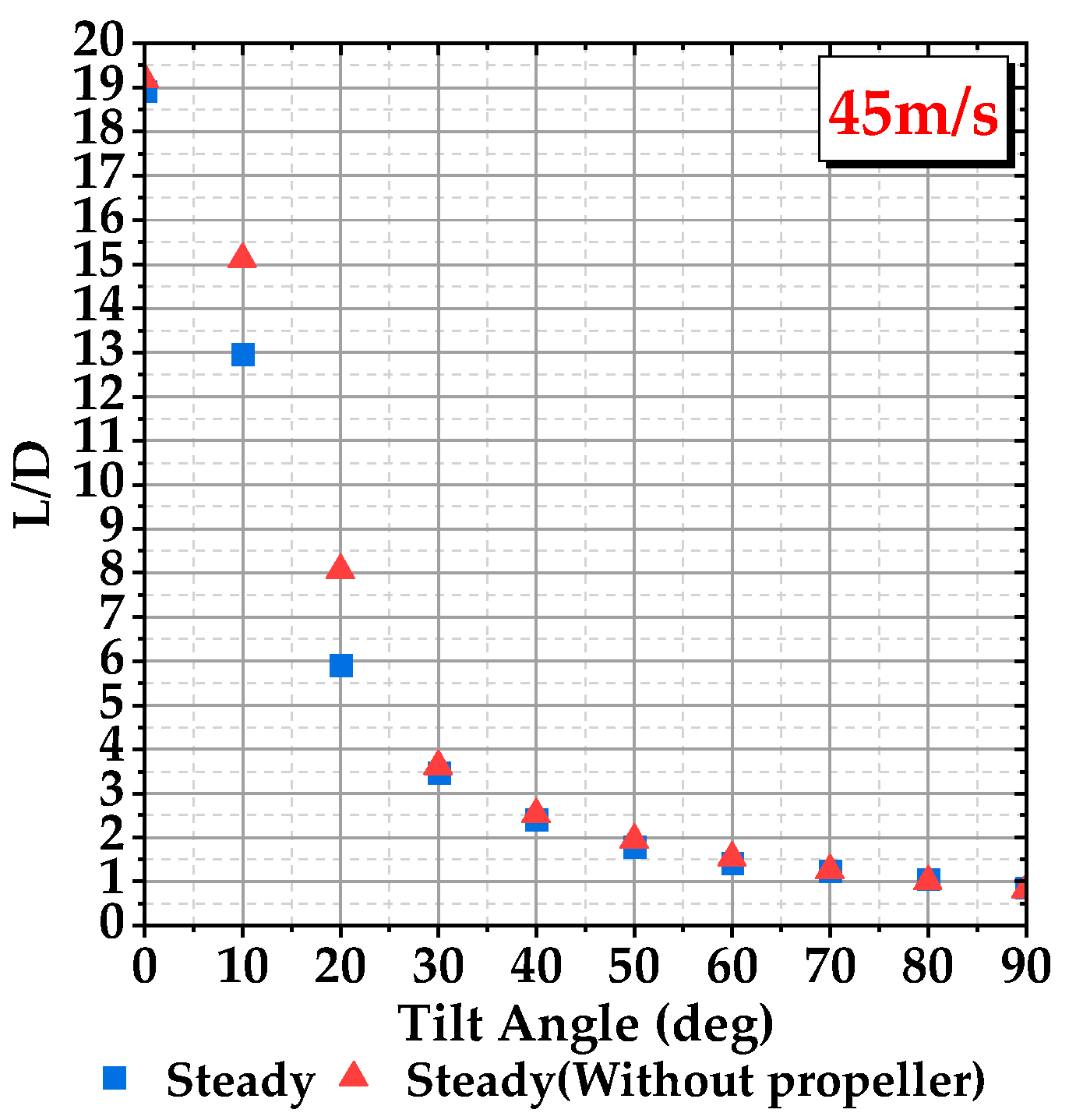
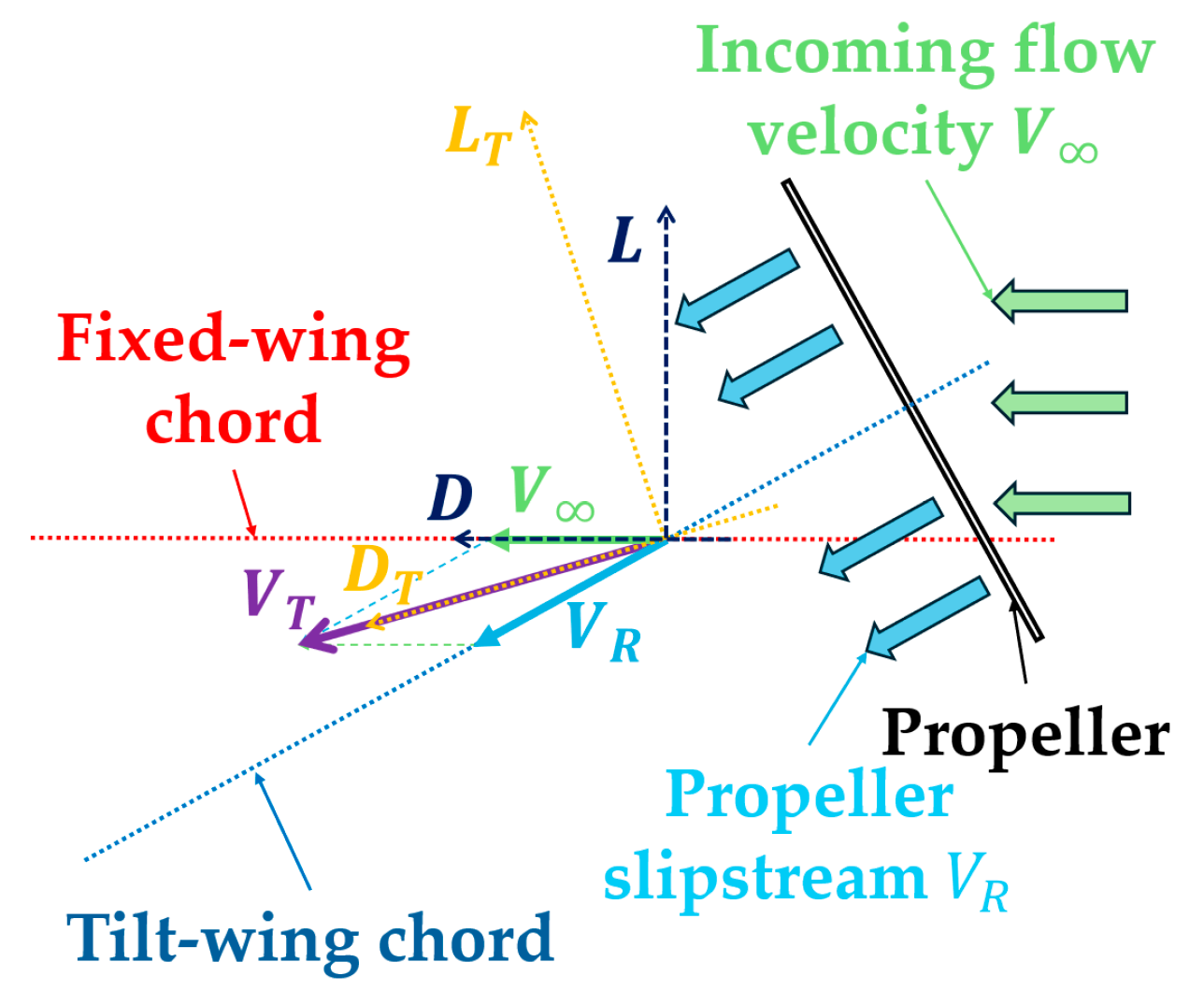
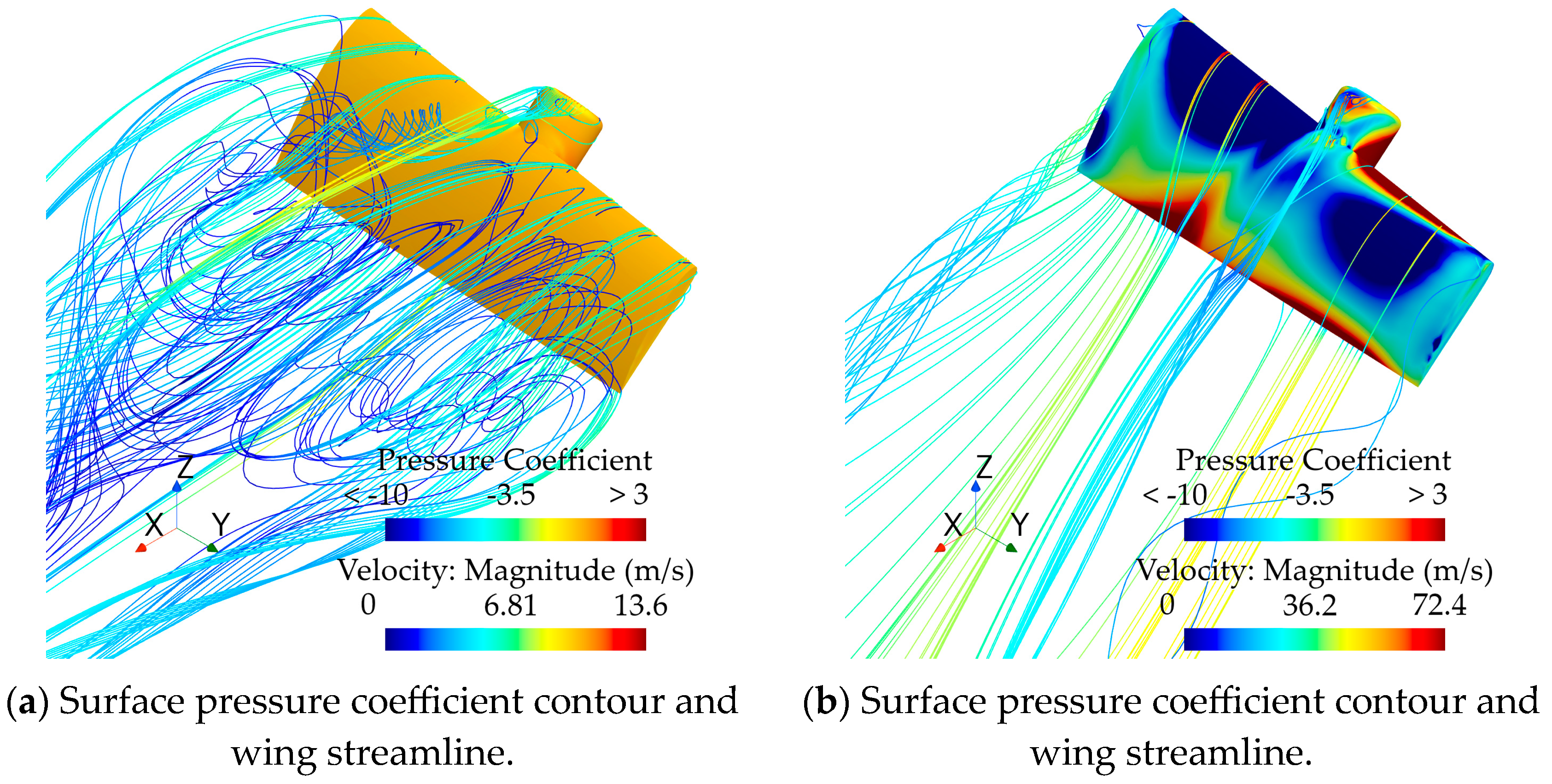
3.1. Impact of Propeller on Tilt-Wing
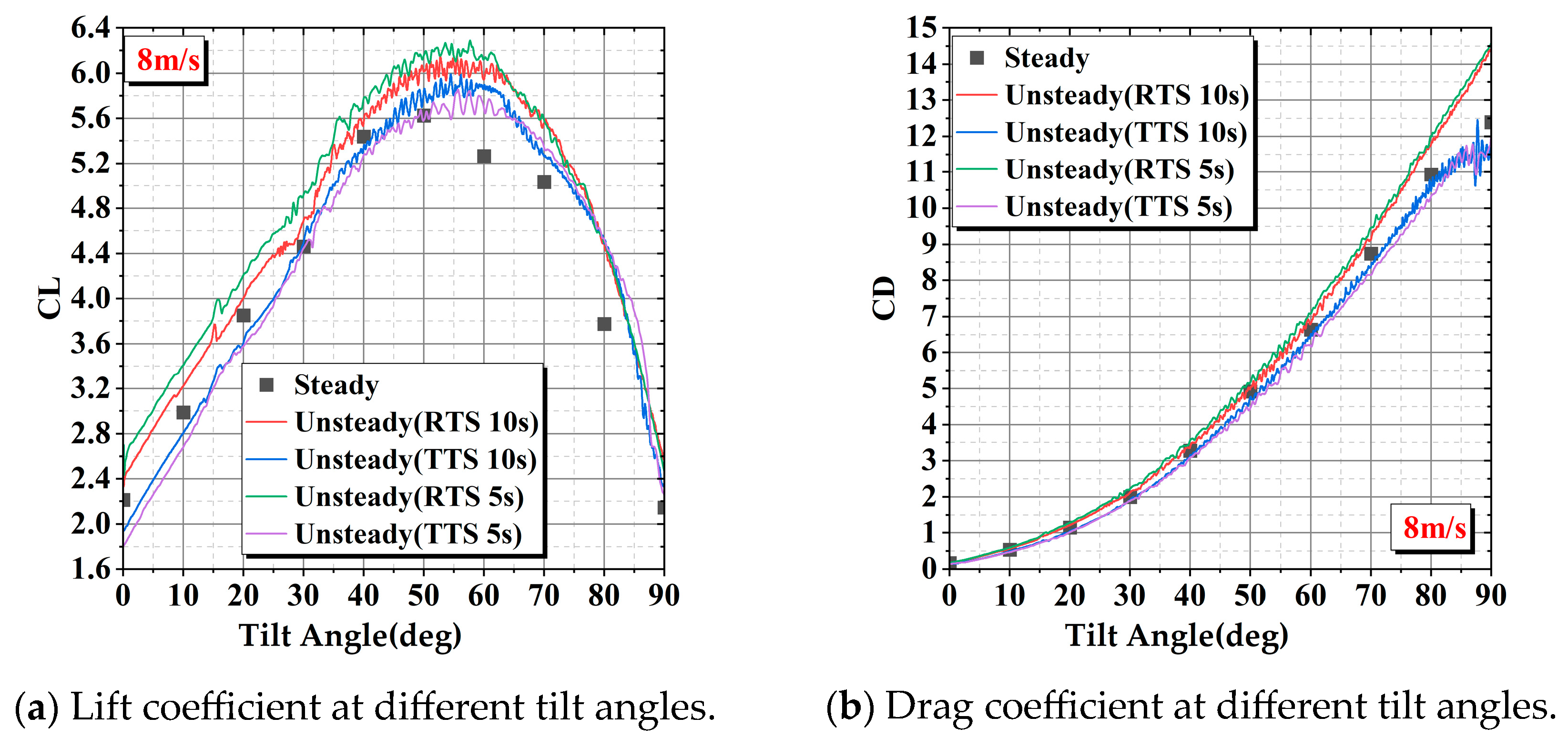
3.2. Aerodynamic Characteristics of the Tilt Transition Section
3.2.1. Explanation of Calculation Results
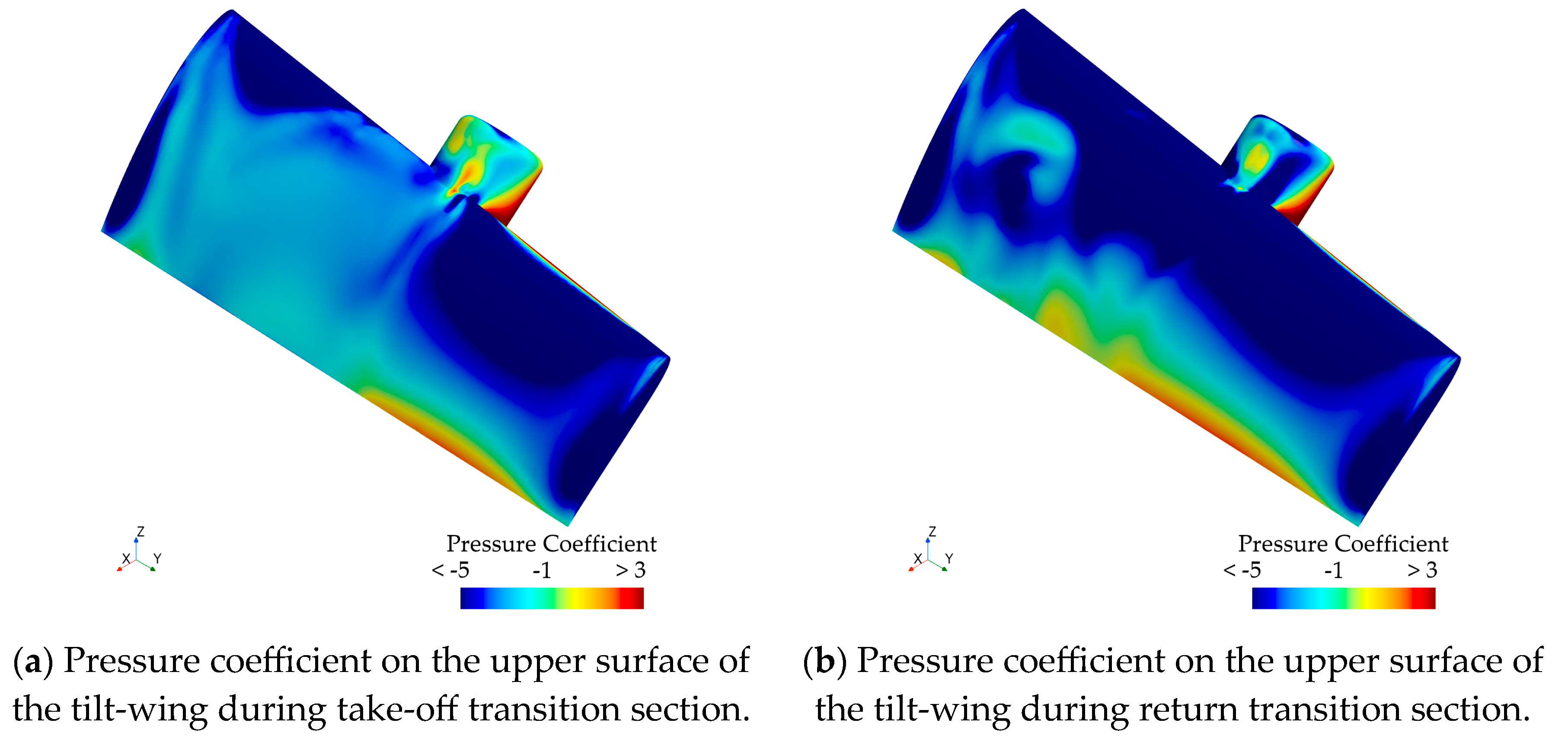
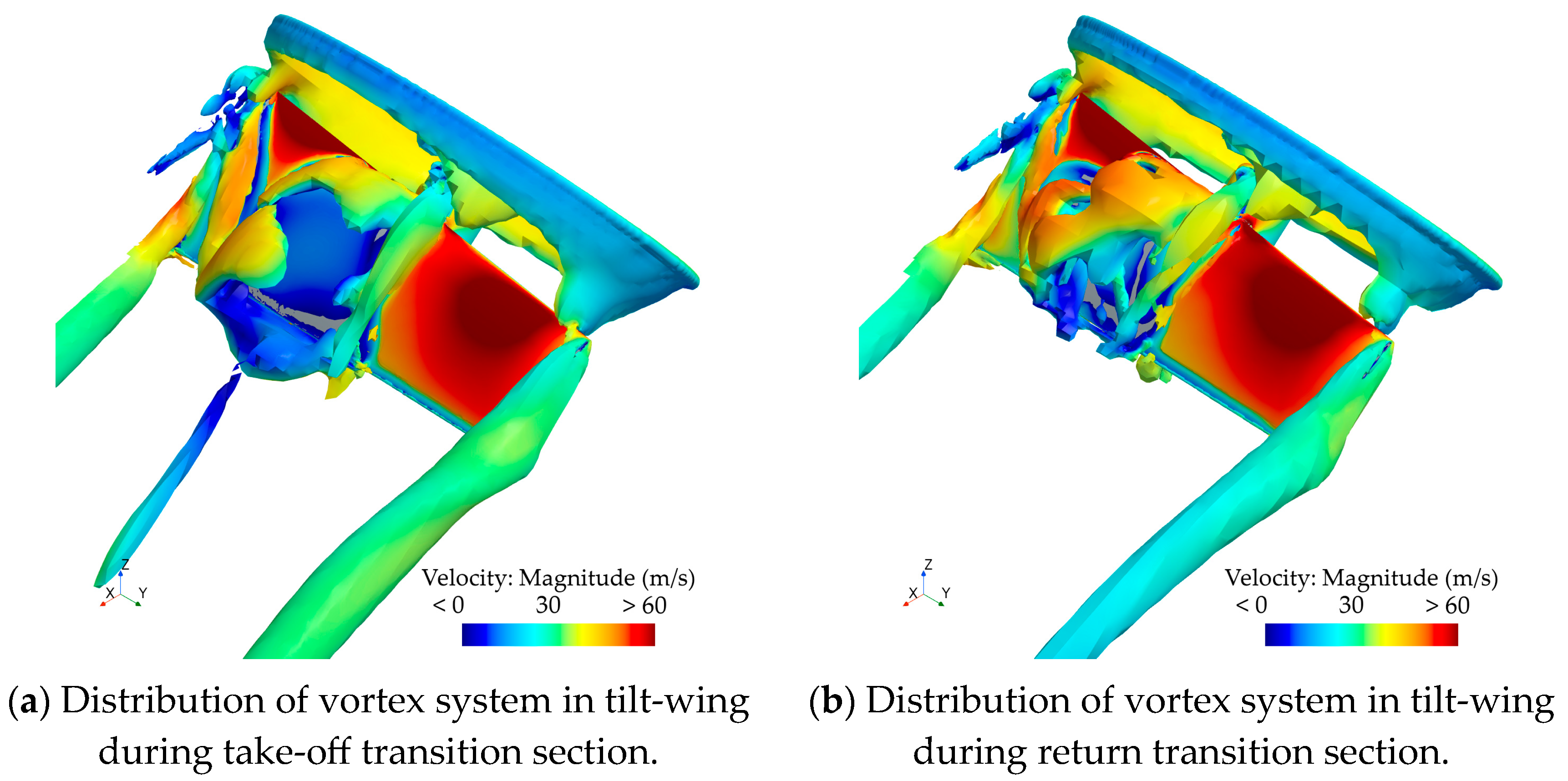
3.2.2. Differences in Aerodynamic Forces between Take-Off Transition and Return Transition Section
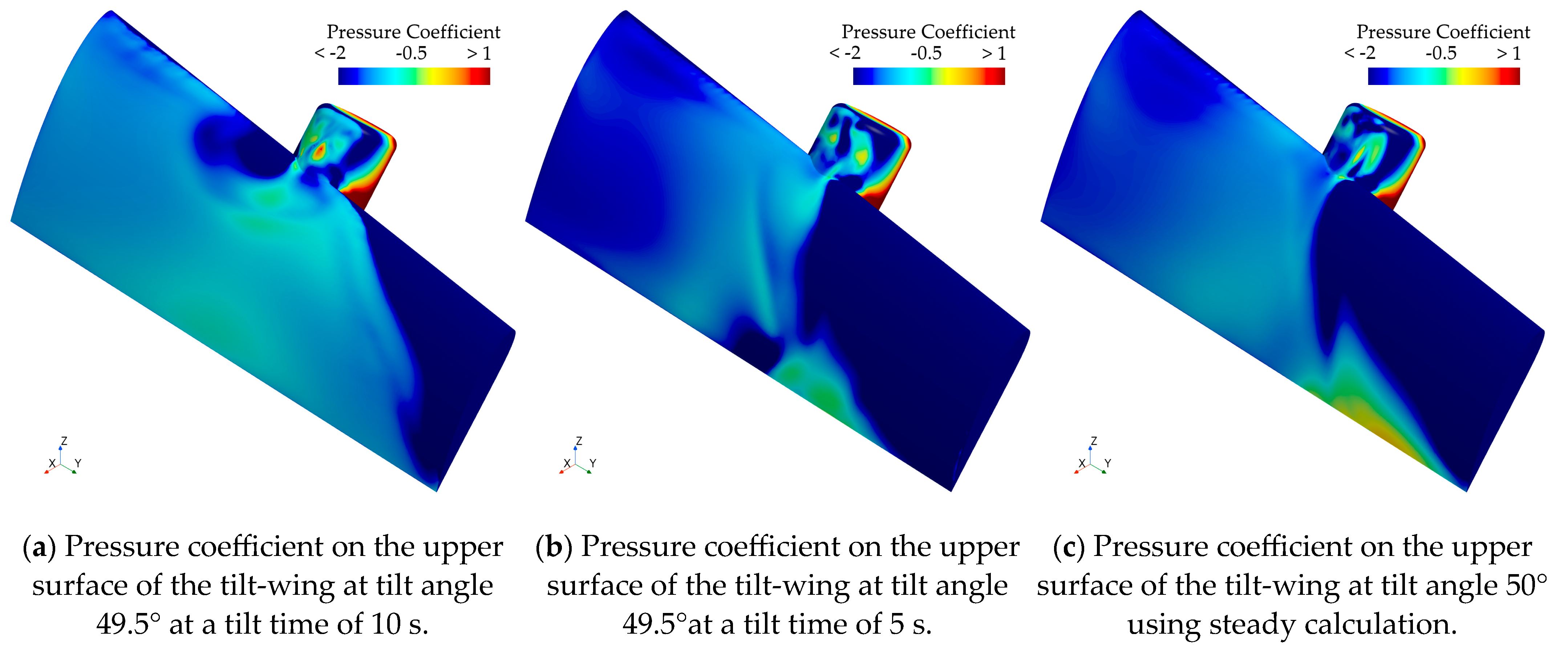
3.2.3. The Effect of Tilt Speed on the Take-Off transition Section at a Freestream Velocity of 30 m/s
4. Conclusions
- (1)
- In the transition section, the slipstream velocity can be increased by adjusting the propeller speed, thereby allowing the aircraft to complete the mode transition faster.
- (2)
- The take-off transition section requires a higher propeller speed compared to the return transition section to overcome the stall problem on the wing’s upper surface.
- (3)
- The tilt process and tilt speed of the tilt-wing aircraft affect the variation in aerodynamic forces in the transition section. If the aerodynamic data at a fixed tilt angle are calculated as the input for the flight control system, it may result in difficulties in maintaining altitude during transition section, which could affect flight safety.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Straubinger, A.; Rothfeld, R.; Shamiyeh, M.; Büchter, K.-D.; Kaiser, J.; Plötner, K.O. An Overview of Current Research and Developments in Urban Air Mobility—Setting the Scene for UAM Introduction. J. Air Transp. Manag. 2020, 87, 101852. [Google Scholar] [CrossRef]
- Kuhn, H.; Falter, C.; Sizmann, A. Renewable Energy Perspectives for Aviation. In Proceedings of the 3rd CEAS Air&Space Conference/21st AIDAA Congress, Venice, Italy, 24–28 October 2011. [Google Scholar]
- Doo, J.T.; Pavel, M.D.; Didey, A.; Hange, C.; Field, M.; Diller, N.P.; Tsairides, M.A.; Smith, M.; Textron, B.; Bennet, E.; et al. NASA Electric Vertical Takeoff and Landing (eVTOL) Aircraft Technology for Public Services—A White Paper; NASA: Greenbelt, MD, USA, 2021.
- Bacchini, A.; Cestino, E. Electric VTOL Configurations Comparison. Aerospace 2019, 6, 26. [Google Scholar] [CrossRef]
- Kumar, V.; Michael, N. Opportunities and Challenges with Autonomous Micro Aerial Vehicles. In Robotics Research: The 15th International Symposium ISRR; Christensen, H.I., Khatib, O., Eds.; Springer Tracts in Advanced Robotics; Springer International Publishing: Cham, Switzerland, 2017; pp. 41–58. ISBN 978-3-319-29363-9. [Google Scholar]
- Xiang, S.; Xie, A.; Ye, M.; Yan, X.; Han, X.; Niu, H.; Li, Q.; Huang, H. Autonomous eVTOL: A Summary of Researches and Challenges. Green Energy Intell. Transp. 2024, 3, 100140. [Google Scholar] [CrossRef]
- Hegde, N.T.; George, V.I.; Nayak, C.G.; Kumar, K. Design, Dynamic Modelling and Control of Tilt-Rotor UAVs: A Review. IJIUS 2019, 8, 143–161. [Google Scholar] [CrossRef]
- Huangzhong, P.; Ziyang, Z.; Chen, G. Tiltrotor Aircraft Attitude Control in Conversion Mode Based on Optimal Preview Control. In Proceedings of the 2014 IEEE Chinese Guidance, Navigation and Control Conference, Yantai, China, 8–10 August 2014; pp. 1544–1548. [Google Scholar]
- Tomic, L.; Cokorilo, O.; Vasov, L.; Stojiljkovic, B. ACAS Installation on Unmanned Aerial Vehicles: Effectiveness and Safety Issues. Aircr. Eng. Aerosp. Technol. 2022, 94, 1252–1262. [Google Scholar] [CrossRef]
- Peng, L.I.; Qijun, Z. CFD Calculations on the Interaction Flowfield and Aerodynamic Force of Tiltrotor/Wing in Hover. Acta Aeronaut. Astronaut. Sin. 2014. [Google Scholar] [CrossRef]
- Qi, W.; Xiang-Wei, Z. Research on Dynamics Interference between the Rotor and Wing of Tilt-Rotor Aircraft. Flight Dyn. 2013, 31, 407–410+415. [Google Scholar] [CrossRef]
- Kim, B.M.; Kim, B.S.; Kim, N.W. Trajectory Tracking Controller Design Using Neural Networks for a Tiltrotor Unmanned Aerial Vehicle. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2010, 224, 881–896. [Google Scholar] [CrossRef]
- Sun, J.; Yang, J.; Zhu, X. Robust Flight Control Law Development for Tiltrotor Conversion. Intell. Hum.-Mach. Syst. Cybern. Int. Conf. 2009, 2, 481–484. [Google Scholar] [CrossRef]
- Robust Nonlinear Adaptive Flight Control for Consistent Handling Qualities. Available online: https://ieeexplore.ieee.org/document/1522230/ (accessed on 7 November 2023).
- Maisel, M.; Harris, D. Hover Tests of the XV-15 Tilt Rotor Research Aircraft. In Proceedings of the 1st Flight Test Conference, Las Vegas, NV, USA, 11–13 November 1981; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 1981. [Google Scholar]
- Felker, F.F.; Light, J.S. Aerodynamic Interactions Between a Rotor and Wing in Hover. J. Am. Helicopter Soc. 1988, 33, 53–61. [Google Scholar] [CrossRef]
- Mcveigh, M.A.; Grauer, W.K.; Paisley, D.J. Rotor/Airframe Interactions on Tiltrotor Aircraft. J. Am. Helicopter Soc. 1988, 35, 43–51. [Google Scholar] [CrossRef]
- Felker, F.F.; Light, J.S.; Quackenbush, T.R.; Bliss, D.B. Comparisons of Predicted and Measured Rotor Performance in Hover Using a New Free Wake Analysis. 1988. Available online: https://ntrs.nasa.gov/citations/19890031562 (accessed on 29 February 2024).
- Johnson, W. Calculation of Tilt Rotor Aeroacoustic Model (TRAM DNW) Performance, Airloads, and Structural Loads. In Proceedings of the American Helicopter Society Aeromechanics Specialists’ Meeting, Atlanta, Georgia, 13–15 November 2000. [Google Scholar]
- Potsdam, M.A.; Strawn, R.C. CFD Simulations of Tiltrotor Configurations in Hover. J. Am. Helicopter Soc. 2002, 50, 82–94. [Google Scholar] [CrossRef]
- Kitaplioglu, C.; Johnson, W. Comparison of full-scale XV-15 blade-vortex interaction noise calculations with wind tunnel data. In Proceedings of the American Helicopter Society International Technical Specialist Meeting On Aerodynamics, Acoustics, and Test and Evaluation, San Francisco, CA, USA, 23–25 January 2002. [Google Scholar]
- Cambier, L.; Heib, S.; Plot, S. The Onera elsA CFD Software: Input from Research and Feedback from Industry. Mech. Ind. 2013, 14, 159–174. [Google Scholar] [CrossRef]
- Raddatz, J.; Fassbender, J.K. Block Structured Navier-Stokes Solver FLOWer. In MEGAFLOW—Numerical Flow Simulation for Aircraft Design; Kroll, N., Fassbender, J.K., Eds.; Springer: Berlin/Heidelberg, Germany, 2005; pp. 27–44. [Google Scholar]
- Biava, M.; Woodgate, M.; Barakos, G.N. Fully Implicit Discrete-Adjoint Methods for Rotorcraft Applications. AIAA J. 2016, 54, 735–749. [Google Scholar] [CrossRef]
- Tran, S.A.; Lim, J.W. Investigation of the Interactional Aerodynamics of the XV-15 Tiltrotor Aircraft; The Vertical Flight Society: Fairfax, VA, USA, 2020. [Google Scholar]
- Tran, S.; Lim, J.; Nunez, G.; Wissink, A.; Bowen-Davies, G. CFD Calculations of the XV-15 Tiltrotor during Transition; The Vertical Flight Society: Fairfax, VA, USA, 2019. [Google Scholar]
- Lim, J. Fundamental Investigation of Proprotor and Wing Interactions in Tiltrotor AIrcraft; The Vertical Flight Society: Fairfax, VA, USA, 2019. [Google Scholar]
- Lim, J.; Tran, S. Interactional Structural Loads of the XV-15 Rotor in Airplane Mode. In Proceedings of the 5th European Rotorcraft Forum, Warsaw, Poland, 17–20 September 2019. [Google Scholar]
- Stoll, A.; Milkic, G.V. Transition Performance of Tilt Propeller Aircraft; The Vertical Flight Society: Fairfax, VA, USA, 2022. [Google Scholar]
- Shi, J.; Fang, X.; Zhang, X.; Zhao, G.; Wang, B. Influence of flight speed in transition state on aerodynamic performance of tiltrotor aircraft. Flight Dyn. 2023, 41, 1–6. [Google Scholar] [CrossRef]
- Menter, F.R. Two-Equation Eddy-Viscosity Turbulence Models for Engineering Applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Le Chuiton, F. Actuator Disc Modelling for Helicopter Rotors. Aerosp. Sci. Technol. 2004, 8, 285–297. [Google Scholar] [CrossRef]
- Li, P.; Zhao, Q.; Wang, Z.; Wang, B. Highly-Efficient CFD Method for Predicting Aerodynamic Force of Tiltrotor in Conversion Mode. J. Nanjing Univ. Aeronaut. Astronaut. 2015, 47, 189–197. [Google Scholar] [CrossRef]
- Chen, G.; Chen, B.; Li, P.; Bai, P.; Ji, C. Numerical Simulation Study on Propeller Slipstream Interference of High Altitude Long Endurance Unmanned Air Vehicle. Procedia Eng. 2015, 99, 361–367. [Google Scholar] [CrossRef]
- Xiang, S.; Liu, Y.Q.; Tong, G.; Zhao, W.P.; Tong, S.X.; Li, Y.D. An Improved Propeller Design Method for the Electric Aircraft. Aerosp. Sci. Technol. 2018, 78, 488–493. [Google Scholar] [CrossRef]
- Xu, H.-Y.; Xing, S.-L.; Ye, Z.-Y.; Ma, M.-S. A Simple and Conservative Unstructured Sliding-Mesh Approach for Rotor–Fuselage Aerodynamic Interaction Simulation. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2017, 231, 163–179. [Google Scholar] [CrossRef]
- Steijl, R.; Barakos, G. Sliding Mesh Algorithm for CFD Analysis of Helicopter Rotor-Fuselage Aerodynamics. Int. J. Numer. Methods Fluids 2008, 58, 527–549. [Google Scholar] [CrossRef]
- Zhao, M.; Cao, Y. Numerical Simulation of Rotor Flow Field Based on Overset Grids and Several Spatial and Temporal Discretization Schemes. Chin. J. Aeronaut. 2012, 25, 155–163. [Google Scholar] [CrossRef]
- Ahmad, J.; Duque, E.P. Helicopter rotor blade computation in unsteady flows using moving overset grids. J. Aircr. 1996, 33, 54–60. [Google Scholar] [CrossRef]
- Mckee, J.W.; Naeseth, R.L. Experimental Investigation of the Drag of Flat Plates and Cylinders in the Slipstream of a Hovering Rotor; Technical Report Archive & Image Library; National Aeronautics and Space Administration: Greenbelt, MD, USA, 1958.


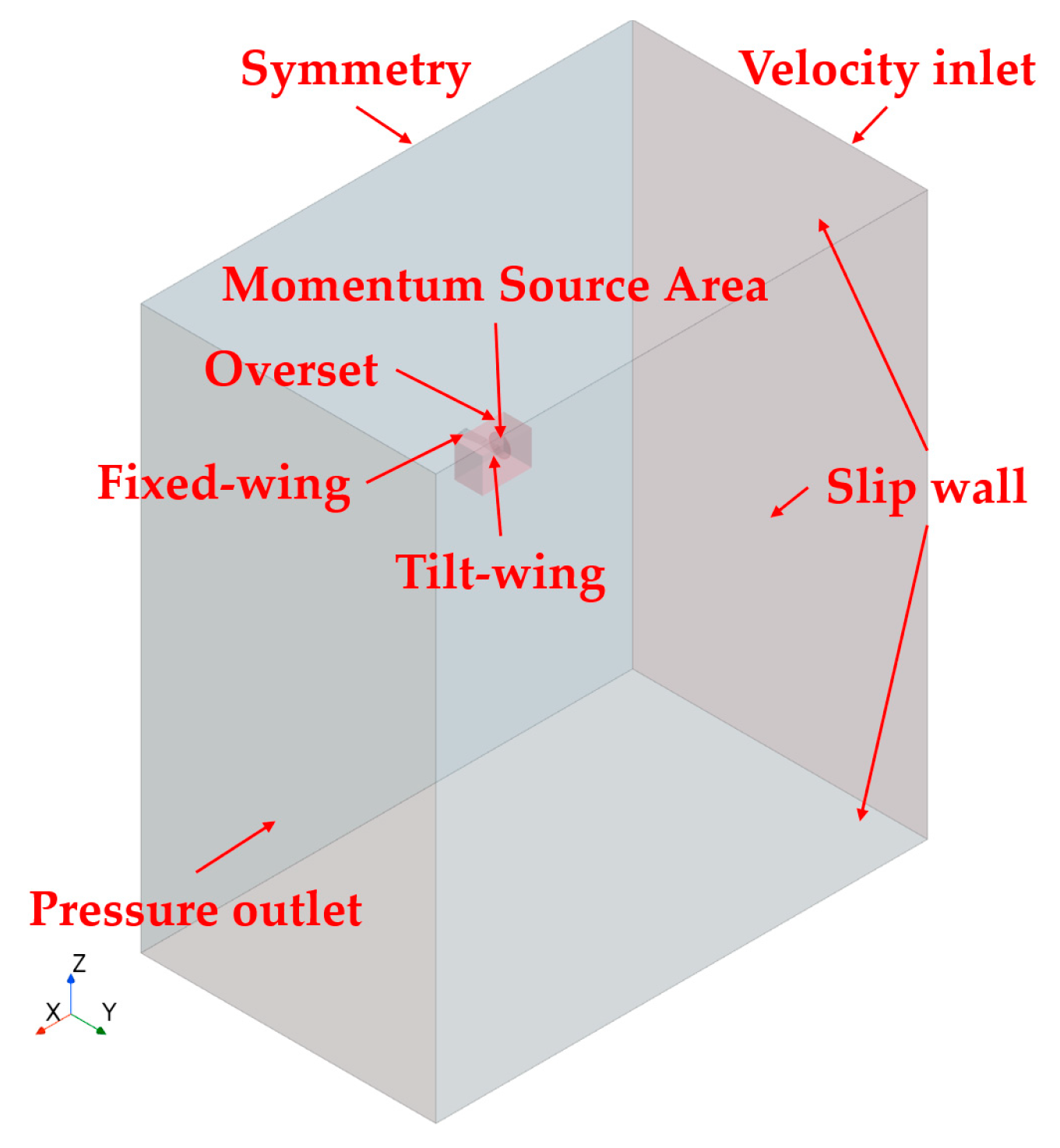
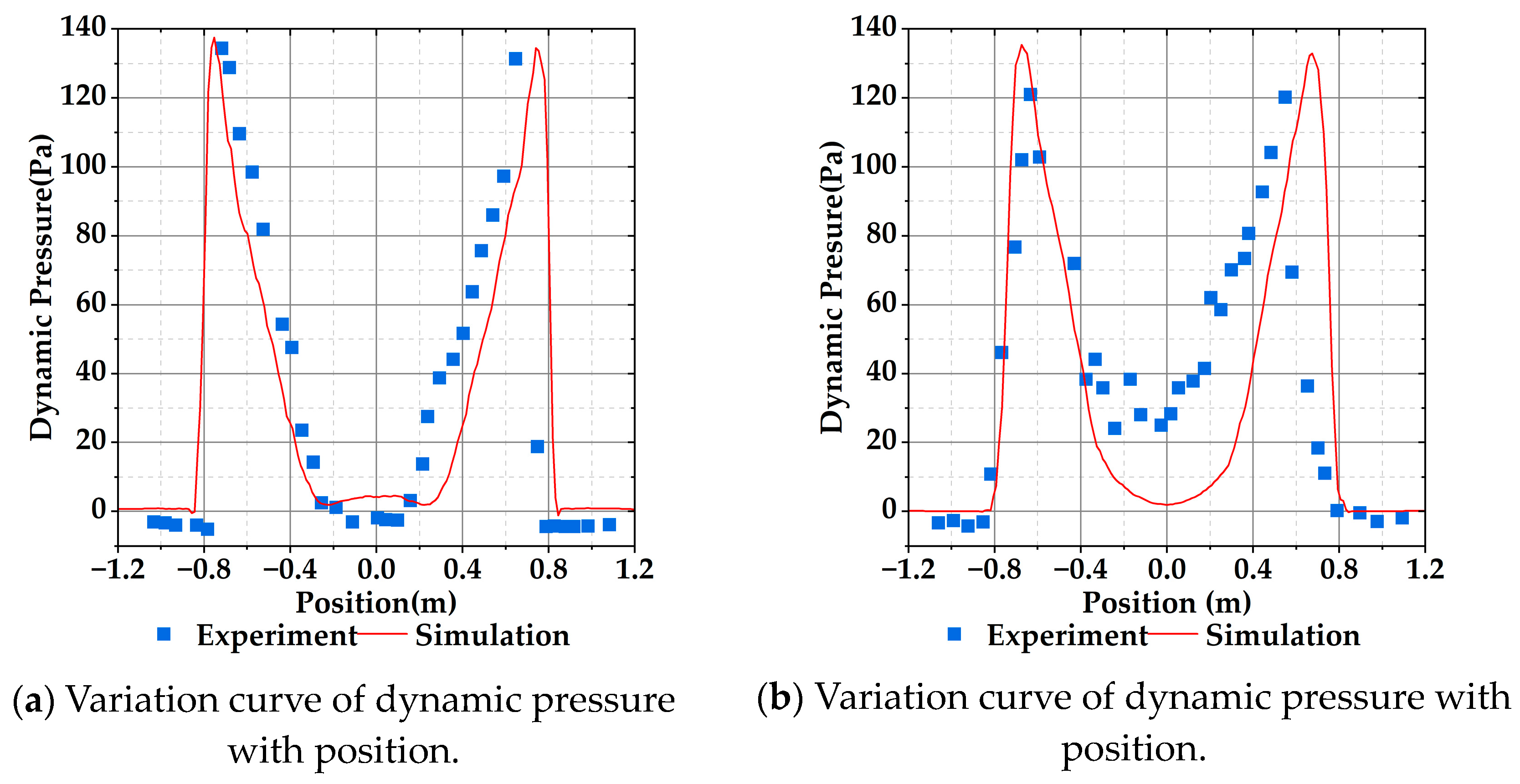
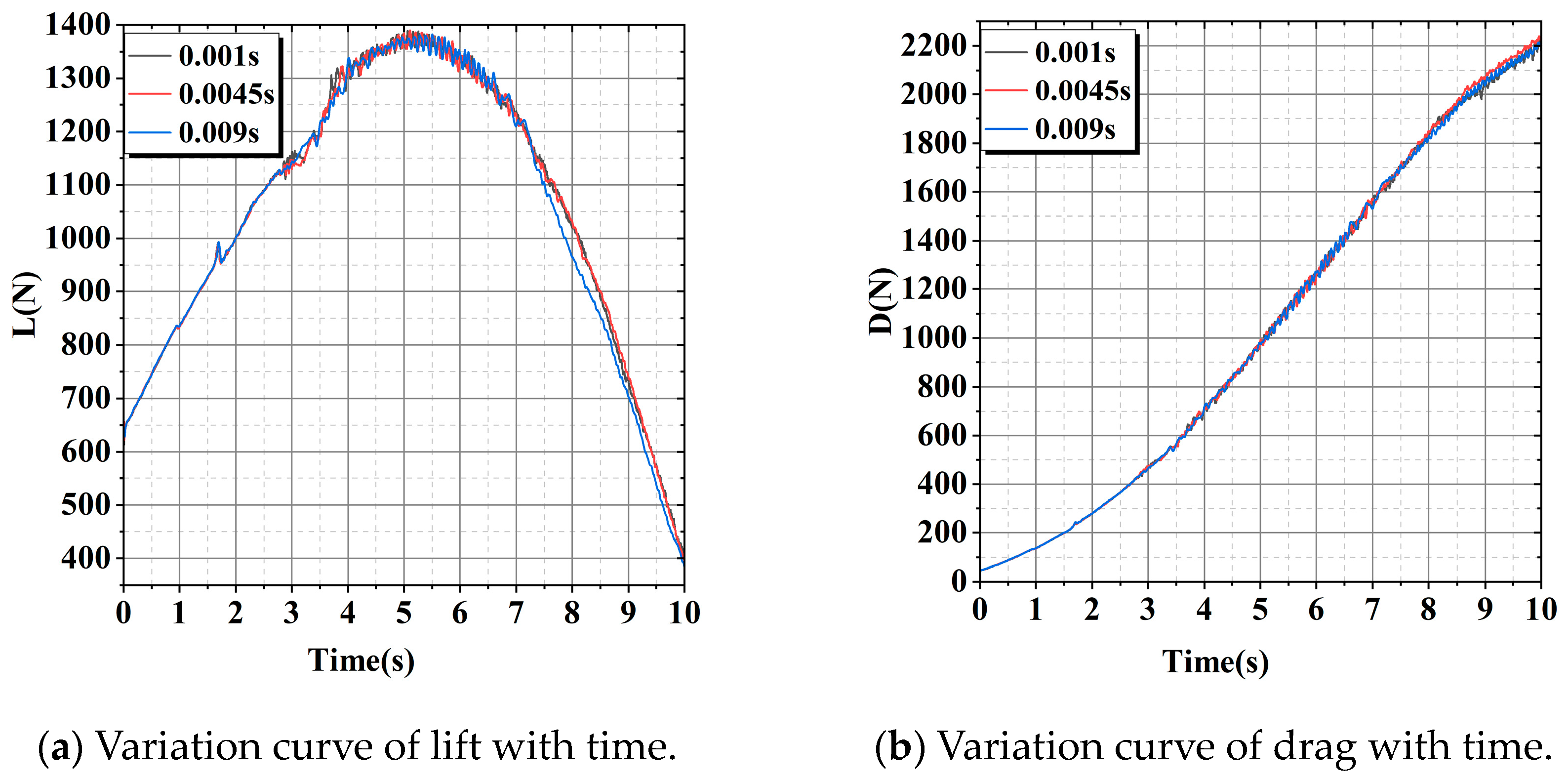
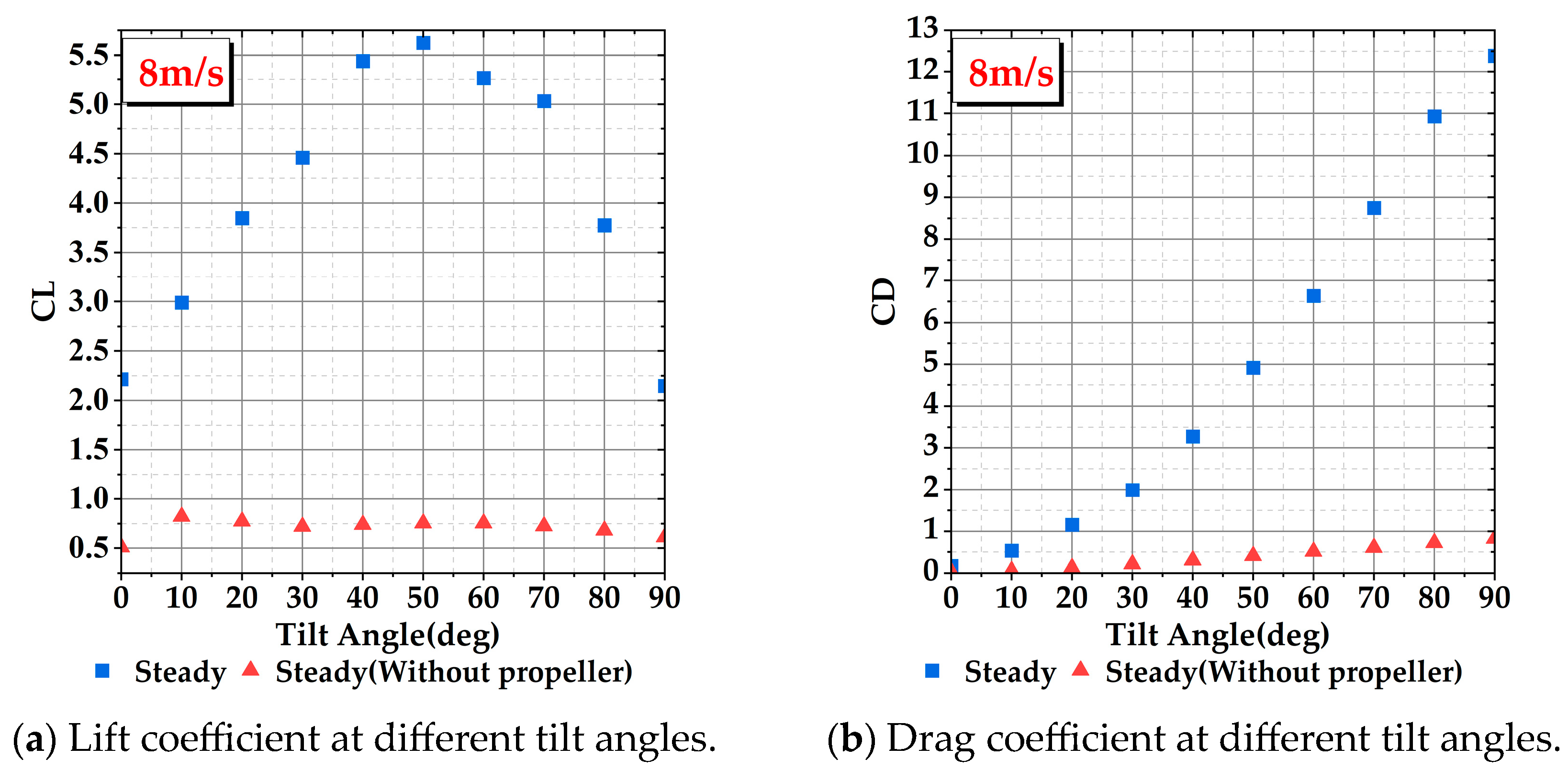





| Positioning (r/R) | Twist (deg) | Chord Length (m) |
|---|---|---|
| 0.1862 | 35.000 | 0.141 |
| 0.25 | 33.001 | 0.136 |
| 0.35 | 31.434 | 0.129 |
| 0.45 | 26.734 | 0.121 |
| 0.55 | 23.601 | 0.114 |
| 0.65 | 20.467 | 0.106 |
| 0.75 | 17.333 | 0.099 |
| 0.85 | 14.200 | 0.091 |
| 0.95 | 11.067 | 0.083 |
| 1 | 9.500 | 0.080 |
| Parameter | Data |
|---|---|
| Rotor airfoil | NACA0012 |
| Number of rotor blades | 2 |
| Rotor blade installation angle (deg) | 11 |
| Rotor radius (m) | 0.914 |
| Rotor blade chord length (m) | 0.1 |
| Rotor rotation speed (rad·s−1) | 122.2 |
| Rotor height above ground | 3.6 R |
| Calculation Method | Propeller Thrust/(N) | Lift/(N) | Drag/(N) |
|---|---|---|---|
| Multiple reference frame | 5990.522 | 575.292 | 41.140 |
| Sliding grid | 5988.837 | 584.166 | 44.316 |
| Momentum source method | 6531.764 | 591.618 | 46.331 |
| Grid Cell Number | Lift | Drag |
|---|---|---|
| 4,809,309 | 615.58 | 50.51 |
| 7,944,514 | 611.10 | 49.97 |
| 9,074,088 | 600.70 | 47.21 |
| 11,748,786 | 591.10 | 45.97 |
| 13,504,231 | 591.62 | 46.33 |
| 19,065,387 | 592.52 | 46.21 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, Q.; He, G.; Jia, J.; Hong, Z.; Yu, F. Numerical Simulation on Aerodynamic Characteristics of Transition Section of Tilt-Wing Aircraft. Aerospace 2024, 11, 283. https://doi.org/10.3390/aerospace11040283
Huang Q, He G, Jia J, Hong Z, Yu F. Numerical Simulation on Aerodynamic Characteristics of Transition Section of Tilt-Wing Aircraft. Aerospace. 2024; 11(4):283. https://doi.org/10.3390/aerospace11040283
Chicago/Turabian StyleHuang, Qingjin, Guoyi He, Jike Jia, Zhile Hong, and Feng Yu. 2024. "Numerical Simulation on Aerodynamic Characteristics of Transition Section of Tilt-Wing Aircraft" Aerospace 11, no. 4: 283. https://doi.org/10.3390/aerospace11040283
APA StyleHuang, Q., He, G., Jia, J., Hong, Z., & Yu, F. (2024). Numerical Simulation on Aerodynamic Characteristics of Transition Section of Tilt-Wing Aircraft. Aerospace, 11(4), 283. https://doi.org/10.3390/aerospace11040283






