The Linear Stability of Liquid Film with Oscillatory Gas Velocity
Abstract
1. Introduction
2. Program Formulation
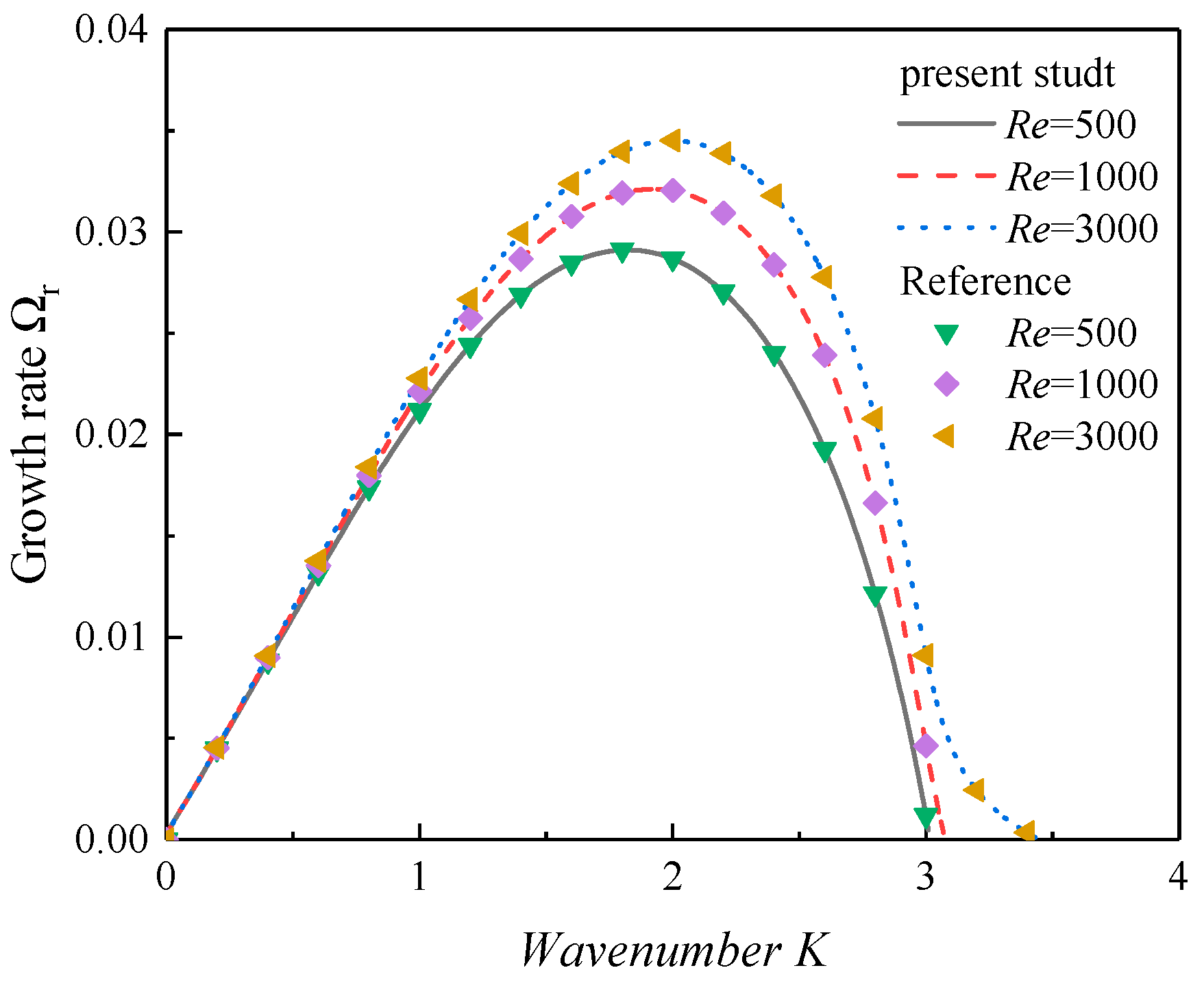
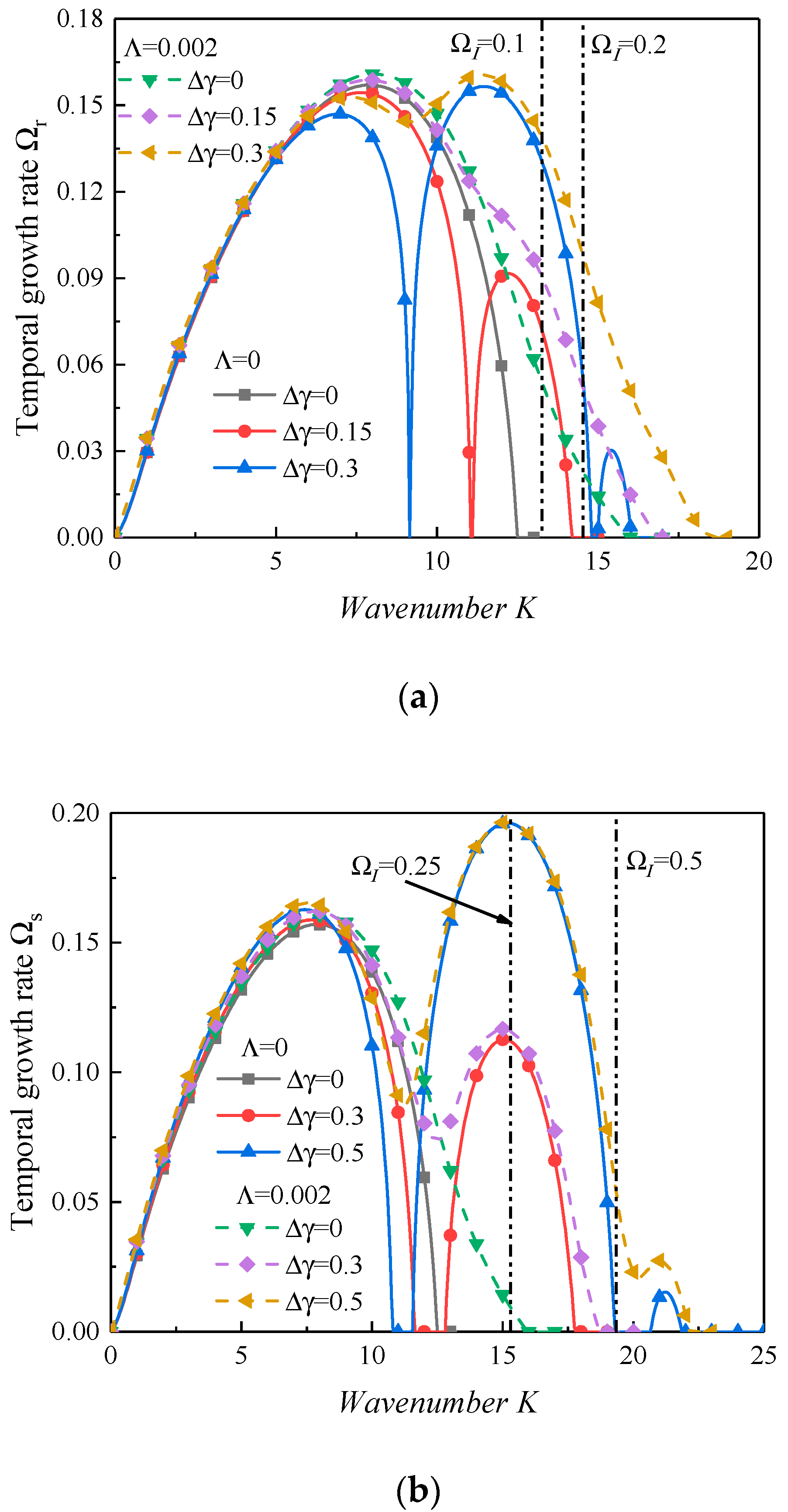
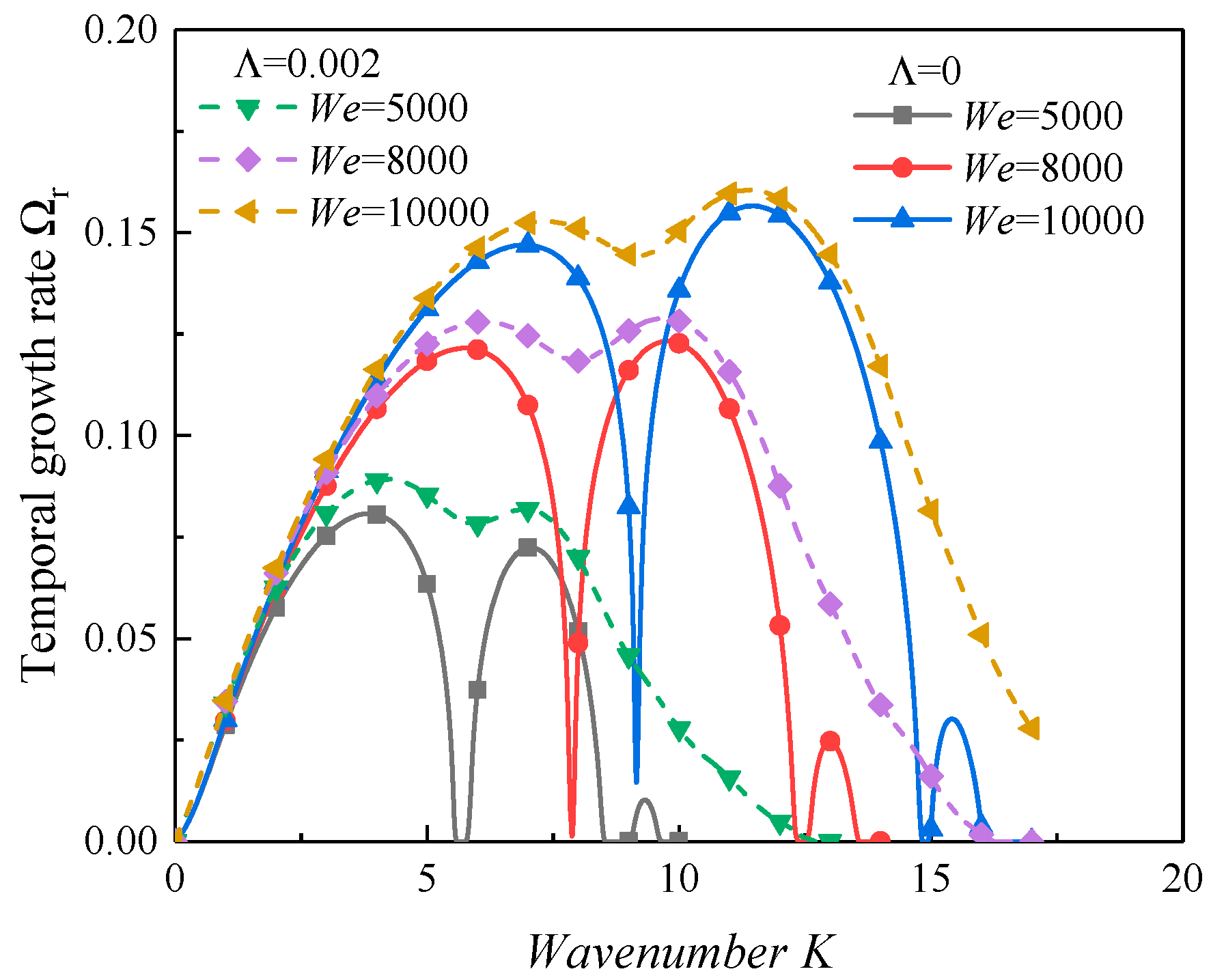
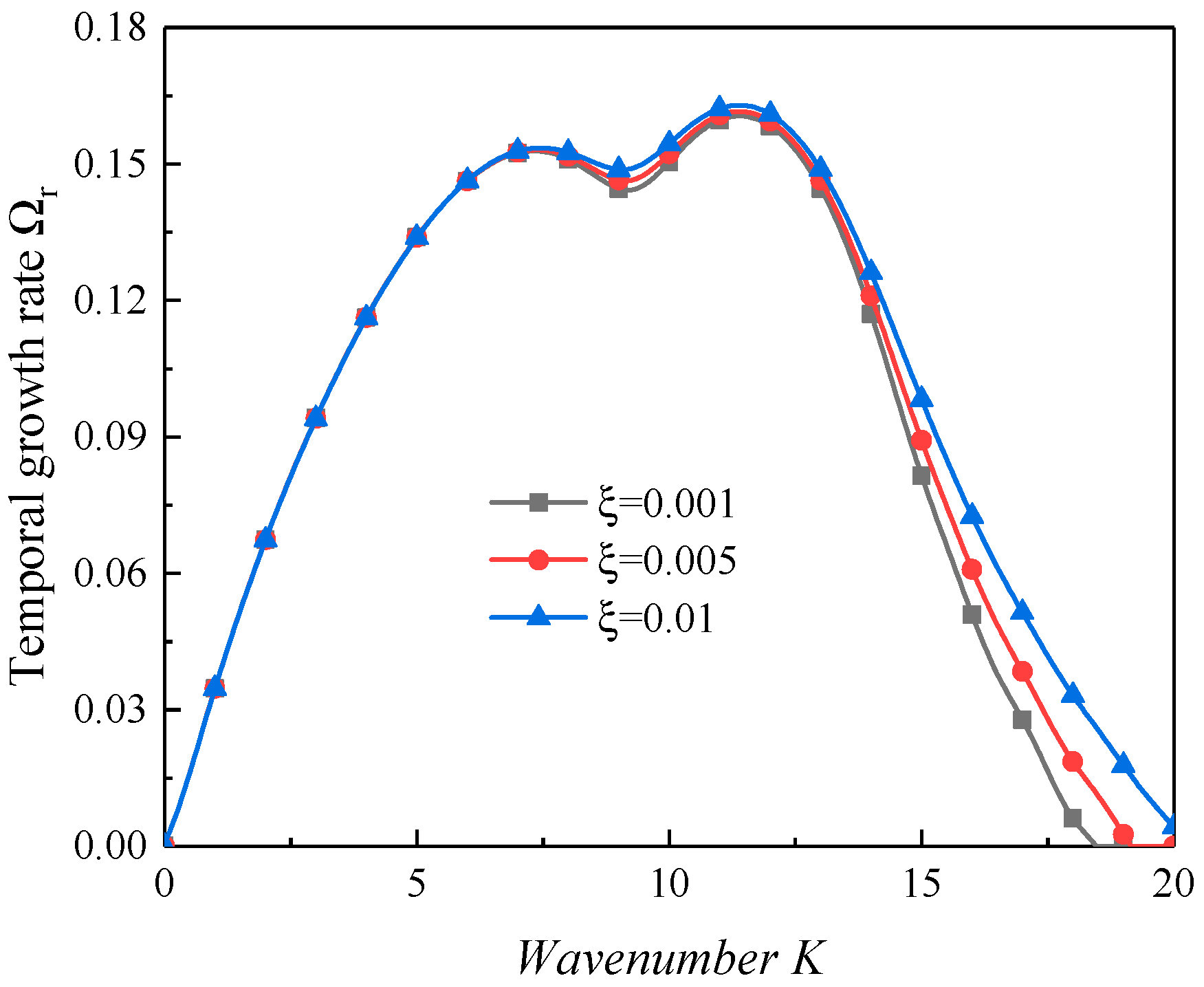
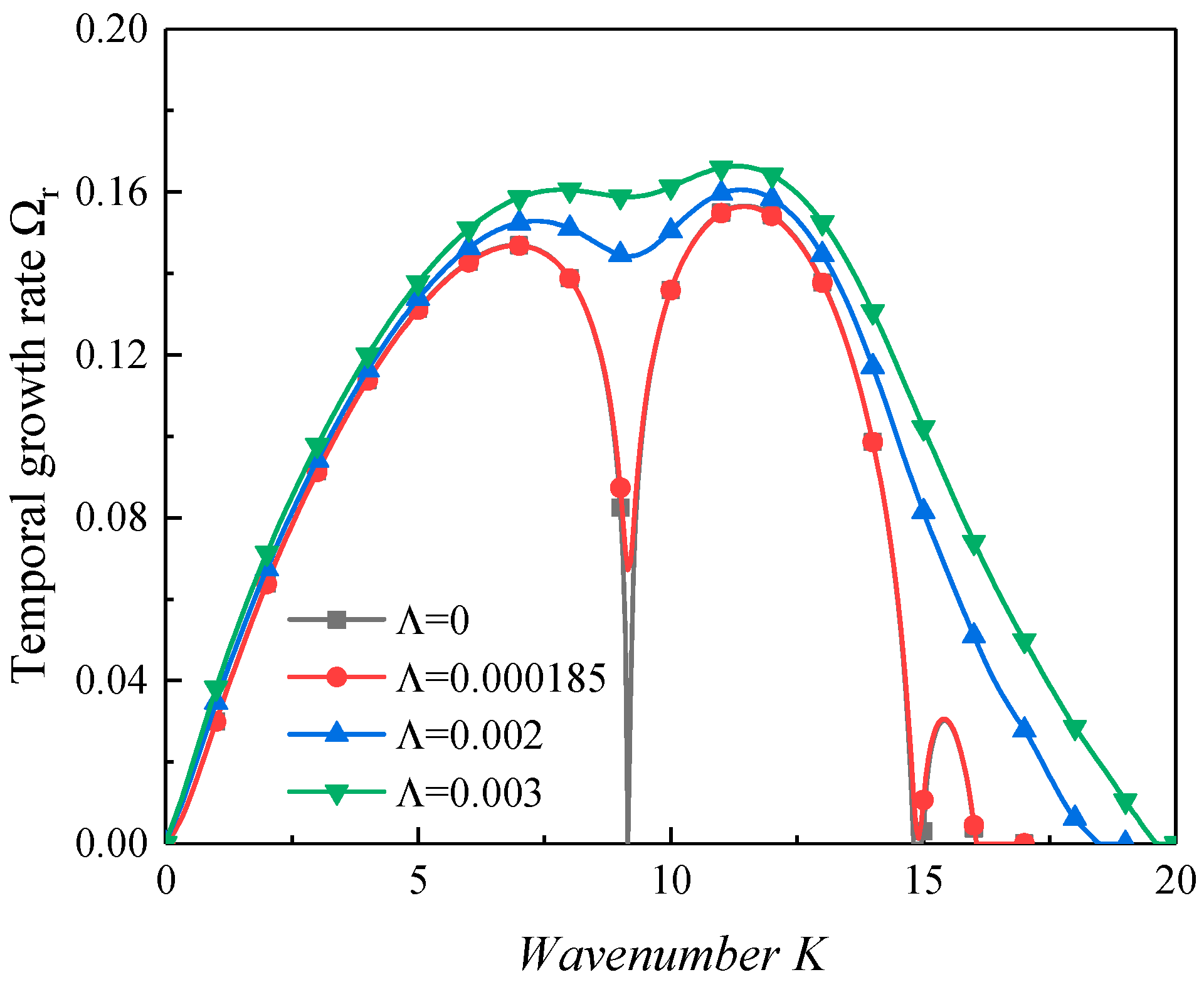
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| undetermined coefficients | |
| undetermined coefficient | |
| undetermined coefficient | |
| undetermined coefficient | |
| undetermined coefficient | |
| a function of time | |
| thickness of gas | |
| thickness of liquid | |
| wavenumber | |
| nondimensional wavenumber | |
| pressure disturbance | |
| disturbed pressure of gas | |
| disturbed pressure of liquid | |
| Reynolds number | |
| time | |
| axial gas velocity in coordinate system moving with liquid jet | |
| axial gas velocity in fixed coordinate system | |
| axial velocity of liquid | |
| axial velocity of gas | |
| axial basic velocity of liquid | |
| axial basic velocity of gas | |
| oscillations amplitude of gas velocity | |
| velocity vector | |
| wavelength | |
| Weber number | |
| thickness ratio between gas and liquid | |
| perturbation potential of gas | |
| perturbation potential of liquid | |
| nondimensional oscillations amplitude | |
| disturbed amplitude | |
| gas viscosity | |
| liquid viscosity | |
| gas–liquid density ratio | |
| gas density | |
| liquid density | |
| surface tension coefficient | |
| heat flux ratio | |
| nondimensional complex growth rate | |
| nondimensional inherent frequency | |
| nondimensional disturbance frequency | |
| nondimensional temporal growth rate | |
| nondimensional forcing oscillation frequency | |
| contribution of viscosity to the instability | |
| complex growth rate | |
| complex disturbance frequency | |
| temporal growth rate | |
| forcing oscillations frequency | |
| gas–liquid viscosity ratio |
Appendix A
Appendix B
References
- Gater, R.A.; L’Ecuyer, M.R. A Fundament Investigation of the Phenomena That Characterize Liquid-Film Cooling. Int. J. Heat Mass Transf. 1970, 13, 1925–1939. [Google Scholar] [CrossRef]
- Petrarolo, A.; Kobald, M.; Schlechtriem, S. Understanding Kelvin–Helmholtz instability in paraffin-based hybrid rocket fuels. Exp. Fluids 2018, 59, 62. [Google Scholar] [CrossRef]
- Petrarolo, A.; Kobald, M.; Schlechtriem, S. Optical analysis of the liquid layer combustion of paraffin-based hybrid rocket fuels. Acta Astronaut. 2019, 158, 313–322. [Google Scholar] [CrossRef]
- Yang, L.J.; Fu, Q.F.; Qu, Y.Y.; Gu, B.; Zhang, M.Z. Breakup of a power-law liquid sheet formed by an impinging jet injector. Int. J. Multiph. Flow 2012, 39, 37–44. [Google Scholar] [CrossRef]
- Fu, Q.F.; Yang, L.J.; Qu, Y.Y. Measurement of annular liquid film thickness in an open-end swirl injector. Aerosp. Sci. Technol. 2011, 15, 117–124. [Google Scholar] [CrossRef]
- Fu, Q.F.; Yang, L.J.; Qu, Y.Y.; Gu, B. Linear Stability Analysis of a Conical Liquid Sheet. J. Propul. Power 2010, 26, 955–968. [Google Scholar] [CrossRef]
- Fu, Q.F.; Yang, L.J.; Wang, X.D. Theoretical and Experimental Study of the Dynamics of a Liquid Swirl Injector. J. Propul. Power 2010, 26, 94–101. [Google Scholar] [CrossRef]
- Yang, L.J.; Qu, Y.Y.; Fu, Q.F.; Gu, B. Linear Stability Analysis of a Non-Newtonian Liquid Sheet. J. Propul. Power 2010, 26, 1212–1224. [Google Scholar] [CrossRef]
- Yang, L.J.; Fu, Q.F.; Qu, Y.Y.; Zhang, W.; Du, M.L.; Xu, B.R. Spray characteristics of gelled propellants in swirl injectors. Fuel 2012, 97, 253–261. [Google Scholar] [CrossRef]
- Lasheras, J.C.; Hopfinger, E.J. Liquid Jet Instability and Atomization in a Coaxial Gas Stream. Annu. Rev. Fluid Mech. 2000, 32, 275–308. [Google Scholar] [CrossRef]
- Varga, C.M.; Lasheras, J.C.; Hopfinger, E.J. Initial breakup of a small-diameter liquid jet by a high-speed gas stream. J. Fluid Mech. 2003, 497, 405–434. [Google Scholar] [CrossRef]
- Aliseda, A.; Hopfinger, E.J.; Lasheras, J.C.; Kremer, D.M.; Berchielli, A.; Connolly, E.K. Atomization of viscous and non-Newtonian liquids by a coaxial, highspeed gas jet. Experiments and droplet size modeling. Int. J. Multiphase Flow. 2008, 34, 161–175. [Google Scholar] [CrossRef]
- Mayer, E. Theory of liquid atomization in high velocity gas streams. ARS J. 1961, 31, 1783–1785. [Google Scholar]
- Qin, L.Z.; Yi, R.; Yang, L.J. Theoretical breakup model in the planar liquid sheets exposed to high-speed gas and droplet size prediction. Int. J. Multiph. Flow 2018, 98, 158–167. [Google Scholar] [CrossRef]
- Fu, Q.F.; Yao, M.W.; Yang, L.J.; Xie, L. Atomization Model of Liquid Jets Exposed to Subsonic Crossflows. AIAA J. 2020, 58, 2347–2351. [Google Scholar] [CrossRef]
- Yang, L.J.; Gao, Y.P.; Li, J.X.; Fu, Q.F. Theoretical atomization model of a coaxial gas–liquid jet. Phys. Fluids 2020, 32, 124108. [Google Scholar] [CrossRef]
- Yang, L.J.; Fu, Q.F. Stability of Confined Gas–Liquid Shear Flows in Recessed Shear Coaxial Injectors. J. Propul. Power 2012, 28, 1413–1424. [Google Scholar] [CrossRef]
- Rayana, F.B.; Cartellier, A.; Hopfinger, E.J. Assisted Atomization of a Liquid Layer: Investigation of the Parameters Affecting the Mean Drop Size Prediction. In Proceedings of the International Conference on Liquid Atomization and Spray Systems (ICLASS), Kyoto, Japan, 27 September 2006; pp. 1–8. [Google Scholar]
- Anderson, W.E.; Yang, V. Liquid Rocket Engine Combustion Instability. In Progress in Astronautics and Aeronautics; AIAA: Washington, DC, USA, 1995; pp. 1–78. [Google Scholar]
- Baillot, F.; Blaisot, J.B.; Boisdron, G.; Dumouchel, C. Behaviour of an air-assisted jet submitted to a transverse high-frequency acoustic field. J. Fluid Mech. 2003, 640, 305–342. [Google Scholar] [CrossRef]
- Ćosić, B.; Moeck, J.P.; Paschereit, C.O. Nonlinear Instability Analysis for Partially Premixed Swirl Flames. Combust. Sci. Technol. 2014, 186, 713–736. [Google Scholar] [CrossRef]
- Kheirkhaha, S.; Cirtwill, J.D.M.; Saini, P.; Venkatesanb, K.; Steinberga, A.M. Dynamics and mechanisms of pressure, heat release rate, and fuel spray coupling during intermittent thermoacoustic oscillations in a model aeronautical combustor at elevated pressure. Combust. Flame 2017, 185, 319–334. [Google Scholar] [CrossRef]
- Jia, B.Q.; Xie, L.; Cui, X.; Yang, L.J.; Fu, Q.F. Linear Stability of Confined Coaxial Jets in the Presence of Gas Velocity Oscillations with Heat and Mass Transfer. Phys. Fluids 2019, 31, 092101. [Google Scholar] [CrossRef]
- Jia, B.Q.; Xie, L.; Cui, X.; Yang, L.J.; Fu, Q.F. Linear instability of viscoelastic planar liquid sheets in the presence of gas velocity oscillations. J. Non-Newton. Fluid Mech. 2019, 273, 104169. [Google Scholar] [CrossRef]
- Jia, B.Q.; Yang, L.J.; Xie, L.; Fu, Q.F.; Cui, X. Linear stability of confined swirling annular liquid layers in the presence of gas velocity oscillations with heat and mass transfer. Int. J. Heat Mass Transf. 2019, 138, 117–125. [Google Scholar] [CrossRef]
- Guan, X.Y.; Jia, B.Q.; Yang, L.J.; Fu, Q.F. Linear instability of an annular liquid jet with gas velocity oscillations. Phys. Fluids 2021, 33, 054110. [Google Scholar] [CrossRef]
- Deng, X.D.; Jia, B.Q.; Cui, X.; Wang, N.F.; Shi, B.L. Temporal Instability of Liquid Jet in Swirling Gas with Axial Velocity Oscillations. AIAA J. 2022, 60, 3852–3862. [Google Scholar] [CrossRef]
- Deng, X.D.; Wang, H.R.; Cui, X.; Xie, L.; Jia, B.Q. Temporal instability of confined three-dimensional liquid jet with heat and mass transfer under longitudinal acoustic oscillations. Phys. Fluids 2022, 34, 102107. [Google Scholar] [CrossRef]
- Fu, Q.F.; Deng, X.D.; Yang, L.J. Kelvin–Helmholtz Instability of Confined Oldroyd-B Liquid Film with Heat and Mass Transfer. J. Non-Newton. Fluid Mech. 2019, 267, 28–34. [Google Scholar] [CrossRef]
- Fu, Q.F.; Deng, X.D.; Jia, B.Q.; Yang, L.J. Temporal instability of a confined liquid film with heat and mass transfer. AIAA J. 2018, 56, 2615–2622. [Google Scholar] [CrossRef]
- Mohanta, L.; Cheung, F.B.; Bajorek, S.M. Stability of coaxial jets confined in a tube with heat and mass transfer. Phys. A 2016, 443, 333–346. [Google Scholar] [CrossRef]
- Hsieh, D.Y. Effects of heat and mass transfer on Rayleigh-Taylor instability. J. Fluid Eng. T. ASME 1972, 94, 156–160. [Google Scholar] [CrossRef]
- Hsieh, D.Y. Interfacial stability with mass and heat transfer. Phys. Fluids 1978, 21, 745–748. [Google Scholar] [CrossRef]
- Asthana, R.; Agrawal, G.S. Viscous potential flow analysis of Kelvin–Helmholtz instability with mass transfer and vaporization. Phys. A 2007, 382, 389–404. [Google Scholar] [CrossRef]
- Asthana, R.; Agrawal, G.S. Viscous potential flow analysis of electrohydrodynamic Kelvin–Helmholtz instability with heat and mass transfer. Int. J. Eng. Sci. 2010, 48, 1925–1936. [Google Scholar] [CrossRef]
- Awasthi, M.K.; Asthana, R.; Agrawal, G.S. Pressure corrections for the potential flow analysis of Kelvin–Helmholtz instability with heat and mass transfer. Int. J. Heat Mass Transf. 2012, 55, 2345–2352. [Google Scholar] [CrossRef]
- Awasthi, M.K.; Asthana, R.; Agrawal, G.S. Viscous correction for the viscous potential flow analysis of Kelvin–Helmholtz instability of cylindrical flow with heat and mass transfer. Int. J. Heat Mass Transf. 2014, 78, 251–259. [Google Scholar] [CrossRef]
- Awasthi, M.K. Kelvin-Helmholtz instability of viscoelastic liquid-viscous gas interface with heat and mass transfer. Int. J. Therm. Sci. 2021, 161, 106710. [Google Scholar] [CrossRef]
- Moatimid, G.M.; Obied Allah, M.H.; Hassan, M.A. Kelvin-Helmholtz instability for flow in porous media under the influence of oblique magnetic fields: A viscous potential flow analysis. Phys. Plasmas 2013, 20, 102111. [Google Scholar] [CrossRef]
- Awasthi, M.K.; Asthana, R.; Uddin, Z. Nonlinear Study of Kelvin-Helmholtz instability of cylindrical flow with mass and heat transfer. Int. J. Commun. Heat Mass 2016, 71, 216–224. [Google Scholar] [CrossRef]
- Scriven, L.E.; Sternling, V.S. The Marangoni effects. Nature 1960, 187, 186–788. [Google Scholar] [CrossRef]
- Oron, A.; Deissler, R.T.; Duh, J.C. Marangoni instability in a liquid sheet. Adv. Space Res. 1995, 16, 83–86. [Google Scholar] [CrossRef]
- Dávalos-Orozco, L.A. Thermocapillar instability of liquid sheets in motion. Colloids Surf. A 1999, 157, 223–233. [Google Scholar] [CrossRef]
- Funada, T. Marangoni instability of thin liquid sheet. J. Phys. Soc. Jpn. 1986, 55, 2191–2202. [Google Scholar] [CrossRef]
- Tong, M.X.; Yang, L.J.; Fu, Q.F. Thermocapillar instability of a two-dimensional viscoelastic planar liquid sheet in surrounding gas. Phys. Fluid 2014, 26, 033105. [Google Scholar] [CrossRef]
- Zhang, S.; Lan, X.D.; Zhou, M. Thermocapillary instability of a liquid sheet with centrifugal force. J. Braz. Soc. Mech. Sci. 2018, 47, 40–47. [Google Scholar] [CrossRef]
- Hu, K.X.; He, M.; Chen, Q.S. Instability of thermocapillary liquid layers for Oldroyd-B fluid. Phys. Fluid 2016, 28, 033105. [Google Scholar] [CrossRef]
- Hu, K.X.; He, M.; Chen, Q.S.; Liu, R. Linear stability of thermocapillary liquid layers of a shear-thinning fluid. Phys. Fluid 2017, 29, 073101. [Google Scholar] [CrossRef]
- Chandrasekhar, S. Hydrodynamic and Hydro-Magnetic Stability; Dover publications, Inc.: New York, NY, USA, 1961. [Google Scholar]
- Hasegawa, K.; Manzaki, Y. Marangoni fireworks: Atomization dynamics of binary droplets on an oil pool. Phys. Fluids 2021, 33, 034124. [Google Scholar] [CrossRef]
- Moezzi, M.; Sajjadi, M.; Hossein Hejazi, S. Thermally driven Marangoni effects on the spreading dynamics of droplets. Int. J. Multiph. Flow 2023, 159, 104335. [Google Scholar] [CrossRef]
- Cui, X.; Jia, B.Q. Thermal Effect on the Instability of Annular Liquid Jet. Aerospace 2021, 8, 382. [Google Scholar] [CrossRef]
- Funada, T.; Joseph, D.D. Viscous potential flow analysis of Kelvin-Helmholtz instability in a channel. J. Fluid Mech. 2001, 445, 263–283. [Google Scholar] [CrossRef]
- Yang, L.; Zhang, Q.; Zhang, H.Q.; Wang, B. Numerical investigation on the performance of internal flow and atomization in the recessed gas-centered swirl coaxial injectors. Aerosp. Sci. Technol. 2022, 129, 107858. [Google Scholar] [CrossRef]
- Benjamin, T.B.; Ursell, F. The stability of the plane free surface of a liquid in vertical periodic motion. Proc. R. Soc. Lond. Ser. A 1954, 225, 505–515. [Google Scholar] [CrossRef]
- Kumar, K. Linear Theory of Faraday Instability in Viscous Liquids. Proc. R. Soc. A 1996, 452, 1113–1126. [Google Scholar] [CrossRef]
- Funada, T.; Joseph, D.D.; Yamashita, S. Stability of a Liquid Jet into Incompressible Gases and Liquids. Int. J. Multiph. Flow 2004, 30, 1279–1310. [Google Scholar] [CrossRef]
- Zeng, P.; Sarholz, S.; Iwainsky, C.; Binninger, B.; Peters, N.; Herrmann, M. Simulation of Primary Breakup for Diesel Spray with Phase Transition. In Recent Advances in Parallel Virtual Machine and Message Passing Interface: 16th European PVM/MPI Users’ Group Meeting, Espoo, Finland, 7–10 September 2009; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Chan, S.H.; Wang, Y.S.; Tan, C.C. The effect of mass transfer on Kelvin-Helmholtz instability at the gas-liquid interface of a sonic reacting and non-reacting gas jet submerged in a liquid. Int. J. Heat Mass Transf. 1994, 37, 1123–1132. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Deng, X.; Shi, B.; Tang, Y.; Wang, N. The Linear Stability of Liquid Film with Oscillatory Gas Velocity. Aerospace 2023, 10, 691. https://doi.org/10.3390/aerospace10080691
Deng X, Shi B, Tang Y, Wang N. The Linear Stability of Liquid Film with Oscillatory Gas Velocity. Aerospace. 2023; 10(8):691. https://doi.org/10.3390/aerospace10080691
Chicago/Turabian StyleDeng, Xiangdong, Baolu Shi, Yong Tang, and Ningfei Wang. 2023. "The Linear Stability of Liquid Film with Oscillatory Gas Velocity" Aerospace 10, no. 8: 691. https://doi.org/10.3390/aerospace10080691
APA StyleDeng, X., Shi, B., Tang, Y., & Wang, N. (2023). The Linear Stability of Liquid Film with Oscillatory Gas Velocity. Aerospace, 10(8), 691. https://doi.org/10.3390/aerospace10080691






