1. Introduction
With the deepening of human space exploration, space on-orbit missions, such as assembly and maintenance in orbit, the refueling of satellite fuel, and the recovery of failed satellites, will continue to increase. Compared with sending astronauts into space to complete these tasks, space robots have stronger adaptability to the harsh space environment and need not the life support system needed to maintain the survival of astronauts. Their long working hours and high efficiency can greatly save the cost of space exploration and perform more complex tasks [
1,
2,
3,
4]. Therefore, it is of great significance to do research on space robots [
5,
6,
7,
8].
To date, there has been a clear trend towards miniaturization of spacecrafts launched into orbit. Considering the carrying capacity of rockets and the effective utilization of cabin space, the micro spacecrafts are usually modular packaged on the ground and assembled on-orbit after being launched into space. The on-orbit operation of insertion and extraction is the key technology for performing these space tasks. However, during the inserting and extracting process, the replacement part at the end of the manipulator will inevitably contact and collide with the external environment. The huge impact force will easily cause damage to the replacement part, the micro spacecraft and even the robot when appropriate control strategies are not implemented. Compared with the robot with a fixed base, the space robot with a free-floating carrier has six more degrees of freedom of motion. There is a strong dynamic coupling between the manipulator and the carrier, and its structure becomes complicated. When the manipulator moves, it will exert a dynamic force or moment on the carrier to change the position and attitude of the carrier, which further increases the difficulty of realizing the space robot’s on-orbit operation of insertion and extraction. For the study of compliance control during contact and collision, the impedance control strategy proposed by Hogan [
9] can establish a dynamic relationship between the end posture and output force by adjusting the impedance parameters, which has been widely used in handling the contact and collision problems between robots and the external environment.
In recent years, in the field of aviation, especially in the field of space robot on-orbit operation, many experts and scholars have considered the impedance control strategy in the problem of on-orbit operation and carried out much research on it. Uyama et al. [
10] proposed an impedance control strategy based on the recovery coefficient to deal with the contact force between a free-floating space robot and a target satellite and verified the effectiveness of the proposed strategy through ground experiments. Flores-Abad et al. [
11] use interference observers to estimate the contact force between the robotic arm and the target satellite and to adjust the input of the impedance controller through the matching degree between the actual contact force and the estimated contact force to achieve the safe capture of the target satellite by the space robot. Ge et al. [
12] designed a coordinated impedance control strategy to coordinate the contact force between the end effector and the target satellite by applying a reference impedance for the coordinated control problem of a multi-arm space robot capturing the target satellite.
To sum up, these scholars have achieved the flexibility of the process of contact and collision between the space robot and the external environment. However, they have not accurately controlled the manipulator end output force at some fixed values. Considering that in the process of on-orbit insertion and extraction of a space robot, it is not only necessary to avoid severe contact and collision but also to accurately control the output force of the replacement component. If the output force is less than the frictional resistance, it is difficult for the insertion and extraction operation to proceed smoothly; If the output force is too large, it is easy to cause damage to the replacement parts. Generally, the output force control accuracy should be better than 1 N. In order to prevent severe contact and collision between the plug and the hole due to the similar size of the two and trajectory control accuracy issues, some high requirements are called for the control of the carrier attitude and the plug posture during the insertion and extraction operation. The control accuracy of the general position should be better than m, and the control accuracy of the posture should be better than rad. In general, it is difficult to accurately determine the system parameters of space robots due to the constant reduction in the mass caused by the fuel consumption of the robot carrier, the huge temperature difference between the positive side and the negative side of the robot arm leading to the center of mass deviation of the robot arm, the inconsistent quality of different replacement parts and other factors. The modeling uncertainties mentioned above will seriously affect the position and pose control precision of the space robot and bring additional interference to the on-orbit insertion and extraction operation.
RBFNN is a feedforward neural network with excellent performance, which can approximate nonlinear functions with arbitrary accuracy and fundamentally solve the local optimal problem of Back Propagation Neural Network (BPNN). RBF neural network consists of three layers: namely input layer, hidden layer and output layer. The transformation from the input layer to the hidden layer is nonlinear, while the transformation from the hidden layer to the output layer is linear. Since there is only one hidden layer, it has good global approximation ability, strong robustness, high memory ability, superior nonlinear mapping ability and self-learning ability. Therefore, it has attracted the attention of many scholars [
13,
14,
15,
16,
17,
18]. In order to achieve accurate force/posture control for on-orbit insertion and extraction of space robots, an adaptive RBFNN impedance control strategy is proposed by combining RBFNN and impedance control principles. To the best of the authors’ knowledge, no one has paid much attention to the application of neural networks in the operation of on-orbit insertion and extraction of space robots. Therefore, this paper considers introducing RBFNN to achieve accurate force/pose control of on-orbit insertion and extraction operation of space robots.
In this paper, the force/posture control of space robots’ on-orbit operation of insertion and extraction is studied. Based on the principle of momentum conservation, a system dynamics model in the form of uncontrolled carrier position and controlled attitude is obtained. Additionally, a second-order linear impedance control model is established by the dynamic relationship between the end force and the position of the space robot. By combining impedance control principle with RBFNN, an adaptive RBFNN impedance control strategy is designed, which has good stability, strong robustness, and high control accuracy for force and posture. The simulation results show that under the action of this control strategy, the space robot can not only realize the operation of in-orbit insertion and extraction but also meet the requirement of the control precision of the mission design, especially in the aspect of the impedance output force of the manipulator end, the control precision reaches 0.5 N.
2. Dynamics and Kinematics Modeling
2.1. System Dynamics Model
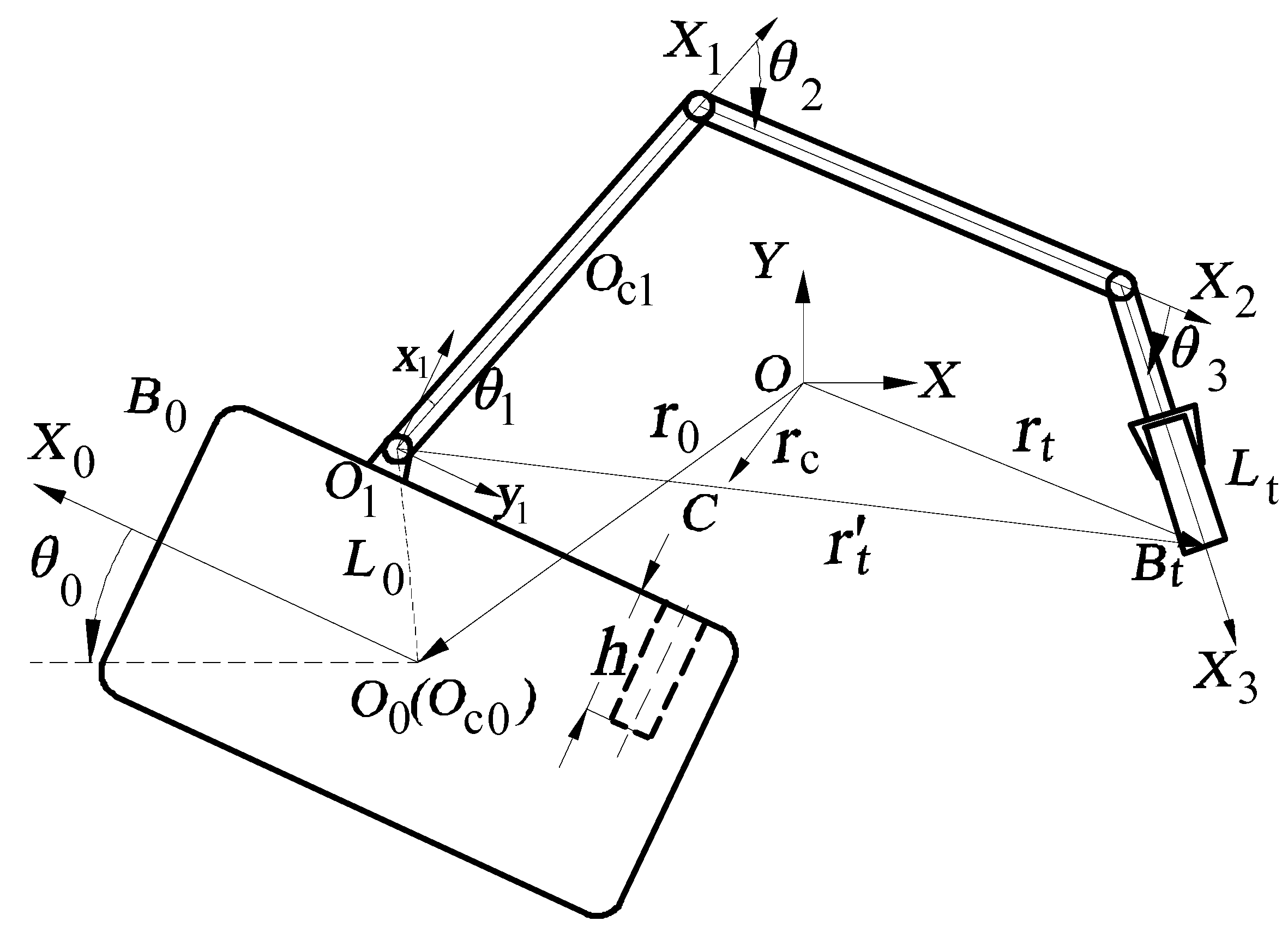
The on-orbit operation of on-orbit insertion and extraction space robots is shown in
Figure 1. Among them,
are the center of mass of the space robot carrier and the center of each joint hinge,
is the end point of the replacement component plug,
are the unit vector of each split spindle of the space robot.
is the unit vector of the main axes of each partition of the space robot.
are the coordinate system connected to the main axis of each partition.
The symbols used in the text are defined as follows: are, respectively, the mass of the carrier, the moment of inertia, and the distances from to ; are, respectively, the mass, moment of inertia, and arm length of the ith robotic arm; are, respectively, the mass, moment of inertia, and length of replace component; are, respectively, the distance between the ith joint hinge center and the center of mass of the robotic arm ; is the depth of the inserting hole; are, respectively, the carrier attitude angle and manipulator angle.
Generally, the dynamic model of a space robot shown in
Figure 1 is:
where,
is the symmetric and positive definite inertia matrix of the space robot system,
is column vector containing Coriolis force and centrifugal force;
is the generalized coordinate of the space robot system,
is the column vector for the angle between the carrier and the manipulator;
is the vector of system control torque,
is the vector of carrier position control torque, and
is the vector of carrier attitude and joint control torque.
It is assumed that the hole is on the carrier during the on-orbit insertion and extraction operation, so the plug moves relative to the carrier. This process only requires controlling the attitude of the carrier and does not require controlling the position of the carrier. Therefore, Equation (1) is divided into the following blocks:
By integrating the first row obtained by multiplying Equation (2) and combining the momentum conservation relationship of the system, a dynamic model of a space robot system in the form of uncontrolled carrier position and controlled attitude can be obtained:
where
,
.
2.2. Replacement Component Kinematics Model
Since it is necessary to precisely control the motion trajectory of the plug relative to the carrier during the on-orbit insertion and extraction process, it is proposed to establish the motion relationship between the point
of the plug end and the carrier. By projecting the position of point
relative to
on the base coordinate system
, it can be obtained that:
where,
,
are the position of
in
and
.
By derivation of Equation (4), the kinematic relation of the point
with respect to the carrier can be written:
where
,
is the augmented motion Jacobian matrix of the plug end
point relative to the carrier.
2.3. Impedance Modeling
Due to the accuracy of the position control of the carrier and the plug, the plug will inevitably be subjected to friction resistance from the hole during the on-orbit operation of the space robot. The plug cannot be inserted or pulled out of the hole when the output force of the plug is too small, and it is easy to cause damage to the replacement parts while the output force of the plug is too large. Therefore, in order to smoothly implement the on-orbit insertion and extraction operation, it is necessary not only to accurately control the position of the plug, but also to accurately control the output force of the plug. Impedance control can accommodate force and posture into the same framework based on the impedance relationship model and can maintain an ideal dynamic relationship between the plug posture and environmental contact forces by adjusting impedance parameters. Considering the force/posture control requirements of space robots during insertion and extraction, impedance control is applied to this operation.
Generally, the mathematical model of plug impedance relationship can be expressed in the form of a second-order differential equation, and the environmental model can be approximated in the form of a second-order nonlinear function:
where
,
are, respectively, expected position and reference position of the plug;
,
,
are the inertia matrix, damping matrix and stiffness matrix of the manipulator, respectively;
,
are environmental damping matrix and stiffness matrix, respectively;
,
are, respectively, the plug output force/moment and contact force/moment.
According to Equation (6), the error between the output force/moment of the plug and the contact force/moment can be calculated as:
3. Controller Design
Since the on-orbit operation of inserting and extracting requires precise control of the position and posture of the plug, the dynamics model of the space robot system shown in Equation (3) is established based on the joint reference space. In order to control the position and posture of the plug more directly, it needs to be converted to the inertial space.
According to Equation (5), it can be obtained that:
By substituting Equation (8) into Equation (3), the dynamics model of space robot system based on inertial reference space can be written as:
where
,
,
.
According to references [
19,
20], there is:
Property 1. The matrix of is oblique symmetry, that is, for any , there are:.
The position and speed errors of the system are defined as:
Based on Equation (10), a linear error function in the following form is defined:
where,
are both the symmetric positive definite gain matrices.
According to Equation (11), it can be obtained that:
Due to carrier fuel consumption, changes in the position of the manipulator’s center of mass, inconsistent quality of replacement parts, etc., the parameters of the space robot system are often unable to be accurately obtained. Generally, the uncertain parameters of the system need to be sorted out as follows:
Since the Gaussian function tends to zero at infinity, that is, the action function is local. Therefore, RBFNN has the advantages of fast convergence, good stability, unique approximation and no local minimum. Based on the above analysis, RBFNN is used to estimate the instability parameters of the system, which can be obtained:
where
is the input of RBFNN,
is the ideal weight matrix of the network,
is the Gaussian base function, and
.
p is the number of neurons in a hidden layer of the network,
,
are the center value and width of the Gaussian base function, and
is the approximation error of uncertain parameters.
Assumption 1. The approximation error of the neural network to the uncertain term is bounded, namely: .
Since the ideal weight matrix
exists, however, its true value is unknown, then the estimated value of the uncertain parameter can be expressed as:
where
is the estimated value of
, and the error between the ideal weight and the estimated weight is defined as
.
The neural network update rate is designed as the following form:
where
and
.
Based on Equations (11)–(15), the control law is designed as the following form:
By substituting Equations (13) and (17) into Equation (12), it can be obtained:
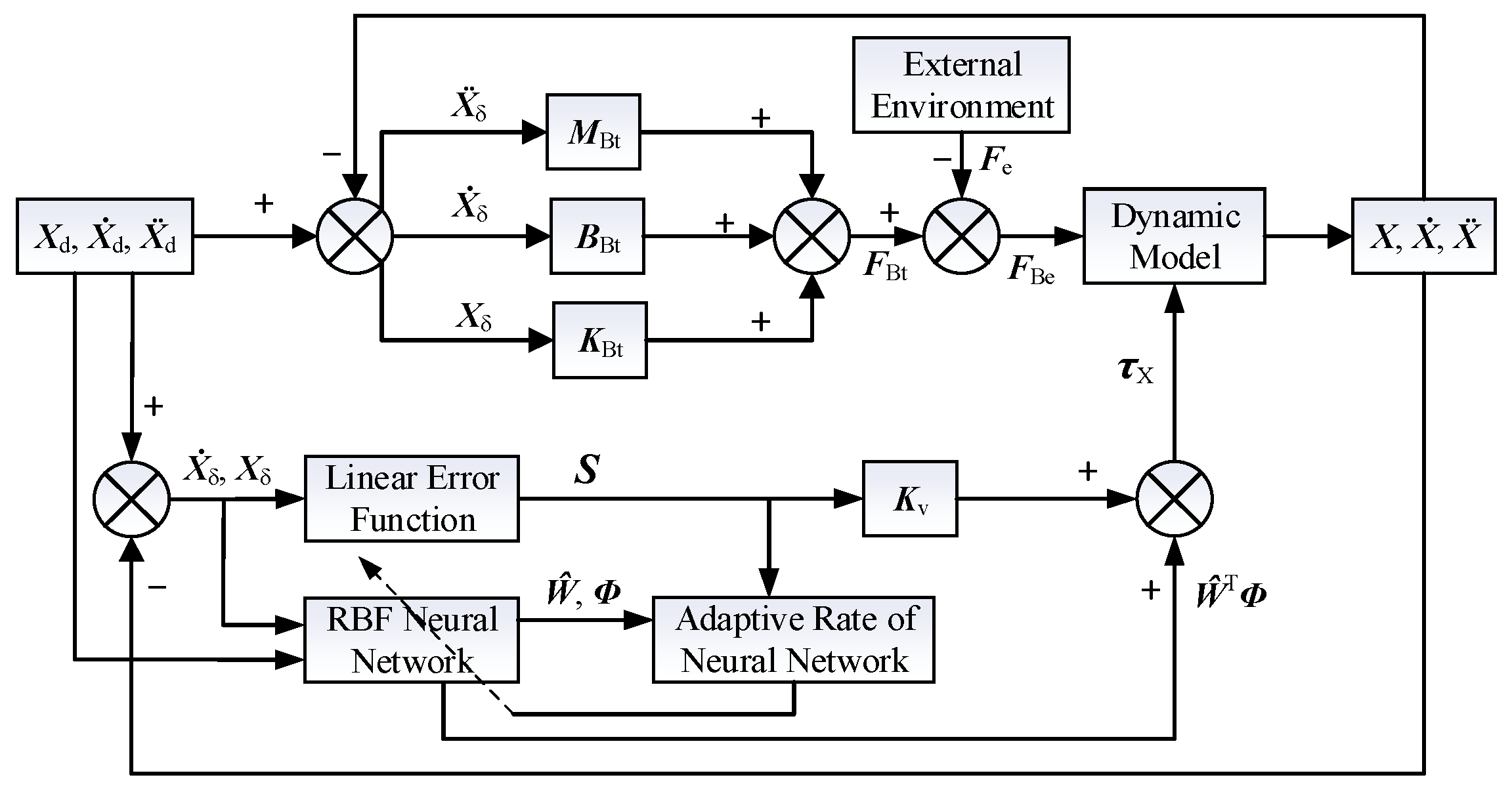
In summary, as for the design of impedance control and neural network control, the system control block diagram is shown in
Figure 2.
5. Simulation Analysis
5.1. Details and Results of Insertion Simulation of Constant Frictional Resistance
The space robot shown in
Figure 1 is used for simulation analysis. Additionally, the dynamic parameters of the system are shown in the
Table 1.
The control parameters of the controller and the neural network can be seen in
Table 2. Additionally, the simulation time is 15 s.
It is assumed that during the insertion process, the friction force applied to the plug of the replacement component remains constant and is set to ; The inserting hole is located on the front of the carrier, and its position relative to O1 is , ; the position of the end of replaceable component relative to O1 is , , also called the initial position.
In order to ensure a precise force/position control of the on-orbit operating, the whole process was divided into three stages:
Stage 1 (
): Preparation stage. The impedance control is turned off. The manipulator end and the plug of the replacement part are controlled to move to the desired position from the initial position. The attitude of the space robot carrier and the plug are adjusted so that the plug moves directly above the carrier hole and the orientation of the plug is consistent with that of the hole.
where the first element of
is the expected attitude of the carrier, and the second through fourth elements are the expected trajectory and attitude of the plug end points of the replacement parts, respectively. The unit of carrier and plug attitude is in rad, the unit of plug position is m, the unit of plug output force is N, and the unit of time is s.
Stage 2 (
): Closing stage. The force/pose impedance control is turned on and the output force is preloaded. The position of the end of the manipulator in the X-direction (also the position of the hole) remains unchanged, and the movement in the Y-direction just above the hole surface is maintained. Keeping the attitude of the space robot base 0 rad and the attitude at the end of the robot arm 3.14 rad to make them perpendicular to each other:
Stage 3 (
): Inserting stage. The impedance control enables the plug to overcome the frictional resistance of the carrier hole along the desired trajectory to complete the insertion operation:
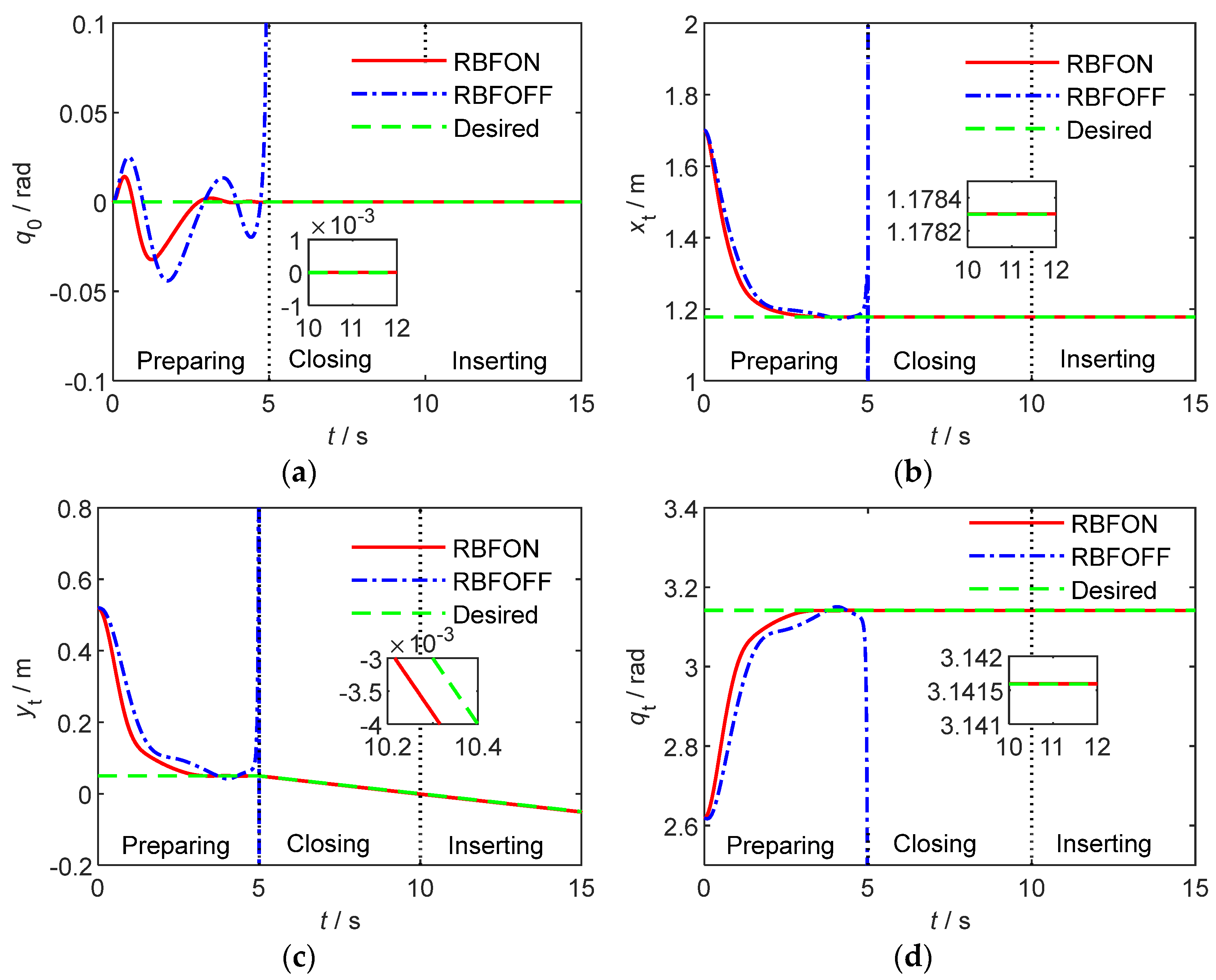
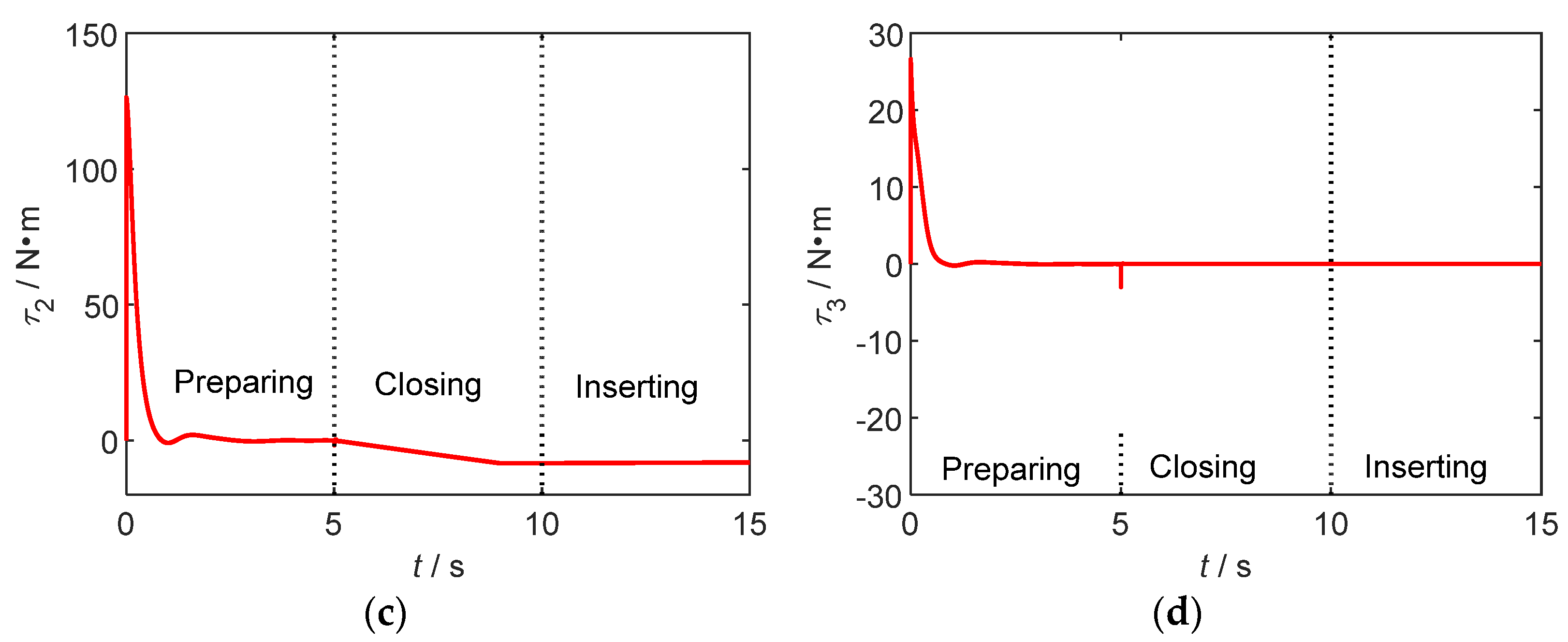
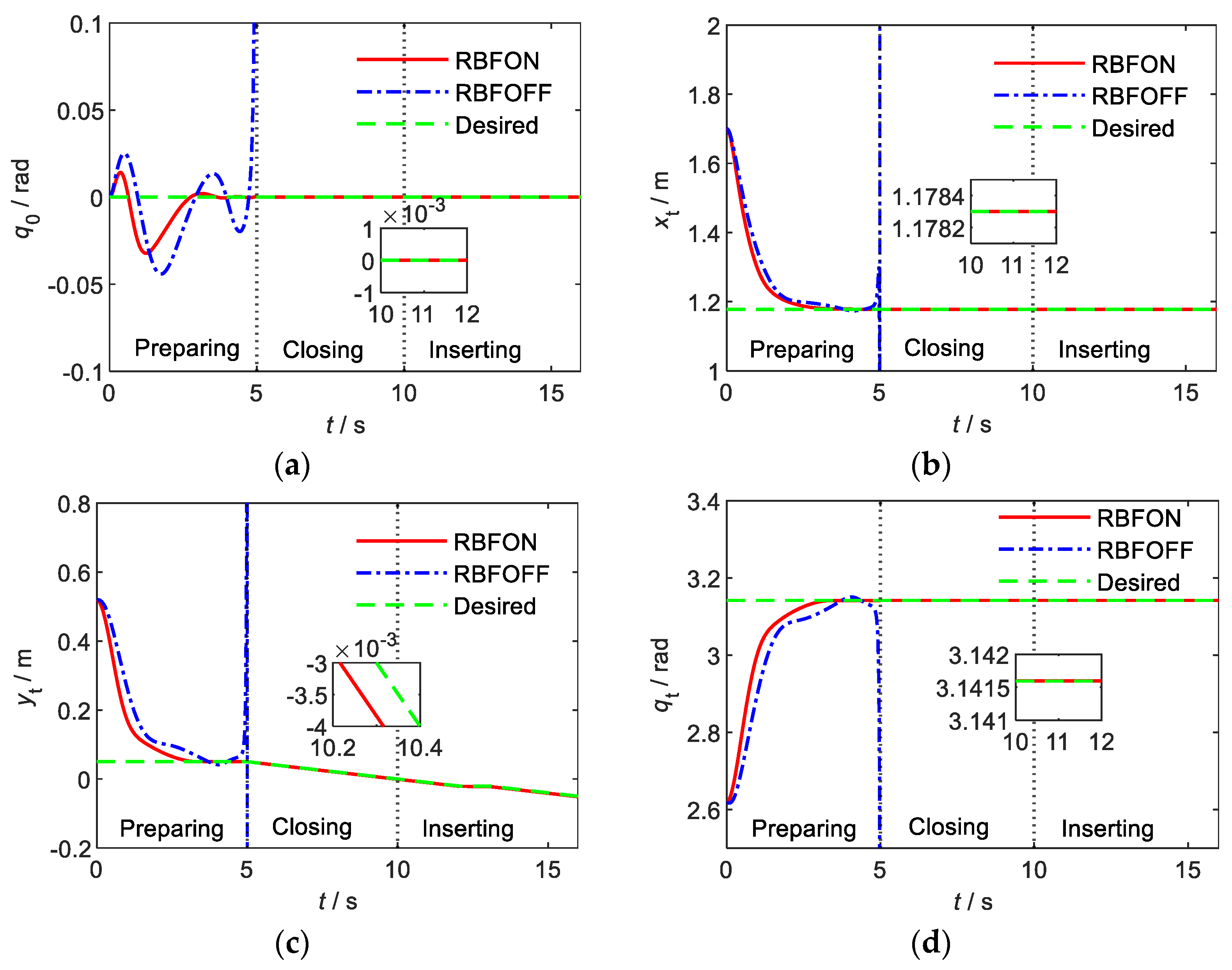
According to
Figure 3a,d, the proposed control algorithm can realize the smooth transition of carrier and plug attitude in the three stages with good stability and better control accuracy than
rad. In accordance with
Figure 3b,c, the position control of the plug is stable, the convergence speed is fast, and the control accuracy is better than
m. From the comparison results in
Figure 3, it is obvious that after turning off the RBFNN, the space robot cannot complete the on-orbit insertion due to error accumulation and other factors.
As can be seen from
Figure 4, the designed neural network impedance control strategy can realize real-time tracking of the output force, and the control accuracy is better than 0.5 N. Since the attitude of the carrier, the attitude of the plug and the control accuracy of the trajectory meet the high requirements, there is no collision between the plug and the carrier resulting in a huge impact force in the third stage.
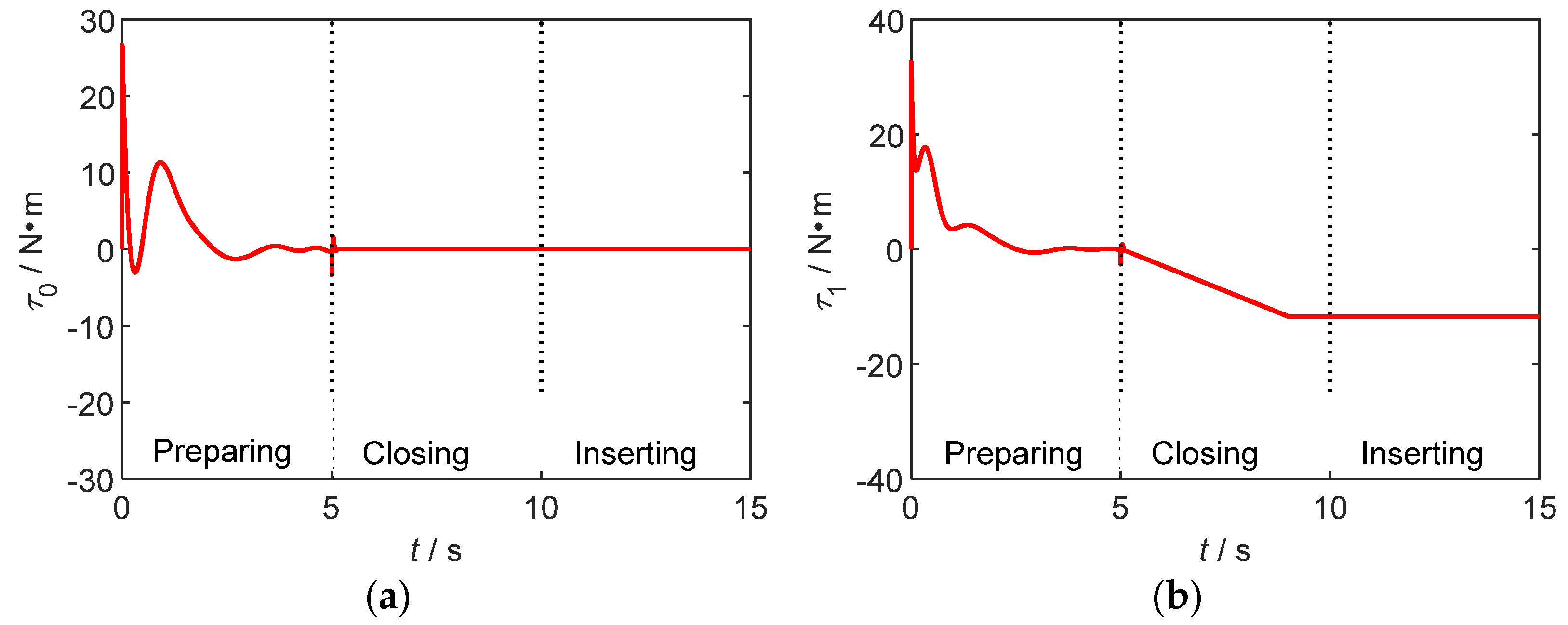
Figure 5 shows the control torques of the base and each joint of space robot.
The tracking errors of the manipulator end of the proposed algorithm are compared with the tracking error based on the preset performance control (PPC) method in the literature [
20] and are listed in the following table.
Table 3 in the form of percentage. And, the up arrow means that the RBF control accuracy is increased relative to PPC while the down arrow means reduced.
The comparison results illustrate that the absolute values of the above errors meet the requirements of task design. Meanwhile, the dynamic relationship between the force/position errors can be obtained by adjusting impedance parameters under impedance control. Therefore, the percentage of error comparison results are also acceptable.
5.2. Details and Results of Insertion Simulation of Frictional Resistance with Sudden Change
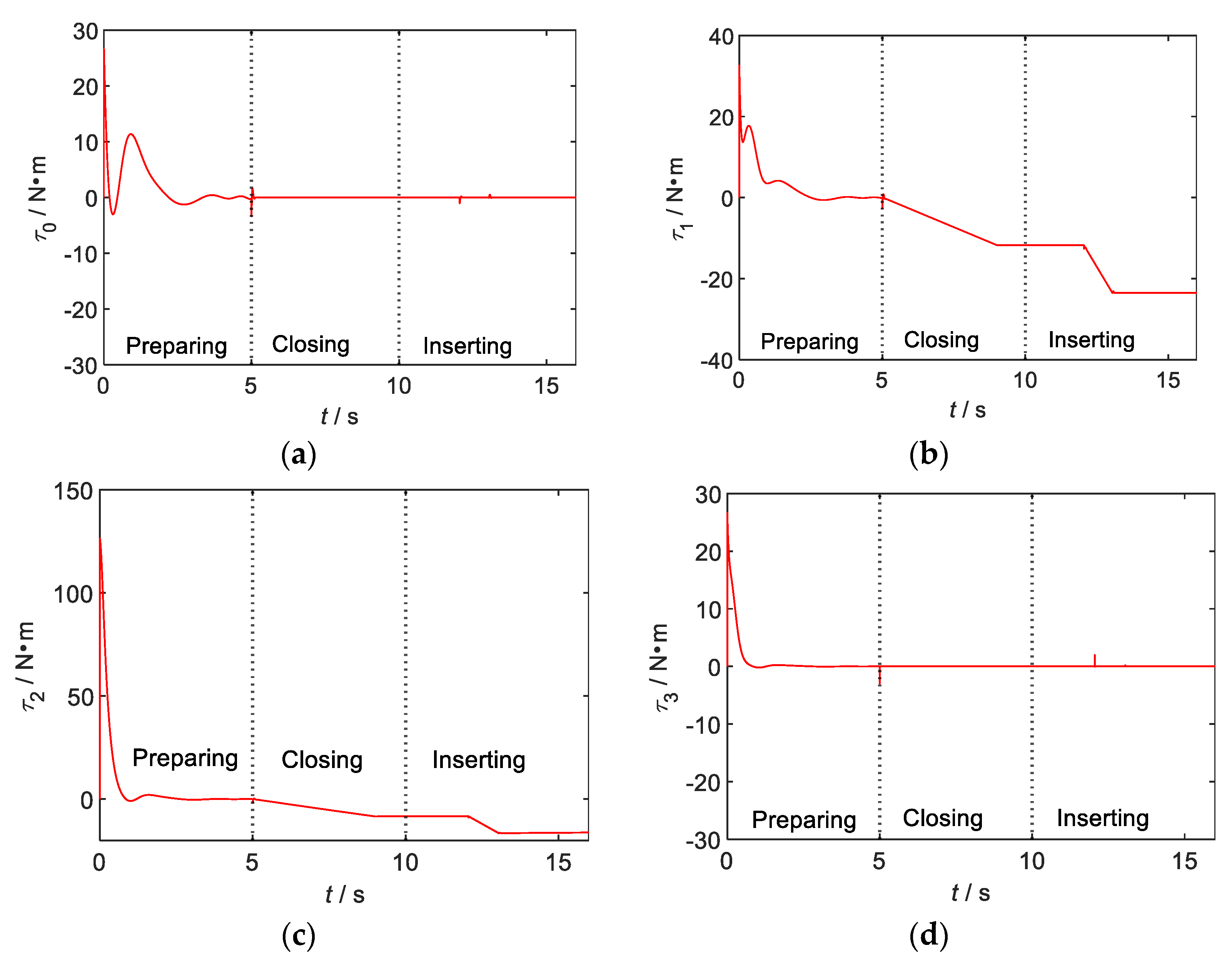
Considering the possibility of sudden change in friction resistance in the actual insertion process, that is, there is stuck phenomenon. Therefore, it is assumed that when the inserting operation is reached at , the friction resistance changes to . At this time, it is necessary to increase the output force of the plug to slightly greater than the friction resistance to continue to complete the operation.
Keep the first two stages unchanged and modify the third stage as follows:
Stage 3 (
): Inserting stage. The impedance control enables the plug to overcome the frictional resistance of the carrier hole along the desired trajectory to complete the insertion operation. Then, at the moment of
, the friction suddenly increases, the blockage appears, and the insertion movement stops. Subsequently, the end of the manipulator starts to increase the output force. Until 1s later, the end output force is greater than the friction force again, and the on-orbit insertion operation will be continued:
As can be seen from
Figure 6 and
Figure 7, the designed control algorithm can stably control the posture of the carrier and plug even when the friction resistance changes, so that no changes will occur.
According to these results, when there is stuck, the position and pose of the plug remain relatively static. After the impedance control increases the output force of the plug to the expected value, the space robot re-executes the insertion operation and successfully completes the tasks.
Additionally, the comparisons of tracking errors are listed in
Table 4.
Figure 8 shows the control torques of the base and each joint of space robot.
5.3. Details and Results of Extraction Simulation of Constant Frictional Resistance
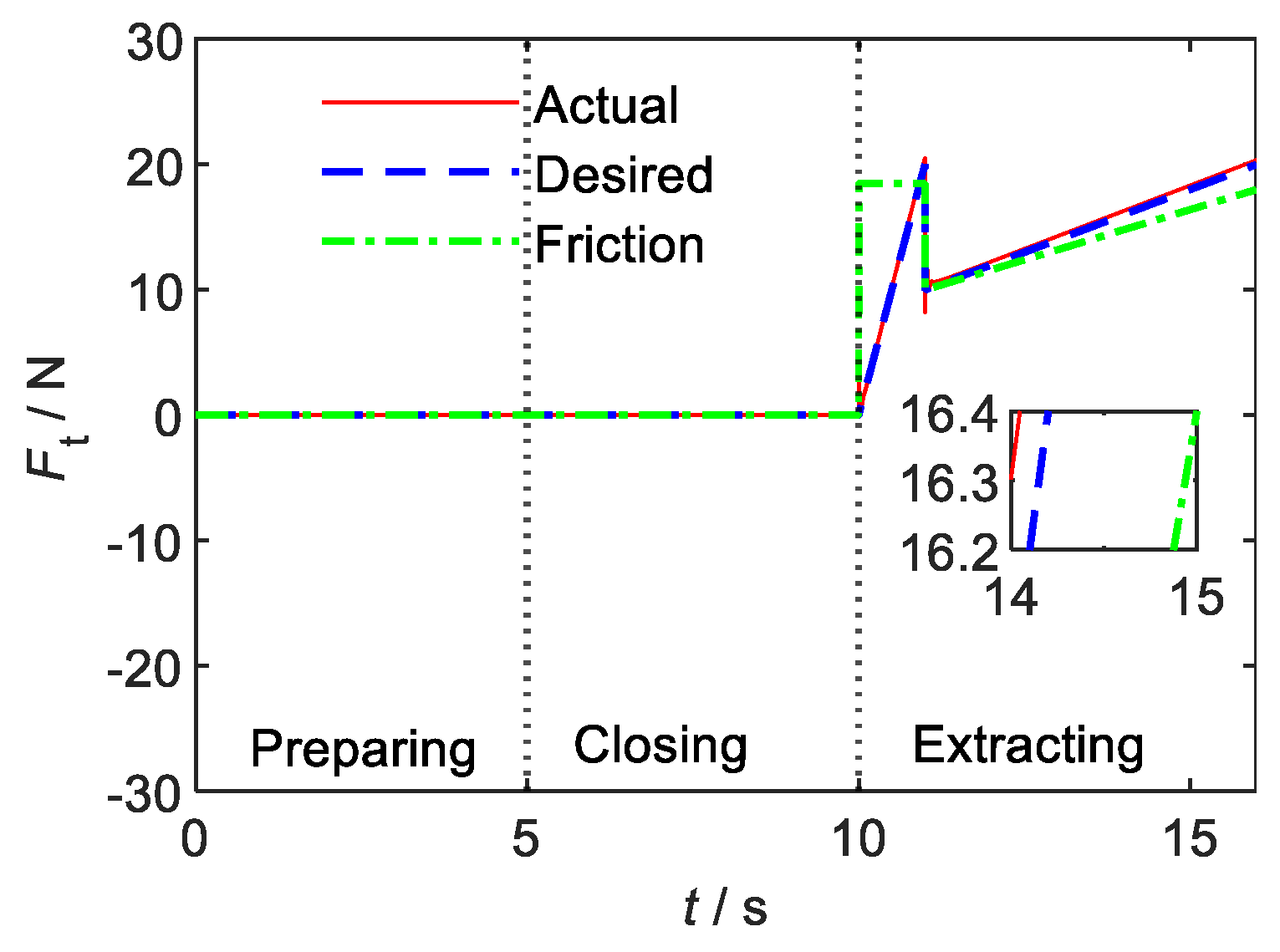
Due to assembly fit and material deformation, it is assumed that during the extraction process, the sliding friction increases as the length of the plug is removed and the maximum static friction force is set to 18.5 N.
The whole process of extracting operation was also divided into three stages:
Stage 1 (
): Preparation stage. The impedance control is turned off. The manipulator end is controlled to move to the desired position from the initial position. The attitude of the space robot carrier and the end-effector are adjusted so that the end-effector moves directly above the carrier hole and the orientation of the plug is consistent with that of the axis of the replaced part:
Stage 2 (
): Closing stage. The impedance control is turned off. The end-effector is controlled to move to be connected to the replaced device:
Stage 3 (
): Extraction stage. The impedance controller is turned on, and the output force is preloaded to overcome the maximum static friction force at 10 s~11 s after the end-effector holding the handle. At 11 s~16 s, the end effector grips the replaced part along the expected trajectory to overcome the friction resistance of the carrier hole and complete the extraction operation:
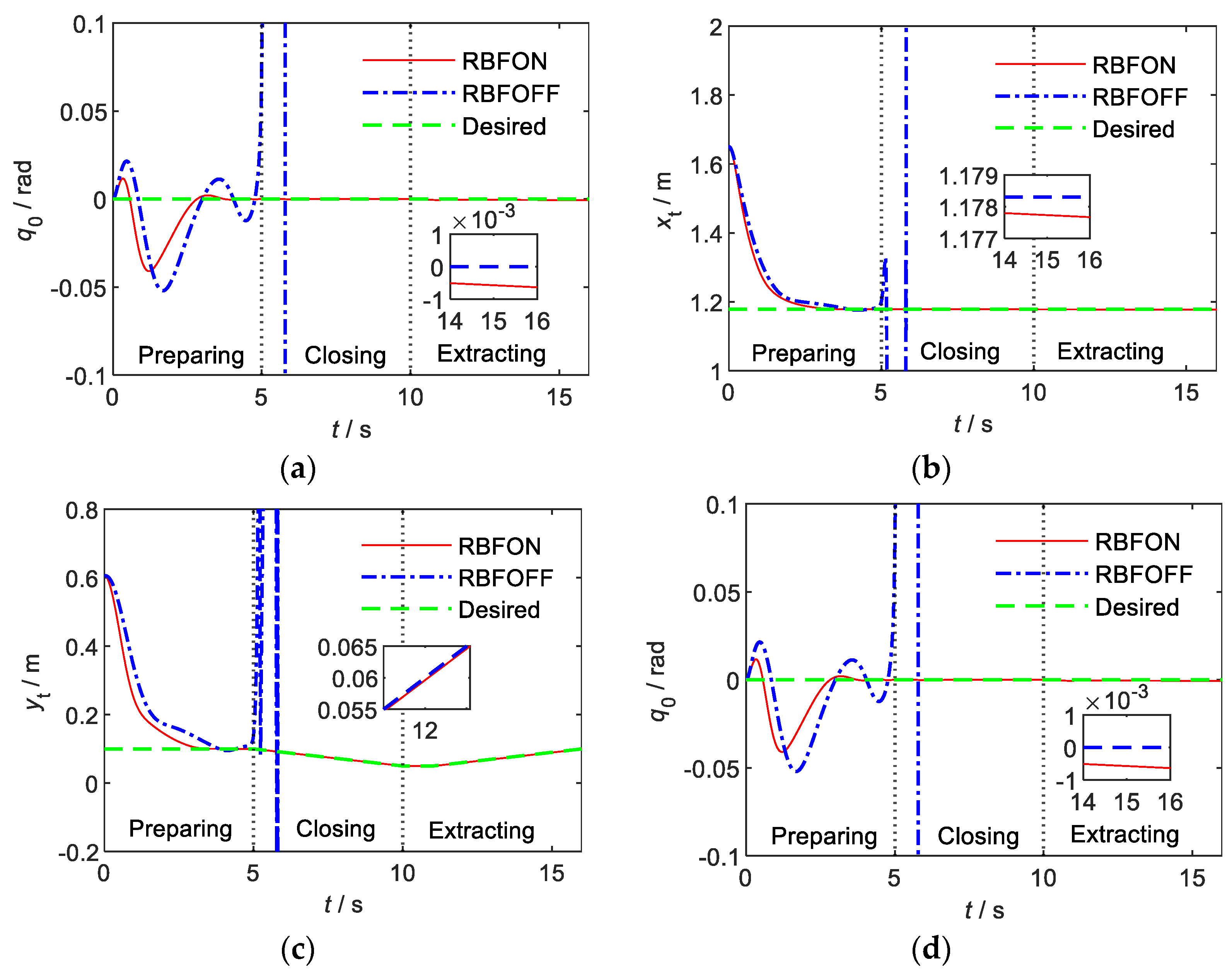
As can be seen from
Figure 9a,d, during the whole extraction process, the attitude of the carrier and the end-effector is well controlled, and the accuracy is better than
rad.
As can be seen from
Figure 9b, the control accuracy of the end-effector in the X direction was maintained at
m in the switching process of the three stages.
According to
Figure 9c and
Figure 10, when the end-effector is clamped by the replacement part, the movement in the Y direction remains static. When the impedance control increases the output force to the expected value of the design, the space robot successfully completes the extraction operation.
According to
Figure 9c and
Figure 10, when the end-effector is clamped by the replacement part, the movement in the Y direction remains static. When the impedance control increases the output force to the expected value of the design, the space robot successfully completes the extraction operation.
Additionally, the comparisons of tracking errors are listed in
Table 5.
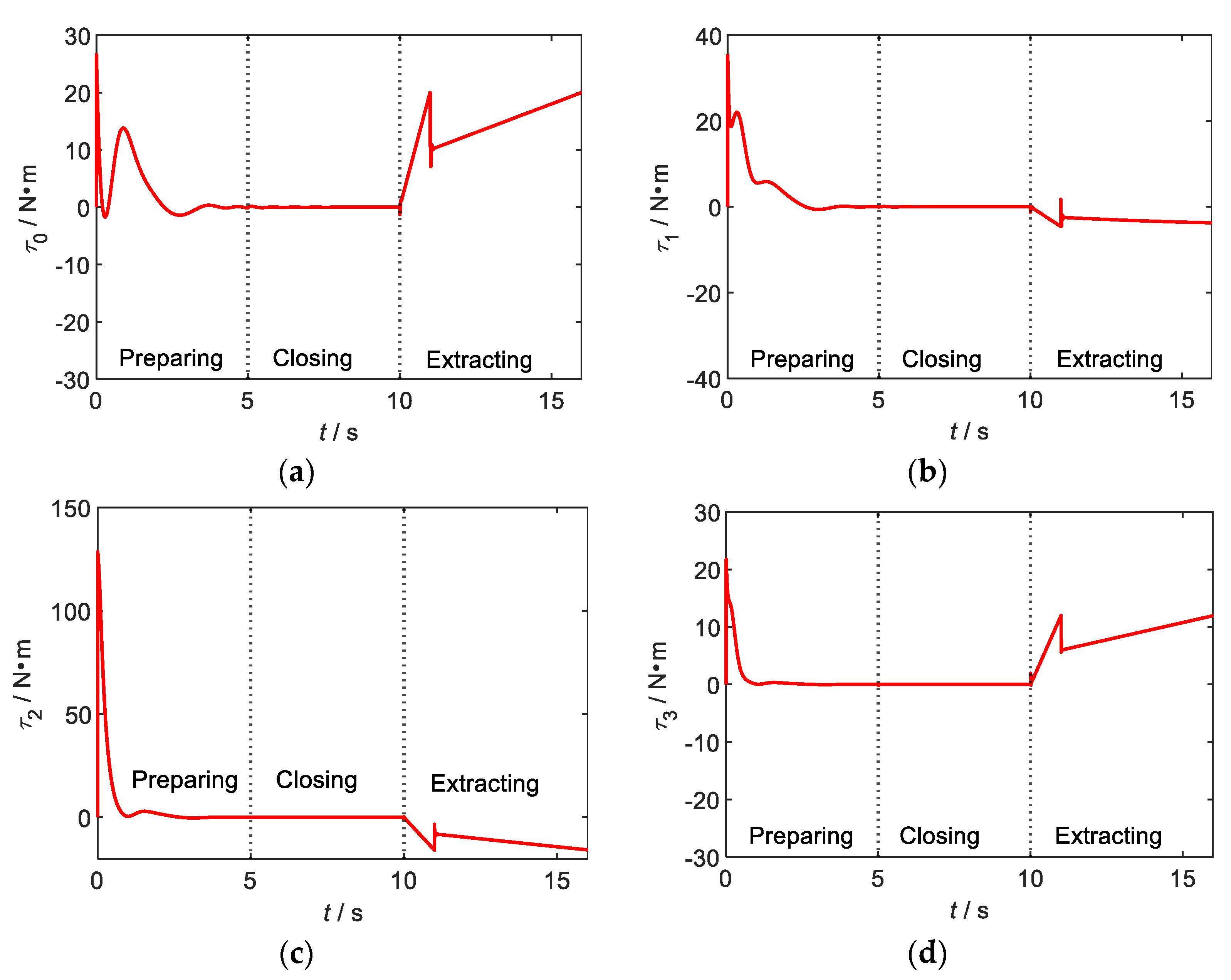
Figure 11 shows the control torques of the base and each joint of space robot.