Design and Flight Performance of a Bio-Inspired Hover-Capable Flapping-Wing Micro Air Vehicle with Tail Wing
Abstract
:1. Introduction
2. System Design and Optimization
2.1. System Overview
2.2. Flapping Mechanism
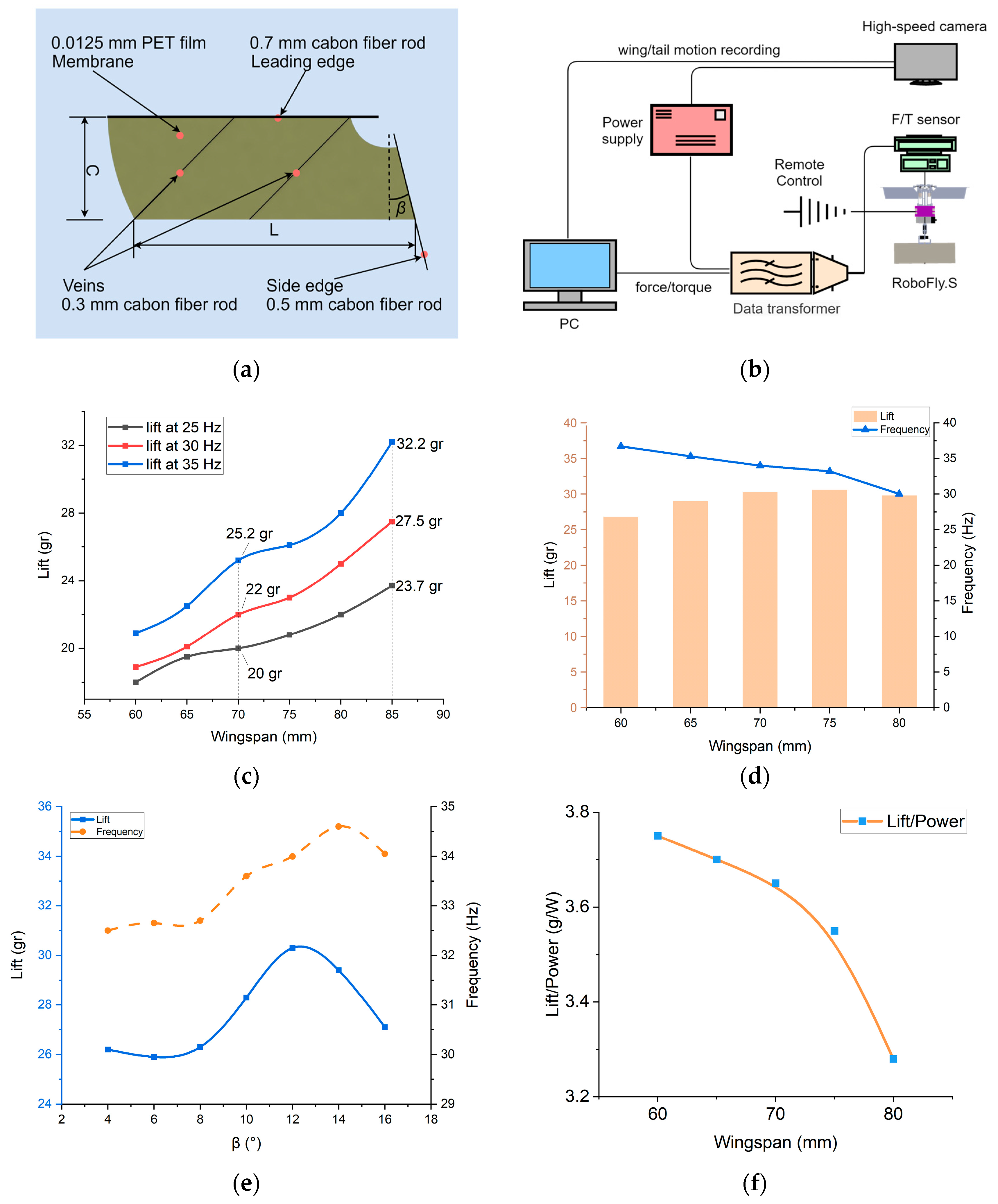
2.3. Bionic Wings
2.4. Control Mode
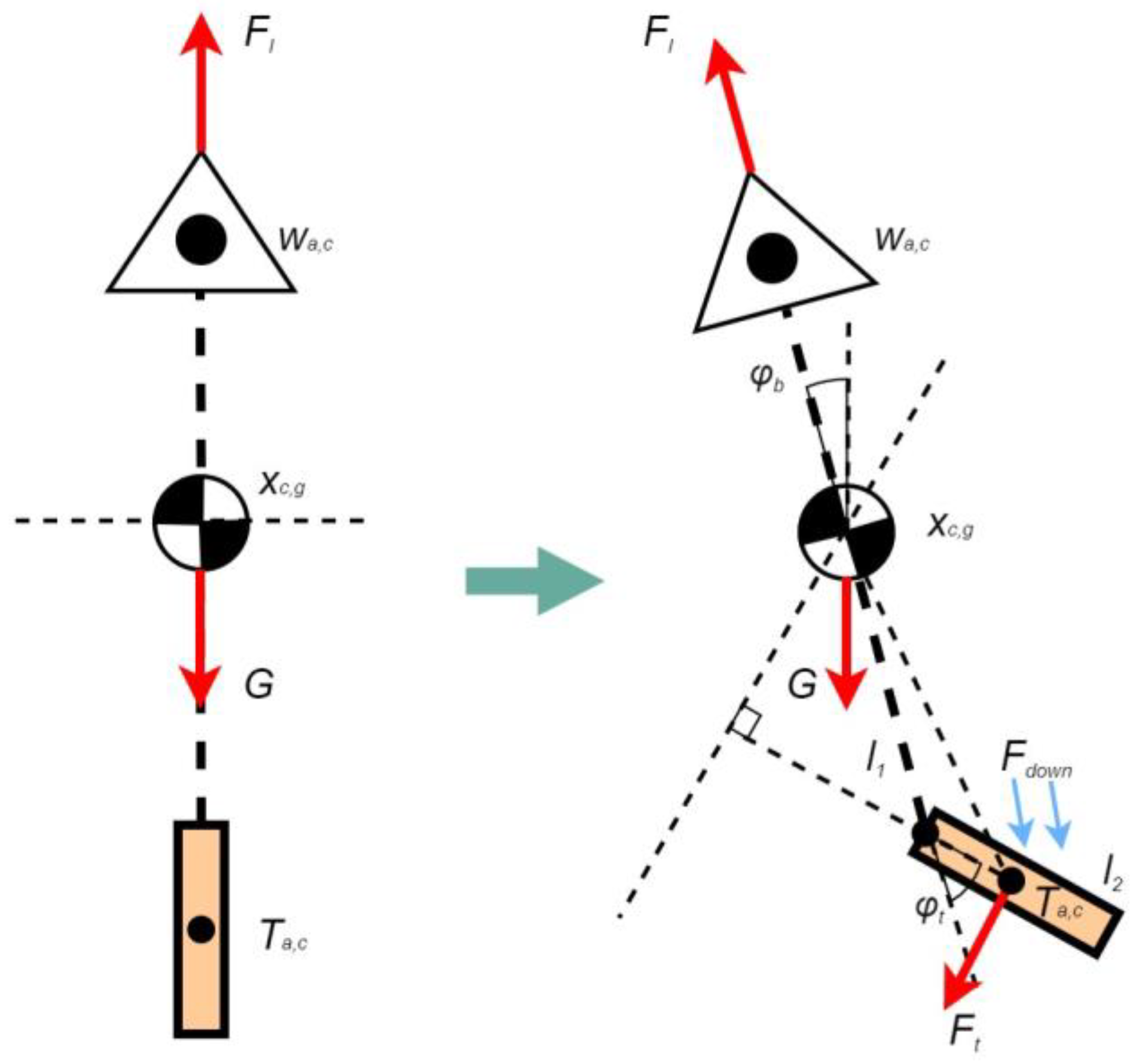
2.5. Stability Analysis
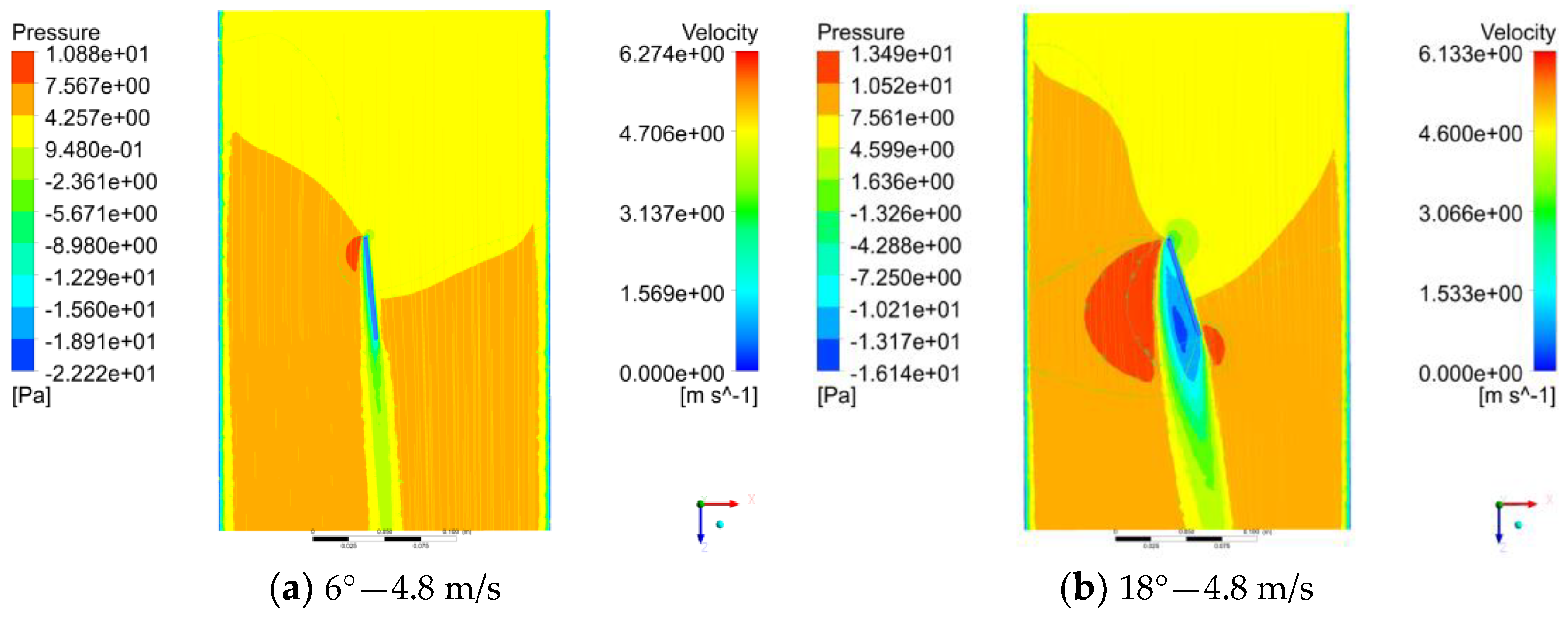
2.6. Analysis of Tail Wing
3. Flight Control System
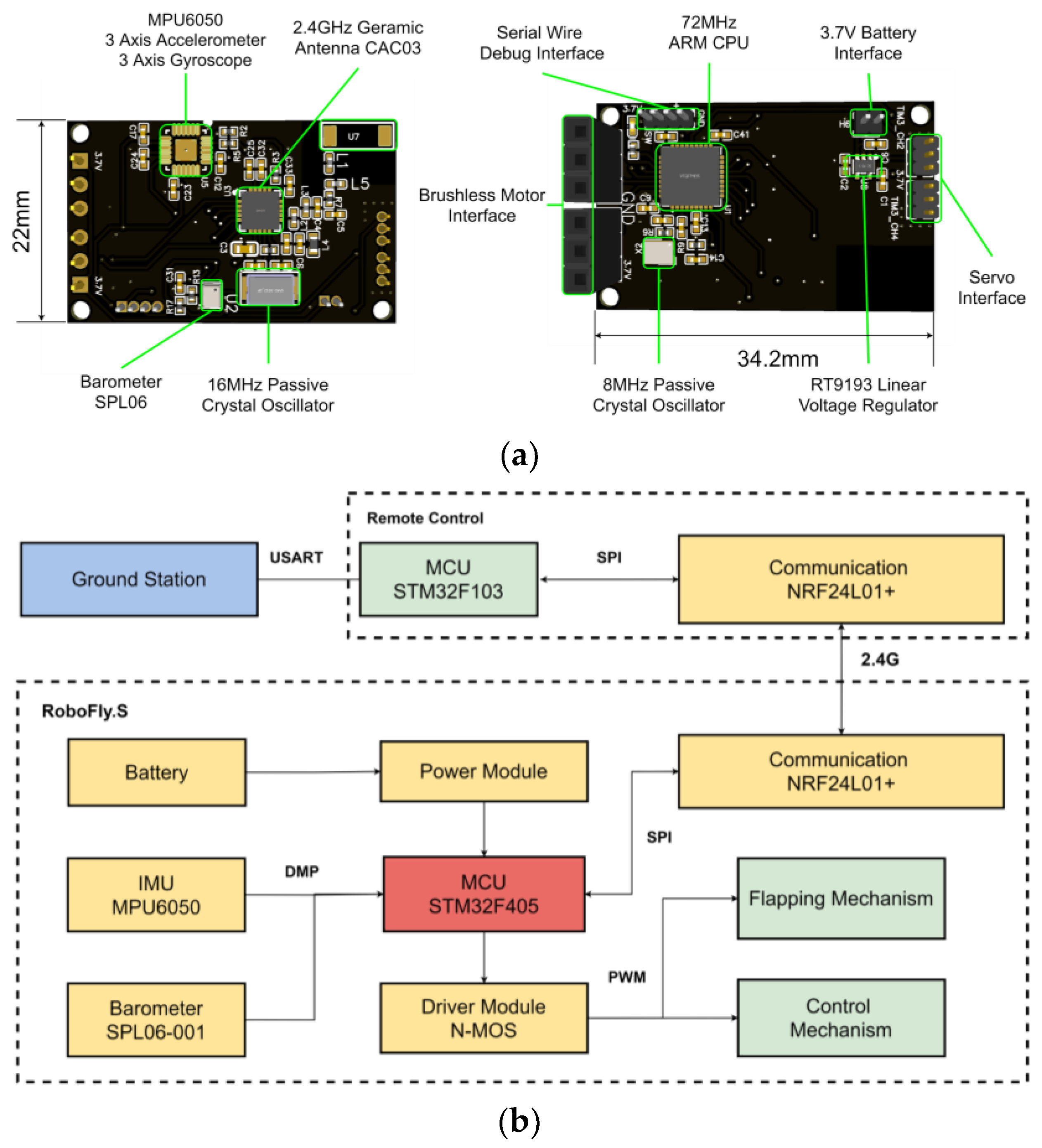
3.1. Autopilot
3.2. Control Algorithm
4. Experiments and Flight Tests
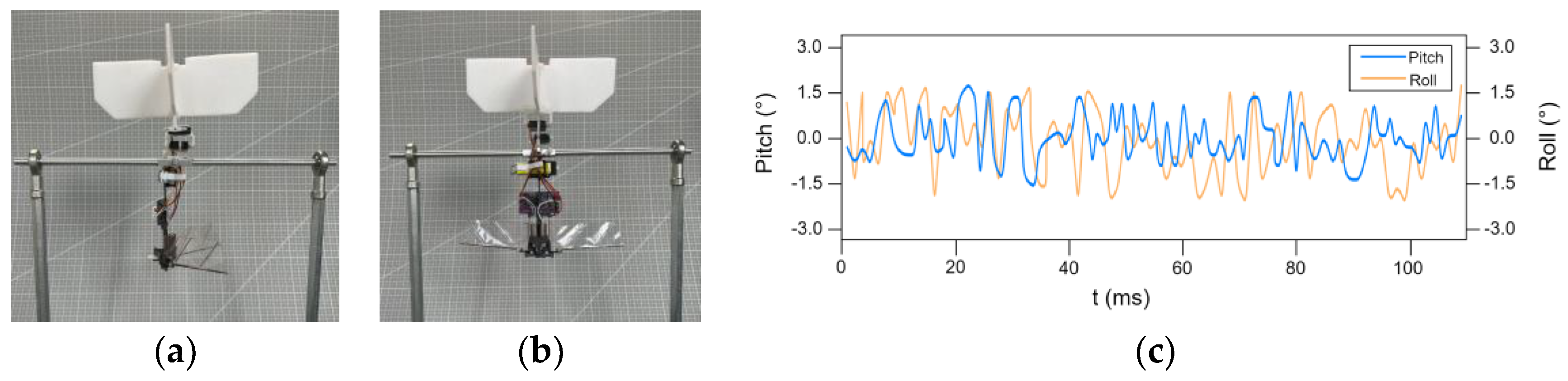
4.1. Attitude Stability Test
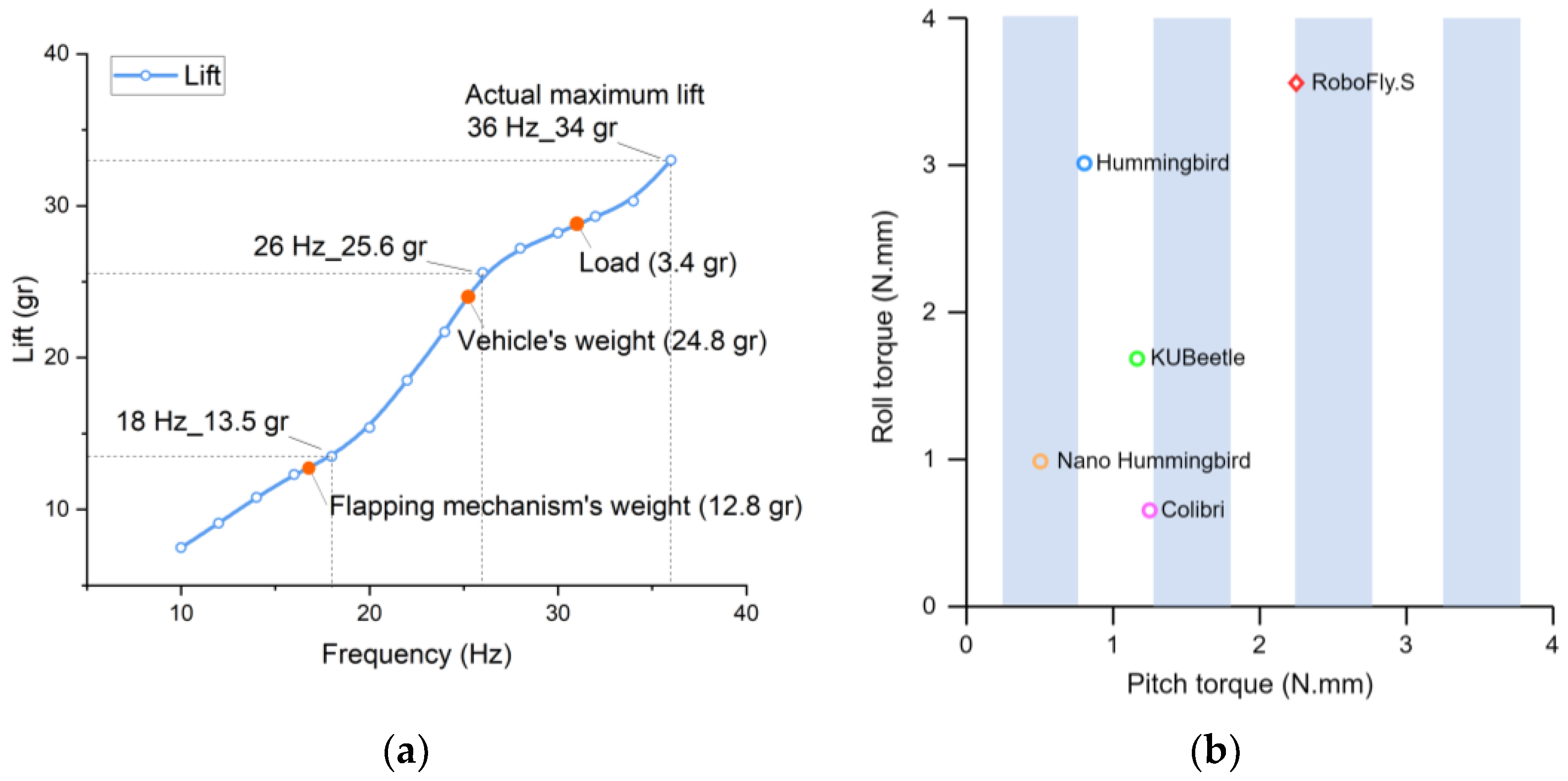
4.2. Aerodynamic Test
4.3. Flight Test
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Gerdes, J.W.; Gupta, S.K.; Wilkerson, S.A. A review of bird-inspired flapping wing miniature air vehicle designs. J. Mech. Robot. 2012, 4, 021003. [Google Scholar] [CrossRef]
- Jones, A.R.; Babinsky, H. Reynolds Number Effects on Leading Edge Vortex Development on a Waving Wing. Exp. Fluids 2011, 51, 197–210. [Google Scholar] [CrossRef]
- Deng, H.; Xiao, S.; Huang, B.; Yang, L.; Xiang, X.; Ding, X. Design optimization and experimental study of a novel mechanism for a hoverable bionic flapping-wing micro air vehicle. Bioinspir. Biomim. 2020, 16, 026005. [Google Scholar] [CrossRef] [PubMed]
- Vejdani, H.R.; Boerma, D.B.; Swartz, S.M.; Breuer, K.S. The dynamics of hovering flight in hummingbirds, insects and bats with implications for aerial robotics. Bioinspir. Biomim. 2018, 14, 016003. [Google Scholar] [CrossRef] [PubMed]
- Keennon, M.; Klingebiel, K.; Won, H. Development of the nano hummingbird: A tailless flapping wing micro air vehicle. In Proceedings of the 50th AIAA Aerospace Sciences Meeting including the New Horizons Forum and Aerospace Exposition, Nashville, TN, USA, 9–12 January 2012; p. 588. [Google Scholar]
- Steltz, E.; Avadhanula, S.; Fearing, R.S. High lift force with 275 Hz wing beat in MFI. In Proceedings of the IEEE/RSJ International Conference on Intelligent Robots and Systems, San Diego, CA, USA, 29 October–2 November 2007; pp. 3993–3998. [Google Scholar]
- Graule, M.; Chirarattananon, P.; Fuller, S.; Jaferis, N.; Ma, K.; Spenko, M. Perching and takeoff of a robotic insect on overhangs using switchable electrostatic adhesion. Science 2016, 352, 978–982. [Google Scholar] [CrossRef]
- Chen, Y.; Wang, H.; Helbling, E.F.; Jaferis, N.T.; Zuferey, R.; Ong, A. A biologically inspired, flapping-wing, hybrid aerial-aquatic microrobot. Sci. Robot. 2017, 2, eaao5619. [Google Scholar] [CrossRef]
- Jaferis, N.T.; Helbling, E.F.; Karpelson, M.; Wood, R.J. Untethered flight of an insect-sized flapping-wing microscale aerial vehicle. Nature 2019, 570, 491–495. [Google Scholar] [CrossRef]
- Zou, Y.; Zhang, W.P.; Zhang, Z. Liftoff of an electromagnetically driven insect-inspired flapping-wing robot. IEEE Trans. Robot. 2016, 32, 1285–1289. [Google Scholar] [CrossRef]
- Lau, G.K.; Lim, H.T.; Teo, J.Y.; Chin, Y.W. Lightweight mechanical amplifers for rolled dielectric elastomer actuators and their integration with bio-inspired wing flappers. Smart Mater. Struct. 2014, 23, 025021. [Google Scholar] [CrossRef]
- Yan, X.J.; Qi, M.J.; Lin, L.W. Self-lifting artificial insect wings via electrostatic flapping actuators. In Proceedings of the IEEE International Conference on Micro Electro Mechanical Systems, Estoril, Portugal, 18–22 January 2015; pp. 22–25. [Google Scholar]
- De Clercq, K.M.; de Kat, R.; Remes, B.; van Oudheusden, B.W.; Bijl, H. Aerodynamic experiments on DelFly II: Unsteady lift enhancement. Int. J. Micro Air Veh. 2009, 1, 255–262. [Google Scholar] [CrossRef]
- Tijmons, S.; Karásek, M.; De Croon, G. Attitude control system for a lightweight flapping wing MAV. Bioinspir. Biomim. 2018, 13, 056004. [Google Scholar] [CrossRef] [PubMed]
- Karásek, M.; Muijres, F.T.; De Wagter, C.; Remes, B.D.; De Croon, G.C. A tailless aerial robotic flapper reveals that flies use torque coupling in rapid banked turns. Science 2018, 361, 1089–1094. [Google Scholar] [CrossRef] [PubMed]
- Karásek, M.; Nan, Y.; Romanescu, I.; Preumont, A. Pitch moment generation and measurement in a robotic hummingbird. Int. J. Micro Air Veh. 2013, 5, 299–309. [Google Scholar] [CrossRef]
- Karasek, M.; Hua, A.; Nan, Y.; Lalami, M.; Preumont, A. Pitch and roll control mechanism for a hovering flapping wing MAV. Int. J. Micro Air Veh. 2014, 6, 253–264. [Google Scholar] [CrossRef]
- Roshanbin, A.; Altartouri, H.; Karásek, M.; Preumont, A. COLIBRI: A hovering flapping twin-wing robot. Int. J. Micro Air Veh. 2017, 9, 270–282. [Google Scholar] [CrossRef]
- Roshanbin, A.; Abad, F.; Preumont, A. Kinematic and aerodynamic enhancement of a robotic hummingbird. AIAA J. 2019, 57, 4599–4607. [Google Scholar] [CrossRef]
- Preumont, A.; Wang, H.; Kang, S.; Wang, K.; Roshanbin, A. A note on the electromechanical design of a robotic hummingbird. Actuators 2021, 10, 52. [Google Scholar] [CrossRef]
- Phan, H.V.; Truong, Q.T.; Park, H.C. An experimental comparative study of the efficiency of twisted and flat flapping wings during hovering flight. Bioinspir. Biomim. 2017, 12, 036009. [Google Scholar] [CrossRef]
- Phan, H.V.; Kang, T.; Park, H.C. Design and stable flight of a 21 g insect-like tailless flapping wing micro air vehicle with angular rates feedback control. Bioinspir. Biomim. 2017, 12, 036006. [Google Scholar] [CrossRef]
- Phan, H.V.; Park, H.C. Mechanisms of collision recovery in flying beetles and flapping-wing robots. Science 2020, 370, 1214–1219. [Google Scholar] [CrossRef]
- Nguyen, Q.V.; Chan, W.L. Development and flight performance of a biologically-inspired tailless flapping-wing micro air vehicle with wing stroke plane modulation. Bioinspir. Biomim. 2018, 14, 016015. [Google Scholar] [CrossRef]
- Tu, Z.; Fei, F.; Zhang, J.; Deng, X. An at-scale tailless flapping-wing hummingbird robot. I. Design, optimization, and experimental validation. IEEE Trans. Robot. 2020, 36, 1511–1525. [Google Scholar] [CrossRef]
- Tu, Z.; Fei, F.; Deng, X. Untethered flight of an at-scale dual-motor hummingbird robot with bio-inspired decoupled wings. IEEE Robot. Autom. Lett. 2020, 5, 4194–4201. [Google Scholar] [CrossRef]
- Zhang, J.; Cheng, B.; Roll, J.A.; Deng, X.; Yao, B. Direct drive of flapping wings under resonance with instantaneous wing trajectory control. In Proceedings of the 2013 IEEE International Conference on Robotics and Automation, Karlsruhe, Germany, 6–10 May 2013; pp. 4029–4034. [Google Scholar]
- Xiao, S.; Deng, H.; Hu, K.; Zhang, S. Design and Control of Hoverable Bionic Flapping Wing Micro Air Vehicle. In Proceedings of the 2021 IEEE International Conference on Electrical Engineering and Mechatronics Technology, Qingdao, China, 2–4 July 2021; pp. 224–227. [Google Scholar]
- Nguyen, Q.V.; Chan, W.; Debiasi, M. Design, Fabrication, and Performance Test of a Hovering-Based Flapping-Wing Micro Air Vehicle Capable of Sustained and Controlled Flight. In Proceedings of the International Micro Air Vehicle Conference and Competition 2014, Delft, The Netherlands, 12–15 August 2014; pp. 18–25. [Google Scholar]
- Hines, L.; Campolo, D.; Sitti, M. Liftoff of a Motor-Driven, Flapping-Wing Microaerial Vehicle Capable of Resonance. IEEE Trans. Robot. 2014, 30, 220–232. [Google Scholar] [CrossRef]
- Guo, W. Mechanisms and Machine Theory; Science Press: Beijing, China, 2013. [Google Scholar]
- Nan, Y.; Karásek, M.; Lalami, M.E.; Preumont, A. Experimental optimization of wing shape for a hummingbird-like flapping wing micro air vehicle. Bioinspir. Biomim 2017, 12, 026010. [Google Scholar] [CrossRef]
- Phan, H.V.; Park, H.C. Design and evaluation of a deformable wing configuration for economical hovering flight of an insect-like tailless flying robot. Bioinspir. Biomim 2018, 13, 036009. [Google Scholar] [CrossRef] [PubMed]
- Li, H.; Nabawy, M.R.A. Wing Planform Effect on the Aerodynamics of Insect Wings. Insects 2022, 13, 459. [Google Scholar] [CrossRef]
- Phillips, N.; Knowles, K.; Bomphrey, R.J. The effect of aspect ratio on the leading-edge vortex over an insect-like flapping wing. Bioinspir. Biomim 2015, 10, 056020. [Google Scholar] [CrossRef] [PubMed]
- Nan, Y.; Chen, Y.; Mcglinchey, D.; Li, Y. Experimental Studies of Tail Shapes for Hummingbird-Like Flapping Wing Micro Air Vehicles. IEEE Access 2020, 8, 52622–52630. [Google Scholar] [CrossRef]
- Li, H.; Li, D.; Shen, T.; Bie, D.; Kan, Z. Numerical Analysis on the Aerodynamic Characteristics of an X-wing Flapping Vehicle with Various Tails. Aerospace 2022, 9, 440. [Google Scholar] [CrossRef]
- Armanini, S.; Caetano, J.; De Visser, V.; Pavel, M. Modelling wing wake and tail aerodynamics of a flapping-wing micro aerial vehicle. Int. J. Micro Air Veh. 2019, 11, 1756829319833674. [Google Scholar] [CrossRef]
- Jiao, Z.X.; Wang, L.; Zhao, L.F.; Jiang, W.Y. Hover flight control of X-shaped flapping wing aircraft considering wing–tail interactions. Aerosp. Sci. Technol 2021, 16, 106870. [Google Scholar] [CrossRef]
- Guzmán, M.M.; Páez, C.R.; Maldonado, F.J.; Zufferey, R.; Tormo-Barbero, J.; Acosta, J.; Ollero, A. Design and comparison of tails for bird-scale flapping-wing robots. In Proceedings of the 2021 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Prague, Czech Republic, 27 September–1 October 2021; pp. 6358–6365. [Google Scholar]
- Zerze, T.; Pandža, V.; Kasalo, M.; Zlatar, D. Discrete mechanics and optimal control optimization of flapping wing dynamics for Mars exploration. Aerosp. Sci. Technol 2020, 106, 106131. [Google Scholar]








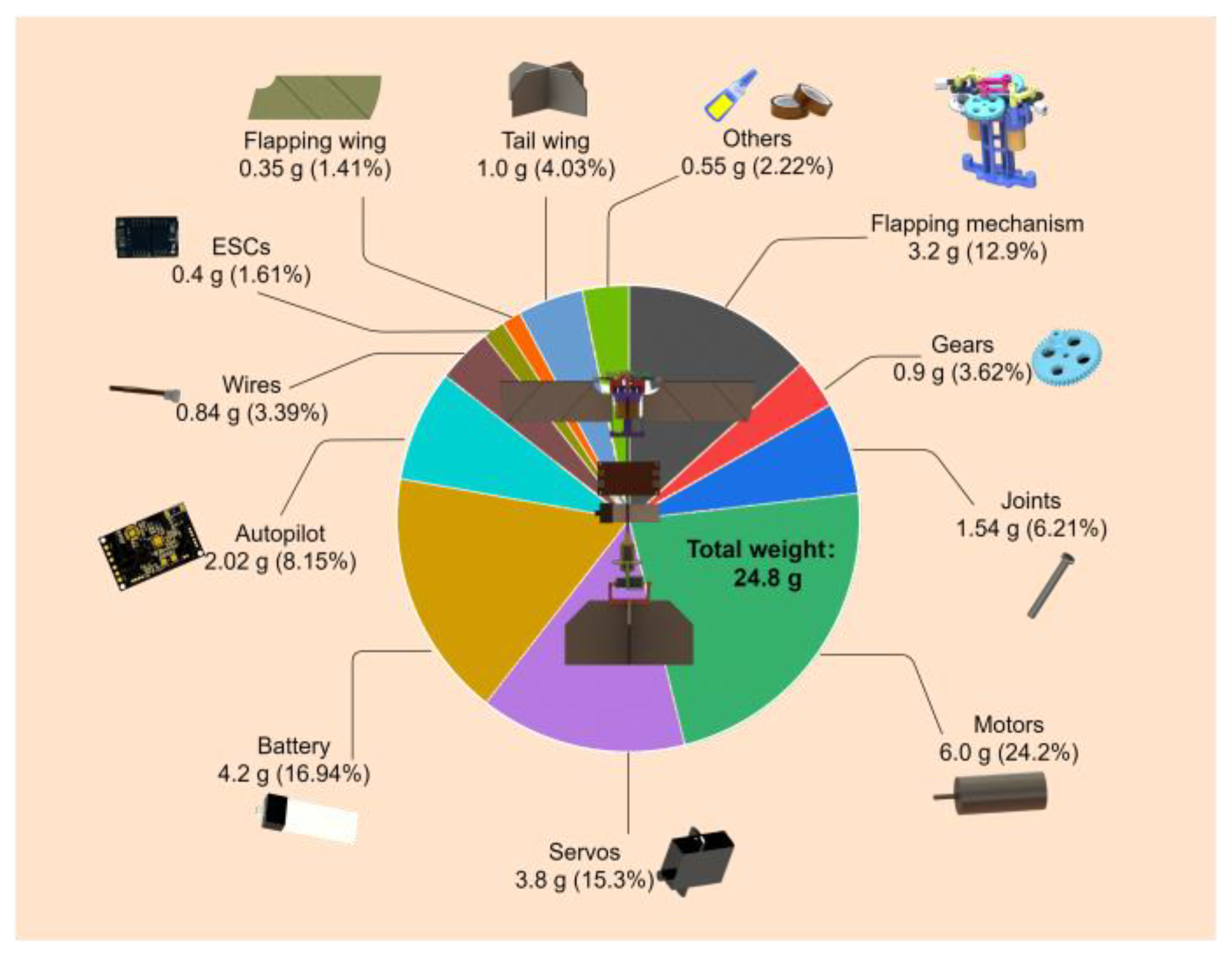


| Weight (g) | Wingspan (cm) | Max-Lift (g) | Max Forward Speed (m/s) | Frequency (Hz) | Amplitude (°) | Flight Endurance |
|---|---|---|---|---|---|---|
| 24.8 | 16.5 | 34 | 2.5 | 27–38 | 162 | 2.5 min |
| Measurement Position | 0.25R | 0.5R | 0.75R | R |
|---|---|---|---|---|
| Rolling direction flow velocity (m/s) | 3.6 | 4.8 | 4.9 | 2.45 |
| Pitching direction flow velocity (m/s) | 2.5 | 2.6 | 2.8 | 1.7 |
| Working Conditions | 30-4.8 (Roll) | 18-4.8 (Roll) | 6-4.8 (Roll) | 30-2.6 (Pitch) | 18-2.6 (Pitch) | 6-2.6 (Pitch) |
|---|---|---|---|---|---|---|
| Torques (N·mm) | 4.625 | 3.746 | 0.914 | 1.743 | 1.065 | 0.254 |
| Torque coefficients | 0.007 | 0.005 | 0.001 | 0.002 | 0.001 | 0.0004 |
| Experimental values | 3.49–3.55 | 0.85~0.91 | 1.47–1.51 | 0.32–0.33 |
| FWMAVs | Flapping Mechanism | Control Mechanism | Wingspan (cm) | Weight (g) | Load (g) | Flight Endurance |
|---|---|---|---|---|---|---|
| Nano Hummingbird | String-based | Wing side-edge twist | 16.5 | 19 | 1 | 4 min |
| Robotic hummingbird | Single-crank and double-rocker | Wing root twist | 30 | 62 | \ | 40 s |
| KUBeetle-S | String-based and linkage | Wing root twist | \ | 15.8 | 2.3 | 8.8 min |
| Colibri | String-based and linkage | Wing side-edge twist | 22 | 23.85 | \ | 5 min |
| Hummingbird | Motor direct drive | Gears/Motors | 17 | 20.4 | \ | 20 s |
| RoboFly.S | Crank-rocker and linkage | Tail wing | 16.5 | 24.8 | 3.4 | 2.5 min |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiao, S.; Sun, Y.; Ren, D.; Hu, K.; Deng, H.; Wang, Y.; Ding, X. Design and Flight Performance of a Bio-Inspired Hover-Capable Flapping-Wing Micro Air Vehicle with Tail Wing. Aerospace 2023, 10, 910. https://doi.org/10.3390/aerospace10110910
Xiao S, Sun Y, Ren D, Hu K, Deng H, Wang Y, Ding X. Design and Flight Performance of a Bio-Inspired Hover-Capable Flapping-Wing Micro Air Vehicle with Tail Wing. Aerospace. 2023; 10(11):910. https://doi.org/10.3390/aerospace10110910
Chicago/Turabian StyleXiao, Shengjie, Yuhong Sun, Dapeng Ren, Kai Hu, Huichao Deng, Yun Wang, and Xilun Ding. 2023. "Design and Flight Performance of a Bio-Inspired Hover-Capable Flapping-Wing Micro Air Vehicle with Tail Wing" Aerospace 10, no. 11: 910. https://doi.org/10.3390/aerospace10110910
APA StyleXiao, S., Sun, Y., Ren, D., Hu, K., Deng, H., Wang, Y., & Ding, X. (2023). Design and Flight Performance of a Bio-Inspired Hover-Capable Flapping-Wing Micro Air Vehicle with Tail Wing. Aerospace, 10(11), 910. https://doi.org/10.3390/aerospace10110910






