Analytical Formulations for Nitrogen Oxides Emissions Estimation of an Air Turbo-Rocket Engine Using Hydrogen
Abstract
:1. Introduction
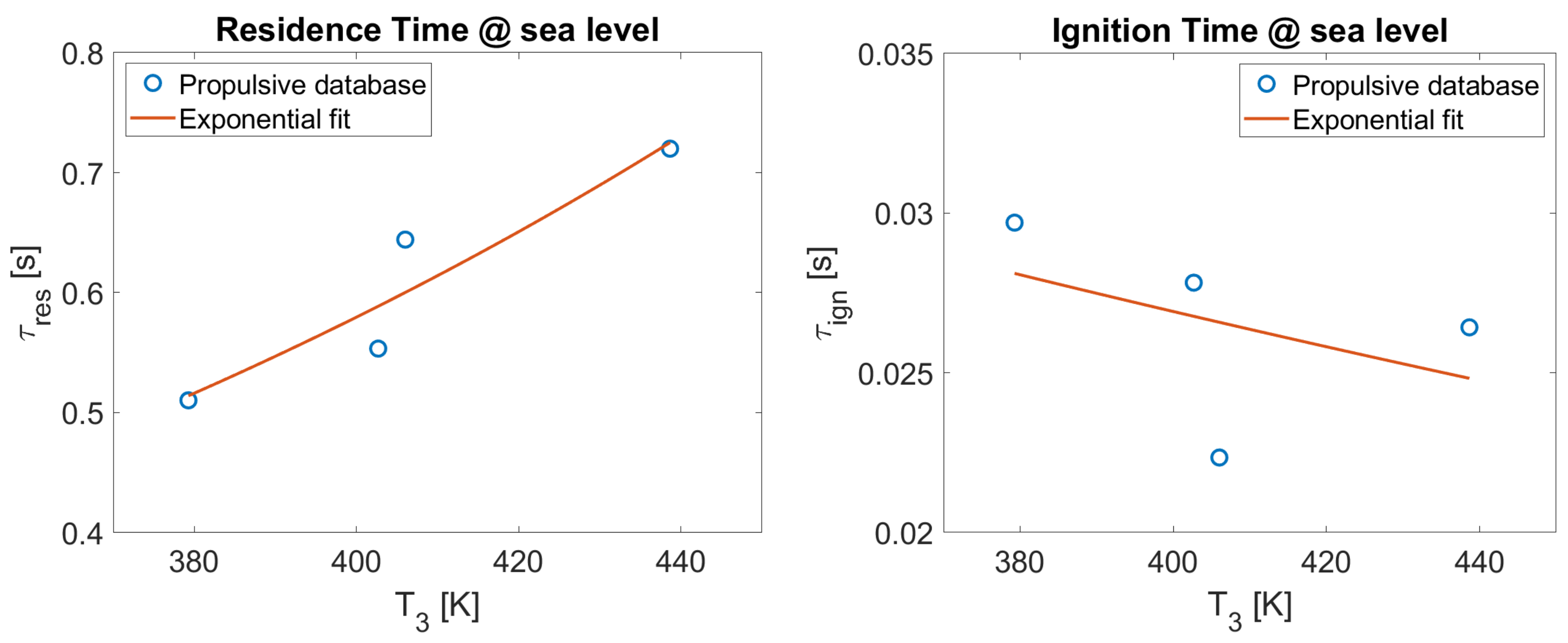
- The H2-P3T3 method follows an approach similar to the original P3-T3 method and allows the prediction of in-flight emissions, knowing the emissions at sea-level conditions together with the ratios of the flight-level and sea-level conditions of the pressure and fuel-to-air ratio at the inlet of the combustion chamber. However, the need to introduce new parameters (i.e., Da) in the analytical formulations to better represent the high-speed hydrogen combustion, modifies the original method, requiring the definition of additional sea-level trends for new variables, including the ignition delay time (i.e., the time elapsing between the start of injection and the start of combustion) and the residence time (i.e., the time spent by the reacting flow inside the thrust chamber).
- While the original P3-T3 method consists of a single analytical formulation with tuneable parameters to effectively represent different engine architectures, the new H2-P3T3 method encompasses three different formulations for the same engine architecture (ATR). The different formulations provide the user with different levels of prediction accuracy and can thus be applied at different stages in the design process, increasing the flexibility in the method.
- The introduction of new correlated factors to improve the analytical formulations is based on an in-depth investigation into the results of the chemical-kinetic analyses coupled with the assessment of the most commonly adopted strategies for NOx minimization in the case of hydrogen-based combustion in high-speed aviation propulsive systems.
- This section reveals an important scientific finding: the emissions of nitrogen oxides from a high-speed engine using hydrogen are well correlated to the Da number. A variation in Da number is usually due to a variation in the residence time of the reacting flow in the combustion chamber, which may lead to a temperature variation in the combustor, thus resulting in a variation in NOx emissions.
- While the formulations are strictly related to the architecture of the analyzed engine, the proposed H2-P3T3 method has a more general validity and can be used as a baseline for developing additional analytical formulations to better represent different engine architectures and technologies.
2. State of the Art in NOx Emissions Modeling for Aeronautical Applications
2.1. Correlation-Based Methods
- Correlation-based methods require a great amount of real engine data, which can only be retrieved from extensive experimental on-ground test campaigns or in-flight direct measurements. This is extremely expensive, both in terms of economic and time resources.
- Correlation-based methods are built for a specific engine and it is nearly impossible to include in the formulation parameters capturing the effect of variation, even minimal, in the engine design on emissions.
- The variables that show better correlation with NOx formation (e.g., pressure at temperature conditions in the combustion chamber, residence times, etc.) are very difficult to estimate in the early design stages.
2.2. P3-T3 Method
- (1)
- The sea-level combustor inlet conditions in terms of pressure (), temperature (T3) and fuel-to-air ratio () corresponding to the four throttle settings prescribed in [9] are estimated or retrieved from the engine manufacturer’s proprietary information. Complementary NOx emission indexes at sea-level condition () are retrieved from the ICAO Aircraft Engine Emissions Databank. , , and are plotted against the T3 value corresponding to the four throttle settings.
- (2)
- In-flight combustor inlet conditions (, ) are usually retrieved from manufacturer proprietary data or, in the case of data unavailability, they can be estimated from accurate and high-fidelity propulsive models.
- (3)
- Starting from the in-flight combustor inlet conditions (, ), the values of , , and , corresponding to combustor inlet temperature at altitude, are obtained from the previously mentioned plots.
- (4)
- can be determined by using corrective factors accounting for the differences between sea-level and in-flight altitude conditions, according to Formula (1):
- Data are unavailable from the ICAO aircraft engine emissions databank for under-development or future engines [9]. In this paper, the authors tackle this issue by setting up extensive 0D chemical-kinetic simulation campaigns. This allows for modeling and simulating sea-level conditions for the combustor to obtain the . set.
- Demonstrated validity of the model is restricted to subsonic engines using conventional fuels. In this paper, the authors tackle this issue by exploiting the results of the 0D chemical-kinetic simulations to upgrade the original analytical formulation (Equation (1)), thus extending its applicability to hydrogen-fueled engines able to operate from the subsonic speed regime to the supersonic and hypersonic ones.
2.3. Fuel-Flow Method
2.4. Simplified Physics-Based Models
2.5. High-Fidelity Simulations
3. Case Study: Air Turbo-Rocket Fueled with Hydrogen
3.1. STRATOFLY MRx and Its Air Turbo-Rocket Engines
3.2. Propulsive and Chemical Emissions Databases
4. H2-P3T3 Methodology and Novel Formulations
4.1. H2-P3T3 Method
4.2. Prediction of Sea-Level Conditions
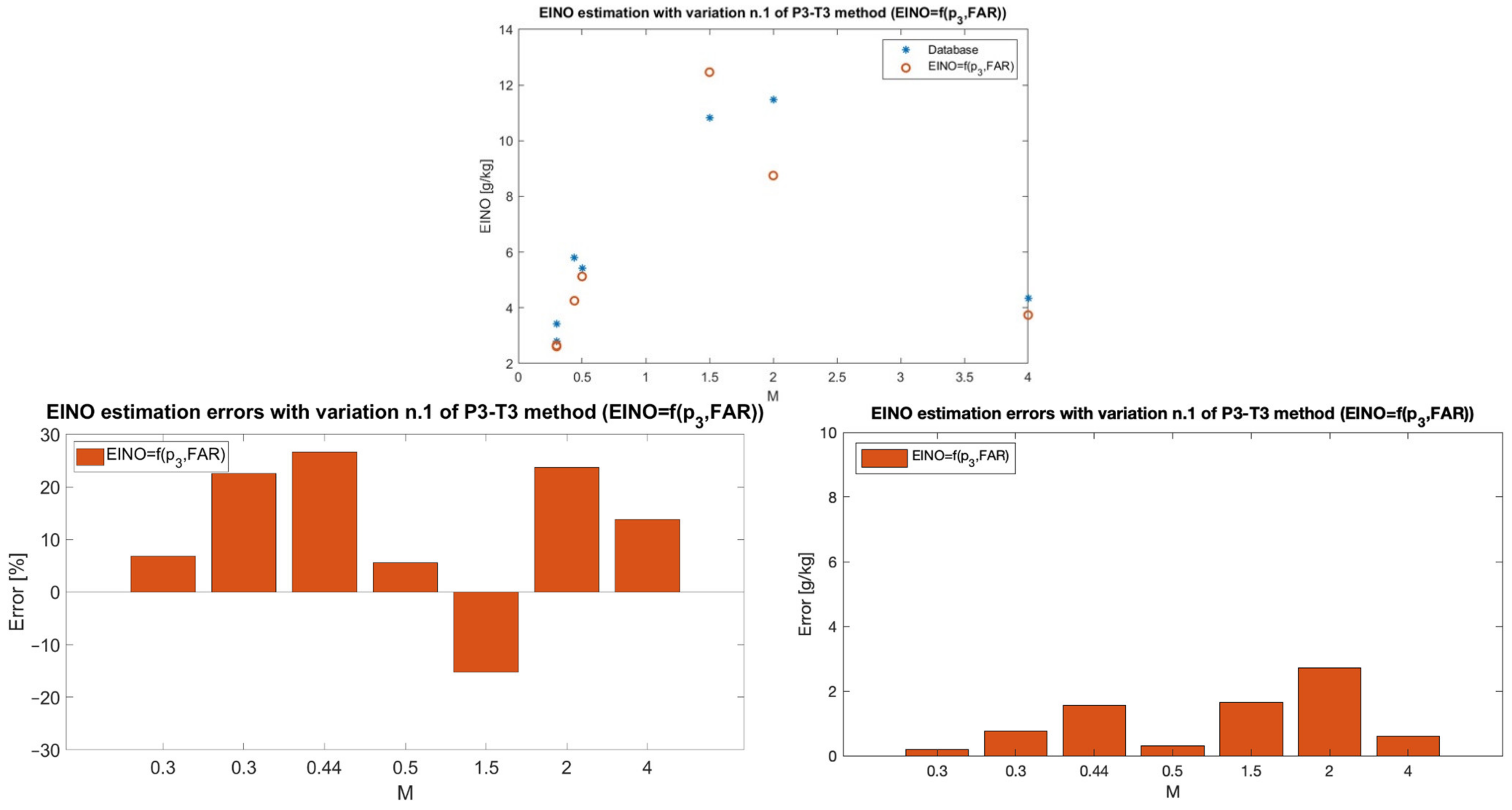
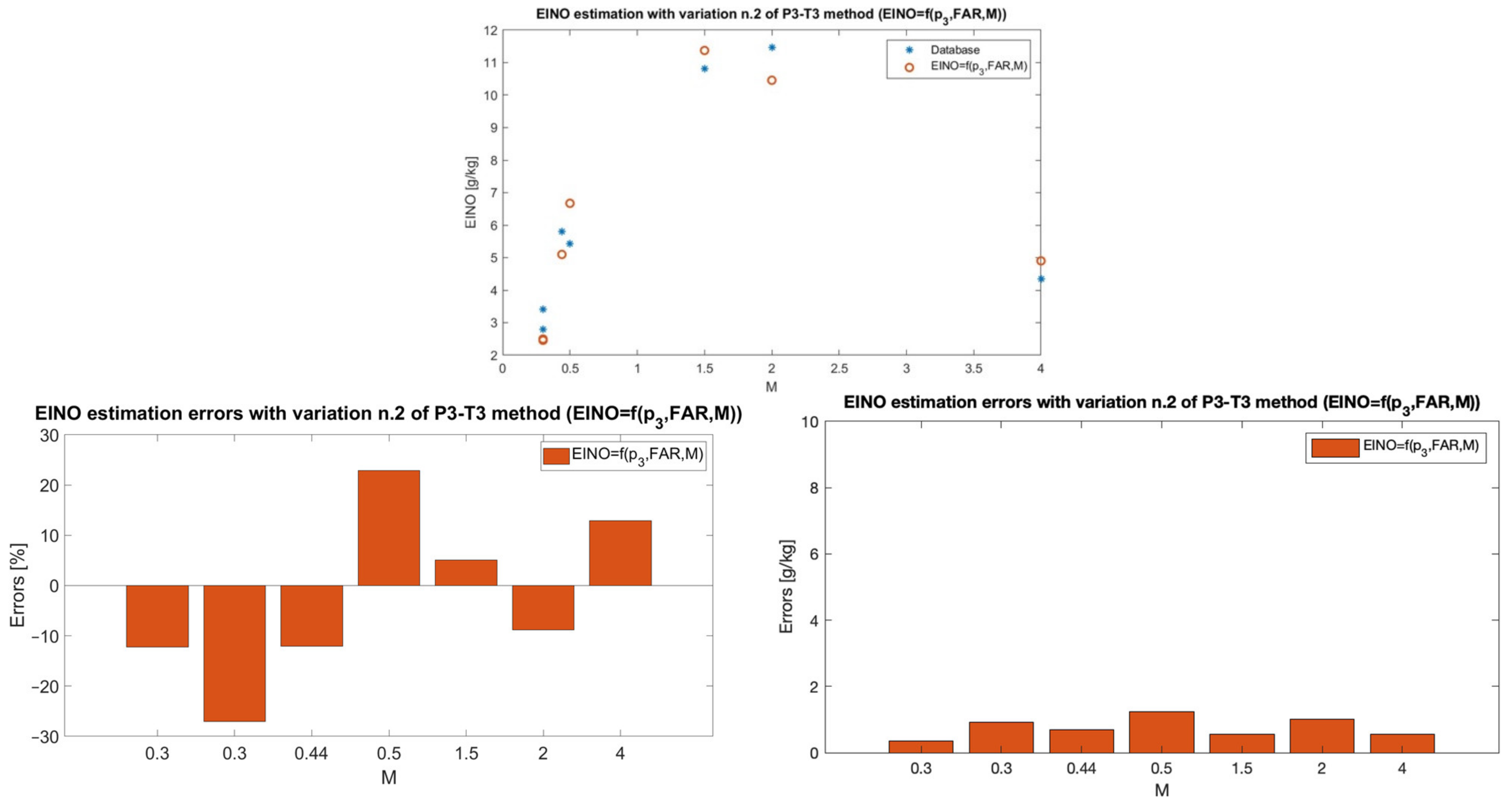
4.3. H2-P3T3 Formulations Derivation for An ATR Engine Fueled with Hydrogen
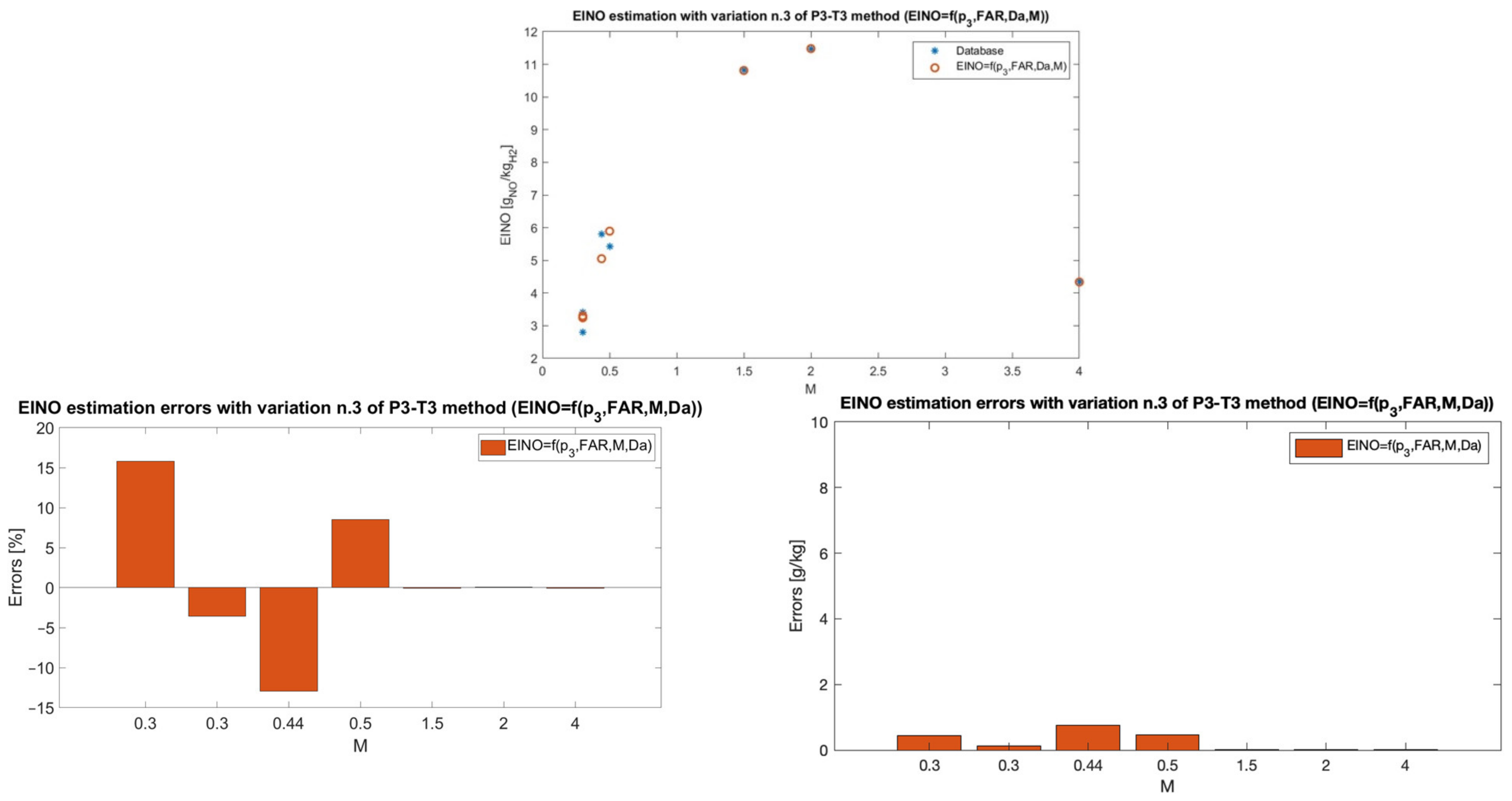
5. Results and Discussion
- ◦
- The positive value of b is in agreement with the ideal gas constitutive law, which implies that an increase in causes a rise in the temperature of the mixture, leading to higher NOx production. However, the impact of the pressure ratio factor in the novel formulation is reduced by a factor of about two with respect to the original P3-T3 method. This is due the nature of the fuel considered in the two formulations. As a matter of fact, the novel formulation is optimized specifically for hydrogen, which, at the instant of injection, is a compressible gas with a significantly higher sensitivity to pressure variation than kerosene, a liquid fuel. Therefore, for the ATR, the same influence on EINO is obtained with a smaller change in pressure than in a conventional turbofan fueled with kerosene.
- ◦
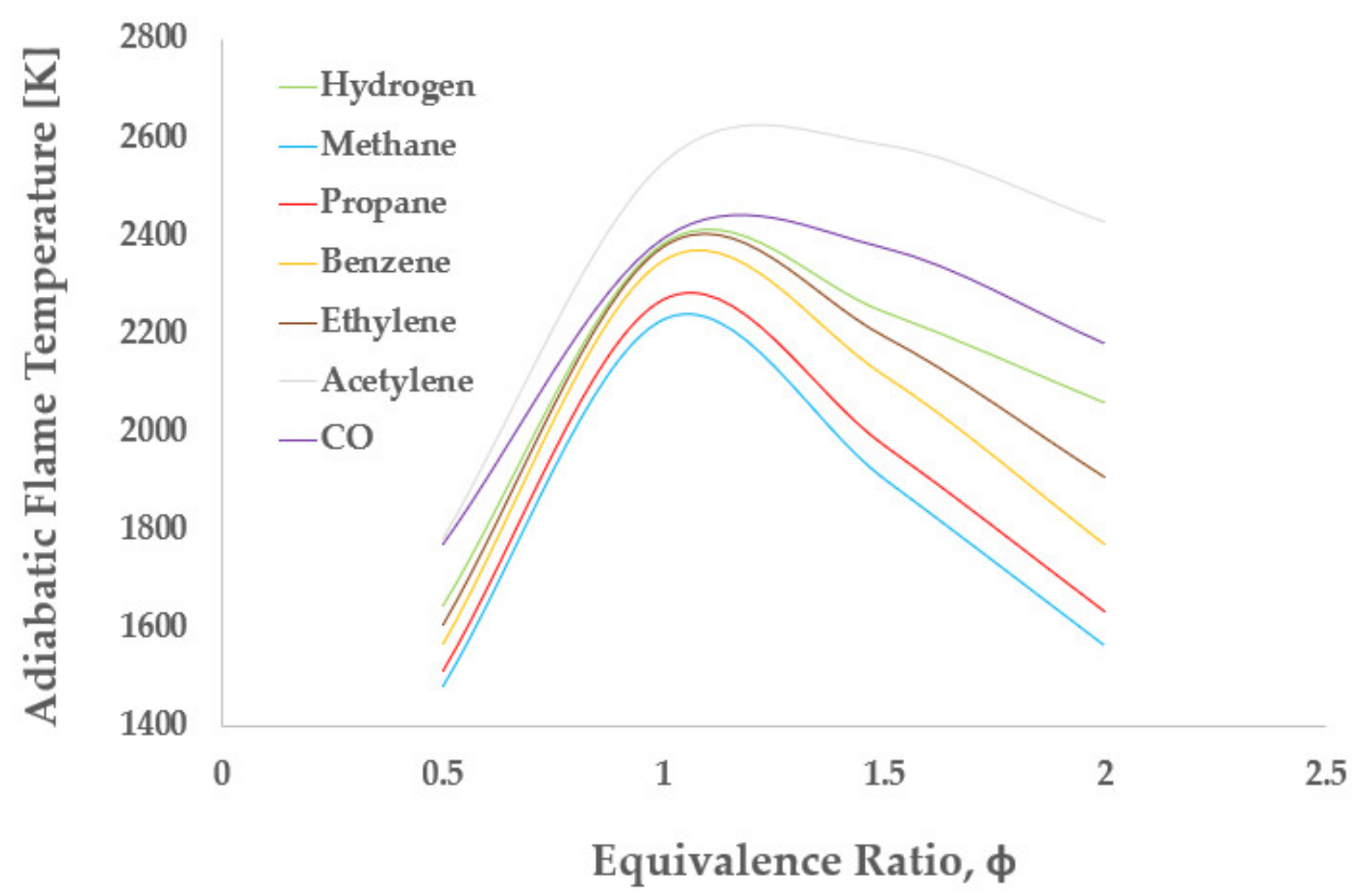
- The value of c is emblematic of a positive contribution of FAR: as a matter of fact, the NOx emissions rise as a result of the increase in the flame temperature caused by the enhancement of FAR. It is worth remembering that the NOx production is greatest for a stoichiometric mixture, while for a lean and rich mixture it gradually reduces. Since the ATR operates at fuel-lean conditions, the increase in FAR mentioned above is intended up to , so that φ < 1. The reason behind the different influence of the FAR term with respect to the original P3-T3 method is still ascribed to the nature of the fuel. Indeed, the FAR related to kerosene is the result of a tradeoff analysis for the minimization of both CO/CO2 and NOx emissions, which respectively decrease and increase as the mixture approaches the stoichiometric conditions. However, since H2 does not generate carbon-related emissions, the FAR has a higher impact since it optimizes both the thrust and the NOx emissions. Therefore, the selection of the FAR is subject to fewer constraints and the parameter has a higher variability.
- ◦
- The Mach number also has a favorable effect for the NOx formation, since its increase leads to a higher combustion temperature causing a rise in EINO. From the optimization of the exponents, results indicate that the Mach number itself counts in the measure of a cube root with the additional contribution by coefficient a. This parameter is inserted since it is a direct indicator of the variation in flight conditions, as explained above.
- ◦
- The Damköhler number is also responsible for a positive impact on EINO levels, meaning the NOx emissions increase as a consequence of the enhancement of the Damköhler number, which generally occurs through an increase in residence time, since the ignition delay is determined based on the chemical composition of the mixture. This parameter is included to account for the matching between the residence and ignition times, which have a strong influence on the formation of NOx in supersonic and hypersonic engines. As a matter of fact, a higher causes a temperature rise in the combustor resulting in an increase in EINO; thus, a value of Da approaching unity is desirable for NOx minimization purposes.
6. Conclusions
- For the first time, a comprehensive review of emissions estimation techniques and their applicability beyond traditional subsonic aeroengines and fuels is reported in a scientific publication, providing the readers with useful and practical guidelines for the selection of the most appropriate technique for predicting emissions.
- The paper exploits a unique dataset, which includes the propulsive and emissive database of the ATR covering very different operating conditions, from takeoff up to a wide range of cruise Mach numbers ranging from 0.3 to 4.
- The novel H2-P3T3 method follows an approach similar to the original P3-T3 method, which allows the prediction of in-flight emissions knowing the emissions at sea-level conditions and the ratios of the flight-level and sea-level conditions of the pressure and fuel-to-air ratio at the inlet of the combustion chamber. However, the introduction of new parameters (i.e., Da) in the analytical formulations produces a modification of the original method, requiring the definition of additional sea-level trends for additional variables, including the ignition delay time and the residence time.
- The original P3-T3 method presents a single analytical formulation with tuneable parameters to effectively represent different engine architectures. The new H2-P3T3 method encompasses three different formulations for the same engine architecture (ATR). The different formulations provide an increasing level of accuracy in the predictions, thus allowing a more flexible application throughout the design process.
- The introduction of new correlated factors to improve the analytical formulations is based on the analysis of the most effective NOx minimization strategies adopted for hydrogen combustion of high-speed aviation propulsive systems.
- The most complete formulation reveals an important scientific finding: emissions of nitrogen oxides from a high-speed engine using hydrogen are well correlated to the Da number, thus they are strongly affected by the characteristic times of the combustion process.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lai, Y.Y.; Christley, E.; Kulanovic, A.; Teng, C.C.; Björklund, A.; Nordensvärd, J.; Karakaya, E.; Urban, F. Analysing the opportunities and challenges for mitigating the climate impact of aviation: A narrative review. Renew. Sustain. Energy Rev. 2022, 156, 111972, ISSN 1364-0321. [Google Scholar] [CrossRef]
- Grewe, V.; Gangoli Rao, A.; Grönstedt, T.; Xisto, C.; Linke, F.; Melkert, J.; Middel, J.; Ohlenforst, B.; Blakey, S.; Christie, S.; et al. Evaluating the climate impact of aviation emission scenarios towards the Paris agreement including COVID-19 effects. Nat. Commun. 2021, 12, 3841. [Google Scholar] [CrossRef]
- Intergovernmental Panel on Climate Change (IPCC) (Ed.) Climate Change 2022—Mitigation of Climate Change: Working Group III Contribution to the Sixth Assessment Report of the Intergovernmental Panel on Climate Change; Cambridge University Press: Cambridge, UK, 2023. [Google Scholar] [CrossRef]
- Thomson, R.; Weichenhain, U.; Sachdeva, N.; Kaufmann, M. Hydrogen|A Future Fuel for Aviation? Roland Berger Study; Roland Berger GMBH: Munich, Germany, 2020. [Google Scholar]
- EUROCONTROL. EUROCONTROL Forecast Update 2022–2024. 2022. Available online: https://www.eurocontrol.int/publication/eurocontrol-forecast-update-2022-2024 (accessed on 10 October 2022).
- Ram, M.; Bogdanov, D.; Aghahosseini, A.; Khalili, S.; Child, M.; Fasihi, M.; Traber, T.; Breyer, C. European Energy System Based on 100% Renewable Energy—Transport Sector. In Mobilität der Zukunft; ATZ/MTZ-Fachbuch; Siebenpfeiffer, W., Ed.; Springer Vieweg: Berlin/Heidelberg, Germany, 2021. [Google Scholar] [CrossRef]
- Chandrasekaran, N.; Guha, A. Study of Prediction Methods for NOx Emission from Turbofan Engines. J. Propuls. Power 2012, 28, 170–180. [Google Scholar] [CrossRef]
- Rizk, N.K.; Mongia, H.C. Semianalytical Correlations for NOx, CO and UHC emissions. Trans. ASME 1993, 115, 612–619. [Google Scholar] [CrossRef]
- ICAO. Annex 16 Volume II Aircraft Engine Emissions; ICAO: Montreal, QC, Canada, 2014.
- Silberhorn, D.; Dahlmann, K.; Görtz, A.; Linke, F.; Zanger, J.; Rauch, B.; Methling, T.; Janzer, C.; Hartmann, J. Climate impact reduction potentials of synthetic kerosene and green hydrogen powered mid-range aircraft concepts. Appl. Sci. 2022, 12, 5950. [Google Scholar] [CrossRef]
- DuBois, D.; Paynter, G.C. ‘Fuel Flow Method2’ for Estimating Aircraft Emissions. SAE Trans. 2006, 115, 1–14. Available online: https://www.jstor.org/stable/44657657 (accessed on 21 September 2023).
- Dinc, A. NOx emissions of turbofan powered unmanned aerial vehicle for complete flight cycle. Chin. J. Aeronaut. 2020, 33, 1683–1691, ISSN 1000-9361. [Google Scholar] [CrossRef]
- Wang, Y.; Yin, H.; Zhang, S.; Yu, X. Multi-objective optimization of aircraft design for emission and cost reductions. Chin. J. Aeronaut. 2014, 27, 52–58, ISSN 1000-9361. [Google Scholar] [CrossRef]
- Fusaro, R.; Viola, N.; Galassini, D. Sustainable Supersonic Fuel Flow Method: An Evolution of the Boeing Fuel Flow Method for Supersonic Aircraft Using Sustainable Aviation Fuels. Aerospace 2021, 8, 331. [Google Scholar] [CrossRef]
- Viola, N.; Fusaro, R.; Saracoglu, B.; Schram, C.; Grewe, V.; Martinez, J.; Marini, M.; Hernandez, S.; Lammers, K.; Vincent, A.; et al. Main Challenges and Goals of the H2020 STRATOFLY Project. Aerotec. Missili Spaz. 2021, 100, 95–110. [Google Scholar] [CrossRef]
- Viola, N.; Fusaro, R.; Ferretto, D.; Gori, O.; Saracoglu, B.; Ispir, A.C.; Schram, C.; Grewe, V.; Plezer, J.F.; Martinez, J.; et al. H2020 STRATOFLY project: FROM Europe to Australia in less than 3 hours. In Proceedings of the 32nd Congress of the International Council of the Aeronautical Sciences, ICAS 2021, Shanghai, China, 6–10 September 2021. [Google Scholar]
- Viola, N.; Fusaro, R.; Gori, O.; Marini, M.; Roncioni, P.; Saccone, G.; Saracoglu, B.; Ispir, A.C.; Fureby, C.; Nilsson, T.; et al. Stratofly mr3—How to reduce the environmental impact of high-speed transportation. In Proceedings of the AIAA Scitech 2021 Forum, Virtual, 11–15 and 19–21 January 2021; pp. 1–21. [Google Scholar]
- Steelant, J.; Varvill, R.; Walton, C.; Defoort, S.; Hannemann, K.; Marini, M. Achievements Obtained for Sustained Hypersonic Flight within the LAPCAT-II project. In Proceedings of the 20th AIAA International Space Planes and Hypersonic Systems and Technologies Conference, Glasgow, UK, 6–9 July 2015. [Google Scholar]
- Villace, V.F.; Steelant, J. The Thermal Paradox of Hypersonic Cruisers. In Proceedings of the 20th AIAA International Space Planes and Hypersonic Systems and Technologies Conference, Glasgow, UK, 6–9 July 2015; p. 14. [Google Scholar]
- Langener, T.; Erb, S.; Steelant, J. Trajectory Simulation and Optimization of the LAPCAT MR2 Hypersonic Cruiser Concept. In Proceedings of the 29th Congress of the International Council of the Aeronautical Sciences, St. Petersburg, Russia, 7–12 September 2014. [Google Scholar]
- Saccone, G.; Ispir, A.C.; Saracoglu, B.H.; Cutrone, L.; Marini, M. Computational evaluations of emissions indexes released by the STRATOFLY air-breathing combined propulsive system. Aircr. Eng. Aerosp. Technol. 2022, 94, 1499–1507. [Google Scholar] [CrossRef]
- Ispir, A.C.; Gonçalves, P.; Kurban, E.; Saracoglu, B.H. Thermodynamic efficiency analysis and investigation of exergetic effectiveness of stratofly mr3 aircraft propulsion plant. In Proceedings of the AIAA Scitech 2020 Forum, Orlando, FL, USA, 6–10 January 2020. [Google Scholar] [CrossRef]
- Nista, L.; Saracoglu, B.H. Numerical investigation of the STRATOFLY MR3 propulsive nozzle during supersonic to hypersonic transition. In Proceedings of the AIAA Propulsion and Energy Forum and Exposition, Indianapolis, IN, USA, 19–22 August 2019. [Google Scholar] [CrossRef]
- Ozden, A.; Nista, L.; Saracoglu, B.H. Performance evaluations of the stratofly mr3 propulsive nozzle at supersonic speeds. In Proceedings of the AIAA Propulsion and Energy 2020 Forum, Virtual, 24–28 August 2020; pp. 1–14. [Google Scholar] [CrossRef]
- Ferretto, D.; Fusaro, R.; Viola, N. Propellant subsystem design for hypersonic cruiser exploiting liquid hydrogen. In Proceedings of the AIAA AVIATION 2022 Forum, Chicago, IL, USA, 27 June–1 July 2022. [Google Scholar] [CrossRef]
- Viola, N.; Roncioni, P.; Gori, O.; Fusaro, R. Aerodynamic characterization of hypersonic transportation systems and its impact on mission analysis. Energies 2021, 14, 3580. [Google Scholar] [CrossRef]
- Roncioni, P.; Cutrone, L.; Marini, M. Aeropropulsive characterization of the hypersonic cruiser vehicle in stratofly project. In Proceedings of the 33rd Congress of the International Council of the Aeronautical Sciences, ICAS 2022, Stockholm, Sweden, 4–9 September 2022; Volume 6, pp. 4564–4575. [Google Scholar]
- Ferretto, D.; Gori, O.; Fusaro, R.; Viola, N. Integrated Flight Control System Characterization Approach for Civil High-Speed Vehicles in Conceptual Design. Aerospace 2023, 10, 495. [Google Scholar] [CrossRef]
- Rodríguez-Segade, M.; Hernández, S.; Díaz, J. Multi-bubble scheme and structural analysis of a hypersonic stratospheric flight vehicle. Aerosp. Sci. Technol. 2022, 124, 107514. [Google Scholar] [CrossRef]
- Rodríguez-Segade, M.; Hernández, S.; Amenedo, D.; Díaz, J. An Application of single and multi-objective optimization to the design of the hypersonic STRATOFLY-MR3 vehicle. In Proceedings of the AIAA Aviation and Aeronautics Forum and Exposition, AIAA AVIATION Forum 2021, Virtual, 2–6 August 2021. [Google Scholar] [CrossRef]
- Fernández-Villacé, V.; Paniagua, G.; Steelant, J. Installed performance evaluation of an air turbo-rocket expander engine. Aerosp. Sci. Technol. 2014, 35, 63–79, ISSN 1270-9638. [Google Scholar] [CrossRef]
- Goodwin, D.; Moffat, H.K.; Speth, R.L. Cantera: An Object-oriented Software Toolkit for Chemical Kinetics, Thermodynamics, and Transport Processes, (Version 2.6). 2023. Available online: http://www.cantera.org (accessed on 20 September 2023).
- Piscitelli, F.; Cutrone, L.; Pezzella, G.; Roncioni, P.; Marini, M. Nose-to-tail analysis of an air-breathing hypersonic vehicle using an in-house simplified tool. Acta Astronaut. 2017, 136, 148–158. [Google Scholar] [CrossRef]
- Tang, Y.; Kim, J.; Sforzo, B.; Scarcelli, R.; Raman, V. Numerical and Experimental Study of an Aircraft Igniter Plasma Jet Discharge. J. Propuls. Power 2023, 1–16. [Google Scholar] [CrossRef]
- Zettervall, N.; Fureby, C. Computational Study of Ramjet, Scramjet and Dual Mode Ramjet/Scramjet Combustion in a Combustor with a Cavity Flameholder. In Proceedings of the AIAA Aerospace Science, Kissimmee, FL, USA, 8–12 January 2018. [Google Scholar] [CrossRef]
- Saccone, G.; Natale, P.; Cutrone, L.; Marini, M. Hydrogen/Air Supersonic Combustion Modelling and Validation for Scramjet Applications. J. Fluid Flow Heat Mass Transf. (JFFHMT) 2022, 9, 136–147. [Google Scholar] [CrossRef]
- Ingenito, A. NOx reduction strategies in scramjet combustion. Aerosp. Sci. Technol. 2016, 59, 189–198. [Google Scholar] [CrossRef]
- Pouech, P.; Duchaine, F.; Poinsot, T. Premixed flame ignition in high-speed flows over a backward facing Step. Combust. Flame 2021, 229, 111398. [Google Scholar] [CrossRef]
- Fedorov, A.V.; Fedorova, N.N.; Vankova, O.S.; Tropin, D.A. Verification of kinetic schemes of hydrogen ignition and combustion in air. AIP Conf. Proc. 2018, 1939, 020019. [Google Scholar] [CrossRef]
- Chiesa, S.; Farfaglia, S.; Fioriti, M.; Viola, N. Design of all electric secondary power system for future advanced medium altitude long endurance unmanned aerial vehicles. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2012, 226, 1255–1270. [Google Scholar] [CrossRef]
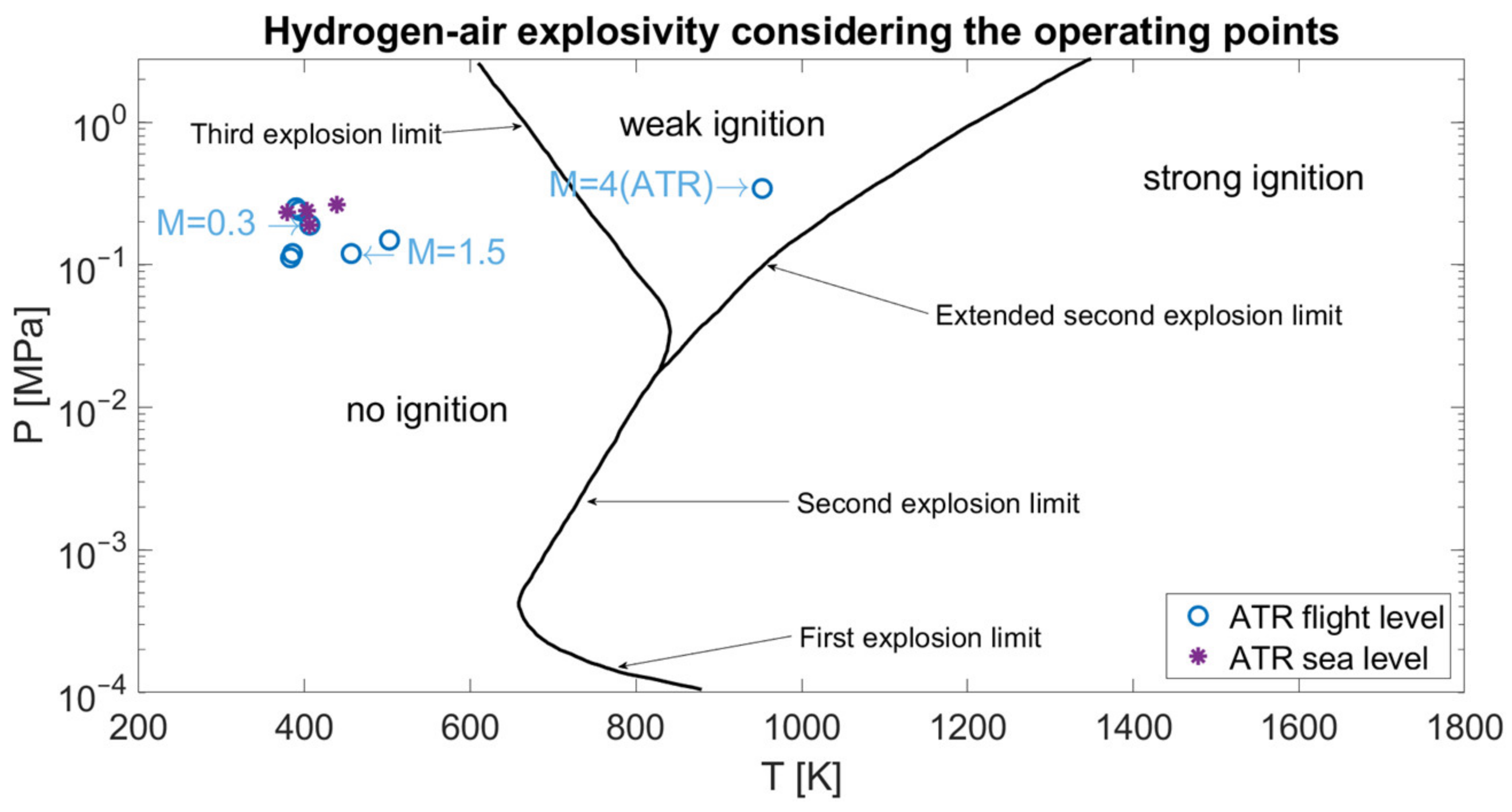

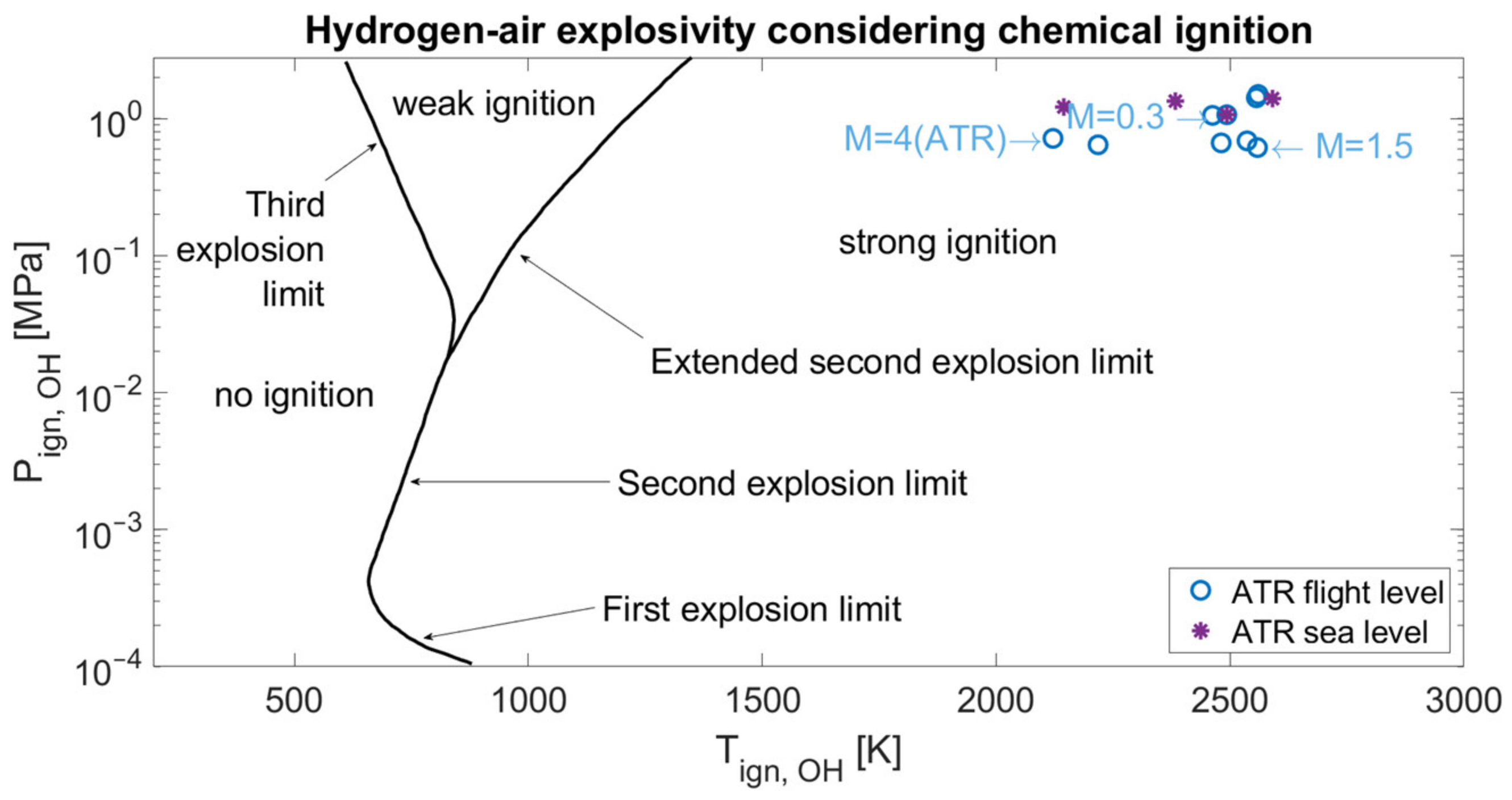




| From Propulsive Database | From Emissive Database | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| M | Z (m) | mfuel (kg/s) | mair (kg/s) | FAR | phi | T3,air (K) | T3,fuel (K) | T3,mix (K) | p3 (Pa) | H | EINO (gNO/kgH2) |
| 0.3 | 0 | 8.88 | 401.41 | 0.022 | 0.759 | 363 | 542 | 406.01 | 190,000 | −0.0307 | 2.12 |
| 0.35 | 0 | 10.09 | 463.83 | 0.022 | 0.759 | 393 | 583 | 438.66 | 265,000 | −0.0307 | 1.84 |
| 0.44 | 0 | 13.63 | 588.74 | 0.023 | 0.793 | 390 | 441 | 402.67 | 240,000 | −0.0307 | 2.37 |
| 0.5 | 0 | 14.41 | 662.62 | 0.022 | 0.759 | 380 | 377 | 379.28 | 232,000 | −0.0307 | 1.4 |
| From Propulsive Database | From Emissive Database | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| M | Z (m) | mfuel (kg/s) | mair (kg/s) | FAR | phi | T3,air (K) | T3,fuel (K) | T3,mix (K) | p3 (Pa) | H | EINO (gNO/kgH2) |
| 0.3 | 400 | 8.88 | 384.49 | 0.023 | 0.793 | 366 | 529 | 406.51 | 190,000 | −0.0133 | 2.8 |
| 0.3 | 800 | 8.88 | 368.13 | 0.024 | 0.828 | 368 | 517 | 406.22 | 190,000 | 0.0025 | 3.42 |
| 0.44 | 2000 | 13.65 | 472.78 | 0.029 | 1.000 | 402 | 374 | 393.76 | 240,000 | 0.0411 | 5.8 |
| 0.5 | 2500 | 14.35 | 507.76 | 0.028 | 0.966 | 410 | 340 | 389.91 | 251,000 | 0.0538 | 5.43 |
| 0.75 | 8000 | 6.62 | 390.10 | 0.017 | 0.586 | 355 | 510 | 385.44 | 120,000 | 0.1154 | 7.19 |
| 0.82 | 8921 | 7.06 | 306.33 | 0.023 | 0.793 | 363 | 444 | 383.13 | 112,000 | 0.117 | 2.76 |
| 1.5 | 16134 | 6.61 | 231.64 | 0.029 | 1.000 | 469 | 426 | 456.35 | 120,000 | 0.119 | 10.82 |
| 2 | 17411 | 8.53 | 271.10 | 0.031 | 1.069 | 550 | 396 | 502.52 | 149,000 | 0.119 | 11.47 |
| 4 | 24152 | 2.68 | 177.65 | 0.015 | 0.517 | 938 | 1019 | 952.37 | 343,000 | 0.1182 | 4.34 |
| Mach | τres [s] | τign,OH [s] | Da |
|---|---|---|---|
| 0.3 | 6.442 × 10−1 | 2.235 × 10−2 | 28.83 |
| 0.35 | 7.200 × 10−1 | 2.642 × 10−2 | 27.25 |
| 0.44 | 5.534 × 10−1 | 2.782 × 10−2 | 19.89 |
| 0.5 | 5.103 × 10−1 | 2.970 × 10−2 | 17.18 |
| Mach | τres (s) | τign,OH (s) | Da |
|---|---|---|---|
| 0.3 | 6.640 × 10−1 | 2.226 × 10−2 | 29.83 |
| 0.3 | 6.862 × 10−1 | 2.225 × 10−2 | 30.85 |
| 0.44 | 6.627 × 10−1 | 2.854 × 10−2 | 23.22 |
| 0.5 | 6.556 × 10−1 | 3.018 × 10−2 | 21.72 |
| 0.75 | 4.669 × 10−1 | 1.601 × 10−2 | 29.16 |
| 0.82 | 5.214 × 10−1 | 1.501 × 10−2 | 34.73 |
| 1.5 | 5.854 × 10−1 | 1.196 × 10−2 | 48.96 |
| 2 | 5.476 × 10−1 | 1.224 × 10−2 | 44.73 |
| 4 | 1.213 × 10 | 1.457 × 10−3 | 832.41 |
| a | b | c | d | f | |
|---|---|---|---|---|---|
| Original formulation | 1 | 0.4 | - | - | - |
| 1 | −0.3614 | 3.8132 | - | - | |
| 1.5996 | 0.3187 | 3.5 | 0.3143 | - | |
| 1.8110 | 0.2273 | 2.4276 | 0.3299 | 0.7742 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Viola, N.; Fusaro, R.; Saccone, G.; Borio, V. Analytical Formulations for Nitrogen Oxides Emissions Estimation of an Air Turbo-Rocket Engine Using Hydrogen. Aerospace 2023, 10, 909. https://doi.org/10.3390/aerospace10110909
Viola N, Fusaro R, Saccone G, Borio V. Analytical Formulations for Nitrogen Oxides Emissions Estimation of an Air Turbo-Rocket Engine Using Hydrogen. Aerospace. 2023; 10(11):909. https://doi.org/10.3390/aerospace10110909
Chicago/Turabian StyleViola, Nicole, Roberta Fusaro, Guido Saccone, and Valeria Borio. 2023. "Analytical Formulations for Nitrogen Oxides Emissions Estimation of an Air Turbo-Rocket Engine Using Hydrogen" Aerospace 10, no. 11: 909. https://doi.org/10.3390/aerospace10110909
APA StyleViola, N., Fusaro, R., Saccone, G., & Borio, V. (2023). Analytical Formulations for Nitrogen Oxides Emissions Estimation of an Air Turbo-Rocket Engine Using Hydrogen. Aerospace, 10(11), 909. https://doi.org/10.3390/aerospace10110909








