Axial symmetry of the potential considerably simplifies the finding of trajectories because particle motion in the polar direction is uncoupled from the motion in the radial directions. In the case of the motion of quantum particles, such separation is impossible. However, axial symmetry can be used to reduce the number of considered impact parameters, since wave functions for rotationally equivalent impact parameters can be generated by the application of the rotation operator.
In the first subsection, the manifestations of the classical rainbow effect will be explained on the example of the proton channeling in SWCNT. Subsequent subsections will be devoted to the analysis of the quantum rainbow channeling of positrons.
3.1. Interpretation of the Classical Rainbow Effect
Here we consider the transmission of the parallel, monochromatic, 1-GeV proton beam through SWCNT (11, 9), which is perfectly aligned with nanotube axis. The radius of the SWCNT is nm, and the number of its atomic strings is . Standard deviation of the carbon thermal motion can be estimated from the Debye theory, which for the room temperature (T = 300 K) gives nm. Screening length of carbon atom is nm.
The motion of the protons in the longitudinal direction is relativistic while its motion in the transverse direction is classical. The equations of motion (
11) still hold. The only differences are that
m should be replaced by the protons relativistic mass
(
), and the relationship between initial kinetic energy
and longitudinal linear momentum
is
, where
c is the speed of light [
9]. The relativistically corrected critical angle is
mrad.
We consider only protons whose impact parameters satisfy inequality
. Since the proton beam is aligned with the SWCNT axes, each proton trajectory is confined to a plane defined by the impact parameter
and the nanotube axis.
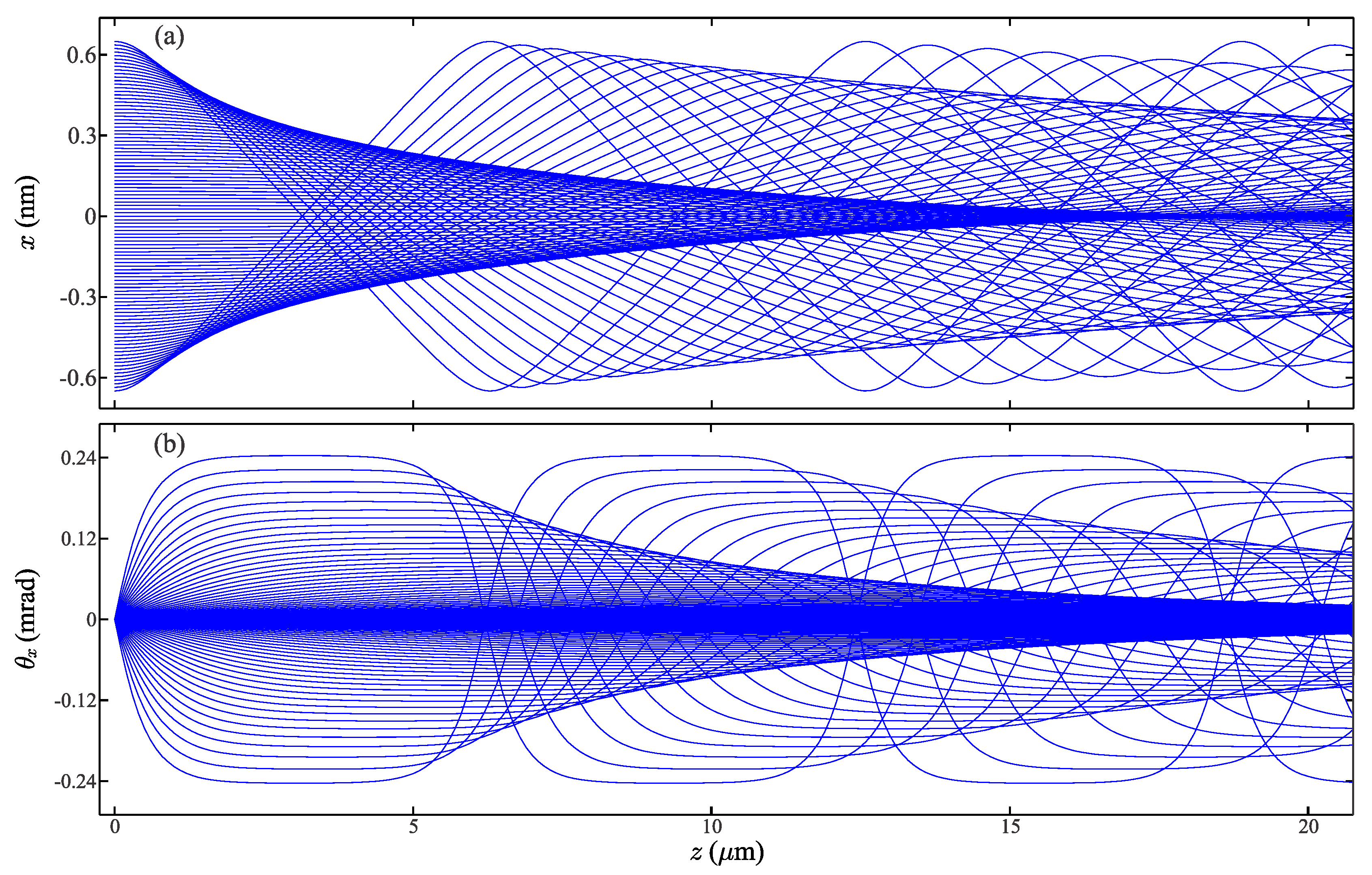
Figure 3 shows the obtained proton trajectories in the
plane. Newton’s equations of motion (
11) were solved by Runge-Kutta method of the 4-th order [
41]. Note that the amplitude of any proton trajectory is constant and corresponds to its impact parameter
. The corresponding trajectories in the angular space are shown in
Figure 3b. The amplitude of any trajectory is also constant. Note that the maximal deflection angle of
corresponds to the trajectory of impact parameter
. All these facts demonstrate that proton trajectories were accurately calculated.
Proton trajectories shown in
Figure 3a,b can be concisely represented as function
,
, depending on the parameter
. For SWCNT of length
L functions
,
define mappings of the impact parameter plane to the final transmitted position and final transmitted angle plane, called the spatial and angular transmission function, respectively, which will be denoted as
and
. The symmetry of the trajectory family requires that both transmission functions are odd functions
,
. Rainbow defining condition (
13) now reduces to
Therefore, the critical points of transmission functions, which occur in symmetrical pairs, are rainbow points. Each critical point pair corresponds to the one circular rainbow line whose radius is equal to the absolute value of the critical point ordinate.
For the 10-
m long SWCNT (11, 9) angular transmission function
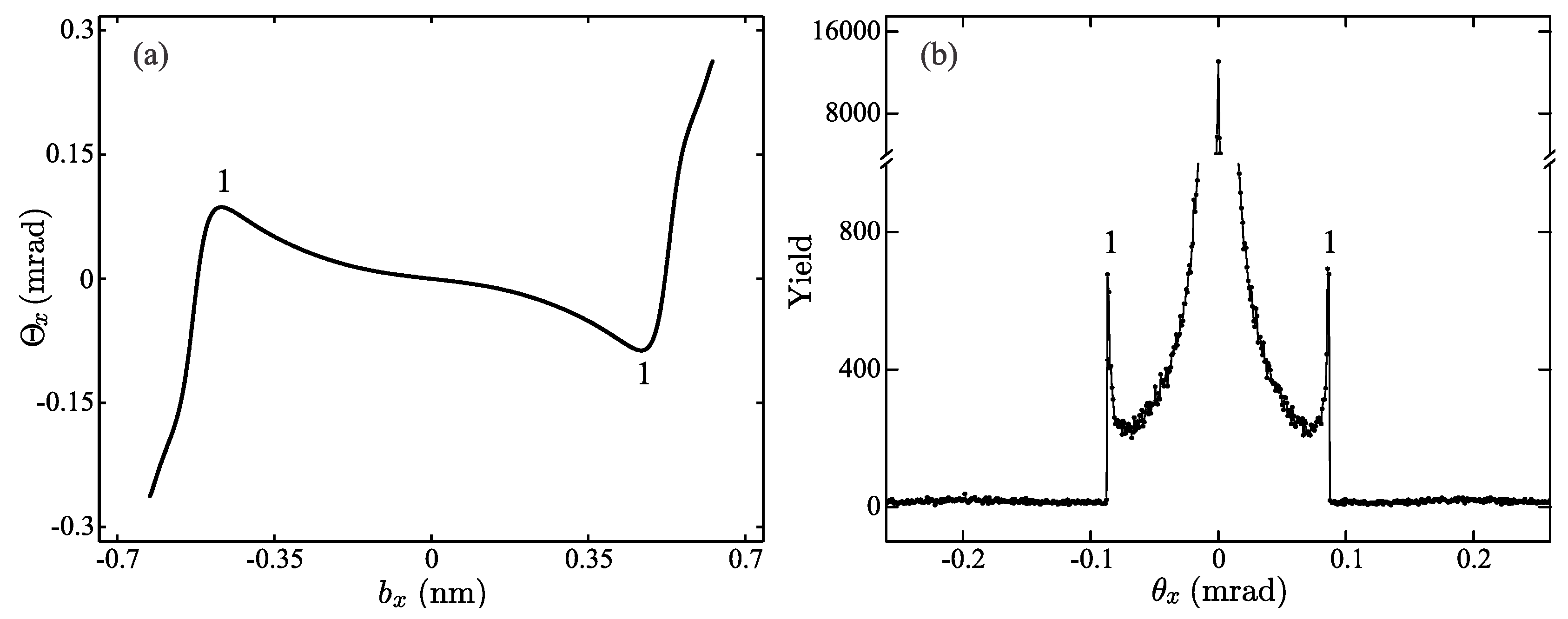
, shown in
Figure 4a, has only one critical point pair
= (
nm, 0.856 mrad), and
nm,
mrad), both labeled 1. This function shows that for any
from the interval
mrad, there are three corresponding impact parameters, while outside of this interval the correspondence is one-to-one. Therefore, there is only one circular angular rainbow line of radius 0.856 mrad. The interior of the line is the rainbow’s bright side, while its exterior is the rainbow’s dark side.
The vertical slice through the corresponding angular distribution is shown in
Figure 4b. The initial number of protons was 16,655,140, while the size of the bin in the
space was 0.866 μrad. Note the small statistical fluctuation of the obtained distribution which reflects the randomness of the impact parameter selection process. Besides this the obtained distribution is perfectly axially symmetric. This distribution contains three prominent peaks. The central peak is the consequence of the fact that potential
has its minimum at the coordinate origin. It represents the undeflected part of the proton beam, and it is not related to the rainbow effect. The two remaining peaks are located symmetrically around the central maximum. Angular positions of the critical points form
Figure 4a are indicated by the number 1. It is obvious that their positions are in the perfect correspondence with the positions of the mentioned peaks. Note also high particle yield inside, and low yield outside the interval enclosed by the observed peak pair, which corresponds to the rainbow light and dark sides. Therefore, the angular distribution contains one circular rainbow line whose properties are determined by the critical points of the transmission function.
3.2. Semi-Classical Interpretation of Quantum Rainbow Effect
This subsection is devoted to the analysis of the channeling of 1-MeV positrons through 200-nm long chiral SWCNT (14, 4). The radius of this nanotube is
nm, while the number of atomic strings is
. Longitudinal motion of 1-MeV positrons is free and relativistic (
) while transverse motion is quantum and nonrelativistic. As in the previous example, Equation (
16) still holds if bear mass
m is replaced with relativistic mass
[
9]. To observe channeling effect one need to use positron beam collimated better then critical channeling angle
. Here we assume that the incoming positron beam has angular standard deviation
, which gives
mrad and
nm. This value was selected because then transverse size of any wave packet is large enough that the self-interference effect becomes significant, while on the other hand it is small enough to allow explicit dependence of their dynamics on impact parameters to be analyzed. Let
M represent the number of wave packets uniformly covering impact parameter plane. Expansion coefficients from Equation (
19) are
. In order that
be a uniform distribution in the entrance plane of the nanotube, the number of wave packets
M must be very large (theoretically infinite). In order to minimize the number
M an algorithm was devised which optimize the values of the coefficients
while keeping the difference between the distribution
and uniform distribution
in the region
below some prescribed tolerance. We have found that accurate representation of the initial distribution of the positron beam can be accomplished with only 142 Gaussian wave packets.
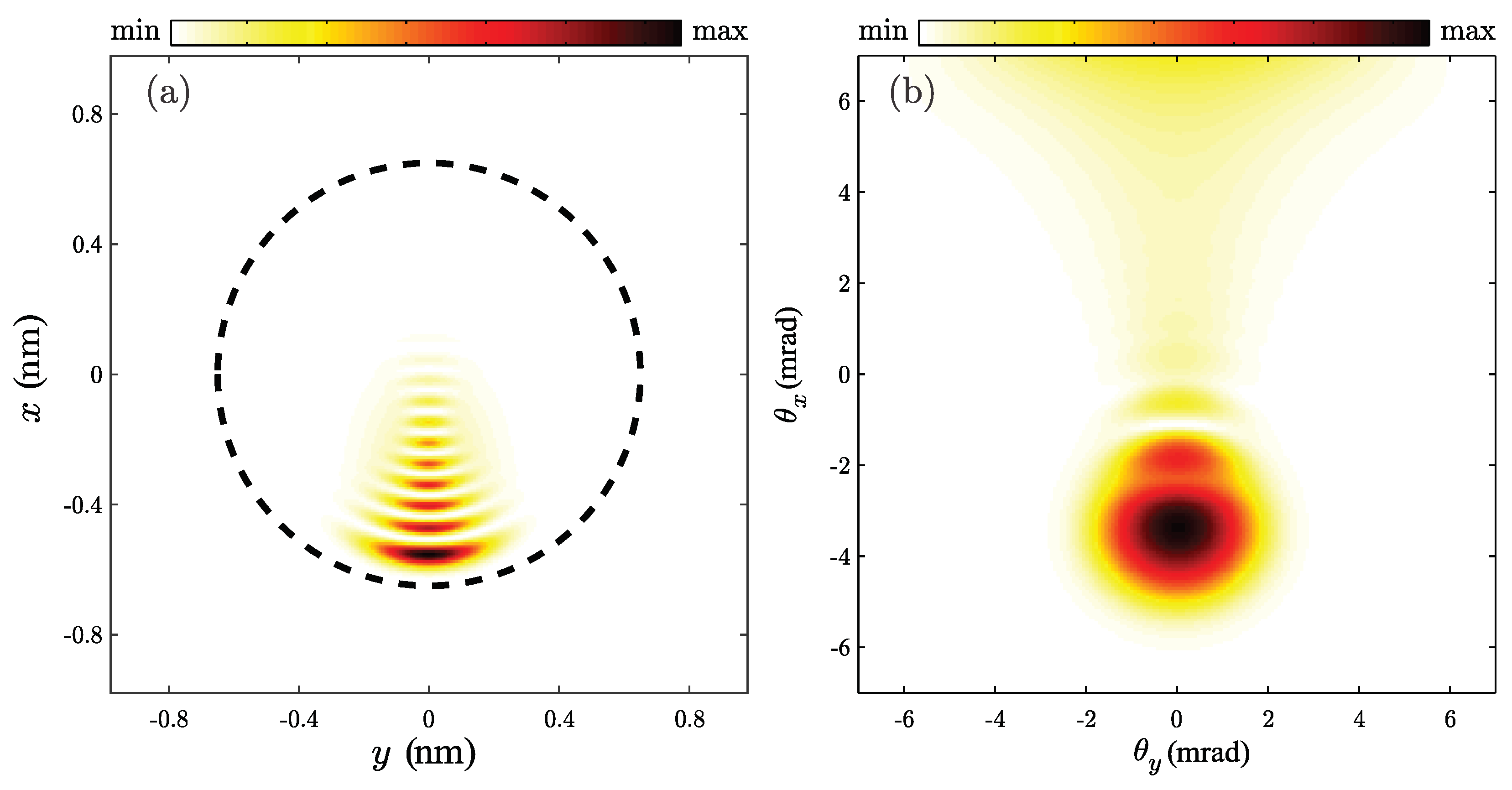
Let us examine the motion of the wave packet of impact parameter
nm. The corresponding Schrödinger Equation (
16) is solved using the method of Chebyshev global propagation [
42]. The obtained probability densities at the exit of the SWCNT are shown in
Figure 5. In both representations, densities have a number of peaks, which can be attributed to self-interference of the incoming part of wave function with the part of wave function already reflected from SWCNT wall. However, since all peaks are the consequence of the wave packet self-interference looking only on the numerically obtained probability densities, it is very difficult to say which peak is connected with the rainbow effect, and which one is a simple manifestation of the positron wave nature.
In order to understand and classify self-interference peaks, a semiclassical approach can be applied. To avoid unnecessary complications here we will focus only on the vertical slices of the wave packets moving along the
x axis. Transmission functions
and
then fully characterize the classical motion of the particle. In the phase space, those two functions define a curve called the rainbow diagram which is shown in
Figure 6a as a thin black line. The transmission function
has two pairs of critical points labeled
and
whose ordinates are
nm, and
nm, respectively, while transmission function
has only one pair of critical points labeled
whose ordinates are
mrad. The positions of the rainbow points in the rainbow diagram are indicated by the black arrows. Note that at rainbow points, the tangents of the rainbow diagram are vertical or horizontal. They represent points where different branches of the mappings
, and
, respectively, meet. Therefore, mapping
has 5 branches, while mapping
has only 3.
In the semiclassical approach the quantum wave function at the time
t can be constructed from classical rainbow diagram as a sum of the contributions of the wave trains coming from all branches [
43]. The contribution of the branch
is of the form
. Let density of the trajectories in the impact parameter plane be
. The number of particles in the interval
around
x coming from the branch
is equal to the number of particles in the interval
around the point
mapped to the corresponding interval. Therefore,
, where
is the density of the points in the branch
. The phase
is a type 2 canonical transformation
in the spatial representation and type 1 canonical transformation
in the angular representation, of the branch
. Therefore, the total semiclassical wave functions in spatial and angular representations are given by the expressions
where indices
, and
count branches of the spatial and angular transmission functions
, and
, respectively,
, and
are referent points of the
-th and
-th branch respectively. Note that
stands for the inverse function of the branch
of spatial transmission function, while
is inverse function of the branch
of the angular transmission function. In the spatial representation the sum should be taken over all branches satisfying equation
, while in the angular representation the sum is over all branches satisfying equation
.
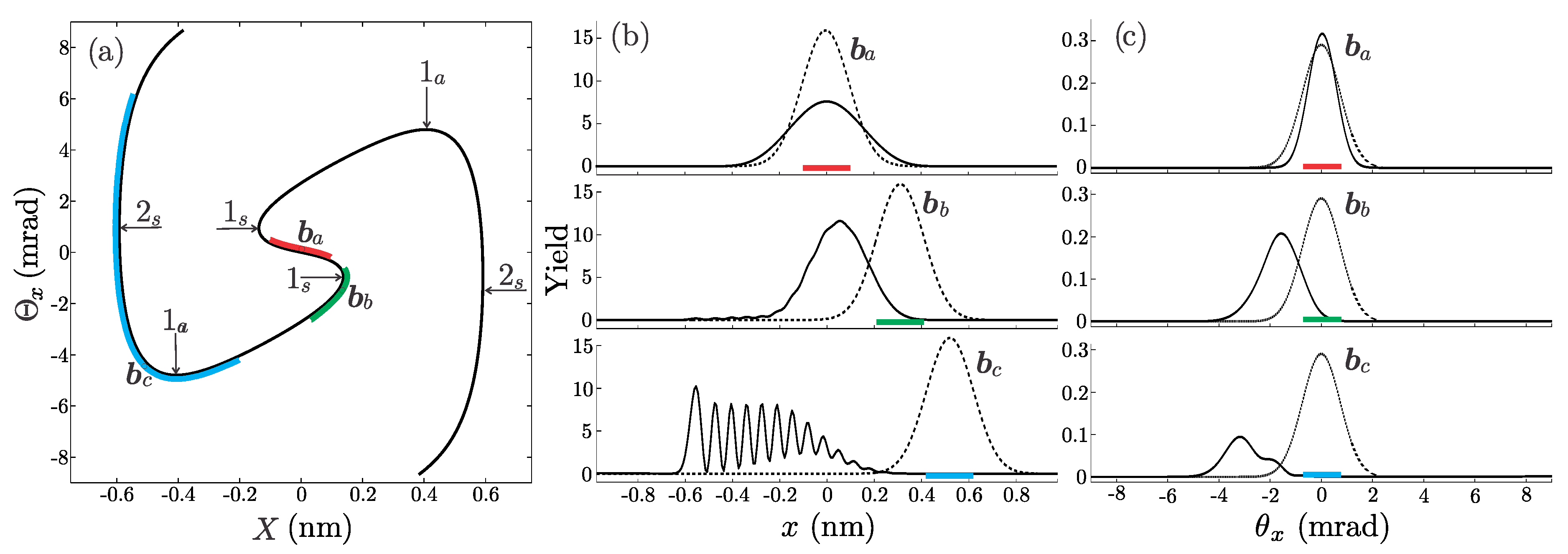
Let us now apply semiclassical reasoning for interpretation of the quantum motion of wave packets labeled
a,
b and
c of impact parameters
= (0, 0),
= (0.31, 0), and
= (0.52, 0) nm, respectively. Their spatial, and angular representations are shown in
Figure 6b,c respectively. Wave packets in the impact parameter plane are shown by the dashed lines, wave packets in the exit plane of the SWCNT are shown by the solid line. Since the initial distributions are Gaussian, the dominant contribution comes from trajectories from the interval
. For reasons of simplicity, the contributions of all other trajectories will be neglected. The dominant intervals are in
Figure 6b shown by the red, green, and blue lines, respectively. In the angular space, the dominant intervals of length of
are in
Figure 6c designated by the same colors. The corresponding exit positions of the trajectories from the dominant intervals are in
Figure 6a indicated by thick red, green and blue lines, respectively. For wave packer
a, the red curve in
Figure 6a covers only one branch of the mappings
and
, respectively. This is the reason why for wave packet
a self-interference is not observable. For wave packet
b the green line in
Figure 6a covers one branch of mapping
completely and slightly extends into the second branch, and covers only one branch of the mapping
. Therefore, the number of trajectories which interfere is small. This explains why in the spatial representation for wave packet
b only weak self-interference can be observed, and there is no observable self-interference in the angular representation. For wave packet
c the blue curve in
Figure 6a covers two branches of mappings
and
, respectively. In this case, the number of trajectories which interfere is large. This fact explains why self-interference is the strongest for wave packet
c.
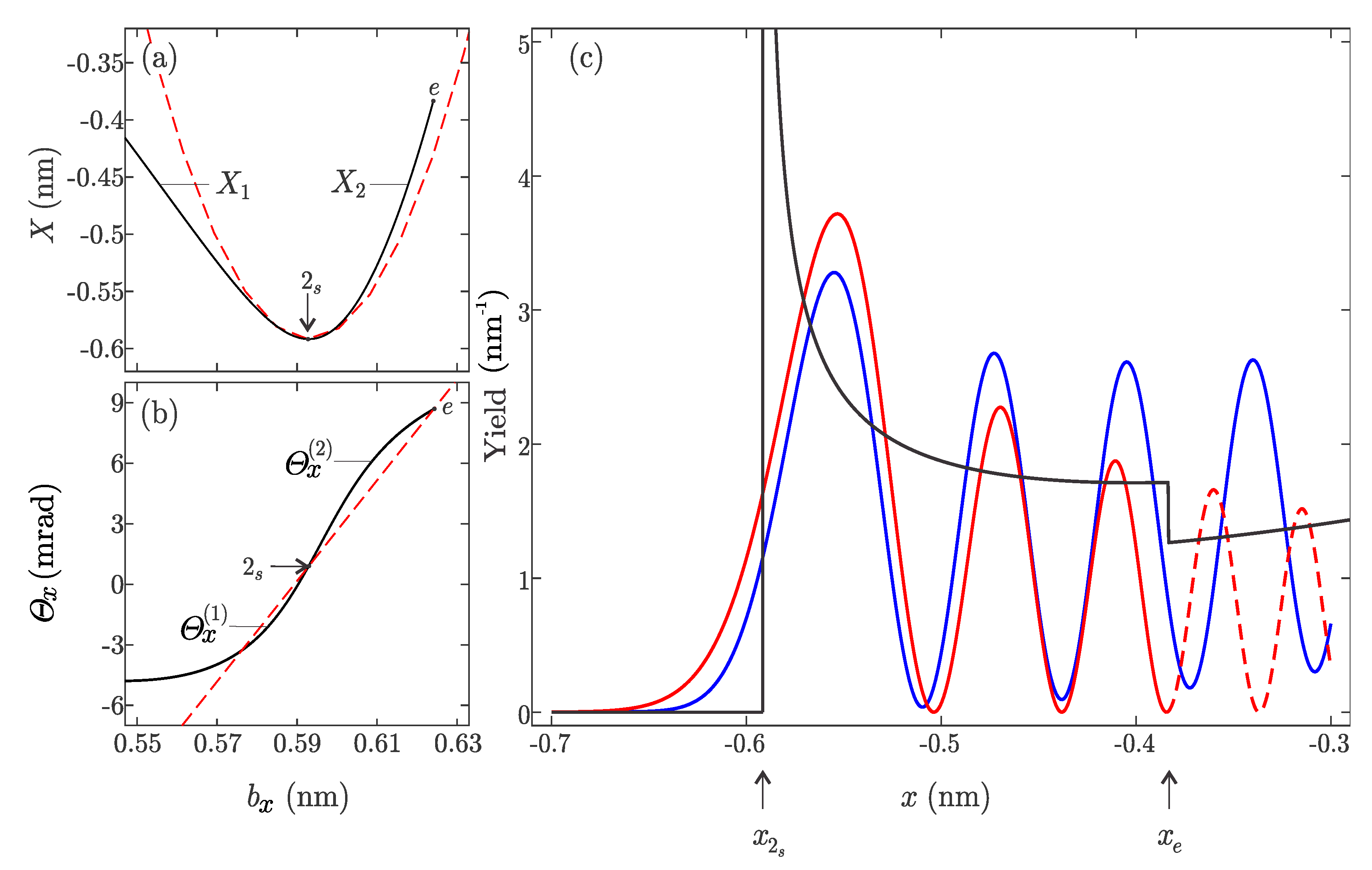
Let us now examine more closely shape of the classical, the semi-classical probability density of the wave packet
c in the vicinity of the point
, and compare it with the exact solution given on
Figure 6b. The spatial transmission function
is shown in
Figure 7a has a minimum labeled
at the point at
. It ends at the point
e which correspond to maximal possible considered impact parameter
. The inverse transmission mapping
have two branches labeled
and
, respectively. The branch
is formed by the end positions of positrons having impact parameters
, while positrons ending on the branch
have impact parameters belong to the interval
. Therefore, for
the mapping
is zero-valued for
x in the interval
, the mapping is double-valued, while for
it is single-valued. The classical probability density is defined by the equation
For an interval of impact parameters that is considered to be small from
Figure 7a, function
can be approximated with a constant (this also means that
). The resulting normed distribution
is in
Figure 7c shown by the black line. Since,
for
both branches give singular contributions to the function
which is infinite at the rainbow point
. For
density
, therefore this region is the dark side of the rainbow. For
x in the interval
the function
is monotonously decreasing. Note an abrupt jump of the function
at
which is consequence of the change of the multiplicity of the mapping
from 2 to 1.
The exact normed probability density
is shown in the
Figure 7c by the blue line. Comparison of these two solutions shows the following. The classical approximation correctly predicts overall order of the magnitude of the exact probability density
. It predicts that the largest contribution to the density is at the rainbow point
, which is very close to the largest peak of the function
, and that density is low for
and high for
. Out of many peaks of the density
, as is clearly visible on
Figure 6b and
Figure 7c, the classical approximation explains the existence of only one, and clearly overestimates its amplitude.
According to the Equation (
24) to construct semiclassical solution
one need to find all solution of the equation
, form semiclassical waves and sum their contributions. For example, if
amplitudes of the individual semiclassical waves are
and
. To obtain phases of the semiclassical waves one need to consider also angular transmission function
shown in
Figure 7b. Since the mapping
is two-valued in the considered interval, the mapping
has also two branches
, and
. Phases of the semiclassical waves are solutions of the equations
, and
.
Note that direct evaluation of the Equation (
24) is not possible at
since both wave amplitudes
diverge. To circumvent this limitation one can use the so-called transitional approximation [
11,
29]. Firstly, the spatial and the angular transmission functions are in the vicinity of the rainbow point
approximated by the following polynomials
Obtained approximations are in
Figure 7a,b shown by the dashed red lines. By the analytical continuation validity of the equation
is extended to the whole complex plane. Therefore, for
equation
has two real solutions, for
, the equation has a double root, while for
the equation has two conjugate complex solutions. Taking into account additional complex solutions it can be shown that transitional semiclassical density
is given by the equation
where
is the Airy function [
37]. The obtained semiclassical distribution
is in
Figure 7c shown by the red line. The largest maxima which is now finite of the function
is the closest to the classical rainbow
. For
interference of the complex rays make density
exponentially decaying. For
a number of smaller peaks can be observed which appear due to the constructive interference of the real rays.
Note that almost identical expression describe semiclassical intensity of the light in the vicinity of the optical rainbow [
44,
45,
46]. Therefore, peaks of the function
can be classified in analogues way as interference peaks of the optical rainbow. The large maximum closest to the position of the classical rainbow is considered to be the primary rainbow maximum, while all other peaks are supernumeraries associated with the observed primary.
Comparison of the red and the blue curve from
Figure 7c reveals that transitional semiclassical approximation almost perfectly predicts the position and size of the dominant peak of the exact distribution
. The constructive interference of real rays explains the existence of all other maxima, while the interference of the complex rays explains how probability density
can have non-zero values in the region where there are no real rays at all. It could be said that the ray interference “assuages” the sharpness of the classical distribution
. Therefore, the semiclassical approximation captures all qualitative features of the quantum rainbow scattering effect. However, its validity is limited. Note that accuracy of the semiclassical solution
actually decreases for
. Its range of validity is limited only to the region
. The reason for this is that in the vicinity of the point
the multiplicity of the inverse transmission function
changes by one, while number of real roots of any polynomial approximation of the
can change only by an integer multiple of 2. This is the reason why semiclassical density
for
in
Figure 7c is shown by the dashed red line. For
the correct semiclassical wave function is given by only one semiclassical wave. According to the Equation (
24) semiclassical density is then
, i.e., it is equal to the classical solution
. Therefore, the semiclassical approximation is not capable of explaining the existence of the peaks of the exact density
in the region where transmission function has only one branch.
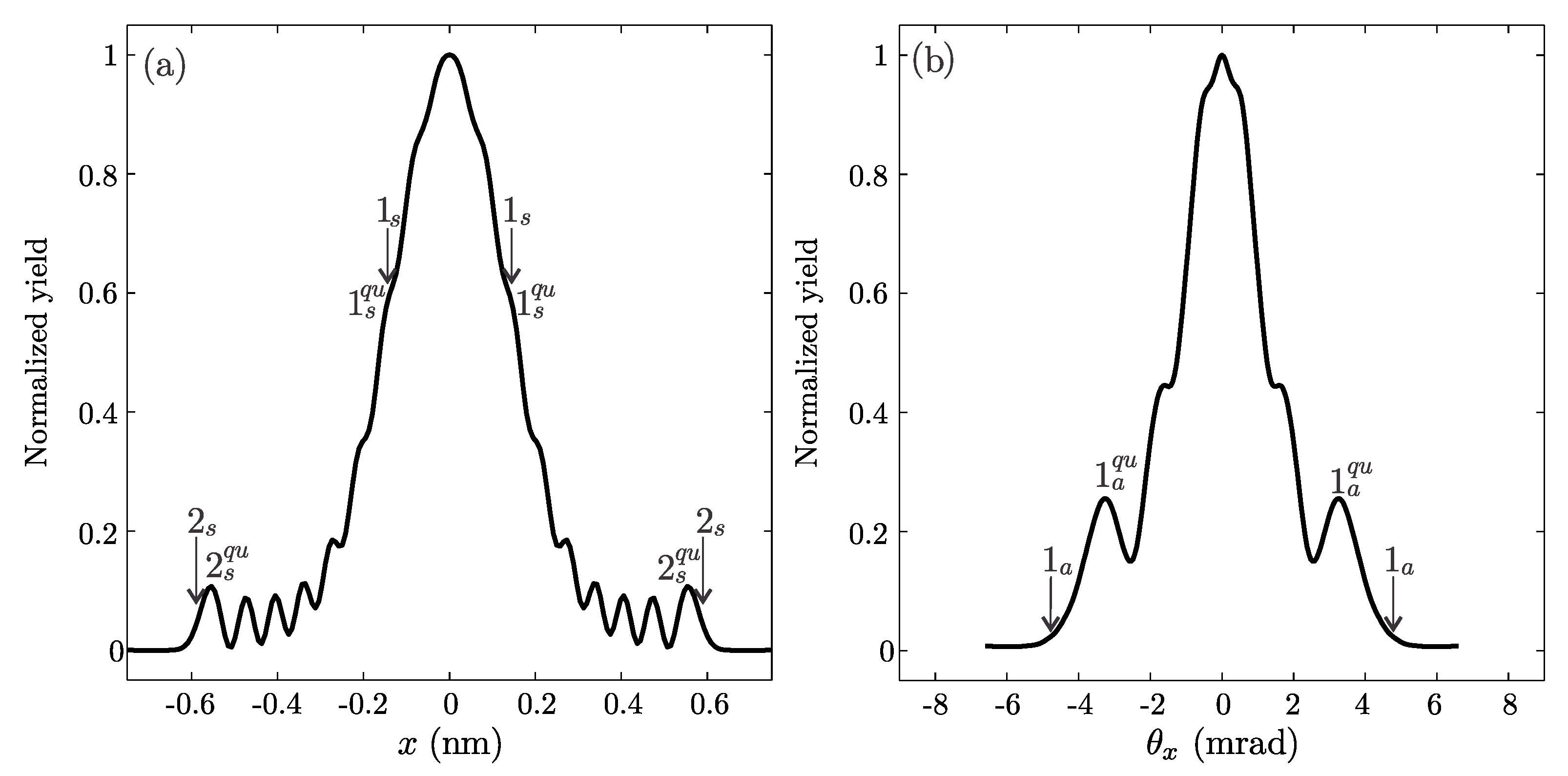
Summing contributions of all wave packets according to Equation (
19) gives distributions of the transmitted positron beam at the exit plane of the SWCNT. The vertical slices through the obtained distributions in spatial and angular space are shown in
Figure 8a,b, respectively. Each pair of symmetric maxima visible in the
Figure 8a,b corresponds to the circular maxima of the 2D distribution. Positions of the classical rainbow point pairs
,
, and
are indicated by the arrows. Large maxima closest to the classical rainbow points, labeled
, and
in the
Figure 8a at
nm and
nm are interpreted as the primary and the secondary rainbow point. All other peaks are considered to be supernumerary rainbows. In the case of angular distribution, the large maximum pair
at
mrad, closest to the classical rainbow peaks
, are interpreted as the primary rainbow points, all remaining peaks are considered to be supernumerary rainbows.
3.3. Morphological Interpretation of Quantum Rainbow Effect
The developed method was applied for classification of angular distributions of 1-MeV positrons transmitted through 200-nm long SWCNTs (7, 3), (8, 5), (9, 7), (14, 4), (16, 5), and (17, 7). The nanotubes considered here are the easiest to produce by the arch discharge method [
1]. The radii of the considered nanotubes are in the range from 0.35 to 0.85 nm. The classical critical angles for the considered SWCNTs are very close to each other, ranging from 7.3 to 7.4 mrad. For all SWCNT we assume that initial angular standard deviation of the positron beam
is always equal to the 10% of the corresponding classical critical angle
. This means that the used angular standard deviations of the positron beams are in the range [0.73, 0.74] mrad, the corresponding range of the standard deviations of the Gaussian wave packets are in range [0.094, 0.095] nm. Note that all wave packets have almost the same size.
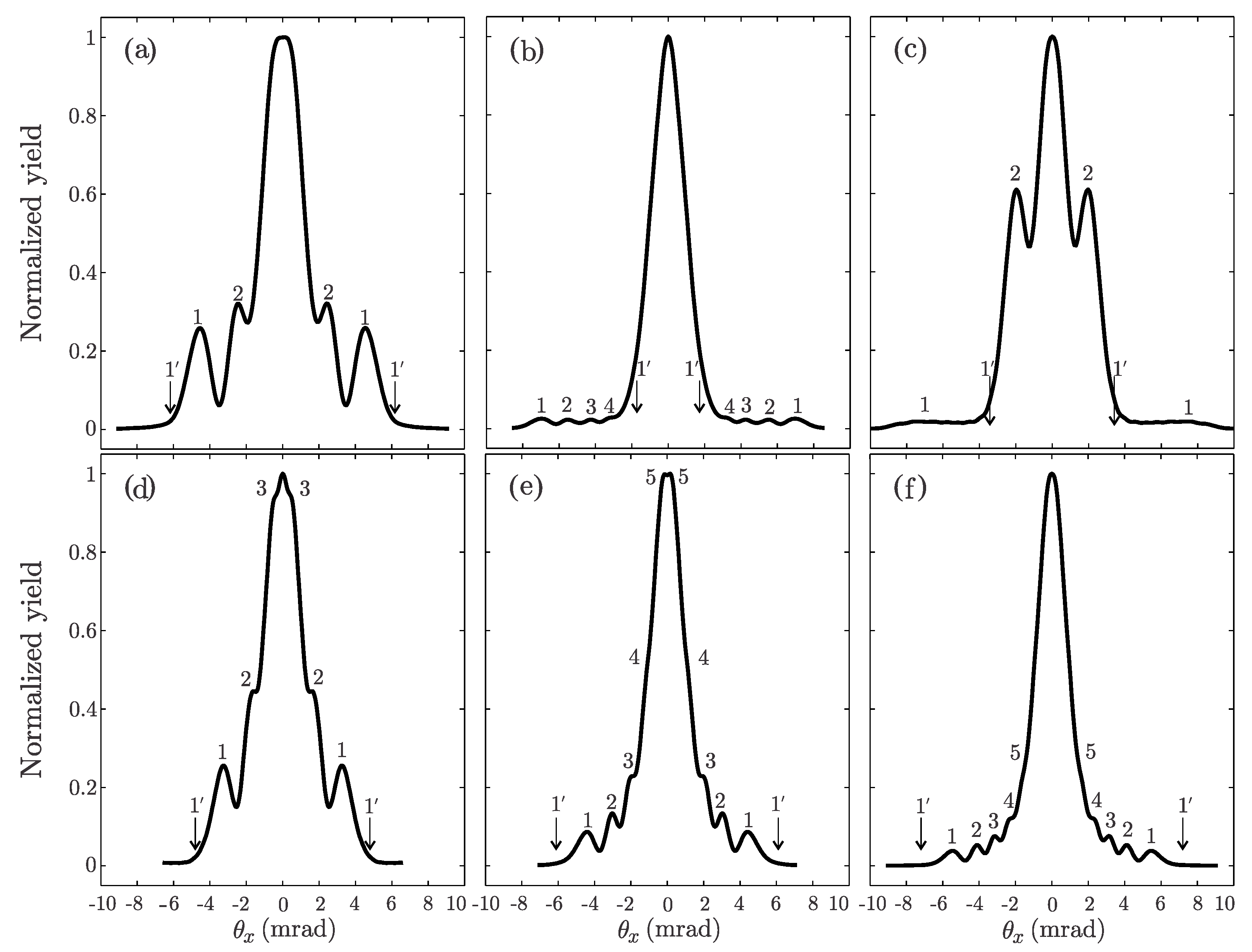
Vertical slices through obtained distributions are shown in
Figure 9. Initial spatial distributions were constructed using: 43, 71, 101, 141, 190, 241 Gaussians, respectively. All prominent peaks excluding central are labeled by numbers, with symmetrical maxima labeled by the same number. Numeration always starts from the outermost rainbow pair. In all analyzed cases there is only one classical rainbow point pair labeled
. Their positions in
Figure 9 are shown by the arrows. It is clear that the quantum peak pairs labeled 1 in
Figure 9a,d–f are quantum primary rainbow points. All other peaks are supernumerary rainbow.
It should be noted that the semiclassical classification of the quantum peaks for the distributions from
Figure 9b,c is ambiguous. This is not surprising since the applicability of the semiclassical approach is limited. Rather, it is surprising that the semiclassical interpretation works at all. It should be noted that accurate wave functions differ considerably from their semiclassical counterparts. If we take a closer look at the wave packet
shown in
Figure 6b,c, the interference is clearly visible in the interval from
nm up to
nm in the spatial representation, and in interval from
mrad up to the
mrad. However, the corresponding relevant parts of the transmission functions are two-valued only in the intervals
nm, and
mrad, respectively. Outside the mentioned intervals the transmission functions are single-valued, and there should be no observable interference effects. Therefore, the semiclassical wave functions drastically underestimate real self-interference of the wave packets.
The problem with the semiclassical interpretation is that it intrinsically relay on classical concepts (such as exact position of the particle in the phase space) which do not have direct quantum analogue. A alternative approach would be to try to link the rainbow effect to certain morphological properties of the family of the classical trajectories, and quantum amplitude and phase function families. If morphological properties were found to be equal then both approaches are merely two descriptions of the same physical reality.
In this subsection it will be shown that it is possible unambiguously to classify quantum peaks relaying only on the information contained in the quantum amplitude and phase function families. In order to show that let us examine channeling of 1-MeV positrons through 400-nm long SWCNT (11, 9). The classical critical angle is
mrad. The obtained trajectory family in
plane is shown in
Figure 10a. The striking features of this family are three pairs of envelope lines, labeled
,
, and
, which are defined as a limiting line formed from intersections points of the neighboring family members [
47]. The mathematical envelope is defined as a set of solutions of the equation
Equation (
28) shows that each envelope is a locus of one critical point of the transmission function, i.e., the envelope is caustic line of the trajectory family [
48]. For example, for a nanotube of length
nm the spatial transmission function has one symmetrical pair of critical points whose ordinates
nm are equal to the positions of envelope points
in
Figure 10a. Another important quantity is the Hamilton’s principal function defined by the equation [
49]
which is directly related to the phase function of the quantum wave packet [
50]. Hamilton’s principal functions for nanotube whose length is
nm is shown in
Figure 10b. It is multivalued singular curve composed of three branches. The caustic lines are also loci of singularities of the Hamilton’s principal function [
48]. Its cusped-like singular points, locally isomorphic to the cusp catastrophe [
51], are also labeled
. Therefore, envelope lines and singularities of the Hamilton’s principal function are inextricably linked to the manifestations of the rainbow effect.
Now let us apply the same logic for the explanation of the quantum rainbow channeling.
Figure 11a shows spatial distribution of the transmitted positron obtained assuming that initially its divergence was
. Spatial and angular standard deviations of the wave packets were
nm and
0.73 mrad. The vertical slice through the obtained spatial distribution of the positron beam, transmitted through 150-nm long SWCNT (11, 9), is shown in
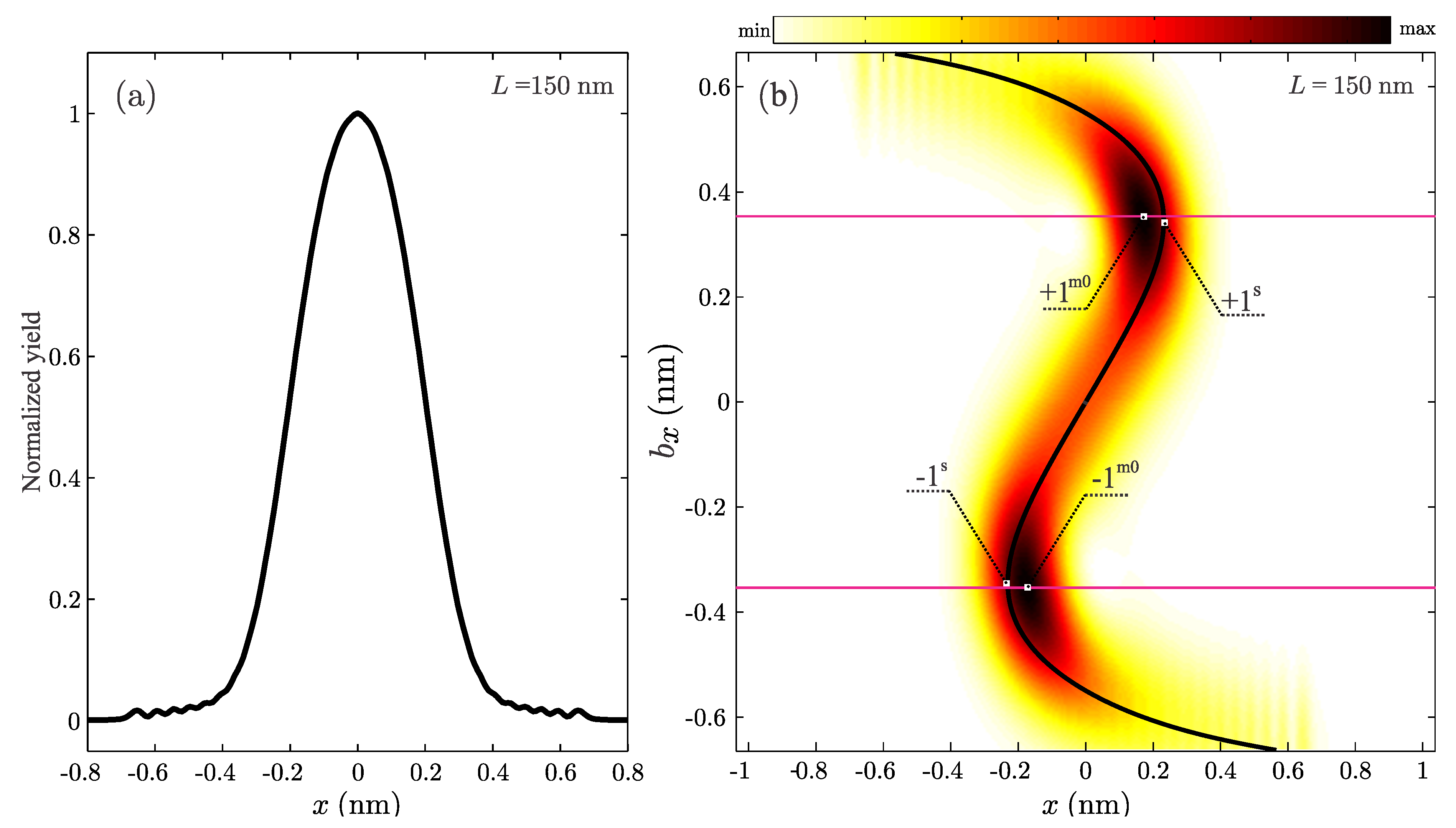
Figure 11a. It consists of a large central peak which shows no signs of any internal structure, and six pairs of smaller peaks. The largest is the outermost peak pair, while remaining peaks are of approximately the same size. We need to provide a classification of the observed behaviour, and an explanation for its formation.
Since the obtained spatial distribution is axially symmetric we have focused only on the motion of wave packets with impact parameters belong to the nanotube vertical cross-section. We have followed evolution of 301 wave packets. Vertical cross-sections through obtained probability densities parameterized by the impact parameter are shown in
Figure 11b. This distribution is dominated by two large maxima labeled
at
nm. Therefore, the dominant contribution to the large central peak in the
Figure 11a actually comes from two smaller maxima. During their evolution, the wave packets become wrinkled (for example see
Figure 5, or
Figure 6b,c). This is the manifestation of the wave packet self-interference caused by the interaction with the nanotube walls. The wave packet ensemble shown in
Figure 11b can be separated in two parts. The first one is formed by the wave packets showing no observable wrinkling (i.e., for
nm). The second subensemble is formed by the wave packets for which self-interference is considerable (i.e., for
nm), which is called the rainbow subensemble. Members of these two subensembles are separated by the magenta lines in
Figure 11b. Note the formation of the vertical yellow stripes which occur for the wave packets of impact parameters approximately in the range
nm. This means that the wave packets wrinkle in the mutually coordinated way. The corresponding inverse classical spatial transmission function is shown by the thick black line. Positions of the classical rainbow points, labeled
, are also indicated. Quantum probability density is concentrated around the classical line. It behaves as if there is a virtual barrier preventing spreading of the wave packets in the areas beyond the line.
It should be noted that the observed behavior is unexplainable by the semiclassical approach. For example, for nm the wave packet wrinkling is the most noticeable in rainbow subensemble and in the region nm, where the inverse transmission function is single valued. On the other hand the semiclassical approximation predicts that the most intense self-interference should be in the regions close to the classical rainbow points , where no wave packet wrinkling can be observed.
Wrinkling, concentration and coordination of the wave packets are elementary processes clearly sufficient for description of the wave packet motion. Out of these three processes, the coordination is the most important for explanation of the rainbow effect. Next, we will show that coordinated evolution of wave packets generate the rainbow effect.
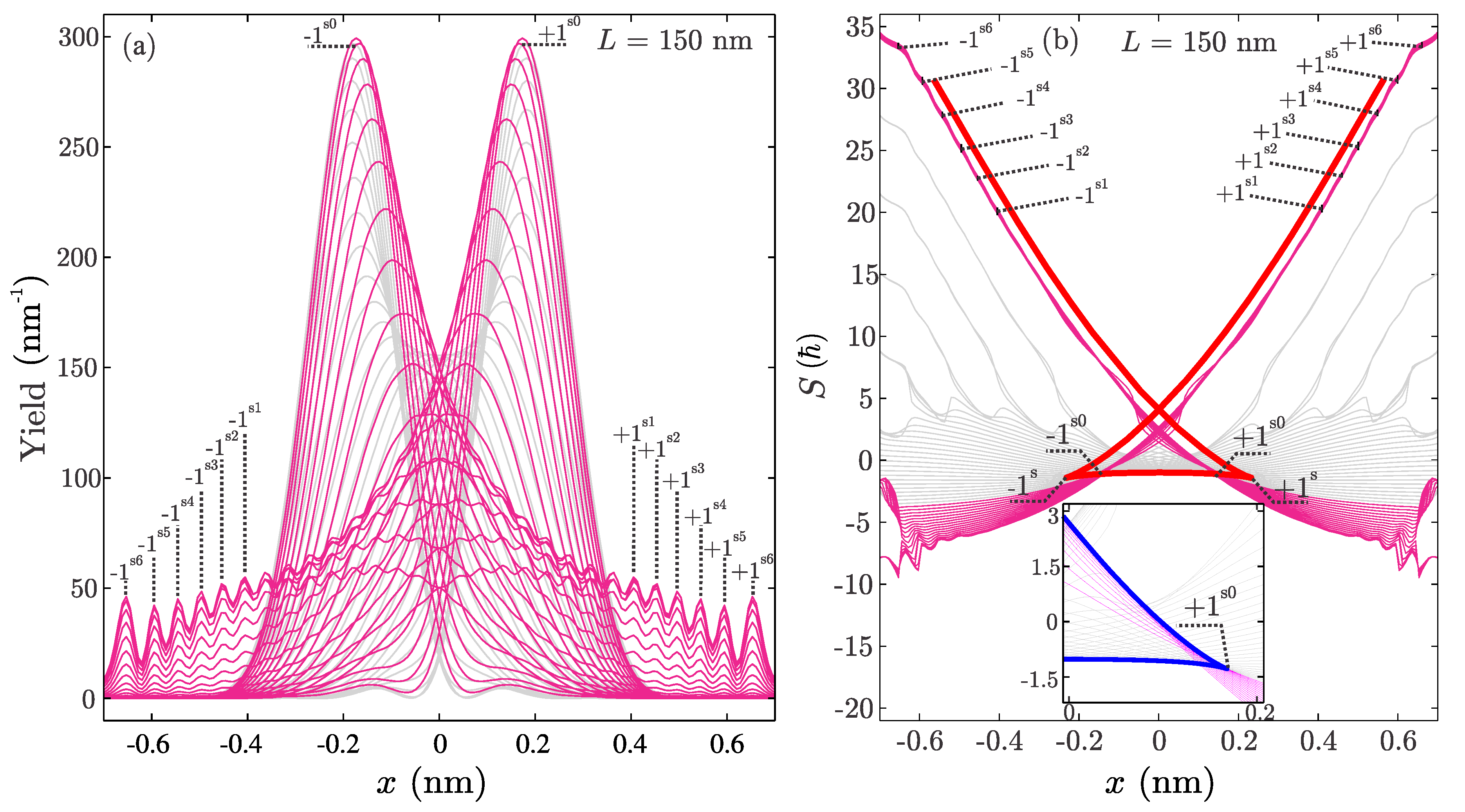
Figure 12a shows the family of quantum probability densities. Members of the rainbow subensemble are designated by the magenta lines. Each member represents the motion of the wave packet reflected form the SWCNT boundary. The largest maxima of any member show the current position of the wave packet center, with a large number of self-interference maxima on its tail. The remaining probability densities are shown by the gray lines. Prominent peak pairs visible in
Figure 11a and
Figure 12a labeled
,
,
,
,
,
, and
, are at
,
,
,
,
, and
nm, respectively. The numbering starts from the innermost peak pairs. The reason for such a convention will become apparent shortly. Note that due to the wave packet coordination, members of the rainbow subensemble have their respective maxima on the exactly same abscissas.
Figure 12b shows the obtained family of quantum phase functions expressed in the units of
ℏ. The red line shows the corresponding classical Hamilton’s principal function form
Figure 10b. Phases of the members of the rainbow subensemble are designated by the magenta lines, remaining phases are shown by the gray lines. The family of phase functions can also be subdivided into subsets of lines which run in parallel, i.e., subsets of coordinated wave packets. Note that the subset of magenta lines is the largest, which also runs in parallel with the classical Hamilton’s principal function. Therefore, wave packet coordination is the strongest in the rainbow subensemble. Detailed analysis of phases functions in the vicinity of classical singular points
have shown that envelope function of phase function family also have two cusp singular points
at
nm. This can be seen in inset in
Figure 12b where the envelope line is shown by the blue line. The "vertical" branch of the envelope is defined by the members of the rainbow subensemble, while the "horizontal" is defined by the remaining phases of the ensemble. Therefore, both subensembles are important for the explanation of the formation of cusp singular points. The position of the points
is also shown in
Figure 12a and it proved to be very close to the peaks
from
Figure 11b. A careful examination revealed that phases of the rainbow subensemble have common inflection points labeled
,
,
,
,
,
, and
, respectively whose abscissas are equal with abscissas of the corresponding points in
Figure 12a. Note that points
,
,
,
,
, and
, respectively belong to the branch generated by the point
.
Now the classification of the prominent peaks of the spatial distribution of the positron beam is straightforward. The central maximum consists of two primary quantum rainbow peaks . Peaks , , , , , and , respectively, are supernumeraries of the primary rainbow , while , , , , , and are supernumeraries of the primary rainbow .
It should be stressed that although quantum mechanical description requires that amplitude and phase functions of any individual wave packet are smooth single valued functions, there is no such restriction regarding the behavior of the ensembles of amplitude or phase function families. The envelope function of the quantum phase functions can develop cusp singularities characteristic for the existence of the rainbow effect. Therefore, the morphological approach for the classification of the system behavior, based on the analysis of the singularities of the appropriate function family, is more general than other approaches considered here. The developed morphological method is geometrical in its nature, which makes it applicable for interpretation of the rainbow pattern for any physical system in which it can be observed. The true limitation of the method is the existence of the some random factors which can destroy the coordinated behavior of individual wave packets.