Calculation of Low-Energy Positron-Atom Scattering with Square-Integrable Wavefunctions
Abstract
1. Introduction
2. Scattering as a Bound-State Problem
2.1. Generalized Eigenvalue Problem
2.2. Scattering Phase Shifts
3. Calculation of Elastic -Wave Positron–Hydrogen Phase Shifts
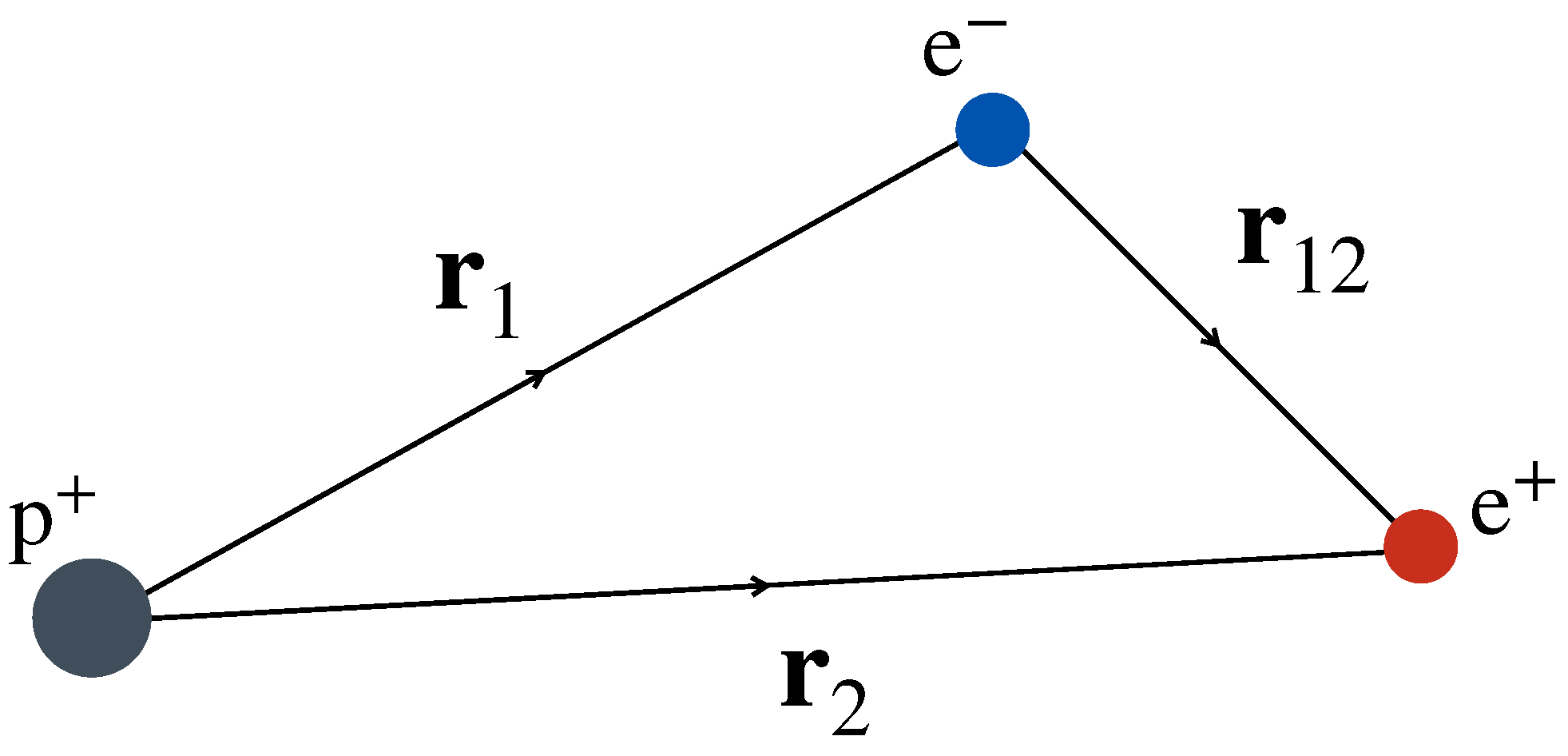
3.1. One-Particle Problem
3.2. Two-Particle Problem
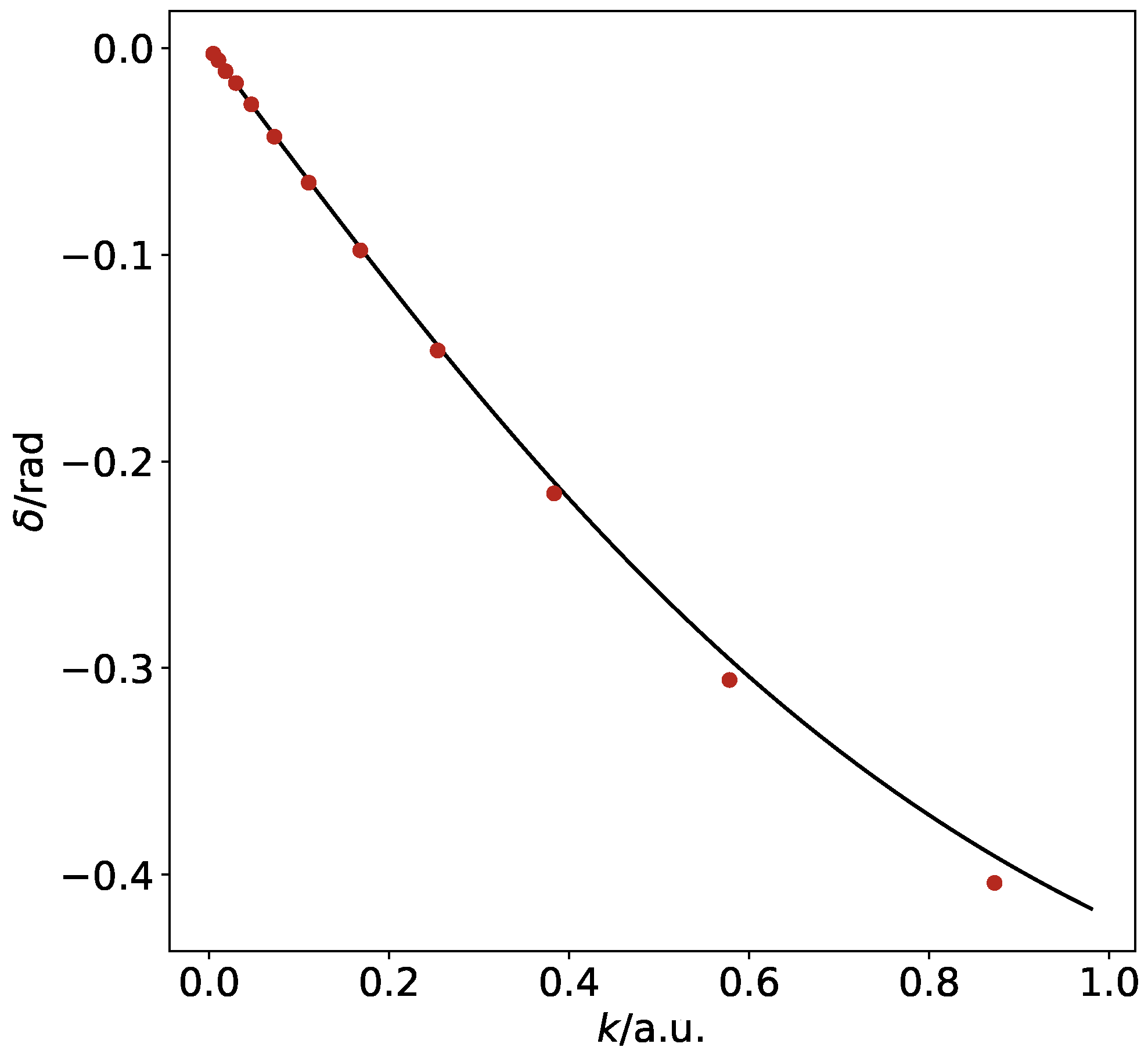
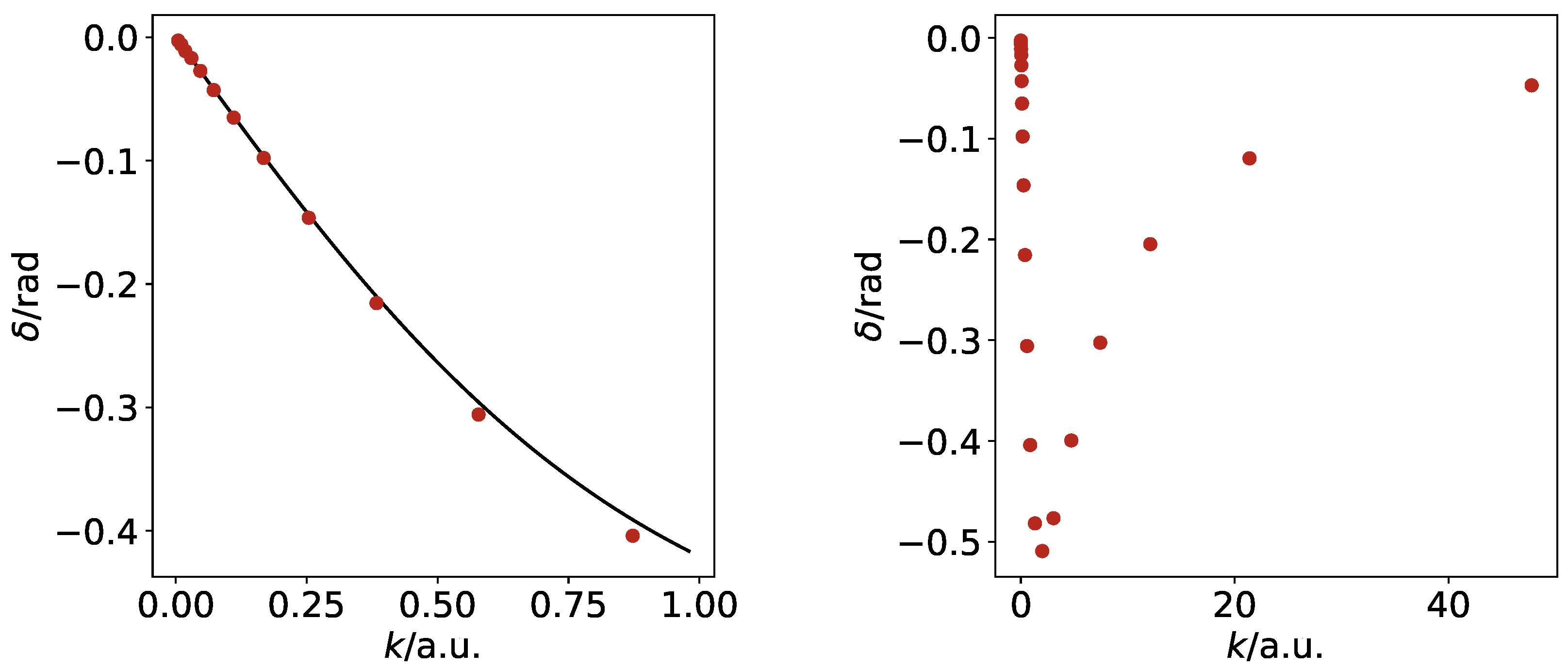
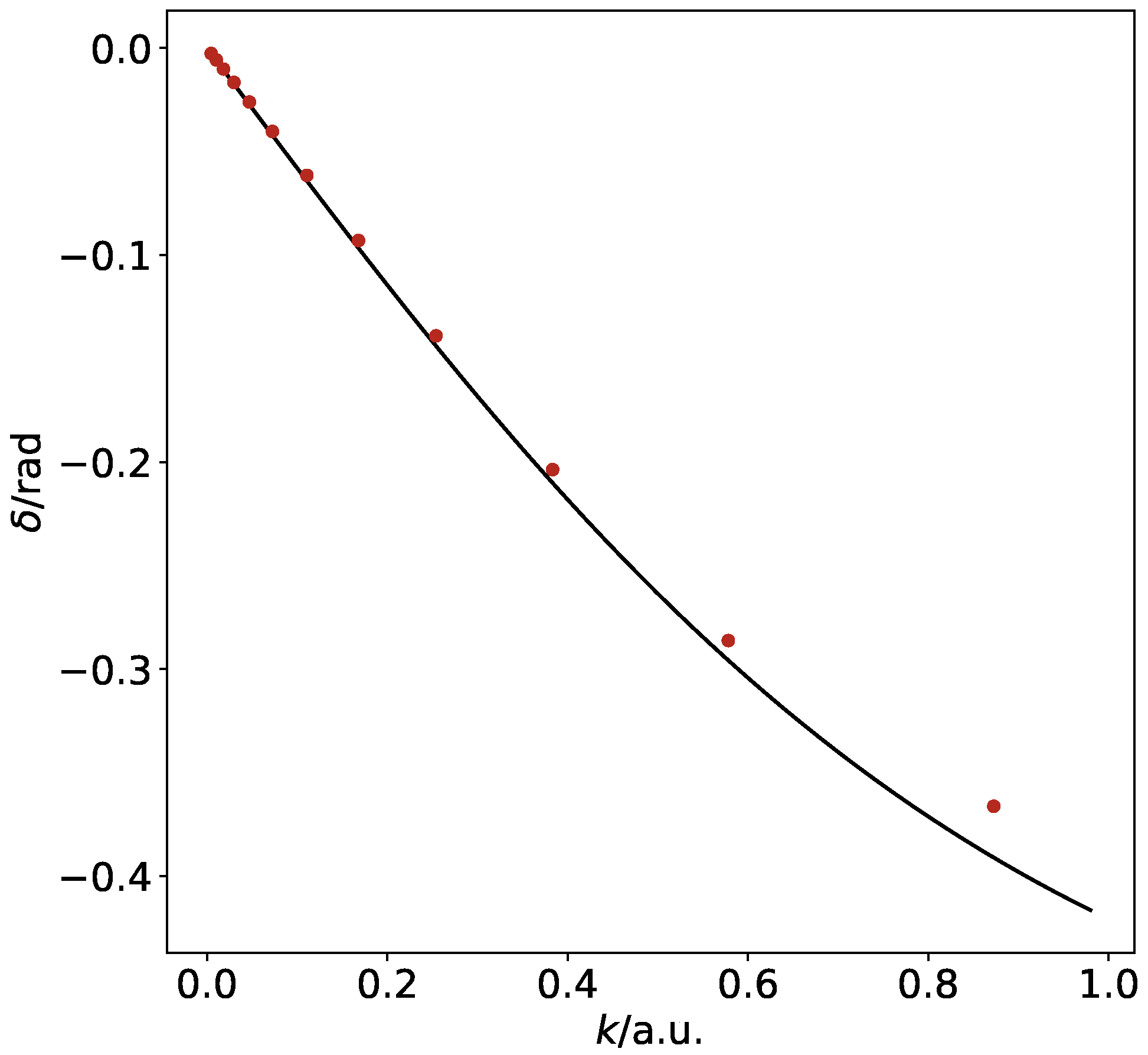
3.2.1. Reproducing the Frozen-Target Results
3.2.2. Variation of : Radial Correlations
3.2.3. Nonzero : Effect of Angular Correlations
4. Calculation of the Annihilation Parameter
4.1. One-Particle Calculation
4.2. Two-Particle Calculation
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Standard Integrals
Appendix B. Parameters for Positron-Scattering Wavefunction Bases
| 1 | 0.80 | 0.04 | 9 | 0.79 | 0.06 | ||
| 3 | 0.80 | 0.05 | 0.99 | 0.40 | 0.11 | ||
| 0.88 | 0.45 | 0.10 | 1.00 | 0.14 | |||
| 0.97 | 0.28 | 0.40 | 0.04 | ||||
| 5 | 0.80 | 0.05 | 0.85 | 0.88 | |||
| 0.98 | 0.46 | 0.06 | 0.29 | 0.40 | |||
| 0.99 | 0.14 | 0.85 | 0.50 | ||||
| 0.45 | 0.12 | 0.84 | |||||
| 0.86 | 0.93 | 0.99 | 0.67 |
| 1 | Atomic units are used throughout (in which , where e is the elementary charge and m is the electron or positron mass). |
| 2 | For a state with a zero total angular momentum, the wavefunction is spherically symmetric, so there is no dependence on the directions of and , except the angle between them, i.e., dependence on . |
References
- Chua, S.; Groves, A. Biomedical Imaging Applications and Advances; Woodhead Publishing: Cambridge, UK, 2014. [Google Scholar]
- Tuomisto, F.; Makkonen, I. Defect identification in semiconductors with positron annihilation: Experiment and theory. Rev. Mod. Phys. 2013, 85, 1583–1631. [Google Scholar] [CrossRef]
- Ishida, A.; Namba, T.; Asai, S.; Kobayashi, T.; Saito, H.; Yoshida, M.; Tanaka, K.; Yamamoto, A. New precision measurement of hyperfine splitting of positronium. Phys. Lett. B 2014, 734, 338. [Google Scholar] [CrossRef]
- Ahmadi, M.; Alves, B.X.R.; Baker, C.J.; Bertsche, W.; Capra, A.; Carruth, C.; Cesar, C.L.; Charlton, M.; Cohen, S.; Collister, R.; et al. Investigation of the fine structure of antihydrogen. Nature 2020, 578, 375–380. [Google Scholar] [CrossRef]
- Dirac, P.A.M. Quantised Singularities in the Electromagnetic Field. Proc. R. Soc. Lond. Ser. A 1931, 133, 60–72. [Google Scholar] [CrossRef]
- Anderson, C.D. The positive electron. Phys. Rev. 1932, 43, 491–494. [Google Scholar] [CrossRef]
- Surko, C.M.; Gribakin, G.F.; Buckman, S.J. Low-energy positron interactions with atoms and molecules. J. Phys. B 2005, 38, R57–R126. [Google Scholar] [CrossRef]
- Amusia, M.Y.; Cherepkov, N.A.; Chernysheva, L.V.; Shapiro, S.G. Elastic scattering of slow positrons by helium. J. Phys. B 1976, 9, L531–L534. [Google Scholar] [CrossRef]
- Amusia, M.Y.; Dolmatov, V.K.; Chernysheva, L.V. Positron elastic scattering by a semifilled-shell atom. J. Phys. B 2021, 54, 185003. [Google Scholar] [CrossRef]
- Gribakin, G.F.; King, W.A. The effect of virtual positronium formation on positron-atom scattering. J. Phys. B 1994, 27, 2639–2645. [Google Scholar] [CrossRef]
- Dzuba, V.A.; Flambaum, V.V.; Gribakin, G.F.; King, W.A. Bound states of positrons and neutral atoms. Phys. Rev. A 1995, 52, 4541. [Google Scholar] [CrossRef]
- Dzuba, V.A.; Flambaum, V.V.; Gribakin, G.F.; King, W.A. Many-body calculations of positron scattering and annihilation from noble-gas atoms. J. Phys. B 1996, 29, 3151. [Google Scholar] [CrossRef][Green Version]
- Gribakin, G.F.; Ludlow, J. Many-body theory of positron-atom interactions. Phys. Rev. A 2004, 70, 032720. [Google Scholar] [CrossRef]
- Green, D.G.; Ludlow, J.A.; Gribakin, G.F. Positron scattering and annihilation on noble-gas atoms. Phys. Rev. A 2014, 90, 032712. [Google Scholar] [CrossRef]
- Hofierka, J.; Cunningham, B.; Rawlins, C.M.; Patterson, C.H.; Green, D.G. Many-body theory of positron binding to polyatomic molecules. Nature 2022, 606, 688–693. [Google Scholar] [CrossRef] [PubMed]
- Bray, I.; Stelbovics, A.T. Convergent close-coupling calculations of low-energy positron–atomic-hydrogen scattering. Phys. Rev. A 1993, 48, 4787–4789. [Google Scholar] [CrossRef]
- Mitroy, J.; Bromley, M.W.J. Convergence of configuration-interaction single-center calculations of positron-atom interactions. Phys. Rev. A 2006, 73, 052712. [Google Scholar] [CrossRef]
- Kadyrov, A.S.; Bray, I. Two-center convergent close-coupling approach to positron-hydrogen collisions. Phys. Rev. A 2002, 66, 012710. [Google Scholar] [CrossRef]
- Humberston, J.; Van Reeth, P. Annihilation in low energy positron-helium scattering. Nucl. Instrum. Methods Phys. Res. B 1998, 143, 127–134. [Google Scholar] [CrossRef]
- Reeth, P.V.; Humberston, J.W. Elastic scattering and positronium formation in low-energy positron-helium collisions. J. Phys. B 1999, 32, 3651–3667. [Google Scholar] [CrossRef]
- Germano, J.S.E.; Lima, M.A.P. Schwinger multichannel method for positron-molecule scattering. Phys. Rev. A 1993, 47, 3976–3982. [Google Scholar] [CrossRef]
- da Silva, E.P.; Germano, J.S.E.; Lima, M.A.P. Annihilation Dynamics of Positrons in Molecular Environments: Theoretical Study of Low-Energy Positron- C2H4 Scattering. Phys. Rev. Lett. 1996, 77, 1028–1031. [Google Scholar] [CrossRef] [PubMed]
- Schwartz, C. Electron Scattering from Hydrogen. Phys. Rev. 1961, 124, 1468–1471. [Google Scholar] [CrossRef]
- Humberston, J.W.; Reeth, P.V.; Watts, M.S.T.; Meyerhof, W.E. Positron-hydrogen scattering in the vicinity of the positronium formation threshold. J. Phys. B 1997, 30, 2477–2493. [Google Scholar] [CrossRef]
- Bhatia, A.K. Positron-Hydrogen Scattering, Annihilation, and Positronium Formation. Atoms 2016, 4, 27. [Google Scholar] [CrossRef]
- Humberston, J.W.; Wallace, J.B.G. The elastic scattering of positrons by atomic hydrogen. J. Phys. B 1972, 5, 1138–1148. [Google Scholar] [CrossRef]
- Ghosh, A.; Sil, N.; Mandal, P. Positron-atom and positron-molecule collisions. Phys. Rep. 1982, 87, 313–406. [Google Scholar] [CrossRef]
- Gien, T.T. Coupled-state calculations of positron-hydrogen scattering. Phys. Rev. A 1997, 56, 1332–1337. [Google Scholar] [CrossRef]
- Ghoshal, A.; Mandal, P. Correlated dipole-polarized basis in Schwinger’s principle for elastic positron-hydrogen collisions. Phys. Rev. A 2005, 72, 042709. [Google Scholar] [CrossRef]
- Available online: https://docs.scipy.org/doc/scipy/reference/generated/scipy.linalg.eigh.html (accessed on 3 February 2021).
- Swann, A.R.; Gribakin, G.F. Model-potential calculations of positron binding, scattering, and annihilation for atoms and small molecules using a Gaussian basis. Phys. Rev. A 2020, 101, 022702. [Google Scholar] [CrossRef]
- Landau, L.D.; Lifshitz, E.M. Quantum Mechanics, 3rd ed.; Pergamon: Oxford, UK, 1977. [Google Scholar]
- Amusia, M.Y.; Chernysheva, L.V. Computation of Atomic Processes: A Handbook for the ATOM Programs; Institute of Physics Publishing: Bristol, UK, 1997. [Google Scholar]
- Hylleraas, E.A. Über den Grundzustand des Heliumatoms. Z. Phys. 1928, 48, 469–494. [Google Scholar] [CrossRef]
- Kadyrov, A.S.; Bray, I.; Stelbovics, A.T. Near-Threshold Positron-Impact Ionization of Atomic Hydrogen. Phys. Rev. Lett. 2007, 98, 263202. [Google Scholar] [CrossRef] [PubMed]
- Fraser, P. Positrons and Positronium in Gases. In Advances in Atomic and Molecular Physics; Academic Press: New York, NY, USA, 1968; Volume 4, pp. 63–107. [Google Scholar] [CrossRef]


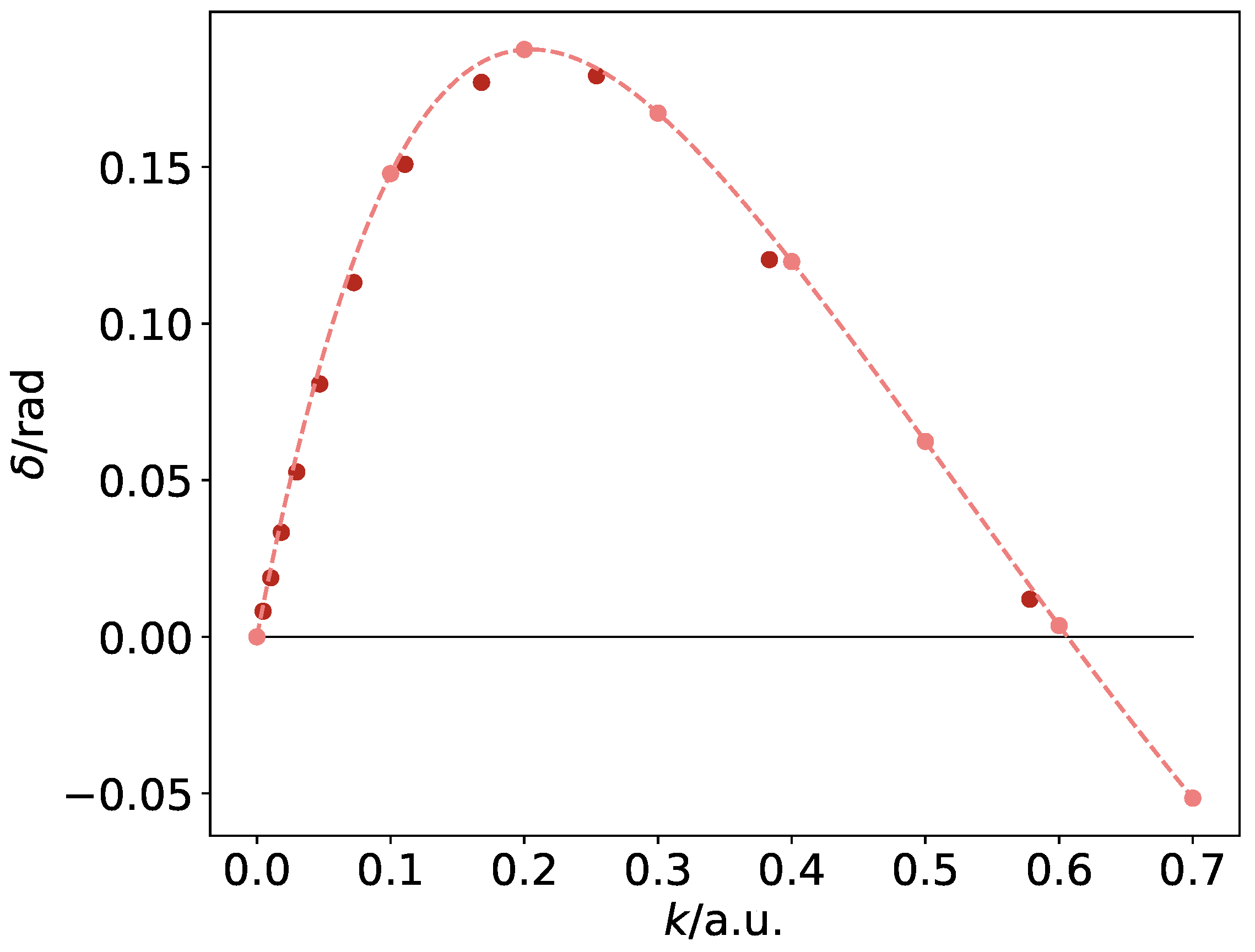
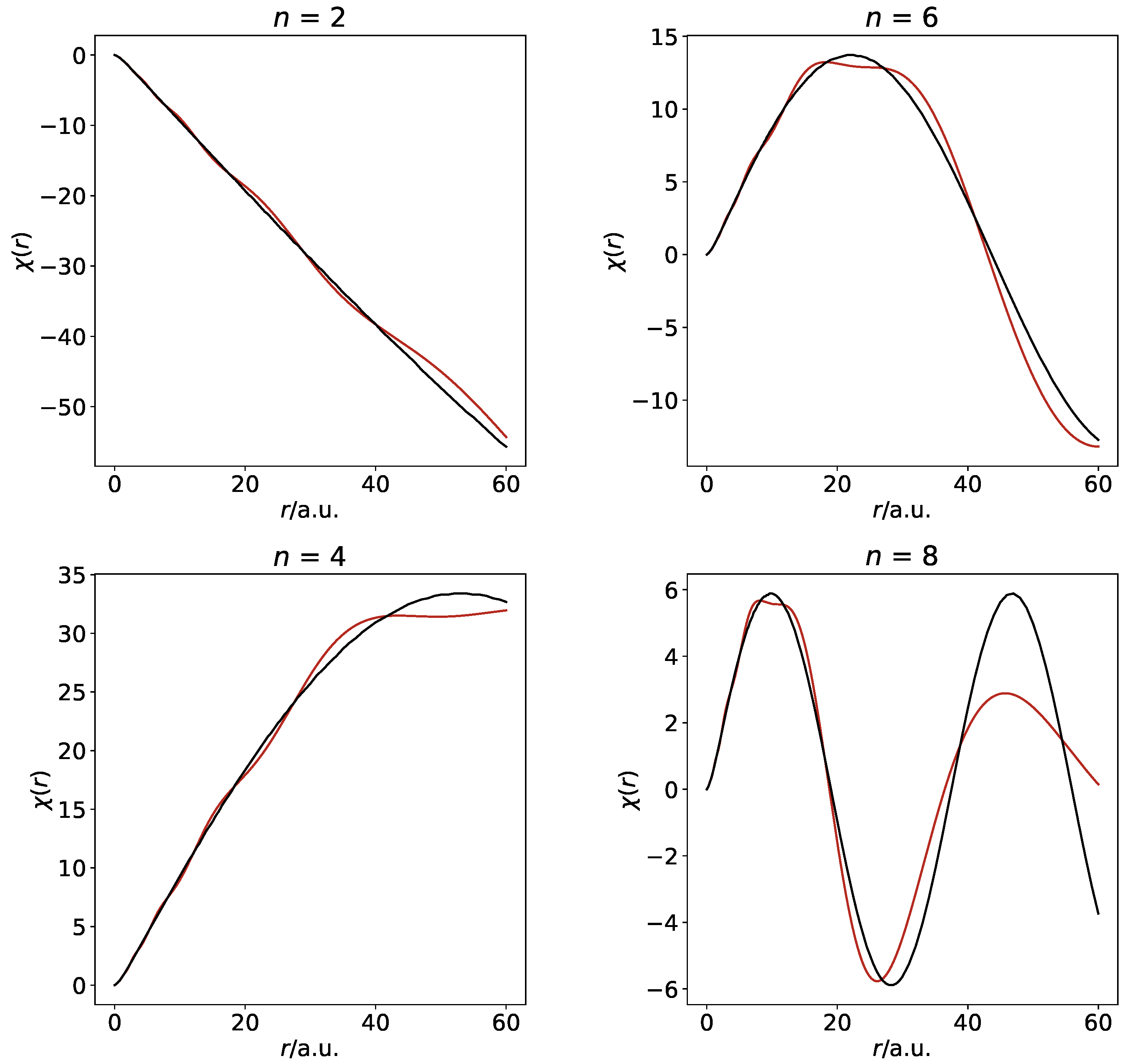
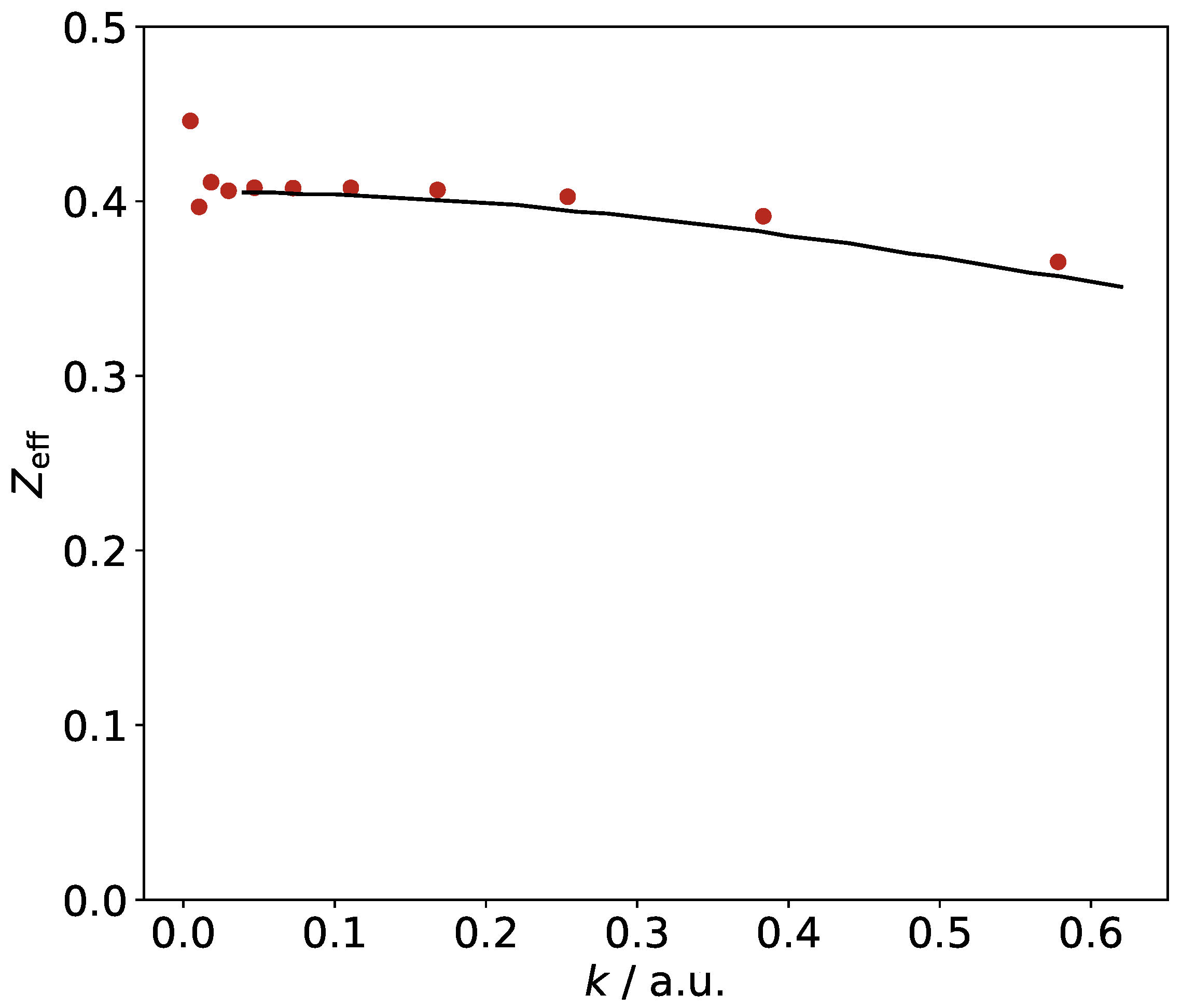
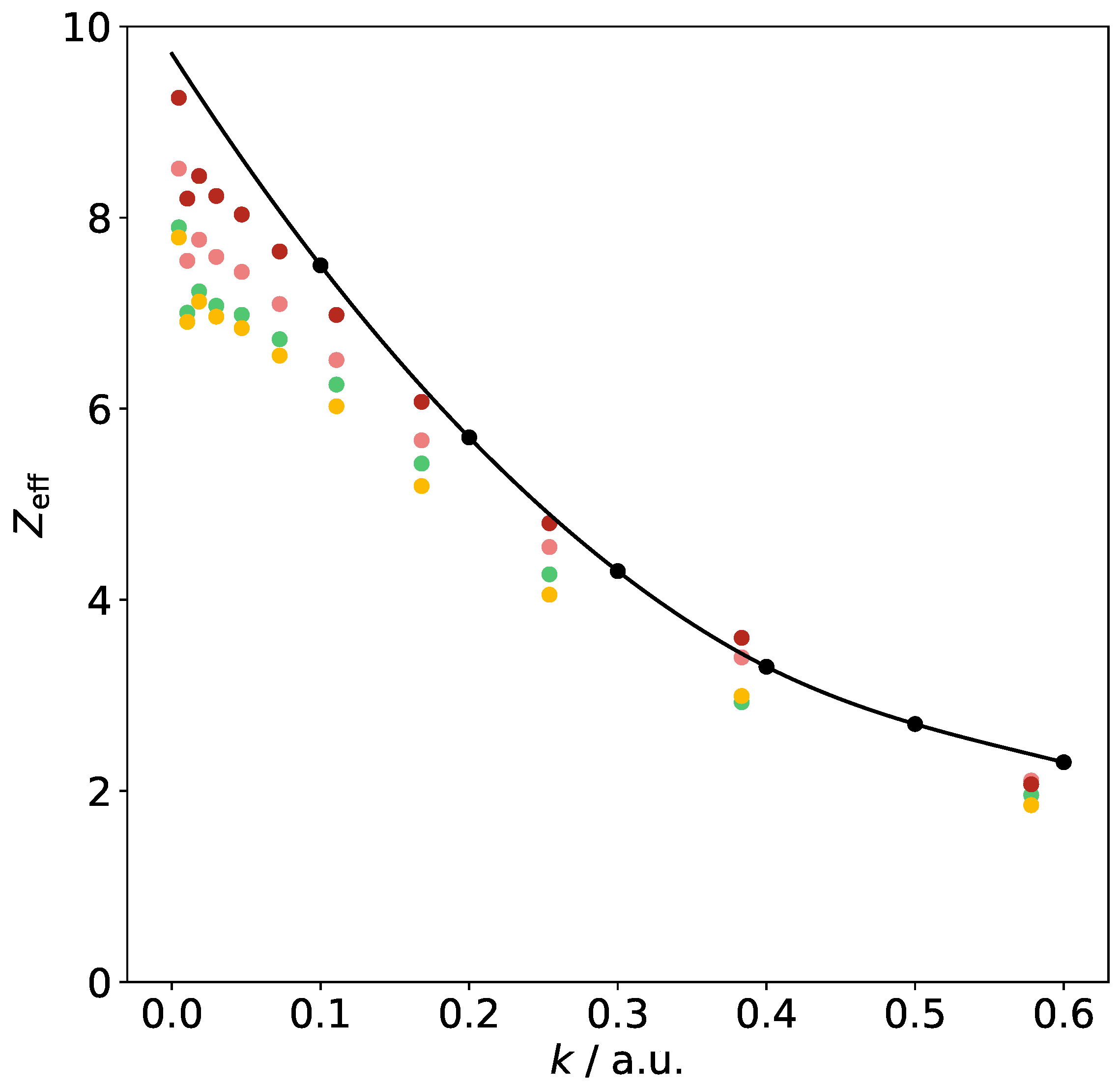
| 1 | 3 | 5 | 9 | |||||
|---|---|---|---|---|---|---|---|---|
| /a.u. | /rad | /a.u. | /rad | /a.u. | /rad | /a.u. | /rad | |
| 1 | 0.0046 | 0.0049 | 0.0046 | 0.0063 | 0.0046 | 0.0075 | 0.0046 | 0.0082 |
| 2 | 0.0104 | 0.0115 | 0.0104 | 0.0145 | 0.0104 | 0.0176 | 0.0104 | 0.0189 |
| 3 | 0.0183 | 0.0201 | 0.0183 | 0.0257 | 0.0183 | 0.0307 | 0.0183 | 0.0334 |
| 4 | 0.0299 | 0.0326 | 0.0299 | 0.0415 | 0.0299 | 0.0494 | 0.0299 | 0.0526 |
| 5 | 0.0470 | 0.0504 | 0.0470 | 0.0638 | 0.0470 | 0.0752 | 0.0470 | 0.0807 |
| 6 | 0.0725 | 0.0748 | 0.0725 | 0.0938 | 0.0725 | 0.1086 | 0.0725 | 0.1130 |
| 7 | 0.1107 | 0.1051 | 0.1107 | 0.1296 | 0.1107 | 0.1449 | 0.1107 | 0.1509 |
| 8 | 0.1680 | 0.1335 | 0.1680 | 0.1597 | 0.1680 | 0.1725 | 0.1680 | 0.1770 |
| 9 | 0.2540 | 0.1384 | 0.2540 | 0.1593 | 0.2540 | 0.1713 | 0.2540 | 0.1791 |
| 10 | 0.3834 | 0.0841 | 0.3834 | 0.0957 | 0.3834 | 0.1176 | 0.3833 | 0.1204 |
| 11 | 0.5782 | 0.5782 | 0.5782 | 0.5781 | 0.0120 | |||
| n | k/a.u. | /rad | /rad | Error/rad |
|---|---|---|---|---|
| 1 | 0.0046 | 0.0082 | 0.0100 | 0.0018 |
| 2 | 0.0104 | 0.0189 | 0.0219 | 0.0030 |
| 3 | 0.0183 | 0.0334 | 0.0375 | 0.0041 |
| 4 | 0.0299 | 0.0526 | 0.0586 | 0.0059 |
| 5 | 0.0470 | 0.0807 | 0.0862 | 0.0055 |
| 6 | 0.0725 | 0.1130 | 0.1202 | 0.0071 |
| 7 | 0.1107 | 0.1509 | 0.1564 | 0.0055 |
| 8 | 0.1680 | 0.1770 | 0.1835 | 0.0065 |
| 9 | 0.2540 | 0.1791 | 0.1815 | 0.0024 |
| 10 | 0.3833 | 0.1204 | 0.1287 | 0.0083 |
| 11 | 0.5781 | 0.0120 | 0.0163 | 0.0043 |
| k/a.u. | Error | ||
|---|---|---|---|
| 0.0046 | 9.2538 | 9.6013 | 0.3475 |
| 0.0104 | 8.1989 | 9.4644 | 1.2655 |
| 0.0183 | 8.4351 | 9.2767 | 0.8416 |
| 0.0299 | 8.2262 | 9.0083 | 0.7821 |
| 0.0470 | 8.0325 | 8.6212 | 0.5887 |
| 0.0725 | 7.6456 | 8.0667 | 0.4211 |
| 0.1107 | 6.9813 | 7.2876 | 0.3063 |
| 0.1680 | 6.0719 | 6.2319 | 0.1600 |
| 0.2540 | 4.8017 | 4.8951 | 0.0934 |
| 0.3833 | 3.6024 | 3.4371 | −0.1653 |
| 0.5781 | 2.0695 | 2.3833 | 0.3138 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gregg, S.; Gribakin, G. Calculation of Low-Energy Positron-Atom Scattering with Square-Integrable Wavefunctions. Atoms 2022, 10, 97. https://doi.org/10.3390/atoms10040097
Gregg S, Gribakin G. Calculation of Low-Energy Positron-Atom Scattering with Square-Integrable Wavefunctions. Atoms. 2022; 10(4):97. https://doi.org/10.3390/atoms10040097
Chicago/Turabian StyleGregg, Sarah, and Gleb Gribakin. 2022. "Calculation of Low-Energy Positron-Atom Scattering with Square-Integrable Wavefunctions" Atoms 10, no. 4: 97. https://doi.org/10.3390/atoms10040097
APA StyleGregg, S., & Gribakin, G. (2022). Calculation of Low-Energy Positron-Atom Scattering with Square-Integrable Wavefunctions. Atoms, 10(4), 97. https://doi.org/10.3390/atoms10040097







